Effects of Running Speed on Coupling between Pantograph of High-Speed Train and Tunnel Based on Aerodynamics and Multi-Body Dynamics Coupling
Abstract
:Featured Application
Abstract
1. Introduction
2. Model Establishment
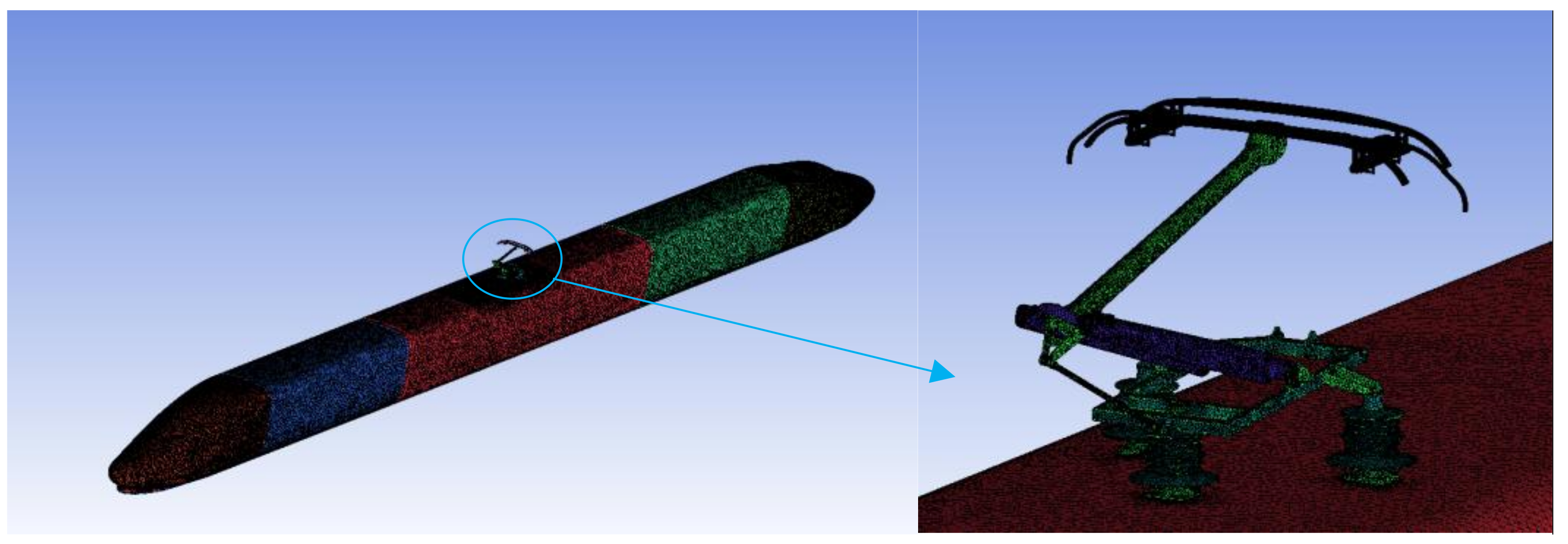
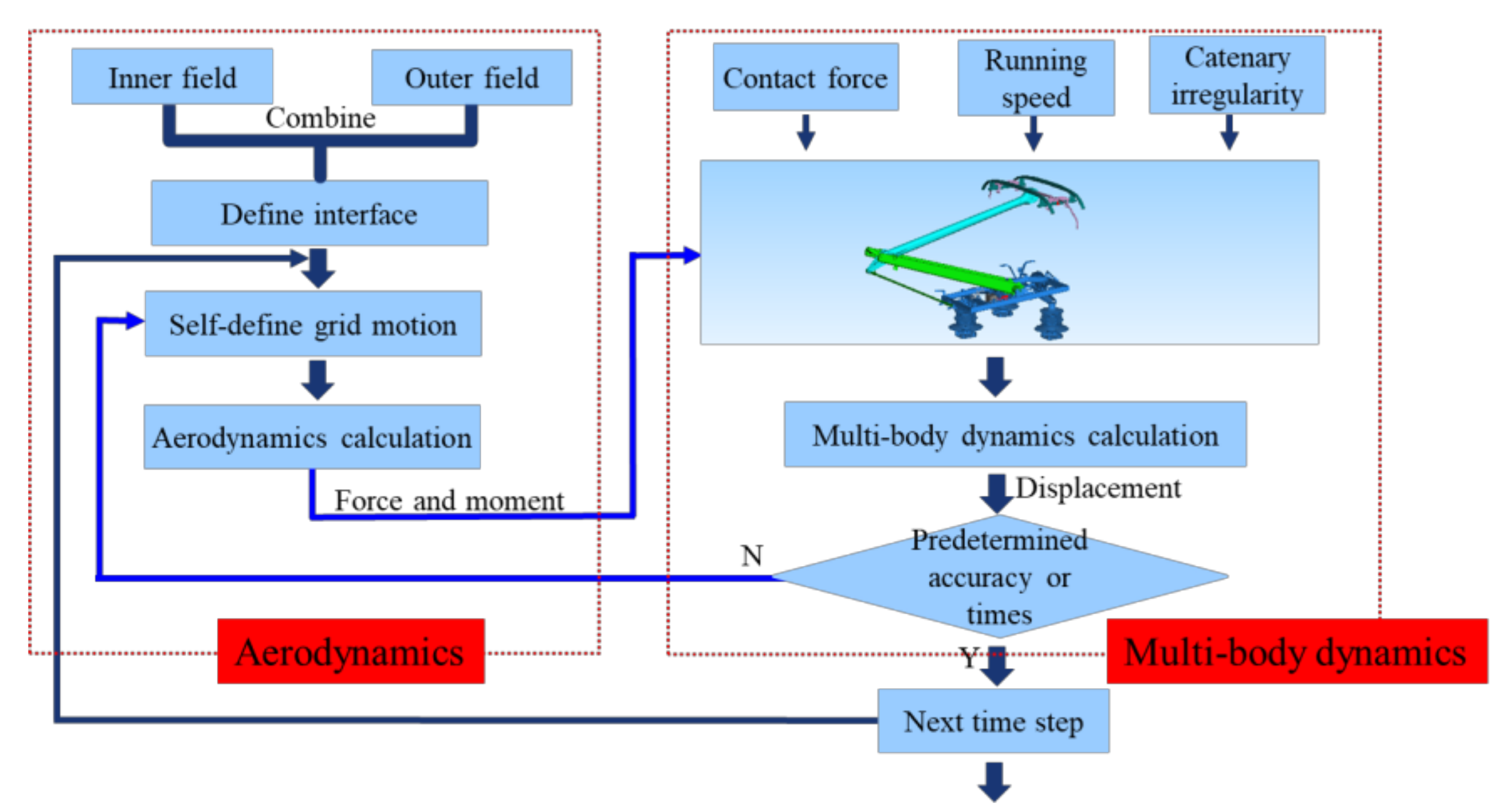
2.1. Multi-Body Dynamics Model
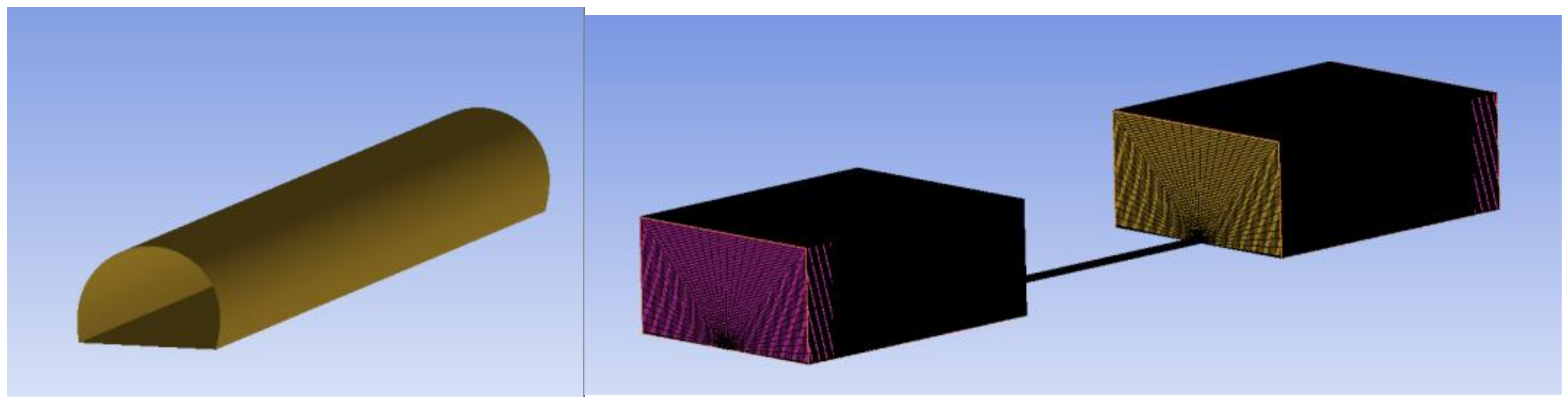
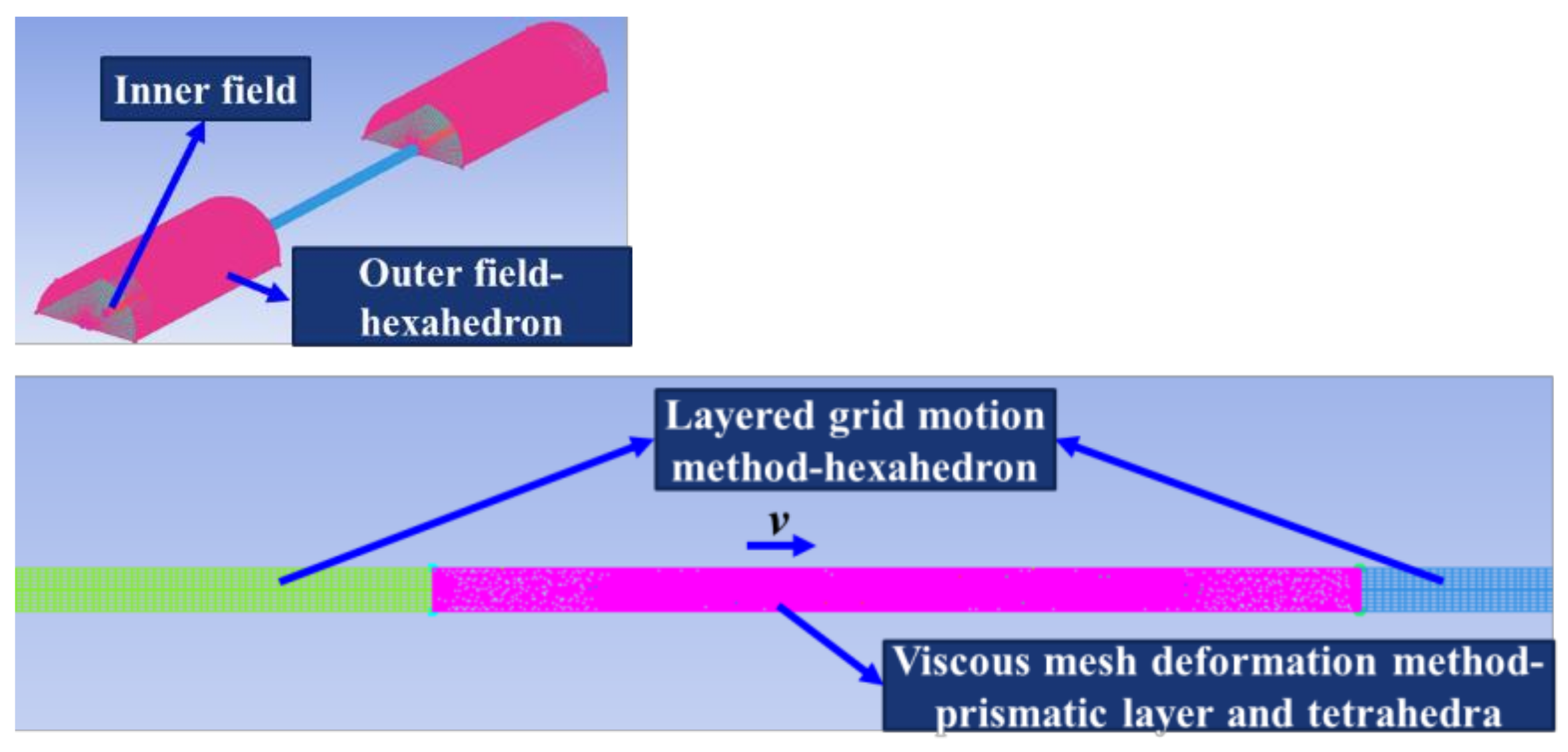
2.2. Aerodynamic Model
3. Coupling between Aerodynamics and Structural Dynamics
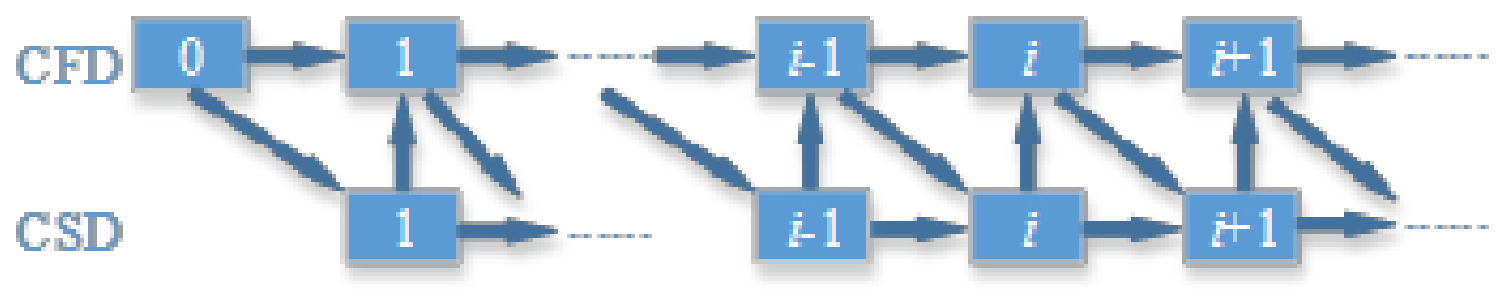
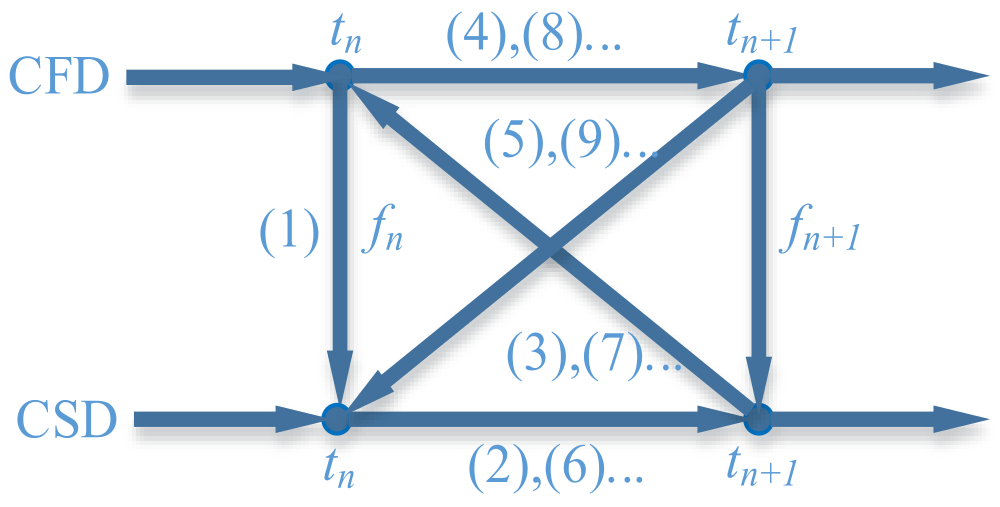
3.1. Coupling Principle
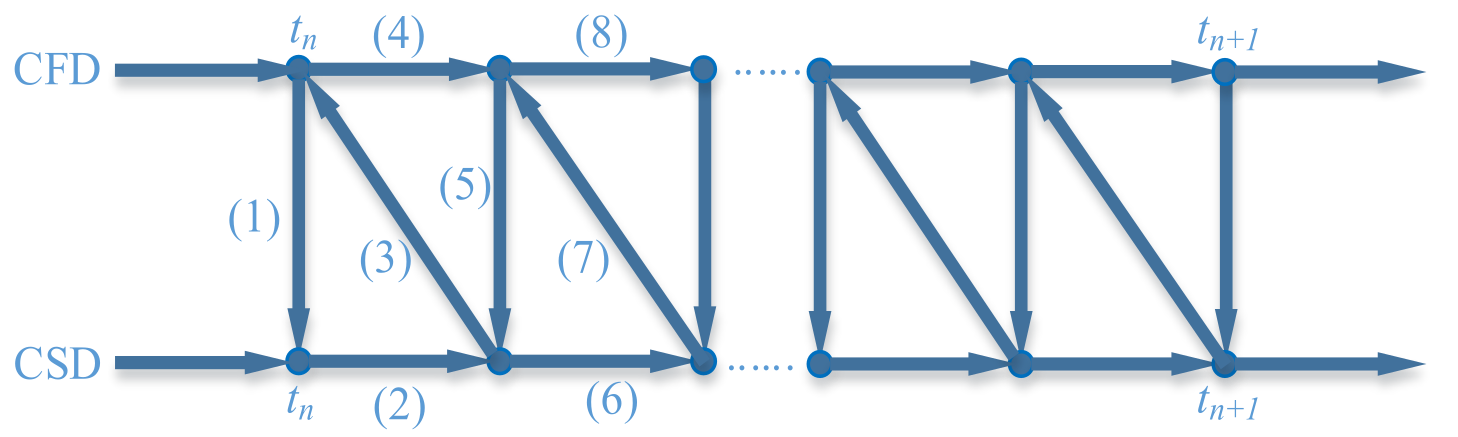
3.2. Coupling Method
3.3. Verification
4. Results and Discussion
4.1. Time-Domain Characteristics
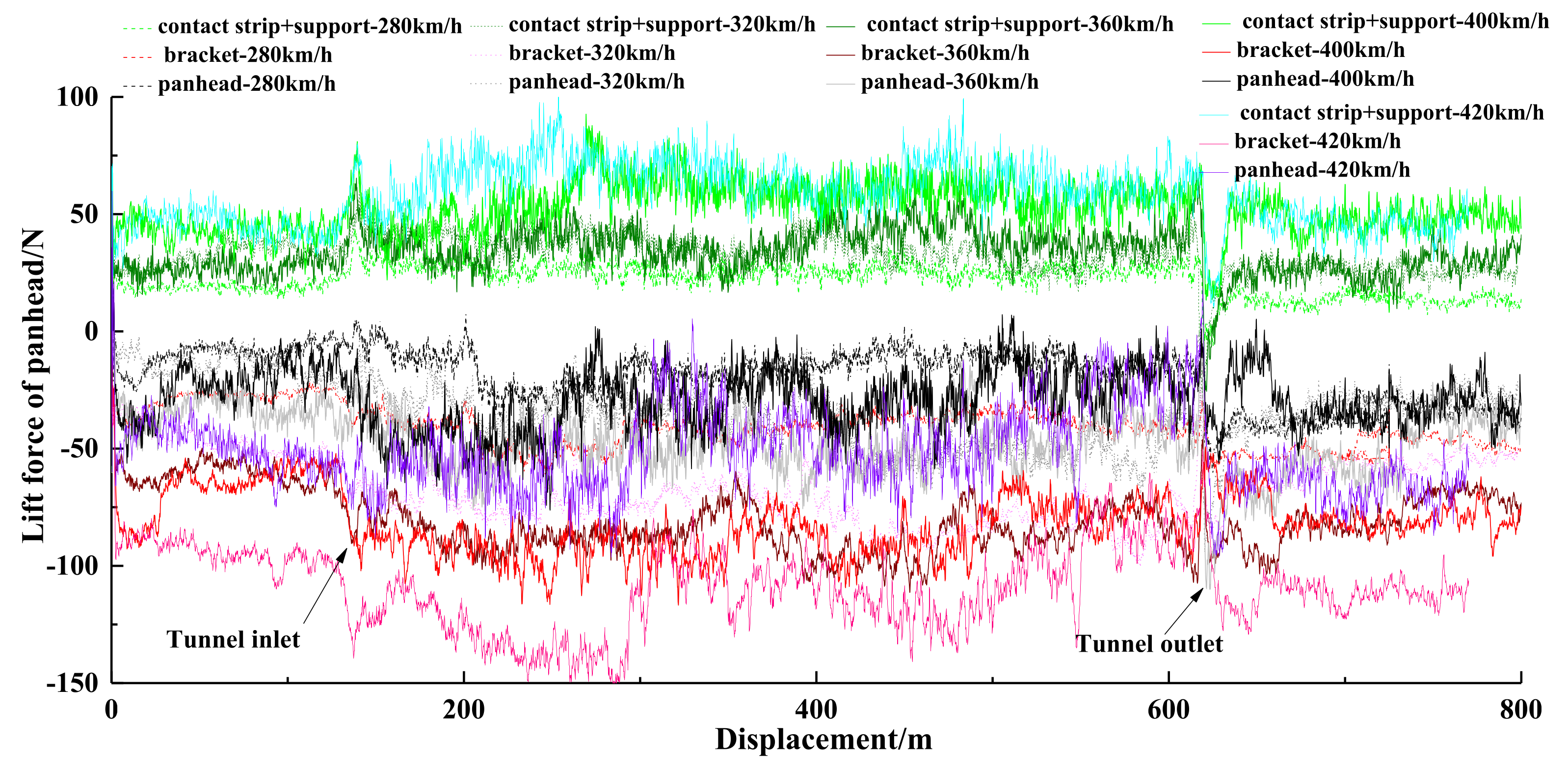
4.1.1. Aerodynamic Lift
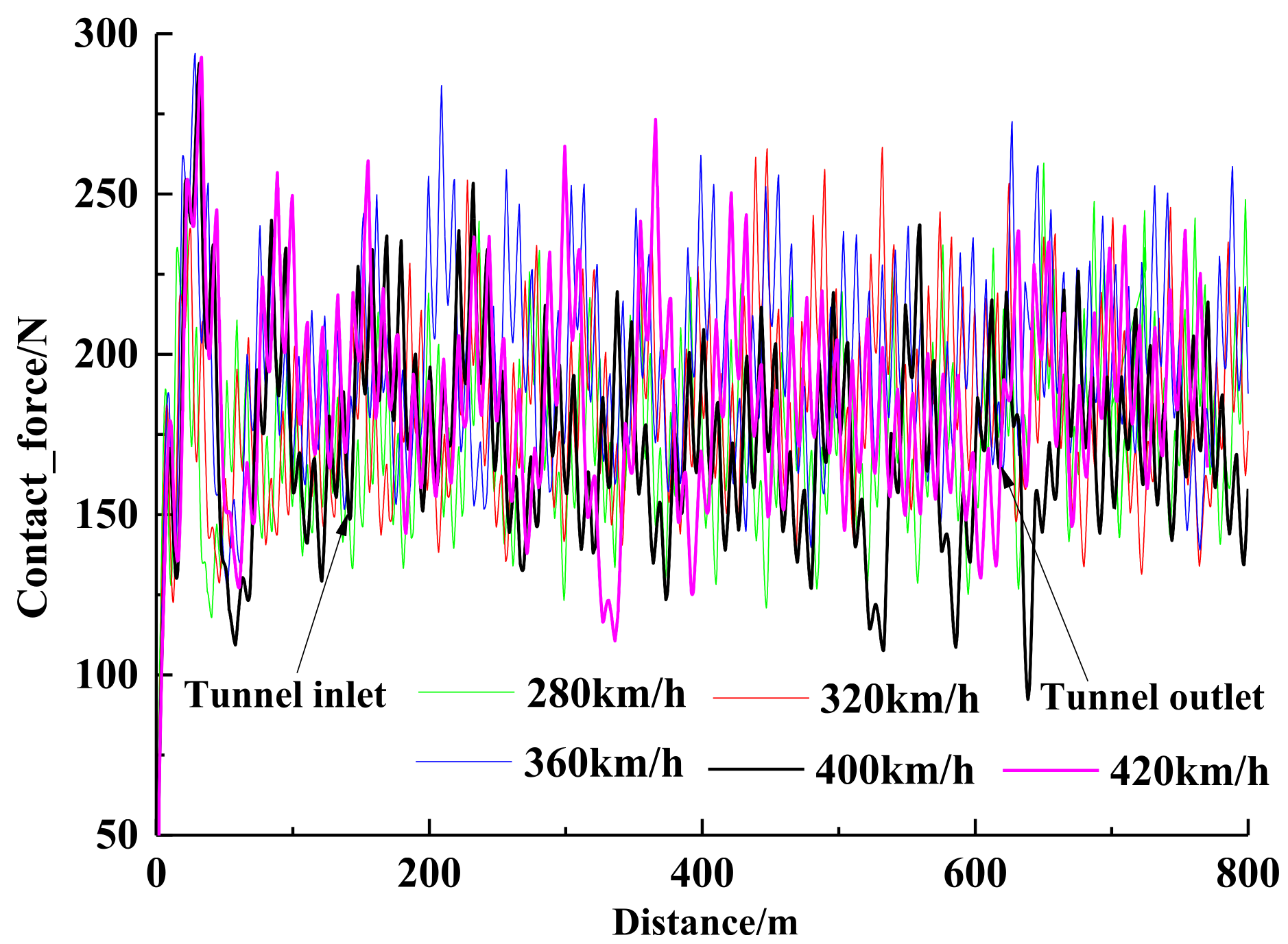
4.1.2. Contact Force between Pantograph and Catenary
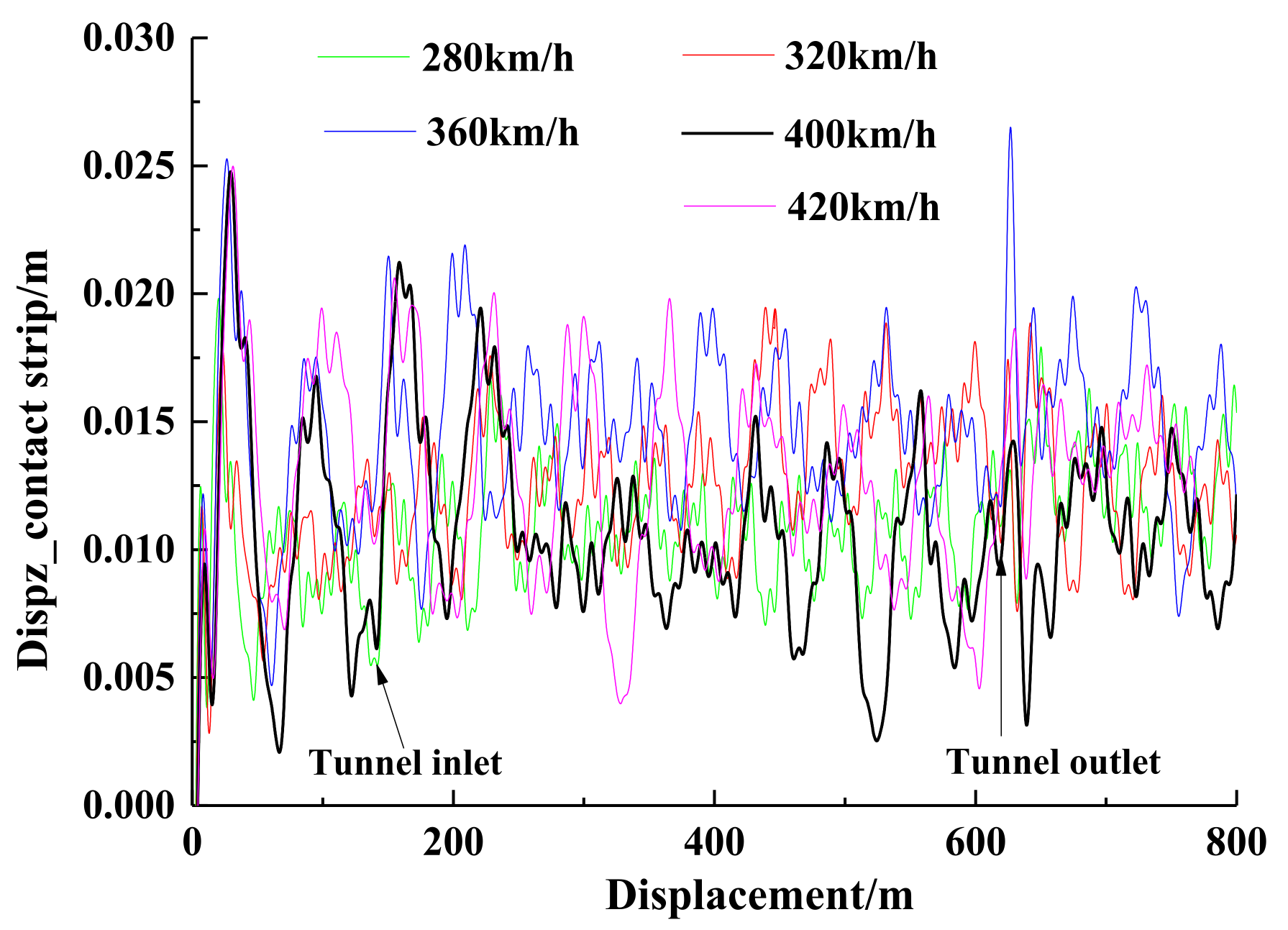
4.1.3. Vertical Displacement of Contact Strip
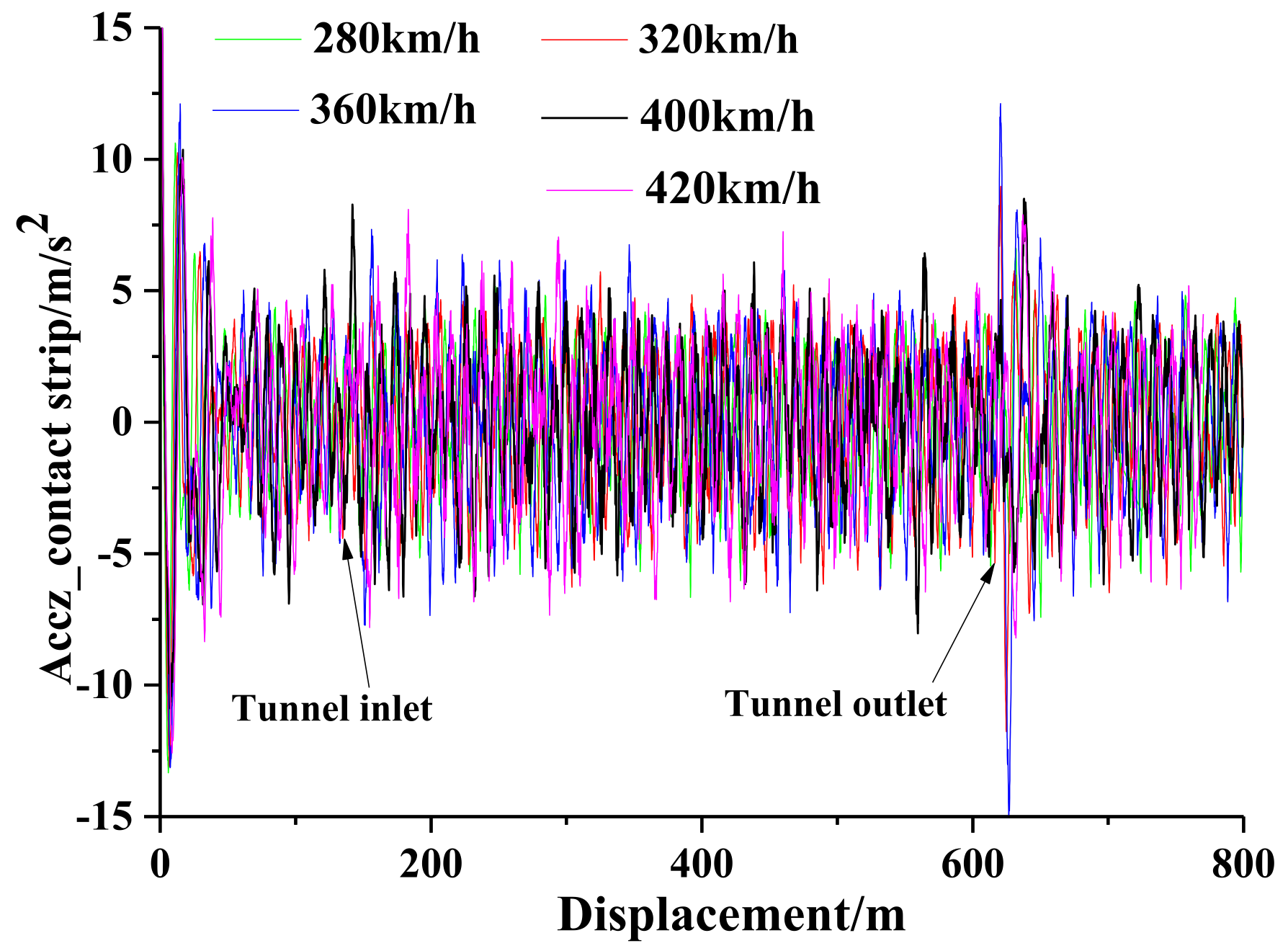
4.1.4. Vertical Acceleration of Contact Strip
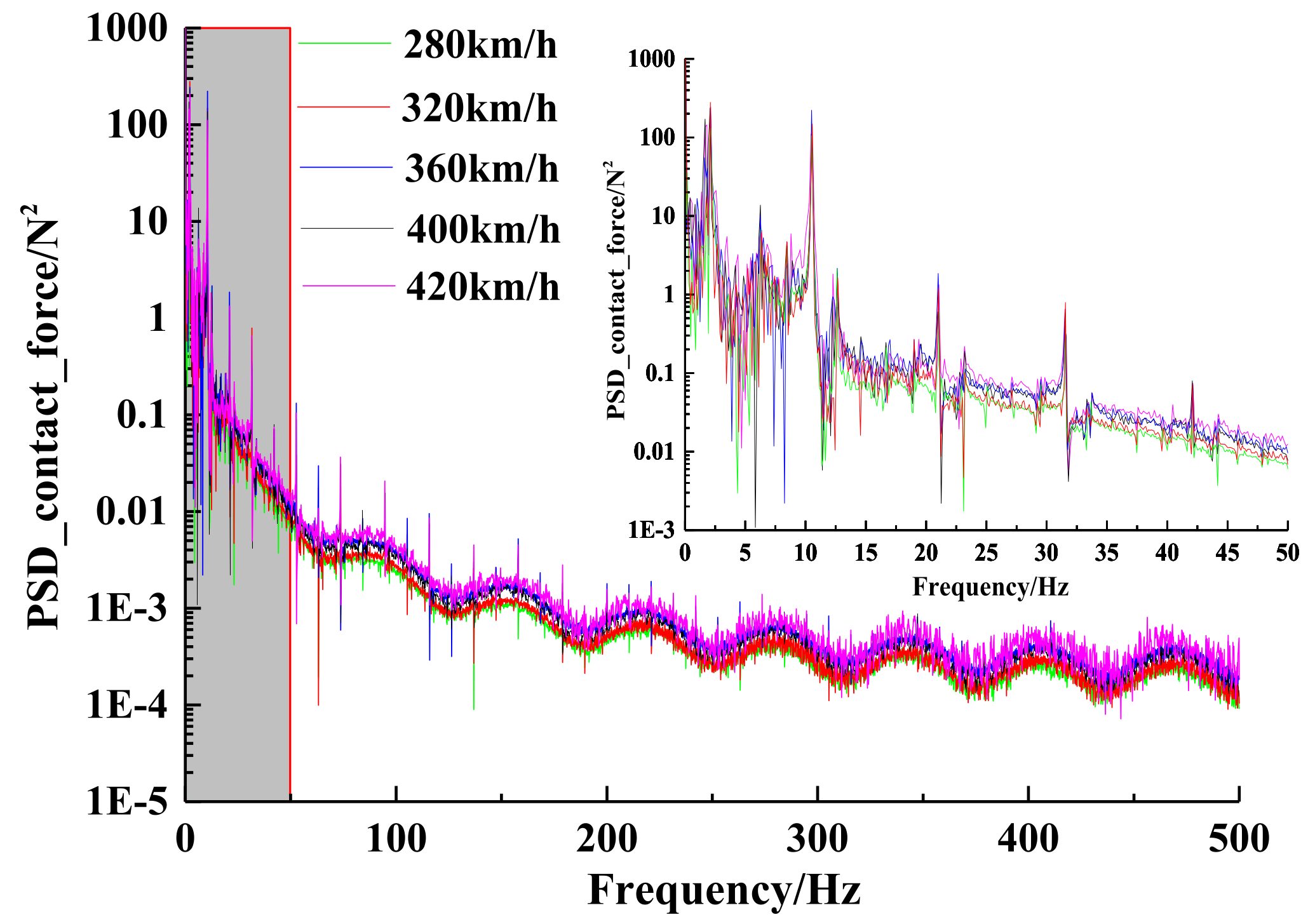
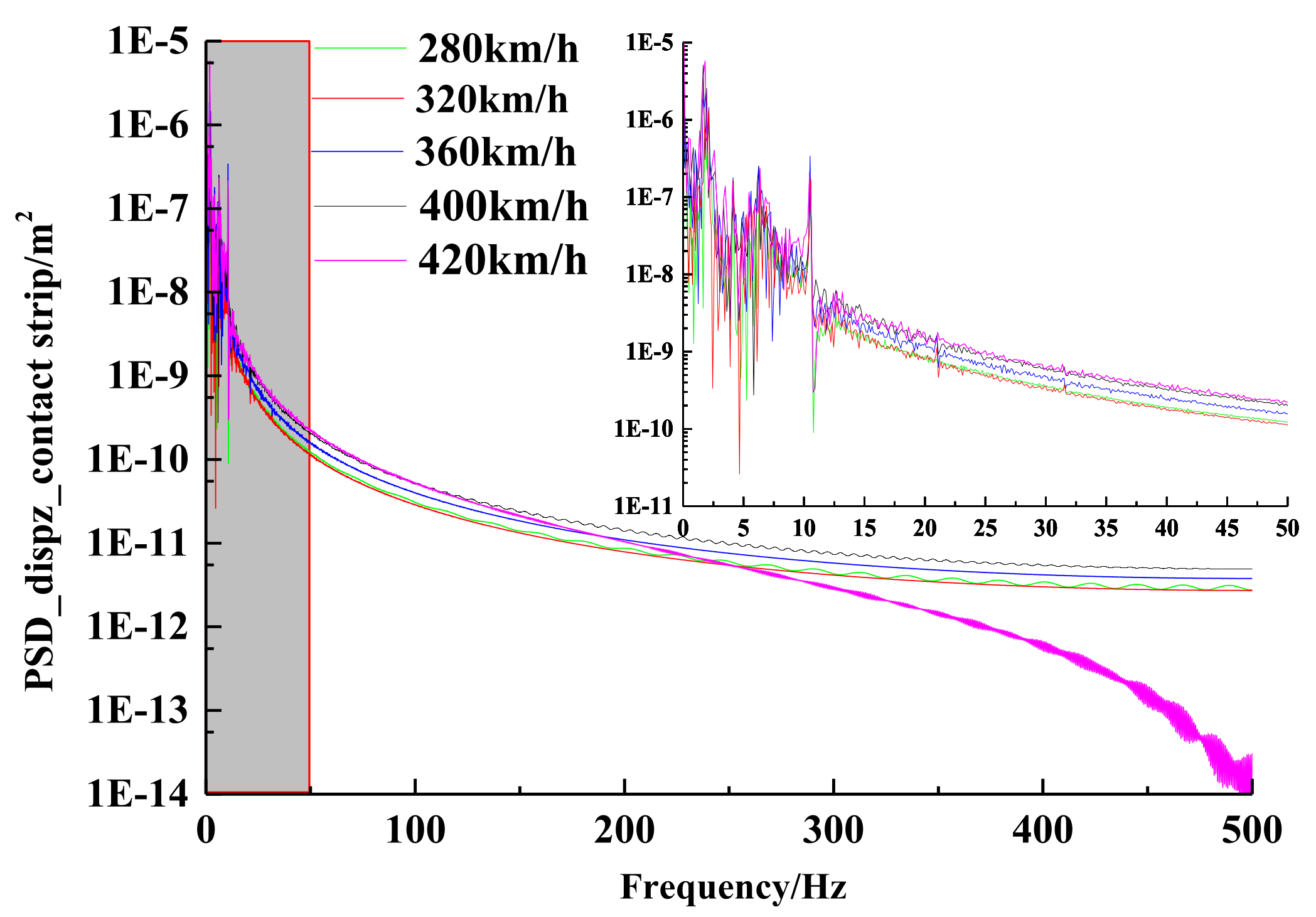
4.2. Frequency-Domain Characteristics
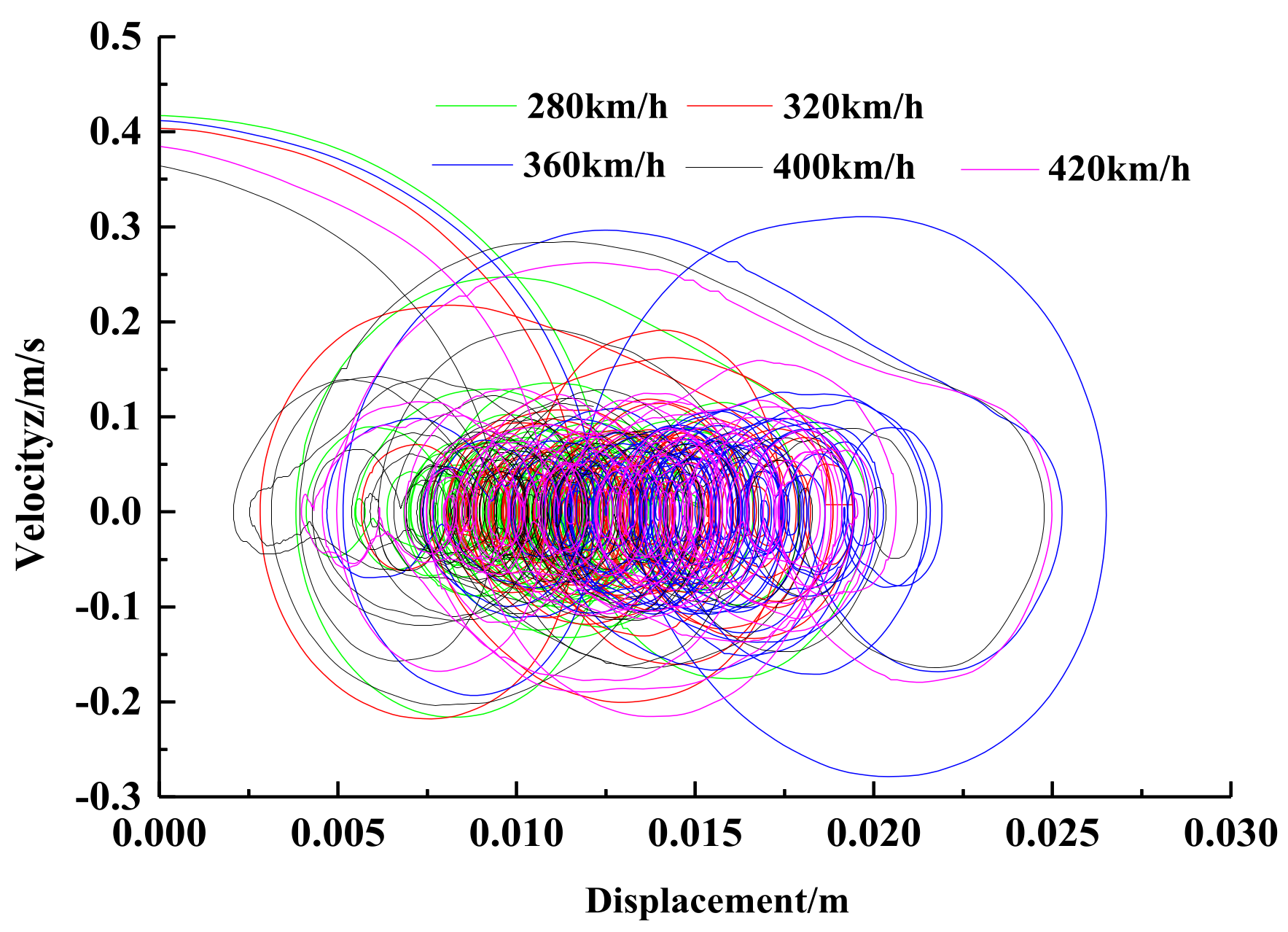
4.3. Phase Diagram of Contact Strip
5. Conclusions
- The aerodynamic lift of the panhead does not strictly increase with the increase in running speed. However, the standard deviation and the mean value are largest at 420 km/h than at other speeds—43.33% and 156.72% higher than those at 280 km/h, respectively.
- Compared with that at 360 km/h, at 400 km/h or 420 km/h, with 280 km/h as the benchmark, the contact strip standard deviations increase by 5.91% and 6.28%, respectively, while the contact strip minimum values decrease by 29.85% and 13.32%, respectively. These changes are detrimental to the stable contact between the pantograph and catenary.
- Compared with that at 360 km/h, at 400 km/h, taking 280 km/h as the benchmark, the mean value of contact strip vertical displacement decreases by 36.36% whereas the standard deviation increases by 21.54%.
- At 360 km/h, the maximum, minimum, and standard deviation of contact strip vertical acceleration are larger than those at other speeds.
- At running speeds of 360 km/h, 400 km/h and 420 km/h, there are larger amplitudes of the contact force between the pantograph and catenary. The larger amplitudes of vertical displacement and vertical acceleration of the contact strip appear at low frequencies (e.g., around 1.75 Hz) and are not multiple frequencies of the basic frequency.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dai, Z.Y.; Li, T.; Deng, J.; Zhou, N.; Zhang, W.H. Effect of the strip spacing on the aerodynamic performance of a high-speed double-strip pantograph. Int. J. Veh. Mech. Mobil. 2021. [Google Scholar] [CrossRef]
- Li, T.; Qin, D.; Zhang, W.H.; Zhang, J.Y. Study on the aerodynamic noise characteristics of high-speed pantographs with different strip spacings. J. Wind. Eng. Ind. Aerod. 2020, 202, 10–19. [Google Scholar] [CrossRef]
- Dai, Z.Y.; Li, T.; Zhang, W.H.; Zhang, J.Y. Numerical study on aerodynamic performance of high-speed pantograph with double strips. Fluid Mech. Mater. Process. 2020, 16, 31–40. [Google Scholar] [CrossRef]
- Sun, Z.K.; Wang, T.T.; Wu, F. Numerical investigation of influence of pantograph parameters and train length on aerodynamic drag of high-speed train. J. Cent. South. Univ. 2020, 27, 1334–1350. [Google Scholar] [CrossRef]
- Simarro, M.; Postigo, S.; Prado-Novoa, M.; Pérez-Blanca, A.; Castillo, J.J. Analysis of contact forces between the pantograph and the overhead conductor rail using a validated finite element model. Eng. Struct. 2020, 225, 1–15. [Google Scholar] [CrossRef]
- Song, Y.; Rønnquist, A.; Nåvik, P. Assessment of the high-frequency response in the railway pantograph-catenary interaction based on numerical simulation. IEEE T. Veh. Technol. 2020, 69, 10596–10605. [Google Scholar] [CrossRef]
- Yao, Y.M.; Zhou, N.; Mei, G.M.; Zhang, W.H. Dynamic analysis of pantograph-catenary system considering ice coating. Shock. Vib. 2020, 1, 1–15. [Google Scholar] [CrossRef]
- Song, Y.; Liu, Z.; Rønnquist, A.; Nåvik, P.; Liu, Z.D. Contact wire irregularity stochastics and effect on high-speed railway pantograph-catenary interactions. IEEE T. Instrum. Meas. 2020, 69, 8196–8206. [Google Scholar] [CrossRef]
- Yao, Y.M.; Zou, D.; Zhou, N.; Mei, G.M.; Wang, J.W.; Zhang, W.H. A study on the mechanism of vehicle body vibration affecting the dynamic interaction in the pantograph–catenary system. Vehicle Syst. Dyn. 2021, 59, 1335–1354. [Google Scholar] [CrossRef]
- Kulkarni, S.; Pappalardo, C.M.; Shabana, A.A. Pantograph/catenary contact formulations. J. Vib. Acoust. 2017, 139, 1–12. [Google Scholar] [CrossRef]
- Vesali, F.; Rezvani, M.A.; Molatefi, H.; Hecht, M. Static form-finding of normal and defective catenaries based on the analytical exact solution of the tensile Euler–Bernoulli beam. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2019, 233, 691–700. [Google Scholar] [CrossRef]
- Wang, W.L.; Liang, Y.W.; Zhang, W.H.; Iwnick, S. Effect of the nonlinear displacement-dependent characteristics of a hydraulic damper on high-speed rail pantograph dynamics. Nonlinear Dyn. 2019, 95, 3439–3464. [Google Scholar] [CrossRef] [Green Version]
- Vesali, F.; Ali Rezvani, M.; Molatefi, H. Simulation of the dynamic interaction of rail vehicle pantograph and catenary through a modal approach. Arch. Appl. Mech. 2020, 90, 1475–1496. [Google Scholar] [CrossRef]
- Song, Y.; Wang, Z.W.; Liu, Z.G.; Wang, R.C. A spatial coupling model to study dynamic performance of pantograph-catenary with vehicle-track excitation. Mech. Syst. Signal Pr. 2021, 151, 1–26. [Google Scholar] [CrossRef]
- Carnevale, M.; Facchinetti, A.; Maggiori, L.; Rocchi, D. Computational fluid dynamics as a means of assessing the influence of aerodynamic forces on the mean contact force acting on a pantograph. Proc. Inst. Mech. Eng. Part F-J. Rail Rapid Transit 2016, 230, 1698–1713. [Google Scholar] [CrossRef]
- Pombo, J.; Ambrósio, J. Environmental and track perturbations on multiple pantograph interaction with catenaries in high-speed trains. Comput. Struct. 2013, 124, 88–101. [Google Scholar] [CrossRef] [Green Version]
- Song, Y.; Liu, Z.G.; Wang, H.R.; Lu, X.B.; Zhang, J. Nonlinear analysis of wind-induced vibration of high-speed railway catenary and its influence on pantograph–catenary interaction. Vehicle Syst. Dyn. 2016, 54, 723–747. [Google Scholar] [CrossRef]
- Li, R.P.; Zhang, W.H.; Ning, Z.; Liu, B.B.; Zou, D.; Liu, W. Influence of a high-speed train passing through a tunnel on pantograph aerodynamics and pantograph–catenary interaction. Proc. Inst. Mech. Eng. Part F-J. Rail Rapid Transit 2017, 231, 198–210. [Google Scholar] [CrossRef]
- Shi, H.J.; Chen, G.; Yang, Y.R. A comparative study on pantograph-catenary models and effect of parameters on pantograph-catenary dynamics under crosswind. J. Wind. Eng. Ind. Aerod. 2021, 211, 1–18. [Google Scholar] [CrossRef]
- Nakade, K.; Masahiro, S.; Hiroshi, F. Interaction between vehicle vibration and aerodynamic force on high-speed train running in tunnel. Veh. Syst. Dyn. 2004, 41, 717–723. [Google Scholar]
- Li, T.; Zhang, J.Y.; Zhang, W.H. An improved algorithm for fluid-structure interaction of high-speed trains under crosswind. J. Mod. Transp. 2011, 19, 75–81. [Google Scholar] [CrossRef] [Green Version]




| Maximum | Minimum | Mean | Standard Deviation | Mean Error | Minimum Error | |
|---|---|---|---|---|---|---|
| Bidirectional coupling | 254.264 | 126.475 | 183.55598 | 25.55415 | −0.05% | 3.92% |
| Wind loads as known loads | 255.9772 | 129.57878 | 182.58699 | 25.02823 | −0.58% | 6.47% |
| No wind loads | 216.15146 | 119.43517 | 162.41813 | 21.661 | 11.56% | −11.56% |
| Fitted results | 242.667 | 121.704 | 183.65 | - | - | - |
| Maximum (N) | Minimum (N) | Mean (N) | Standard Deviation (N) | Standard Deviation Difference | Mean Difference | |
|---|---|---|---|---|---|---|
| Contact strip +support-280 | 44.2242 | −6.24535 | 21.39542 | 6.60428 | - | - |
| Bracket-280 | −21.8436 | −61.0492 | −41.34639 | 8.59548 | - | - |
| Panhead-280 | 7.87 | −60.6928 | −19.95097 | 12.08294 | - | - |
| Contact strip +support-320 | 60.4527 | −26.5484 | 33.04816 | 7.99576 | 21.07% | 54.46% |
| Bracket-320 | −23.7578 | −100.617 | −68.01941 | 13.63352 | 58.61% | 64.51% |
| Panhead-320 | −1.0657 | −93.9362 | −34.97125 | 14.18627 | 17.41% | 75.29% |
| Contact strip +support-360 | 67.7074 | −25.5528 | 33.75717 | 9.68588 | 46.66% | 57.78% |
| Bracket-360 | −43.6264 | −111.306 | −81.01173 | 12.59806 | 46.57% | 95.93% |
| Panhead-360 | 2.8301 | −110.0323 | −47.25456 | 13.16243 | 8.93% | 136.85% |
| Contact strip +support-400 | 92.6164 | 13.3197 | 52.56508 | 10.52163 | 37.23% | 145.68% |
| Bracket-400 | −49.2168 | −116.733 | −81.84115 | 12.75881 | 48.44% | 97.79% |
| Panhead-400 | 7.1519 | −76.2796 | −29.27607 | 12.59056 | 4.20% | 46.74% |
| Contact strip +support-420 | 102.565 | 10.0293 | 58.27943 | 12.97636 | 96.48% | 172.39% |
| Bracket-420 | −50.0785 | −152.777 | −109.49819 | 16.78258 | 95.25% | 164.83% |
| Panhead-420 | 18.2573 | −96.3173 | −51.21876 | 17.33287 | 43.33% | 156.72% |
| Maximum (N) | Minimum (N) | Mean (N) | Standard Deviation (N) | Standard Deviation Difference | Mean Difference | |
|---|---|---|---|---|---|---|
| 280 km/h | 259.666 | 117.859 | 178.09312 | 26.84069 | - | - |
| 320 km/h | 264.556 | 122.616 | 184.08905 | 26.12482 | −2.67% | 3.37% |
| 360 km/h | 293.885 | 127.550 | 196.55243 | 28.08317 | 4.63% | 10.36% |
| 400 km/h | 290.781 | 92.366 | 173.37707 | 29.66925 | 10.54% | −2.65% |
| 420 km/h | 292.640 | 110.554 | 186.40273 | 29.76878 | 10.91% | 4.67% |
| Maximum (m) | Minimum (m) | Mean (m) | Standard Deviation (m) | Standard Deviation Difference | Mean Difference | |
|---|---|---|---|---|---|---|
| 280 km/h | 0.01982 | 0.00383 | 0.01092 | 0.00246 | - | - |
| 320 km/h | 0.01947 | 0.00282 | 0.01252 | 0.00276 | 12.20% | 14.65% |
| 360 km/h | 0.0265 | 0.00469 | 0.01468 | 0.00331 | 34.55% | 34.43% |
| 400 km/h | 0.02477 | 0.00208 | 0.01071 | 0.00384 | 56.10% | −1.92% |
| 420 km/h | 0.02498 | 0.00398 | 0.01293 | 0.00387 | 57.32% | 18.41% |
| Maximum (m/s2) | Minimum (m/s2) | Mean (m/s2) | Standard Deviation (m/s2) | Standard Deviation Difference | Minimum Difference | |
|---|---|---|---|---|---|---|
| 280 km/h | 10.6145 | −7.42214 | −0.2054 | 2.51441 | - | - |
| 320 km/h | 10.243 | −11.7679 | 0.01795 | 2.6966 | 7.25% | 58.55% |
| 360 km/h | 12.1125 | −15.0945 | 0.0103 | 3.10342 | 23.43% | 103.37% |
| 400 km/h | 10.3612 | −8.02659 | 0.04289 | 2.75807 | 9.69% | 8.14% |
| 420 km/h | 10.0105 | −8.3469 | 0.02085 | 2.9433 | 17.06% | 12.46% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, Z.; Guo, Y.; Guo, D.; Yang, G.; Liu, Y. Effects of Running Speed on Coupling between Pantograph of High-Speed Train and Tunnel Based on Aerodynamics and Multi-Body Dynamics Coupling. Appl. Sci. 2021, 11, 10008. https://doi.org/10.3390/app112110008
Ji Z, Guo Y, Guo D, Yang G, Liu Y. Effects of Running Speed on Coupling between Pantograph of High-Speed Train and Tunnel Based on Aerodynamics and Multi-Body Dynamics Coupling. Applied Sciences. 2021; 11(21):10008. https://doi.org/10.3390/app112110008
Chicago/Turabian StyleJi, Zhanling, Yi Guo, Dilong Guo, Guowei Yang, and Yubiao Liu. 2021. "Effects of Running Speed on Coupling between Pantograph of High-Speed Train and Tunnel Based on Aerodynamics and Multi-Body Dynamics Coupling" Applied Sciences 11, no. 21: 10008. https://doi.org/10.3390/app112110008
APA StyleJi, Z., Guo, Y., Guo, D., Yang, G., & Liu, Y. (2021). Effects of Running Speed on Coupling between Pantograph of High-Speed Train and Tunnel Based on Aerodynamics and Multi-Body Dynamics Coupling. Applied Sciences, 11(21), 10008. https://doi.org/10.3390/app112110008






