Functionally Graded Plate Fracture Analysis Using the Field Boundary Element Method
Abstract
:1. Introduction
2. Materials and Methods
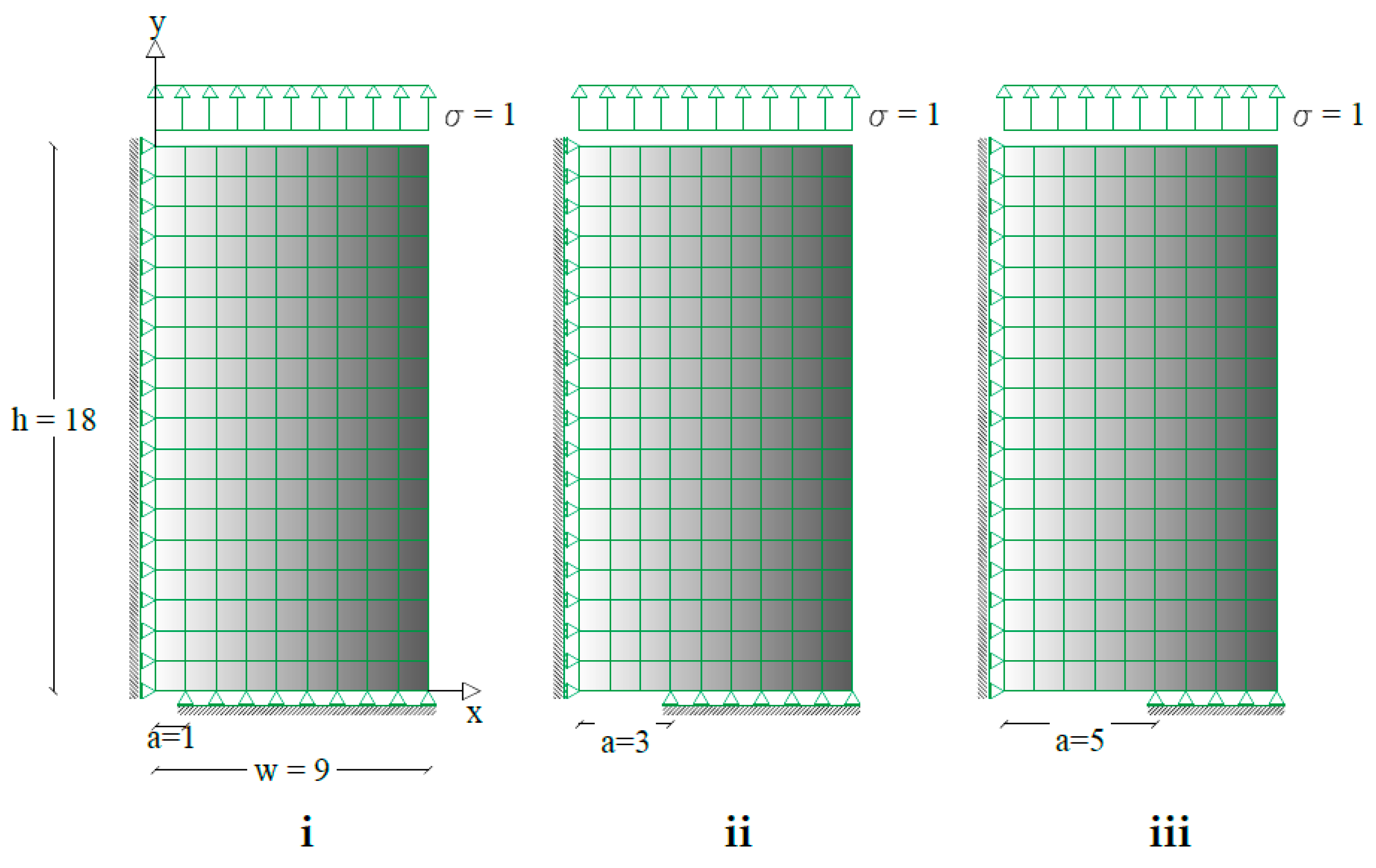
2.1. Mesh and Constraints
2.2. Material Properties
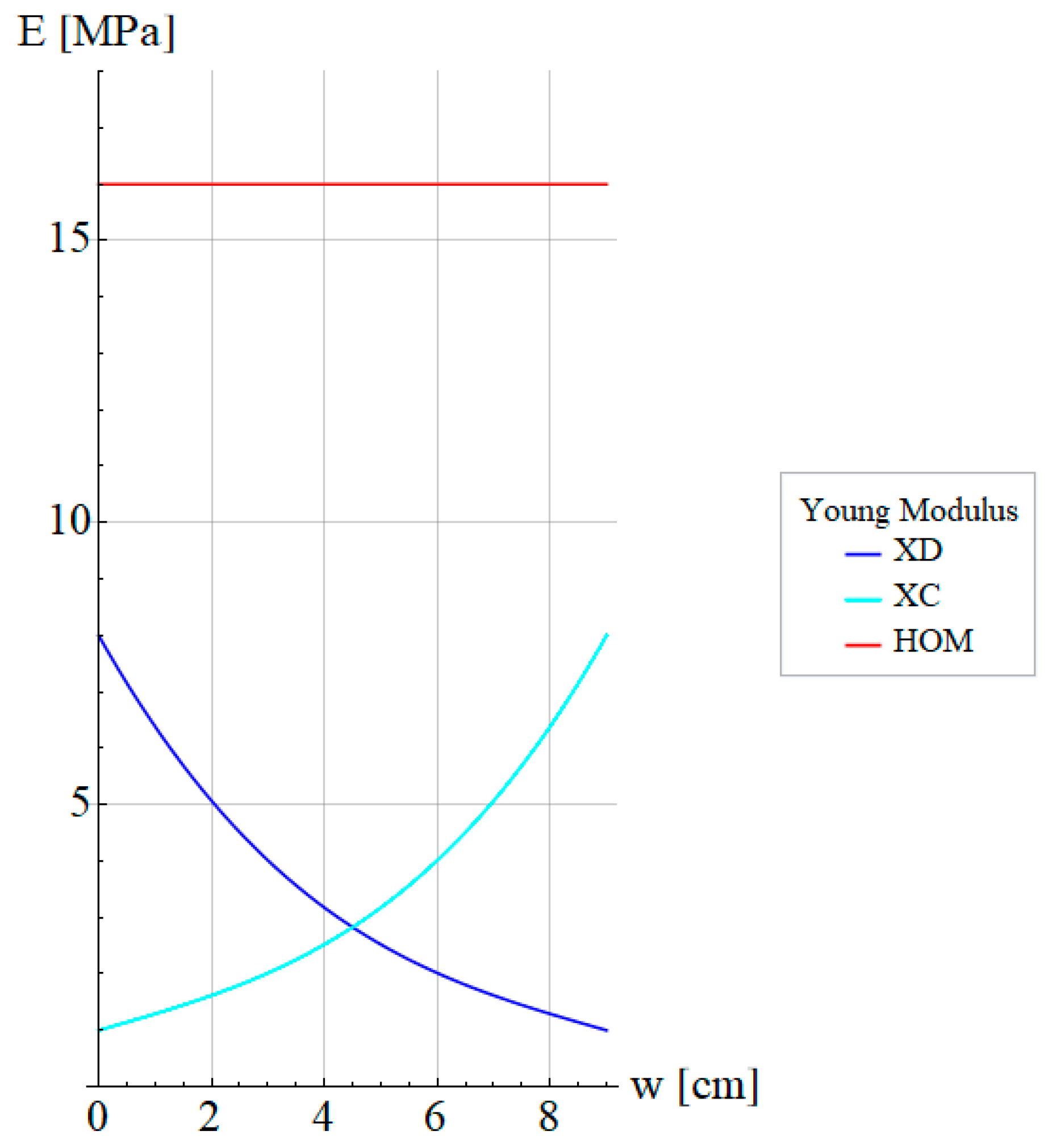
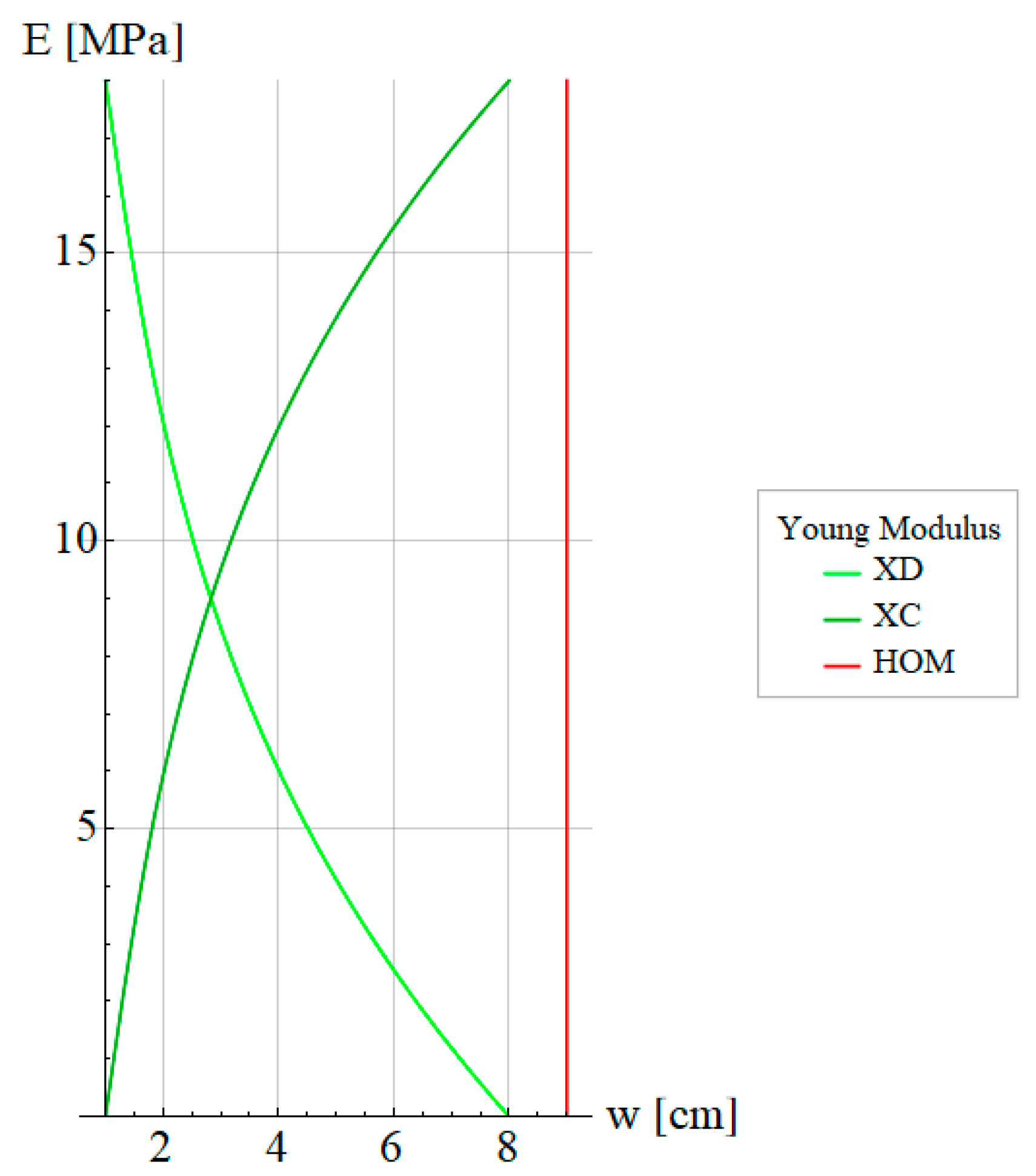
- : Young Modulus decreasing in the x-direction with spanning from 8 to 1:
- : Young Modulus increasing in the x-direction with spanning from 1 to 8:
- : Young Modulus decreasing in the y-direction with spanning from 8 to 1:
- : Young Modulus increasing in the y-direction with spanning from 1 to 8:
3. Results
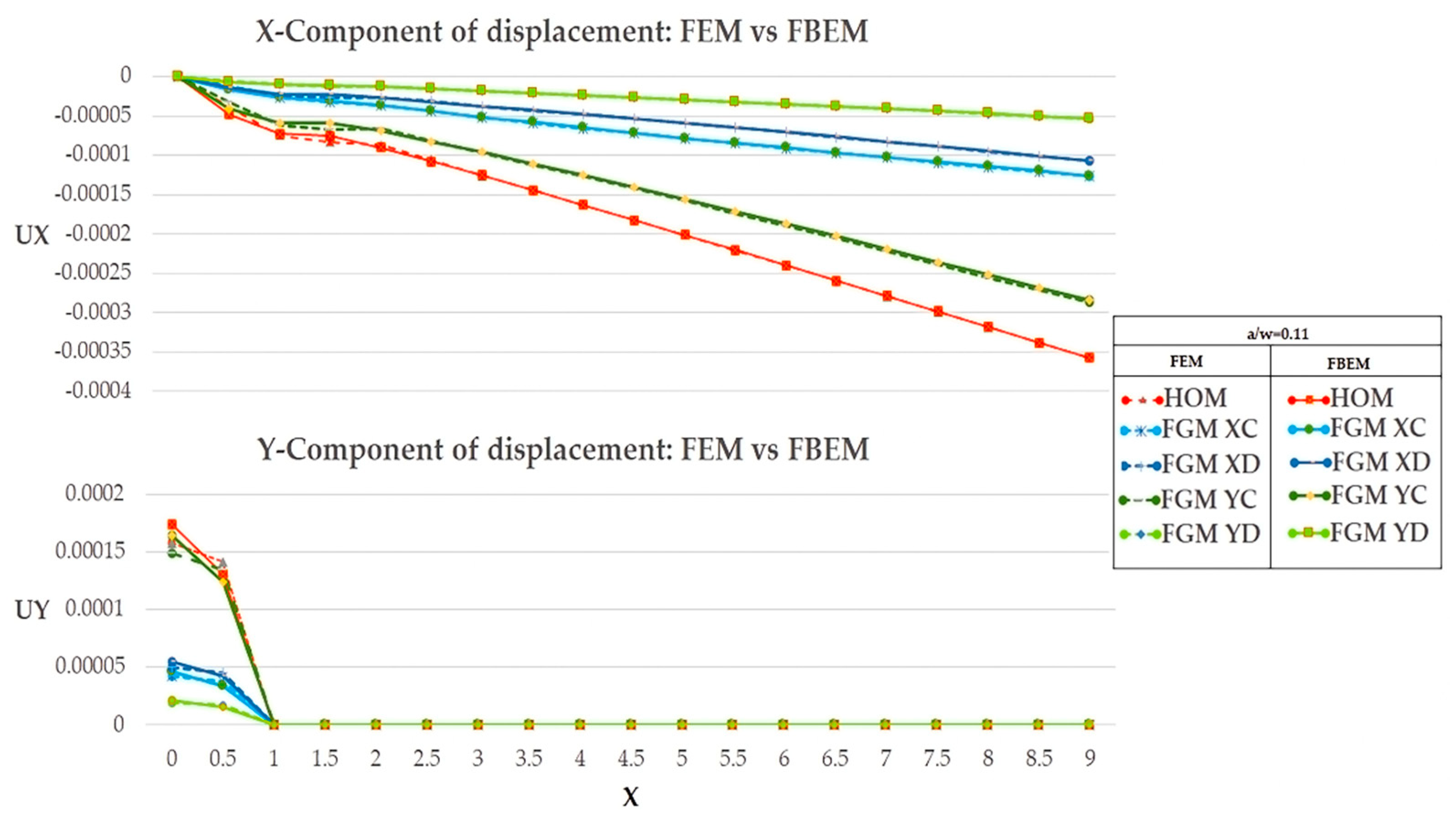
3.1. Displacement’s Comparison FEM vs. FBEM
3.2. Numerical Results
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ichikawa, K. Functionally Graded Materials in the 21st Century; Kluwer Academic Publishers: Norwell, MA, USA, 2001. [Google Scholar]
- Watanabe, K.; Ziegler, F. Dynamics of Advanced Materials and Smart Structures. In Proceedings of the IUTAM Symposium, Dordrecht, The Netherlands, 20–24 May 2002. [Google Scholar]
- Pan, W.; Gong, J.; Zhang, L.; Chen, L. Functionally Graded Materials. In Proceedings of the VII Int. Symposium (FGM2002), Beijing, China, 15–18 October 2003. [Google Scholar]
- Irwin, G.R. Analysis of Stresses and Strains Near the End of a Crack Traversing a Plate. J. Appl. Mech. 1957, 24, 361–364. [Google Scholar] [CrossRef]
- Li, R.; Yang, M.; Liang, B. The Homogenized Transformation Method for the Calculation of Stress Intensity Factor in Cracked FGM Structure. Int. J. Comput. Methods 2021, 18, 2050014. [Google Scholar] [CrossRef]
- Minutolo, V.; Esposito, L.; Sacco, E.; Fraldi, M. Designing stress for optimizing and toughening truss-like structures. Meccanica 2020, 55, 1603–1622. [Google Scholar] [CrossRef]
- Wang, H.-T.; Wu, G.; Pang, Y.-Y. Theoretical and Numerical Study on Stress Intensity Factors for FRP-Strengthened Steel Plates with Double-Edged Cracks. Sensors 2018, 18, 2356. [Google Scholar] [CrossRef] [Green Version]
- Seifi, R. Stress intensity factors for internal surface cracks in autofrettaged functionally graded thick cylinders using weight function method. Theor. Appl. Fract. Mech. 2015, 75, 113–123. [Google Scholar] [CrossRef]
- Eshraghi, I.; Soltani, N. Stress intensity factor calculation for internal circumferential cracks in functionally graded cylinders using the weight function approach. Eng. Fract. Mech. 2015, 134, 1–19. [Google Scholar] [CrossRef]
- Chen, Y. Stress intensity factors in a finite cracked cylinder made of functionally graded materials. Int. J. Press. Vessel. Pip. 2004, 81, 941–947. [Google Scholar] [CrossRef]
- Mi, Y.; Aliabadi, M. Dual boundary element method for three-dimensional fracture mechanics analysis. Eng. Anal. Bound. Elements 1992, 10, 161–171. [Google Scholar] [CrossRef]
- Chen, T.; Wang, B.; Cen, Z.; Wu, Z. A symmetric Galerkin multi-zone boundary element method for cohesive crack growth. Eng. Fract. Mech. 1999, 63, 591–609. [Google Scholar] [CrossRef]
- Minutolo, V.; Ruocco, E. Boundary element modelling and optimisation of structures made of functionally graded materials. In Advances in Boundary Element Techniques; EC., Ltd.: Montreal, UK, 2005. [Google Scholar]
- Sutradhar, A.; Paulino, G.H. The simple boundary element method for transient heat conduction in functionally graded materials. Comput. Methods Appl. Mech. Eng. 2004, 4511–4539. [Google Scholar] [CrossRef]
- Chandran, K.S.R.; Imad, B. Determination of stress intensity factor solutions for cracks in finite-width functionally graded materials. Int. J. Fract. 2003, 121, 183–203. [Google Scholar] [CrossRef]
- Minutolo, V.; Ruocco, E. Wall structure finite-element by BEM coupling. Int. J. Mason. Res. Innov. 2019, 4, 113. [Google Scholar] [CrossRef]
- Fraldi, M.; Minutolo, V.; Ruocco, E. The derivation of the domain boundary integral equation governing the material nonlinearity of heterogeneous spongy structures. In Proceedings of the Third International Conference on BETEQ, Beijing, China, 10–12 September 2002. [Google Scholar]
- Cutolo, A.; Carotenuto, A.R.; Palumbo, S.; Esposito, L.; Minutolo, V.; Fraldi, M.; Ruocco, E. Stacking sequences in composite laminates through design optimization. Meccanica 2021, 56, 1555–1574. [Google Scholar] [CrossRef]
- Esposito, L.; Cutolo, A.; Barile, M.; Lecce, L.; Mensitieri, G.; Sacco, E.; Fraldi, M. Topology optimization-guided stiffening of composites realized through Automated Fiber Placement. Compos. Part B Eng. 2019, 164, 309–323. [Google Scholar] [CrossRef]
- Ruocco, E.; Minutolo, V. Two-dimensional stress analysis of multiregion functionally graded materials using a field boundary element model. Compos. Part B Eng. 2012, 43, 663–672. [Google Scholar] [CrossRef]
- Wang, B.-L.; Tian, Z.-H. Application of finite element–finite difference method to the determination of transient temperature field in functionally graded materials. Finite Elements Anal. Des. 2005, 41, 335–349. [Google Scholar] [CrossRef]
- Kim, J.-H.; Paulino, G.H. Finite element evaluation of mixed mode stress intensity factors in functionally graded materials. Int. J. Numer. Methods Eng. 2001, 53, 1903–1935. [Google Scholar] [CrossRef]
- Delale, F.; Erdogan, F. The Crack Problem for a Nonhomogeneous Plane. J. Appl. Mech. 1983, 50, 609–614. [Google Scholar] [CrossRef]
- Erdogan, F. The Crack Problem for Bonded Nonhomogeneous Materials Under Antiplane Shear Loading. J. Appl. Mech. 1985, 52, 823–828. [Google Scholar] [CrossRef]
- Erdogan, F. Fracture mechanics of functionally graded materials. Compos. Eng. 1995, 5, 753–770. [Google Scholar] [CrossRef]
- Eischen, J. Fracture of nonhomogeneous materials. Int. J. Fract. 1987, 34, 3–22. [Google Scholar]
- Konda, N.; Erdogan, F. The mixed mode crack problem in a nonhomogeneous elastic medium. Eng. Fract. Mech. 1994, 47, 533–545. [Google Scholar] [CrossRef]
- Gu, P.; Asaro, R. Crack deflection in functionally graded materials. Int. J. Solids Struct. 1997, 34, 3085–3098. [Google Scholar] [CrossRef]
- Gu, P.; Asaro, R. Cracks in functionally graded materials. Int. J. Solids Struct. 1997, 34, 1–17. [Google Scholar] [CrossRef]
- Anlas, G.; Santare, M.; Lambros, J. Numerical Calculation of Stress Intensity Factors in Functionally Graded Materials. Int. J. Fract. 2000, 104, 131–143. [Google Scholar] [CrossRef]
- Marur, P.R.; Tippur, H.V. Numerical analysis of crack-tip fields in functionally graded materials with a crack normal to the elastic gradient. Int. J. Solids Struct. 2000, 37, 5353–5370. [Google Scholar] [CrossRef]
- Nasirmanesh, A.; Mohammadi, S. Eigenvalue buckling analysis of cracked functionally graded cylindrical shells in the framework of the extended finite element method. Compos. Struct. 2017, 159, 548–566. [Google Scholar] [CrossRef]
- Ruocco, E.; Mallardo, V.; Minutolo, V.; Di Giacinto, D. Analytical solution for buckling of Mindlin plates subjected to arbitrary boundary conditions. Appl. Math. Model. 2017, 50, 497–508. [Google Scholar] [CrossRef]
- Ruocco, E.; Minutolo, V. Buckling Analysis of Mindlin Plates Under the Green–Lagrange Strain Hypothesis. Int. J. Struct. Stab. Dyn. 2015, 15, 1450079. [Google Scholar] [CrossRef]
- Dolbow, J.; Gosz, M. On the computation of mixed-mode stress intensity factors in functionally graded materials. Int. J. Solids Struct. 2002, 39, 2557–2574. [Google Scholar] [CrossRef]
- Zou, Z.; Wu, S.X.; Li, C. On the Multiple Isoparametric Finite Element Method and Computation of Stress Intensity Factor for Cracks in FGMs. Key Eng. Mater. 2000, 183–187, 511–516. [Google Scholar] [CrossRef]
- Minutolo, V.; Ruocco, E.; Ciaramella, S. Isoparametric FEM vs. BEM for elastic Functionally Graded Materials. CMES—Comput. Modeling Eng. Sci. 2009, 41, 27–48. [Google Scholar]
- Zhang, C.; Sládek, J.; Sládek, V. Numerical Analysis of Cracked Functionally Graded Materials. Key Eng. Mater. 2003, 251–252, 463–472. [Google Scholar] [CrossRef]
- Sutradhar, A.; Paulino, G.H.; Gray, L.J. On hypersingular surface integrals in the symmetric Galerkin boundary element method: Application to heat conduction in exponentially graded materials. Int. J. Numer. Methods Eng. 2004, 62, 122–157. [Google Scholar] [CrossRef]
- Zhang, C.; Sládek, J.; Sladek, V. Effects of material gradients on transient dynamic mode-III stress intensity factors in a FGM. Int. J. Solids Struct. 2003, 40, 5251–5270. [Google Scholar] [CrossRef]
- Zhang, C.; Sládek, J.; Sladek, V. Antiplane crack analysis of a functionally graded material by a BIEM. Comput. Mater. Sci. 2005, 32, 611–619. [Google Scholar] [CrossRef]
- Zhang, C.; Sladek, J.; Sladek, V. Transient dynamic analysis of cracked functionally graded materials. In Advances in Fracture and Damage Mechanics IV; EC Press: London, UK, 2005. [Google Scholar]
- Chan, Y.-S.; Paulino, G.H.; Fannjiang, A.C. The crack problem for nonhomogeneous materials under antiplane shear loading—A displacement based formulation. Int. J. Solids Struct. 2001, 38, 2989–3005. [Google Scholar] [CrossRef]
- Yue, Z.; Xiao, H.; Tham, L. Boundary element analysis of crack problems in functionally graded materials. Int. J. Solids Struct. 2003, 40, 3273–3291. [Google Scholar] [CrossRef]
- Nguyen, B.; Tran, H.; Anitescu, C.; Zhuang, X.; Rabczuk, T. An isogeometric symmetric Galerkin boundary element method for two-dimensional crack problems. Comput. Methods Appl. Mech. Eng. 2016, 306, 252–275. [Google Scholar] [CrossRef]
- Falini, A.; Kanduč, T. A Study on Spline Quasi-Interpolation Based Quadrature Rules for the Isogeometric Galerkin BEM; Springer Science and Business Media LLC: Berlin, Germany, 2019; pp. 99–125. [Google Scholar]
- Chen, L.; Wang, Z.; Peng, X.; Yang, J.; Wu, P.; Lian, H. Modeling pressurized fracture propagation with the isogeometric BEM. Géoméch. Geophys. Geo-Energy Geo-Resour. 2021, 7, 1–16. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Emad, S.; Amir, R.M. Free vibration analysis of functionally graded hybrid matrix/fiber nanocomposite conical shells using multiscale method. Aerosp. Sci. Technol. 2020, 105, 105998. [Google Scholar] [CrossRef]
- Jia, B.Y.; Xin, D.; Tao, W. Behaviors of novel sandwich composite beams with normal weight concrete. Steel Compos. Struct. 2021, 38, 599–615. [Google Scholar]
- Rezaiee-Pajand, M.; Masoodi, A. Stability Analysis of Frame Having FG Tapered Beam–Column. Int. J. Steel Struct. 2018, 19, 446–468. [Google Scholar] [CrossRef]
- Sobhani, E.; Masoodi, A.R.; Ahmadi-Pari, A.R. Vibration of FG-CNT and FG-GNP Sandwich Composite Coupled Conical-Cylindrical-Conical Shell. Compos. Struct. 2021, 273, 114281. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Masoodi, A.R.; Alepaighambar, A. Lateral-torsional buckling of functionally graded tapered I-beams considering lateral bracing. Steel Compos. Struct. 2018, 28, 403–414. [Google Scholar]
- Rezaiee-Pajand, M.; Masoodi, A.R. Analyzing FG shells with large deformations and finite rotations. World J. Eng. 2019, 16, 636–647. [Google Scholar] [CrossRef]
- Mohammad, R.-P.; Niloofar, R.-S.; Masoodi, A.R. An efficient curved beam element for thermo-mechanical nonlinear analysis of functionally graded porous beams. Structures 2020, 28, 1035–1049. [Google Scholar]
- Brebbia, C.; Dominguez, J. Boundary Elements. An Introductory Course; WIT: Southempton, UK, 1990. [Google Scholar]
- Diligenti, M.; Monegato, G. Integral evaluation in the BEM solution of (hyper)singular integral equations. 2D problems on polygonal domains. J. Comput. Appl. Math. 1997, 81, 29–57. [Google Scholar] [CrossRef]
- Belytschko, T.; Bathe, K.J. Computational Methods in the Mechanics of Fracture; Atluri, S., Ed.; North Holland Publishing Company: Atlanta, GA, USA, 1986; Volume 2. [Google Scholar]
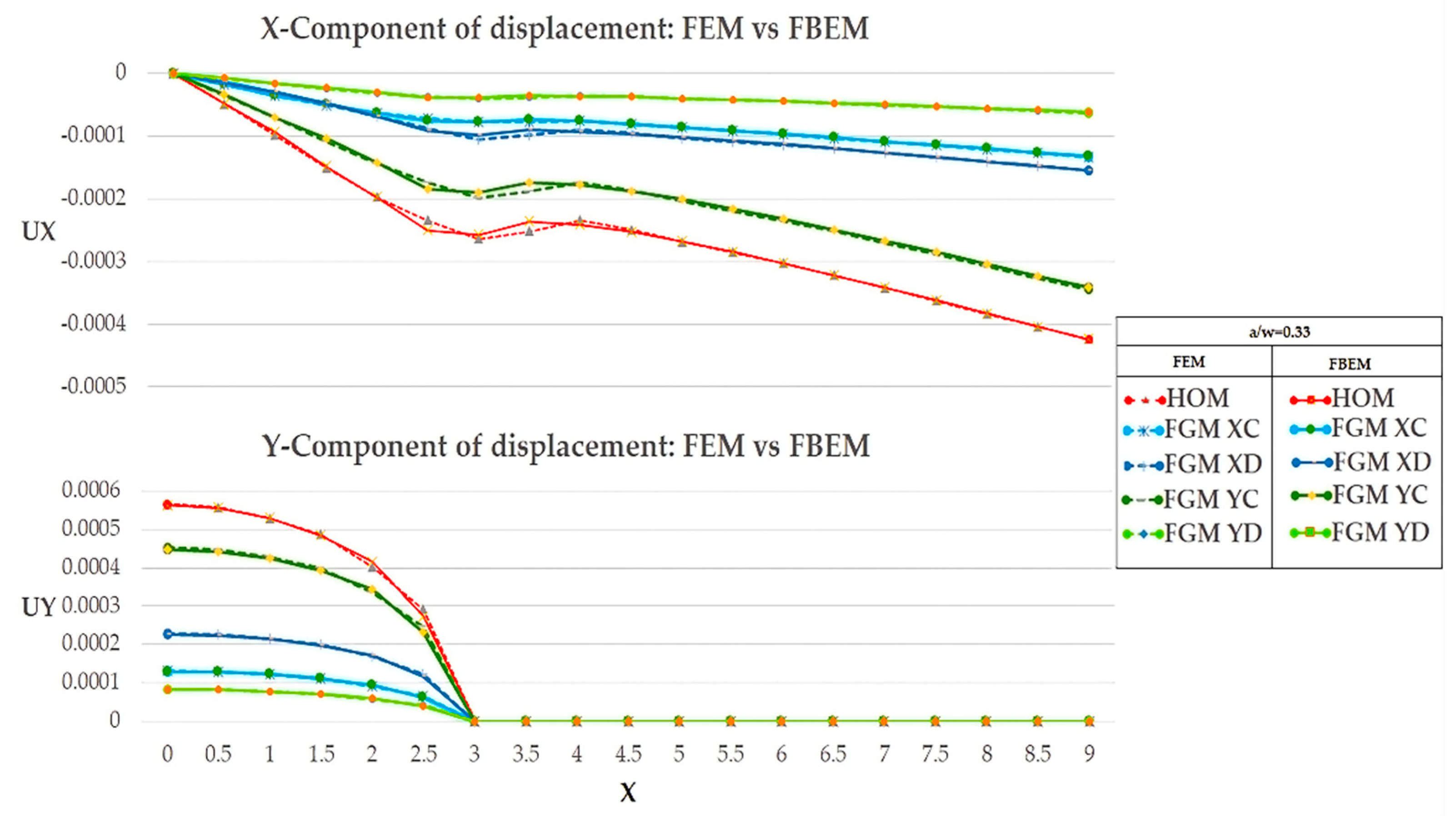
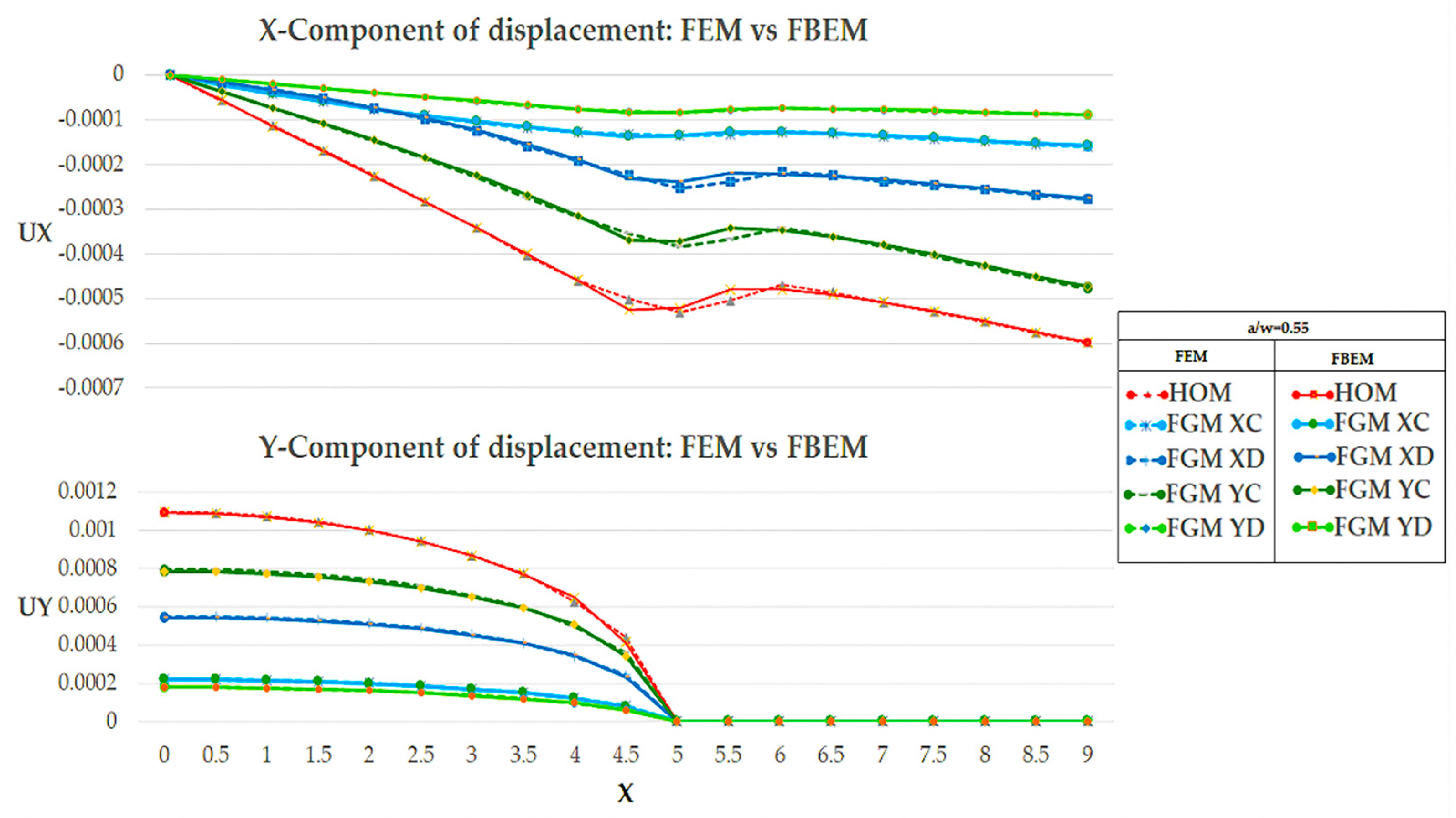
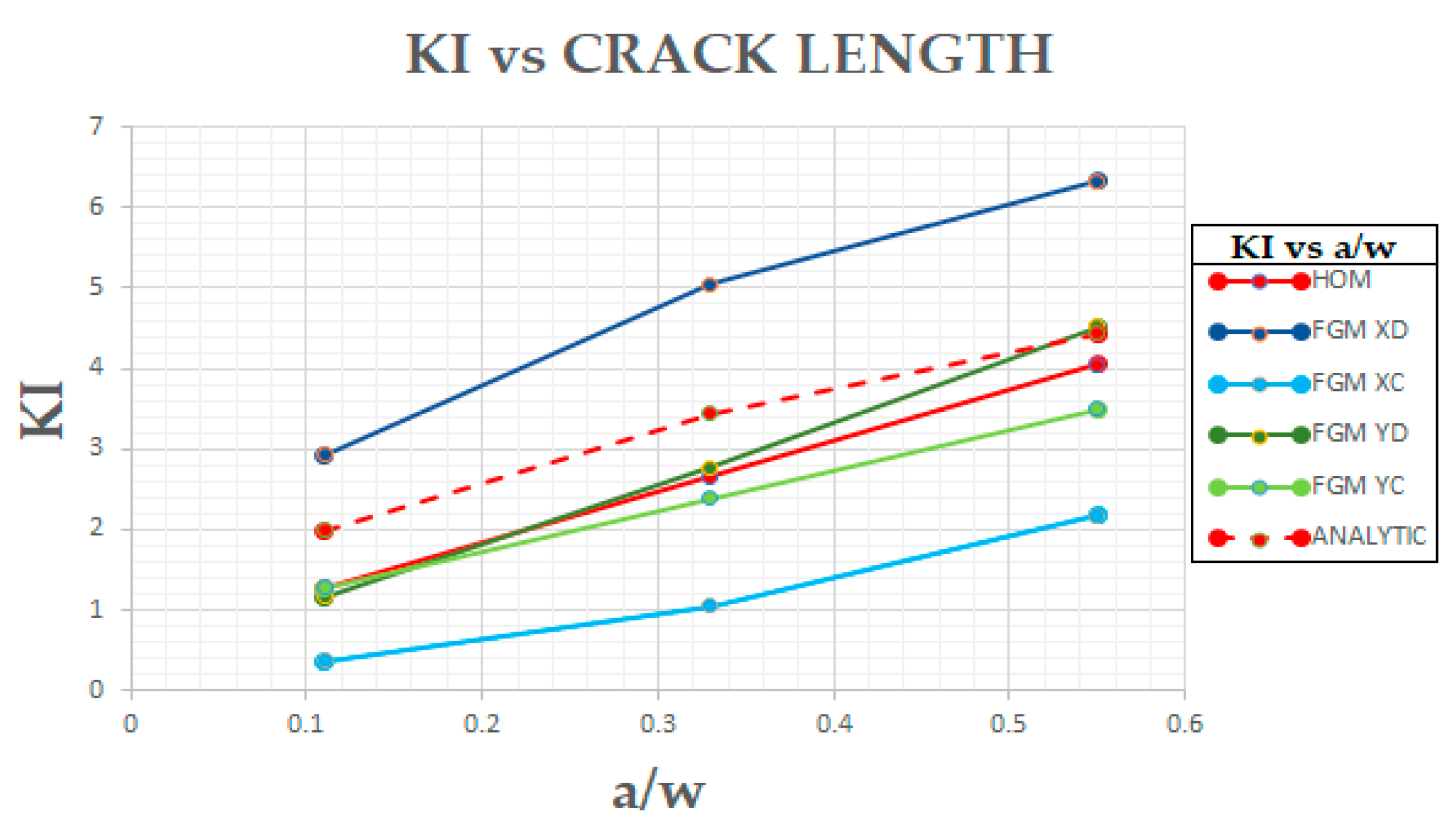
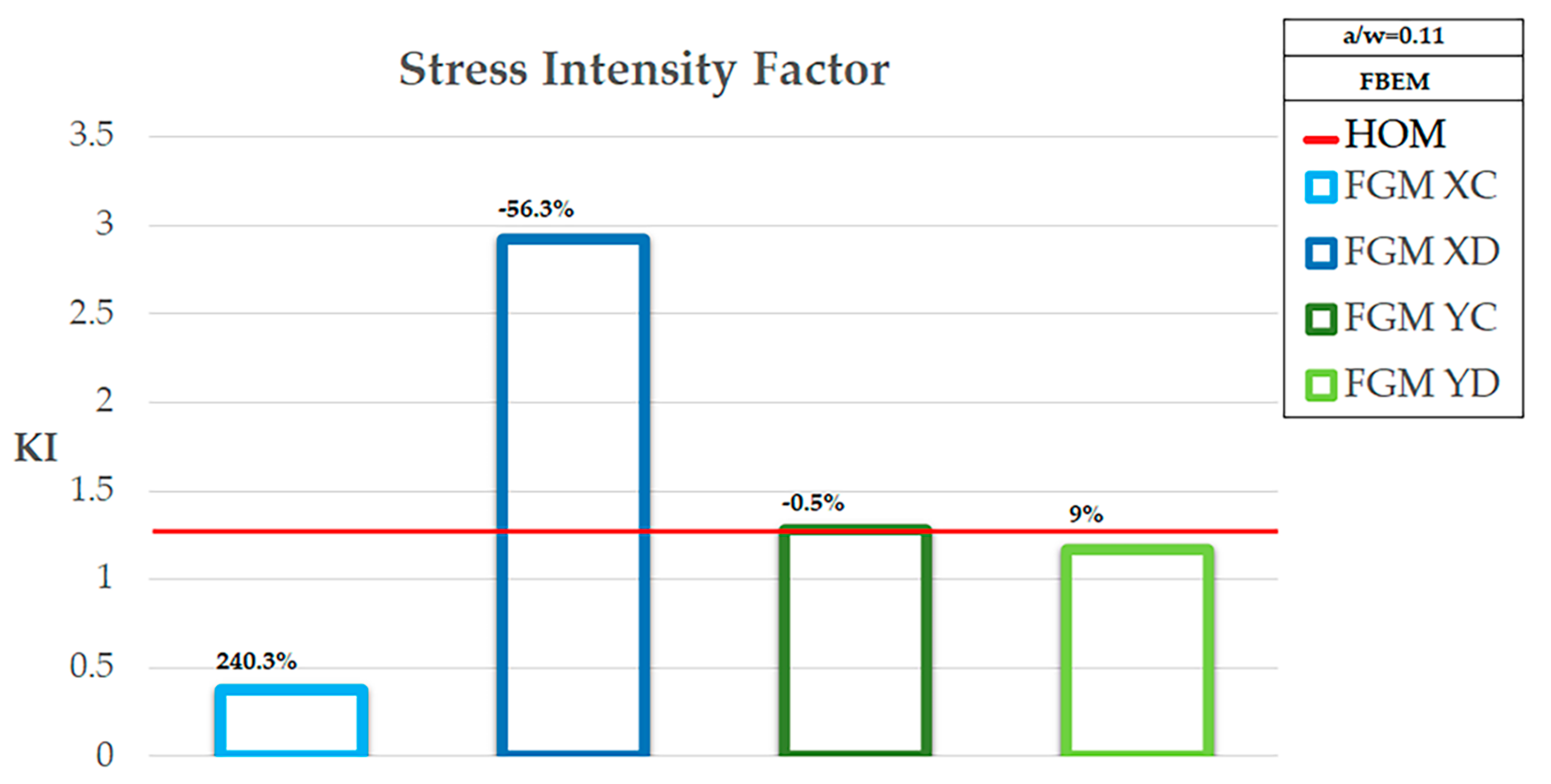
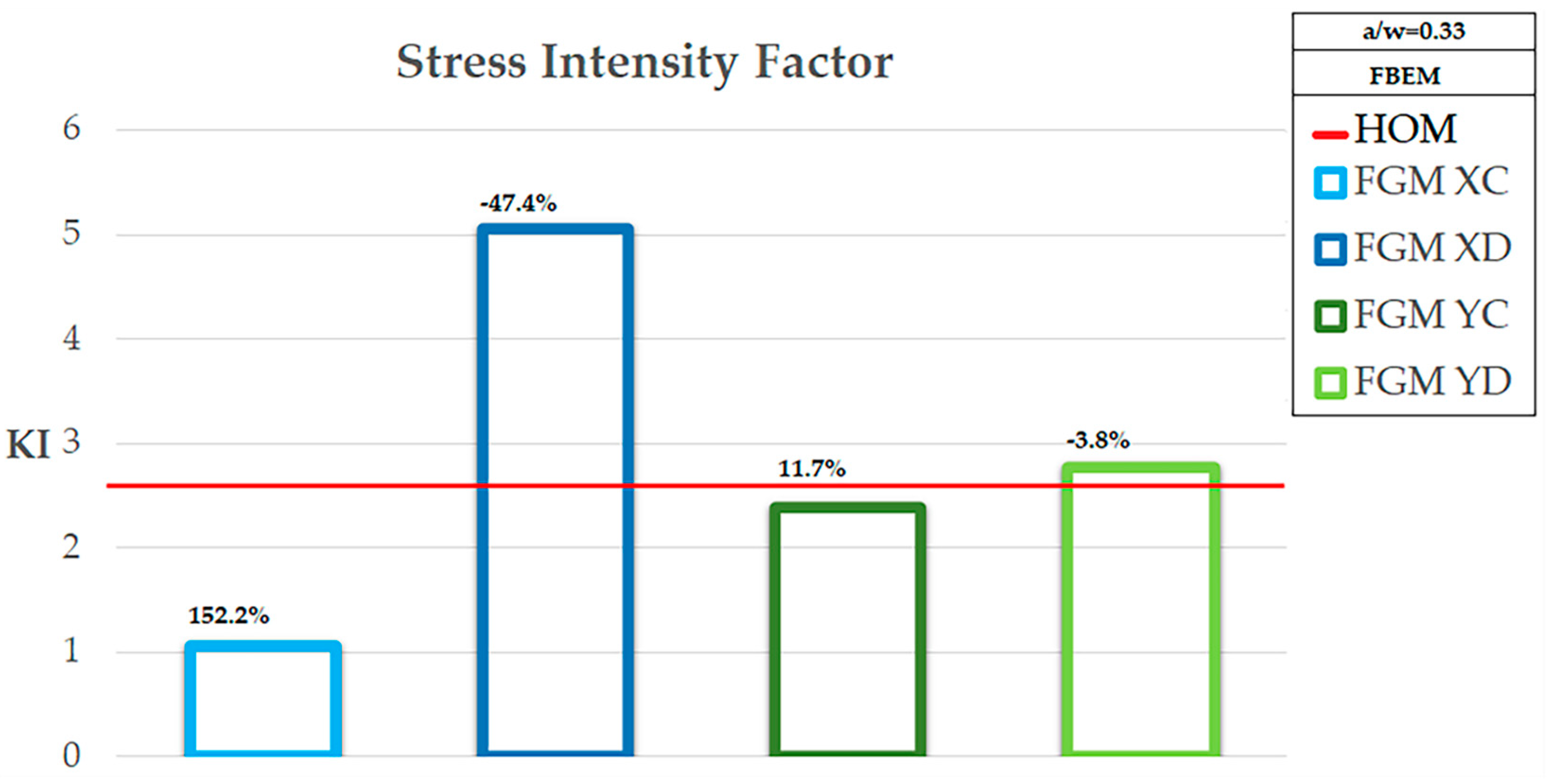
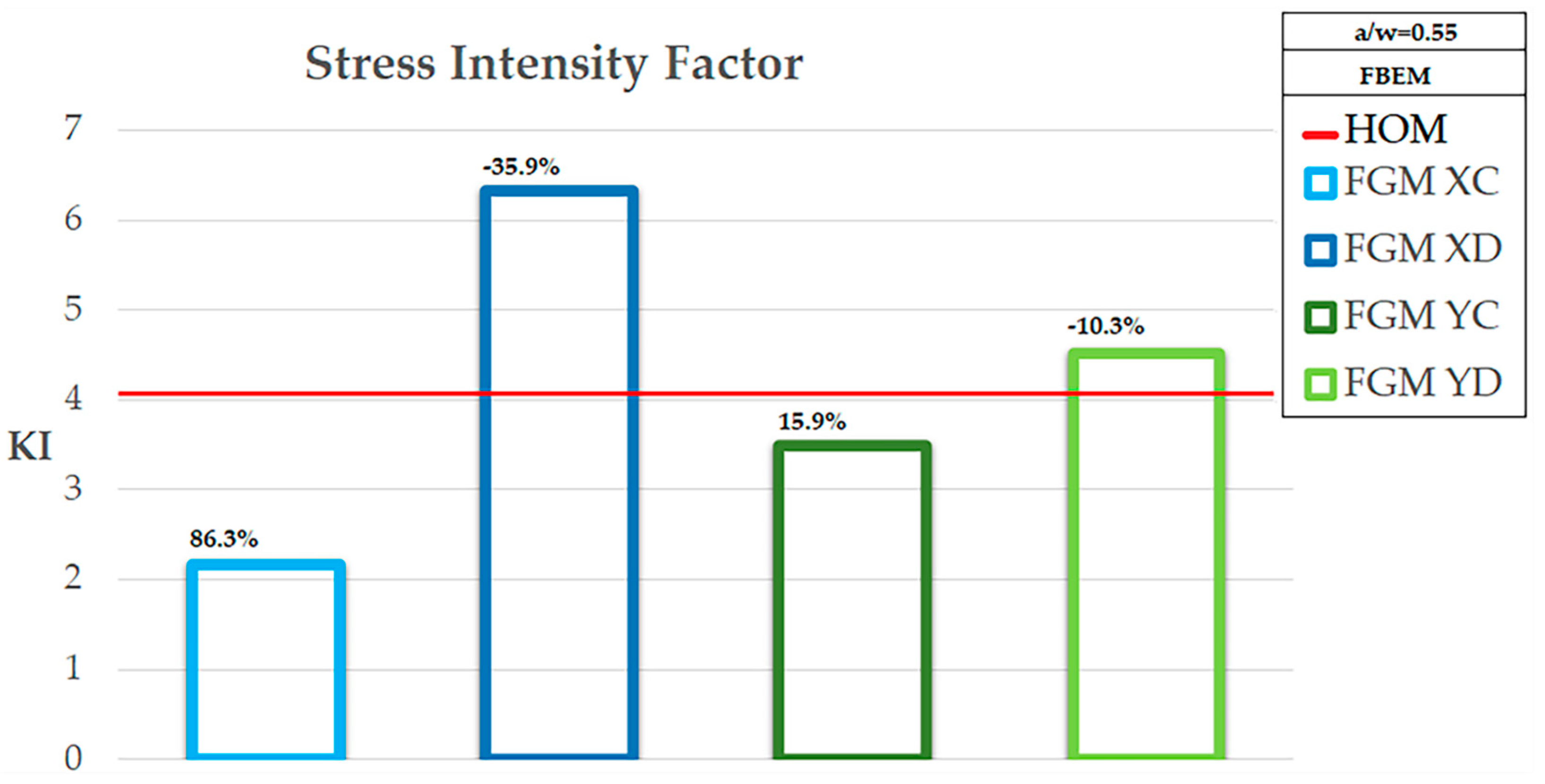
| [w = l] | ||||||
|---|---|---|---|---|---|---|
| 0.11 | 1.98515 | 1.27675 | 2.92406 | 0.37523 | 1.17085 | 1.28338 |
| 0.33 | 3.43838 | 2.66062 | 5.05537 | 1.05479 | 2.76551 | 2.38274 |
| 0.55 | 4.43893 | 4.05522 | 6.32927 | 2.17639 | 2.26057 | 3.49758 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palladino, S.; Esposito, L.; Ferla, P.; Zona, R.; Minutolo, V. Functionally Graded Plate Fracture Analysis Using the Field Boundary Element Method. Appl. Sci. 2021, 11, 8465. https://doi.org/10.3390/app11188465
Palladino S, Esposito L, Ferla P, Zona R, Minutolo V. Functionally Graded Plate Fracture Analysis Using the Field Boundary Element Method. Applied Sciences. 2021; 11(18):8465. https://doi.org/10.3390/app11188465
Chicago/Turabian StylePalladino, Simone, Luca Esposito, Paolo Ferla, Renato Zona, and Vincenzo Minutolo. 2021. "Functionally Graded Plate Fracture Analysis Using the Field Boundary Element Method" Applied Sciences 11, no. 18: 8465. https://doi.org/10.3390/app11188465
APA StylePalladino, S., Esposito, L., Ferla, P., Zona, R., & Minutolo, V. (2021). Functionally Graded Plate Fracture Analysis Using the Field Boundary Element Method. Applied Sciences, 11(18), 8465. https://doi.org/10.3390/app11188465








