Control of a Variable Blade Pitch Wind Turbine Subject to Gust Wind and Actuators Saturation
Abstract
:1. Introduction
2. Materials and Methods
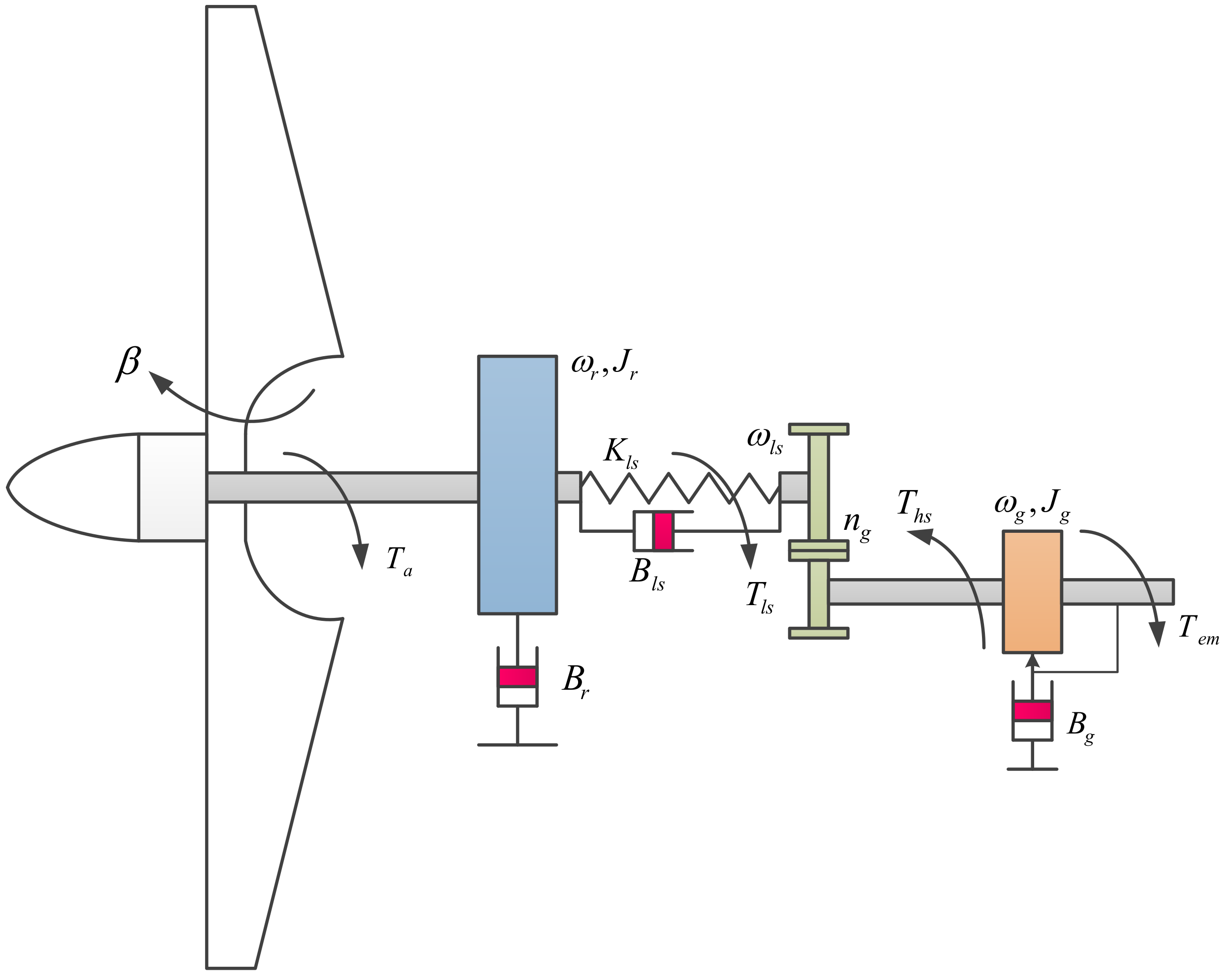
2.1. Wind Generator Dynamic Model
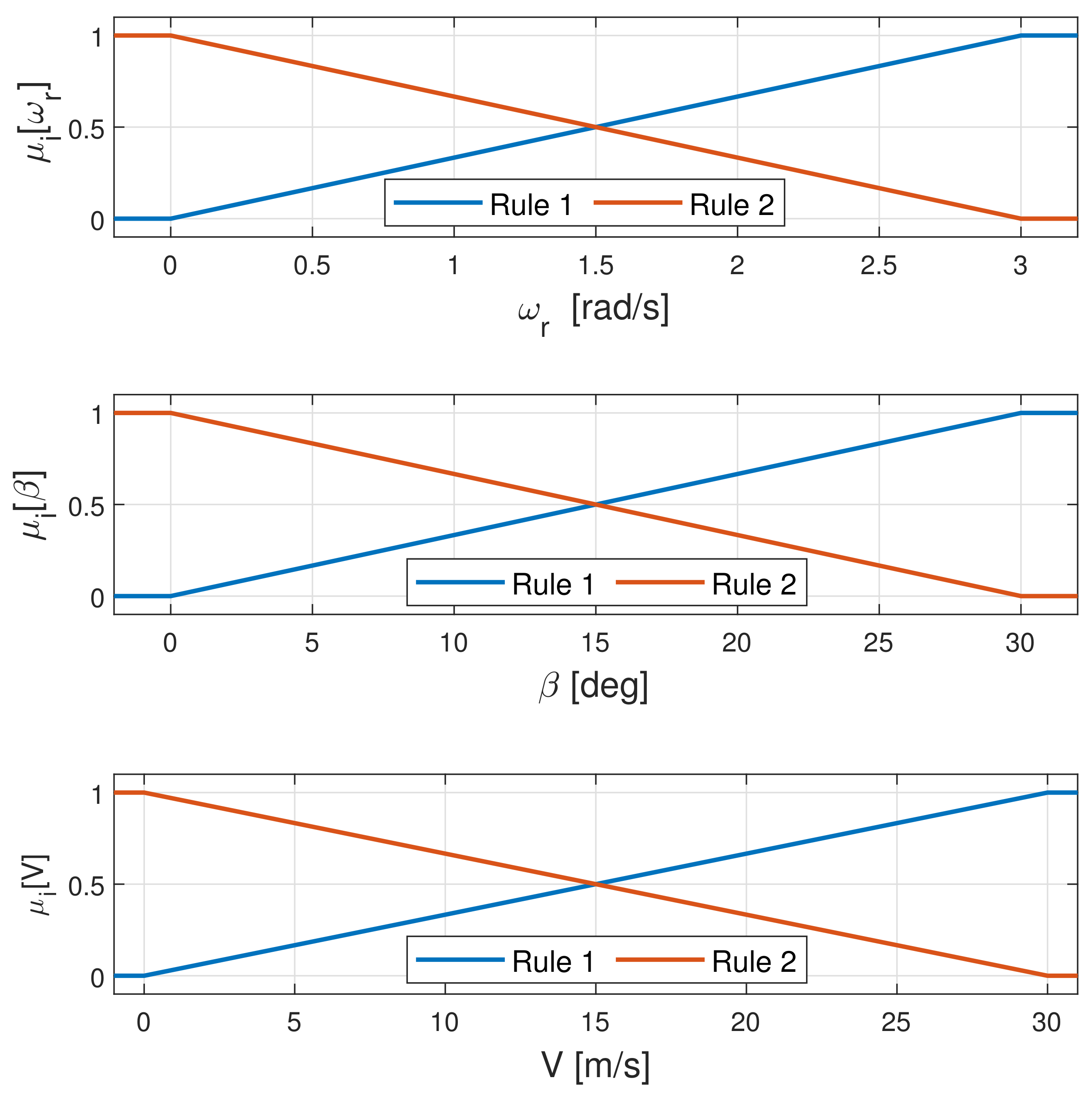
2.2. Takagi–Sugeno Fuzzy Model
- They are cheaper to develop, they cover a wider range of operating conditions, and they are more readily customizable in natural language terms.
- They are quick to comprehend conceptually, the ideas underlying them are fundamental.
- They are flexible, they enable emerging Fuzzy structures to be applied to their features by applying new information to established rules.
- They are tolerant of incorrect data, complex uncertainty, and unmodeled dynamics.
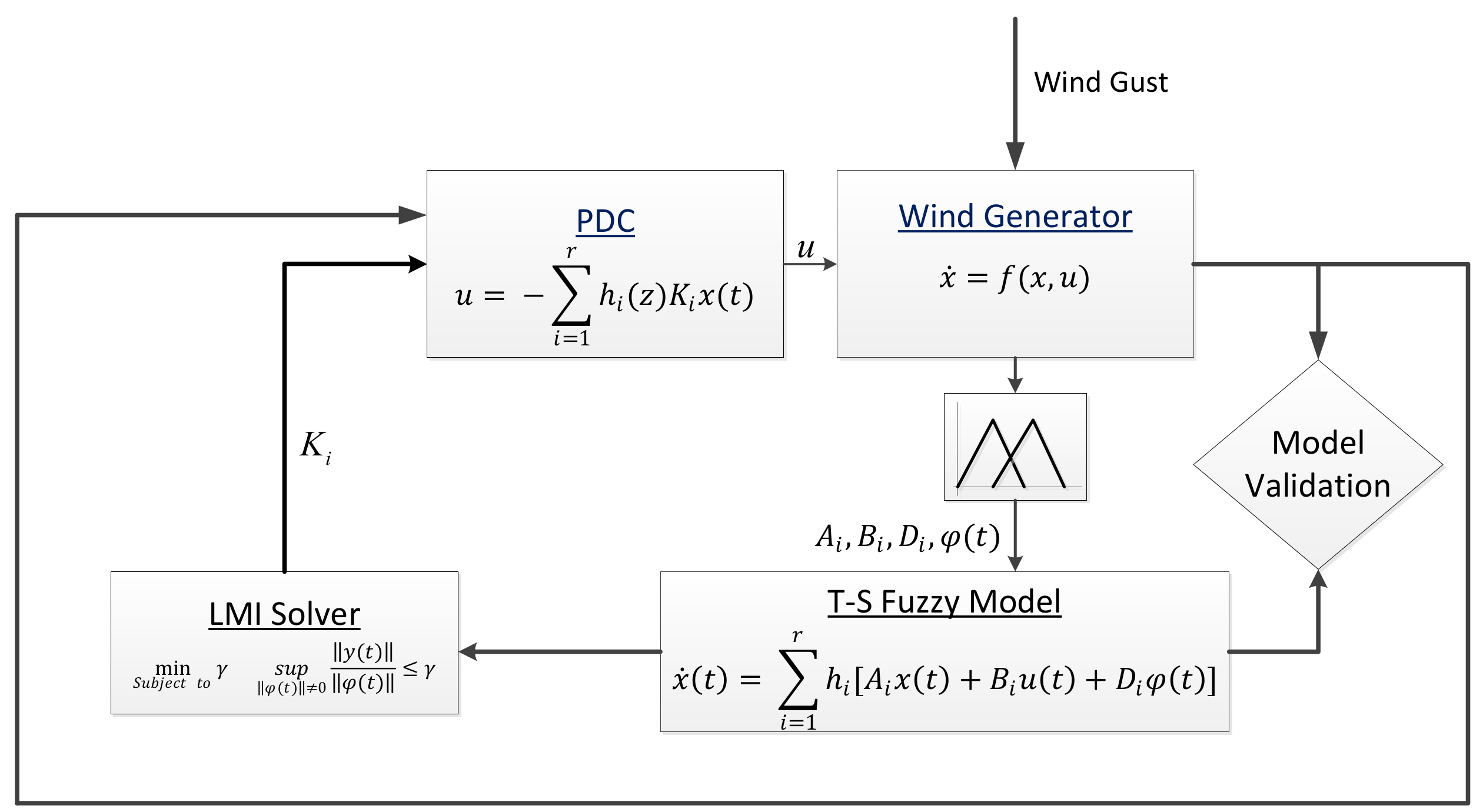
2.3. Fuzzy Controller Design
2.4. Parallel Distributed Compensation Control
2.5. Controller Structure
3. Results
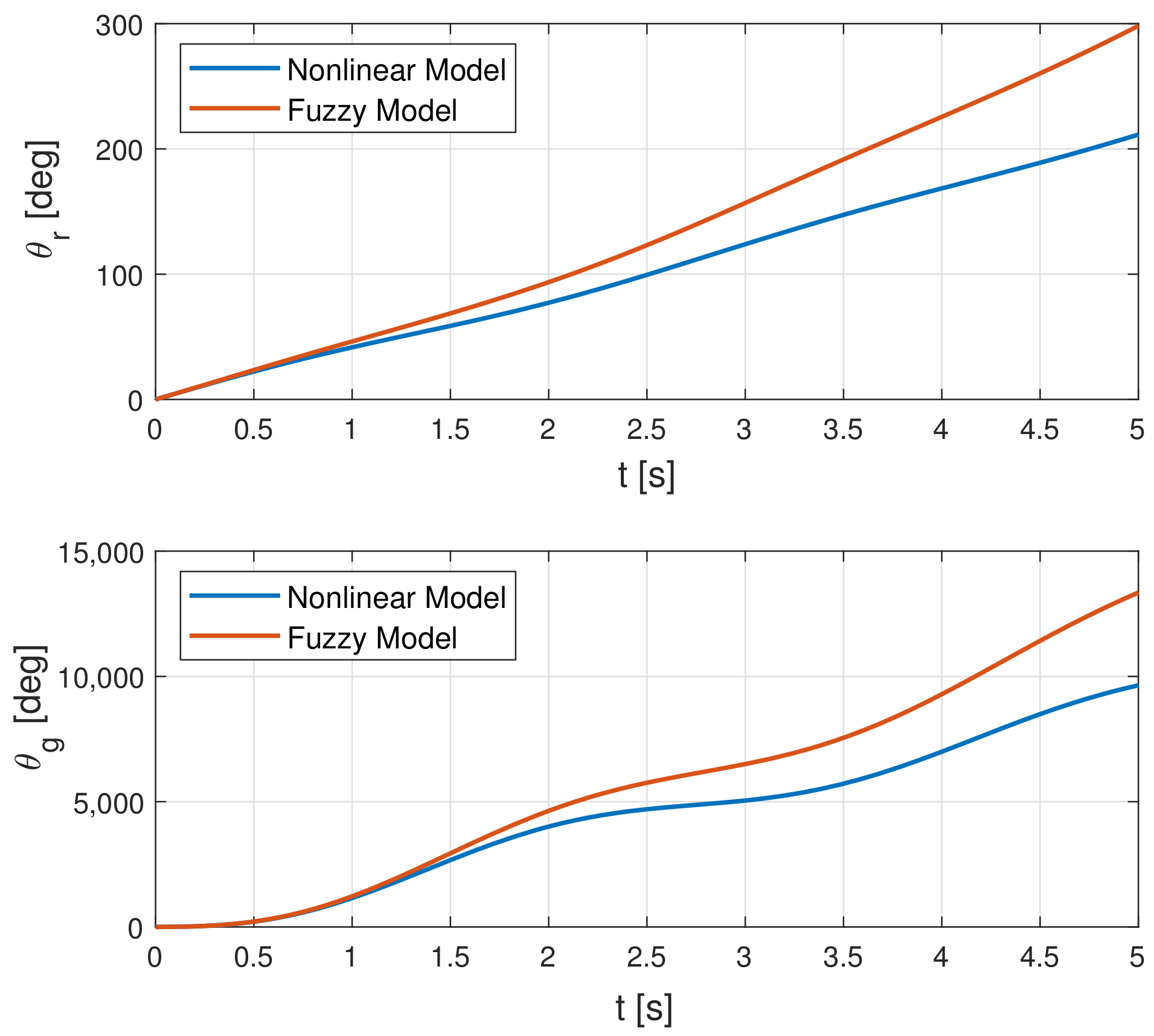
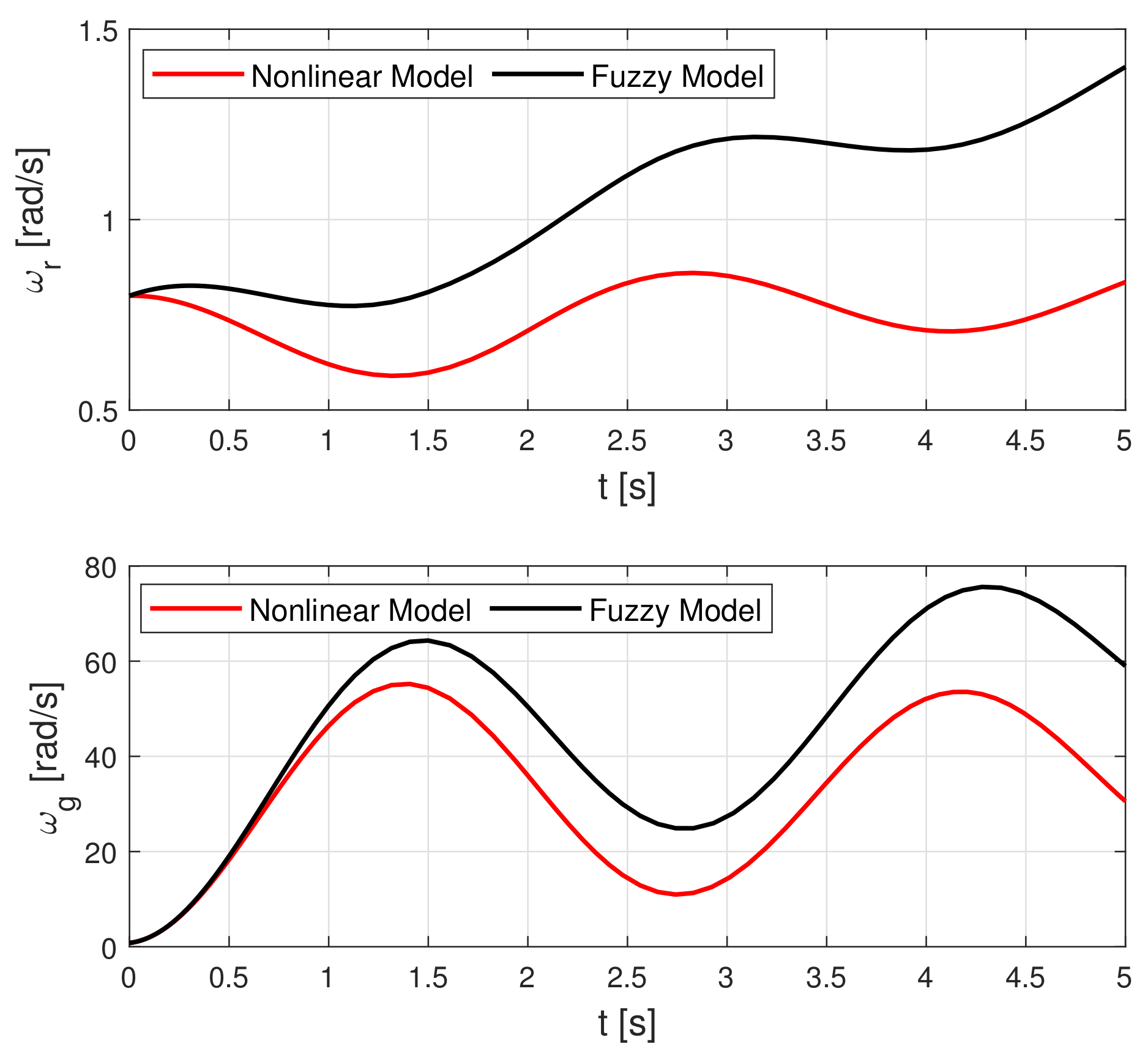
3.1. Fuzzy Model Validation
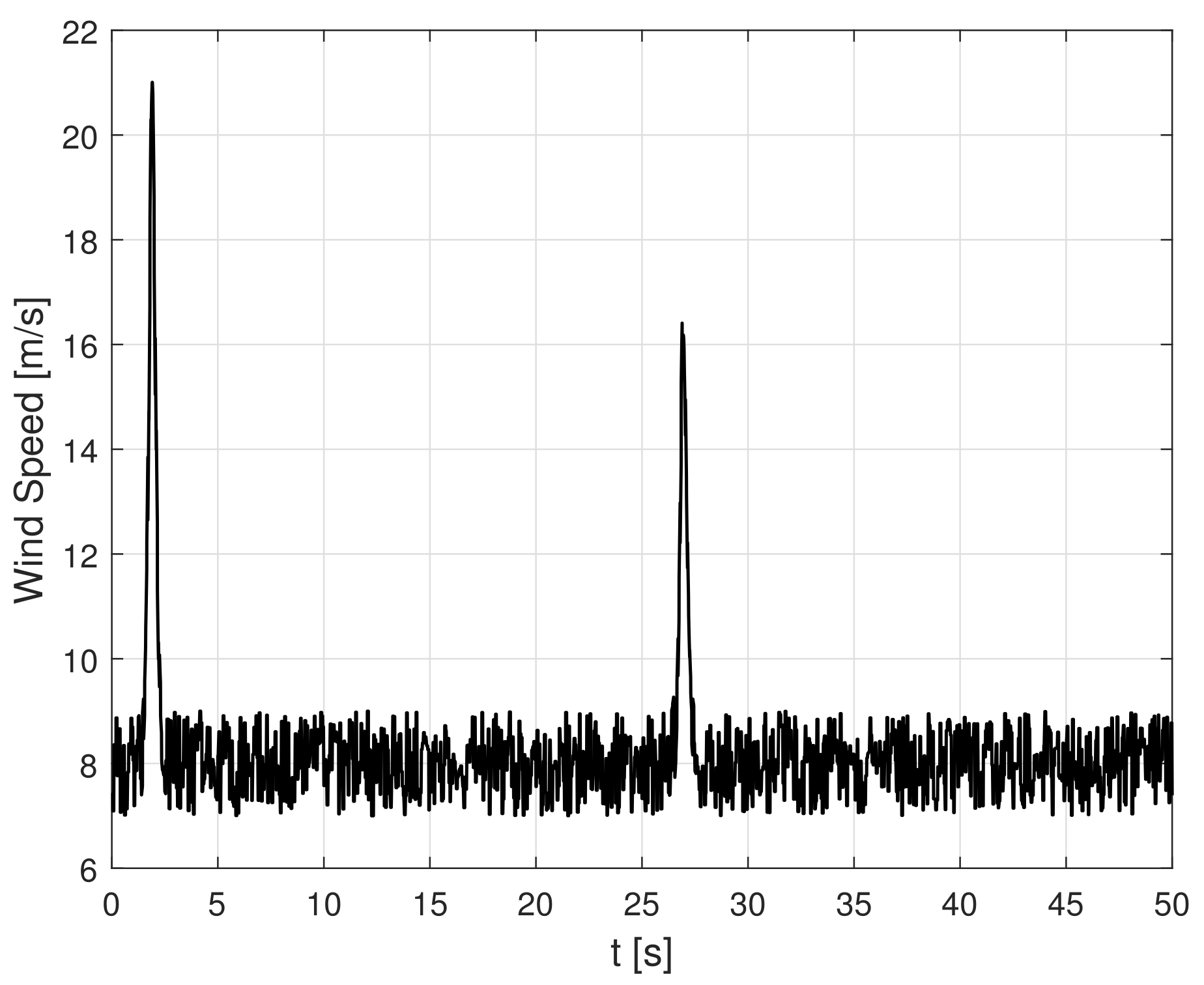
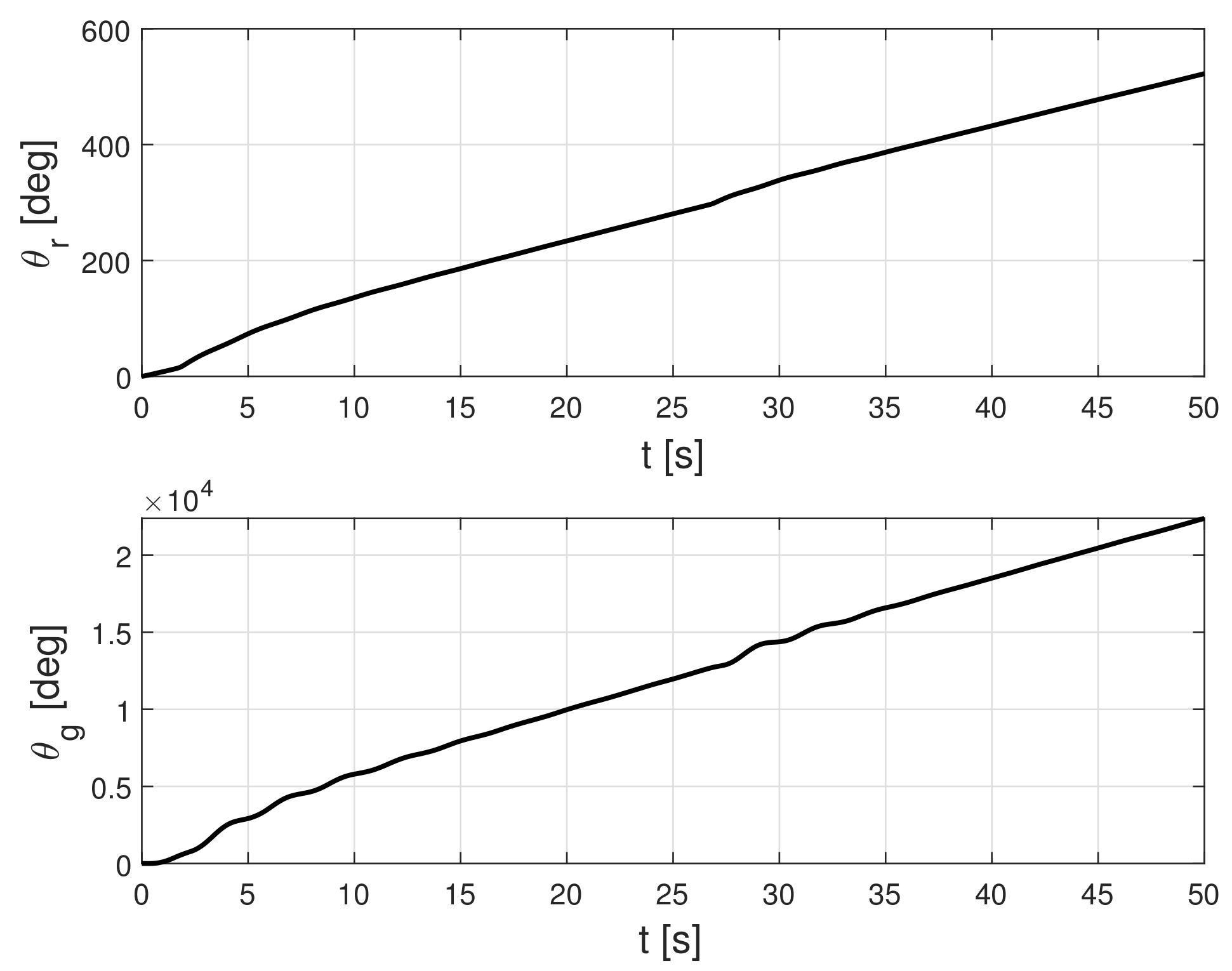
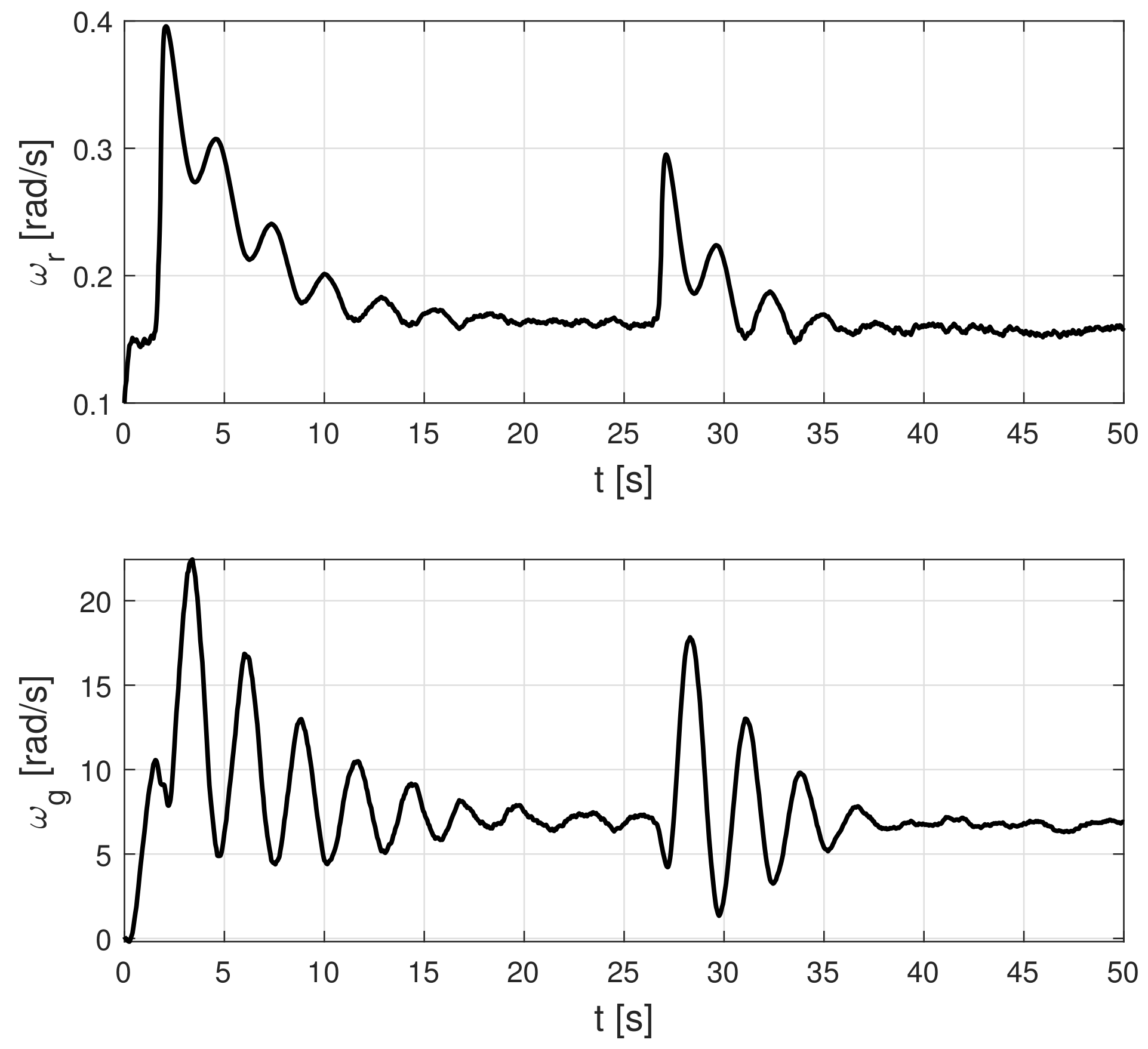
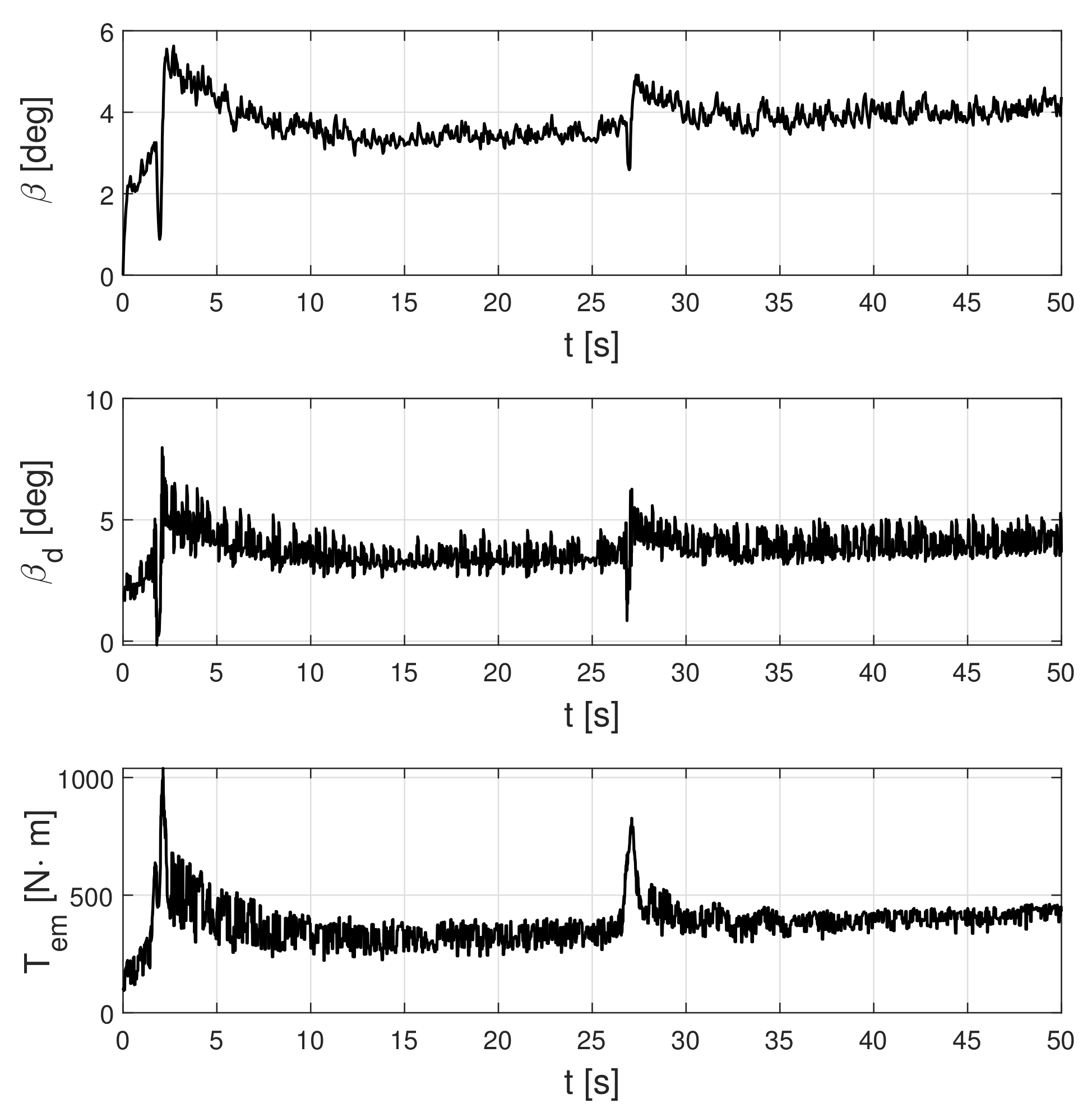
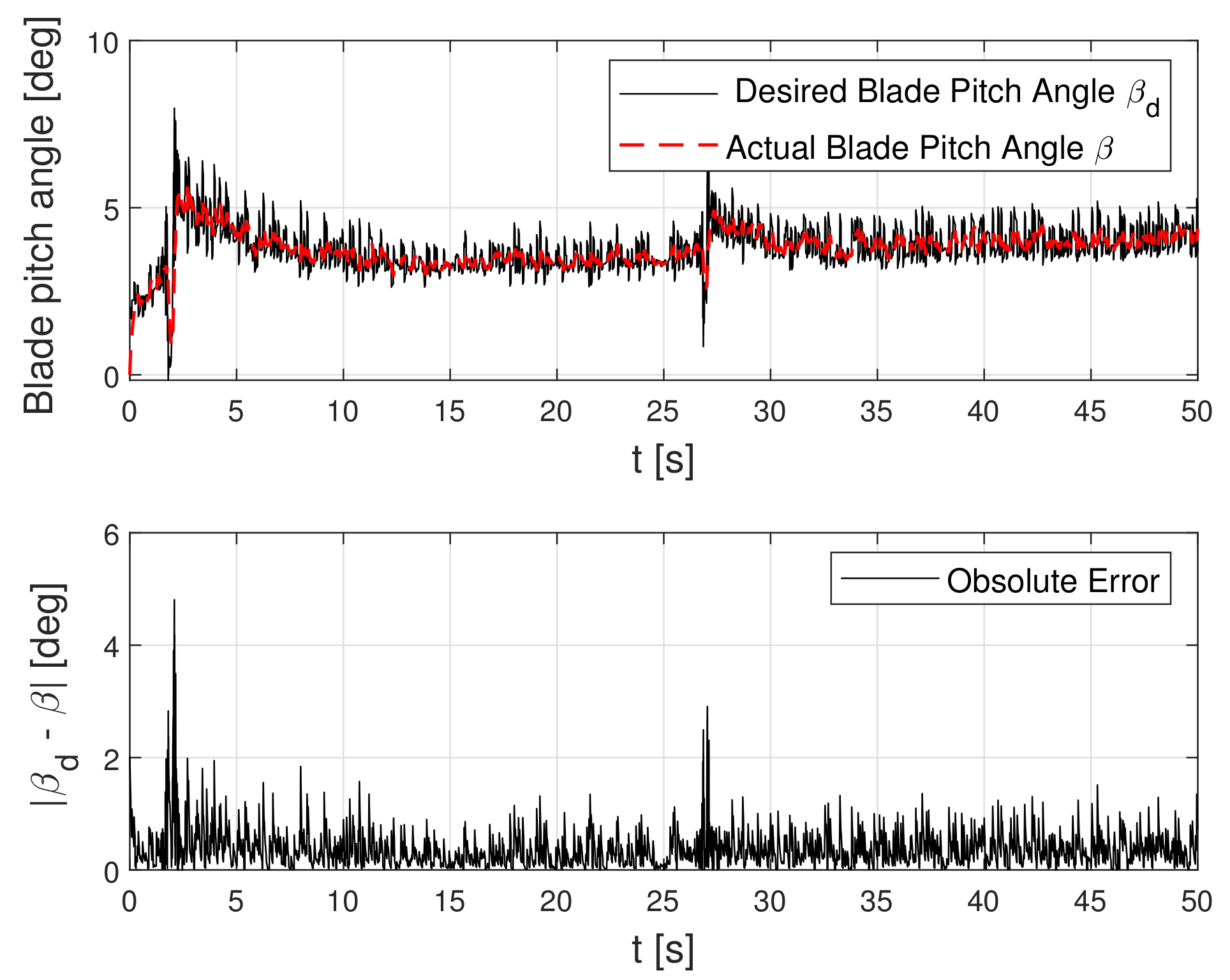
3.2. Numerical Results
4. Conclusions and Future Work
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ragheb, M.; Ragheb, A.M. Wind Turbines Theory—The Betz Equation and Optimal Rotor Tip Speed Ratio. In Fundamental and Advanced Topics in Wind Power; IntechOpen: London, UK, 2011. [Google Scholar] [CrossRef] [Green Version]
- Parvathy, R.; Daniel, A.E. A survey on active disturbance rejection control. In Proceedings of the 2013 International Mutli-Conference on Automation, Computing, Communication, Control and Compressed Sensing (iMac4s), Kottayam, India, 22–23 March 2013; pp. 330–335. [Google Scholar] [CrossRef]
- Rezaei, V. Advanced control of wind turbines: Brief survey, categorization, and challenges. In Proceedings of the 2015 American Control Conference (ACC), Chicago, IL, USA, 1–3 July 2015; pp. 3044–3051. [Google Scholar] [CrossRef]
- Achour, D.; Kesraoui, M.; Chaib, A.; Achour, A. Frequency Control of a Wind Turbine Generator–flywheel System. Wind Eng. 2017, 41, 397–408. Available online: https://www.jstor.org/stable/90015240 (accessed on 16 August 2021). [CrossRef]
- Colombo, L.; Corradini, M.L.; Ippoliti, G.; Orlando, G. Pitch angle control of a wind turbine operating above the rated wind speed: A sliding mode control approach. ISA Trans. 2020, 96, 95–102. [Google Scholar] [CrossRef] [PubMed]
- Martinez, M.I.; Susperregui, A.; Tapia, G.; Xu, L. Sliding-mode control of a wind turbine-driven double-fed induction generator under non-ideal grid voltages. IET Renew. Power Gener. 2013, 7, 370–379. [Google Scholar] [CrossRef]
- Sahoo, S.; Subudhi, B.; Panda, G. Torque and pitch angle control of a wind turbine using multiple adaptive neuro-fuzzy control. Wind Eng. 2020, 44, 125–141. [Google Scholar] [CrossRef]
- Hur, S.; Leithead, W.E. Model predictive and linear quadratic Gaussian control of a wind turbine. Optim. Control. Appl. Methods 2017, 38, 88–111. [Google Scholar] [CrossRef] [Green Version]
- Mechter, A.; Kemih, K.; Ghanes, M. Backstepping control of a wind turbine for low wind speeds. Nonlinear Dyn. 2016, 84, 2435–2445. [Google Scholar] [CrossRef]
- Vepa, R. Nonlinear, Optimal Control of a Wind Turbine Generator. IEEE Trans. Energy Convers. 2011, 26, 468–478. [Google Scholar] [CrossRef]
- Pedersen, N.H.; Johansen, P.; Andersen, T.O. Optimal control of a wind turbine with digital fluid power transmission. Nonlinear Dyn. 2018, 91, 591–607. [Google Scholar] [CrossRef]
- Asgharnia, A.; Shahnazi, R.; Jamali, A. Performance and robustness of optimal fractional fuzzy PID controllers for pitch control of a wind turbine using chaotic optimization algorithms. ISA Trans. 2018, 79, 27–44. [Google Scholar] [CrossRef]
- Feng, G. A Survey on Analysis and Design of Model-Based Fuzzy Control Systems. IEEE Trans. Fuzzy Syst. 2006, 14, 676–697. [Google Scholar] [CrossRef] [Green Version]
- Takagi, T.; Sugeno, M. Fuzzy Identification of Systems and its Applications to Modeling and Control. IEEE Trans. Syst. Man Cybern. 1985, 1, 116–132. [Google Scholar] [CrossRef]
- Tanaka, K.; Sugeno, M. Stability analysis of fuzzy systems using Lyapunov’s direct method. In Proceedings of the NAFIPS’90, Toronto, ON, Canada, 6–8 June 1990; pp. 133–136. [Google Scholar]
- Zheng, W.; Wang, H.; Wang, H.; Wen, S. Stability analysis and dynamic output feedback controller design of T–S fuzzy systems with time-varying delays and external disturbances. J. Comput. Appl. Math. 2019, 358, 111–135. [Google Scholar] [CrossRef]
- Zheng, K.; Hu, Y.; Wu, B. Intelligent fuzzy sliding mode control for complex robot system with disturbances. Eur. J. Control 2020, 51, 95–109. [Google Scholar] [CrossRef]
- Hong, S.-K.; Langari, R. Robust fuzzy control of a magnetic bearing system subject to harmonic disturbances. IEEE Trans. Control Syst. Technol. 2000, 8, 366–371. [Google Scholar] [CrossRef]
- Cao, Y.-Y.; Frank, P.M. Robust H∞ disturbance attenuation for a class of uncertain discrete-time fuzzy systems. IEEE Trans. Fuzzy Syst. 2000, 8, 406–415. [Google Scholar] [CrossRef]
- Yoneyama, J. H∞ filtering for fuzzy systems with immeasurable premise variables: An uncertain system approach. Fuzzy Sets Syst. 2009, 160, 1738–1748. [Google Scholar] [CrossRef]
- Gao, Z.; Shi, X.; Ding, S.X. Fuzzy state/disturbance observer design for (T–S) fuzzy systems with application to sensor fault estimation. IEEE Trans. Syst. Man Cybern. Part B (Cybern.) 2008, 38, 875–880. [Google Scholar] [CrossRef]
- Vu, V.; Wang, W. State/Disturbance Observer and Controller Synthesis for the T–S Fuzzy System With an Enlarged Class of Disturbances. IEEE Trans. Fuzzy Syst. 2018, 26, 3645–3659. [Google Scholar] [CrossRef]
- Kim, E. A fuzzy disturbance observer and its application to control. IEEE Trans. Fuzzy Syst. 2002, 10, 77–84. [Google Scholar] [CrossRef]
- Zhang, J.; Shi, P.; Xia, Y. Robust Adaptive Sliding-Mode Control for Fuzzy Systems With Mismatched Uncertainties. IEEE Trans. Fuzzy Syst. 2010, 18, 700–711. [Google Scholar] [CrossRef]
- Chwa, D. Fuzzy Adaptive Tracking Control of Wheeled Mobile Robots With State-Dependent Kinematic and Dynamic Disturbances. IEEE Trans. Fuzzy Syst. 2012, 20, 587–593. [Google Scholar] [CrossRef]
- Chen, W.; Jiao, L.; Li, R.; Li, J. Adaptive Backstepping Fuzzy Control for Nonlinearly Parameterized Systems with Periodic Disturbances. IEEE Trans. Fuzzy Syst. 2010, 18, 674–685. [Google Scholar] [CrossRef]
- Yue, H.; Li, J. Output-feedback adaptive fuzzy control for a class of nonlinear systems with input delay and unknown control directions. J. Frankl. Inst. 2013, 350, 129–154. [Google Scholar] [CrossRef]
- Zhou, Q.; Shi, P.; Xu, S.; Li, H. Adaptive Output Feedback Control for Nonlinear Time-Delay Systems by Fuzzy Approximation Approach. IEEE Trans. Fuzzy Syst. 2013, 21, 301–313. [Google Scholar] [CrossRef]
- González-Yero, G.; Ramírez Leyva, R.; Ramírez Mendoza, M.; Albertos, P.; Crespo-Lorente, A.; Reyes Alonso, J.M. Neuro-Fuzzy System for Compensating Slow Disturbances in Adaptive Mold Level Control. Metals 2021, 11, 56. [Google Scholar] [CrossRef]
- Civelek, Z. Optimization of fuzzy logic (Takagi-Sugeno) blade pitch angle controller in wind turbines by genetic algorithm. Eng. Sci. Technol. 2020, 23, 1–9. [Google Scholar] [CrossRef]
- Teng, L.; Wang, Y.; Cai, W.; Li, H. Efficient Robust Fuzzy Model Predictive Control of Discrete Nonlinear Time-Delay Systems via Razumikhin Approach. IEEE Trans. Fuzzy Syst. 2018, 27, 262–272. [Google Scholar] [CrossRef]
- Li, H.; Wang, J.; Wu, L.; Lam, H.K.; Gao, Y. Optimal Guaranteed Cost Sliding-Mode Control of Interval Type-2 Fuzzy Time-Delay Systems. IEEE Trans. Fuzzy Syst. 2018, 26, 246–257. [Google Scholar] [CrossRef] [Green Version]
- Boukhezzar, B.; Siguerdidjane, H. Comparison between linear and nonlinear control strategies for variable speed wind turbines. Control Eng. Pract. 2010, 18, 1357–1368. [Google Scholar] [CrossRef]
- Waleed, K. Mechanical modelling of wind turbine: Comparative study. Int. J. Renew. Energy Res. 2013, 3, 94–97. [Google Scholar]
- Milivojevic, N.; Schofield, N.; Stamenkovic, I.; Gurkaynak, Y. Field weakening control of PM generator used for small wind turbine aplication. In Proceedings of the IET Conference on Renewable Power Generation (RPG 2011), Edinburgh, UK, 6–8 September 2011; p. 191. [Google Scholar] [CrossRef]
- Bozhko, S.; Rashed, M.; Hill, C.I.; Yeoh, S.S.; Yang, T. Flux-Weakening Control of Electric Starter–Generator Based on Permanent-Magnet Machine. IEEE Trans. Transp. Electrif. 2017, 3, 864–877. [Google Scholar] [CrossRef]
- Gallegos-Lopez, G.; Gunawan, F.S.; Walters, J.E. Optimum torque control of permanent-magnet AC machines in the field-weakened region. IEEE Trans. Ind. Appl. 2005, 41, 1020–1028. [Google Scholar] [CrossRef]
- Maric, D.S.; Hiti, S.; Stancu, C.C.; Nagashima, J.M. Two improved flux weakening schemes for surface mounted permanent magnet synchronous machine drives employing space vector modulation. In Proceedings of the 24th Annual Conference of the IEEE Industrial Electronics Society (IECON’98), Aachen, Germany, 31 August–4 September 1998; Volume 1, pp. 508–512. [Google Scholar]
- Guo, X.; Wen, X.; Zhao, F.; Song, X. PI parameter design of the flux weakening control for PMSM based on small signal and transfer function. In Proceedings of the 2009 International Conference on Electrical Machines and Systems (ICEMS), Tokyo, Japan, 15–18 November 2009; pp. 1–6. [Google Scholar]
- Bolognani, S.; Calligaro, S.; Petrella, R. Adaptive flux-weakening controller for interior permanent magnet synchronous motor drives. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 236–248. [Google Scholar] [CrossRef]
- Ganjefar, S.; Mohammadi, A. Variable speed wind turbines with maximum power extraction using singular perturbation theory. Energy 2016, 106, 510–519. [Google Scholar] [CrossRef]
- Georg, S.; Schulte, H.; Aschemann, H. Control-oriented modelling of wind turbines using a Takagi-Sugeno model structure. In Proceedings of the 2012 IEEE International Conference on Fuzzy Systems, Brisbane, QLD, Australia, 10–15 June 2012; pp. 1–8. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.O.; Tanaka, K. Parallel Distributed Compensation of Nonlinear Systems by Takagi-Sugeno Fuzzy Model. In Proceedings of the 1995 IEEE International Conference on Fuzzy Systems, Yokohama, Japan, 20–24 March 1995; Volume 2, pp. 531–538. [Google Scholar] [CrossRef]
- Tanaka, K.; Wang, H.O. Fuzzy Control Systems Design and Analysis: A Linear Matrix Inequality Approach; John Wiley & Sons: New York, NY, USA, 2001; pp. 66–83. [Google Scholar]
- Wang, L.X.; Mendel, J.M. Fuzzy basis functions, universal approximation, and orthogonal least-squares learning. IEEE Trans. Neural Netw. 1992, 3, 807–814. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wan, F.; Shang, H.; Wang, L.-X.; Sun, Y.-X. How to determine the minimum number of fuzzy rules to achieve given accuracy: A computational geometric approach to SISO case. Fuzzy Sets Syst. 2005, 150, 199–209. [Google Scholar] [CrossRef]
- L<i>o</i>¨fberg, J. YALMIP: A toolbox for modeling and optimization in MATLAB. In Proceedings of the CACSD Conference, Taipei, Taiwan, 2–4 September 2004. [Google Scholar]
- Simani, S. Overview of Modelling and Advanced Control Strategies for Wind Turbine Systems. J. Energ. 2015, 8, 13395–13418. [Google Scholar] [CrossRef] [Green Version]

| Parameters | Symbole | Values |
|---|---|---|
| Rotor radius | R | 21.65 m |
| Rotor inertia | 34.4 kg·m | |
| Generator inertia | 34.4 kg·m | |
| Shaft damping coeff | 9500 N·m/rad·s | |
| Shaft stiffness coeff | 2.691×10 N·m/rad·s | |
| Rotor friction coeff | 27.36 N·m/rad·s | |
| Generator friction coeff | 0.2 N·m/rad·s | |
| Gearbox ratio | 43.165 | |
| Air density | 1.225 kg/m | |
| time delay | 0.1 s | |
| torque coeffs | 0.5176, 116, 0.4, 5, 21, 0.0068 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sendi, C. Control of a Variable Blade Pitch Wind Turbine Subject to Gust Wind and Actuators Saturation. Appl. Sci. 2021, 11, 7865. https://doi.org/10.3390/app11177865
Sendi C. Control of a Variable Blade Pitch Wind Turbine Subject to Gust Wind and Actuators Saturation. Applied Sciences. 2021; 11(17):7865. https://doi.org/10.3390/app11177865
Chicago/Turabian StyleSendi, Chokri. 2021. "Control of a Variable Blade Pitch Wind Turbine Subject to Gust Wind and Actuators Saturation" Applied Sciences 11, no. 17: 7865. https://doi.org/10.3390/app11177865
APA StyleSendi, C. (2021). Control of a Variable Blade Pitch Wind Turbine Subject to Gust Wind and Actuators Saturation. Applied Sciences, 11(17), 7865. https://doi.org/10.3390/app11177865






