Numerical Modeling of Single Pile Behaviors Due to Groundwater Level Rising
Abstract
1. Introduction
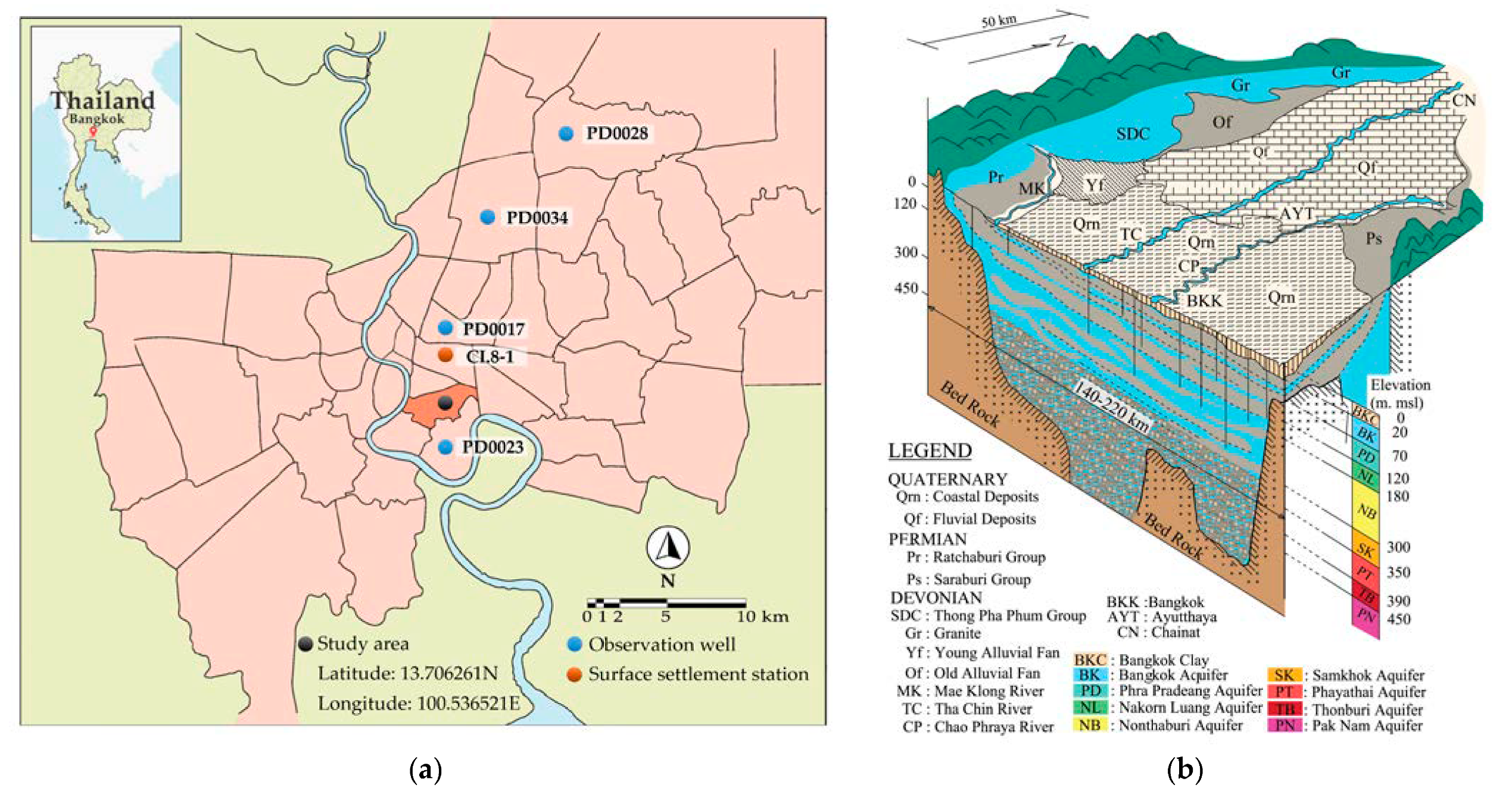
2. Study Area
2.1. Geology and Hydrogeology of Bangkok Area
2.2. Bangkok Subsoil and Pile Foundation
- (1)
- Weathered crust of backfill material, 0 m to 2 m thick. The high soil density layer was usually affected by human activities.
- (2)
- Very soft clay, Soft Bangkok Clay, low strength and highly compressible clay, from 2 m to 14 m. The color varies from medium gray to dark gray. The natural water content of the soil layer varies from 60 to 105%.
- (3)
- Medium clay, 14 m to 20 m thick. The color is dark gray to brownish gray. The natural water content ranges from 31 to 62%.
- (4)
- Stiff to very stiff clay (referred to as Stiff Bangkok Clay), usually 5 m to 10 m, but extending up to the depth of 20 m to 30 m. The water content is relatively low, 15 to 32%.
- (5)
- First sand layer, 8 m to 16 m thick, generally found at 20 m to 30 m. It can be fine to medium sand and occasionally contains traces of clay or silt. The color is yellowish to grayish brown. The water content varies from 12 to 25%.
- (6)
- Very stiff to hard clay layer, found below the medium to dense sandy layer, color varying from light gray to grayish brown, about 10 m to 15 m thick, extending to a depth of 30 m to 40 m. The water content ranges from 15 to 22%. It should be noted that the hard clay layer at some location appears below the second sand layer, a depth greater than 65 m.
- (7)
- Second sand layer (very dense sand layer) is found below the very stiff to hard clay layer, all the way down to the end of the borehole, at 50 to 65 m. The sand is silty, yellowish brown to brownish gray in color. Most of the large bore piles for the high–rise buildings require pile tips to be based in this layer to yield their maximum capacities.
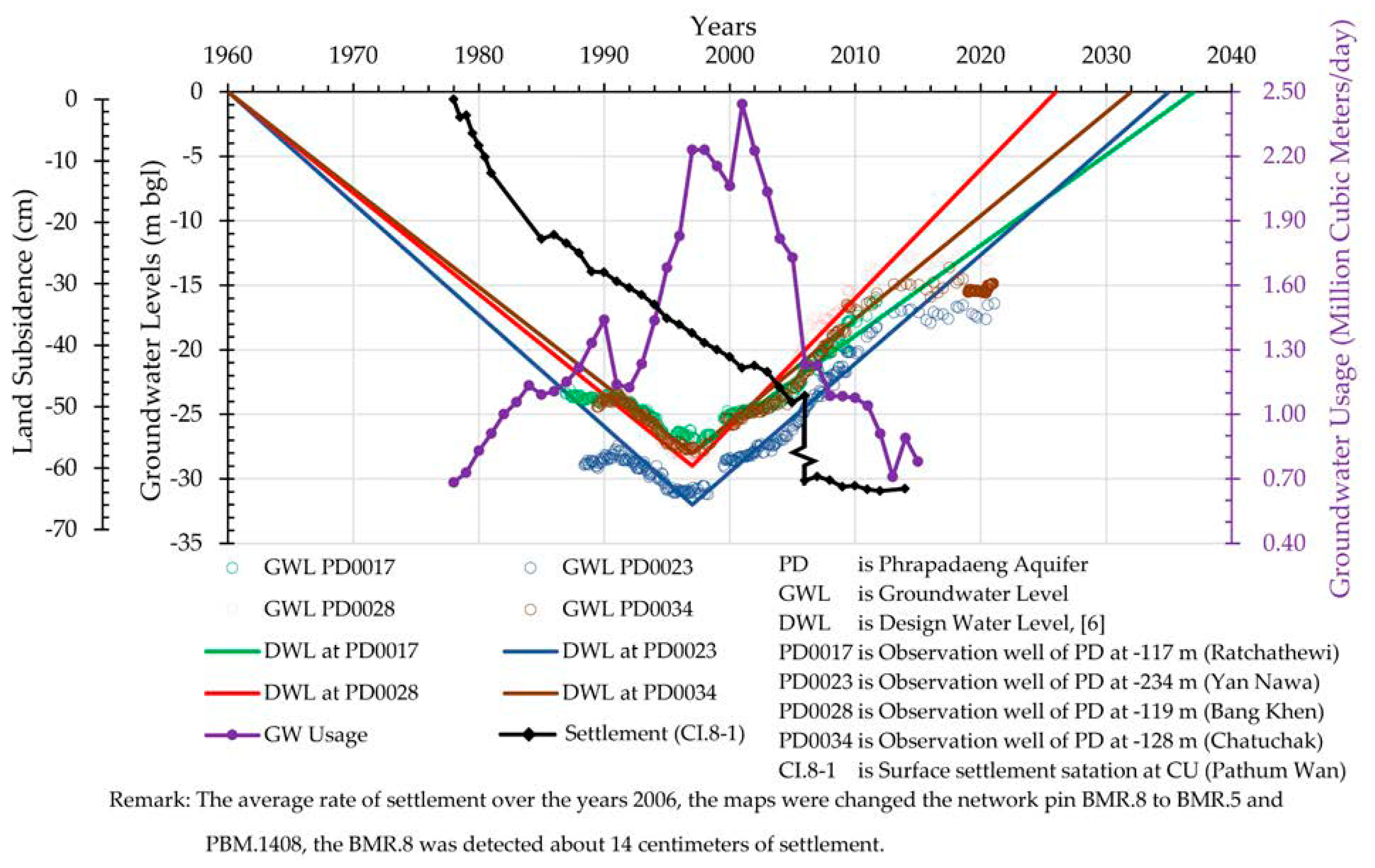
2.3. Groundwater Levels Trend in the Bangkok Aquifer System
2.4. Reference Pile
3. Numerical Modeling
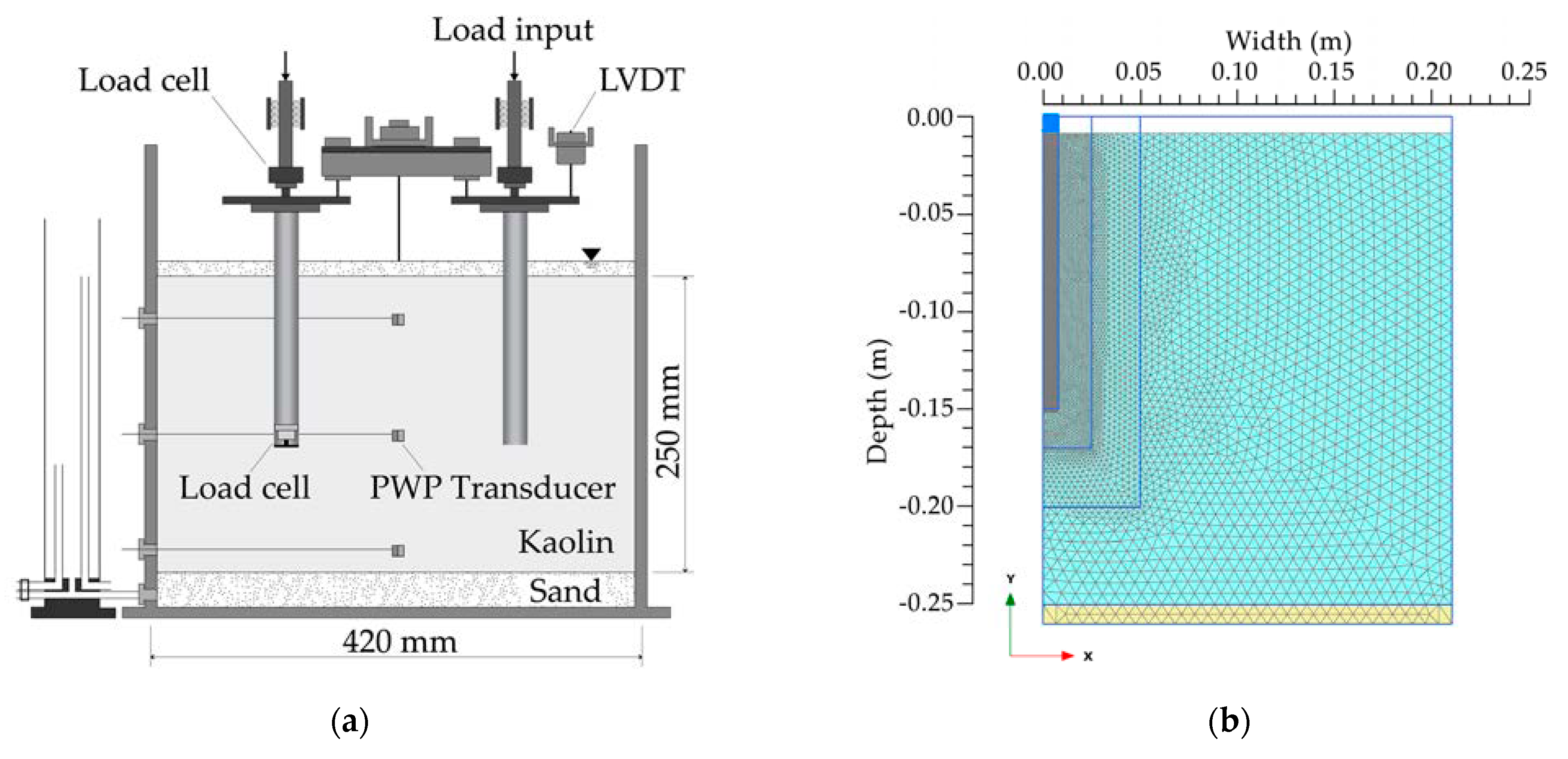
3.1. Geometric Model and Boundary Conditions
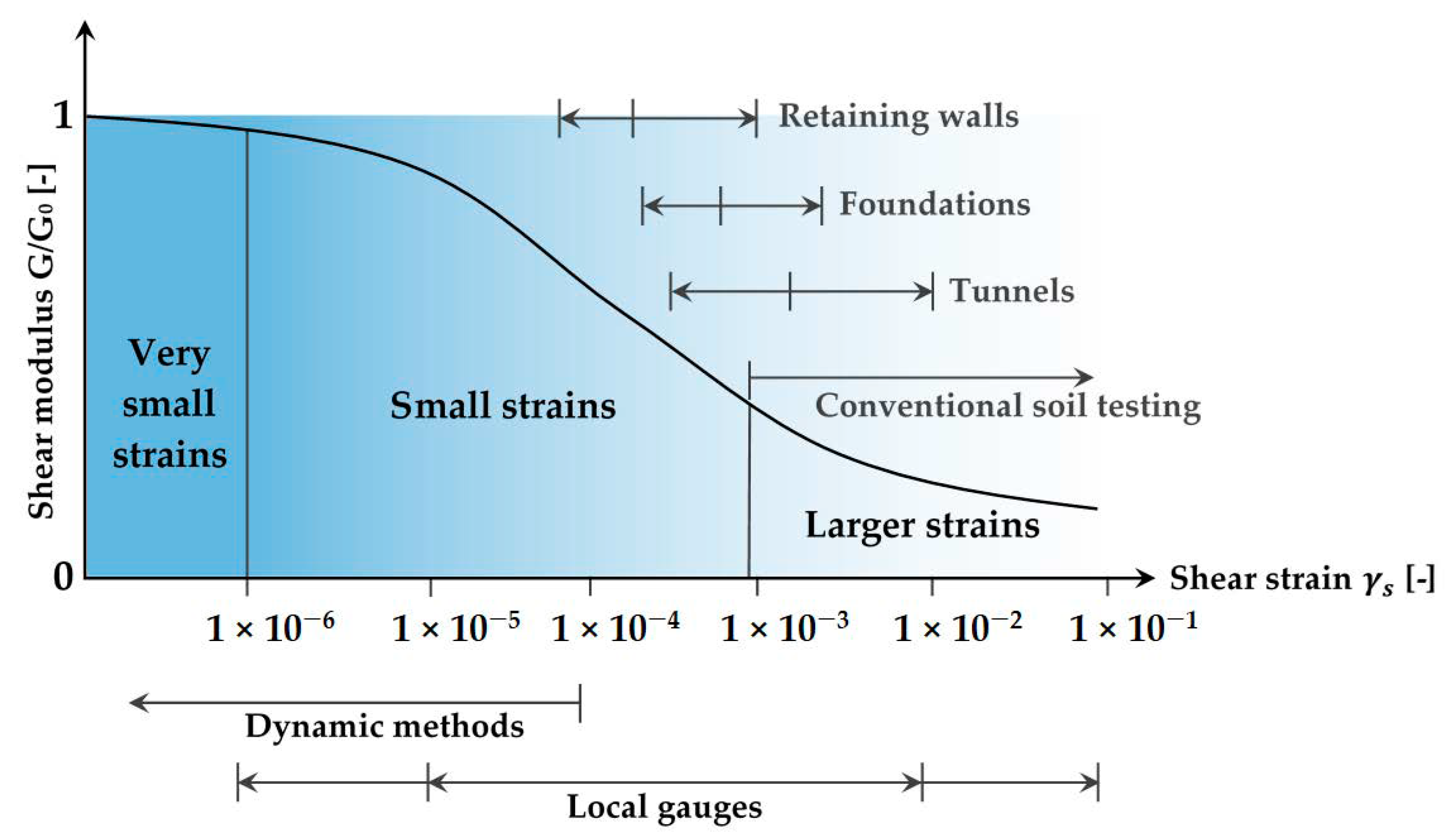
3.2. Constitutive Model and Model Parameters
3.3. Head Function of Groundwater Level Change
3.4. Fully Coupled Flow Deformation Analysis
4. Numerical Analysis
4.1. Verification
4.2. Applied Load on the Pile Head
4.3. Procedure of FE Simulation
- (i.)
- Initial (In 1957, t = 0): This stage was the generation of initial distribution of vertical and horizontal stresses, the soil unit weight, and the coefficient of soil pressure was used to calculate the initial soil stress state. The initial pore water pressure was assigned assuming hydrostatic pressure only.
- (ii.)
- Groundwater levels dropped (t = 0 to 14,600 days): The fully coupled flow and deformation analysis was used for calculation. The groundwater head function was defined from −0.0 to −30.0 m, decreasing at a rate of −0.75 m/year. At the end of this step, the groundwater level was the lowest.
- (iii.)
- Pile installation (In 1997, t = 14,600 days): The piles were installed and loaded axially with the working load. The model assumed that no soil displacement occurred while the piles were installed. The pore water pressure was set as in the previous step. The piles were installed at a minimum groundwater level before rising. One sub-step (iii-substage) was included in this step: the piles were tested when the groundwater level was lowest, for comparing with the load settlement curve after the groundwater stopped rising.
- (iv.)
- Groundwater levels rose (t = 14,600 to 29,200 days): The fully coupled flows and deformations were calculated in this step. The groundwater level rose from −30.0 to −1.0 m at +0.725 m/year. Note that the groundwater level rise was constrained to 1.0 m to prevent groundwater seeping onto the surface. The working load of each pile remained constant. The pile head and soil surface movement, stress distribution along with the pile and the occurrence of plastic points in each time step, was registered.
- (v.)
- End of groundwater rise (In 2037, t = 29,200 days): The groundwater level was highest and close to the hydrostatic pressure. The ultimate load of piles was determined from the load settlement curve, using the pile diameter displacement (10%D), which was defined as the ultimate load [35]. For (v–substage), the pile load settlement curve was compared with the piles at the lowest groundwater level in (iii–substage). Pile load reduction in each pile settlement was computed.
5. Results and Discussion
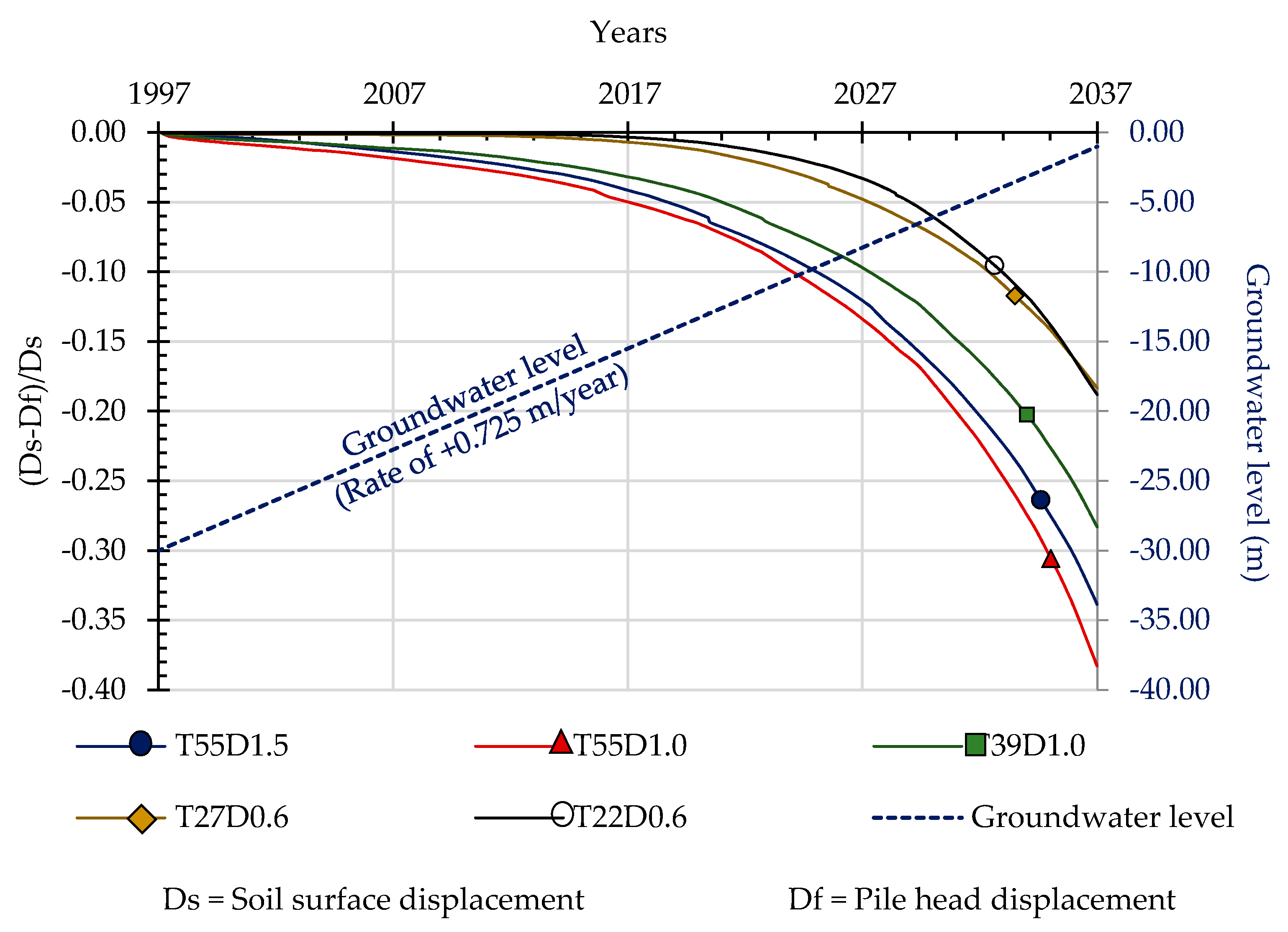
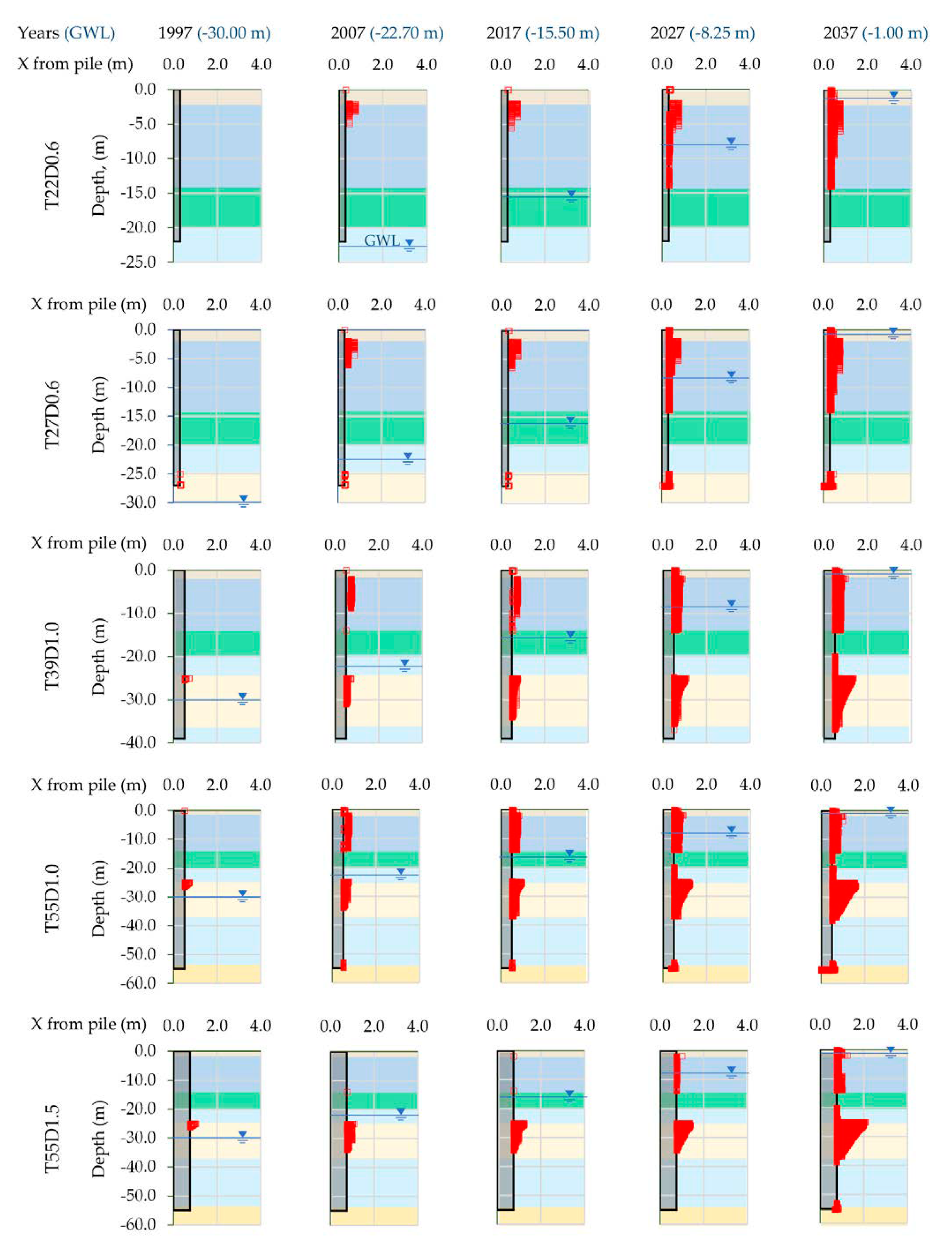
5.1. Pile–Soil Movement
5.2. Stress Distribution along the Pile
5.3. Plastic Points
 red cube) was observed. In the year when the groundwater level was lowest (1997), the plastic points of the soils around the pile appeared in the first sand layer. When the groundwater level rose, plastic points developed. The plastic points expanded downwards in the first sand layer and simultaneously in the soft clay. For the pile through the first sand layer, a larger number of plastics points appeared surrounding the soil layer, because the groundwater level rise had most effect on the sand layer, around the pile, with deceasing effective stress. It is postulated that the permeability of sand layer is much greater than that of clay layer.
red cube) was observed. In the year when the groundwater level was lowest (1997), the plastic points of the soils around the pile appeared in the first sand layer. When the groundwater level rose, plastic points developed. The plastic points expanded downwards in the first sand layer and simultaneously in the soft clay. For the pile through the first sand layer, a larger number of plastics points appeared surrounding the soil layer, because the groundwater level rise had most effect on the sand layer, around the pile, with deceasing effective stress. It is postulated that the permeability of sand layer is much greater than that of clay layer.5.4. Reduction of Pile Capacity
6. Conclusions
- (1)
- Groundwater rises caused both an upward movement of the ground and the pile foundation. The pile settlement relative to the ground surface increased with groundwater level.
- (2)
- A reduction of stress distribution along with the pile with rising groundwater level was caused by soil swelling around the pile. The pile shaft friction was reduced It was accompanied by development of plastic points (failure points).
- (3)
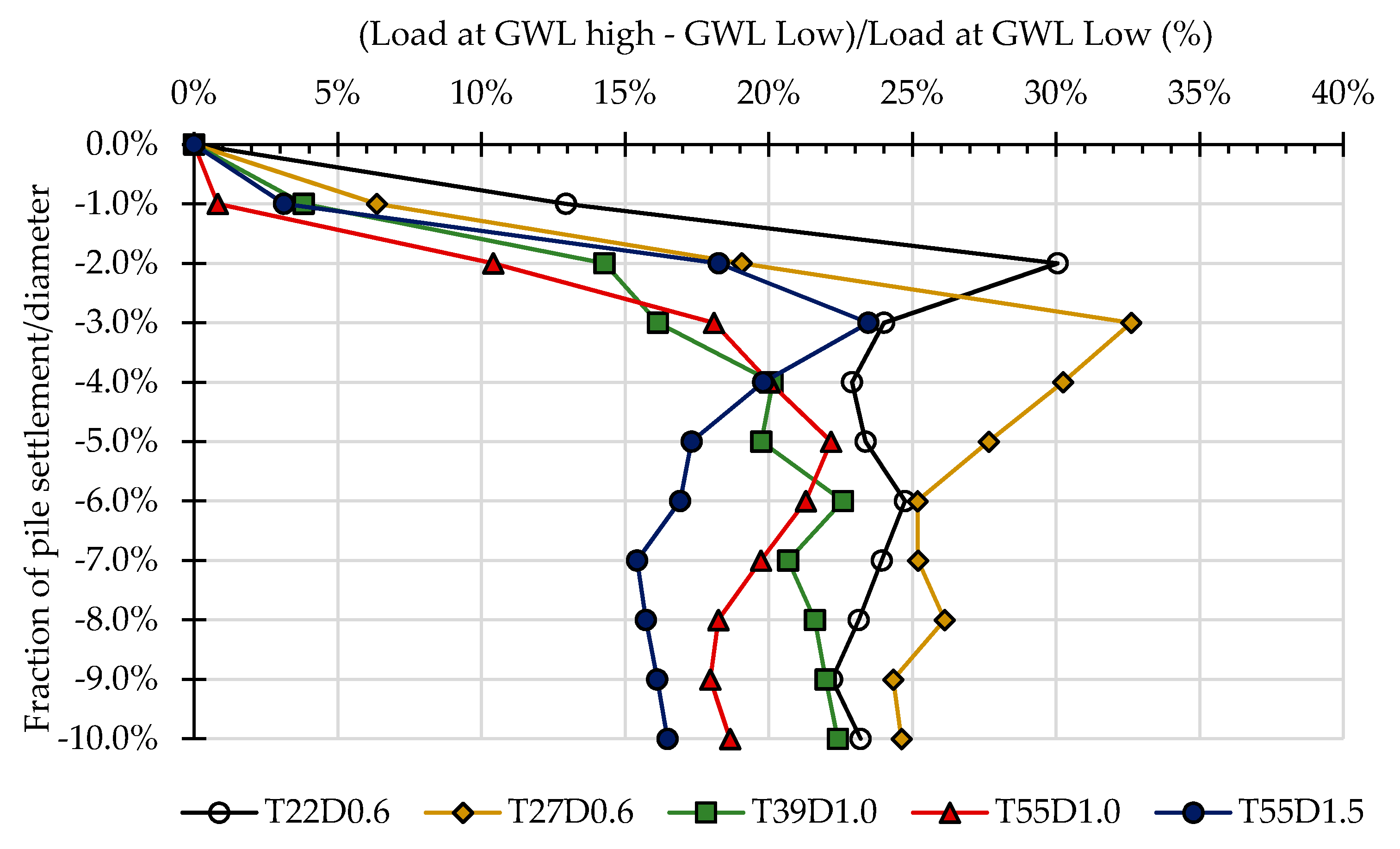
- Groundwater rises caused a loss in pile foundation capacity. For any percentage of pile settlement/diameter in the analysis, the percentages of pile load reduction have not reach to 35%, the pile that was working load with factor of safety less than 2.0, the pile stability should be concerned when the groundwater level is changed. In this study, the evaluation of pile stability due to groundwater level rising for preliminary guidelines to protect existing structures are proposed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cox, D.W. The effects of changing groundwater levels on construction in the City of London. In Groundwater Problems in Urban Areas; Thomas Telford: London, UK, 1994; pp. 263–277. [Google Scholar] [CrossRef]
- Al-Sefry, S.A.; Şen, Z. Groundwater rise problem and risk evaluation in major cities of arid lands—Jedddah case in Kingdom of Saudi Arabia. Water Resour. Manag. 2006, 20, 91–108. [Google Scholar] [CrossRef]
- Allocca, V.; Coda, S.; De Vita, P.; Iorio, A.; Viola, R. Rising groundwater levels and impacts in urban and semirural areas around Naples (Southern Italy). Rend. Online Soc. Geol. Ital. 2016, 41, 14–17. [Google Scholar] [CrossRef]
- Gattinoni, P.; Scesi, L. The groundwater rise in the urban area of Milan (Italy) and its interactions with underground structures and infrastructures. Tunn. Undergr. Space Technol. 2017, 62, 103–114. [Google Scholar] [CrossRef]
- Wang, G.-Y.; Zhu, J.-Q.; Zhang, D.; Wu, J.-Q.; Yu, J.; Gong, X.-L.; Gou, F.-G. Land subsidence and uplift related to groundwater extraction. Q. J. Eng. Geol. Hydrogeol. 2020, 53, 609–619. [Google Scholar] [CrossRef]
- Seven Associated Consultants, Co. Ltd. Study of the Effects on the Substructures due to the Recovery of Piezometric Pressure in the Aquifers in the Bangkok Area and Its Vicinity, Final Report; Department of Groundwater Resources DGR: Bangkok, Thailand, 1999. [Google Scholar]
- Nikos, S.; Ioannis, P.; Constantinos, L.; Paraskevas, T.; Anastasia, K.; Charalambos, K. Land subsidence rebound detected via multi-temporal InSAR and ground truth data in Kalochori and Sindos regions, Northern Greece. Eng. Geol. 2016, 209, 175–186. [Google Scholar] [CrossRef]
- Wang, G.Y.; Zhu, J.Q.; You, G.; Yu, J.; Gong, X.-L.; Li, W.; Gou, F.G. Land rebound after banning deep groundwater extraction in Changzhou, China. Eng. Geol. 2017, 229, 13–20. [Google Scholar] [CrossRef]
- Wilkinson, W. Rising groundwater levels in London and possible effects on engineering structures. In Hydrogeology in the Service of Man, Memoires of the 18th Congress of the International Association of Hydrogeologists; IAH: Cambridge, UK, 1985; pp. 145–157. [Google Scholar]
- Shahriar, M.A.; Sivakugan, N.; Urquhart, A.; Tapiolas, M.; Das, B.M. A Study on the Influence of Ground Water Level on Foundation Settlement in Cohesionless Soils. In Proceedings of the 18th International Conference on Soil Mechanics and Geotechnical Engineering, Paris, France, 2–6 September 2013; pp. 953–956. [Google Scholar]
- Cox, J.W.; Armishaw, D.W. 18. The effects of changes in pore water pressures on the carrying capacities and settlements of driven piles end bearing in a sand and gravel stratum. In Recent Developments in the Design and Construction of Piles; ICE: London, UK, 1979. [Google Scholar]
- Challa, P.K.; Poulos, H.G. Behaviour of single pile in expansive clay. J. Southeast Asian Soc. Soil Eng. 1992, 22, 189–216. [Google Scholar]
- Morrison, P.R.J.; Taylor, R.N. 17. Foundations in a rising groundwater environment. In Groundwater Problems in Urban Areas; Thomas Telford: London, UK, 1994; pp. 342–354. [Google Scholar] [CrossRef]
- Morrison, P.R.J.; Taylor, R.N. Modelling of foundations in a rising groundwater environment. In Proceedings of the International Conference on Soil Mechanics and Foundation Engineering, New Delhi, India, 5–10 January 1994; pp. 655–658. [Google Scholar]
- Nutalaya, P.; Rau, J.L. Bangkok: The sinking metropolis. Episodes 1981, 1981, 3–8. [Google Scholar] [CrossRef][Green Version]
- Phien-Wej, N.; Giao, P.H.; Nutalaya, P. Land subsidence in Bangkok, Thailand. Eng. Geol. 2006, 82, 187–201. [Google Scholar] [CrossRef]
- Intachai, K. The Thesis of Analytical Assessment on Pile Capacity in View of Effects of Groundwater Drawdown and Rebound Associated with Deep Well Pumping in Bangkok. Master’s Thesis, Asian Institute of Technology, Pathum Thani, Thailand, May 2016. [Google Scholar]
- Intui, S.; Soralump, S. The Vertical Bearing Capacity Behavior of Single Pile by Geotechnical Centrifuge. Kasetsart Eng. J. 2018, 31, 55–68. [Google Scholar]
- Roh, Y.; Kim, I.; Kim, G.; Lee, J. Comparative Analysis of Axial Load Capacity for Piled-Raft Foundation with Changes in Groundwater Level. KSCE J. Civ. Eng. 2019, 23, 1–9. [Google Scholar] [CrossRef]
- Dassault; Systemes Simulia Corporation Province ABAQUS/CAE User’s Manual 6.13. Available online: http://130.149.89.49:2080/v6.13/books/usi/default.htm (accessed on 11 September 2020).
- Saowiang, K.; Huy, P. Numerical analysis of subsurface deformation induced by groundwater level changes in the Bangkok aquifer system. Acta Geotech. 2020, 8, 1265–1279. [Google Scholar] [CrossRef]
- Brinkgreve, R.; Vermeer, P.A. PLAXIS 2D Reference Manual CONNECT EDITION V20; Delft University: Delft, The Netherlands, 2019. [Google Scholar]
- Galavi, V. Internal Report Groundwater flow, fully coupled flow deformation and undrained analyses in PLAXIS 2D and 3D Vahid Galavi Research department. Plaxis Rep. 2010. [Google Scholar] [CrossRef]
- Japan International Cooperation Agency (JICA). The Study on Management of Groundwater and Land Subsidence in the Bangkok Metropolitan Area and Its Vicinity; JICA: Tokyo, Japan, 1995; pp. 1-1–11-5. [Google Scholar]
- Tanachaichoksirikun, P.; Seeboonruang, U.; Fogg, G.E. Improving Groundwater Model in Regional Sedimentary Basin Using Hydraulic Gradients. KSCE J. Civ. Eng. 2020, 24, 1655–1669. [Google Scholar] [CrossRef]
- Nutalaya, P.; Rau, J.L. Structural framework of the Chao Phraya basin, Thailand. In Proceedings of the Symposium on Cenozoic Basins Thailand: Geology and Resources, Chiang Mai, Thailand, 24 October 1984; pp. 106–129. [Google Scholar]
- Prust, R.; Davies, J.; Hu, S. Part 6: Tunnels and Underground Structures: Pressuremeter Investigation for Mass Rapid Transit in Bangkok, Thailand. Transp. Res. Rec. J. Transp. Res. Board 2005, 1928, 205–217. [Google Scholar] [CrossRef]
- Atkinson, J.H.; Sallfors, G. Experimental determination of soil properties. Proc. ECSMFE 1991, 3, 915–956. [Google Scholar]
- Brinkgreve, R.B.J.; Kappert, M.H.; Bonnier, P.B. Hysteretic damping in a small-strain stiffness model. In Numerical Models in Geomechanics—NUMOG X; Taylor & Francis Group: London, UK, 2007; pp. 737–742. [Google Scholar] [CrossRef]
- Rukdeechuai, T.; Jongpradist, P.; Wonglert, A.; Kaewsri, T. Influence of Soil Models on Numerical Simulation of Geotechnical works in Bangkok subsoil. Res. Dev. J. Vol. 2009, 20, 17–28. [Google Scholar]
- Likitlersuang, S.; Teachavorasinskun, S.; Surarak, C.; Oh, E.; Balasubramaniam, A. Small strain stiffness and stiffness degradation curve of Bangkok Clays. Soils Found. 2013, 53, 498–509. [Google Scholar] [CrossRef]
- Kamchoom, V.; Leung, A.K. A simplified modelling method of seasonal tree root-water uptake effects on pore-water pressure and ground subsidence. In Proceedings of the 7th International Conference on Unsaturated Soils, HKUST, Hong Kong, China, 3–5 August 2018. [Google Scholar]
- Biot, M.A. General theory of three dimensional consolidation. J. Appl. Phys. 1941, 12, 155–164. [Google Scholar] [CrossRef]
- Bishop, A.W.; Blight, G.E. Some aspects of effective stress in saturated and partly saturated soils. Geotechnique 1963, 13, 177–197. [Google Scholar] [CrossRef]
- Whitaker, T.; Cooke, R.W. An investigation of the shaft and base resistance of large bored piles in London Clay. In Large Bored Piles; Institution of Civil Engineers: London, UK, 1966; pp. 7–49. [Google Scholar]
- Al-Tabbaa, A.; Arup, O. Heave induced pile tension: A simple one-dimensional analysis. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1990, 27, 366. [Google Scholar] [CrossRef]
- Hirany, A.; Kulhawy, F.H. Conduct and Interpretation of Load Tests on Drilled Shaft Foundations; Detailed Guidelines: Final Report; Electric Power Research Institute: Palo Alto, CA, USA, 1988; Volume 1, p. 376. [Google Scholar]
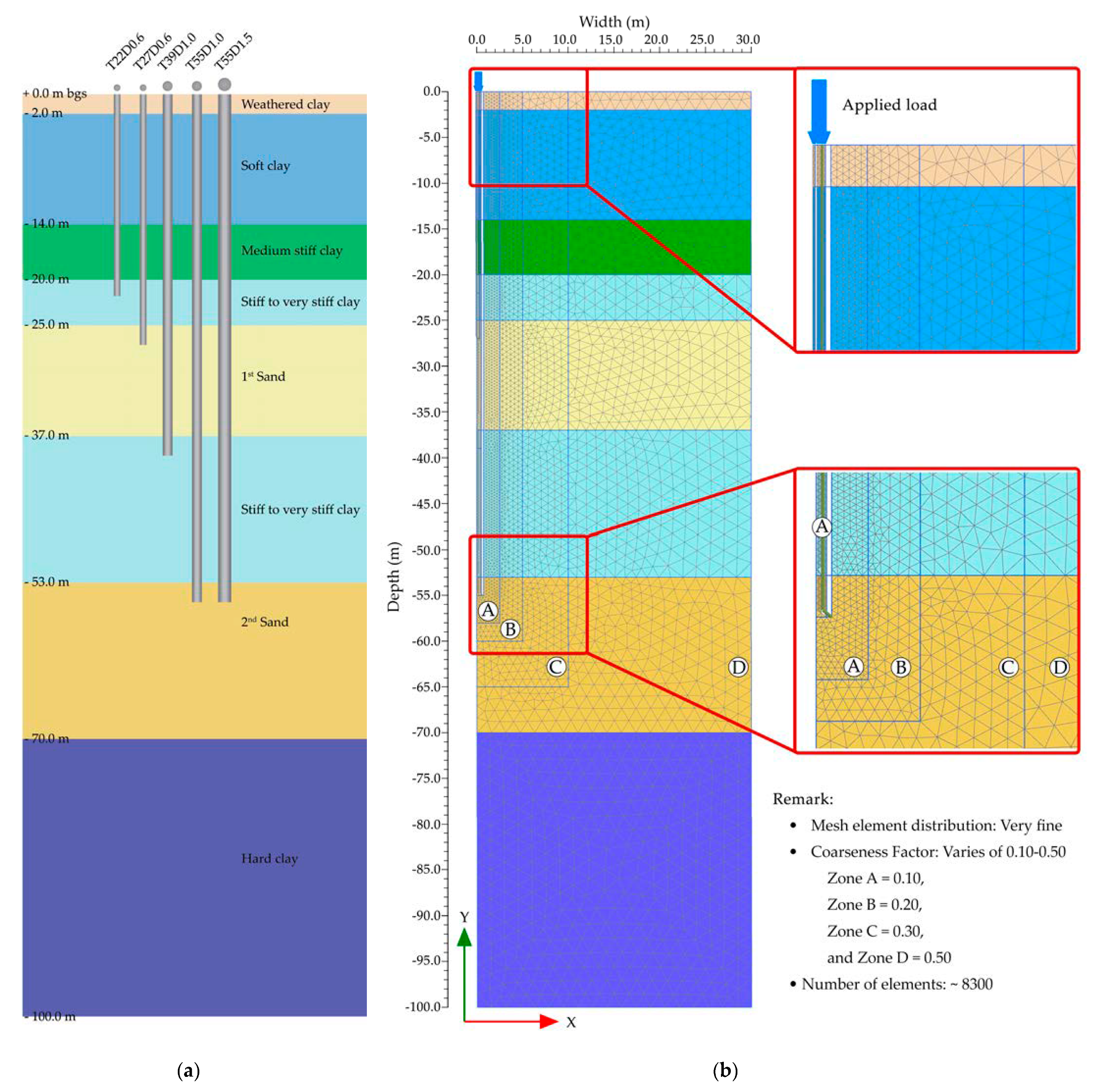

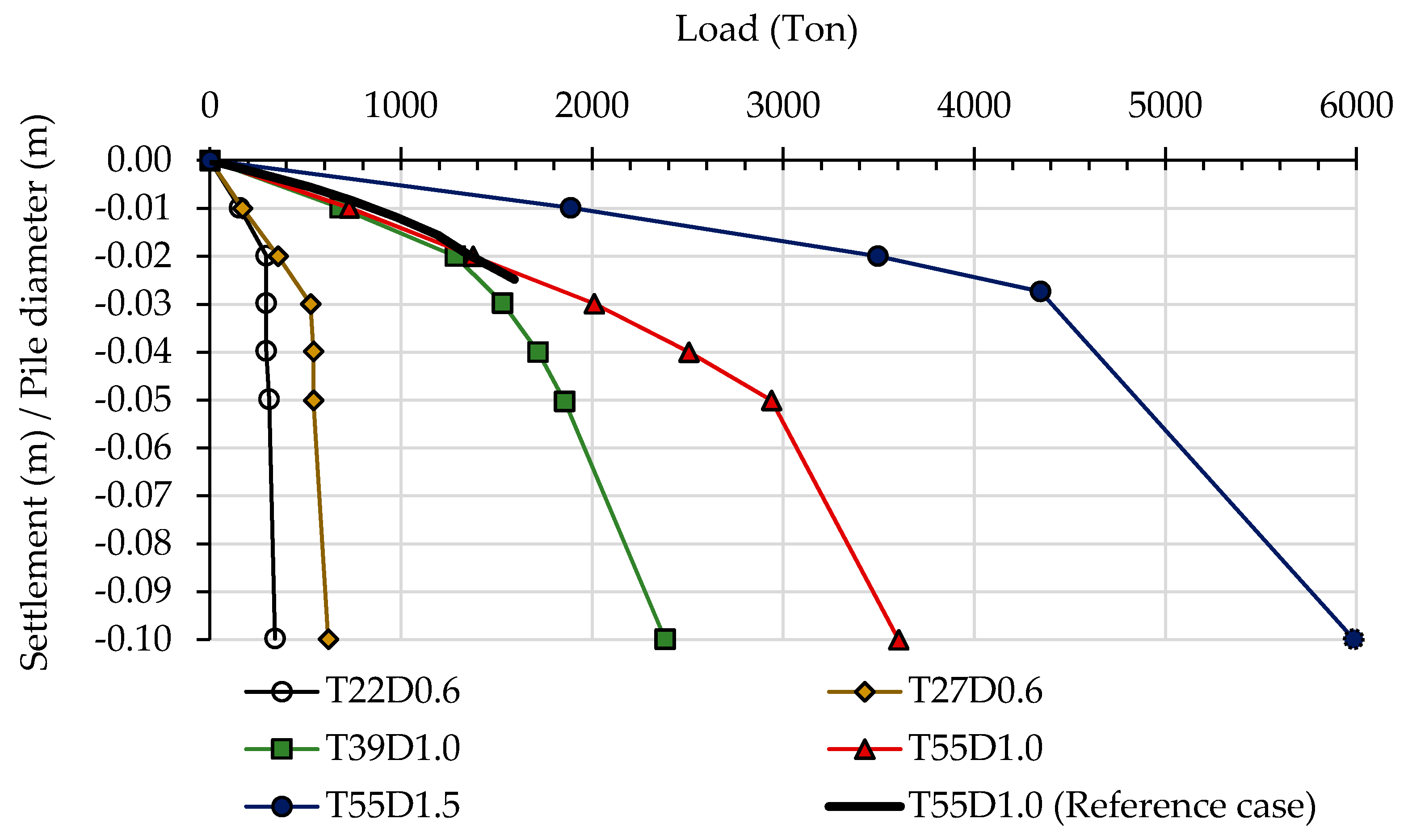




| Piles | Type of Pile | Length (m) | Diameter (m) | Soil at Pile Base | |
|---|---|---|---|---|---|
| T22D0.6 | Bored | 22.0 | 0.6 | Stiff clay | |
| T27D0.6 | Bored | 27.0 | 0.6 | 1st Sand | |
| T39D1.0 | Bored | 39.0 | 1.0 | Stiff clay | |
| T55D1.0 | Bored | 55.0 | 1.0 | 2nd Sand | |
| T55D1.5 | Bored | 55.0 | 1.5 | 2nd Sand | |
| Parameters | Symbol | Unit | Speswhite Kaolin Clay | Pile |
|---|---|---|---|---|
| Material property | ||||
| Material model | - | - | HSsmall | LE |
| Drainage type | - | - | Drained | NP |
| Unit weight below phreatic level | kN/m3 | 17 | - | |
| Unit weight above phreatic level | kN/m3 | 10.5 | 24 | |
| Secant stiffness in standard drained triaxial test | E50ref | kN/m2 | 1500 | E = 2.7 × 107 |
| Tangent stiffness for primary oedometer loading | Eoedref | kN/m2 | 750 | - |
| Unloading/reloading stiffness | Eurref | kN/m2 | 8000 | - |
| Power for stress level dependency of stiffness | power (m) | - | 1.00 | - |
| Cohesion | C’ref | kN/m2 | 0.00 | - |
| Friction angle | degree | 21.00 | - | |
| Dilatancy angle | degree | 0 | - | |
| Shear strain at which Gs = 0.722G0 | - | 0.0002 | - | |
| Shear modulus at very small strain | G0ref | kN/m2 | 33300 | - |
| Poisson’s ratio | v’ur | - | 0.20 | v(nu) = 0.15 |
| Reference pressure | Pref | kN/m2 | 100 | - |
| Coefficient of lateral earth pressure for a normally | K0nc | - | 0.64 | - |
| Failure ratio | Rf | - | 0.9 | - |
| Interface reduction factor | Rinter | - | 0.75 | 1 |
| Over consolidation ratio | OCR | - | 1.00 | - |
| References | - | - | after Benz [29] | |
| Groundwater property | ||||
| Data set | - | User-defined | Non porous | |
| Model | - | Van Genuchten | - | |
| Residual saturation | Sres | - | 0.0500 | - |
| Saturated saturation | Ssat | - | 1.000 | - |
| Fitting parameters (Van Genuchten) | gn | - | 1.600 | - |
| Fitting parameters (Van Genuchten) | ga | 1/m | 0.0400 | - |
| Fitting parameters (Van Genuchten) | gl | - | 0.5000 | - |
| Horizontal Permeability | kx | m/day | 8.64 × 10−3 | - |
| Vertical Permeability | ky | m/day | 8.640 × 10−3 | - |
| References | Gallipoli et al. [32] |
| Parameters | Unit | Weathered Clay | Soft Clay | Medium Stiff Clay | Stiff to Very Stiff Clay | 1st Sand | 2nd sand | Hard Clay | Pile |
|---|---|---|---|---|---|---|---|---|---|
| Material property | |||||||||
| Model | - | MC | HSsmall | HSsmall | HSsmall | MC | MC | HSsmall | LE |
| Material type | - | Undrained | Drained | Drained | Drained | Drained | Drained | Drained | NP |
| kN/m3 | 17 | 16.5 | 18 | 20 | 20 | 20 | 20 | - | |
| kN/m3 | 16 | 15 | 17 | 18 | 19 | 19 | 18 | 24 | |
| E50ref | kN/m2 | E′ = 6000 | 800 | 1650 | 8500 | E′ = 85,800 | E′ = 85,800 | 30,000 | E = 2.70 × 107 |
| Eoedref | kN/m2 | - | 850 | 1650 | 9000 | - | - | 30,000 | - |
| Eurref | kN/m2 | - | 8000 | 5400 | 30,000 | - | - | 120,000 | - |
| power (m) | - | - | 1.00 | 1.00 | 1.00 | - | - | 1.00 | - |
| C’ref | kN/m2 | Su = 40 | 1.00 | 10.00 | 25.00 | 0.1 | 0.1 | 40.00 | - |
| degree | 0 | 23 | 25 | 26 | 36 | 37 | 24 | - | |
| degree | 0 | 0 | 0 | 0 | 5 | 5 | 0 | - | |
| - | - | 0.05000 | 0.00100 | 0.00100 | - | - | 0.00150 | - | |
| G0ref | kN/m2 | - | 10,000 | 16,000 | 50,000 | - | - | 124,000 | - |
| v’ur | - | 0.30 | 0.20 | 0.20 | 0.20 | 0.30 | 0.30 | 0.20 | v(nu) = 0.15 |
| Pref | kN/m2 | - | 100 | 100 | 100 | - | - | 100 | - |
| K0nc | - | - | 0.74 | 0.65 | 0.50 | 0.5 | 0.5 | 0.50 | - |
| Rf | - | - | 0.90 | 0.90 | 0.90 | - | - | 0.90 | - |
| Rinter | - | - | 0.75 | 0.75 | 0.75 | 0.75 | 0.75 | 0.75 | 1.00 |
| OCR | - | - | 1.2 | 2.0 | 2.0 | - | - | 1.1 | - |
| Groundwater property | |||||||||
| Data set | - | Standard | Standard | Standard | Standard | Standard | Standard | Standard | - |
| Soil type | - | Coarse | Very fine | Fine | Fine | Coarse | Fine | Fine | - |
| kx | m/day | 8.64 × 10−7 | 8.64 × 10−5 | 8.64 × 10−4 | 8.64 × 10−4 | 1.60 | 0.80 | 8.64 × 10−5 | - |
| ky | m/day | 8.64 × 10−7 | 8.64 × 10−5 | 8.64 × 10−4 | 8.64 × 10−4 | 1.60 | 0.80 | 8.64 × 10−5 | - |
| Piles | Stress 10%D 1 (kN/m2) | Load 10%D 1 (Ton) | Applied Stress 2 (kN/m2) | Applied Load 2 (Ton) |
|---|---|---|---|---|
| T22D0.6 | 12,000 | 340 | 4800 | 136 |
| T27D0.6 | 22,000 | 625 | 8800 | 250 |
| T39D1.0 | 30,000 | 2365 | 12,000 | 945 |
| T55D1.0 | 45,700 | 3600 | 18,280 | 1440 |
| T55D1.5 | 33,850 | 6000 | 13,540 | 2400 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Phoban, H.; Seeboonruang, U.; Lueprasert, P. Numerical Modeling of Single Pile Behaviors Due to Groundwater Level Rising. Appl. Sci. 2021, 11, 5782. https://doi.org/10.3390/app11135782
Phoban H, Seeboonruang U, Lueprasert P. Numerical Modeling of Single Pile Behaviors Due to Groundwater Level Rising. Applied Sciences. 2021; 11(13):5782. https://doi.org/10.3390/app11135782
Chicago/Turabian StylePhoban, Harutus, Uma Seeboonruang, and Prateep Lueprasert. 2021. "Numerical Modeling of Single Pile Behaviors Due to Groundwater Level Rising" Applied Sciences 11, no. 13: 5782. https://doi.org/10.3390/app11135782
APA StylePhoban, H., Seeboonruang, U., & Lueprasert, P. (2021). Numerical Modeling of Single Pile Behaviors Due to Groundwater Level Rising. Applied Sciences, 11(13), 5782. https://doi.org/10.3390/app11135782






