DC 4-Point Measurement for Total Electrical Conductivity of SOFC Cathode Material
Abstract
:1. Introduction
2. Materials and Methods
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mahato, N.; Banerjee, A.; Gupta, A.; Omar, S.; Balani, K. Progress in material selection for solid oxide fuel cell technology: A review. Prog. Mater. Sci. 2015, 72, 141–337. [Google Scholar] [CrossRef]
- Zhou, J.; Chen, G.; Wu, K.; Cheng, Y. La0.8Sr1.2CoO4+δ–CGO composite as cathode on La0.9Sr0.1Ga0.8Mg0.2O3−δ electrolyte for intermediate temperature solid oxide fuel cells. J. Power Sources 2013, 232, 332–337. [Google Scholar] [CrossRef]
- Brandon, N.P.; Skinner, S.; Steele, B.C. Recent Advances in Materials for Fuel Cells. Ann. Rev. Mater. Res. 2003, 33, 183–213. [Google Scholar] [CrossRef]
- Hauch, A.; Jensen, S.H.; Ramousse, S.; Mogensen, M. Performance and Durability of Solid Oxide Electrolysis Cells. J. Electrochem. Soc. 2006, 153, A1741. [Google Scholar] [CrossRef] [Green Version]
- Zou, X.; Li, X.; Shen, B.; Lu, X.; Xu, Q.; Zhou, Z.; Ding, W. CeO2-Y2O3-ZrO2 Membrane with Enhanced Molten Salt Corrosion Resistance for Solid Oxide Membrane (SOM) Electrolysis Process. Metall. Mater. Trans. 2017, 48, 678–691. [Google Scholar] [CrossRef]
- Maguire, E.; Gharbage, B.; Marques, F.M.B.; Labrincha, J.A. Cathode materials for intermediate temperature SOFCs. Solid State Ion. 2000, 127, 329–335. [Google Scholar] [CrossRef]
- Mizutani, Y.; Tamura, M.; Kawai, M.; Yamamoto, O. Development of high-performance electrolyte in SOFC. Solid State Ion. 1994, 72, 271–275. [Google Scholar] [CrossRef]
- Sun, C.; Hui, R.; Roller, J. Cathode materials for solid oxide fuel cells: A review. J Solid State Electrochem. 2010, 14, 1125–1144. [Google Scholar] [CrossRef]
- Zakaria, Z.; Mat, Z.A.; Hassan, S.H.A.; Kar, Y.B. A review of solid oxide fuel cell component fabrication methods toward lowering temperature. Int. J. Energy Res. 2020, 44, 594–611. [Google Scholar] [CrossRef]
- Chen, D.; Huang, C.; Ran, R.; Park, H.J.; Kwak, C.; Shao, Z. New Ba0.5Sr0.5Co0.8Fe0.2O3−δ + Co3O4 composite electrode for IT-SOFCs with improved electrical conductivity and catalytic activity. Electrochem. Commun. 2011, 13, 197–199. [Google Scholar] [CrossRef]
- Hussain, S.; Yangping, L. Review of solid oxide fuel cell materials: Cathode, anode, and electrolyte. Energy Transit. 2020, 4, 113–126. [Google Scholar] [CrossRef]
- Two-Wire Versus Four-Wire Resistance Measurements: Which Configuration Makes Sense for Your Application. Available online: http://www.tek.com/sites/tek.com/files/media/document/resources/2Wire_4WireResistanceArticle.pdf (accessed on 27 May 2021).
- Garcia-Garcia, F.J.; Tang, Y.; Gotor, F.J.; Sayagués, M.J. Development by Mechanochemistry of La0.8Sr0.2Ga0.8Mg0.2O2.8 Electrolyte for SOFCs. Materials 2020, 13, 1366. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Garcia-Garcia, F.J.; Sayagués, M.J.; Gotor, F.J. A Novel, Simple and Highly Efficient Route to Obtain PrBaMn2O5+δ Double Perovskite: Mechanochemical Synthesis. Nanomaterials 2021, 11, 380. [Google Scholar] [CrossRef] [PubMed]
- International Organization for Standardization (ISO). Fine Ceramics (Advanced Ceramics, Advanced Technical Ceramics)—Test Method for Total Electrical Conductivity of Conductive Fine Ceramics; ISO/FDIS 23331; ISO: Geneva, Switzerland.
- Mizusaki, J.; Yonemura, Y.; Kamata, H.; Ohyama, K.; Mori, N.; Takai, H.; Tagawa, H.; Dokiya, M.; Naraya, K.; Sasamoto, T.; et al. Electronic conductivity, Seebeck coefficient, defect and electronic structure of nonstoichiometric La1−xSrxMnO3. Solid State Ion. 2000, 132, 167–180. [Google Scholar] [CrossRef]
- Paydar, S.; Shariat, M.H.; Javadpour, S. Investigation on electrical conductivity of LSM/YSZ8, LSM/Ce0.84Y0.16O0.96 and LSM/Ce0.42Zr0.42Y0.16O0.96 composite cathodes of SOFCs. Int. J. Hydrog. Energy. 2016, 41, 23145–23155. [Google Scholar] [CrossRef]
- Ahmed, B.; Lee, S.B.; Song, R.H.; Lee, J.W.; Lim, T.H.; Park, S.J. A Study on Sintering Inhibition of La0.8Sr0.2MnO3−δ Cathode Material for Cathode-Supported Fuel Cells. J. Korean Ceram. Soc. 2016, 53, 494–499. [Google Scholar]
- Bai, Y.; Liu, M.; Ding, D.; Blinn, K.; Qin, W.; Liu, J.; Liu, M. Electrical and electrocatalytic properties of a La0.8Sr0.2Co0.17Mn0.83O3−δ cathode for intermediate-temperature solid oxide fuel cells. J. Power Sources 2012, 205, 80–85. [Google Scholar] [CrossRef]
- Noh, T.; Kim, J.; Kim, Y.; Chun, H.H.; Jeon, M.-S.; Lee, H. Mn valence state and electrode performance of perovskite-type cathode La0·8Sr0·2Mn1−xCuxO3−δ (x = 0, 0·2) for intermediate-temperature solid oxide fuel cells. Bull. Mater. Sci. 2013, 36, 1261–1266. [Google Scholar] [CrossRef] [Green Version]


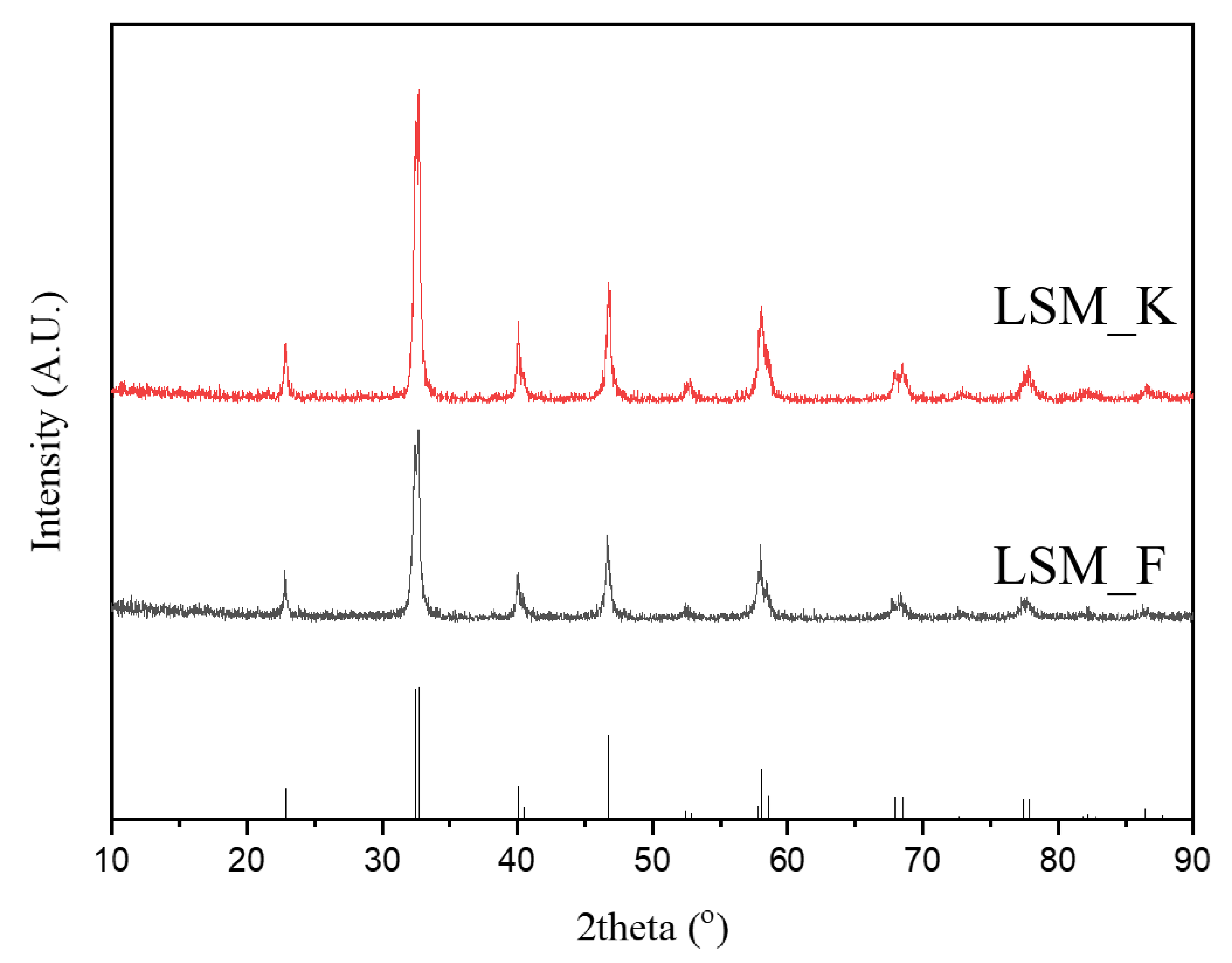
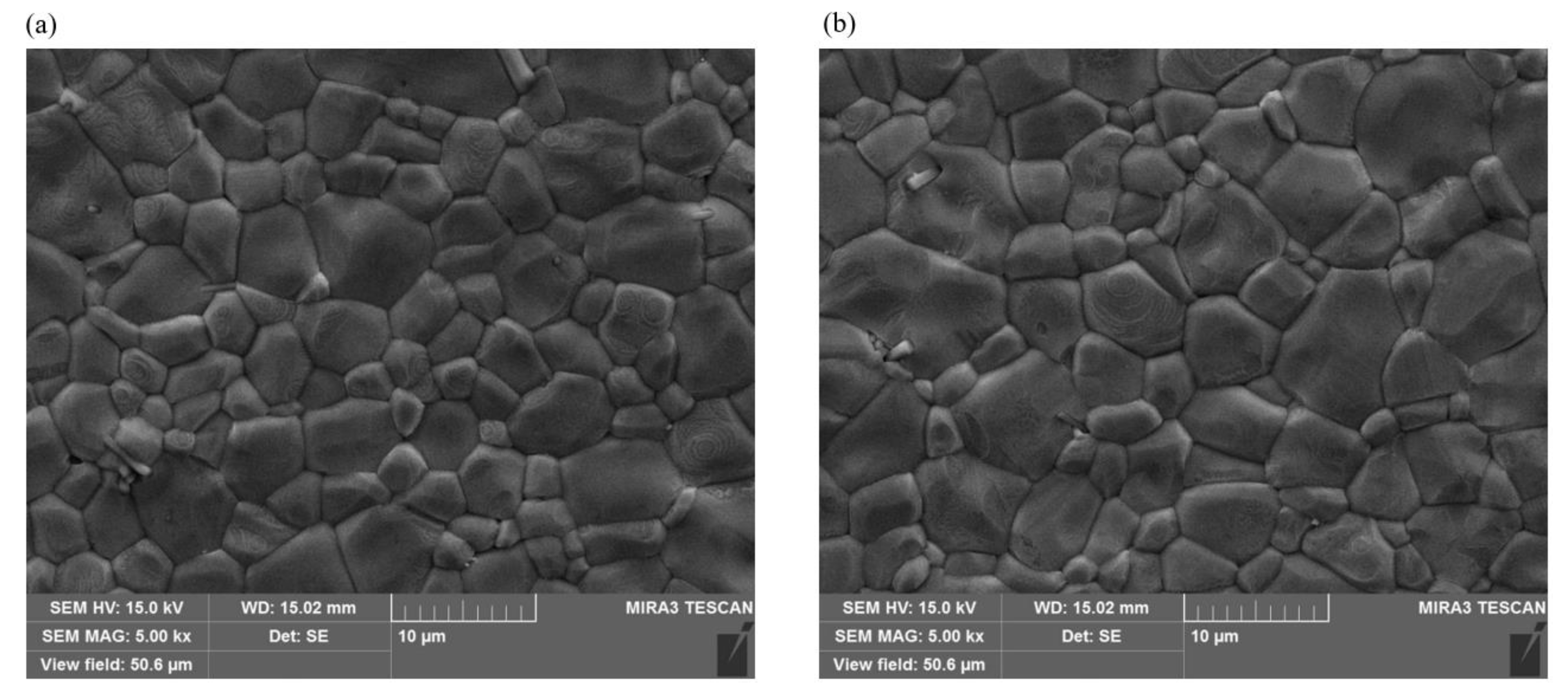
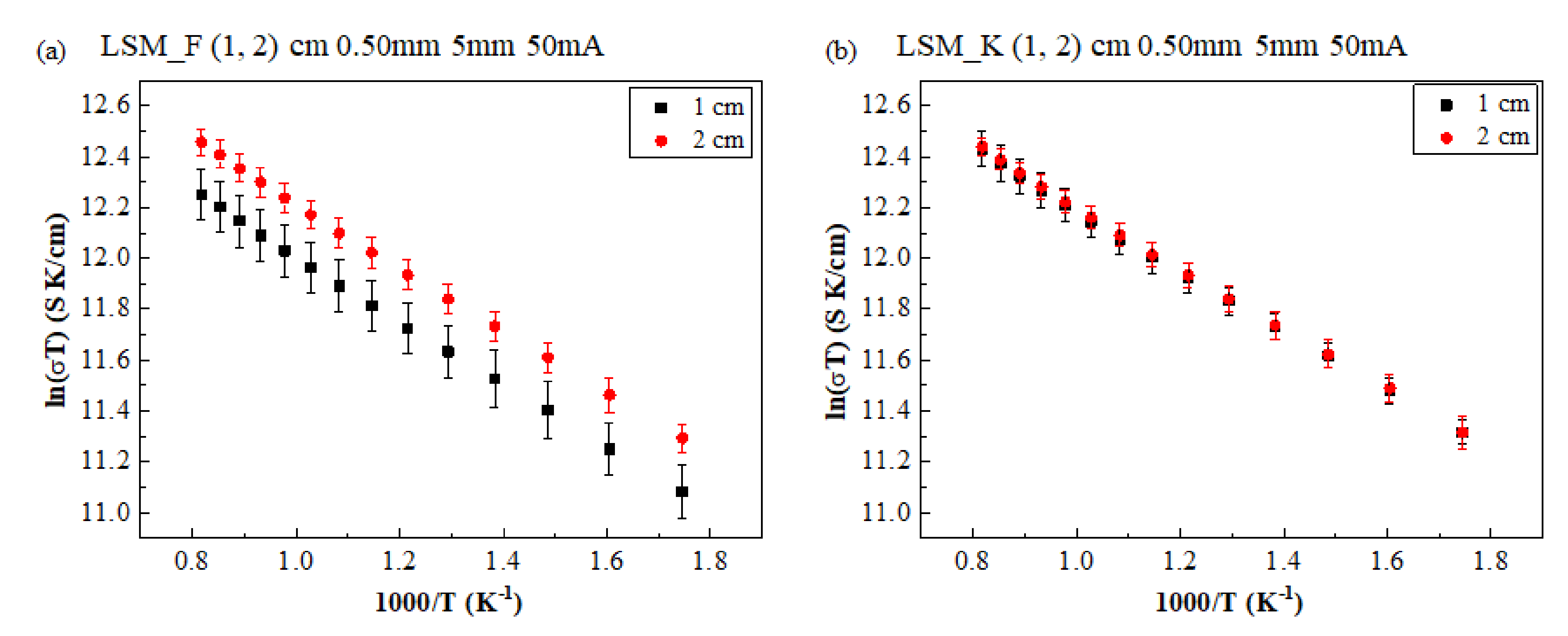
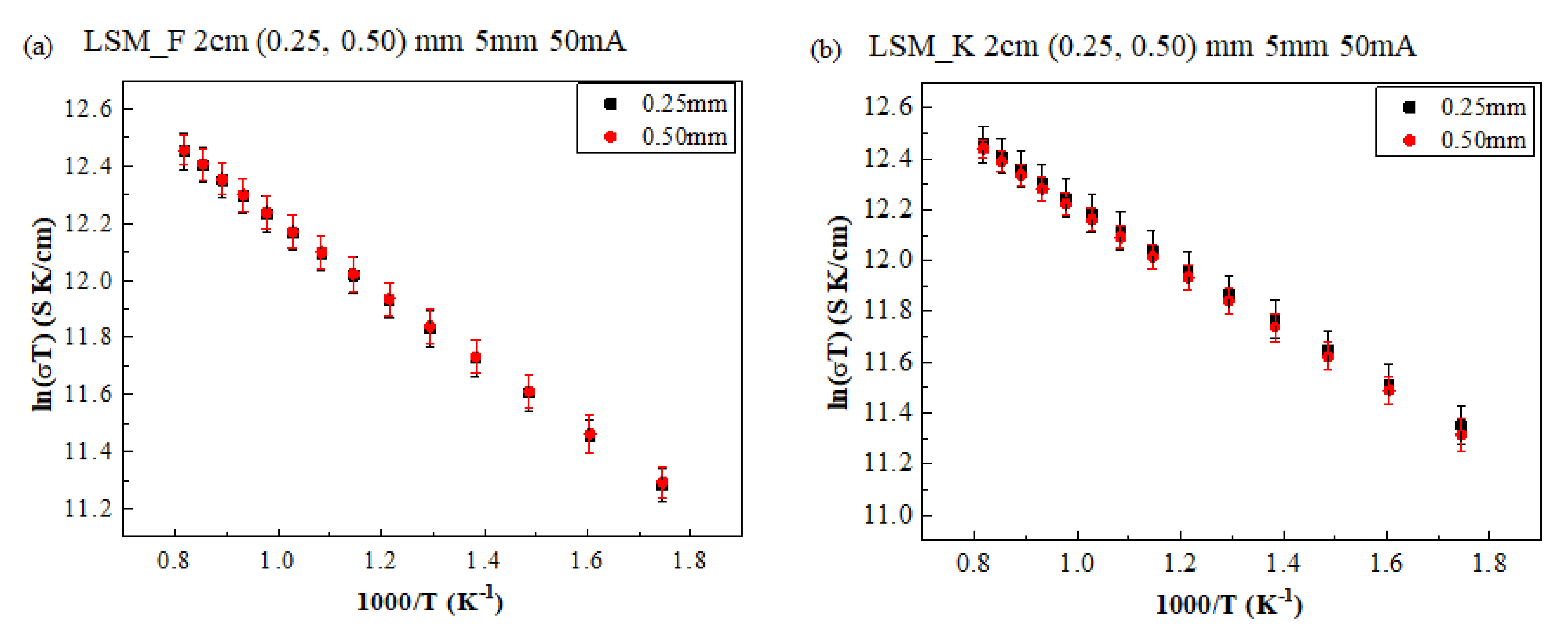


| Variables | Dimension |
|---|---|
| Width (w) | 3 mm |
| Sample thickness (t) | 3, 4, 5 mm |
| Spacing between the measurement terminals (L) | 10, 20 mm |
| Diameter of lead wire (ϕ) | 0.25, 0.50 mm |
| Applied current (I) | 10, 50, 100 mA |
| Material | Electrical Conductivity at 900 °C (S/cm) | Fabrication Method | Sintering Condition (Temperature, Hour) | Reference |
|---|---|---|---|---|
| LSM82 | 209 | Acetate aqueous solution | 1400 °C | [16] |
| LSM73 | 235 | Commercial | 1450 °C, 5 h | [17] |
| LSM82 | 270 | Commercial | 1300 °C, 5 h | [18] |
| LSM82 | 229 (800 °C, Van der Pauw) | Sol-gel method | 1050 °C, 2 h (Brush coating) | [19] |
| LSM82 | 190 | ECCP | 1250 °C, 4 h | [20] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jo, K.; Ha, J.; Ryu, J.; Lee, E.; Lee, H. DC 4-Point Measurement for Total Electrical Conductivity of SOFC Cathode Material. Appl. Sci. 2021, 11, 4963. https://doi.org/10.3390/app11114963
Jo K, Ha J, Ryu J, Lee E, Lee H. DC 4-Point Measurement for Total Electrical Conductivity of SOFC Cathode Material. Applied Sciences. 2021; 11(11):4963. https://doi.org/10.3390/app11114963
Chicago/Turabian StyleJo, Kanghee, Jooyeon Ha, Jiseung Ryu, Eunkyung Lee, and Heesoo Lee. 2021. "DC 4-Point Measurement for Total Electrical Conductivity of SOFC Cathode Material" Applied Sciences 11, no. 11: 4963. https://doi.org/10.3390/app11114963
APA StyleJo, K., Ha, J., Ryu, J., Lee, E., & Lee, H. (2021). DC 4-Point Measurement for Total Electrical Conductivity of SOFC Cathode Material. Applied Sciences, 11(11), 4963. https://doi.org/10.3390/app11114963





