Perspective on Some Recent and Future Developments in Casimir Interactions
Abstract
1. Introduction
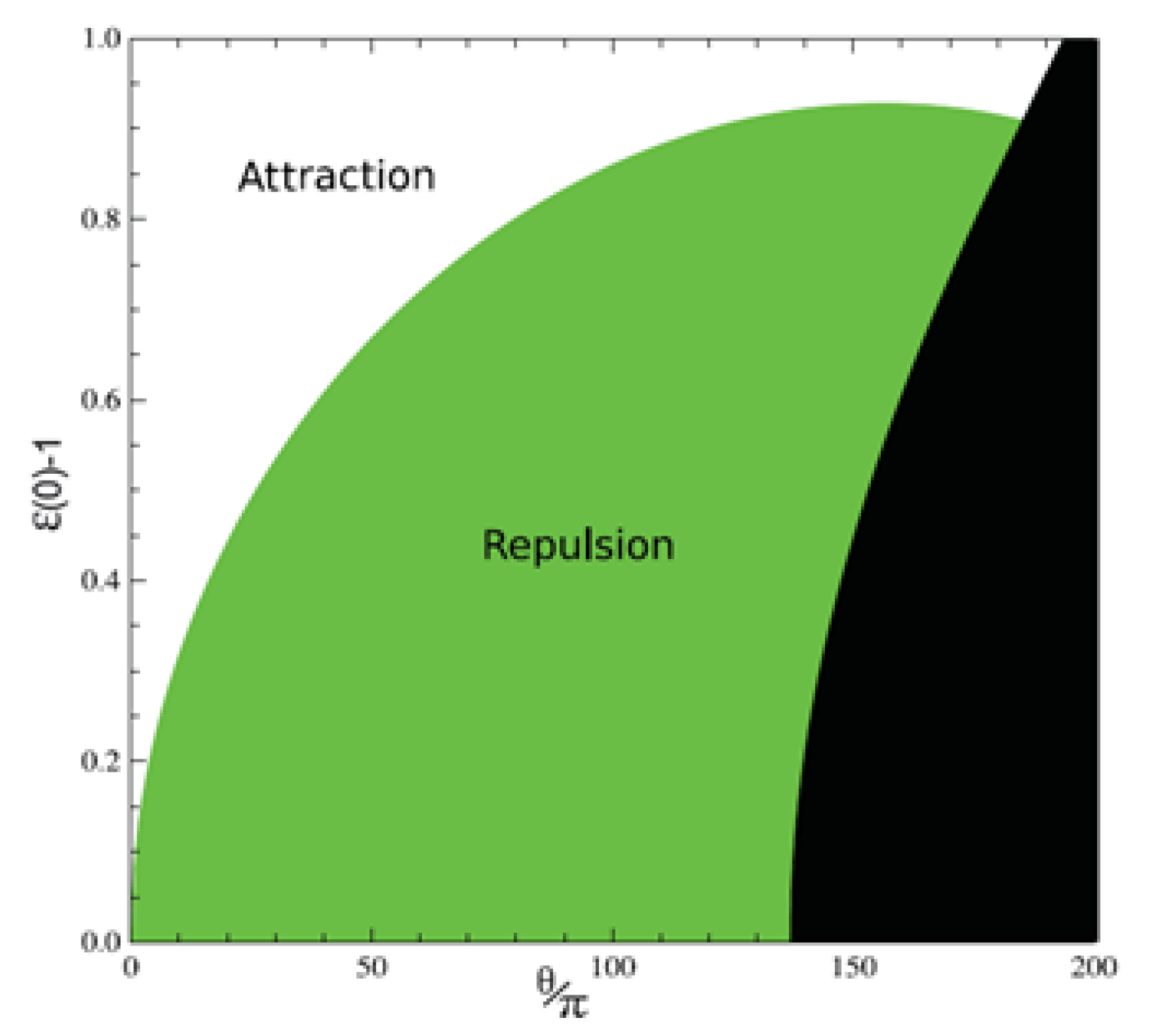
2. Nontrivial Topology in Materials
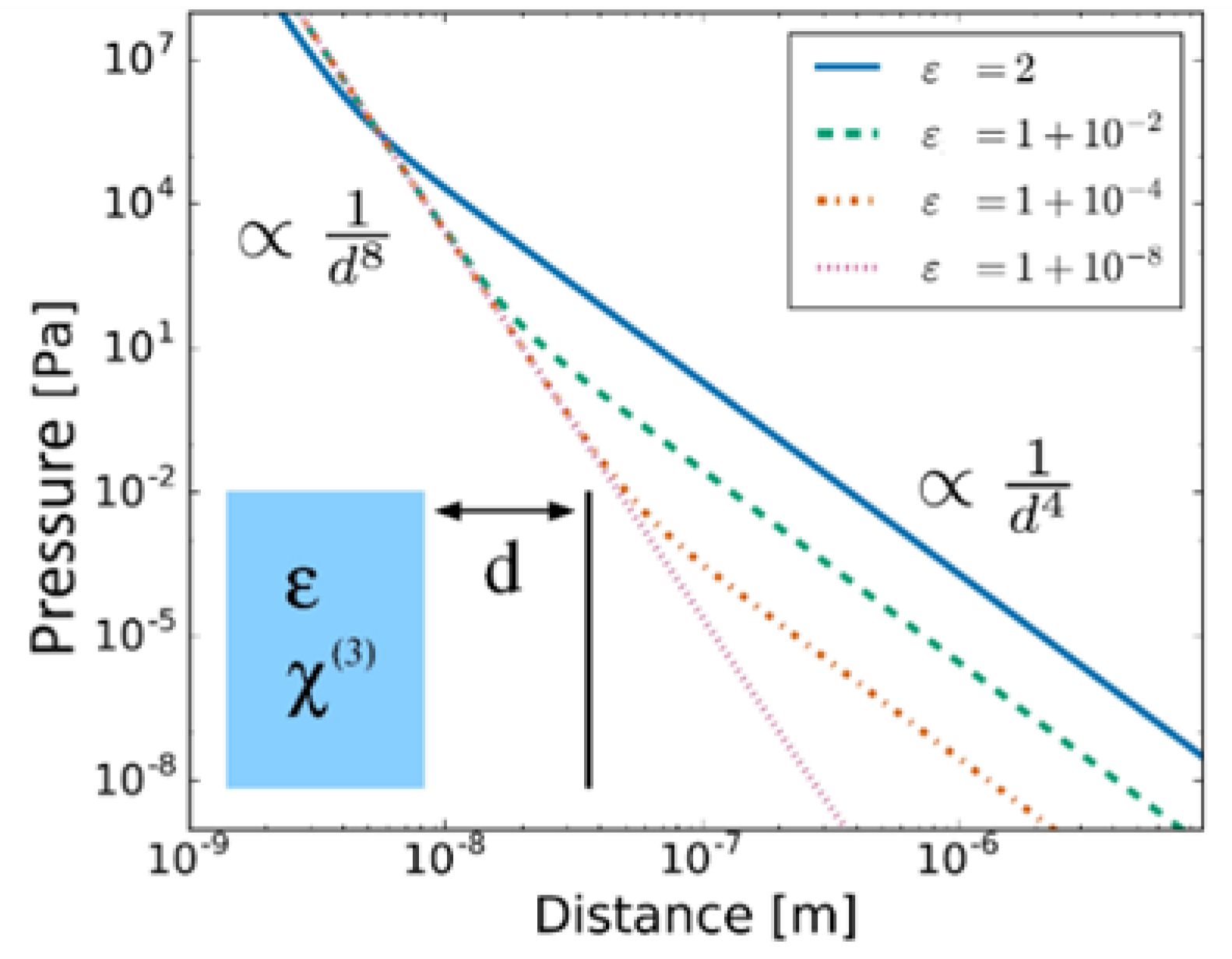
3. Nonlinear Optical Response
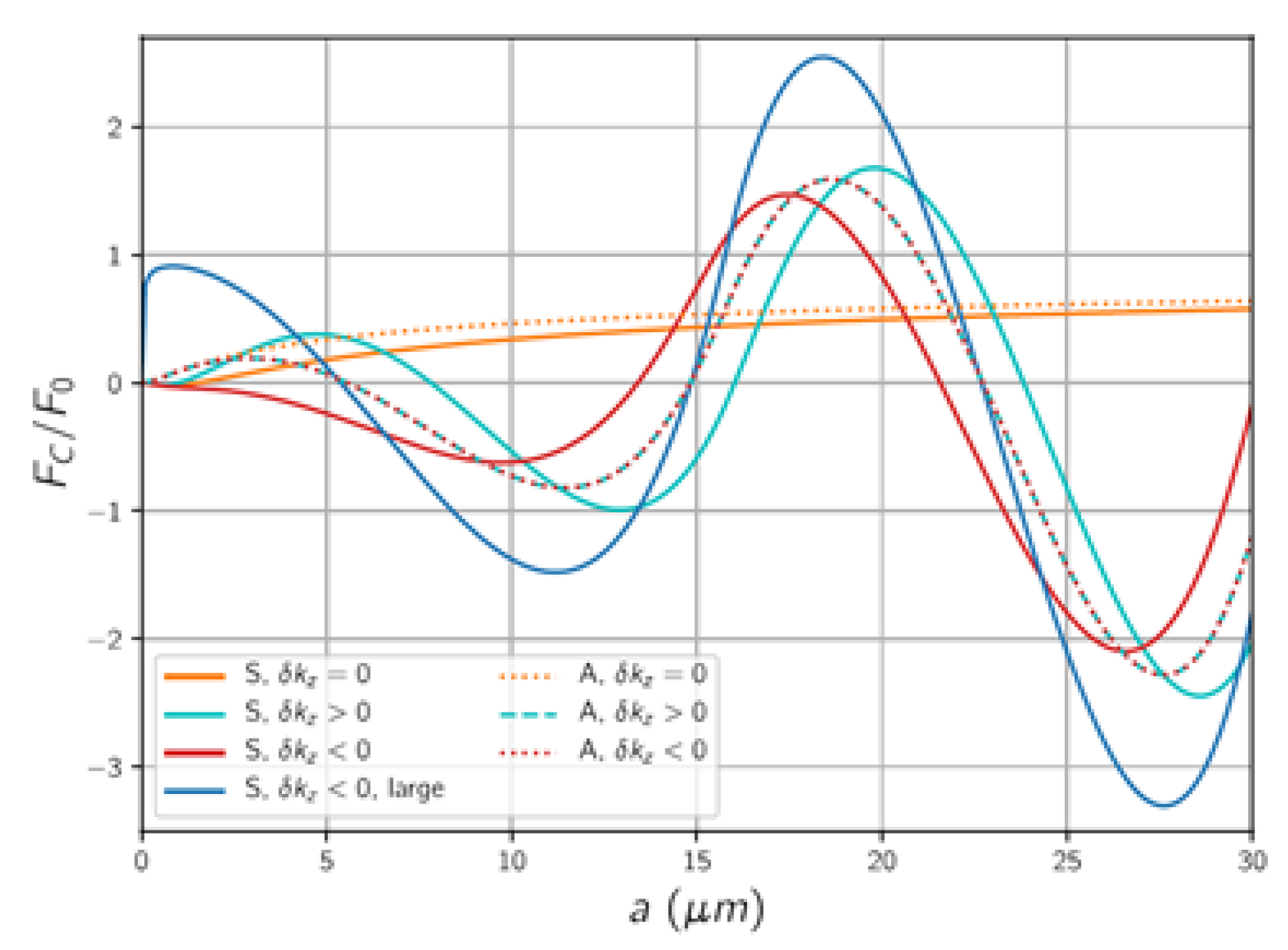
4. Dynamical Casimir Effect
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Woods, L.M.; Dalvit, D.A.R.; Tkatchenko, A.; Rodriguez-Lopez, P.; Rodriguez, A.W.; Podgornik, R. Materials perspective on Casimir and van der Waals interactions. Rev. Mod. Phys. 2016, 88, 045003. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. The Casimir force between real materials: Experiment and theory. Rev. Mod. Phys. 2009, 81, 1827. [Google Scholar] [CrossRef]
- Barcenas, J.; Reyes, L.; Esquivel-Sirvent, R. Scaling of micro- and nanodevices actuated by Casimir forces. Appl. Phys. Lett. 2005, 87, 263106. [Google Scholar] [CrossRef]
- Duong, D.L.; Yun, S.J.; Lee, Y.H. Van der Waals layered materials: Opportunities and challenges. ACS Nano 2017, 11, 11803. [Google Scholar] [CrossRef]
- Hermann, J.; DiStasio, R.A., Jr.; Tkatchenko, A. First-principles models for van der Waals interactions in molecules and materials: Concepts, theory, and applications. Chem. Rev. 2017, 117, 4714. [Google Scholar] [CrossRef] [PubMed]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological insulators. Rev. Mod. Phys. 2011, 83, 1057. [Google Scholar] [CrossRef]
- Wehling, T.O.; Black-Schaffer, A.M.; Balatsky, A.V. Dirac materials. Adv. Phys. 2014, 63, 1–76. [Google Scholar] [CrossRef]
- Armitage, N.P.; Mele, E.J.; Vishwanath, A. Weyl and Dirac semimetals in three-dimensional solids. Rev. Mod. Phys. 2018, 90, 015001. [Google Scholar] [CrossRef]
- Wen, X.; Gong, Z.; Li, D. Nonlinear optics of two-dimensional transition metals dichalcogenides. InfoMat 2019, 1, 317. [Google Scholar] [CrossRef]
- Xu, Q.; Zhang, Y.; Koepernik, K.; Shi, W.; van der Brink, J.; Felser, C.; Sun, Y. Comprehensive scan for nonmagnetic Weyl semimetals with nonlinear optical response. NPJ Comp. Mater. 2020, 32, 1–7. [Google Scholar] [CrossRef]
- Wu, L.; Patankar, S.; Morimoto, T.; Nair, N.L.; Thewalt, E.; Little, A.; Analytis, J.G.; Moore, J.E.; Ornstein, J. Giant anisotropic nonlinear optical response in transition metal monopnictide Weyl semimetal. Nat. Phys. 2017, 13, 350. [Google Scholar] [CrossRef]
- De Huan, F.; Grushin, A.G.; Morimoto, T.; Moore, J.E. Quantized circular photogalvanic effect in Weyl semimetals. Nat. Comm. 2017, 8, 15995. [Google Scholar]
- Moore, G.T. Quantum theory of the electromagnetic field in a variable-length one-dimensional cavity. J. Math. Phys. 1970, 11, 2679. [Google Scholar] [CrossRef]
- Wilson, C.M.; Johansson, G.; Pourkabirian, A.; Simoen, M.; Johansson, J.R.; Duty, T.; Nori, F.; Delsing, P. Observation of the dynamical Casimir effect in a superconducting circuit. Nature 2011, 479, 376. [Google Scholar] [CrossRef]
- Hasan, M.Z.; Moore, J.E. Three-dimensional topological insulators. Annu. Rev. Condens. Matter Phys. 2011, 2, 55. [Google Scholar] [CrossRef]
- Qi, X.-L.; Li, R.; Zang, J.; Zhang, S.-C. Inducing a magnetic monopole with topological surface states. Science 2009, 323, 1184. [Google Scholar] [CrossRef]
- Grushin, A.G.; Cortijo, A. Tunable casimir repulsion with three-dimensional topological insulators. Phys. Rev. Lett. 2011, 106, 020403. [Google Scholar] [CrossRef]
- Grushin, A.G.; Rodriguez-Lopez, P.; Cortijo, A. Effect of finite temperature and uniaxial anisotropy on the Casimir effect with three-dimensional topological insulators. Phys. Rev. B 2011, 84, 045119. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P. Casimir repulsion between topological insulators in the diluted regime. Phys. Rev. B 2011, 84, 165409. [Google Scholar] [CrossRef]
- Nie, W.; Zeng, R.; Lan, Y.; Zhu, S. Casimir force between topological insulator slabs. Phys. Rev. B 2013, 88, 085412. [Google Scholar] [CrossRef]
- Lu, B.-S. Van der Waals torque and force between anisotropic topological insulator slabs. Phys. Rev. B 2018, 97, 045427. [Google Scholar] [CrossRef]
- Zeng, R.; Chen, L.; Nie, W.J.; Ni, M.H.; Yang, Y.P.; Zhu, S.Y. Enhancing Casimir repulsion via topological insulator multilayers. Phys. Lett. A 2016, 380, 2861. [Google Scholar] [CrossRef]
- Martin-Ruiz, A.; Urrutia, L.F. Interaction of a hydrogenlike ion with a planar topological insulator. Phys. Rev. A 2018, 97, 022502. [Google Scholar] [CrossRef]
- Li, L.L.; Peeters, F.M. Optical conductivity of topological insulator thin films. J. Appl. Phys. 2015, 117, 175305. [Google Scholar] [CrossRef]
- Li, Z.; Carbotte, J.P. Magneto-optical conductivity in a topological insulator. Phys. Rev. B 2013, 88, 045414. [Google Scholar] [CrossRef]
- Rahim, K.; Ullah, A.; Tahir, M.; Sabeeh, K. Magneto-optical properties of topological insulator thin films with broken inversion symmetry. J. Phys. Condens. Matter 2017, 29, 425304. [Google Scholar] [CrossRef] [PubMed]
- Haldane, F.D.M. Model for a quantum Hall effect without landau levels: Condensed matter realization of the “Parity Anomaly”. Phys. Rev. Lett. 1988, 61, 2015. [Google Scholar] [CrossRef]
- Tang, E.; Mei, J.-W.; Wen, X.-G. High-temperature fractional Quantum Hall States. Phys. Rev. Lett. 2011, 106, 236802. [Google Scholar] [CrossRef]
- Verberos, J.W.F.; Kourtis, S.; van der Brink, J.; Daghofer, M. Fractional quantum-hall liquid spontaneously generated by strongly correlated t2g electrons. Phys. Rev. Lett. 2012, 108, 125405. [Google Scholar]
- Rachel, S. Interacting topological insulators: A review. Rep. Prog. Phys. 2018, 81, 116501. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P.; Grushin, A. Repulsive Casimir effect with Chern insulators. Phys. Rev. Lett. 2014, 112, 056804. [Google Scholar] [CrossRef] [PubMed]
- Fialkovsky, N.K.; Vassilevich, D. Quest for Casimir repulsion between Chern-Simons surfaces. Phys. Rev. B 2018, 97, 165432. [Google Scholar] [CrossRef]
- Vassilevich, D. On the (im) possibility of Casimir repulsion between Chern-Simons surfaces. Mod. Phys. Lett. A 2020, 35, 2040017. [Google Scholar] [CrossRef]
- Marachevsky, V.N. Casimir effect for Chern-Simons layers in the vacuum. Theor. Math. Phys. 2017, 190, 315. [Google Scholar] [CrossRef]
- Marachevsky, V.N. Casimir interaction of two dielectric half spaces with Chern-Simons boundary layers. Phys. Rev. B 2019, 99, 075420. [Google Scholar] [CrossRef]
- Marachevsky, V.N. Chern-Simons boundary layers in the Casimir effect. Mod. Phys. Lett. A 2020, 35, 2040015. [Google Scholar] [CrossRef]
- Marachevsky, V.N.; Pis’mak, Y.M. Casimir-Polder effect for a plane with Chern-Simons interaction. Phys. Rev. D 2010, 81, 065005. [Google Scholar] [CrossRef]
- Vogt, P.; de Padova, P.; Quaresima, C.; Avila, J.; Frantzeskakis, E.; Arsenio, M.C.; Resta, A.; Ealet, B.; le Lay, G. Silicene: Compelling experimental evidence for graphene-like two-dimensional silicon. Phys. Rev. Lett. 2012, 108, 155501. [Google Scholar] [CrossRef]
- Da’vila, M.E.; Xian, L.; Cahangirov, S.; Rubio, A.; Lay, G.L. Germanene: A novel two-dimensional germanium allotrope akin to graphene and silicene. New J. Phys. 2014, 16, 095002. [Google Scholar] [CrossRef]
- Zhu, F.-F.; Chen, W.-J.; Gao, C.-L.; Guan, D.-D.; Liu, C.-H.; Qian, D.; Zhang, S.-C.; Jia, J.-F. Epitaxial growth of two-dimensional stanene. Nat. Mater. 2015, 14, 1020–1026. [Google Scholar] [CrossRef]
- Ezawa, M. Photoinduced topological phase transition and a single Dirac-cone state in silicene. Phys. Rev. Lett. 2013, 110, 026603. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez-Lopez, P.; Kort-Kamp, W.J.M.; Dalvit, D.A.R.; Woods, L.M. Casimir force phase transitions in the graphene family. Nat. Comm. 2017, 8, 14699. [Google Scholar] [CrossRef] [PubMed]
- Gómez-Santos, G. Thermal van der Waals interaction between graphene layers. Phys. Rev. B 2009, 80, 245424. [Google Scholar] [CrossRef]
- Drosdoff, D.; Woods, L.M. Casimir forces and graphene sheets. Phys. Rev. B 2010, 82, 155459. [Google Scholar] [CrossRef]
- Bordag, M.; Klimchitskaya, G.L.; Mostepanenko, V.M. Thermal Casimir effect in the interaction of graphene with dielectric and metals. Phys. Rev. B 2012, 86, 165429. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P.; Kort-Kamp, W.J.M.; Dalvit, D.A.R.; Woods, L.M. Nonlocal optical response in topological phase transitions in the graphene family. Phys. Rev. Mater. 2018, 2, 014003. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M.; Sernelius, B.E. Two approaches for describing the Casimir interaction in graphene: Density-density correlation function versus polarization tensor. Phys. Rev. B 2014, 89, 125407. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Theory of the Casimir interaction from graphene-coated substrates using the polarization tensor and comparison with experiment. Phys. Rev. B 2014, 89, 115419. [Google Scholar] [CrossRef]
- Fialkovsky, V.; Marachevsky, N.; Vassilevich, D.V. Finite-temperature Casimir effect for graphene. Phys. Rev. B 2011, 84, 035446. [Google Scholar] [CrossRef]
- Khusnutdinov, N.; Kashapov, R.; Woods, L.M. Casmir effect for a stack of conductive planes. Phys. Rev. D 2015, 92, 045002. [Google Scholar] [CrossRef]
- Khusnutdinov, N.; Kashapov, R.; Woods, L.M. Thermal Casimir and Casimir-Polder effects in N parallel 2D Dirac materials. 2D Mater. 2018, 5, 035032. [Google Scholar] [CrossRef]
- Henkel, C.; Klimchitskaya, G.L.; Mostepanenko, V.M. Influence of the chemical potential on the Casimir-Polder interaction between an atom and gapped graphene or graphene-coated substrate. Phys. Rev. A 2018, 97, 032504. [Google Scholar] [CrossRef]
- Cycne, T.; Kort-Kamp, W.J.M.; Oliver, D.; Pinheiro, F.A.; Rosa, F.S.S.; Farina, C. Tuning the Casimir-Polder interaction via magneto-optical effects in graphene. Phys. Rev. A 2014, 90, 052511. [Google Scholar]
- Drosdoff, D.; Bondarev, I.V.; Widom, A.; Podgornik, R.; Woods, L.M. Charge induced fluctuation forces in graphitic nanostructures. Phys. Rev. X 2016, 6, 011004. [Google Scholar] [CrossRef]
- Li, M.S.; Reimers, J.R.; Dobson, J.F.; Gould, T. Faraday cage screening reveals intrinsic aspects of the van der Waals attraction. Proc. Natl. Acad. Sci. USA 2018, 115, E10295. [Google Scholar] [CrossRef]
- Farias, M.B.; Kort-Kamp, W.J.M.; Dalvit, D.A.R. Quantum friction in two-dimensional topological materials. Phys. Rev. B 2018, 97, 161407. [Google Scholar] [CrossRef]
- Tabert, C.J.; Carbotte, J.P. Optical conductivity of Weyl semimetals and signatures of the gapped semimetal phase transition. Phys. Rev. B 2016, 93, 085442. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P.; Popescu, A.; Fialkovsky, I.; Khusnutdinov, N.; Woods, L.M. Signatures of complex optical response in Casimir interactions of type I and II Weyl semimetals. Comm. Mater. 2020, 1, 14. [Google Scholar] [CrossRef]
- Wilson, J.H.; Allocca, A.A.; Galistki, V. Repulsive casimir force between Weyl semimetals. Phys. Rev. B 2015, 91, 235115. [Google Scholar] [CrossRef]
- Farias, M.B.; Zyuzin, A.Z.; Schmidt, T.L. Casimir force between Weyl semimetals in a chiral medium. Phys. Rev. B 2020, 101, 235446. [Google Scholar] [CrossRef]
- Jiang, Q.-D.; Wilczek, F. Chiral Casimir force: Repulsive, enhanced, tunable. Phys. Rev. B 2019, 99, 125403. [Google Scholar] [CrossRef]
- Franken, P.A.; Hill, A.E.; Peters, C.W.; Weinreich, G. Generation of optical harmonics. Phys. Rev. Lett. 1961, 7, 118. [Google Scholar] [CrossRef]
- You, J.-S.; Fang, S.; Xu, S.-Y.; Kaxiras, E.; Low, T. Berry curvature dipole current in the transition metal dichalcogenide family. Phys. Rev. B 2018, 98, 121109. [Google Scholar] [CrossRef]
- Rostami, H.; Polini, M. Nonlinear anomalous photocurrents in Weyl semiemtals. Phys. Rev. B 2018, 97, 195151. [Google Scholar] [CrossRef]
- Drummond, P.D. Electromagnetic quantization in dispersive inhomogeneous nonlinear dielectrics. Phys. Rev. A 1990, 42, 6845. [Google Scholar] [CrossRef]
- Scheel, S.; Welsch, D.G. Quantum theory of light and noise polarization in nonlinear optics. Phys. Rev. Lett. 2006, 96, 073601. [Google Scholar] [CrossRef]
- Kysylychyn, D.; Piatnytsia, V.; Lozovski, V. Electrodynamical interaction between a nanoparticle and the surface of a solid. Phys. Rev. E 2013, 88, 052403. [Google Scholar] [CrossRef]
- Makhnovets, K.; Kolezhuk, A. On short-range enhancement of Van-der-Waals forces. Materwiss. Werksttech. 2016, 47, 222. [Google Scholar] [CrossRef]
- Soo, H.; Dean, D.S.; Krüger, M. Particles with nonlinear electric response: Suppressing van der Waals forces by an external field. Phys. Rev. E 2017, 95, 012151. [Google Scholar] [CrossRef]
- Soo, H.; Krüger, M. Fluctuational electrodynamics for nonlinear media. Eur. Lett. 2016, 115, 41002. [Google Scholar] [CrossRef][Green Version]
- Soo, H.; Krüger, M. Fluctuational electrodynamics for nonlinear materials in and out of thermal equilibrium. Phys. Rev. B 2017, 97, 045412. [Google Scholar] [CrossRef]
- Karvonen, L.; Rönn, J.; Kujala, S.; Chen, Y.; Säynätjoki, A.; Tervonen, A.; Svirko, Y.; Honkanen, S. High non-resonant third-order optical nonlinearity of Ag-glass nanocomposite fabricated by two-step ion exchange. Opt. Mater. 2013, 36, 328. [Google Scholar] [CrossRef]
- Kheirandish, F.; Amooghorban, E.; Soltani, M. Finite-temperature Casimir effect in the presence of nonlinear dielectrics. Phys. Rev. A 2011, 83, 032507. [Google Scholar] [CrossRef]
- Khandekar, F.; Pick, A.; Johnson, S.G.; Rodriguez, A.W. Radiative heat thermal transfer in nonlinear Kerr media. Phys. Rev. B 2015, 91, 115406. [Google Scholar] [CrossRef]
- Khandekar, C.; Rodriguez, A.W. Near-field thermal upconversion and energy transfer through a Kerr medium. Opt. Express 2017, 25, 23164. [Google Scholar] [CrossRef]
- Neto, P.A.M.; Machado, L.A.S. Quantum radiation generated by a moving mirror in free space. Phys. Rev. A 1996, 54, 3420. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Klimov, A.B. Generation and detection of photons in a cavity with a resonantly oscillating boundary. Phys. Rev. A 1996, 53, 2664. [Google Scholar] [CrossRef]
- Lambrecht, A.; Jaekel, M.T.; Reynaud, S. Motion induced radiation from a vibrating cavity. Phys. Rev. Lett. 1996, 77, 615. [Google Scholar] [CrossRef]
- Crocce, M.; Dalvit, D.A.R.; Mazzitelli, F.D. Resonant photon creation in a three-dimensional oscillating cavity. Phys. Rev. A 2001, 64, 013808. [Google Scholar] [CrossRef]
- Uhlmann, M.; Plunien, G.; Schützhold, R.; Soff, G. Resonant cavity photon creation via the dynamical Casimir effect. Phys. Rev. Lett. 2004, 93, 193601. [Google Scholar] [CrossRef]
- Yablonovitch, E. Accelerating reference frame for electromagnetic waves in a rapidly growing plasma: Unruh-Davies-Fulling-De Witt radiation and the nonadiabatic Casimir effect. Phys. Rev. Lett. 1989, 62, 1742. [Google Scholar] [CrossRef]
- Srivastava, Y.; Widom, A. Quantum electrodynamic processes in electrical engineering circuits. Phys. Rep. 1987, 148, 1–65. [Google Scholar] [CrossRef]
- Segev, E.; Abdo, B.; Shtempluck, O.; Buks, E.; Yurke, B. Prospects of employing superconducting stripline resonators for studying the dynamical Casimir effect experimentally. Phys. Lett. A 2007, 370, 202. [Google Scholar] [CrossRef][Green Version]
- Fuji, T.; Matsuo, S.; Hatakenaka, N.; Kurihara, S.; Zeilinger, A. Quantum circuit analog of the dynamical Casimir effect. Phys. Rev. B 2011, 84, 174521. [Google Scholar] [CrossRef]
- Lähteenmäki, P.; Paraoanu, G.S.; Hassel, J.; Hakonen, P.J. Dynamical Casimir effect in a Josephson metamaterial. Proc. Nat. Acad. Sci. 2013, 110, 4234. [Google Scholar] [CrossRef]
- Johansson, J.R.; Johansson, G.; Wilson, C.M.; Delsing, P.; Nori, F. Nonclassical microwave radiation from the dynamical Casimir effect. Phys. Rev. A 2013, 87, 043804. [Google Scholar] [CrossRef]
- Nation, P.D.; Johansson, J.R.; Blencowe, M.P.; Nori, F. Colloquium: Stimulating uncertainty: Amplifying the quantum vacuum with superconducting circuits. Rev. Mod. Phys. 2012, 84, 1. [Google Scholar] [CrossRef]
- Nation, P.D.; Suh, J.; Blencowe, M.P. Ultrastrong optomechanics incorporating the dynamical Casimir effect. Phys. Rev. A 2016, 93, 022510. [Google Scholar] [CrossRef]
- Wustmann, W.; Shumeiko, V. Parametric effects in circuit quantum electrodynamics. Low Temp. Phys. 2019, 45, 848. [Google Scholar] [CrossRef]
- Dodonov, V.V. Fifty years of the dynamical Casimir effect. Physics 2020, 2, 67. [Google Scholar] [CrossRef]
- Yablonovitch, E.; Heritage, J.P.; Aspnes, D.E.; Yafet, Y. Virtual photoconductivity. Phys. Rev. Lett. 1989, 63, 976. [Google Scholar] [CrossRef]
- Okushima, T.; Shimizu, A. Photon emission from a false vacuum of semiconductors. Jpn. J. Appl. Phys. 1995, 34, 4508. [Google Scholar] [CrossRef]
- Lozovik, Y.E.; Tsvetus, V.G.; Vinogradov, E.A. Parametric excitation of vacuum by use of femtosecond laser pulses. Phys. Scr. 1995, 52, 184. [Google Scholar] [CrossRef]
- Braggio, C.; Bressi, G.; Carugno, G.; del Noce, C.; Galeazzi, G.; Lombardi, A.; Palmieri, A.; Ruoso, G.; Zanello, D. A novel experimental approach for the detection of the dynamic Casimir effect. Eur. Lett. 2005, 70, 754. [Google Scholar] [CrossRef][Green Version]
- Agnesi, A.; Braggio, C.; Carugno, G.; della Valle, F.; Galeazzi, G.; Messineo, G.; Pirzio, F.; Reali, G.; Ruoso, G. A laser system for the parametric amplification of electromagnetic fields in a microwave cavity. Rev. Sci. Instr. 2011, 82, 115107. [Google Scholar] [CrossRef]
- Kim, W.-J.; Brownell, J.H.; Onofrio, R. Comment on ‘Novel experimental approach for the detection of the dynamical Casimir effect’ by C. Braggio et al. EPL Europhys. Lett. 2007, 78, 21002. [Google Scholar] [CrossRef]
- Hagenmüller, D. All-optical dynamical Casimir effect in a three-dimensional terahertz photonic band gap. Phys. Rev. B 2016, 93, 235309. [Google Scholar] [CrossRef]
- Dezael, F.X.; Lambrecht, A. Analogue Casimir radiation using an optical parametric oscillator. EPL Europhys. Lett. 2010, 89, 14001. [Google Scholar] [CrossRef][Green Version]
- Faccio, D.; Carusotto, I. Dynamical Casimir effect in optically modulated cavities. EPL Europhys. Lett. 2011, 96, 24006. [Google Scholar] [CrossRef][Green Version]
- Hizhnyakov, V.; Kaasik, H.; Tehver, I. Spontaneous nonparametric down-conversion of light. Appl. Phys. A 2014, 115, 563. [Google Scholar] [CrossRef]
- Hizhnyakov, V.; Loot, A.; Azizabadi, S.C. Enhanced dynamical Casimir effect for surface and guided waves. Appl. Phys. A 2016, 122, 333. [Google Scholar] [CrossRef]
- Vezzoli, S.; Mussot, A.; Westerberg, N.; Kudlinski, A.; Saleh, H.D.; Prain, A.; Biancalana, F.; Lantz, E.; Faccio, D. Optical analogue of the dynamical Casimir effect in a dispersion-oscillating fibre. Comm. Phys. 2019, 2, 84. [Google Scholar] [CrossRef]
- Braggio, C.; Carugno, G.; Borghesani, A.F.; Dodonov, V.V.; Pirzio, F.; Ruoso, G. Generation of microwave fields in cavities with laser-excited nonlinear media: Competition between the second- and third-order optical nonlinearities. J. Opt. 2018, 20, 095502. [Google Scholar] [CrossRef]
- Sanz, M.; Wieczorek, W.; Groblacher, S.; Solano, E. Electro-mechanical Casimir effect. Quantum 2018, 2, 91. [Google Scholar] [CrossRef]
- Wang, H.; Blencowe, M.P.; Wilson, C.M.; Rimberg, A.J. Mechanically generating entangled photons from the vacuum: A microwave circuit-acoustic resonator analog of the oscillatory Unruh effect. Phys. Rev. A 2019, 99, 053833. [Google Scholar] [CrossRef]
- Qin, W.; Macrì, V.; Miranowicz, A.; Savasta, S.; Nori, F. Emission of photon pairs by mechanical stimulation of the squeezed vacuum. Phys. Rev. A 2019, 100, 062501. [Google Scholar] [CrossRef]
- Carusotto, I.; Balbinot, R.; Fabbri, A.; Recati, A. Density correlations and analog dynamical Casimir emission of Bogoliubov phonons in modulated atomic Bose-Einstein condensates. Eur. Phys. J. D 2010, 56, 391. [Google Scholar] [CrossRef]
- Jaskula, J.-C.; Partridge, G.B.; Bonneau, M.; Lopes, R.; Ruaudel, J.; Boiron, D.; Westbrook, C.I. Acoustic analog to the dynamical Casimir effect in a Bose-Einstein condensate. Phys. Rev. Lett. 2012, 109, 220401. [Google Scholar] [CrossRef]
- Balbinot, R.; Fabbri, A. Amplifying the Hawking signal in BECs. Adv. High. Energy Phys. 2014, 2014, 713574. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Mendonca, J.T. Dynamical Casimir effect in ultra-cold matter with a time-dependent effective charge. Phys. Scr. 2014, T160, 014008. [Google Scholar] [CrossRef]
- Eckel, S.; Kumar, A.; Jacobson, T.; Spielman, I.B.; Campbell, G.K. A rapidly expanding Bose-Einstein condensate: An expanding universe in the lab. Phys. Rev. X 2018, 8, 021021. [Google Scholar] [CrossRef] [PubMed]
- Michael, M.H.; Schmiedmayer, J.; Demler, E. From the moving piston to the dynamical Casimir effect: Explorations with shaken condensates. Phys. Rev. A 2019, 99, 053615. [Google Scholar] [CrossRef]
- Lawandy, N.M. Scattering of vacuum states by dynamic plasmon singularities: Generating photons from vacuum. Opt. Lett. 2006, 31, 3650. [Google Scholar] [CrossRef]
- Ciuti, C.; Bastard, G.; Carusotto, I. Quantum vacuum properties of the intersubband cavity polariton field. Phys. Rev. B 2005, 72, 115303. [Google Scholar] [CrossRef]
- Koghee, S.; Wouters, M. Dynamical Casimir emission from polariton condensates. Phys. Rev. Lett. 2014, 112, 036406. [Google Scholar] [CrossRef] [PubMed]
- Hizhnyakov, V.; Loot, A.; Azizabadi, S.C. Dynamical Casimir effect for surface plasmon polaritons. Phys. Lett. A 2015, 379, 501. [Google Scholar] [CrossRef]
- Naylor, W. Vacuum-excited surface plasmon polaritons. Phys. Rev. A 2015, 91, 053804. [Google Scholar] [CrossRef]
- Wang, X.; Qin, W.; Miranowicz, A.; Savasta, S.; Nori, F. Unconventional cavity optomechanics: Nonlinear control of phonons in the acoustic quantum vacuum. Phys. Rev. A 2019, 100, 063827. [Google Scholar] [CrossRef]
- Wittemer, M.; Hakelberg, F.; Kiefer, P.; Schröder, J.-P.; Fey, C.; Schützhold, R.; Warring, U.; Schaetz, T. Phonon pair creation by inflating quantum fluctuations in an ion trap. Phys. Rev. Lett. 2019, 123, 180502. [Google Scholar] [CrossRef]
- Maclay, G.J.; Forward, R.L. A Gedanken spacecraft that operates using the quantum vacuum (dynamic Casimir effect). Found. Phys. 2004, 34, 477. [Google Scholar] [CrossRef][Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Woods, L.M.; Krüger, M.; Dodonov, V.V. Perspective on Some Recent and Future Developments in Casimir Interactions. Appl. Sci. 2021, 11, 293. https://doi.org/10.3390/app11010293
Woods LM, Krüger M, Dodonov VV. Perspective on Some Recent and Future Developments in Casimir Interactions. Applied Sciences. 2021; 11(1):293. https://doi.org/10.3390/app11010293
Chicago/Turabian StyleWoods, Lilia M., Matthias Krüger, and Victor V. Dodonov. 2021. "Perspective on Some Recent and Future Developments in Casimir Interactions" Applied Sciences 11, no. 1: 293. https://doi.org/10.3390/app11010293
APA StyleWoods, L. M., Krüger, M., & Dodonov, V. V. (2021). Perspective on Some Recent and Future Developments in Casimir Interactions. Applied Sciences, 11(1), 293. https://doi.org/10.3390/app11010293






