Parametrized Analysis and Multi-Objective Optimization of Supercritical CO2 (S-CO2) Power Cycles Coupled with Parabolic Trough Collectors
Abstract
1. Introduction
- Three S-CO2 power cycles coupled with PTC (namely, simple regeneration, intercooling and re-compression) are established and their parameters are analyzed for preliminary comparison.
- A multi-objective optimization method using genetic algorithm (GA) is presented to compare the performance, including thermodynamic performance and preliminary economic cost of cycles. The advantages and disadvantages of the three cycle kinds are thoroughly compared.
- The sensitivity analysis of a typical optimization result of a simple regeneration cycle is carried out; the influence of different parameters on system performance is obtained.
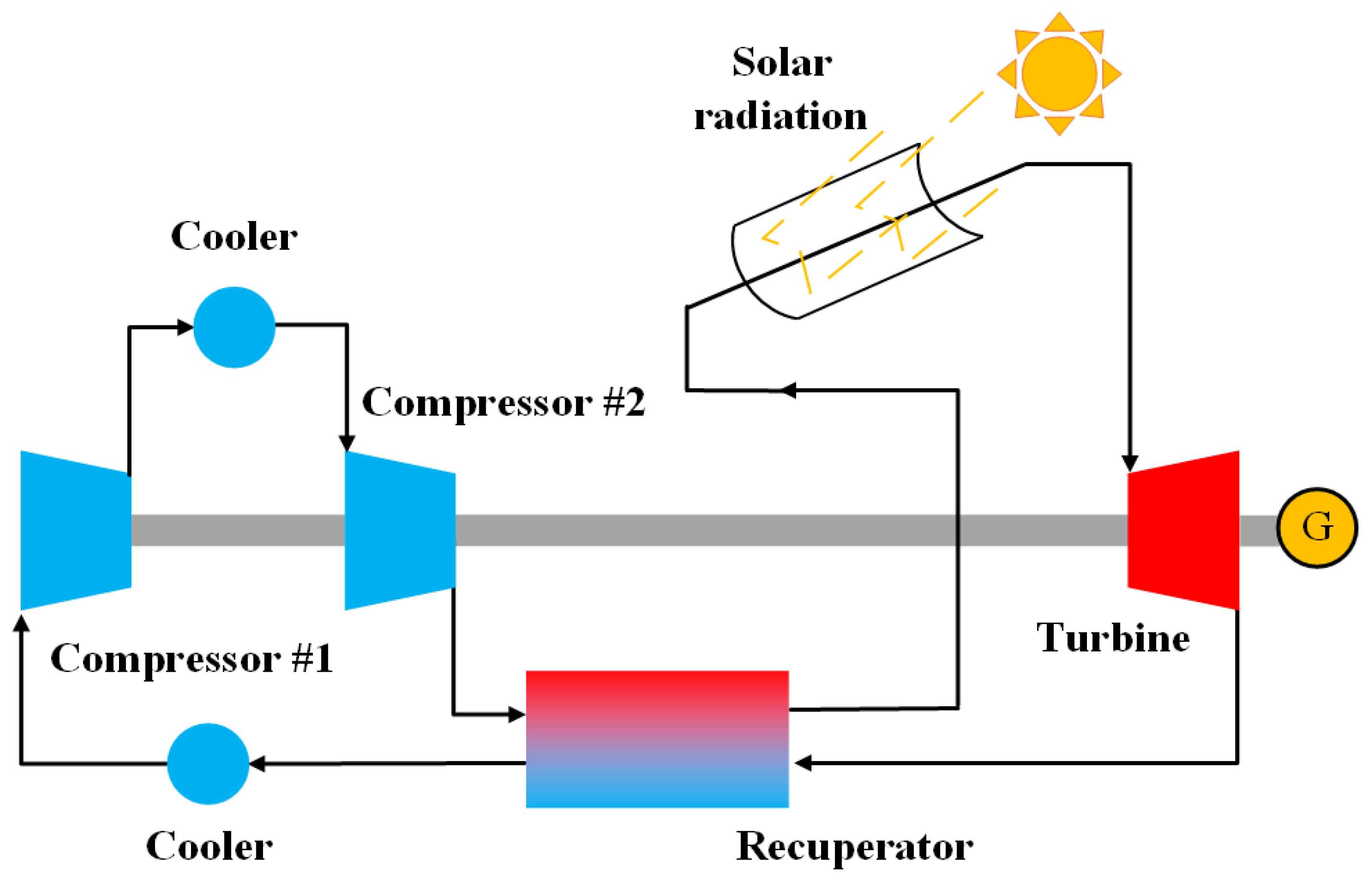
2. Description of the Research Systems
3. Modeling of the System
- The cycle is considered steady during calculation.
- The effects of kinetic and gravitational terms are neglected in the calculations.
- The pressure drops and heat loss are neglected.
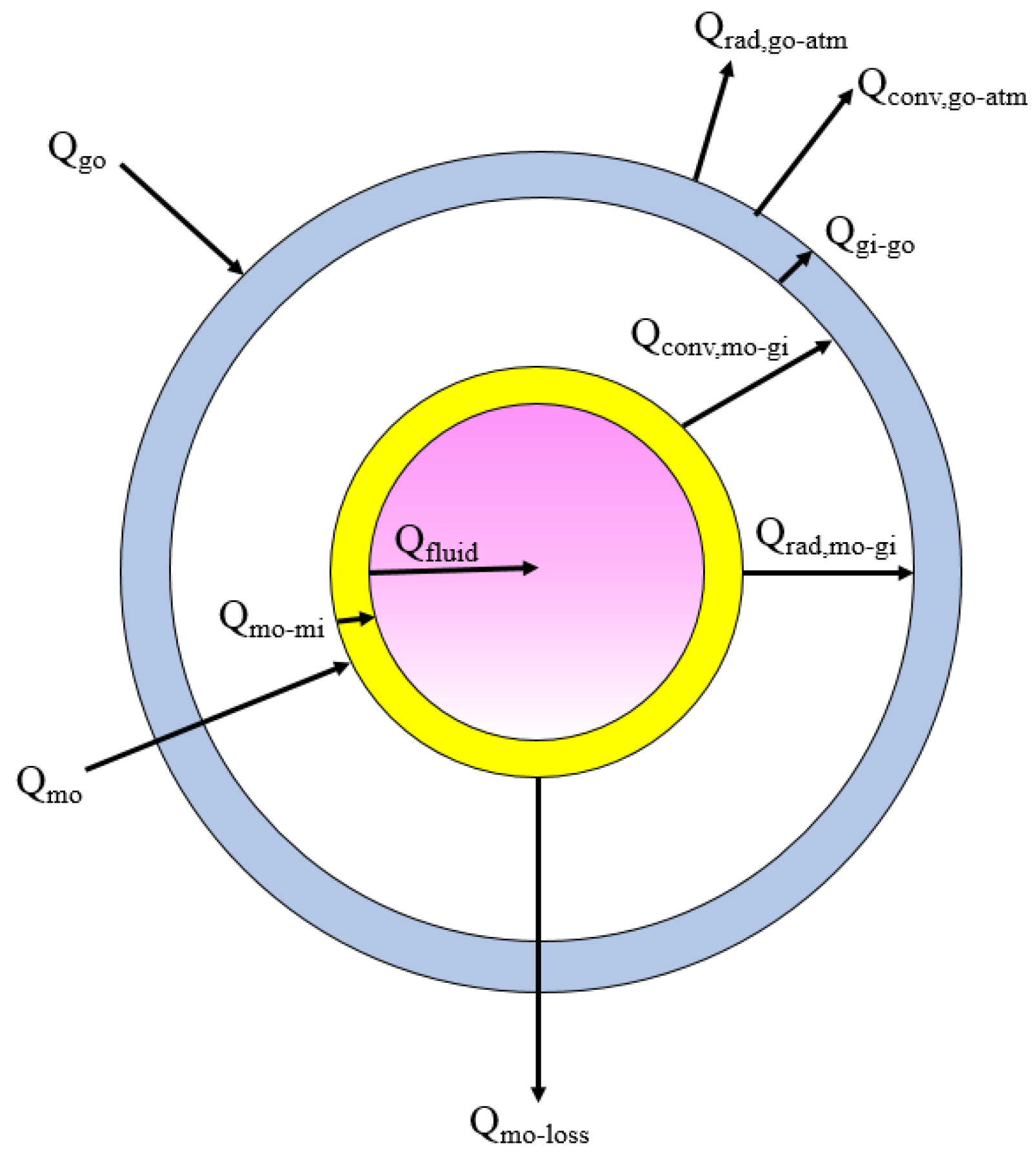
3.1. Solar Collector
3.2. Other Thermodynamic Components
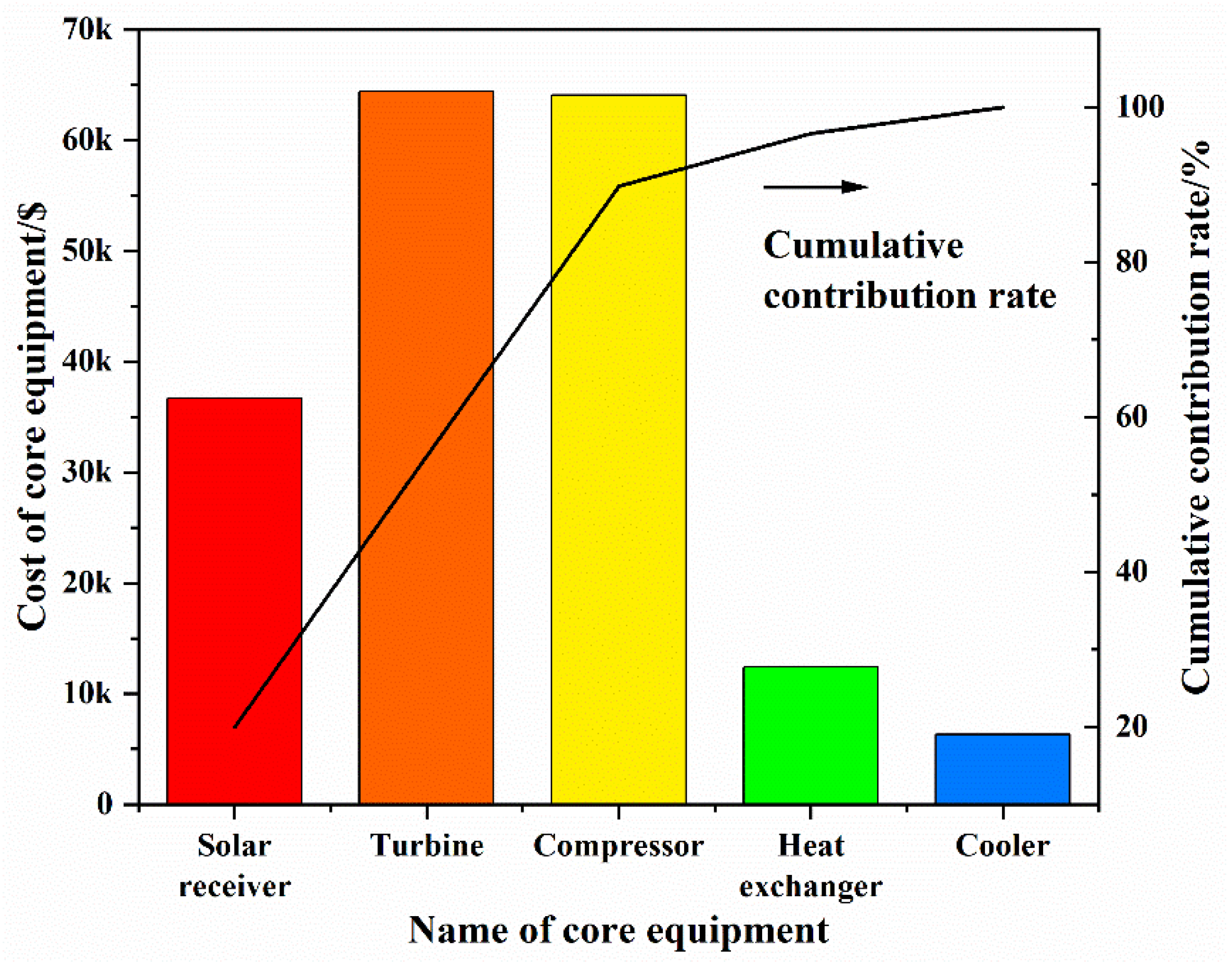
3.3. Equipment Cost Accountings
- The cost of the core equipment is considered the total cost of the system. In actuality, the cost of the core equipment consists of only part of the total cost, with pipes, construction fee, and engineering design as additions. Nevertheless, in this design process, we assume these costs are proportional to the total amount. Hence, we can obtain sufficient analysis to conduct the comparison of the proposed three system configurations, considering thermodynamics and thermoeconomic performance.
- Characteristic parameters are used to calculate the cost of different core equipment. However, geometric design parameters and flow forms of turbomachinery will affect the purchasing price of the device, which will undoubtedly lead to some deviations compared to actual cost of the S-CO2 system. However, this deviation can be acceptable for these three kinds of systems because we can consider it as consistent.
3.4. Performance Criteria
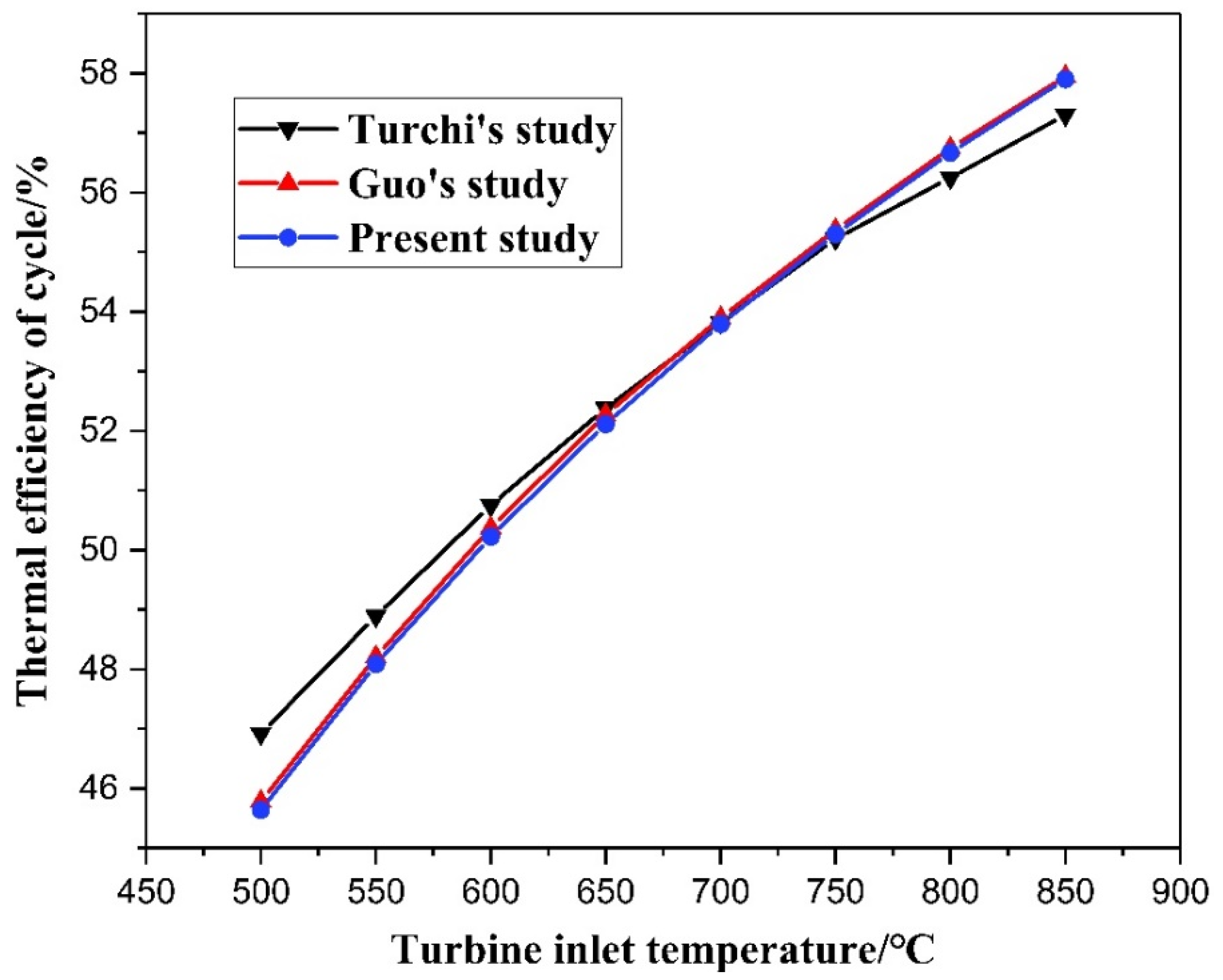
3.5. Model Validation
4. Results and Discussion
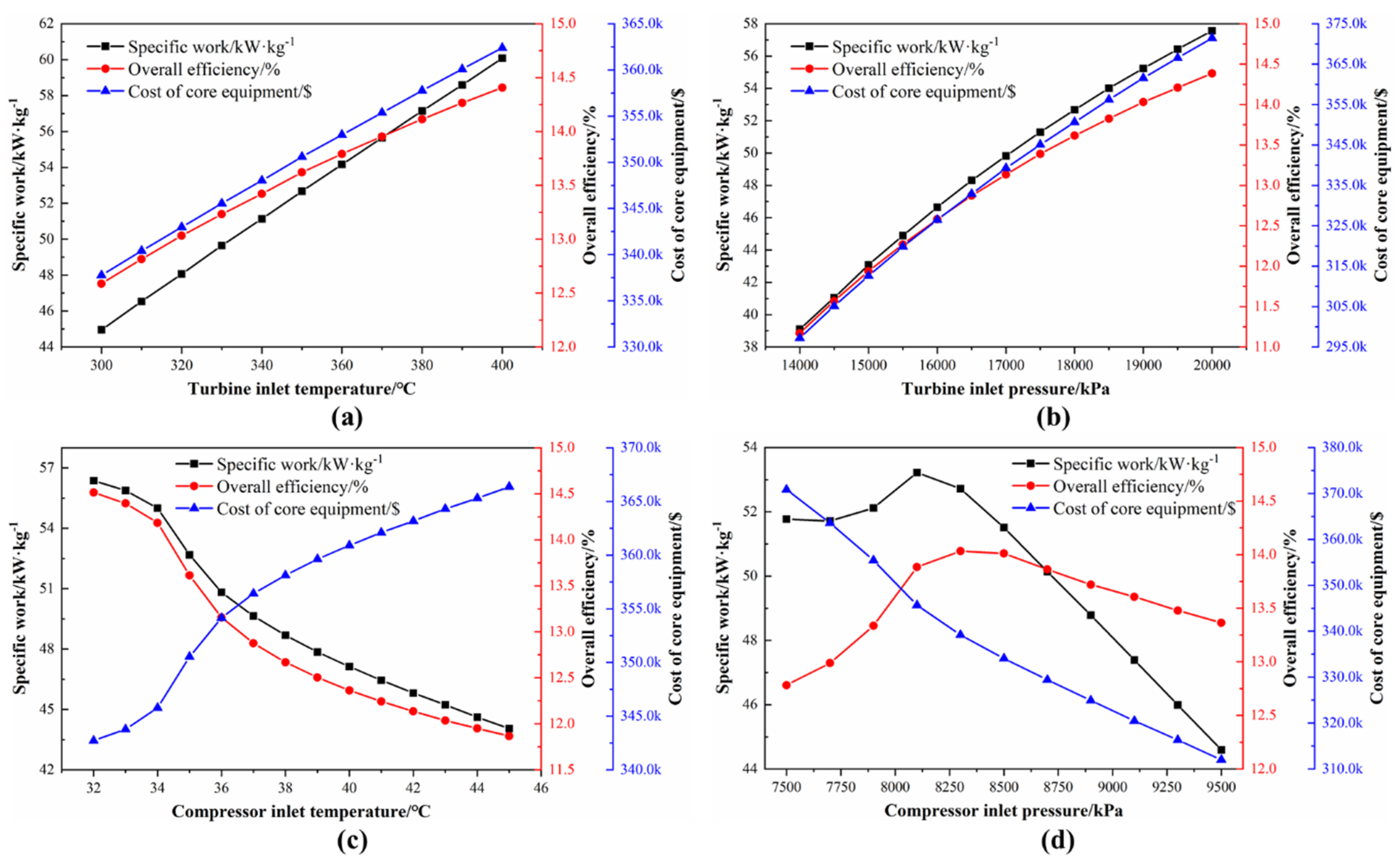
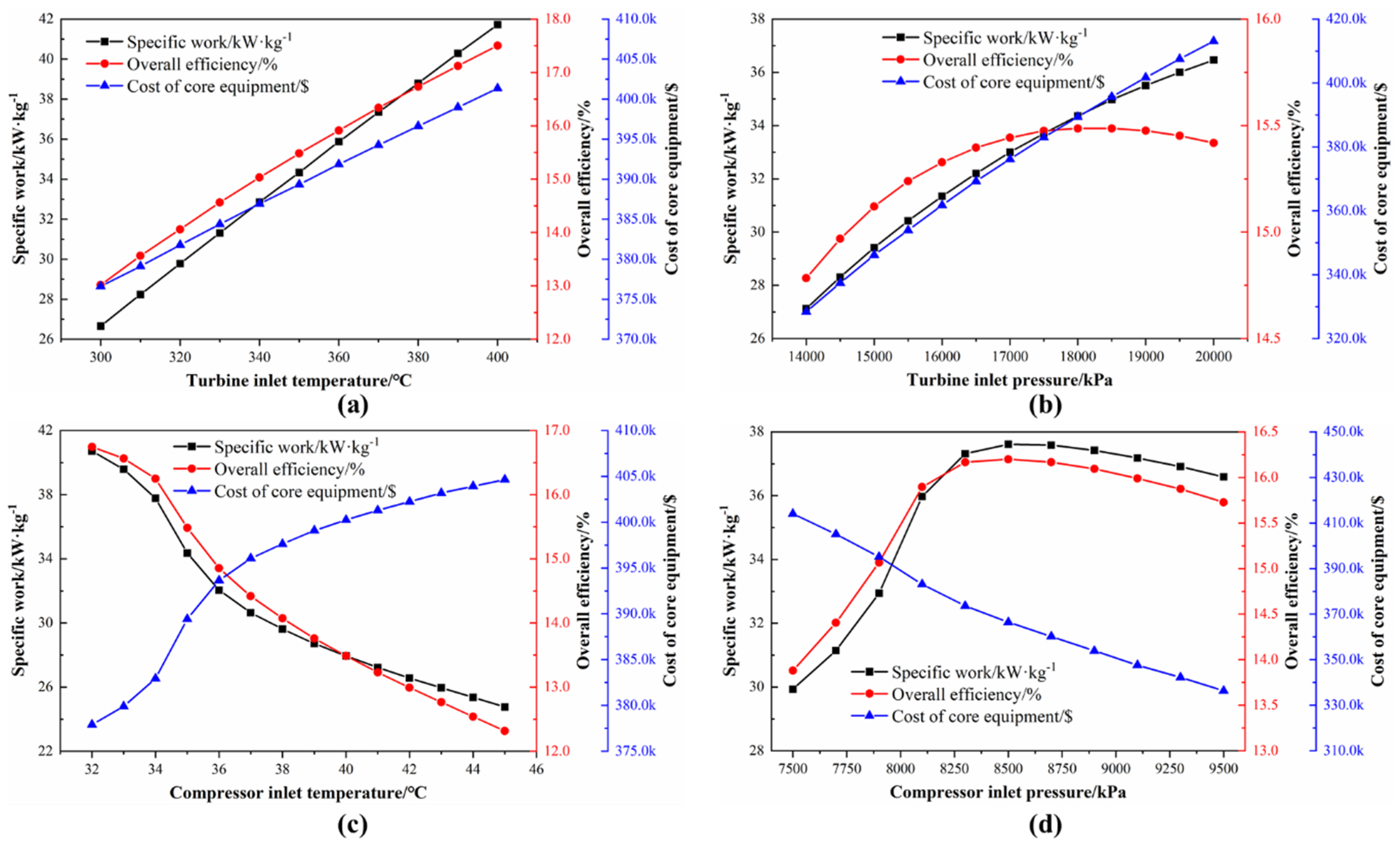
4.1. Parameter Study
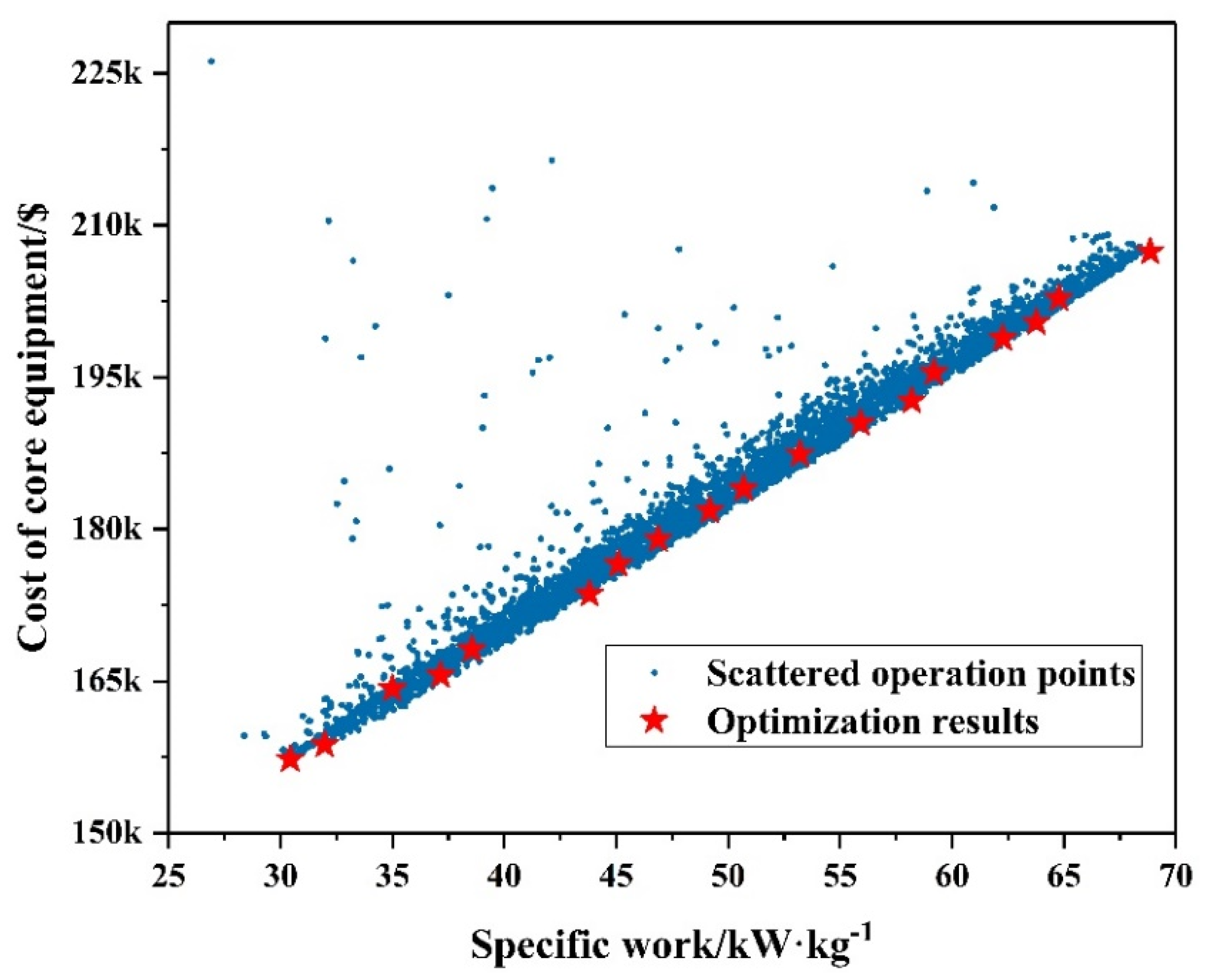
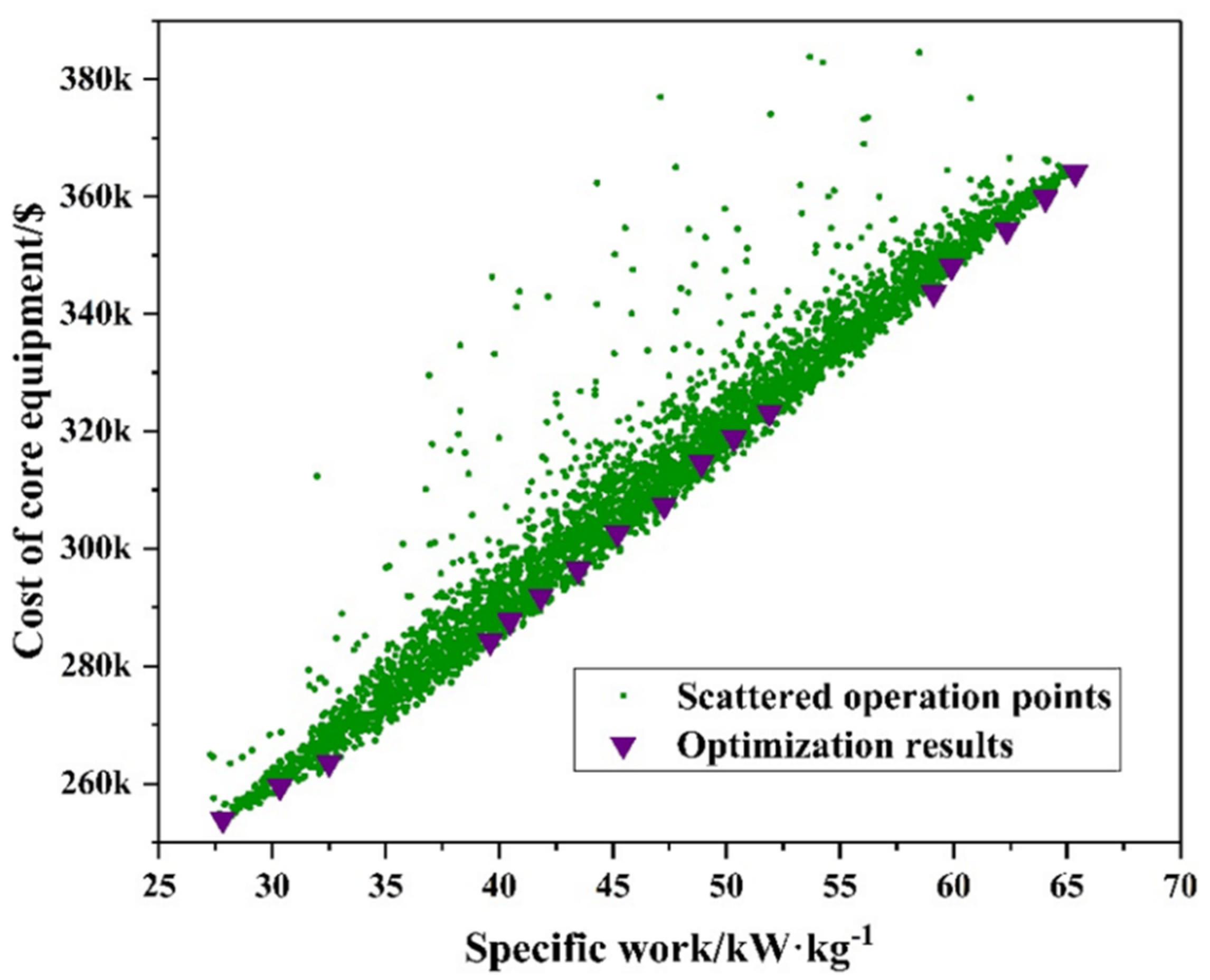
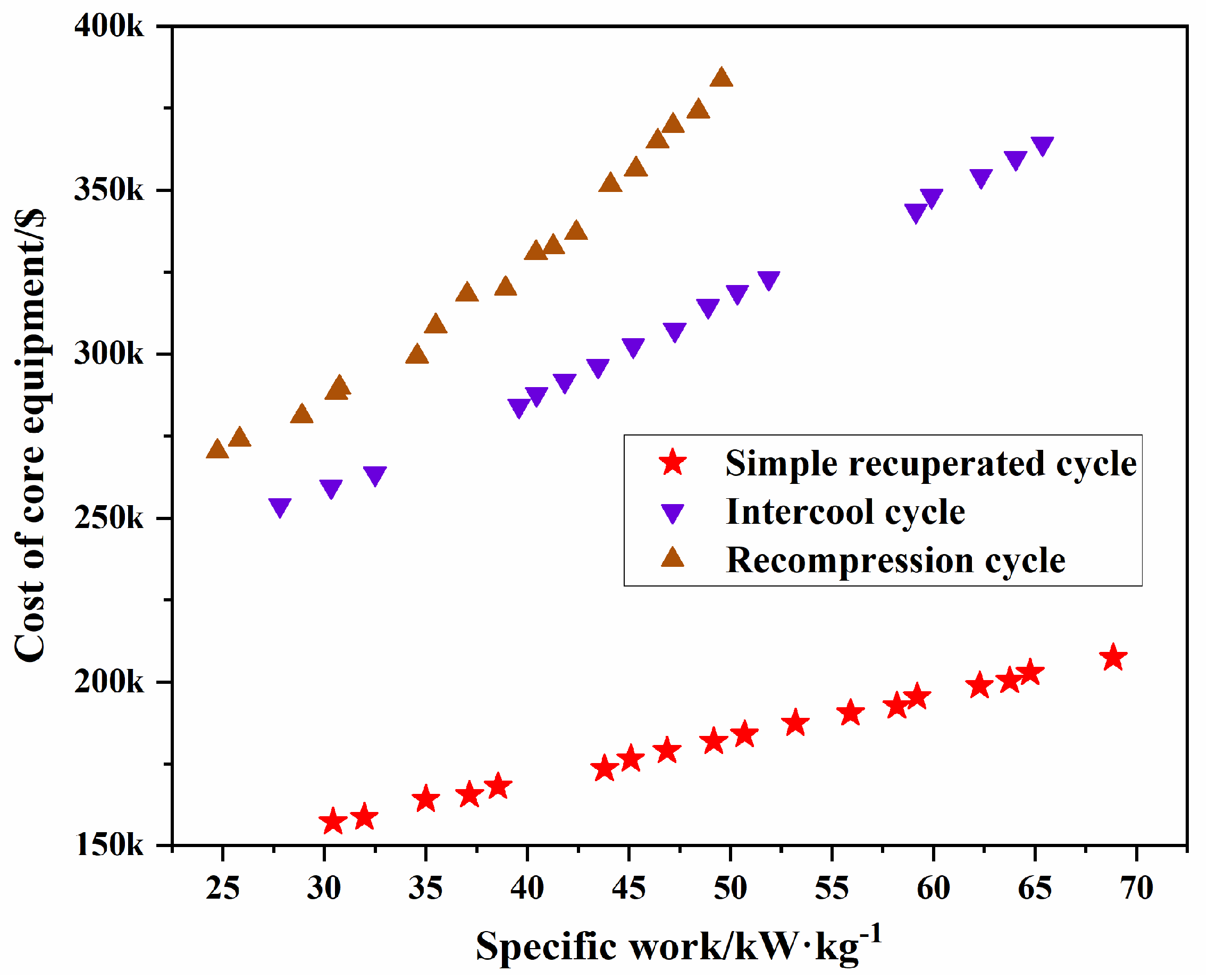
4.2. Multi-Objective Optimization
4.3. Sensitivity Analysis of a Typical Result
5. Conclusions
- (1)
- It results in an optimal specific work ranging between 30 and 70 kW/kg, and optimal cost of core equipment ranging between $155,000 and $210,000 USD for the simple recuperated cycle. The values of the optimization objectives extend between 27 and 65 kW/kg for specific work and between $255,000 and $365,000 USD for cost of core equipment in the intercooling cycle. Moreover, the values of the optimization objectives extend between 25 and 50 kW/kg for specific work and from $270,000 and $370,000 USD for cost of core equipment in the recompression cycle.
- (2)
- The simple recuperation cycle layout shows more excellent performance than the intercooling cycle layout and the recompression cycle layout in terms of cost, while the advantage in specific work of the intercooling cycle layout and the recompression cycle layout is not obvious.
- (3)
- The sensitivity analysis of a typical optimization result of a simple regeneration cycle shows that the change of parameters have little influence on the cost of core equipment and much influence on specific work.
- (4)
- Through this study, it can be found that the turbine inlet temperature is low using a parabolic trough solar collector, and the complex SCO2 Brayton cycles are not dominant.
- (5)
- The preliminary analysis and systematic comparison in this study can be useful in selecting cycle layout using solar energy by a parabolic trough solar collector when there are requirements for the specific work and the cost of core equipment. Moreover, it can also provide reference for the comparison and selection of other cycle types.
Author Contributions
Conflicts of Interest
Nomenclature
| a | thermal adaptation coefficient of solar collector |
| b | interaction coefficient of solar collector |
| CR | concentration ratio |
| D | diameter (m) |
| f | friction coefficient |
| h | enthalpy (kJ·kg-1) |
| hc | convective heat transfer coefficient (kW·m-2·K-1) |
| k | conductivity (kW·m-1·K-1) |
| l | length (m) |
| Nu | Nusselt number |
| P | Pressure (MPa) |
| Pr | Prandtl number |
| Q | heat transfer rate (kW) |
| Re | Reynolds number |
| T | temperature (K) |
| U | heat transfer coefficient (kW·m-2·K-1) |
| t | plate thickness (mm) |
| Greeks | |
| α | absorptivity |
| γ | heat capacity ratio |
| δ | molecular diameter of air (cm) |
| ε | emissivity |
| η | efficiency |
| λ | mean free path of molecular (cm) |
| ρ | reflectivity of trough reflector |
| σ | Boltzmann constant |
| τ | transmittance |
| Subscripts | |
| atm | atmosphere |
| c | compressor |
| conv | convective |
| fluid | working fluid |
| gi | inner surface of glass envelope |
| go | outer surface of glass envelope |
| id | ideal state with no entropy increase |
| j | number of transfer units |
| mi | inner surface of metal absorber |
| mo | outer surface of metal absorber |
| out | outlet condition |
| rad | radiative |
References
- Mellit, A.; Massi, P.A.; Ogliari, E.; Leva, S.; Lughi, V. Advanced Methods for Photovoltaic Output Power Forecasting: A Review. Appl. Sci. 2020, 10, 487. [Google Scholar] [CrossRef]
- Cucchiella, F.; D’Adamo, I.; Gastaldi, M. A profitability assessment of small-scale photovoltaic systems in an electricity market without subsidies. Energ. Convers. Manag. 2016, 129, 62–74. [Google Scholar] [CrossRef]
- Fthenakis, V.; Raugei, M. Environmental life-cycle assessment of photovoltaic systems. In The Performance of Photovoltaic (PV) Systems; Pearsall, N., Ed.; Woodhead Publishing: Cambridge, UK, 2017; pp. 209–232. [Google Scholar]
- Li, L.; Sun, J.; Li, Y. Prospective fully-coupled multi-level analytical methodology for concentrated solar power plants: General modelling. Appl. Therm. Eng. 2017, 118, 171–187. [Google Scholar] [CrossRef]
- Qiu, Y.; He, Y.L.; Cheng, Z.D.; Wang, K. Study on optical and thermal performance of a linear Fresnel solar reflector using molten salt as HTF with MCRT and FVM methods. Appl. Energy 2015, 146, 162–173. [Google Scholar] [CrossRef]
- Du, B.C.; He, Y.L.; Zheng, Z.J.; Cheng, Z.D. Analysis of thermal stress and fatigue fracture for the solar tower molten salt receiver. Appl. Therm. Eng. 2016, 99, 741–750. [Google Scholar] [CrossRef]
- ACCIONA. Nevada Solar One. Available online: http://www.acciona.us/projects/energy/concentrating-solar-power/nevada-solar-one/ (accessed on 1 January 2017).
- Fernández-García, A.; Zarza, E.; Valenzuela, L.; Pérez, M. Parabolic-trough solar collectors and their applications. Renew. Sustain. Energy Rev. 2010, 14, 1695–1721. [Google Scholar] [CrossRef]
- Cayer, E.; Galanis, N.; Desilets, M.; Nesreddine, H.; Roy, P. Analysis of a carbon dioxide transcritical power cycle using a low temperature source. Appl. Energ. 2009, 86, 1055–1063. [Google Scholar] [CrossRef]
- Price, H.; Lüpfert, E.; Kearney, D.; Zarza, E.; Cohen, G.; Gee, R.; Mahoney, R. Advances in parabolic trough solar power technology. J. Energ. Eng. 2002, 124, 109–125. [Google Scholar] [CrossRef]
- Usman, M.; Imran, M.; Yang, Y.; Lee, D.H.; Park, B.-S. Thermo-economic comparison of air-cooled and cooling tower based Organic Rankine Cycle (ORC) with R245fa and R1233zde as candidate working fluids for different geographical climate conditions. Energy 2017, 123, 353–366. [Google Scholar] [CrossRef]
- Mecheri, M.; Le Moullec, Y. Supercritical CO2 Brayton cycles for coal-fired power plants. Energy 2016, 103, 758–771. [Google Scholar] [CrossRef]
- Singh, R.; Miller, S.A.; Rowlands, A.S.; Jacobs, P.A. Dynamic characteristics of a direct-heated supercritical carbon-dioxide Brayton cycle in a solar thermal power plant. Energy 2013, 50, 194–204. [Google Scholar] [CrossRef]
- Hiroaki, T.; Niichi, N.; Masaru, H.; Ayao, T. Forced convection heat transfer to fluid near critical point flowing in circular tube. Int. J. Heat Mass Transfer. 1971, 14, 739–750. [Google Scholar] [CrossRef]
- Monje, B.; Sanchez, D.; Savill, M.; Pilidis, P.; Sanchez, T. A Design Strategy for Supercritical CO2 Compressors; American Society Mechanical Engineers: New York, NY, USA, 2014. [Google Scholar]
- Kim, Y.M.; Kim, C.G.; Favrat, D. Transcritical or supercritical CO2 cycles using both low- and high-temperature heat sources. Energy 2012, 43, 402–415. [Google Scholar] [CrossRef]
- Osorio, J.D.; Hovsapian, R.; Ordonez, J.C. Dynamic analysis of concentrated solar supercritical CO2-based power generation closed-loop cycle. Appl. Therm. Eng. 2016, 93, 920–934. [Google Scholar] [CrossRef]
- Valdes, M.; Abbas, R.; Rovira, A.; Martin-Aragon, J. Thermal efficiency of direct, inverse and SCO2 gas turbine cycles intended for small power plants. Energy 2016, 100, 66–72. [Google Scholar] [CrossRef]
- De la Calle, A.; Bayon, A.; Hinkley, J.; Pye, J. System-level simulation of a novel solar power tower plant based on a sodium receiver, PCM storage and sCO2 power block. AIP Conf. Proc. 2018, 2033, 210003. [Google Scholar]
- Binotti, M.; Astolfi, M.; Campanari, S.; Manzolini, G.; Silva, P. Preliminary assessment of SCO2 cycles for power generation in CSP solar power plants. Appl. Energ. 2017, 204, 1007–1017. [Google Scholar] [CrossRef]
- Turchi, C.S.; Ma, Z.; Neises, T.W.; Wagner, M.J. Thermodynamic study of advanced supercritical carbon dioxide power cycle for concentrating solar power. J. Sol. Energy Eng. 2013, 135, 041007. [Google Scholar] [CrossRef]
- Padilla, R.V.; Too, Y.C.S.; Benito, R.; Stein, W. Exergetic analysis of supercritical CO2 Brayton cycles integrated with solar central receivers. Appl. Energ. 2015, 148, 348–365. [Google Scholar] [CrossRef]
- Chapman, D.J.; Arias, D.A. An Assessment of the Supercritical Carbon Dioxide Cycle for Use in a Solar Parabolic Trough Power Plant. In Proceedings of the Supercritical CO2 Power Cycle Symposium, New York, NY, USA, 29–30 April 2009. [Google Scholar]
- Singh, R.; Rowlands, A.S.; Miller, S.A. Effects of relative volume-ratios on dynamic performance of a direct-heated supercritical carbon-dioxide closed Brayton cycle in a solar-thermal power plant. Energy 2013, 55, 1025–1032. [Google Scholar] [CrossRef]
- Wang, K.; Li, M.J.; Guo, J.Q.; Li, P.W.; Liu, Z.B. A systematic comparison of different S-CO2 Brayton cycle layouts based on multi-objective optimization for applications in solar power tower plants. Appl. Energ. 2018, 212, 109–121. [Google Scholar] [CrossRef]
- Vasquez Padilla, R.; Soo Too, Y.C.; Benito, R.; McNaughton, R.; Stein, W. Multi-objective thermodynamic optimisation of supercritical CO2 Brayton cycles integrated with solar central receivers. Int. J. Sustain. Energy 2018, 37, 1–20. [Google Scholar] [CrossRef]
- Ho, C.K.; Carlson, M.; Garg, P.; Kumar, P. Technoeconomic Analysis of Alternative Solarized s-CO2 Brayton Cycle Configurations. J. Solar Energy Eng. 2016, 138, 051008. [Google Scholar] [CrossRef]
- Feher, E.G. The supercritical thermodynamic power cycle. Energ. Convers. 1968, 8, 85–90. [Google Scholar] [CrossRef]
- Ahn, Y.; Bae, S.J.; Kim, M.; Cho, S.K.; Baik, S.; Lee, J.I.; Cha, J.E. Review of supercritical CO2 power cycle technology and current status of research and development. Nucl. Eng. Technol. 2015, 47, 647–661. [Google Scholar] [CrossRef]
- Moisseytsev, A.; Sienicki, J.J. Investigation of alternative layouts for the supercritical carbon dioxide Brayton cycle for a sodium-cooled fast reactor. Nucl. Eng. Des. 2009, 239, 1362–1371. [Google Scholar] [CrossRef]
- Calle, A.D.L.; Bayon, A.; Too, Y.C.S. Impact of ambient temperature on supercritical CO2 recompression Brayton cycle in arid locations: Finding the optimal design conditions. Energy 2018, 153, 1016–1027. [Google Scholar] [CrossRef]
- Wang, X.; Li, X.; Li, Q.; Liu, L.; Liu, C. Performance of a solar thermal power plant with direct air-cooled supercritical carbon dioxide Brayton cycle under off-design conditions. Appl. Energy 2020, 261, 114359. [Google Scholar] [CrossRef]
- Guo, J.; Huai, X.; Cheng, K. The comparative analysis on thermal storage systems for solar power with direct steam generation. Renew. Energ. 2018, 115, 217–225. [Google Scholar] [CrossRef]
- Gnielinski, V. New equations for heat mass transfer in turbulent pipe and channel flows. Int. Chem. Eng. 1976, 16, 359–368. [Google Scholar]
- Ratzel, A.; Hickox, C.; Gartling, D. Techniques for reducing thermal conduction and natural convection heat losses in annular receiver geometries. J. Sol. Energ-T. Asme 1979, 101, 108–113. [Google Scholar] [CrossRef]
- Swinbank, W.C. Long-wave radiation from clear skies. Q. J. Roy. Meteor. Soc. 1963, 89, 339–348. [Google Scholar] [CrossRef]
- Dostal, V.; Driscoll, M.J.; Hejzlar, P. A supercritical carbon dioxide cycle for next generation nuclear reactors. Mass. Inst. Technol. 2004, 154, 265–282. [Google Scholar]
- Henchoz, S.; Buchter, F.; Favrat, D.; Morandin, M.; Mercangoz, M. Thermoeconomic analysis of a solar enhanced energy storage concept based on thermodynamic cycles. Energy 2012, 45, 358–365. [Google Scholar] [CrossRef]
- Yoon, S.H.; Kim, J.H.; Hwang, Y.W.; Kim, M.S.; Min KKim, Y. Heat transfer and pressure drop characteristics during the in-tube cooling process of carbon dioxide in the supercritical region. Int. J. Refrig. 2003, 26, 857–864. [Google Scholar] [CrossRef]
- Guo, J.Q.; Li, M.J.; He, Y.L.; Xu, J.L. A study of new method and comprehensive evaluation on the improved performance of solar power tower plant with the CO2-based mixture cycles. Appl. Energ. 2019, 256, 113837. [Google Scholar] [CrossRef]
- He, Y.L.; Mei, D.H.; Tao, W.Q.; Yang, W.W.; Liu, H.L. Simulation of the parabolic trough solar energy generation system with Organic Rankine Cycle. Appl. Energy 2012, 97, 630–641. [Google Scholar] [CrossRef]
- Al-Sulaiman, F.A. Energy and sizing analyses of parabolic trough solar collector integrated with steam and binary vapor cycles. Energy 2013, 58, 561–570. [Google Scholar] [CrossRef]





| Parameters | Value |
|---|---|
| Inner diameter of absorber/m | 0.066 |
| Outer diameter of absorber/m | 0.070 |
| Inner diameter of glass envelope/m | 0.109 |
| Outer diameter of glass envelope/m | 0.115 |
| Opening width of collector/m | 5.0 |
| Focal length/m | 0.98 |
| Concentration ratio | 71 |
| Equipment Unit | Sizing Factor X | Purchasing Cost/$ |
|---|---|---|
| Compressor | Shaft power/kW | 9000·X0.6 + 20,000 |
| Turbine | Shaft power/kW | 2000·X0.6 + 40,000 |
| Heat exchanger | Surface/m2 | 900·X0.82 + 10,000 |
| Cooler | Surface/m2 | 450·X0.82 + 5000 |
| Solar receiver | Surface/m2 | 222·X |
| Constant Parameters in the Cycle | Value | Unit |
|---|---|---|
| Turbine inlet pressure | 25 | MPa |
| Turbine inlet temperature | 500–850 | °C |
| Compressor inlet pressure | 7.38 | MPa |
| Compressor inlet temperature | 32 | °C |
| Re-heating pressure | 16.19 | MPa |
| Turbine efficiency | 93 | % |
| Compressor efficiency | 89 | % |
| Recuperator effectiveness | 95 | % |
| Constant Parameters in the Cycle | Value | Unit |
|---|---|---|
| Temperature of environment | 25 | °C |
| Isentropic efficiency of turbine | 85 | % |
| Isentropic efficiency of compressor | 80 | % |
| End temperature difference in regenerator | 10 | °C |
| Direct solar radiation intensity | 1000 | W/m2 |
| Recuperator effectiveness | 95 | % |
| Turbine inlet pressure | 18 | MPa |
| Turbine inlet temperature | 350 | °C |
| Compressor inlet pressure | 8.0 | MPa |
| Compressor inlet temperature | 35 | °C |
| Decision Variables | Range | Unit |
|---|---|---|
| Turbine inlet temperature | 300–400 | °C |
| Turbine inlet pressure | 14–20 | MPa |
| Compressor inlet pressure | 7.5–9.5 | MPa |
| Compressor inlet temperature | 32–45 | °C |
| Variables | Value | Variables | Value |
|---|---|---|---|
| Turbine inlet temperature | 382.76 °C | Cost of core equipment | 183.98k$ |
| Turbine inlet pressure | 17.15 MPa | ||
| Compressor inlet pressure | 8.82 MPa | Specific work | 50.69 kW·kg−1 |
| Compressor inlet temperature | 32.35 °C |
| Variables | Value | Cost of Core Equipment/k$ | Specific Work / kW·kg−1 |
| Turbine inlet temperature | 363.62 °C | 182.33(–0.90%) | 48.36(–4.60%) |
| 401.90 °C | 185.57(+0.86%) | 53.00(4.56%) | |
| Turbine inlet pressure | 16.29 MPa | 179.40(–2.49%) | 47.42(–6.45%) |
| 18.00 MPa | 188.16(+2.27%) | 53.64(+5.82%) | |
| Compressor inlet pressure | 8.38 MPa | 188.54(+2.48%) | 54.19(+6.90%) |
| 9.26 MPa | 179.38(–2.50%) | 47.29(–6.71%) | |
| Isentropic efficiency of turbine | 80.75% | 182.77(–0.65%) | 47.45(–6.39%) |
| 89.25% | 185.17(+0.64%) | 53.93(+6.39%) | |
| Isentropic efficiency of compressor | 76% | 185.30(+0.72%) | 49.95(–1.46%) |
| 84% | 182.71(–0.69%) | 51.37(+1.34%) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, L.; Wang, Y.; Wang, D.; Xie, Y. Parametrized Analysis and Multi-Objective Optimization of Supercritical CO2 (S-CO2) Power Cycles Coupled with Parabolic Trough Collectors. Appl. Sci. 2020, 10, 3123. https://doi.org/10.3390/app10093123
Sun L, Wang Y, Wang D, Xie Y. Parametrized Analysis and Multi-Objective Optimization of Supercritical CO2 (S-CO2) Power Cycles Coupled with Parabolic Trough Collectors. Applied Sciences. 2020; 10(9):3123. https://doi.org/10.3390/app10093123
Chicago/Turabian StyleSun, Lei, Yuqi Wang, Ding Wang, and Yonghui Xie. 2020. "Parametrized Analysis and Multi-Objective Optimization of Supercritical CO2 (S-CO2) Power Cycles Coupled with Parabolic Trough Collectors" Applied Sciences 10, no. 9: 3123. https://doi.org/10.3390/app10093123
APA StyleSun, L., Wang, Y., Wang, D., & Xie, Y. (2020). Parametrized Analysis and Multi-Objective Optimization of Supercritical CO2 (S-CO2) Power Cycles Coupled with Parabolic Trough Collectors. Applied Sciences, 10(9), 3123. https://doi.org/10.3390/app10093123




