Steady-Suction-Based Flow Control of Flutter of Long-Span Bridge
Abstract
Featured Application
Abstract
1. Introduction
2. Experimental Set-Up
2.1. Models
2.2. Wind Tunnel Set-Up and Test Measurements
3. Results and Discussion
3.1. Experiment Validation
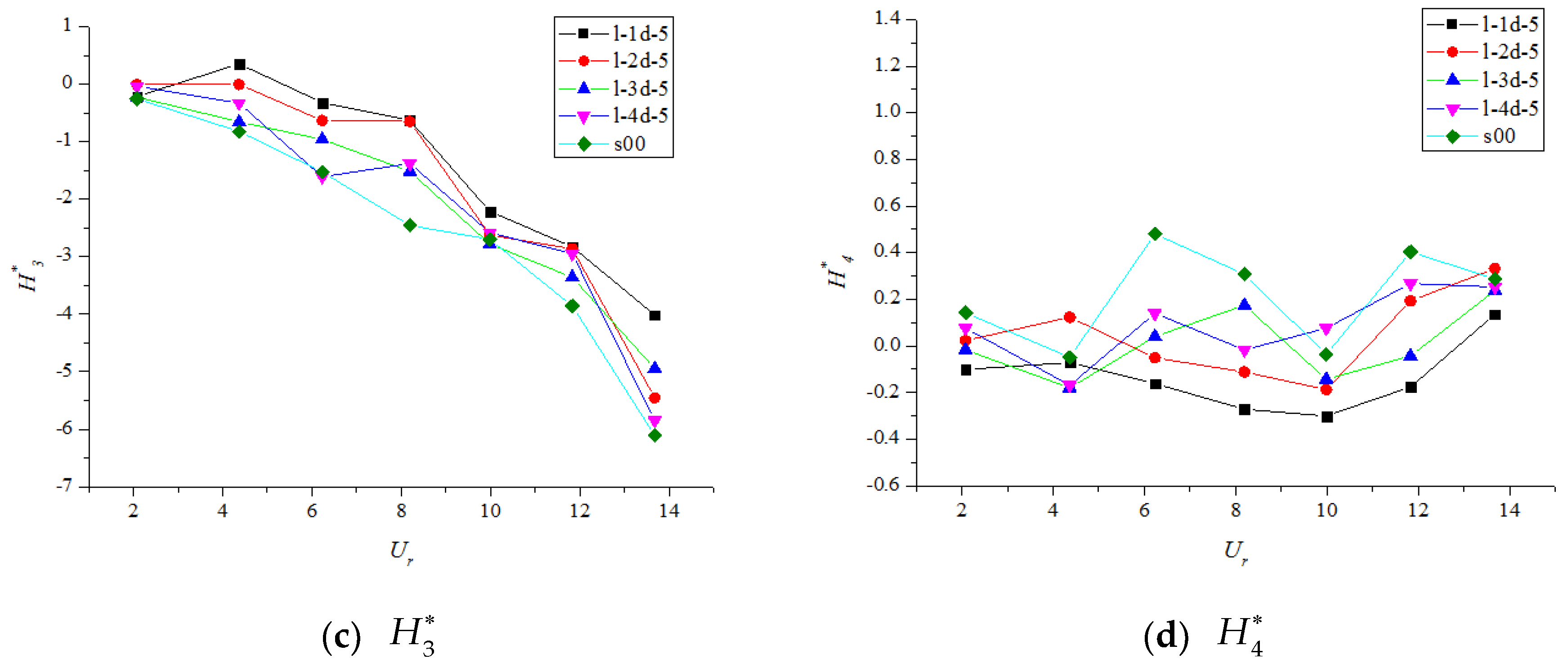
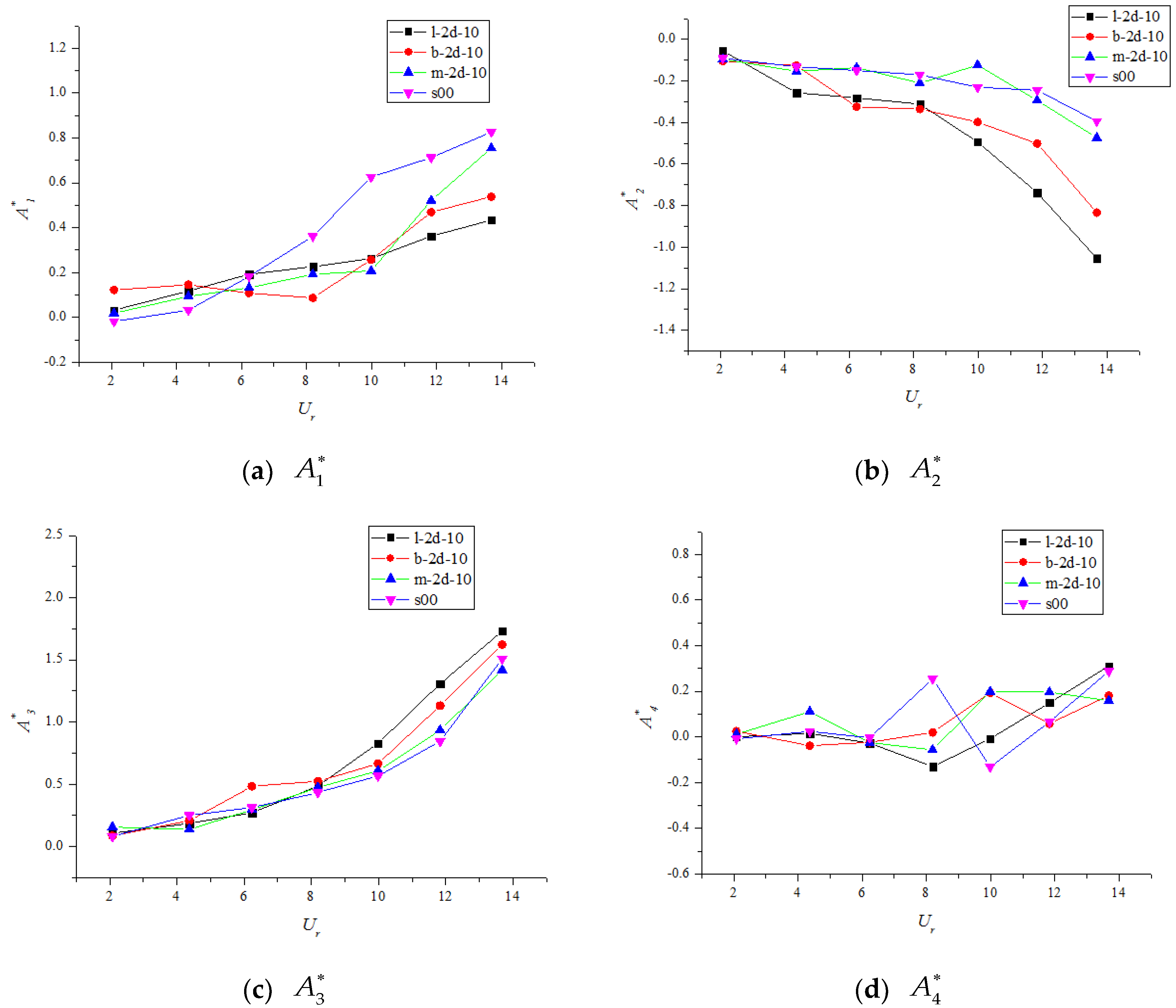
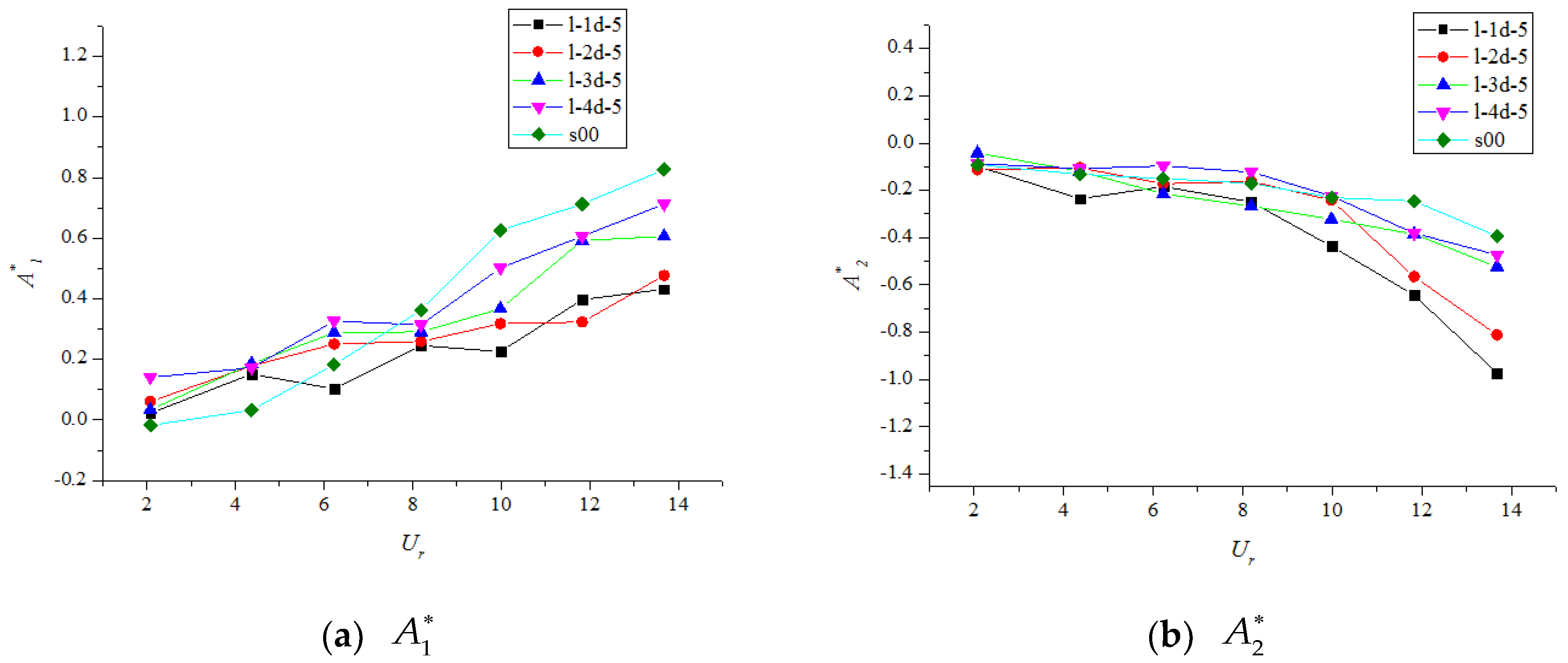
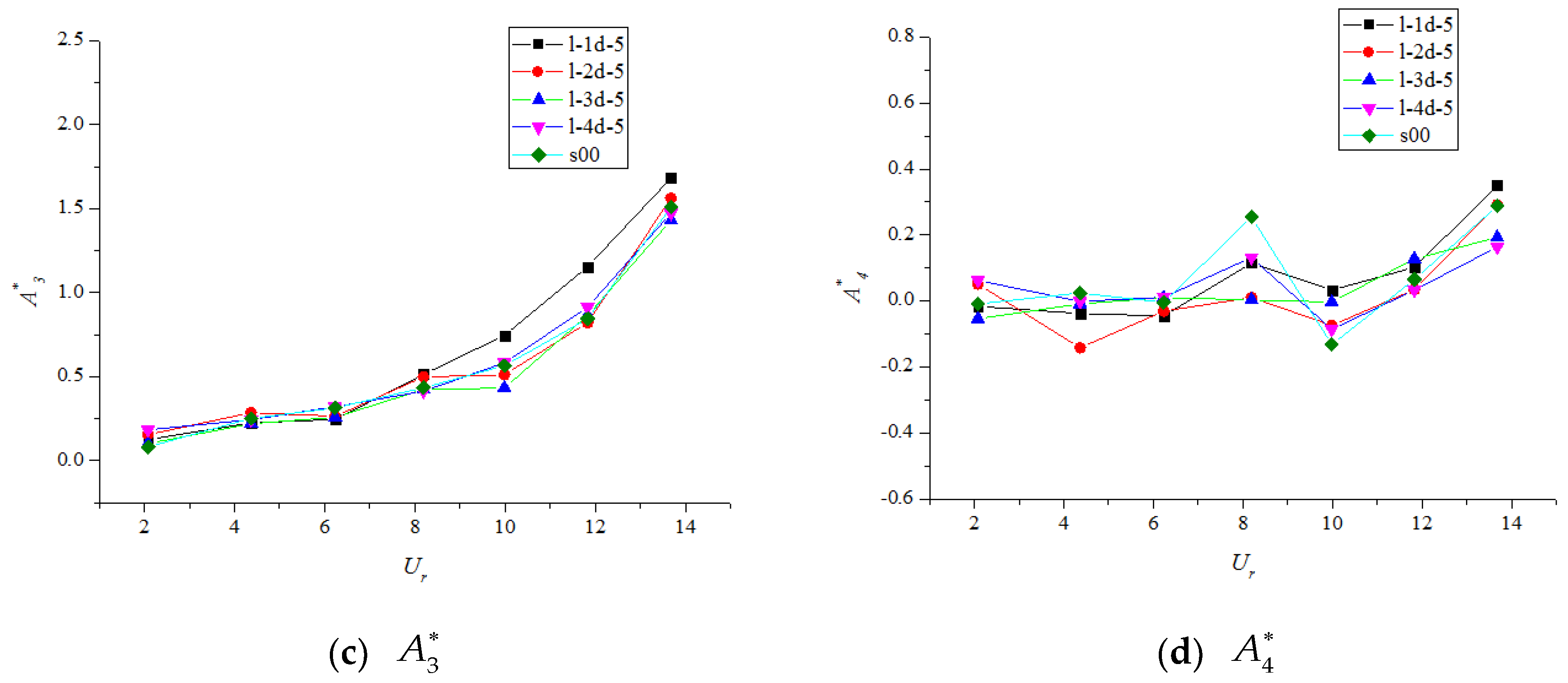
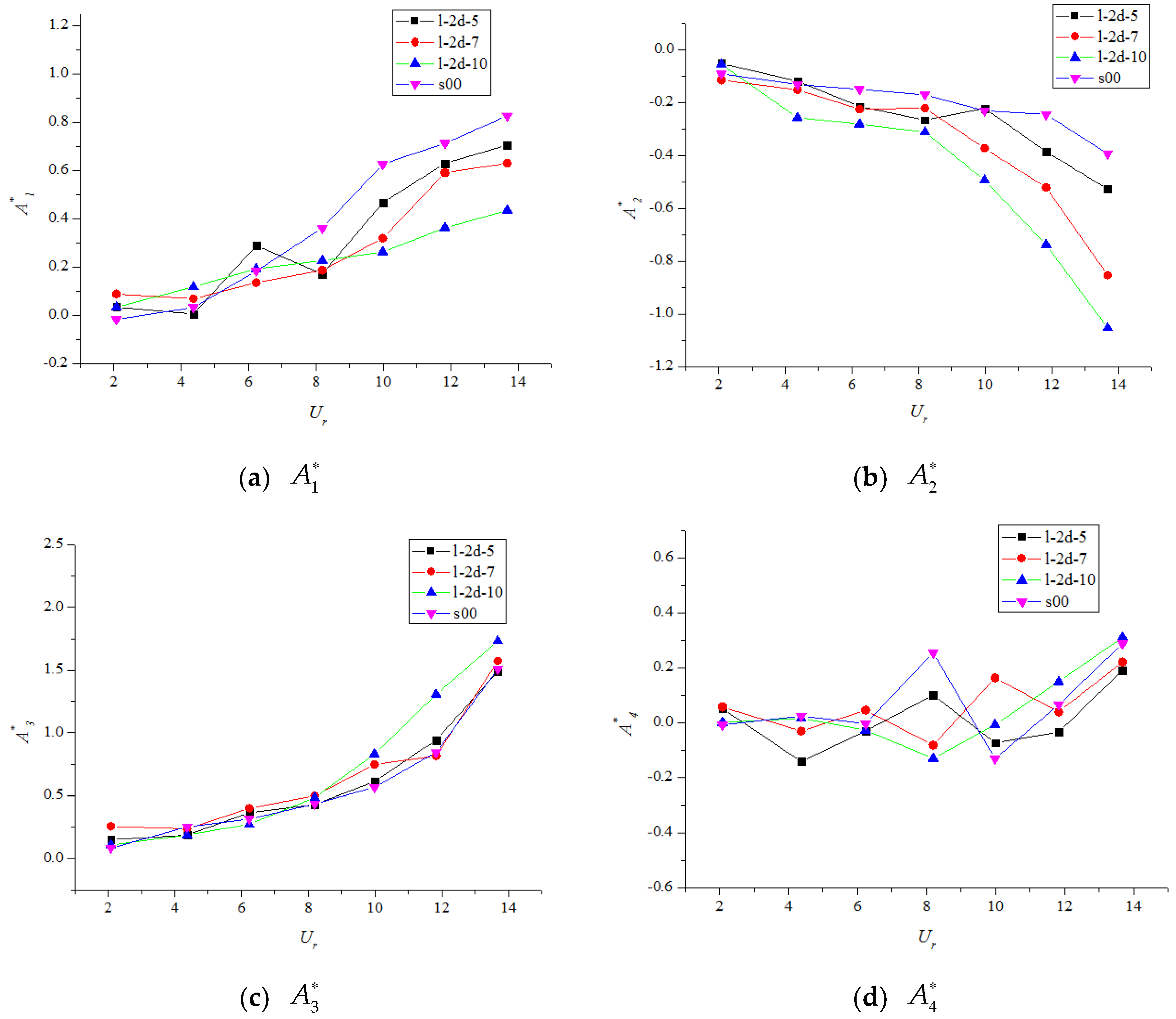
3.2. Effects of Steady-Suction-Based Flow Control on Flutter Derivatives
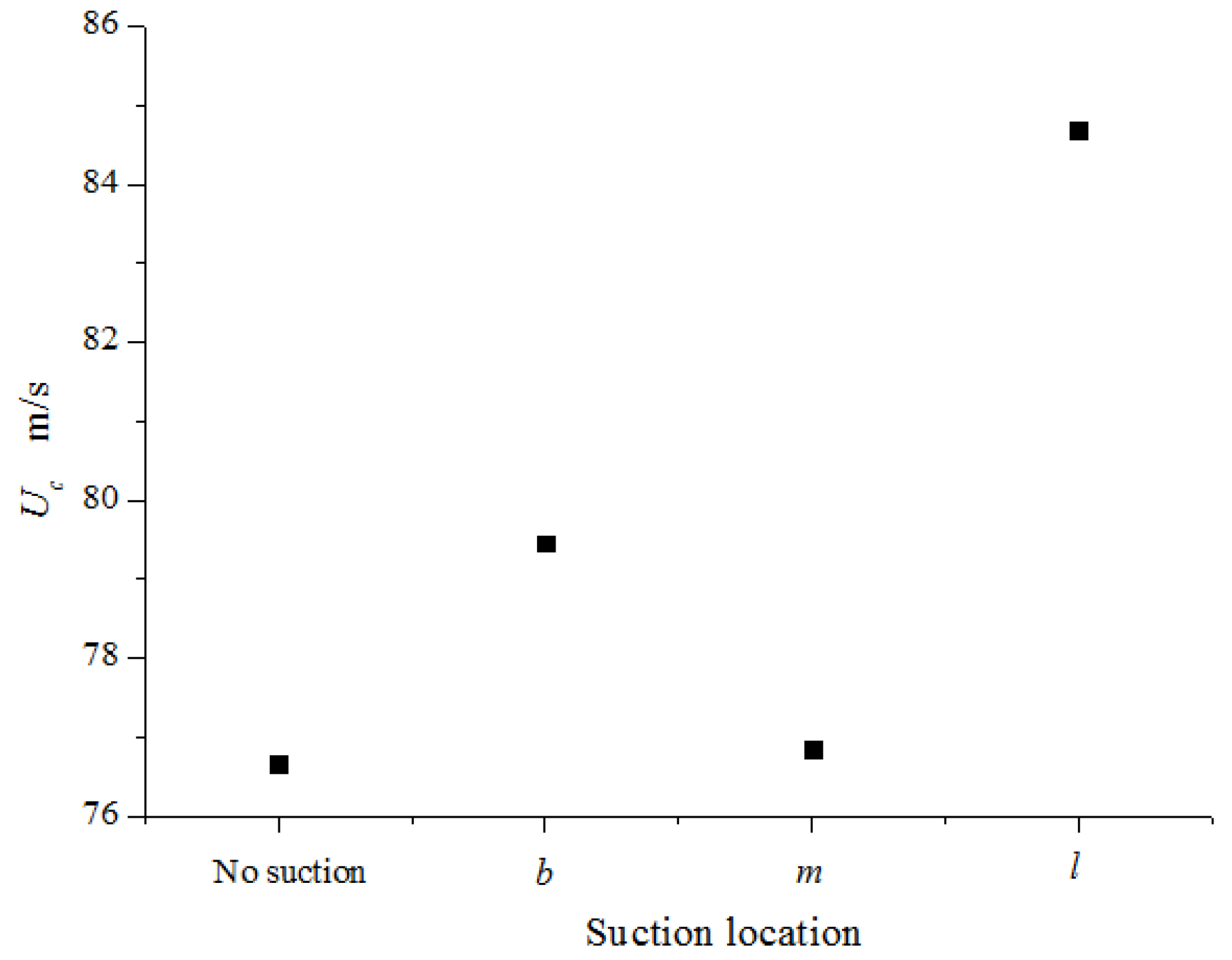
3.3. Influence of Steady-Suction-Based Flow Control on Critical Flutter Speed
3.3.1. Influence of Suction Slot Position
3.3.2. Influence of Suction Interval
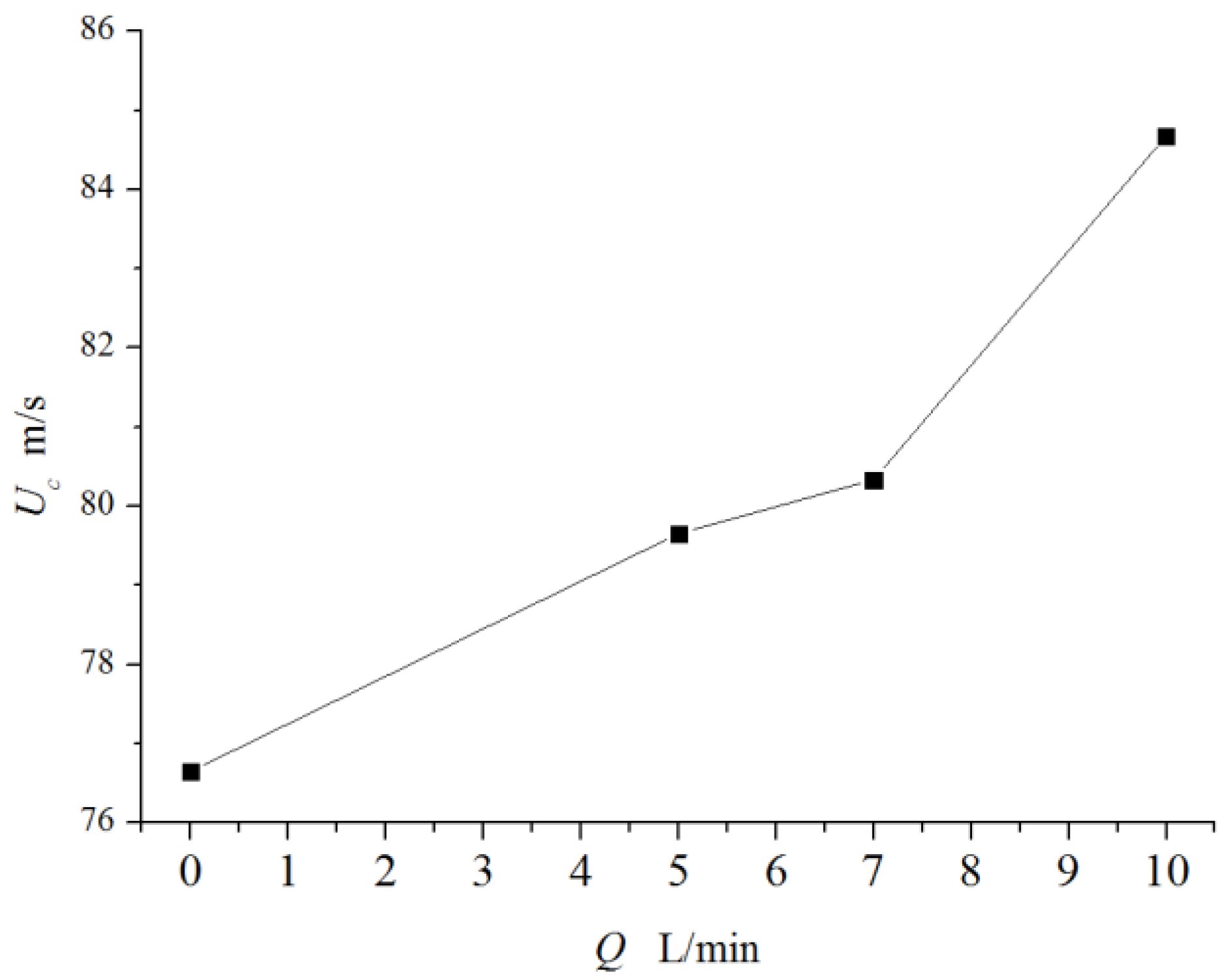
3.3.3. Influence of Suction Flow Rate
4. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nobuto, J.; Fujino, Y.; Ito, M. A study on the effectiveness of TMD to suppress a coupled flutter of bridge deck. Doboku Gakkai Ronbunshu 1988, 398, 413–416. [Google Scholar] [CrossRef][Green Version]
- Pourzeynali, S.; Datta, T. Control of flutter of suspension bridge deck using TMD. Wind Struct. 2002, 5, 407–422. [Google Scholar] [CrossRef]
- Igusa, T.; Xu, K. Wide-Band Response of Multiple Subsystems with High Modal Density; Springer: Berlin, Germany, 1991. [Google Scholar] [CrossRef]
- Kwon, S.D.; Park, K.S. Suppression of bridge flutter using tuned mass dampers based on robust performance design. J. Wind Eng. Ind. Aerodyn. 2004, 92, 919–934. [Google Scholar] [CrossRef]
- Li, C.; Qu, W. Optimum properties of multiple tuned mass dampers for reduction of translational and torsional response of structures subject to ground acceleration. Eng. Struct. 2006, 28, 472–494. [Google Scholar] [CrossRef]
- Guo, Y.; Chen, W. Dynamic analysis of space structures with multiple tuned mass dampers. Eng. Struct. 2007, 29, 3390–3403. [Google Scholar] [CrossRef]
- Körlin, R.; Starossek, U. Wind tunnel test of an active mass damper for bridge decks. J. Wind Eng. Ind. Aerody. 2007, 95, 267–277. [Google Scholar] [CrossRef]
- Ge, Y.; Xiang, H. Aerodynamic stabilization for box-girder suspension bridges with super-long span. In Proceedings of the 5th European and African Conference Wind Engineering, Florence, Italy, 19–23 July 2009. [Google Scholar]
- Munshi, S.; Modi, V.; Yokomizo, T. Aerodynamics and dynamics of rectangular prisms with momentum injection. J. Fluids Struct. 1997, 11, 873–892. [Google Scholar] [CrossRef]
- Kubo, Y.; Yukoku, E.; Modi, V.J.; Yamaguchi, E.; Kato, K.; Kawamura, S.-I. Control of flow separation from leading edge of a shallow rectangular cylinder through momentum injection. J. Wind Eng. Ind. Aerody. 1999, 83, 503–514. [Google Scholar] [CrossRef]
- Farajpour, A.; Farokhi, H.; Ghayesh, M.H.; Hussain, S. Nonlinear mechanics of nanotubes conveying fluid. Int. J. Eng. Sci. 2018, 133, 132–143. [Google Scholar] [CrossRef]
- Ghayesh, M.H.; Farokhi, H.; Farajpour, A. Global dynamics of fluid conveying nanotubes. Int. J. Eng. Sci. 2019, 135, 37–57. [Google Scholar] [CrossRef]
- Farajpour, M.R.; Shahidi, A.R.; Farajpour, A. Elastic waves in fluid-conveying carbon nanotubes under magneto-hygro-mechanical loads via a two-phase local/nonlocal mixture model. Mater. Res. Express 2019, 6. [Google Scholar] [CrossRef]
- Chen, W.; Xin, D.; Xu, F.; Li, H.; Ou, J.; Hu, H. Suppression of vortex-induced vibration of a circular cylinder using suction-based flow control. J. Fluids Struct. 2013, 42, 25–39. [Google Scholar] [CrossRef]
- Xin, D.; Ou, J. Numerical study on improving wind-induced static characteristics of bridge section by steady air-suction. J. Shenyang Jianzhu Univ. 2008, 24, 1–5. (In Chinese) [Google Scholar]
- Xin, D.; Ou, J.; Li, H.; Li, Z. Suppression method for wind-induced flutter of long-span bridge based on steady air-suction. J. Jilin Univ. (Eng. Technol. Ed.) 2011, 41, 1273–1278. (In Chinese) [Google Scholar]
- Scanlan, R.H.; Tomo, J. Air foil and bridge deck flutter derivatives. J. Soil Mech. Found. Div. 1971, 97, 1717–1737. [Google Scholar]
- Sarkar, P.P.; Jones, N.P.; Scanlan, R.H. System identification for estimation of flutter derivatives. J. Wind Eng. Ind. Aerody. 1992, 42, 1243–1254. [Google Scholar] [CrossRef]
- Singh, L.; Jones, N.; Scanlan, R.; Lorendeaux, O. Identification of lateral flutter derivatives of bridge decks. J. Wind Eng. Ind. Aerody. 1996, 60, 81–89. [Google Scholar] [CrossRef]
- Chowdhury, A.G.; Sarkar, P.P. A new technique for identification of eighteen flutter derivatives using a three-degree-of-freedom section model. Eng. Struct. 2003, 25, 1763–1772. [Google Scholar] [CrossRef]
- Poulsen, N.K.; Damsgaard, A.; Reinhold, T.A. Determination of flutter derivatives for the Great Belt Bridge. J. Wind Eng. Ind. Aerody. 1992, 41, 153–164. [Google Scholar] [CrossRef]
- Xiang, H. Modern Theory and Practice on Bridge Wind Resistance; China Communications Press: Beijing, China, 2005; pp. 307–312. [Google Scholar]
- Matsumoto, M.; Daito, Y.; Yoshizumi, F.; Ichikawa, Y.; Yabutani, T. Torsional flutter of bluff bodies. J. Wind Eng. Ind. Aerody. 1997, 69, 871–882. [Google Scholar] [CrossRef]
- Gu, M.; Zhang, R.; Xiang, H. Identification of flutter derivatives of bridge decks. J. Wind Eng. Ind. Aerody. 2000, 84, 151–162. [Google Scholar] [CrossRef]
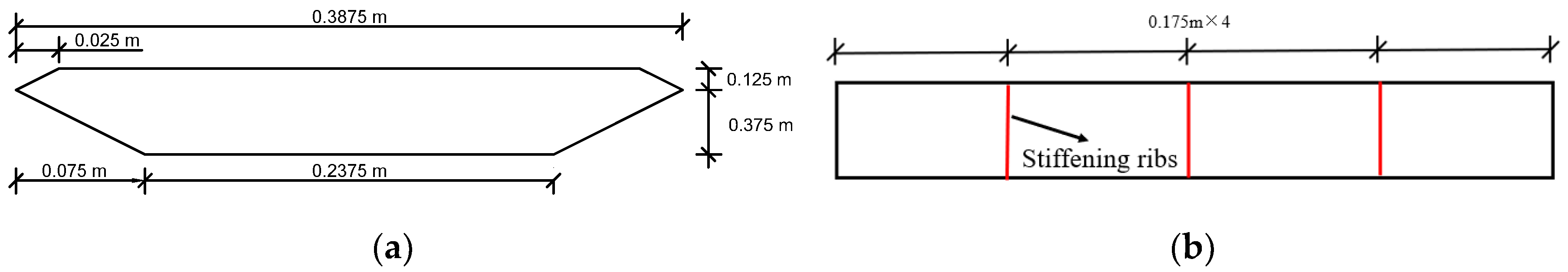
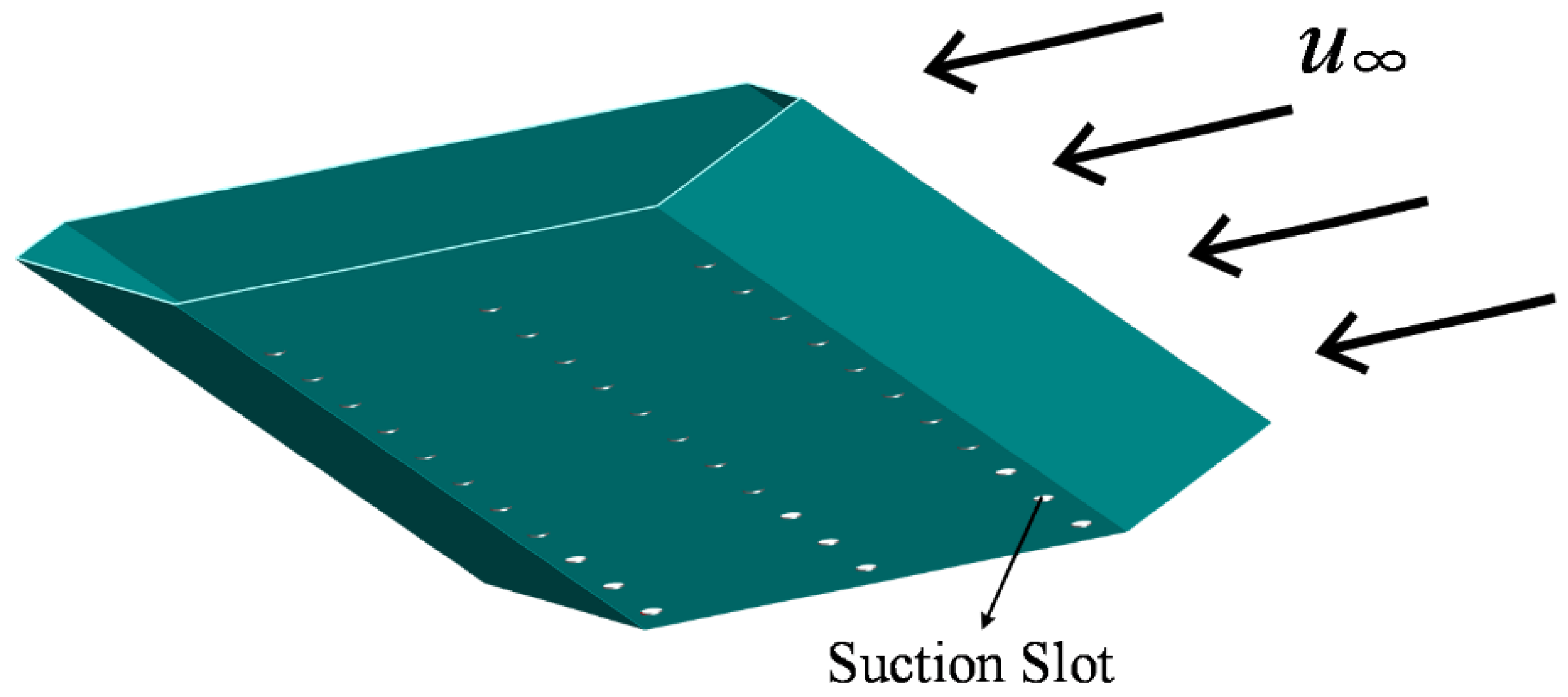







| Case | Wind Velocity (m/s) | Position | Interval | Flow Rate |
|---|---|---|---|---|
| s00 | 2, 4, 6, 8, 10, 12, 14 | - | - | - |
| l-2d-10 | 2, 4, 6, 8, 10, 12, 14 | windward | 2H | 10 L/min |
| m-2d-10 | 2, 4, 6, 8, 10, 12, 14 | middle | 2H | 10 L/min |
| b-2d-10 | 2, 4, 6, 8, 10, 12, 14 | leeward | 2H | 10 L/min |
| l-1d-5 | 2, 4, 6, 8, 10, 12, 14 | windward | 1H | 5 L/min |
| l-2d-5 | 2, 4, 6, 8, 10, 12, 14 | windward | 2H | 5 L/min |
| l-3d-5 | 2, 4, 6, 8, 10, 12, 14 | windward | 3H | 5 L/min |
| l-4d-5 | 2, 4, 6, 8, 10, 12, 14 | windward | 4H | 5 L/min |
| l-2d-7 | 2, 4, 6, 8, 10, 12, 14 | windward | 2H | 7 L/min |
| Property | Unit | Real Bridge | Similarity Ratio | Section Model |
|---|---|---|---|---|
| Length/L | m | 1624 | 0.7 | |
| Breadth/B | m | 31 | 1/80 | 0.3875 |
| Height/H | m | 4 | 1/80 | 0.05 |
| Equivalent mass | kg/m | 23,687 | 1/802 | 3.701 |
| Equivalent mass moment of inertia | kg·m2/m | 2.501 × 105 | 1/804 | 0.061 |
| Frequency of heaving mode | Hz | 0.097 | 26.66 | 2.586 |
| Frequency of pitching mode | Hz | 0.27 | 25.19 | 6.8 |
| Frequency ratio | 2.78 | 0.95/1 | 2.63 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhan, J.; Zhang, H.; Liu, Z.; Liu, H.; Xin, D.; Ou, J. Steady-Suction-Based Flow Control of Flutter of Long-Span Bridge. Appl. Sci. 2020, 10, 1372. https://doi.org/10.3390/app10041372
Zhan J, Zhang H, Liu Z, Liu H, Xin D, Ou J. Steady-Suction-Based Flow Control of Flutter of Long-Span Bridge. Applied Sciences. 2020; 10(4):1372. https://doi.org/10.3390/app10041372
Chicago/Turabian StyleZhan, Jian, Hongfu Zhang, Zhiwen Liu, Huan Liu, Dabo Xin, and Jinping Ou. 2020. "Steady-Suction-Based Flow Control of Flutter of Long-Span Bridge" Applied Sciences 10, no. 4: 1372. https://doi.org/10.3390/app10041372
APA StyleZhan, J., Zhang, H., Liu, Z., Liu, H., Xin, D., & Ou, J. (2020). Steady-Suction-Based Flow Control of Flutter of Long-Span Bridge. Applied Sciences, 10(4), 1372. https://doi.org/10.3390/app10041372




