Influence of Phase Change Phenomena on the Performance of a Desiccant Dehumidification System
Abstract
:1. Introduction
2. Materials and Methods
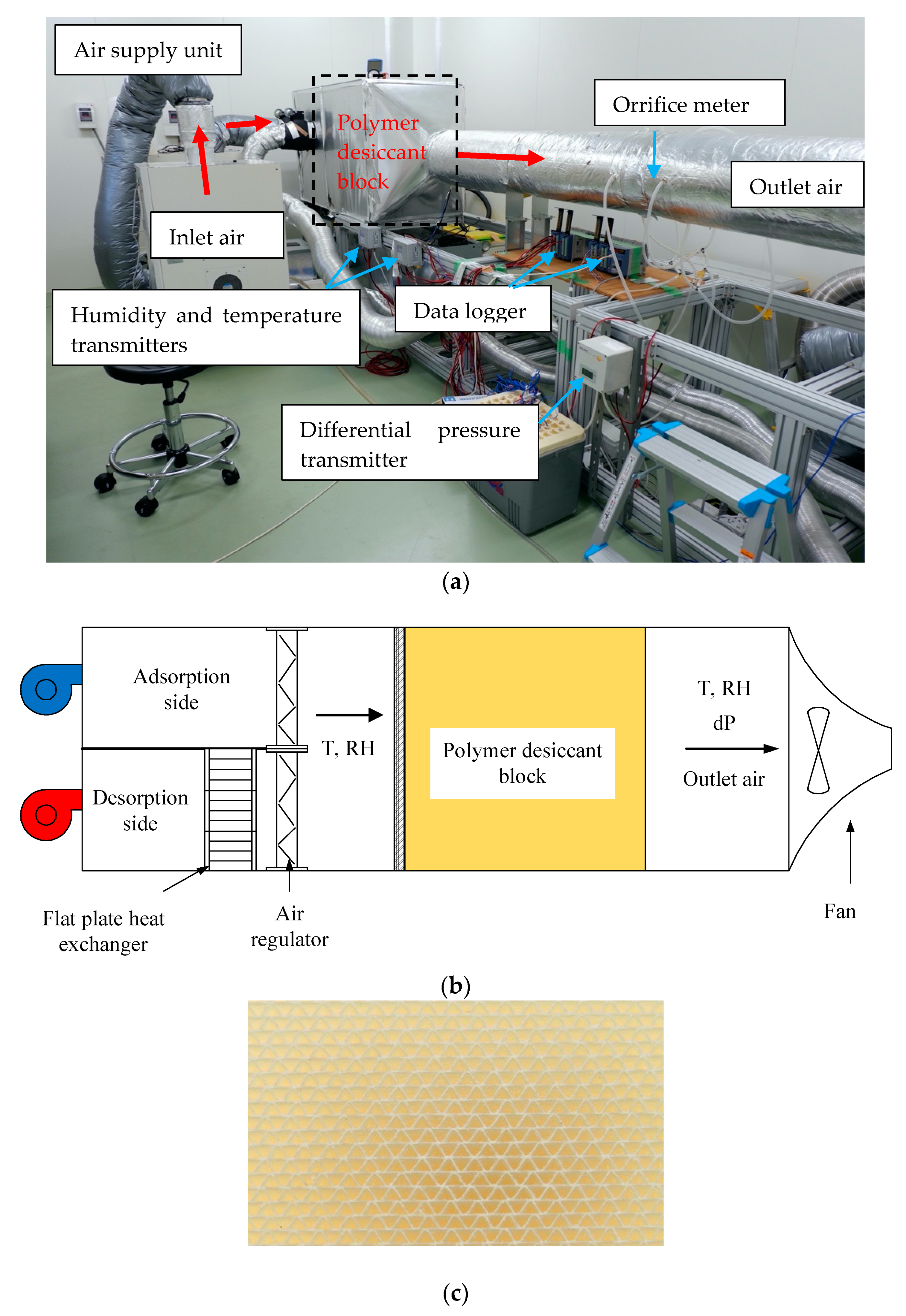
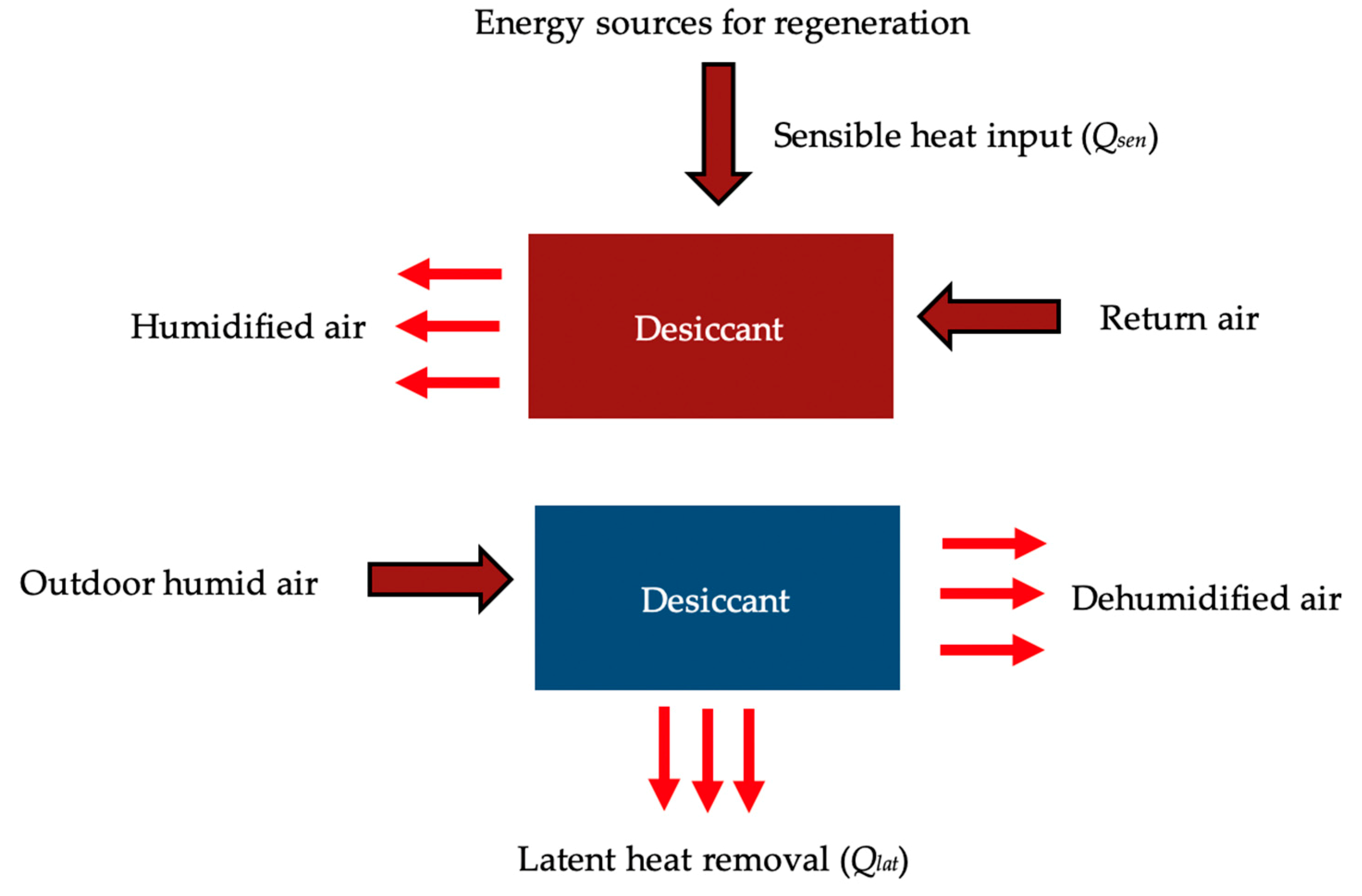
2.1. Experimental Section
- (1)
- Firstly, the desiccant material was heated by the hot air, which was provided by the air supply unit for the desorption phase. This process allowed the water vapor molecules, which were trapped in the desiccant material, to evaporate. Therefore, the desiccant material was regenerated and recovered the ability of moisture adsorption.
- (2)
- When the desiccant material reached the equilibrium, the process was switched into the adsorption process by using the air regulator. The desiccant material adsorbed the water vapor molecules. At the same time, in the interface of the desiccant material, the heat of adsorption was released.
- (3)
- Lastly, the process was repeated for three up to four cycles to obtain adequate data.
2.2. Data Reduction Equations
2.2.1. Dehumidification Index
2.2.2. The Effect of Latent Heat on the Performance
2.2.3. Uncertainty Analysis
3. Results and Discussion
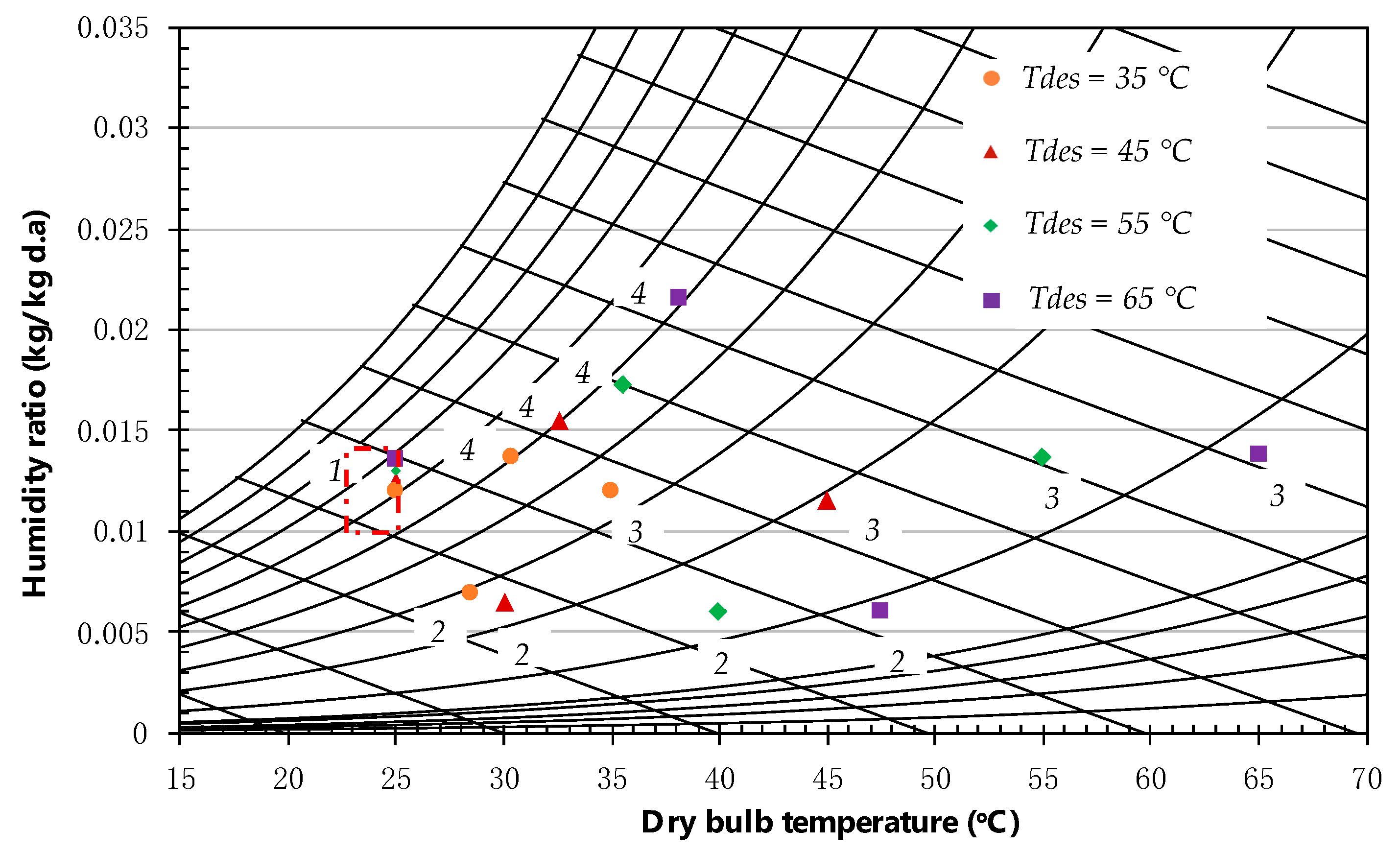
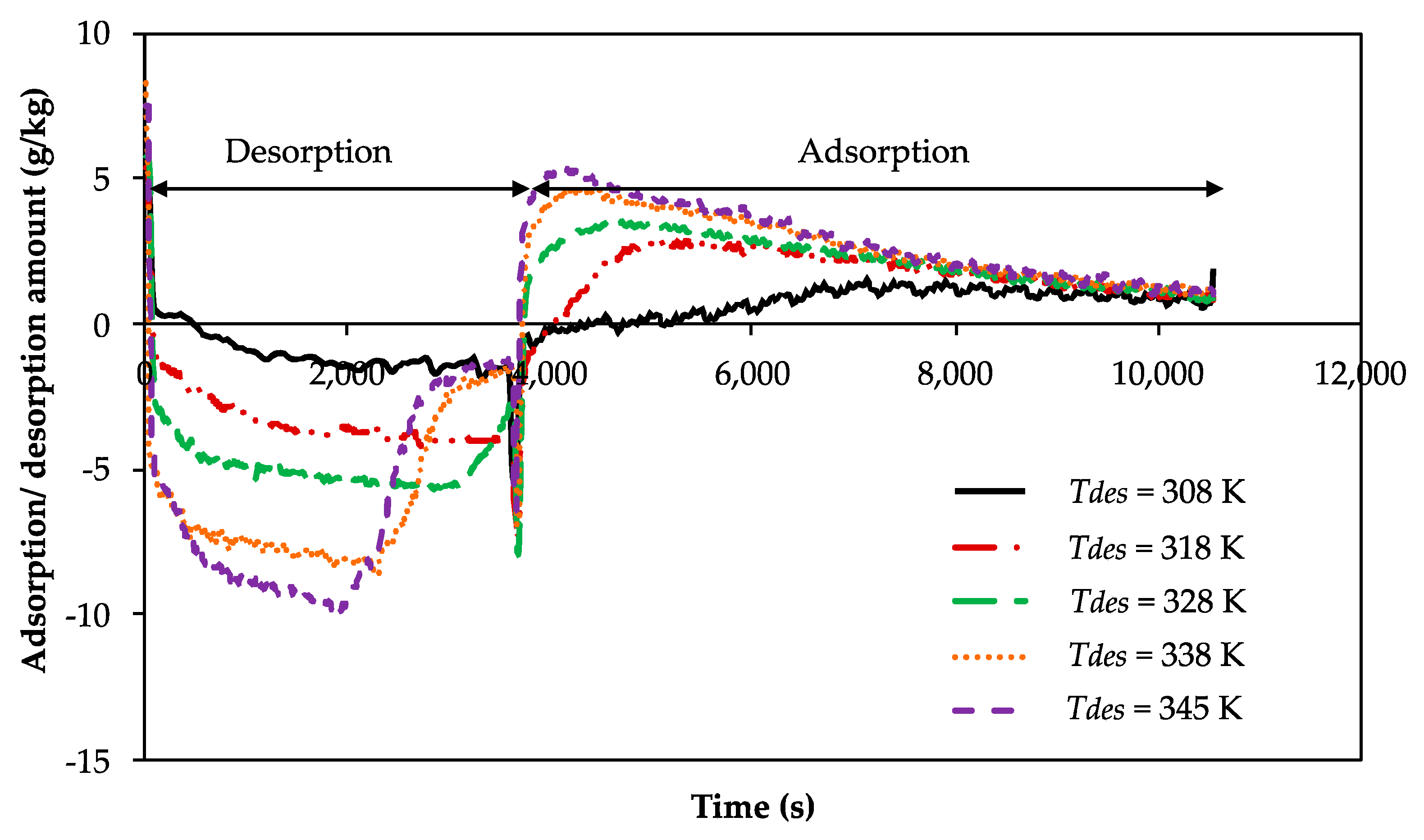
3.1. Influence of Desorption Temperature on the Water Content Removal
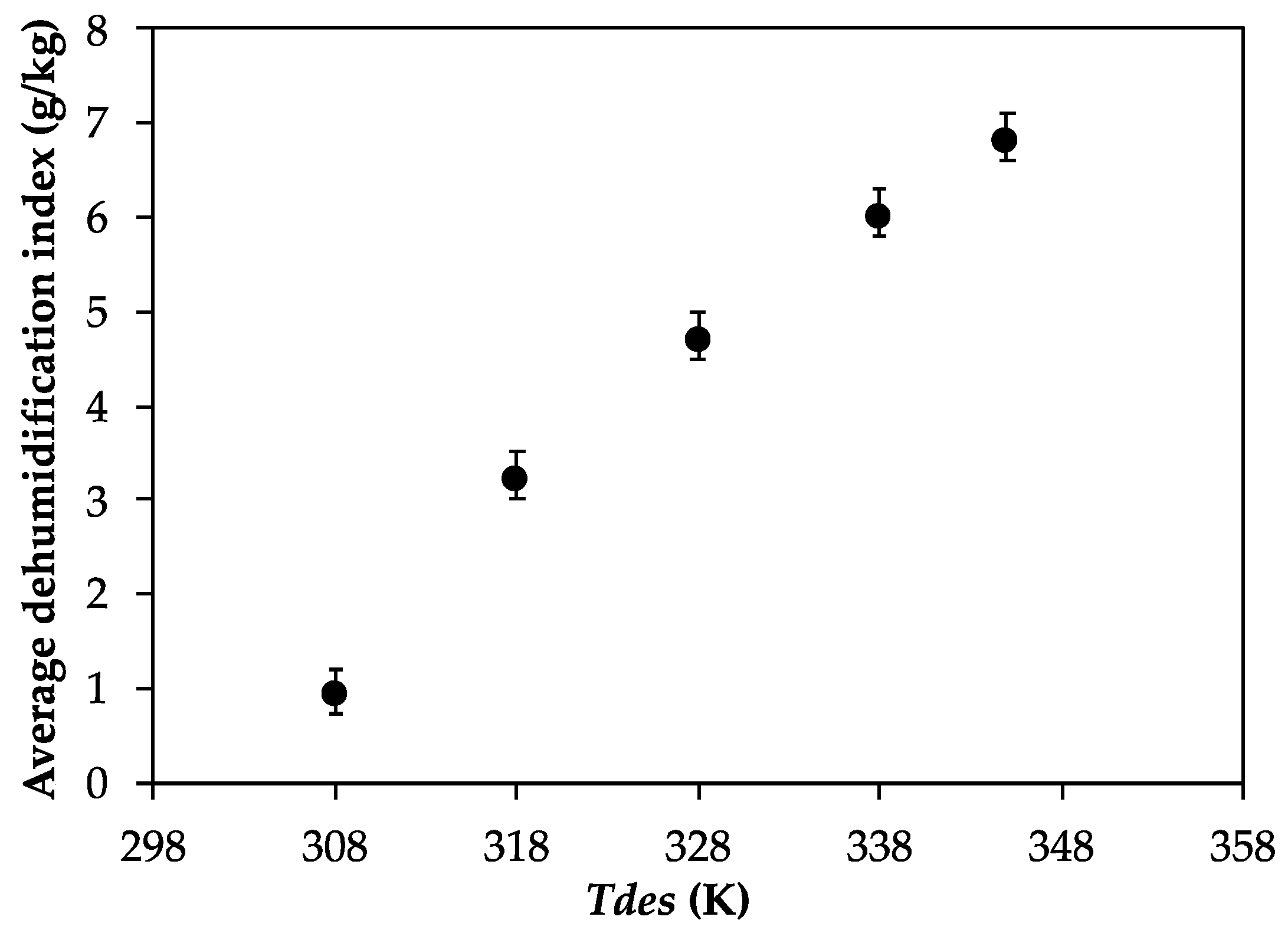
3.2. Influence of Desorption Temperature on Dehumidification Index
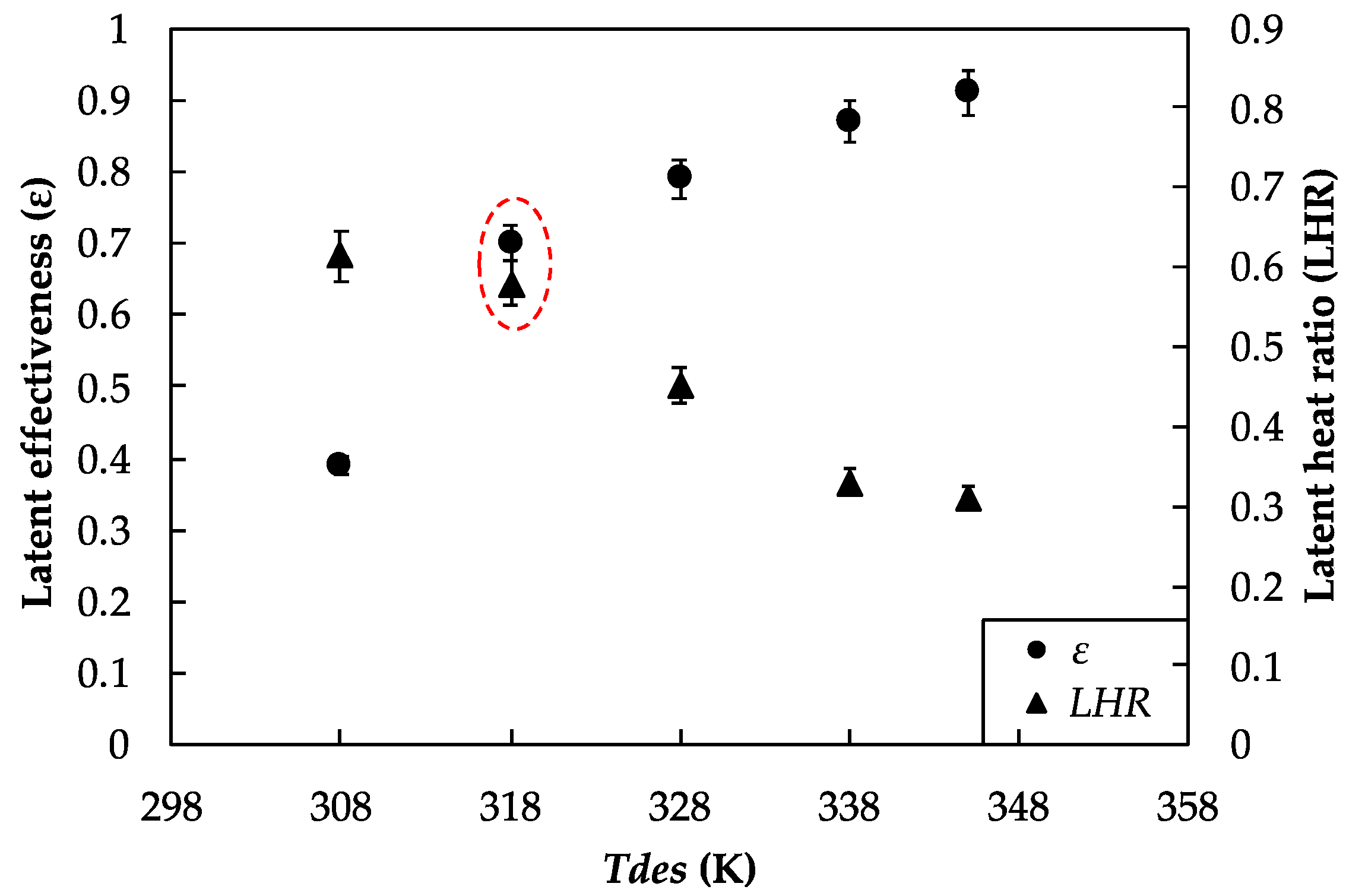
3.3. The Effect of Latent Heat on the Performance of the System
3.4. Energy Analysis
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | adsorption potential (kJ/kg) |
| cp | specific heat capacity (J/kg·K) |
| cp,b | specific heat capacity of the bed (kJ/kg·K) |
| d | moisture removal capacity (kg/kg) |
| Dh | hydraulic diameter of the air channel (m) |
| Di | dehumidification index (kg/kg) |
| E | adsorption characteristics parameter (kJ/kg) |
| Et | total energy demand (W) |
| h | channel height (air layer) |
| H | desiccant block height (m) |
| kd | thermal conductivity of sorbent (W/m·K) |
| L | desiccant block length (m) |
| LHR | latent heat ratio |
| n | fitting constant |
| p | fin spacing (m) |
| P | partial pressure (kPa) |
| Po | saturation pressure (kPa) |
| Qads | average of enthalpy exchange in adsorption process (W) |
| Qdes | average of heat exchange in the desorption process (W) |
| Qlat | latent heat transfer (W) |
| Qsen | sensible heat transfer (W) |
| r | radius of the adsorption particle (m) |
| R | specific gas constant for water (kJ/kg·K) |
| t | dehumidification time (s) |
| T | temperature (K) |
| Re | Reynolds number |
| RH | relative humidity (%) |
| Ua | air velocity (m/s) |
| w | desiccant block width (m) |
| , | volume flow rate (m3/s) |
| W | equilibrium adsorption uptake (kg/kg) |
| Wfan | electricity for fan (W) |
| Wo | maximum adsorption capacity (kg/kg) |
| X | humidity ratio (kg/kg) |
| Xideal | ideal humidity ratio of air stream at the outlet of dehumidification system (kg/kg) |
| ∆H | heat of adsorption (kJ/kg) |
| Greek symbol | |
| ε | latent heat effectiveness |
| εfan | efficiency of the fan (%) |
| δ | sorbent thickness (m) |
| γ | latent heat of vaporization (kJ/s) |
| μ | dynamic viscosity of the air (kg/m.s) |
| ρa | air density (kg/m3) |
| ρb | bed density (kg/m3) |
| ϕ | porosity |
| Subscript | |
| ads | adsorption |
| des | desorption |
| in | inlet |
| out | outlet |
References
- Al-Homoud, M.S. Optimum Thermal Design of Air-Conditioned Residential Buildings. Build Environ. 1997, 32, 203–210. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Ge, T.S.; Dai, Y.J.; Zhao, Y.; Wang, R.Z. Experimental Investigation on Solar Powered Desiccant Coated Heat Exchanger Humidification Air Conditioning System in Winter. Energy J. 2017, 137, 468–478. [Google Scholar] [CrossRef]
- Zhou, Y.P.; Wu, J.Y.; Wang, R.Z.; Shiochi, S. Energy Simulation in the Variable Refrigerant Flow Air-conditioning System under Cooling Conditions. Energ. Build. 2007, 39, 212–220. [Google Scholar] [CrossRef]
- Mohhamadi, S.M.H.; Ameri, M. Energy and Exergy Comparison of a Cascade Air Conditioning System using Different Cooling Strategies. Int. J. Refrig. 2014, 41, 14–26. [Google Scholar] [CrossRef]
- Fermanel, F.; Miriel, J. Air Heating System: Influence of a Humidifier on Thermal Comfort. Appl. Therm. Eng. 1999, 19, 1107–1127. [Google Scholar] [CrossRef]
- Antonellis, S.D.; Intini, M.; Joppolo, C.M.; Molinaroli, L.; Romano, F. Desiccant wheels for air humidification: An Experimental and Numerical Analysis. Energy Convers. Manag. 2015, 106, 355–364. [Google Scholar] [CrossRef]
- Kaseminejad, H. Analysis of One-dimensional Fin Assembly Heat Transfer with Dehumidification. Int. J. Heat Mass Transf. 1995, 38, 445–462. [Google Scholar] [CrossRef]
- Su, W.; Zhang, X. Thermodynamics Analysis of a Compression-absorption Refrigeration Air-conditioning System Coupled with Liquid Desiccant Dehumidification. Appl. Therm. Eng. 2017, 115, 575–585. [Google Scholar] [CrossRef]
- Yaningsih, I.; Wijayanta, A.T.; Miyazaki, T.; Koyama, S. Analysis of heat and mass transfer characteristics of desiccant dehumidifier system with honeycomb configuration. Appl. Therm. Eng. 2018, 144, 658–669. [Google Scholar] [CrossRef]
- Zhang, L.Z. Progress on Heat and Moisture Recovery with Membranes: From Fundamentals to Engineering Applications. Energy Convers. Manag. 2012, 63, 173–195. [Google Scholar]
- Miyazaki, T.; Akisawa, A.; Nikai, I. The Cooling Performance of a Building Integrated Evaporative Cooling System Driven by Solar Energy. Energ. Build. 2011, 43, 2211–2218. [Google Scholar] [CrossRef]
- Wrobel, J.; Walter, P.S.; Schmitz, G. Performance of a Solar Assisted Air Conditioning System at Different Locations. Sol. Energy 2013, 92, 69–83. [Google Scholar] [CrossRef]
- Benhammou, M.; Draoui, B.; Zerrouki, M.; Marif, Y. Performance Analysis of an Earth-to-air exchanger Assisted by a Wind Tower for Passive Cooling of Buildings in Arid and Hot Climate. Energy Convers. Manag. 2015, 91, 1–11. [Google Scholar] [CrossRef]
- Daher, N.A.; Raoofat, M.; Saad, M.; Mougharbel, I.; Asber, D.; Beltran-Galindo, J. Improve the HVAC Contribution in Wind Power Smoothing. Electr. Pow. Syst. Res. 2017, 171, 219–229. [Google Scholar] [CrossRef]
- Garimella, S.; Brown, A.M.; Nagavapura, A.K. Waste Heat Driven Absorption/vapor-compression Cascade Refrigeration System for Megawatt Scale, High-flux, Low-temperature Cooling. Int. J. Refrig. 2011, 34, 1776–1785. [Google Scholar] [CrossRef]
- Ali, S.M.; Chakraborty, A. Thermodynamic Modelling and Performance Study of an Engine Waste Heat Driven Adsorption Cooling for Automotive Air-conditioning. Appl. Therm. Eng. 2015, 90, 54–63. [Google Scholar] [CrossRef]
- Ling, J.; Kuwabara, O.; Hwang, Y.; Radermacher, R. Enhancement Options for Separate Sensible and Latent Cooling Air-conditioning Systems. Int. J. Refrig. 2013, 36, 45–57. [Google Scholar] [CrossRef]
- Tso, C.Y.; Chao, C.Y.H. Activated Carbon, Silica-gel, Calcium Chloride Composite Adsorbents for Energy Efficient Solar Adsorption Cooling and Dehumidification Systems. Int. J. Refrig. 2012, 35, 1626–1638. [Google Scholar] [CrossRef]
- Sultan, M.; Miyazaki, T.; Koyama, S. Optimization of Adsorption Isotherm Types for Desiccant Air-Conditioning Applications. Renew. Energ. 2018, 121, 441–450. [Google Scholar] [CrossRef]
- Aristov, Y.I. Challenging offers of Material Science for Adsorption Heat Transformation: A Review. Appl. Therm. Eng. 2013, 50, 1610–1618. [Google Scholar] [CrossRef]
- Enteria, N.; Yoshino, H.; Takaki, H.; Mochida, A.; Satake, A.; Yoshie, R. Effect of the Regeneration Temperature in the Exergetic Performances of the Develop Desiccant-Evaporative Air-Conditioning System. Int. J. Refrig. 2013, 36, 2323–2342. [Google Scholar] [CrossRef]
- Chen, Y.; Yin, Y.; Zhang, X. Performance Analysis of a Hybrid Air-Conditioning System Dehumidified by Liquid Desiccant with Low Temperature and Low Concentration. Energ. Build. 2014, 77, 91–102. [Google Scholar] [CrossRef]
- Jia, C.X.; Dai, Y.J.; Wu, J.Y.; Wang, R.Z. Experimental Comparison of Two Honeycombed Desiccant Wheels Fabricated with Silica Gel and Composite Desiccant Material. Energy Convers. Manag. 2006, 47, 2523–2534. [Google Scholar] [CrossRef]
- Angrisani, G.; Roselli, C.; Sasso, M. Experimental Assessment of The Energy Performance of a Hybrid Desiccant Cooling System and Comparison with other Air-conditioning Technologies. Appl. Energy 2015, 138, 533–545. [Google Scholar] [CrossRef]
- Lu, S.M.; Shyu, R.J.; Yan, W.J.; Chung, T.W. Development and Experimental Validation of Two Novel Solar Desiccant—Dehumidification-Regeneration Systems. Energy J. 1995, 20, 751–757. [Google Scholar] [CrossRef]
- Oliveira, A.C.; Afonso, C.F.; Riffat, S.B.; Doherty, P.S. Thermal Performance of a Novel Air Conditioning System using a Liquid Desiccant. Appl. Therm. Eng. 2000, 20, 1213–1223. [Google Scholar] [CrossRef]
- Kanoğlu, M.; Bolattürk, A.; Altuntop, N. Effect of Ambient Conditions on the First and Second Law Performance of an Open Desiccant Cooling Process. Renew. Energy 2007, 32, 931–946. [Google Scholar] [CrossRef]
- Jeong, J.; Yamaguchi, S.; Saito, K.; Kawai, S. Performance Analysis of Desiccant Dehumidification Systems Driven by Low-grade Heat Source. Int. J. Refrig. 2011, 34, 928–945. [Google Scholar] [CrossRef]
- Panaras, G.; Mathioulakis, E.; Belessiotis, V. Solid Desiccant Air-conditioning Systems-Design Parameters. Energy J. 2011, 36, 2399–2406. [Google Scholar] [CrossRef]
- Ge, T.S.; Dai, Y.J.; Wang, R.Z.; Li, Y. Performance of Two-stage Rotary Desiccant Cooling System with Different Regeneration Temperatures. Energy J. 2015, 80, 556–566. [Google Scholar] [CrossRef]
- Lee, H.; Lin, X.; Hwang, Y.; Radermacher, R. Performance Investigation on Solid Desiccant Assisted Mobile Air Conditioning System. Appl. Therm. Eng. 2016, 103, 1370–1380. [Google Scholar] [CrossRef]
- Lock, G.S.H. Latent Heat Transfer: An Introduction to Fundamentals; Oxford University Press: New York, NY, USA, 1994. [Google Scholar]
- Chung, J.D.; Lee, D.; Yoon, S.M. Optimization of Desiccant Wheel Speed and Area Ratio of Regeneration to Dehumidification as a Function of Regeneration Temperature. Sol. Energy 2009, 83, 625–635. [Google Scholar] [CrossRef]
- Datta, N.; Chakraborty, A.; Ali, S.M.; Choo, F.H. Experimental Investigation od Multi-effect Regenerator for Desiccant Dehumidifier: Effects of Various Regeneration Temperatures and Solution Flow Rates on System Performances. Int. J. Refrig. 2017, 76, 7–18. [Google Scholar] [CrossRef]
- Ruivo, C.R.; Goldsworthy, M.; Intini, M. Interpolation Methods to Predict the Influence of Inlet Airflow States on Desiccant Wheel Performance at Low Regeneration Temperature. Energy J. 2014, 68, 765–772. [Google Scholar] [CrossRef]
- Mandegari, M.A.; Pahlavanzadeh, H. Introduction of a New Definition for Effectiveness of Desiccant Wheels. Energy J. 2009, 34, 797–803. [Google Scholar] [CrossRef]
- Islam, M.R.; Alan, S.W.L.; Chua, K.J. Studying the Heat and Mass Transfer Process of Liquid Desiccant for Dehumidification and Cooling. Appl. Energy 2018, 221, 334–347. [Google Scholar]
- Kirkup, L.; Frenkel, B. An Introduction to Uncertainty in Measurement; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar]
- Amer, E.H.; Kotb, H.; Mostafa, G.H.; El-Ghalban, A.R. Theoretical and Experimental Investigation of Humidification-dehumidification Desalination Unit. Desalination 2009, 249, 949–959. [Google Scholar] [CrossRef]
- Sultan, M.; El-Sharkawy, I.I.; Miyazaki, T.; Saha, B.B.; Koyama, S.; Maruyama, T.; Maeda, S.; Nakamura, T. Water Vapor Sorption Kinetics of Polymer-Based Sorbent: Theory and Experiments. Appl. Therm. Eng. 2016, 106, 192–202. [Google Scholar] [CrossRef]
- Simavilla, D.N.; Huang, W.; Vanderstrick, P.; Ryckaert, J.P.; Sferrazza, M.; Napolitano, S. Mechanism of Polymer Adsorption onto Solid Substrate. ACS Macro Lett. 2017, 6, 975–979. [Google Scholar] [CrossRef]
- Chung, J.D.; Lee, D. Effect of desiccant isotherm on the performance of the desiccant wheel. Int. J. Refrig. 2009, 32, 720–726. [Google Scholar] [CrossRef]
- Schild, P.G.; Mysen, M. Recommendation on Spesific Fan Power and Fan System Efficiency; AIVC Technical Note 65; Air Infiltration and Ventilation Centre: Sint-Stevens-Woluwe, Belgium, 2009. [Google Scholar]
- Subiantoro, A. Feasibility Analysis of the Hybrid Dehumidifier-air conditioner technology for small-scale household applications in the tropis. Sci. Technol. Built Environ. 2019, 25, 177–188. [Google Scholar] [CrossRef]
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Width | w | 0.2 | m |
| Length | L | 0.2 | m |
| Height | H | 0.2 | m |
| Thickness of sorbent | δ | 3 × 10−4 | m |
| Channel height (air layer) | h | 1.7 × 10−3 | m |
| Fin spacing | p | 3.6 × 10−3 | m |
| Heat of adsorption | ΔH | 2500 | kJ/kg |
| Density | ρb | 1500 | kg/m3 |
| Specific heat capacity of the bed | cp,b | 805 | kJ/kg·K |
| Thermal conductivity of sorbent | kd | 0.33 | W/m·K |
| Porosity | ϕ | 0.9 | - |
| Radius of the adsorbent particle | r | 1.29 × 10−6 | m |
| Process | Parameters | ||||
|---|---|---|---|---|---|
| Tin (K) | Xin (g/kg) | RHin (%) | Ua (m/s) | Re | |
| Desorption | 308 | 12.2 | 35.23 | 0.16 | 447.6 |
| 318 | 11.5 | 20.19 | 0.16 | 526.6 | |
| 328 | 13.7 | 15.5 | 0.16 | 593.7 | |
| 338 | 13.8 | 9.10 | 0.15 | 701.9 | |
| 345 | 13.5 | 6.63 | 0.15 | 712.7 | |
| Adsorption | 293 | 9.2 | 60.25 | 0.16 | 304.4 |
| Parameter | Symbol | Value |
|---|---|---|
| Humidity ratio | X | 2.37% |
| Dehumidification index | Di | 4.61% |
| Latent effectiveness | ε | 4.8% |
| Latent heat ratio | LHR | 5.31% |
| Reynolds number | Re | 3.72% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yaningsih, I.; Wijayanta, A.T.; Thu, K.; Miyazaki, T. Influence of Phase Change Phenomena on the Performance of a Desiccant Dehumidification System. Appl. Sci. 2020, 10, 868. https://doi.org/10.3390/app10030868
Yaningsih I, Wijayanta AT, Thu K, Miyazaki T. Influence of Phase Change Phenomena on the Performance of a Desiccant Dehumidification System. Applied Sciences. 2020; 10(3):868. https://doi.org/10.3390/app10030868
Chicago/Turabian StyleYaningsih, Indri, Agung Tri Wijayanta, Kyaw Thu, and Takahiko Miyazaki. 2020. "Influence of Phase Change Phenomena on the Performance of a Desiccant Dehumidification System" Applied Sciences 10, no. 3: 868. https://doi.org/10.3390/app10030868
APA StyleYaningsih, I., Wijayanta, A. T., Thu, K., & Miyazaki, T. (2020). Influence of Phase Change Phenomena on the Performance of a Desiccant Dehumidification System. Applied Sciences, 10(3), 868. https://doi.org/10.3390/app10030868






