Simulation of Electronic Quantum Devices: Failure of Semiclassical Models
Abstract
1. Introduction
2. Fundamentals of Semiclassical Device Modeling
2.1. Phenomenological Dissipation and Thermalization Models
2.2. Kinetic Dissipation and Thermalization Models
3. Partially Quantum-Mechanical Device Modeling Based on the Wigner-Function Formalism
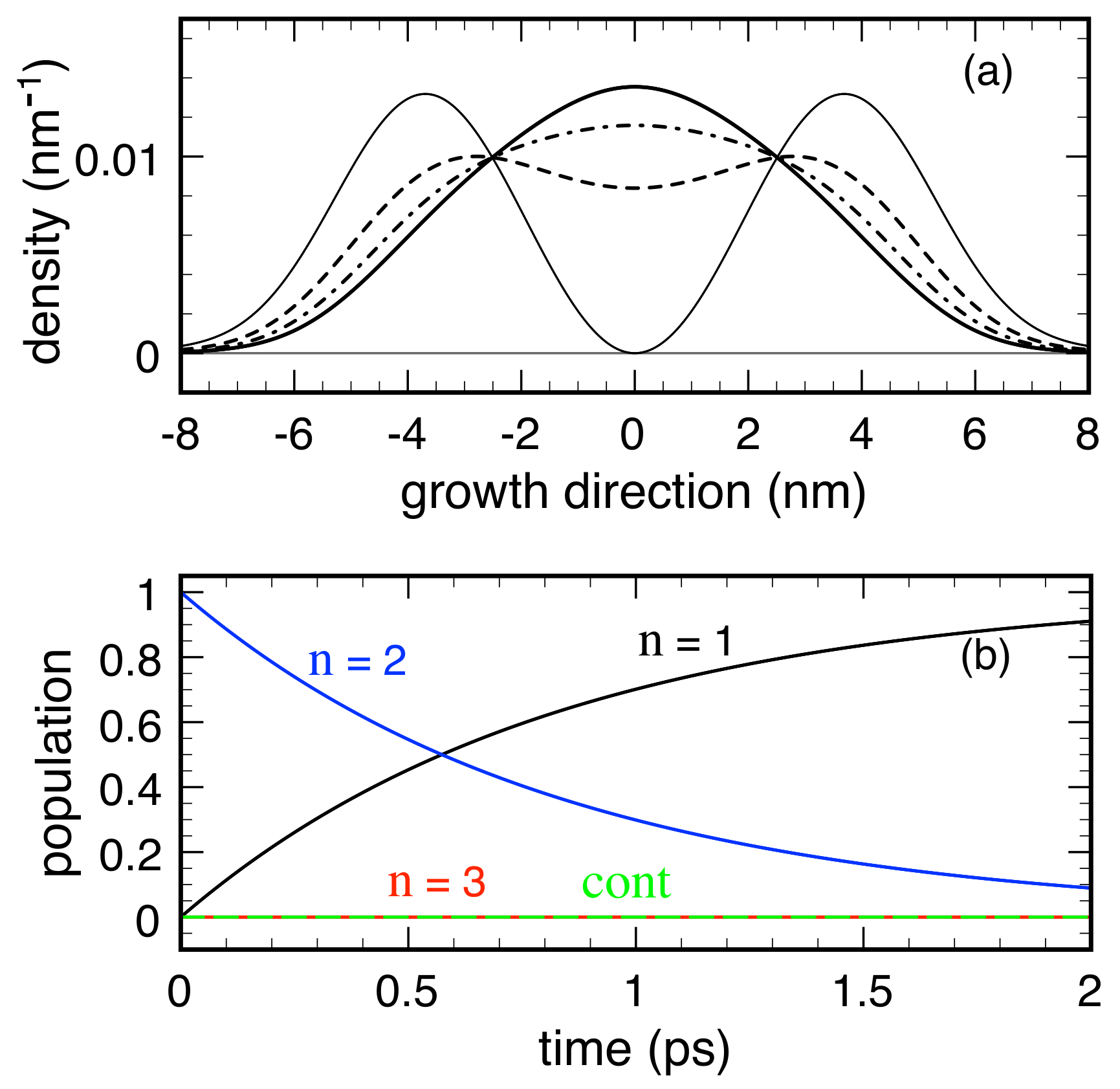
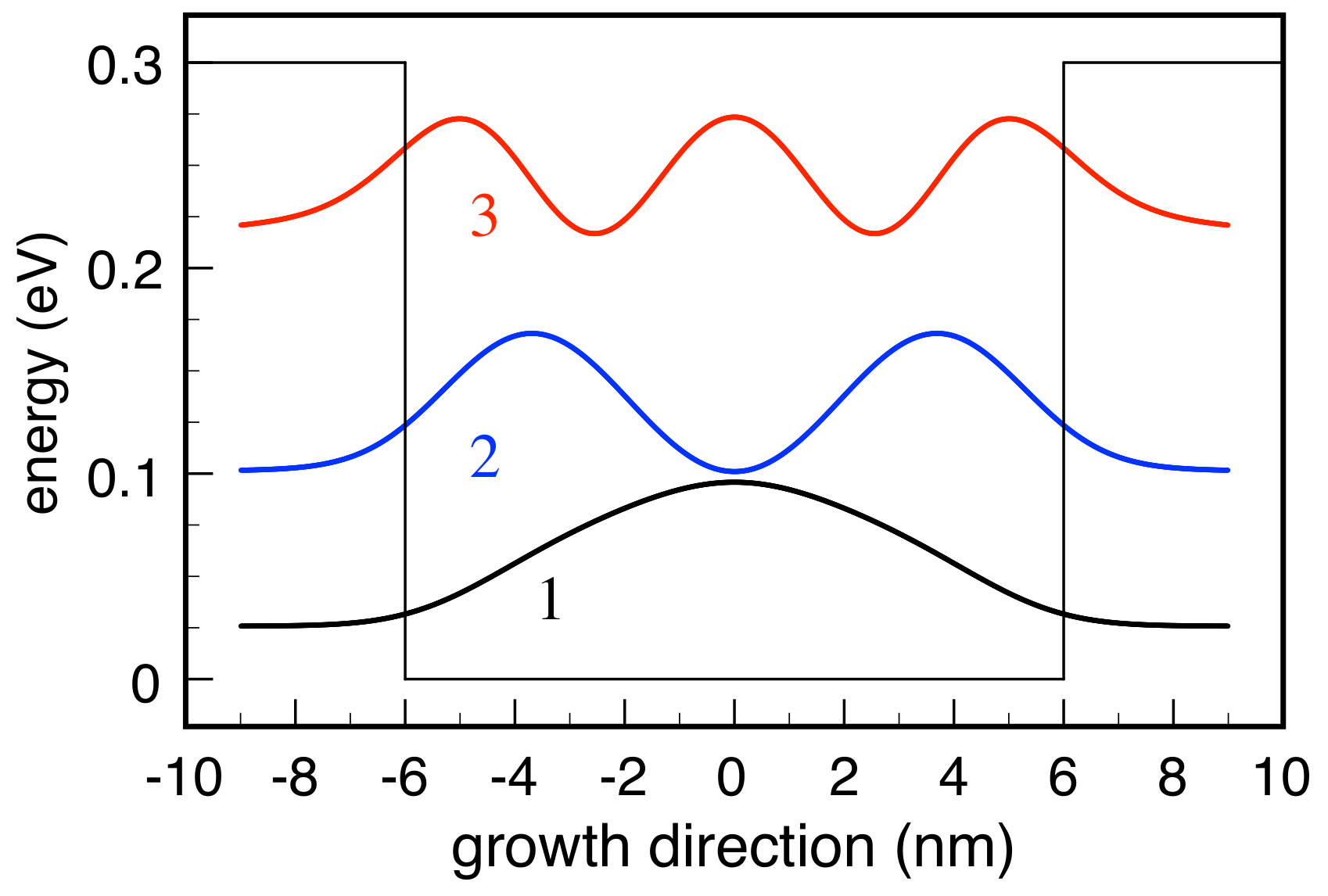
3.1. Failure of Local Dissipation Models
3.2. Failure of Local Thermalization Models
4. Fully Quantum-Mechanical Device Modeling Based on the Density-Matrix Formalism
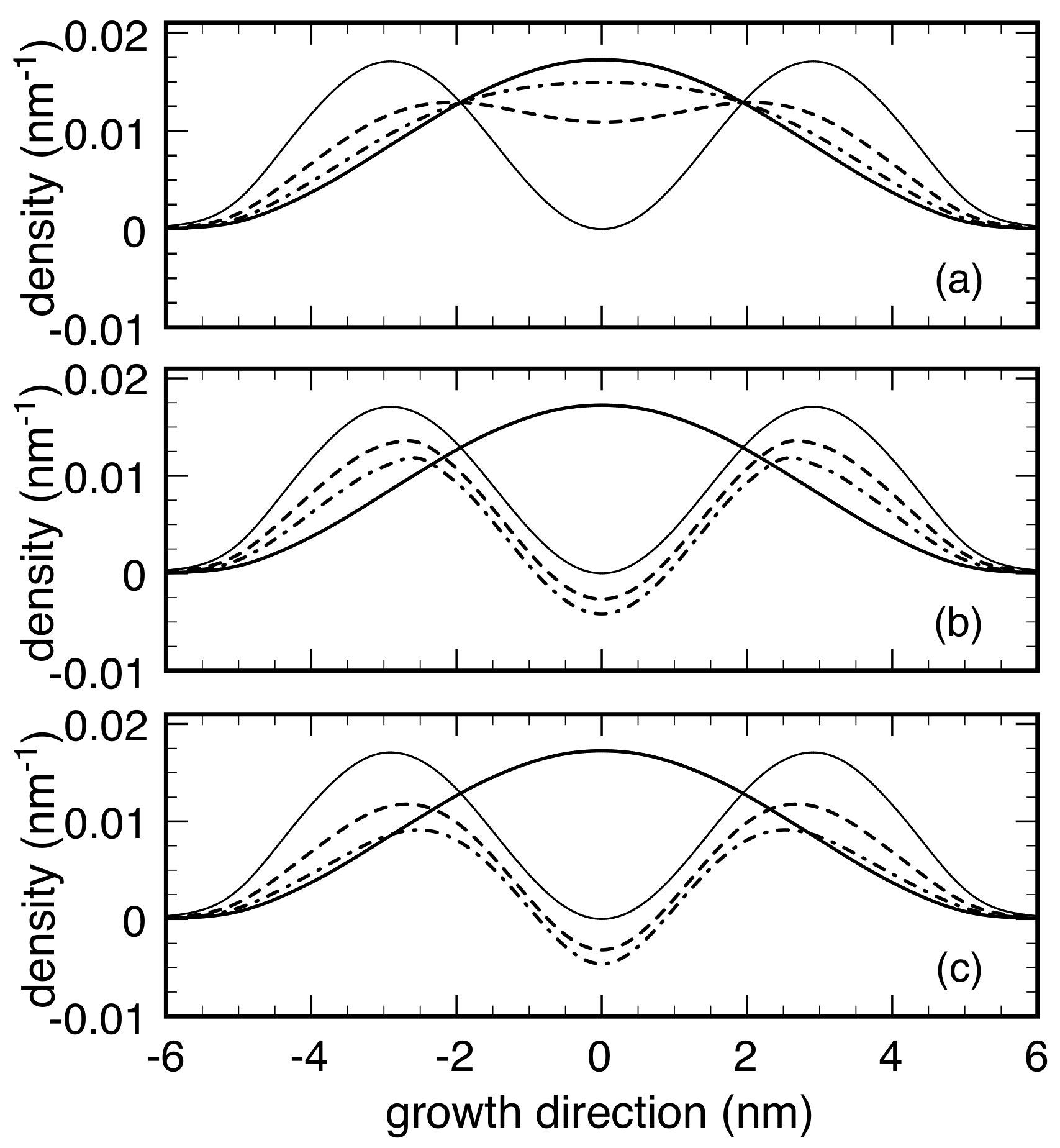
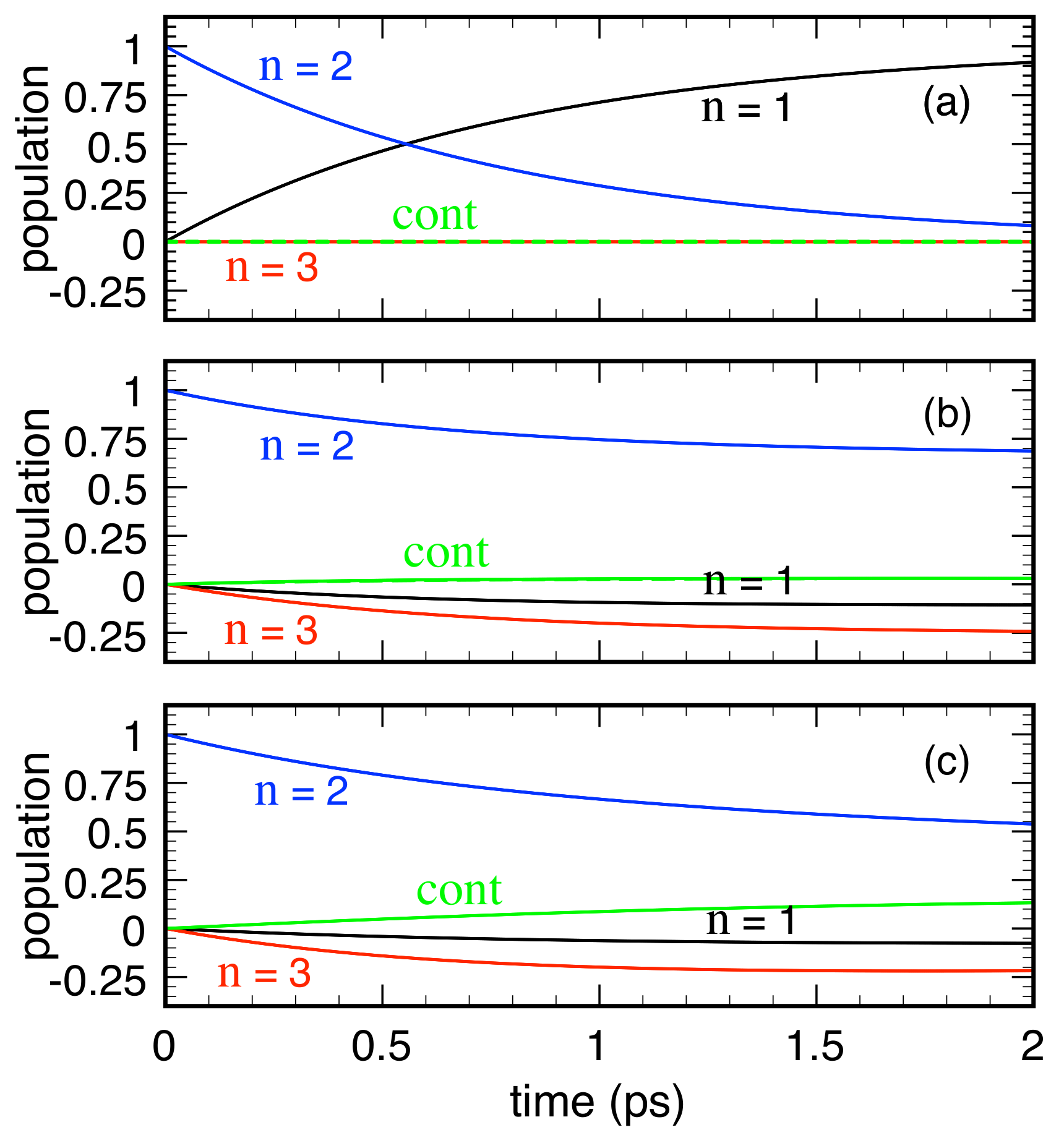
4.1. Non-Local Relaxation-Time Models
4.2. Non-Local Boltzmann-Type Models
5. Summary and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ashcroft, N.; Mermin, N. Solid State Physics; Cengage Learning India Private Limited: New Delhi, India, 2011. [Google Scholar]
- Jacoboni, C.; Lugli, P. The Monte Carlo Method for Semiconductor Device Simulation; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar]
- Yu, P.; Cardona, M. Fundamentals of Semiconductors: Physics and Materials Properties; Number v. 3 in Advanced Texts in Physics; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Bastard, G. Wave Mechanics Applied to Semiconductor Heterostructures; Monographies de Physique, Les Éditions de Physique; EDP Sciences: Les Ulis, France, 1988. [Google Scholar]
- Frensley, W.R. Boundary-conditions for open quantum-systems driven far from equilibrium. Rev. Mod. Phys. 1990, 62, 745–791. [Google Scholar] [CrossRef]
- Lent, C.S.; Tougaw, P.D.; Porod, W.; Bernstein, G.H. Quantum cellular automata. Nanotechnology 1993, 4, 49. [Google Scholar] [CrossRef]
- Di Carlo, A.; Vogl, P.; Pötz, W. Theory of Zener tunneling and Wannier-Stark states in semiconductors. Phys. Rev. B 1994, 50, 8358–8377. [Google Scholar] [CrossRef] [PubMed]
- Savasta, S.; Girlanda, R. Quantum Optical Effects and Nonlinear Dynamics in Interacting Electron Systems. Phys. Rev. Lett. 1996, 77, 4736–4739. [Google Scholar] [CrossRef]
- Fischetti, M.V. Master-equation approach to the study of electronic transport in small semiconductor devices. Phys. Rev. B 1999, 59, 4901–4917. [Google Scholar] [CrossRef]
- Datta, S. Nanoscale device modeling: The Green’s function method. Superlattice Microst. 2000, 28, 253–278. [Google Scholar] [CrossRef]
- Rossi, F.; Kuhn, T. Theory of ultrafast phenomena in photoexcited semiconductors. Rev. Mod. Phys. 2002, 74, 895–950. [Google Scholar] [CrossRef]
- Axt, V.M.; Kuhn, T. Femtosecond spectroscopy in semiconductors: A key to coherences, correlations and quantum kinetics. Rep. Prog. Phys. 2004, 67, 433–512. [Google Scholar] [CrossRef]
- Jacoboni, C.; Bordone, P. The Wigner-function approach to non-equilibrium electron transport. Rep. Prog. Phys. 2004, 67, 1033. [Google Scholar] [CrossRef]
- Pecchia, A.; Di Carlo, A. Atomistic theory of transport in organic and inorganic nanostructures. Rep. Prog. Phys. 2004, 67, 1497–1561. [Google Scholar] [CrossRef]
- Iotti, R.C.; Rossi, F. Microscopic theory of semiconductor-based optoelectronic devices. Rep. Prog. Phys. 2005, 68, 2533–2571. [Google Scholar] [CrossRef]
- Datta, S. Quantum Transport: Atom to Transistor; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Haug, H.; Jauho, A. Quantum Kinetics in Transport and Optics of Semiconductors; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Jacoboni, C. Theory of Electron Transport in Semiconductors: A Pathway from Elementary Physics to Nonequilibrium Green Functions; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Haug, H.; Koch, S. Quantum Theory of the Optical and Electronic Properties of Semiconductors; World Scientific: Singapore, 2004. [Google Scholar]
- Rossi, F. Theory of Semiconductor Quantum Devices: Microscopic Modeling and Simulation Strategies; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Buot, F. Nonequilibrium Quantum Transport Physics in Nanosystems: Foundation of Computational Nonequilibrium Physics in Nanoscience and Nanotechnology; World Scientific: Singapore, 2009. [Google Scholar]
- Frensley, W.R. Transient Response of a Tunneling Device Obtained from the Wigner Function. Phys. Rev. Lett. 1986, 57, 2853–2856. [Google Scholar] [CrossRef] [PubMed]
- Kluksdahl, N.C.; Kriman, A.M.; Ferry, D.K.; Ringhofer, C. Self-consistent study of the resonant-tunneling diode. Phys. Rev. B 1989, 39, 7720–7735. [Google Scholar] [CrossRef]
- Buot, F.A.; Jensen, K.L. Lattice Weyl-Wigner formulation of exact many-body quantum-transport theory and applications to novel solid-state quantum-based devices. Phys. Rev. B 1990, 42, 9429–9457. [Google Scholar] [CrossRef]
- Jensen, K.; Buot, F. The effects of scattering on current-voltage characteristics, transient response, and particle trajectories in the numerical simulation of resonant tunneling diodes. J. Appl. Phys. 1990, 67, 7602–7607. [Google Scholar] [CrossRef]
- Miller, D.R.; Neikirk, D.P. Simulation of intervalley mixing in double-barrier diodes using the lattice Wigner function. Appl. Phys. Lett. 1991, 58, 2803–2805. [Google Scholar] [CrossRef]
- McLennan, M.J.; Lee, Y.; Datta, S. Voltage drop in mesoscopic systems: A numerical study using a quantum kinetic equation. Phys. Rev. B 1991, 43, 13846–13884. [Google Scholar] [CrossRef] [PubMed]
- Tso, H.C.; Horing, N.J.M. Wigner-function formulation of nonlinear electron-hole transport in a quantum well and analysis of the linear transient and steady state. Phys. Rev. B 1991, 44, 11358–11380. [Google Scholar] [CrossRef]
- Gullapalli, K.K.; Miller, D.R.; Neikirk, D.P. Simulation of quantum transport in memory-switching double-barrier quantum-well diodes. Phys. Rev. B 1994, 49, 2622–2628. [Google Scholar] [CrossRef]
- Fernando, C.L.; Frensley, W.R. Intrinsic high-frequency characteristics of tunneling heterostructure devices. Phys. Rev. B 1995, 52, 5092–5104. [Google Scholar] [CrossRef]
- El Sayed, K.; Kenrow, J.A.; Stanton, C.J. Femtosecond relaxation kinetics of highly excited electronic wave packets in semiconductors. Phys. Rev. B 1998, 57, 12369–12377. [Google Scholar] [CrossRef]
- Pascoli, M.; Bordone, P.; Brunetti, R.; Jacoboni, C. Wigner paths for electrons interacting with phonons. Phys. Rev. B 1998, 58, 3503–3506. [Google Scholar] [CrossRef]
- Kim, K.Y.; Lee, B. Wigner-function formulation in anisotropic semiconductor quantum wells. Phys. Rev. B 2001, 64, 115304. [Google Scholar] [CrossRef]
- Proietti Zaccaria, R.; Rossi, F. On the problem of generalizing the semiconductor Bloch equation from a closed to an open system. Phys. Rev. B 2003, 67, 113311. [Google Scholar] [CrossRef]
- Nedjalkov, M.; Kosina, H.; Selberherr, S.; Ringhofer, C.; Ferry, D.K. Unified particle approach to Wigner-Boltzmann transport in small semiconductor devices. Phys. Rev. B 2004, 70, 115319. [Google Scholar] [CrossRef]
- Nedjalkov, M.; Vasileska, D.; Ferry, D.K.; Jacoboni, C.; Ringhofer, C.; Dimov, I.; Palankovski, V. Wigner transport models of the electron-phonon kinetics in quantum wires. Phys. Rev. B 2006, 74, 035311. [Google Scholar] [CrossRef]
- Taj, D.; Genovese, L.; Rossi, F. Quantum-transport simulations with the Wigner-function formalism: Failure of conventional boundary-condition schemes. Europhys. Lett. 2006, 74, 1060–1066. [Google Scholar] [CrossRef]
- Weetman, P.; Wartak, M.S. Self-consistent model of a nanoscale semiconductor laser using Green and Wigner functions in two bases. Phys. Rev. B 2007, 76, 035332. [Google Scholar] [CrossRef]
- Querlioz, D.; Saint-Martin, J.; Bournel, A.; Dollfus, P. Wigner Monte Carlo simulation of phonon-induced electron decoherence in semiconductor nanodevices. Phys. Rev. B 2008, 78, 165306. [Google Scholar] [CrossRef]
- Morandi, O. Multiband Wigner-function formalism applied to the Zener band transition in a semiconductor. Phys. Rev. B 2009, 80, 024301. [Google Scholar] [CrossRef]
- Wójcik, P.; Spisak, B.; Wołoszyn, M.; Adamowski, J. Self-consistent Wigner distribution function study of gate-voltage controlled triple-barrier resonant tunnelling diode. Semicond. Sci. Tech. 2009, 24, 095012. [Google Scholar] [CrossRef]
- Barraud, S. Phase-coherent quantum transport in silicon nanowires based on Wigner transport equation: Comparison with the nonequilibrium-Green-function formalism. J. Appl. Phys. 2009, 106, 063714. [Google Scholar] [CrossRef]
- Yoder, P.D.; Grupen, M.; Smith, R. Demonstration of Intrinsic Tristability in Double-Barrier Resonant Tunneling Diodes With the Wigner Transport Equation. IEEE Trans. Electron Devices 2010, 57, 3265–3274. [Google Scholar] [CrossRef]
- Álvaro, M.; Bonilla, L.L. Two miniband model for self-sustained oscillations of the current through resonant-tunneling semiconductor superlattices. Phys. Rev. B 2010, 82, 035305. [Google Scholar] [CrossRef]
- Jiang, H.; Cai, W. Effect of boundary treatments on quantum transport current in the Green’s function and Wigner distribution methods for a nano-scale DG-MOSFET. J. Comput. Phys. 2010, 229, 4461–4475. [Google Scholar] [CrossRef]
- Jiang, H.; Cai, W.; Tsu, R. Accuracy of the Frensley inflow boundary condition for Wigner equations in simulating resonant tunneling diodes. J. Comput. Phys. 2011, 230, 2031–2044. [Google Scholar] [CrossRef]
- Savio, A.; Poncet, A. Study of the Wigner function at the device boundaries in one-dimensional single- and double-barrier structures. J. Appl. Phys. 2011, 109, 033713. [Google Scholar] [CrossRef]
- Trovato, M.; Reggiani, L. Quantum maximum-entropy principle for closed quantum hydrodynamic transport within a Wigner function formalism. Phys. Rev. E 2011, 84, 061147. [Google Scholar] [CrossRef]
- Nedjalkov, M.; Schwaha, P.; Selberherr, S.; Sellier, J.; Vasileska, D. Wigner quasi-particle attributes—An asymptotic perspective. Appl. Phys. Lett. 2013, 102, 163113. [Google Scholar] [CrossRef]
- Sellier, J.; Amoroso, S.; Nedjalkov, M.; Selberherr, S.; Asenov, A.; Dimov, I. Electron dynamics in nanoscale transistors by means of Wigner and Boltzmann approaches. Physica A 2014, 398, 194–198. [Google Scholar] [CrossRef]
- Sellier, J.; Dimov, I. A Wigner approach to the study of wave packets in ordered and disordered arrays of dopants. Physica A 2014, 406, 185–190. [Google Scholar] [CrossRef]
- Jacoboni, C.; Bordone, P. Wigner transport equation with finite coherence length. J. Comput. Electron. 2014, 13, 257–263. [Google Scholar] [CrossRef]
- Jonasson, O.; Knezevic, I. Dissipative transport in superlattices within the Wigner function formalism. J. Comput. Electron. 2015, 14, 879–887. [Google Scholar] [CrossRef]
- Hamerly, R.; Mabuchi, H. Quantum noise of free-carrier dispersion in semiconductor optical cavities. Phys. Rev. A 2015, 92, 023819. [Google Scholar] [CrossRef]
- Cabrera, R.; Bondar, D.I.; Jacobs, K.; Rabitz, H.A. Efficient method to generate time evolution of the Wigner function for open quantum systems. Phys. Rev. A 2015, 92, 042122. [Google Scholar] [CrossRef]
- Kim, K.Y.; Kim, S. Effect of uncertainty principle on the Wigner function-based simulation of quantum transport. Solid State Electron. 2015, 111, 22–26. [Google Scholar] [CrossRef]
- Dorda, A.; Schürrer, F. A WENO-solver combined with adaptive momentum discretization for the Wigner transport equation and its application to resonant tunneling diodes. J. Comput. Phys. 2015, 284, 95–116. [Google Scholar] [CrossRef]
- Schulz, L.; Schulz, D. Application of a slowly varying envelope function onto the analysis of the wigner transport equation. IEEE Trans. Nanotechnol. 2016, 15, 801–809. [Google Scholar] [CrossRef]
- Woloszyn, M.; Spisak, B. Dissipative transport of thermalized electrons through a nanodevice. Phys. Rev. B 2017, 96, 075440. [Google Scholar] [CrossRef]
- Lee, J.H.; Shin, M. Quantum transport simulation of nanowire resonant tunneling diodes based on a Wigner function model with spatially dependent effective masses. IEEE Trans. Nanotechnol. 2017, 16, 1028–1036. [Google Scholar] [CrossRef]
- Iotti, R.C.; Dolcini, F.; Rossi, F. Wigner-function formalism applied to semiconductor quantum devices: Need for nonlocal scattering models. Phys. Rev. B 2017, 96, 115420. [Google Scholar] [CrossRef]
- Weinbub, J.; Ferry, D. Recent advances in Wigner function approaches. Appl. Phys. Rev. 2018, 5, 055304. [Google Scholar] [CrossRef]
- Ferry, D.; Welland, I. Relativistic Wigner functions in transition metal dichalcogenides. J. Comput. Electron. 2018, 17, 110–117. [Google Scholar] [CrossRef]
- Iotti, R.; Rossi, F. Microscopic Theory of Energy Dissipation and Decoherence in Solid-State Quantum Devices: Need for Nonlocal Scattering Models. Entropy 2018, 20, 726. [Google Scholar] [CrossRef]
- Lee, J.H.; Shin, M.; Seo, J. Performance limitations of nanowire resonant-tunneling transistors with steep switching analyzed by Wigner transport simulation. J. Appl. Phys. 2019, 125, 174502. [Google Scholar] [CrossRef]
- Ballicchia, M.; Ferry, D.K.; Nedjalkov, M.; Weinbub, J. Investigating Quantum Coherence by Negative Excursions of the Wigner Quasi-Distribution. Appl. Sci. 2019, 9, 1344. [Google Scholar] [CrossRef]
- Rosati, R.; Dolcini, F.; Iotti, R.C.; Rossi, F. Wigner-function formalism applied to semiconductor quantum devices: Failure of the conventional boundary condition scheme. Phys. Rev. B 2013, 88, 035401. [Google Scholar] [CrossRef]
- Proietti Zaccaria, R.; Ciancio, E.; Iotti, R.C.; Rossi, F. Modeling of open quantum devices within the closed-system paradigm. Phys. Rev. B 2004, 70, 195311. [Google Scholar] [CrossRef]
- Proietti Zaccaria, R.; Iotti, R.C.; Rossi, F. Monte Carlo simulation of hot-carrier phenomena in open quantum devices: A kinetic approach. Appl. Phys. Lett. 2004, 84, 139–141. [Google Scholar] [CrossRef]
- Moyal, J.E. Quantum mechanics as a statistical theory. Math. Proc. Camb. Phil. Soc. 1949, 45, 99–124. [Google Scholar] [CrossRef]
- Zhan, Z.; Colomés, E.; Oriols, X. Unphysical features in the application of the Boltzmann collision operator in the time-dependent modeling of quantum transport. J. Comput. Electron. 2016, 15, 1206–1218. [Google Scholar] [CrossRef]
- Dolcini, F.; Iotti, R.C.; Rossi, F. Interplay between energy dissipation and reservoir-induced thermalization in nonequilibrium quantum nanodevices. Phys. Rev. B 2013, 88, 115421. [Google Scholar] [CrossRef]
- Rosati, R.; Iotti, R.C.; Dolcini, F.; Rossi, F. Derivation of nonlinear single-particle equations via many-body Lindblad superoperators: A density-matrix approach. Phys. Rev. B 2014, 90, 125140. [Google Scholar] [CrossRef]


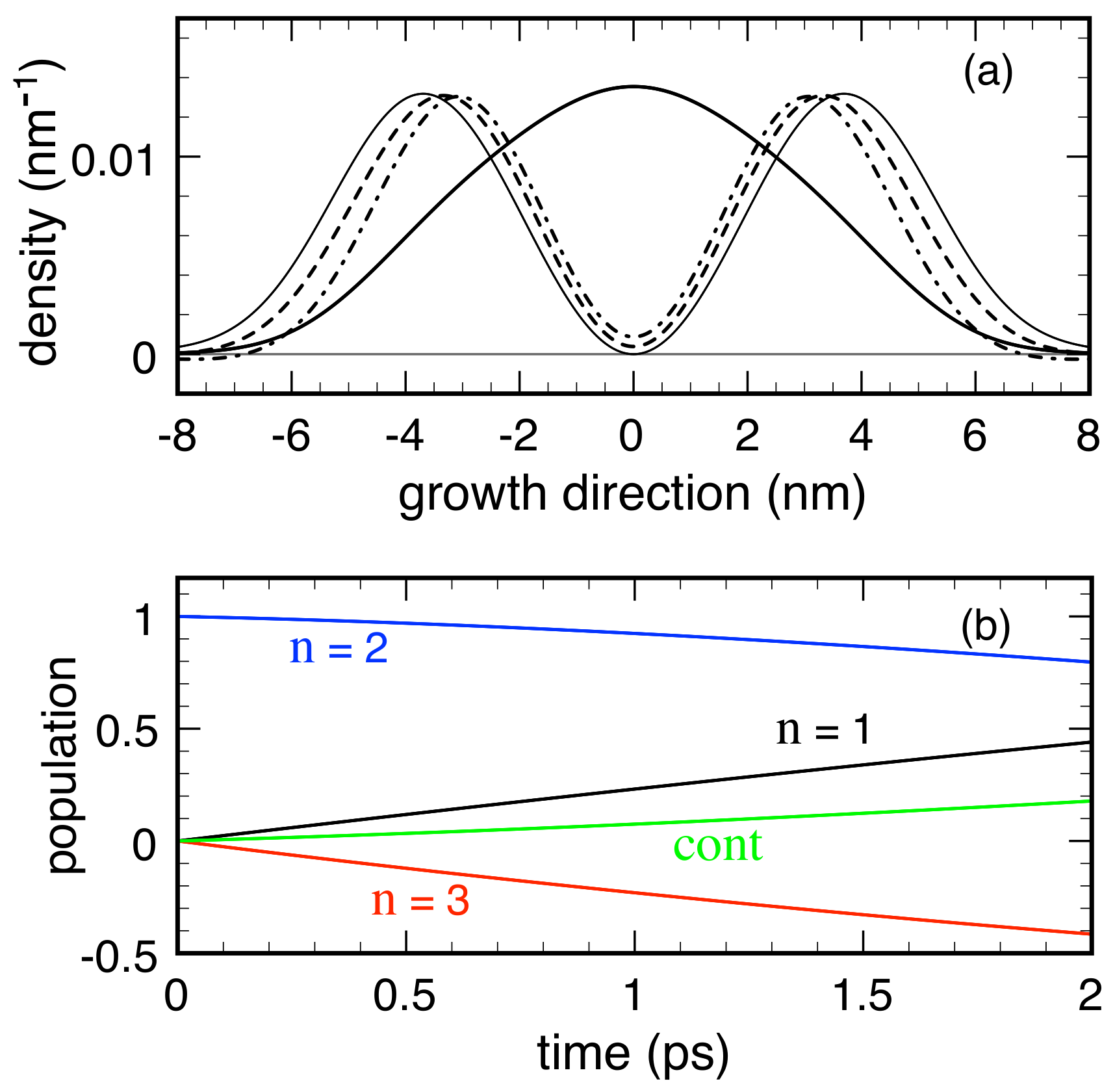

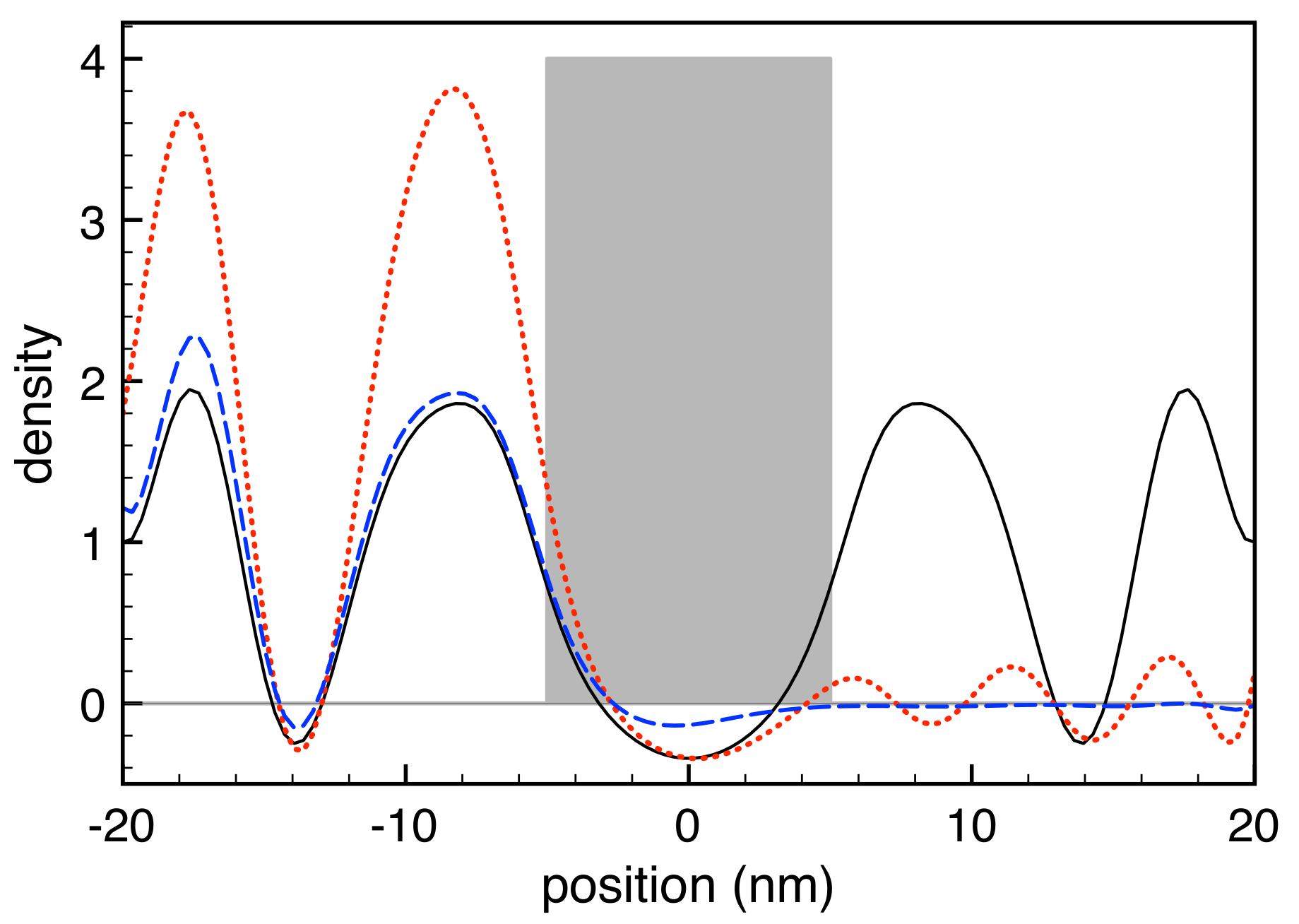
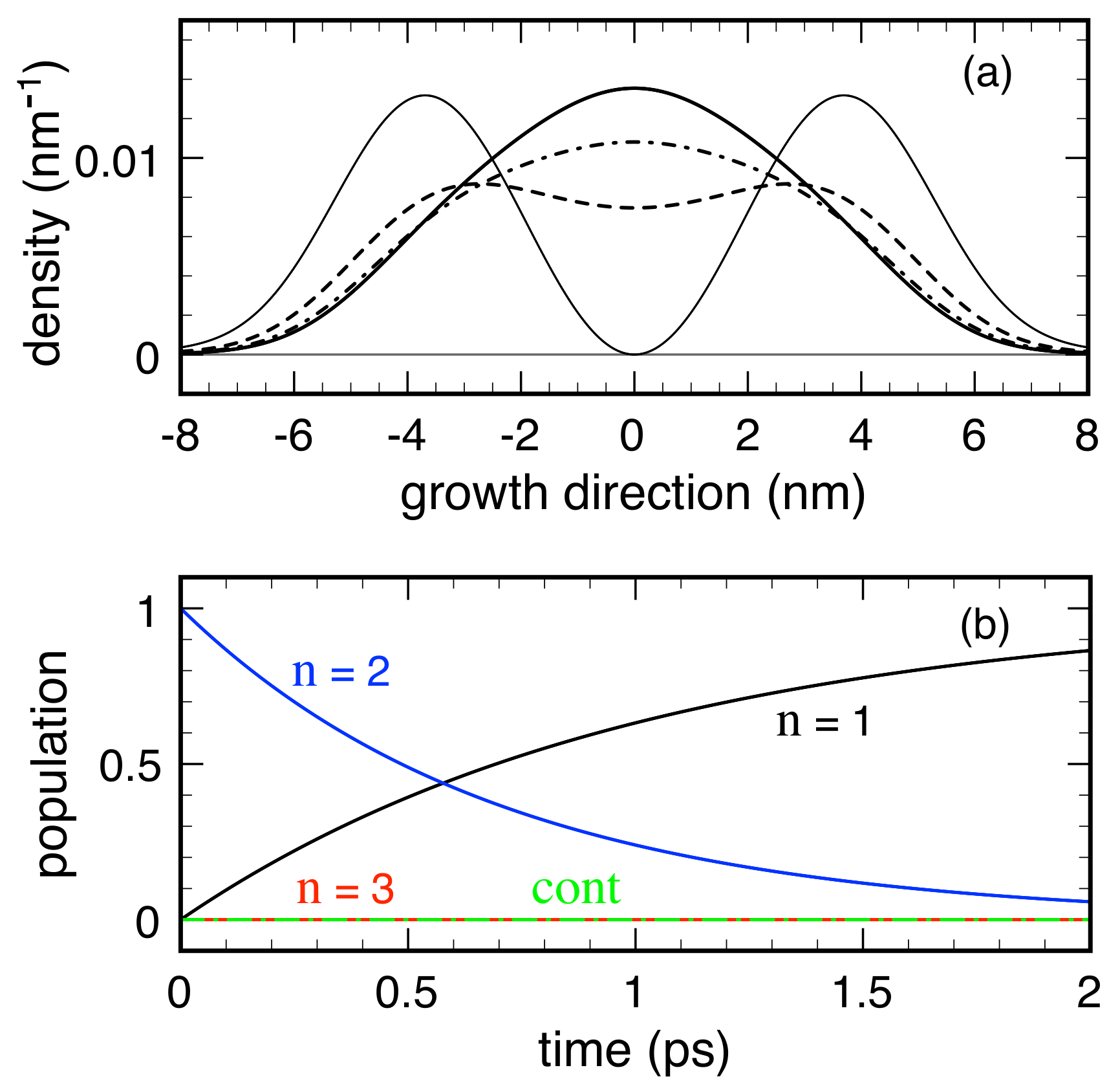

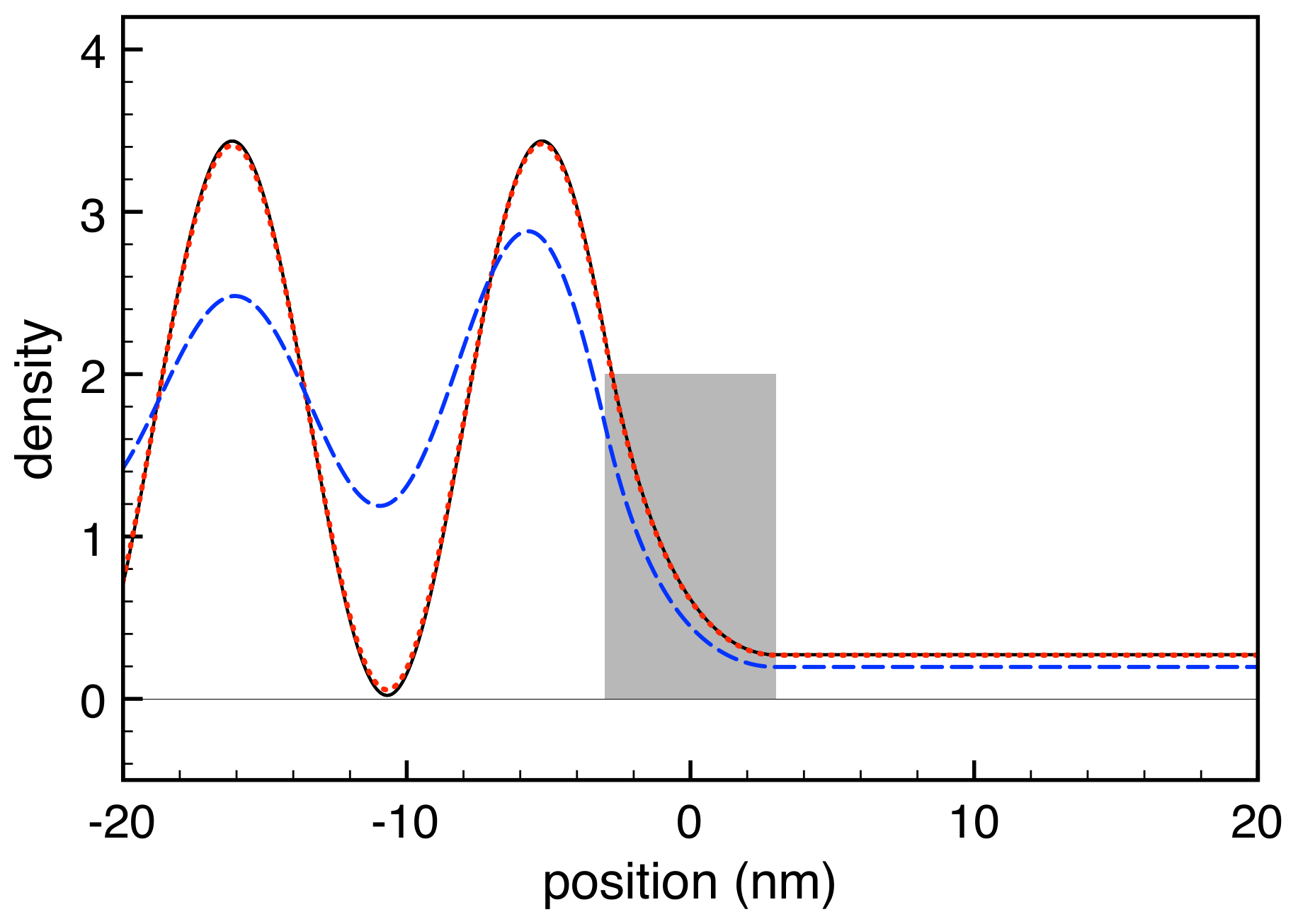
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iotti, R.C.; Rossi, F. Simulation of Electronic Quantum Devices: Failure of Semiclassical Models. Appl. Sci. 2020, 10, 1114. https://doi.org/10.3390/app10031114
Iotti RC, Rossi F. Simulation of Electronic Quantum Devices: Failure of Semiclassical Models. Applied Sciences. 2020; 10(3):1114. https://doi.org/10.3390/app10031114
Chicago/Turabian StyleIotti, Rita Claudia, and Fausto Rossi. 2020. "Simulation of Electronic Quantum Devices: Failure of Semiclassical Models" Applied Sciences 10, no. 3: 1114. https://doi.org/10.3390/app10031114
APA StyleIotti, R. C., & Rossi, F. (2020). Simulation of Electronic Quantum Devices: Failure of Semiclassical Models. Applied Sciences, 10(3), 1114. https://doi.org/10.3390/app10031114