Parametric Analysis of Tensegrity Plate-Like Structures: Part 1—Qualitative Analysis
Abstract
:Featured Application
Abstract
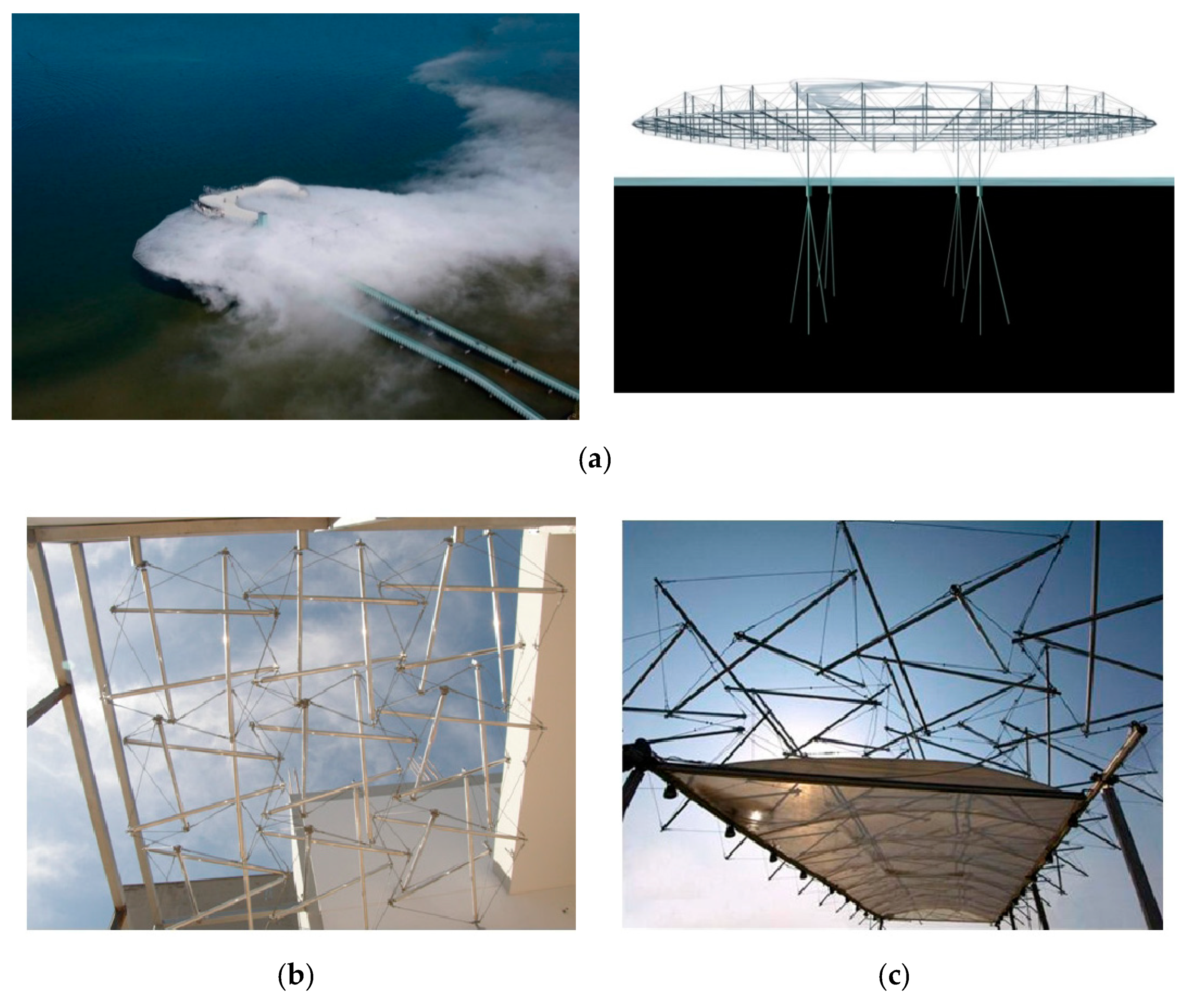
1. Introduction
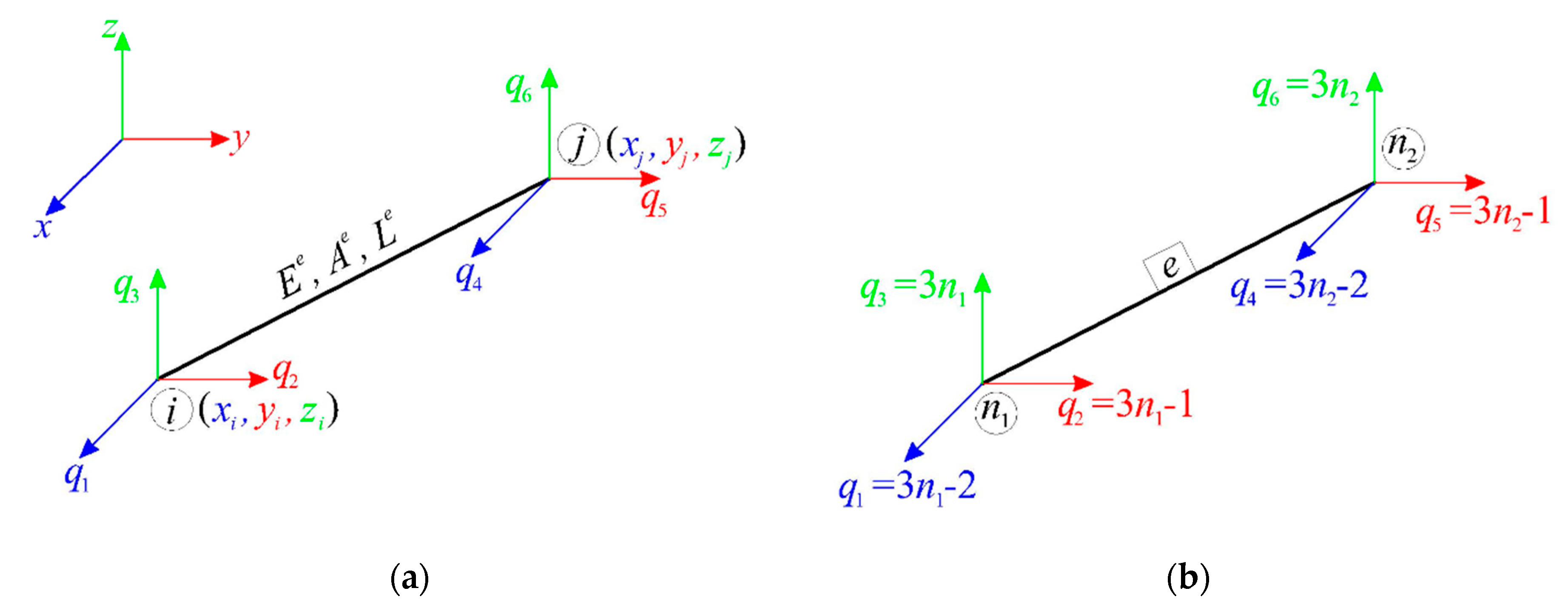
2. Mathematical Description
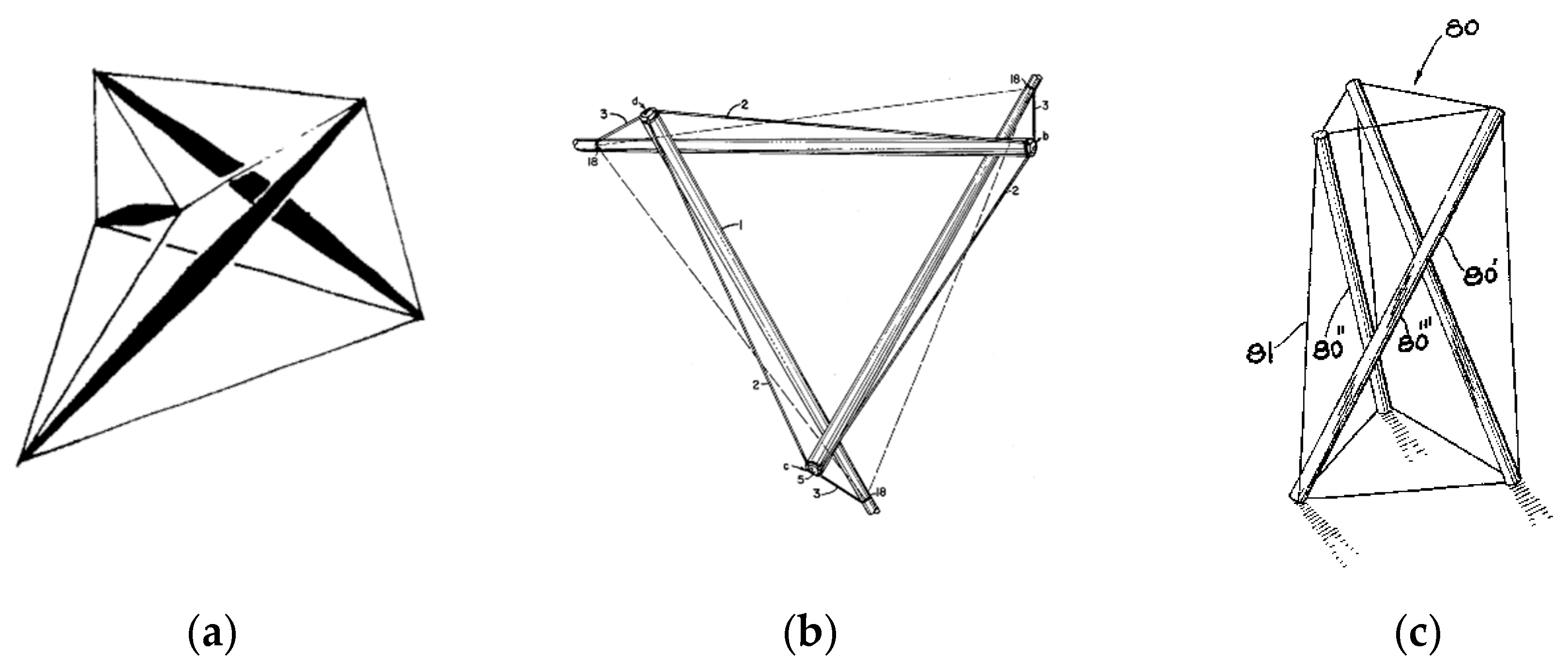
3. Classification of Tensegrity Structures
- T — the structure is a truss,
- S — there is a self-stress state,
- C — tensile elements are cables and have no rigidity in compression,
- M — there is an infinitesimal mechanism stiffened by the self-stress state,
- I — the set of struts is contained within the continuous net of tensile elements,
- D — compressed elements form a discontinuous set so its extremities do not touch each other.
- ideal tensegrity—structures which meet all requirements (T, S, C, M, D, I) and all self-stress states (including the superposed one) must ensure the stability of the structure,
- “pure” tensegrity—structures satisfy the first five requirements, that is, T, S, C, M, D, and all self-stress states ensure the stability of the structure,
- structures with tensegrity features of class 1—structures meet the conditions of the first four features (T, S, C, M) and at least one self-stress state that ensures the stability of the structure,
- structures with tensegrity features of class 2—structures meet only the obligatory requirements (T, S, C) and additionally either feature I or D.
4. Examples
- —eigenvalues of matrix ,
- —eigenvectors of corresponding to the zero eigenvalues (if any) in responsible for the existence of the self-stress state (S),
- —eigenvalues of matrix ,
- —eigenvectors of corresponding to the zero eigenvalues (if any) in responsible for the existence of the mechanism (M),
- —eigenvalues of matrix .
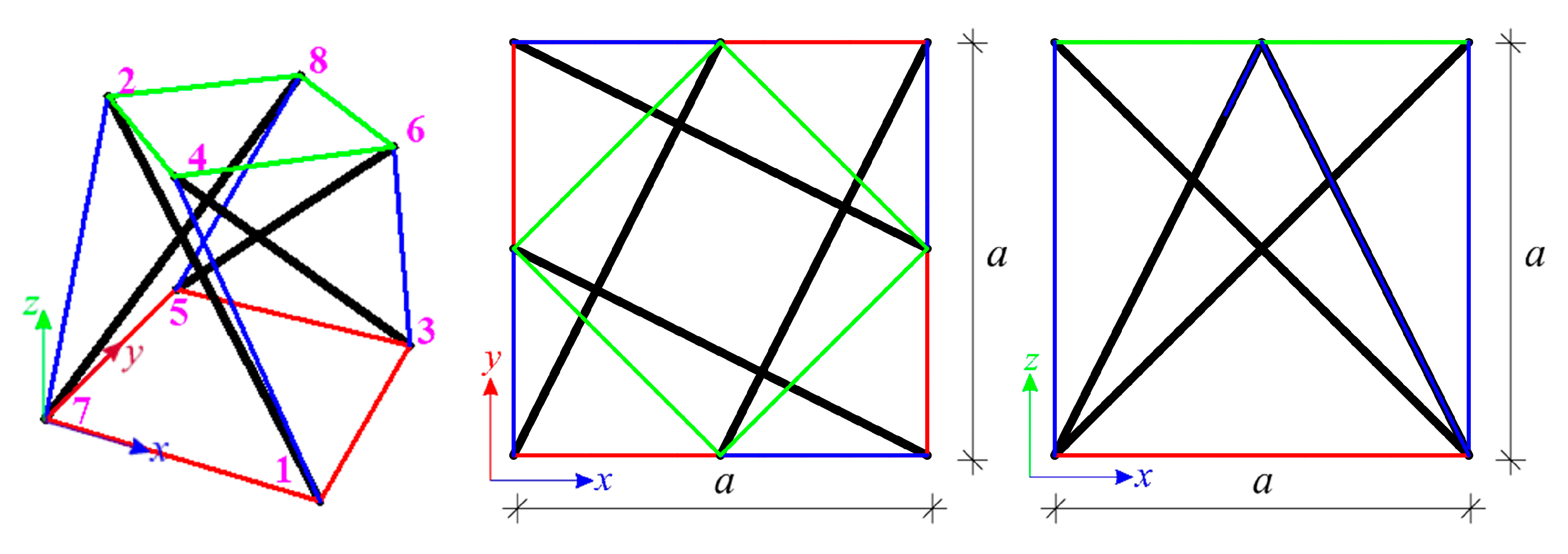
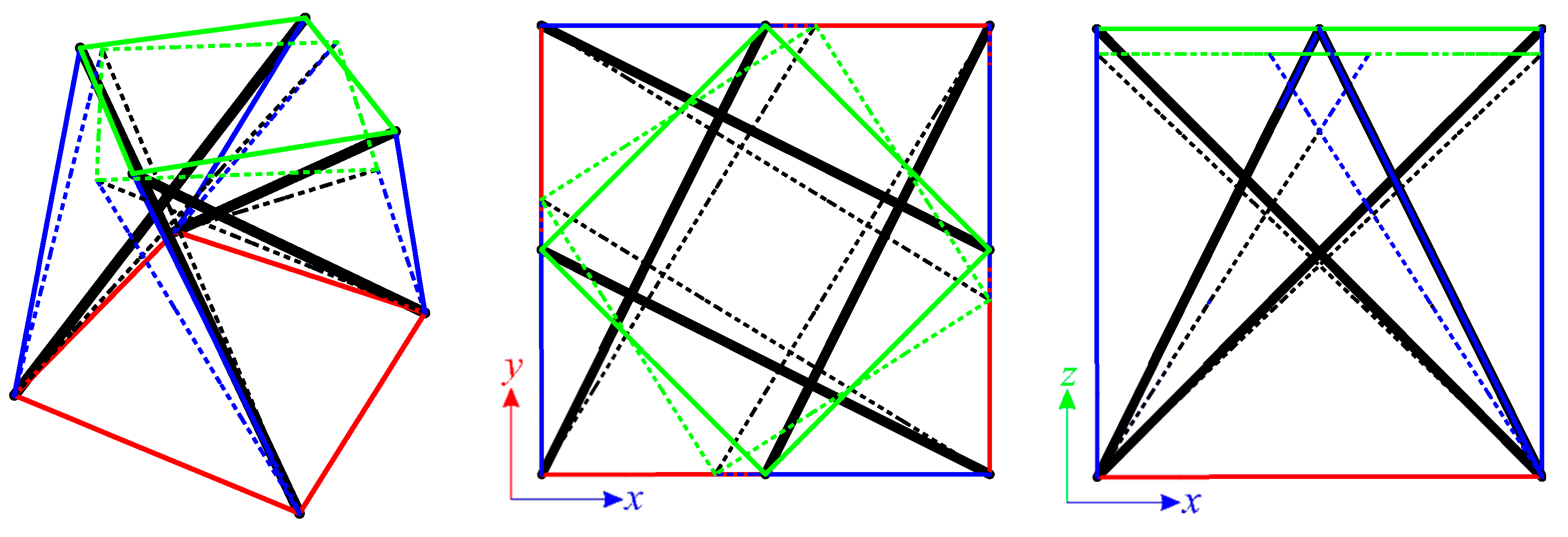
4.1. Single Modified Quartex Module
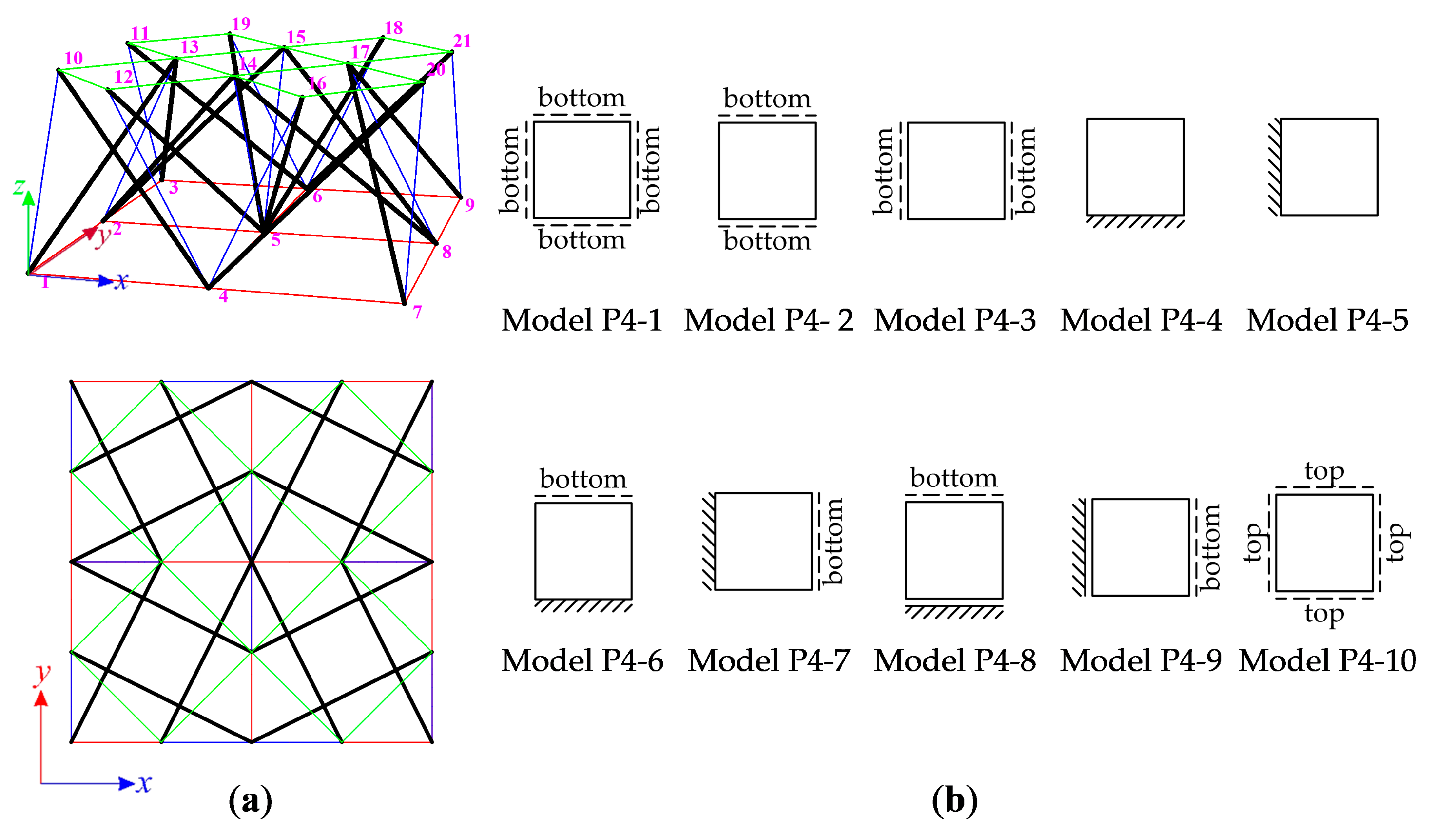
4.2. Four-Module Tensegrity Plate-Like Structures
- model P4-1—the truss (T) with 39 d.o.f. (m = 39),
- model P4-2—the truss (T) with 45 d.o.f. (m = 45),
- model P4-3—the truss (T) with 45 d.o.f. (m = 45),
- model P4-4—the truss (T) with 48 d.o.f. (m = 48),
- model P4-5—the truss (T) with 48 d.o.f. (m = 48),
- model P4-6—the truss (T) with 39 d.o.f. (m = 39),
- model P4-7—the truss (T) with 39 d.o.f. (m = 39),
- model P4-8—the truss (T) with 38 d.o.f. (m = 38),
- model P4-9—the truss (T) with 38 d.o.f. (m = 38),
- model P4-10—the truss (T) with 39 d.o.f. (m = 39).
4.3. Sixteen-Module Tensegrity Plate-Like Structures
- model P16-1—the truss (T) with 153 d.o.f. (m = 153),
- model P16-2—the truss (T) with 156 d.o.f. (m = 156),
- model P16-3—the truss (T) with 105 d.o.f. (m = 105),
- model P16-4—the truss (T) with 129 d.o.f. (m = 129),
- model P16-5—the truss (T) with 122 d.o.f. (m = 122),
- model P16-6—the truss (T) with 146 d.o.f. (m = 146).
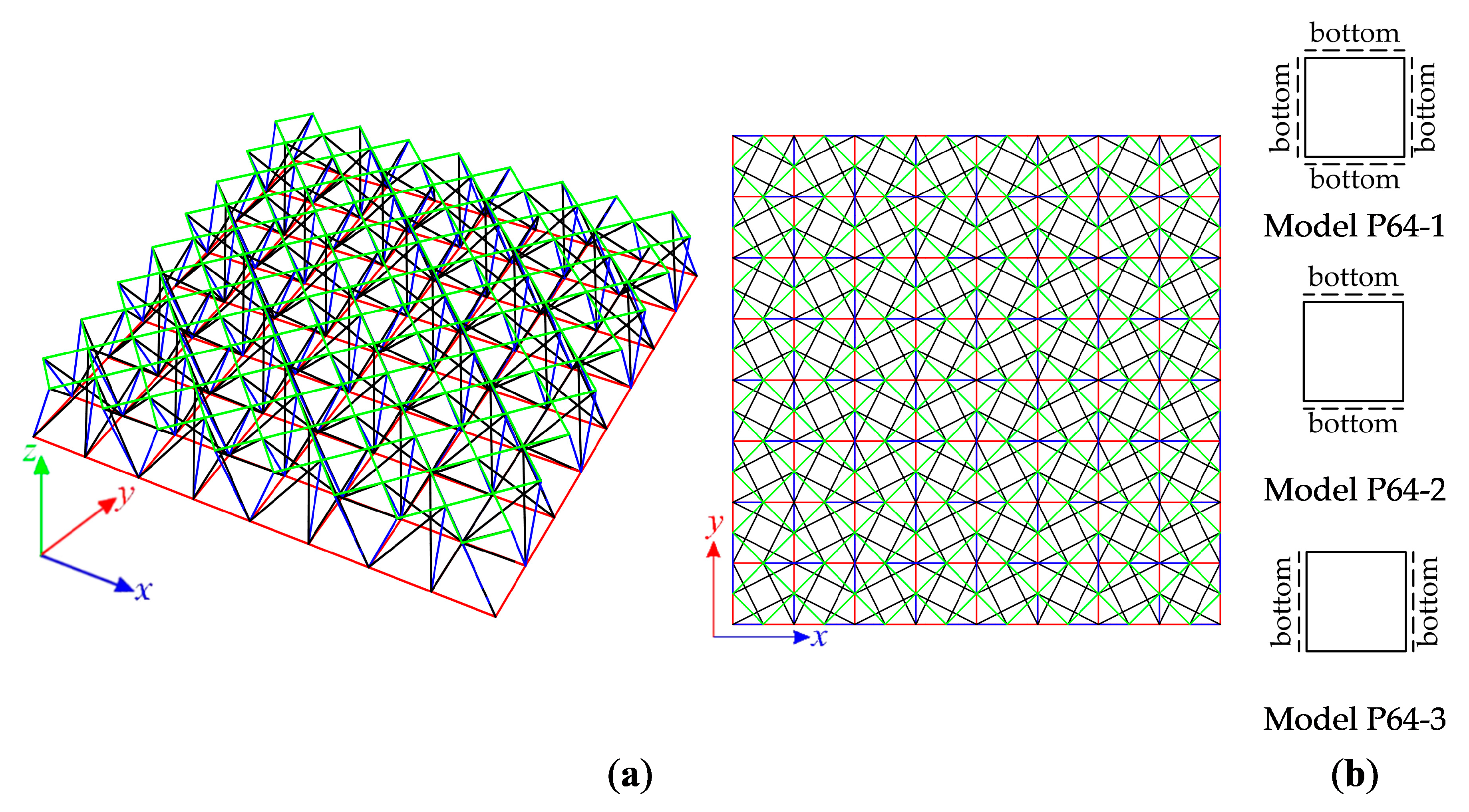
4.4. Sixty-Four-Module Tensegrity Plate-Like Structures
- model P64-1—the truss (T) with 579 d.o.f. (m = 579),
- model P64-2—the truss (T) with 621 d.o.f. (m = 621),
- model P64-3—the truss (T) with 621 d.o.f. (m = 621).
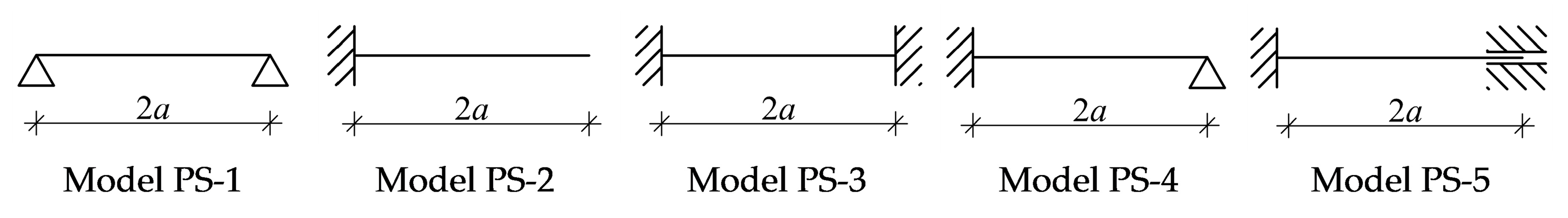
4.5. Plate Strips
- model PS-1—the truss (T) with 39 d.o.f. (m = 39),
- model PS-2—the truss (T) with 40 d.o.f. (m = 45),
- model PS-3—the truss (T) with 27 d.o.f. (m = 27),
- model PS-4—the truss (T) with 33 d.o.f. (m = 33),
- model PS-5—the truss (T) with 32 d.o.f. (m = 32).
5. Conclusions
- Only the single modified Quartex module is the ideal tensegrity. This module meets all tensegrity features, i.e., the structure is a truss (T) with a continuous net of tensile components—cables (C)—including a discontinuous set (D) of the compressed elements (I) and it features the existence of one self-stress state (S) and one mechanism (M).
- The plates built with four, sixteen or sixty-four modules cannot be the ideal tensegrities because of the way of connecting the modules. These structures do not satisfy the condition for the discontinuity of struts (D).
- All of the plates are characterised by the existence of more than one self-stress state (S). Due to the fact that only one self-stress state accurately identifies the elements, they cannot be considered as “pure” tensegrities. Actually, the only one appropriate self-stress state for the all considered plate-like structures is the one that is a superposition of the self-stress state of the single module.
- Considered plates can be classified as structures with features of class 1, if there is a mechanism, or class 2, if there is no mechanism (see Table 4).
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Gilewski, W.; Obara, P.; Kłosowska, J. Self-stress control of real civil engineering tensegrity structures. AIP Conf. Proc. 2018, 1922, 150004. [Google Scholar]
- Gilewski, W.; Kłosowska, J.; Obara, P. The influence of self-stress on the behavior of tensegrity-like real structure. MATEC Web Conf. 2017, 117, 00079. [Google Scholar] [CrossRef] [Green Version]
- Gilewski, W.; Kłosowska, J.; Obara, P. Parametric analysis of some tensegrity structures. MATEC Web Conf. 2019, 262, 10003. [Google Scholar] [CrossRef]
- Obara, P. Analysis of orthotropic tensegrity plate strips using a continuum two-dimensional model. MATEC Web Conf. 2019, 262, 10010. [Google Scholar] [CrossRef]
- Obara, P. Application of linear six-parameter shell theory to the analysis of orthotropic tensegrity plate-like structures. J. Theor. App. Mech. 2019, 57, 167–178. [Google Scholar] [CrossRef]
- Emmerich, D.G. Construction de Reseaux Autotendants. French Patent 1,377,290, 28 September 1964. [Google Scholar]
- Fuller, R.B. Tensile-Integrity Structures. U.S. Patent 3,063,521, 13 November 1962. [Google Scholar]
- Snelson, K. Continuous Tension, Discontinuous Compression Structures. U.S. Patent 3,169,611, 16 February 1965. [Google Scholar]
- Estrada, G.; Bungartz, H.-J.; Mohrdieck, C. Numerical form-finding of 2D tensegrity structures. In Proceedings of the 5th International Conference on Computation of Shell and Spatial Structures, Salzburg, Austria, 1–4 June 2005. [Google Scholar]
- Skelton, R.E.; de Oliveira, M.C. Tensegrity Systems; Springer: London, UK, 2009. [Google Scholar]
- Chen, Y.; Feng, J. Initial prestress distribution and natural vibration analysis of tensegrity structures based on group theory. Int. J. Struct. Stab. Dyn. 2012, 12. [Google Scholar] [CrossRef]
- Zhang, L.-Y.; Li, Y.; Cao, Y.-P.; Feng, X.-Q. Stiffness matrix based form-finding method of tensegrity structures. Eng. Struct. 2014, 58, 36–48. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhang, D.; Dobah, Y.; Scarpa, F.; Fernando Fraternali, F.; Skelton, R. Tensegrity cell mechanical metamaterial with metal rubber. Appl. Phys. Lett. 2018, 113, 031906. [Google Scholar] [CrossRef] [Green Version]
- Motro, R. Tensegrity. Structural Systems for the Future; Kogan Page: London, UK, 2003. [Google Scholar]
- Wang, B.B. Free-Standing Tension Structures. From Tensegrity Systems to Cable-Strut System; CRC Press: New York, NY, USA, 2004. [Google Scholar]
- Ali, N.; Rhode-Barbarigos, L.; Smith, I. Analysis of clustered tensegrity structures using a modified dynamic relaxation algorithm. Int. J. Solids Struct. 2011, 48, 637–664. [Google Scholar]
- Zhang, L.; Gao, Q.; Liu, Y.; Zhang, H. An efficient finite element formulation for nonlinear analysis of clustered tensegrity. Eng. Comput. 2016, 33, 252–273. [Google Scholar] [CrossRef]
- Feron, J.; Boucher, L.; Denoël, V.; Latteur, P. Optimization of Footbridges Composed of Prismatic Tensegrity Modules. J. Bridge Eng. 2019, 24, 04019112. [Google Scholar] [CrossRef] [Green Version]
- Kono, Y.; Kunieda, H. Tensegrity grids transformed from double-layer space grids. In Conceptual Design of Structures, Proceedings of the International Symposium, Stuttgart, Germany, 7–11 October 1996; IASS: Stuttgart, Germany, 1996; pp. 293–300. [Google Scholar]
- Gómez-Jáuregui, V.; Arias, R.; Otero, C.; Manchado, C. Novel Technique for Obtaining Double-Layer Tensegrity Grids. Int. J. Solids Struct. 2012, 27, 155–166. [Google Scholar] [CrossRef]
- Olejnikova, T. Double Layer Tensegrity Grids. Acta Polytech. Hung. 2012, 9, 95–106. [Google Scholar]
- Wang, Y.; Xian, X. Prestress Design of Tensegrity Structures Using Semidefinite Programming. Adv. Civ. Eng. 2019, 2019, 1–9. [Google Scholar] [CrossRef]
- Faroughi, S.; Lee, J. Geometrical nonlinear analysis of tensegrity based on a co-rotational method. Adv. Struct. Eng. 2014, 17, 41–51. [Google Scholar] [CrossRef]
- Sulaiman, S.; Parthasarathi, N.L.; Geetha, B.; Satyanarayanan, K.S. The Performance of Half-Cuboctahedron Grid Tensegrity Systems in Roof Structures. Indian, J. Sci. Technol. 2016, 9, 1–6. [Google Scholar] [CrossRef]
- Wang, B.B. Cable-strut systems: Part I—Tensegrity. J. Constr. Steel Res. 1998, 45, 281–289. [Google Scholar] [CrossRef]
- Wang, B.B. Realizing Cable-Strut Systems. Int. J. Architect. Techn. 2012, 42, 42–53. [Google Scholar]
- Hanaor, A. Double-layer tensegrity grids: Static load response. II—Experimental study. J. Struct. Eng. 1991, 117, 1675–1684. [Google Scholar] [CrossRef]
- Hanaor, A. Aspects of design of double-layer tensegrity domes. Int. J. Space Struct. 1992, 7, 101–113. [Google Scholar] [CrossRef]
- Hanaor, A. Double-layer tensegrity grids as deployable structures. Int. J. Space Struct. 1993, 8, 135–145. [Google Scholar] [CrossRef]
- Hanaor, A. Geometrically rigid double-layer tensegrity grids. Int. J. Space Struct. 1994, 9, 227–238. [Google Scholar]
- Hanaor, A.; Liao, M.K. Double-Layer Tensegrity Grids: Static Load Response. Part I: Analytical Study. J. Struct. Eng. 1991, 117, 1660–1674. [Google Scholar] [CrossRef]
- Motro, R. Tensegrity Systems and Geodesic Domes. Int. J. Space Struct. 1990, 5, 341–351. [Google Scholar] [CrossRef]
- Raducanu, V.; Motro, R. New tensegrity grids. In Proceedings of the International Symposium on Theory, Design and Realization of Shell and Spatial Structures, Nagoya, Japan, 9–13 October 2001; pp. 320–321. [Google Scholar]
- Raducanu, V. Architecture et système constructif: Cas des systèmes de tenségrité. Ph.D. Thesis, Montpellier University, Montpellier, France, 2001. [Google Scholar]
- Averseng, J. Mise en œuvre et contrôle des systèmes de tenségrité. Ph.D. Thesis, Université Montpellier, Montpellier, France, 2004. [Google Scholar]
- Averseng, J.; Jamin, F.; Quirant, J. Système de tenségrité déployable et modulaire pour le développement de l’accessibilité. In Proceedings of the Rencontres Universitaires de Génie Civil, Nantes, France, 22–24 May 2017. [Google Scholar]
- Averseng, J.; Jamin, F.; Quirant, J. Les systèmes de tenségrité déployables: Application à l’accessibilité de la baignade en mer. In Proceedings of the Rencontres Universitaires de Génie Civil, Bayonne, France, 26–29 May 2015. [Google Scholar]
- Papantoniou, A. Parametric models of tensegrity structures with double curvature. ArchiDOCT 2017, 5, 63–76. [Google Scholar]
- Gilewski, W.; Al Sabouni-Zawadzka, A. On orthotropic properties of tensegrity structures. Procedia Eng. 2016, 153, 887–894. [Google Scholar]
- Gilewski, W.; Al Sabouni-Zawadzka, A. Smart tensegrity plate structures in communication engineering (in Polish). Logistyka 2014, 6, 1390–1399. [Google Scholar]
- Gilewski, W.; Al Sabouni-Zawadzka, A. Smart Metamaterial Based on the Simplex Tensegrity Pattern. Materials 2018, 11, 673. [Google Scholar]
- Fest, E.; Shea, K.; Domer, B.; Smith, I.F.C. Adjustable Tensegrity Structures. J. Struct. Eng. 2003, 129, 515–526. [Google Scholar] [CrossRef] [Green Version]
- Fest, E.; Shea, K.; Smith, I.F.C. Active tensegrity structure. J. Struct. Eng. 2004, 130, 1454–1465. [Google Scholar] [CrossRef] [Green Version]
- Crawfordt, L. Transgender Architectonics: The Shape of Change in Modernist Space; Routledge: London, UK, 2015. [Google Scholar]
- Gilewski, W.; Kłosowska, J.; Obara, P. Verification of Tensegrity Properties of Kono Structure and Blur Building. Procedia Eng. 2016, 153, 173–179. [Google Scholar] [CrossRef] [Green Version]
- Blur Building. Available online: https://dsrny.com/project/blur-building (accessed on 5 September 2020).
- Liapi, K.A.; Kim, J. Tensegrity Structures of Helical Shape: A Parametric Approach. In Proceedings of the Conference on Computation: The New Realm of Architectural Design, Istanbul, Turkey, 16–19 September 2009; pp. 53–58. [Google Scholar]
- Falk, A. Architectural and structural development of plate tensegrity. In Proceedings of the International Association for Shell and Spatial Structures 2006 Symposium, Beijing, China, 16–19 October 2006. [Google Scholar]
- Liapi, K.; Kim, J. A Parametric Approach to the Design of a Tensegrity Vaulted Dome for an Ephemeral Structure for the 2004 Olympics. In Proceedings of the the 2003 Annual Conference of the Association for Computer Aided Design in Architecture, Indianapolis, IN, USA, 24–27 October 2003; pp. 301–309. [Google Scholar]
- Bathe, K.J. Finite Element Procedures in Engineering Analysis; Prentice Hall: New York, NY, USA, 1996. [Google Scholar]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method: The Basis; Elsevier Butterworth-Heinemann: London, UK, 2000; Volume 1. [Google Scholar]
- Gilewski, W.; Kasprzak, A. Introduction to mechanics of tensegrity modules. In Theoretical Foundation of Civil Engineering. Mechanics of Structures and Materials; Jemioło, S., Lutomirski, S., Eds.; OWPW: Warsaw, Poland, 2012; Volume 1, pp. 83–94. [Google Scholar]
- Calladine, C.R. Buckminster Fuller’s “tensegrity” structures and clerk Maxwell’s rules for the construction of stiff frames. Int. J. Solids Struct. 1978, 14, 161–172. [Google Scholar] [CrossRef]
- Calladine, C.R. Modal stiffnesses of a pretensioned cable net. Int. J. Solids Struct. 1982, 18, 829–846. [Google Scholar] [CrossRef]
- Pellegrino, S.; Calladine, C.R. Matrix analysis of statically and kinematically indeterminate frameworks. Int. J. Solids Struct. 1986, 22, 409–428. [Google Scholar] [CrossRef]
- Pellegrino, S. Analysis of prestressed mechanisms. Int. J. Solids Struct. 1990, 26, 1329–1350. [Google Scholar] [CrossRef]
- Calladine, C.R.; Pellegrino, S. First order infinitesimal mechanisms. Int. J. Solids Struct. 1991, 27, 505–515. [Google Scholar] [CrossRef]
- Obara, P.; Kłosowska, J.; Gilewski, W. Truth and myths about 2D tensegrity trusses. Appl. Sci. 2019, 9, 179. [Google Scholar] [CrossRef] [Green Version]
- Gilewski, W.; Kłosowska, J.; Obara, P. Form finding of tensegrity structures via Singular Value Decomposition of compability matrix. In Advances in Mechanics: Theoretical, Computational and Interdisciplinary Issues, Proceedings of the 21st International Conference on Computer Methods in Mechanics, Gdansk, Poland, 8–11 September 2015; CRC Press: Boca Raton, FL, USA, 2015; pp. 191–195. [Google Scholar]
- Golub, G.; Kahan, W. Calculating the singular values and pseudo-inverse of a matrix. J. SIAM Numer. Anal. Ser. B 1965, 2, 205–224. [Google Scholar] [CrossRef]
- Klema, V.C. The singular value decomposition: It’s computation and some applications. IEEE Trans. Autom. Contr. 1980, 25, 164–176. [Google Scholar] [CrossRef] [Green Version]
- Leon, S.J. Linear Algebra with Applications; Macmillan: New York, NY, USA, 1994. [Google Scholar]
- Long, C. Visualization of matrix singular value decomposition. Math. Mag. 1983, 56, 161–167. [Google Scholar] [CrossRef]
- Mc Guire, W.; Gallagher, R.H. Matrix Structural Analysis; Wiley: New York, NY, USA, 1979. [Google Scholar]
- Stewart, G.W. Matrix Algorithms: Basic Decompositions; SIAM: Philadelphia, PA, USA, 1998. [Google Scholar]
- Strang, G. Introduction to Linear Algebra; Wellesley-Cambridge Press: Wellesley, MA, USA, 1993. [Google Scholar]
- Gilewski, W.; Kłosowska, J.; Obara, P. Application of singular value decomposition for qualitative analysis of truss and tensegrity structures. ACTA Sci. Polon. Ser. Archit. 2015, 14, 3–20. [Google Scholar]
- Pellegrino, S. Structural computations with the singular value decomposition of the equilibrium matrix. Int. J. Solids Struct. 1993, 30, 3025–3035. [Google Scholar] [CrossRef]
- Rahami, H.; Kaveh, A.; Ardalan Asl, M.; Mirghaderi, S.R. Analysis of near-regular structures with node irregularity using SVD of equilibrium matrix. Int. J. Civ. Eng. 2013, 11, 226–239. [Google Scholar]
- Obara, P. Dynamic and Dynamic Stability of Tensegrity Structures; Wydawnictwo Politechniki Świętokrzyskiej: Kielce, Poland, 2019. (In Polish) [Google Scholar]
- Kasprzak, A. Assessment of the possibility of using tensegrity in bridge structures. Ph.D. Thesis, Warsaw University of Technology, Warsaw, Poland, 2014. (In Polish). [Google Scholar]
- Kebiche, K.; Kazi-Aoual, M.N.; Motro, R. Geometrical non-linear analysis of tensegrity systems. Eng. Struct. 1999, 21, 864–876. [Google Scholar] [CrossRef]
- Tran, H.C.; Lee, J. Initial self-stress design of tensegrity grid structures. Comput. Struct. 2010, 88, 558–566. [Google Scholar] [CrossRef]
- Oliveto, N.D.; Sivaselvan, M.V. Dynamic analysis of tensegrity structures using a complementary framework. Comput. Struct. 2011, 89, 2471–2483. [Google Scholar] [CrossRef]
- Shekastehband, B.; Abedi, K.; Dianat, N.; Chenaghlou, M.R. Experimental and numerical studies on the collapse behavior of tensegrity systems considering cable rupture and strut collapse with snap-through. Int. J. Nonlin. Mech. 2012, 47, 751–768. [Google Scholar] [CrossRef]




| T | S | C | M | I | D | |
|---|---|---|---|---|---|---|
| ideal tensegrity | + | + | + | + | + | + |
| “pure” tensegrity | + | + | + | + | + | – |
| structures with tensegrity features of class 1 | + | + | + | + | – | – |
| structures with tensegrity features of class 2 | + | + | + | – | + | – |
| – | + |
| No. of Node | x | y | z |
|---|---|---|---|
| 1 | a | 0 | 0 |
| 2 | 0 | 0.5a | a |
| 3 | a | a | 0 |
| 4 | 0.5a | 0 | a |
| 5 | 0 | a | 0 |
| 6 | a | 0.5a | a |
| 7 | 0 | 0 | 0 |
| 8 | 0.5a | a | a |
| No. of Element | No. of First Node | No. of Second Node | |
|---|---|---|---|
| struts | 1 | 1 | 2 |
| 2 | 3 | 4 | |
| 3 | 5 | 6 | |
| 4 | 7 | 8 | |
| bottom cables | 5 | 1 | 3 |
| 6 | 1 | 7 | |
| 7 | 5 | 7 | |
| 8 | 3 | 5 | |
| middle cables | 9 | 3 | 6 |
| 10 | 1 | 4 | |
| 11 | 5 | 8 | |
| 12 | 2 | 7 | |
| upper cables | 13 | 2 | 8 |
| 14 | 2 | 4 | |
| 15 | 4 | 6 | |
| 16 | 6 | 8 |
| No. of Single Modules | Model | No. of Nodes (w) | No. of Elements (n) | Degrees of Freedom (m) | No. of Mechanisms | No. of Self-Stress States | Classification |
|---|---|---|---|---|---|---|---|
| 1 | SM | 8 | 16 | 16 | 1 | 1 | ideal tensegrity |
| 4 | P4-1 | 21 | 56 | 39 | 1 | 18 | structures with tensegrity features of class 1 |
| P4-2 | 45 | 2 | 13 | ||||
| P4-3 | 45 | 3 | 14 | ||||
| P4-4, P4-5 | 48 | 2 | 10 | ||||
| P4-6, P4-7 | 39 | 0 | 17 | structures with tensegrity features of class 2 | |||
| P4-8, P4-9 | 38 | 0 | 18 | ||||
| P4-10 | 39 | 0 | 17 | ||||
| 16 | P16-1 | 69 | 212 | 153 | 1 | 60 | structures with tensegrity features of class 1 |
| P16-2 | 156 | 0 | 56 | structures with tensegrity features of class 2 | |||
| P16-2 | 105 | 0 | 107 | ||||
| P16-3 | 129 | 0 | 83 | ||||
| P16-4 | 122 | 0 | 90 | ||||
| P16-6 | 146 | 1 | 67 | structures with tensegrity features of class 1 | |||
| 64 | P64-1 | 225 | 800 | 579 | 1 | 222 | structures with tensegrity features of class 1 |
| P64-2, P64-3 | 621 | 1 | 180 | ||||
| 4 | PS-1 | 21 | 56 | 39 | 1 | 18 | structures with tensegrity features of class 1 |
| PS-2 | 40 | 0 | 16 | structures with tensegrity features of class 2 | |||
| PS-3 | 27 | 0 | 29 | ||||
| PS-4 | 33 | 0 | 23 | ||||
| PS-5 | 32 | 0 | 24 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Obara, P.; Tomasik, J. Parametric Analysis of Tensegrity Plate-Like Structures: Part 1—Qualitative Analysis. Appl. Sci. 2020, 10, 7042. https://doi.org/10.3390/app10207042
Obara P, Tomasik J. Parametric Analysis of Tensegrity Plate-Like Structures: Part 1—Qualitative Analysis. Applied Sciences. 2020; 10(20):7042. https://doi.org/10.3390/app10207042
Chicago/Turabian StyleObara, Paulina, and Justyna Tomasik. 2020. "Parametric Analysis of Tensegrity Plate-Like Structures: Part 1—Qualitative Analysis" Applied Sciences 10, no. 20: 7042. https://doi.org/10.3390/app10207042
APA StyleObara, P., & Tomasik, J. (2020). Parametric Analysis of Tensegrity Plate-Like Structures: Part 1—Qualitative Analysis. Applied Sciences, 10(20), 7042. https://doi.org/10.3390/app10207042