Development of Energy-Based Impact Formula-Part II: Scabbing Depth, Scabbing Limit, and Perforation Limit
Abstract
:1. Introduction
2. Literature Review
3. Development of Scabbing Depth Formula
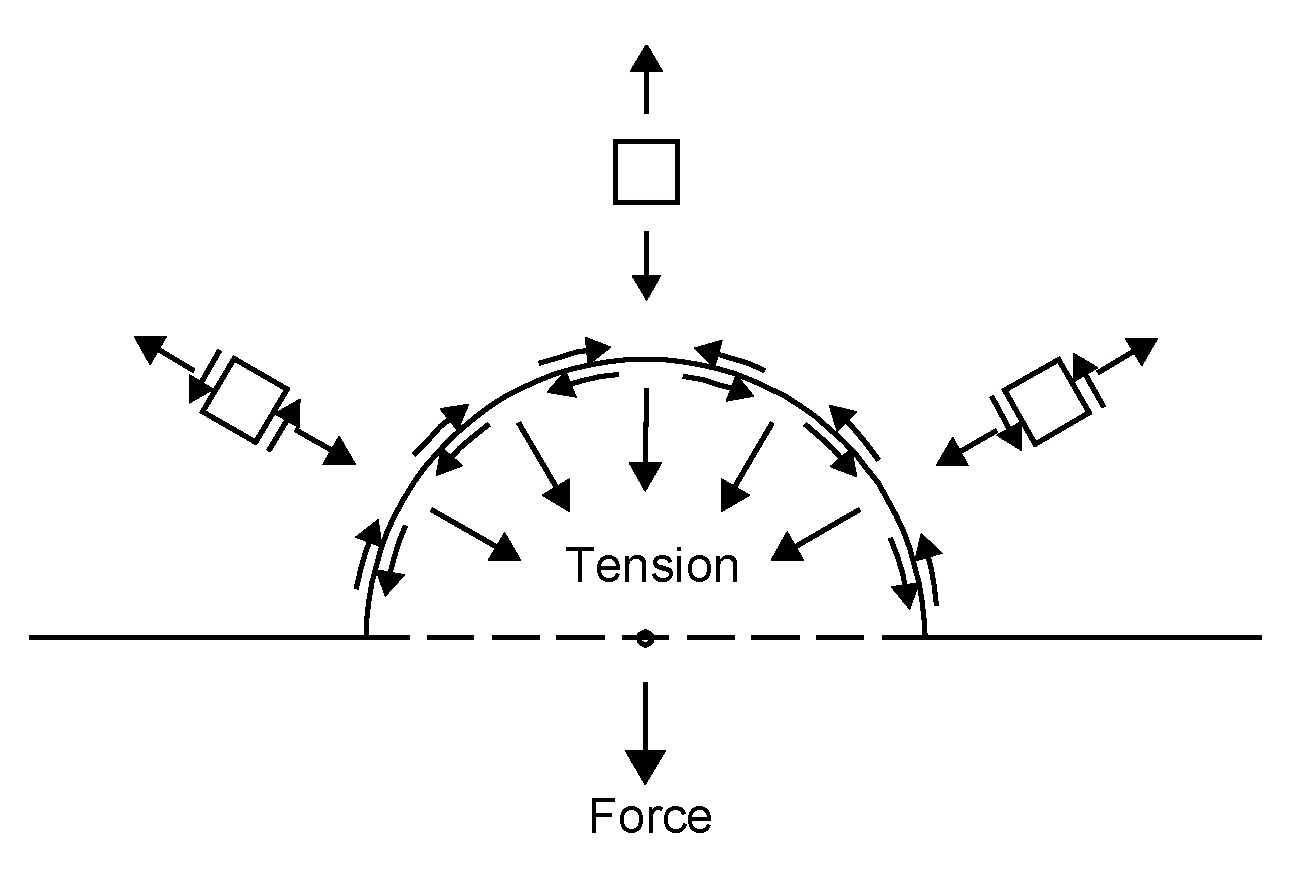
3.1. Scabbing-Resistant Energy
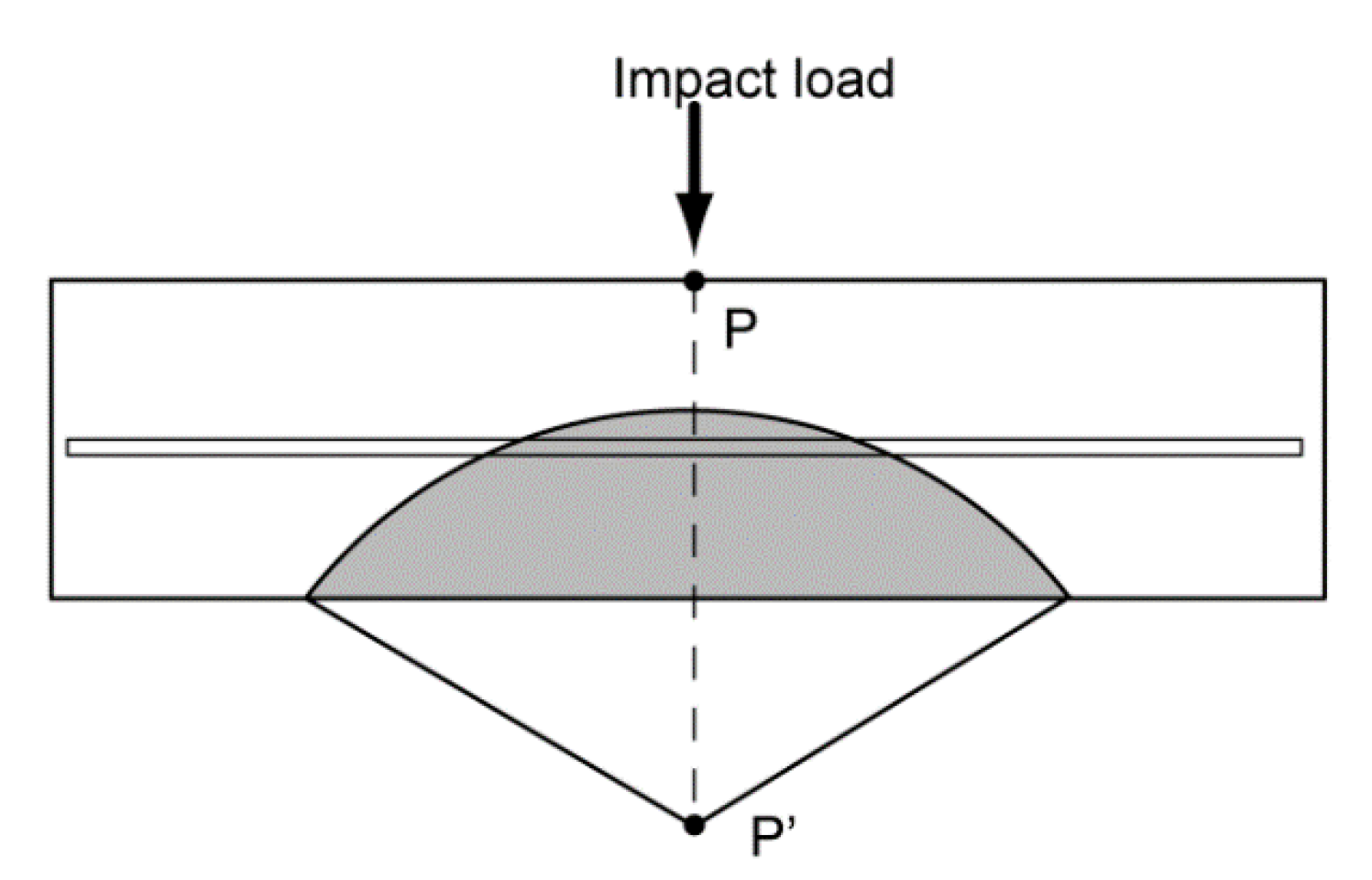
3.2. Derivation of Scabbing Depth Formula
3.3. Derivation of Scabbing Limit Formula
3.4. Derivation of Perforation Limit Formula
4. Verification of Energy-Based Impact Formula
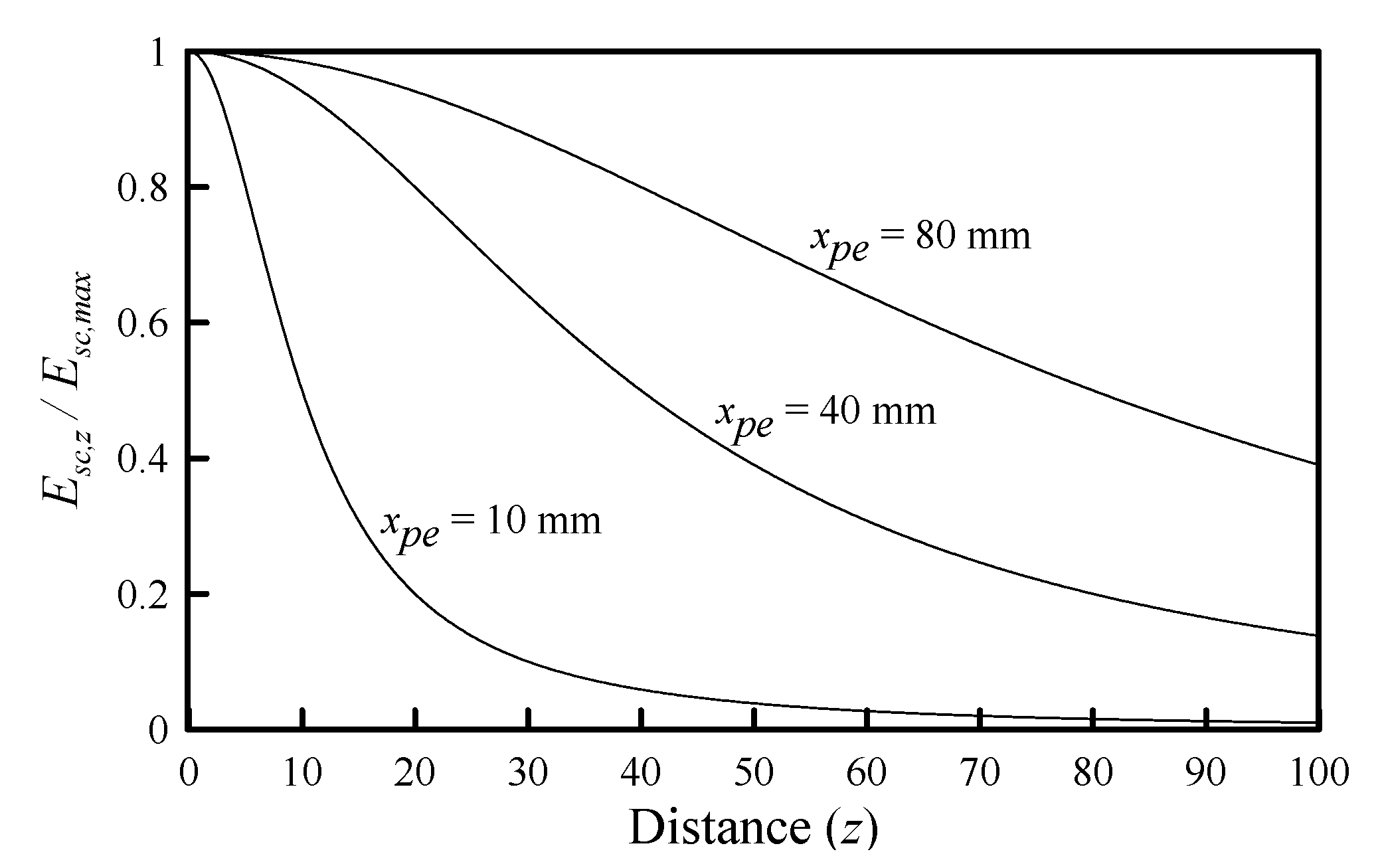
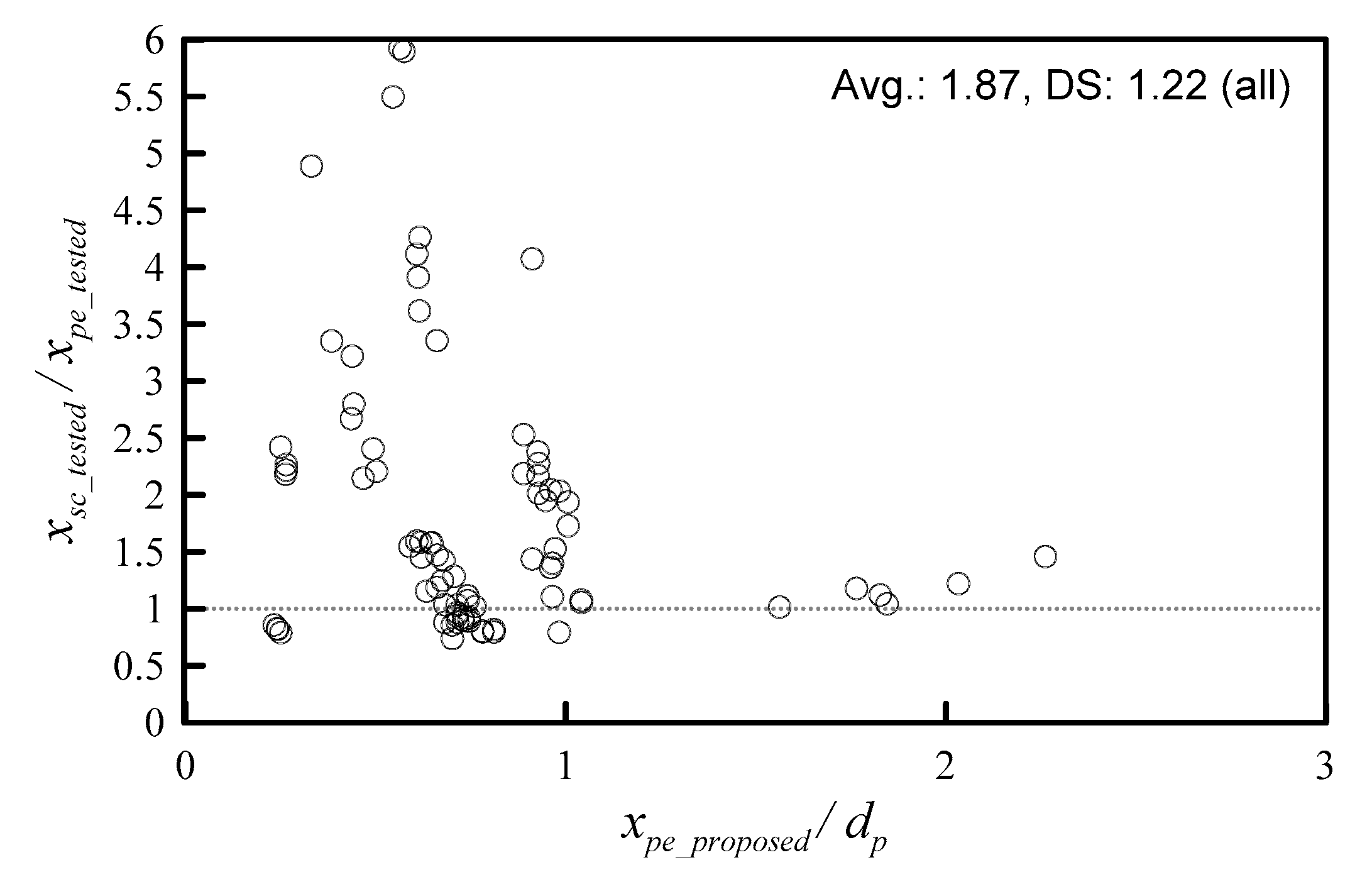
4.1. Scabbing Depth Assessment
4.2. Scabbing Limit Assessment
4.3. Perforation Limit Assessment
5. Conclusions
- The scabbing depth formula is derived using the same concept as that of penetration depth, based on Hertzian contact theory, but is not applied with the energy concentration effect of the impact velocity and projectile shape. The scabbing depth formula is proposed and verified using the experimental data. The mean value of the tested-to-predicted scabbing depth ratio is 1.87 and the standard deviation is 1.22. The scabbing depth formula is less accurate than the penetration depth formula. This is because it is difficult to predict the scabbing depth due to the energy amplified by reinforcing bars and/or aggregate and reflection angle. Despite these challenges, in 52 of the 81 specimens (64%), the scattering ratio is 2 and the average ratio is 1.16. Therefore, the scabbing depth formula is good overall.
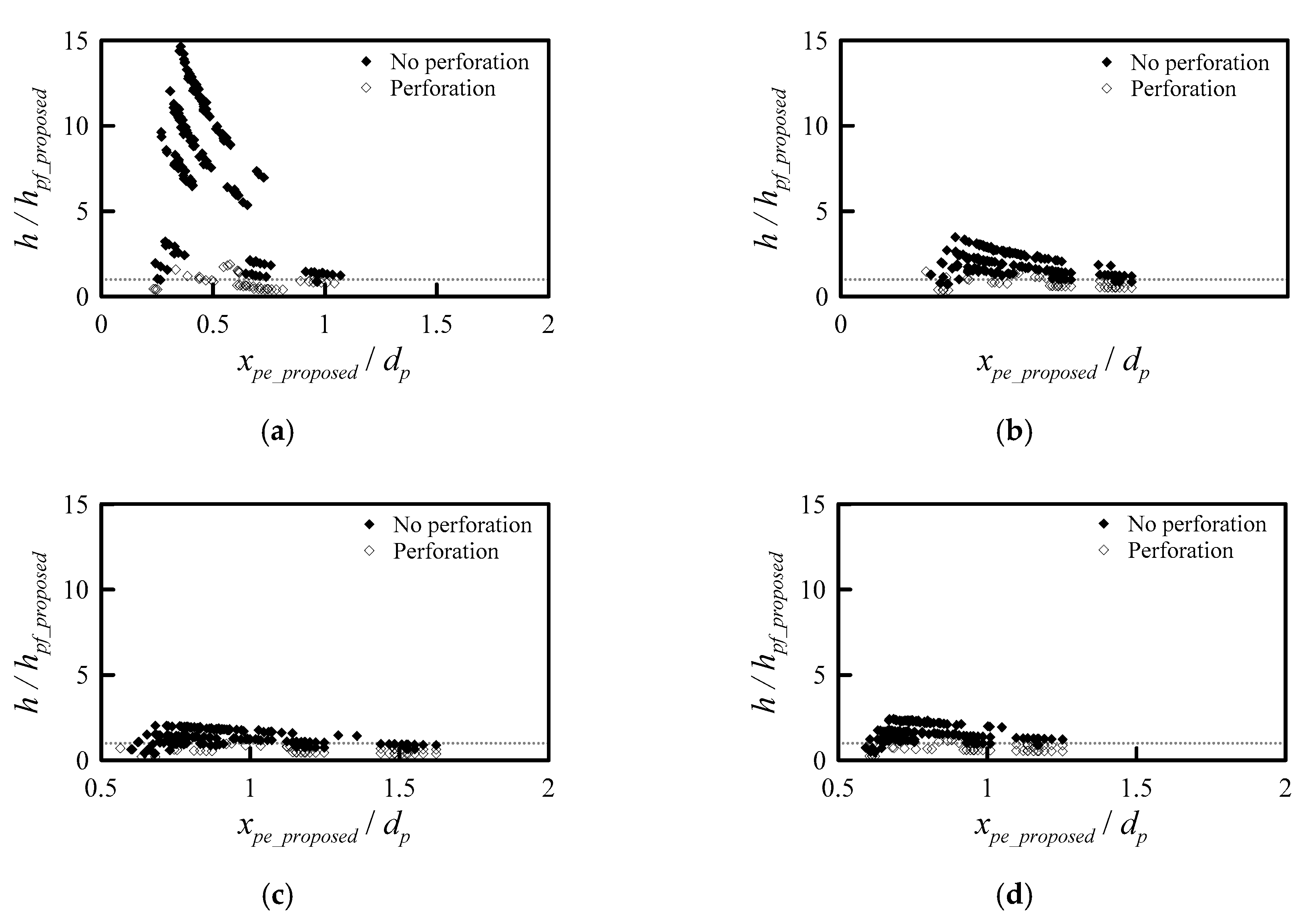
- The scabbing limit formula can be obtained from the scabbing depth formula. The scabbing limit thickness, which is equal to the thickness that makes the scabbing depth zero. The Hughes formula is the best for predicting perforation failure, while the proposed equation is the most conservative. However, the proposed formula is the best for predicting non-perforation, with 99% accuracy. The interface between perforation and non-perforation is properly divided.
- The perforation limit thickness can be obtained from the penetration depth, tunneling depth, and scabbing depth, whose sum is equivalent to the minimum thickness required to prevent perforation. This is equal to the perforation limit thickness. To prevent perforation, the slab thickness should be equal to or greater than the sum of the penetration depth, tunneling depth, and scabbing depth.
- A new formula for predicting the scabbing limit and perforation limit thickness is derived using the theoretical framework and verified using experimental data. The proposed formulae predict all scabbing failures. However, non-scabbing failure is predicted with an accuracy of 81%. This formula is found to be the most accurate.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Probst, A.D.; Kang, T.H.K.; Ramseyer, C.; Kim, U. Composite flexural behavior of full-scale concrete-filled tubes without axial loads. J. Struct. Eng. 2010, 136, 1401–1412. [Google Scholar] [CrossRef]
- Kang, T.H.K.; Kim, W.; Massone, L.M.; Galleguillos, T.A. Shear-flexure coupling behavior of steel fiber-reinforced concrete beams. ACI Struct. J. 2012, 109, 435–444. [Google Scholar] [CrossRef]
- Kang, T.H.K.; Martin, R.D.; Park, H.G.; Wilkerson, R.; Youssef, N. Tall building with steel plate shear walls subject to load reversal. Struct. Des. Tall Spéc. Build. 2011, 22, 500–520. [Google Scholar] [CrossRef]
- Kang, T.H.K.; Ha, S.S.; Choi, D.U. Bar pullout tests and seismic tests of small-headed bars in beam-column joints. ACI Struct. J. 2010, 107, 32–42. [Google Scholar]
- Hong, S.; Kang, T.H.K. Dynamic strength properties of concrete and reinforcing steel subject to extreme loads. ACI Struct. J. 2016, 113, 983–995. [Google Scholar] [CrossRef]
- Kim, S.; Kang, T.H.K.; Yun, H.D. Evaluation of impact resistance of steel fiber-reinforced concrete panels using design equations. ACI Struct. J. 2017, 114, 911–921. [Google Scholar] [CrossRef]
- Li, Q.; Reid, S.; Wen, H.; Telford, A. Local impact effects of hard missiles on concrete targets. Int. J. Impact Eng. 2005, 32, 224–284. [Google Scholar] [CrossRef]
- Kim, S.; Kang, T.H.K.; Jang, S.J.; Kim, K.S.; Yun, H.D. High-velocity impact experiment of concrete panels reinforced with crimped wire mesh and steel fibers. Struct. Concr. 2018, 19, 1818–1828. [Google Scholar] [CrossRef]
- ACE. Fundamentals of Protective Structures (Report No. AT120 AT1207821); Army Corps of Engineering: Washington, DC, USA, 1946. [Google Scholar]
- White, M.P. Effects of Impact and Explosion; Defense Technical Information Center (DTIC): Fort Belvoir, VA, USA, 1946; Volume 1. [Google Scholar]
- Kennedy, R. A review of procedures for the analysis and design of concrete structures to resist missile impact effects. Nucl. Eng. Des. 1976, 37, 183–203. [Google Scholar] [CrossRef]
- Hwang, H.J.; Kim, S.; Kang, T.H.K. Energy-based penetration model for local impact-damaged concrete members. ACI Struct. J. 2017, 114, 1189–1200. [Google Scholar] [CrossRef]
- Luk, V.; Forrestal, M. Penetration into semi-infinite reinforced-concrete targets with spherical and ogival nose projectiles. Int. J. Impact Eng. 1987, 6, 291–301. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Luk, V.K. Dynamic spherical cavity-expansion in a compressible elastic-plastic solid. J. Appl. Mech. 1988, 55, 275–279. [Google Scholar] [CrossRef]
- Haldar, A.; Miller, F.J. Penetration depth in concrete for nondeformable missiles. Nucl. Eng. Des. 1982, 71, 79–88. [Google Scholar] [CrossRef]
- Haldar, A.; Hamieh, H.A. Local effect of solid missiles on concrete structures. J. Struct. Eng. 1984, 110, 948–960. [Google Scholar] [CrossRef]
- Hughes, G. Hard missile impact on reinforced concrete. Nucl. Eng. Des. 1984, 77, 23–35. [Google Scholar] [CrossRef]
- Barr, P. Guidelines for the Design and Assessment of Concrete Structures subjected to Impact (Report No. SRD-R-439); UK Atomic Energy Authority, Safety and Reliability Directorate: Abingdon, UK, 1990.
- Reid, S.R.; Wen, H.M. Predicting Penetration, Cone Cracking, Scabbing and Perforation of Reinforced Concrete Targets Struck by Flat-faced Projectiles (Report No. ME/AM/02.01/TE/G/018507/Z); University of Manchester institute of Science and Technology (UMIST): Manchester, UK, 2001. [Google Scholar]
- BNFL. Appendix H Reinforced concrete slab local damage assessment. In R3 Impact Assessment Procedure; Magnox Electric plc & Nuclear Electric Limited: Berkely, UK, 2003; Volume 3. [Google Scholar]
- Li, Q.M.; Tong, D.J. Perforation thickness and ballistic limit of concrete target subjected to rigid projectile impact. J. Eng. Mech. 2003, 129, 1083–1091. [Google Scholar] [CrossRef]
- Kim, S.; Kang, T.H.K. Development of energy-based impact formula—Part I: Penetration depth. Appl. Sci. 2020, 10, 4964. [Google Scholar] [CrossRef]
- Park, H.G.; Choi, K.K.; Chung, L. Strain-based strength model for direct punching shear of interior slab–column connections. Eng. Struct. 2011, 33, 1062–1073. [Google Scholar] [CrossRef]
- Choi, K.K.; Shin, D.W.; Park, H.G. Shear strength model for slab-column connections subjected to unbalanced moment. ACI Struct. J. 2014, 111, 491–502. [Google Scholar] [CrossRef]
- fib. Model Code for Concrete Structures 2010 (fib Model Code 2010); Ernst & Sohn: Berlin, Germany, 2010. [Google Scholar]
- Society of Material Science of Japan. Impact Engineering; Nikkan Kogyo Shimbun Ltd.: Tokyo, Japan, 1998. (In Japanese) [Google Scholar]
- Williams, J.A.; Dwyer-Joyce, R.S. Contact Between Solid Surfaces, Chapter 3 in Modern Tribology Hanbook, Two Volume Set; Bhushan, B., Ed.; CRC Press: Boca Raton, FL, USA, 2000; pp. 121–162. [Google Scholar]
- Abdel-Kader, M.; Fouda, A. Effect of reinforcement on the response of concrete panels to impact of hard projectiles. Int. J. Impact Eng. 2014, 63, 1–17. [Google Scholar] [CrossRef]
- Kim, S. Impact Resistance of High Performance Concrete Panels under High Velocity Projectile Collision. Ph.D. Thesis, Seoul National University, Seoul, Korea, August 2018. [Google Scholar]
- Williams, M.S. Modeling of local impact effects on plain and reinforced concrete. ACI Struct. J. 1994, 91, 178–187. [Google Scholar] [CrossRef]



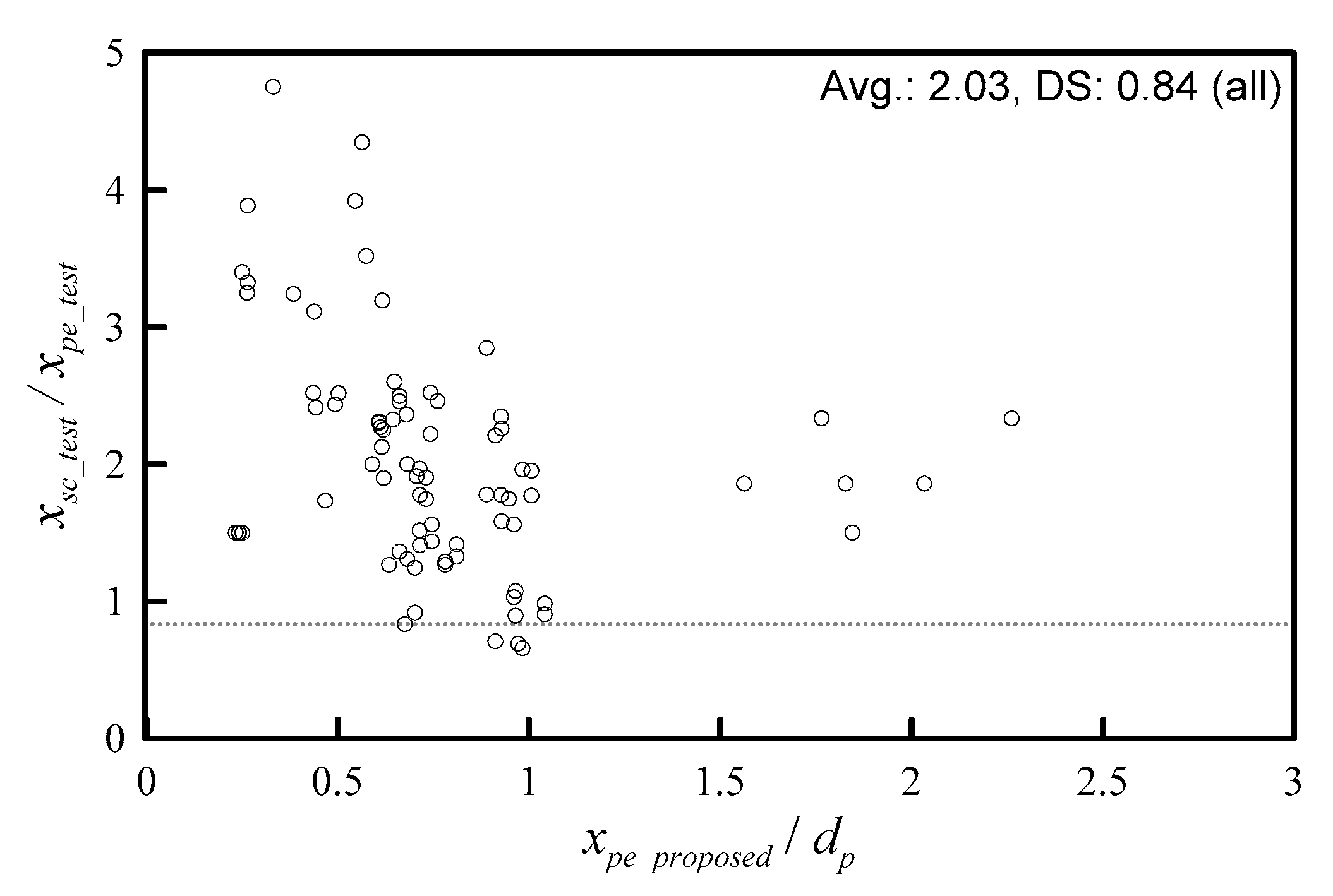

| Ratio Range | Number of Specimens | Average Ratio |
|---|---|---|
| Under 1 | 19 | 0.85 |
| Under 1.5 | 43 | 1.05 |
| Under 2 | 52 | 1.16 |
| Over 2 | 29 | 3.14 |
| Formula | Scabbing Failure (Predicted/Tested) | No Scabbing Failure (Predicted/Tested) |
|---|---|---|
| Proposed | 100% (75 of 75 specimens) | 81% (139 of 172 specimens) |
| Modified NDRC | 100% (75 of 75 specimens) | 66% (113 of 172 specimens) |
| Hughes | 100% (75 of 75 specimens) | 43% (74 of 172 specimens) |
| ACE | 100% (75 of 75 specimens) | 66% (113 of 172 specimens) |
| Formula | Perforation Failure | No Perforation Failure |
|---|---|---|
| Proposed | 63% (41 of 65 specimens) | 99% (180 of 182 specimens) |
| Modified NDRC | 77% (50 of 65 specimens) | 93% (170 of 182 specimens) |
| Hughes | 100% (65 of 65 specimens) | 67% (125 of 182 specimens) |
| ACE | 88% (57 of 65 specimens) | 91% (166 of 182 specimens) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, S.; Kang, T.H.-K. Development of Energy-Based Impact Formula-Part II: Scabbing Depth, Scabbing Limit, and Perforation Limit. Appl. Sci. 2020, 10, 5481. https://doi.org/10.3390/app10165481
Kim S, Kang TH-K. Development of Energy-Based Impact Formula-Part II: Scabbing Depth, Scabbing Limit, and Perforation Limit. Applied Sciences. 2020; 10(16):5481. https://doi.org/10.3390/app10165481
Chicago/Turabian StyleKim, Sanghee, and Thomas H.-K. Kang. 2020. "Development of Energy-Based Impact Formula-Part II: Scabbing Depth, Scabbing Limit, and Perforation Limit" Applied Sciences 10, no. 16: 5481. https://doi.org/10.3390/app10165481
APA StyleKim, S., & Kang, T. H.-K. (2020). Development of Energy-Based Impact Formula-Part II: Scabbing Depth, Scabbing Limit, and Perforation Limit. Applied Sciences, 10(16), 5481. https://doi.org/10.3390/app10165481






