Robust Phase Estimation of Gaussian States in the Presence of Outlier Quantum States
Abstract
1. Introduction
2. Preliminaries
2.1. M-estimator
2.2. Tuning Parameter
2.3. Iterative Algorithm
3. Robustness of M-estimator
3.1. Classical Contaminated Model
3.2. Asymptotic Breakdown Point
3.3. Finite Breakdown Point
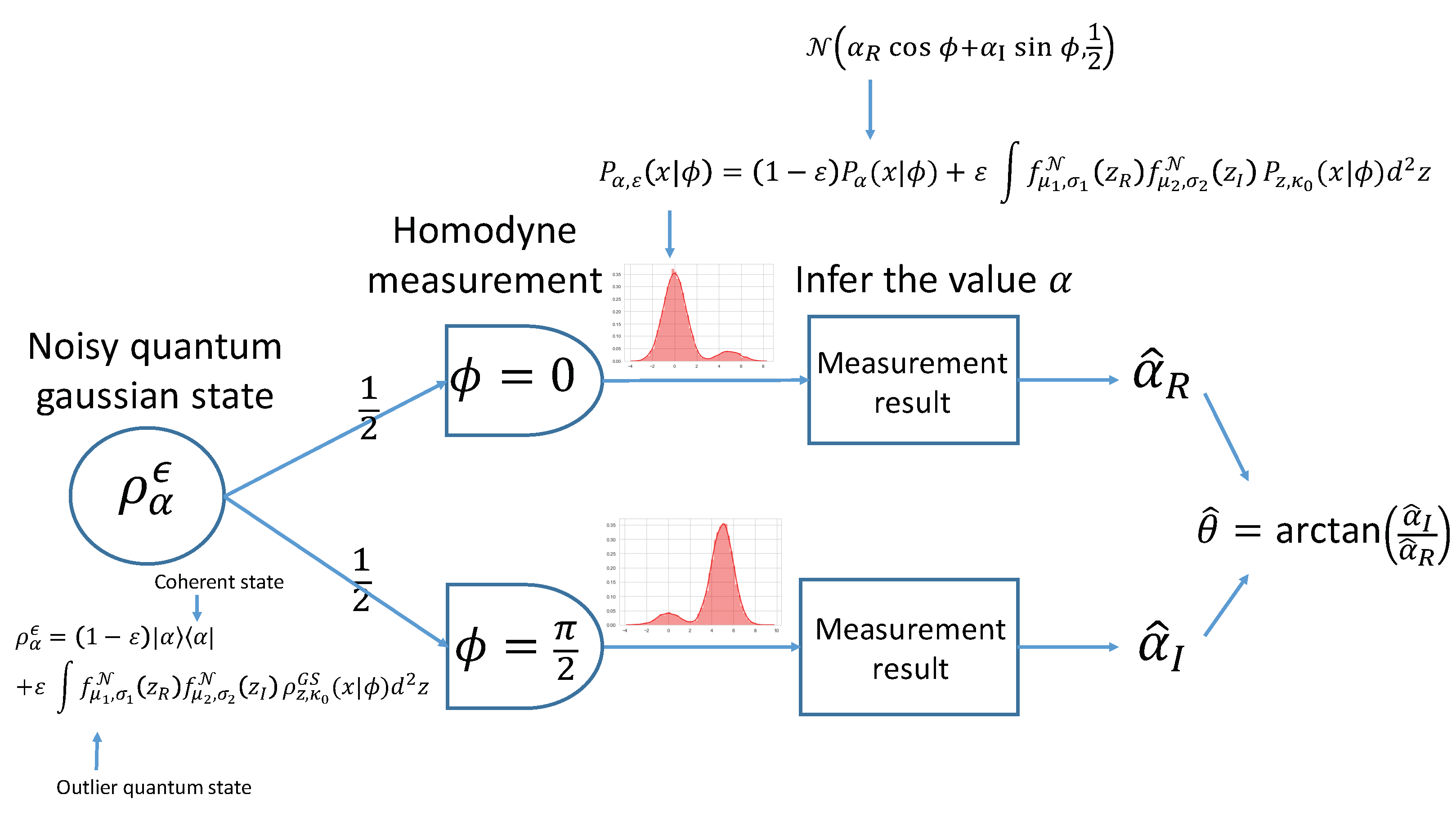
4. Quantum Statistical Model with Outliers
4.1. Quantum Statistical Model with Outlier Quantum States
4.2. Quantum Gaussian State with Outliers
4.3. Homodyne Measurement on the Noisy Quantum Gaussian States
5. Phase Estimation of Noisy Coherent State
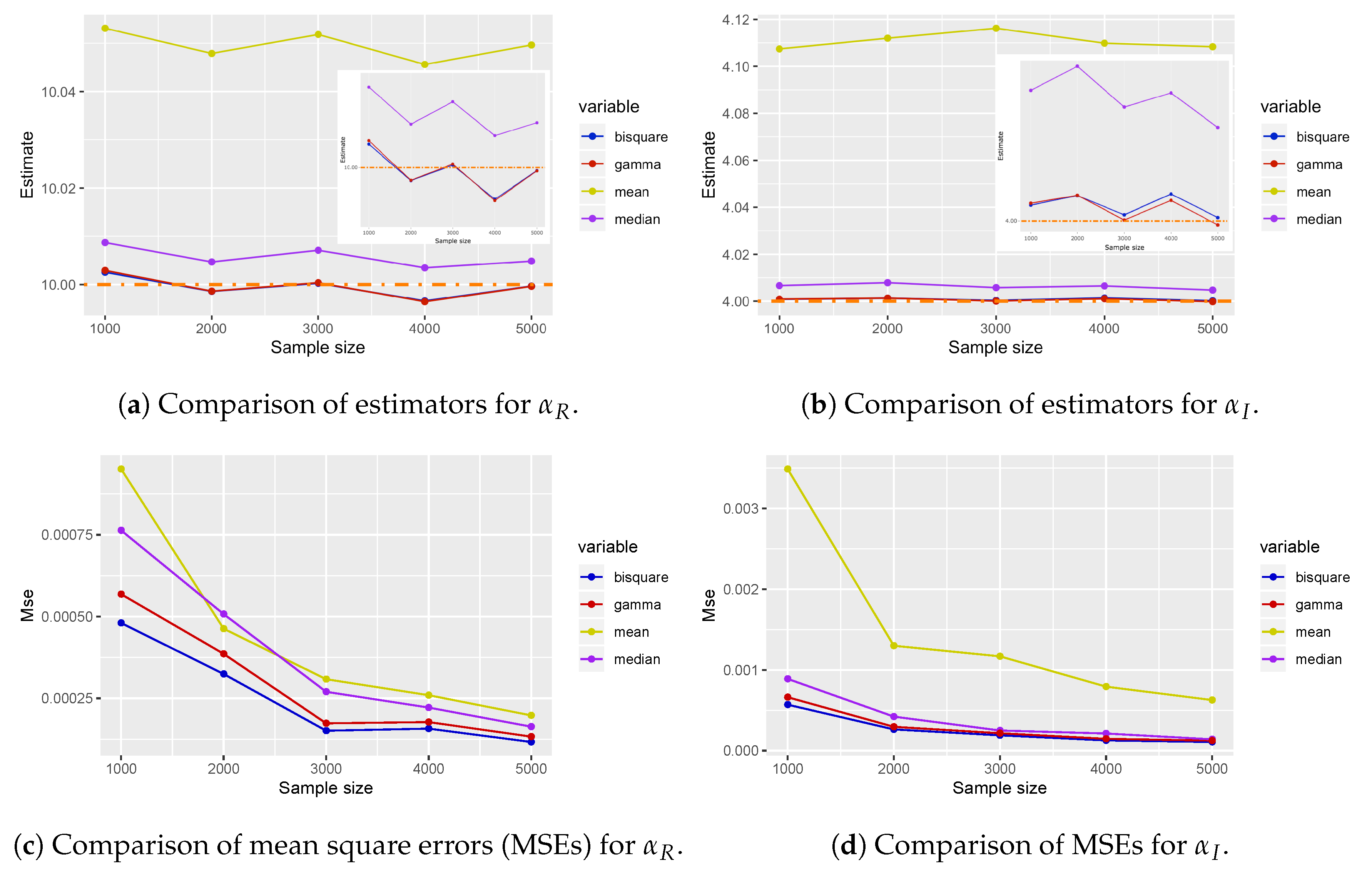
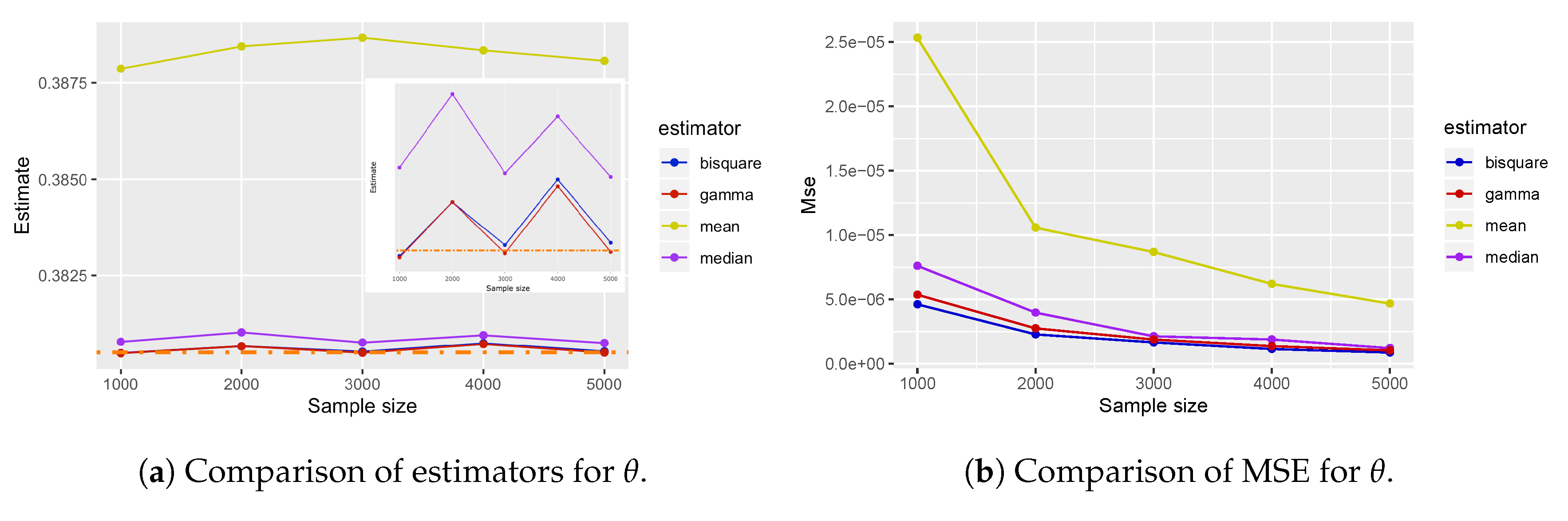
5.1. Numerical Simulation
5.2. Single Outlier Quantum State
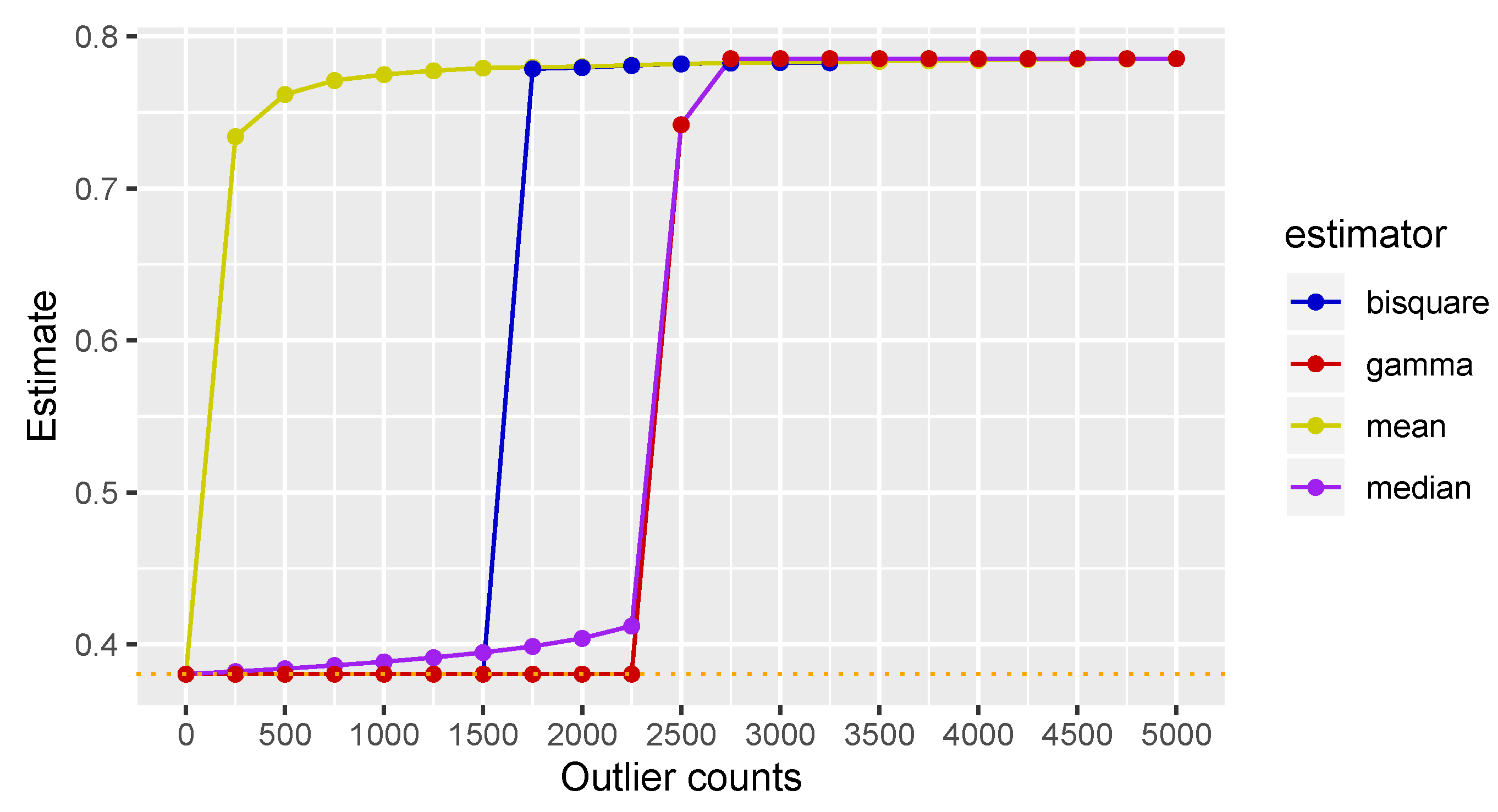
5.3. Robustness of M-estimators
5.4. Distributed Outlier Quantum States
5.5. Discussion
6. Conclusions and Outlook
Author Contributions
Funding
Conflicts of Interest
References
- Merkel, S.T.; Gambetta, J.M.; Smolin, J.A.; Poletto, S.; Córcoles, A.D.; Johnson, B.R.; Ryan, C.A.; Steffen, M. Self-consistent quantum process tomography. Phys. Rev. A 2013, 87, 062119. [Google Scholar] [CrossRef]
- Ferrie, C. Self-guided quantum tomography. Phys. Rev. Lett. 2014, 113, 190404. [Google Scholar] [CrossRef] [PubMed]
- Sugiyama, T.; Imori, S.; Tanaka, F. Reliable characterization of super-accurate quantum operations. arXiv 2018, arXiv:1806.02696. [Google Scholar]
- Huber, P.J. Robust Statistics; John Wiley & Sons: Hoboken, NJ, USA, 2004; Volume 523. [Google Scholar]
- Andrews, D.; Hampel, F. Robust Estimates of Location: Survey and Advances; Princeton University Press: Princeton, NJ, USA, 2015. [Google Scholar]
- Wilcox, R.R. Introduction to Robust Estimation and Hypothesis Testing; Academic Press: Cambridge, MA, USA, 2011. [Google Scholar]
- Hampel, F.R.; Ronchetti, E.M.; Rousseeuw, P.J.; Stahel, W.A. Robust Statistics: The Approach Based on Influence Functions; John Wiley & Sons: Hoboken, NJ, USA, 2011; Volume 196. [Google Scholar]
- Maronna, R.A.; Martin, R.D.; Yohai, V.J.; Salibián-Barrera, M. Robust Statistics: Theory and Methods (with R); John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Wasserstein, R.L.; Lazar, N.A. The ASA Statement on p-Values: Context, Process, and Purpose. Am. Stat. 2016, 70, 129–133. [Google Scholar] [CrossRef]
- Camerer, C.F.; Dreber, A.; Holzmeister, F.; Ho, T.H.; Huber, J.; Johannesson, M.; Kirchler, M.; Nave, G.; Nosek, B.A.; Pfeiffer, T.; et al. Evaluating the replicability of social science experiments in Nature and Science between 2010 and 2015. Nat. Hum. Behav. 2018, 2, 637–644. [Google Scholar] [CrossRef]
- Wasserstein, R.L.; Schirm, A.L.; Lazar, N.A. Moving to a World Beyond “p < 0.05”. Am. Stat. 2019, 73, 1–19. [Google Scholar] [CrossRef]
- Braunstein, S.L.; Van Loock, P. Quantum information with continuous variables. Rev. Mod. Phys. 2005, 77, 513. [Google Scholar] [CrossRef]
- Wang, X.B.; Hiroshima, T.; Tomita, A.; Hayashi, M. Quantum information with Gaussian states. Phys. Rep. 2007, 448, 1–111. [Google Scholar] [CrossRef]
- Serafini, A. Quantum Continuous Variables: A Primer of Theoretical Methods; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Fujisawa, H.; Eguchi, S. Robust parameter estimation with a small bias against heavy contamination. J. Multivar. Anal. 2008, 99, 2053–2081. [Google Scholar] [CrossRef]
- Helstrom, C.W. The minimum variance of estimates in quantum signal detection. IEEE Trans. Inf. Theory 1968, 14, 234–242. [Google Scholar] [CrossRef]
- Helstrom, C.W. Quantum detection and estimation theory. J. Stat. Phys. 1969, 1, 231–252. [Google Scholar] [CrossRef]
- Helstrom, C.W.; Kennedy, R. Noncommuting observables in quantum detection and estimation theory. IEEE Trans. Inf. Theory 1974, 20, 16–24. [Google Scholar] [CrossRef]
- Yuen, H.; Lax, M. Multiple-parameter quantum estimation and measurement of nonselfadjoint observables. IEEE Trans. Inf. Theory 1973, 19, 740–750. [Google Scholar] [CrossRef]
- Helstrom, C.W. Quantum Detection and Estimation Theory; Academic Press: Cambridge, MA, USA, 1976. [Google Scholar]
- Holevo, A.S. Probabilistic and Statistical Aspects of Quantum Theory; Edizioni della Normale: Pisa, Italy, 2011. [Google Scholar]
- Fujiwara, A.; Nagaoka, H. An estimation theoretical characterization of coherent states. J. Math. Phys. 1999, 40, 4227–4239. [Google Scholar] [CrossRef]
- D’ariano, G.M.; Paris, M.G.; Sacchi, M.F. Parameter estimation in quantum optics. Phys. Rev. A 2000, 62, 023815. [Google Scholar] [CrossRef]
- Paris, M.G.A.; Řeháček, J.E. Quantum State Estimation; Springer: Berlin, Germany, 2004. [Google Scholar]
- Hayashi, M. Quantum Information Theory. In Graduate Texts in Physics; Springer: Berlin, Germany, 2017. [Google Scholar]
- Suzuki, J. Nuisance parameter problem in quantum estimation theory: Tradeoff relation and qubit examples. J. Phys. A: Math. Theor. 2020, 53, 264001. [Google Scholar] [CrossRef]
- Suzuki, J.; Yang, Y.; Hayashi, M. Quantum state estimation with nuisance parameters. J. Phys. A Math. Theor. 2020. [Google Scholar] [CrossRef]
- Aspachs, M.; Calsamiglia, J.; Muñoz-Tapia, R.; Bagan, E. Phase estimation for thermal Gaussian states. Phys. Rev. A 2009, 79, 033834. [Google Scholar] [CrossRef]
- Pinel, O.; Jian, P.; Treps, N.; Fabre, C.; Braun, D. Quantum parameter estimation using general single-mode Gaussian states. Phys. Rev. A 2013, 88, 040102. [Google Scholar] [CrossRef]
- Bradshaw, M.; Lam, P.K.; Assad, S.M. Ultimate precision of joint quadrature parameter estimation with a Gaussian probe. Phys. Rev. A 2018, 97, 012106. [Google Scholar] [CrossRef]
- Oh, C.; Lee, C.; Rockstuhl, C.; Jeong, H.; Kim, J.; Nha, H.; Lee, S.Y. Optimal Gaussian measurements for phase estimation in single-mode Gaussian metrology. Npj Quantum Inf. 2019, 5, 1–9. [Google Scholar] [CrossRef]
- Lee, C.; Oh, C.; Jeong, H.; Rockstuhl, C.; Lee, S.Y. Using states with a large photon number variance to increase quantum Fisher information in single-mode phase estimation. J. Phys. Commun. 2019, 3, 115008. [Google Scholar] [CrossRef]
- Arnhem, M.; Karpov, E.; Cerf, N.J. Optimal Estimation of Parameters Encoded in Quantum Coherent State Quadratures. Appl. Sci. 2019, 9, 4264. [Google Scholar] [CrossRef]
- Oh, C.; Lee, C.; Lie, S.H.; Jeong, H. Optimal distributed quantum sensing using Gaussian states. Phys. Rev. Res. 2020, 2, 023030. [Google Scholar] [CrossRef]
- Assad, S.M.; Li, J.; Liu, Y.; Zhao, N.; Zhao, W.; Lam, P.K.; Ou, Z.; Li, X. Accessible precisions for estimating two conjugate parameters using Gaussian probes. Phys. Rev. Res. 2020, 2, 023182. [Google Scholar] [CrossRef]
- Donoho, D.L.; Huber, P.J. The notion of breakdown point. In A Festschrift for Erich L. Lehmann; CRC Press: Boca Raton, FL, USA, 1983; pp. 157–184. [Google Scholar]
- Huber, P.J. Finite sample breakdown of M-and P-estimators. Ann. Stat. 1984, 12, 119–126. [Google Scholar] [CrossRef]
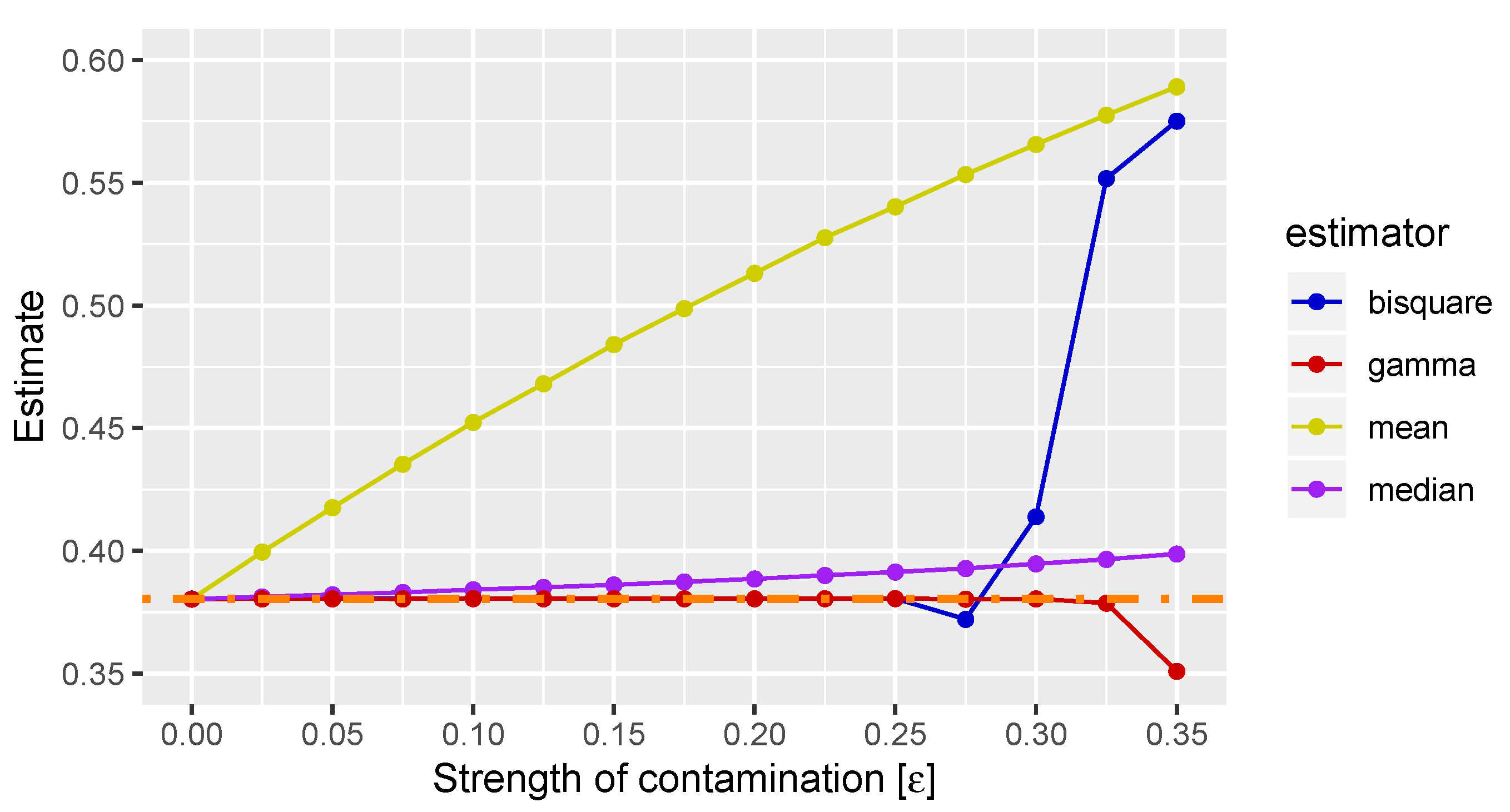
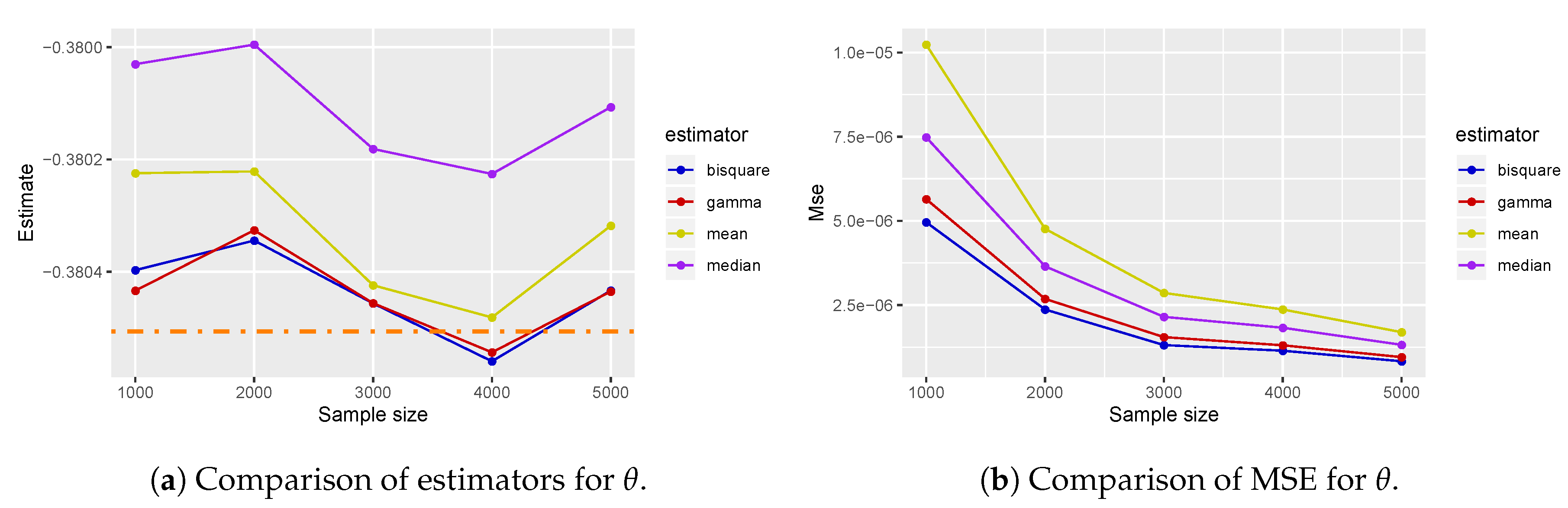
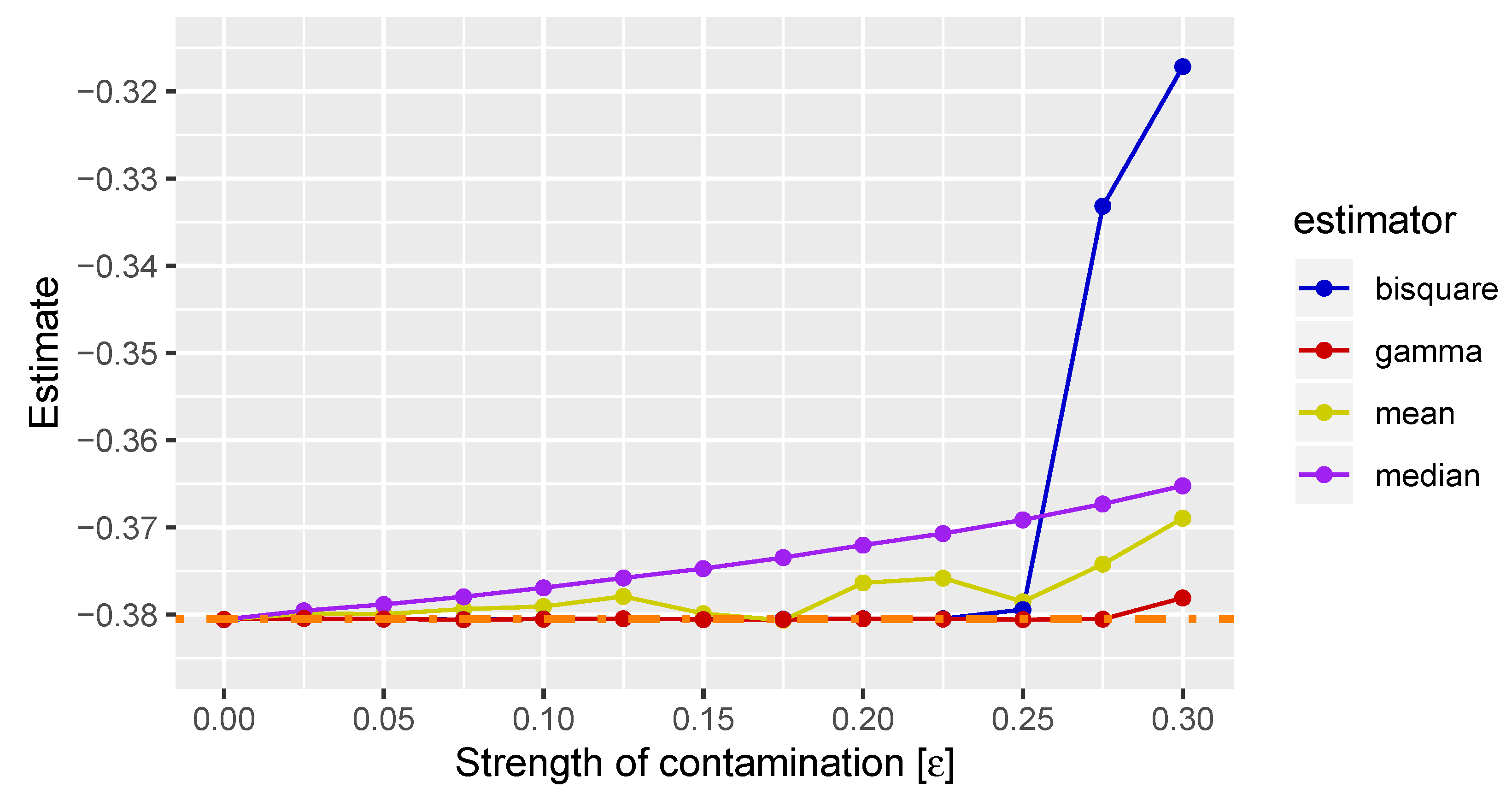
| 0 | 0.025 | 0.05 | 0.075 | 0.1 | 0.125 | 0.15 | 0.175 | 0.2 | 0.225 | 0.25 | 0.275 | 0.3 | 0.325 | 0.35 | |
| bisquare | 3.21 | 5.00 | 5.01 | 5.00 | 5.00 | 5.11 | 5.99 | 6.00 | 6.08 | 7.11 | 9.07 | 20.02 | 11.00 | 6.67 | 5.29 |
| gamma | 7.97 | 7.99 | 7.99 | 8.00 | 8.02 | 8.04 | 8.12 | 8.28 | 8.57 | 8.85 | 9.00 | 9.20 | 9.89 | 11.60 | 17.77 |
| 0 | 0.025 | 0.05 | 0.075 | 0.1 | 0.125 | 0.15 | 0.175 | 0.2 | 0.225 | 0.25 | 0.275 | 0.3 | 0.325 | 0.35 | |
| bisquare | 3.19 | 5.00 | 5.00 | 4.96 | 4.05 | 4.67 | 5.00 | 5.00 | 5.41 | 6.06 | 7.07 | 9.64 | 20.06 | 9.99 | 6.50 |
| gamma | 7.97 | 7.99 | 8.01 | 8.01 | 8.02 | 8.02 | 8.05 | 8.09 | 8.09 | 8.12 | 8.18 | 8.20 | 8.21 | 8.23 | 8.38 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mototake, Y.; Suzuki, J. Robust Phase Estimation of Gaussian States in the Presence of Outlier Quantum States. Appl. Sci. 2020, 10, 5475. https://doi.org/10.3390/app10165475
Mototake Y, Suzuki J. Robust Phase Estimation of Gaussian States in the Presence of Outlier Quantum States. Applied Sciences. 2020; 10(16):5475. https://doi.org/10.3390/app10165475
Chicago/Turabian StyleMototake, Yukito, and Jun Suzuki. 2020. "Robust Phase Estimation of Gaussian States in the Presence of Outlier Quantum States" Applied Sciences 10, no. 16: 5475. https://doi.org/10.3390/app10165475
APA StyleMototake, Y., & Suzuki, J. (2020). Robust Phase Estimation of Gaussian States in the Presence of Outlier Quantum States. Applied Sciences, 10(16), 5475. https://doi.org/10.3390/app10165475





