A Novel Negative Stiffness Amplification System Based Isolation Method for the Vibration Control of Underground Structures
Abstract
1. Introduction
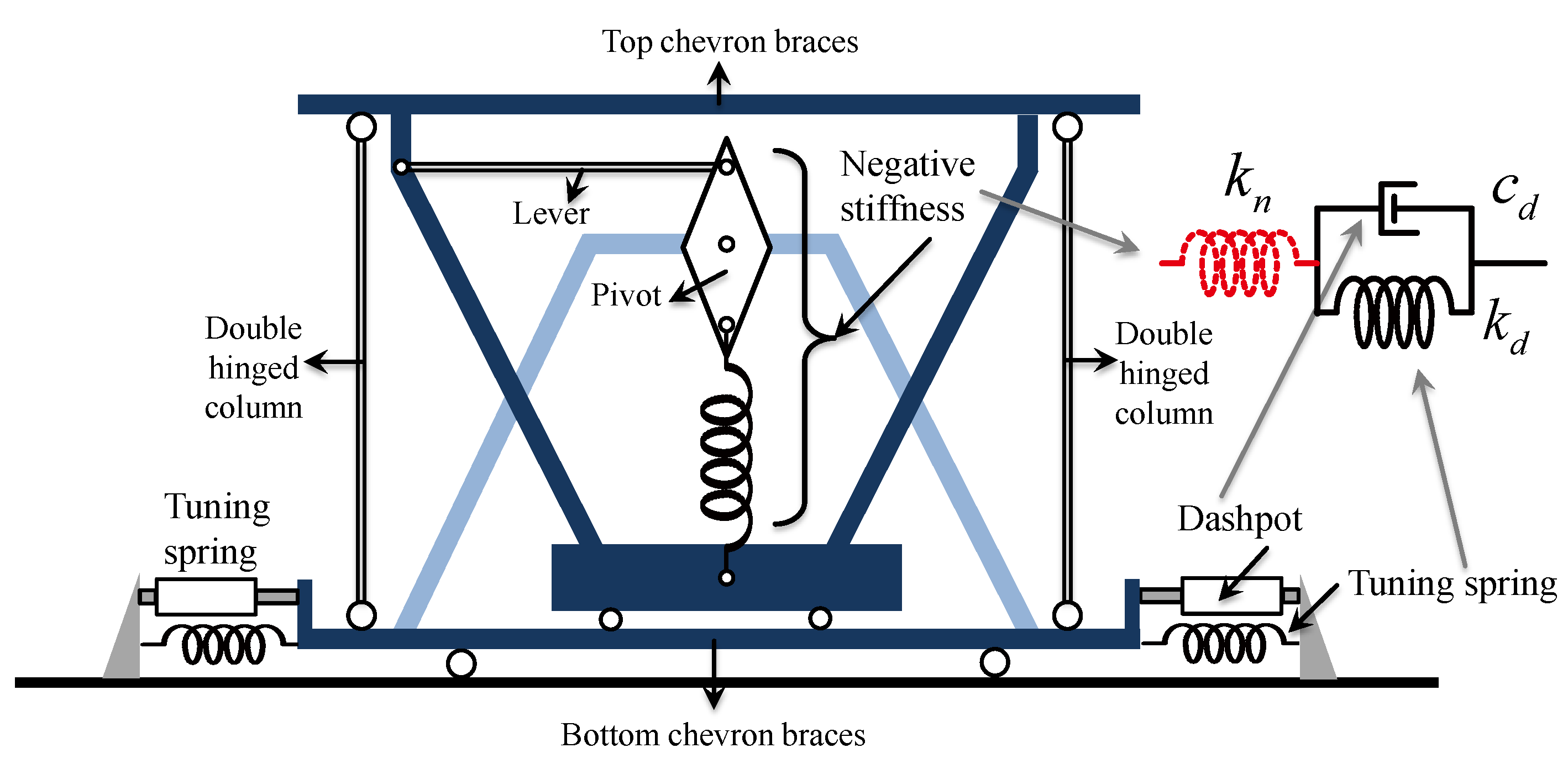
2. NSAS
2.1. NSAS Model
2.2. Governing Equation
2.3. Energy-Dissipation-Enhancement Effect
3. NSAS-Based Underground Structure
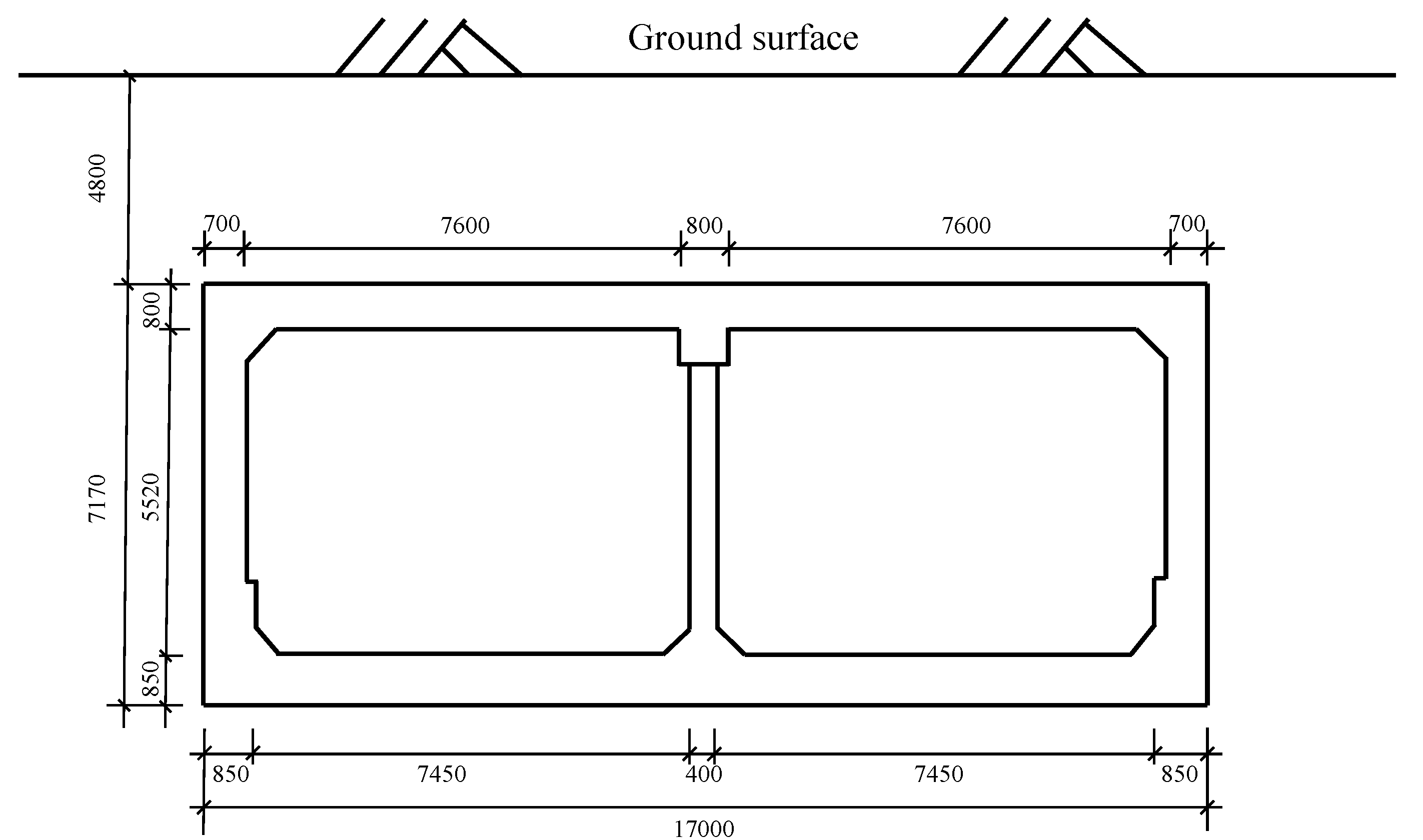
3.1. Mechanical Model
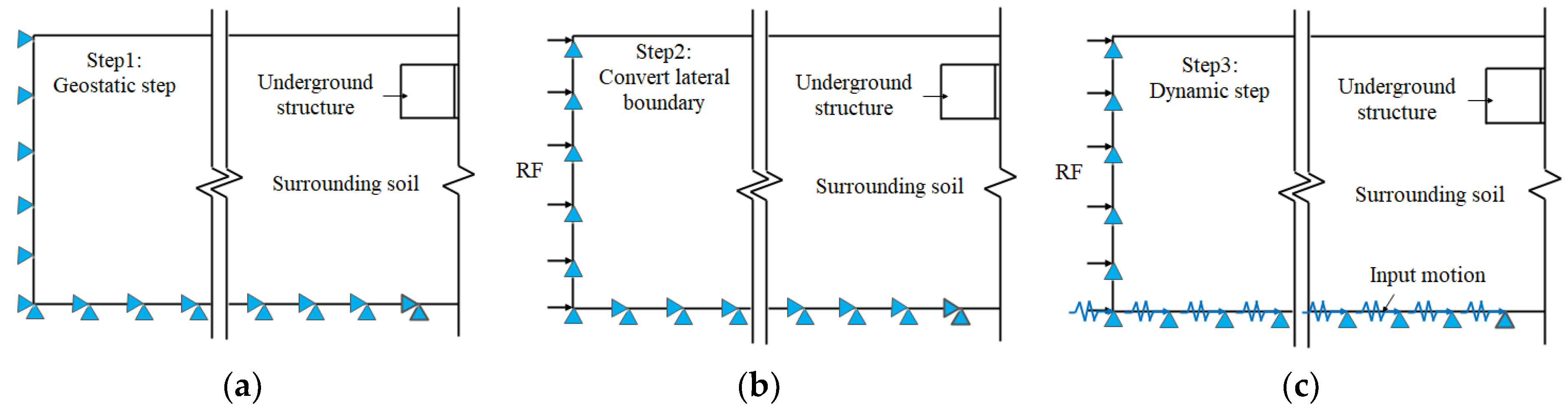
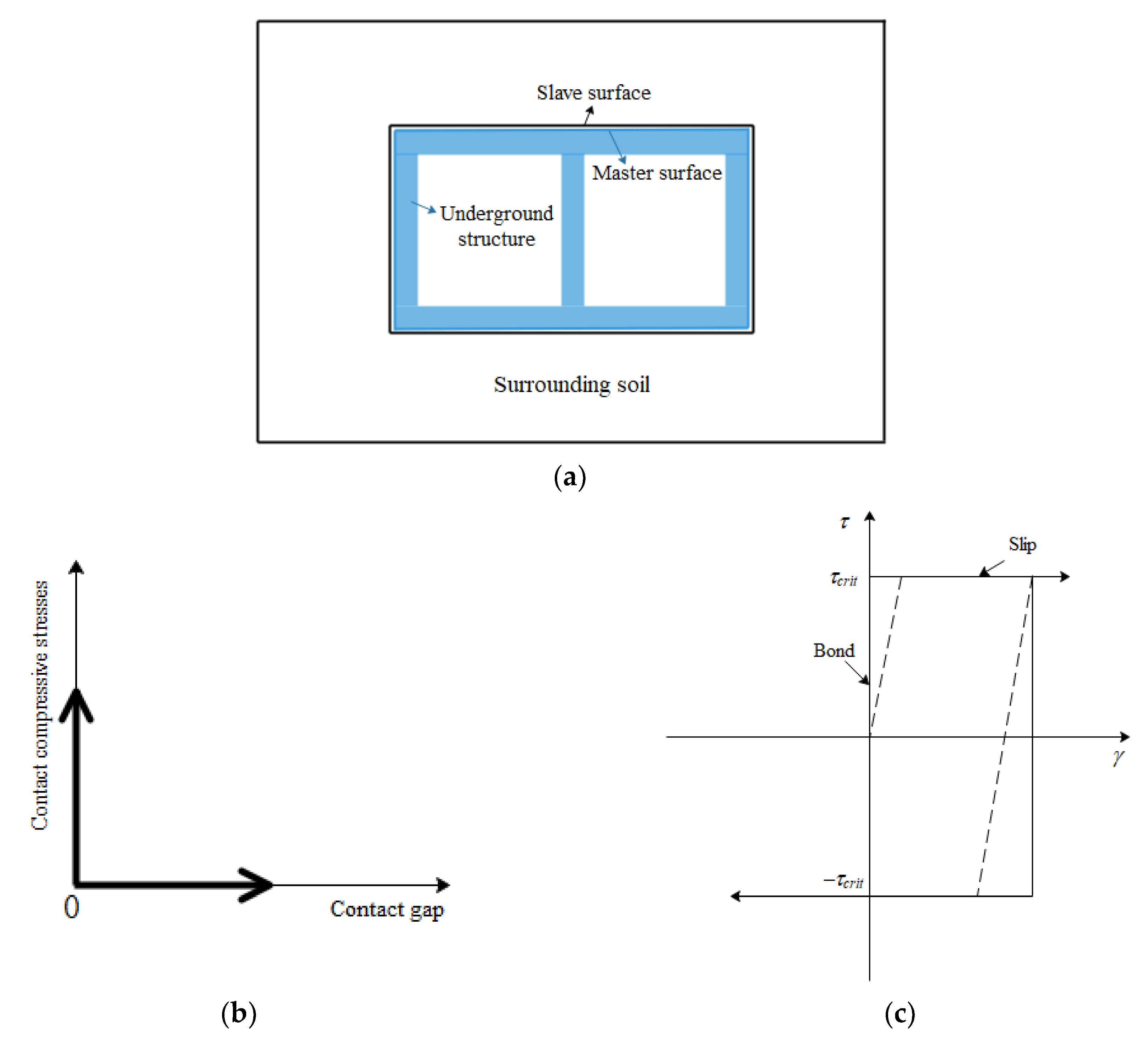
3.2. Soil and Boundary Conditions
4. NSAS Effectiveness for Underground Structures
4.1. Measurement Definition
4.2. Displacement Control
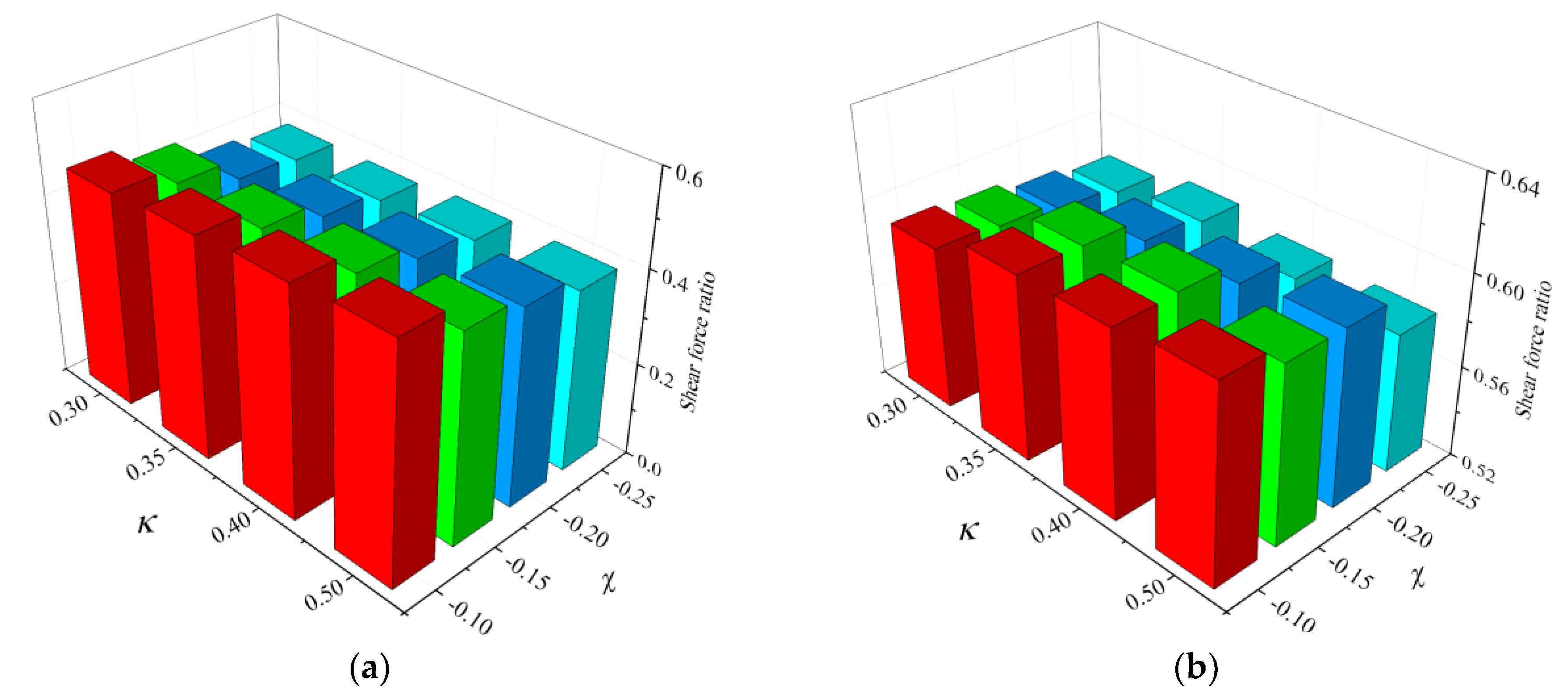
4.3. Shear Force Control
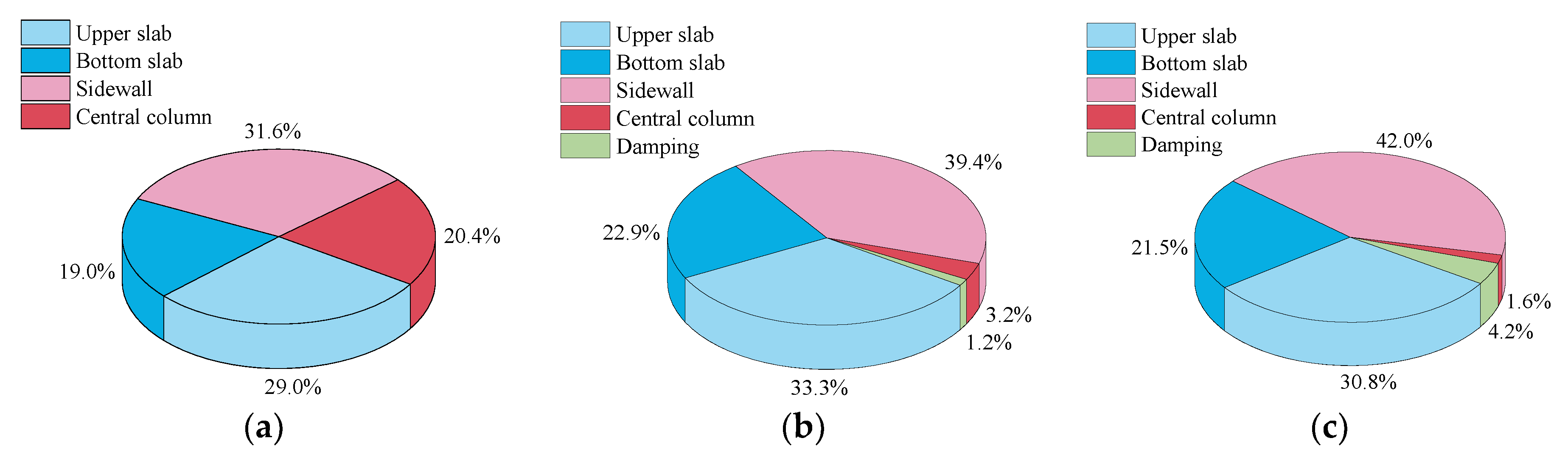
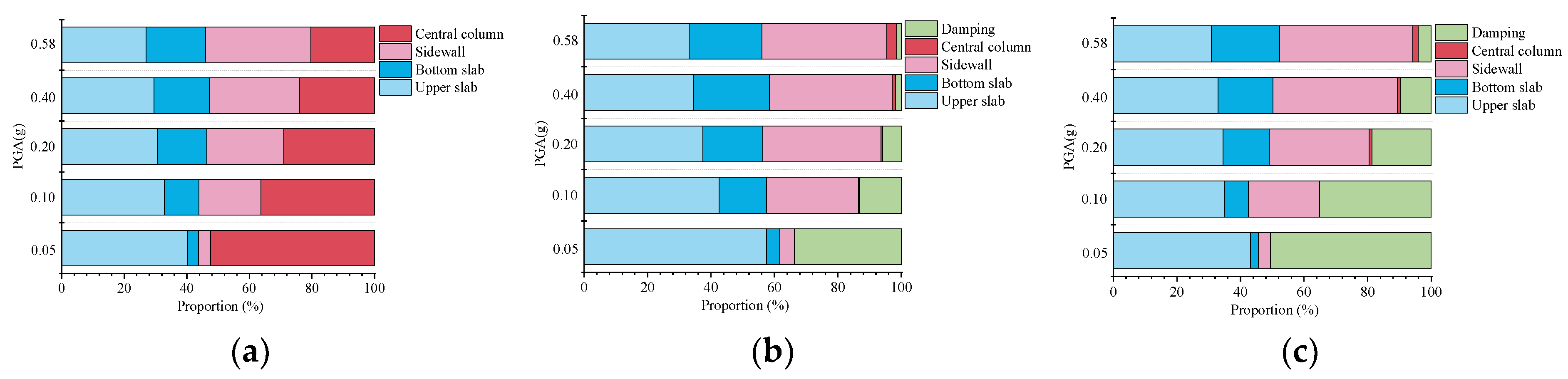
4.4. Energy-Based Damage Control
5. Conclusions
- (1)
- The system is effective in the vibration control of underground structures, and it exhibited a significant energy-dissipation-enhancement effect in which the series connection of the negative and positive stiffnesses amplified dashpot deformation for enhanced energy-dissipation capacity and efficiency.
- (2)
- In comparison to a conventional isolator with the same damping coefficient, the NSAS isolation system provides underground structures with a multiperformance effect. Particularly, the simultaneous relative displacement of the central column and shear force responses can be mitigated more effectively by the NSAS.
- (3)
- Benefiting from the energy-dissipation-enhancement effect, more vibration energy can be dissipated by the NSAS, thereby reducing the energy-dissipation burden of the central column. Under seismic excitation with different intensity levels, the enhanced energy-dissipation capacity of the NSAS isolation system held true, which demonstrated the robustness of the proposed system for the multilevel safety of underground structures.
- (4)
- The objective of this study was to develop a novel isolation system, the NSAS, for the vibration mitigation of underground structures. In future studies, the parameter design of NSAS for underground structures should be detailed. Correspondingly, the design method and the energy-dissipation-enhancement effect of NSAS should be further verified by experiment analysis.
Author Contributions
Funding
Conflicts of Interest
References
- Lanzano, G.; Bilotta, E.; Russo, G. Tunnels under seismic loading: A review of damage case histories and protection methods. In Strategies for Reduction of the Seismic Risk; StreGa: Ripalimosani (CB), Italy, 2008; pp. 65–75. [Google Scholar]
- Zhang, Z.M.; Bilotta, E.; Yuan, Y.; Yu, H.T.; Zhao, H.L. Experimental assessment of the effect of vertical earthquake motion on underground metro station. Appl. Sci. 2019, 9, 5182. [Google Scholar] [CrossRef]
- Pitilakis, K.; Tsinidis, G. Performance and Seismic Design of Underground Structures, Earthquake Geotechnical Engineering Design; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Iida, H.; Hiroto, T.; Yoshida, N.; Iwafuji, M. Damage to Daikai subway station. Soils Found. 1996, 36, 283–300. [Google Scholar] [CrossRef]
- An, X.; Shawky, A.A.; Maekawa, K. The collapse mechanism of a subway station during the Great Hanshin earthquake. Cem. Concr. Compos. 1997, 19, 241–257. [Google Scholar] [CrossRef]
- Ming, W.J.; Litehiser, J.J. The distribution of earthquake damage to underground facilities during the 1976 Tang-Shan earthquake. Earthq. Spectra 1985, 1, 741–757. [Google Scholar] [CrossRef]
- Gazetas, G.; Gerolymos, N.; Anastasopoulos, I. Response of three Athens metro underground structures in the 1999 Parnitha earthquake. Soil Dyn. Earthq. Eng. 2005, 25, 617–633. [Google Scholar] [CrossRef]
- Wang, W.L.; Wang, T.T.; Su, J.J.; Lin, C.H.; Seng, C.R.; Huang, T.H. Assessment of damage in mountain tunnels due to the Taiwan Chi-Chi Earthquake. Tunn. Undergr. Space Technol. 2001, 16, 133–150. [Google Scholar] [CrossRef]
- Lai, J.; He, S.; Qiu, J.; Chen, J.; Wang, L.; Wang, K.; Wang, J. Characteristics of seismic disasters and aseismic measures of tunnels in Wenchuan earthquake. Environ. Earth Sci. 2017, 76, 94. [Google Scholar] [CrossRef]
- Chen, Z.Y.; Zhao, H.; Lou, M.L. Seismic performance and optimal design of framed underground structures with lead-rubber bearings. Struct. Eng. Mech. 2016, 58, 259–276. [Google Scholar] [CrossRef]
- Ma, C.; Lu, D.C.; Du, X.L. Seismic performance upgrading for underground structures by introducing sliding isolation bearings. Tunn. Undergr. Space Technol. 2018, 74, 1–9. [Google Scholar] [CrossRef]
- Mikami, A.; Konagai, K.; Sawada, T. Stiffness design of isolation rubber for center columns of tunnel. Doboku Gakkai Ronbunshu 2001, 415–420. [Google Scholar] [CrossRef]
- Buckle, I.G.; Mayes, R.L. Seismic isolation: History, application, and performance—A world view. Earthq. Spectra 1990, 6, 161–201. [Google Scholar] [CrossRef]
- Kiryu, S.; Murono, Y.; Morikawa, H. Seismic response of a cut-and-cover tunnel isolated by polymer material. Earthq. Eng. Struct. Dyn. 2012, 41, 2043–2057. [Google Scholar] [CrossRef]
- Li, H.; Liu, J.L.; Ou, J.P. Seismic response control of a cable-stayed bridge using negative stiffness dampers. Struct. Control Health Monit. 2011, 18, 265–288. [Google Scholar] [CrossRef]
- Iemura, H.; Pradono, M.H. Passive and semi-active seismic response control of a cable-stayed bridge. J. Struct. Control 2002, 9, 189–204. [Google Scholar] [CrossRef]
- Hogsberg, J.B. The role of negative stiffness in semi-active control of magneto-rheological dampers. Struct. Control Health Monit. 2011, 18, 289–304. [Google Scholar] [CrossRef]
- Sarlis, A.A.; Pasala, D.T.R.; Constantinou, M.C.; Reinhorn, A.M.; Nagarajaiah, S.; Taylor, D.P. Negative stiffness device for seismic protection of structures. J. Struct. Eng. 2013, 139, 1124–1133. [Google Scholar] [CrossRef]
- Pasala, D.T.R.; Sarlis, A.A.; Nagarajaiah, S.; Reinhorn, A.M.; Constantinou, M.C.; Taylor, D.P. Adaptive negative stiffness: New structural modification approach for seismic protection. J. Struct. Eng. 2013, 139, 1112–1123. [Google Scholar] [CrossRef]
- Kwon, I.Y.; Yang, H.T.; Hansma, P.K.; Randall, C. Implementable bio-inspired passive negative spring actuator for full-scale structural control under seismic excitation. J. Struct. Eng. 2016, 142, 04015079. [Google Scholar] [CrossRef]
- Attary, N.; Symans, M.D.; Nagarajaiah, S. Development of a rotation-based negative stiffness device for seismic protection of structures. J. Vib. Control 2017, 23, 853–867. [Google Scholar] [CrossRef]
- Kalathur, H.; Lakes, R.S. Column dampers with negative stiffness: High damping at small amplitude. Smart Mater. Struct. 2013, 22, 084013. [Google Scholar] [CrossRef]
- Iemura, H.; Pradono, M.H. Advances in the development of pseudo-negative-stiffness dampers for seismic response control. Struct. Control Health Monit. 2009, 16, 784–799. [Google Scholar] [CrossRef]
- Riosgarcia, G.; Benaventcliment, A. New rocking column with control of negative stiffness displacement range and its application to RC frames. Eng. Struct. 2020, 206, 110133. [Google Scholar] [CrossRef]
- Wang, M.; Sun, F.F.; Yang, J.Q.; Nagarajaiah, S. Seismic protection of SDOF systems with a negative stiffness amplifying damper. Eng. Struct. 2019, 190, 128–141. [Google Scholar] [CrossRef]
- Zhuang, H.Y.; Hu, Z.H.; Wang, X.J.; Chen, G.X. Seismic responses of a large underground structure in liquefied soils by FEM numerical modelling. Bull. Earthq. Eng. 2015, 13, 3645–3668. [Google Scholar] [CrossRef]
- ABAQUS. Theory and Analysis User’s Manual Version 6.12; Dassault Systems SIMULIA Corp: Providence, RI, USA, 2012. [Google Scholar]
- Li, W.; Chen, Q.J. Effect of vertical ground motions and overburden depth on the seismic responses of large underground structures. Eng. Struct. 2020, 205, 110073. [Google Scholar] [CrossRef]
- Li, W.; Chen, Q.J. Seismic damage evaluation of an entire underground subway system in dense urban areas by 3D FE simulation. Tunn. Undergr. Space Technol. 2020, 99, 103351. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. Plastic-damage model for cyclic loading of concrete structures. J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
- Chopra, A.K.; Chintanapakdee, C. Inelastic deformation ratios for design and evaluation of structures: Single-degree-of-freedom bilinear systems. J. Struct. Eng. 2004, 130, 1309–1319. [Google Scholar] [CrossRef]
- Martin, P.P.; Seed, H.B. One-dimensional dynamic ground response analyses. J. Geotech. Eng. Div. 1982, 108, 935–952. [Google Scholar] [CrossRef]
- Ge, Q.; Xiong, F.; Xie, L.W.; Chen, J.; Yu, M.J. Dynamic interaction of soil—Structure cluster. Soil Dyn. Earthq. Eng. 2019, 123, 16–30. [Google Scholar] [CrossRef]
- Chen, Q.J.; Wang, Y.C.; Zhao, Z.P. Energy spectrum study and optimal design of an inerter-based structure considering the underlying soil. Appl. Sci. 2020, 10, 2999. [Google Scholar] [CrossRef]
- JGS-4001-2004. Principles for Foundation Designs Grounded on a Performance-Based Design Concept; Japanese Geotechnical Society: Tokyo, Japan, 2006. [Google Scholar]
- GB/T-51336-2018. Standard for Seismic Design of Underground Structures; Architecture and Building Press: Beijing, China, 2018. [Google Scholar]
- Huo, H.; Bobet, A.; Fernández, G.; Ramirez, J. Load transfer mechanisms between underground structure and surrounding ground: Evaluation of the failure of the Daikai station. J. Geotech. Geoenviron. Eng. 2005, 131, 1522–1533. [Google Scholar] [CrossRef]
- Cai, X.S.; Yang, C.Y.; Yuan, Y. Hybrid simulation of seismic responses of a typical station with a reinforced concrete column. Appl. Sci. 2020, 10, 1331. [Google Scholar] [CrossRef]
- Wang, Y.C.; Chen, Q.J. Parametric fitting of soft soil sites’ Davidenkov model based on PSO algorithm and its application. J. Vib. Shock 2019, 38, 8–16. [Google Scholar]
- Parramontesinos, G.J.; Bobet, A.; Ramirez, J.A. Evaluation of soil-structure interaction and structural collapse in Daikai subway station during kobe earthquake. ACI Struct. J. 2006, 103, 113–122. [Google Scholar] [CrossRef]
- GB-50011-2010. Code for Seismic Design of Buildings; Architecture and Building Press: Beijing, China, 2016. [Google Scholar]
- Zhao, Z.P.; Chen, Q.J.; Zhang, R.F.; Pan, C.; Jiang, Y.Y. Energy dissipation mechanism of inerter systems. Int. J. Mech. Sci. 2020, 184, 105845. [Google Scholar] [CrossRef]
- Zhao, Z.P.; Zhang, R.F.; Jiang, Y.Y.; Pan, C. Seismic response mitigation of structures with a friction pendulum inerter system. Eng. Struct. 2019, 193, 110–120. [Google Scholar] [CrossRef]
- Zhao, Z.P.; Zhang, R.F.; Jiang, Y.Y.; Pan, C. A tuned liquid inerter system for vibration control. Int. J. Mech. Sci. 2019, 164, 105171. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Galelamuela, D.; Donaireavila, J. Energy capacity and seismic performance of RC waffle-flat plate structures under two components of far-field ground motions: Shake table tests. Earthq. Eng. Struct. Dyn. 2019, 48, 949–969. [Google Scholar] [CrossRef]
- Benavent-Climent, A. Design, testing, and evaluation of the web plastifying damper for the aseismic protection of buildings. In Encyclopedia of Earthquake Engineering; Springer: Berlin/Heidelberg, Germany, 2014; pp. 1–8. [Google Scholar] [CrossRef]




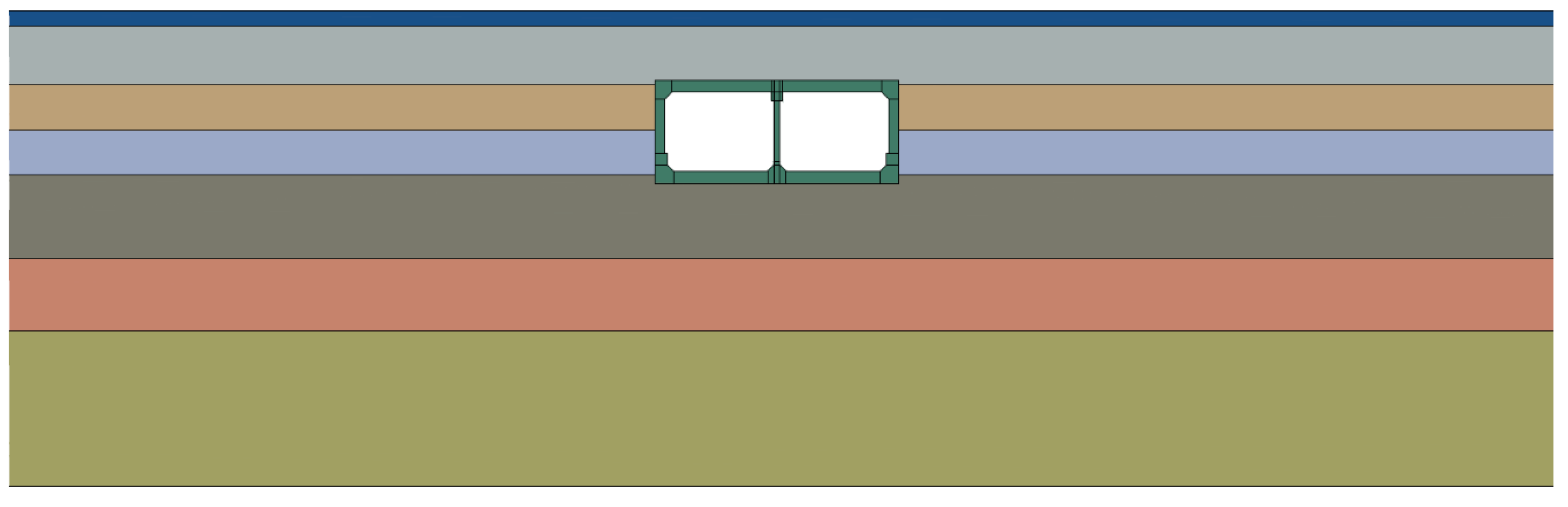
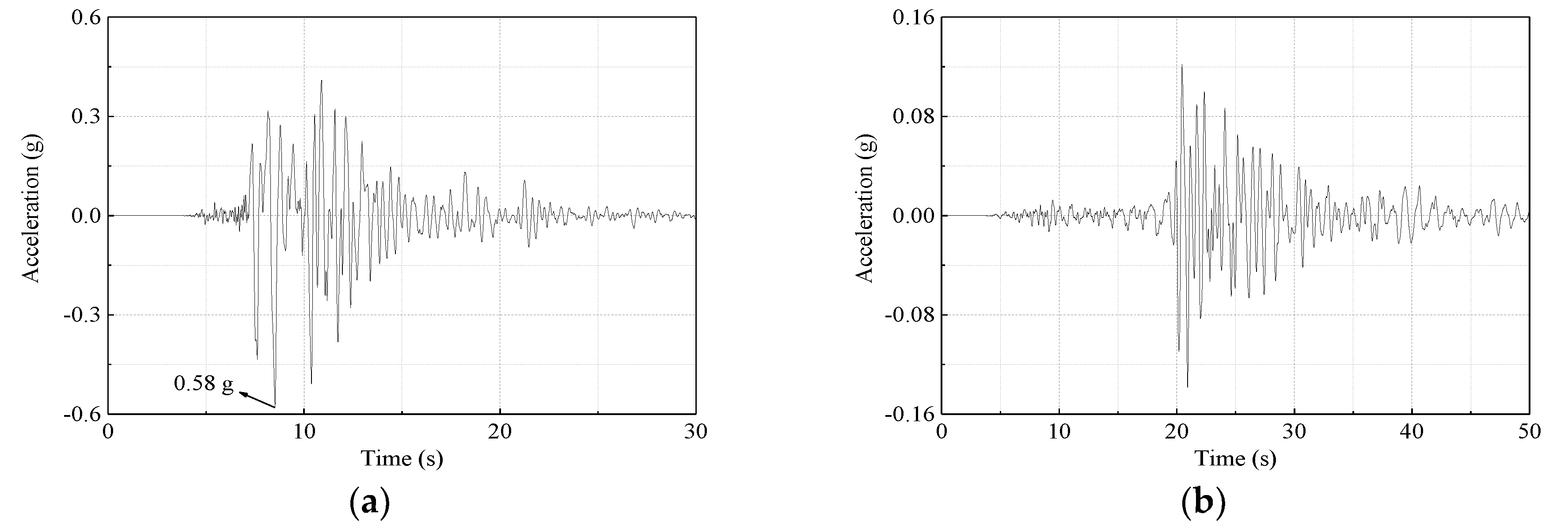




| No. | Type | Thickness (m) | Density (kg/m3) | Vs (m/s) | Gmax (MPa) | Poisson’s Ratio |
|---|---|---|---|---|---|---|
| 1 | Filled | 1.0 | 1900 | 140 | 38 | 0.333 |
| 2 | Sand | 4.1 | 1900 | 140 | 38 | 0.32 |
| 3 | Sand | 3.2 | 1900 | 170 | 56 | 0.32 |
| 4 | Clay | 3.1 | 1900 | 190 | 70 | 0.40 |
| 5 | Clay | 5.8 | 1900 | 240 | 112 | 0.30 |
| 6 | Sand | 5.0 | 2000 | 330 | 222 | 0.26 |
| 7 | Sand | 11.8 | 2000 | 1000 | 2040 | 0.26 |
| −0.10 | −0.15 | −0.20 | −0.25 | |
|---|---|---|---|---|
| 0.30 | 0.88 | 0.85 | 0.89 | 1.06 |
| 0.35 | 0.87 | 0.88 | 0.85 | 0.89 |
| 0.40 | 0.93 | 0.90 | 0.88 | 0.87 |
| 0.50 | 1.03 | 0.95 | 0.89 | 0.90 |
| −0.10 | −0.15 | −0.20 | −0.25 | |
|---|---|---|---|---|
| 0.30 | 0.91 | 0.90 | 0.90 | 1.06 |
| 0.35 | 0.92 | 0.91 | 0.87 | 0.90 |
| 0.40 | 0.96 | 0.95 | 0.92 | 0.89 |
| 0.50 | 1.04 | 0.96 | 0.91 | 0.91 |
| −0.10 | −0.15 | −0.20 | −0.25 | |
|---|---|---|---|---|
| 0.30 | 0.50 | 0.44 | 0.42 | 0.39 |
| 0.35 | 0.49 | 0.44 | 0.41 | 0.38 |
| 0.40 | 0.48 | 0.43 | 0.40 | 0.38 |
| 0.50 | 0.47 | 0.42 | 0.39 | 0.37 |
| −0.10 | −0.15 | −0.20 | −0.25 | |
|---|---|---|---|---|
| 0.30 | 0.61 | 0.60 | 0.60 | 0.58 |
| 0.35 | 0.60 | 0.60 | 0.58 | 0.58 |
| 0.40 | 0.60 | 0.60 | 0.59 | 0.59 |
| 0.50 | 0.59 | 0.59 | 0.59 | 0.58 |
| Parts | Plastic-Energy Density (J/m3) | ||
|---|---|---|---|
| Uncontrolled US | US-CIS | US-NSAS Isolation System | |
| Upper slab | 555 | 678 | 641 |
| Bottom slab | 213 | 294 | 267 |
| Sidewall | 314 | 513 | 483 |
| Central column | 2221 | 210 | 138 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Q.; Wang, Y.; Zhao, Z. A Novel Negative Stiffness Amplification System Based Isolation Method for the Vibration Control of Underground Structures. Appl. Sci. 2020, 10, 5421. https://doi.org/10.3390/app10165421
Chen Q, Wang Y, Zhao Z. A Novel Negative Stiffness Amplification System Based Isolation Method for the Vibration Control of Underground Structures. Applied Sciences. 2020; 10(16):5421. https://doi.org/10.3390/app10165421
Chicago/Turabian StyleChen, Qingjun, Yanchao Wang, and Zhipeng Zhao. 2020. "A Novel Negative Stiffness Amplification System Based Isolation Method for the Vibration Control of Underground Structures" Applied Sciences 10, no. 16: 5421. https://doi.org/10.3390/app10165421
APA StyleChen, Q., Wang, Y., & Zhao, Z. (2020). A Novel Negative Stiffness Amplification System Based Isolation Method for the Vibration Control of Underground Structures. Applied Sciences, 10(16), 5421. https://doi.org/10.3390/app10165421






