Disturbance and Uncertainty Suppression Control for a Saucer-Shaped Unmanned Aerial Vehicle Based on Extended State Observer
Abstract
1. Introduction
2. Model Description of the Saucer-Shaped UAV
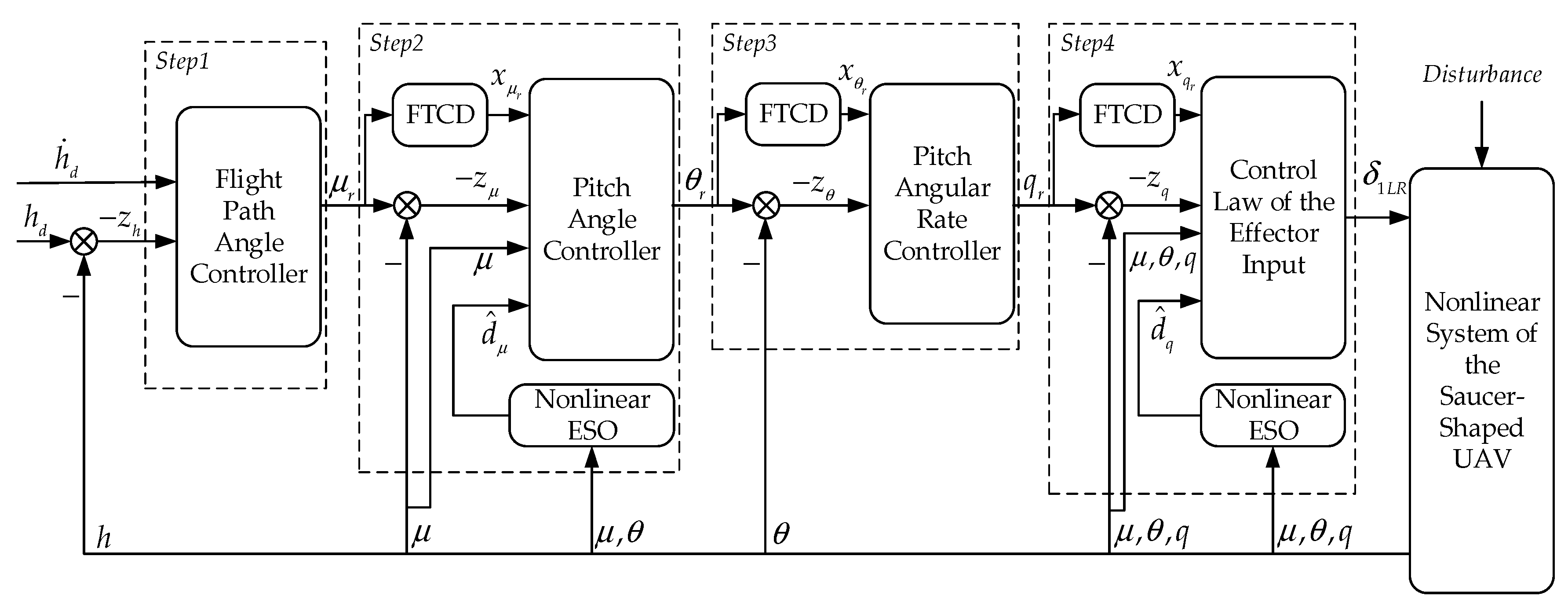
3. Control System Design
3.1. Finite-Time-Convergent Second-Order Differentiator
3.2. Nonlinear ESO Design
3.3. Backstepping Control Law Design
4. Stability and Altitude Tracking Performance Analysis
- (1)
- All the signals of the resulting closed-loop system remain bounded.
- (2)
- The system output tracking error converges to a sufficiently small neighbourhood of zero by selecting appropriate design parameters.
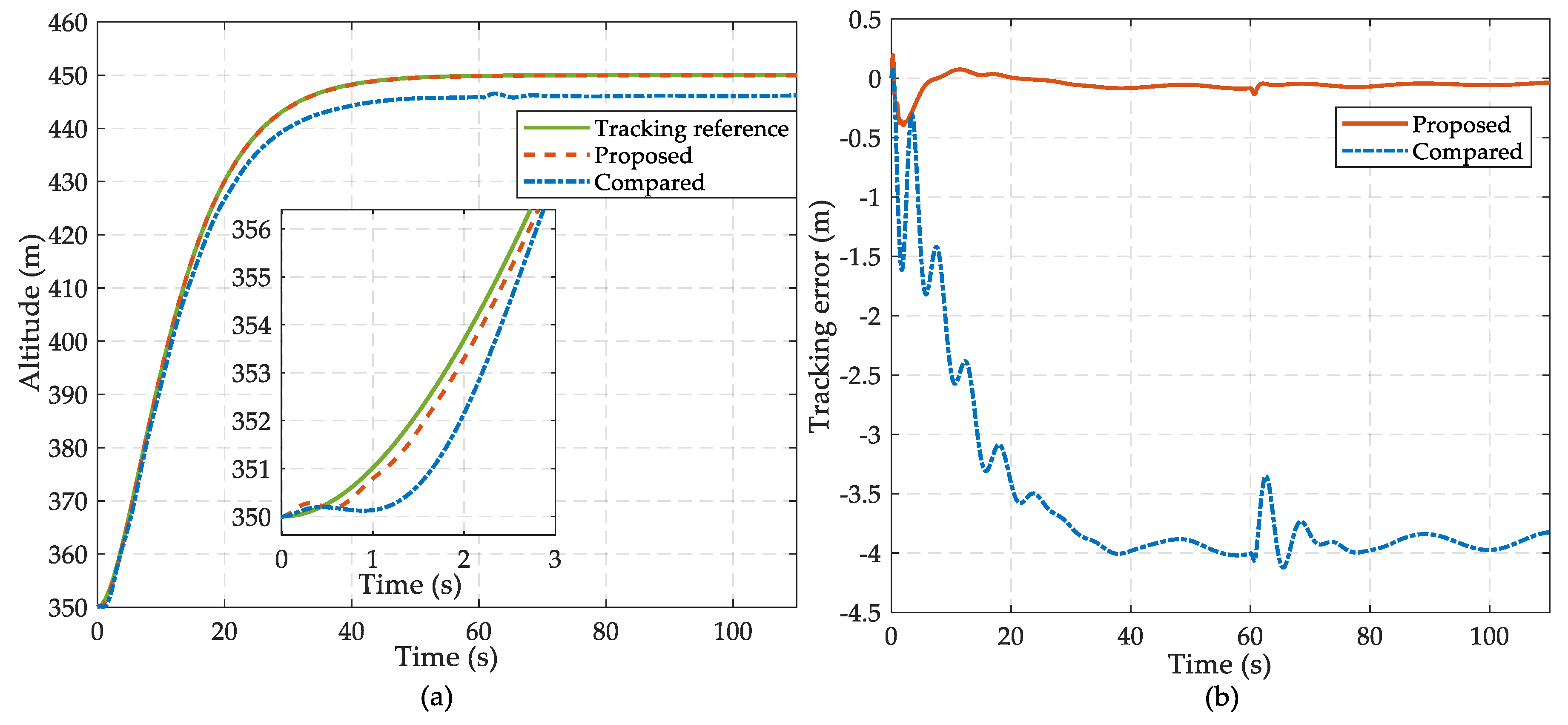
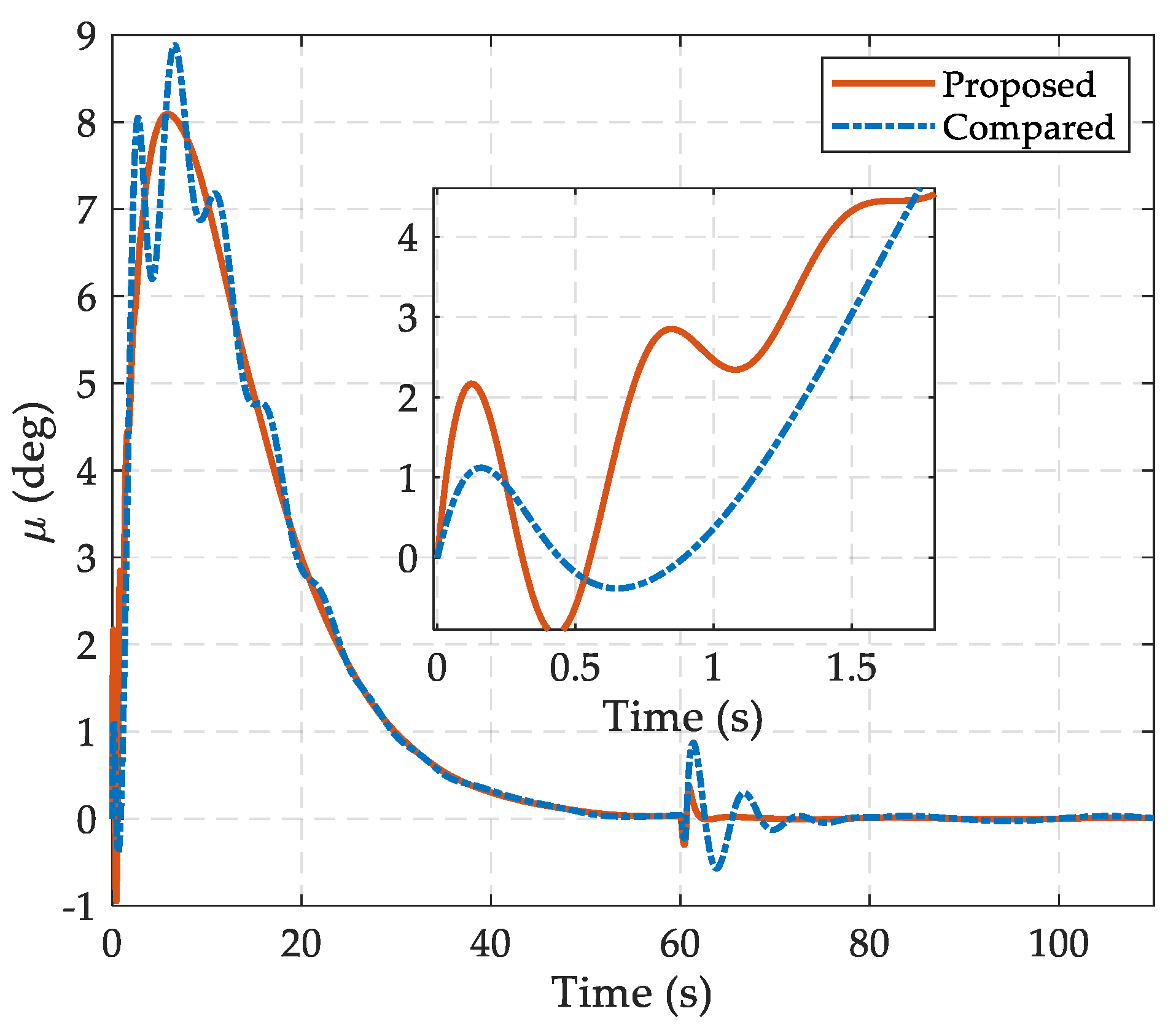
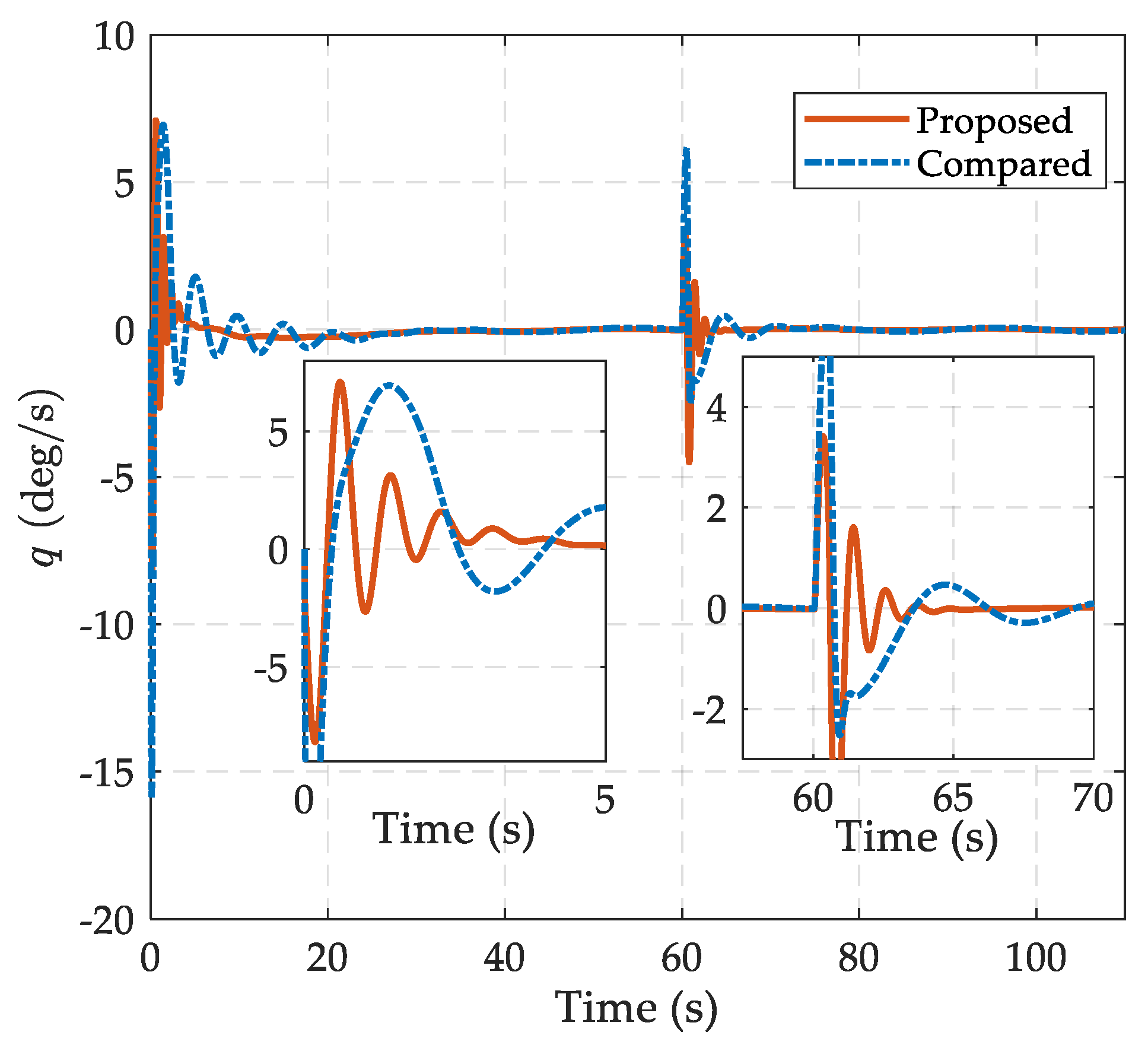
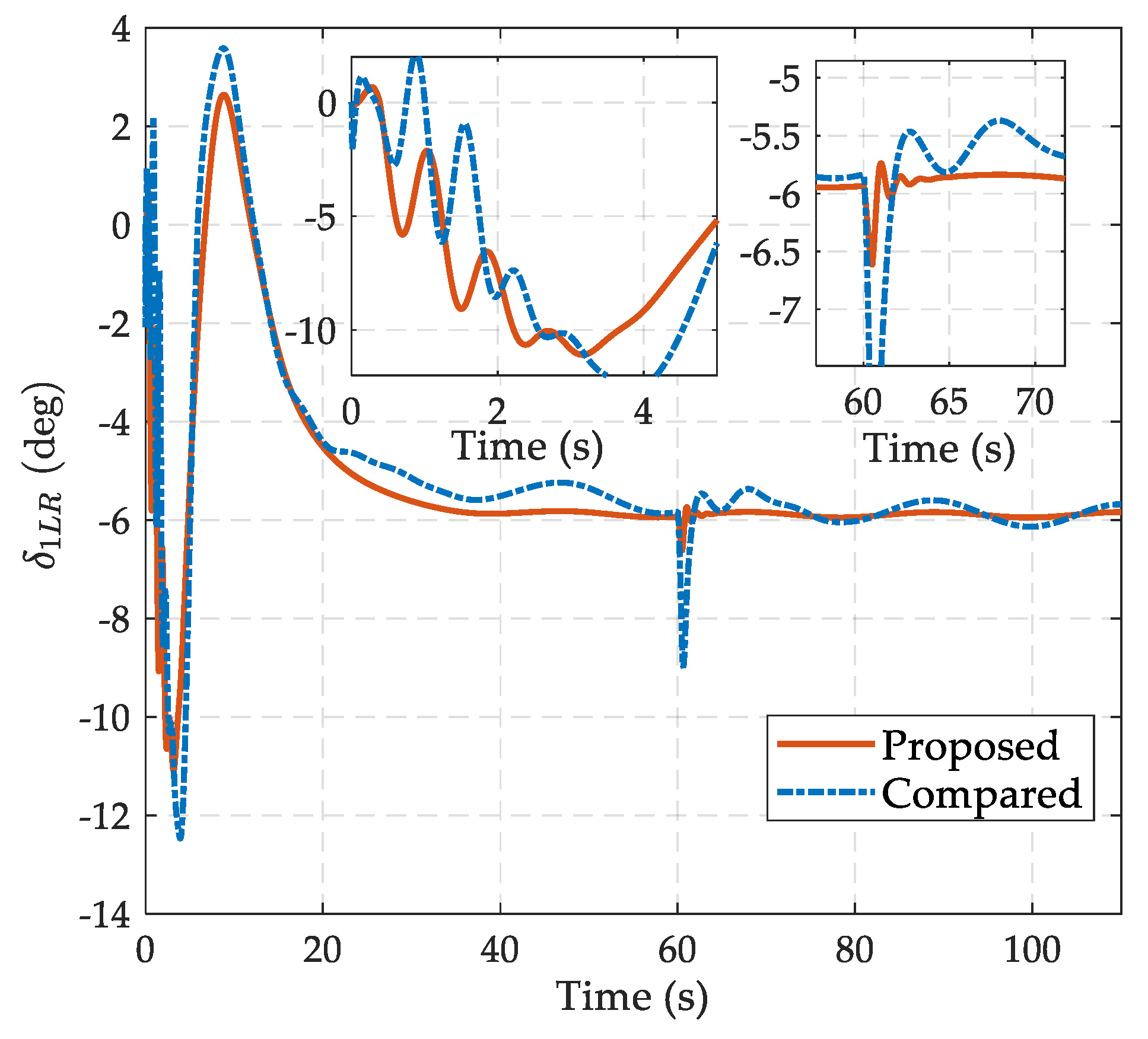
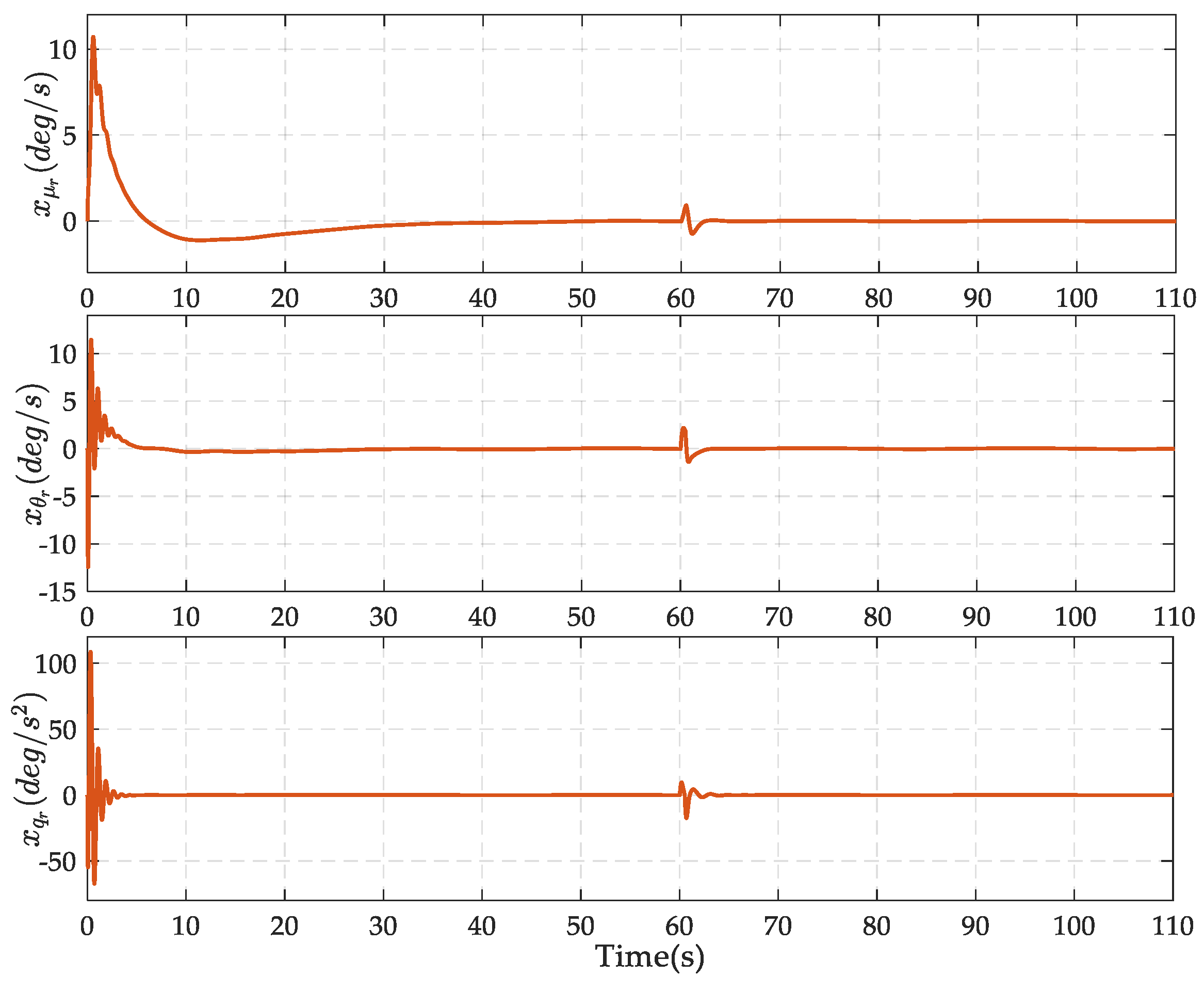
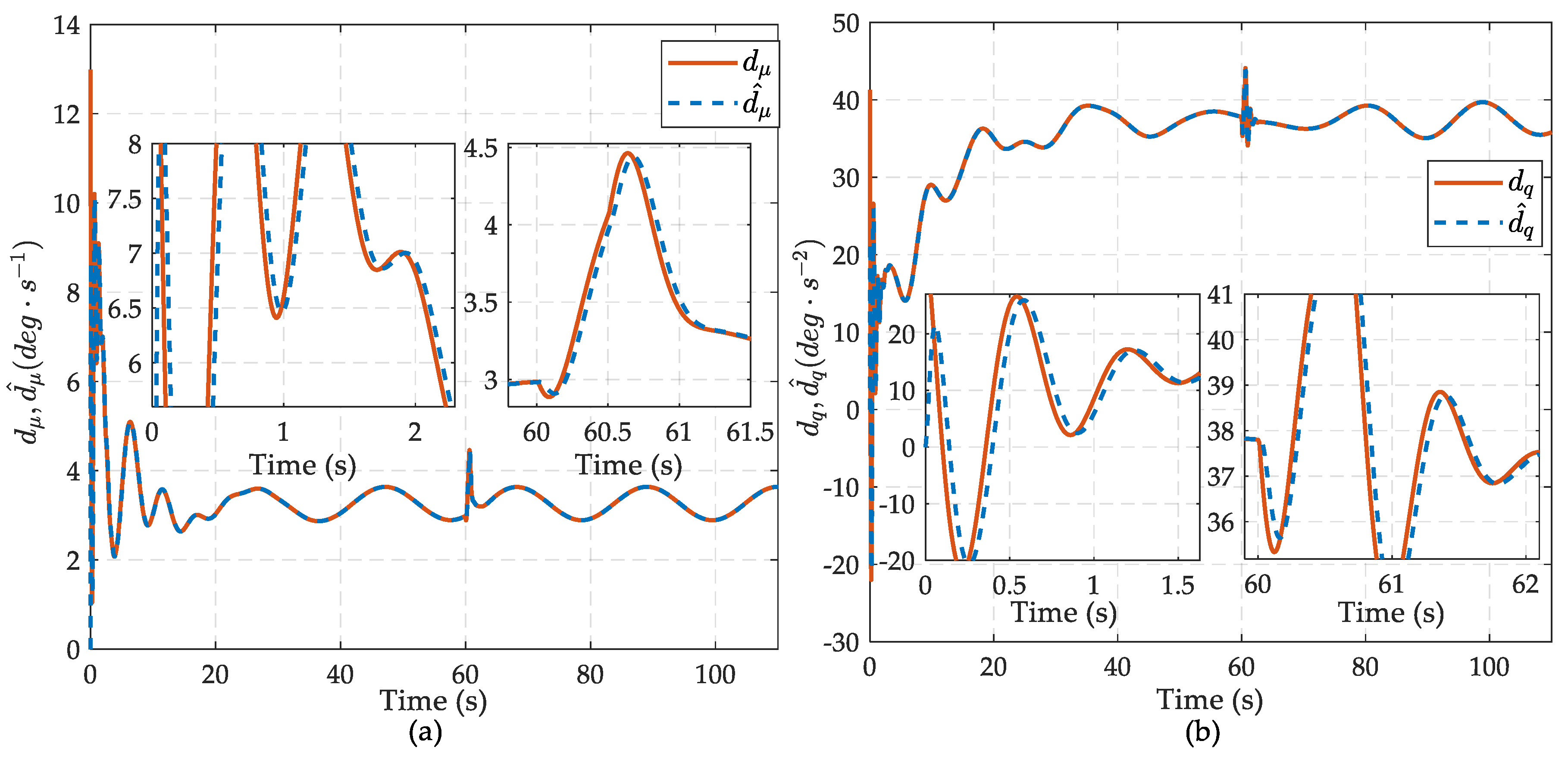
5. Numerical Simulation
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Proof of Step 1 in Theorem 1
References
- Richard, W.; Bauer, X. Flying wings/flying fuselages. In Proceedings of the 39th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2011. [Google Scholar]
- De Vries Pieter, S.; van Kampen, E.-J. Reinforcement Learning-based Control Allocation for the Innovative Control Effectors Aircraft. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar]
- Okonkwo, P.; Smith, H. Review of evolving trends in blended wing body aircraft design. Prog. Aerosp. Sci. 2016, 82, 1–23. [Google Scholar] [CrossRef]
- Liu, C.J.; Chen, W.H.; Andrews, J. Tracking control of small-scale helicopters using explicit nonlinear MPC augmented with disturbance observers. Control. Eng. Pract. 2012, 20, 258–268. [Google Scholar] [CrossRef]
- Pu, Z.; Tan, X.; Fan, G.; Yi, J. Uncertainty analysis and robust trajectory linearization control of a flexible air-breathing hypersonic vehicle. Acta Astronaut. 2014, 101, 16–32. [Google Scholar] [CrossRef]
- Qiu, B.B.; Wang, G.F.; Fan, Y.S. Trajectory Linearization-Based Adaptive PLOS Path Following Control for Unmanned Surface Vehicle with Unknown Dynamics and Rudder Saturation. Appl. Sci. 2020, 10, 3538. [Google Scholar] [CrossRef]
- Tian, B.L.; Qun, Z.Q. Quasi-continuous high-order sliding mode controller and observer design for vehicle. In Proceedings of the 17th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, San Francisco, CA, USA, 11–14 April 2011. [Google Scholar]
- Denieul, Y.; Bordeneuve-Guibe, J.; Alazard, D.; Toussaint, C.; Taquin, G. Integrated design of flight control surfaces and laws for new aircraft configurations. IFAC-Pap. Online 2017, 50, 14180–14187. [Google Scholar] [CrossRef]
- Wen, N.; Liu, Z.H.; Sun, Y.; Zhu, L.P. Design of LPV-based sliding mode controller with finite time convergence for a morphing aircraft. Int. J. Aerosp. Eng. 2017, 7, 81515–81531. [Google Scholar] [CrossRef]
- Yang, J.; Li, S.H.; Yu, X.H. Sliding-mode control for systems with mismatched uncertainties via a disturbance observer. IEEE Trans. Ind. Electron. 2012, 60, 160–169. [Google Scholar] [CrossRef]
- Chen, W.H.; Yang, J.; Guo, L.; Li, S.H. Disturbance-observer-based control and related methods—An overview. IEEE Trans. Ind. Electron. 2015, 63, 1083–1095. [Google Scholar] [CrossRef]
- Kim, K.S.; Park, Y.J.; Oh, S.H. Designing robust sliding hyperplanes for parametric uncertain systems: A Riccati approach. Automatica 2000, 36, 1041–1048. [Google Scholar] [CrossRef]
- Guo, B.Z.; Wu, Z.H. Active disturbance rejection control approach to output-feedback stabilization of lower triangular nonlinear systems with stochastic uncertainty. Int. J. Robust Nonlin. Control. 2017, 27, 2773–2797. [Google Scholar] [CrossRef]
- Zhao, Z.L.; Guo, B.Z. Active disturbance rejection control approach to stabilization of lower triangular systems with uncertainty. Int. J. Robust Nonlin. Control. 2016, 16, 2314–2337. [Google Scholar] [CrossRef]
- Sun, H.B.; Guo, L. Composite adaptive disturbance observer based control and back-stepping method for nonlinear system with multiple mismatched disturbances. J. Frankl. Inst. 2014, 351, 1027–1041. [Google Scholar] [CrossRef]
- Zhai, D.; An, L.W.; Dong, J.X.; Zhang, Q.L. Robust adaptive fuzzy control of a class of uncertain nonlinear systems with unstable dynamics and mismatched disturbances. IEEE Trans. Cybern. 2017, 48, 3105–3115. [Google Scholar] [CrossRef]
- Shi, X.Y.; Cheng, Y.H.; Yin, C.; Huang, X.G.; Zhong, S.M. Design of adaptive backstepping dynamic surface control method with RBF neural network for uncertain nonlinear system. Neurocomputing 2019, 330, 490–503. [Google Scholar] [CrossRef]
- Yu, J.P.; Ma, Y.M.; Yu, H.S.; Lin, C. Adaptive fuzzy dynamic surface control for induction motors with iron losses in electric vehicle drive systems via backstepping. Inf. Sci. 2017, 376, 172–189. [Google Scholar] [CrossRef]
- Yu, J.P.; Shi, P.; Dong, W.J.; Yu, H.S. Observer and command-filter-based adaptive fuzzy output feedback control of uncertain nonlinear systems. IEEE Trans. Ind. Electron. 2015, 62, 5962–5970. [Google Scholar] [CrossRef]
- Jin, Z.J.; Zhang, W.M.; Liu, S.; Gu, M. Command-Filtered Backstepping Integral Sliding Mode Control with Prescribed Performance for Ship Roll Stabilization. Appl. Sci. 2019, 9, 4288. [Google Scholar] [CrossRef]
- Jiang, T.; Lin, D.F.; Song, T. Finite-time backstepping control for quadrotors with disturbances and input constraints. IEEE Access. 2018, 6, 62037–62049. [Google Scholar] [CrossRef]
- Yu, J.P.; Shi, P.; Zhao, L. Finite-time command filtered backstepping control for a class of nonlinear systems. Automatica 2018, 9, 173–180. [Google Scholar] [CrossRef]
- Zhao, Z.L.; Guo, B.Z. Extended state observer for uncertain lower triangular nonlinear systems. Syst. Control. Lett. 2015, 85, 100–108. [Google Scholar] [CrossRef]
- Gong, L.G.; Wang, Q.; Dong, C.Y. Disturbance rejection control of morphing aircraft based on switched nonlinear systems. Nonlinear Dyn. 2019, 96, 975–995. [Google Scholar] [CrossRef]
- Zhang, L.; Li, Z.; Yang, C. Adaptive neural network based variable stiffness control of uncertain robotic systems using disturbance observer. IEEE Trans. Ind Electron. 2017, 64, 2236–2245. [Google Scholar] [CrossRef]
- Yao, J.; Jiao, Z.; Ma, D. Extended-state-observer-based output feedback nonlinear robust control of hydraulic systems with backstepping. IEEE Trans. Ind. Electron. 2014, 61, 6285–6293. [Google Scholar] [CrossRef]
- Shao, X.L.; Liu, J.; Wang, H.L. Robust back-stepping output feedback trajectory tracking for quadrotors via extended state observer and sigmoid tracking differentiator. Mech. Syst. Signal. Process. 2018, 104, 631–647. [Google Scholar] [CrossRef]
- Xiong, S.; Xie, H.; Song, K.; Zhang, G.H. A Speed Tracking Method for Autonomous Driving via ADRC with Extended State Observer. Appl. Sci. 2019, 9, 3339. [Google Scholar] [CrossRef]
- Li, S.H.; Yang, J.; Chen, W.H.; Chen, X.S. Generalized extended state observer based control for systems with mismatched uncertainties. IEEE Trans. Ind. Electron. 2011, 59, 4792–4802. [Google Scholar] [CrossRef]
- Guo, B.Z.; Zhao, Z.L. On the convergence of an extended state observer for nonlinear systems with uncertainty. Syst. Control. Lett. 2011, 60, 420–430. [Google Scholar] [CrossRef]
- Xing, Z.H.; Wu, S.T.; Wu, X.L. Explicit nonlinear model predictive control for a saucer-shaped unmanned aerial vehicle. Adv. Mech. Eng. 2013, 5, 706453. [Google Scholar] [CrossRef]
- Liu, J.L.; Zhang, W.G.; Liu, X.X.; He, Q.Z.; Qin, Y.X. Gust response stabilization for rigid aircraft with multi-control-effectors based on a novel integrated control scheme. Aerosp. Sci. Technol. 2018, 79, 625–635. [Google Scholar] [CrossRef]
- Wong, K.Y.; Kariya, N.; Tanaka, M.; Tanaka, K. Longitudinal Fuzzy Model Construction of a Flying-Wing Unmanned Aerial Vehicle and a Nonlinear Guaranteed Cost Control Approach to Altitude Stabilization. In Proceedings of the 2019 International Conference on Fuzzy Theory and Its Applications (iFUZZY), New Taipei City, Taiwan, 7–10 November 2019. [Google Scholar]
- Ran, M.P.; Wang, Q.; Dong, C.Y. Active disturbance rejection control for uncertain nonaffine-in-control nonlinear systems. IEEE T. Automat. Contr. 2016, 62, 5830–5836. [Google Scholar] [CrossRef]
- Guo, B.Z.; Han, J.Q.; Xi, F.B. Linear tracking-differentiator and application to online estimation of the frequency of a sinusoidal signal with random noise perturbation. Int. J. Syst. Sci. 2002, 33, 351–358. [Google Scholar] [CrossRef]
- Levant, A. Higher-order sliding modes, differentiation and output-feedback control. Int. J. Control. 2003, 76, 924–941. [Google Scholar] [CrossRef]
- Shao, X.L.; Wang, H.L. Back-stepping robust trajectory linearization control for hypersonic reentry vehicle via novel tracking differentiator. J. Franklin Inst. 2016, 353, 1957–1984. [Google Scholar] [CrossRef]
- Wang, X.H.; Chen, Z.Q.; Yang, G. Finite-time-convergent differentiator based on singular perturbation technique. IEEE Trans. Automat. Contr. 2007, 52, 1731–1737. [Google Scholar] [CrossRef]
- Kuo, C.W.; Tsai, C.C.; Lee, C.T. Intelligent leader-following consensus formation control using recurrent neural networks for small-size unmanned helicopters. IEEE Trans. Syst. Man Cybern. Syst. 2019, 99, 1–14. [Google Scholar] [CrossRef]
- Choi, Y.H.; Sung, J.Y. A simple fuzzy-approximation-based adaptive control of uncertain unmanned helicopters. Int. J. Control. Autom. Syst. 2016, 14, 340–349. [Google Scholar] [CrossRef]
- He, W.; Meng, T.T.; He, X.Y.; Sun, C.Y. Iterative learning control for a flapping wing micro aerial vehicle under distributed disturbances. IEEE Trans. Cybern. 2018, 49, 1524–1535. [Google Scholar] [CrossRef]
- Yu, J.L.; Wang, L.L.; Gao, G. Using wing tip devices to improve performance of saucer-shaped aircraft. Chin. J. Aeronaut. 2006, 19, 309–314. [Google Scholar] [CrossRef]
- Rahman, N.U.; Whidborne, J.F.; Cooke, A.K. Longitudinal control system design and handling qualities assessment of a blended wing body aircraft. In Proceedings of the 6th International Bhurban Conference on Applied Sciences & Technology, Islamabad, Pakistan, 19–22 January 2009; pp. 177–186. [Google Scholar]
- Hao, A.; Xia, H.W.; Wang, C.H. Barrier Lyapunov function-based adaptive control for hypersonic flight vehicles. Nonlinear Dyn. 2017, 88, 1833–1853. [Google Scholar]


| Symbol | Variable | Symbol | Variable |
|---|---|---|---|
| Mass | Velocity | ||
| Flight path angle andbank angle | Three principal moments of inertia and the product of inertia about the x and z axes | ||
| Angle of attack and sideslip | Dynamic pressure | ||
| Pitch angle | Atmospheric density | ||
| Roll, pitch and yaw angular rate | Aerodynamic lift coefficients | ||
| Lift force, side force and thrust | Aerodynamic pitch coefficients | ||
| Pitch moment | Reference area | ||
| Position of the engine in the body axis | Chord | ||
| Gravitational acceleration | Altitude |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Mass (kg) | 13 | 0.0752 | |
| Reference area (m2) | 2.097 | 0.03865 | |
| Chord (m) | 0.97991 | −0.0181 | |
| Air density (kg/m3) | 1.225064 | −0.0261 | |
| Inertial moment (kgm2) | 1.79715 | −0.04 | |
| Gravitational acceleration (m/s2) | 9.8 | −0.18 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, J.; Feng, C.; Zhao, H.; Wen, Y.; Wu, S. Disturbance and Uncertainty Suppression Control for a Saucer-Shaped Unmanned Aerial Vehicle Based on Extended State Observer. Appl. Sci. 2020, 10, 4884. https://doi.org/10.3390/app10144884
Deng J, Feng C, Zhao H, Wen Y, Wu S. Disturbance and Uncertainty Suppression Control for a Saucer-Shaped Unmanned Aerial Vehicle Based on Extended State Observer. Applied Sciences. 2020; 10(14):4884. https://doi.org/10.3390/app10144884
Chicago/Turabian StyleDeng, Jia, Cong Feng, Hongbo Zhao, Yongming Wen, and Sentang Wu. 2020. "Disturbance and Uncertainty Suppression Control for a Saucer-Shaped Unmanned Aerial Vehicle Based on Extended State Observer" Applied Sciences 10, no. 14: 4884. https://doi.org/10.3390/app10144884
APA StyleDeng, J., Feng, C., Zhao, H., Wen, Y., & Wu, S. (2020). Disturbance and Uncertainty Suppression Control for a Saucer-Shaped Unmanned Aerial Vehicle Based on Extended State Observer. Applied Sciences, 10(14), 4884. https://doi.org/10.3390/app10144884





