Quasi-Static Characteristics and Vibration Responses Analysis of Helical Geared Rotor System with Random Cumulative Pitch Deviations
Abstract
1. Introduction
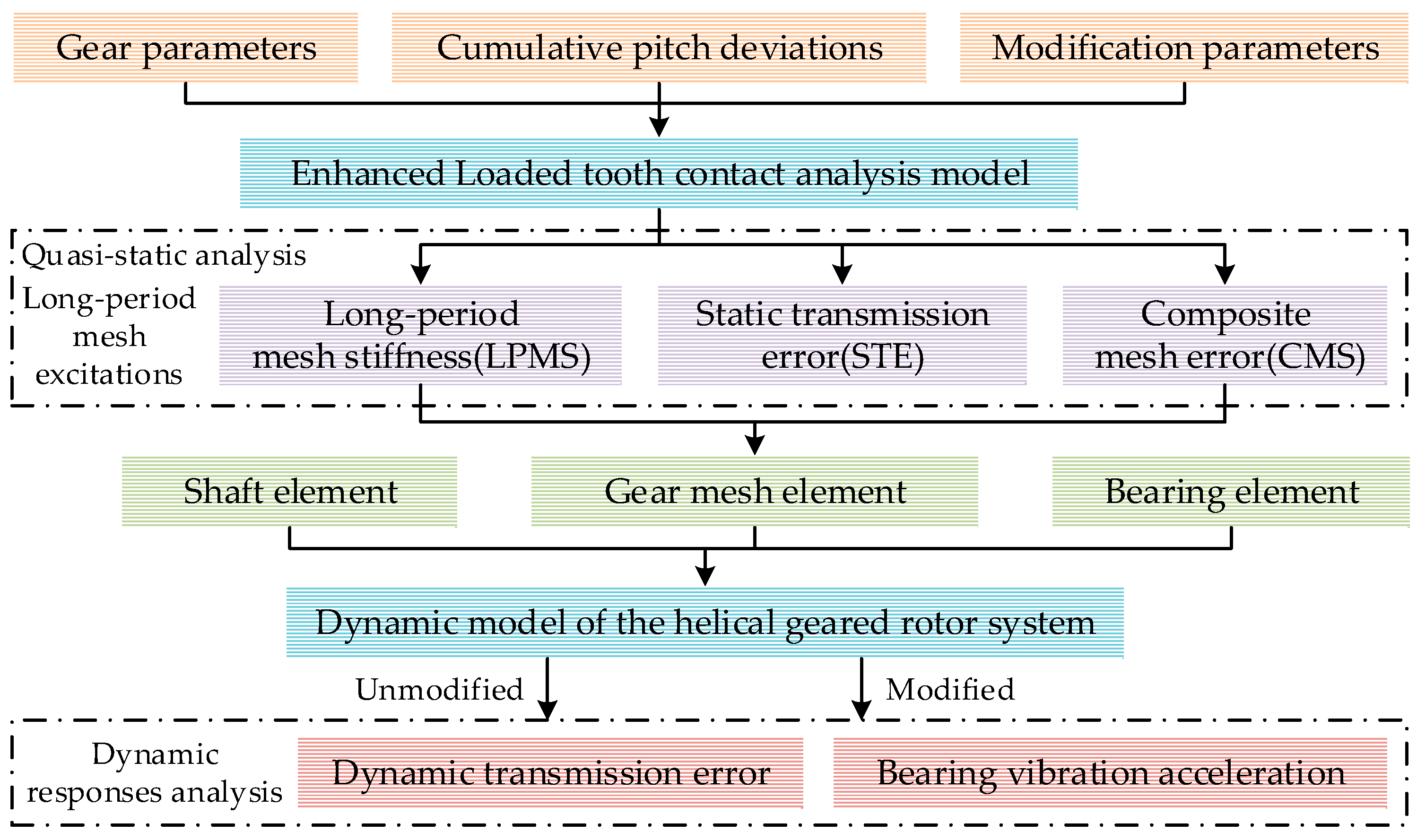
2. Description of the Models
2.1. Calculation Model of Mesh Excitations for Helical Gears with Cumulative Pitch Deviations
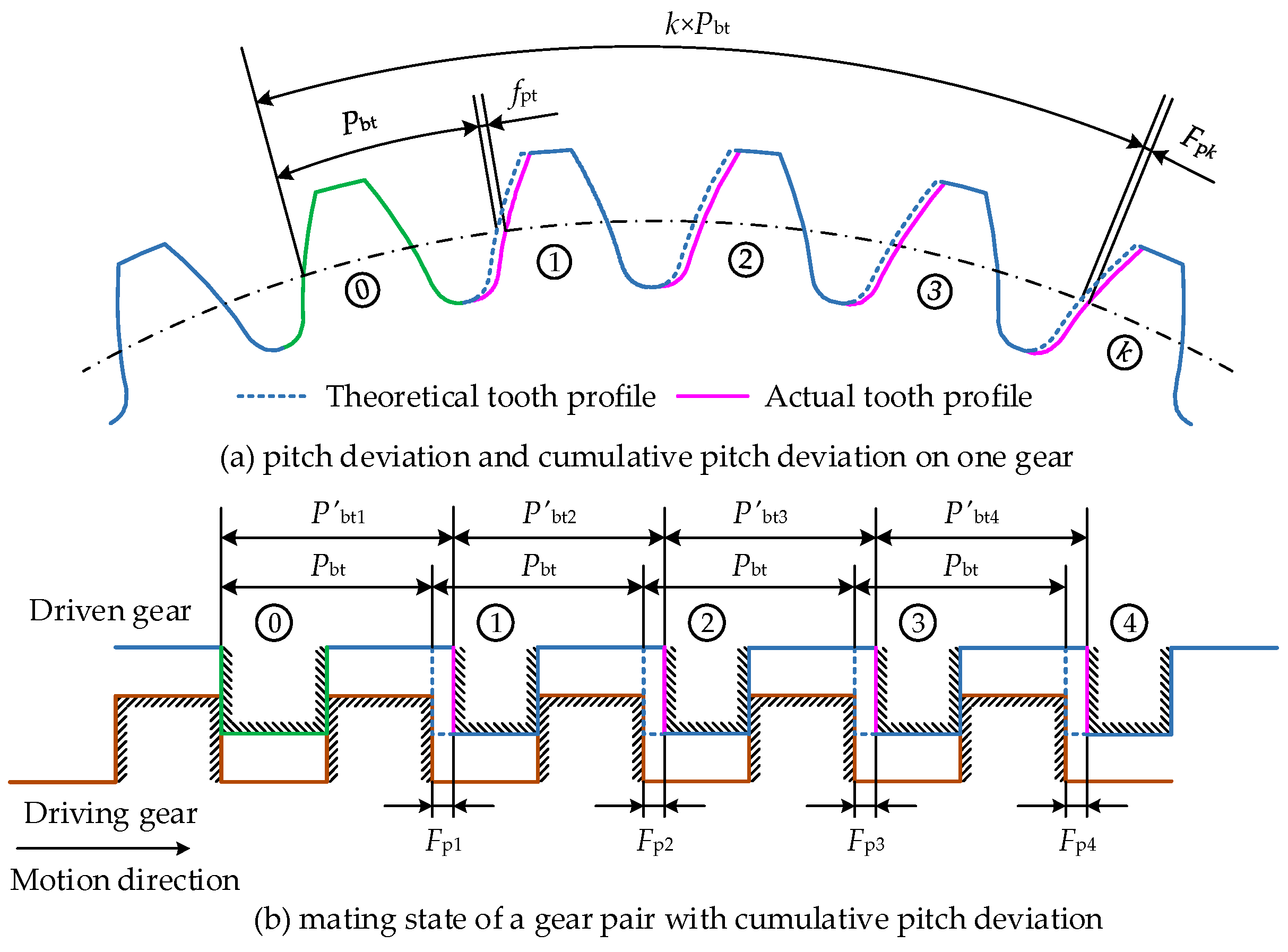
2.1.1. Description of Cumulative Pitch Deviations for Helical Gears
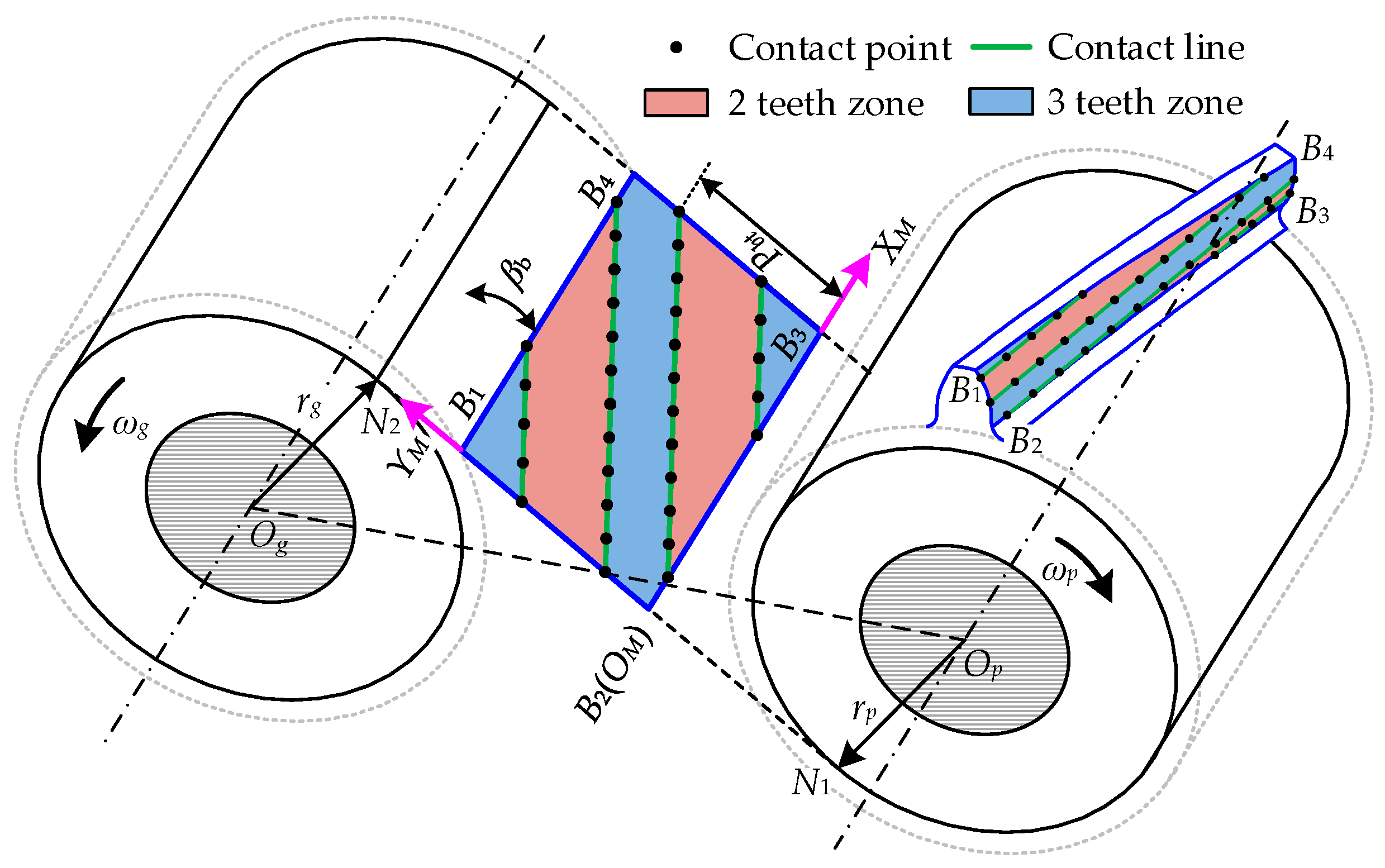
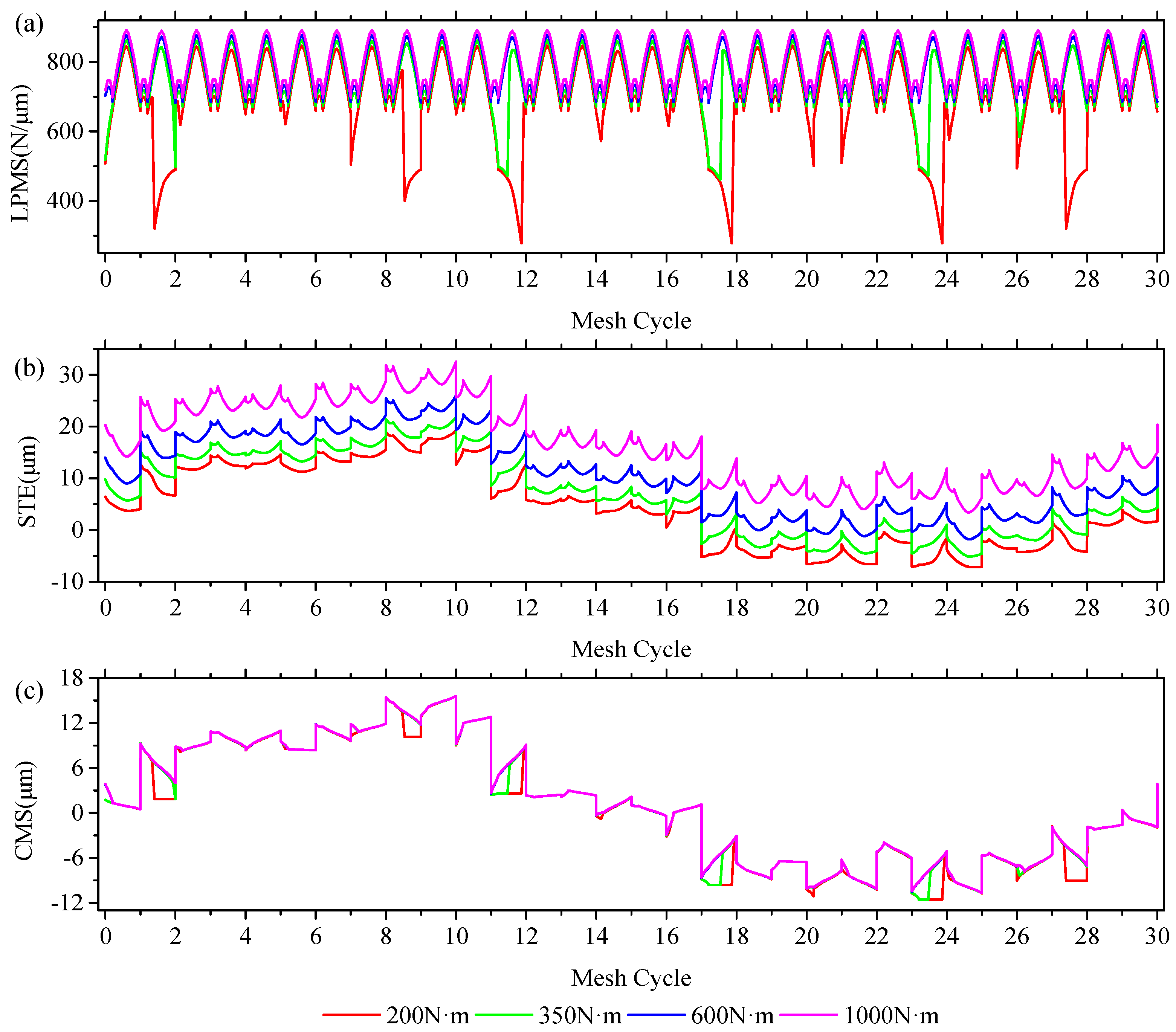
2.1.2. Determination of LPMS, STE, and CMS
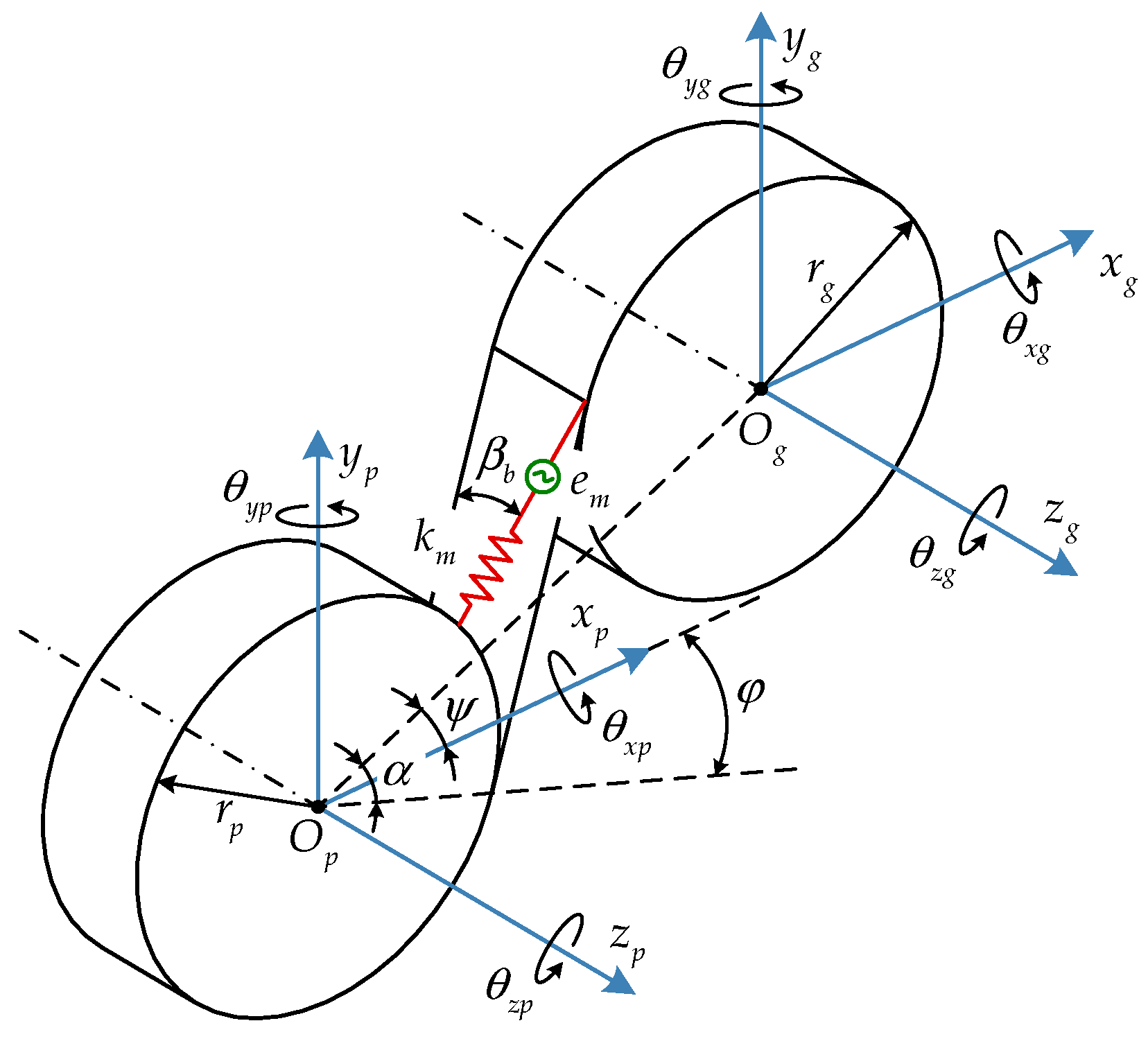
2.2. Dynamic Model of HGRS
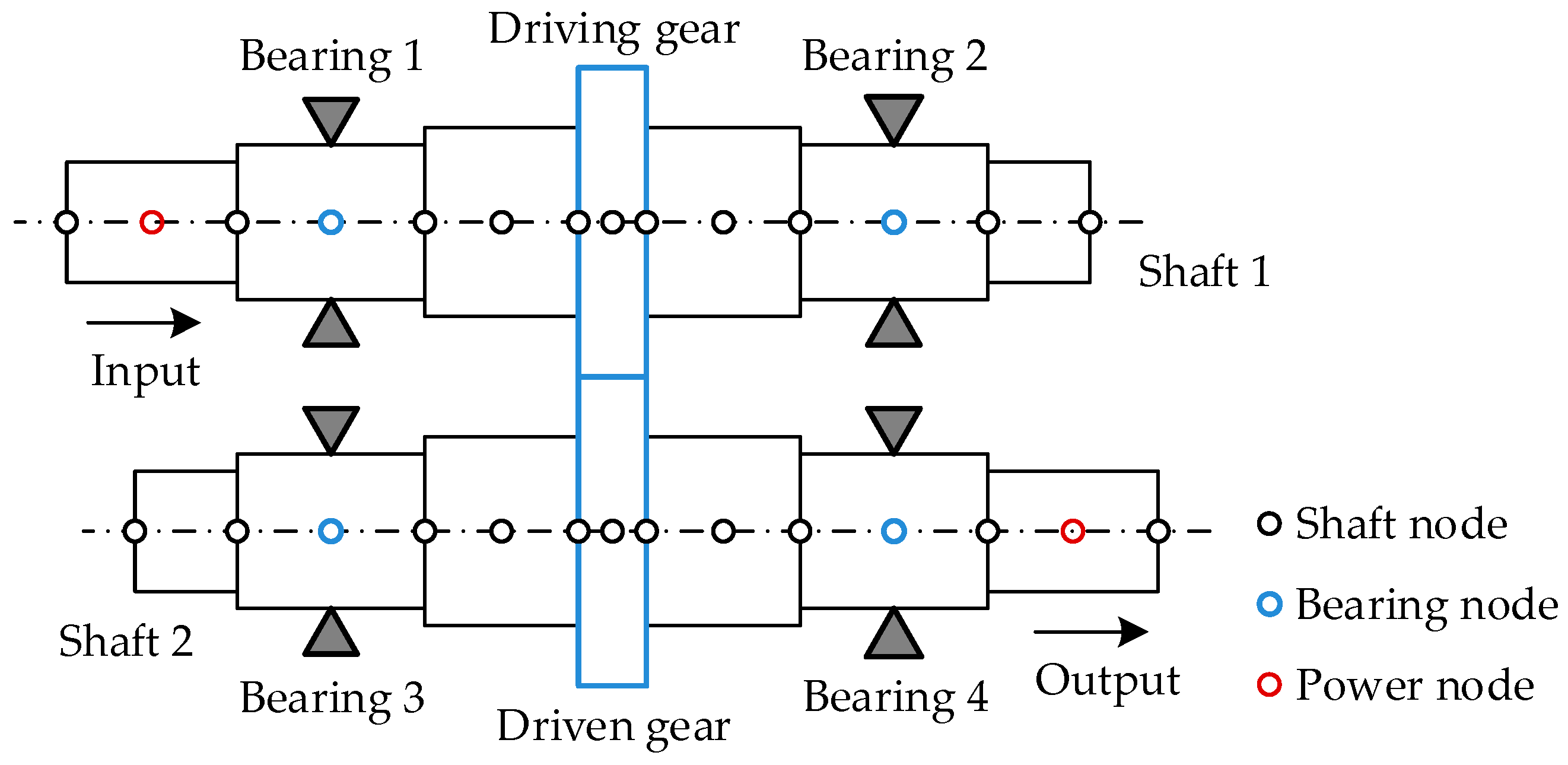
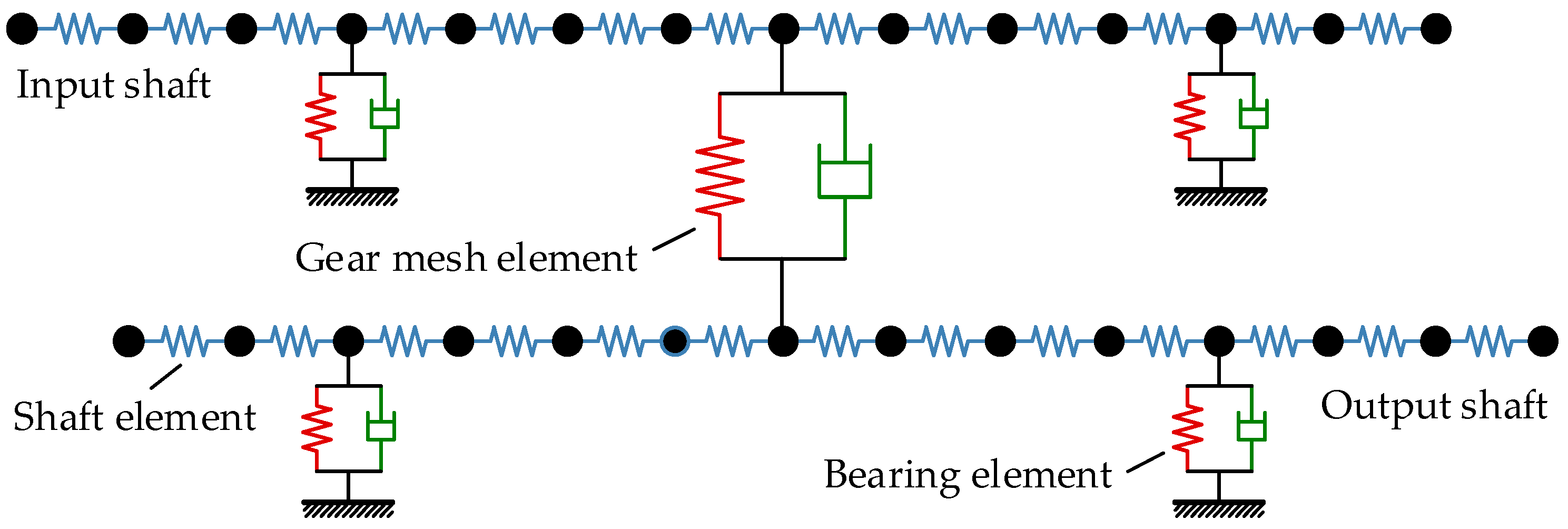
2.2.1. Element Models
2.2.2. System Model
3. Numerical Results and Discussion
3.1. Effect of Random Cumulative Pitch Deviations on Unmodified HGRS
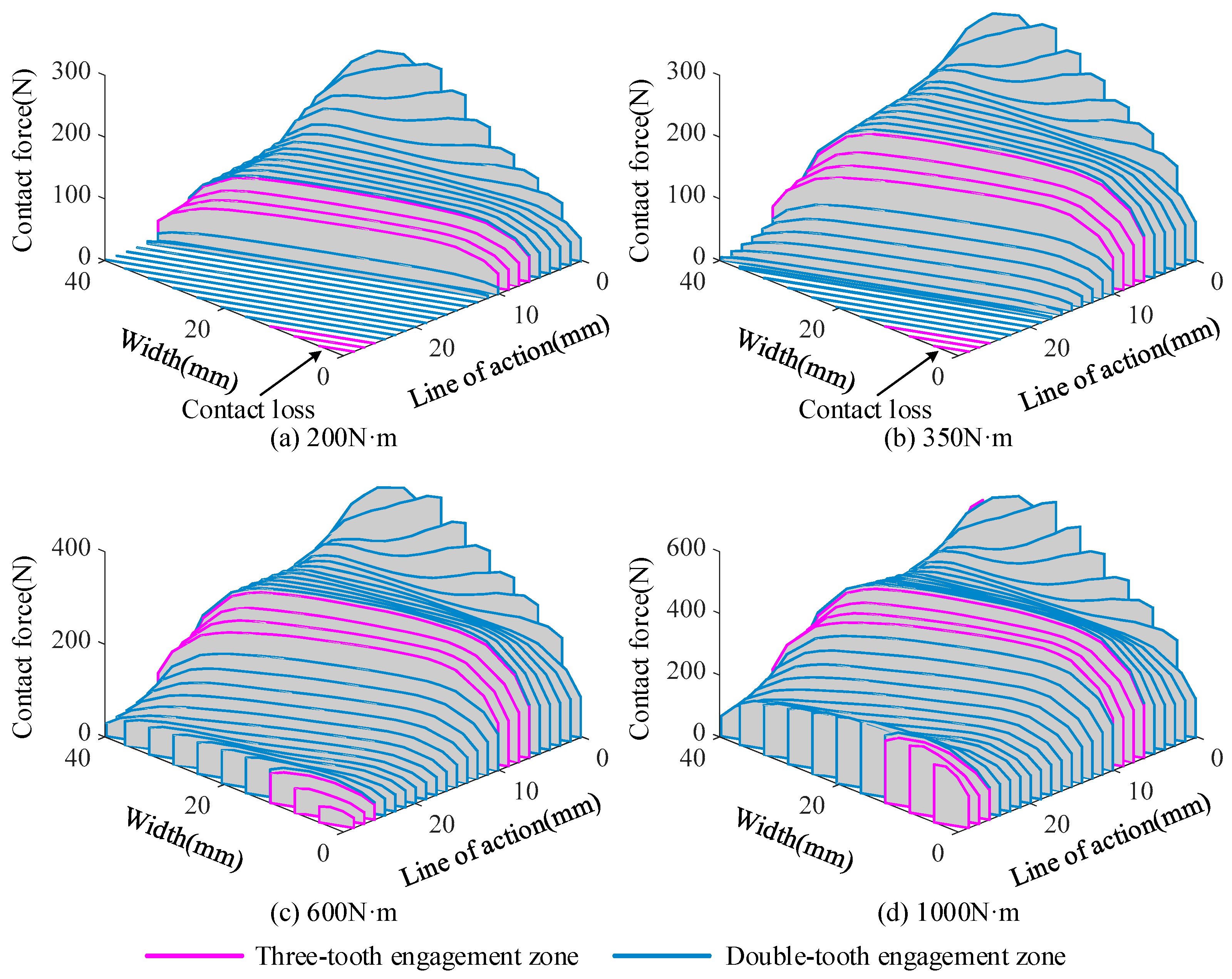
3.1.1. Quasi-Static Analysis
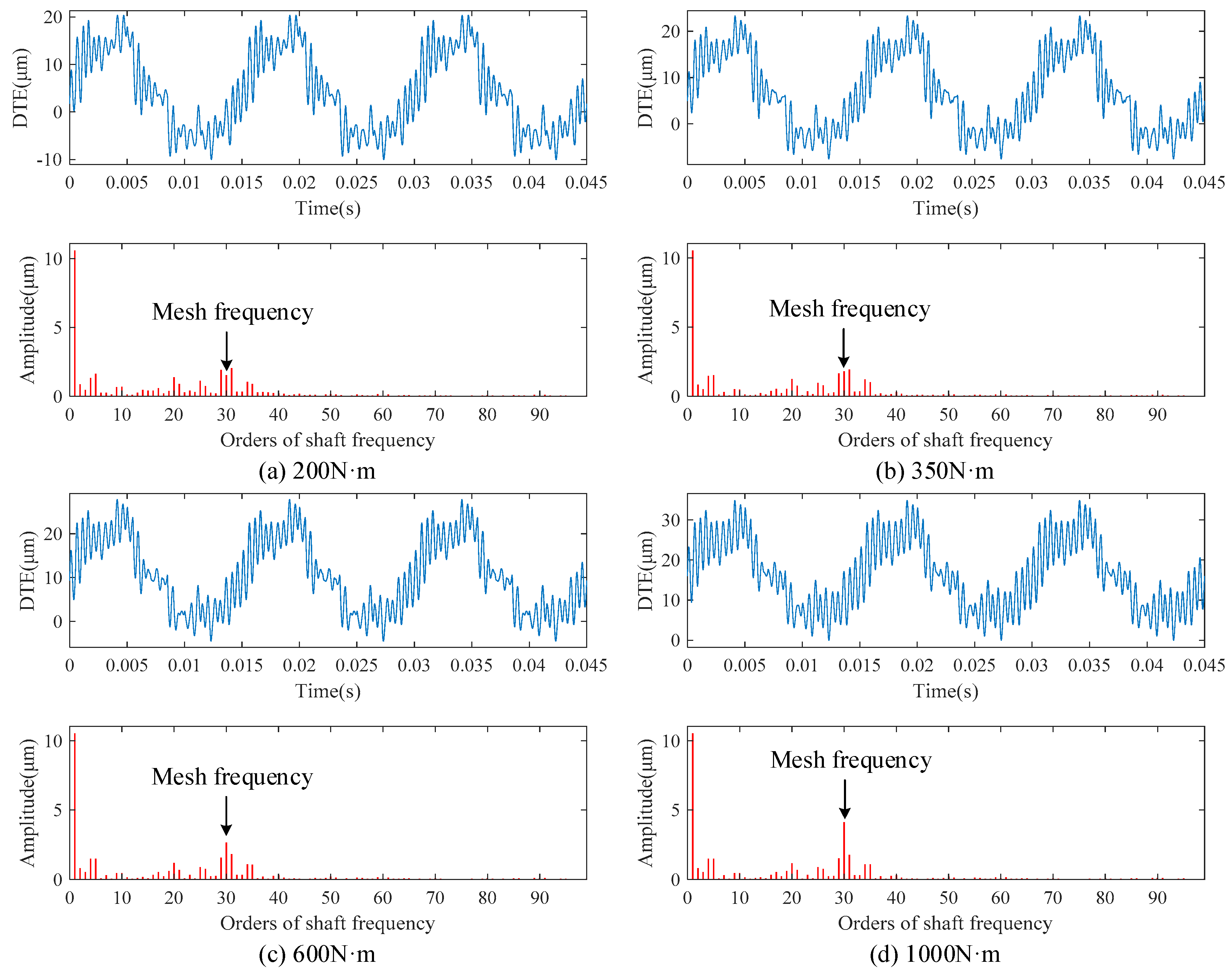
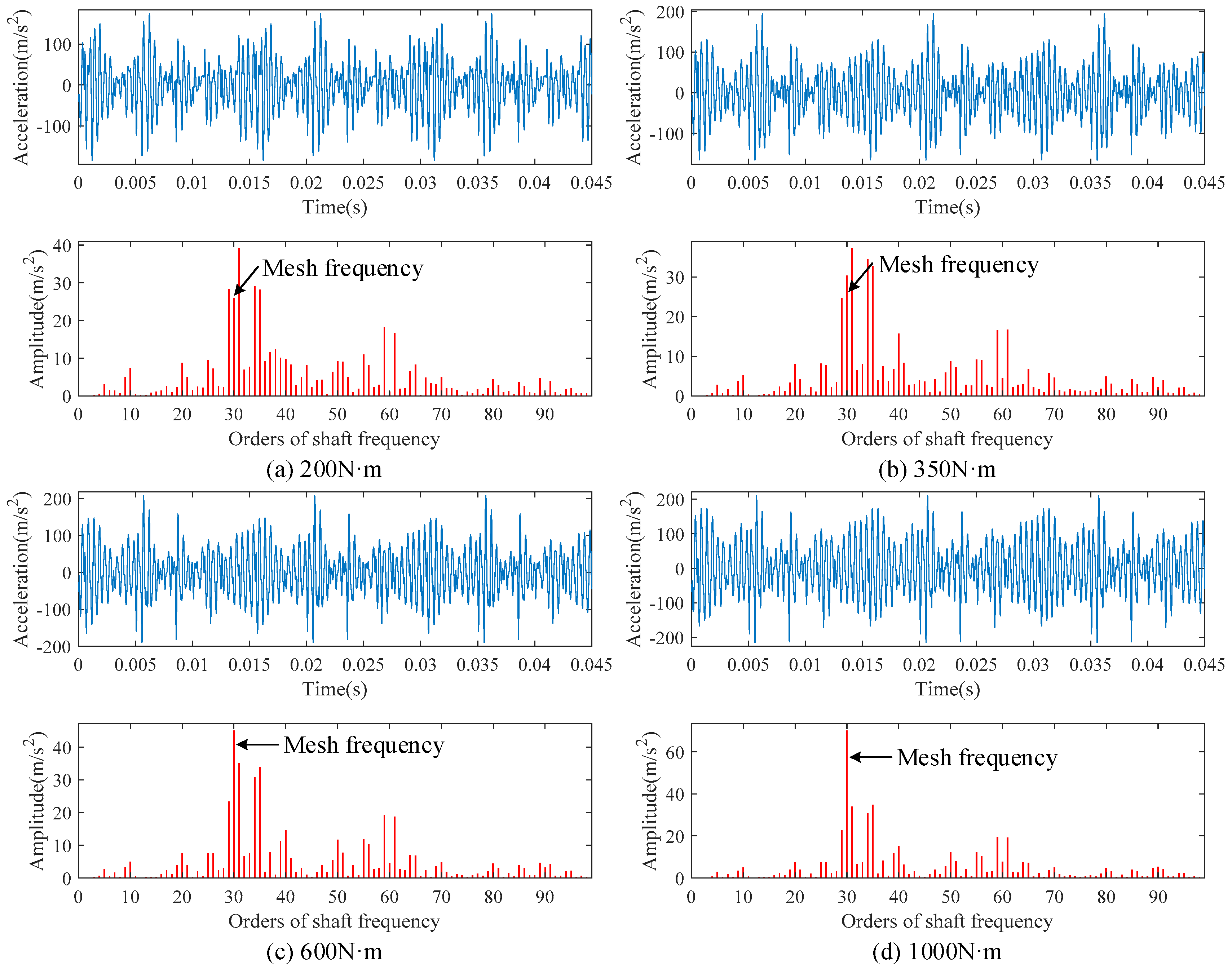
3.1.2. Dynamic Responses Analysis
3.2. Effect of Random Cumulative Pitch Deviations on Modified HGRS
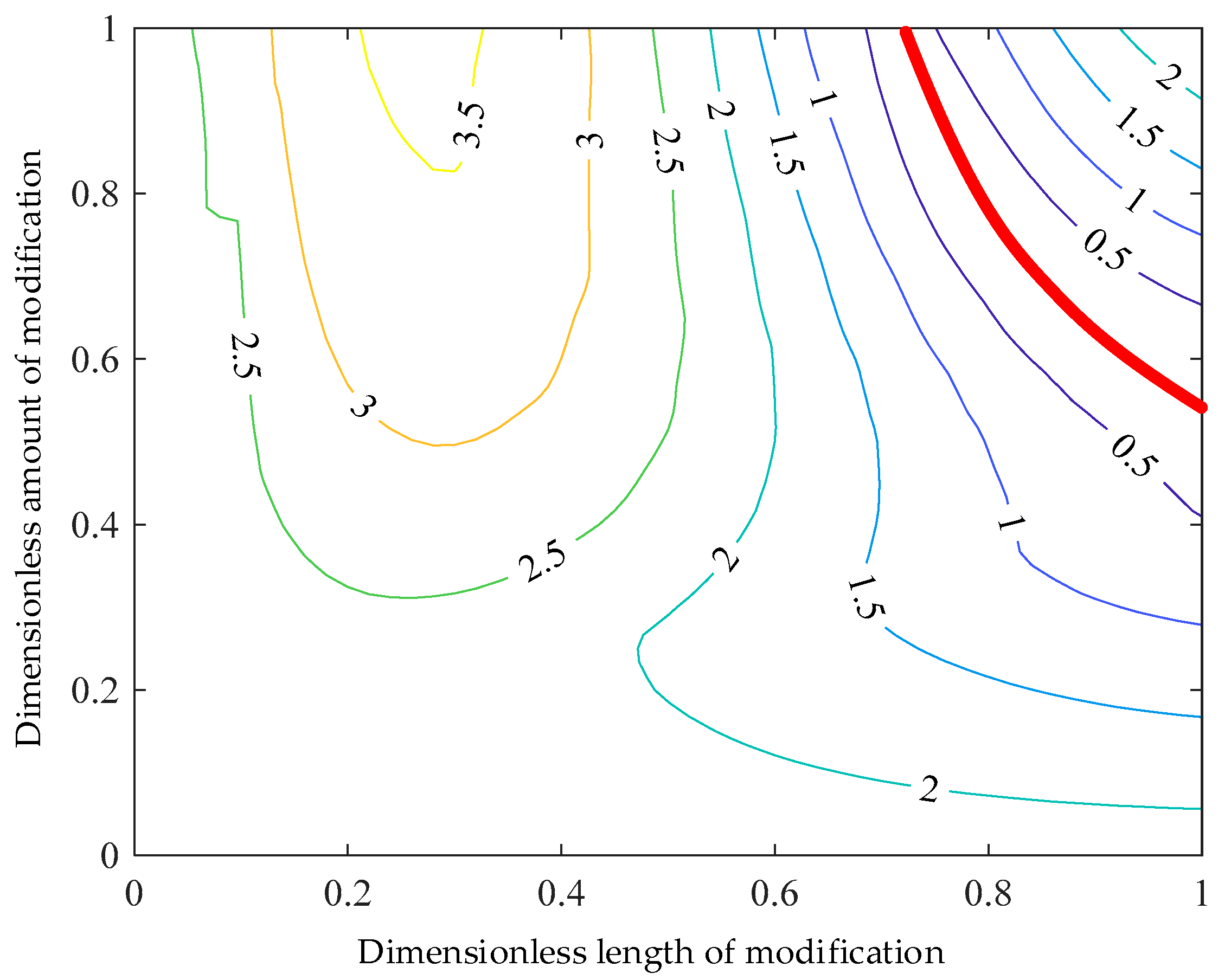
3.2.1. Design of Modification Parameters
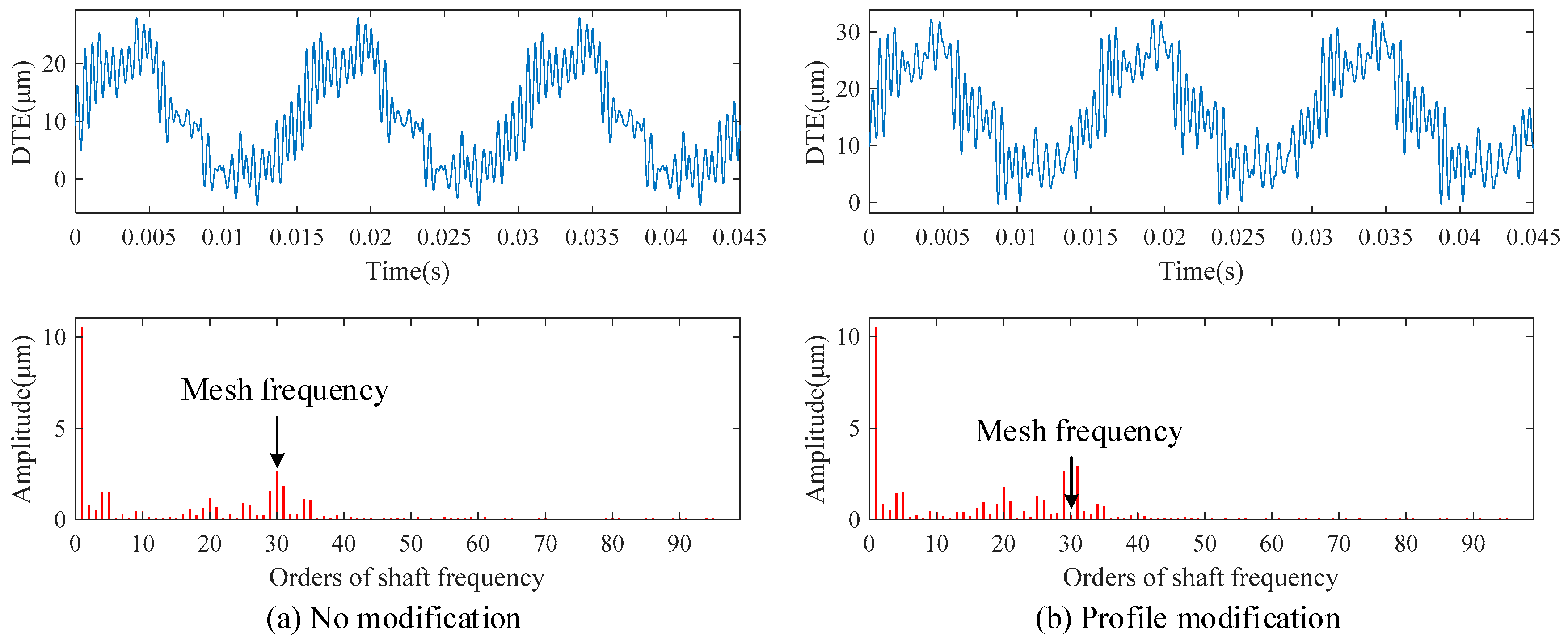
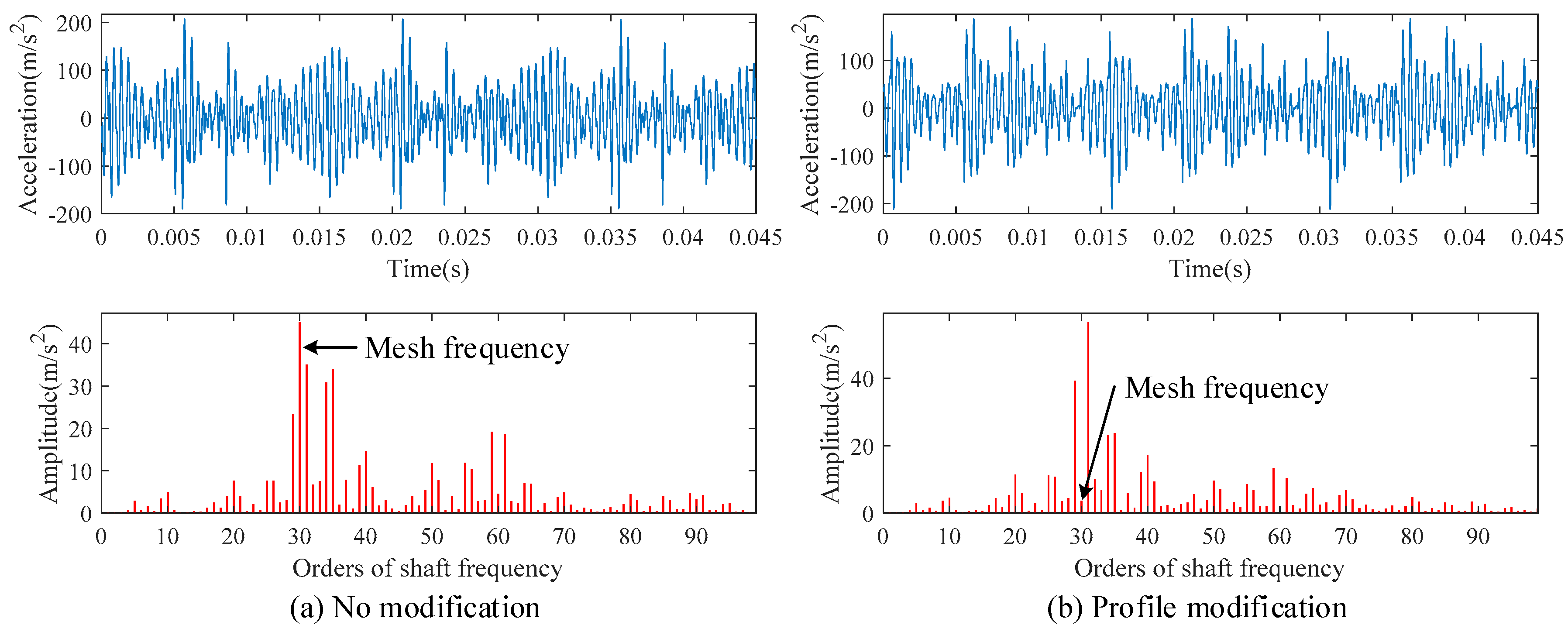
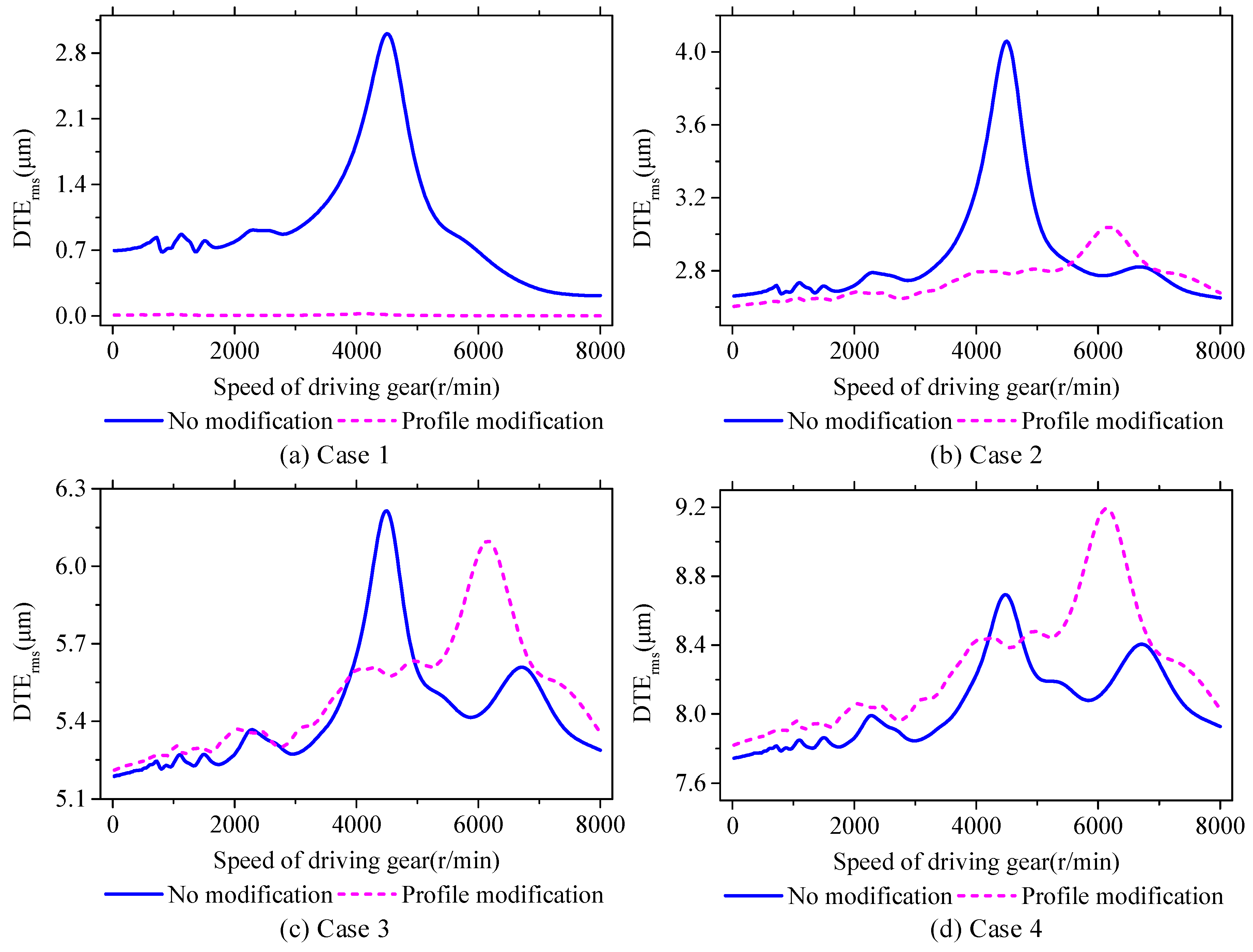
3.2.2. Dynamic Responses Analysis
4. Conclusions
- (1)
- considering random cumulative pitch deviations, the curve shapes of STE and CMS become irregular. When the load torque is relatively low, the LPMS in some mesh cycles will decrease due to partial contact loss. As the load torque increases, the actual contact region will become larger. When the contact patterns in each mesh cycle extend to full tooth surface, the curve shape of LPMS will become regular;
- (2)
- vibration modulation phenomenon can be observed in dynamic responses of HGRS with random cumulative pitch deviation, and the random characteristic of cumulative pitch deviations makes the vibration frequency spectrum complex. When HGRS is under light load conditions, the amplitudes of some sideband frequencies are larger than that of MFIHs because of relatively high contact ratio. With the increase of load torque, the amplitudes of MFIHs will increase and become larger than that of sideband frequencies;
- (3)
- profile modification can reduce the amplitudes of MFIHs in DTEs and bear vibration acceleration. However, with the increase of the amplitude of cumulative pitch deviations, the effectiveness of profile modification in reducing system vibration will become weaker. In some working conditions, profile modification will lead to stronger system vibration for HGRS, owing to the increased amplitudes of sideband frequencies.
Author Contributions
Funding
Conflicts of Interest
References
- Byrtus, M.; Zeman, V. On modeling and vibration of gear drives influenced by nonlinear couplings. Mech. Mach. Theory 2011, 46, 375–397. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, L.; Li, H.; Dai, Y. Nonlinear dynamic response of a spur gear pair based on the modeling of periodic mesh stiffness and static transmission error. Appl. Math. Model. 2019, 72, 444–469. [Google Scholar] [CrossRef]
- Chen, S.; Tang, J.; Luo, C.; Wang, Q. Nonlinear dynamic characteristics of geared rotor bearing systems with dynamic backlash and friction. Mech. Mach. Theory 2011, 46, 466–478. [Google Scholar]
- Yang, Y.; Xu, M.; Du, Y.; Zhao, P.; Dai, Y. Dynamic analysis of nonlinear time-varying spur gear system subjected to multi-frequency excitation. J. Vib. Control 2019, 25, 1210–1226. [Google Scholar] [CrossRef]
- Hu, Z.; Tang, J.; Zhong, J.; Chen, S. Frequency spectrum and vibration analysis of high speed gear-rotor system with tooth root crack considering transmission error excitation. Eng. Fail. Anal. 2015, 60, 405–441. [Google Scholar] [CrossRef]
- Ye, S.Y.; Tsai, S.J. A computerized method for loaded tooth contact analysis of high-contact-ratio spur gears with or without flank modification considering tip corner contact and shaft misalignment. Mech. Mach. Theory 2016, 97, 190–214. [Google Scholar] [CrossRef]
- Fernández-del-Rincón, A.; Iglesias, M.; de-Juan, A.; García, P.; Sancibrián, R.; Viadero, F. Gear transmission dynamic: Effects of tooth profile deviations and support flexibility. Appl. Acoust. 2014, 77, 138–149. [Google Scholar] [CrossRef]
- Li, S. Effects of misalignment error, tooth modifications and transmitted torque on tooth engagements of a pair of spur gears. Mech. Mach. Theory 2015, 83, 125–136. [Google Scholar] [CrossRef]
- Sánchez, M.B.; Pleguezuelos, M.; Pedrero, J.I. Influence of profile modifications on meshing stiffness, load sharing, and transmission error of involute spur gears. Mech. Mach. Theory 2019, 139, 506–525. [Google Scholar] [CrossRef]
- Kim, J.; Gang, G.A.; Cho, S.; Lee, G.H.; Park, Y.-J. Dynamic stiffness effect of mechanical components on gear mesh misalignment. Appl. Sci. 2018, 8, 844. [Google Scholar] [CrossRef]
- Wei, J.; Sun, W.; Wang, L. Effects of flank deviation on load distributions for helical gear. J. Mech. Sci. Technol. 2011, 25, 1781–1789. [Google Scholar] [CrossRef]
- Velex, P.; Maatar, M. A mathematical model for analyzing the influence of shape deviations and mounting errors on gear dynamic behaviour. J. Sound Vib. 1996, 191, 629–660. [Google Scholar] [CrossRef]
- Peng, Y.; Zhao, N.; Qiu, P.; Zhang, M.; Li, W.; Zhou, R. An efficient model of load distribution for helical gears with modification and misalignment. Mech. Mach. Theory 2018, 121, 151–168. [Google Scholar] [CrossRef]
- Shehata, A.; Adnan, M.A.; Mohammed, O.D. Modeling the effect of misalignment and tooth microgeometry on helical gear pair in mesh. Eng. Fail. Anal. 2019, 106, 104190. [Google Scholar] [CrossRef]
- Chang, L.; Cao, X.; He, Z.; Liu, G. Load-related dynamic behaviors of a helical gear pair with tooth flank errors. J. Mech. Sci. Technol. 2018, 32, 1473–1487. [Google Scholar] [CrossRef]
- Wang, Q.; Zhang, Y. A model for analyzing stiffness and stress in a helical gear pair with tooth profile errors. J. Vib. Control 2017, 23, 272–289. [Google Scholar] [CrossRef]
- Chen, Z.; Shao, Y. Mesh stiffness calculation of a spur gear pair with tooth profile modification and tooth root crack. Mech. Mach. Theory 2013, 62, 63–74. [Google Scholar] [CrossRef]
- Sun, Y.; Ma, H.; Huangfu, Y.; Chen, K.; Che, L.; Wen, B. A revised time-varying mesh stiffness model of spur gear pairs with tooth modifications. Mech. Mach. Theory 2018, 129, 261–278. [Google Scholar] [CrossRef]
- Wang, Q.; Ma, H.; Kong, X.; Zhang, Y. A distributed dynamic mesh model of a helical gear pair with tooth profile errors. J. Cent. South Univ. 2018, 25, 287–303. [Google Scholar] [CrossRef]
- Yuan, B.; Chang, S.; Liu, G.; Chang, L.; Liu, L. Optimization of bias modification and dynamic behavior analysis of helical gear system. Adv. Mech. Eng. 2017, 9, 3252. [Google Scholar] [CrossRef]
- Benatar, M.; Handschuh, M.; Kahraman, A.; Talbot, D. Static and dynamic transmission error measurements of helical gear pairs with various tooth modifications. J. Mech. Des. 2019, 141, 103301. [Google Scholar] [CrossRef]
- Fernández-del-Rincón, A.; Iglesias, M.; de-Juan, A.; Diez-Ibarbia, A.; García, P.; Viadero, F. Gear transmission dynamics: Effects of index and run out errors. Appl. Acoust. 2016, 108, 63–83. [Google Scholar] [CrossRef]
- Talbot, D.; Sum, A.; Kahraman, A. Impact of tooth indexing errors on dynamic factors of spur gears: Experiments and model simulations. J. Mech. Des. 2016, 138, 093302. [Google Scholar] [CrossRef]
- Inalpolat, M.; Handschuh, M.; Kahraman, A. Influence of indexing errors on dynamic response of spur gear pairs. Mech. Syst. Signal Process. 2015, 60–61, 391–405. [Google Scholar] [CrossRef]
- Yuan, B.; Chang, S.; Liu, G.; Wu, L. Quasi-static and dynamic behaviors of helical gear system with manufacturing errors. Chin. J. Mech. Eng. 2018, 31, 1–9. [Google Scholar] [CrossRef]
- Chang, L.H.; Liu, G.; Wu, L.Y. A robust model for determining the mesh stiffness of cylindrical gears. Mech. Mach. Theory 2015, 87, 93–114. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, Q.; Ma, H.; Huang, J.; Zhao, C. Dynamic analysis of three-dimensional helical geared rotor system with geometric eccentricity. J. Mech. Sci. Technol. 2013, 27, 3231–3242. [Google Scholar] [CrossRef]
- Handschuh, M.J.; Kahraman, A.; Milliren, M.R. Impact of tooth spacing errors on the root stresses of spur gear pairs. J. Mech. Des. 2014, 136, 061010. [Google Scholar] [CrossRef]
- Chen, Y. Time-varying dynamic analysis of a helical-geared rotor-bearing system with three-dimensional motion due to shaft deformation. Appl. Sci. 2020, 10, 1542. [Google Scholar] [CrossRef]
- Kubur, M.; Kahraman, A.; Zini, D.M.; Kienzle, K. Dynamic analysis of a multi-shaft helical gear transmission by finite elements: Model and experiment. J. Mech. Des. 2004, 126, 398–406. [Google Scholar] [CrossRef]
- Hu, Z.H.; Tang, J.Y.; Zhong, J.; Chen, S. Effects of tooth profile modification on dynamic responses of a high speed gear-rotor-bearing system. Mech. Syst. Signal Process. 2016, 76, 294–318. [Google Scholar] [CrossRef]
- Hong, J.; Talbot, D.; Kahraman, A. Effects of tooth indexing errors on load distribution and tooth load sharing of splines under combined loading conditions. J. Mech. Des. 2015, 137, 032601. [Google Scholar] [CrossRef]
- Wei, J.; Zhang, A.; Wang, G.; Qin, D.; Lim, T.C.; Wang, Y.; Lin, T. A study of nonlinear excitation modeling of helical gears with modification: Theoretical analysis and experiments. Mech. Mach. Theory 2018, 128, 314–335. [Google Scholar] [CrossRef]
- Bruyère, J.; Velex, P.; Guilbert, B.; Houser, D.R. An analytical study on the combination of profile relief and lead crown minimizing transmission error in narrow-faced helical gears. Mech. Mach. Theory 2019, 136, 224–243. [Google Scholar] [CrossRef]

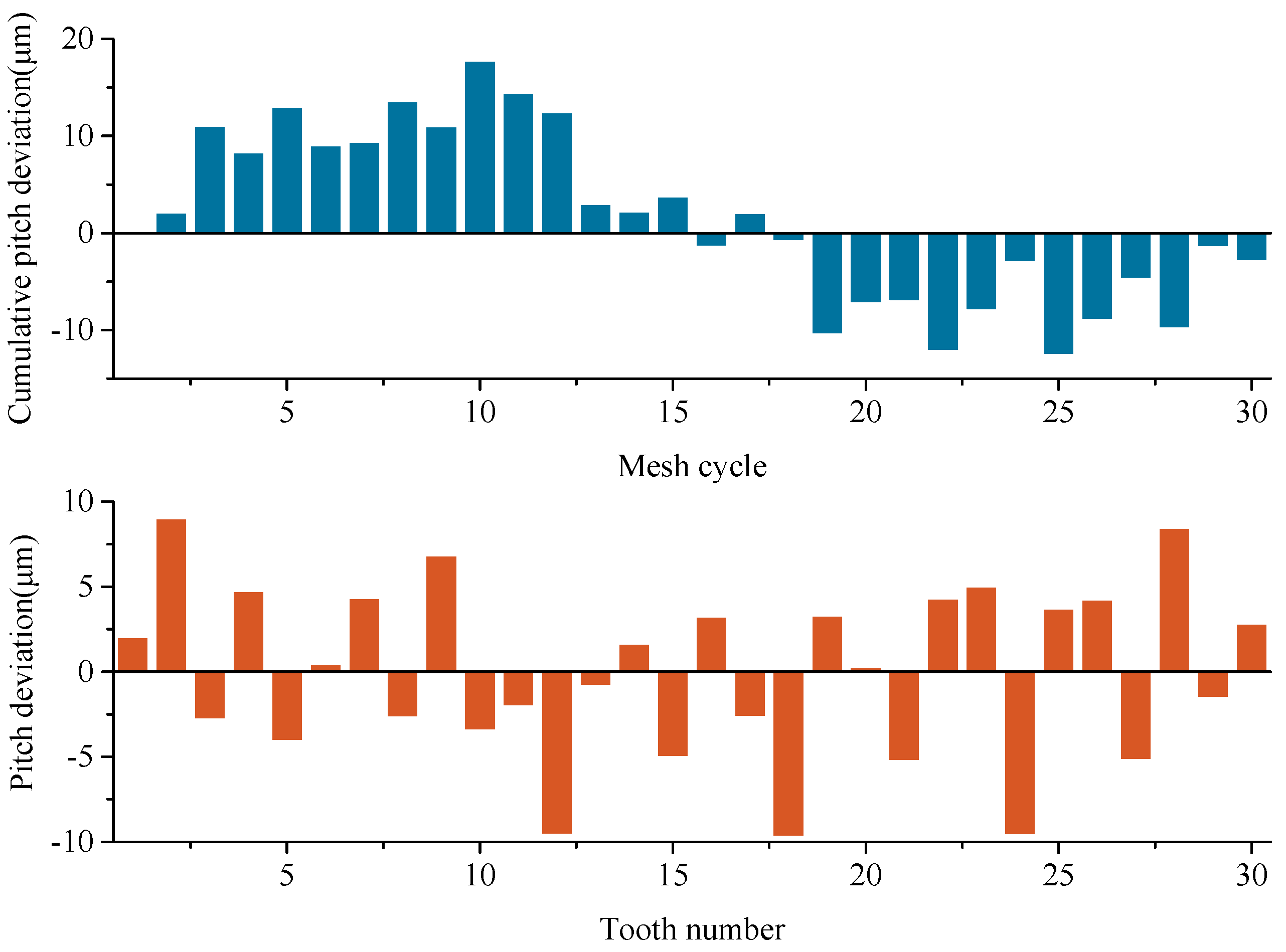

| Number of Teeth | 30/30 | Addendum Coefficient | 1 |
|---|---|---|---|
| Normal module/mm | 6 | Bottom clearance coefficient | 0.25 |
| Pressure angle/° | 20 | Width/mm | 40 |
| Helix angle/° | ±18 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, B.; Liu, G.; Liu, L. Quasi-Static Characteristics and Vibration Responses Analysis of Helical Geared Rotor System with Random Cumulative Pitch Deviations. Appl. Sci. 2020, 10, 4403. https://doi.org/10.3390/app10124403
Yuan B, Liu G, Liu L. Quasi-Static Characteristics and Vibration Responses Analysis of Helical Geared Rotor System with Random Cumulative Pitch Deviations. Applied Sciences. 2020; 10(12):4403. https://doi.org/10.3390/app10124403
Chicago/Turabian StyleYuan, Bing, Geng Liu, and Lan Liu. 2020. "Quasi-Static Characteristics and Vibration Responses Analysis of Helical Geared Rotor System with Random Cumulative Pitch Deviations" Applied Sciences 10, no. 12: 4403. https://doi.org/10.3390/app10124403
APA StyleYuan, B., Liu, G., & Liu, L. (2020). Quasi-Static Characteristics and Vibration Responses Analysis of Helical Geared Rotor System with Random Cumulative Pitch Deviations. Applied Sciences, 10(12), 4403. https://doi.org/10.3390/app10124403





