An Advanced Shear Strength Criterion for Rock Discontinuities Considering Size and Low Shear Rate
Abstract
1. Introduction
2. Method
2.1. Establishment of the New Shear Strength Equation
2.2. Design of the Direct Shear Test
2.3. Verification of the New Strength Equation
3. Results
3.1. Experimental Results
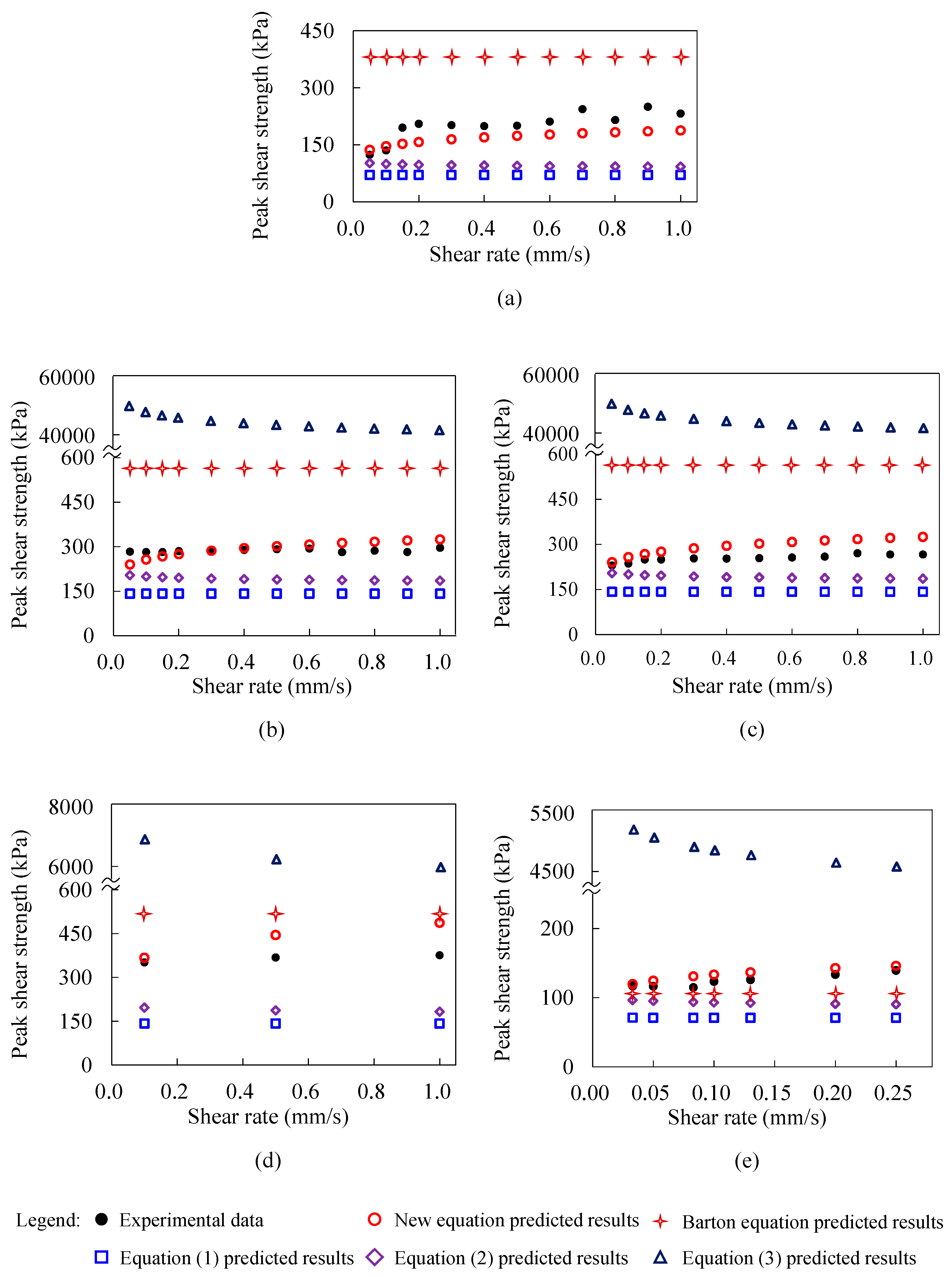
3.2. Comparison Experimental Data with Predicted Results
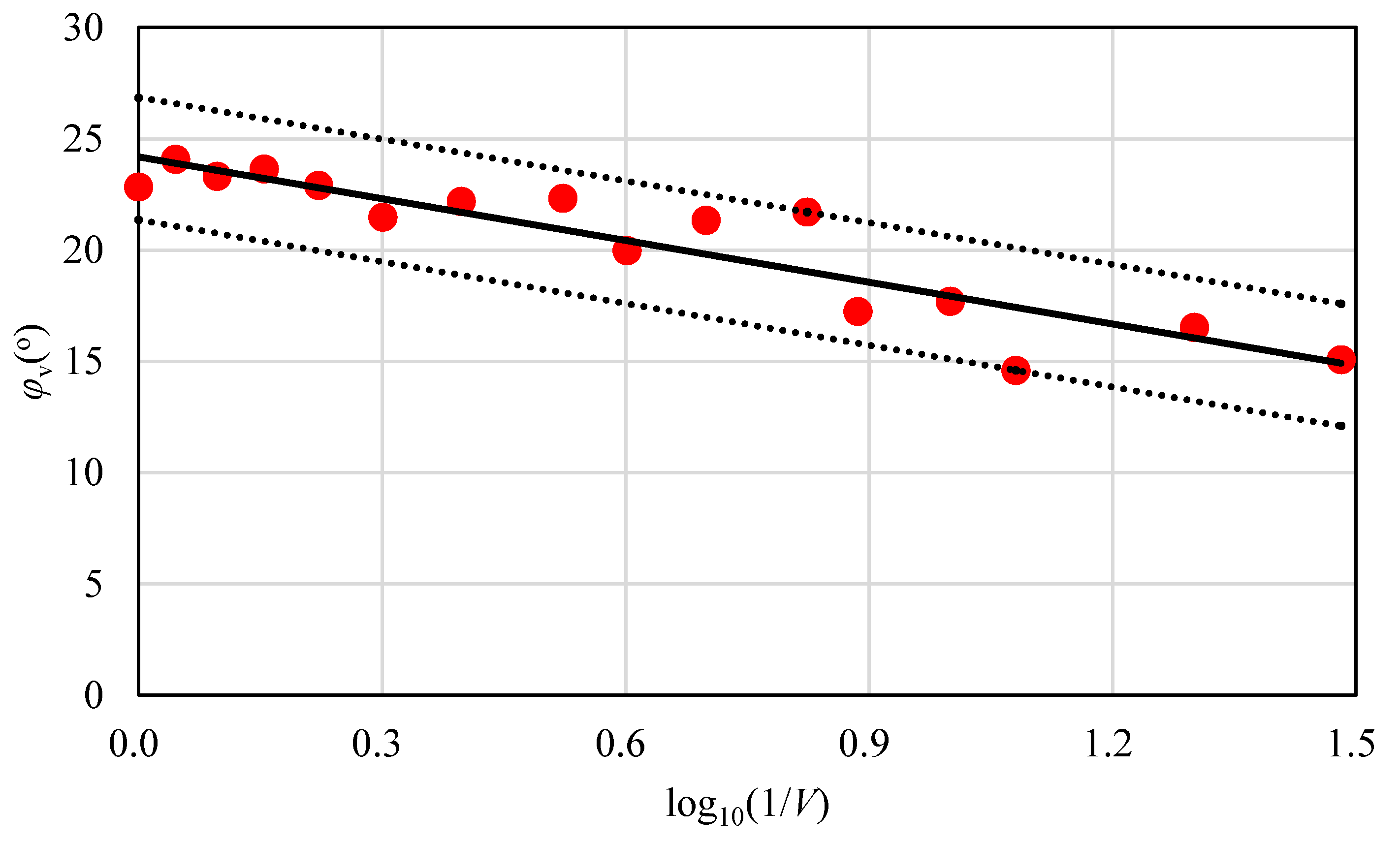
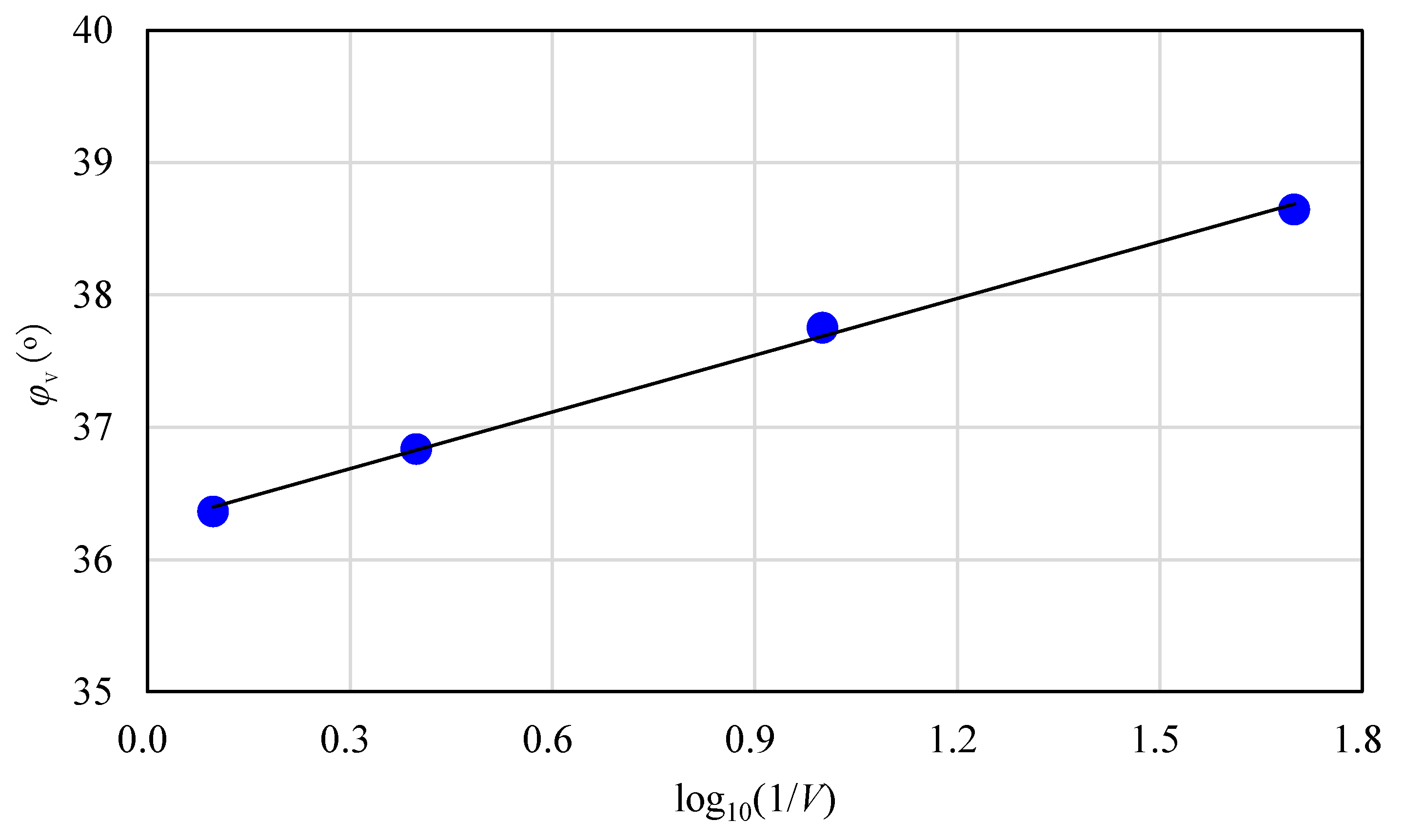
3.2.1. Rate-Dependent Friction Angle φv
3.2.2. Peak Shear Strength
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Sample Number | Normal Stress (kPa) | Shear Rate (mm/s) | Peak Shear Strength (kPa) |
|---|---|---|---|
| Sample 1# | 100 | 0.05 | 123.85 |
| 0.1 | 135.4 | ||
| 0.15 | 195.7 | ||
| 0.2 | 205.55 | ||
| 0.3 | 202.25 | ||
| 0.4 | 199.5 | ||
| 0.5 | 200.6 | ||
| 0.6 | 211.6 | ||
| 0.7 | 243.9 | ||
| 0.8 | 215.4 | ||
| 0.9 | 250.5 | ||
| 1.0 | 232.4 | ||
| Sample 2# | 200 | 0.05 | 283.7 |
| 0.1 | 282.6 | ||
| 0.15 | 282.6 | ||
| 0.2 | 285.9 | ||
| 0.3 | 289.75 | ||
| 0.4 | 289.75 | ||
| 0.5 | 291.9 | ||
| 0.6 | 294.1 | ||
| 0.7 | 282.05 | ||
| 0.8 | 286.95 | ||
| 0.9 | 282.6 | ||
| 1.0 | 296.85 | ||
| Sample 3# | 200 | 0.05 | 229.5 |
| 0.1 | 236.05 | ||
| 0.15 | 249.2 | ||
| 0.2 | 249.2 | ||
| 0.3 | 253.05 | ||
| 0.4 | 252.5 | ||
| 0.5 | 254.15 | ||
| 0.6 | 256.35 | ||
| 0.7 | 259.05 | ||
| 0.8 | 270.55 | ||
| 0.9 | 266.2 | ||
| 1.0 | 266.2 | ||
| Sample 4# | 200 | 0.1 | 351.35 |
| 0.5 | 368.35 | ||
| 1.0 | 376.05 | ||
| Sample 5# | 100 | 0.033 | 116.788 |
| 0.05 | 116.664 | ||
| 0.083 | 114.876 | ||
| 0.1 | 123.496 | ||
| 0.13 | 126.148 | ||
| 0.2 | 133.58 | ||
| 0.25 | 139.364 |
| Sample Type | Shear Rate V (mm/s) | Peak Shear Strength τ (MPa) | Normal Stress σ (MPa) | Arctan (τ/σ) (°) | JRCn∙log10 (JCSn/σ) (°) |
|---|---|---|---|---|---|
| Granite discontinuity (17°) | 0.05 | 0.124 | 0.1 | 51.082 | 36.994 |
| 0.05 | 0.284 | 0.2 | 54.817 | 33.404 | |
| 0.05 | 0.230 | 0.2 | 48.929 | 33.404 | |
| 0.1 | 0.135 | 0.1 | 53.552 | 36.994 | |
| 0.1 | 0.283 | 0.2 | 54.712 | 33.404 | |
| 0.1 | 0.236 | 0.2 | 49.726 | 33.404 | |
| 0.15 | 0.196 | 0.1 | 62.934 | 36.994 | |
| 0.15 | 0.283 | 0.2 | 54.712 | 33.404 | |
| 0.15 | 0.249 | 0.2 | 51.251 | 33.404 | |
| 0.2 | 0.206 | 0.1 | 64.057 | 36.994 | |
| 0.2 | 0.286 | 0.2 | 55.025 | 33.404 | |
| 0.2 | 0.249 | 0.2 | 51.251 | 33.404 | |
| 0.3 | 0.202 | 0.1 | 63.690 | 36.994 | |
| 0.3 | 0.290 | 0.2 | 55.385 | 33.404 | |
| 0.3 | 0.253 | 0.2 | 51.679 | 33.404 | |
| 0.4 | 0.200 | 0.1 | 63.378 | 36.994 | |
| 0.4 | 0.290 | 0.2 | 55.385 | 33.404 | |
| 0.4 | 0.253 | 0.2 | 51.618 | 33.404 | |
| 0.5 | 0.201 | 0.1 | 63.504 | 36.994 | |
| 0.5 | 0.292 | 0.2 | 55.582 | 33.404 | |
| 0.5 | 0.254 | 0.2 | 51.799 | 33.404 | |
| 0.6 | 0.212 | 0.1 | 64.705 | 36.994 | |
| 0.6 | 0.294 | 0.2 | 55.783 | 33.404 | |
| 0.6 | 0.256 | 0.2 | 52.039 | 33.404 | |
| 0.7 | 0.244 | 0.1 | 67.706 | 36.994 | |
| 0.7 | 0.282 | 0.2 | 54.660 | 33.404 | |
| 0.7 | 0.259 | 0.2 | 52.330 | 33.404 | |
| 0.8 | 0.215 | 0.1 | 65.097 | 36.994 | |
| 0.8 | 0.287 | 0.2 | 55.124 | 33.404 | |
| 0.8 | 0.271 | 0.2 | 53.527 | 33.404 | |
| 0.9 | 0.251 | 0.1 | 68.238 | 36.994 | |
| 0.9 | 0.283 | 0.2 | 54.712 | 33.404 | |
| 0.9 | 0.266 | 0.2 | 53.082 | 33.404 | |
| 1 | 0.232 | 0.1 | 66.718 | 36.994 | |
| 1 | 0.297 | 0.2 | 56.030 | 33.404 | |
| 1 | 0.266 | 0.2 | 53.082 | 33.404 | |
| Granite discontinuity (16°) | 0.1 | 0.351 | 0.2 | 60.350 | 42.677 |
| 0.5 | 0.368 | 0.2 | 61.500 | 42.677 | |
| 1 | 0.376 | 0.2 | 61.994 | 42.677 | |
| Granite discontinuity (13°) | 0.033 | 0.117 | 0.1 | 49.428 | 34.363 |
| 0.05 | 0.117 | 0.1 | 49.398 | 34.363 | |
| 0.083 | 0.115 | 0.1 | 48.960 | 34.363 | |
| 0.1 | 0.123 | 0.1 | 51.001 | 34.363 | |
| 0.13 | 0.126 | 0.1 | 51.595 | 34.363 | |
| 0.2 | 0.134 | 0.1 | 53.181 | 34.363 | |
| 0.25 | 0.139 | 0.1 | 54.339 | 34.363 |
| Sample Type | Shear Rate V (mm/s) | Average Value φv (°) |
|---|---|---|
| Granite discontinuity | 0.033 | 15.065 |
| 0.05 | 16.515 | |
| 0.083 | 14.597 | |
| 0.1 | 17.700 | |
| 0.13 | 17.232 | |
| 0.15 | 21.698 | |
| 0.2 | 21.337 | |
| 0.25 | 19.976 | |
| 0.3 | 22.317 | |
| 0.4 | 22.193 | |
| 0.5 | 21.477 | |
| 0.6 | 22.908 | |
| 0.7 | 23.631 | |
| 0.8 | 23.315 | |
| 0.9 | 24.077 | |
| 1 | 22.836 |
| Sample Type | Shear Rate V (mm/s) | Peak Shear Strength τ (MPa) | Normal Stress σ (MPa) | Arctan (τ/σ) (°) | JRCn∙log10 (JCSn/σ) (°) |
|---|---|---|---|---|---|
| Cement discontinuity | 0.02 | 1.695 | 1 | 14.213 | 17.737 |
| 0.02 | 2.39 | 1 | 20.762 | 25.911 | |
| 0.02 | 2.71 | 1 | 40.309 | 50.306 | |
| 0.02 | 3.11 | 2 | 14.213 | 13.459 | |
| 0.02 | 4.208 | 2 | 20.762 | 19.661 | |
| 0.02 | 4.58 | 2 | 40.309 | 38.171 | |
| 0.02 | 4.45 | 3 | 14.213 | 10.956 | |
| 0.02 | 6.289 | 3 | 20.762 | 16.005 | |
| 0.02 | 6.69 | 3 | 40.309 | 31.073 | |
| 0.1 | 1.675 | 1 | 14.213 | 17.737 | |
| 0.1 | 2.33 | 1 | 20.762 | 25.911 | |
| 0.1 | 2.63 | 1 | 40.309 | 50.306 | |
| 0.1 | 3.02 | 2 | 14.213 | 13.459 | |
| 0.1 | 4.02 | 2 | 20.762 | 19.661 | |
| 0.1 | 4.31 | 2 | 40.309 | 38.171 | |
| 0.1 | 4.3 | 3 | 14.213 | 10.956 | |
| 0.1 | 5.96 | 3 | 20.762 | 16.005 | |
| 0.1 | 6.27 | 3 | 40.309 | 31.073 | |
| 0.4 | 1.64 | 1 | 14.213 | 17.737 | |
| 0.4 | 2.29 | 1 | 20.762 | 25.911 | |
| 0.4 | 2.57 | 1 | 40.309 | 50.306 | |
| 0.4 | 2.83 | 2 | 14.213 | 13.459 | |
| 0.4 | 3.81 | 2 | 20.762 | 19.661 | |
| 0.4 | 4.14 | 2 | 40.309 | 38.171 | |
| 0.4 | 4.09 | 3 | 14.213 | 10.956 | |
| 0.4 | 5.79 | 3 | 20.762 | 16.005 | |
| 0.4 | 5.99 | 3 | 40.309 | 31.073 | |
| 0.8 | 1.63 | 1 | 14.213 | 17.737 | |
| 0.8 | 2.28 | 1 | 20.762 | 25.911 | |
| 0.8 | 2.55 | 1 | 40.309 | 50.306 | |
| 0.8 | 2.75 | 2 | 14.213 | 13.459 | |
| 0.8 | 3.75 | 2 | 20.762 | 19.661 | |
| 0.8 | 4.03 | 2 | 40.309 | 38.171 | |
| 0.8 | 3.95 | 3 | 14.213 | 10.956 | |
| 0.8 | 5.61 | 3 | 20.762 | 16.005 | |
| 0.8 | 5.89 | 3 | 40.309 | 31.073 |
| Sample Type | Shear Rate V (mm/s) | Average Value φv (°) |
|---|---|---|
| Cement discontinuity | 0.02 | 38.647 |
| 0.1 | 37.754 | |
| 0.4 | 36.835 | |
| 0.8 | 36.362 |
| Sample Number | Normal Stress (kPa) | Shear Rate (mm/s) | Experimental Result (kPa) | Predicted Result (kPa) | Error Value (kPa) | Ratio of Error Value to Experimental Result (%) |
|---|---|---|---|---|---|---|
| Sample 1# | 100 | 0.05 | 123.85 | 136.91 | 13.06 | 10.55 |
| 0.1 | 135.4 | 146.81 | 11.41 | 8.43 | ||
| 0.15 | 195.7 | 153.05 | −42.65 | −21.79 | ||
| 0.2 | 205.55 | 157.71 | −47.84 | −23.28 | ||
| 0.3 | 202.25 | 164.62 | −37.63 | −18.61 | ||
| 0.4 | 199.5 | 169.79 | −29.71 | −14.89 | ||
| 0.5 | 200.6 | 173.98 | −26.62 | −13.27 | ||
| 0.6 | 211.6 | 177.51 | −34.09 | −16.11 | ||
| 0.7 | 243.9 | 180.58 | −63.32 | −25.96 | ||
| 0.8 | 215.4 | 183.31 | −32.09 | −14.90 | ||
| 0.9 | 250.5 | 185.77 | −64.73 | −25.84 | ||
| 1.0 | 232.4 | 188.01 | −44.39 | −19.10 | ||
| Sample 2# | 200 | 0.05 | 283.7 | 240.62 | −43.08 | −15.19 |
| 0.1 | 282.6 | 257.37 | −25.23 | −8.93 | ||
| 0.15 | 282.6 | 267.84 | −14.76 | −5.22 | ||
| 0.2 | 285.9 | 275.60 | −10.30 | −3.60 | ||
| 0.3 | 289.75 | 287.05 | −2.70 | −0.93 | ||
| 0.4 | 289.75 | 295.56 | 5.81 | 2.01 | ||
| 0.5 | 291.9 | 302.40 | 10.50 | 3.60 | ||
| 0.6 | 294.1 | 308.16 | 14.06 | 4.78 | ||
| 0.7 | 282.05 | 313.15 | 31.10 | 11.03 | ||
| 0.8 | 286.95 | 317.56 | 30.61 | 10.67 | ||
| 0.9 | 282.6 | 321.53 | 38.93 | 13.77 | ||
| 1.0 | 296.85 | 325.14 | 28.29 | 9.53 | ||
| Sample 3# | 200 | 0.05 | 229.5 | 240.62 | 11.12 | 4.84 |
| 0.1 | 236.05 | 257.37 | 21.32 | 9.03 | ||
| 0.15 | 249.2 | 267.84 | 18.64 | 7.48 | ||
| 0.2 | 249.2 | 275.60 | 26.40 | 10.59 | ||
| 0.3 | 253.05 | 287.05 | 34.00 | 13.44 | ||
| 0.4 | 252.5 | 295.56 | 43.06 | 17.05 | ||
| 0.5 | 254.15 | 302.40 | 48.25 | 18.99 | ||
| 0.6 | 256.35 | 308.16 | 51.81 | 20.21 | ||
| 0.7 | 259.05 | 313.15 | 54.10 | 20.88 | ||
| 0.8 | 270.55 | 317.56 | 47.01 | 17.38 | ||
| 0.9 | 266.2 | 321.53 | 55.33 | 20.78 | ||
| 1.0 | 266.2 | 325.14 | 58.94 | 22.14 | ||
| Sample 4# | 200 | 0.1 | 351.35 | 367.17 | 15.82 | 4.50 |
| 0.5 | 368.35 | 444.89 | 76.54 | 20.78 | ||
| 1.0 | 376.05 | 487.08 | 111.03 | 29.52 | ||
| Sample 5# | 100 | 0.033 | 116.788 | 119.60 | 2.81 | 2.40 |
| 0.05 | 116.664 | 124.50 | 7.84 | 6.72 | ||
| 0.083 | 114.876 | 130.82 | 15.94 | 13.88 | ||
| 0.1 | 123.496 | 133.24 | 9.74 | 7.89 | ||
| 0.13 | 126.148 | 136.75 | 10.60 | 8.40 | ||
| 0.2 | 133.58 | 142.78 | 9.20 | 6.89 | ||
| 0.25 | 139.364 | 146.04 | 6.68 | 4.79 |
References
- Goodman, R.E.; Taylor, R.L.; Brekke, T.A. A model for the mechanics of jointed rock. J. Soil Mech. Found. Div. 1968, 94, 637–659. [Google Scholar]
- Hoek, E. Strength of jointed rock masses. Geotechnique 1983, 33, 187–223. [Google Scholar] [CrossRef]
- Barton, N.; Bandis, S.; Bakhtar, K. Strength, deformation and conductivity coupling of rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 121–140. [Google Scholar] [CrossRef]
- Saroglou, C.; Qi, S.W.; Guo, S.F.; Wu, F.Q. ARMR, a new classification system for the rating of anisotropic rock masses. Bull. Eng. Geol. Environ. 2018, 78, 3611–3626. [Google Scholar] [CrossRef]
- Plesha, M.E. Constitutive models for rock discontinuities with dilatancy and surface degradation. Int. J. Numer. Anal. Methods Geomech. 1987, 11, 345–362. [Google Scholar] [CrossRef]
- Grasselli, G. Shear Strength of Rock Joints Based on Quantified Surface Description. Ph.D. Thesis, Swiss Federal Institute of Technology, Zürich, Switzerland, 2001. [Google Scholar]
- Guo, S.F.; Qi, S.W. Numerical study on progressive failure of hard rock samples with an unfilled undulate joint. Eng. Geol. 2015, 193, 173–182. [Google Scholar] [CrossRef]
- Bahaaddini, M.; Hagan, P.C.; Mitra, R.; Khosravi, M.H. Experimental and numerical study of asperity degradation in the direct shear test. Eng. Geol. 2016, 204, 41–52. [Google Scholar] [CrossRef]
- Guo, S.F.; Qi, S.W.; Zhan, Z.F.; Zheng, B.W. Plastic-strain-dependent strength model to simulate the cracking process of brittle rocks with an existing non-persistent joint. Eng. Geol. 2017, 231, 114–125. [Google Scholar] [CrossRef]
- Qi, S.W.; Xu, Q.; Lan, H.X.; Zhang, B.; Liu, J.Y. Spatial distribution analysis of landslides triggered by 2008.5.12 Wenchuan Earthquake, China. Eng. Geol. 2010, 116, 95–108. [Google Scholar] [CrossRef]
- Qi, S.W.; Xu, Q.; Zhang, B.; Zhou, Y.D.; Lan, H.X.; Li, L.H. Source characteristics of long runout rock avalanches triggered by the 2008 Wenchuan earthquake, China. J. Asian Earth Sci. 2011, 40, 896–906. [Google Scholar] [CrossRef]
- Qi, S.W.; Lan, H.X.; Dong, J.Y. An analytical solution to slip buckling slope failure triggered by earthquake. Eng. Geol. 2015, 194, 4–11. [Google Scholar] [CrossRef]
- Huang, X.L.; Qi, S.W.; Guo, S.F.; Dong, W.L. Experimental study of ultrasonic waves propagating through a rock mass with a single joint and multiple parallel joints. Rock Mech. Rock Eng. 2014, 47, 549–559. [Google Scholar] [CrossRef]
- Huang, X.L.; Qi, S.W.; Williams, A.; Zou, Y.; Zheng, B.W. Numerical simulation of stress wave propagating through filled joints by particle model. Int. J. Solids Struct. 2015, 69, 23–33. [Google Scholar] [CrossRef]
- Huang, X.L.; Qi, S.W.; Xia, K.W.; Shi, X.S. Particle crushing of a filled fracture during compression and its effect on stress wave propagation. J. Geophys. Res. Solid Earth 2018, 123, 5559–5587. [Google Scholar] [CrossRef]
- Muralha, J.; Grasselli, G.; Tatone, B.; Blümel, M.; Chryssanthakis, P.; Jiang, Y.J. ISRM suggested method for laboratory determination of the shear strength of rock joints: Revised version. Rock Mech. Rock Eng. 2014, 47, 291–302. [Google Scholar] [CrossRef]
- Qi, S.W.; Macciotta, R.; Shou, K.J.; Saroglou, C. Preface to the Special Issue on “Advances in Rock Mass Engineering Geomechanics”. Eng. Geol. 2020, 272, 105642. [Google Scholar] [CrossRef]
- Patton, F.D. Multiple Modes of Shear Failure in Rock and Related Materials. Ph.D. Thesis, University of IIIinois, Urbana, IL, USA, 1966. [Google Scholar]
- Patton, F.D. Multiple modes of shear failure in rock. In Proceedings of the 1st Congress of International Society for Rock Mechanics, Lisbon, Portugal, 25 September–1 October 1966; pp. 509–513. [Google Scholar]
- Ladanyi, B.; Archambault, G. Simulation of shear behavior of a jointed rock mass. In Proceedings of the 11th Symposium on Rock Mechanics: Theory and Practice, Berkeley, CA, USA, 16–19 June 1969; pp. 105–125. [Google Scholar]
- Barton, N. Review of a new shear-strength criterion for rock joints. Eng. Geol. 1973, 7, 287–332. [Google Scholar] [CrossRef]
- Jing, L.; Nordlund, E.; Stephansson, O. An experimental study on the anisotropy and stress-dependency of the strength and deformability of rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1992, 29, 535–542. [Google Scholar] [CrossRef]
- Huang, X.; Haimson, B.C.; Plesha, M.E.; Qiu, X. An investigation of the mechanics of rock joints—Part I. Laboratory investigation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 257–269. [Google Scholar] [CrossRef]
- Qiu, X.; Plesha, M.E.; Huang, X.; Haimson, B.C. An investigation of the mechanics of rock joints—Part II. Analytical investigation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 271–287. [Google Scholar] [CrossRef]
- Kulatilake, P.; Shou, G.; Huang, T.H.; Morgan, R.M. New peak shear strength criteria for anisotropic rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1995, 32, 673–697. [Google Scholar] [CrossRef]
- Zhao, J. A new JRC-JMC shear strength criterion for rock joint. Chin. J. Rock Mech. Eng. 1998, 17, 349–357. (In Chinese) [Google Scholar]
- Homand, F.; Belem, T.; Souley, M. Friction and degradation of rock joint surfaces under shear loads. Int. J. Numer. Anal. Methods Geomech. 2001, 25, 973–999. [Google Scholar] [CrossRef]
- Grasselli, G.; Egger, P. Constitutive law for the shear strength of rock joints based on three-dimensional surface parameters. Int. J. Rock Mech. Min. Sci. 2003, 40, 25–40. [Google Scholar] [CrossRef]
- Xia, C.C.; Tang, Z.C.; Xiao, W.M.; Song, Y.L. New peak shear strength criterion of rock joints based on quantified surface description. Rock Mech. Rock Eng. 2014, 47, 387–400. [Google Scholar] [CrossRef]
- Tse, R.; Cruden, D.M. Estimating joint roughness coefficients. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1979, 16, 303–307. [Google Scholar] [CrossRef]
- Maerz, N.H.; Franklin, J.A.; Bennett, C.P. Joint roughness measurement using shadow profilometry. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1990, 27, 329–343. [Google Scholar] [CrossRef]
- Beer, A.J.; Stead, D.; Coggan, J.S. Technical note estimation of the joint roughness coefficient (JRC) by visual comparison. Rock Mech. Rock Eng. 2002, 35, 65–74. [Google Scholar] [CrossRef]
- Jang, H.S.; Kang, S.S.; Jang, B.A. Determination of joint roughness coefficients using roughness parameters. Rock Mech. Rock Eng. 2014, 47, 2061–2073. [Google Scholar] [CrossRef]
- Li, Y.R.; Huang, R.Q. Relationship between joint roughness coefficient and fractal dimension of rock fracture surfaces. Int. J. Rock Mech. Min. Sci. 2015, 75, 15–22. [Google Scholar] [CrossRef]
- Zheng, B.W.; Qi, S.W. A new index to describe joint roughness coefficient (JRC) under cyclic shear. Eng. Geol. 2016, 212, 72–85. [Google Scholar] [CrossRef]
- Bao, H.; Zhang, G.B.; Lan, H.X.; Yan, C.G.; Xu, J.B.; Xu, W. Geometrical heterogeneity of the joint roughness coefficient revealed by 3D laser scanning. Eng. Geol. 2020, 265, 105415. [Google Scholar] [CrossRef]
- Barton, N.; Choubey, V. The shear strength of rock joints in theory and practice. Rock Mech. 1977, 10, 1–54. [Google Scholar] [CrossRef]
- International society for rockmechanics commission on standardization of laboratory and field tests. Suggested methods for the quantitative description of discontinuities in rock masses. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1978, 15, 319–368. [Google Scholar]
- Bandis, S.; Lumsden, A.; Barton, N. Experimental studies of scales effects on the shear behaviour of rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 1–21. [Google Scholar] [CrossRef]
- Barton, N.; Bandis, S. Effects of block size on the shear behavior of jointed rock. In Proceedings of the 23rd U.S Symposium on Rock Mechanics, Berkeley, CA, USA, 25–27 August 1982; pp. 739–760. [Google Scholar]
- Cunha, A.P. Scale effects in rock mechanics. In Proceedings of the 1st International Workshop on Scale Effects in Rock Masses, Loen, Norway, 7–8 June 1990; pp. 3–27. [Google Scholar]
- Castelli, M.; Savia, S.; Zanineti, A. Experimental evaluation of scale effect on the mechanical behavior of rock joints. In Proceedings of the International Eurock Symposium, Espoo, Finland, 4–7 June 2001; pp. 205–210. [Google Scholar]
- Du, S.G.; Hu, Y.J.; Hu, X.F. Measurement of joint roughness coefficient by using profilograph and roughness ruler. J. Earth Sci. 2009, 20, 890–896. [Google Scholar] [CrossRef]
- Vallier, F.; Mitani, Y.; Boulon, M.; Esaki, T.; Pellet, F. A new shear model for accounting scale effect in rock joints behavior. Rock Mech. Rock Eng. 2010, 43, 581–595. [Google Scholar] [CrossRef]
- Chen, S.J.; Zhu, W.C.; Yu, Q.L.; Liu, X.G. Characterization of anisotropy of joint surface roughness and aperture by variogram approach based on digital image processing technique. Rock Mech. Rock Eng. 2015, 49, 855–876. [Google Scholar] [CrossRef]
- Song, L.B.; Jiang, Q.; Li, Y.H.; Yang, C.X.; Ran, S.G.; Wang, B.L.; Liu, T. Description of discontinuities morphology based on shear behavior. Rock Soil Mech. 2017, 38, 525–533. (In Chinese) [Google Scholar]
- Schneider, H.J. The time dependence of friction of rock joints. Bull. Int. Assoc. Eng. Geol. 1977, 16, 235–239. [Google Scholar] [CrossRef]
- Dieterich, J.H. Time-dependent friction and the mechanics of stick-slip. Pure Appl. Geophys. 1978, 116, 790–806. [Google Scholar] [CrossRef]
- Crawford, A.M.; Curran, J.H. The influence of shear velocity on the frictional resistance of rock discontinuities. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 505–515. [Google Scholar] [CrossRef]
- Gillette, D.R.; Sture, S.; Ko, H.K.; Gould, M.C.; Scott, G.A. Dynamic behavior of rock joints. In Proceedings of the 24th US Symposium on Rock Mechanics, College Station, TX, USA, 20–23 June 1983; pp. 163–179. [Google Scholar]
- Li, H.B.; Feng, H.P.; Liu, B. Study on strength behaviors of rock joints under different shearing deformation velocities. Chin. J. Rock Mech. Eng. 2006, 25, 2435–2440. (In Chinese) [Google Scholar]
- Atapour, H.; Moosavi, M. The influence of shearing velocity on shear behavior of artificial joints. Rock Mech. Rock Eng. 2014, 47, 1745–1761. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, X.P.; Jiang, Y.J.; Zhang, Y.Z. New shear strength criterion for rough rock joints considering shear velocity. Chin. J. Geotech. Eng. 2015, 37, 1399–1404. (In Chinese) [Google Scholar]
- Zheng, B.W.; Qi, S.W.; Zhan, Z.F.; Zou, Y.; Zhang, S.S. Effect of shear rate on the strength characteristics of rock joints. J. Earth Sci. Environ. 2015, 37, 101–110. (In Chinese) [Google Scholar]
- Mehrishal, S.; Sharifzadeh, M.; Shahriar, K.; Song, J.J. An experimental study on normal stress and shear rate dependency of basic friction coefficient in dry and wet limestone joints. Rock Mech. Rock Eng. 2016, 49, 4607–4629. [Google Scholar] [CrossRef]
- Tang, Z.C.; Wong, L.Y.N. Influences of normal loading rate and shear velocity on the shear behavior of artificial rock joints. Rock Mech. Rock Eng. 2016, 49, 2165–2172. [Google Scholar] [CrossRef]
- Meng, F.Z.; Wong, L.N.Y.; Zhou, H.; Wang, Z.Q. Comparative study on dynamic shear behavior and failure mechanism of two types of granite joint. Eng. Geol. 2018, 245, 356–369. [Google Scholar] [CrossRef]
- Qi, S.W.; Zheng, B.W.; Wu, F.Q.; Huang, X.L.; Zhan, Z.F.; Zou, Y. A Test System and Test Method for the Properties of Discontinuities. China Patent ZL201410078860.7, 6 July 2016. (In Chinese). [Google Scholar]
- Qi, S.W.; Zheng, B.W.; Wu, F.Q.; Huang, X.L.; Guo, S.F.; Zhan, Z.F.; Zou, Y.; Barla, G. A new dynamic direct shear testing device on rock joints. Rock Mech. Rock Eng. 2020. (under review). [Google Scholar]
- Hu, Y.X.; Liu, S.C.; Dong, W. Earthquake Engineering; CRC Press: London, UK, 1996. [Google Scholar]
- Zhang, Q.B.; Zhao, J. A review of dynamic experimental techniques and mechanical behaviour of rock materials. Rock Mech. Rock Eng. 2014, 47, 1411–1478. [Google Scholar] [CrossRef]
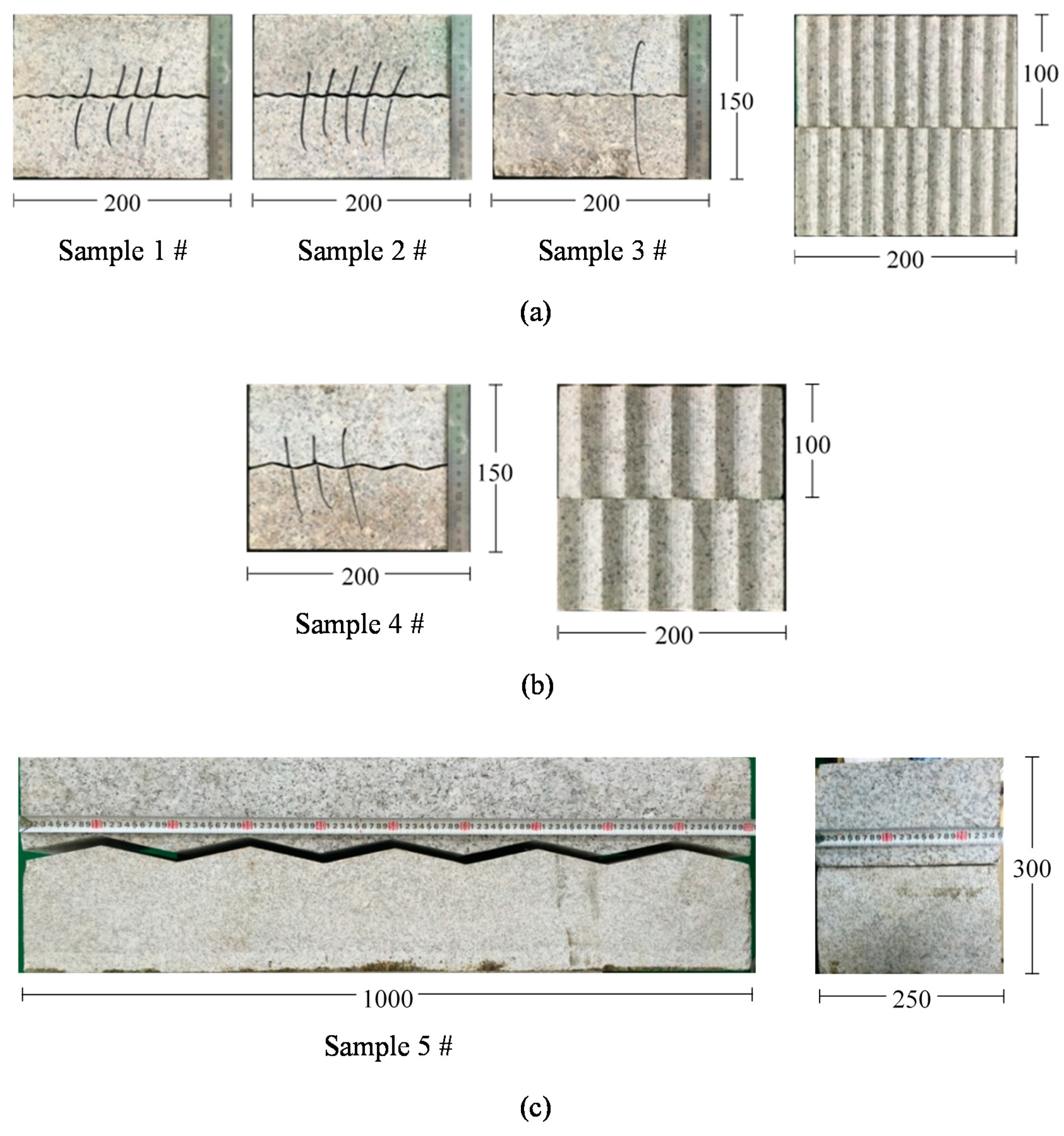


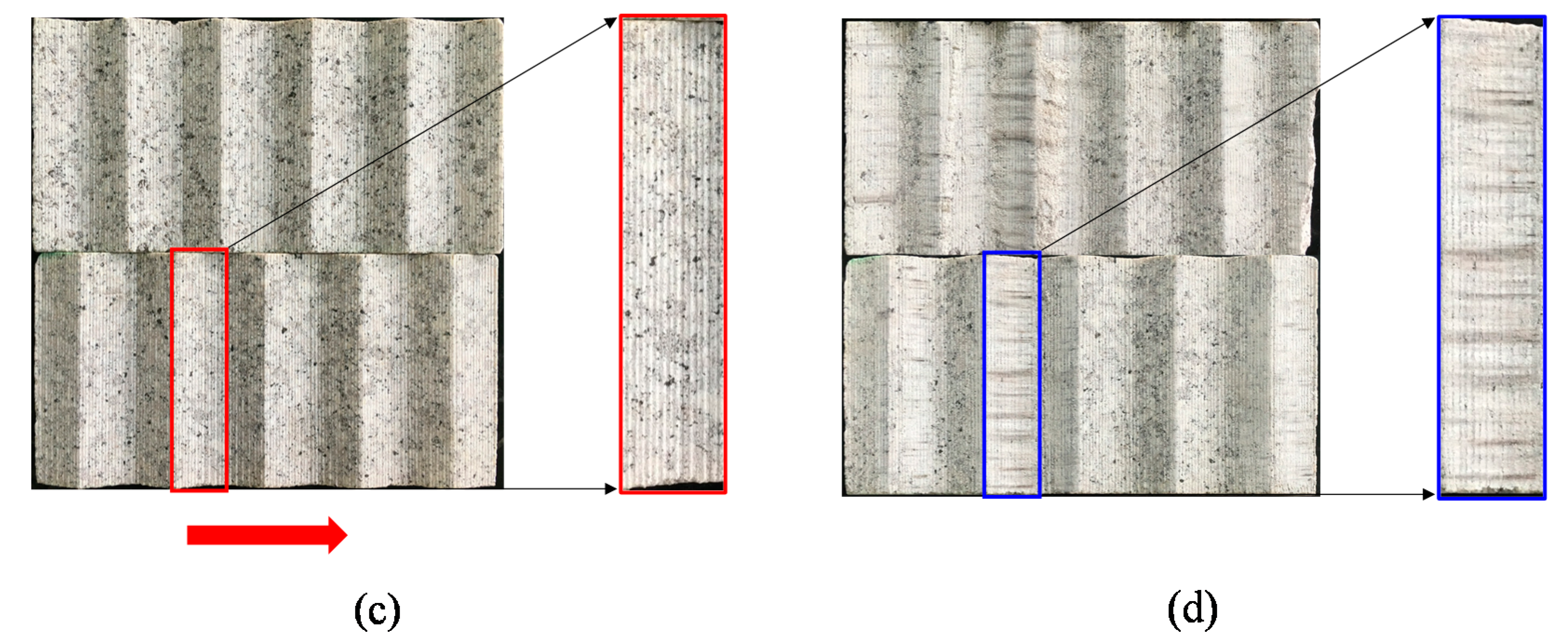
| Sample Type | Shear Rate (mm/s) | Empirical Equations | References |
|---|---|---|---|
| Granite, Diamond-rock | 0~0.1 | …(a) | [48] |
| Cement | 0.02~0.8 | …(b) | [51] |
| Cement mortar | 0.01~0.4 | …(c) | [53] |
| Syenite, Sandstone, Cement, Gypsum, Concrete | 0.005~0.8 | …(d) | [54] |
| Hot metamorphic limestone, Chemically deposited limestone | 0.0017~0.83 | …(e) | [55] |
| Sample Type and Number | Sample Length, Height, Width (mm) | Regular Sawtooth Number | Sawtooth Angle (°) | Sawtooth Length, Height (mm) | Average Value of Uniaxial Compressive Strength (MPa) | Average Value of Basic Friction Angle (°) |
|---|---|---|---|---|---|---|
| Small sample 1#, 2#, 3#, 4# | 200, 150, 100 | 10 | 17 | 20, 3.057 | 200 | 25 |
| 200, 150, 100 | 5 | 16 | 40, 5.735 | |||
| Large sample 5# | 1000, 300, 250 | 5 | 13 | 200, 23.087 |
| Sample Type | Undulated Angle (°) | Sample Length (mm) | JRCn | JCSn (MPa) |
|---|---|---|---|---|
| Granite discontinuity | 17 | 200 | 11.924 | 126.582 |
| 16 | 200 | 15.179 | 129.589 | |
| 13 | 1000 | 12.362 | 60.234 |
| Sample Type | Undulated Angle (°) | Ln (mm) | hn (mm) | JCS0 (MPa) | φb (°) | JRCn | JCSn (MPa) |
|---|---|---|---|---|---|---|---|
| Cement discontinuities with regular sawtooth shape | 15 | 150 | 4.019 | 22.5 | 36.22 | 14.213 | 17.701 |
| 30 | 4.33 | 20.762 | |||||
| 45 | 7.5 | 40.309 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, B.; Qi, S.; Huang, X.; Guo, S.; Wang, C.; Zhan, Z.; Luo, G. An Advanced Shear Strength Criterion for Rock Discontinuities Considering Size and Low Shear Rate. Appl. Sci. 2020, 10, 4095. https://doi.org/10.3390/app10124095
Zheng B, Qi S, Huang X, Guo S, Wang C, Zhan Z, Luo G. An Advanced Shear Strength Criterion for Rock Discontinuities Considering Size and Low Shear Rate. Applied Sciences. 2020; 10(12):4095. https://doi.org/10.3390/app10124095
Chicago/Turabian StyleZheng, Bowen, Shengwen Qi, Xiaolin Huang, Songfeng Guo, Chonglang Wang, Zhifa Zhan, and Guangming Luo. 2020. "An Advanced Shear Strength Criterion for Rock Discontinuities Considering Size and Low Shear Rate" Applied Sciences 10, no. 12: 4095. https://doi.org/10.3390/app10124095
APA StyleZheng, B., Qi, S., Huang, X., Guo, S., Wang, C., Zhan, Z., & Luo, G. (2020). An Advanced Shear Strength Criterion for Rock Discontinuities Considering Size and Low Shear Rate. Applied Sciences, 10(12), 4095. https://doi.org/10.3390/app10124095






