Stability Tests of Agricultural and Operating Machines by Means of an Installation Composed by a Rotating Platform (the “Turntable”) with Four Weighting Quadrants
Abstract
Featured Application
Abstract
1. Introduction
2. Materials and Methods
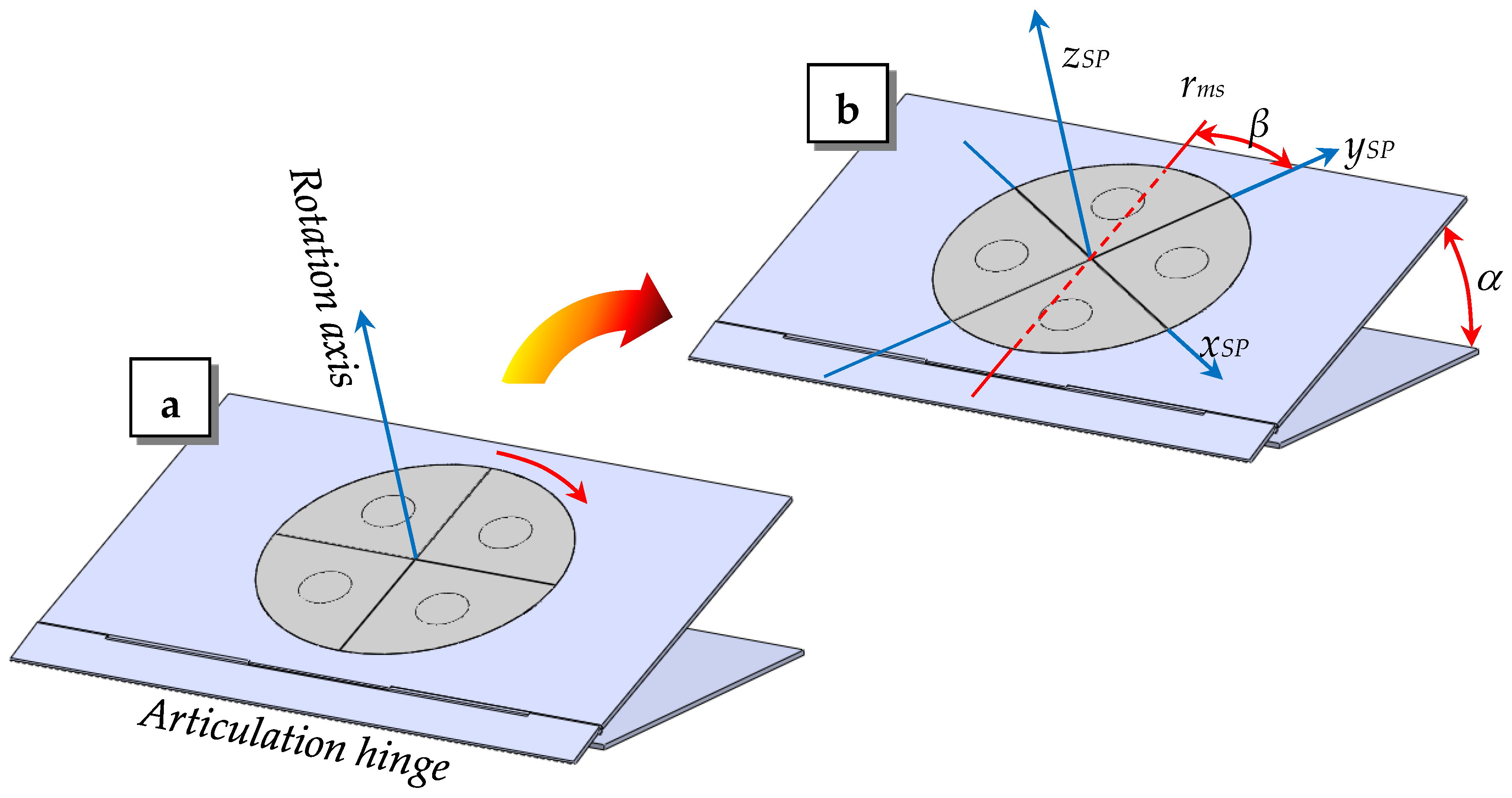
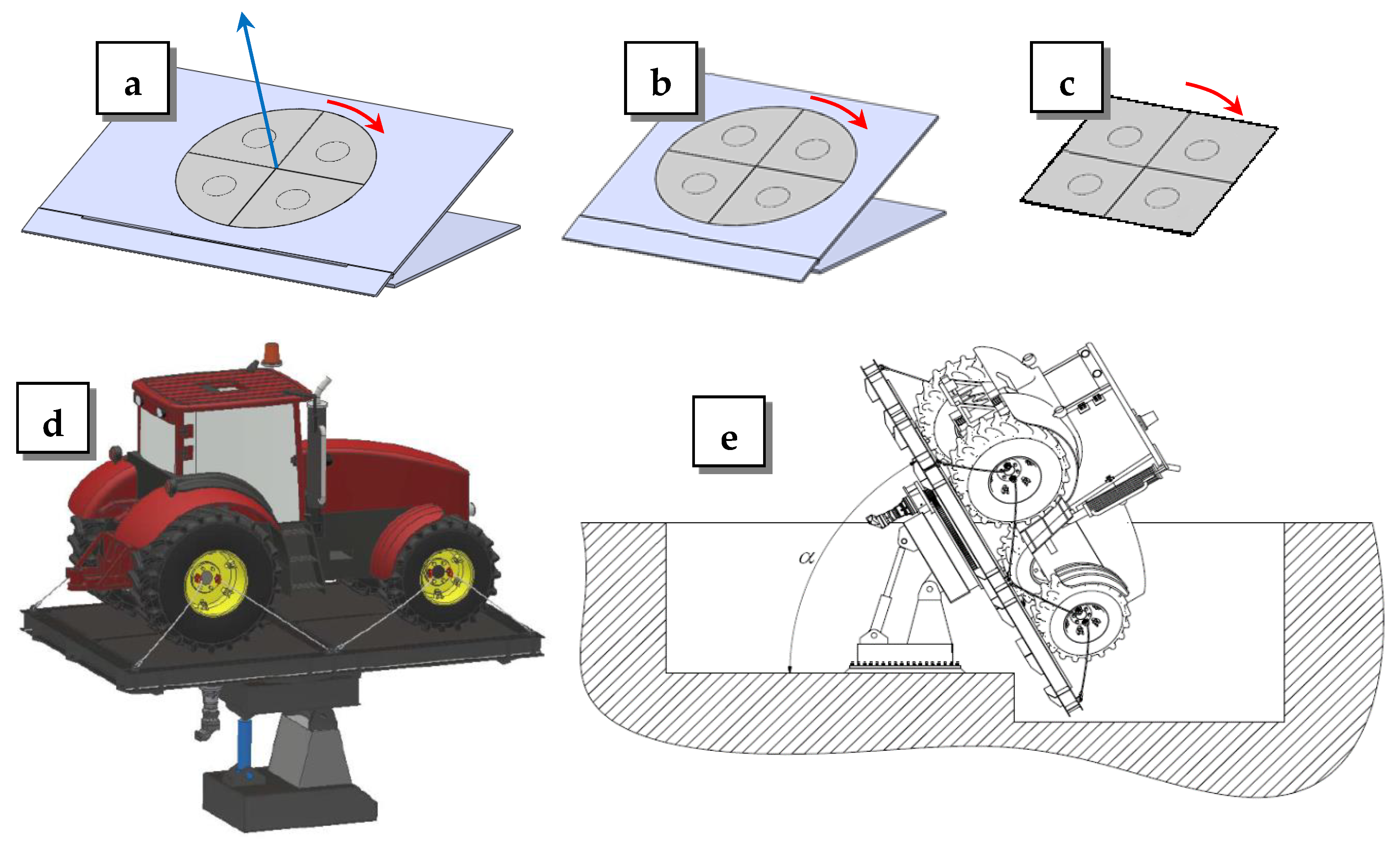
2.1. The Test Rig
2.2. The Approach
3. Results and Discussion
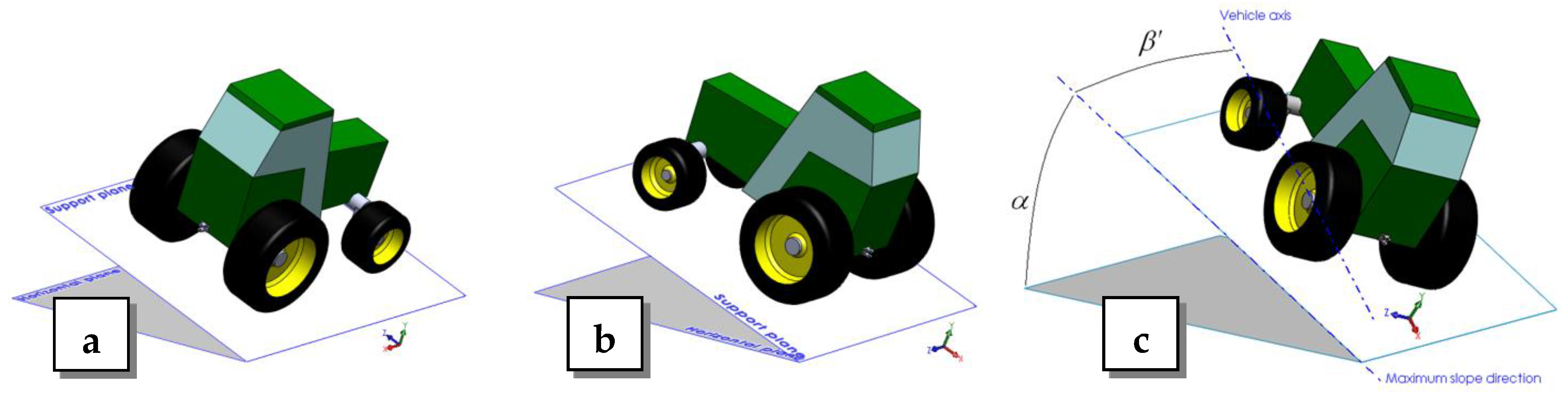
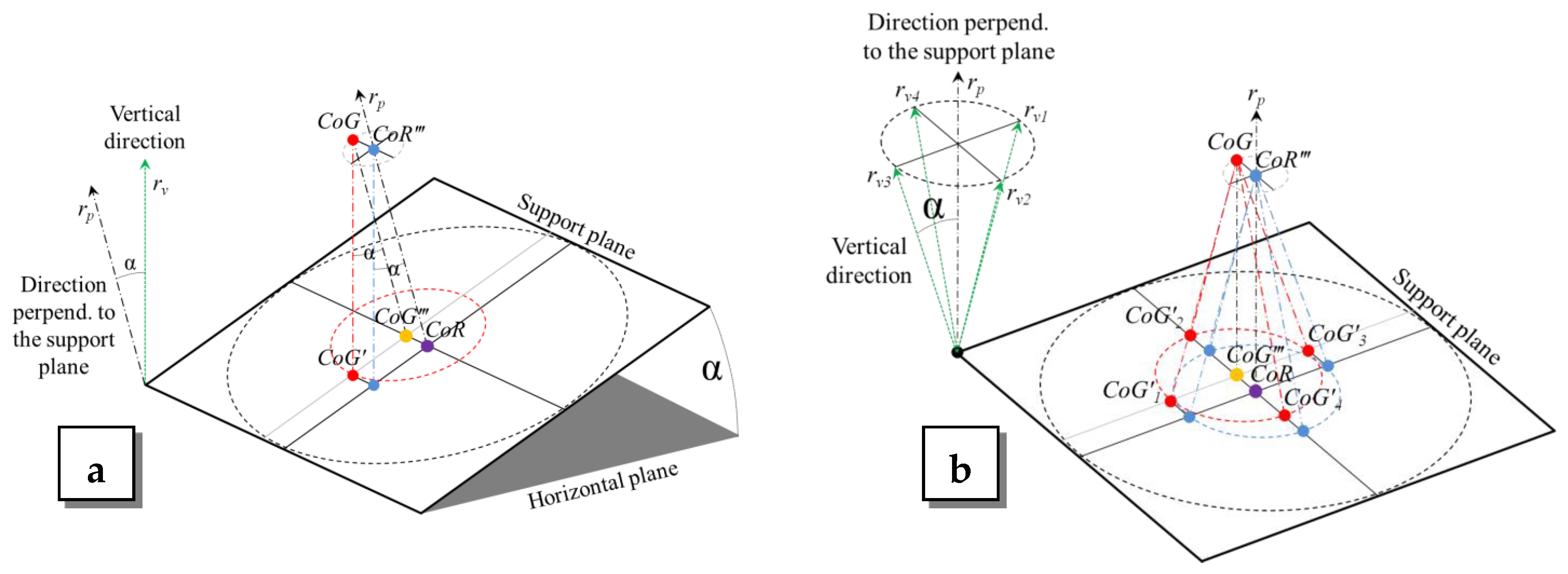
3.1. Correlation between the Operative Angles of the Rig and the Orientation Angles of a Vehicle under Test
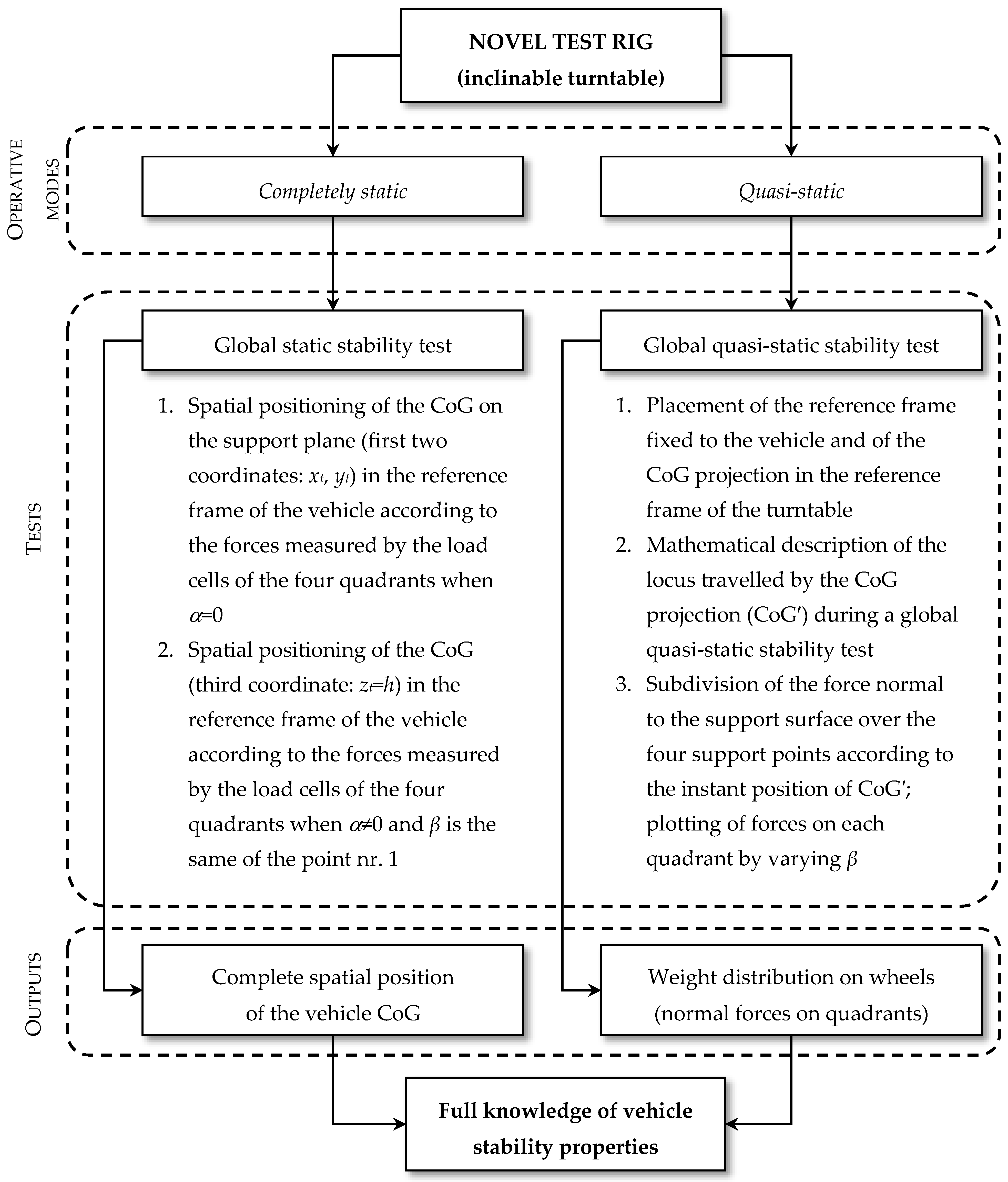
3.2. Possible Operative Modes of the Test Rig
- completely static mode (i.e., no revolution of the turntable around its axis of rotation) to have a global static stability test; (phase 1) the vehicle is placed on the platform when the support structure is horizontal (α = 0°) and the sides of the quadrant are aligned with the inclination hinge (β = 0° or β = ±90° or β = ±180°), thanks to the measurement of the weights supported by each quadrant, it is possible to place the center of gravity in relation to the center of the platform; (phase 2) subsequently, without moving the vehicle (its wheels are fixed to the quadrants), the support structure is tilted at a fixed angle (however far from the vehicle rollover); by doing so it is therefore possible to identify the height of the CoG of the machines under test; (phase 3) the support structure is tilted until the (lateral, frontal) overturning angle is reached;
- quasi-static mode (i.e., very slow rotation of the turntable and of the vehicle on it) to have a global quasi-static stability test; (phase 1) when the support structure is horizontal, the vehicle is positioned on the platform and the vehicle wheels are fixed to the quadrants; (phase 2) the support structure is tilted at different angles with respect to the horizontal plane and, at each fixed inclinations, the rotating subsystem is then activated thus making the vehicle revolute around the turntable rotation axis (the rotation speed should be around 0.026 rad·s−1 as declared in [41], i.e., a full rotation is completed in about 4 min); in the meantime, the weights supported by each quadrant (hence, supported by each wheel of the vehicle) are measured, thus characterizing the balance of the vehicle at different angles of roll, pitch and yaw (see next paragraphs); (phase 3) the procedure described in the previous phase is repeated till reaching an overturning condition. It is worth noting that the slowness of movement should make it possible to avoid (or, at least, mitigate) the vibrations that unfortunately occur in structures in which there are rotating organs [44], that can be a serious disturbance when measurements are made. However, another possibility to consider after first real-scale tests is eventually transforming the continuous movement into an intermittent one. This would allow exploring the stability of vehicles at a limited number of angles, equally-spaced by a fixed angle (e.g., 10° or 20°) until the turntable performs a complete rotation with a low number of stop-and-go not to lengthen a test too much. Then, it will be possible to thicken the measurements close to the angle of rotation where the stability conditions will be more critical.
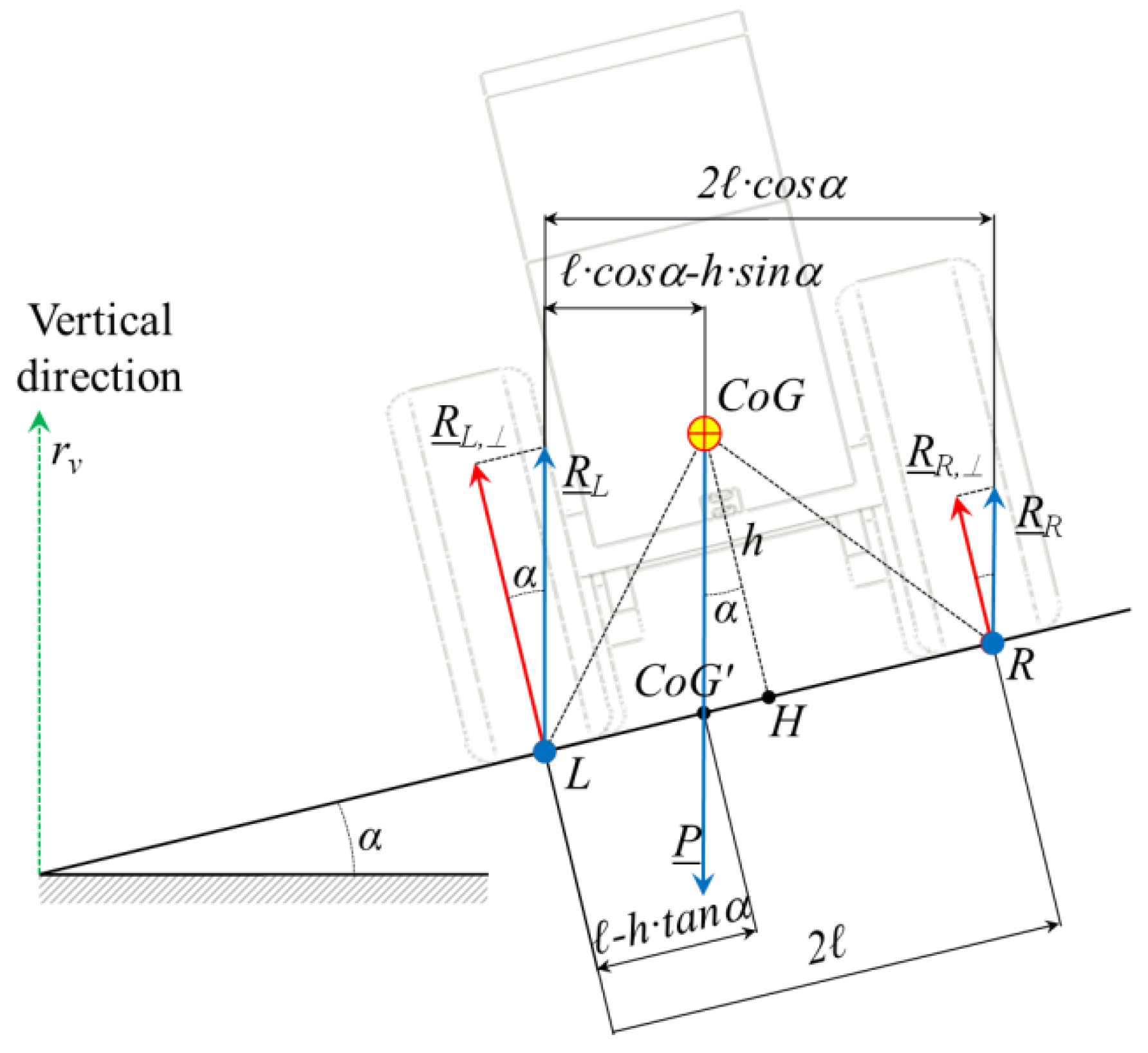
3.3. Possibility of Using the Position of the CoG Projection to Express the Incipient Roll-over Condition
- the front and rear tracks have the same width, hence L and R (i.e., the centers of the left/right tires) are limit points for the rollover also in the transversal plane containing the CoG;
- the tractor CoG is exactly in the median longitudinal plane of the vehicle (hence: ).
3.4. Completely Static Operative Mode of the Test Rig
3.4.1. Setting of a Frame of Reference Fixed to the Vehicle
- xt axis: aligned with the tractor median axis, contained in the tractor support plane;
- yt axle: aligned with the rear axle of the tractor, passing through the center of the rear tyre traces and, hence, contained in the tractor support plane; the point of the passage of the yt axle can be identified by taking the center of the rear wheel hub as reference (see Figure 6);
- zt axis: perpendicular to the other two axles, and therefore also perpendicular to the support plane of the tractor;
- Origin Ot: positioned at the center of the tractor rear axle, contained in the support plane of the tractor.
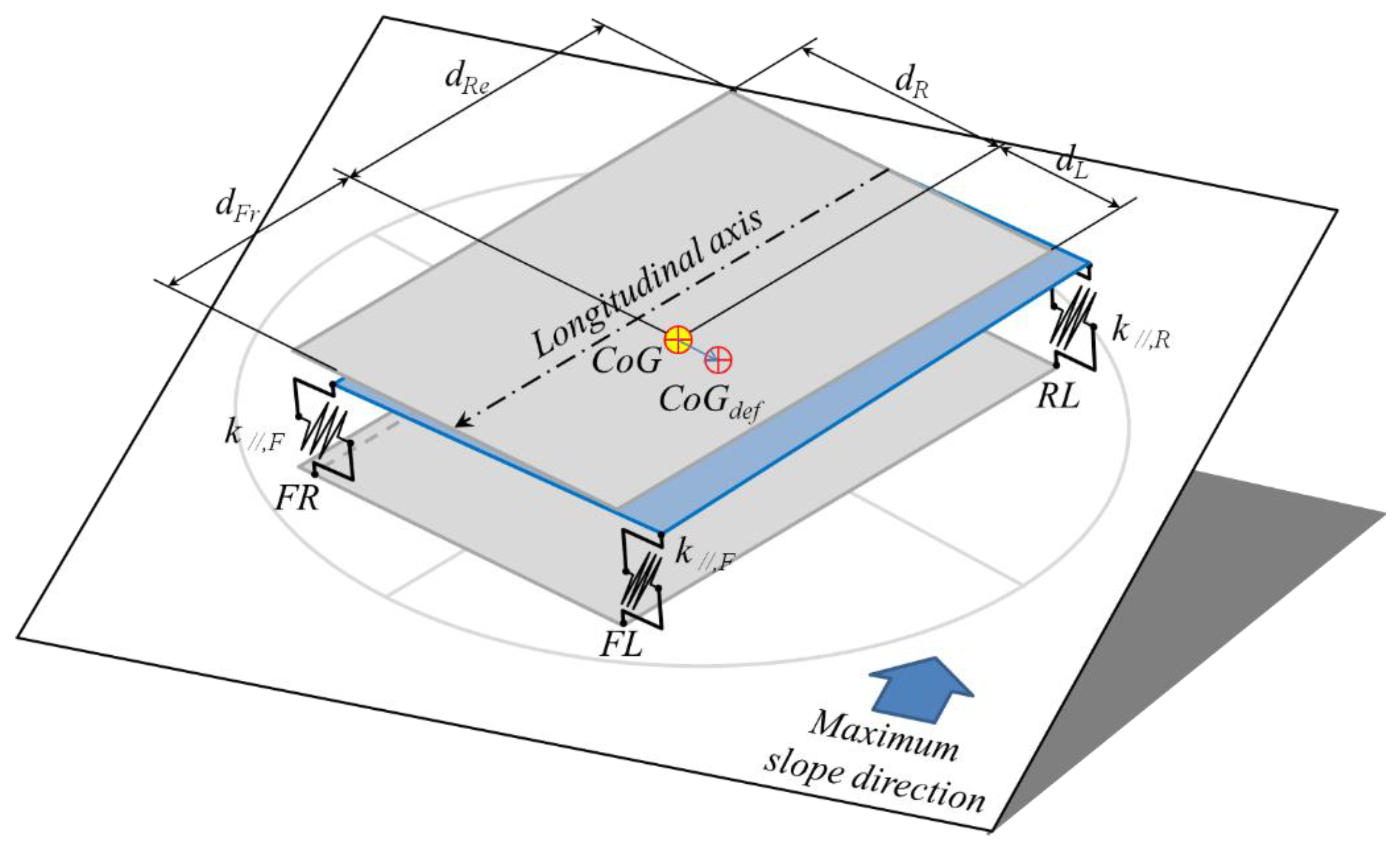
3.4.2. Positioning of the CoG Projection in the Support Plane Frame of Reference According to the Forces Measured by the Load Cells of the Four Quadrants
3.4.3. Calculation of the CoG Height
3.5. Quasi-Static Operative Mode of the Test Rig
3.5.1. Expressing the Coordinates of CoG′ in the Support Plane Frame of Reference
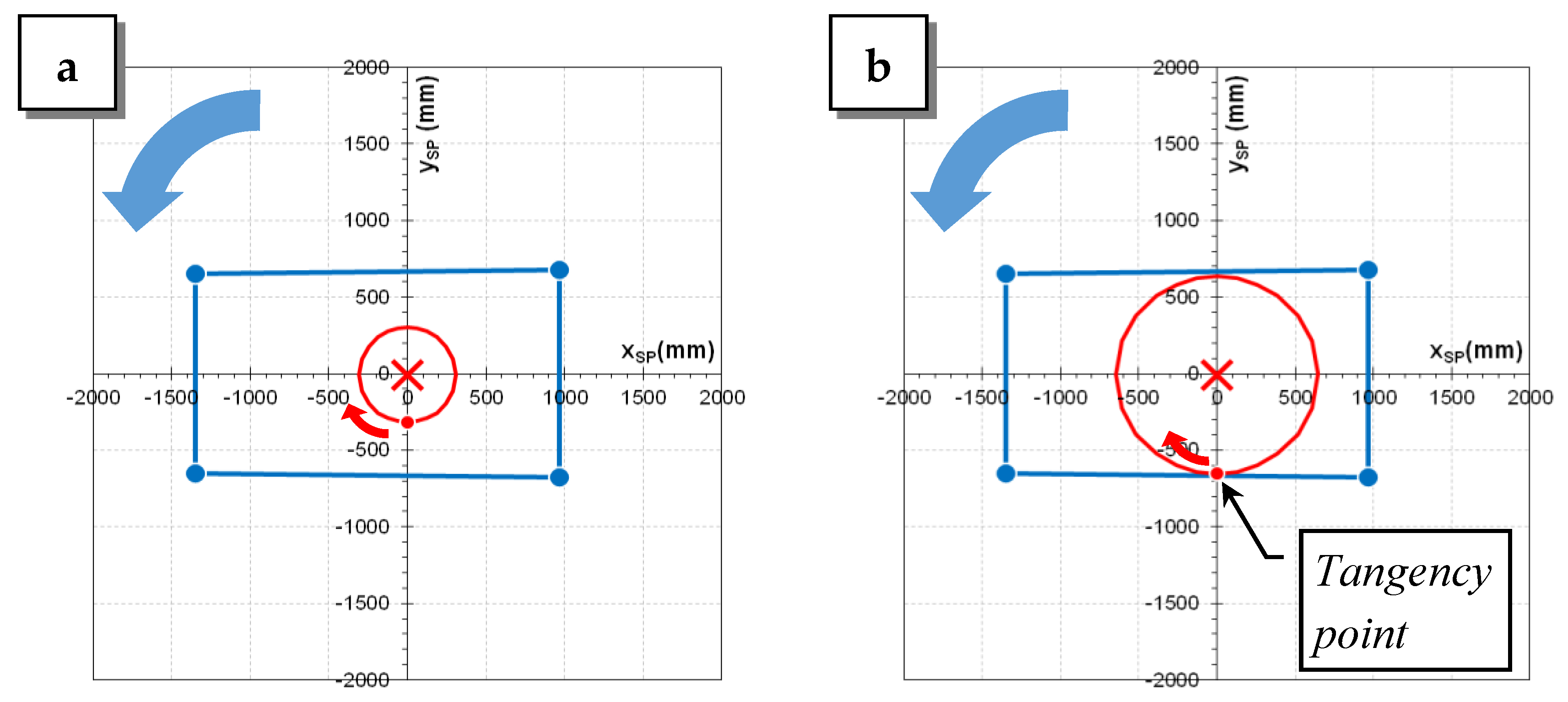
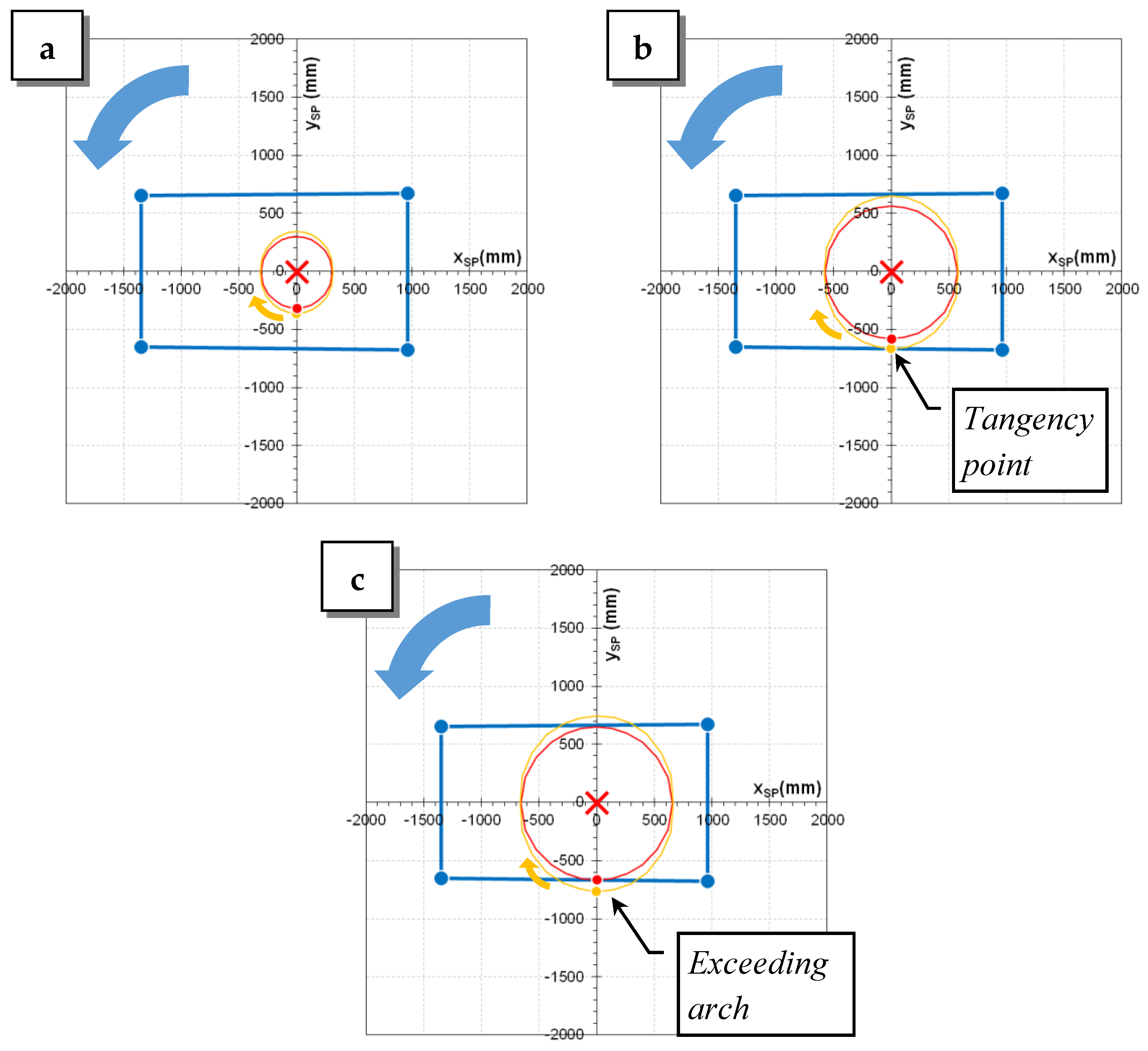
3.5.2. Locus Described by the CoG Projection during a Global Quasi-Static Stability Test
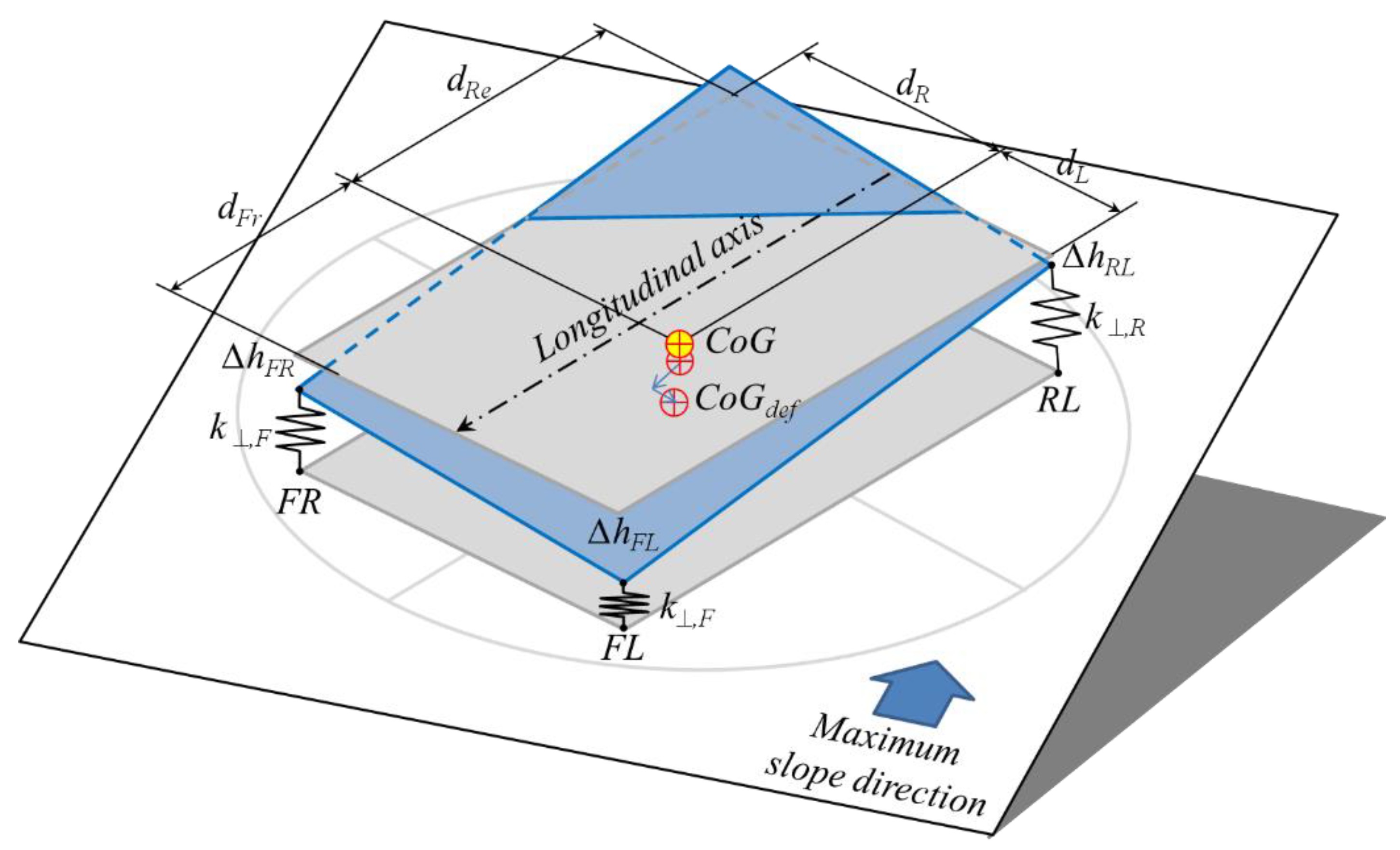
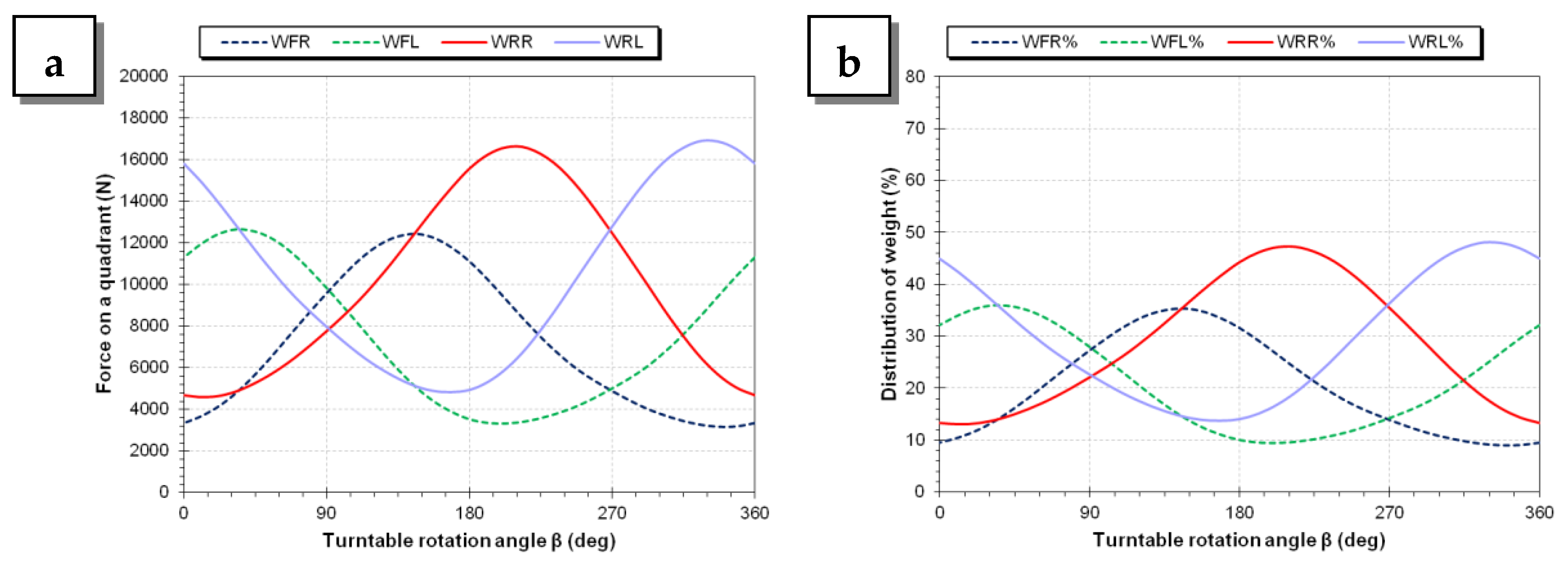
3.5.3. Subdivision of the Force Normal to the Support Surface over the Four Support Points According to the Position of the CoG Projection (CoG′)
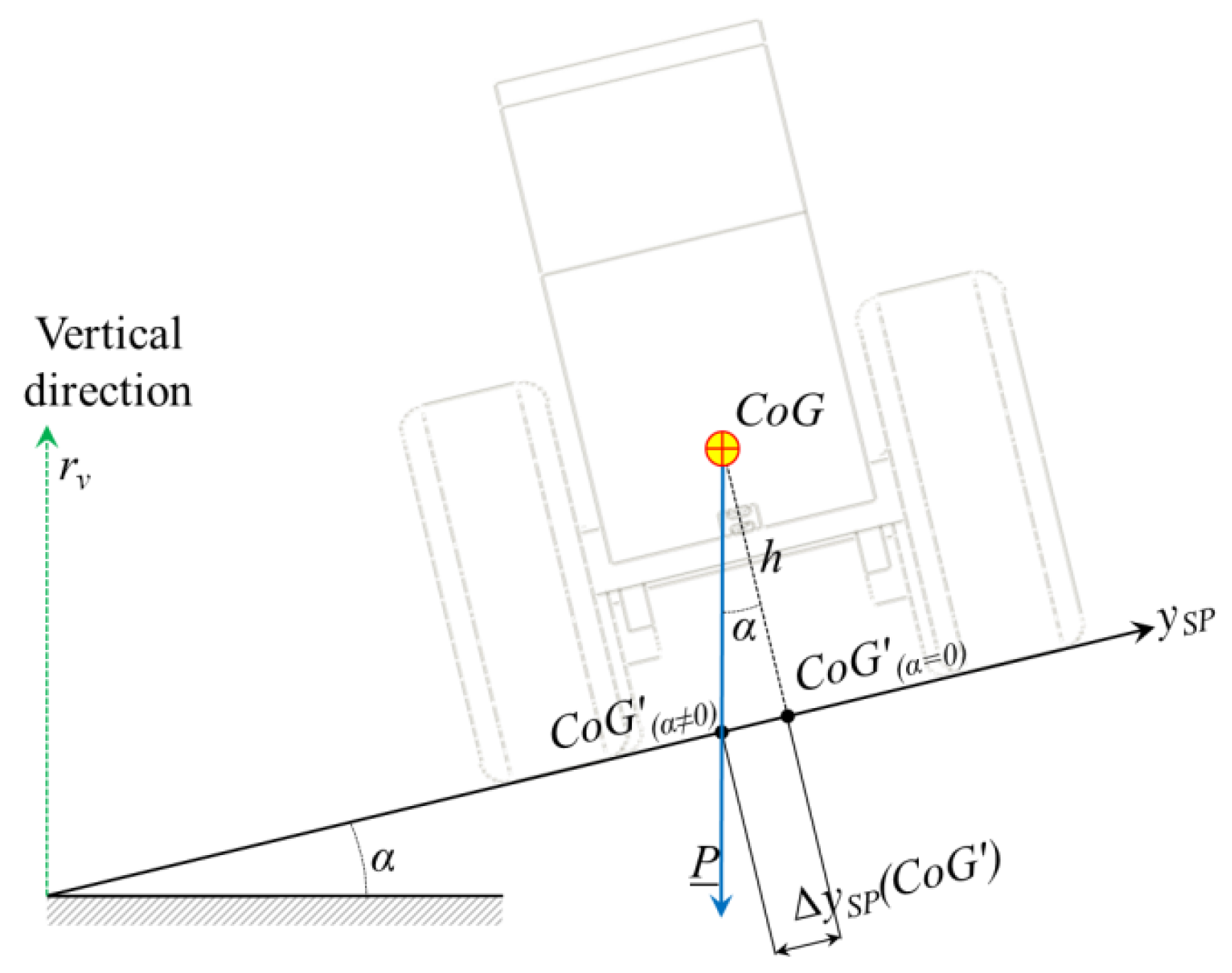
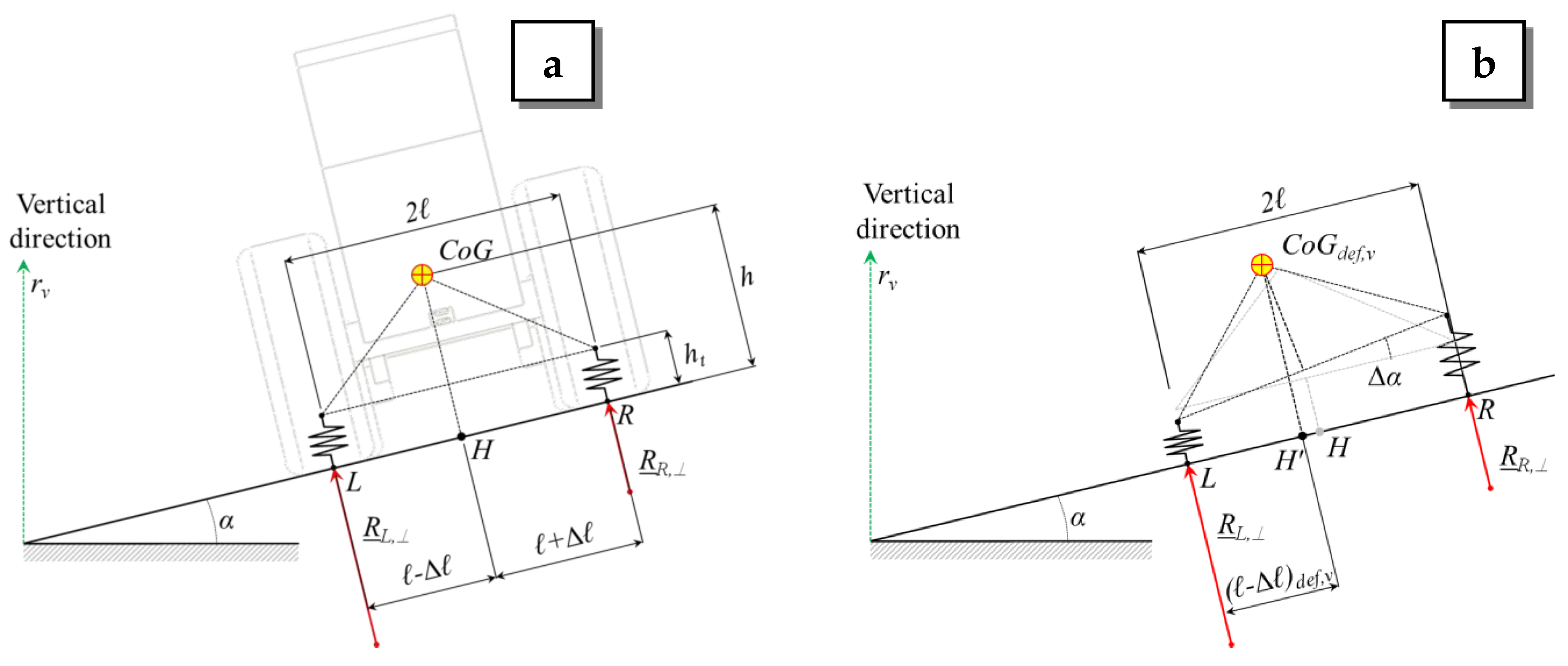
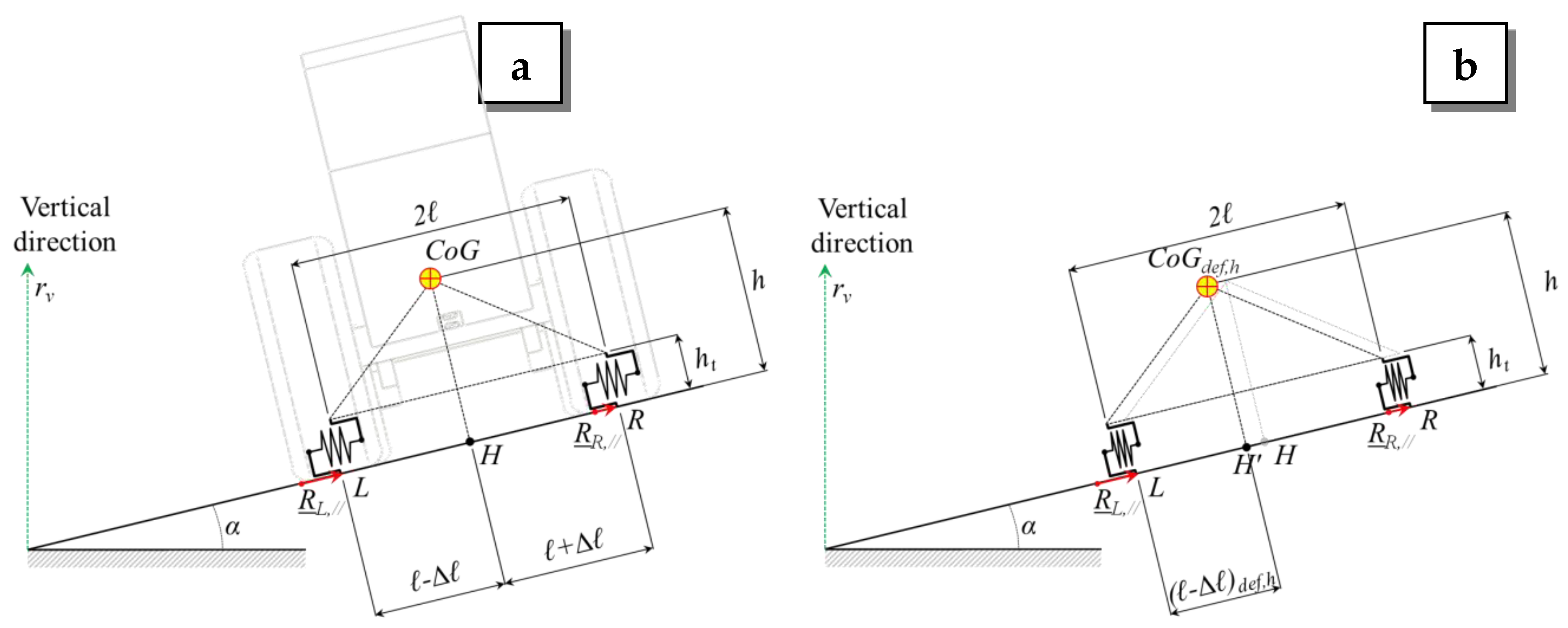
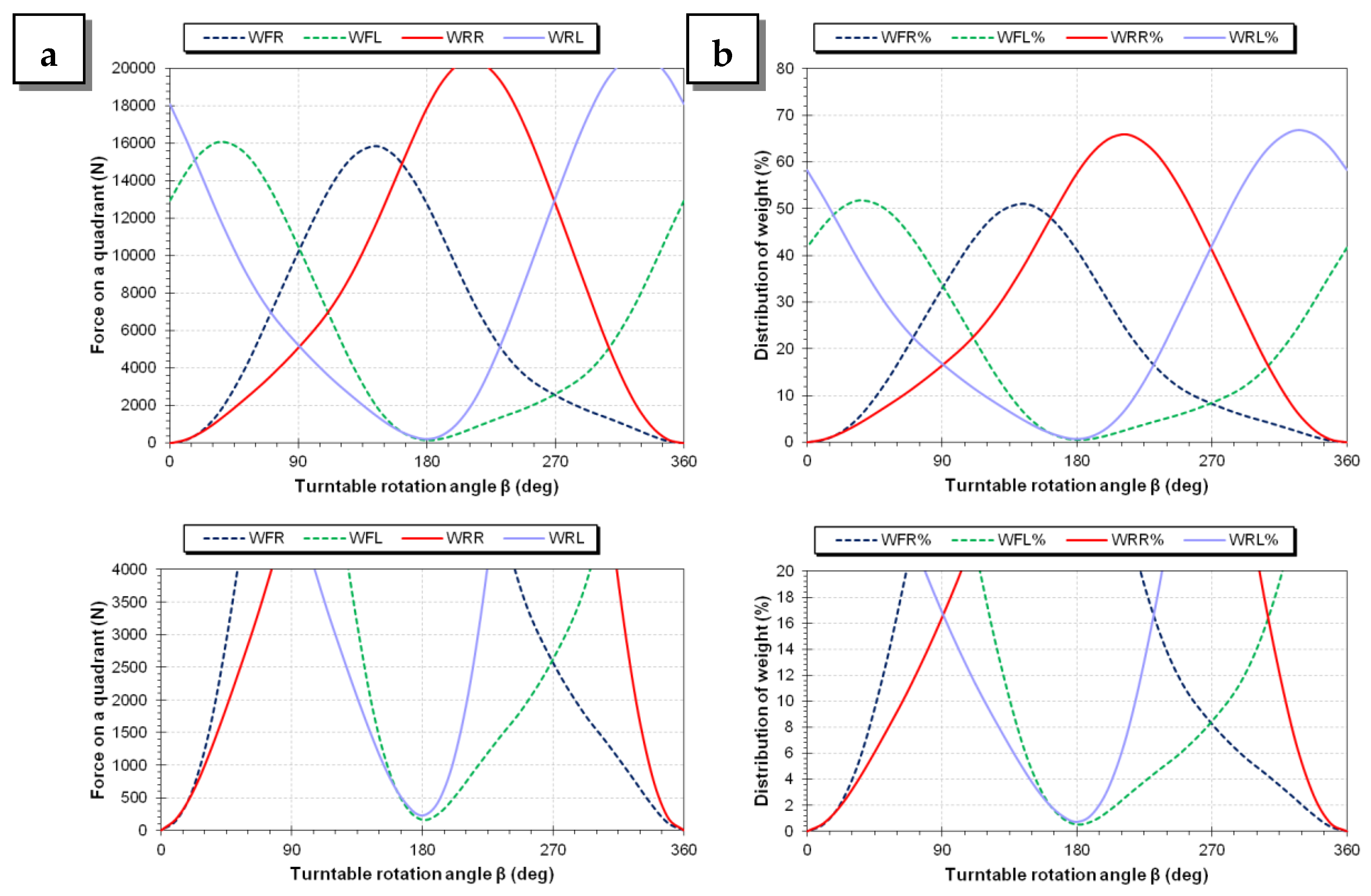
3.6. Effect of the Tyres Deformation on the Vehicle Stability
- a load perpendicular to the support plane, which causes a flattening of the tyres (i.e., they undergo a decrease in height), thus causing the chassis having a rigid rotation downstream as a consequence of the increase in the roll and pitch angles;
- a load parallel to the supporting plane; in particular, the component of this load parallel to the axis of the wheel (called: the lateral horizontal load) is the one of greatest interest, as it is responsible for a lateral deformation of the tyre and, therefore, it causes a translation of the entire chassis downstream.
3.6.1. Effect on the CoG Position of a Load on the Tyres Perpendicular to the Vehicle Support Plane
3.6.2. Effect on the CoG Position of a Load on the Tyres Parallel to the Vehicle Support Plane
3.7. Case-Study: Simulation of the Test of a Farm Tractor
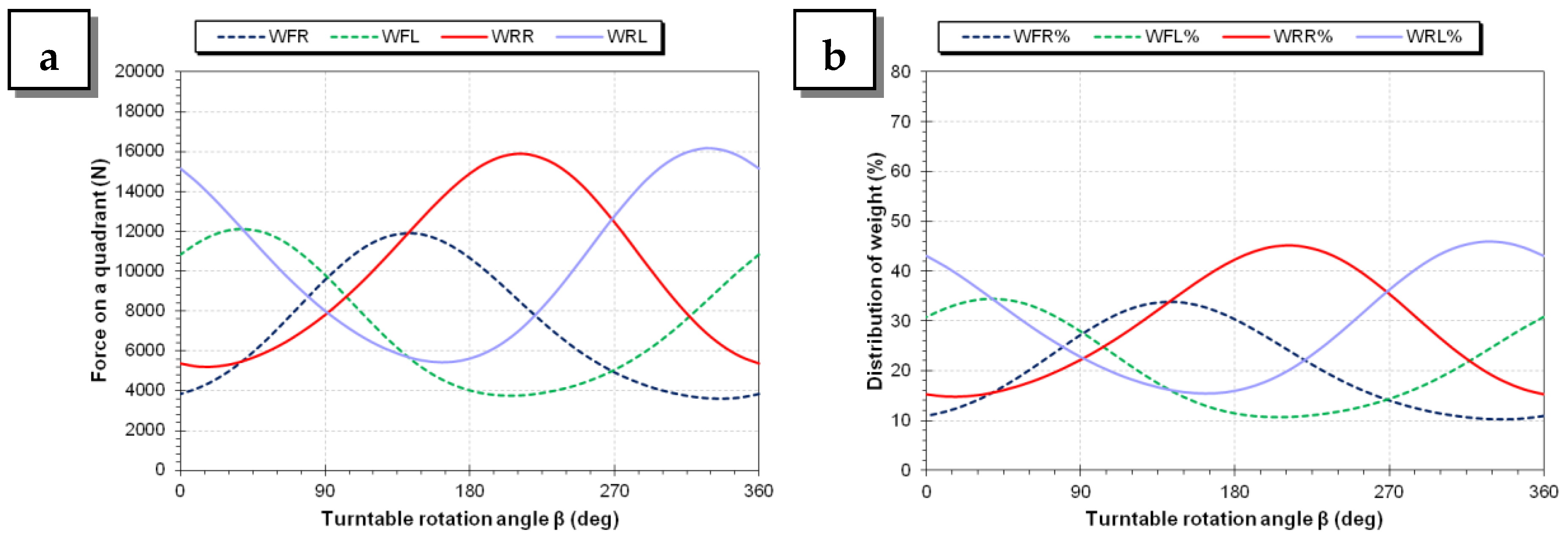
3.7.1. Static Test (Without Tyres Deflection)
3.7.2. Quasi-Static Test (Without Tyres Deflection)
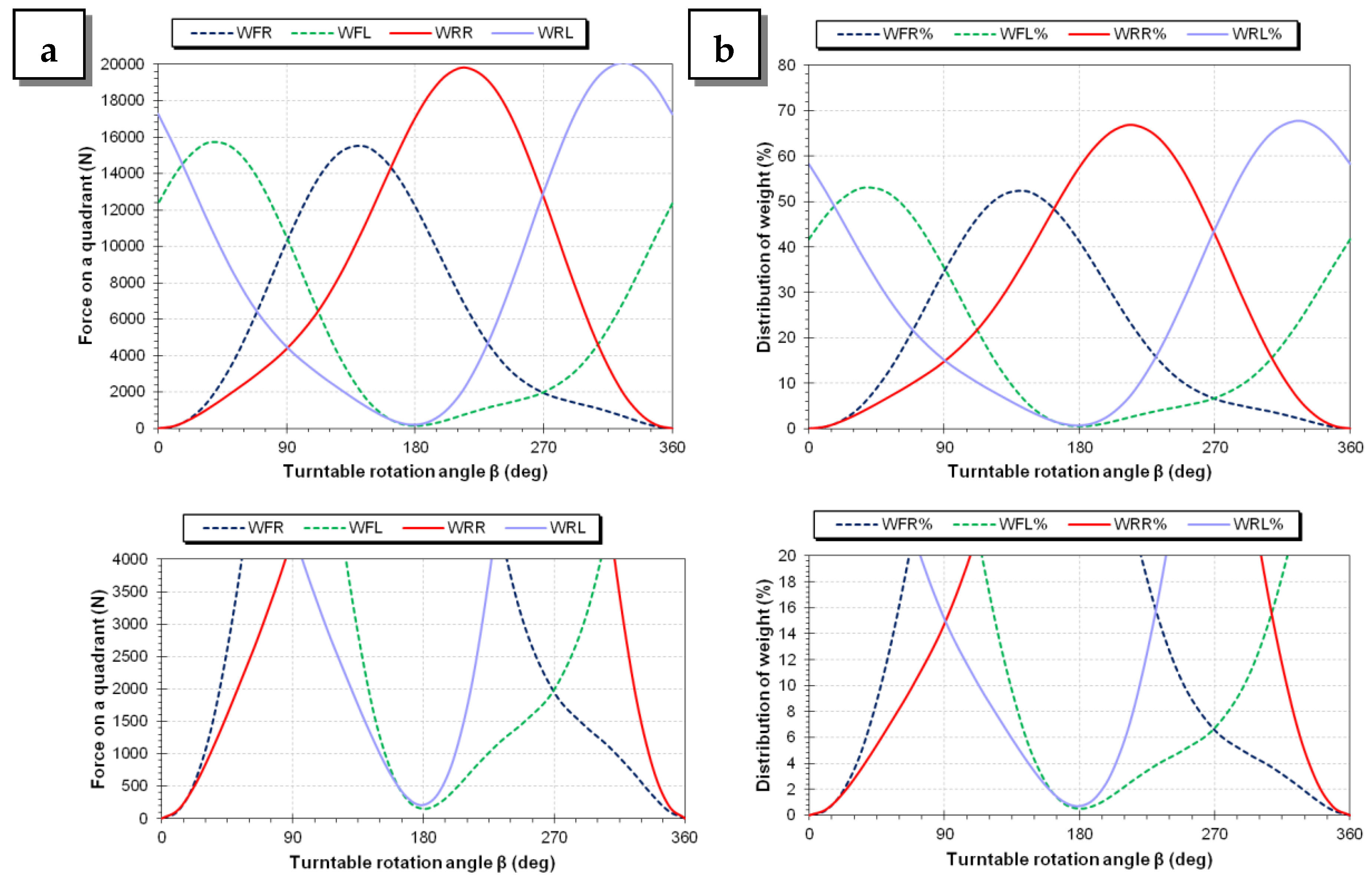
3.7.3. Quasi-Static Test (Considering Also the Tyres Deflection)
- for a 20° inclination of the support, with the assumption of tyre deformability, the maximum loads are higher than 5% whilst the minimum loads are lower than 10%, a clear sign of the worsening of the situation due to the deformation of the tyres downstream, which has affected the position of the CoG and caused a load transfer;
- for an inclination of the support at the limit of incipient rollover for the tractor (respectively of 37.73° and 33.95° in the two examined cases), the maximum loads recorded under the assumption of tyre deformability are greater by 2–3%, due to the lower inclination of the support platform, which results in a greater normal component on each wheel.
3.8. Possible Realization of the Described Test Rig
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Abbreviation | Significance |
| CoG | center of gravity (of a vehicle or, in general, of a body) |
| CoG′ | projection of the CoG of a vehicle (or, in general, of a body) on the vehicle support plane |
| CoG″ | projection of the CoG of a vehicle (or, in general, of a body) on the vehicle support plane when the support plane is horizontal |
| CoR | center of rotation (of the rotating system) on the support plane |
| CoR″ | center of rotation (of the rotating system) at a height h from the support plane |
| DoF(s) | degree(s) of freedom |
| OECD | Organization for Economic Co-operation and Development |
| Q1, … Q4 | quadrants of the turntable on which a vehicle under test is positioned, subdividing the support plane |
| Symbol | Significance, units |
| α | inclination angle (always positive) of the support plane (in the frame of reference of the test system); together with β is one of the “operative angles” of the test rig [°] |
| αlim,L, αlim,R | limit angle of inclination of the support plane α, corresponding to an incipient rollover condition on the left (L) or right (R) side of the vehicle [°] |
| β | rotation angle (positive if counter-clockwise) of the turntable, measured between the ySP axis of the turntable and the maximum slope direction of the support plane; together with α is one of the “operative angles” of the test rig [°] |
| β′ | rotation angle (positive if counter-clockwise) of vehicle longitudinal axis with the maximum slope direction; it coincides with the rotation angle of the turntable only when the vehicle longitudinal axis xt is parallel to the ySP axis of the turntable [°] |
| δ | angle (positive if counter-clockwise) between the abscissa/ordinate axis of the coordinate system of the support plane (xSP or ySP) and the abscissa/ordinate axis of the coordinate system of the vehicle (xt or yt) [°] |
| ϑ | pitch angle of a vehicle (in the vehicle frame of reference) [°] |
| k⊥ | coefficient of elasticity in the direction perpendicular to the support plane for each of the two sides of the vehicle under test [N·mm−1] |
| k// | coefficient of elasticity in the direction parallel to the support plane and to the wheel rotational axis for each of the two sides of the vehicle under test [N·mm−1] |
| k⊥,FL/k⊥,FR/k⊥,RL/k⊥,RR | coefficient of elasticity in the direction perpendicular to the support plane for the front-left/front-right/rear-left/rear-right tyre of the vehicle under test [N·mm−1] |
| k//,FL/k//,FR/k//,RL/k//,RR | coefficient of elasticity in the direction parallel to the support plane and to the wheel rotational axis for the front-left/front-right/rear-left/rear-right tyre of the vehicle under test [N·mm−1] |
| φ | roll angle of a vehicle (in the vehicle frame of reference) [°] |
| ψ | yaw angle of a vehicle (in the vehicle frame of reference) [°] |
| (a, b) | coordinates of CoG′ in the reference frame of the vehicle placed on the turntable [m; m] |
| Δℓ | distance of the CoG of a vehicle under test from its longitudinal axis/plane [m]; in other words, in a plane transverse to the longitudinal axis of the vehicle, offset from the centreline of the vertical line passing through the CoG |
| ΔySP(CoG′) | shift of CoG′ downstream the inclined support plane in the frame of reference fixed to the turntable as an effect of an increase in the support plane inclination [m] |
| d1, d2 | dimensions of the quadrilateral linking the load cells under the quadrants (i.e., distance along xSP and ySP between the load cells under the quadrants) [m] |
| dFr, dRe | distances of the CoG′ from the front and the rear axles of a vehicle under test [m] |
| dFL, dFR, dRL, dRR | respectively the distances of the line passing through CoG′ from the front/rear left/right wheels of the tractor |
| FT | front track width of the vehicle under test (positioned on the support plane) [m] |
| g | modulus of the gravitational acceleration [m·s−2] |
| h = zt | distance of the CoG from the vehicle support plane (height of the CoG) [m] |
| ℓ | half-track width of a vehicle under test (positioned on the support plane) or half-width of a vehicle under test (positioned on the support plane) in correspondence to the CoG [m] |
| m | mass of the vehicle under test [kg] |
| P | modulus of the weight force of a vehicle under test [N] |
| rBR | ratio between the components perpendicular to the support plane of the binding reaction in the points R and L, i.e., in the center of the right and left rear wheel of a vehicle under test (positioned on the support plane) [-] |
| rdist | ratio of the distances of CoG′ from L and from R, i.e., from the centers of the right and left rear wheels of a vehicle under test (positioned on the support plane) [-] |
| rms | maximum slope direction of the support plane of the test system |
| rp | direction perpendicular to the support plane, on which the vehicle under test is placed |
| rv | (absolute) vertical direction, perpendicular to the horizontal plane on which the test rig is placed |
| RR⊥, RL⊥ | modulus of the component perpendicular to the support plane of the binding reaction in the points R (L), i.e., in the center of the right (left) rear wheel of a vehicle under test, i.e., positioned on the support plane [N] |
| RR, RL | modulus of the binding reaction in the points R (L), i.e., in the center of the right (left) rear wheel of a vehicle under test (positioned on the support plane) [N] |
| RT | rear track width of the vehicle under test (positioned on the support plane) [m] |
| W1, … W4 | weights measured by the load cell placed under each quadrant [N] |
| W1,%, … W4,% | percentage distribution of weights on each quadrant [%] |
| WB | wheelbase of the vehicle under test (positioned on the support plane) [m] |
| WF, WR | weight supported by the front (rear) axles of the vehicle under test (positioned on the support plane) [N] |
| WFR, WFL | weight supported by the front right (left) wheel of the vehicle under test (positioned on the support plane) [N] |
| WRR, WRL | weight supported by the rear right (left) wheel of the vehicle under test (positioned on the support plane) [N] |
| Wtot | total weight supported by the wheels of the vehicle under test (positioned on the support plane); NB if the support plane inclination is not null, it is different from the weight force P of the same vehicle under test [N] |
| xSP-ySP-zSP-CoR | Cartesian frame of reference of the support plane fixed to the turntable (i.e., rotating with it) |
| xt-yt-zt-Ot | Cartesian frame of reference of a vehicle under test (positioned on the support plane, NB variously inclined with respect to the horizontal plane) |
References
- Genta, G. Motor Vehicle Dynamics: Modeling and Simulation—Series on Advances in Mathematics for Applied Sciences; World Scientific Publishing Co Pte Ltd.: Singapore, 1997. [Google Scholar]
- Resnick, R.; Halliday, D.; Krane, K.S. Physics; Wiley: Hoboken, NJ, USA, 2001; Volume 1. [Google Scholar]
- Mazzetto, F.; Bietresato, M.; Vidoni, R. Development of a dynamic stability simulator for articulated and conventional tractors useful for real-time safety devices. Appl. Mech. Mater. 2013, 394, 546–553. [Google Scholar] [CrossRef]
- Vidoni, R.; Bietresato, M.; Gasparetto, A.; Mazzetto, F. Evaluation and stability comparison of different vehicle configurations for robotic agricultural operations on side-slopes. Biosyst. Eng. 2015, 129, 197–211. [Google Scholar] [CrossRef]
- Melzi, S.; Sabbioni, E.; Vignati, M.; Cutini, M.; Brambilla, M.; Bisaglia, C.; Cavallo, E. Multibody model of fruit harvesting trucks: Comparison with experimental data and rollover analysis. J. Agric. Eng. 2018, 49, 92–99. [Google Scholar] [CrossRef]
- Hicks, D.; Grzebieta, R.; Mongiardini, M.; Rechnitzer, G.; Simmons, K.; Olivier, J. Investigation of when quad bikes rollover in the farming environment. Saf. Sci. 2018, 106, 28–34. [Google Scholar]
- Sun, C.; Nakashima, H.; Shimizu, H.; Miyasaka, J.; Ohdoi, K. Physics engine application to overturning dynamics analysis on banks and uniform slopes for an agricultural tractor with a rollover protective structure. Biosyst. Eng. 2019, 185, 150–160. [Google Scholar] [CrossRef]
- Sun, C.; Nakashima, H.; Tsukamoto, S.; Minagawa, K.; Takahashi, H.; Shimizu, H.; Miyasaka, J.; Ohdoi, K. Numerical analysis of overturning of a three-wheeled agricultural carrier with a safety frame on a slope. Eng. Agric. Environ. Food 2017, 10, 249–258. [Google Scholar] [CrossRef]
- Bietresato, M.; Mazzetto, F. Morphometry as a Key to Investigate the Stability to a Wind-Induced Rollover of Agricultural Equipment for Irrigation. Int. J. Saf. Secur. Eng. 2020, 10, 129–139. [Google Scholar]
- Mazzetto, F.; Bietresato, M.; Gasparetto, A.; Vidoni, R. Simulated stability tests of a small articulated tractor designed for extreme-sloped vineyards. J. Agric. Eng. 2013, XLIV, 663–668. [Google Scholar] [CrossRef][Green Version]
- Bietresato, M.; Carabin, G.; Vidoni, R.; Mazzetto, F.; Gasparetto, A. A parametric approach for evaluating the stability of agricultural tractors using implements during side-slope activities. Contemp. Eng. Sci. 2015, 8, 1289–1309. [Google Scholar]
- Guzzomi, A.L. A revised kineto-static model for Phase I tractor rollover. Biosyst. Eng. 2012, 113, 65–75. [Google Scholar] [CrossRef]
- Chisholm, C.J. A mathematical model of tractor overturning and impact behaviour. J. Agric. Eng. Res. 1979, 24, 375–394. [Google Scholar] [CrossRef]
- Coombes, G.B. Slope stability of Tractors. In Farm Machine Design Engineering; Design Engineering Publications: London, UK, 1968; pp. 18–33. [Google Scholar]
- Hunter, A.G.M. A review of research into machine stability on slopes. Saf. Sci. 1993, 16, 325–339. [Google Scholar] [CrossRef]
- Li, Z.; Zhu, Z.; Qin, J.; Mao, E.; Mitsuoka, M.; Inoue, E.; Shin, S. Factorial significance on tractor stability under variation of terrain roughness employing the Taguchi method. Int. J. Agric. Biol. Eng. 2018, 11, 88–94. [Google Scholar] [CrossRef]
- Zhang, S.; Li, Z.; Zhu, Z.; Mao, E.; Mitsuoka, M.; Inoue, E. Scale Model Experiment on Lateral Stability of Tractor Affected by Multi-factors. Nongye Jixie Xuebao Trans. Chin. Soc. Agric. Mach. 2017, 48, 358–363. [Google Scholar]
- Inotsume, H.; Sutoh, M.; Nagaoka, K.; Nagatani, K.; Yoshida, K. Slope traversability analysis of reconfigurable planetary rovers. In Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems, IEEE, Vilamoura, Algarve, Portugal, 7–12 October 2012; pp. 4470–4476. [Google Scholar]
- Bekker, M.G. Theory of Land Locomotion: The Mechanics of Vehicle Mobility; The University of Michigan Press: Ann Arbor, MI, USA, 1956. [Google Scholar]
- Bietresato, M.; Belotti, R.; von Ellenrieder, K.D.; Mazzetto, F. A Preliminary Study of Active Stabilization for Agricultural Machines Using a Movable Mass. In Volume 4: Dynamics, Vibration, and Control; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- Ahmadi, I. Dynamics of tractor lateral overturn on slopes under the influence of position disturbances (model development). J. Terramechanics 2011, 48, 339–346. [Google Scholar] [CrossRef]
- Chisholm, C.J. The effect of parameter variation on tractor overturning and impact behaviour. J. Agric. Eng. Res. 1979, 24, 417–440. [Google Scholar] [CrossRef]
- Yisa, M.G.; Terao, H.; Noguchi, N.; Kubota, M. Stability criteria for tractor-implement operation on slopes. J. Terramechanics 1998, 35, 1–19. [Google Scholar] [CrossRef]
- Baker, V.; Guzzomi, A.L. A model and comparison of 4-wheel-drive fixed-chassis tractor rollover during Phase I. Biosyst. Eng. 2013, 116, 179–189. [Google Scholar] [CrossRef]
- Previati, G.; Gobbi, M.; Mastinu, G. Mathematical models for farm tractor rollover prediction. Int. J. Veh. Des. 2014, 64, 280–303. [Google Scholar]
- Lindemann, R.A.; Voorhees, C.J. Mars Exploration Rover Mobility Assembly Design, Test and Performance. In Proceedings of the 2005 IEEE International Conference on Systems, Man and Cybernetics, IEEE, Waikoloa, HI, USA, 12 October 2005; Volume 1, pp. 450–455. [Google Scholar]
- Cameron, J.; Jain, A.; Huntsberger, T.; Sohl, G.; Mukherjee, R. Vehicle-Terrain Interaction Modeling and Validation for Planetary Rovers; Jet Propulsion Laboratory, National Aeronautics and Space Administration: Pasadena, CA, USA, 2009.
- UNI; ISO. UNI ISO 22915-4:2012 Carrelli Industriali—Verifica Della Stabilità—Parte 4; UNI: Milan, Italy, 2012. [Google Scholar]
- UNI—Ente Nazionale Italiano di Unificazione. UNI EN 1459-3:2015 Carrelli Elevatori Fuoristrada-Requisiti di Sicurezza e Verifica-Parte 3; UNI: Milan, Italy, 2015. [Google Scholar]
- ISO. ISO 16231-2:2015—Self-Propelled Agricultural Machinery—Assessment of Stability—Part. 2: Determination of Static Stability and Test Procedures; ISO: Geneva, Switzerland, 2015. [Google Scholar]
- OECD (Organization for Economic Co-operation and Development) Code 6—OECD Standard Code for the Official Testing of Front Mounted Rollover Protective Structures on Narrow Track Wheeled Agricultural and Forestry Tractors; OECD: Paris, France, 1990.
- Scarlett, A.J.; Reed, J.N.; Semple, D.A.; Seward, P.C.; Stockton, A.D.; Price, J.S. Operator Roll-Over Protection on Small Vehicles—Research Report 432; HSE—Health and Safety Executive: Silsoe, UK, 2006.
- Gravalos, I.; Gialamas, T.; Loutridis, S.; Moshou, D.; Kateris, D.; Xyradakis, P.; Tsiropoulos, Z. An experimental study on the impact of the rear track width on the stability of agricultural tractors using a test bench. J. Terramechanics 2011, 48, 319–323. [Google Scholar]
- Zhao, X.T.; Jiang, H.Z.; Zheng, S.T.; Han, J.W. Precision Gravity Center Position Measurement System for Heavy Vehicles. Key Eng. Mater. 2006, 315–316, 788–791. [Google Scholar] [CrossRef]
- Franceschetti, B.; Lenain, R.; Rondelli, V. Comparison between a rollover tractor dynamic model and actual lateral tests. Biosyst. Eng. 2014, 127, 79–91. [Google Scholar] [CrossRef]
- ISO. ISO 789-6:1982—Agricultural Tractors—Test. Procedures—Part. 6: Centre of Gravity; ISO—International Organization for Standardization: Geneva, Switzerland, 1982. [Google Scholar]
- Bietresato, M.; Mazzetto, F. Increasing the safety of agricultural machinery operating on sloping grounds by performing static and dynamic tests of stability on a new-concept facility. Int. J. Saf. Secur. Eng. 2018, 8, 77–89. [Google Scholar]
- Pahl, G.; Beitz, W.; Feldhusen, J.; Grote, K.-H. Engineering Design—A Systematic Approach; Springer: London, UK, 2007. [Google Scholar]
- Hsu, W.; Liu, B. Conceptual design: Issues and challenges. Comput. Des. 2000, 32, 849–850. [Google Scholar] [CrossRef]
- Deng, Y.-M.; Tor, S.B.; Britton, G.A. A dual-stage functional modelling framework with multi-level design knowledge for conceptual mechanical design. J. Eng. Des. 2000, 11, 347–375. [Google Scholar] [CrossRef]
- Bietresato, M.; Mazzetto, F. Definition of the Layout for a New Facility to Test the Static and Dynamic Stability of Agricultural Vehicles Operating on Sloping Grounds. Appl. Sci. 2019, 9, 4135. [Google Scholar] [CrossRef]
- Bietresato, M.; Mazzetto, F. Proposal of an advanced facility to perform static and dynamic tests of stability on agricultural machines. Chem. Eng. Trans. 2017, 58, 151–156. [Google Scholar]
- OECD (Organization for Economic Co-operation and Development) Code 4—OECD Standard Code for the Official Testing of Protective Structures on Agricultural and Forestry Tractors (Static Test); OECD: Paris, France, 2020.
- Fu, C.; Xu, Y.; Yang, Y.; Lu, K.; Gu, F.; Ball, A. Response analysis of an accelerating unbalanced rotating system with both random and interval variables. J. Sound Vib. 2020, 466, 115047. [Google Scholar] [CrossRef]
- Kulikowski, K.; Szpica, D. Determination of directional stiffnesses of vehicles’ tires under a static load operation. Maint. Reliab. 2014, 16, 66–72. [Google Scholar]
- FEDERUNACOMA—Federazione Nazionale Costruttori Macchine per l’Agricoltura/Italian Agricultural Machinery Manufacturers Federation Annuari FEDERUNACOMA. Available online: http://www.federunacoma.it/en/index.php (accessed on 2 April 2020).
- Bietresato, M.; Calcante, A.; Mazzetto, F. A neural network approach for indirectly estimating farm tractors engine performances. Fuel 2015, 143, 144–154. [Google Scholar]
- CNH Industrial New Holland—Agricultural & Farm Machinery. Available online: https://agriculture.newholland.com/eu/en-uk?market=uk (accessed on 1 April 2020).
- Istituto per la Meccanizzazione Agricola. Test in Accordance with the OECD Code 1 for the Official Testing of Agricultural Tractors—New Holland TL 90 4WD—OECD Approval No.: 1/1915—Report No. 2648/T/2000; Consiglio Nazionale delle Ricerche—Istituto per la Meccanizzazione Agricola: Torino, Italy, 2000. [Google Scholar]
- Bietresato, M.; Bisaglia, C.; Merola, M.; Brambilla, M.; Cutini, M.; Mazzetto, F. An application of morphometry to artificial systems: The evolutionary study of farm tractors. Chem. Eng. Trans. 2017, 58, 145–150. [Google Scholar]
- ETRTO—European Tyre and Rim Technical Organisation. Standards Manual 2020; ETRTO—European Tyre and Rim Technical Organisation: Brussels, Belgium, 2020. [Google Scholar]
- Gorshkov, Y.G.; Starunova, I.N.; Kalugin, A.A.; Troyanovskaya, I.P. Investigation of the slope angle influence on the loading imbalance of the wheeled vehicle sides and the change in the center of gravity vector direction. J. Phys. Conf. Ser. 2019, 1177, 012005. [Google Scholar] [CrossRef]
- Brassart, F.P.; Wright, M.E. A Machine to Study Vertical Tire Stiffness and Damping Coefficient; SAE Technical Papers; SAE International: Warrendale, PA, USA, 1993. [Google Scholar]
- Anifantis, A.S.; Cutini, M.; Bietresato, M. An Experimental–Numerical Approach for Modelling the Mechanical Behaviour of a Pneumatic Tyre for Agricultural Machines. Appl. Sci. 2020, 10, 3481. [Google Scholar] [CrossRef]


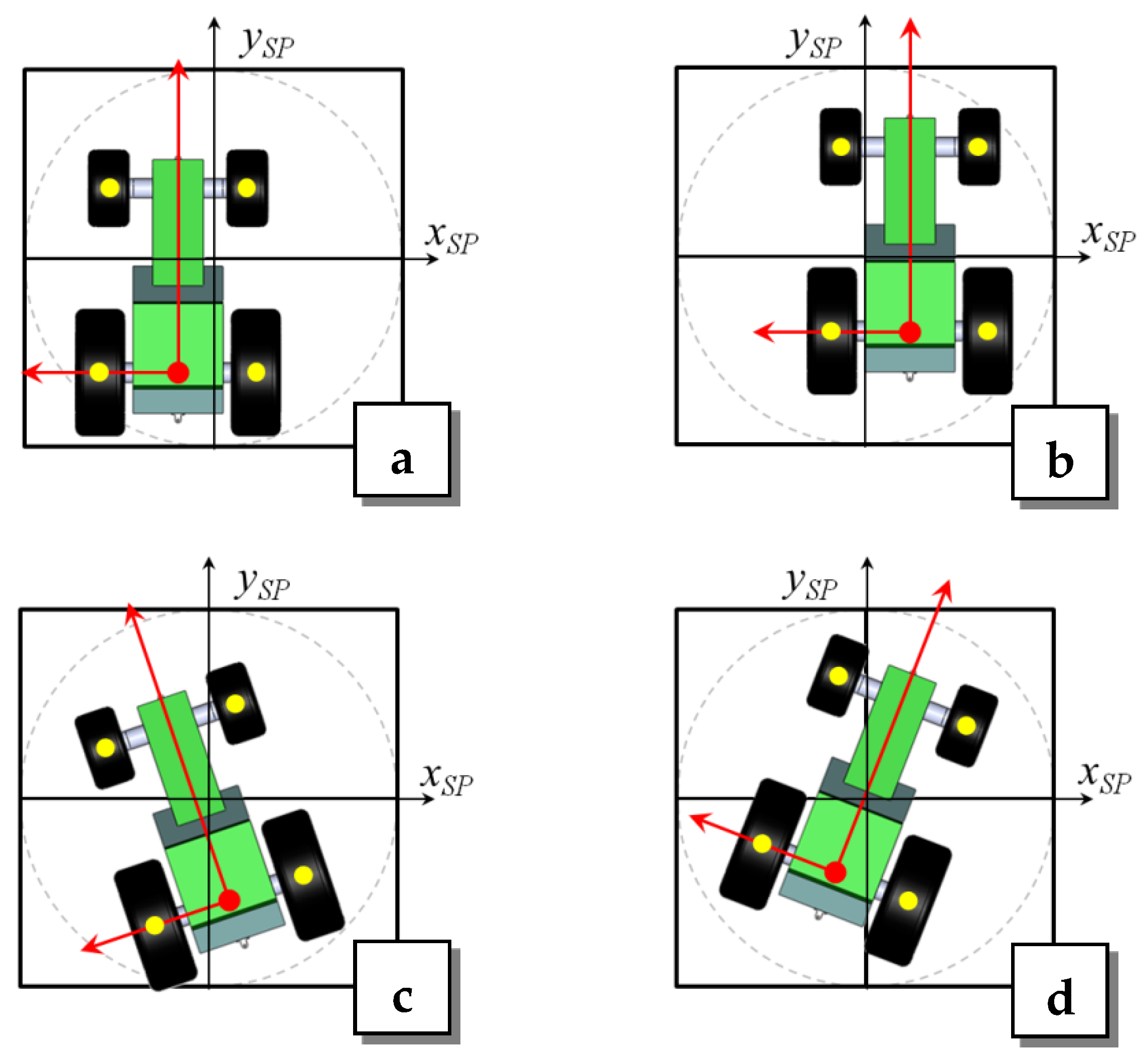
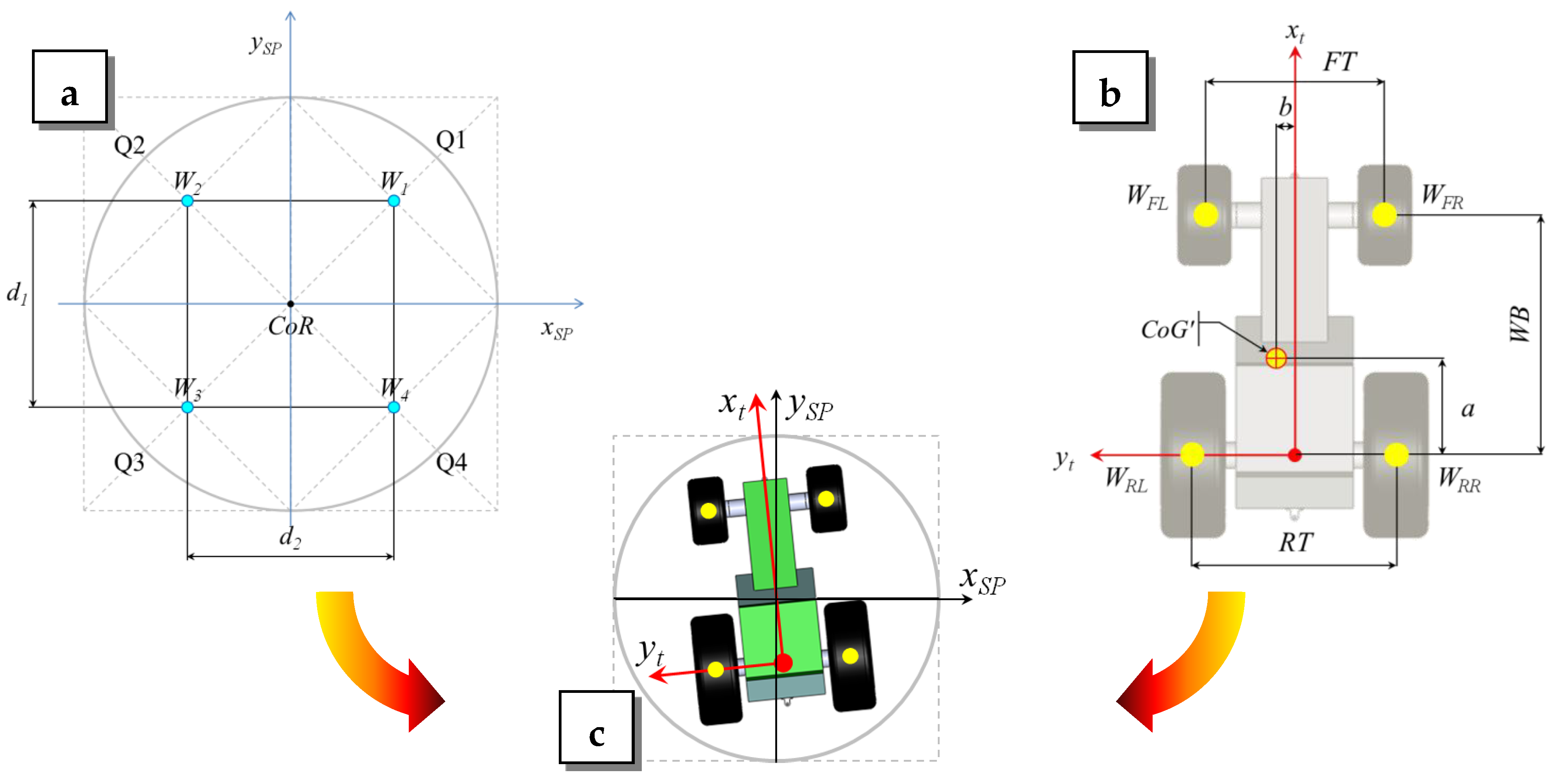
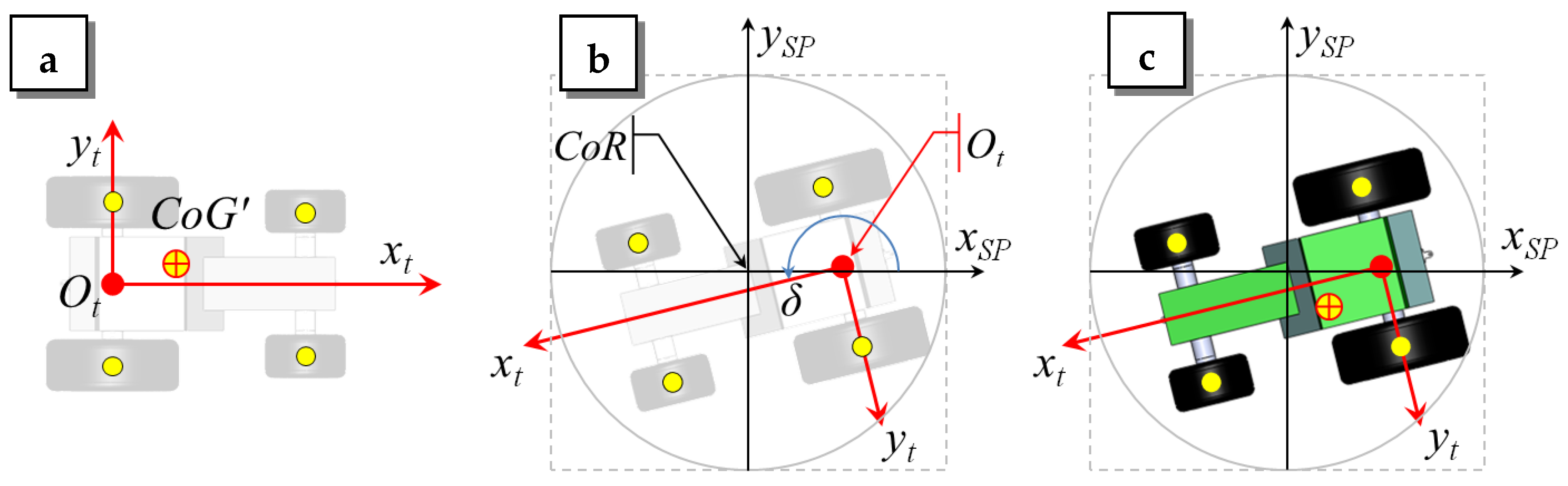

| Test Scenario | Pure Lateral Inclination | Pure Frontal Inclination | Generic Inclination (Realistic Situation) |
|---|---|---|---|
| Characteristic angles of the vehicle in the frame of reference of the test system (support plane inclination, vehicle longitudinal axis direction) | (α, ±90°) | (α, 0°/±180°) | (α, β′) |
| Characteristic angles of vehicle (roll, pitch, yaw) | (φ, 0, 0) = (α, 0, 0) | (0, ϑ, 0) = (0, α, 0) | (φ, ϑ, ψ) |
| Test able to generate the described scenario | Traditional test; turntable test (in partic.: global completely static tests with β′ = ±90°) | Traditional test; turntable test (in partic.: global completely static tests with β′ = 0° or ±180°) | Turntable test (in partic.: global completely static tests, global quasi-static test) |
| Quantity | Symbol | Value | Unit | |
|---|---|---|---|---|
| Support polygon dimensions | Wheelbase | WB | 2314 | mm |
| Front track width | FT | 1305 | mm | |
| Rear track width | RT | 1350 | mm | |
| CoG position | CoG height | h | 850 | mm |
| CoG distance from the rear axle | dR | 965 | mm | |
| CoG distance from the longitudinal axis (leftward if seen from the rear part) | dF | 8 | mm | |
| Total mass | m | 3820 | kg | |
| Point | Coordinates | Quadrant | ||
|---|---|---|---|---|
| xSP (mm) | ySP (mm) | |||
| CoG″ | Projection of CoG on the support surface when this is horizontal | +0.0 | −8.0 | 1–4 |
| FR | Centre of the front right wheel | −1349.0 | +652.5 | 2 |
| FL | Centre of the front left wheel | −1349.0 | −652.5 | 3 |
| RR | Centre of the rear right wheel | +965.0 | +675.0 | 1 |
| RL | Centre of the rear left wheel | +965.0 | −675.0 | 4 |
| Quantity | Meas. Unit | α | ||
|---|---|---|---|---|
| 0.00° | 20.00° | 37.73° | ||
| WFR (≡ W2) | N | 7717 | 3840 | 0 |
| WFL (≡ W3) | N | 7905 | 10,840 | 12,356 |
| WRR (≡ W1) | N | 10,788 | 5368 | 0 |
| WRL (≡ W4) | N | 11,051 | 15,154 | 17,273 |
| Normal force (∑Wi) | N | 37,461 | 35,202 | 29,629 |
| WA ( = W2 + W3; front part of the tractor) | N | 15,622 | 14,680 | 12,356 |
| WB ( = W1 + W4; rear part of the tractor) | N | 21,839 | 20,522 | 17,273 |
| WC ( = W1 + W2; right part of the tractor) | N | 18,506 | 9209 | 0 |
| WD ( = W3 + W4; left part of the tractor) | N | 18,956 | 25,994 | 29,629 |
| x(CoG′)SP | mm | 0.0 | 0.0 | 0.0 |
| y(CoG′)SP | mm | −8.0 | −317.4 | −665.6 |
| Wheels Loads (N) | Wheels Load Distribution (%) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| WFR | WFL | WRR | WRL | WFR,% | WFL,% | WRR,% | WRL,% | ||
| 20.00° | min | 3601 | 3755 | 5210 | 5446 | 10.2 | 10.7 | 14.8 | 15.5 |
| max | 11,910 | 12,121 | 15,862 | 16,141 | 33.8 | 34.4 | 45.1 | 45.9 | |
| 37.73° | min | 0 | 149 | 0 | 208 | 0.0 | 0.5 | 0.0 | 0.7 |
| max | 15,518 | 15,729 | 19,801 | 20,068 | 52.4 | 53.1 | 66.8 | 67.7 | |
| Tyre Denomination | Rim Diameter (in.) | Load Index (-) | Overall Diameter (mm) | Load Capacity (kgf) | Nominal Inflation Press (bar) | Speed Radius Index (-) |
|---|---|---|---|---|---|---|
| 14.9–24 | 24 | 126 | 1245 | 1700 | 1.6 | 600 |
| 18.4–34 | 34 | 144 | 1645 | 2800 | 1.6 | 775 |
| Wheels Loads (N) | Wheels Load Distribution (%) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| WFR | WFL | WRR | WRL | WFR,% | WFL,% | WRR,% | WRL,% | ||
| 20.00 | min | 3182 | 3338 | 4601 | 4843 | 9.0 | 9.5 | 13.1 | 13.8 |
| max | 12,446 | 12,669 | 16,618 | 16,899 | 35.4 | 36.0 | 47.2 | 48.0 | |
| 33.95 | min | 0 | 163 | 0 | 228 | 0.0 | 0.5 | 0.0 | 0.7 |
| max | 15,869 | 16,102 | 20,394 | 20,686 | 51.1 | 51.8 | 65.6 | 66.6 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bietresato, M.; Mazzetto, F. Stability Tests of Agricultural and Operating Machines by Means of an Installation Composed by a Rotating Platform (the “Turntable”) with Four Weighting Quadrants. Appl. Sci. 2020, 10, 3786. https://doi.org/10.3390/app10113786
Bietresato M, Mazzetto F. Stability Tests of Agricultural and Operating Machines by Means of an Installation Composed by a Rotating Platform (the “Turntable”) with Four Weighting Quadrants. Applied Sciences. 2020; 10(11):3786. https://doi.org/10.3390/app10113786
Chicago/Turabian StyleBietresato, Marco, and Fabrizio Mazzetto. 2020. "Stability Tests of Agricultural and Operating Machines by Means of an Installation Composed by a Rotating Platform (the “Turntable”) with Four Weighting Quadrants" Applied Sciences 10, no. 11: 3786. https://doi.org/10.3390/app10113786
APA StyleBietresato, M., & Mazzetto, F. (2020). Stability Tests of Agricultural and Operating Machines by Means of an Installation Composed by a Rotating Platform (the “Turntable”) with Four Weighting Quadrants. Applied Sciences, 10(11), 3786. https://doi.org/10.3390/app10113786






