Performance of Tilting Pad Journal Bearings with the Same Sommerfeld Number
Abstract
:1. Introduction
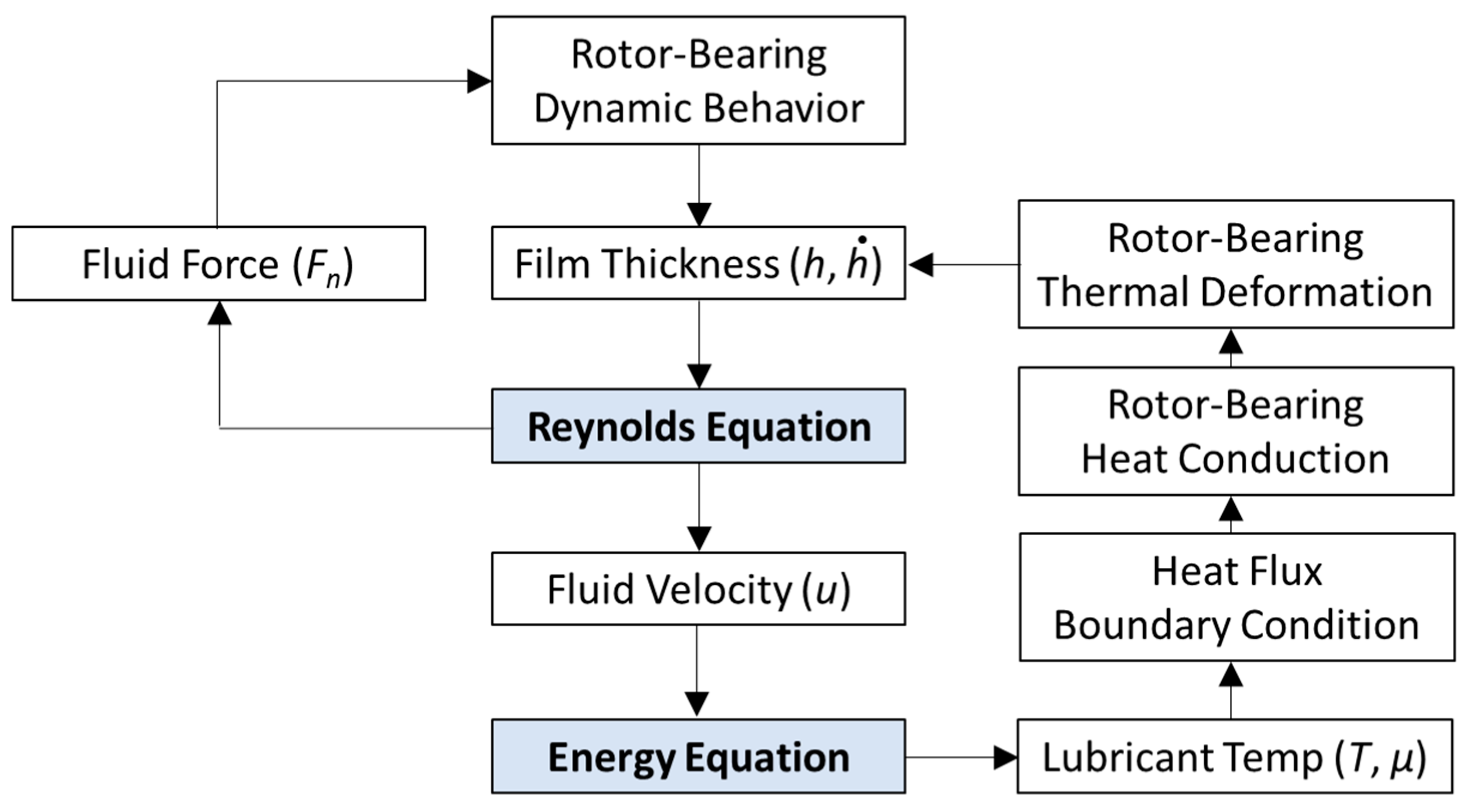
2. Numerical Modeling of Tilting Pad Journal Bearing
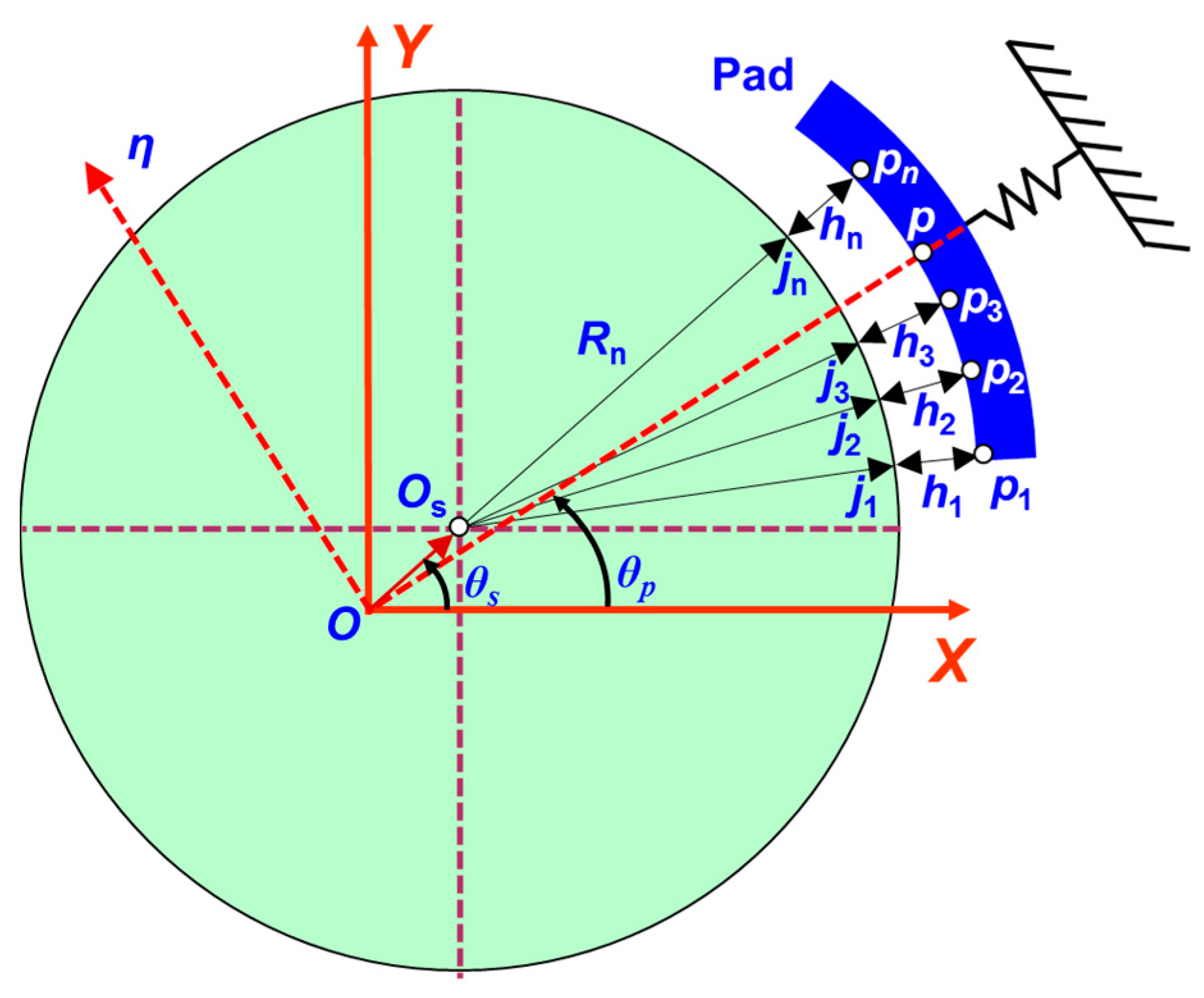
2.1. Generalized Reynolds Equation
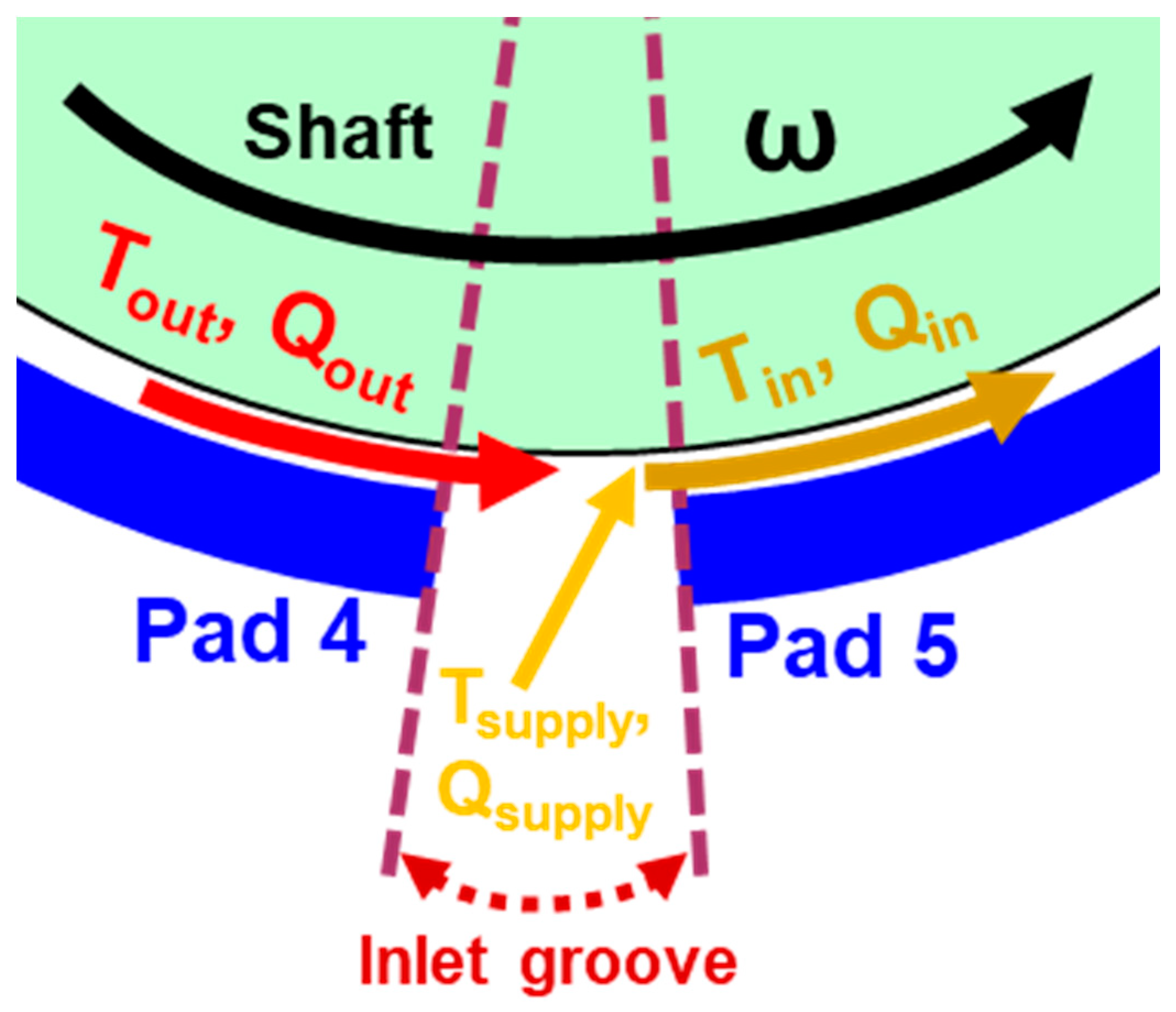
2.2. Energy Equation
2.3. Flexible Pad Model and Nonlinear Pivot Model
2.4. Bearing-Journal Model
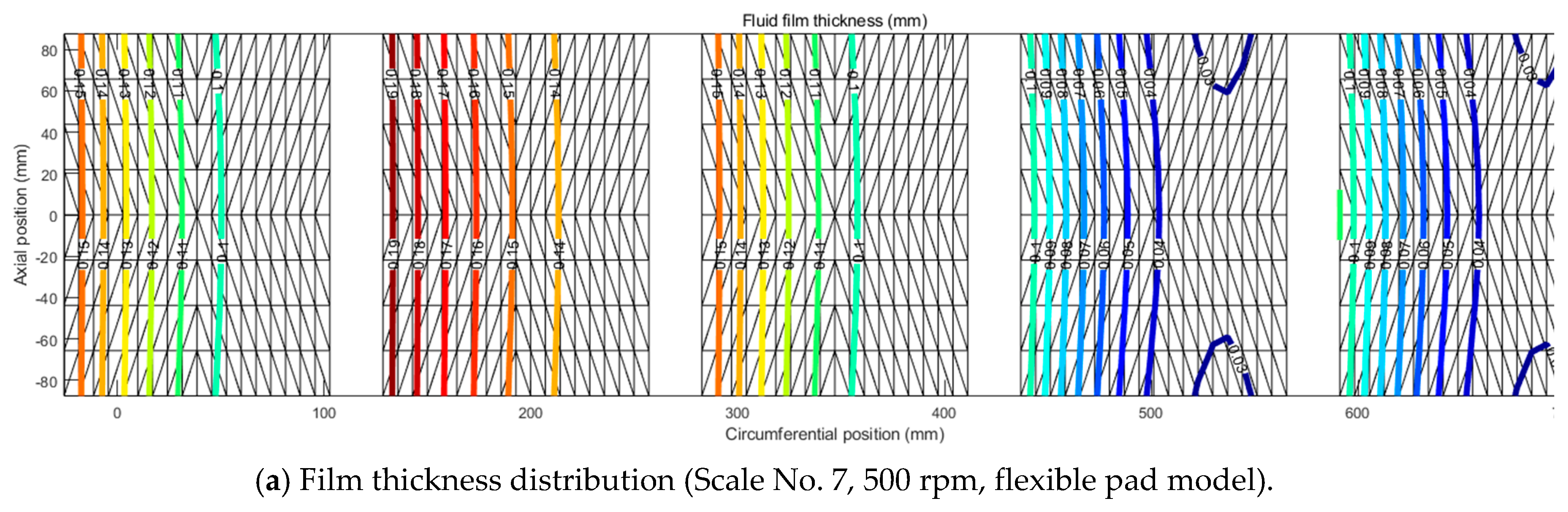
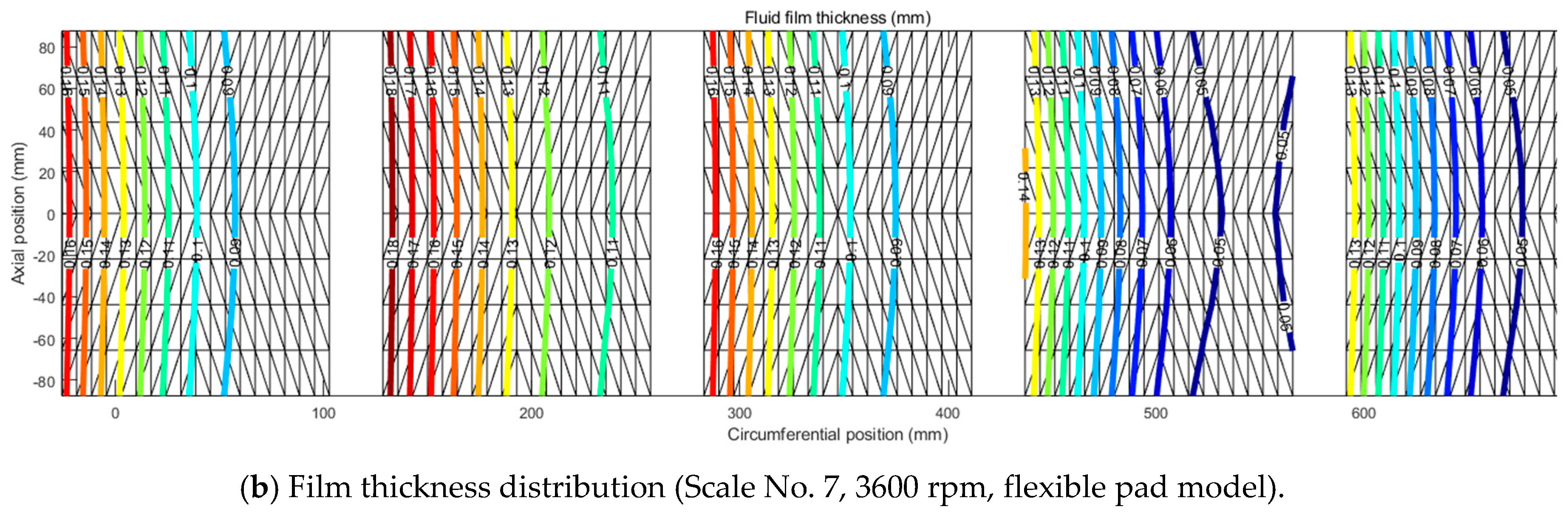
3. Simulation Results and Discussions
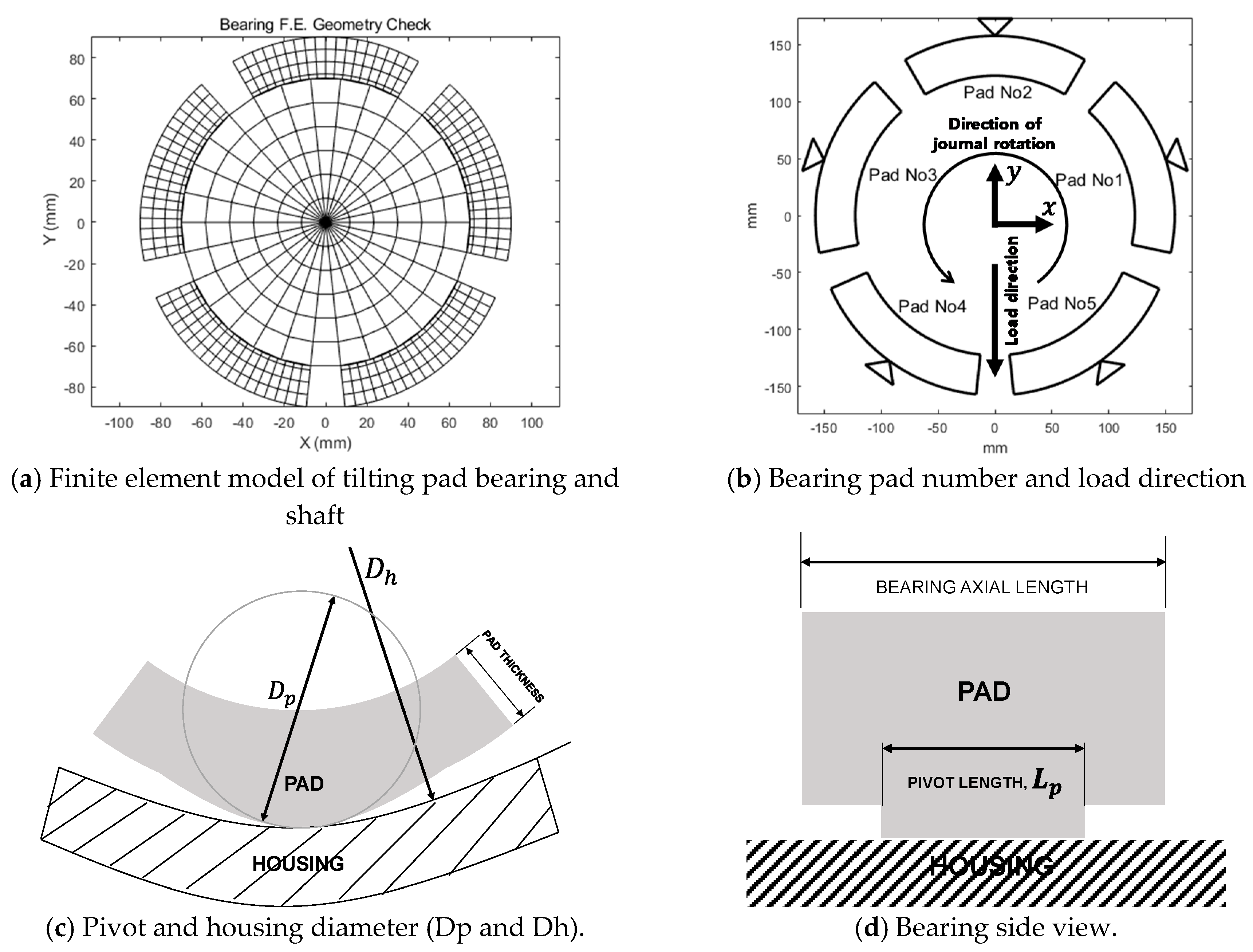
3.1. Simulation Model
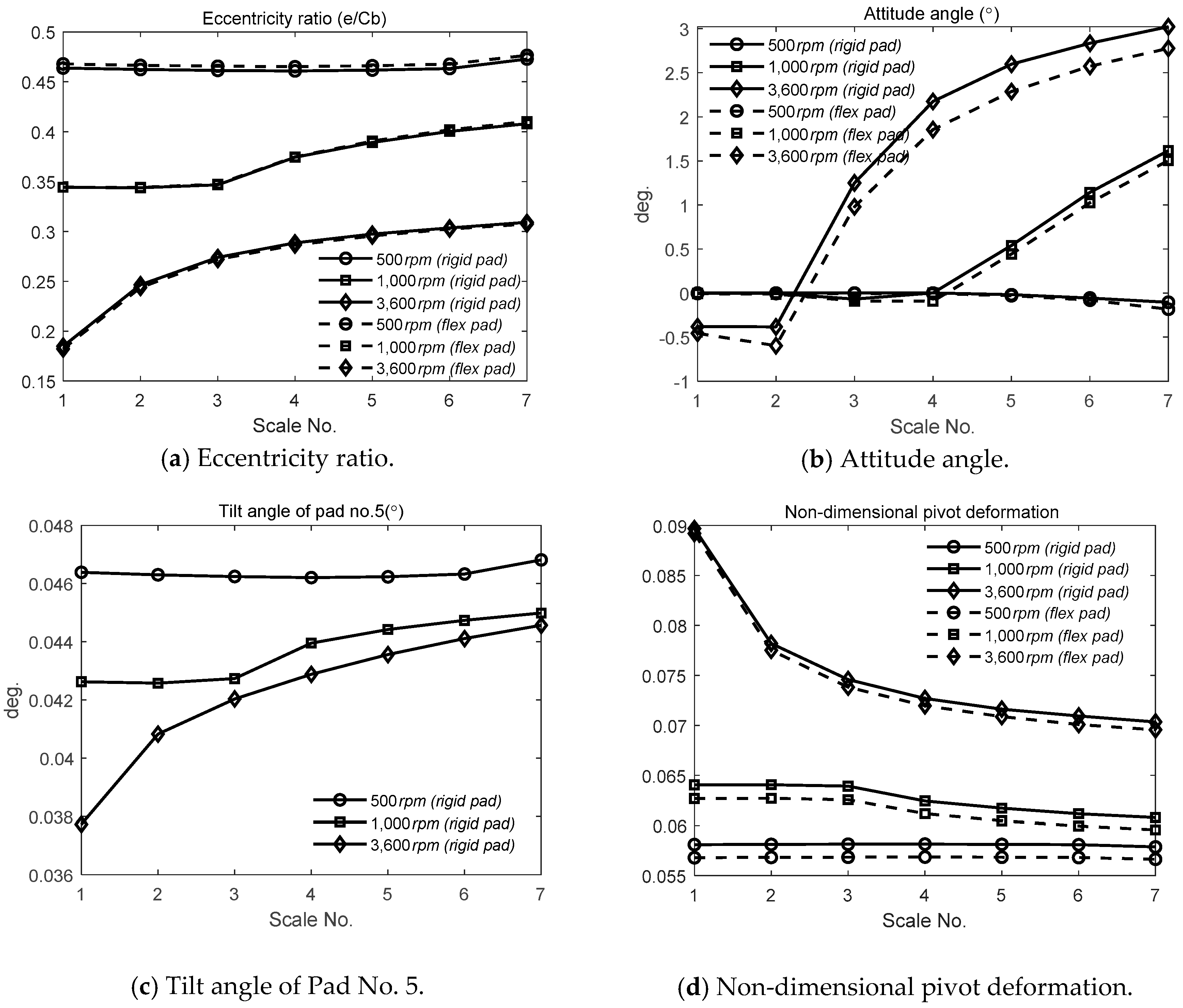
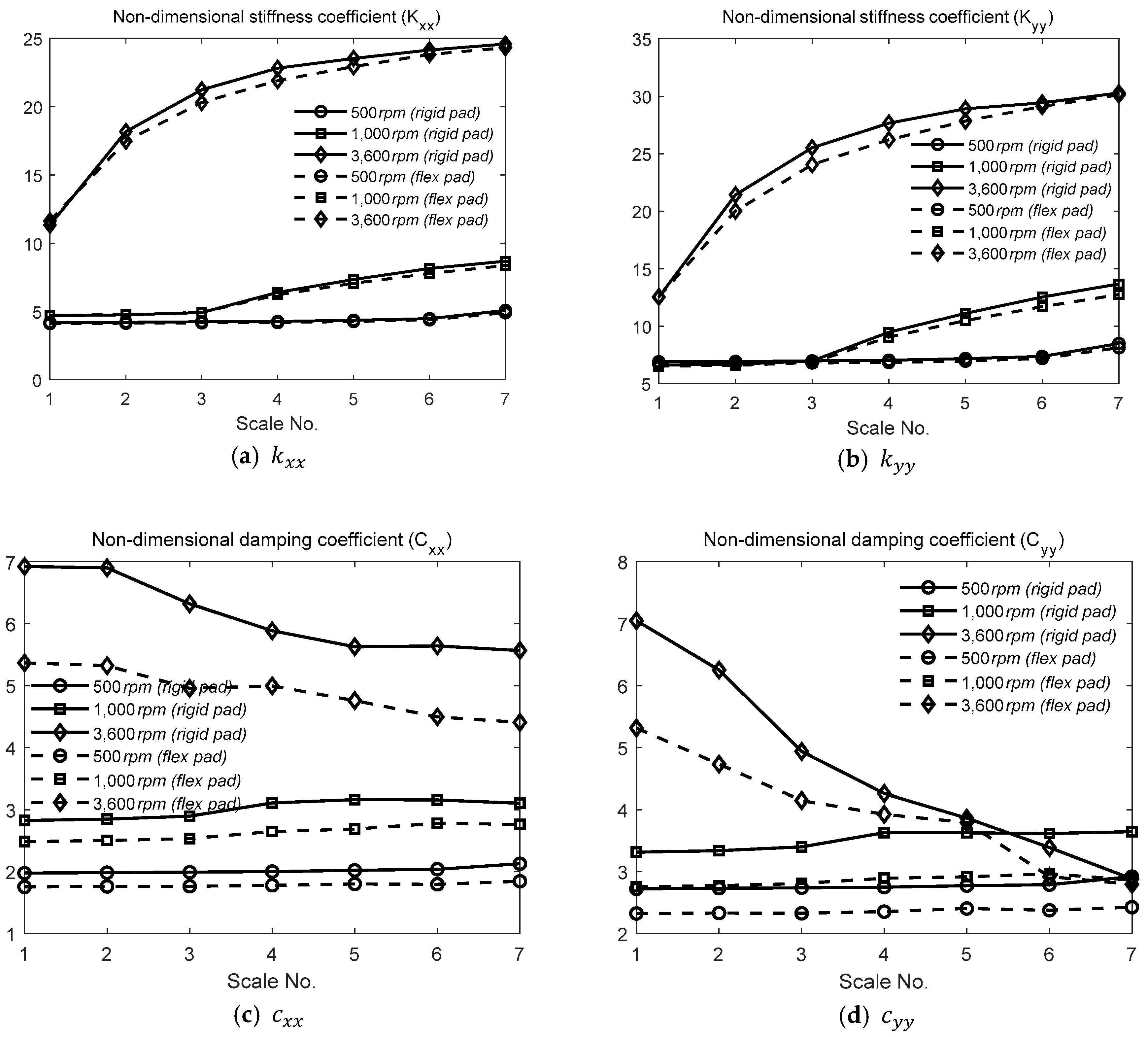
3.2. Bearing Static and Dynamic Characteristics
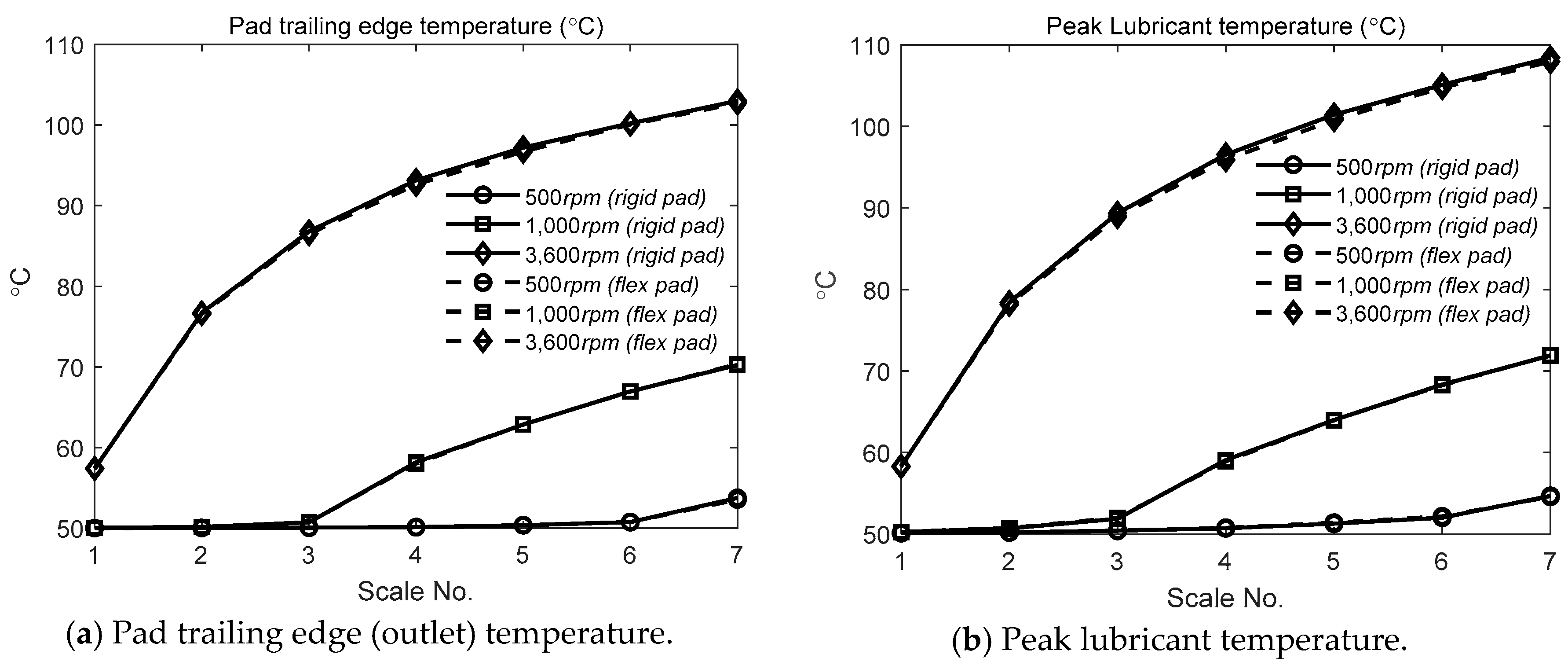
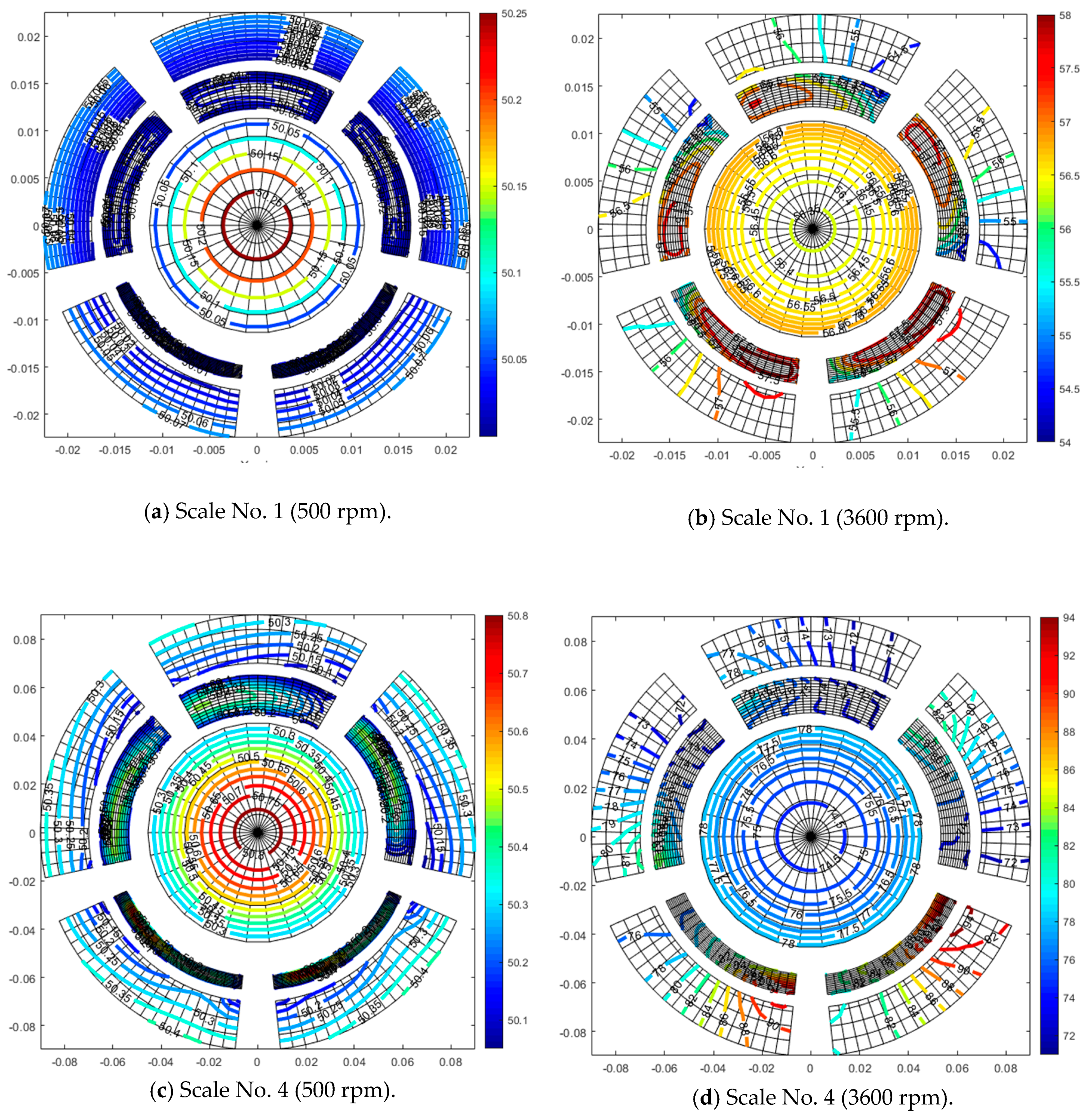
3.3. Bearing Thermal Behavior
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Film Thickness | |
| Lubricant viscosity | |
| Shaft surface linear velocity | |
| Time | |
| Nodal normal fluid force acting on the bearing pad and spinning journal | |
| X-Component of Journal Position | |
| Y-Component of Journal Position | |
| Bearing Clearance | |
| Pad number | |
| Nodal position in circumferential direction | |
| Nodal position in axial direction | |
| Number of nodes in circumferential direction of the lubricant FE model | |
| Number of nodes in axial direction of the lubricant FE model | |
| Journal radial center | |
| Angular position of node () | |
| Moment of inertia of the pad in axis | |
| Moment of inertia of the pad in axis | |
| Moment of inertia of the pad in axis | |
| Local axis for the journal radial position | |
| Local axis for the journal angular position | |
| Local axis for the pad angular position | |
| Pad tilt angle | |
| Pad pitch angle | |
| Pad yaw angle | |
| Pivot displacement | |
| Circumferential nodal position | |
| Axial nodal position | |
| Pad clearance | |
| Journal angular position in x direction | |
| Journal angular position in y direction | |
| Pivot angular position | |
| Journal radius |
References
- Someya, T. Journal-Bearing Databook; Springer: Berlin, Germany, 1989. [Google Scholar]
- Lund, J.W. Spring and Damping Coefficients for the Tilting-Pad Journal Bearing. ASLE Trans. 1964, 7, 342–352. [Google Scholar] [CrossRef]
- Dowson, D.; Hudson, J.D.; Hunter, B.; March, C.N. Paper 3: An Experimental Investigation of the Thermal Equilibrium of Steadily Loaded Journal Bearings. Proc. Inst. Mech. Eng. Conf. Proc. 1966, 181, 70–80. [Google Scholar] [CrossRef]
- Khonsari, M.M.; Jang, J.Y.; Fillon, M. On the Generalization of Thermohydrodynamic Analyses for Journal Bearings. J. Tribol. 1996, 118, 571–579. [Google Scholar] [CrossRef]
- Brockett, T.S.; Barrett, L.E.; Allaire, P.E. Thermoelastohydrodynamic Analysis of Fixed Geometry Thrust Bearings Including Runner Deformation. Tribol. Trans. 1996, 39, 555–562. [Google Scholar] [CrossRef]
- Kim, J.; Palazzolo, A.B.; Gadangi, R.K. TEHD Analysis for Tilting-Pad Journal Bearings Using Upwind Finite Element Method. Tribol. Trans. 1994, 37, 771–783. [Google Scholar] [CrossRef]
- Fillon, M.; Bligoud, J.-C.; Frêne, J. Experimental Study of Tilting-Pad Journal Bearings—Comparison with Theoretical Thermoelastohydrodynamic Results. J. Tribol. 1992, 114, 579–587. [Google Scholar] [CrossRef]
- Monmousseau, P.; Fillon, M. Frequency Effects on the TEHD Behavior of a Tilting-Pad Journal Bearing Under Dynamic Loading. J. Tribol. 1999, 121, 321–326. [Google Scholar] [CrossRef]
- Nilsson, L. The Influence of Bearing Flexibility on the Dynamic Performance of Radial Oil Film Bearings. In Proceedings of the 5th Leeds-Lyon Symposium on Tribology, Lyon, France, 6–9 September 1978; pp. 311–319. [Google Scholar]
- Kirk, R.G.; Reedy, S.W. Evaluation of Pivot Stiffness for Typical Tilting-Pad Journal Bearing Designs. J. Vib. Acoust. 1988, 110, 165–171. [Google Scholar] [CrossRef]
- Wilkes, J.C.; Childs, D.W. Tilting Pad Journal Bearings—A Discussion on Stability Calculation, Frequency Dependence, and Pad and Pivot Flexibility. ASME J. Eng. Gas Turbines Power 2012, 134, 122508. [Google Scholar] [CrossRef]
- Gaines, J. Examining the Impact of Pad Flexibility on the Rotordynamic Coefficients of Rocker-Pivot-Pad Tiling-Pad Journal Bearings. Master’s Thesis, Mechanical Engineering, Texas A&M University, College Station, TX, USA, 2014. [Google Scholar]
- Andres, L.S.; Li, Y. Effect of Pad Flexibility on the Performance of Tilting Pad Journal Bearings—Benchmarking a Predictive Model. J. Eng. Gas Turbines Power 2015, 137, 122503. [Google Scholar] [CrossRef]
- Andres, L.S.; Tao, Y.; Li, Y. Tilting Pad Journal Bearings: On Bridging the Hot Gap Between Experimental Results and Model Predictions. J. Eng. Gas Turbines Power 2014, 137, 022505. [Google Scholar] [CrossRef]
- DeCamillo, S.M.; He, M.; Cloud, C.H.; Byrne, J.M. Journal Bearing Vibration and SSV Hash. In Proceedings of the 37th Turbomachinery Symposium, Houston, TX, USA, 7–11 September 2008. [Google Scholar]
- Gomiciaga, R.; Keogh, P.S. Orbit Induced Journal Temperature Variation in Hydrodynamic Bearings. J. Tribol. 1999, 121, 77–84. [Google Scholar] [CrossRef]
- Andres, L.S.; Koo, B.; Hemmi, M. A Flow Starvation Model for Tilting Pad Journal Bearings and Evaluation of Frequency Response Functions: A Contribution Toward Understanding the Onset of Low Frequency Shaft Motions. J. Eng. Gas Turbines Power 2018, 140, 052506. [Google Scholar] [CrossRef]
- Dimond, T.W.; Younan, A.A.; Allaire, P. Comparison of Tilting-Pad Journal Bearing Dynamic Full Coefficient and Reduced Order Models Using Modal Analysis (GT2009-60269). J. Vib. Acoust. 2010, 132, 051009. [Google Scholar] [CrossRef]
- Suh, J.; Palazzolo, A. Three-Dimensional Dynamic Model of TEHD Cylindrical-Pivot Tilting-Pad Bearing, Part I: Numerical Modeling. J. Tribol. 2015, 117, 041703. [Google Scholar] [CrossRef]
- Suh, J.; Palazzolo, A. Three-Dimensional Dynamic Model of TEHD Cylindrical-Pivot Tilting-Pad Bearing, Part II: Parametric Studies. J. Tribol. 2015, 117, 041704. [Google Scholar] [CrossRef]
- Suh, J.; Palazzolo, A.B. Three-Dimensional Thermohydrodynamic Morton Effect Simulation—Part I: Theoretical Model. J. Tribol. 2014, 136, 031706. [Google Scholar] [CrossRef]
- Young, W.C.; Budynas, R.G. Roark’s Formulas for Stress and Strain; McGraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Suh, J.; Choi, Y.-S. Pivot design and angular misalignment effects on tilting pad journal bearing characteristics: Four pads for load on pad configuration. Tribol. Int. 2016, 102, 580–599. [Google Scholar] [CrossRef]
- Dimond, T.; Younan, A.; Allaire, P. A Review of Tilting Pad Bearing Theory. Int. J. Rotating Mach. 2011, 2011, 1–23. [Google Scholar] [CrossRef] [Green Version]



| Lubricant Properties | Value |
|---|---|
| Viscosity at 40 °C (N·s/m2) | 0.0365 |
| Viscosity coefficient (Pa·s) | 0.0297 |
| Heat capacity (J/kg °C) | 1886 |
| Heat conductivity (W/(mK)) | 0.136 |
| Lubricant supply temperature (°C) | 50 |
| Density (kg/m3) | 877 |
| Bearing | |
| Load type | LBP |
| Number of pads | 5 |
| Pad arc length (°) | 60 |
| Offset | 0.5 |
| Preload | 0.4 |
| Load direction (deg.) | 270 (−y) |
| Journal Material | |
| Young’s Modulus (Pa) | 2.05 × 1011 |
| Density (kg/m3) | 7850 |
| Poison’s Ratio | 0.3 |
| Heat Capacity (J/(kg °C)) | 453.6 |
| Heat Conductivity (W/(m °C)) | 42.6 |
| Therm. Exp. Coeff (1/ °C) | 0.0000122 |
| Ref. Temp. for T.exp (°C) | 25 |
| Pad Material | |
| Young’s Modulus (Pa) | 2.00 × 1011 |
| Density (kg/m3) | 7858 |
| Poison’s Ratio | 0.3 |
| Heat Capacity (J/(kg °C) | 453.6 |
| Heat Conductivity (W/(m °C)) | 51.9 |
| Therm. Exp. Coeff (1/°C) | 0.0000121 |
| Ref. Temp. for Texp (°C) | 25 |
| Babbit Material | |
| Young’s Modulus (Pa) | 5.3 × 1010 |
| Density (kg/m3) | 7390 |
| Poison’s Ratio | 0.3 |
| Heat Capacity (J/(kg °C) | 230 |
| Heat Conductivity (W/(m °C)) | 55 |
| Therm. Exp. Coeff (1/°C) | 0.000021 |
| Ref. Temp. for Texp (°C) | 25 |
| Housing Material | |
| Young’s Modulus (Pa) | 1.86 × 1011 |
| Poison’s Ratio | 0.3 |
| Scale No. | Journal Diameter (mm) | Pad Thickness (mm) | Babbit Thickness (mm) | Dp (mm) | Dh (mm) | Pad Clearance (mm) | Pad Length (mm) | Bearing Load (N) |
|---|---|---|---|---|---|---|---|---|
| 1 | 35 | 5 | 0.5 | 38 | 45 | 0.032 | 25 | 1230 |
| 2 | 70 | 10 | 1 | 76 | 90 | 0.064 | 50 | 4920 |
| 3 | 105 | 15 | 1.5 | 114 | 135 | 0.096 | 75 | 11,070 |
| 4 | 140 | 20 | 2 | 152 | 180 | 0.128 | 100 | 19,680 |
| 5 | 175 | 25 | 2.5 | 190 | 225 | 0.16 | 125 | 30,750 |
| 6 | 210 | 30 | 3 | 228 | 270 | 0.192 | 150 | 44,280 |
| 7 | 245 | 35 | 3.5 | 266 | 315 | 0.224 | 175 | 60,270 |
| Running Condition | 1 | 2 | 3 |
|---|---|---|---|
| Rotor spin speed (rpm) | 500 | 1000 | 3600 |
| Sommerfeld Number | 0.065 | 0.129 | 0.466 |
| Unit Load | 1.41 | 1.41 | 1.41 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jeung, S.-H.; Suh, J.; Yoon, H.S. Performance of Tilting Pad Journal Bearings with the Same Sommerfeld Number. Appl. Sci. 2020, 10, 3529. https://doi.org/10.3390/app10103529
Jeung S-H, Suh J, Yoon HS. Performance of Tilting Pad Journal Bearings with the Same Sommerfeld Number. Applied Sciences. 2020; 10(10):3529. https://doi.org/10.3390/app10103529
Chicago/Turabian StyleJeung, Sung-Hwa, Junho Suh, and Hyun Sik Yoon. 2020. "Performance of Tilting Pad Journal Bearings with the Same Sommerfeld Number" Applied Sciences 10, no. 10: 3529. https://doi.org/10.3390/app10103529
APA StyleJeung, S.-H., Suh, J., & Yoon, H. S. (2020). Performance of Tilting Pad Journal Bearings with the Same Sommerfeld Number. Applied Sciences, 10(10), 3529. https://doi.org/10.3390/app10103529






