Teacher Intervention During Collaborative Problem Solving in Mathematics Classrooms in Mainland China
Abstract
1. Introduction
2. Literature Review
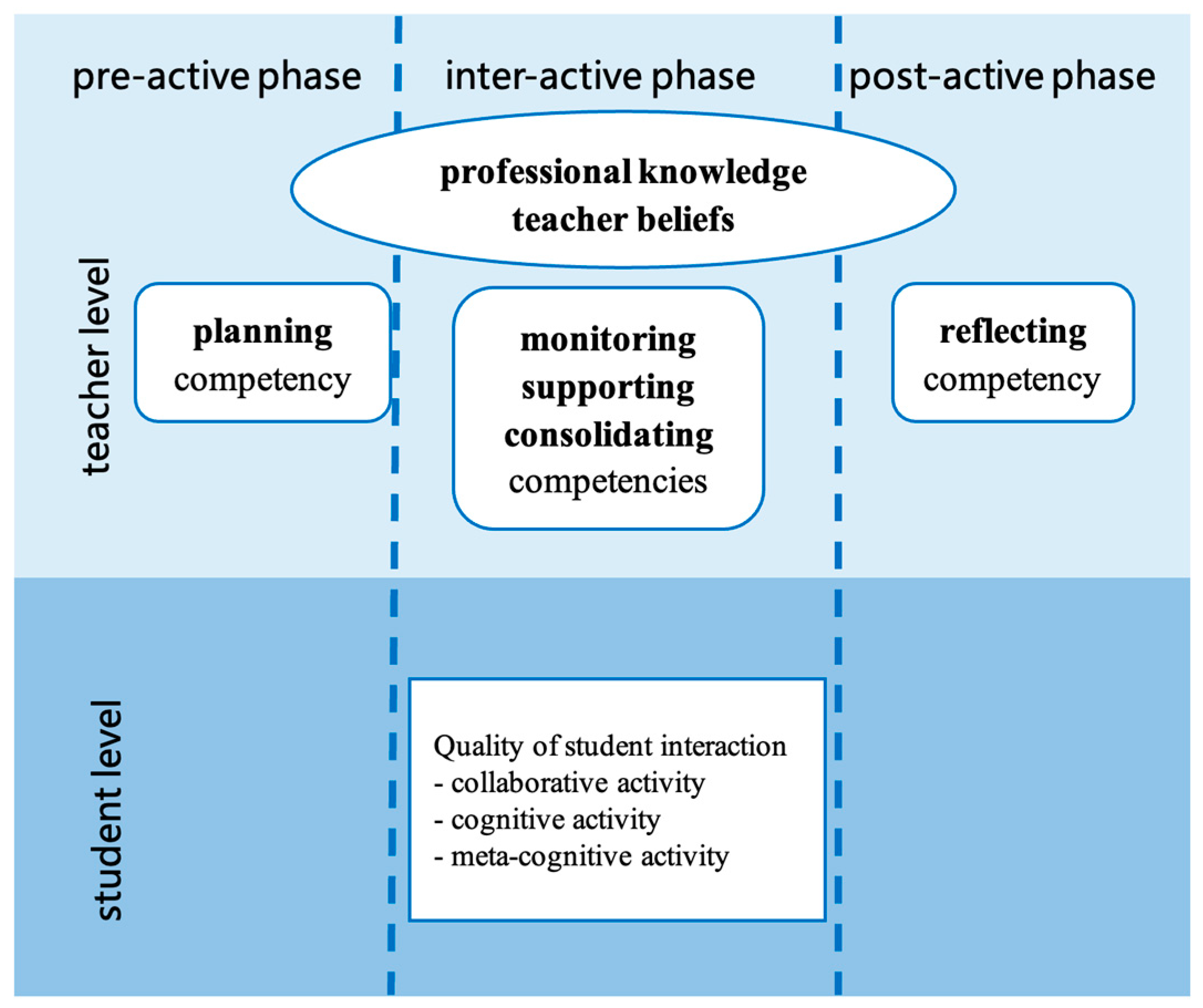
2.1. Teacher Intervention During Collaborative Problem Solving
2.2. Focus and Means of Teacher Intervention
2.3. Teacher–Group Interactions in China’s Mathematics Classrooms
2.4. Research Questions
3. Methodology
3.1. Participants
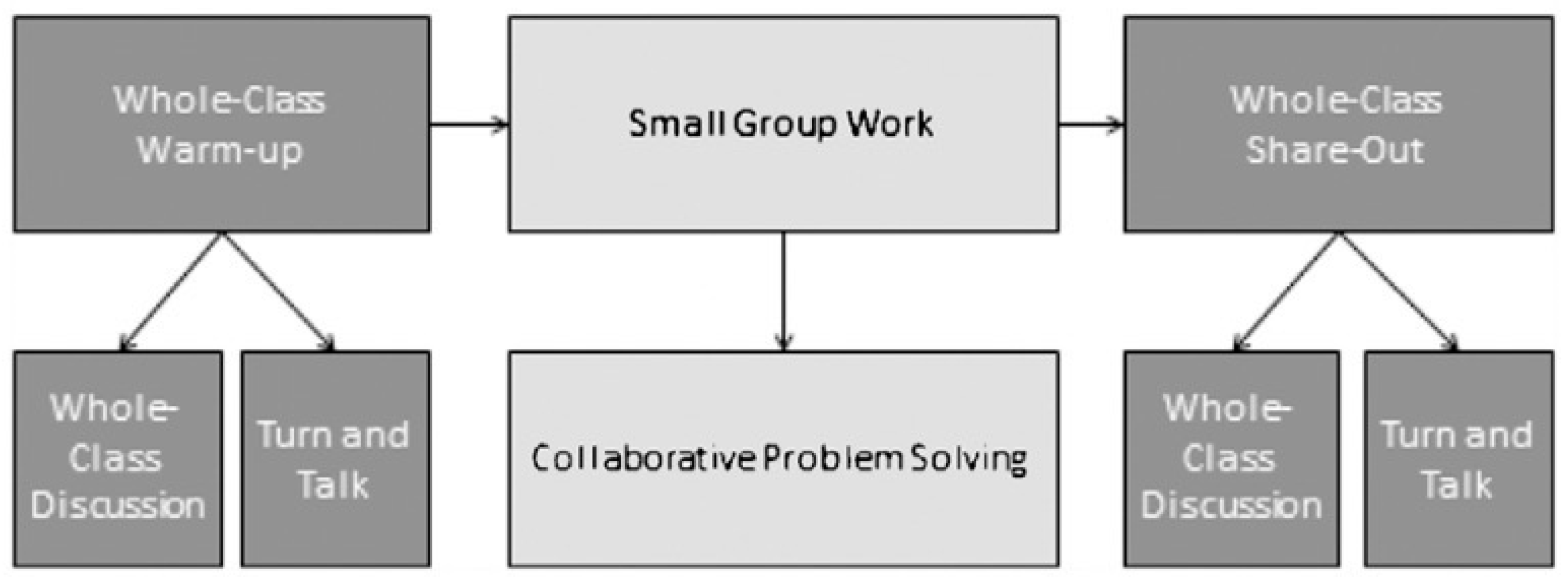
3.2. Tasks
- Paired task
- Small-group task
- (1)
- 请画一幅图展示小明的公寓。
- (2)
- 标注其中每一个房间的功能及尺寸(长和宽)。
3.3. Data Collection
3.4. Data Analysis
3.4.1. Scoring of Task Sheet
3.4.2. Analysis of Teacher–Student Dialogues
4. Results
4.1. Task Sheets
4.2. Dialogue
5. Discussions
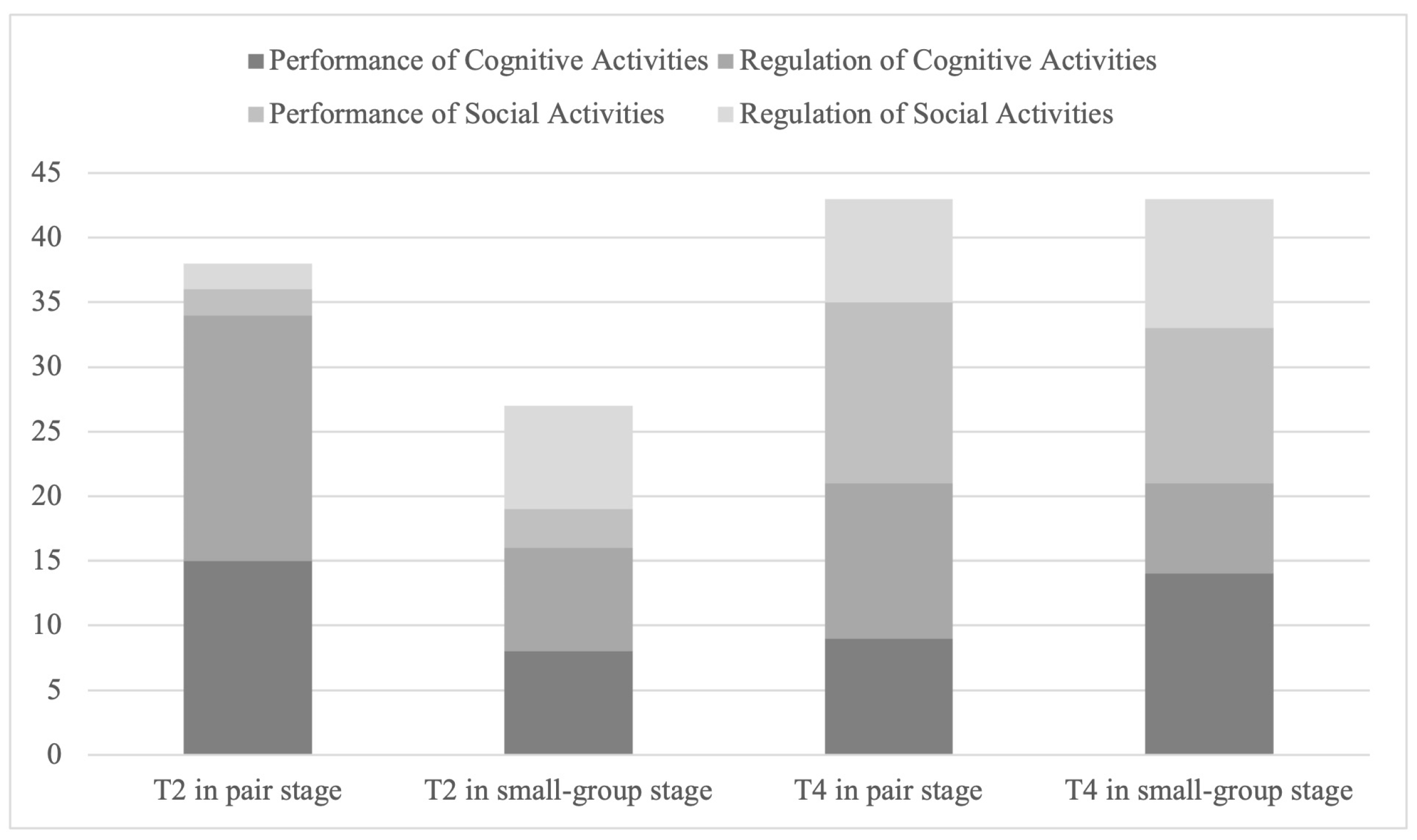
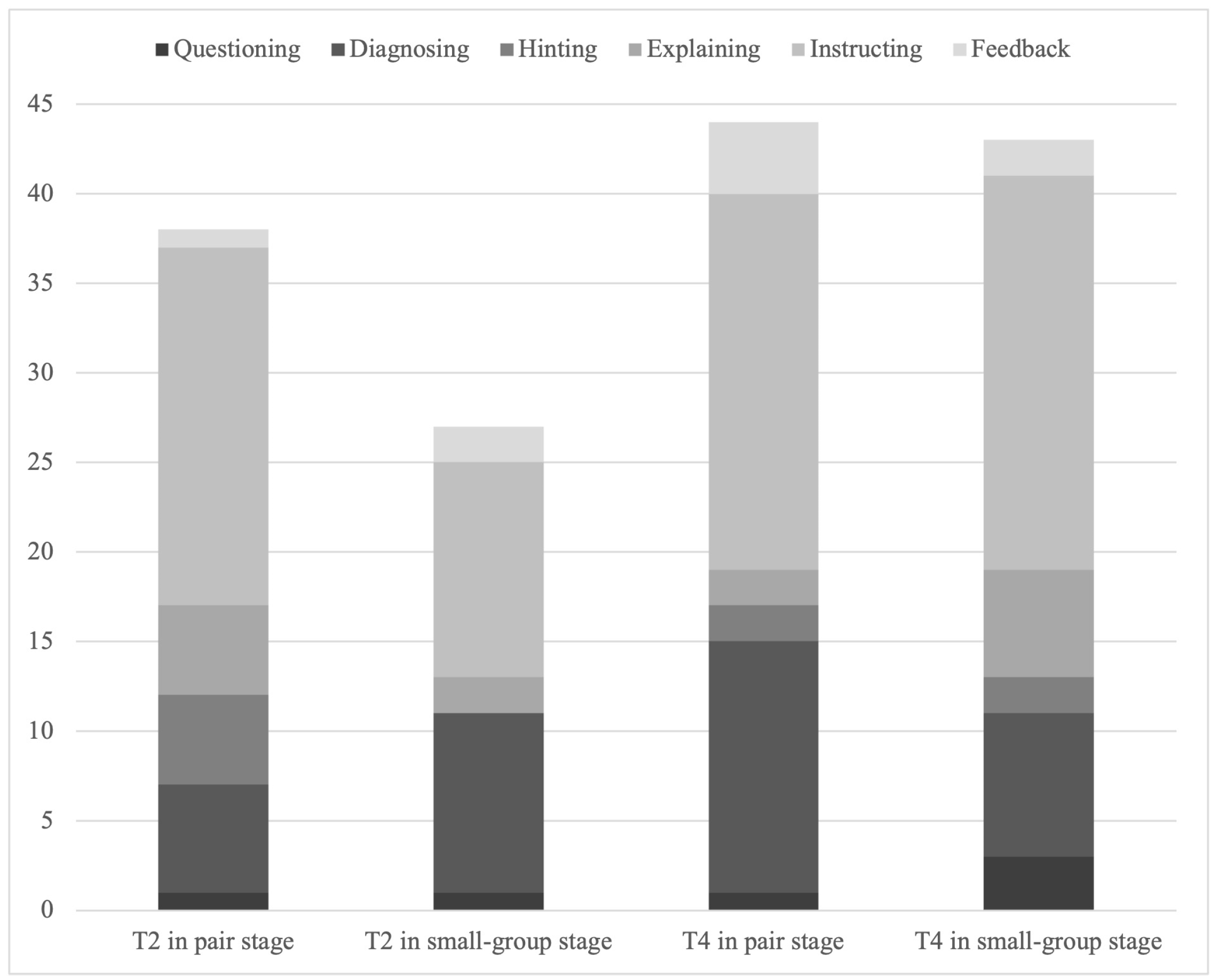
5.1. Social Activities and Diagnosing in Teacher Intervention
5.2. Intervention Style
6. Conclusions and Limitations
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Anitha, D., & Kavitha, D. (2023). Improving problem-solving skills through technology assisted collaborative learning in a first year engineering mathematics course. Interactive Technology and Smart Education, 20, 534–553. [Google Scholar] [CrossRef]
- Bellanca, J. A. (2010). 21st century skills: Rethinking how students learn. Solution Tree Press. [Google Scholar]
- Bender, W. N. (2012). Project-based learning: Differentiating instruction for the 21st century. Corwin Press. [Google Scholar]
- Bossert, S. (1988). Cooperative activities in the classroom. Review of Research in Education, 15, 225–250. [Google Scholar] [CrossRef]
- Bryan, C. A., Wang, T., Perry, B., Wong, N.-Y., & Cai, J. (2007). Comparison and contrast: Similarities and differences of teachers’ views of effective mathematics teaching and learning from four regions. ZDM—Mathematics Education, 39(4), 329–340. [Google Scholar] [CrossRef]
- Cao, Y., & He, C. (2009). Research of the type of teacher-student interaction behavior subject in junior middle school mathematics classroom on the LPS video date. Journal of Mathematics Education, 18(5), 38–41. (In Chinese). [Google Scholar]
- Chan, M. C. E., & Clarke, D. (2017). Structured affordances in the use of open-ended tasks to facilitate collaborative problem solving. ZDM—Mathematics Education, 49(6), 951–963. [Google Scholar] [CrossRef]
- Chan, M. C. E., Clarke, D., & Cao, Y. (2018). The social essentials of learning: An experimental investigation of collaborative problem solving and knowledge construction in mathematics classrooms in Australia and China. Mathematics Education Research Journal, 30, 39–50. [Google Scholar] [CrossRef]
- Chen, J., Wang, M., Kirschner, P. A., & Tsai, C.-C. (2018). The role of collaboration, computer use, learning environments, and supporting strategies in CSCL: A meta-analysis. Review of Educational Research, 88(6), 799–843. [Google Scholar] [CrossRef]
- Clarke, D. (1996). Assessment. In A. J. Bishop, K. Clements, C. Keitel, J. Kilpatrick, & C. Laborde (Eds.), International handbook of mathematics education (Vol. 4, pp. 327–370). Springer. [Google Scholar] [CrossRef]
- Clarke, D., & Sullivan, P. (1990). Is a question the best answer? Australian Mathematics Teacher, 46(3), 30–33. [Google Scholar]
- Cobb, P. (1995). Mathematical learning and small-group interaction: Four case studies. In The emergence of mathematical meaning: Interaction in classroom cultures (pp. 25–129). Routledge. [Google Scholar]
- Common Core State Standards Initiative. (2022). Common core state standards for mathematics. Available online: https://www.nctm.org/uploadedFiles/Standards_and_Positions/Common_Core_State_Standards/Math_Standards.pdf (accessed on 2 January 2025).
- Davidson, N. (1990). Cooperative learning in mathematics: A handbook for teachers. Addison-Wesley Publishing Company, Inc. [Google Scholar]
- Department of Education. (2022). National curriculum in England: Mathematics programmes of study. Available online: https://www.gov.uk/government/publications/national-curriculum-in-england-mathematics-programmes-of-study/national-curriculum-in-england-mathematics-programmes-of-study (accessed on 2 January 2025).
- Ding, M., Li, X., Piccolo, D., & Kulm, G. (2007). Teacher interventions in cooperative-learning mathematics classes. The Journal of Educational Research, 100(3), 162–175. [Google Scholar] [CrossRef]
- Dong, L., & Cao, Y. (2013). Teacher Interventions as an analytical entry point to study cooperative learning in middle school mathematics classrooms in China. Journal of Mathematics Education, 6(2), 1–16. [Google Scholar]
- Engeness, I., & Edwards, A. (2017). The complexity of learning: Exploring the interplay of different mediational means in group learning with digital tools. Scandinavian Journal of Educational Research, 61(6), 650–667. [Google Scholar] [CrossRef]
- Griffin, P., & Care, E. (2015). The ATC21S method. In P. Griffin, & E. Care (Eds.), Assessment and teaching of 21st century skills: Methods and approach (pp. 3–33). Springer. [Google Scholar] [CrossRef]
- Haataja, E., Moreno-Esteva, E. G., Salonen, V., Laine, A., & Hannula, M. S. (2019). Teacher’s visual attention when scaffolding collaborative mathematical problem solving. Teaching and Teacher Education, 86, 102877. [Google Scholar] [CrossRef]
- Heinonen, P., Karvonen, U., & Tainio, L. (2020). Hand-on-shoulder touch as a resource for constructing a pedagogically relevant participation framework. Linguistics and Education, 56, 100795. [Google Scholar] [CrossRef]
- Hogan, K., Nastasi, B. K., & Pressley, M. (1999). Discourse patterns and collaborative scientific reasoning in peer and teacher-guided discussions. Cognition and Instruction, 17(4), 379–432. [Google Scholar] [CrossRef]
- Huang, L., Doorman, M., & van Joolingen, W. (2021). Inquiry-based learning practices in lower-secondary mathematics education reported by students from China and The Netherlands. International Journal of Science and Mathematics Education, 19(7), 1505–1521. [Google Scholar] [CrossRef]
- Ing, M., Webb, N. M., Franke, M. L., Turrou, A. C., Wong, J., Shin, N., & Fernandez, C. H. (2015). Student participation in elementary mathematics classrooms: The missing link between teacher practices and student achievement? Educational Studies in Mathematics, 90(3), 341–356. [Google Scholar] [CrossRef]
- Janssen, J., Erkens, G., Kirschner, P. A., & Kanselaar, G. (2012). Task-related and social regulation during online collaborative learning. Metacognition and Learning, 7(1), 25–43. [Google Scholar] [CrossRef]
- Kaendler, C., Wiedmann, M., Rummel, N., & Spada, H. (2015). Teacher competencies for the implementation of collaborative learning in the classroom: A framework and research review. Educational Psychology Review, 27(3), 505–536. [Google Scholar] [CrossRef]
- Kosyvas, G. (2016). Levels of arithmetic reasoning in solving an open-ended problem. International Journal of Mathematical Education in Science and Technology, 47(3), 356–372. [Google Scholar] [CrossRef]
- Kyndt, E., Raes, E., Lismont, B., Timmers, F., Cascallar, E., & Dochy, F. (2013). A meta-analysis of the effects of face-to-face cooperative learning. Do recent studies falsify or verify earlier findings? Educational Research Review, 10, 133–149. [Google Scholar] [CrossRef]
- Lan, X., Ponitz, C. C., Miller, K. F., Li, S., Cortina, K., Perry, M., & Fang, G. (2009). Keeping their attention: Classroom practices associated with behavioral engagement in first grade mathematics classes in China and the United States. Early Childhood Research Quarterly, 24(2), 198–211. [Google Scholar] [CrossRef]
- Le, H., Janssen, J., & Wubbels, T. (2018). Collaborative learning practices: Teacher and student perceived obstacles to effective student collaboration. Cambridge Journal of Education, 48(1), 103–122. [Google Scholar] [CrossRef]
- Leung, F. K. S. (2001). In search of an east Asian identity in mathematics education. Educational Studies in Mathematics, 47(1), 35–51. [Google Scholar] [CrossRef]
- Li, D., Ding, M., & Cai, J. (2022). Teacher learning about collaborative learning: A case study. ZDM—Mathematics Education, 54(3), 721–735. [Google Scholar] [CrossRef]
- Liljedahl, P., & Cai, J. (2021). Empirical research on problem solving and problem posing: A look at the state of the art. ZDM—Mathematics Education, 53(4), 723–735. [Google Scholar] [CrossRef]
- Ministry of Education of the People’s Republic of China. (2011). Compulsory education mathematics curriculum standards (2011 ed.). Beijing Normal University Publishing Group. [Google Scholar]
- Ministry of Education of the People’s Republic of China. (2022). Compulsory education mathematics curriculum standards (2022 ed.). Beijing Normal University Publishing Group. [Google Scholar]
- MOE. (2021). Mathematics syllabus primary one to six. Available online: https://www.moe.gov.sg/-/media/files/primary/2021-primary-mathematics-syllabus-p1-to-p6.pdf (accessed on 2 January 2025).
- Molenaar, I., Chiu, M. M., Sleegers, P., & van Boxtel, C. (2011). Scaffolding of small groups’ metacognitive activities with an avatar. International Journal of Computer-Supported Collaborative Learning, 6(4), 601–624. [Google Scholar] [CrossRef]
- Ng, O.-L., & Cui, Z. (2021). Examining primary students’ mathematical problem-solving in a programming context: Towards computationally enhanced mathematics education. ZDM—Mathematics Education, 53(4), 847–860. [Google Scholar] [CrossRef]
- Nieminen, J. H., Chan, M. C. E., & Clarke, D. (2022). What affordances do open-ended real-life tasks offer for sharing student agency in collaborative problem-solving? Educational Studies in Mathematics, 109(1), 115–136. [Google Scholar] [CrossRef]
- OECD. (2017a). PISA 2015 assessment and analytical framework: Science, reading, mathematic, financial literacy and collaborative problem solving. OECD. [Google Scholar] [CrossRef]
- OECD. (2017b). PISA 2015 results (volume V): Collaborative problem solving. OECD. [Google Scholar] [CrossRef]
- Pijls, M., & Dekker, R. (2011). Students discussing their mathematical ideas: The role of the teacher. Mathematics Education Research Journal, 23(4), 379–396. [Google Scholar] [CrossRef]
- Ros, A. (1993). Peer work groups in Dutch classroom practice. American Educational Research Association. Available online: https://files.eric.ed.gov/fulltext/ED361870.pdf (accessed on 2 January 2025).
- Saadati, F., & Felmer, P. (2021). Assessing impact of a teacher professional development program on student problem-solving performance. ZDM—Mathematics Education, 53(4), 799–816. [Google Scholar] [CrossRef]
- Salmon, G. (2000). E-moderating: The key to teaching and learning online. Routledge. [Google Scholar]
- Saqr, M., Nouri, J., & Jormanainen, I. (2019). A Learning analytics study of the effect of group size on social dynamics and performance in online collaborative learning. In M. Scheffel, J. Broisin, V. Pammer-Schindler, A. Ioannou, & J. Schneider (Eds.), Transforming learning with meaningful technologies (pp. 466–479). Springer International Publishing. [Google Scholar] [CrossRef]
- Schindler, M., & Bakker, A. (2020). Affective field during collaborative problem posing and problem solving: A case study. Educational Studies in Mathematics, 105(3), 303–324. [Google Scholar] [CrossRef]
- Sekiguchi, Y. (2021). Activity systems analysis of classroom teaching and learning of mathematics: A case study of Japanese secondary schools. Educational Studies in Mathematics, 108(3), 493–511. [Google Scholar] [CrossRef]
- Shi, N., Wang, S., & General Senior Secondary Mathematics Curriculum Standards Revision Team. (2018). General senior secondary mathematics curriculum standards (2017 edition) explanation. Higher Education Press. (In Chinese) [Google Scholar]
- State Council of the People’s Republic of China. (2001, May 29). Decision of the state council on the reform and development of basic education. Available online: http://www.gov.cn/gongbao/content/2001/content_60920.htm (accessed on 2 January 2025). (In Chinese)
- Sullivan, P., Knott, L., & Yang, Y. (2015). The relationships between task design, anticipated pedagogies, and student learning. In Task design in mathematics education (pp. 83–114). New ICMI Study Series. Springer. [Google Scholar] [CrossRef]
- Tang, P., Liu, H., & Wen, H. (2021). Factors predicting collaborative problem solving: Based on the data from PISA 2015. Frontiers in Education, 6, 619450. [Google Scholar] [CrossRef]
- van Boxtel, C., van der Linden, J., & Kanselaar, G. (2000). The use of textbooks as a tool during collaborative physics learning. Journal of Experimental Education, 69(1), 57–76. [Google Scholar] [CrossRef]
- van de Pol, J., Volman, M., & Beishuizen, J. (2010). Scaffolding in teacher-student interaction: A decade of research. Educational Psychology Review, 22(3), 271–296. [Google Scholar] [CrossRef]
- van Leeuwen, A., & Janssen, J. (2019). A systematic review of teacher guidance during collaborative learning in primary and secondary education. Educational Research Review, 27, 71–89. [Google Scholar] [CrossRef]
- van Leeuwen, A., Janssen, J., Erkens, G., & Brekelmans, M. (2013). Teacher interventions in a synchronous, co-located CSCL setting: Analyzing focus, means, and temporality. Computers in Human Behavior, 29(4), 1377–1386. [Google Scholar] [CrossRef]
- Vermunt, J. D., & Verloop, N. (1999). Congruence and friction between learning and teaching. Learning and Instruction, 9(3), 257–280. [Google Scholar] [CrossRef]
- Wang, X., & Wang, T. (2022). The mutability of pedagogical practice and space use: A case study of collaborative learning and classroom space in a Chinese primary school. Compare: A Journal of Comparative and International Education, 52(5), 729–747. [Google Scholar] [CrossRef]
- Wang, X., & Wu, L. (2012). A study of changes in primary school mathematics classroom teaching in ten years of curriculum reform. China Educational Technology, 8, 111–114+128. (In Chinese). [Google Scholar]
- Webb, N. M. (2009). The teacher’s role in promoting collaborative dialogue in the classroom. British Journal of Educational Psychology, 79(1), 1–28. [Google Scholar] [CrossRef]
- Webb, N. M., Franke, M. L., Ing, M., Turrou, A. C., Johnson, N. C., & Zimmerman, J. (2019). Teacher practices that promote productive dialogue and learning in mathematics classrooms. International Journal of Educational Research, 97, 176–186. [Google Scholar] [CrossRef]
- Webb, N. M., Franke, M. L., Johnson, N. C., Ing, M., & Zimmerman, J. (2021). Learning through explaining and engaging with others’ mathematical ideas. Mathematical Thinking and Learning, 25(4), 438–464. [Google Scholar] [CrossRef]
- Webb, N. M., Nemer, K. M., & Zuniga, S. (2002). Short circuits or superconductors? Effects of group composition on high-achieving students’ science assessment performance. American Educational Research Journal, 39(4), 943–989. [Google Scholar] [CrossRef]
- Wilson, J. M., Straus, S. G., & McEvily, B. (2006). All in due time: The development of trust in computer-mediated and face-to-face teams. Organizational Behavior and Human Decision Processes, 99(1), 16–33. [Google Scholar] [CrossRef]
- Wu, L., Xia, M., & Xu, Y. (2020). Review and prospect of the research on the mathematics cooperative learning of Chinese primary and middle schools since the 21st century. Journal of Tianjin Academy of Educational Science, 6, 60–66. (In Chinese). [Google Scholar]
- Yager, S., Johnson, R., Johnson, D., & Snider, B. (1986). The impact of group processing on achievement in cooperative learning groups. Journal of Social Psychology, 126(3), 389–397. [Google Scholar] [CrossRef]
- Yang, Q.-F., Lin, C.-J., & Hwang, G.-J. (2021). Research focuses and findings of flipping mathematics classes: A review of journal publications based on the technology-enhanced learning model. Interactive Learning Environments, 29(6), 905–938. [Google Scholar] [CrossRef]
- Yang, X., König, J., & Kaiser, G. (2021). Growth of professional noticing of mathematics teachers: A comparative study of Chinese teachers noticing with different teaching experiences. ZDM—Mathematics Education, 53(1), 29–42. [Google Scholar] [CrossRef]
- Zhang, S., Cao, Y., Chan, M. C. E., & Wan, M. E. V. (2022). A comparison of meaning negotiation during collaborative problem solving in mathematics between students in China and Australia. ZDM—Mathematics Education, 54, 287–302. [Google Scholar] [CrossRef]
- Zheng, J. Q., Cheung, K. C., & Sit, P. S. (2023). The Effects of perceptions toward interpersonal relationships on collaborative problem-solving competence: Comparing four ethnic Chinese communities assessed in PISA 2015. The Asia-Pacific Education Researcher, 33, 481–493. [Google Scholar] [CrossRef]
| Teacher | Control Classes | Intervention Classes | Differences | |||||
|---|---|---|---|---|---|---|---|---|
| N1 | M1 | SD1 | N2 | M2 | SD2 | M2–M1 | p | |
| T1 | 34 | 83.55 | 16.54 | 34 | 84.06 | 13.08 | −0.51 | 0.888 |
| T2 | 32 | 79.75 | 16.75 | 33 | 81.56 | 13.43 | −1.81 | 0.635 |
| T3 | 40 | 82.95 | 14.32 | 39 | 81.84 | 13.51 | 1.11 | 0.727 |
| T4 | 39 | 79.14 | 16.68 | 41 | 77.65 | 19.28 | 1.49 | 0.720 |
| Focus | Descriptions | Examples |
|---|---|---|
| Performance of cognitive activities | Discourse about task content | “How do you calculate the size of the room?” |
| Regulation of cognitive activities | Discourse about planning of the task/time management Discourse about task strategies | “Read the problem again.” “Explain your answer.” |
| Performance of social activities | Discourse about the mood or other social activities within a group or the class | “Students should communicate well with each other.” |
| Regulation of social activities | Discourse about the collaboration process/about strategies for collaboration | “Distribute the tasks in the group.” |
| Means | Descriptions | Examples |
|---|---|---|
| Questioning | Request for a piece of information. | “How do you calculate the size of the room?” |
| Diagnosing | Questions to understand the current situation, without giving help. Asking what the problem is/about students’ understanding of the topic on hand. | “What is the question that you have not solved right now?” |
| Hinting | Giving a hint or a reminder without supplying the solution or detailed instructions. Students are still required to think for themselves. A hint can take the form of an instruction. | Student: “Is a balcony a room?” Teacher: “Is the area of the balcony counted as the area of your apartment?” |
| Explaining | Providing direct answers or information to elaborate on something, to make it clearer. After giving an explanation, the students(s) are able to continue their task immediately. | Student: “How big is a ten square meter bedroom?” Teacher: “It can probably fit a bed.” |
| Instructing | The teacher instructs students to do something. Recognisable mostly by the use of an imperative, but this is not necessary. | “Explain your answer.” |
| Feedback | Direct evaluation of the behaviour/work of the students. | “The state of your discussion is great.” |
| Other | Remaining utterances. Correcting a previous statement. | “That’s the last problem.” |
| Teacher | T1 | T2 | T3 | T4 | Total |
|---|---|---|---|---|---|
| Control Class | 3.94 (1.14) | 4.28 (0.76) | 4.20 (0.89) | 3.76 (1.29) | 4.04 (1.06) |
| Intervention Class | 4.61 (0.54) | 3.31 (1.83) | 4.50 (0.68) | 4.30 (0.75) | 4.21 (1.15) |
| Teacher | T1 | T2 | T3 | T4 | Total |
|---|---|---|---|---|---|
| Control Class | 2.81 (1.21) | 2.81 (1.60) | 3.11 (1.33) | 2.94 (1.12) | 2.93 (1.41) |
| Intervention Class | 3.69 (1.20) | 3.94 (1.37) | 3.22 (0.73) | 3.11 (1.41) | 3.47 (1.30) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Cao, Y. Teacher Intervention During Collaborative Problem Solving in Mathematics Classrooms in Mainland China. Behav. Sci. 2025, 15, 377. https://doi.org/10.3390/bs15030377
Liu Y, Cao Y. Teacher Intervention During Collaborative Problem Solving in Mathematics Classrooms in Mainland China. Behavioral Sciences. 2025; 15(3):377. https://doi.org/10.3390/bs15030377
Chicago/Turabian StyleLiu, Yixuan, and Yiming Cao. 2025. "Teacher Intervention During Collaborative Problem Solving in Mathematics Classrooms in Mainland China" Behavioral Sciences 15, no. 3: 377. https://doi.org/10.3390/bs15030377
APA StyleLiu, Y., & Cao, Y. (2025). Teacher Intervention During Collaborative Problem Solving in Mathematics Classrooms in Mainland China. Behavioral Sciences, 15(3), 377. https://doi.org/10.3390/bs15030377






