Next Article in Journal
Exposure to Terrorism, Post-Traumatic Stress Disorder and University Teachers’ Performance: Underpinning the Role of Social Support
Previous Article in Journal
Perceptions of Stakeholders Regarding China’s Special Education and Inclusive Education Legislation, Law, and Policy: Implications for Student Wellbeing and Mental Health
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Behavioral Sciences
Volume 13
Issue 6
10.3390/bs13060516
behavsci-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Brief Report

Latent Regression Analysis Considering Student, Teacher, and Parent Variables and Their Relationship with Academic Performance in Primary School Students in Chile

by
Sonia Salvo-Garrido
1,*,
José Zayas-Castro
2,
Karina Polanco-Levicán
3 and
José Luis Gálvez-Nieto
4
1
Departamento de Matemática y Estadística, Universidad de La Frontera, Temuco 4780000, Chile
2
Department of Industrial and Management Systems Engineering, University of South Florida, Tampa, FL 33612, USA
3
Programa de Doctorado en Ciencias Sociales, Universidad de La Frontera, Temuco 4780000, Chile
4
Departamento de Trabajo Social, Universidad de La Frontera, Temuco 4780000, Chile
*
Author to whom correspondence should be addressed.
Behav. Sci. 2023, 13(6), 516; https://doi.org/10.3390/bs13060516
Submission received: 26 April 2023 / Revised: 9 June 2023 / Accepted: 14 June 2023 / Published: 19 June 2023
(This article belongs to the Section Educational Psychology)
Browse Figure
Versions Notes

Abstract

:
Academic performance in primary students is fundamental to future school success; however, simultaneous analysis of different key individual, family, and teaching factors must be considered to improve understanding and benefit the development of students’ potential. This article presents a latent regression analysis model that examines the relationship between the latent variables (self-efficacy, interest in reading, bullying, parental expectations, and discrimination/exclusion, and teacher violence/aggression) and the academic performance of first-cycle primary students. The model investigates the impact of the latent variables on the standardized endogenous variables of SIMCE Mathematics and Language test scores using a quantitative, non-experimental, correlational, and cross-sectional design. The study involved 70,778 students (53.4% female), with an average age of 9.5 years (SD = 0.6), from Chilean public (33.6%) and subsidized (66.4%) schools. The results indicate that the model accounted for 49.8% and 47.7% of the mean variability in SIMCE Mathematics and Language test scores, respectively. The goodness-of-fit indices demonstrated satisfactory fits for both models. In both tests, student self-efficacy emerged as the most significant factor explaining test score variability, followed by parental expectations. Bullying was identified as a relevant factor in reducing mean performance on both tests. The findings suggest that education decision makers should address these issues to improve student outcomes.

1. Introduction

Several studies have demonstrated the link between students’ personal and social characteristics and academic performance, as noted in the standardized test scores in Chile and other Latin American countries [1,2,3,4,5]. This region has the highest segregation by socio-economic level [6], which affects students’ academic development. It also negatively impacts teachers and students’ families, linking with the families’ low expectations [6,7]. Specifically, Chile has a highly segregated education system [7], and with it, other conditions of psycho-social adversity are shown to be prevalent among students and have been the subject of different studies for decades, with the observation that they hinder learning and socialization, limiting students’ potential [6,8,9,10,11,12,13].
To understand the factors associated with academic performance in vulnerable populations, studies have shifted focus toward identifying individual and environmental variables related to academic success [14,15,16,17,18]. Understanding the factors that reinforce or hinder the educational development of vulnerable populations is crucial for creating equitable conditions and ensuring that all students have adequate opportunities for future success [19,20,21]. Protective and risk factors have been identified in socio-educational development processes that influence student performance and stability over time [22,23,24,25,26]. In addition, a consensus has been established regarding the reciprocal influence between individuals and the environment in these processes [16].
In this sense, self-efficacy (understood as the perception of self-efficacy) is considered an individual characteristic that reflects self-confidence in one’s cognitive capacities and internal attributes related to learning and performance [27]. According to Bandura [28], self-efficacy refers to the judgments a person makes about their own ability and their chances of achieving positive results by virtue of the proposed objectives. This influences their behavior, thoughts, and emotions, as well as their decisions regarding the effort they will invest in a particular task and persistence in the face of obstacles [28]. Self-efficacy has been studied in several fields, including mathematics, where it has been noted that self-efficacy is a significant factor in mathematics performance in arithmetic and problem-solving tasks for both students and teachers, being demonstrated in cross-sectional and longitudinal studies [29,30,31,32,33,34].
Studies have also identified other factors that contribute to satisfactory math performance, including gender, age, school resources, and socioeconomic level, as well as the student’s motivation for a certain activity [33,35,36,37,38]. Fisher et al. [39] studied the relevance of gender in math performance and found that the low number of women in science, technology, engineering, and mathematics (STEM) disciplines is mainly due to their lower degree of self-efficacy. Consequently, it is important to consider the gender construct when studying performance in mathematics, as it is a culturally stereotypical domain [40,41,42,43]. For example, Borgonovi and Pokropek [44] found higher means for boys than girls in the perception of self-efficacy in mathematics, suggesting the need to consider and control for the influence of the gender variable in the link between academic performance and self-efficacy [29].
Self-efficacy in language is understood as the students’ perceptions of their abilities and possibilities of achieving a suitable performance in this specific area [45], while interest in reading is defined as the level of pleasure or enjoyment taken from reading a text [46,47]. When established in early development, the perception of self-efficacy in language and interest in reading are considered important factors for coping with the difficulties that can arise at school [24,48,49]. In addition, the ability to read is also associated with attitudes toward autonomy, i.e., with undertaking activities in this area alone and asking for help only when required, as well as toward the use of information and communication technologies to reinforce these skills [50,51,52,53].
Bullying is defined as a behavior of intentional and repeated aggression toward a victim over time [54]. Traditional bullying and cyberbullying are linked to academic performance, acting as precursor and consequence [55,56]. Moreover, bullying can affect the adjustment capacities and social efficacy of victims [17,57,58,59]. In the case of cyberbullying, a sense of self-efficacy is crucial to developing defensive behaviors against cyber-attacks [60,61]. Finally, it is worth noting that teachers sometimes observe bullying and aggression between students, which negatively affect the teaching–learning process, social relations generated in the class, and academic performance [62].
On the other hand, the link between family and school is also relevant to expectation development [63,64], with a sense of commitment to family obligations increasing students’ motivation to study [65,66] and parental and community emotional support reinforcing the academic capacities of children with difficulties in the socio-affective domain [67,68,69]. In addition, parental involvement, particularly from the mother, and parental expectations can reduce the negative and stressful effects of adverse socio-affective and contextual conditions [70,71], which are predictors of school performance, along with a positive school climate and resilient teachers [72,73].
Gutiérrez et al. [74] found that permanent family and teacher support contribute to school involvement and the satisfaction of youths. Ansong et al. [75] emphasized the importance of encouraging students regarding academic expectations and a prospective view of life for satisfactory school performance. Randall and Burley [76] suggest that teachers’ self-efficacy beliefs influence their commitment to their profession, impacting their contribution to students’ academic processes. Bonneville-Roussy et al. [77] suggest that cultural differences affect the extent of teachers’ self-efficacy development on youths’ self-efficacy in teaching practices. Miller et al. [78] indicate that teachers’ sense of self-efficacy is associated with the competence perceived by the students and the respect perceived by the teachers. Theron and Theron [79] argue that good academic performance requires an active connection between the teacher, family, community, and the student’s ability to respond.
The preceding provides evidence of the relevance of considering factors from various domains (personal, teachers, and family) to analyze the relationship with primary students’ academic performance. This is based on previously conducted studies [24,80] that demonstrate, for example, the importance of the variable self-efficacy and its connection to academic performance. In addition, it is considered that standardized mathematics and language tests assess knowledge and skills required in other areas of learning [24,80]. Hence, this study uses a latent regression model to explore the association between variables related to the student (self-efficacy in mathematics, self-efficacy in language, and interest in reading), teachers (Violence/Aggression), and parents (Parents’ expectations, Discrimination/Exclusion) to academic performance on the SIMCE tests in mathematics and language of students in the first cycle of public and private-subsidized primary schools in contexts of significant family and social vulnerability in Chile. Therefore, the following research question is posited: What are the relations between the latent variables of students, teachers, families, and the academic performance of first-cycle primary students in Chile?

2. Materials and Methods

2.1. Participants

A total of 70,708 fourth-grade primary students (46.6% male and 53.4% female) in Chile who took the SIMCE tests in 2015 were considered in this study, representing 46% of the population. As a criterion of student inclusion, it was believed that there were no missing values in the analysis variables. Regarding place of residence, 90.3% of the students reported living in urban settings (9.7% rural). Considering the type of school, 23,780 (33.6%) children belonged to public schools, and the remaining (66.4%) to private-subsidized schools. The mean age of the sample was 9.5 (SD = 0.6), with a minimum of 9 and a maximum of 10 years old.

2.2. Instruments

The census SIMCE tests evaluate the learning of all students in Chile, in relation to the current national curriculum, in different subjects and school levels, to contribute to improving the quality and equity of education. In addition, it collects information from students, teachers, and parents/guardians, through context questionnaires about Chilean education [81].

2.3. Data

The database of the SIMCE mathematics and language test results of fourth-grade primary students from 2015 and the context questionnaires provided by the Chilean Education Quality Assurance Agency were used under strict confidentiality. For more information, see https://www.agenciaeducacion.cl/ (accessed on 10 March 2022).

2.4. Procedure

The processes for applying the instruments (tests and context questionnaires) were carried out according to instructions, manuals, and action protocols based on the international standardization criteria defined in the document Standards for Educational and Psychological Testing [82]. Such documents are intended to provide equivalent conditions for all students when taking the test, while ensuring confidentiality of the information and safeguarding the materials applied [81].
With respect to the application of contextual tests and questionnaires, the examiner reviews the material received by the Education Quality Assurance Agency and reports possible inconsistencies. Next, the classroom is prepared based on the seating arrangement and booklet allocation criteria established in the Application Manual. Then, the students are instructed to enter the classroom, attendance is taken, and the general instructions for each test, contained on the cover of booklets, are read aloud. These instructions are key to the students being familiar with the general conditions before taking the test. Once the test is finished, the material is carefully collected, packaged, and the box containing the test is sealed for dispatch to the corresponding collection center [81].

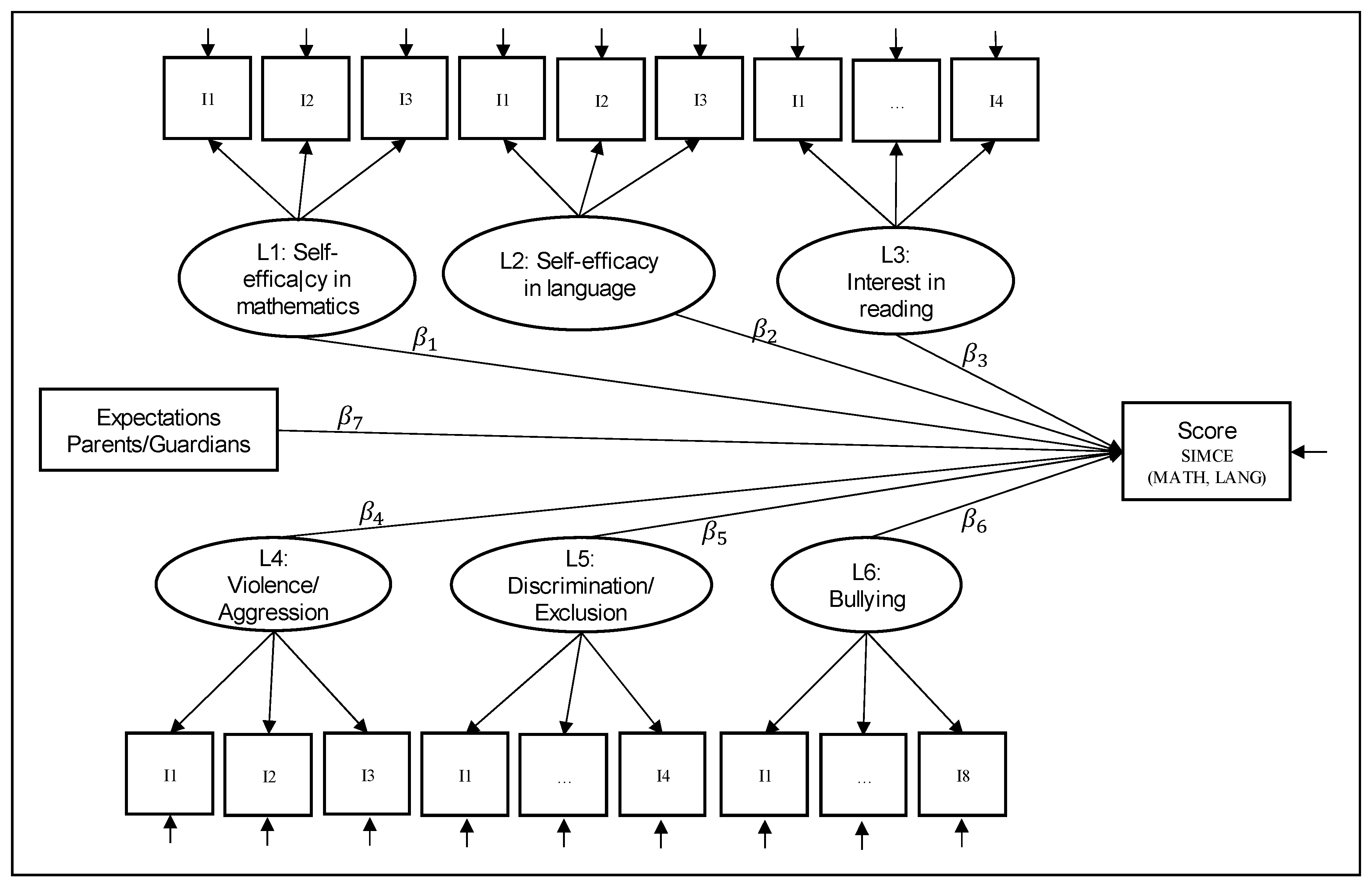
2.5. Method

This is a quantitative, non-experimental, correlational, and cross-sectional study [83]. A latent regression analysis [84] was carried out considering covariates, where the endogenous variables were the SIMCE Mathematics and Language test scores, with seven variables exogenous. Six latent variables comprised 24 items measured with an ordinal scale of four categories (1 = Never/Very untrue, 2 = Few times/Untrue, 3 = Many times/True, 4 = Always/Very true). They were distributed as follows: L1: Interest in reading, made up of four items; L2: Bullying, made up of eight items; L3: Self-efficacy in mathematics, made up of three items; L4: Self-efficacy in reading, made up of three items; L5: Violence perceived by parents, made up of four items; L6: School discrimination perceived by teachers, made up of four items; and standardized parental educational expectations, corresponding to the perception of the highest level of education that the student would be able to complete in the future, and the covariates included were gender and type of school. SEMs (Structural Equation Model) with latent variables allow us to specify regression analyses at the level of latent variables (factors) that are corrected for measurement error. This procedure has the advantage that error of measurement can be taken into account explicitly for both the independent and the dependent variable(s). Furthermore, SEMs with latent variables allow us to estimate the reliabilities of the manifest variables. The explicit consideration of measurement error leads to a more precise estimation of the parameters of the regression model compared to manifest regression analyses with observed variables that are not adjusted for measurement error. Multiple indicators are required for each latent variable in a latent regression model (LRM) [84].
To estimate the models, the polychoric correlation matrix [85] was used along with the method of weighted least squares means and variance adjusted (WLSMV) [86]. The following goodness-of-fit indicators were used to assess the quality of the models: comparative fit index (CFI), Tucker–Lewis index (TLI), root mean square error of approximation (RMSEA), and standardized root mean square residual (SRMR). An SRMR value of <0.08 is considered a good fit [87]; a CFI and TLI value of ≥0.90 is regarded as a reasonable fit and ≥0.95, an excellent fit [88]; and an RMSEA value of < 0.08 is considered a reasonable fit [87], and ≤0.06, an excellent fit [89]. The reliability of each latent variable was determined using the McDonald’s Omega coefficient [90], which must be between 0.70 and 0.90 to be acceptable [91].
Figure 1 shows the LRM to be estimated. All analyses were performed with the Mplus program, version 8.3 [92].

3. Results

Table 1 presents the descriptive statistics, mean and standard deviation, of the standardized variable scores by school type and gender. The means of the standardized scores of the endogenous variables are below the mean of the overall standardized score in public schools, regardless of gender. However, in private-subsidized schools, these values are above the mean. For example, boys have a higher mean in math self-efficacy (L1) than girls. On the other hand, in language self-efficacy (L2), only girls from private-subsidized schools are above the overall mean; boys from school public have the highest mean in bullying. On the other hand, parents/guardians at private-subsidized schools have above-mean academic expectations for their children.
Two LRMs were adjusted for the endogenous variables SIMCE mathematics (M1) and language (M2) scores. For M1, the goodness-of-fit indices had the following values: WLSMV-χ2 (304) = 70,933.2, p < 0.001; CFI = 0.937; TLI = 0.928; RMSEA = 0.057 (90%C.I. = 0.057, 0.058); SRMR = 0.068. These values indicate that the proposed M1 model fits the data reasonably. R2 = 0.498, 49.8% of the variability of the SIMCE mathematics score is explained by the exogenous variables. For M2, the goodness-of-fit indices had the following values: WLSMV-χ2 (304) = 71,542.4, p < 0.001; CFI = 0.937; TLI = 0.927; RMSEA = 0.058 (90%C.I. = 0.057, 0.058); SRMR = 0.069. These values indicate that the proposed M2 model fits the data reasonably. R2 = 0.477, 47.7% of the variability of the SIMCE language score is explained by the exogenous variables. Both R2 values are greater than 0.3. In social and behavioral science applications, the R2 value is usually not higher than 0.3 because relevant exogenous variables that are difficult to measure may not be included in the model [93]. These results show the relevance of considering, on the one hand, a latent regression analysis, and on the other, considering the variables gender and type of school as covariates.
Table 2 shows the standardized factor loadings, all greater than 0.64. They are therefore considered adequate to define the latent factor. For the latent variable self-efficacy in mathematics, the omega coefficient was 0.85; self-efficacy in language was 0.74; interest in reading was 0.84; violence/aggression was 0.87; discrimination/exclusion was 0.89; and bullying was 0.92. These reliability values are considered acceptable for all factors.
Table 3 shows the values of the standardized estimated coefficients of the LRM for M1 and M2. All the estimated coefficients were statistically significant, p < 0.001. For M1, the most relevant variables that explain a positive change in the SIMCE score were self-efficacy in mathematics, self-efficacy in language, expectations (parents/guardians), and bullying for a negative change. For M2, they were self-efficacy in language, expectations (parents/guardians), and bullying, respectively.

4. Discussion

The results reveal latent factors that tend to configure a cross section of variables significantly influencing academic performance. To achieve this, a latent regression analysis was adjusted to the measurement scale of the items and their interrelationships with excellent indicators of fit. For M1, self-efficacy in mathematics was the most relevant latent factor to explain the mean variability of the SIMCE mathematics test scores, followed by self-efficacy in language and parent/guardian expectations. By contrast, bullying and parent/guardian discrimination/exclusion were the most relevant factors to decrease, in the mean, this performance. For M2, self-efficacy in Language was the most relevant latent factor to explain the mean variability of the SIMCE language test scores, followed by parents’/guardians’ expectations and self-efficacy in mathematics. On the contrary, bullying and parent/guardian discrimination/exclusion were the most relevant to decrease, in the mean, this performance. These results present an important degree of theoretical consistency with previous studies in terms of the prevalence of the self-efficacy factor in mathematics [25,29,30,31,33,34,37,57], and indicate that this internal attribute is associated with the learning processes responsible for satisfactory academic performance. In the field of language, the self-efficacy factor proves to be a relevant variable related to the development of language skills and interest in reading [24,53,94]. In this sense, the predominance of the level of self-efficacy as a protective factor of the student body is consistent with Salvo-Garrido et al. [27] and Anghel [26], who emphasize the need to develop or reinforce students’ confidence in their own cognitive skills, which will allow them to overcome the obstacles that arise at school and in their life in general. Similarly, the relationship established by Pitzer and Skinner [95] between self-efficacy and self-appreciation as factors that influence mathematics performance is interesting.
In the same vein, the literature tends to conceive the perception of self-efficacy as an individual internal characteristic, so it could be argued that confidence in one’s abilities is significantly associated with socio-educational and affective relationships, which the student has or does not have the chance to develop [96]. Semanchin et al. [96] and Salvo-Garrido et al. [26] refer to the significant emotional support offered to the student by adults or professional figures as benefitting their education outcomes. This would increase the capacity for self-regulation and self-directed learning, factors that have been linked to the variable of self-efficacy [97,98]. In this sense, the emergence of expectations also theoretically refers to a positive socio-affective context anchored in the family [71] and teaching dimensions [32]. According to several studies, this factor (expectations) contributes to youths’ increased motivation for study, greater involvement and school satisfaction, and the development of a prospective view of life. These are all socio-affective processes that can have a significant impact on academic performance [17,24,26,68,74,75].
However, latent factors that allude to bullying, discrimination, exclusion, and the deployment of aggressive behaviors, whether they are perpetrated in person or virtually [60], also constitute risk variables that need to be addressed in the development of educational strategies, reinforcing the sense of self-efficacy [61]. Specifically, bullying is recognized as a major problem that affects children’s academic performance all over the world, impacting their academic commitment and class attendance [56]. Moreover, while students who are spectators of cyberbullying and traditional bullying are key to reducing the aggressions and consequences for the victim, their actions will depend on how confident they feel about their ability to stop the aggressions on a social and emotional level [61]. Generally, aggressions experienced and observed as well as the difficulties schools have in managing good interpersonal relations affect the coexistence of the members of the education community and, therefore, academic performance [55]. The relevance that students involved in bullying (online and offline) receive social support from teachers and other students, offering them prompt attention, is highlighted, adding that preventive actions are carried out constantly, promoting suitable behavioral adjustment and appropriate interpersonal relationships [55]. In addition, regulations in the schools need to be effective in protecting children from aggression phenomena, while at the same time applying laws and policies [56] that favor the optimal development of the students in a safe environment [17,26,57,58,59].
Concerning the findings linked to the perception of self-efficacy and gender differences, it may be stated that the highest mean in self-efficacy in mathematics observed in boys compared to girls could condition students’ future academic choices, clarifying that there are a small number of women in the areas of science, technology, engineering, and mathematics [39]. This could be related to gender stereotypes associated with the mathematics domain, which would culturally be associated with the skills of boys and, to a lesser degree, girls [41]. In this sense, cultural gender stereotypes are relevant to consider, since they may be at the basis of the higher means obtained by boys compared to girls in terms of their mathematics self-concept [44]. Taking into account the socioeconomic segregation that Chile and Latin America generally experience [6,7], which has a detrimental impact on academic performance on standardized tests [1,2,3,4,5], the situation becomes more complex, preventing boys, and especially girls, from reaching their full potential and lowering family expectations [6,8,9,10,11,12,13]. Consequently, it is important to make families and teachers aware of the issue so they can offer support and positive reinforcement to change this situation. In addition, it is suggested that this variable should be controlled in studies on academic performance and the development of school self-efficacy [29].
The latent factors indicated concur in academic processes as conceptual devices, but also as strategic, when developing programs that support the vulnerable student population. Thus, emphasis is placed on the need for socio-educational and affective convergence between the student, parental and educational resources, and institutional devices. Moreover, it is crucial to create a school and family climate free from violence and disqualification, with a consistent normative dimension, which can strengthen the relationship between self-efficacy and expectations while reinforcing academic provisions and the positive development of a prospective view of life in each boy and girl who must continually face the vicissitudes of adversity. The evidence suggests that education decision makers should include efforts to address this issue in the education community.
Finally, future lines of research are proposed to explore the longitudinal relationships among the constructs addressed in this study. In addition, it would be relevant to include the analysis of school climate in light of its association with several variables, such as cyberbullying and academic performance, among others. As for the limitations of this study, it is important to emphasize that the results obtained are cross-sectional, which provides limited evidence in terms of temporality and causality. Moreover, it is worth noting that the context questionnaires comprising the SIMCE are based on self-reported responses, which implies that the results reflect the participants’ perceptions and have not been contrasted with other data sources.

Author Contributions

Conceptualization, S.S.-G. and K.P.-L.; methodology, J.Z.-C., S.S.-G. and J.L.G.-N.; validation, J.Z.-C., S.S.-G. and J.L.G.-N.; formal analysis, J.Z.-C., S.S.-G. and J.L.G.-N.; investigation, J.Z.-C. and S.S.-G.; resources, S.S.-G.; data curation, J.Z.-C. and S.S.-G.; writing—original draft preparation, S.S.-G., J.Z.-C. and K.P.-L.; writing—review and editing, S.S.-G., K.P.-L. and J.L.G.-N.; visualization, S.S.-G. and K.P.-L.; supervision, S.S.-G.; project administration, S.S.-G.; funding acquisition, S.S.-G. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by Agencia Nacional de Investigación y Desarrollo: FONDECYT Projects 1160429 and 1210551.

Institutional Review Board Statement

The study was conducted in accordance with the Declaration of Helsinki, and approved by the Ethics Committee of Universidad de La Frontera (protocol code 007/16, approval date 11 May 2016).

Informed Consent Statement

Informed consent was obtained from all subjects involved in the study.

Data Availability Statement

The data presented in this study are available on request from the Ministry of Education of Chile.

Acknowledgments

The authors wish to thank the Chilean Ministry of Education for providing access to the corresponding database.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Aliaga, R.M. ¿Puede La Escuela Corregir Inequidades Sociales? Aportes Para Una Comprensión Amplia Del Fenómeno De La Desigualdad. Rev. Enfoques Educ. 2022, 19, 1–25. [Google Scholar] [CrossRef]
  2. Mullis, I.; Martin, M.; Foy, P.; Arora, A. Timss 2011 International Results in Mathematics; TIMSS & PIRLS International Study Center, Lynch School of Education, Boston College: Chestnut Hill, MA, USA, 2012; Volume 43, ISBN 9781889938639. [Google Scholar]
  3. Murillo, F.J. Measuring the School Segregation in Latin America. A Methodological Analysis Using TERCE. REICE Rev. Iberoam. Sobre Calid. Efic. Cambio Educ. 2016, 14, 33–60. [Google Scholar] [CrossRef] [Green Version]
  4. OCDE. ¿Cómo Algunos Estudiantes Superan Su Entorno Socioeconómico de Origen? Pisa Focus 2011, 5, 1–4. Available online: https://www.educacionyfp.gob.es/inee/publicaciones/publicaciones-periodicas/pisa-in-focus/2011.html (accessed on 15 August 2021).
  5. Vera-Bachmann, D.; Osses, S.; Schiefelbein, E. Las Creencias de Los Profesores Rurales: Una Tarea Pendiente Para La Investigación Educativa. Estud. Pedagog. 2012, 38, 311–324. [Google Scholar] [CrossRef]
  6. Murillo, F.J.; Martínez-Garrido, C.; Graña, R. Segregación Escolar Por Nivel Socioeconómico En Educación Primaria En América Latina y El Caribe. REICE. Rev. Iberoam. Sobre Calid. Efic. Cambio Educ. 2023, 21, 87–117. [Google Scholar] [CrossRef]
  7. Murillo, F.J.; Martínez-Garrido, C. La Ségrégation Sociale Dans Les Écoles Publiques et Privées En Amérique Latine. Educ. Soc. 2017, 38, 727–750. [Google Scholar] [CrossRef] [Green Version]
  8. Atria, F. La Mala Educación. Ideas Que Inspiran al Movimiento Estudiantil en Chile; Primera: Santiago, Chile, 2012; ISBN 978-956-324-114-3. [Google Scholar]
  9. Espinoza, V.; Santa Cruz, C.; Rosas, R. Developmental Trajectories of Written Language Precursors According to Socioeconomic Status. Read. Writ. Q. 2021. [Google Scholar] [CrossRef]
  10. Murillo, F.J.; Duk, C. Evolución de La Segregación Socioeconómica de Las Escuelas de América Latina. Estud. Pedagógicos 2018, 157–179. [Google Scholar] [CrossRef] [Green Version]
  11. Núñez, J.; Miranda, L. La Movilidad Intergeneracional Del Ingreso y La Educación En Chile. In El Arte de Clasificar a Los Chilenos. Enfoques Sobre los Modelos de Estratificación en Chile; Joignant, A., Güell, P., Eds.; Ediciones Universidad Diego Portales: Santiago, Chile, 2009; p. 153. ISBN 978-956-314-070-5. [Google Scholar]
  12. Ponce Mancilla, T.; Vielma Hurtado, C.; Bellei Carvacho, C. Experiencias Educativas de Niñas, Niños y Adolescentes Chilenos Confinados Por La Pandemia COVID-19. Rev. Iberoam. Educ. 2021, 86, 97–115. [Google Scholar] [CrossRef]
  13. Vargas-Diaza, C.; Matus-Correa, C. Persistent Mathematical Gender Gap in Chilean National Assessment Simce. Estud. Pedagog. 2022, 48, 389–400. [Google Scholar] [CrossRef]
  14. Dueñas, X.; Godoy, S.; Duarte, J.; López, D. Resilience in the Educational Achievement of Colombian Students. Rev. Colomb. Educ. 2019, 69–90. [Google Scholar] [CrossRef]
  15. Garagiola, E.R.; Lam, Q.; Wachsmuth, L.S.; Tan, T.Y.; Ghali, S.; Asafo, S.; Swarna, M. Behavioral Sciences Adolescent Resilience during the COVID-19 Pandemic: A Review of the Impact of the Pandemic on Developmental Milestones. Behav. Sci. 2022, 12, 2–24. [Google Scholar] [CrossRef] [PubMed]
  16. Paulus, L.; Spinath, F.M.; Hahn, E. How Do Educational Inequalities Develop? The Role of Socioeconomic Status, Cognitive Ability, Home Environment, and Self-Efficacy along the Educational Path. Intelligence 2021, 86, 101528. [Google Scholar] [CrossRef]
  17. Sandoval-Hernández, A.; Białowolski, P. Factors and Conditions Promoting Academic Resilience: A TIMSS-Based Analysis of Five Asian Education Systems. Asia Pac. Educ. Rev. 2016, 17, 511–520. [Google Scholar] [CrossRef] [Green Version]
  18. Suárez Cretton, X.; Castro Méndez, N. Socio-Emotional Competences and Resilience of Students from Vulnerable Schools and Their Relationship with Academic Performance. Rev. Psicol. 2022, 40, 879–904. [Google Scholar] [CrossRef]
  19. Diaz-Vargas, C.; Tapia-Figueroa, A.; Valdebenito-Villalobos, J.; Gutiérrez-Echavarria, M.A.; Acuña-Zuñiga, C.C.; Parra, J.; Arias, A.M.; Castro-Durán, L.; Chávez-Castillo, Y.; Cristi-Montero, C.; et al. Academic Performance According to School Coexistence Indices in Students from Public Schools in the South of Chile. Behav. Sci. 2023, 13, 154. [Google Scholar] [CrossRef]
  20. Martín-Lagos López. Education and Inequality: A Meta-Synthesis after the 50th Anniversary of Coleman’s Report. Rev. Educ. 2018, 380, 186–209. [Google Scholar] [CrossRef]
  21. Rodríguez-Garcés, C.; Padilla-Fuentes, G.; Suazo-Ruíz, C. Etnia Mapuche y Vulnerabilidad: Una Mirada Desde Los Indicadores de Carencialidad Socioeducativa. Rev. Encuentros 2020, 18, 84–92. [Google Scholar] [CrossRef]
  22. Bravo, M.; Salvo-Garrido, S.; Muñoz, C. Profiles of Chilean Students According to Academic Performance in Mathematics: An Exploratory Study Using Classification Trees and Random Forests. Stud. Educ. Eval. 2015, 44, 50–59. [Google Scholar] [CrossRef]
  23. Gomez, G.; Valenzuela, J.P.; Sotomayor, C. Against All Odds: Outstanding Reading Performance among Chilean Youth in Vulnerable Conditions. Comp. Educ. Rev. 2015, 59, 693–716. [Google Scholar] [CrossRef] [Green Version]
  24. Salvo-Garrido, S.; Miranda-Vargas, H.; Vivallo-Urra, O.; Gálvez-Nieto, J.; Miranda-Zapata, E. Students with Socioeconomic Disadvantages Who Have Academic Success in Language: Examining Academic Resilience in South America. Preprints.org 2019, 2019120043. [Google Scholar] [CrossRef] [Green Version]
  25. Salvo-Garrido, S.; Vargas, H.M.; Urra, O.V.; Gálvez-Nieto, J.L.; Miranda-Zapata, E. Resilient Students in the Area of Mathematics: Examining Protective and Risk Factors in an Emerging Country. Rev. Iberoam. Diagn. Eval. Psicol. 2020, 55, 43–57. [Google Scholar] [CrossRef]
  26. Salvo-Garrido, S.; San Martín, S.; Acuña-Cabrera, J.; Vivallo-Urra, O. Desarrollo de Factores Protectores y de Riesgo de La Resiliencia Socioeducativa de Niños Chilenos En Situación de Vulnerabilidad Social, Según La Perspectiva Docente. Rev. Colomb. Educ. 2020, 83, 1–18. [Google Scholar] [CrossRef]
  27. Anghel, R.E. Psychological and Educational Resilience in High vs. Low-Risk Romanian Adolescents. Procedia-Soc. Behav. Sci. 2015, 203, 153–157. [Google Scholar] [CrossRef] [Green Version]
  28. Bandura, A. Self-Referent Thought: A Developmental Analysis of Self-Efficacy; Flavell, J.H., Ross, L., Eds.; Cambridge University Press: Cambridge, UK, 1981. [Google Scholar]
  29. Arens, A.K.; Frenzel, A.C.; Goetz, T. Self-Concept and Self-Efficacy in Math: Longitudinal Interrelations and Reciprocal Linkages with Achievement. J. Exp. Educ. 2020, 90, 615–633. [Google Scholar] [CrossRef]
  30. Alivernini, F.; Manganelli, S.; Lucidi, F. The Last Shall Be the First: Competencies, Equity and the Power of Resilience in the Italian School System. Learn. Individ. Differ. 2016, 51, 19–28. [Google Scholar] [CrossRef]
  31. Borman, G.D.; Overman, L.T. Academic Resilience in Mathematics among Poor and Minority Students. Elem. Sch. J. 2004, 104, 177–195. [Google Scholar] [CrossRef] [Green Version]
  32. Kaskens, J.; Segers, E.; Lin, S.; van Luit, J.E.H. Impact of Children’ s Math Self-Concept, Math Self-Ef Fi Cacy, Math Anxiety, and Teacher Competencies on Math Development. Teach. Teach. Educ. 2020, 94, 103096. [Google Scholar] [CrossRef]
  33. Mercader, J.; Presentación, M.-J.; Siegenthaler, R.; Molinero, V.; Miranda, A. Motivación y Rendimiento Académico En Matemáticas: Un Estudio Longitudinal En Las Primeras Etapas Educativas. Rev. Psicodidact. 2017, 22, 157–163. [Google Scholar] [CrossRef]
  34. Stankov, L. Unforgiving Confucian Culture: A Breeding Ground for High Academic Achievement, Test Anxiety and Self-Doubt? Learn. Individ. Differ. 2010, 20, 555–563. [Google Scholar] [CrossRef]
  35. Akben-Selcuk, E. Personality, Motivation, and Math Achievement Among Turkish Students: Evidence from PISA Data. Percept. Mot. Skills 2017, 124, 514–530. [Google Scholar] [CrossRef] [PubMed]
  36. Bakan, D. The Influence of Socioeconomic Status, Self-Efficacy, and Anxiety on Mathematics Achievement in England, Greece, Hong Kong, the Netherlands, Turkey, and the USA. Educ. Sci. Theory Pract. 2015, 15, 1391–1401. [Google Scholar] [CrossRef] [Green Version]
  37. Recber, S.; Isiksal, M.; Koç, Y. Investigating Self-Efficacy, Anxiety, Attitudes and Mathematics Achievement Regarding Gender and School Type. Ann. Psicol. 2018, 34, 41–51. [Google Scholar] [CrossRef] [Green Version]
  38. Ryan, R.M.; Deci, E.L. Self-Determination Theory and the Facilitation of Intrinsic Motivation, Social Development, and Well-Being. Am. Psychol. 2000, 55, 68–78. [Google Scholar] [CrossRef]
  39. Fisher, C.R.; Thompson, C.D.; Brookes, R.H. Gender Differences in the Australian Undergraduate STEM Student Experience: A Systematic Review. High. Educ. Res. Dev. 2020, 39, 1155–1168. [Google Scholar] [CrossRef] [Green Version]
  40. del Río, M.F.; Susperreguy, M.I.; Strasser, K.; Cvencek, D.; Iturra, C.; Gallardo, I.; Meltzoff, A.N. Early Sources of Children’s Math Achievement in Chile: The Role of Parental Beliefs and Feelings about Math. Early Educ. Dev. 2020, 32, 637–652. [Google Scholar] [CrossRef]
  41. Goetz, T.; Bieg, M.; Lüdtke, O.; Pekrun, R.; Hall, N.C. Do Girls Really Experience More Anxiety in Mathematics? Psychol. Sci. 2013, 24, 2079–2087. [Google Scholar] [CrossRef] [Green Version]
  42. Levine, S.C.; Pantoja, N. Development of Children’s Math Attitudes: Gender Differences, Key Socializers, and Intervention Approaches. Dev. Rev. 2021, 62, 100997. [Google Scholar] [CrossRef]
  43. McCoy, S.; Byrne, D.; O’Connor, P. Gender Stereotyping in Mothers’ and Teachers’ Perceptions of Boys’ and Girls’ Mathematics Performance in Ireland. Oxford Rev. Educ. 2022, 48, 341–363. [Google Scholar] [CrossRef]
  44. Borgonovi, F.; Pokropek, A. Seeing Is Believing: Task-Exposure Specificity and the Development of Mathematics Self-Efficacy Evaluations. J. Educ. Psychol. 2019, 111, 268–283. [Google Scholar] [CrossRef]
  45. Zimmerman, B. Self-Efficacy: An Essential Motive to Learn. Contemp. Educ. Psychol. 2000, 25, 82–91. [Google Scholar] [CrossRef] [PubMed]
  46. Zhang, W.J.; Xin, T. A Cross-Culture Comparison Study of the Impact of Reading Engagement on Reading Literacy: Based on PISA 2009. Psychol. Dev. Educ. 2012, 28, 175–183. [Google Scholar] [CrossRef]
  47. Zhao, W.; Song, Y.; Zhao, Q.; Zhang, R. The Effect of Teacher Support on Primary School Students’ Reading Engagement: The Mediating Role of Reading Interest and Chinese Academic Self-Concept. Educ. Psychol. 2018, 39, 236–253. [Google Scholar] [CrossRef]
  48. García-Crespo, F.J.; Fernández-Alonso, R.; Muñiz, J. Academic Resilience in European Countries: The Role of Teachers, Families, and Student Profiles. PLoS ONE 2021, 16, e0253409. [Google Scholar] [CrossRef]
  49. Kennedy, E. Engaging Children as Readers and Writers in High-Poverty Contexts. J. Res. Read. 2018, 41, 716–731. [Google Scholar] [CrossRef]
  50. Fernández Batanero, J.M.; Montenegro Rueda, M.; Fernández Cerero, J.; Román Gravan, P. Impact of ICT on Writing and Reading Skills: A Systematic Review (2010–2020). Texto Livre 2021, 14, 1–12. [Google Scholar] [CrossRef]
  51. Liu, I.F.; Ko, H.W. Roles of Paper-Based Reading Ability and ICT-Related Skills in Online Reading Performance. Read. Writ. 2019, 32, 1037–1059. [Google Scholar] [CrossRef]
  52. Moreno, R.; Martinez, R. Aprendizaje autónomo. Desarrollo de una definición. Acta Comport. Rev. Lat. Análisis Comport. 2007, 15, 51–62. Available online: https://www.redalyc.org/articulo.oa?id=274520891004 (accessed on 13 January 2023).
  53. Xiao, Y.; Liu, Y.; Hu, J. Regression Analysis of ICT Impact Factors on Early Adolescents’ Reading Proficiency in Five High-Performing Countries. Front. Psychol. 2019, 10, 1646. [Google Scholar] [CrossRef] [Green Version]
  54. Smith, P.K.; Cowie, H.; Olafsson, R.F.; Liefooghe, A.P.D. Definitions of Bullying: A Comparison of Terms Used, and Age and Gender Differences, in a Fourteen-Country International Comparison. Child Dev. 2002, 73, 1119–1133. [Google Scholar] [CrossRef]
  55. Cerda, G.; Pérez, C.; Elipe, P.; Casas, J.A.; Del Rey, R. School Coexistence and Its Relationship with Academic Performance Among Primary Education Students. Rev. Psicodidact. 2018, 24, 46–52. [Google Scholar] [CrossRef]
  56. Laith, R.; Vaillancourt, T. The Temporal Sequence of Bullying Victimization, Academic Achievement, and School Attendance: A Review of the Literature. Aggress. Violent Behav. 2022, 64, 101722. [Google Scholar] [CrossRef]
  57. Bravo-Sanzana, M.; Pavez, M.; Salvo-Garrido, S.; Mieres-Chacaltana, M. Autoeficacia, Expectativas y Violencia Escolar Como Mediadores Del Aprendizaje En Matemática. Rev. Espac. 2019, 40, hal-03450819. [Google Scholar]
  58. Erberber, E.; Stephens, M.; Mamedova, S.; Ferguson, S.; Kroeger, T. Socioeconomically Disadvantaged Students Who Are Academically Successful: Examining Academic Resilience Cross-Nationally. IEA’s Policy Brief Ser. 2015, 5, 1–12. Available online: https://www.iea.nl/publications/series-journals/policy-brief/march-2015-socioeconomically-disadvantaged-students-who (accessed on 16 September 2022).
  59. Gómez-Ortiz, O.; Romera-Félix, E.-M.; Ortega-Ruiz, R. Multidimensionalidad de La Competencia Social: Medición Del Constructo y Su Relación Con Los Roles Del Bullying. Rev. Psicodidáctica 2017, 22, 37–44. [Google Scholar] [CrossRef]
  60. Clark, M.; Bussey, K. The Role of Self-Efficacy in Defending Cyberbullying Victims. Comput. Human Behav. 2020, 109, 106340. [Google Scholar] [CrossRef]
  61. Polanco-Levicán, K.; Salvo-Garrido, S. Bystander Roles in Cyberbullying: A Mini-Review of Who, How Many, and Why. Front. Psychol. 2021, 12, 1719. [Google Scholar] [CrossRef]
  62. Lucas-Molina, B.; Williamson, A.; Pulido, R. Longitudinal Prediction of 1st and 2nd Grade English Oral Reading Fluency in ELL. Psychol. Sch. 2015, 53, 298–315. [Google Scholar] [CrossRef]
  63. Graham, A.; Truscott, J.; O’Byrne, C.; Considine, G.; Hampshire, A.; Creagh, S.; Western, M. Disadvantaged Families’ Experiences of Home-School Partnerships: Navigating Agency, Expectations and Stigma. Int. J. Incl. Educ. 2019, 25, 1236–1251. [Google Scholar] [CrossRef]
  64. Lai, T.; Liu, F.; Huang, Y. The Influence of Parental Educational Expectations on Children’s Higher Education Attainment: Re-Estimation Based on Instrumental Variables. Front. Psychol. 2022, 13, 1–11. [Google Scholar] [CrossRef]
  65. King, R.B.; Ganotice, F.A. Does Family Obligation Matter for Students’ Motivation, Engagement, and Well-Being?: It Depends on Your Self-Construal. Pers. Individ. Dif. 2015, 86, 243–248. [Google Scholar] [CrossRef]
  66. Mudrák, J.; Zábrodská, K.; Takács, L. Systemic Approach to the Development of Reading Literacy: Family Resources, School Grades, and Reading Motivation in Fourth-Grade Pupils. Front. Psychol. 2020, 11, 37. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  67. Gan, Y.; Bilige, S. Parental Involvement in Home-Based Education and Children’s Academic Achievement in China. Soc. Behav. Pers. 2019, 47, 1–15. [Google Scholar] [CrossRef]
  68. Holmes, M.R.; Yoon, S.; Berg, K.A.; Cage, J.L.; Perzynski, A.T. Promoting the Development of Resilient Academic Functioning in Maltreated Children. Child Abus. Negl. 2018, 75, 92–103. [Google Scholar] [CrossRef] [PubMed]
  69. Kallia, E.; Dermitzaki, I.; Paraskeva, V.; Bonoti, F.; Nisiotou, I. A Six Months’ Prospective Study of the Relations between Children’s Self-Regulated Learning Skills and Maternal Supportive Behaviors. Cogn. Dev. 2022, 63, 101220. [Google Scholar] [CrossRef]
  70. Bravo, M.; Salvo-Garrido, S.; Mieres, M.; Mansilla, J.; Hederich, C. Perfiles de Desempeño Académico: La Importancia de Las Expectativas Familiares. Perf. Latinoam. 2017, 25, 361–386. [Google Scholar] [CrossRef] [Green Version]
  71. Murillo, F.J.; Hernández-Castilla, R. Does Parental Involvement Matter in Children’s Performance? A Latin American Primary School Study. Rev. Psicodidact. 2020, 25, 13–22. [Google Scholar] [CrossRef]
  72. Olmo-Extremera, M.; Townsend, A.; Segovia, J.D. Resilient Leadership in Principals: Case Studies of Challenged Schools in Spain. Int. J. Leadersh. Educ. 2022. [Google Scholar] [CrossRef]
  73. Ţibu, S.L.; Horga, I. Empathic and Supportive Teachers, Key to Quality and Efficiency in Education. Rev. Rom. Pentru Educ. Multidimens. 2018, 10, 166–181. [Google Scholar]
  74. Gutiérrez, M.; Tomás, J.M.; Romero, I.; Barrica, J.M. Apoyo Social Percibido, Implicación Escolar y Satisfacción Con La Escuela. Rev. Psicodidact. 2017, 22, 111–117. [Google Scholar] [CrossRef]
  75. Ansong, D.; Eisensmith, S.R.; Okumu, M.; Chowa, G.A. The Importance of Self-Efficacy and Educational Aspirations for Academic Achievement in Resource-Limited Countries: Evidence from Ghana. J. Adolesc. 2019, 70, 13–23. [Google Scholar] [CrossRef] [PubMed]
  76. Randall Chesnut, S.; Burley, H. Self-Efficacy as a Predictor of Commitment to the Teaching Profession: A Meta-Analysis. Educ. Res. Rev. 2015, 15, 1–16. [Google Scholar] [CrossRef]
  77. Bonneville-Roussy, A.; Bouffard, T.; Palikara, O.; Vezeau, C. The Role of Cultural Values in Teacher and Student Self-Efficacy: Evidence from 16 Nations. Contemp. Educ. Psychol. 2019, 59, 101798. [Google Scholar] [CrossRef]
  78. Miller, A.D.; Ramirez, E.M.; Murdock, T.B. The Influence of Teachers’ Self-Efficacy on Perceptions: Perceived Teacher Competence and Respect and Student Effort and Achievement. Teach. Teach. Educ. 2017, 64, 260–269. [Google Scholar] [CrossRef]
  79. Theron, L.C.; Theron, A.M.C. Education Services and Resilience Processes: Resilient Black South African Students’ Experiences. Child. Youth Serv. Rev. 2014, 47, 297–306. [Google Scholar] [CrossRef] [Green Version]
  80. Salvo-Garrido, S.I.; Gálvez-Nieto, J.L.; Martín-Parihuén, S.S. Academic Resilience: Understanding Family Relationships That Promote It. Rev. Electron. Educ. 2021, 25, 1–18. [Google Scholar] [CrossRef]
  81. Agencia de Calidad de la Educación. Informe Técnico SIMCE 2015. 2018, 1–65. Available online: https://www.agenciaeducacion.cl/simce/ (accessed on 23 April 2022).
  82. American Educational Research Association; American Psychological Association; National Council on Measurement in Education. Standards for Educational and Psychological Testing; American Educational Research Association: Washington, DC, USA, 2014. [Google Scholar]
  83. Toro, I.; Parra, R. Fundamentos Epistemológicos de la Investigación y la Metodología de la Investigación: Cualitativa/Cuantitativa; Universidad EAFIT: Medellín, Colombia, 2010; ISBN 978-958-720-057-7. [Google Scholar]
  84. Geiser, C. Data Analysis with Mplus; The Guilford Press: New York, NY, USA, 2010. [Google Scholar]
  85. Asun, R.A.; Rdz-Navarro, K.; Alvarado, J.M. Developing Multidimensional Likert Scales Using Item Factor Analysis: The Case of Four-Point Items. Sociol. Methods Res. 2016, 45, 109–133. [Google Scholar] [CrossRef]
  86. Finney, S.J.; DiStefano, C. Non-normal and categorical data in structural equation modeling. In Structural Equation Modeling: A Second Course; Hancock, G.R., Mueller, R.O., Eds.; Information Age: Charlotte, NC, USA, 2006. [Google Scholar]
  87. Hu, L.; Bentler, P.M. Cutoff Criteria for Fit Indexes in Covariance Structure Analysis: Conventional Criteria versus New Alternatives, Structural Equation Modeling. Struct. Equ. Model. A Multidiscip. J. 1999, 6, 1–55. [Google Scholar] [CrossRef]
  88. Schumacher, R.E.; Lomax, R.G. A Beginner’s Guide to Structural Equation Modeling, 3rd ed.; Routledge, Taylor & Francis Group: New York, NY, USA, 2015. [Google Scholar]
  89. Schreiber, J.B.; Amaury, N.; Stage, F.K.; Barlow, E.A.; King, J. Reporting Structural Equation Modeling and Confirmatory Factor Analysis Results: A Review. J. Educ. Res. 2006, 99, 323–338. [Google Scholar] [CrossRef]
  90. Trizano-Hermosilla, I.; Alvarado, J.M. Best Alternatives to Cronbach’s Alpha Reliability in Realistic Conditions: Congeneric and Asymmetrical Measurements. Front. Psychol. 2016, 7, 769. [Google Scholar] [CrossRef] [Green Version]
  91. Campo-Arias, A.; Oviedo, H.C. Propiedades Psicométricas de Una Escala: La Consistencia Interna. Rev. Salud Pública 2008, 10, 831–839. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  92. Muthén, L.; Muthén, B.O. Mplus User’s Guide, 8th ed.; 1998–2017 Muthén & Muthén Program; Muthén & Muthén: Los Angeles, CA, USA, 2017. [Google Scholar]
  93. Muthén, B.O.; Muthén, L.K.; Tihomir, A. Regression and Mediation Analysis Using Mplus; Muthén & Muthén: Los Angeles, CA, USA, 2016; ISBN 978-0-9829983-1-1. [Google Scholar]
  94. García-Crespo, F.J.; Galián, B.; Fernández-Alonso, R.; Muñiz, J. Educational Resilience in Reading Comprehension: Determinant Factors in PIRLS-Europe. Rev. Educ. 2019, 384, 71–96. [Google Scholar] [CrossRef]
  95. Pitzer, J.; Skinner, E. Predictors of Changes in Students’ Motivational Resilience over the School Year: The Roles of Teacher Support, Self-Appraisals, and Emotional Reactivity. Int. J. Behav. Dev. 2016, 41, 15–29. [Google Scholar] [CrossRef]
  96. Semanchin Jones, A.; Bowen, E.; Ball, A. School Definitely Failed Me, the System Failed Me: Identifying Opportunities to Impact Educational Outcomes for Homeless and Child Welfare-Involved Youth. Child. Youth Serv. Rev. 2018, 91, 66–76. [Google Scholar] [CrossRef]
  97. Cattelino, E.; Morelli, M.; Baiocco, R.; Chirumbolo, A. From External Regulation to School Achievement: The Mediation of Self-Efficacy at School. J. Appl. Dev. Psychol. 2018, 60, 127–133. [Google Scholar] [CrossRef]
  98. Gaxiola, J.C.; González, S.; Gaxiola, E. Autorregulación, Resiliencia y Metas Educativas: Variables Protectoras Del Rendimiento Académico de Bachilleres. Rev. Colomb. Psicol. 2013, 22, 241–252. [Google Scholar]
Behavsci 13 00516 g001 550
Figure 1. Latent regression analysis on the SIMCE mathematics and language scores. Where: I1,…,I8 represent the items of each of the latent factors and βi,i = 1,…,6 are the parameters of the model to be estimated.
Figure 1. Latent regression analysis on the SIMCE mathematics and language scores. Where: I1,…,I8 represent the items of each of the latent factors and βi,i = 1,…,6 are the parameters of the model to be estimated.
Behavsci 13 00516 g001
Table
Table 1. Descriptive statistics, mean, and standard deviation.
Table 1. Descriptive statistics, mean, and standard deviation.
SchoolGenderLangMathL1L2L3L4L5L6Expect.
PublicMale−0.36 (1.03)−0.30 (1.04)0.02 (0.66)−0.09 (0.49)−0.05 (0.61)0.23 (0.80)0.18 (0.57)0.26 (0.70)−0.36 (1.18)
Female−0.18 (0.99)−0.35 (1.01)−0.07 (0.64)−0.04 (0.49)0.15 (0.59)0.20 (0.80)0.17 (0.60)0.07 (0.70)−0.30 (1.15)
Private-subsidizedMale0.06 (1.00)0.20 (0.95)0.04 (0.66)−0.01 (0.49)−0.16 (0.62)−0.04 (0.70)0.07 (0.52)0.07 (0.63)0.15 (0.88)
Female0.20 (0.94)0.13 (0.93)−0.07 (0.67)0.04 (0.48)0.05 (0.62)−0.08 (0.70)0.04 (0.53)−0.12 (0.61)0.18 (0.84)
Expect. = Expectations parents/guardians, (SD). Note: The mean differences by school type and gender were statistically significant, p < 0.001.
Table
Table 2. Description of the latent factors and their respective standardized factor loadings.
Table 2. Description of the latent factors and their respective standardized factor loadings.
Latent VariableItemsEst.SEp-Value
Self-efficacy in mathematicsDoing math problems is entertaining.0.7420.002<0.001
I do better in math than my classmates.0.7490.002<0.001
I learn math easily and quickly.0.9190.002<0.001
Self-efficacy in languageReading is easier for me than for many of my classmates.0.6560.003<0.001
Reading is easier for me than any other class activity.0.7360.003<0.001
I am simply good at reading.0.6870.003<0.001
Interest in readingI would be happy if someone gifted me a book.0.7260.002<0.001
I would like to have more time for reading.0.7740.002<0.001
I like reading.0.8690.002<0.001
I read for fun.0.6490.003<0.001
Violence/AggressionInsults, slurs, derision, and disqualifications among students.0.8990.002<0.001
Threats or harassment among students.0.8780.002<0.001
Students break or damage the establishment.0.7100.003<0.001
Discrimination/ExclusionGender of the student.0.7600.004<0.001
Physical characteristics of the student.0.8690.002<0.001
Personality of the student.0.7410.003<0.001
Way of dressing or physical appearance of the student.0.8810.003<0.001
BullyingSome school students have stolen from another classmate.0.7590.002<0.001
Some school students have scared another classmate and forced them to do things they did not want to.0.8420.002<0.001
Some school students have threatened to beat another classmate.0.8270.002<0.001
Some school students have forced another classmate to give them their belongings or their money.0.8620.002<0.001
Some school students have intentionally broken another classmate’s belongings.0.8070.002<0.001
Some school students have sent messages to annoy or threaten another classmate.0.7400.003<0.001
Est. = Estimate, SE = Standard error.
Table
Table 3. Standardized coefficient estimates of the latent regression model.
Table 3. Standardized coefficient estimates of the latent regression model.
MathematicsLanguage
VariableParticipantEst. Coef.SEp-ValueEst. Coef.SEp-Value
Intercept 0.0390.005<0.001−0.2530.007<0.001
Self-efficacy in mathematicsStudent0.3970.004<0.0010.2030.004<0.001
Self-efficacy in languageStudent0.3090.004<0.0010.4350.004<0.001
Interest in readingStudent0.0340.0040.0310.0990.004<0.001
ExpectationsParents/guardians0.2800.003<0.0010.2660.004<0.001
Violence/AggressionTeacher−0.1580.004<0.001−0.1440.004<0.001
Discrimination/ExclusionParents/guardians−0.1820.005<0.001−0.1680.004<0.001
BullyingStudent−0.2510.004<0.001−0.2970.004<0.001
Gender (Female = 1, Male = 0) −0.0560.004<0.0010.0140.0040.001
Type of school (Private-subsidized = 1, Public = 0) 0.0480.004<0.001−0.0010.0040.735
Est. coef = Estimate coefficient; SE = Standard error.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Salvo-Garrido, S.; Zayas-Castro, J.; Polanco-Levicán, K.; Gálvez-Nieto, J.L. Latent Regression Analysis Considering Student, Teacher, and Parent Variables and Their Relationship with Academic Performance in Primary School Students in Chile. Behav. Sci. 2023, 13, 516. https://doi.org/10.3390/bs13060516

AMA Style

Salvo-Garrido S, Zayas-Castro J, Polanco-Levicán K, Gálvez-Nieto JL. Latent Regression Analysis Considering Student, Teacher, and Parent Variables and Their Relationship with Academic Performance in Primary School Students in Chile. Behavioral Sciences. 2023; 13(6):516. https://doi.org/10.3390/bs13060516

Chicago/Turabian Style

Salvo-Garrido, Sonia, José Zayas-Castro, Karina Polanco-Levicán, and José Luis Gálvez-Nieto. 2023. "Latent Regression Analysis Considering Student, Teacher, and Parent Variables and Their Relationship with Academic Performance in Primary School Students in Chile" Behavioral Sciences 13, no. 6: 516. https://doi.org/10.3390/bs13060516

APA Style

Salvo-Garrido, S., Zayas-Castro, J., Polanco-Levicán, K., & Gálvez-Nieto, J. L. (2023). Latent Regression Analysis Considering Student, Teacher, and Parent Variables and Their Relationship with Academic Performance in Primary School Students in Chile. Behavioral Sciences, 13(6), 516. https://doi.org/10.3390/bs13060516

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop