Kinetics of White Soft Minerals (WSMs) Decomposition under Conditions of Interest for Astrobiology: A Theoretical and Experimental Study
Abstract
:1. Introduction
2. Evaluation of Delivery Scenarios Involving WSMs: Computer Modelling
3. Theory and Experiment: Calcite Decomposition
- problematic carbonate samples, in which there is no clear evidence of controlled or induced biomineralization [40];
- microbialites, i.e., bio-induced carbonate deposits, and stromatolites, i.e., the laminated fabric of microbialites, some of which are among the most primitive traces of biological activity on Earth [41];
- different parts of the same carbonate rock to discriminate the presence, nature and degree of biogenicity of various micrite types (i.e., detrital vs autochthonous) and to distinguish them from the skeletal grains, each one characterized by different organic matter content [42].
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Jenniskens, P.; Wilson, M.A.; Packan, D.; Laux, C.O.; Krüger, C.H.; Boyd, I.D.; Popova, O.P.; Fonda, M. Meteors: A delivery mechanism of organic matter to the early Earth. Earth Moon Planets 1998, 82, 57–70. [Google Scholar] [CrossRef]
- Chyba, C.; Sagan, C. Endogenous production, exogenous delivery and impact-shock synthesis of organic molecules: An inventory for the origins of life. Nature 1992, 355, 125–132. [Google Scholar] [CrossRef] [PubMed]
- Horneck, G.; Rettberg, P.; Reitz, G.; Panitz, C.; Rabbow, E. Protection of bacterial spores in space, a contribution to the discussion on panspermia. Orig. Life Evol. Biosph. 2001, 31, 527–547. [Google Scholar] [CrossRef] [PubMed]
- Burchell, M.J. Panspermia today. Int. J. Astrobiol. 2004, 3, 73–80. [Google Scholar] [CrossRef]
- McKay, D.S.; Gibson, E.K., Jr.; Thomas-Keprta, K.L.; Vali, H.; Romanek, C.S.; Clemett, S.J.; Chillier, X.D.; Maechling, C.R.; Zare, R.N. Search for past life on Mars: Possible relic biogenic activity in Martian meteorite ALH84001. Science 1996, 273, 924–930. [Google Scholar] [CrossRef] [PubMed]
- Glavin, D.P.; Bada, J.L. Survival of amino acids in micrometeorites during atmospheric entry. Astrobiology 2001, 1, 259–269. [Google Scholar] [CrossRef] [PubMed]
- Matrajt, G.; Brownlee, D.; Sadilek, M.; Kruse, L. Survival of organic phases in porous IDPs during atmospheric entry: A pulse-heating study. Meteorit. Planet. Sci. 2006, 41, 903–911. [Google Scholar] [CrossRef]
- Flynn, G.; Keller, L.P.; Jacobsen, C.; Wirick, S.; Miller, M.A. Organic carbon in interplanetary dust particles. Bioastronomy 99 2000, 213, 191–194. [Google Scholar]
- Pizzarello, S.; Cooper, G.W.; Flynn, G.J. The nature and distribution of the organic material in carbonaceous chondrites and interplanetary dust particles. Meteorit. Early Sol. Syst. II 2000, 1, 625–651. [Google Scholar]
- Matrajt, G.; Messenger, S.; Brownlee, D.; Joswiak, D. Diverse forms of primordial organic matter identified in interplanetary dust particles. Meteorit. Planet. Sci. 2012, 47, 525–549. [Google Scholar] [CrossRef]
- Peters, C.A.; Piazolo, S.; Webb, G.E.; Dutkiewicz, A.; George, S.C. In search of early life: Carbonate veins in Archean metamorphic rocks as potential hosts of biomarkers. Earth Planet. Sci. Lett. 2016, 453, 44–55. [Google Scholar] [CrossRef]
- Peters, C.A.; George, S.C. Hydrocarbon biomarkers preserved in carbonate veins of potentially Paleoproterozoic age, and implications for the early biosphere. Geobiology 2018, 16, 577–596. [Google Scholar] [CrossRef] [PubMed]
- Gendrin, A.; Mangold, N.; Bibring, J.P.; Langevin, Y.; Gondet, B.; Poulet, F.; Bonello, G.; Quantin, C.; Mustard, J.; Arvidson, R.; et al. Sulfates in Martian layered terrains: the OMEGA/Mars Express view. Science 2005, 307, 1587–1591. [Google Scholar] [CrossRef] [PubMed]
- Langevin, Y.; Poulet, F.; Bibring, J.P.; Gondet, B. Sulfates in the north polar region of Mars detected by OMEGA/Mars Express. Science 2005, 307, 1584–1586. [Google Scholar] [CrossRef] [PubMed]
- Ehlmann, B.L.; Mustard, J.F.; Murchie, S.L.; Poulet, F.; Bishop, J.L.; Brown, A.J.; Calvin, W.M.; Clark, R.N.; Marais, D.J.; Milliken, R.E.; et al. Orbital identification of carbonate-bearing rocks on Mars. Science 2008, 322, 1828–1832. [Google Scholar] [CrossRef] [PubMed]
- Boynton, W.V.; Ming, D.W.; Kounaves, S.P.; Young, S.M.; Arvidson, R.E.; Hecht, M.H.; Hoffman, J.; Niles, P.B.; Hamara, D.K.; Quinn, R.C.; et al. Evidence for calcium carbonate at the Mars Phoenix landing site. Science 2009, 325, 61–64. [Google Scholar] [CrossRef] [PubMed]
- Palomba, E.; Zinzi, A.; Cloutis, E.A.; D’Amore, M.; Grassi, D.; Maturilli, A. Evidence for Mg-rich carbonates on Mars from a 3.9 μm absorption feature. Icarus 2009, 203, 58–65. [Google Scholar] [CrossRef]
- Wray, J.J.; Murchie, S.L.; Bishop, J.L.; Ehlmann, B.L.; Milliken, R.E.; Wilhelm, M.B.; Seelos, K.D.; Chojnacki, M. Orbital evidence for more widespread carbonate-bearing rocks on Mars. J. Geophys. Res. Planets 2016, 121, 652–677. [Google Scholar] [CrossRef]
- Borg, L.E.; Connelly, J.N.; Nyquist, L.E.; Shih, C.Y.; Wiesmann, H.; Reese, Y. The age of the carbonates in Martian meteorite ALH84001. Science 1999, 286, 90–94. [Google Scholar] [CrossRef] [PubMed]
- Thomas-Keprta, K.L.; Clemett, S.J.; Mckay, D.S.; Gibson, E.K.; Wentworth, S.J. Origins of magnetite nanocrystals in Martian meteorite ALH84001. Geochim. Cosmochim. Acta 2009, 73, 6631–6677. [Google Scholar] [CrossRef]
- Gooding, J.L.; Wentworth, S.J.; Zolensky, M.E. Calcium carbonate and sulfate of possible extraterrestrial origin in the EETA 79001 meteorite. Geochim. Cosmochim. Acta 1988, 52, 909–915. [Google Scholar] [CrossRef]
- Gooding, J.L.; Wentworth, S.J.; Zolensky, M.E. Aqueous alteration of the Nakhla meteorite. Meteoritics 1991, 26, 135–143. [Google Scholar] [CrossRef]
- Treiman, A.H.; Barrett, R.A.; Gooding, J.L. Preterrestrial aqueous alteration of the Lafayette (SNC) meteorite. Meteoritics 1993, 28, 86–97. [Google Scholar] [CrossRef]
- Wentworth, S.J.; Gooding, J.L. Carbonates and sulfates in the Chassigny meteorite: Further evidence for aqueous chemistry on the SNC parent planet. Meteoritics 1994, 29, 860–863. [Google Scholar] [CrossRef]
- Friedmann, E.I.; Wierzchos, J.; Ascaso, C.; Winklhofer, M. Chains of magnetite crystals in the meteorite ALH84001: Evidence of biological origin. Proc. Natl. Acad. Sci. USA 2001, 98, 2176–2181. [Google Scholar] [CrossRef] [PubMed]
- Gibson, E.K., Jr.; McKay, D.S.; Thomas-Keprta, K.L.; Wentworth, S.J.; Westall, F.; Steele, A.; Romanek, C.S.; Bell, M.S.; Toporski, J. Life on Mars: evaluation of the evidence within Martian meteorites ALH84001, Nakhla, and Shergotty. Precambrian Res. 2001, 106, 15–34. [Google Scholar] [CrossRef]
- Rivkin, A.S.; Volquardsen, E.L.; Clark, B.E. The surface composition of Ceres: Discovery of carbonates and iron-rich clays. Icarus 2006, 185, 563–567. [Google Scholar] [CrossRef]
- de Sanctis, M.C.; Raponi, A.; Ammannito, E.; Ciarniello, M.; Toplis, M.J.; McSween, H.Y.; Castillo-Rogez, J.C.; Ehlmann, B.L.; Carrozzo, F.G.; Marchi, S.; et al. Bright carbonate deposits as evidence of aqueous alteration on (1) Ceres. Nature 2016, 536, 54–57. [Google Scholar] [CrossRef] [PubMed]
- De Sanctis, M.C.; Ammannito, E.; McSween, H.Y.; Raponi, A.; Marchi, S.; Capaccioni, F.; Capria, M.T.; Carrozzo, F.G.; Ciarniello, M.; Fonte, S.; et al. Localized aliphatic organic material on the surface of Ceres. Science 2017, 355, 719–722. [Google Scholar] [CrossRef] [PubMed]
- De Sanctis, M.C.; Vinogradoff, V.; Raponi, A.; Ammannito, E.; Ciarniello, M.; Carrozzo, F.G.; De Angelis, S.; Raymond, C.A.; Russell, C.T. Characteristics of organic matter on Ceres from VIR/Dawn high spatial resolution spectra. Mon. Notices R. Astron. Soc. 2018, 482, 2407–2421. [Google Scholar] [CrossRef]
- Busemann, H.; Nguyen, A.N.; Cody, G.D.; Hoppe, P.; Kilcoyne, A.L.D.; Stroud, R.M.; Zega, T.; Nittler, L.R. Ultra-primitive interplanetary dust particles from the comet 26P/Grigg-Skjellerup dust stream collection. Earth Planet. Sci. Lett. 2009, 288, 44–57. [Google Scholar] [CrossRef]
- Fomenkova, M.N.; Kerridge, J.F.; Marti, K.; McFadden, L.A. Compositional trends in rock-forming elements of comet Halley dust. Science 1992, 258, 266–269. [Google Scholar] [CrossRef] [PubMed]
- Love, S.G.; Brownlee, D.E. Heating and thermal transformation of micrometeoroids entering the Earth’s atmosphere. Icarus 1991, 89, 26–43. [Google Scholar] [CrossRef]
- Micca Longo, G.; Longo, S. Thermal decomposition of MgCO3 during the atmospheric entry of micrometeoroids. Int. J. Astrobiol. 2017, 16, 368–378. [Google Scholar] [CrossRef]
- Micca Longo, G.; Longo, S. Theoretical analysis of the atmospheric entry of sub-mm meteoroids of MgxCa1−xCO3 composition. Icarus 2018, 310, 194–202. [Google Scholar] [CrossRef]
- Micca Longo, G.; Piccinni, V.; Longo, S. Evaluation of CaSO4 micrograins in the context of organic matter delivery: Thermochemistry and atmospheric entry. Int. J. Astrobiol. 2018, 1–8. [Google Scholar] [CrossRef]
- Bisceglia, E.; Micca Longo, G.; Longo, S. Thermal decomposition rate of MgCO3 as an inorganic astrobiological matrix in meteorites. Int. J. Astrobiol. 2017, 16, 130–136. [Google Scholar] [CrossRef]
- Orofino, V.; Blanco, A.; D’Elia, M.; Licchelli, D.; Fonti, S. Infrared transmission spectroscopy of carbonate samples of biotic origin relevant to Mars exobiological studies. Icarus 2007, 187, 457–463. [Google Scholar] [CrossRef]
- Orofino, V.; Blanco, A.; D’Elia, M.; Fonti, S.; Licchelli, D. Time-dependent degradation of biotic carbonates and the search for past life on Mars. Planet. Space Sci. 2009, 57, 632–639. [Google Scholar] [CrossRef]
- Blanco, A.; Orofino, V.; D’Elia, M.; Fonti, S.; Mastandrea, A.; Guido, A.; Russo, F. A spectroscopic method for identifying terrestrial biocarbonates and application to Mars. Icarus 2011, 213, 473–479. [Google Scholar] [CrossRef]
- Blanco, A.; Orofino, V.; D’Elia, M.; Fonti, S.; Mastandrea, A.; Guido, A.; Russo, F. Infrared spectroscopy of microbially induced carbonates and past life on Mars. Icarus 2013, 226, 119–126. [Google Scholar] [CrossRef]
- Blanco, A.; D’Elia, M.; Orofino, V.; Mancarella, F.; Fonti, S.; Mastandrea, A.; Guido, A.; Tosti, F.; Russo, F. Microbialites vs. detrital micrites: Degree of biogenicity, parameter suitable for Mars analogues. Planet. Space Sci. 2014, 97, 34–42. [Google Scholar] [CrossRef]
- Grün, E.; Zook, H.A.; Fechtig, H.; Giese, R.H. Collisional balance of the meteoritic complex. Icarus 1985, 62, 244–272. [Google Scholar] [CrossRef]
- Kerridge, J.F.; Matthews, M.S. Meteorites and the Early Solar System; University of Arizona Press: Tucson, AR, USA, 1988. [Google Scholar]
- Flynn, G.J.; Keller, L.P.; Feser, M.; Wirick, S.; Jacobsen, C. The origin of organic matter in the solar system: Evidence from the interplanetary dust particles. Geochim. Cosmochim. Acta 2003, 67, 4791–4806. [Google Scholar] [CrossRef]
- Love, S.G.; Brownlee, D.E. A direct measurement of the terrestrial mass accretion rate of cosmic dust. Science 1993, 262, 550–553. [Google Scholar] [CrossRef] [PubMed]
- Foucher, F.; Westall, F.; Brandstaetter, F.; Demets, R.; Parnell, J.; Cockell, C.; Edwards, H.; Jean-Michel, B.; Brack, A.; Kurat, G. Testing the survival of microfossils in artificial Martian sedimentary meteorites during entry into Earth’s atmosphere: the STONE 6 experiment. Icarus 2010, 207, 616–630. [Google Scholar] [CrossRef]
- Opik, E.J. Physics of Meteor Flight in the Atmosphere; Courier Corporation: Chelmsford, MA, USA, 2004. [Google Scholar]
- L’vov, B.V. Thermal Decomposition of Solids and Melts: New Thermochemical Approach to the Mechanism, Kinetics and Methodology (Vol. 7); Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Briani, G.; Pace, E.; Shore, S.N.; Pupillo, G.; Passaro, A.; Aiello, S. Simulations of micrometeoroid interactions with the Earth atmosphere. Astron. Astrophys. 2013, 552, A53. [Google Scholar] [CrossRef]
- Orofino, V.; Blanco, A.; Fonti, S.; Marra, A.C.; Polimeno, N. The complex refractive index of limestone particles: An extension to the FIR range for Mars applications. Planet. Space Sci. 2002, 50, 839–847. [Google Scholar] [CrossRef]
- Hills, A.W.D. The mechanism of the thermal decomposition of calcium carbonate. Chem. Eng. Sci. 1968, 23, 297–320. [Google Scholar] [CrossRef]
- Barker, R. The reversibility of the reaction CaCO3 CaO+ CO2. J. Appl. Chem. Biotechnol. 1973, 23, 733–742. [Google Scholar] [CrossRef]
- Houst, Y.F.; Wittmann, F.H. Influence of porosity and water content on the diffusivity of CO2 and O2 through hydrated cement paste. Cement Concr. Res. 1994, 24, 1165–1176. [Google Scholar] [CrossRef]
- Schenk, A.S.; Kim, Y.-Y. Unraveling the internal microstructure of biogenic and bioinspired calcite single crystals. MRS Bull. 2015, 40, 499–508. [Google Scholar] [CrossRef]
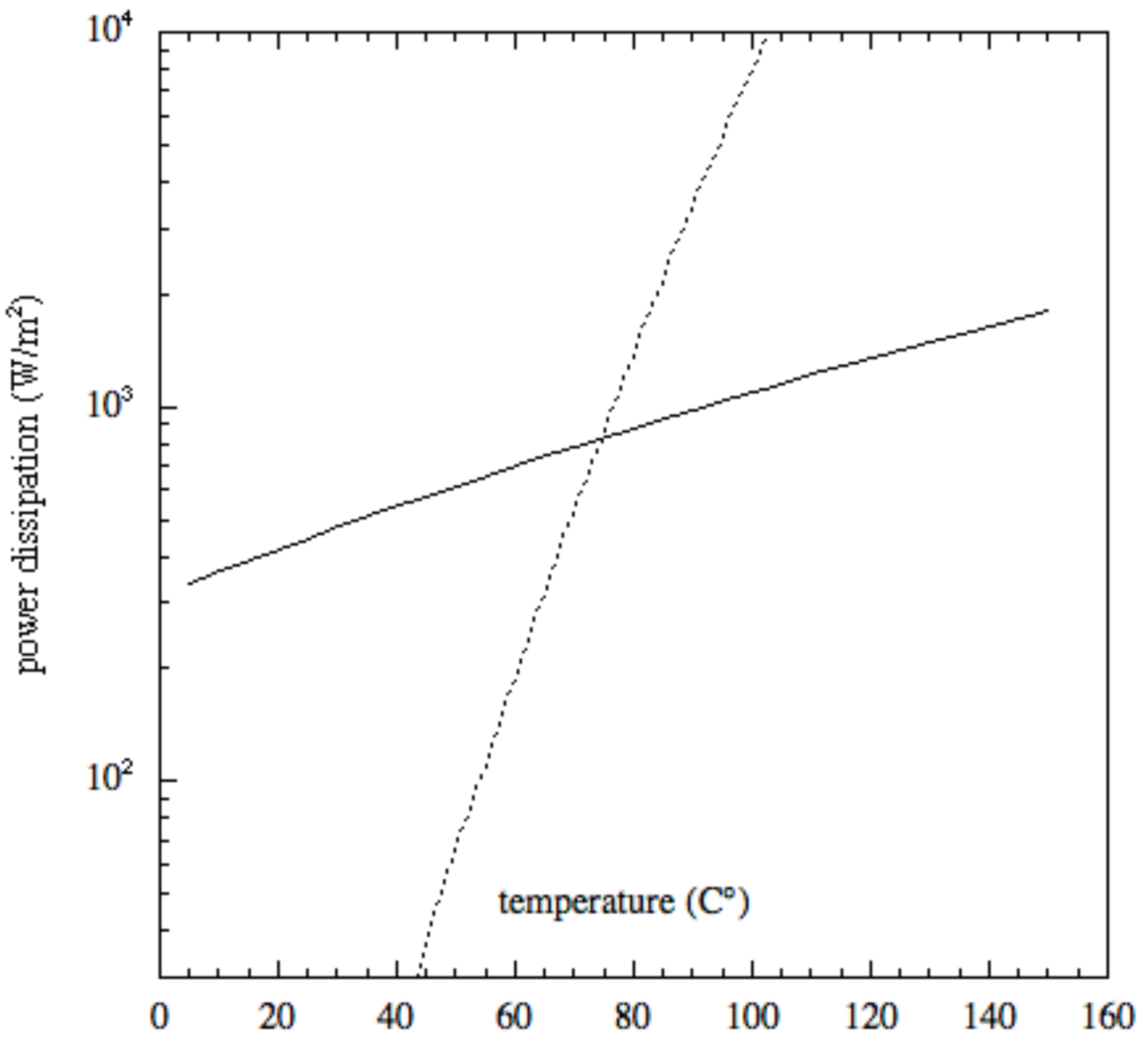



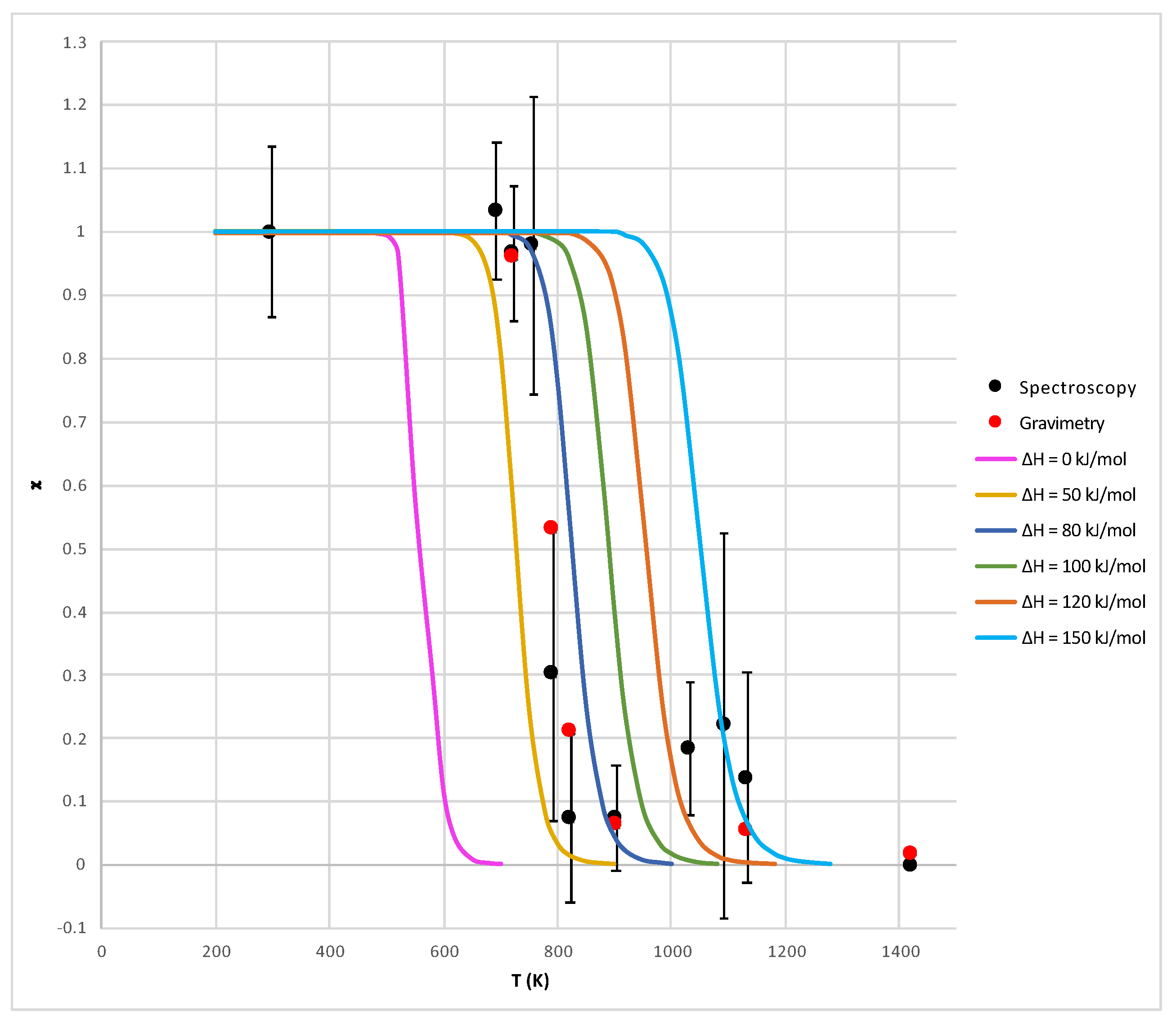

| Temperature (C) | Temperature (K) | ||||
|---|---|---|---|---|---|
| 25 | 298 | 1.00 | 0.14 | ||
| 420 | 693 | 1.03 | 0.11 | ||
| 450 | 723 | 0.97 | 0.11 | 0.960 | 0.004 |
| 485 | 758 | 0.98 | 0.24 | ||
| 520 | 793 | 0.30 | 0.23 | 0.532 | 0.003 |
| 550 | 823 | 0.07 | 0.13 | 0.212 | 0.002 |
| 630 | 903 | 0.07 | 0.08 | 0.065 | 0.002 |
| 760 | 1033 | 0.19 | 0.10 | ||
| 820 | 1093 | 0.22 | 0.31 | ||
| 860 | 1133 | 0.14 | 0.17 | 0.056 | 0.002 |
| 1150 | 1423 | 0.00 | 0.00 | 0.019 | 0.002 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Micca Longo, G.; D’Elia, M.; Fonti, S.; Longo, S.; Mancarella, F.; Orofino, V. Kinetics of White Soft Minerals (WSMs) Decomposition under Conditions of Interest for Astrobiology: A Theoretical and Experimental Study. Geosciences 2019, 9, 101. https://doi.org/10.3390/geosciences9020101
Micca Longo G, D’Elia M, Fonti S, Longo S, Mancarella F, Orofino V. Kinetics of White Soft Minerals (WSMs) Decomposition under Conditions of Interest for Astrobiology: A Theoretical and Experimental Study. Geosciences. 2019; 9(2):101. https://doi.org/10.3390/geosciences9020101
Chicago/Turabian StyleMicca Longo, Gaia, Marcella D’Elia, Sergio Fonti, Savino Longo, Francesca Mancarella, and Vincenzo Orofino. 2019. "Kinetics of White Soft Minerals (WSMs) Decomposition under Conditions of Interest for Astrobiology: A Theoretical and Experimental Study" Geosciences 9, no. 2: 101. https://doi.org/10.3390/geosciences9020101
APA StyleMicca Longo, G., D’Elia, M., Fonti, S., Longo, S., Mancarella, F., & Orofino, V. (2019). Kinetics of White Soft Minerals (WSMs) Decomposition under Conditions of Interest for Astrobiology: A Theoretical and Experimental Study. Geosciences, 9(2), 101. https://doi.org/10.3390/geosciences9020101






