Combined Gravimetric-Seismic Moho Model of Tibet
Abstract
1. Introduction
2. Study Area and Data Acquisition
2.1. Study Area
2.2. Seismic Data
2.3. Gravity Data
2.3.1. Free-Air Gravity Data
2.3.2. Bouguer Gravity Data
2.3.3. Isostatic Gravity Data
2.4. Gravity Maps
3. Method
3.1. Seismic Moho Model
3.2. Isostatic Moho Model
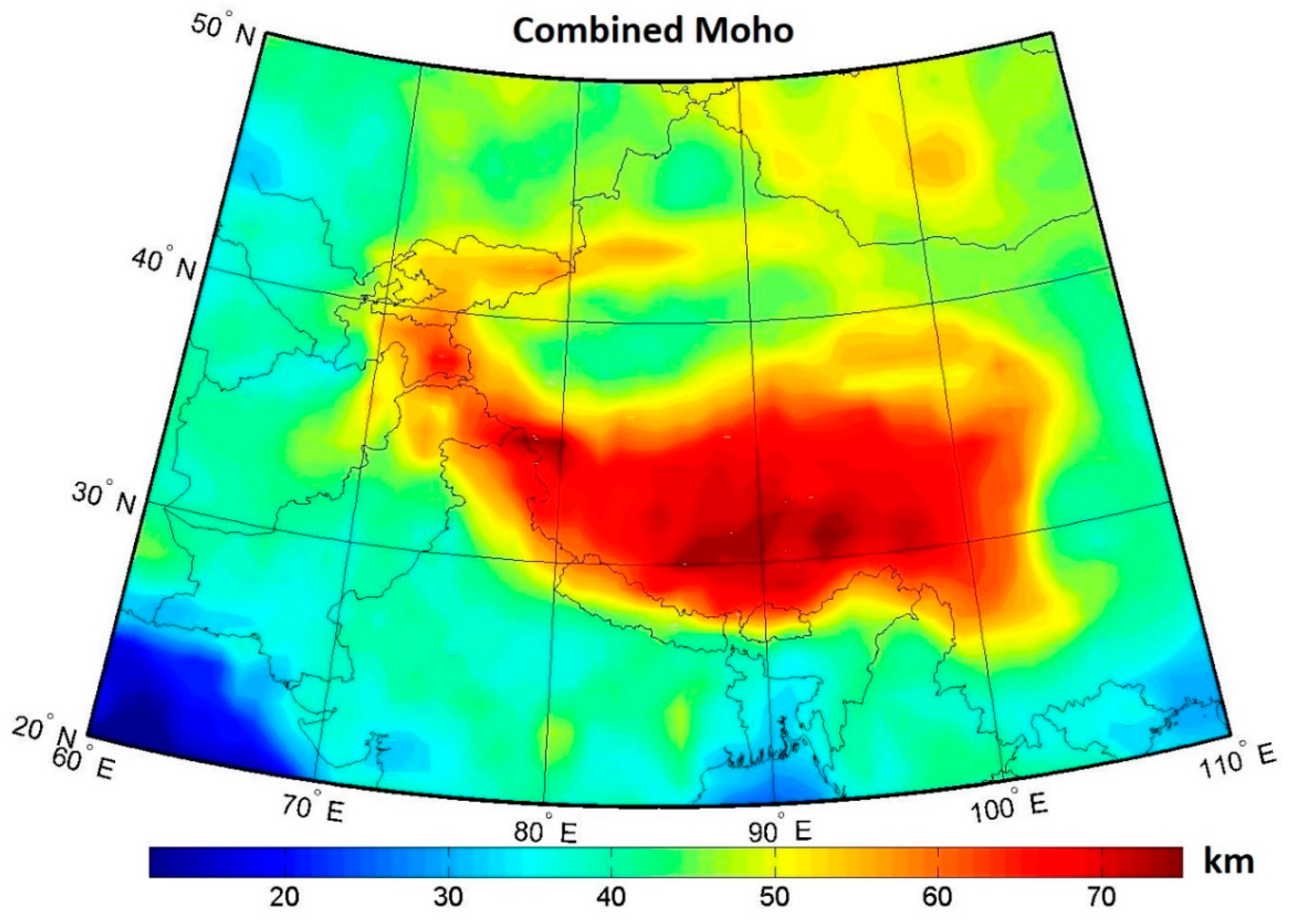
3.3. Combined Moho Model
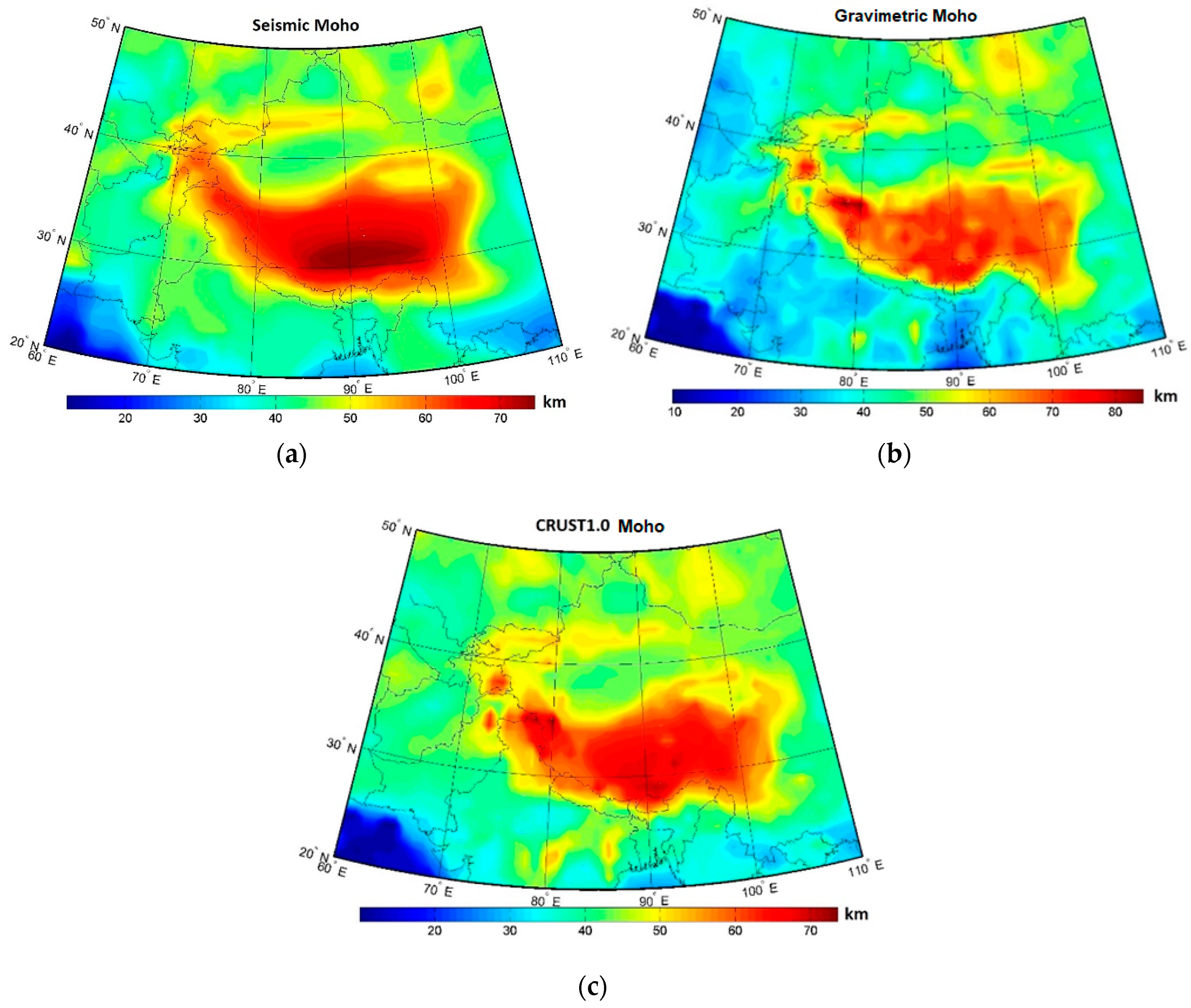
4. Results
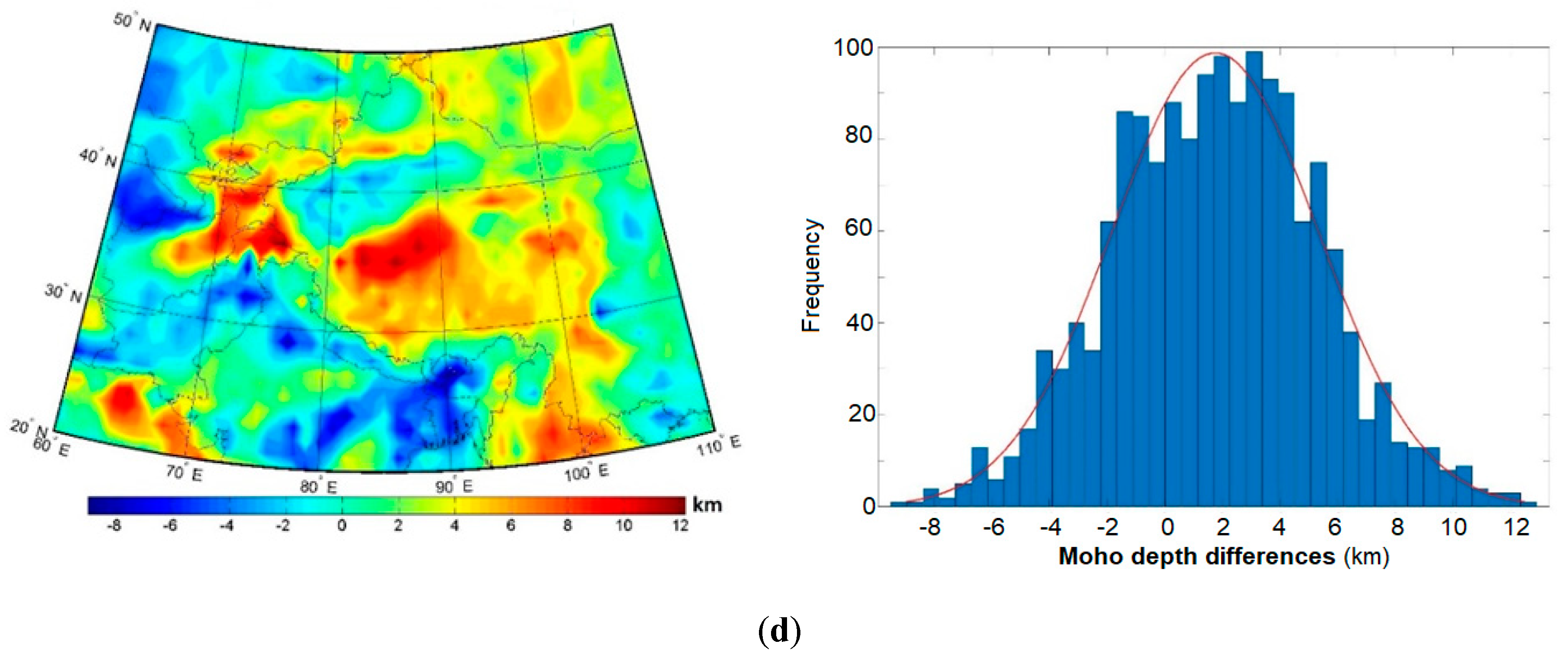
Comparison of Results
5. Discussion
Accuracy Assessment
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Teng, J.W.; Yin, Z.X.; Wnag, X.T.; Lu, D.Y. Structure of the crust and upper mantle pattern and velocity distributional characteristics in the Northern Himalayan mountain region. Acta Geophys. Sin. 1983, 26, 525–540. [Google Scholar] [CrossRef]
- Tilmann, F.; Ni, J.; INDEPTH III Seismic Team. Seismic imaging of the downwelling Indian lithosphere beneath central Tibet. Science 2003, 300, 1424–1427. [Google Scholar] [CrossRef]
- Allègre, C.J.; Courtillot, V.; Tapponnier, P.A.; Hirn, M.; Mattauer, C.; Coulon, J.J.; Jaeger, J.; Achache, U.; Schärer, J.; Marcoux, J.P.; et al. Structure and evolution of the Himalaya-Tibet orogenic belt. Nature 1984, 307, 17–22. [Google Scholar] [CrossRef]
- Zhao, W.; Nelson, K.D.; Che, J.; Quo, J.; Lu, D.; Wu, C.; Liu, X. Deep seismic reflection evidence for continental underthrusting beneath southern Tibet. Nature 1993, 366, 557–559. [Google Scholar] [CrossRef]
- Rai, S.S.; Priestley, K.; Gaur, V.K.; Mitra, S.; Singh, M.P.; Searle, M. Configuration of the Indian Moho beneath the NW Himalaya and Ladakh. Geophys. Res. Lett. 2006, 33, L15308. [Google Scholar] [CrossRef]
- Wittlinger, G.; Vergne, J.; Tapponnier, P.; Farra, V.; Poupinet, G.; Jiang, M.; Su, H.; Herquel, G.; Paul, A. Teleseismic imaging of subducting lithosphere and Moho offsets beneath western Tibet. Earth Planet. Sci. Lett. 2004, 221, 117–130. [Google Scholar] [CrossRef]
- Tenzer, R.; Bagherbandi, M.; Hwang, C.H.; Chang, E.T.Y. Moho interface modeling beneath Himalayas, Tibet and central Siberia using GOCO02S and DTM2006.0. Special issue on geophysical and climate change studies in Tibet, Xinjiang, and Siberia from satellite geodesy. Terr. Atm. Ocean. Sci. 2013, 24, 581–590. [Google Scholar] [CrossRef]
- Reigber, C.; Bock, R.; Forste, C.; Grunwaldt, L.; Jakowski, N.; Lühr, H.; Schwintzer, P.; Tilgner, C. CHAMP Phase-B Executive Summary; STR96/13; G.F.Z.: Potsdam, Germany, 1996. [Google Scholar]
- Reigber, C.; Schwintzer, P.; Lühr, H. The CHAMP geopotential mission. Boll. Geofis. Teor. Appl. 1999, 40, 285–289. [Google Scholar]
- Reigber, C.; Lühr, H.; Schwintzer, P. CHAMP mission status. Adv. Space Res. 2002, 30, 129–134. [Google Scholar] [CrossRef]
- Tapley, B.D.; Bettadpur, S.; Watkins, M.; Reigber, C. The gravity recovery and climate experiment: Mission overview and early results. Geophys. Res. Lett. 2004, 31, L09607. [Google Scholar] [CrossRef]
- Drinkwater, M.R.; Floberghagen, R.; Haagmans, R.; Muzi, D.; Popescu, A. GOCE: ESA’s First Earth Explorer Core Mission. In Earth Gravity Field from Space-From Sensors to Earth Science; Beutler, G., Ed.; Kluwer Academic: Dordrecht, The Netherlands, 2003; Volume 18, pp. 419–432. [Google Scholar]
- Floberghagen, R.; Fehringer, M.; Lamarre, D.; Muzi, D.; Frommknecht, B.; Steiger, C.; Piñeiro, J.; da Costa, A. Mission design, operation and exploitation of the gravity field and steady-state ocean circulation explorer mission. J. Geod. 2011, 85, 749–758. [Google Scholar] [CrossRef]
- Braitenberg, C.; Zadro, M.; Fang, J.; Wang, Y.; Hsu, H.T. The gravity and isostatic Moho undulations in Qinghai-Tibet plateau. J. Geodyn. 2000, 30, 489–505. [Google Scholar] [CrossRef]
- Braitenberg, C.; Pettenati, F.; Zadro, M. Spectral and classical methods in the evaluation of Moho undulations from gravity data: The N-E Italian Alps and isostasy. J. Geodyn. 1997, 23, 5–22. [Google Scholar] [CrossRef]
- Braitenberg, C.; Drigo, R. A crustal model from gravity inversion in Karakorum. In Proceedings of the International Symposium on Current Crustal Movement and Hazard Reduction in East Asia and Soth-East Asia, Wuhan, China, 4–7 November 1997; pp. 325–341. [Google Scholar]
- Caporali, A. Buckling of the lithosphere in western Himalaya: Constraints from gravity and topography data. J. Geophys. Res. 2000, 105, 3103–3113. [Google Scholar] [CrossRef]
- Shin, Y.H.; Shum, C.K.; Braitenberg, C.; Lee, S.M.; Na, S.-H.; Choi, K.S.; Hsu, H.; Park, Y.-S.; Lim, M. Moho topography, ranges and folds of Tibet by analysis of global gravity models and GOCE data. Sci. Rep. 2015, 5, 11681. [Google Scholar] [CrossRef]
- Tenzer, R.; Bagherbandi, M.; Sjöberg, L.E.; Novák, P. Isostatic crustal thickness under the Tibetan Plateau and Himalayas from satellite gravity gradiometry data. Earth Sci. Res. J. 2015, 19, 97–106. [Google Scholar] [CrossRef]
- Tenzer, R.; Chen, W. Regional gravity inversion of crustal thickness beneath the Tibetan plateau. Earth Sci. Inform. 2014, 7, 265–276. [Google Scholar] [CrossRef]
- Xu, C.; Liu, Z.; Luo, Z.; Wu, Y.; Wang, H. Moho topography of the Tibetan Plateau using multiscale gravity analysis and its tectonic implications. J. Asian Earth Sci. 2017, 138, 378–386. [Google Scholar] [CrossRef]
- Parker, R.L. The rapid calculation of potential anomalies. Geophys. J. R. Astr. Soc. 1972, 31, 447–455. [Google Scholar] [CrossRef]
- Oldenburg, D.W. The inversion and interpretation of gravity anomalies. Geophysics 1974, 39, 526–536. [Google Scholar] [CrossRef]
- Tenzer, R.; Vajda, P. A global correlation of the step-wise consolidated crust-stripped gravity field quantities with the topography, bathymetry, and the CRUST 2.0 Moho boundary. Contr. Geophys. Geod. 2009, 39, 133–147. [Google Scholar] [CrossRef]
- Tenzer, R.; Bagherbandi, M. Theoretical deficiencies of isostatic schemes in modelling the crustal thickness along the convergent continental tectonic plate boundaries. J. Earth Sci. 2016, 27, 1045–1053. [Google Scholar] [CrossRef]
- Tenzer, R.; Chen, W.; Jin, S. Effect of the upper mantle density structure on the Moho geometry. Pure App. Geophys. 2015, 172, 1563–1583. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, Y.; Wang, G.; Teng, J.; Klemperer, S.; Li, J.; Fan, J.; Chen, Y. East-west crustal structure and “down-bowing” Moho under the northern Tibet revealed by wide-angle seismic profile. Sci. Chin. Ser. D Earth Sci. 2002, 45, 550–558. [Google Scholar] [CrossRef]
- Li, X.; Wei, D.; Yuan, X.; Kind, R.; Kumar, P.; Zhou, H. Details of the doublet Moho structure beneath Lhasa, Tibet, obtained by comparison of P and S receiver functions. Bull. Seismol. Soc. Am. 2011, 101, 1259–1269. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, Y.; Houseman, G.A.; Xu, T.; Wu, Z.; Yuan, X.; Chen, Y.; Tian, X.; Bai, Z.; Teng, J. The Moho beneath western Tibet: Shear zones and eclogitization in the lower crust. Earth Planet. Sci. Lett. 2014, 408, 370–377. [Google Scholar] [CrossRef]
- Teng, J.; Zhang, Z.; Zhang, X.; Wang, C.; Gao, R.; Yang, B.; Qiao, Y.; Deng, Y. Investigation of the Moho discontinuity beneath the Chinese mainland using deep seismic sounding profiles. Tectonophysics 2013, 609, 202–216. [Google Scholar] [CrossRef]
- Baranov, A.; Tenzer, R.; Bagherbandi, M. Combined gravimetric-seismic crustal model for Antarctica. Surv. Geophys. 2018, 39, 23–56. [Google Scholar] [CrossRef]
- Vening Meinesz, F.A. Une nouvelle méthode pour la réduction isostatique régionale de Í intensité de la pesanteur. Bull. Geod. 1931, 29, 33–51. [Google Scholar] [CrossRef]
- Moritz, H. The Figure of the Earth; Wichmann: Karlsruhe, Germany, 1990. [Google Scholar]
- Sjöberg, L.E. Solving Vening Meinesz-Moritz inverse problem in isostasy. Geophys. J. Int. 2009, 179, 1527–1536. [Google Scholar] [CrossRef]
- Sjöberg, L.E. On the isostatic gravity anomaly and disturbance and their applications to Vening Meinesz-Moritz inverse problem of isostasy. Geophys. J. Int. 2013, 193, 1277–1282. [Google Scholar] [CrossRef]
- Sjöberg, L.E.; Bagherbandi, M. A method of estimating the Moho density contrast with a tentative application by EGM08 and CRUST2.0. Acta Geophys. 2011, 58, 1–24. [Google Scholar] [CrossRef]
- Mitra, S.; Priestley, K.; Bhattacharyya, A.; Gaur, V. Crustal Structure and Earthquake Focal Depths Beneath Norteastern India and Southern Tibet. Geophys. J. Int. 2005, 160, 227–248. [Google Scholar] [CrossRef]
- Mitra, S.; Bhattacharya, S.N.; Nath, S.K. Crustal structure of the Western Bengal Basin from joint analysis of teleseismic receiver functions and Rayleigh wave dispersion. Bull. Seismol. Soc. Am. 2008, 98, 2715–2723. [Google Scholar] [CrossRef]
- Maggi, A.; Jackson, J.; Priestley, K.; Baker, C. A Reassessment of Focal Depth Distributions in Southern Iran, the Tien Shan and Northern India: Do Earthquakes Really Occur in the Continental Mantle? Geophys. J. Int. 2000, 143, 629–661. [Google Scholar] [CrossRef]
- Mandal, P. Sedimentary and Crustal Structure Beneath Kachchh and Saurashtra Regions, Gujarat, India. Phys. Earth Planet. Int. 2006, 155, 286–299. [Google Scholar] [CrossRef]
- Kumar, R.; Saul, J.; Sarkar, D.; Kind, R.; Shulda, A. Crustal Structure of the Indian Shield: New Constraints from teleseismic receiver functions. Geophys. Res. Lett. 2001, 28, 1339. [Google Scholar] [CrossRef]
- Priestley, K.; Jackson, J.; McKenzie, D. Lithospheric Structure and Deep Earthquakes Beneath India, the Himalaya and Southern Tibet. Geophys. J. Int. 2008, 172, 345–362. [Google Scholar] [CrossRef]
- Saikia, C. Modeling of the 21 May 1997 Jabalpur Earthquake in Central India: Source Parameters and Regional Path Calibration. Bull. Seismol. Soc. Am. 2006, 96, 1396–1421. [Google Scholar] [CrossRef]
- Jagadeesh, J.; Rai, S. Thickness, composition, and evolution of the Indian Precambrian crust inferred from broadband seismological measurements. Precambrian Res. 2008, 162, 4–15. [Google Scholar] [CrossRef]
- Kayal, J.R.; Srivastava, V.K.; Kumar, P.; Chatterjee, R.; Khan, P.K. Evaluation of crustal and upper mantle structures using receiver function analysis: ISM broadband observatory data. J. Geol. Soc. India 2011, 78, 76–80. [Google Scholar] [CrossRef]
- Acton, C.E.; Priestley, K.; Mitra, S.; Gaur, V.K. Crustal structure of the Darjeeling–Sikkim Himalaya and Southern Tibet. Geophys. J. Int. 2011, 184, 829–852. [Google Scholar] [CrossRef]
- Li, A.; Mashele, B. Crustal structure in the Pakistan Himalaya from teleseismic receiver functions. Geochem. Geophys. Geosyst. 2009, 10, Q12010. [Google Scholar] [CrossRef]
- Li, Y.; Gao, M.; Wu, Q. Crustal thickness map of the Chinese mainland from teleseismic receiver functions. Tectonophysics 2014, 611, 51–60. [Google Scholar] [CrossRef]
- Baranov, A. A new crustal model for Central and Southern Asia. Izvest. Phys. Solid Earth 2010, 46, 34–46. [Google Scholar] [CrossRef]
- Li, S.; Mooney, W.; Fan, J. Crustal Structure of Mainland China from Deep Seismic Sounding Data. Tectonophysics 2006, 420, 239–252. [Google Scholar] [CrossRef]
- He, R.; Shang, X.; Yu, CH.; Zhang, H.; Van der Hilst, R. A unified map of Moho depth and Vp/Vs ratio of continental China by receiver function analysis. Geophys. J. Int. 2014, 199, 1910–1918. [Google Scholar] [CrossRef]
- Yue, H.; Chen, Y.J.; Sandvol, E.; Ni, J.; Hearn, T.; Zhou, S.; Feng, Y.; Ge, Z.; Trujillo, A.; Wang, Y.; Jin, G. Lithospheric and upper mantle structure of the northeastern Tibetan Plateau. J. Geophys. Res. 2012, 117, B05307. [Google Scholar] [CrossRef]
- Murodov, D.; Zhao, J.; Xu, Q.; Liu, H.; Pei, S. Complex N–S variations in Moho depth and Vp/Vs ratio beneath the western Tibetan Plateau as revealed by receiver function analysis. Geophys. J. Int. 2018, 214, 895–906. [Google Scholar] [CrossRef]
- Jia, S.; Zhang, X.; Zhao, J.; Wang, F.; Zhang, C.; Xu, Z.; Pan, J.; Liu, Z.; Pan, S.; Sun, G. Deep seismic sounding data reveal the crustal structures beneath Zoigê basin and its surrounding folded orogenic belts. Sci China Earth Sci. 2010, 53, 203–212. [Google Scholar] [CrossRef]
- Liu, Q.M.; Zhao, J.M.; Lu, F.; Liu, H. Crustal structure of northeastern margin of the Tibetan Plateau by receiver function inversion. Sci China Earth Sci. 2014, 57, 741. [Google Scholar] [CrossRef]
- Xu, X.M.; Ding, Z.F.; Shi, D.N.; Li, X.F. Receiver function analysis of crustal structure beneath the eastern Tibetan plateau. J. Asian Earth Sci. 2013, 73, 121–127. [Google Scholar] [CrossRef]
- Wang, C.Y.; Han, W.B.; Wu, J.P.; Lou, H.; Chan, W.W. Crustal structure beneath the eastern margin of the Tibetan Plateau and its tectonic implications. J. Geophys. Res. 2007, 112, B07307. [Google Scholar] [CrossRef]
- Xu, X.; Li, H.Y.; Gong, M.; Ding, Z.F. Three-dimensional S-Wave velocity structure in eastern Tibet from ambient noise Rayleigh and love wave tomography. J. Earth Sci. 2011, 22, 195–204. [Google Scholar] [CrossRef]
- Zhang, Z.J.; Yuan, X.H.; Chen, Y.; Tian, X.B.; Kind, R.; Li, X.Q.; Teng, J.W. Seismic signature of the collision between the east Tibetan escape flow and the Sichuan Basin. Earth Planet. Sci. Lett. 2010, 292, 254–264. [Google Scholar] [CrossRef]
- Xu, L.; Rondenay, S.; van der Hilst, R.D. Structure of the crust beneath the southeastern Tibetan Plateau from teleseismic receiver functions. Phys. Earth Planet. Int. 2007, 165, 176–193. [Google Scholar] [CrossRef]
- Xu, Q.; Zhao, J.M.; Cui, Z.X.; Pei, S.P.; Liu, H.B. Moho offset beneath the central Bangong–Nujiang suture of Tibetan Plateau. Chin. Sci. Bull. 2010, 55, 607–613. [Google Scholar] [CrossRef]
- Tong, W.; Wang, L.; Mi, N.; Ming, H.; Li, H.; Yu, D.; Li, C.; Liu, S.; Liu, M.; Sandvol, E. Receiver function analysis for seismic structure of the crust and uppermost mantle in the Liupanshan area, China. Sci. Chin. Ser. D Earth Sci. 2007, 50, 227. [Google Scholar] [CrossRef]
- Gao, Z.; Zhao, Y.; Deng, X.; Yang, Y. Crustal structure along the Zhenkang–Luxi deep seismic sounding profile in Yunnan derived from receiver functions. Geod. Geodyn. 2018, 9, 334–341. [Google Scholar] [CrossRef]
- Yan, Q.; Zhang, G.; Kan, R.; Hu, H. The crustal structure of Simao to Malong profile, Yunnan province, China. J. Seism. Res. 1985, 8, 249–280. [Google Scholar]
- Wang, C.; Lou, H.; Wang, X.; Qin, J.; Yang, R.; Zhao, J. Crustal structure in Xiaojiang fault zone and its vicinity. Earth Sci. 2009, 22, 347–356. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y. Crustal and upper mantle velocity structure in Yunnan, Southwest China. Tectonophysics 2009, 471, 171–185. [Google Scholar] [CrossRef]
- Förste, C.; Bruinsma, S.L.; Abrikosov, O.; Lemoine, J.-M.; Marty, J.C.; Flechtner, F.; Balmino, G.; Barthelmes, F.; Biancale, R. EIGEN-6C4—The Latest Combined Global Gravity Field Model Including GOCE Data up to Degree and Order 2190 of GFZ Potsdam and GRGS Toulouse; GFZ Data Services; Tapley, B.D., Bettadpur, S., Watkins, M., Eds.; GFZ German Research Centre for Geosciences: Potsdam, Germany, 2014. [Google Scholar]
- Moritz, H. Geodetic Reference System 1980. J. Geod. 2000, 74, 128–162. [Google Scholar] [CrossRef]
- Heiskanen, W.A.; Moritz, H. Physical Geodesy; W.H. Freeman and Co.: San Francisco, CA, USA; London, UK, 1967. [Google Scholar]
- Tenzer, R.; Vajda, P. Global maps of the CRUST2.0 components stripped gravity disturbances. J. Geophys. Res. 2009, 114, B05408. [Google Scholar] [CrossRef]
- Tenzer, R.; Gladkikh, V.; Vajda, P.; Novák, P. Spatial and spectral analysis of refined gravity data for modelling the crust-mantle interface and mantle-lithosphere structure. Surv. Geophys. 2012, 33, 817–839. [Google Scholar] [CrossRef]
- Tenzer, R.; Chen, W.; Tsoulis, D.; Bagherbandi, M.; Sjöberg, L.E.; Novák, P.; Jin, S. Analysis of the refined CRUST1.0 crustal model and its gravity field. Surv. Geophys. 2015, 36, 139–165. [Google Scholar] [CrossRef]
- Hirt, C.; Rexer, M. Earth2014: 1 arc-min shape, topography, bedrock and ice-sheet models—Available as gridded data and degree-10,800 spherical harmonics. Int. J. Appl. Earth Obs. Geoinf. 2015, 39, 103–112. [Google Scholar] [CrossRef]
- Gladkikh, V.; Tenzer, R. A mathematical model of the global ocean saltwater density distribution. Pure Appl. Geophys. 2011, 169, 249–257. [Google Scholar] [CrossRef]
- Tenzer, R.; Novák, P.; Gladkikh, V. The bathymetric stripping corrections to gravity field quantities for a depth-dependent model of the seawater density. Mar. Geod. 2012, 35, 198–220. [Google Scholar] [CrossRef]
- Tenzer, R.; Vajda, P.; Hamayun, P. A mathematical model of the bathymetry-generated external gravitational field. Contrib. Geophys. Geod. 2010, 40, 31–44. [Google Scholar] [CrossRef]
- Cutnell, J.D.; Kenneth, W.J. Physics, 3rd ed.; Wiley: New York, NY, USA, 1995. [Google Scholar]
- Laske, G.; Masters, G.; Ma, Z.; Pasyanos, M.E. Update on CRUST1.0—A 1-degree global model of Earth’s crust. Geophys. Res. Abstr. 2013, 15, 2658. [Google Scholar]
- Tenzer, R.; Vajda, P.; Hamayun, P. Global atmospheric corrections to the gravity field quantities. Contr. Geophys. Geod. 2009, 39, 221–236. [Google Scholar] [CrossRef]
- Molnar, P.; Tapponnier, P. Cenozoic tectonics of Asia: Effects of a continental collision. Science 1975, 189, 419–426. [Google Scholar] [CrossRef] [PubMed]
- Dewey, J.F.; Cande, S.; Pitman, W.C. Tectonic evolution of the Indian/Eurasia collision zone. Eclog. Geol. Helv. 1989, 82, 717–734. [Google Scholar]
- Kanao, M.; Fujiwara, A.; Miyamachi, H.; Toda, S.; Tomura, M.; Ito, K.; Ikawa, T. Reflection imaging of the crust and the lithospheric mantle in the Lützow–Holm Complex, Eastern Dronning Maud Land, Antarctica, derived from the SEAL Transects. Tectonophysics 2011, 508, 73–84. [Google Scholar] [CrossRef]
- Zhu, L.; Kanamori, H. Moho depth variation in Southern California from teleseismic receiver functions. J. Geophys. Res. 2000, 105, 2969–2980. [Google Scholar] [CrossRef]
- Hansen, S.; Nyblade, A.; Pyle, M.; Wiens, D.; Anandakrishnan, S. Using S wave receiver functions to estimate crustal structure beneath ice sheets: An application to the Transantarctic Mountains and East Antarctic craton. Geoch. Geophys. Geosyst. 2009, 10, Q08014. [Google Scholar] [CrossRef]
- Danesi, S.; Morelli, A. Structure of the upper mantle under the Antarctic Plate from surface wave tomography. Geophys. Res. Lett. 2001, 28, 4395–4398. [Google Scholar] [CrossRef]
- Ritzwoller, M.H.; Shapiro, N.M.; Levshin, A.L.; Leahy, G.M. Crustal and upper mantle structure beneath Antarctica and surrounding oceans. J. Geophys. Res. B 2001, 106, 30645–30670. [Google Scholar] [CrossRef]
- Pail, R.; Goiginger, H.; Schuh, W.-D.; Höck, E.; Brockmann, J.M.; Fecher, T.; Gruber, T.; Mayer-Gürr, T.; Kusche, J.; Jäggi, A.; et al. Combined satellite gravity field model GOCO01S derived from GOCE and GRACE. Geophys. Res. Lett. 2010, 37, L20314. [Google Scholar] [CrossRef]
- Pail, R.; Bruinsma, S.; Migliaccio, F.; Förste, C.; Goiginger, H.; Schuh, W.-D.; Höck, E.; Reguzzoni, M.; Brockmann, J.M.; Abrikosov, O.; et al. First GOCE gravity field models derived by three different approaches. J. Geod. 2011, 85, 819–843. [Google Scholar] [CrossRef]



| Gravity Disturbance | Min (mGal) | Max (mGal) | Mean (mGal) | STD (mGal) |
|---|---|---|---|---|
| Free-air | −195 | 177 | 23 | 45 |
| Bouguer | −483 | 436 | 54 | 185 |
| Isostatic | −945 | -353 | -614 | 94 |
| Moho Model | Min (km) | Max (km) | Mean (km) | STD (km) |
|---|---|---|---|---|
| Seismic | 8.0 | 75.8 | 31.1 | 13.9 |
| Gravimetric | 9.7 | 85.9 | 46.3 | 12.0 |
| Combined | 11.7 | 76.3 | 46.5 | 10.9 |
| CRUST1.0 | 10.0 | 74.8 | 44.7 | 9.8 |
| Moho Differences | Min (km) | Max (km) | Mean (km) | RMS (km) |
|---|---|---|---|---|
| Seismic-Combined | −10.3 | 10.9 | 0.3 | 3.2 |
| Seismic-CRUST1.0 | −14.5 | 19.9 | 2.0 | 5.1 |
| Gravimetric-CRUST1.0 | −13.2 | 15.1 | 1.5 | 5.1 |
| Combined-CRUST1.0 | −9.0 | 12.5 | 1.7 | 4.0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baranov, A.; Bagherbandi, M.; Tenzer, R. Combined Gravimetric-Seismic Moho Model of Tibet. Geosciences 2018, 8, 461. https://doi.org/10.3390/geosciences8120461
Baranov A, Bagherbandi M, Tenzer R. Combined Gravimetric-Seismic Moho Model of Tibet. Geosciences. 2018; 8(12):461. https://doi.org/10.3390/geosciences8120461
Chicago/Turabian StyleBaranov, Alexey, Mohammad Bagherbandi, and Robert Tenzer. 2018. "Combined Gravimetric-Seismic Moho Model of Tibet" Geosciences 8, no. 12: 461. https://doi.org/10.3390/geosciences8120461
APA StyleBaranov, A., Bagherbandi, M., & Tenzer, R. (2018). Combined Gravimetric-Seismic Moho Model of Tibet. Geosciences, 8(12), 461. https://doi.org/10.3390/geosciences8120461





