Co-Extrusive Magma Transport and Volcanic Dome Formation: Implications for Triggering Explosive Volcanic Eruptions

Abstract

Polymer co-extrusion experiments are described simulating the dynamics of two different magmas (e.g., silicic and mafic having different viscosities) flowing simultaneously in a vertical volcanic pipe or conduit which results in the effusion of composite lava domes on the surface. These experiments, involving geologically realistic conduit length-to-diameter aspect ratios of 130:1 or 380:1, demonstrate that co-extrusion of magmas having different viscosities can explain not only the observed normal zoning observed in planar dikes and the pipelike conduits that evolve from dikes but also the compositional layering of effused lava domes. The new results support earlier predictions, based on observations of induced core-annular flow (CAF), that dike and conduit zoning along with dome layering are found to depend on the viscosity contrast of the non-Newtonian (shear-thinning) magmas. Any magma properties creating viscosity differences, such as crystal content, bubble content, water content and temperature may also give rise to the CAF regime. Additionally, codependent flow behavior involving the silicic and mafic magmas may play a significant role in modifying the nature of volcanic eruptions. For example, lubrication of the flow by an annulus of a more mafic, lower-viscosity component allows a more viscous but more volatile-charged magma to be injected rapidly to greater vertical distances along a dike into a lower pressure regime that initiates exsolving of a gas phase, further assisting ascent to the surface. The rapid ascent of magmas exsolving volatiles in a dike or conduit is associated with explosive silicic eruptions.

1. Introduction

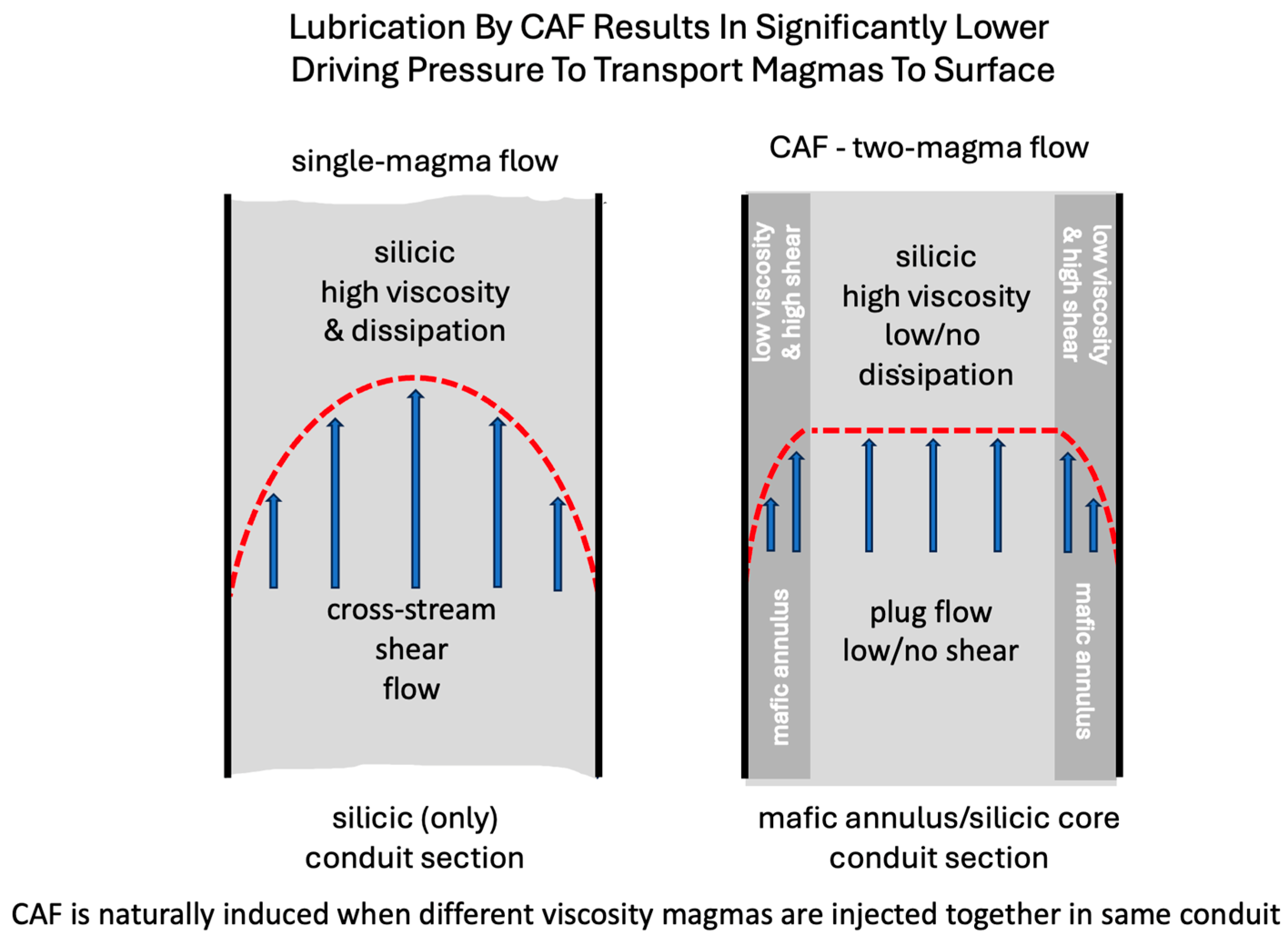
1.1. Normal Zoning of Mafic and Silicic Magmas in Conduits, and Core-Annular Flow (CAF)

Normal zoning or layering is a common mafic-margin (lower silica content) and silicic-core (higher silica content) arrangement of igneous rocks in volcanic dikes (vertical trending magma-filled fractures or channels) and pipelike conduits. The contrast in silica content, here correlated with viscosity, can be relatively large, as in the occurrence of basalts (lower silica) and rhyolites (higher silica) together. Petrologic evidence strongly supports the view that these rocks were both in the liquid state at the time that the multi-layered or composite domes were formed in the East Volcanic Zone of Iceland (Gibson and Walker [1], Walker and Skelhorn [2], Blake and Campbell [3]). This has also been found where the chemical contrast and hence inferred viscosity contrast is smaller, as in the case of the cored conduit of the Obsidian Dome rhyodacite-rhyolite flow in Long Valley, California (Vogel et al. [4]; Carrigan and Eichelberger [5]). Sequential emplacement of the mafic and silicic components has occasionally been suggested as the cause of this chemical or compositional zoning ([2,3]). However, the transport of a mafic component rapidly followed by a silicic magma often fails to explain surface observations of composite lava flows (e.g., overlying layer plan views that are exactly coincidental). These are better understood by the simultaneous co-extrusion of viscous and less viscous components (Carrigan [6]). Unfortunately, the details of magma transport and emplacement dynamics are typically not observable during the emplacement of the resulting volcanic edifice. Furthermore, subsurface flow processes in dikes or conduits must necessarily be inferred, after the fact, either through drilling and coring the surface flow and its associated dike/conduit [4] or by finding conduit and dome structures that have been fortuitously emplaced and subjected to sufficient erosion to allow geological mapping [1,2].

Well-designed analog experiments offer one means of probing fluid dynamics pertinent to magma transport and eruption at the surface. While analog experiments are not new to exploring the fluid dynamics of volcanism (see reviews by Kavanaugh et al. [7]; Poppe et al. [8]), previous experimental work considering the low-Reynolds number co-extrusion of two or more fluids having contrasting viscosities injected into pipes or conduits have largely focused on industrial applications involving polymer co-extrusion (e.g., Han [9]).

In this article, we consider new, analog co-extrusion experiments, with geologically realistic length-to-diameter aspect ratios of 130:1 or 380:1, having the objective of validating an earlier magmatic transport model for two-component co-extrusive, self-lubricated or encapsulated flow ([5,6]). Also called core-annular-flow (CAF), the lower-viscosity component naturally encapsulates the higher-viscosity component of the flow isolating it from the high-shear flow zones adjacent to conduit or dike walls. This results in significant drag reduction during transport between the magma chamber and the surface (Figure 1). Furthermore, we show that the layering character of lavas in the resulting volcanic dome is strongly dependent on the viscosity contrast between the two magmas. Finally, because magma co-extrusion involves lubrication and the enhancement of the rate of ascent of the bulk flow in the dike or conduit for a given driving pressure exerted at the source, CAF is potentially significant for driving explosive eruptions in volcanic regimes having bimodal/multimodal compositions.

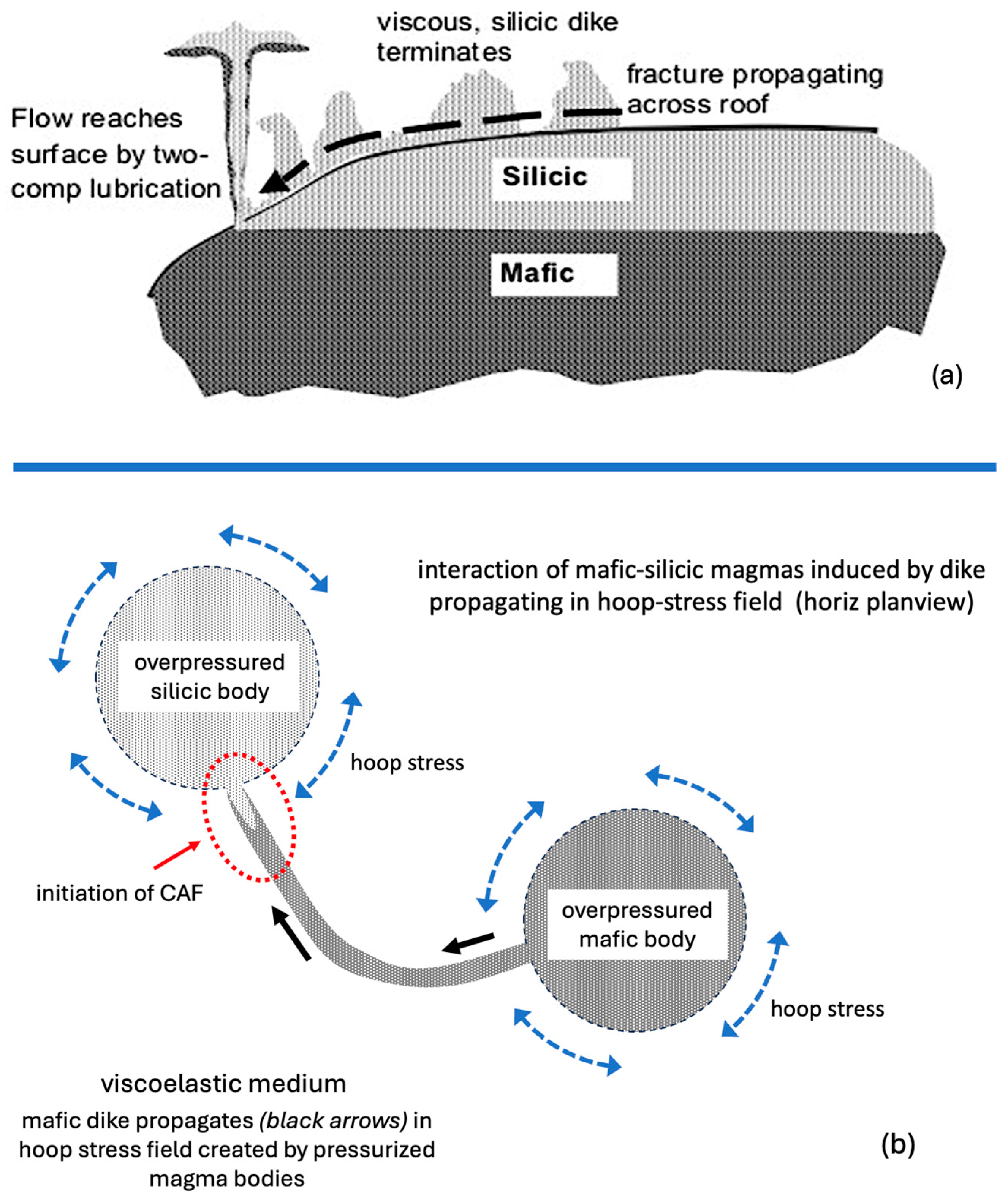
1.2. Dike/Conduit Inlet Condition: Simultaneous Injection of Compositionally Distinct Magmas

A requirement for CAF is that magmas with contrasting viscosity are present in the dike or conduit at the same time, which has been observed in the field ([1,2,5,6]). For a magma chamber in which compositional layering has already evolved, Figure 2a illustrates just one possibility for how the eruption of a magmatic dome might occur. In this case, the sloping part of a chamber roof intersects multiple layers while the horizontal part of the ceiling comes into contact with typically only one layer. For fractures propagating along horizontal parts of the chamber boundary, only high withdrawal rates can produce the entrainment of different layers required for simultaneous, two-component flow to occur (Trial et al. [10]). However, for even very small withdrawal rates, both layers can be drawn into a dike or fracture on a sloping boundary that intersects both the silicic and underlying mafic magma layers. Thus, such an intersection gives rise to the possibility of co-injection of the mafic and silicic magmas and the onset of CAF. Figure 2a additionally suggests that injection of highly viscous silicic magma alone into a propagating dike may be unsuccessful in reaching the surface, as a result of its very high viscosity, until it finally intersects a silicic-mafic interface allowing CAF to form and lubricated flow to occur.

Alternatively, a recent experimental study on dike propagation (Pansino and Taisne [11]) indicates that mafic and silicic magmas, which have evolved separately, may potentially be co-mingled and flow together in the same dike (Figure 2b). The experimental study found that a propagating analog dike is “attracted” by the hoop-stress field created by an over-pressured fluid-filled body embedded in a viscoelastic medium. The authors further found that such dikes breaching a magmatic reservoir do not necessarily cease propagation once they have connected to the over-pressured body, and their analog dike, after contacting the pressurized body, continued to propagate. They concluded that the silicic magma in a reservoir would be equally or more buoyant than the magma in a propagating mafic dike. It could therefore flow inside of the dike and take advantage of the existing fracture to move toward the surface. Such a model for co-mingling two magmas may be more pertinent to the basalt-rhyolite domes mapped in eastern Iceland [1] than a layered magma body with a sloping boundary. Urbani et al. [12] also performed a series of lab experiments that produced an additional understanding of conditions characterizing the viscoelastic medium causing vertical versus horizontal propagation of a dike, which can affect the probability of eruption.

These two possible models for bringing magmas together in a dike or conduit consider the cases where the contrasting magmas have either evolved together in the same chamber or have evolved separately. For this paper, it suffices to conclude that the co-injection of different magmas into a conduit or dike is reasonable, and no preference is given here regarding the origin of contrasting magmas in the discussion that follows.

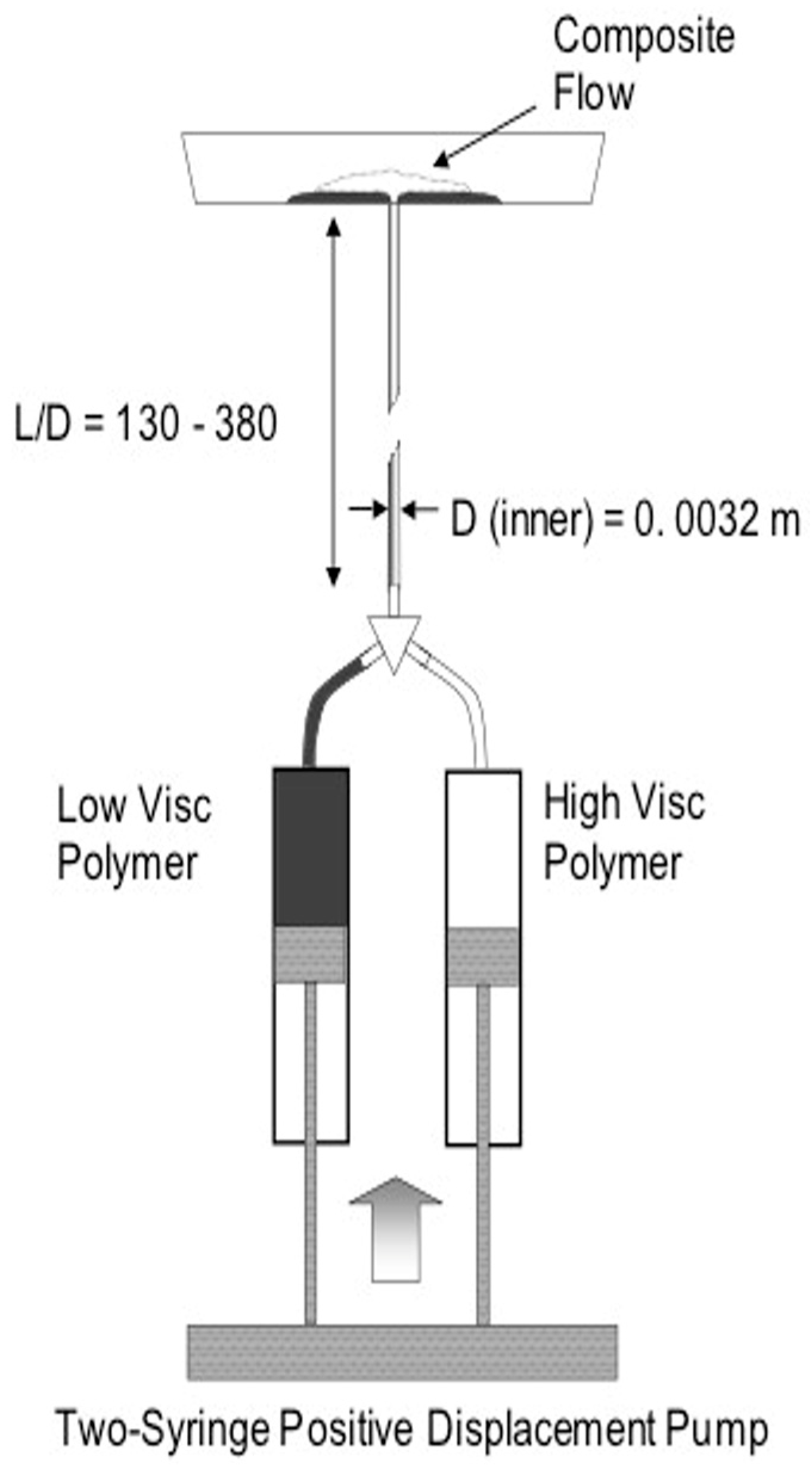
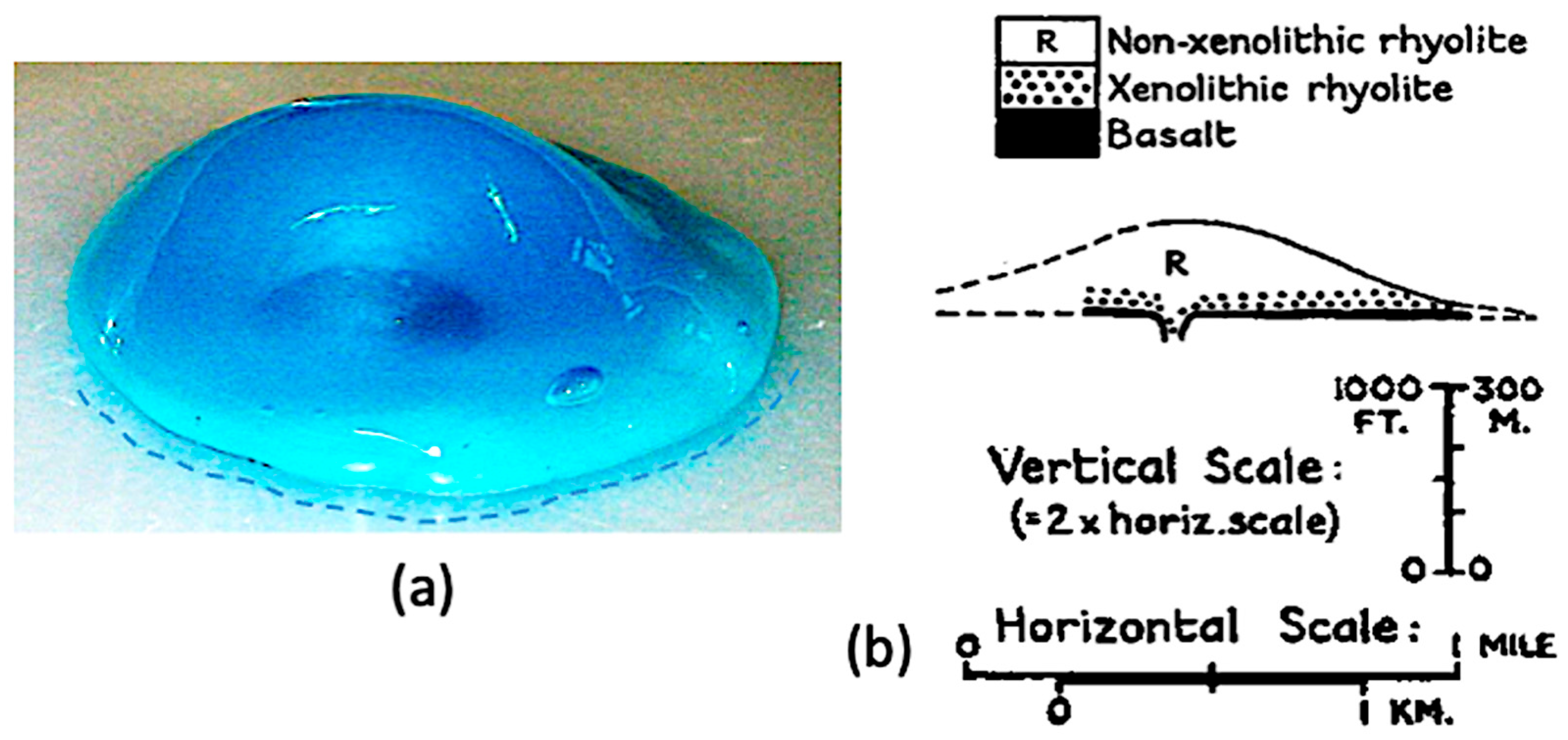
2. Materials and Methods

Experiment Simulating Two-Component Flow in a Vertical Conduit or Dike

Given a laboratory experiment that is scaled to simulate the geometry of a conduit as well as the flow behavior of different magmas flowing together, it is the degree of dynamic similitude that determines the level of correspondence between flows in the experiment and the magmatic case. For the miscible flows to be dynamically similar, the ratios of the magnitudes of different fluid-dynamic forces must be comparable in both the laboratory and magmatic regimes. One ratio that is particularly important for relating the results of laboratory co-extrusion experiments to two-component magma transport is given by the Reynolds number, Re, which compares the characteristic magnitude of inertial forces in the flow to viscous forces. Very small Reynolds numbers, that is, values that are much less than one, indicate that viscous forces dominate inertial forces as is anticipated for magmatic effusion. Typical estimates of the Reynolds number for polymeric co-extrusion experiments are less than unity. For example, in the set of CAF-extrusion experiments described here approximate values of the flow speed (u), polymer-melt kinematic viscosity (ν) and capillary-tube diameter (d) are 0.02 m/s, 0.01 m²/s and 0.0032 m, respectively. These quantities yield a Reynolds number Re = (ud/ν) of about 0.03 << 1. Other co-extrusion experiments referred to here ([9]) are characterized by comparable Reynolds numbers of about 0.001–0.005. Such values imply dynamical similarity with a 0.003–0.1 m/s flow of rhyolitic magma (ν = 10 m²/s) in a three-meter dike (d)—a most reasonable description for an effusive flow regime. In this range of Reynolds number, the earlier industrially motivated polymer—co-extrusion experiments show that a CAF transition occurs during co-extrusion into cylindrical dies with length-to-diameter ratios of only about 20:1 implying that the CAF regime will be initiated at an early stage during flow along a magma conduit.

Figure 3 illustrates the setup with a dual-syringe, positive displacement pump feeding two separate miscible polymer streams into the bottom end of a high-aspect-ratio (tube length/inner diameter = 130–380:1) vertical tube. The outlet end of the tube penetrates the bottom of a pan acting as a surface onto which the composite polymer flows. The polymer used is Naturosol HHR™, a hydroxyethylcellulose powder that hydrates in dilute (0.5–3%) aqueous solutions to form a highly viscous, shear-thinning (non-Newtonian) liquid. Higher concentrations are very weakly negatively buoyant in lower concentrations of the solution. While shear-thinning behavior has also been identified in magmas, shear-dependent rheology is not a requirement for the CAF transition to occur. For sufficiently low shear rates, higher-concentration solutions of Naturosol exhibit a pseudo-plastic behavior broadly similar to the character of more viscous magmas subjected to low shear stresses. The viscous and shear-thinning behavior of Naturosol solutions has been evaluated by Jones et al. [13]. No attempt has been made to match actual magmatic viscosities or their shear-thinning nature other than to show that a viscosity contrast and non-Newtonian behavior in a scaled conduit geometry can simulate both observed subsurface and surface mafic/silicic zoning. Several experiments were performed in which the effect of viscosity contrast was evaluated using colored, high- and low-viscosity, miscible solutions simultaneously injected into the bottom of the vertical tube and then allowed to flow through the vent and out onto the surface of the pan at the top (Figure 3) simulating a volcanic conduit breaching the surface.

3. Results

3.1. Co-Extrusion Involving Fluids Having Same Viscosity

To examine the limit of small-to-vanishing viscosity contrast, two miscible, polymeric solutions with the same viscosity were co-extruded side-by-side. Figure 4 shows the flow that was obtained. Because of the equal viscosities, no core-annular flow developed along the length of the tube (130:1). The flow onto the surface shows no evidence of vertical layering of the type characterizing Inyo Domes. Rather, the flow is layered in the horizontal with the polymer solutions flowing away from each other at the vent. In this case, the side of the vent that each stream exits from corresponds to the side of the tube into which each iso-viscous fluid was injected at the bottom. Some mixing of the miscible liquids occurs along the length of the tube as is indicated by the intermediate green zone made up of mixing some of the yellow and blue components. It is important to note that mixing is limited to only a fraction of the polymers vented. (Some mixing may occur on the surface during flow from the vent). When the viscosities are drastically different, the mixing is less even though the liquids remain miscible.

3.2. Co-Extrusion Involving Fluids Having Contrasting Viscosity

Co-extrusion of polymers with an extreme viscosity contrast (0.5% and 3% solutions) produces a somewhat different result. The core-annular flow regime now develops quickly in the vertical tubing of the experiment (380:1 aspect-ratio) with the high-viscosity component (originally yellow but appears green when overlain by blue annular fluid) forming the core of the flow which is encapsulated by the lower viscosity component that forms an annular zone adjacent to the conduit wall (Figure 5a, bottom). A photo-enhanced section of the tubing illustrates more clearly the non-uniform boundary between the annular and core regions of the flow (Figure 5a’). On the surface of the pan, the lower viscosity (blue) component becomes a vehicle to transport the higher viscosity component that is too viscous to flow from the vent alone in the 380:1 geometry (Figure 5a, top). The higher viscosity component is pseudo-plastic producing a broken or “patchy” appearance after ejection from the core of the conduit flow. This sluggish, overlying, non-Newtonian, high-viscosity layer is carried out away from the vent on the surface breaking up into patches when it is stretched too thin by the faster-flowing underlying layer. As it is carried outward to the advancing edge of the flow by the underlying lower-viscosity component, the high-viscosity material is observed to fall before the advancing front with the top layer rolling under the lower layer at the edge of the dome as it moves outward (Figure 5b). This results in a third, base layer that may be a mixture of the other two (Figure 5b). Analyses of drill cores that captured the layering of a dome and its associated conduit in Long Valley, California support the model proposed here (Figure 6). If lower silica content in rock samples implies lower viscosity during the flow of magma in the conduit, then the distribution of silica content measured in the conduit, as shown in the lower plot of Figure 6, would be expected if core-annular flow in the conduit occurred during the eruption of the dome. After venting at the surface, the measured silica distribution in the drill cores taken from the dome indicates that the higher viscosity layer appears to mix with the lower viscosity layer presumably as a result of blocks of breccia being carried over the edge or front of the advancing lower-viscosity flow and then falling beneath it (Figure 6, upper plot).

The effect of self-lubrication by the lower viscosity component is highly apparent. Intermediate viscosity solutions (2% Naturosol™ solutions) are the upper limit for polymers that can be pumped singly by the injection pump used in the experiments described here. In the dual or co-injection mode, lower viscosity polymer (0.5% solution) and very high viscosity polymer (3%) result in a lower drag, core-annular regime that can be driven up the tube much more rapidly than the 2% case by the injection pump motor. The core-annular flow regime can similarly explain how extremely viscous magmas that could not be extruded alone in a limited-pressure magmatic system can reach the surface in the presence of a lower viscosity component.

In our experiment, a moderate viscosity contrast (nominally, 0.5% and 1%) between the co-extruded fluids yields a different doming structure. Rather than the thin, patchy upper layer of very high viscosity non-Newtonian extrudate that is carried away from the vent by the far more mobile extruded lower layer, the upper, more viscous layer builds in volume over the vent as the lower, less viscous layer spreads below it (Figure 7a). However, the upper layer’s moderate viscosity along with its shear-thinning nature allows it also to spread along with the expanding lower layer and even over-run it slightly as a result of the back-pressure of the domed fluid. Regarding the features discussed here, the result (Figure 7a) appears strikingly similar to the volcanic dome (Figure 7b) in the Eastern Iceland Tertiary region mapped by Gibson and Walker [1].

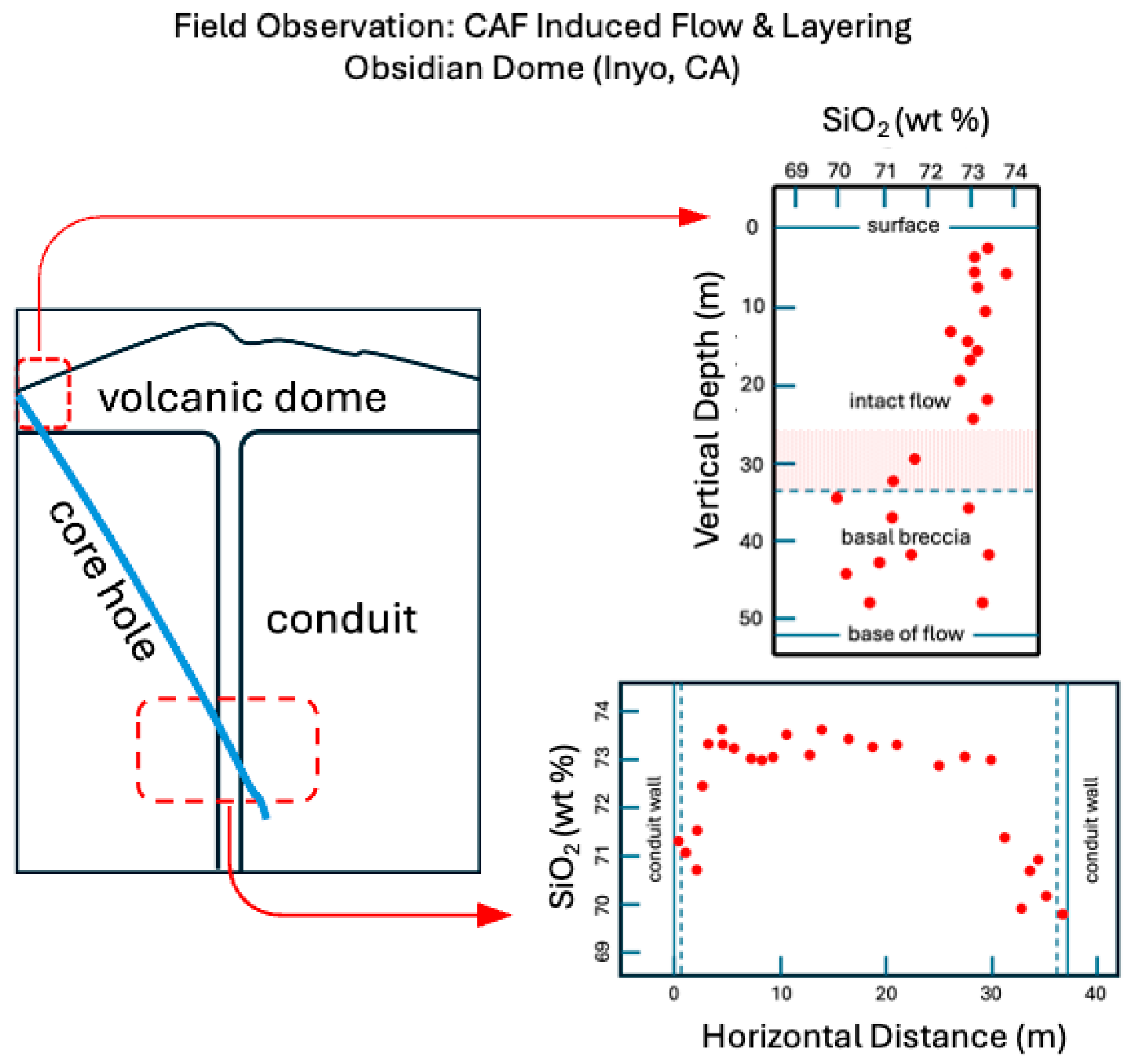
4. Discussion

A dynamical model has now been qualitatively demonstrated spanning the magma transport and dome-formation processes. The results of the experiments clearly show that sequential injections of different magmas are not required to explain vertically zoned lava flows. Gibson and Walker [1] and Carrigan and Eichelberger [5] have both argued that their field observations support a simultaneous two-magma transport process as opposed to sequential injection.

While the occurrence of weak interfacial flow instabilities may produce irregularities of the interface between the annular and core regions, the general CAF structure as shown in Figure 5a,a’ is preserved along the length of the pathway to the surface even in the 380:1 aspect ratio case. Close inspection shows the topography of the interface between the co-extruding polymers resembling the observed interface between mafic and silicic layers (Skelhorn et al. [14]) which can be irregular and may contain enclaves or xenoliths in layered flows. High magmatic viscosities and the relatively small cross-stream length scale (i.e., low Reynolds number) will tend to inhibit large amplitude mixing processes that might effectively destroy the gross normal-zoning features. The stability at the interface between the two magmas may be further enhanced by any local interfacial cooling of the more refractory phase resulting in a “skin” of particularly sluggish magma that will dampen mixing.

What is the effect of cooling on the core-annular regime? Numerical models of cooling fluid with a temperature-dependent viscosity developed using a lattice-gas simulator (Stockman et al. [15]) suggest that cooling of magma at a boundary will cause the magma to gradually become more viscous downstream. This leads to instability within the lubricating layer itself that causes an exchange within the same layer between the cooler and more viscous, “wall” magma and the hotter, lower viscosity “interior” magma of the annular zone. This exchange appears analogous to the mechanism responsible for the formation of core-annular flow involving compositionally distinct magmas except that here we are dealing with cooler and warmer regions of the same magma. Calculations suggest that such an intra-layer exchange instability need only provide a very small transverse component to offset boundary cooling (Carrigan et al. [16]).

Regarding composite flows, our new laboratory observations qualitatively demonstrate that viscosity contrast has an important influence on the nature of layering in volcanic flows. Additionally, the non-Newtonian, shear thinning nature of the polymer solutions in conjunction with viscosity differences also produce secondary doming features (e.g., dome shape, higher viscosity upper layer over-running edge of lower viscosity bottom layer) in agreement with the observations. This gross layering structure (Figure 5), including the underflow of the bottom layer by the top layer (Figure 5b), is borne out by the drilling studies of Obsidian Dome (Figure 6). Of course, even finer details of the flow structure are less easily modeled by the experiments described here. While the Naturosol™ polymer solutions exhibit non-Newtonian, shear-thinning behavior, they cannot exactly replicate the rheological behavior of lavas including the crystallization in the more viscous lavas owing to loss of volatiles during transport.

Lubrication of a viscous magma flowing in a propagating dike by a less viscous magma may also play a significant role in triggering volcanic eruptions. Observations of energetic eruptions involving two compositionally distinct magmas with significantly different inferred viscosities are common although there is no general model for how the bimodal composition produces an eruption (e.g., Pallister et al. [17], Takeuchi and Nakamura [18], Leonard et al. [19], Devine et al. [20]). However, it is generally accepted that viscous losses resulting from magma flow in narrow fractures are far more important than rock-fracture toughness in estimating whether the level of pressurization in a magma chamber is adequate to propagate a dike large distances (Spence and Turcotte [21], Lister and Kerr [22]). With an approximate analytical model, it has already been shown that basalt-lubricated rhyolite can propagate more than 100 times the rate of rhyolite alone for the same magma-chamber-pressure history (Carrigan [6]). While the mafic magma may provide the necessary lubrication for the co-extrusive flow, it may in certain scenarios not contain adequate volatiles for exsolution to reduce the bulk magma density ρ_dike to the level required to enhance the ascent rate of magma in the dike by forming a foam. (The excess inlet pressure available to drive magma upward within a dike is equal to the magma-chamber pressure p_mag, at the inlet, less the pressure associated with the weight of the vertical column of length, l, of magma in the dike subject to gravity, g, that is, p_mag − ρ_dikegl). However, the silicic component can potentially have a higher volatile content that exsolves during the decompression associated with sufficiently rapid transport upward in a dike or conduit (Eichelberger et al. [23], Jaupart and Allegre [24], Mangan et al. [25]). This results in a reduced bulk density ρ_dike and a correspondingly greater driving pressure, p_mag − ρ_dikegl. Again, this is only possible because the wetter silicic magma is enabled, by the lubrication provided by the mafic component, to rise sufficiently fast in the dike (reducing gas loss) to a lower pressure regime where it acquires a lower density owing to exsolution.

Finally, the co-extrusion model for magmas originally proposed by Carrigan and Eichelberger [5] to explain the layering of Obsidian Dome has been criticized on grounds relating to the miscibility of co-extruded magmas and the small Reynolds number associated with effusive transport. Such criticisms (e.g., Koyaguchi and Takada [26]) result from a general misunderstanding of both the CAF transition as well as the less significant dynamical role of miscibility in low Reynolds number, two-component flows. Koyaguchi and Takada have assumed that the CAF transition applies only to larger Reynolds number, immiscible cases such as that characterizing water-oil transport in pipelines (Joseph et al. [27], Preziosi et al. [28]) while overlooking the more dynamically relevant body of research on the successful CAF transition of miscible polymers at low Reynolds number (Han [9], Minigawa and White [29]). The low-Reynolds number, miscible flows described here are the most realistic laboratory evaluations of the magmatic co-extrusive model of dome formation to date. They clearly show the roles of the transition to CAF in a low Reynolds number, miscible flow regime along with the effects of viscosity contrast and shear-thinning rheology on the production of compositionally layered volcanic flows.

5. Conclusions

The main purpose of this study was to identify conditions relevant to magma transport, eruption and the formation of volcanic domes in the context of CAF. To our knowledge, this is the first laboratory effort specifically intended to simulate aspects of magmatic co-extrusion by employing realistic geometry, boundary conditions, flow conditions and differing rheologies of the liquids used in the study. The inlet boundary condition of this experiment simulates what might occur when a dike or conduit is fed by either a layered reservoir containing magmas of contrasting viscosity or two reservoirs containing different magmas (Figure 2a,b). These magma scenarios seem reasonable and permit an injection boundary condition resulting in co-extrusion and CAF. We find that CAF develops rapidly after co-injection of the liquids into the high-aspect-ratio tubing at low Reynolds numbers relevant to magma transport. The layering observed in the tubing is analogous to what has been documented in the field ([1,5]). In addition, layered extrudate vented on the platform of the experiment resembles different observed doming features depending on the degrees of viscosity contrast and shear-thinning (non-Newtonian) behavior. The results presented here would seem to offer a realistic basis for developing improved dynamical models of magma transport and eruption that include the multiple effects of ascent rate, which Gonnermann and Manga [30], Papale [31], Sparks [32], Wilson et al. [33] and others have argued control the nature of an eruption. While we have focused attention on the case of two distinct magmas having different silica contents, it is important to point out that magmas are inherently complex materials that may contain non-uniform distributions of crystals, bubbles and volatiles that affect viscosity. Shear flow associated with the near-wall regions of a dike or conduit can induce redistribution of magmas as well as the crystals and bubbles in the magma. Future laboratory and theoretical studies might consider the effects of viscous heating, melt-crystal and bubble segregation during flow as well as heat loss from the annular zone on the ability of this lubricating layer to reduce pressure losses during the magma co-extrusion process.