Experimental Studies to Evaluate the Effects of Different Unloading Stress Paths on Strength Properties of Unconsolidated Sands
Abstract
1. Introduction
2. Sample Preparation and Material Characterization
3. Experimental Methodology
3.1. Loading-Based Yield Behavior
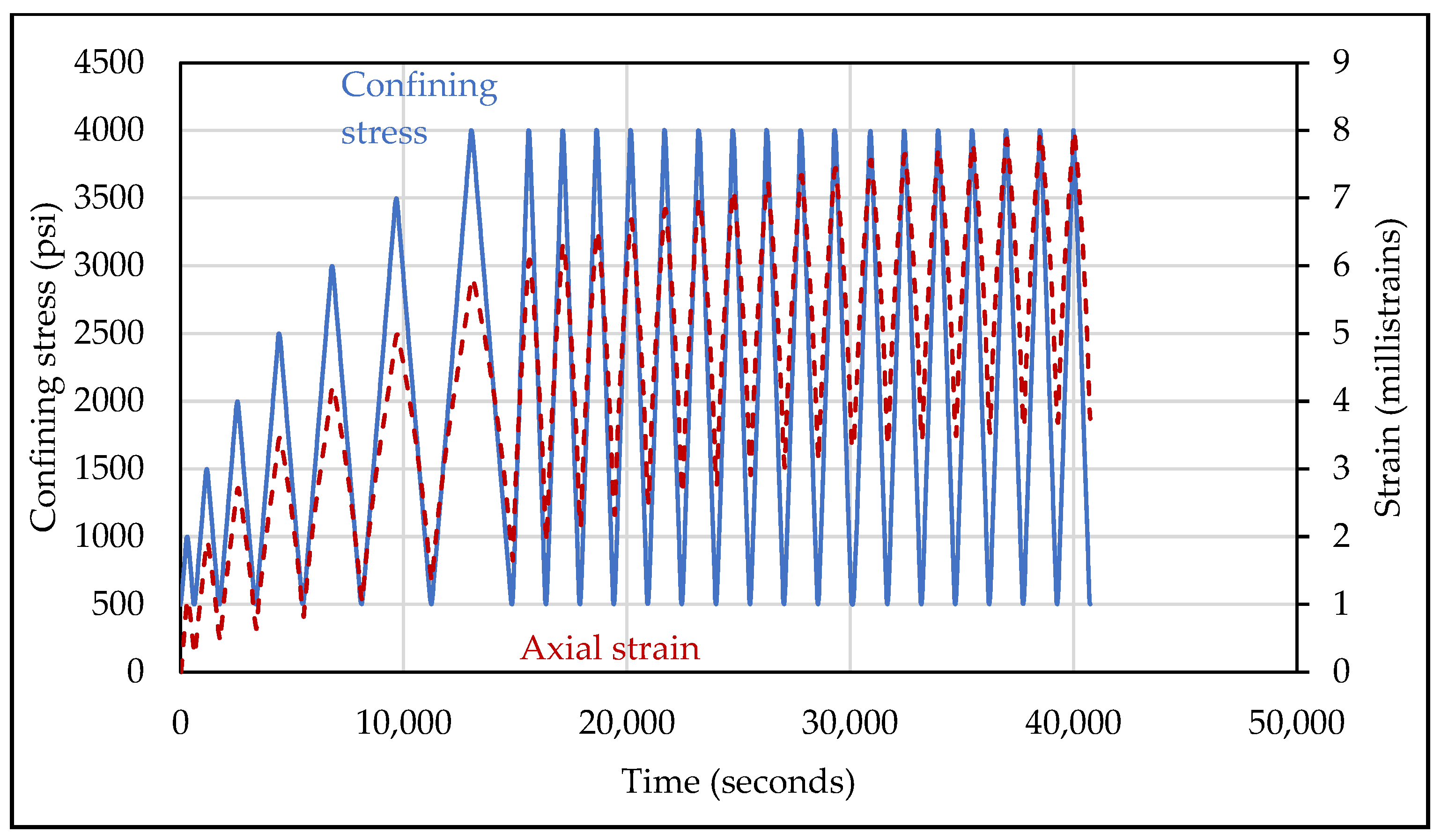
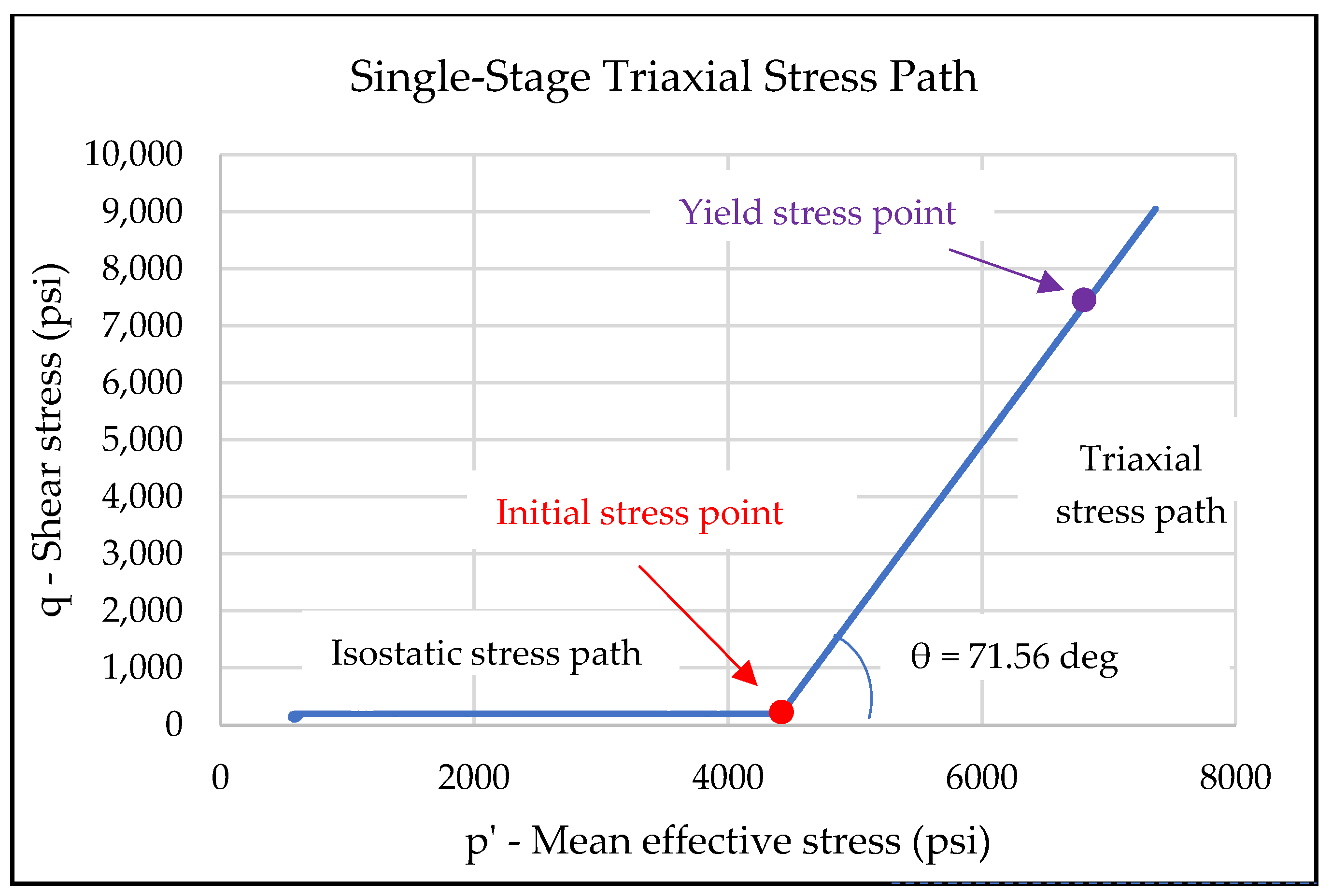
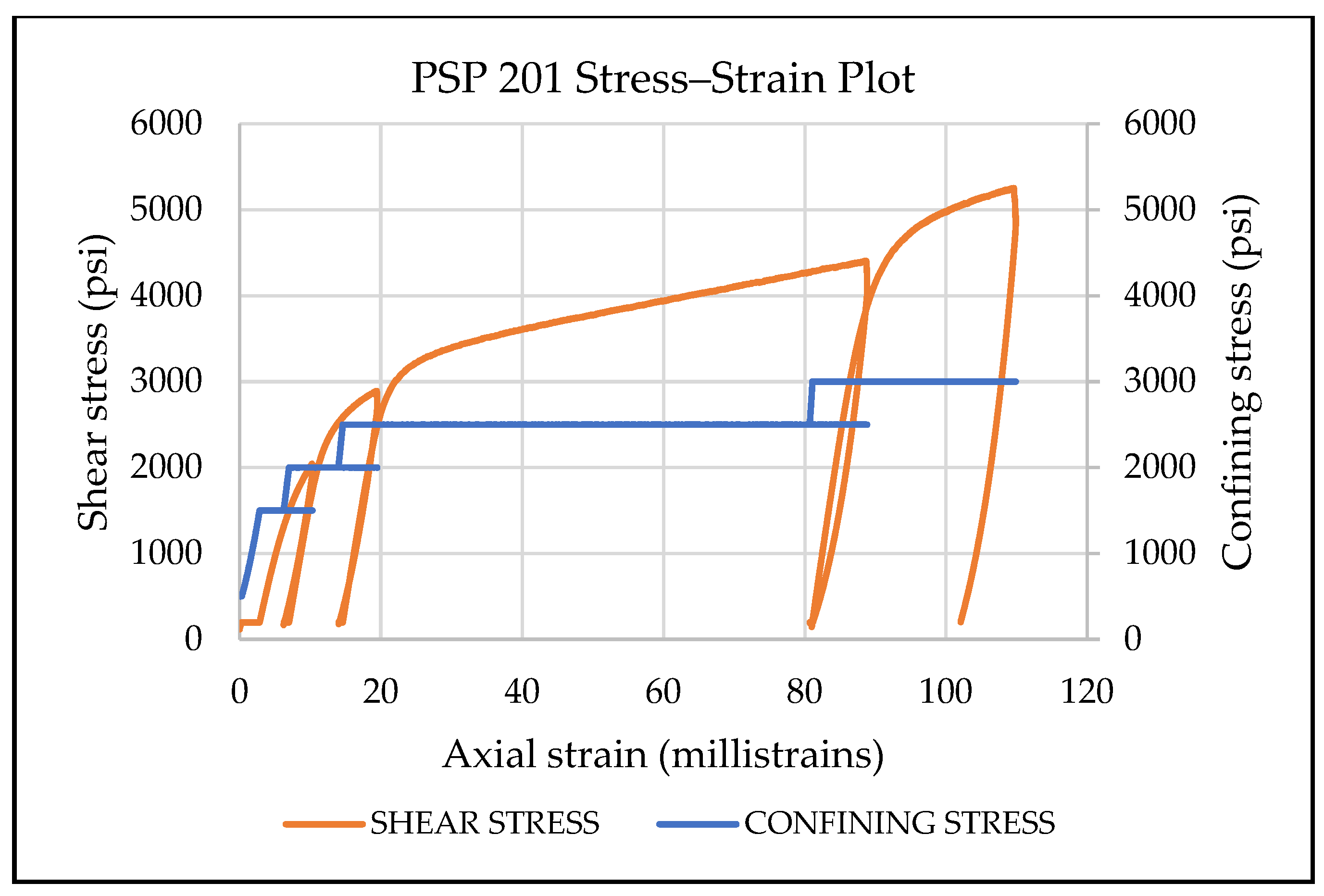
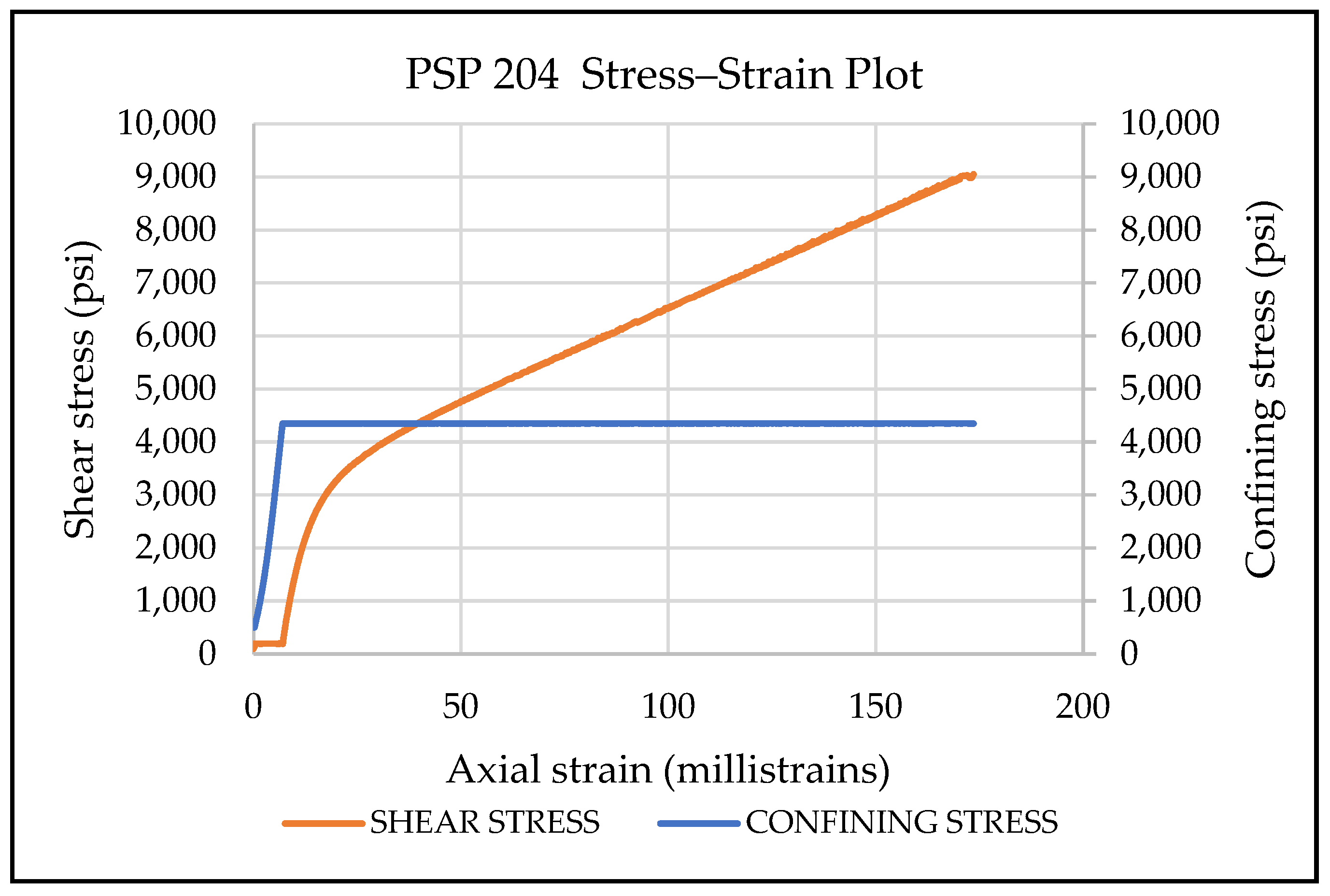
3.1.1. Single-Stage Triaxial Test
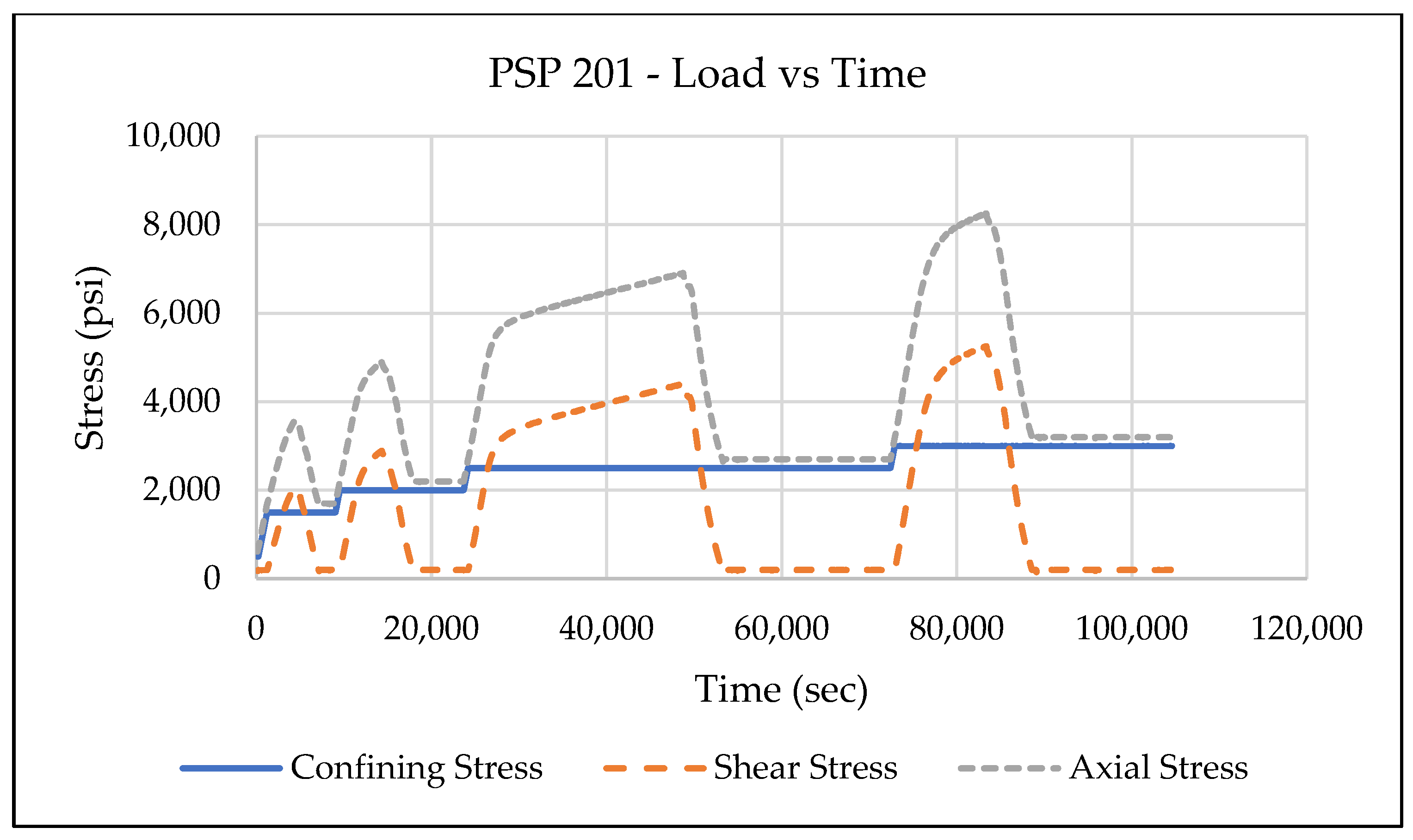
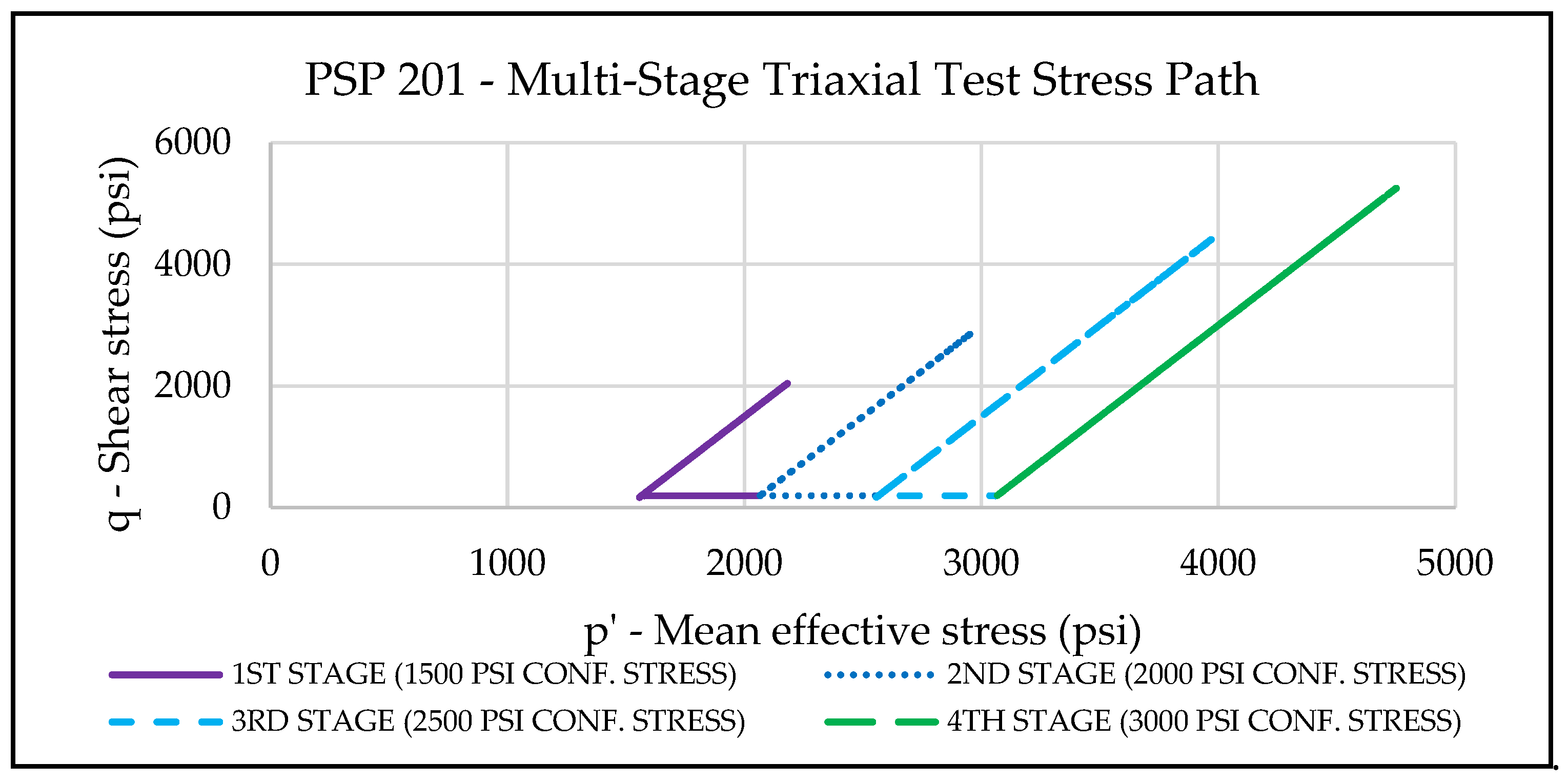
3.1.2. Multi-Stage Triaxial Test
3.2. Unloading-Based Yield Behavior
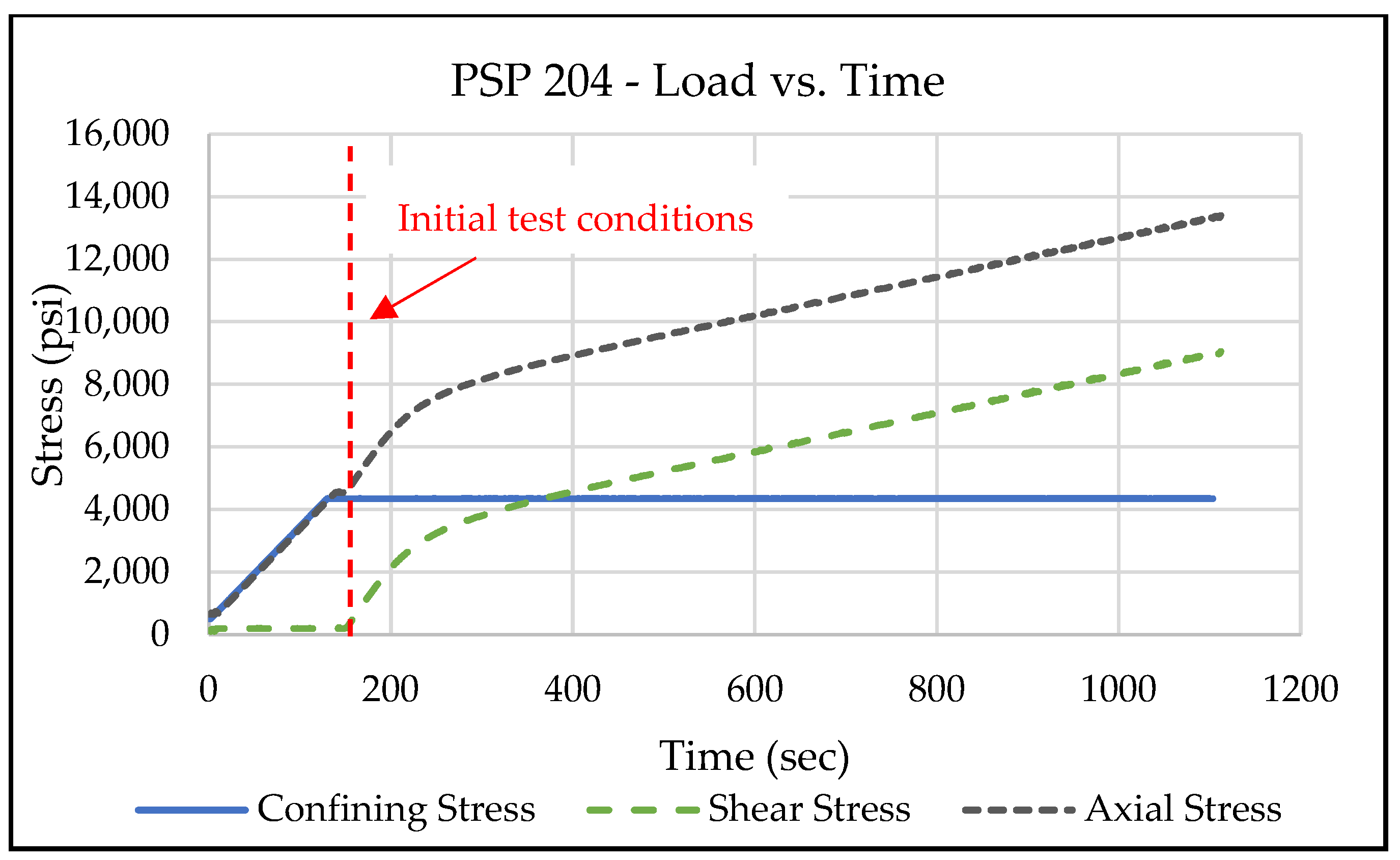
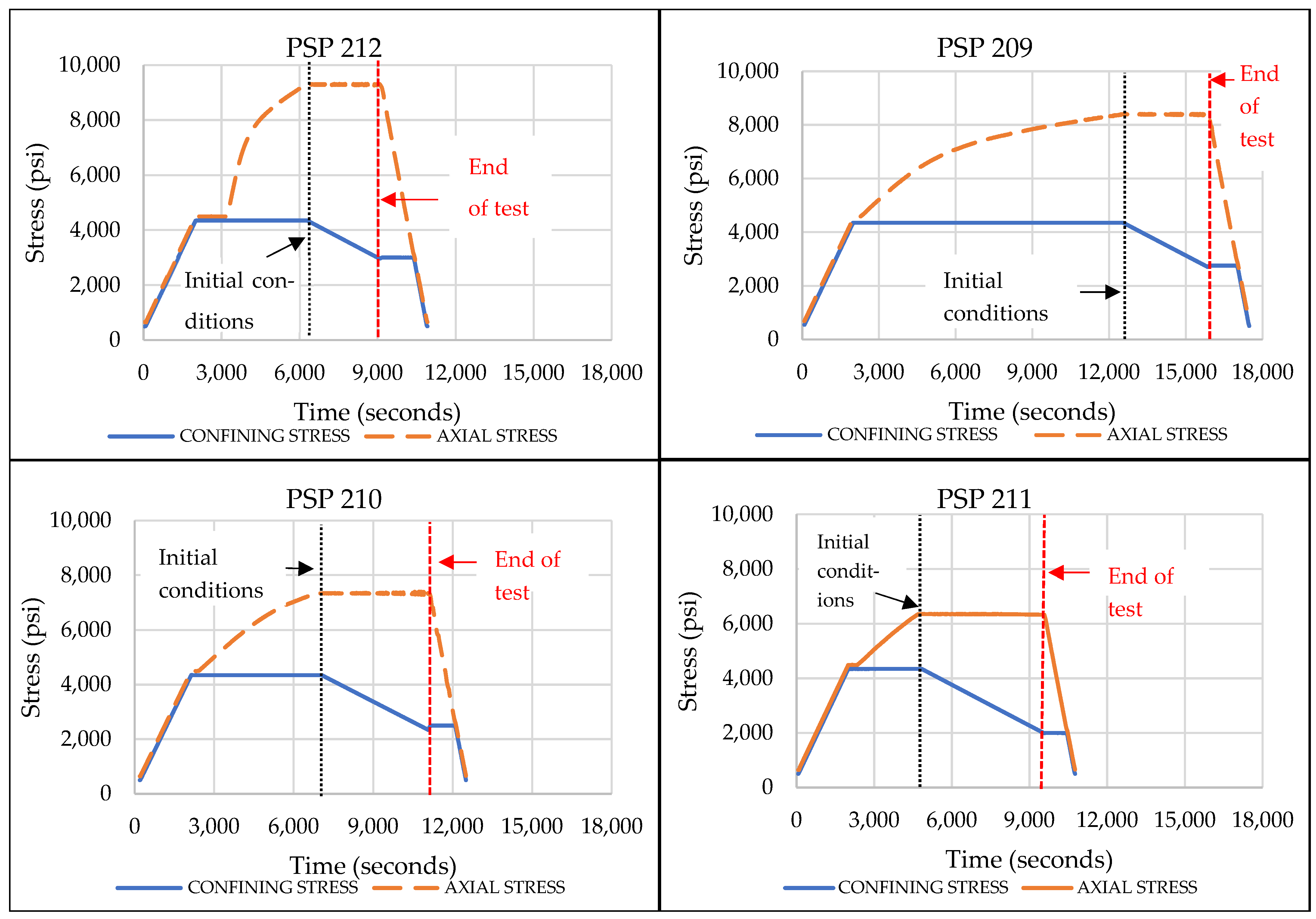
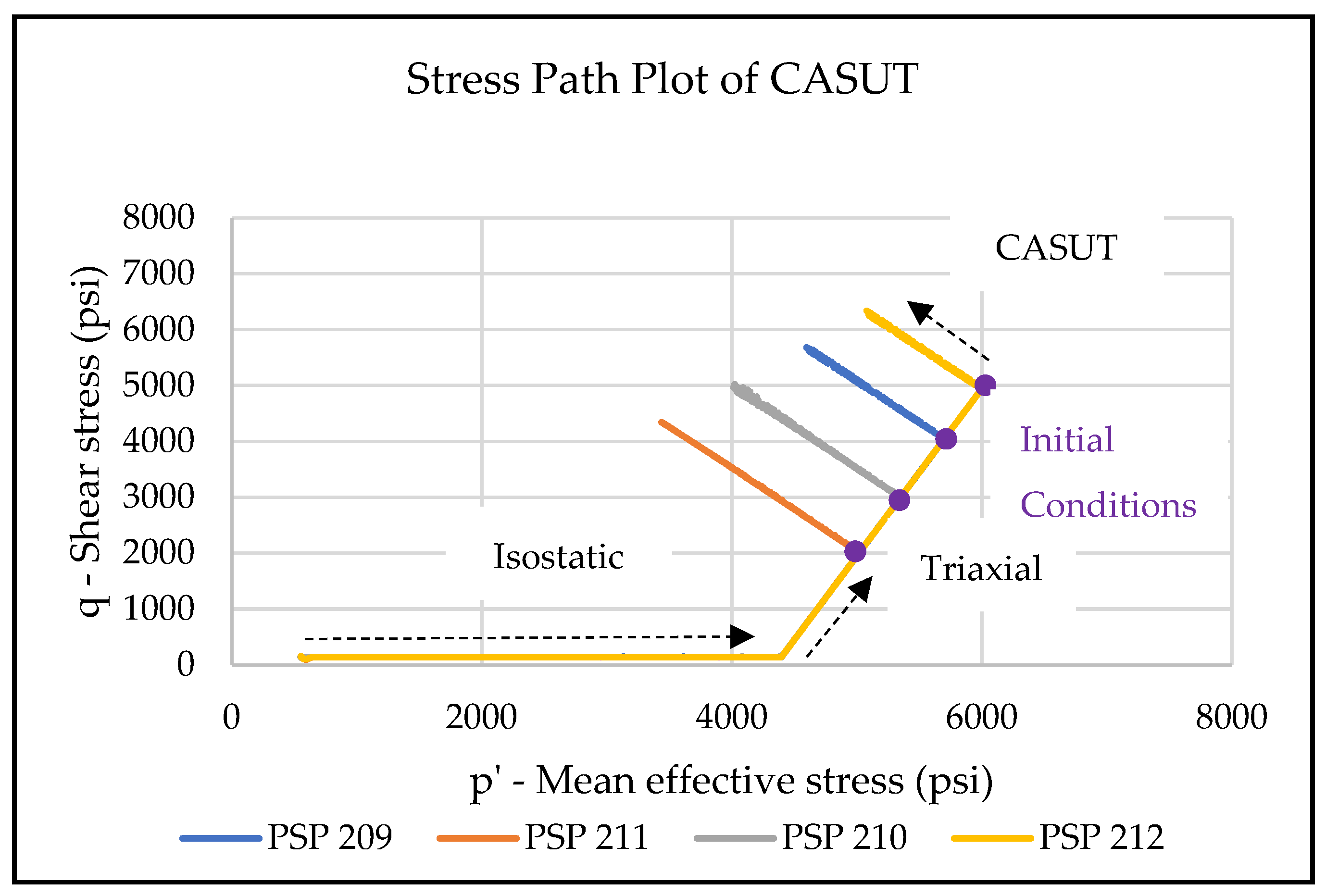
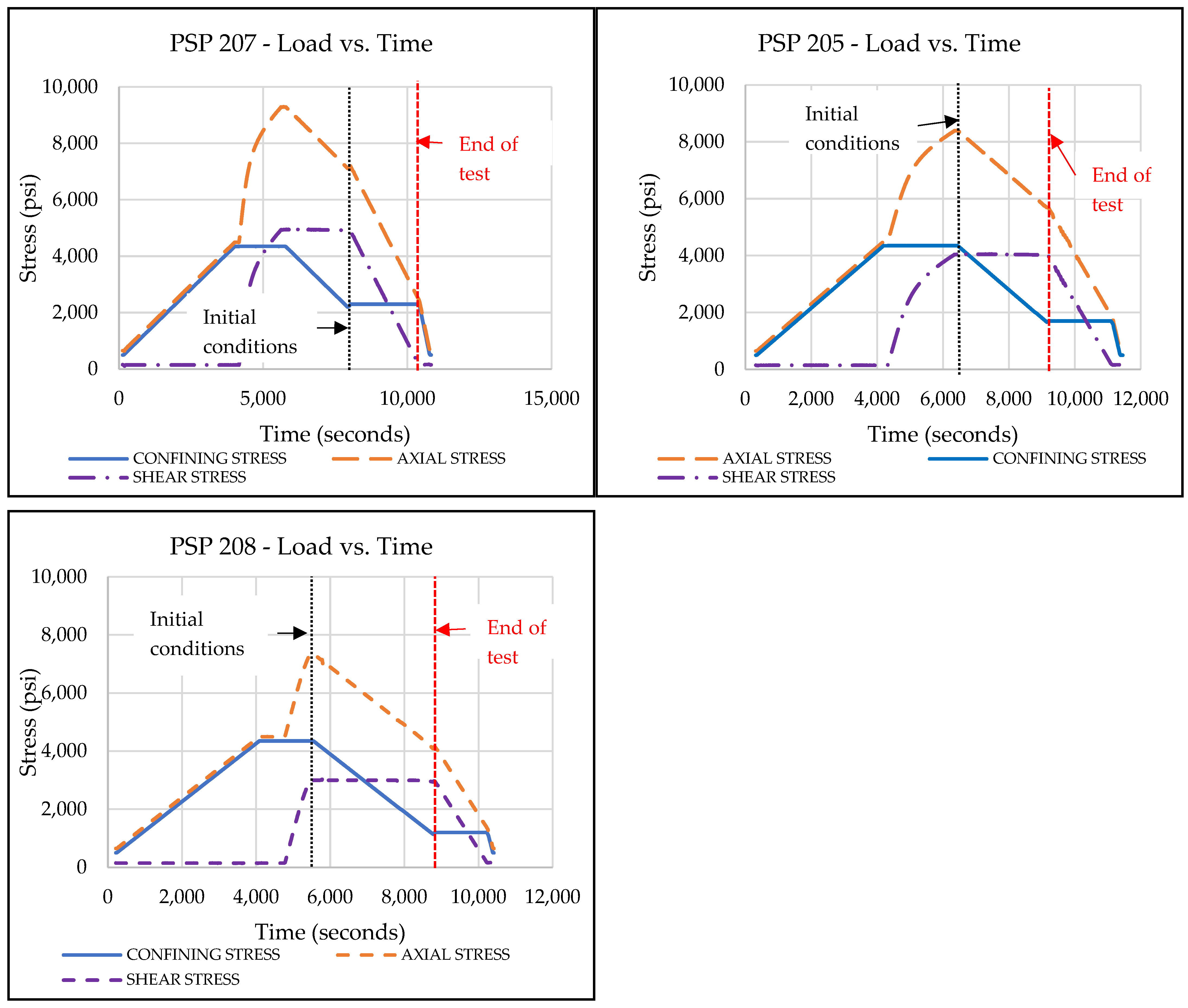
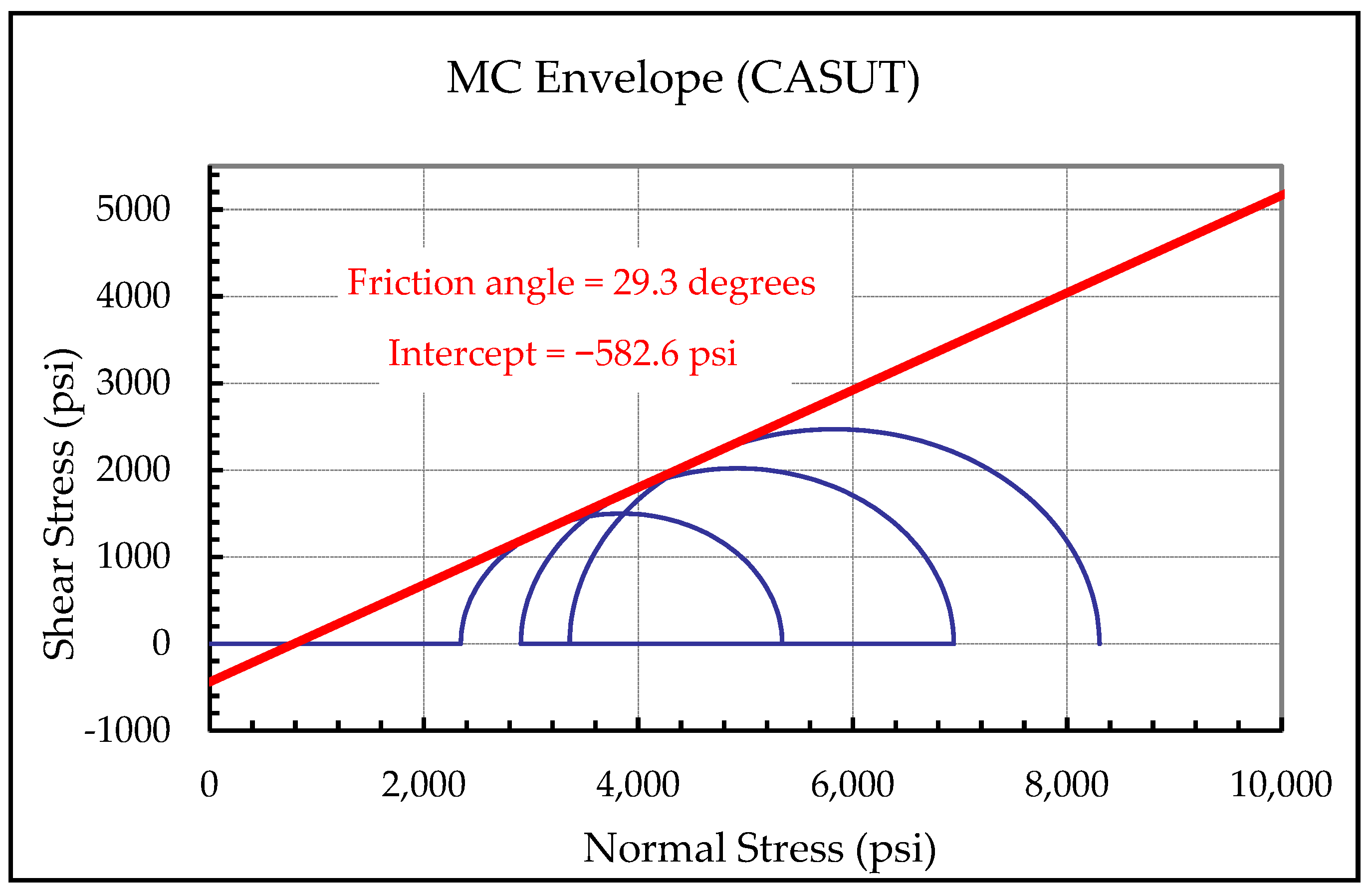
3.2.1. Constant Axial Stress Unloading Tests (CASUTs)
- We start with the isostatic load to the confining stress of 4350 psi (30 MPa).
- Then, a triaxial stress path is followed, and the sample is loaded to the specified initial axial stress point.
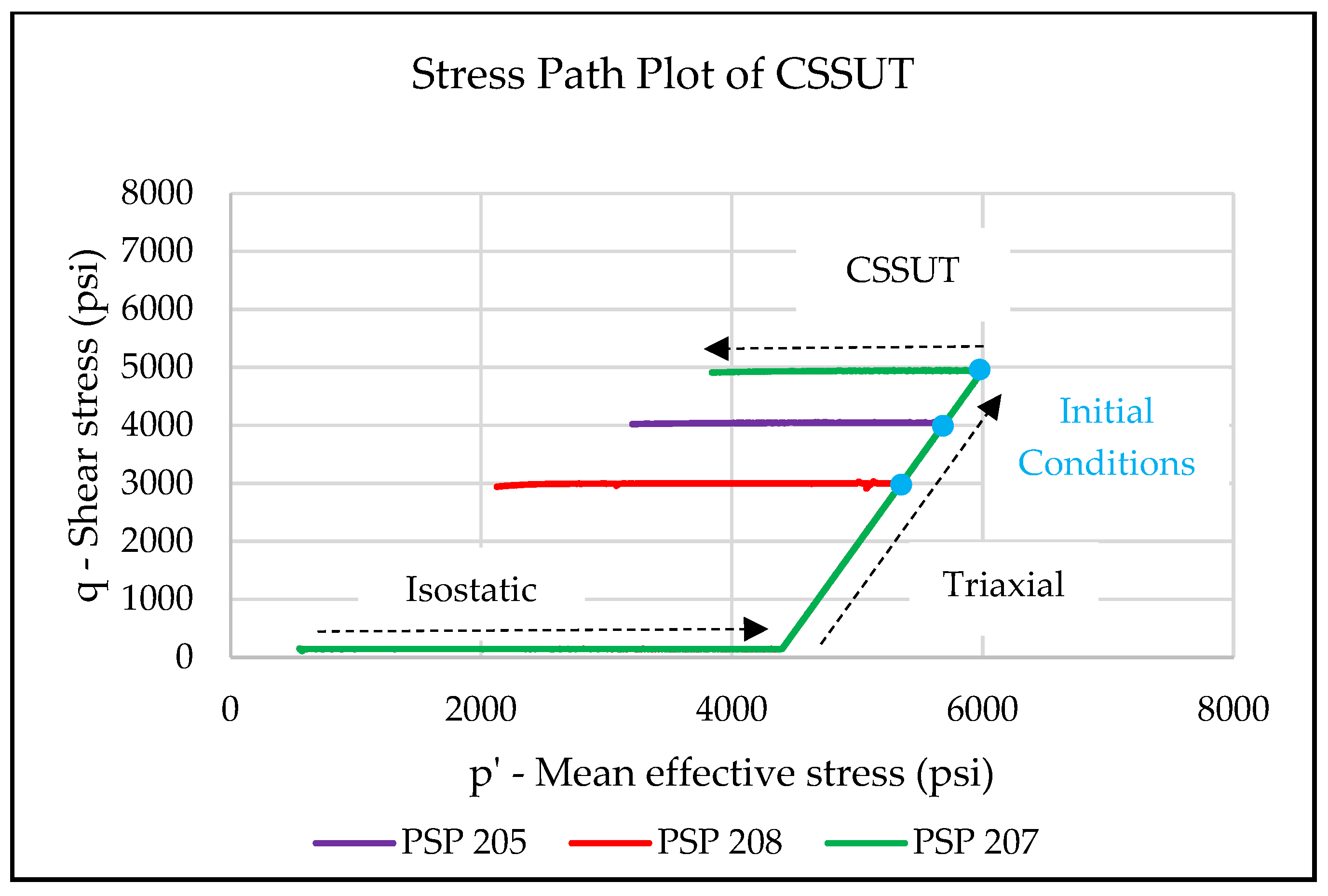
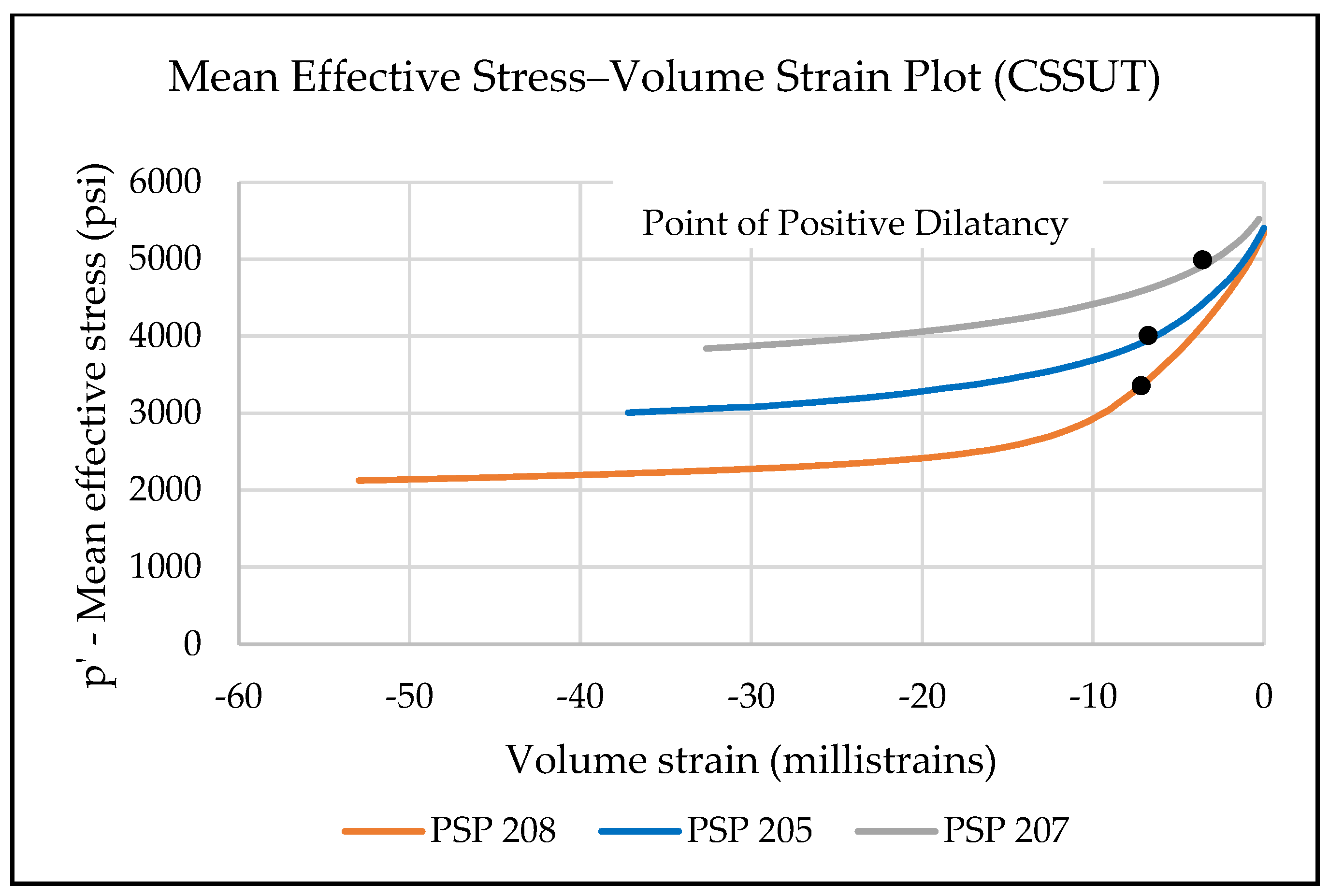
3.2.2. Constant Shear Stress Unloading Tests (CSSUTs)
- Unload the sample by decreasing the confining stress at 0.5 psi per second while keeping the shear stress constant. This is accomplished by controlling the axial load such that for a given confining stress change, the system maintains the shear stress constant.
- Terminate the unloading test when the axial strain gauges reach their measurement limit.
4. Data Analysis
4.1. Loading-Based Triaxial Tests
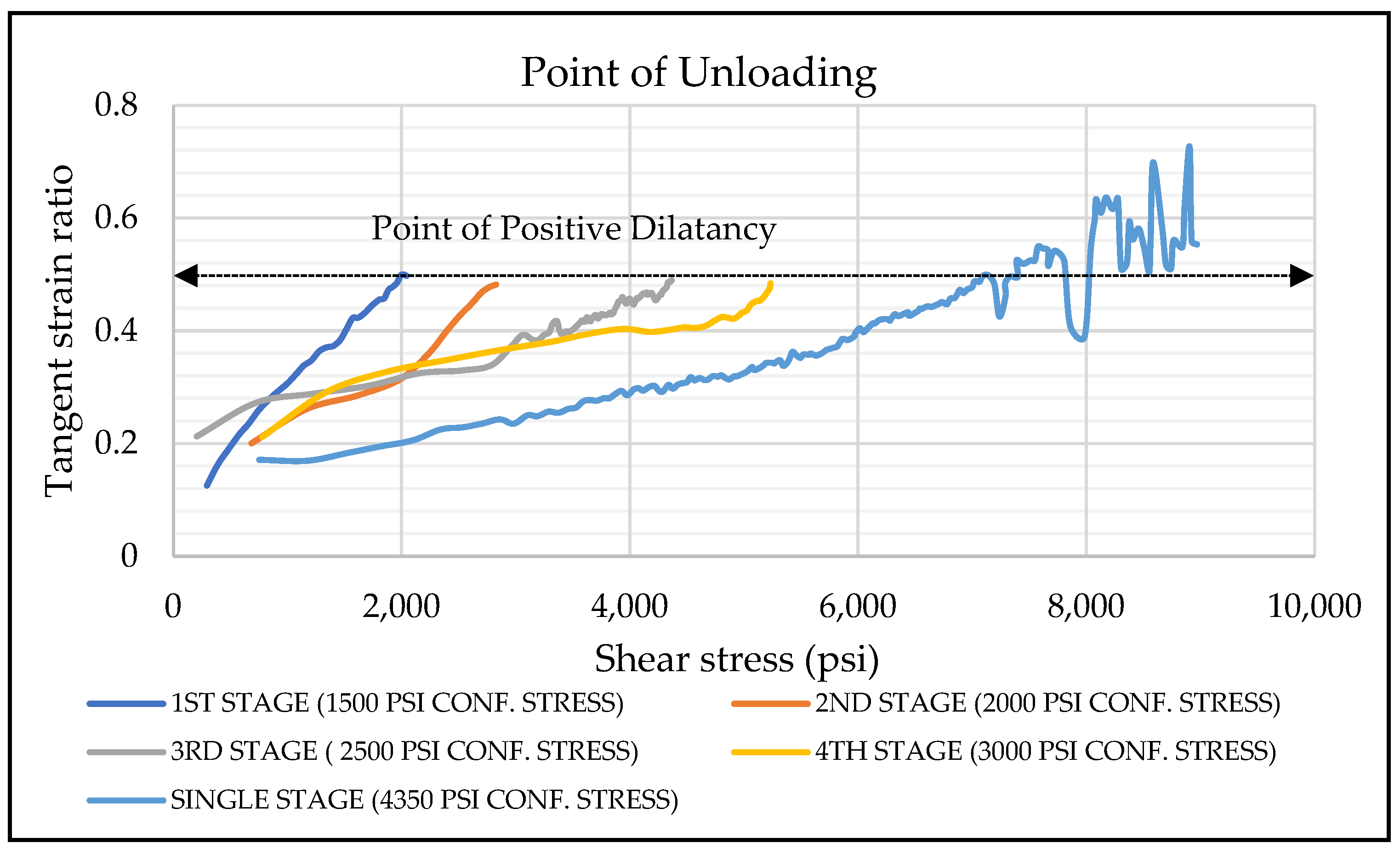
4.1.1. Multi-Stage Triaxial Test Analysis
4.1.2. Single-Stage Triaxial Test Analysis
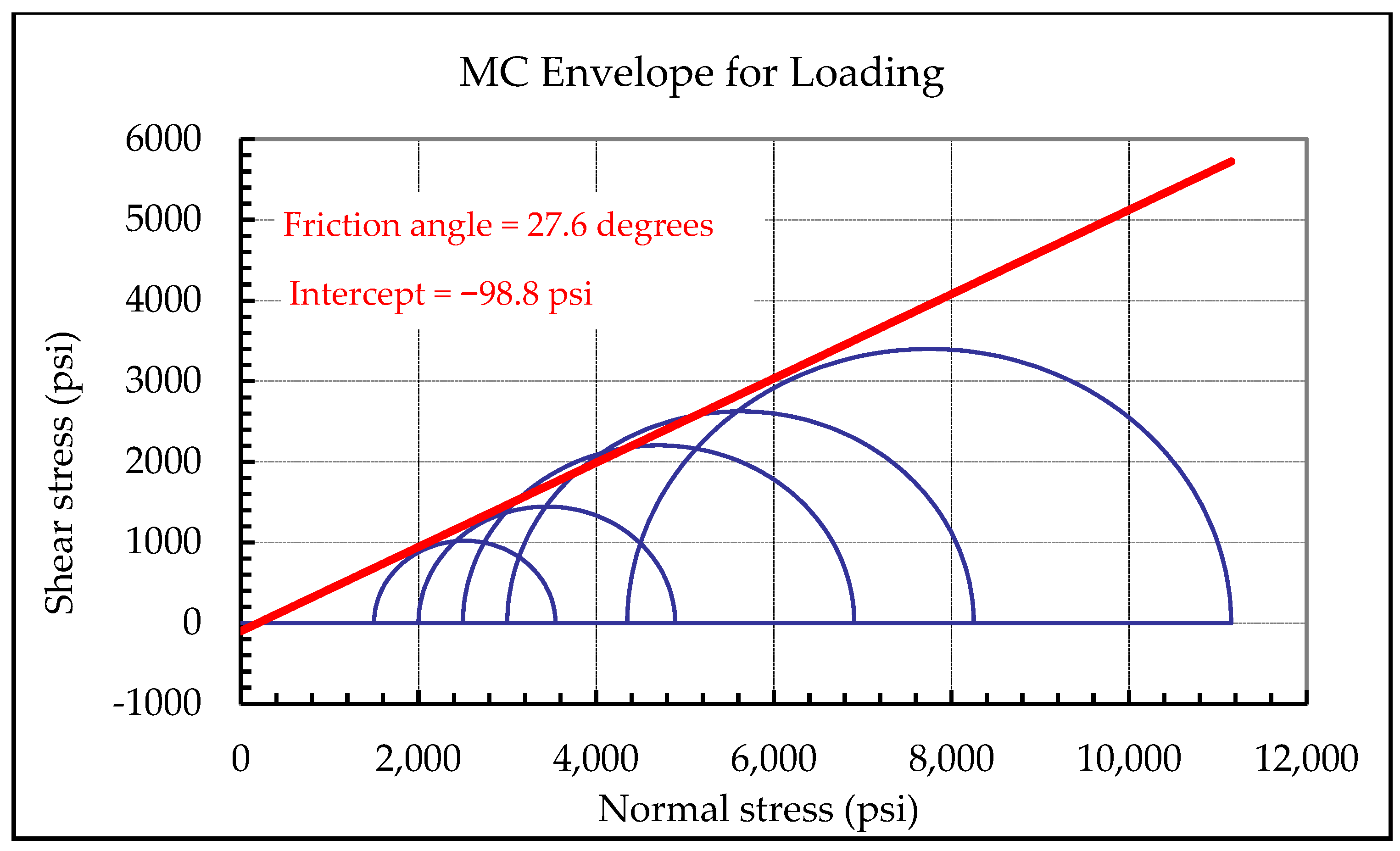
4.1.3. Summary Analysis of Loading-Based Triaxial Tests
4.2. Unloading-Based Triaxial Tests
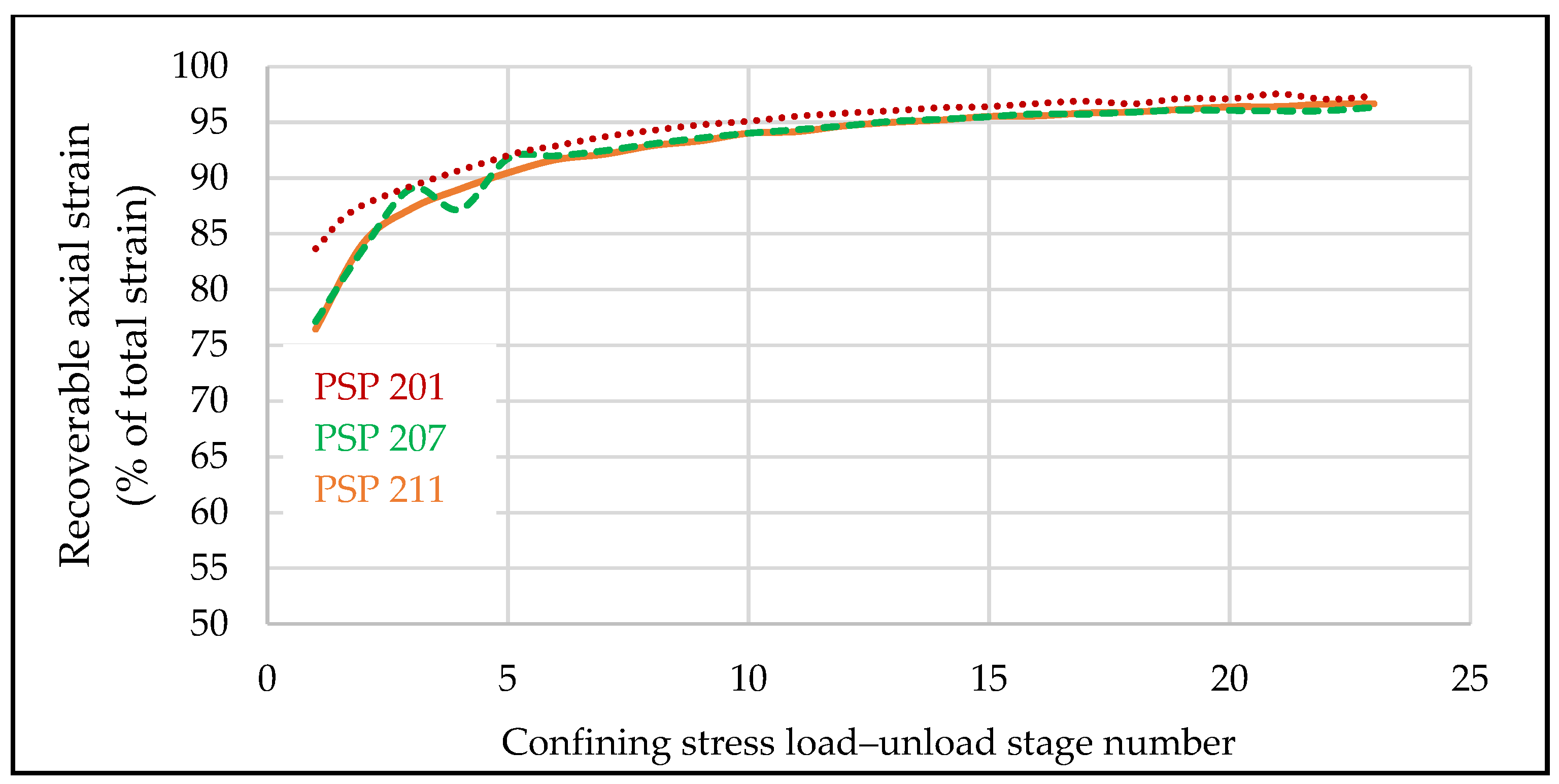
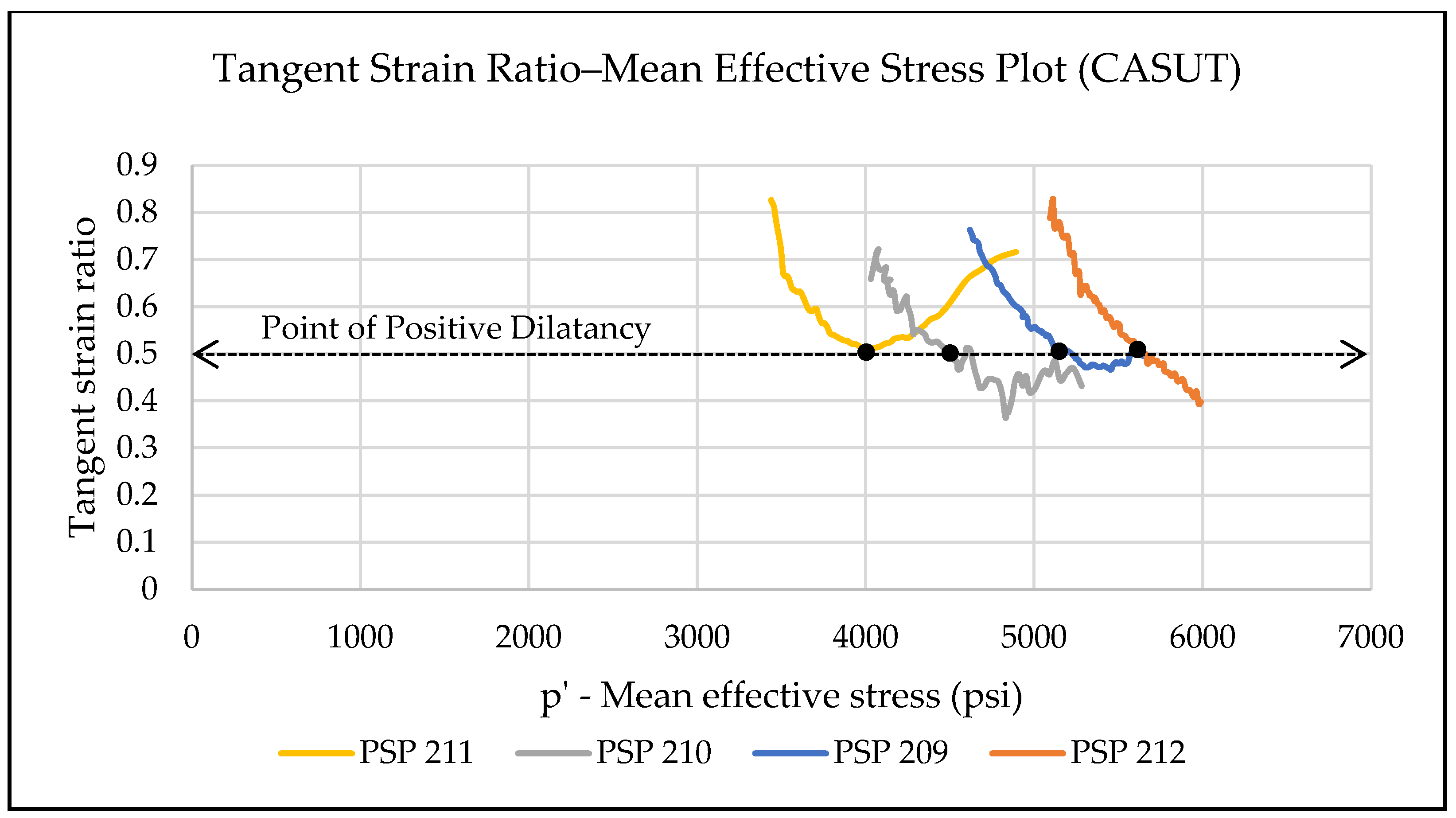
4.2.1. Constant Axial Stress Unloading Tests (CASUTs) Analysis
- An increase in the initial value of mean effective stress makes the sample stronger (it can support more additional load), whereas an increase in the initial value of shear stress makes the sample weaker (it can support less additional load).
- If the initial stress state of the sample is closer to the yield surface, the sample behaves similarly to PSP 212. It would start with a low value of tangent strain ratio and monotonically increase to the point of positive dilatancy and beyond.
- If the sample’s initial stress state is farther away, the applied mean effective stress is higher than the required mean effective stress to support the applied shear stress. Reducing the mean effective stress does not affect the sample response. The sample behaves as if it is unloading in both directions, and the tangent strain ratio decreases.
- At some point during the unloading test, the mean effective stress reaches a point where the value of the mean effective stress is no longer higher than the required mean effective stress to support the applied shear stress. At this point, the tangent strain ratio changes direction and starts to increase.
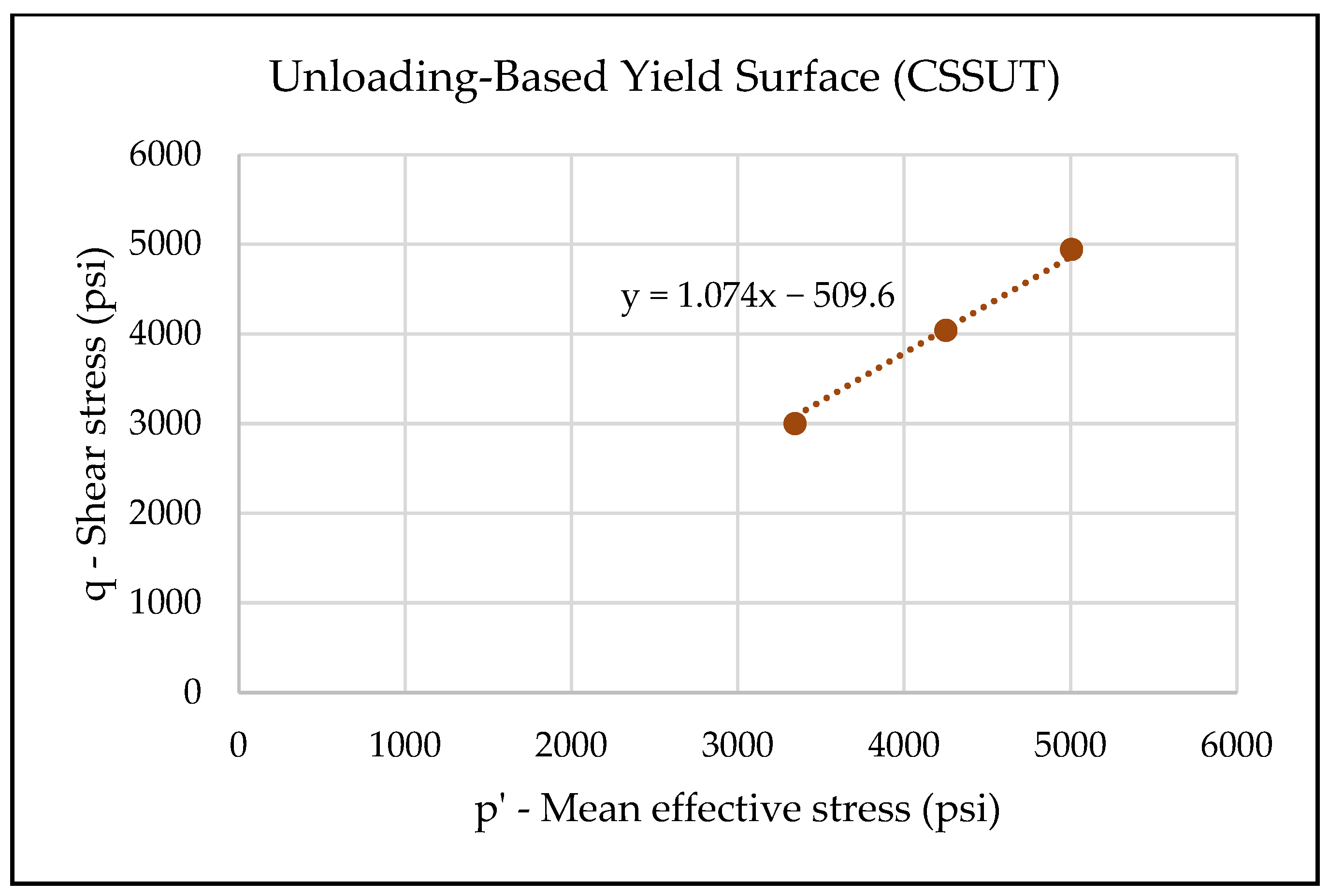
4.2.2. Constant Shear Stress Unloading Tests (CSSUTs) Analysis
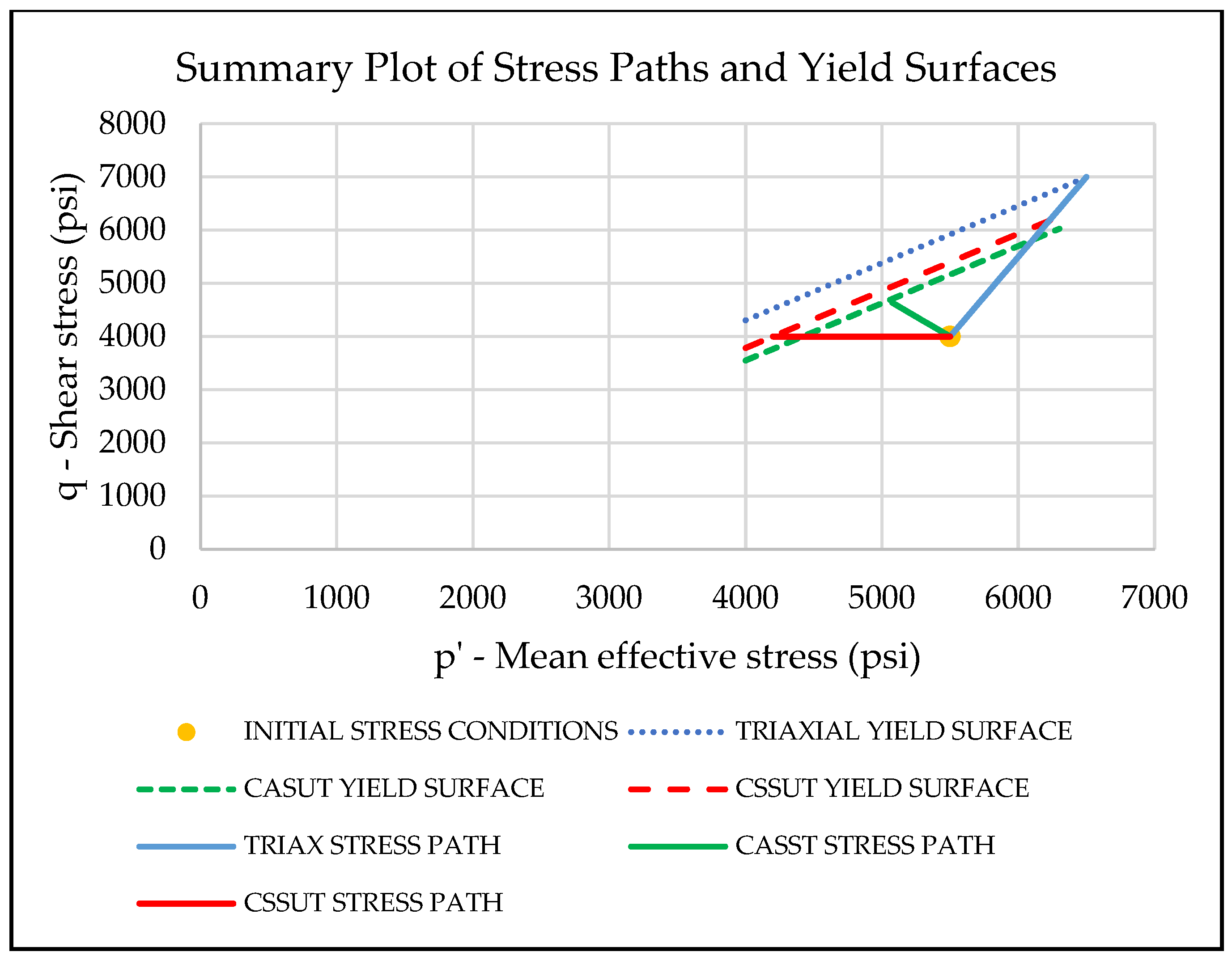
5. Results and Discussion
- A multi-stage triaxial test with one sample to locate the loading-based yield surface.
- A constant axial stress unloading test (CASUT) to locate a yield point on the CASUT yield surface. Since all yield surfaces are parallel, the CASUT yield surface line can be determined geometrically using a slope (same as the triaxial yield surface slope) and the yield point.
- A constant shear stress unloading test (CSSUT) to locate a yield point on the CSSUT yield surface. The CSSUT yield surface line can also be determined as 2.
- The minimum surface can be determined as described earlier in this section.
6. Conclusions and Future Work
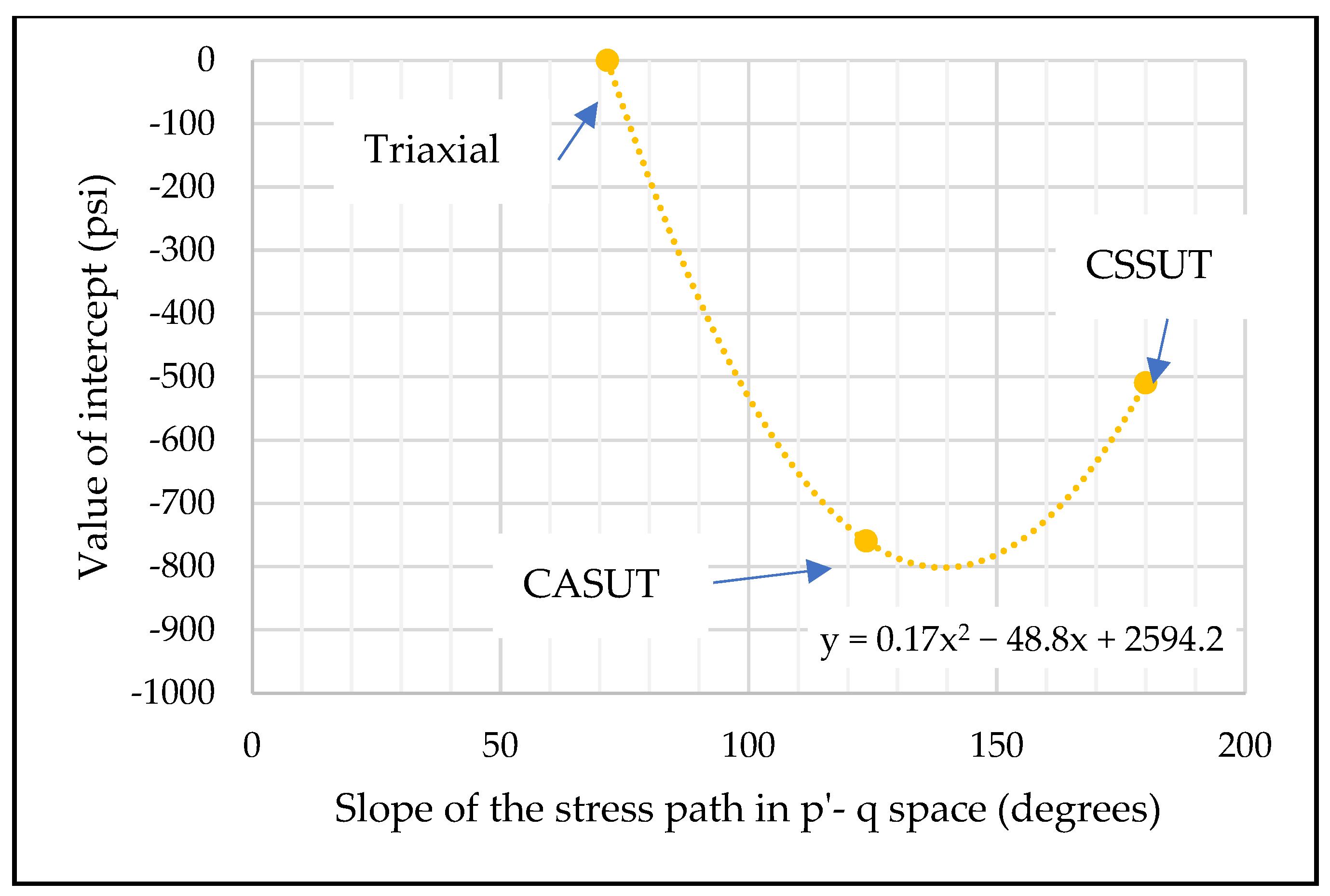
- Yield surfaces for all three sets of experiments were found to be different.
- Yield surfaces for all three sets of experiments were parallel to each other with different values of intercepts.
- The yielding behavior of a material depends on the stress path it traverses. For the same initial stress magnitudes, traversing different stress paths will produce different yield stresses. In the current research work, the triaxial loading stress path produced the highest yield stress, while the constant axial stress unloading path produced the lowest yield stress.
- This work proposes a minimum global yield surface model. The material will have a minimum yield stress in the stress path perpendicular to the yield surfaces.
- With a quadratic fit, all other yield surfaces from different stress paths (with bounds −180 deg to +72 deg) can be predicted.
- With a three-experiment design as described, the global minimum yield surface and all yield points can be determined for a new material.
- Validate the model with more stress paths and expand the upper and lower bounds to different stress paths and magnitudes.
- Include different materials in the study if similar behavior is observed.
- Building a global repository of different materials to quickly predict the yield stress of a material if the stress path is known.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fjær, E.; Holt, R.; Horsrud, P.; Raaen, A.; Risnes, R. Petroleum Related Rock Mechanics, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2008; pp. 1–93. [Google Scholar]
- Jaeger, J.C.; Cook, N.G.W.; Zimmerman, R.W. Fundamentals of Rock Mechanics, 4th ed.; Blackwell Publishing: Hoboken, NJ, USA, 2007; pp. 93–116. [Google Scholar]
- Lambe, T.W. Stress Path Method. J. Soil Mech. Found. Div. 1967, 93, 309–331. [Google Scholar] [CrossRef]
- Zhang, J. Applied Petroleum Geomechanics; Gulf Professional Publishing: Oxford, UK, 2020; pp. 2–8. [Google Scholar]
- Lade, P.V.; Duncan, J.M. Stress-Path Dependent Behavior of Cohesionless Soil. J. Geotech. Eng. Div. 1976, 102, 51–68. [Google Scholar] [CrossRef]
- Zoback, M.D. Reservoir Geomechanics; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Xie, H.Q.; He, C.H. Study of the Unloading Characteristics of a Rock Mass Using the Triaxial Test and Damage Mechanics. Int. J. Rock Mech. Min. Sci. 2004, 41, 74–80. [Google Scholar] [CrossRef]
- Huang, D.; Li, Y. Conversion of Strain Energy in Triaxial Unloading Tests on Marble. Int. J. Rock Mech. Min. Sci. 2014, 66, 160–168. [Google Scholar] [CrossRef]
- Dai, B.; Zhao, G.Y.; Yang, C.; Dong, L.J. Experimental of Unloading Mechanical Properties of Rock Under Different Stress Paths. In International Society for Rock Mechanics and Rock Engineering; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Li, D.; Sun, Z.; Xie, T.; Li, X.; Ranjith, P.G. Energy Evolution Characteristics of Hard Rock During Triaxial Failure with Different Loading and Unloading Paths. Eng. Geol. 2017, 228, 270–281. [Google Scholar] [CrossRef]
- Yin, Z.; Li, X.; Jin, J.; He, X.; Du, K. Failure Characteristics of High Stress Rock Induced by Impact Disturbance Under Confining Pressure Unloading. Trans. Nonferrous Met. Soc. China 2012, 22, 175–184. [Google Scholar] [CrossRef]
- Zhu, T.; Huang, D. Experimental Investigation of the Shear Mechanical Behavior of Sandstone Under Unloading Normal Stress. Int. J. Rock Mech. Min. Sci. 2019, 114, 186–194. [Google Scholar] [CrossRef]
- Huang, R.; Wang, X.; Chan, L. Triaxial unloading test of rocks and its implication for rock burst. Bull. Eng. Geol. Environ. 2001, 60, 37–41. [Google Scholar] [CrossRef]
- Du, K.; Li, X.; Li, D.; Weng, L. Failure Properties of Rocks in True Triaxial Unloading Compressive Test. Trans. Nonferrous Met. Soc. China 2015, 25, 571–581. [Google Scholar] [CrossRef]
- Wang, S.; Xu, W.; Yan, L.; Feng, X.; Xie, W.; Chen, H. Experimental Investigation and Failure Mechanism Analysis for Dacite Under True Triaxial Unloading Conditions. Eng. Geol. 2020, 264, 105407. [Google Scholar] [CrossRef]
- Chen, W.Z.; Liu, D.; Yang, J. Power Function Based Mohr Strength Criterion for Marble with Unloading Confining Pressures. Chin. J. Rock Mech. Eng. 2008, 11, 2214–2220. [Google Scholar]
- Wang, H.; Chen, W.; Tan, X.; Tian, H.; Cao, J. Development of a New Type of Foam Concrete and its Application on Stability Analysis of Large-Span Soft Rock Tunnel. J. Cent. South Univ. 2012, 19, 3305–3310. [Google Scholar] [CrossRef]
- Gao, F.; Zhou, K.; Luo, X.; Zhai, J. Effect of Induction Unloading on Weakening of Rock Mechanics Properties. Trans. Non-Ferr. Met. Soc. China 2012, 22, 419–424. [Google Scholar] [CrossRef]
- He, M.C.; Miao, J.L.; Feng, J.L. Rock Burst Process of Limestone and its Acoustic Emission Characteristics Under True Triaxial Unloading Conditions. Int. J. Rock Mech. Min. Sci. 2010, 47, 286–298. [Google Scholar] [CrossRef]
- Guo, Y.; Yang, C.; Fu, J. Experimental Research on Mechanical Characteristics of Salt Rock Under Triaxial Unloading Test. Rock Soil Mech. 2012, 3, 725–730. Available online: http://ytlx.whrsm.ac.cn/EN/abstract/abstract11031.shtml (accessed on 2 May 2025).
- Zhang, X.; Zhang, D.; Leo, C. Damage Evolution and Post-peak Gas Permeability of Raw Coal Under Loading and Unloading Conditions. Transp. Porous Media 2017, 117, 465–480. [Google Scholar] [CrossRef]
- Liu, Q.; Cheng, Y.; Jin, K.; Tu, Q.; Zhao, W.; Zhang, R. Effect of Confining Pressure Unloading on Strength Reduction of Soft Coal in Borehole Stability Analysis. Environ. Earth Sci. 2017, 76, 173. [Google Scholar] [CrossRef]
- Barla, M. Numerical Simulation of the Swelling Behavior Around Tunnels Based on Special Tri-axial Tests. Tunneling Undergr. Space Technol. 2007, 23, 508–521. [Google Scholar] [CrossRef]
- Zhou, Q. Tests and Test Methods of Unloading Strength of Soft Soil. J. Yangtze River Sci. Res. Inst. 2013, 30, 35–39. [Google Scholar]
- Huang, W.; Wen, K.; Liu, D.; Dong, Q.; Li, J.; Li, Y.; Li, L. Experimental Study on Influence of Excess Pore Water Pressure and Unloading Ratio on Unloading Mechanical Properties of Marine Sedimentary Soft Soils. Ocean. Eng. 2020, 195, 106680. [Google Scholar] [CrossRef]
- Hamoud, M. Influence of Geomechanical Processes on Relative Permeability. Master’s Thesis, University of Alberta, Edmonton, AB, Canada, 2012. [Google Scholar]
- Oldakowski, K. Stress Induced Permeability Changes in Athabasca Oil Sands. Master’s Thesis, University of Alberta, Edmonton, AB, Canada, 1994. [Google Scholar]
- Touhidi, B.A. Absolute Permeability of McMurray Formation Oil Sands at Low Confining Stresses. Ph.D. Thesis, University of Alberta, Edmonton, AB, Canada, 1998. [Google Scholar]
- Chalaturnyk, R.J. Geomechanics of the Steam Assisted Gravity Drainage Process in Heavy Oil Reservoirs. Ph.D. Thesis, University of Alberta, Edmonton, AB, Canada, 1996. [Google Scholar]
- Prakash, S.; Myers, M.; Wong, G.; Hathon, L.; Mikulencak, D. Experimental Study: Stress Path Coefficient in unconsolidated Sands: Effects of Re-Pressurization and Depletion Hysteresis. Geosciences 2024, 14, 327. [Google Scholar] [CrossRef]
- Kovari, K.; Tisa, A. Multiple failure state and strain controlled triaxial tests. In Rock Mechanics and Rock Engineering; Springer: Berlin/Heidelberg, Germany, 1975; Volume 7, pp. 17–33. [Google Scholar]
- Kim, M.M.; Ko, H.Y. Multistage triaxial testing of rocks. Geotech. Test. J. 1979, 2, 98–105. [Google Scholar] [CrossRef]
- Crawford, A.; Wylie, D. A modified multiple failure triaxial testing method. In Proceedings of the ARMA US Rock Mechanics/Geomechanics Symposium, Tucson, AZ, USA, 29 June 1987. ARMA-87-0133. [Google Scholar]
- Holt, A.M.; Fjaer, E. Validity of Multiple Failure State Triaxial Tests in Sandstones. In Proceedings of the 7th ISRM Congress, Aachen, Germany, 16 September 1991. ISRM-7CONGRESS-1991-102. [Google Scholar]
- Pagoulatos, A. Evaluation of Multistage Triaxial Testing on Berea Sandstone. M.S. Thesis, University of Oklahoma, Norman, OK, USA, 2004. [Google Scholar]
- Jambunathan, V. Study of Mechanical Properties of Carbonates. Master’s Thesis, University of Oklahoma, Norman, OK, USA, 2008. [Google Scholar]
- Taheri, A.; Tani, K. Proposal of a New Multiple-Step Loading Triaxial Compression Testing Method. In Proceedings of the 5th Asian Rock Mechanics Symposium, Tehran, Iran, 24 November 2008. ISRM-ARMS5-2008-058. [Google Scholar]
- Alsalman, M.E.; Myers, M.T.; Sharf-Aldin, M.H. Comparison of multi-stage to single-stage tri-axial test. In Proceedings of the 49th US Rock Mechanics/ Geomechanics Symposium, Seattle, WA, USA, 28 June–1 July 2015. ARMA-2015-767. [Google Scholar]
- Bilal, A.; Myers, M.; Hathon, L. An Investigation of Static and Dynamic Data Using Multistage Triaxial Tests. In Proceedings of the Society of Exploration Geophysicists Conference, Dallas, TX, USA, 20 October 2016. SEG 16-13969660. [Google Scholar]
- Dessouki, M.; Myers, M.T.; Hathon, L.A. A New Approach to Calibrate the Modified Cam Clay Model by Using Multi-stage Triaxial Tests on Resedimented Mudrocks. In Proceedings of the 54th U.S. Rock Mechanics/Geomechanics Symposium, Golden, CO, USA, 28 June–1 July 2020. ARMA-2020-1558. [Google Scholar]
- Prakash, S.; Myers, M.T.; Hathon, L.A. Analysis of Damage Induced in Multistage Triaxial Tests Using Acoustic Emissions Data. In Proceedings of the 52nd U.S. Rock Mechanics/Geomechanics Symposium, Seattle, WA, USA, 17 June 2018. ARMA-2018-1223. [Google Scholar]
- Mesri, G.; Vardhanabhuti, B. Compression of granular materials. Can. Geotech. J. 2009, 46, 369–392. [Google Scholar] [CrossRef]
- Nguyen, V.H.; Gland, N.; Dautriat, J.; David, C.; Wassermann, J.; Guelard, J. Compaction, permeability evolution and stress path effects in unconsolidated sand and weakly consolidated sandstone. Int. J. Rock Mech. Min. Sci. 2014, 67, 226–239. [Google Scholar] [CrossRef]
- Wong, T.F.; David, C.; Zhu, W. The transition from brittle faulting to cataclastic flow in porous sandstone: Mechanical deformation. J. Geophys. Res. 1997, 102, 3009–3025. [Google Scholar] [CrossRef]
- Yale, D.P.; Mayer, T.; Wang, J. Geomechanics of Oil Sands Under Injection. In Proceedings of the 44th U.S. Rock Mechanics Symposium, Salt Lake City, UT, USA, 27–30 June 2010. ARMA-10-257. [Google Scholar]
- Gheibi, S.; Holt, R.M.; Vilarrasa, V. Effect of Faults on Stress Path Evolution During Reservoir Pressurization. Int. J. Greenh. Gas Control 2017, 63, 412–430. [Google Scholar] [CrossRef]
- Lynch, T.; Fisher, Q.; Angus, D.; Lorinczi, P. Investigating Stress Path Hysteresis in a CO2 Injection Scenario Using Coupled Geomechanical-Fluid Flow Modelling. Energy Procedia 2013, 37, 3833–3841. [Google Scholar] [CrossRef]
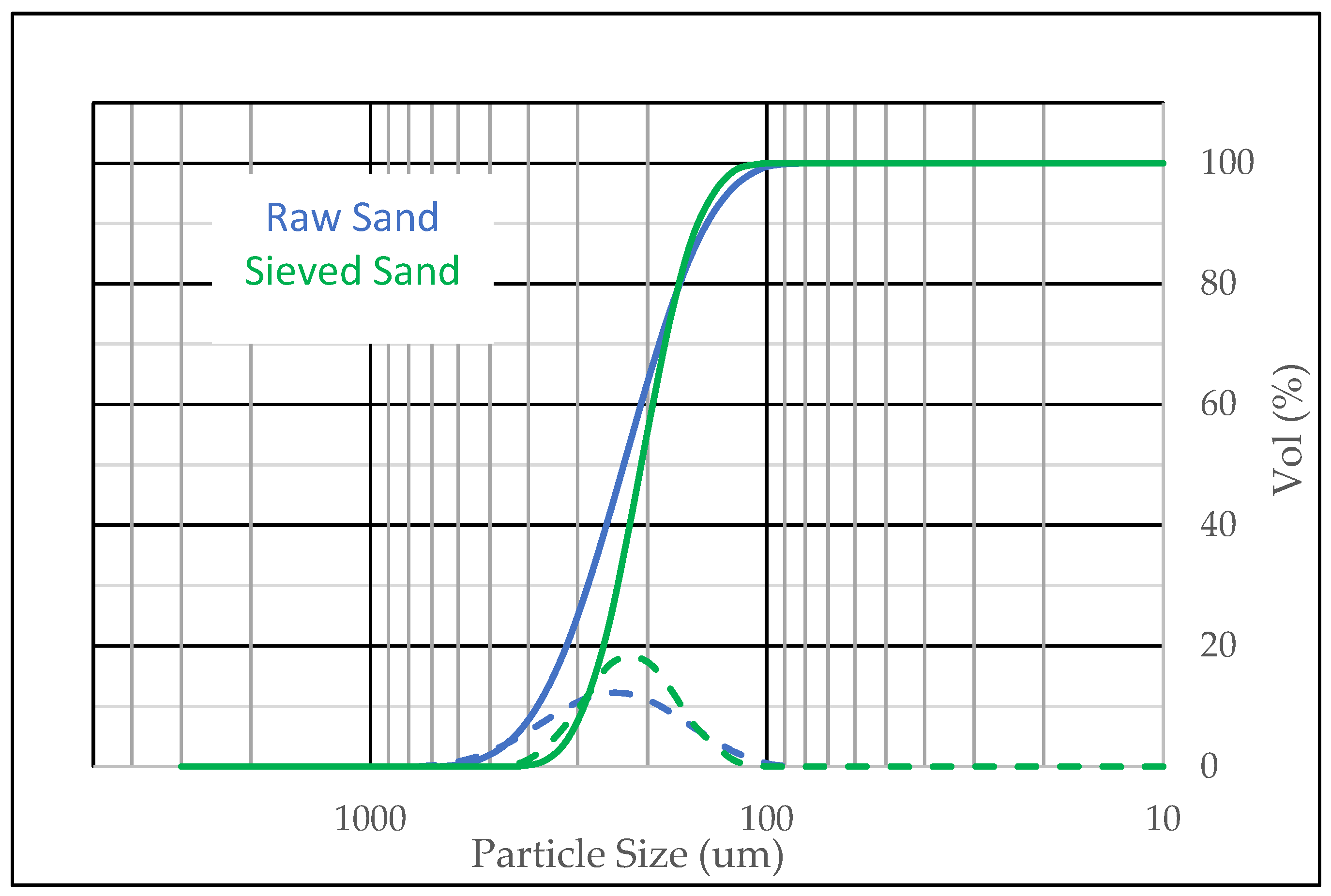










| Material Property | Value |
|---|---|
| Grain Size (d50) | 203 microns |
| Grain Density | 2.65 |
| Mineralogy | 100% Quartz |
| Average Porosity | 35% |
| Sample Length | 2 in. |
| Sample Diameter | 1 in. |
| Test Type | Dry (no pore fluid) |
| Sample | σ1 | σ3 | Initial p’ | Initial q |
|---|---|---|---|---|
| PSP 212 | 9300 | 4350 | 6000 | 4950 |
| PSP 209 | 8400 | 4350 | 5700 | 4050 |
| PSP 210 | 7350 | 4350 | 5350 | 3000 |
| PSP 211 | 6360 | 4350 | 5020 | 2010 |
| Sample | σ1 | σ3 | Initial p’ | Initial q |
|---|---|---|---|---|
| PSP 207 | 9300 | 4350 | 6000 | 4950 |
| PSP 205 | 8400 | 4350 | 5700 | 4050 |
| PSP 208 | 7350 | 4350 | 5350 | 3000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prakash, S.; Myers, M.; Wong, G.; Hathon, L.; Mikulencak, D. Experimental Studies to Evaluate the Effects of Different Unloading Stress Paths on Strength Properties of Unconsolidated Sands. Geosciences 2025, 15, 173. https://doi.org/10.3390/geosciences15050173
Prakash S, Myers M, Wong G, Hathon L, Mikulencak D. Experimental Studies to Evaluate the Effects of Different Unloading Stress Paths on Strength Properties of Unconsolidated Sands. Geosciences. 2025; 15(5):173. https://doi.org/10.3390/geosciences15050173
Chicago/Turabian StylePrakash, Sabyasachi, Michael Myers, George Wong, Lori Hathon, and Duane Mikulencak. 2025. "Experimental Studies to Evaluate the Effects of Different Unloading Stress Paths on Strength Properties of Unconsolidated Sands" Geosciences 15, no. 5: 173. https://doi.org/10.3390/geosciences15050173
APA StylePrakash, S., Myers, M., Wong, G., Hathon, L., & Mikulencak, D. (2025). Experimental Studies to Evaluate the Effects of Different Unloading Stress Paths on Strength Properties of Unconsolidated Sands. Geosciences, 15(5), 173. https://doi.org/10.3390/geosciences15050173






