Experimental Investigation on the Effect of Sequences of Unsteady Flows on Bedload Sediment Transport

Abstract

Flash floods in ephemeral streams are rare, short and difficult to forecast and thus to monitor. During these events, bedload transport reaches very high rates and most sediment transport occurs within a limited number of hours during the course of a year. Because monitoring of bedload in ephemeral rivers is challenging, here we present the results of a series of flume experiments designed to simulate short, flashy floods. Since most flume experiments usually involve single events, here we add to existing evidence by testing the effects of sequences of multiple floods in rapid succession. The flume is 10 m long, 0.3 m wide and 0.5 m deep. Two bed sediment mixtures (well sorted and poorly sorted) with similar median grain size but a different standard deviation were used. Bedload was monitored continuously during each hydrograph, but no sediment was fed. The flume experiments used six triangular hydrographs with peak flows ranging from 0.0147 to 0.02 m³ ${\mathrm{s}}^{-1}$ and durations ranging from 150 to 400 s. Results indicate that the sediment transport rate decreases progressively from the first to the third hydrograph, and that this pattern is consistent for all permutations of peak discharge and flood duration. In all of the runs, the sediment transport rate at a specified flow was higher during the rising limb than the falling limb of the hydrograph, indicating clockwise hysteresis. Furthermore, in the subsequent repetitions of the same hydrograph, the degree of hysteresis generally diminishes in magnitude from the first to the last repetition for all the experiments, irrespective of their magnitude and duration.

1. Introduction

Ephemeral channels in dryland regions typically have distinctive characteristics if compared to permanent rivers. For instance, the bed material is not consolidated into a coarser armour layer and coarse sediment coexists with much finer fractions. There is an abundant source of loose, unstructured sediment available for transport. Sediment transport rates reach high intensities that can be one or two orders of magnitude higher than in perennial streams, mostly due to general conditions of unlimited sediment supply and even very large sediment sizes (i.e., boulders) can be transported. Bedload fluxes can reach extremely high levels, up to ~600 $\mathrm{k}\mathrm{g}{\mathrm{s}}^{-1}{\mathrm{m}}^{-1}$, far exceeding typical values in perennial streams [1,2]. Except for flow regime, streams that possess a comparatively low sediment supply are prone to exhibit a substantial level of armouring. Conversely, streams that experience adequately high sediment supply rates are unlikely to exhibit notable vertical sediment sorting and, as a result, are expected to display a diminished degree of armouring [3]. The main difference between a perennial river and ephemeral streams is the frequency and duration of water flow. Perennial rivers have continuous or nearly continuous water flow, while ephemeral streams only flow during and shortly after rainfall events. Ephemeral streams differ from perennial streams in many ways and they tend to have higher sediment transport rates [4]. Flash floods in ephemeral streams are unforeseeable, rare and short [5]. Understanding the impact of sediment on flash flood hydrodynamics is imperative for precise flood risk assessment and mapping. However, investigating sediment transport in flash floods poses significant challenges due to the high sediment loads, flashy hydrograph rising and recession limbs, and the intermittent nature of these events [3,6]. Measuring bedload transport is the main process responsible for fluvial morphodynamics [7]; bedload is only rarely measured in ephemeral streams [7,8]. Ephemeral gravel bed streams typically have bed surfaces that are relatively unarmoured compared to the substrate below, while gravel bed streams in humid and snowmelt areas typically have well-armoured surfaces [3]. This is because the flashy flow regime impedes the sorting of bed sediment, resulting in unarmoured beds, as noted by [3,5]. Understanding bedload sediment transport provides an essential key to predicting changes in river channel form and hydraulic function, and understating the dynamic of bedload transport overtime can help explaining the conditions of sediment availability in the channel bed during single flood events. There are relatively few flume experiments carried out using unsteady flow [9]. During unsteady flow, sediment transport can be higher in the rising limb of hydrographs if compared to the falling limb, and in this case plotting the bedload versus the liquid discharge shows a clockwise trend [10,11]. Flash floods are characterized by short duration and fast rising limbs, result from intense rainfall events and often exhibit sediment hysteresis [2]. The types of hysteresis are divided into the following groups based on their loop: (1) clockwise, (2) anticlockwise or counterclockwise, (3) single value, (4) single value with loop and figure eight [12]. If flume experiments conducted using unsteady flow are rather limited [9], those carried out with repetition of hydrographs are even scarcer. Amongst the few, ref. [11] showed that even under conditions of sediment recirculation, a previous event decreases the rates of sediment transported of a following hydrograph by around 40% if a high-magnitude event precedes another one, and around 70% if a low-magnitude event precedes another event of similar magnitude. Ref. [13] showed that sequences of hydrographs generated a flow stress history that affects channel microtopography along with bedload transport rates. Here we simulate sequences of hydrographs of different magnitude and duration using two different sediment mixtures (well and poorly sorted) without sediment feeding, to study the dynamics of bedload transport and sediment bed. Well sorted and poorly sorted are uniform sediments and non-uniform sediment mixtures, respectively. The objective of this research is to investigate the potential impact of the hydrograph sequence on sediment transport, considering the alterations in bed morphology during each repetition.

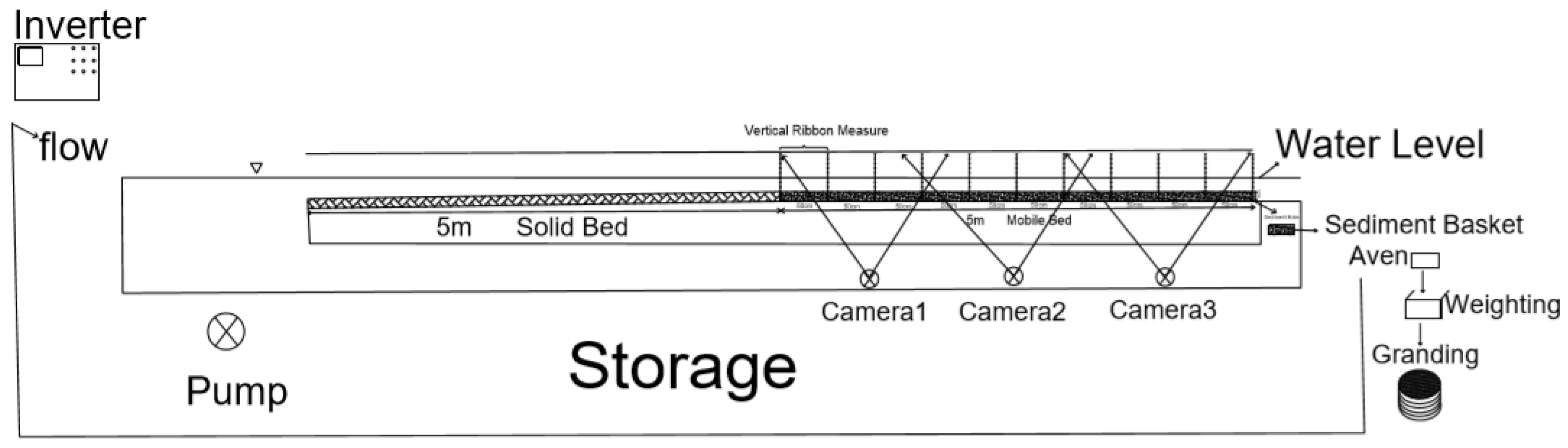
2. Materials and Methods

The experiments were carried out in a rectangular flume 10 m long, 0.3 m wide and 0.5 m deep (Figure 1). The first 5 m of the flume were roughened by gluing sediments on the bed and the second half of the flume was filled with a well-mixed layer of 10 cm of sediments. The water depth was measured at 10 points (spaced 50 cm) along the portion of the flume filled with loose sediments. Also, three cameras were installed on the side of the flume to take pictures every 20 s and to record the water depth (Figure 1). Two types of sediment mixtures (

Table 1. Sediment characteristics.

Classification	${\mathit{\sigma }}_{\mathit{g}}$(mm) *	${\mathit{D}}_{16}$(mm)	${\mathit{D}}_{84}$(mm)	${\mathit{D}}_{50}$(mm)	${\mathit{D}}_{\mathit{m}\mathit{i}\mathit{n}}$(mm)	${\mathit{D}}_{\mathit{m}\mathit{a}\mathit{x}}$(mm)
Poorly sorted	1.60	6	2.2	4.1	2	8
Well sorted	1.29	3.1	5.2	4.1	3	5

* Standard deviation of the sediment mixture.

), poorly sorted and well sorted, with the same ${\mathit{D}}_{\mathbf{50}} (4.1 \mathrm{m}\mathrm{m})$ were used in the experiments. The experiment was conducted without sediment supply from the upstream end of the flume. The entire active layer of the bed was meticulously mixed to prevent vertical layering. Sediment sizes coarser than 2 mm were categorized as gravel. The porosity was calculated 0.5 and 0.39 for well-sorted and poorly sorted sediments, respectively.

The duration and magnitude of the hydrographs was not scaled from a field model, but were designed to study the sediment transport processes; the short duration represents the flashiness (flashy means that the hydrograph depicts sharp vertical jumps and equally steep vertical declines) of events typical of ephemeral streams that these experiments aimed to investigate. The water input consisted of symmetrical triangular hydrographs with different duration and magnitude. Six different hydrographs were used (Figure 2). Four hydrographs featured approximately the same duration of 150 s but differed in terms of peak (14.77 $\mathrm{L}{\mathrm{s}}^{-1}$) for type A, (16.2$\mathrm{L}{\mathrm{s}}^{-1}$) for type D, (17.4 $\mathrm{L}{\mathrm{s}}^{-1}$) for type B and (20.2 $\mathrm{L}{\mathrm{s}}^{-1}$) for type C, and further two hydrographs had the same peak as type D (16.2$\mathrm{L}{\mathrm{s}}^{-1}$) but had longer durations of 300 s (type E) and 400 s (type F). At the beginning of every series experiment (first hydrograph), the bed was flattened and then was left untouched for the second and third hydrographs. Sediment transport rates were measured using a sediment trap located at the downstream end of the flume that was extracted and substituted with an empty one every 30 s. The frequency of collection of the basket depended on the transport rate (i.e., less frequently when the transport rate was lower). In all the tests, the slope was constant at 0.0089 $\mathrm{m}{\mathrm{m}}^{-1}$. After every run, the bed topography was surveyed using a laser distance meter.

3. Results and Discussion

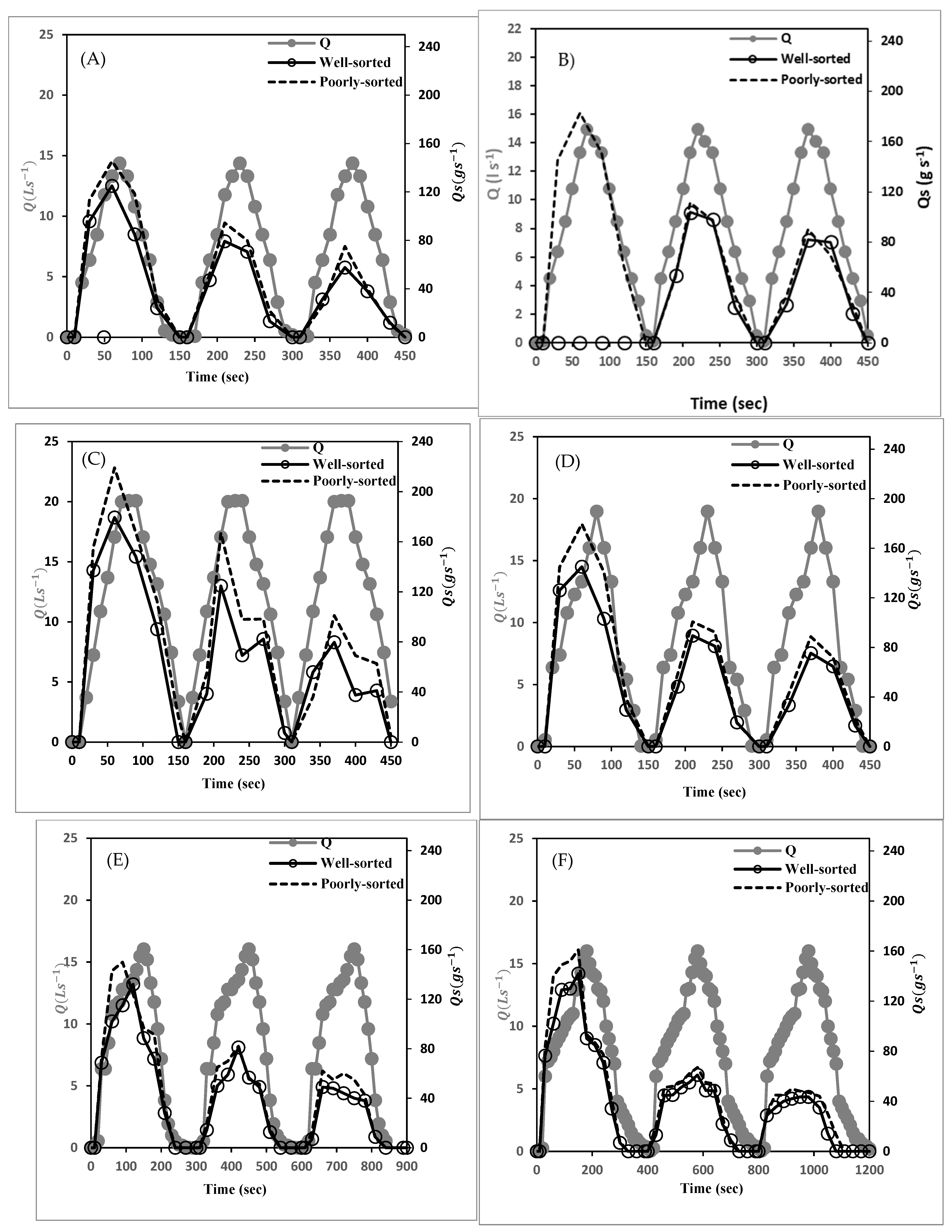
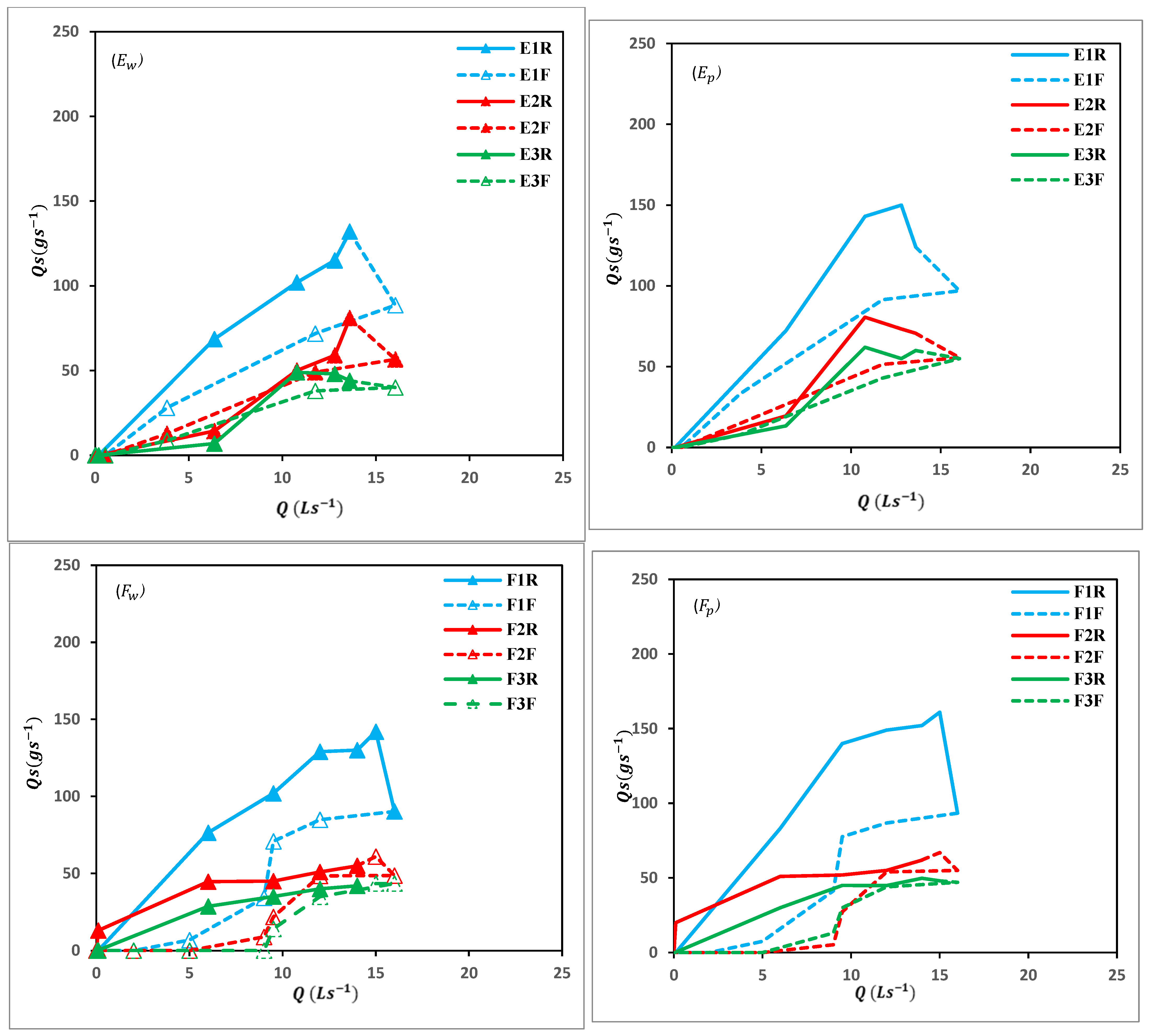
3.1. Dynamics of Sediment Transport during Repetitions of Hydrographs

Figure 3 shows the liquid and the solid discharge for the repetitions of the six types of hydrographs. Figure 3 shows that the first hydrograph of each series is the one with the highest transport rate, and that the transport rate diminishes for the second and then for the third repetition of the same hydrograph. For example, at the peak liquid discharge of 14.77 $\mathrm{L}{\mathrm{s}}^{-1}$ for hydrograph A, the first, second and third hydrographs peak at 125, 79.3 and 57.6 $\mathrm{g}{\mathrm{s}}^{-1}$, respectively, for the experiments with well-sorted sediments. For the poorly sorted sediments, the peak values of sediment discharge were 145.3, 94.3 and 61.3 $\mathrm{g}{\mathrm{s}}^{-1}$ for the first, second and third repetition, respectively. Figure 3 shows that this trend of reduction in sediment intensity at comparable discharges from the first to the third repetition is consistent for all the types of hydrographs simulated in the flume runs. The hydrograph type C, peaking at the highest discharge of 20 $\mathrm{L}{\mathrm{s}}^{-1}$, featured a peak sediment transport rate of 179.83, 124.7 and 113 $\mathrm{g}{\mathrm{s}}^{-1}$ for the well-sorted sediments and 219, 144.6 and 98 $\mathrm{g}{\mathrm{s}}^{-1}$ for the poorly sorted sediments, moving from the first to the third repetition. Figure 3 also shows that the sediment transport during the falling limb of the hydrographs tends to be lower than during the rising limbs for all hydrographs on each run. In hydrographs A, B, C and D, characterized by the same duration but different peak discharge, sediment transport rate increases with peak discharge. For instance, in the peak of the hydrographs A, B, C and D, peaking at 14.7, 17.4, 20 and 16.4 ${\mathrm{Ls}}^{-1},$ sediment transport reached values of 145,183, 219 and 179 $\mathrm{g}{\mathrm{s}}^{-1}$, respectively. Sediment transport rates for the longer duration hydrograph E varied between 159 and 49 and 149 and 62 $\mathrm{g}{\mathrm{s}}^{-1}$ for well-sorted and poorly sorted sediments, respectively. Similarly, the event F featured sediment transport rate ranging from 150 to 54 and 142 to 48 $\mathrm{g}{\mathrm{s}}^{-1}$ from the repetition 1 to the repetition 3 for poorly sorted and well-sorted sediments, respectively.

The decrease in sediment transport from the first to the second and then the first to the third repetition of a hydrograph, irrespective of their duration and magnitude and the homogeneity of the grain size, can mainly be attributed to the diminished availability of sediment due to the lack of sediment supply from the upstream end of the flume. However, it is interesting to notice that the reduction in sediment transport is influenced by the magnitude of the preceding event. The reduction in sediment transport between the first and the third event is lower for short hydrographs if compared to the longer E and F hydrographs. For instance, the reduction in sediment transport rate for hydrographs E and F from the first to the third run is 58% and 62%, respectively (for poorly sorted sediments). For the shorter hydrographs (A, B, C and D), the reduction is 53%, 53%, 54% and 50%, respectively. Interestingly, this reduction in sediment transport from one hydrograph to the following one has been reported for flume simulations with sediment recirculation, but in this case, the reduction had been attributed to the vertical winnowing of fine sediment and the progressive restructuring of coarser clasts in the first layer of sediments [5].

However, regarding the relationship between the sediment transport rate and the time duration of the hydrograph, other factors such as the shape of the hydrograph, the amount and the unsteadiness of the hydrograph are also effective in determining the rate of sediment transport. Overall, for all runs the transport rate for the poorly sorted mixture is larger than for the well sorted, in any runs. Namely, the transport rates at the peak of hydrographs were higher for the poorly sorted mixture: A (145,125) $\mathrm{g}{\mathrm{s}}^{-1},$ B (183,167) $\mathrm{g}{\mathrm{s}}^{-1},$ C (219,179) $\mathrm{g}{\mathrm{s}}^{-1},$ D (179,145) $\mathrm{g}{\mathrm{s}}^{-1},$ E (159,132) $\mathrm{g}{\mathrm{s}}^{-1}$ and F (150,129) $\mathrm{g}{\mathrm{s}}^{-1}$. In poorly sorted sediment, the coarse sediments are more exposed to the flow and the finer fractions increase the transport rate of the coarse fractions compared to well-sorted sediment. This has been observed previously too by [3,14]. In these experiments did not indicate armouring because the duration of the experiments was not sufficient for winnowing (the natural removal of fine material from a coarser sediment by flowing water) to play an important role in vertical sediment sorting either, which contributes to hampering the chances of the surface sediments to develop an armour layer. Indeed, the powerful eddies created by the larger grains can disrupt the smaller, more mobile grains, potentially causing them to be carried to the flux-measuring station. Set against this is the counter-process that proud larger grains will develop a separation ‘bubble’ in their wake and this low pressure zone will draw in fine grains to produce a wake tail (or shadow) to a pebble cluster. Also set against the disturbance and transport of finer grains by eddy shedding will be that the proudness of the coarser grains and/or the formation of clusters will take energy out of the flow and, at least as shown by previous studies [6], reduce transport ‘capacity’ and lower bedload flux. The (more) uniform bed material will undoubtedly produce fewer eddy shedding events. So, while less energy will be dissipated in the flow as a whole, the likelihood of generating large burst-sweep events will be lower. This means that fewer eddies will penetrate. The boundary layer will impinge on bed particles—this will mean less stress and lower bedload flux. In addition, in flume experiments, ref. [15] reported that the transport rate of poorly sorted sand was 1.27 to 3.19 times larger than that of well-sorted sediment under unsteady flow conditions. Ref. [15] also showed that the magnitude of bedload rate fluctuations tends to be larger in the rising limb than in the falling limb of hydrographs, which can result in a decrease in bedload sediment transport rate in subsequent hydrographs.

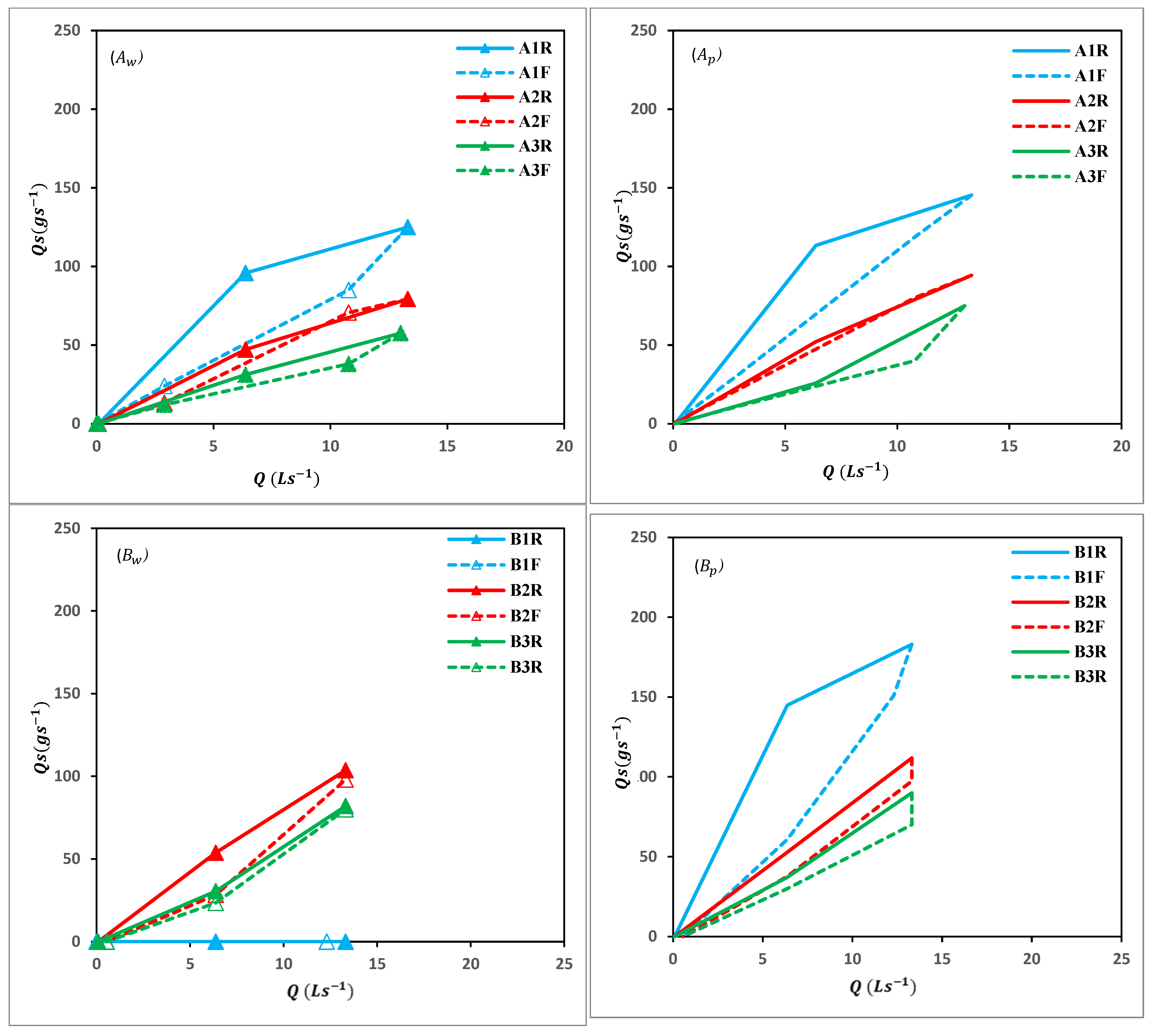
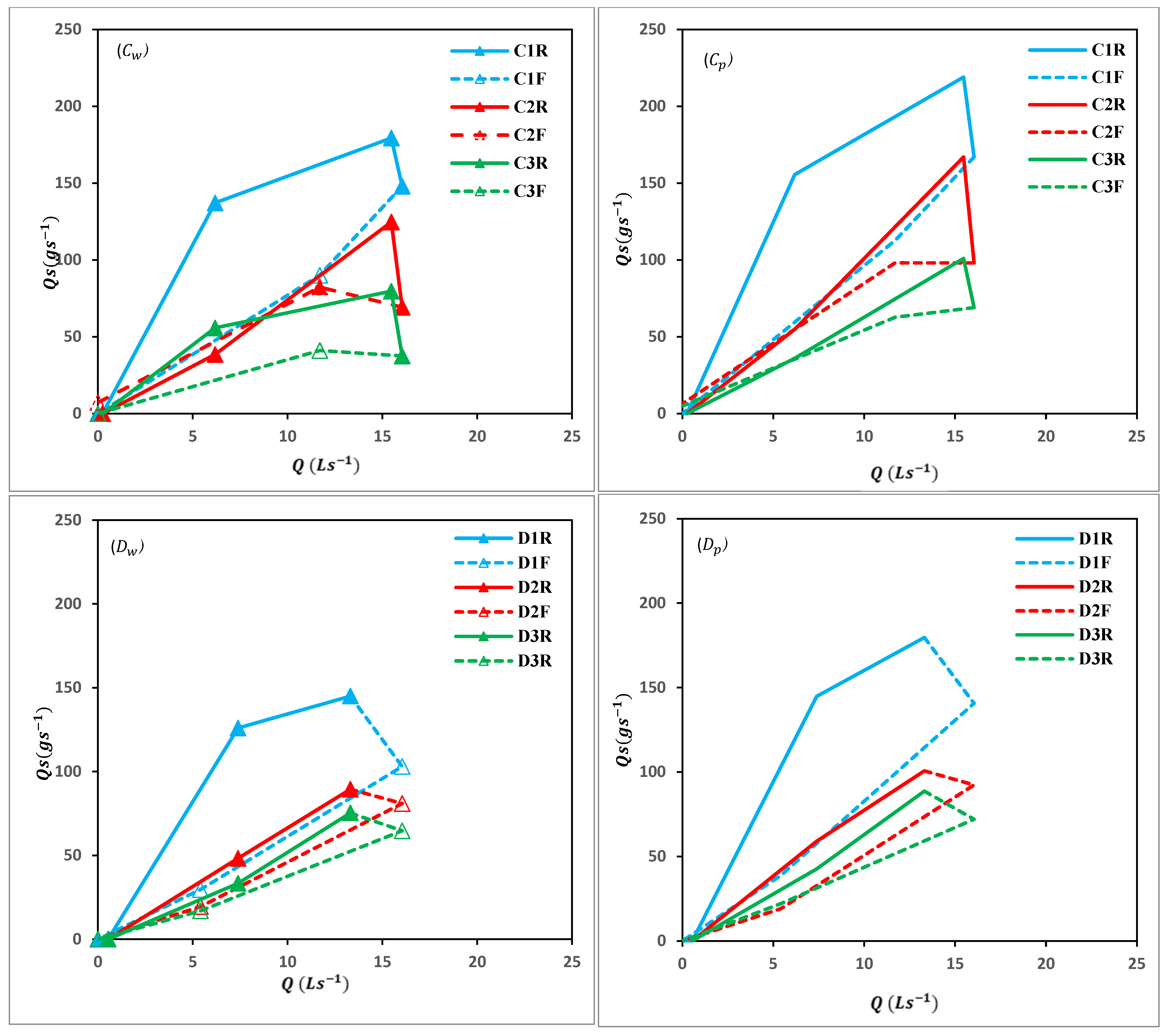
3.2. Trends of Hysteresis in the Transport of Sediments during Repetition of Hydrographs

The relationship between sediment transport rates and liquid discharge is not linear, and when there is a different trend between the rising and falling limb of a hydrograph, a hysteretic pattern can be identified. When the sediment transport rate is higher in the rising than in the falling limb of the hydrograph, then the hysteresis is clockwise, being counterclockwise when the transport rate is higher in the falling limb of the hydrograph. A recent review conducted by [10] demonstrated that gravel-bed streams, operating under no sediment feeding conditions, predominantly exhibit clockwise hysteresis. The morphology, duration and amplitude of the hydrograph, in conjunction with sediment supply conditions, dictate the hysteresis patterns and the underlying mechanisms accountable for such phenomena. Figure 4 shows that in all types of hydrographs, the sediment transport rate is higher in the rising limb, making the hysteresis clockwise. For example, during the first C repetition, at the intermediate discharge of 12 $\mathrm{L}{\mathrm{s}}^{-1}$, sediment transport rates reached 180,80 and 145,75 $\mathrm{g}{\mathrm{s}}^{-1}$ during the rising and falling limb, for poorly sorted and well-sorted sediment, respectively. It is interesting to notice that, although all hydrograph types are clockwise, the degree of hysteresis diminishes on the second and third repetition. This suggests that since there is no sediment supply and high shear stress and turbulent flow conditions, the sediment rate in the rising limb of the hydrographs is higher than in the falling limb. For all hydrographs in the first, second and third repetitions, there is no armouring and the size of sediment particles transported was coarser than the median size of the bed, which can influence the dynamics of sediment transport. For example, coarser sediment particles tend to be more mobile and can be transported more easily during the rising hydrograph limb, leading to clockwise hysteresis [4]. As a consequence, sediment size plays a significant role in the emergence of hysteresis patterns in sediment transport. The magnitude of sediment particles exerts influence over the direction of hysteresis, sediment availability, sediment transport thresholds, sediment dynamics and the processes of sediment deposition and resuspension. Many factors have been suggested to contribute towards creating hysteresis in sediment transport rates. Hysteretic behaviour is thought to be, in part, a function of the availability of sediment in the river that can be transported [2]. Given the lack of sediment supply, at the upstream end of the flume a scour developed progressively, thus causing a reduction in sediment transport. Ref. [3] showed that if the run time is not too long, the flume is not depleted of sediment, and so winnowing may not be the dominant phenomenon governing coarsening. The sediment supply significantly influences the formation of bed surface armouring. Apart from the flow regime, streams characterized by a relatively limited sediment supply are prone to exhibit a substantial degree of armouring. Conversely, streams with adequately high rates of sediment supply are unlikely to undergo significant vertical sediment sorting, thus resulting in a diminished degree of armouring. In the case of the short-duration hydrographs, the duration of the experiment was not sufficient for winnowing to play an important role in vertical sediment sorting. It is interesting to note that the hysteresis remains clockwise for the second and third repetitions for the hydrographs $\left({\mathit{A}}_{\mathit{w}},{\mathit{A}}_{\mathit{p}}\right),\left({\mathit{B}}_{\mathit{w}},{\mathit{B}}_{\mathit{p}}\right),\left({\mathit{C}}_{\mathit{w}},{\mathit{C}}_{\mathit{p}}\right),{(\mathit{D}}_{\mathit{w}}$,${\mathit{D}}_{\mathit{p}}),$ (${\mathit{E}}_{\mathit{w}},{\mathit{E}}_{\mathit{p}})$ and $\left({\mathit{F}}_{\mathit{w}},{\mathit{F}}_{\mathit{p}}\right)$, where the subscripts w and p refer to the well-sorted and poorly sorted sediment mixtures, respectively. Specifically, a reduction in sediment availability during the ascending limbs of hydrographs can contribute to the occurrence of clockwise hysteresis [16]. One explanation is that a less armoured bed allows for easier breakage and mobilization of the coarse sediment particles during the rising limb of the hydrograph, leading to higher transport rates compared to the falling limb. The armoured bed requires higher shear stresses to initiate sediment motion, resulting in lower transport rates on the rising limb compared to the falling limb when the bed has been partially mobilized [5]. Ref. [3] inferred from their experiments that, since winnowing is not reasonable for the development of armouring in rivers with flash floods (i.e., in arid streams), the hysteresis is due to the size of the transported material or morphodynamic factors. In this study, short hydrographs do not develop evident armouring either, and therefore, hysteresis is weak due to the large amount of fine sediment moved during the falling limb of the hydrograph. This occurrence can be attributed to the exceedingly high sediment transfer within the riverbed, resulting from the exceptionally high flow rate. [6] investigated the sedimentary conditions of some ephemeral streams and showed that the transport of sediments in a sequence of flood reveals clockwise hysteresis, and argued that the lack of armour layer could be due to the short duration of floods that does not allow the progressive removal of finer fractions form the channel bed as it would instead occur in permanent rivers characterized by much longer flood events. Further investigation in the relative role of the duration of hydrographs on sediment transport and armour layer development could shed further light on the impact that climate change could cause on the duration of floods and sequencing of flood events, and in turn to the size of sediments on river beds, which determines important ecological properties of habitats for macroinvertebrates and fish.

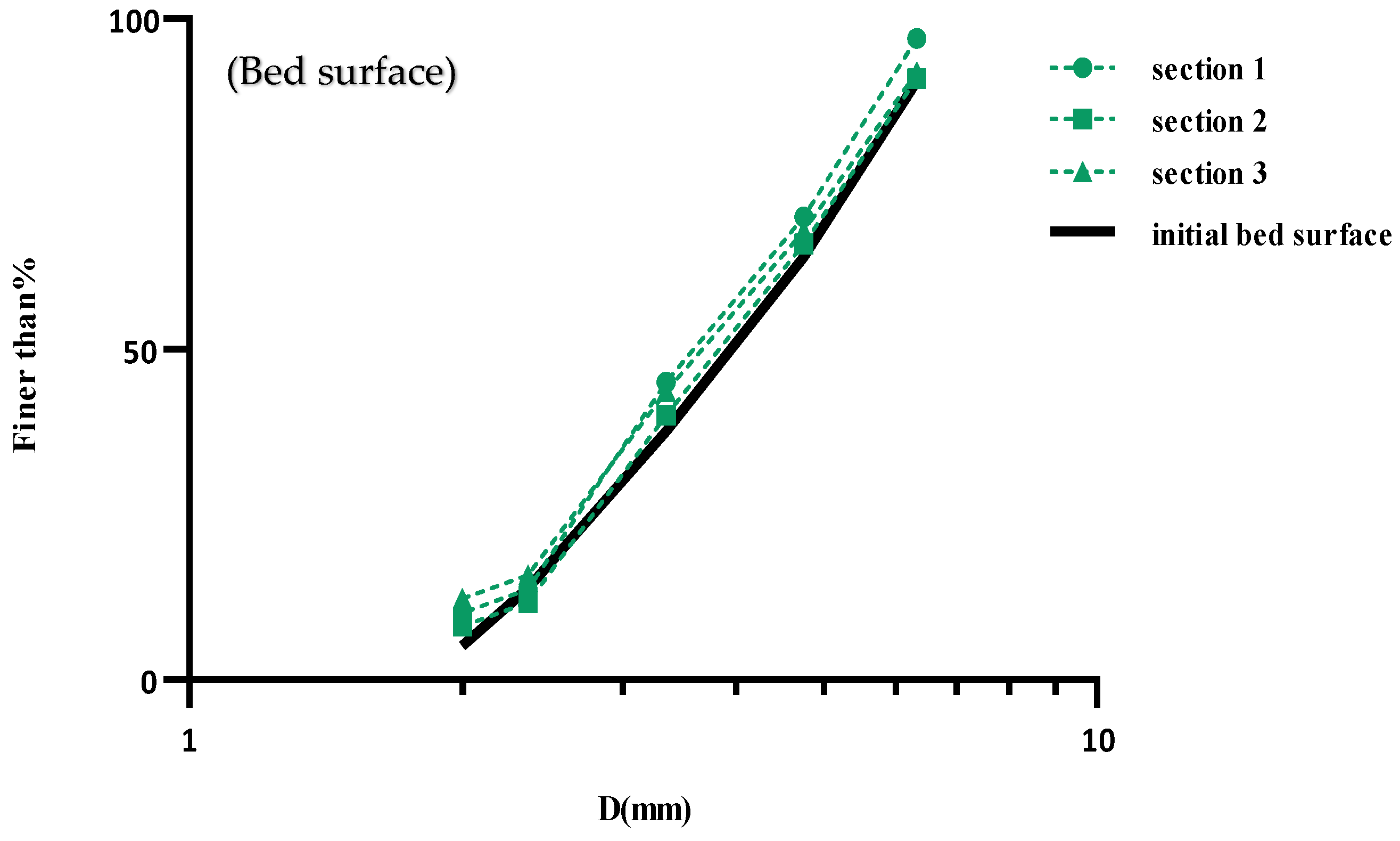
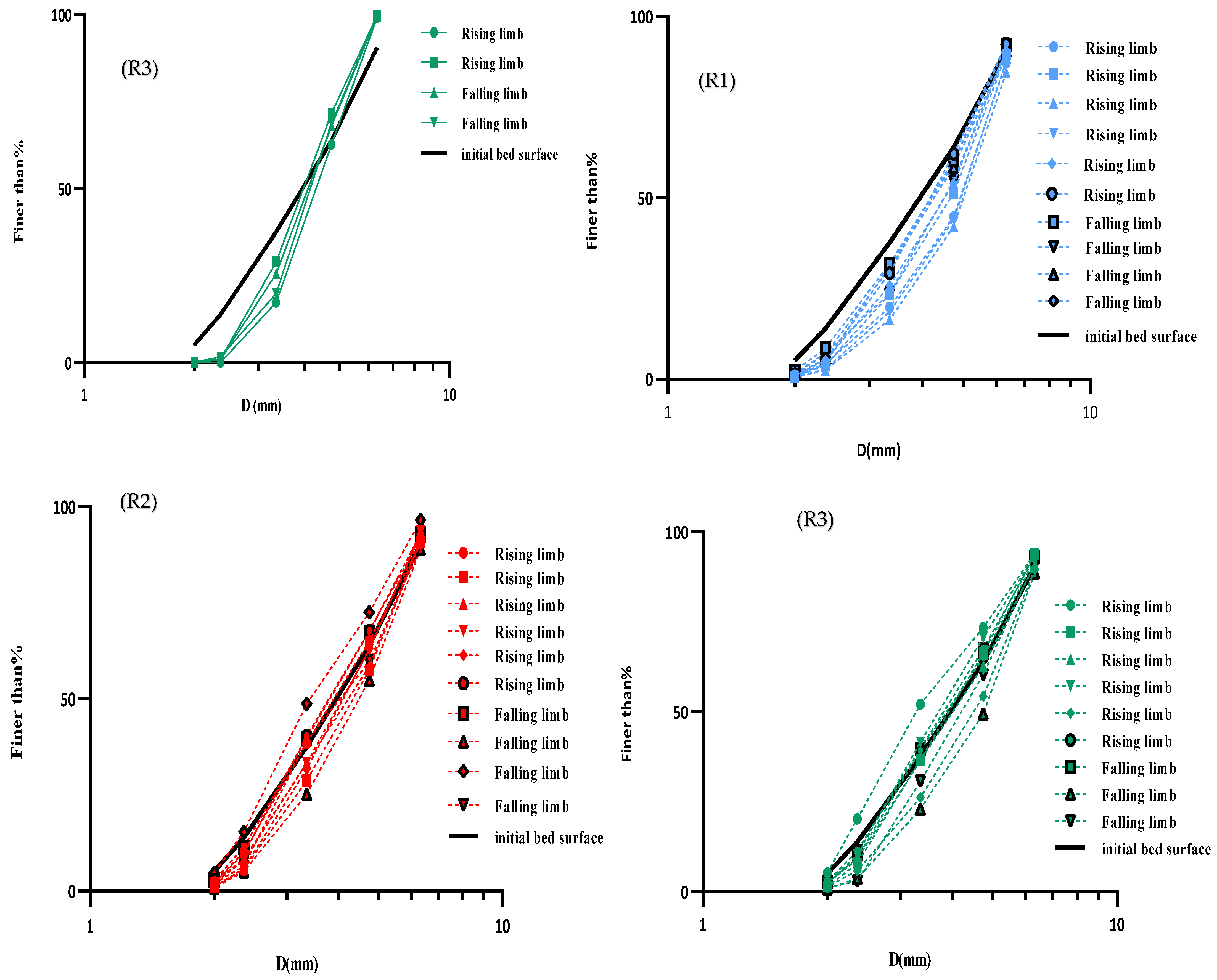
3.3. Changes in Bed Grain Size during the Repetition of Hydrographs

For some of the experiments, after the third repetition, in three sections of the flume (upstream, central and the downstream end of the flume), samples 30 cm wide, 20 cm long and 3 cm deep of sediments were taken to analyse the grain size. The results in Figure 5 show that the sediment of bed surface did not become coarser after the hydrographs, as one would have expected, and also show that there is no downstream trend in terms of grain size. Ref. [3] also showed that armouring was not developing in experiments with sand and gravel, sediment-starving conditions and short hydrographs. Sediment sorting depends on a range of factors such as initial bed surface, sediment supply, flow magnitude and sediment grain size distribution. The literature suggests that the surface grain size in flume experiments under no-feeding conditions should become coarser due to the selective transport of finer fractions [2]. However, the runs in our experiments are so short that this selective transport cannot develop properly, and even the longer hydrographs are not long enough to have a falling limb with enough competence to transport finer fractions and leave just the coarser grains on the first sediment layer. Figure 6 shows the grain size of the transported sediments captured at the downstream end of the flume during the experiments. The larger particles are transported in the rising limb of the hydrograph and smaller particles are transported in the falling limb of the hydrograph. This is observed in the repetitions too, where the transported sediment size is larger if compared to the initial substrate particles. The outcome of this analysis aligns with previous observations [3], indicating that the size distribution of the bed surface material during the experiment for a short hydrograph was comparatively finer than the initial bed and for sediment transported greater than falling limb, which confirms the lack of armouring. The ${\mathit{D}}_{\mathbf{84}}$ of transported sediment peaks at 6.2 mm during the peak discharge of the first run of the C event. Interestingly, the grain size of the transported sediment decreased in the subsequent hydrographs, being ${\mathit{D}}_{\mathbf{84}}$ 5.9 mm and 5.6 mm for the peak of the second and third repetitions, respectively [3]. The symmetrical shape of the hydrograph plays a significant role in the development of the bed surface when the time duration of the hydrograph is short. Moreover, the sediment grain size distribution of the surface becomes finer than the initial surface sediments. The reduction in the size of transported sediments in the rising limb compared to the falling limb in the first repetition of hydrograph C was observed to be 4.8% and 13% for D₈₄ and D₅₀, respectively. Similarly, for hydrograph F, the reduction in transported sediments size was observed to be 9% and 14% for ${\mathit{D}}_{\mathbf{84}}$ and ${\mathit{D}}_{\mathbf{50}}$, respectively. For the second repetitions in the hydrograph sequences C and F, the grain size of the transported sediments decreased to 1.6% and 4.8%, 5.4% and 11% in terms of D₈₄ and D₅₀, respectively. Ref. [5] also showed a progressive reduction in the size of transported sediments on repetitions of hydrographs, but in that case that was due to the progressive vertical sorting of sediments because the runs were conducted under sediment recirculating conditions. Here the results are similar, but refer to no-feeding conditions, thus this is likely to be due to the particularly short duration of the hydrographs. Notably, for hydrograph C, the standard deviation of bedload transport rate around the mean exhibited a considerable difference between the highest discharges (0.067 ${\mathrm{m}}^2{\mathrm{s}}^{-1}$) and the lowest discharges (0.049 ${\mathrm{m}}^2{\mathrm{s}}^{-1}$), with the former displaying fluctuations up to 30% greater than the latter. The standard deviation of bedload transport rate between the shortest and longest time durations (D and F) with equal peak discharge and variable time (150 and 400 s) was 26%. The sediment transport rate in all hydrographs during the initial run shows a higher magnitude in the rising limb compared to the falling limb. For example, the sediment transport rate for the highest peak in hydrograph C was recorded at 80% and 75% for poorly sorted and well sorted, respectively. Furthermore, between rising and falling limbs in the longest hydrograph (F), there was a disparity in sediment transport rate, amounting to 50% and 48% for non-uniform and uniform mixtures, respectively. These findings underscore the significant impact of the hydrograph’s peak flow rate during the rising limb on sediment transport.

4. Conclusions

Given the of heavy and convective rains in arid and semi-arid regions, floods are generally flashy and short. Short floods are not explored enough in flume studies, and here we investigated the impact of flow sequence (i.e., repetition of floods) on sediment transport, using two types of beds, namely well sorted and poorly sorted. During all simulated runs, sediment transport was higher in the rising limb of hydrographs than in the falling limb, exhibiting clockwise hysteresis for both well-sorted and poorly sorted sediment mixtures. We also found that sediment transport rates were higher in the poorly sorted bed compared to the well-sorted bed. Additionally, in the second and third executions for both types of beds, there was a noticeable decrease in the amount of sediment transport, due to the progressive reduction in sediment availability. However, armouring phenomena were not detected during any of the conducted experiments, and in some cases the bed surface became finer. The lack of sediment feeding at the upstream end of the flume did not create armouring and the bed surface size tended to be finer. Although this is not unexpected for the uniform grain size mixture, for the heterogeneous mixture it reveals that the very flashy nature of the hydrographs did not allow for the surface sediments to coarsen due to selective transport. Indeed, channel beds of streams subject to flash floods in arid areas are typically not armoured. Moreover, our findings revealed that the size of transported sediment particles in the rising limb of the hydrograph exceeded those in the descending limb. Furthermore, with each successive run, the size of transported sediment particles consistently decreased. In conclusion, our study provides compelling evidence regarding the influence of flow sequence on sediment transport. The observed hysteresis patterns, differences in sediment transport rates between well-sorted and poorly sorted beds and the consistent reduction in sediment particle size with each run contribute to a comprehensive understanding of this complex phenomenon.