Assessment of Site Effects and Numerical Modeling of Seismic Ground Motion to Support Seismic Microzonation of Dushanbe City, Tajikistan
Abstract
1. Introduction

2. Study Area
2.1. Seismicity of the Region
2.2. Seismic Hazard Affecting Dushanbe City
3. Materials and Methods
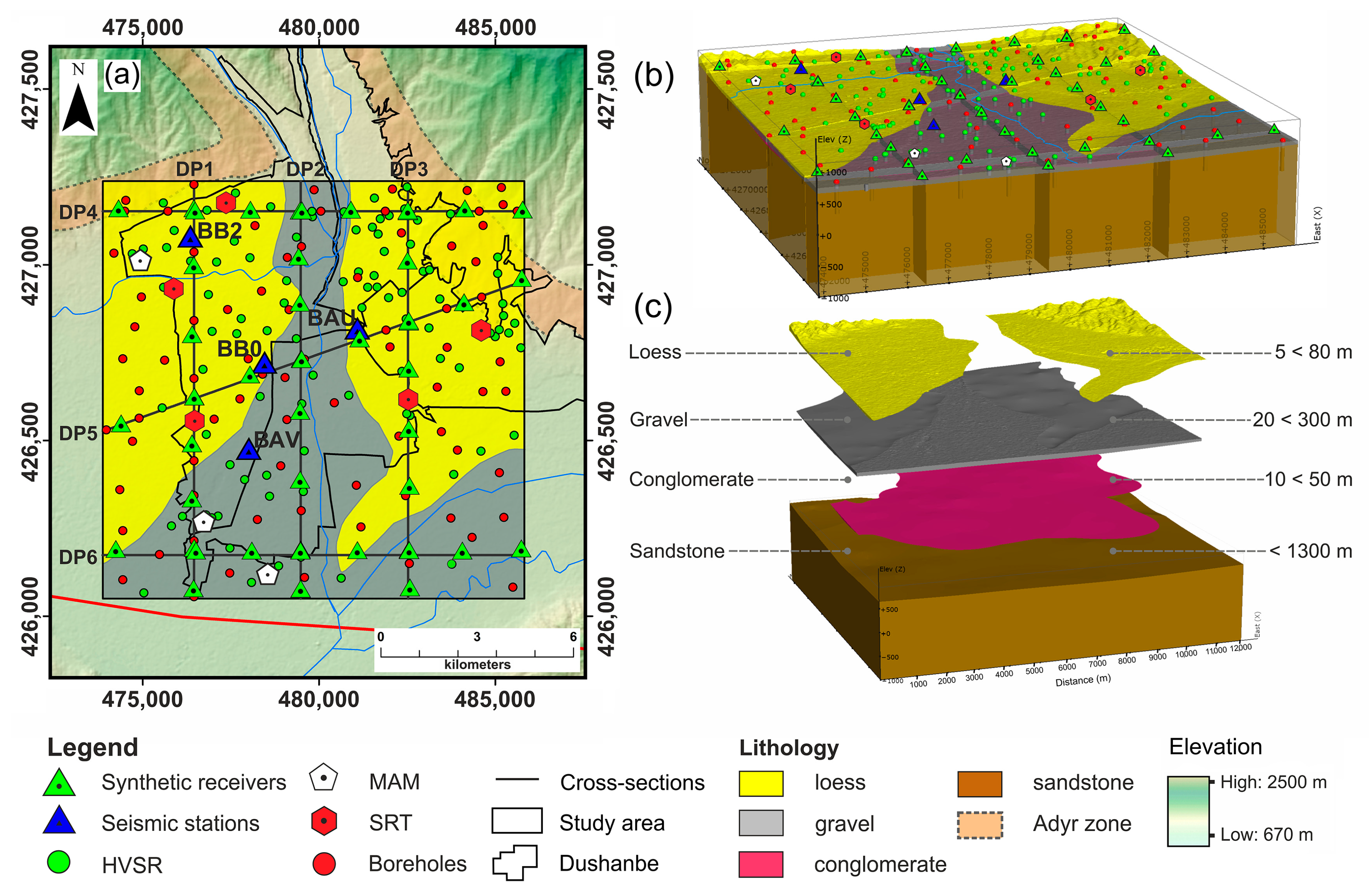
3.1. Geophysical Surveys in Dushanbe
3.2. Summary of Ambient Noise Measurements
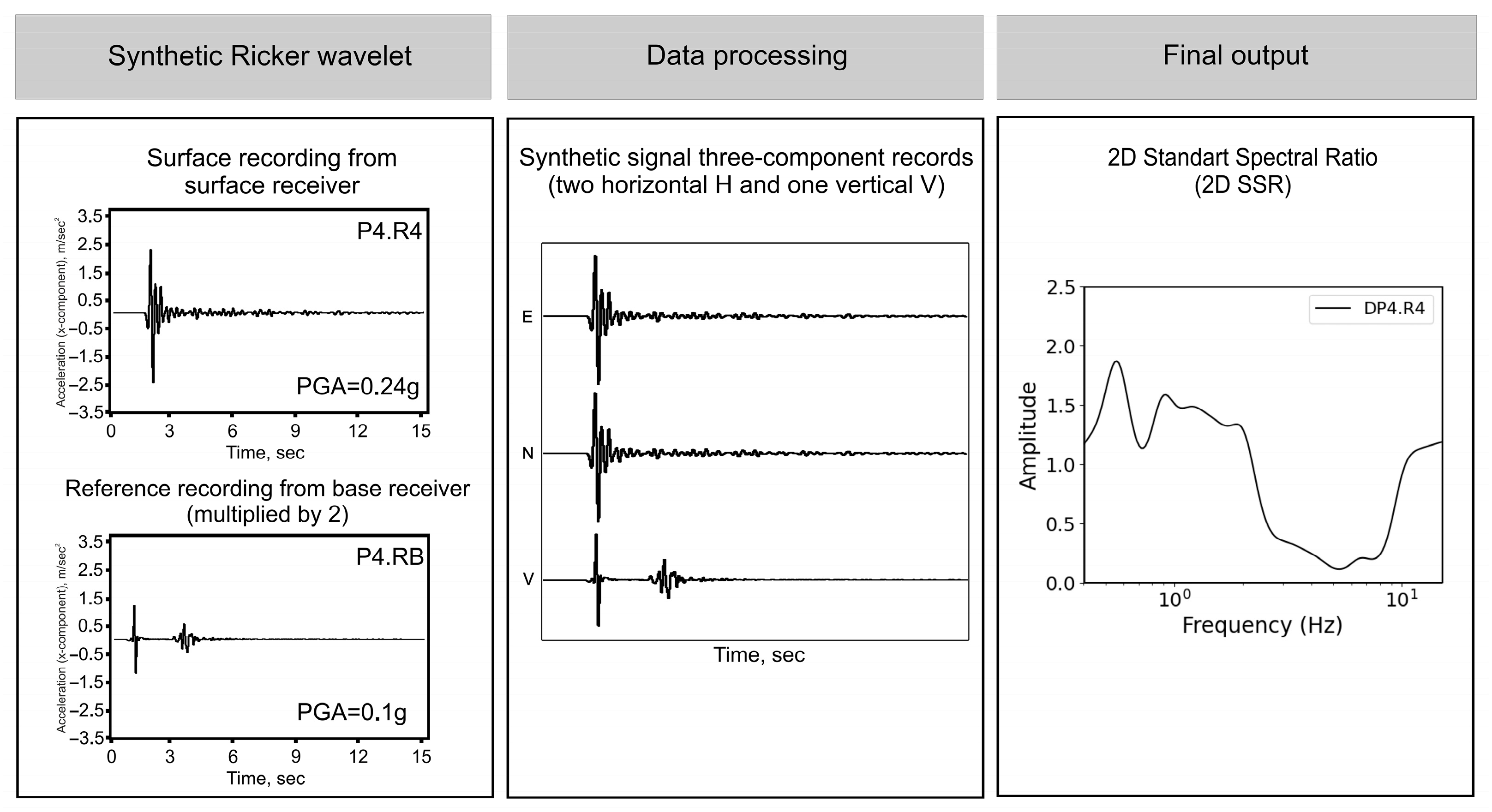
3.3. Earthquake Records and SSR Method
3.4. Microtremor Array Measurements and Seismic Refraction Tomography for the Vs30 Distribution Map
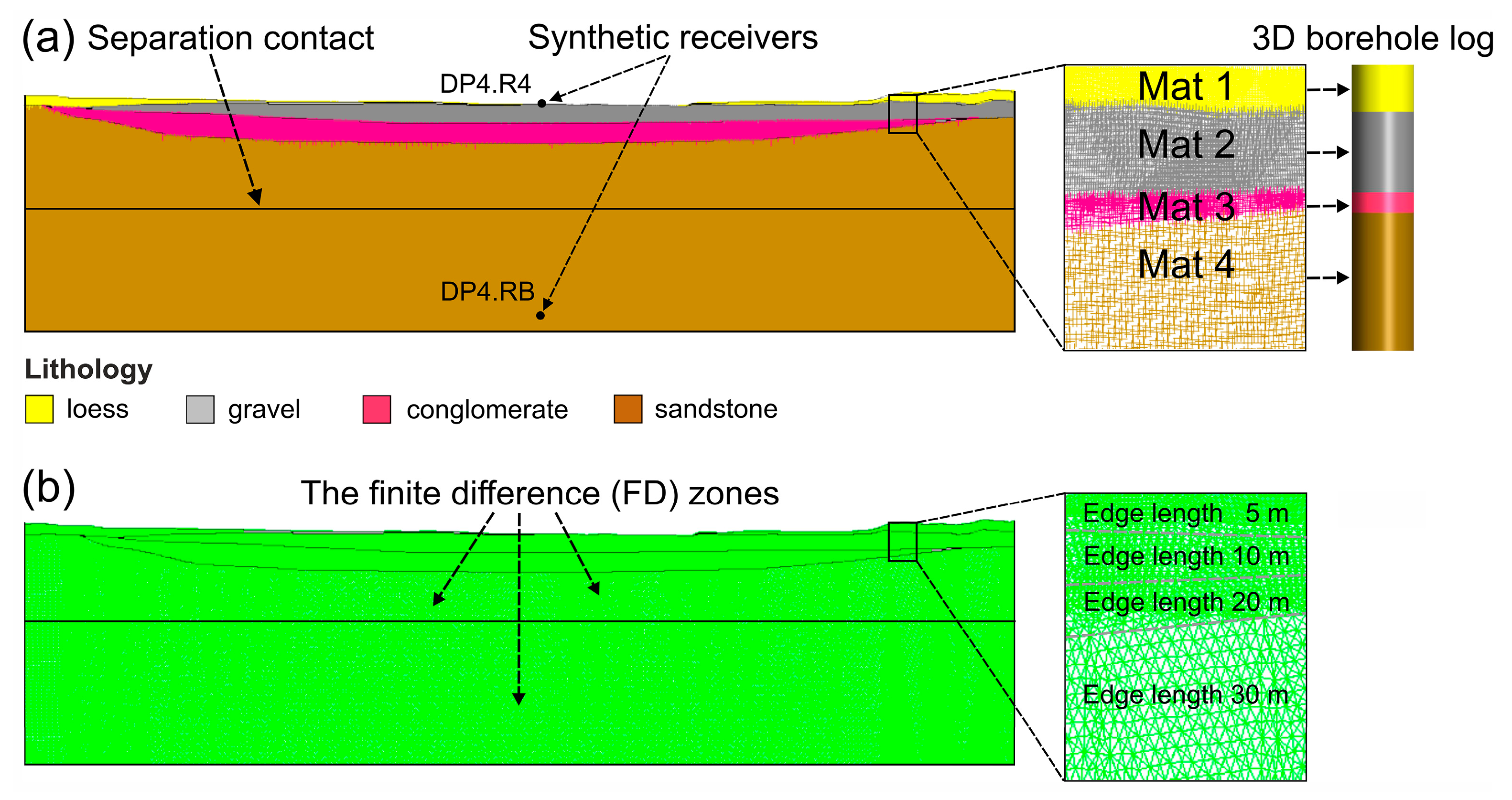
3.5. Three-Dimensional Geomodeling
3.6. Two-Dimensional Dynamic Numerical Modeling Methodology
3.6.1. The Static Model
3.6.2. The Dynamic Model
4. Results
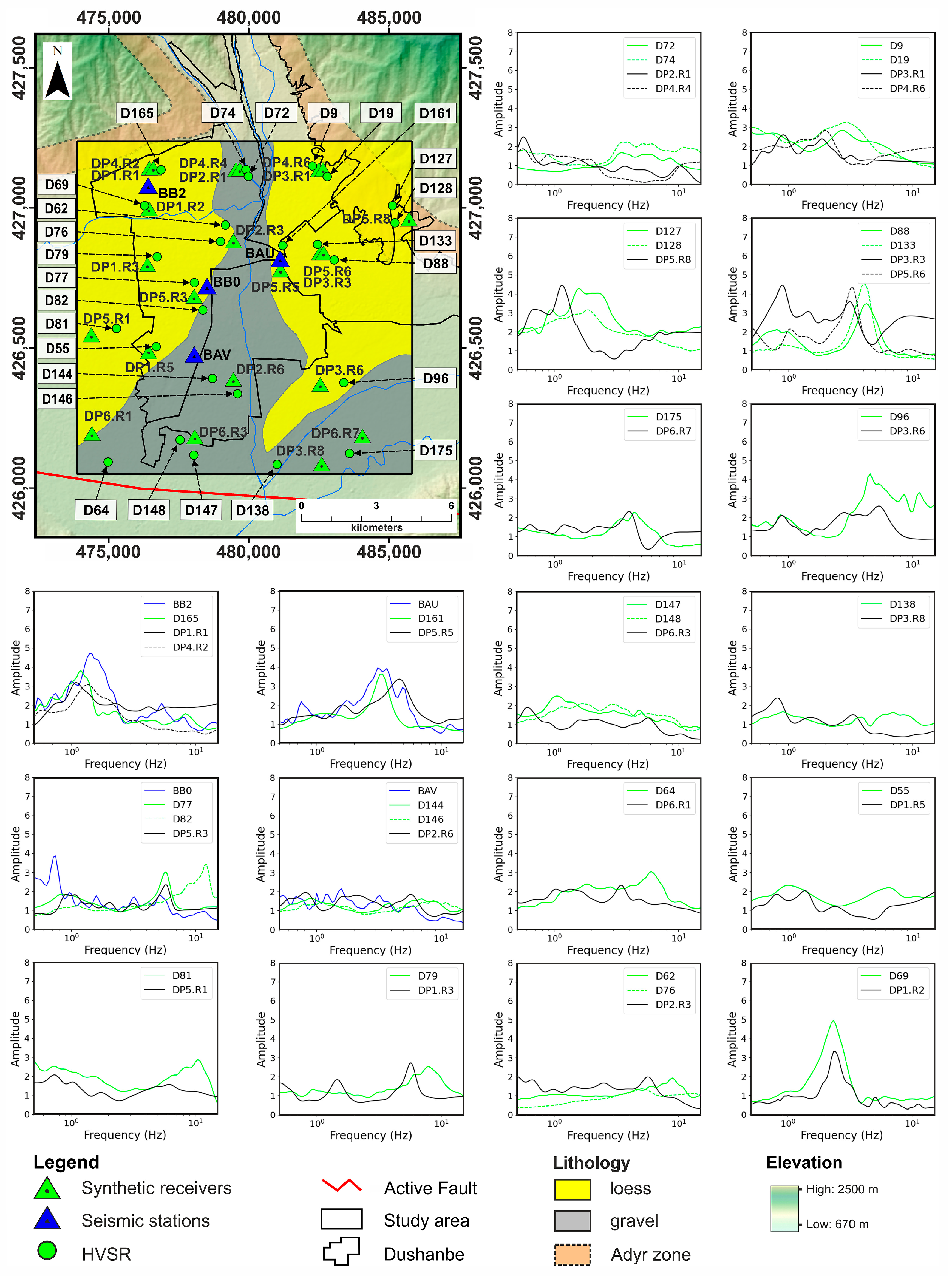
4.1. SSR Results
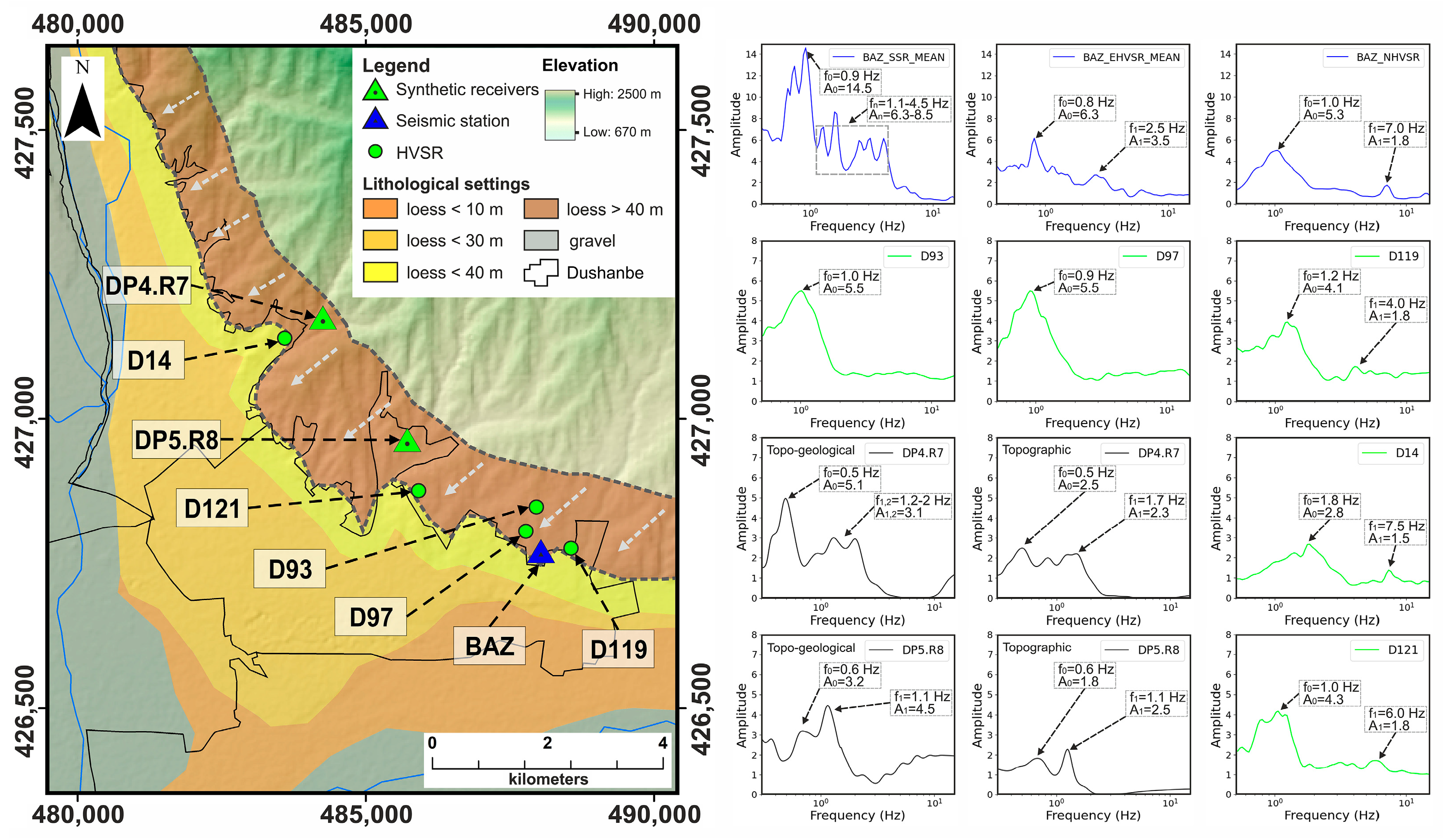
4.2. Maps of the Distribution of Site Effect Inferred from HVSR Results in the Study Area
4.3. Three-Dimensional Geological Model Development
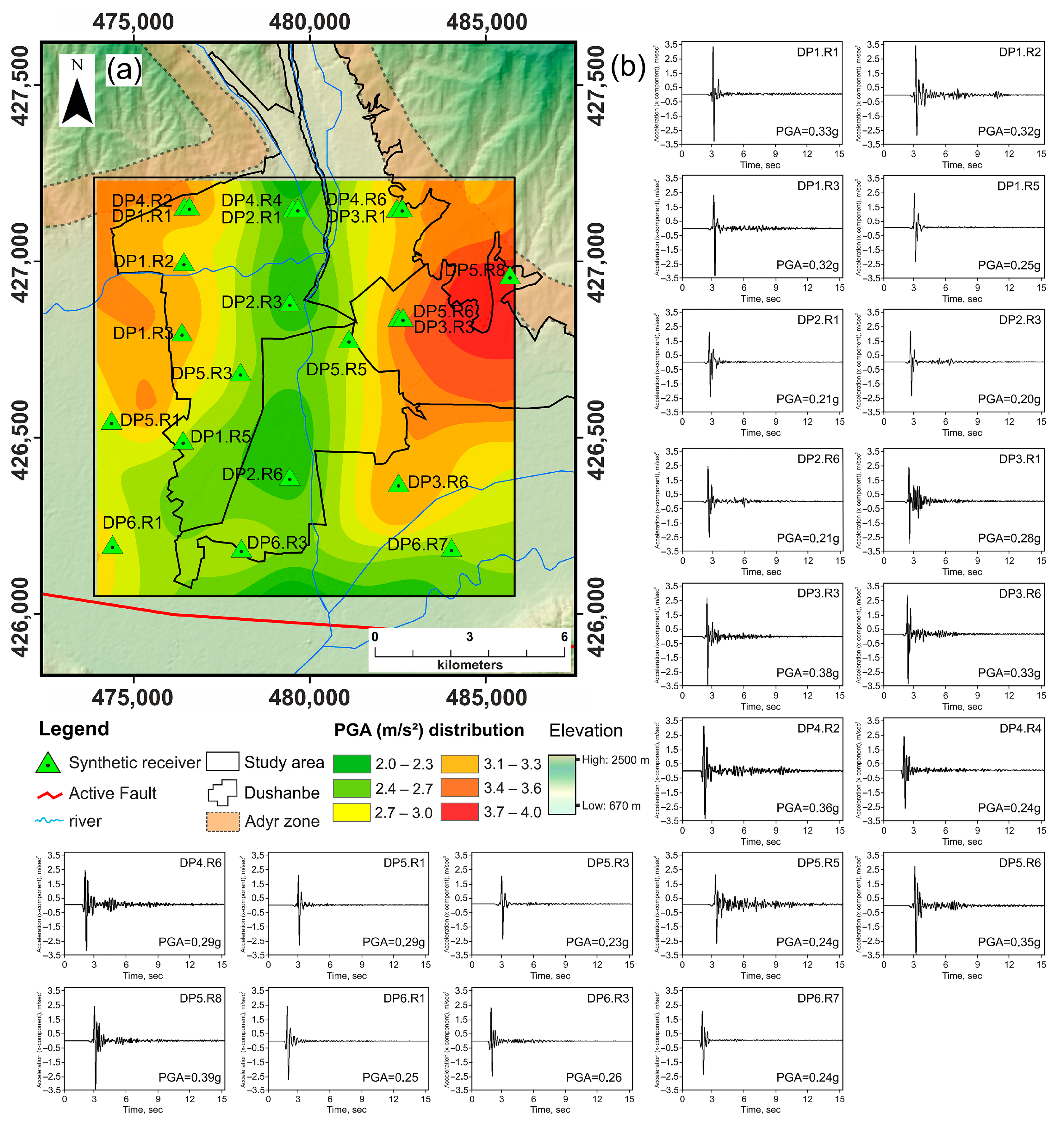
4.4. Two-Dimensional Dynamic Numerical Modeling Results
4.4.1. Two-Dimensional Dynamic Numerical Modeling Applied to Six Profiles
4.4.2. Two-Dimensional Dynamic Numerical Model Processing
4.5. Results of 2D Dynamic Numerical Models: 2D SSR Analysis
4.5.1. Comparison of Results in the SW to the Central Part of the Study Area on Gravels
4.5.2. Comparison of Results in the NW, NE, and SE Part of the Study Area on Loess
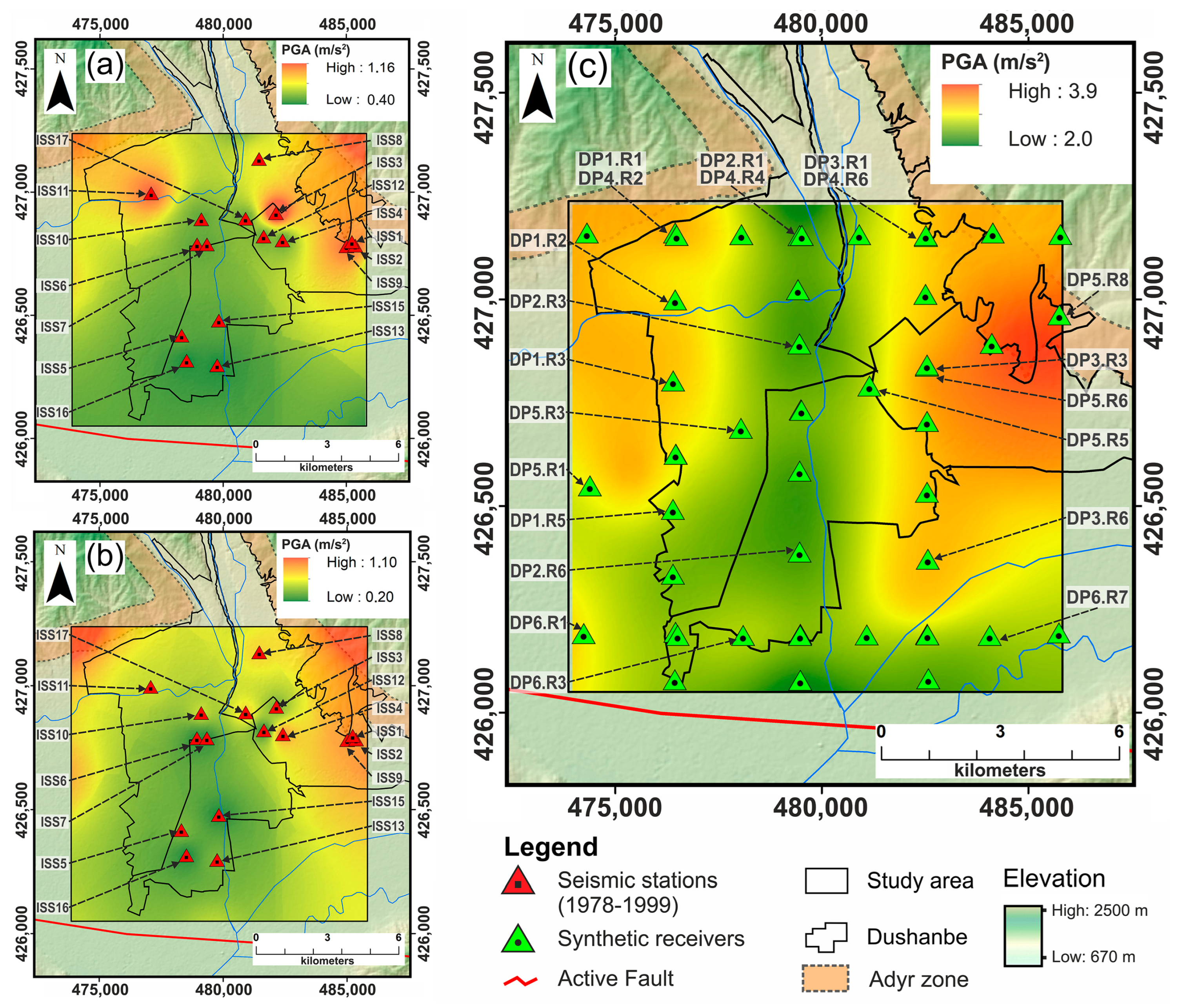
4.6. PGA Distribution Inferred from 2D Dynamic Numerical Simulations
5. Discussion
5.1. Analysis of the Site and Topographic Effects and Their Impact on the NE and SE Sites Using Dynamic Numerical Modeling and, in Comparison, with the Results of HVSRs and SSR
5.2. Comparing 2D Dynamic Numerical Modeling Results with Historical Earthquakes in Dushanbe
5.3. Comparison of the Results of 2D Dynamic Numerical Modeling of PGA with the Results of the HVSR Site Effects: The Importance of the Topographic Amplification Effects
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Equations Used to Determine Model Parameters on the Base of the P-Wave Velocity Vp (m/s), Shear Wave Velocity Vs (m/s) and Dry Density of Rock ρ (kg/m³)

References
- Boncio, P.; Lavecchia, G.; Pace, B. Defining a model of 3D seismogenic sources for Seismic Hazard Assessment applications: The case of central Apennines (Italy). J. Seismol. 2004, 8, 407–425. [Google Scholar] [CrossRef]
- Frischknecht, C.; Rosset, P.; Wagner, J.-J. Toward Seismic Microzonation—2-D Modeling and Ambient Seismic Noise Measurements: The Case of an Embanked, Deep Alpine Valley. Earthq. Spectra 2005, 21, 635–651. [Google Scholar] [CrossRef]
- Smerzini, C.; Pitilakis, K. Seismic risk assessment at urban scale from 3D physics-based numerical modeling the case of Thessaloniki. Bull. Earthq. Eng. 2018, 16, 2609–2631. [Google Scholar] [CrossRef]
- Primofiore, I.; Baron, J.; Klin, P.; Laurenzano, G.; Muraro, C.; Capotorti, F.; Amanti, M.; Vessia, G. 3D numerical modelling for interpreting topographic effects in rocky hills for Seismic Microzonation: The case study of Arquata del Tronto hamlet. Eng. Geol. 2020, 279, 105868. [Google Scholar] [CrossRef]
- Priolo, E. Numerical Simulation of the Reference Ground Motion in Fabriano. 1999. Available online: http://www.iitk.ac.in/nicee/wcee/article/2497.pdf (accessed on 2 October 2023).
- Laurenzano, G.; Priolo, E. Numerical modelling of earthquake strong ground motion in the area of Vittorio Veneto (NE Italy). Boll. Geofis. Teor. Appl. 2008, 49, 401–425. Available online: https://ricerca.ogs.it/retrieve/de024c95-3d7f-4ad9-e053-3a05fe0aa3e3/632%20LAURENZANO_.pdf (accessed on 21 October 2023).
- Pilz, M.; Parolai, S.; Stupazzini, M.; Paolucci, R.; Zschau, J. Modelling basin effects on earthquake ground motion in the Santiago de Chile basin by a spectral element code. Geophys. J. Int. 2011, 187, 929–945. [Google Scholar] [CrossRef]
- Maufroy, E.; Chaljub, E.; Hollender, F.; Bard, P.-Y.; Kristek, J.; Moczo, P.; De Martin, F.; Theodoulidis, N.; Manakou, M.; Guyonnet-Benaize, C.; et al. 3D numerical simulation and ground motion prediction: Verification, validation and beyond–Lessons from the E2VP project. Soil. Dyn. Earthq. Eng. 2016, 91, 53–71. [Google Scholar] [CrossRef]
- Pilz, M.; Parolai, S.; Leyton, F.; Campos, J.; Zschau, J. A comparison of site response techniques using earthquake data and ambient seismic noise analysis in the large urban areas of Santiago de Chile. Geophys. J. Int. 2009, 178, 713–728. [Google Scholar] [CrossRef]
- Soto, V.; Sáez, E.; Magna-Verdugo, C. Numerical modelling of 3D site-city effects including partially embedded buildings using spectral element methods. Application to the case of Viña del Mar city, Chile. Eng. Struct. 2020, 223, 0141–0296. [Google Scholar] [CrossRef]
- Pilz, M.; Bindi, D.; Boxberger, T.; Hakimov, F.; Moldobekov, B.; Murodkulov, S.; Orunbaev, S.; Pittore, M.; Stankiewicz, J.; Ullah, S. First Steps toward a Reassessment of the Seismic Risk of the City of Dushanbe (Tajikistan). Seismol. Res. Lett. 2013, 84, 1026–1038. [Google Scholar] [CrossRef]
- Petrovic, B.; Bildi, D.; Pilz, M.; Serio, M.; Orunbaev, S.; Niyazov, J.; Hakimov, F.; Yasunov, P.; Begaliev, U.; Parolai, S. Building monitoring in Bishkek and Dushanbe by the use of ambient vibration analysis. Ann. Geophys-Italy 2015, 58, S0110. [Google Scholar] [CrossRef]
- Vessia, G.; Laurenzano, G.; Pagliaroli, A.; Pilz, M. Seismic site response estimation for microzonation studies promoting the resilience of urban centers. Eng. Geol. 2021, 284, 106031. [Google Scholar] [CrossRef]
- Ulysse, S.; Boisson, D.; Prépetit, C.; Havenith, H.B. Site Effect Assessment of the Gros-Morne Hill Area in Port-au-Prince, Haiti, Part A: Geophysical-Seismological Survey Results. Geosciences 2018, 8, 142. [Google Scholar] [CrossRef]
- Brando, G.; Pagliaroli, A.; Cocco, G.; Di Buccio, F. Site effects and damage scenarios: The case study of two historic centers following the 2016 Central Italy earthquake. Eng. Geol. 2020, 272, 105647. [Google Scholar] [CrossRef]
- Bard, P.-Y. Microtremor measurements: A Tool for Site Effect Estimation? Eff. Surf. Geol. Seism. Motion 1999, 3, 1251–1279. [Google Scholar]
- Bard, P.-Y. Effects of Surface Geology on Ground Motion: Recent Results and Remaining Issues. In Proceedings of the 10th European Conference on Earthquake Engineering, Vienna, Austria, 28 August–2 September 1994; Duma, G., Ed.; Balkema: Rotterdam, The Netherlands, 1995; pp. 305–323. Available online: https://www.researchgate.net/profile/Pierre-Yves-Bard/publication/235623104_Effects_of_surface_geology_on_ground_motion_Recent_results_and_remaining_issues/links/56faa62f08aef6d10d904c02/Effects-of-surface-geology-on-ground-motion-Recent-results-and-remaining-issues.pdf (accessed on 2 October 2023).
- Wald, D.J.; Allen, T.I. Topographic Slope as a Proxy for Seismic Site Conditions and Amplification. Bull. Seismol. Soc. Am. 2007, 97, 1379–1395. [Google Scholar] [CrossRef]
- Fäh, D.; Rüttener, E.; Noack, T. Kruspan P. Microzonation of the city of Basel. J. Seismol. 1997, 1, 87–102. [Google Scholar] [CrossRef]
- Lacave, C.; Bard, P.-Y.; Koller, M.G. Microzonation: Techniques and examples. Block 1999, 15, 23. [Google Scholar]
- Havenith, H.-B.; Fäh, D.; Polom, U.; Roullé, A. S-wave velocity measurements applied to the seismic microzonation of Basel, Upper Rhine Graben. Geophys. J. Int. 2007, 170, 346–358. [Google Scholar] [CrossRef]
- Bonnefoy-Claudet, S.; Cotton, F.; Bard, P.-Y. The nature of noise wave field and its applications for site effects studies. Earth Sci. Rev. 2006, 79, 205–227. [Google Scholar] [CrossRef]
- Chaljub, E.; Cornou, C.; Bard, P.-Y.; Cotton, F.; Guéguen, P. Numerical benchmark of 3D ground motion simulation in the valley of Grenoble, French Alps. In Proceedings of the Third International Symposium on the Effects of Surface Geology on Seismic Motion, Grenoble, France, 30 August 2006; p. SB1. [Google Scholar]
- Laurenzano, G.; Priolo, E.; Tondi, E. 2D numerical simulations of earthquake ground motion: Examples from the Marche Region, Italy. J. Seismol. 2008, 12, 395–412. [Google Scholar] [CrossRef]
- Baron, J.; Primofiore, I.; Klin, P.; Vessia, G.; Laurenzano, G. Investigation of topographic site effects using 3D waveform modelling: Amplification, polarization and torsional motions in the case study of Arquata del Tronto (Italy). Bull. Earthq. Eng. 2022, 20, 677–710. [Google Scholar] [CrossRef]
- Wang, F.; Ma, Q.; Tao, D.; Xie, Q. A numerical study of 3D topographic site effects considering wavefield incident direction and geomorphometric parameters. Front. Earth Sci. 2023, 10, 996389. [Google Scholar] [CrossRef]
- Hesheng, B.; Bielak, J.; Ghattas, O.; Kallivokas, L.F.; O’Hallaron, D.R.; Shewchuk, J.R.; Xu, J. Earthquake ground motion modeling on parallel computers. Supercomputing 1996, 96. [Google Scholar] [CrossRef]
- Chaljub, E.; Maufroy, E.; Moczo, P.; Kristek, J.; Hollender, F.; Bard, P.-Y.; Priolo, E.; Klin, P.; De Martin, F.; Zhang, Z.; et al. 3-D numerical simulations of earthquake ground motion in sedimentary basins: Testing accuracy through stringent models. Geophys. J. Int. 2015, 201, 90–111. [Google Scholar] [CrossRef]
- Wenk, T.; Fäh, D. Seismic Microzonation of the Basel Area. In Proceedings of the 15th World Conference on Earthquake Engineering, Lisboa, Portugal, 24 September 2012; p. 10. Available online: https://www.iitk.ac.in/nicee/wcee/article/WCEE2012_5455.pdf (accessed on 2 October 2023).
- Mreyen, A.S.; Donati, D.; Elmo, D.; Donze, F.V.; Havenith, H.B. Dynamic numerical modelling of co-seismic landslides using the 3D distinct element method: Insights from the Balta rockslide (Romania). Eng. Geol. 2022, 307, 106774. [Google Scholar] [CrossRef]
- Guillier, B.; Cornou, C.; Kristek, J.; Moczo, P.; Bonnefoy-Claudet, S.; Bard, P.-Y.; Fäh, D. Simulation of seismic ambient vibrations: Does the H/V provide quantitative information in 2D-3D structures. In Proceedings of the Third International Symposium on the Effects of Surface Geology on Seismic Motion, Grenoble, France, 30 August 2006; pp. 185–193. [Google Scholar]
- Graves, R.W.; Aagaard, B.T. Testing Long-Period Ground-Motion Simulations of Scenario Earthquakes Using the Mw 7.2 El Mayor–Cucapah Mainshock: Evaluation of Finite-Fault Rupture Characterization and 3D Seismic Velocity Models. Bull. Seismol. Soc. Am. 2011, 101, 895–907. [Google Scholar] [CrossRef]
- Shi, Z.; Day, S.M. Rupture dynamics and ground motion from 3-D rough-fault simulations. J. Geophys. Res. Solid. Earth 2013, 118, 1122–1141. [Google Scholar] [CrossRef]
- Hakimov, F.; Domej, G.; Ischuk, A.; Reicherter, K.; Cauchie, L.; Havenith, H.-B. Site Amplification Analysis of Dushanbe City Area, Tajikistan to Support Seismic Microzonation. Geosciences 2021, 11, 154. [Google Scholar] [CrossRef]
- Ischuk, A.R.; Lindholm, C.; Ilyasova, Z.; Murodkulov, S. Probabilistic Seismic Hazard Analysis of the Area of Tajikistan. Seismol. Probl. 2022, 4, 29–49. (In Russian) [Google Scholar]
- Abdrakhmatov, K. Establishment of the Central Asia Seismic Risk Initiative (CASRI). In ISTC Project No. KR 1176, 2009. Technical Report on the Work Performed from: 02.01.2006 to 04.30.2009; Institute of Seismology, National Academia of Sciences: Bishkek, Kyrgyzstan, 2009. [Google Scholar]
- Mikhailova, N.; Mukambayev, A.; Aristova, I.; Kulakova, G.; Ullah, S.; Pilz, M.; Bindi, B. Central Asia earthquake catalogue from ancient time to 2009. Ann. Geophys. 2015, 58, 102–111. [Google Scholar] [CrossRef]
- Negmatullaev, S.K.; Rodzhan, K.; Lunev, A.A.; Zolotarev, A.I. Service of Strong Movements of Tajikistan; Donish: Dushanbe, Tajikistan, 1987; p. 150. (In Russian) [Google Scholar]
- Negmatullaev, S.K.; Babaev, A.M.; Ruziev, D.R.; Ishchuk, N.R.; Djuraev, R.U. Analysis of the Seismic Vulnerability of Residential Buildings, and Development of an Earthquake Scenario for Dushanbe to Reduce Risk; World of Polygraphy: Dushanbe, Tajikistan, 2009; p. 30. (In Russian) [Google Scholar]
- Kogan, L.A.; Nechaev, V.A.; Romanov, O.A. Seismic Microzonation in Tajikistan; Donish: Dushanbe, Tajikistan, 1975; p. 379. (In Russian) [Google Scholar]
- Babaev, A.M.; Ishchuk, A.R.; Negmatullaev, S.K. Seismic Conditions of the Territory of Tajikistan; National University of Tajikistan: Dushanbe, Tajikistan, 2005; pp. 93–98. (In Russian) [Google Scholar]
- Nazarov, A.G.; Karapetyan, B.K.; Musayelyan, A.A. Preliminary Results of the Work of the Engineering-Seismological Squad TKSE in the Area of Dushanbe; Proc. Acad. Sci.: Dushanbe, Tajikistan, 1959; p. 30. (In Russian) [Google Scholar]
- Medvedev, S.; Sponheuer, W.; Karník, V. Neue seismische Skala Intensity Scale of Earthquakes, 7. In Tagung der Europäischen Seismologischen Kommission vom 24. 9. bis 30. 9. 1962 in Jena, DDR; Akademie: Berlin, Germany, 1964; pp. 69–76. [Google Scholar]
- Babaev, A.M.; Lyskov, L.M.; Mirzoev, K.M. Seismogenic Zones. Map Scale 1:500,000; Natural Resources of the Tajik SSR; State Geodetic and Cartographic Administration of the USSR: Moscow, Russia, 1984. (In Russian)
- Tschokher, V.O. Seismic Zoning of Urban Territory and Anti-Seismic Building Codes and Regulations; Academy of Sciences of the USSR: Moscow, Russia, 1938; p. 103. (In Russian) [Google Scholar]
- Medvedev, S.V. Seismic Microzonation of Cities; Geophysical Institute of the Academy of Sciences of the USSR: Moscow, Russia, 1952; pp. 78–89. (In Russian) [Google Scholar]
- Oripov, G.O. Map of Seismic Microzonation of the Territory of Dushanbe; Quarterly Report; HIETSCCT: Dushanbe, Tajikistan, 1975; p. 150. (In Russian) [Google Scholar]
- Kopylov, A.L. Map of Seismic Microzonation of the Territory of Dushanbe, Made Using the Method of Acoustic Stiffness; Quarterly Report; HIETSCCT: Dushanbe, Tajikistan, 1989; p. 170. (In Russian) [Google Scholar]
- Steimen, S. Uncertainties in Earthquake Scenarios (Diss. ETH No. 15740). Ph.D. Thesis, Swiss Federal Institute of Technology Zurich, Zurich, Switzerland, 2004; p. 170. [Google Scholar]
- Foti, S.; Aimar, M.; Ciancimino, A.; Passeri, F. Recent developments in seismic site response evaluation and microzonation. In Proceedings of the XVII European Conference on Soil Mechanics and Geotechnical Engineering, Reykjavík, Iceland, 1 September 2019. [Google Scholar] [CrossRef]
- Head Institute of Engineering and Technical Surveys of the State Construction Committee of Tajikistan (HIETSCCT). Collection of Borehole Data from the Dushanbe City Area; HIETSCCT: Dushanbe, Tajikistan, 2021; Personal Communication. [Google Scholar]
- Wathelet, M.; Chatelain, J.L.; Cornou, C.; Di Giulio, G.; Guillier, B.; Ohrnberger, M.; Savvaidis, A. Geopsy: A user friendly open-source tool set for ambient vibration processing. Seismol. Res. Lett. 2020, 91, 1878–1889. [Google Scholar] [CrossRef]
- Konno, K.; Ohmachi, T. Ground-motion characteristics estimated from spectral ratio between horizontal and vertical components of microtremor. Bull. Seismol. Soc. Am. 1998, 88, 228–241. [Google Scholar] [CrossRef]
- Albarello, D.; Lunedei, E. Combining horizontal ambient vibration components for H/V spectral ratio estimates. Geophys. J. Int. 2013, 194, 936–951. [Google Scholar] [CrossRef]
- Fäh, D.; Kind, F.; Giardini, D. A theoretical investigation of average H/V ratios. Geophys. J. Int. 2001, 145, 535–549. [Google Scholar] [CrossRef]
- Pilz, M.; Parolai, S.; Picozzi, M.; Wang, R.; Leyton, F.; Campos, J.; Zschau, J. Shear wave velocity model of the Santiago de Chile basin derived from ambient noise measurements: A comparison of proxies for seismic site conditions and amplification. Geophys. J. Int. 2010, 182, 355–367. [Google Scholar] [CrossRef]
- Abdialim, S.; Hakimov, F.; Kim, J.; Ku, T.; Moon, S.-W. Seismic site classification from HVSR data using the Rayleigh wave ellipticity inversion: A case study in Singapore. Earthq. Struct. 2021, 21, 231–238. [Google Scholar] [CrossRef]
- Bonnefoy-Claudet, S.; Baize, S.; Bonilla, L.F.; Berge-Thierry, C.; Pasten, C.R.; Campos, J.; Volant, P.; Verdugo, R. Site effect evaluation in the basin of Santiago de Chile using ambient noise measurements. Geophys. J. Int. 2009, 176, 925–937. [Google Scholar] [CrossRef]
- Building Seismic Safety Council (BSSC). NEHRP Recommended Provisions for Seismic Regulations for New Buildings and Other Structures, 2003 edition (FEMA 450); Building Seismic Safety Council, National Institute of Building Sciences: Washington DC, USA, 2004. Available online: https://www.nehrp.gov/pdf/fema450provisions.pdf (accessed on 2 October 2023).
- Cundall, P.A. A Computer Model for Simulating Progressive, Large-Scale Movements in Blocky Rock Systems. Rock. Mech. 1971, 8, 129–136. [Google Scholar]
- Bathe, K.-J.; Wilson, E.L. Numerical Methods in Finite Element Analysis; Prentice-Hall Inc.: Englewood Cliffs, NJ, USA, 1976. [Google Scholar] [CrossRef]
- Wolter, A.; Gischig, V.; Stead, D. Investigation of Geomorphic and Seismic Effects on the 1959 Madison Canyon, Montana, Landslide Using an Integrated Field, Engineering Geomorphology Mapping, and Numerical Modelling Approach. Rock Mech. Rock Eng. 2016, 49, 2479–2501. [Google Scholar] [CrossRef]
- Itasca Consulting Group, Inc. UDEC—Universal Distinct Element Code, Version 4.0 User’s Manual; Itasca: Minneapolis, MN, USA, 2006. [Google Scholar]
- Gholamy, A.; Krienovich, V. Why Ricker wavelets are successful in processing seismic data: Towards a theoretical explanation. In Proceedings of the Computational Intelligence for Engineering Solutions (CIES), 2014 IEEE Symposium, Orlando, FL, USA, 9–12 September 2014; pp. 11–16. Available online: https://scholarworks.utep.edu/cs_techrep/861 (accessed on 15 July 2023).
- Ricker, N. The form and laws of propagation of seismic wavelets. Geophysics 1953, 18, 10–40. [Google Scholar] [CrossRef]
- Kuhlemeyer, R.L.; Lysmer, J. Finite Element Method Accuracy for Wave Propagation Problems. J. Soil. Mech. Found. 1973, 99, 421–427. [Google Scholar] [CrossRef]
- Chávez-García, F.J.; Sánchez, L.R.; Hatzfeld, D. Topographic site effects and HVSR. A comparison between observations and theory. Bull. Seismol. Soc. Am. 1996, 86, 1559–1573. [Google Scholar] [CrossRef]
- Pagliaroli, A.; Pitilakis, K.; Chávez-García, F.J.; Raptakis, D.; Apostolidis, P.; Ktenidou, O.-J.; Manakou, M.; Lanzo, G. Experimental study of topographic effects using explosions and microtremor recordings. In Proceedings of the Fourth International Conference on Earthquake Geotechnical Engineering, Thessaloniki, Greece, 25 June 2007. [Google Scholar]
- Panzera, F.; Lombardo, G.; Rigano, R. Evidence of Topographic Effects through the Analysis of Ambient Noise Measurements. Seismol. Res. Let. 2011, 82, 413–419. [Google Scholar] [CrossRef]
- Mirzobayev, K.M.; Kinyapina, T.A.; Djuraev, R.U. Macro-Seismic Description of Earthquakes. In Coll. Earthquakes of Central Asia and Kazakhstan in 1980; Donish: Dushanbe, Tajikistan, 1982; pp. 46–65. (In Russian) [Google Scholar]
- Ishihara, K.; Okusa, S.; Oyagi, N.; Ischuk, A. Liquefaction induced flow slide in the collapsible loess deposit in Soviet Tajik. Soils Found. 1990, 30, 73–89. [Google Scholar] [CrossRef] [PubMed]
- Bouchon, M. Effect of topography on surface motion. Bull. Seismol. Soc. Am. 1973, 63, 615–632. [Google Scholar] [CrossRef]
- Bard, P.-Y. Diffracted waves and displacement field over two-dimensional elevated topographies. Geophys. J. Int. 1982, 71, 731–760. [Google Scholar] [CrossRef]
- Geli, L.; Bard, P.-Y.; Jullien, B. The effect of topography on earthquake ground motion: A review and new results. Bull. Seismol. Soc. Am. 1988, 78, 42–62. [Google Scholar] [CrossRef]
- Zhang, D.; Wang, G. Study of the 1920 Haiyuan earhquake-induced landslides in loess (China). Eng. Geol. 2007, 94, 76–88. [Google Scholar] [CrossRef]
- Napolitano, F.; Gervasi, A.; La Rocca, M.; Guerra, I.; Scarpa, R. Site Effects in the Pollino Region from the HVSR and polarization of seismic noise and earthquakes. Bull. Seismol. Soc. Am. 2018, 108, 309–321. [Google Scholar] [CrossRef]

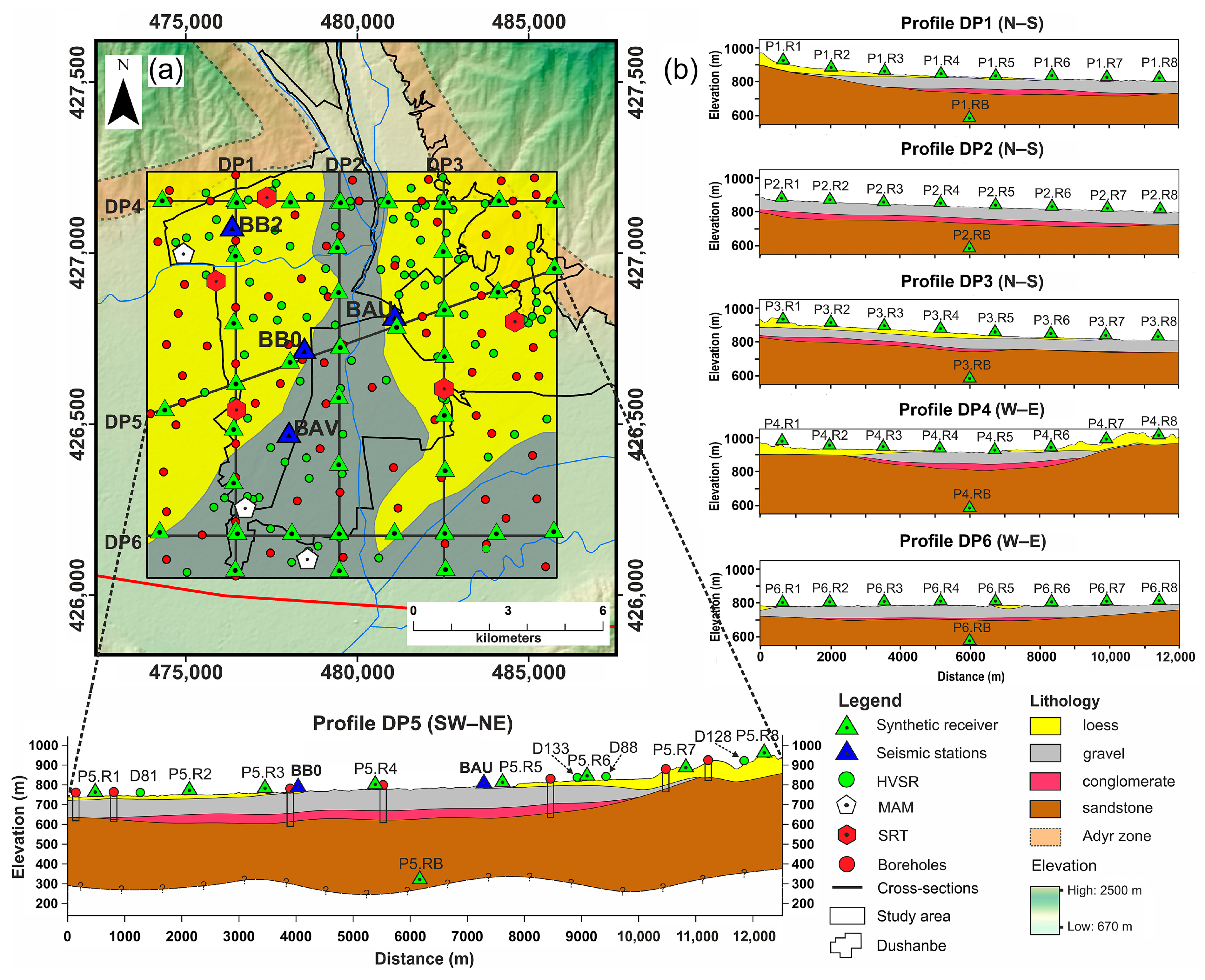
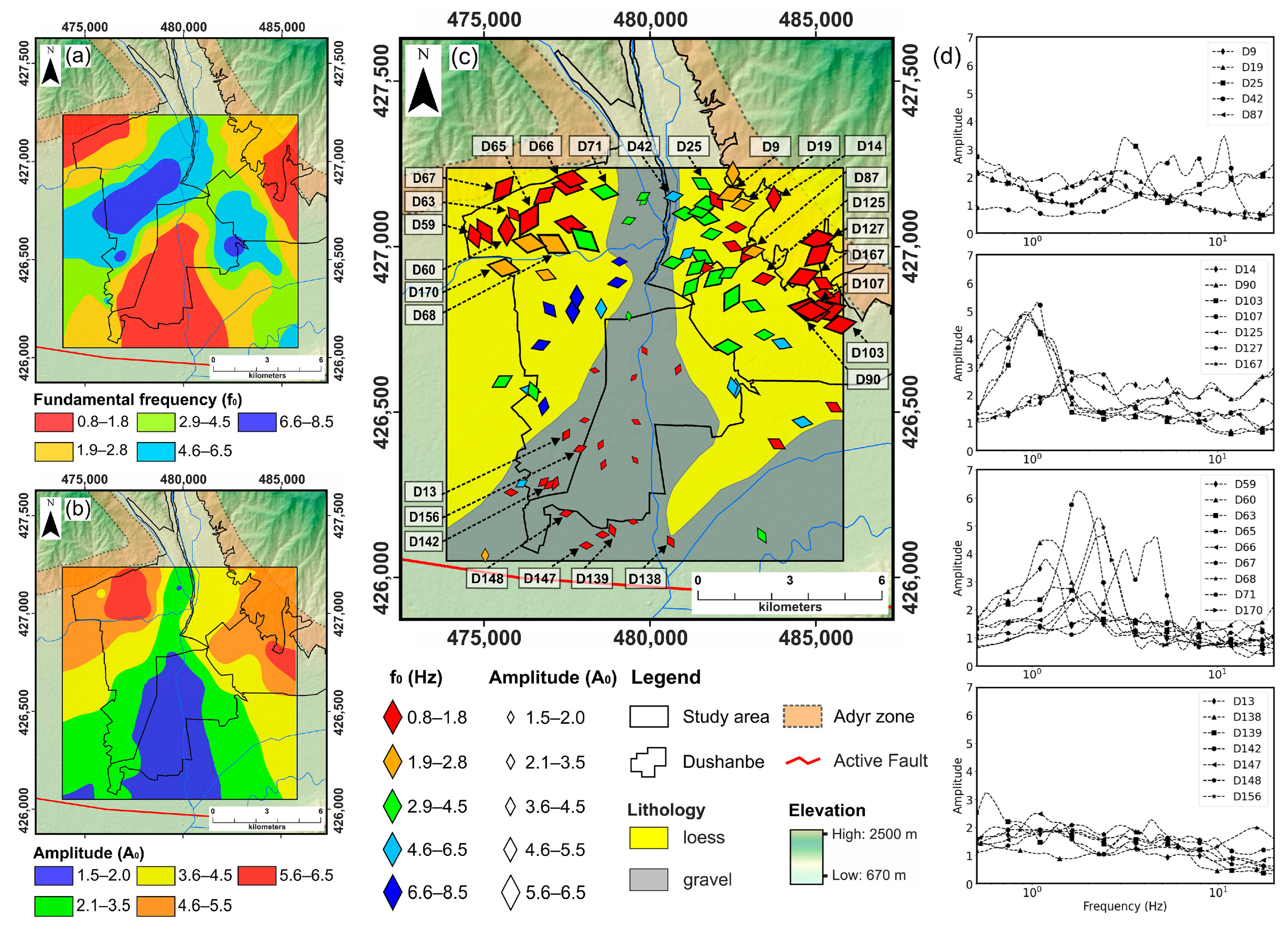
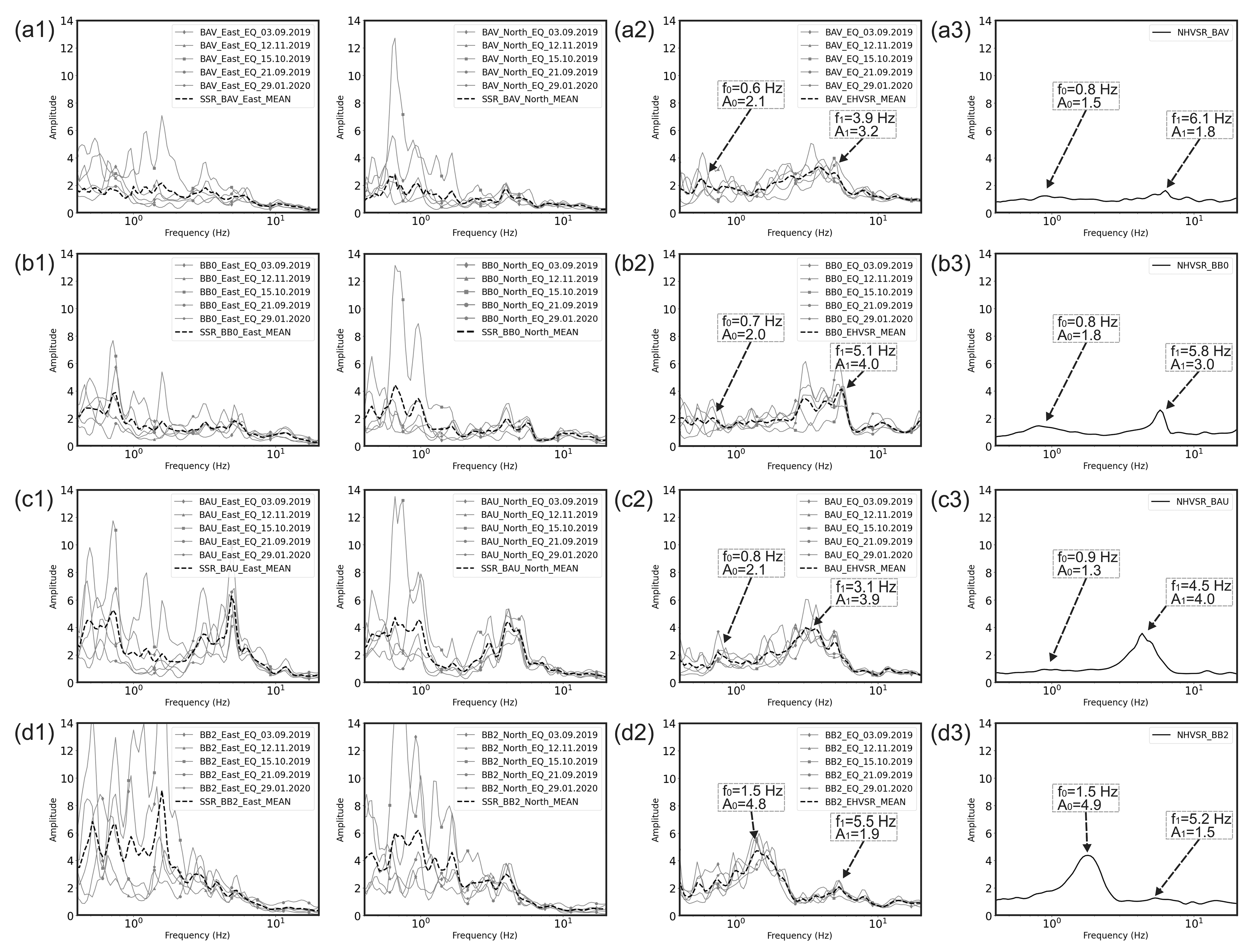
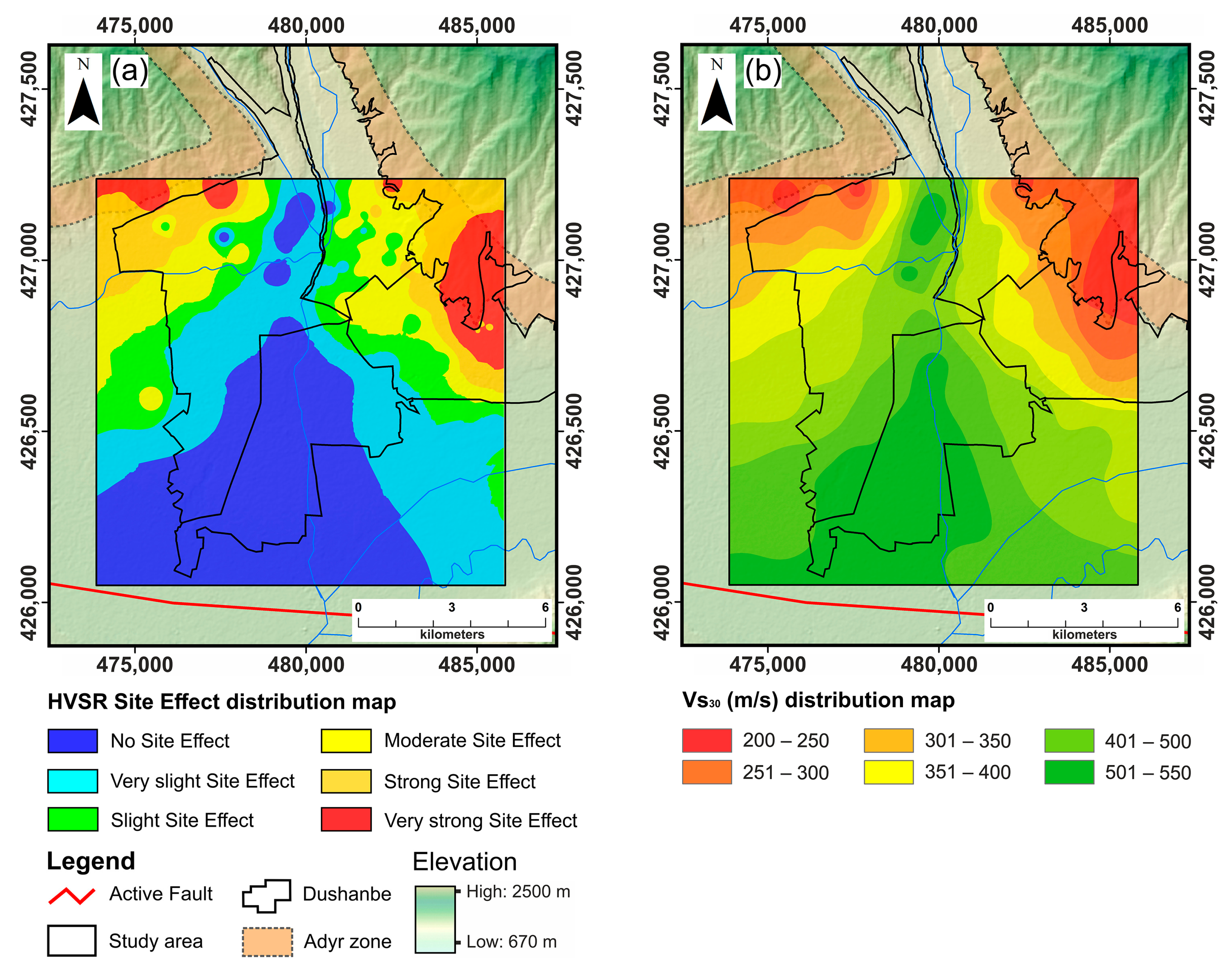
| Earthquakes | Datum | Latitude | Longitude | Magnitude (Mw) | Depth (km) | Distance to Dushanbe (km) | Intensity (MSK–64 Scale) |
|---|---|---|---|---|---|---|---|
| Karatag | 21.10.1907 | 38.70 | 68.10 | 7.4 | 25 | 60 | VII |
| Chuyanchi | 27.10.1907 | 38.80 | 68.40 | 6.2 | 24 | 38 | VI |
| Fayzabad | 11.01.1943 | 38.53 | 69.31 | 6.2 | 12 | 40 | VI |
| Khait | 10.07.1949 | 39.17 | 70.87 | 7.4 | 18 | 190 | VI |
| Stalinabad | 27.02.1952 | 38.60 | 68.90 | 5.0 | 8 | 0 | VI–VII |
| Hissar-Babatag | 04.08.1953 | 38.50 | 68.50 | 4.0 | 8 | 14 | IV–V |
| Andzhir | 07.07.1953 | 38.40 | 68.90 | 4.3 | 5 | 21 | IV–V |
| Yavroz | 16.09.1960 | 38.67 | 69.17 | 4.9 | 14 | 40 | V–VI |
| Chimtepa | 02.01.1966 | 38.47 | 68.70 | 3.9 | 10 | 11 | IV |
| Hissar | 21.04.1968 | 38.47 | 68.65 | 4.9 | 8 | 15 | V–VI |
| Lyaur | 24.04.1970 | 38.37 | 68.71 | 4.6 | 8 | 22 | IV–V |
| Sultanabad | 17.06.1976 | 38.47 | 68.97 | 3.7 | 3 | 21 | III |
| Sultanabad | 10.07.1979 | 38.45 | 68.94 | 4.0 | 2 | 20 | III |
| Dushanbe | 16.12.1980 | 38.48 | 68.75 | 5.0 | 5 | 8 | V |
| Hissar | 22.01.1989 | 38.49 | 68.67 | 5.8 | 7 | 13 | V–VI |
| Hissar-Babatag | 27.03.1999 | 38.47 | 68.50 | 4.3 | 5 | 25 | IV–V |
| Dushanbe | 18.08.2006 | 38.52 | 68.88 | 4.3 | 5 | 10 | V–VI |
| Method | Assessed Data | Processing | Results |
|---|---|---|---|
| 175—HVSR measurements | passive seismic (ambient noise records at 30 min intervals) | HVSR method | fundamental resonance frequency map |
| 9—SRT measurements | active seismic (P-wave) | P-wave inversion | P-wave velocity (Vp) patterns, subsurface structures |
| 5—MAM measurements | passive seismic (ambient noise records at two-hour intervals) | spatial autocorrelation (SPAC), Rayleigh wave | dispersion curves, S-wave velocity (Vs) patterns |
| 5—temporary seismic station recordings and 1 permanent reference station | passive seismic (instrumental data) | SSR from earthquake | amplification factors |
| data compilation | lithological maps and cross-sections data from 80 boreholes | data evaluation and adaption | surface lithology, subsurface structures |
| Station | Position | Terrace | Uppermost Sediment Types |
|---|---|---|---|
| BB2 | 1 km N of the Hissar canal (left bank) | 3rd | loess (<45 m) |
| BB0 | 1 km W of the Varzob river (right bank) | 2nd | loess (<10 m) |
| BAU | 800 m E of the Varzob river (left bank) | 1st | loess (<20 m) |
| BAV (reference site) | 2 km W of the Varzob river (right bank) | 3rd | gravel |
| Material | Lithology | Vp (m/s) | Vs (m/s) | ν | ρ (kg/m3) | E (GPa) | K (MPa) | G (MPa) |
|---|---|---|---|---|---|---|---|---|
| Mat1 | loess | 600 | 300 | 0.33 | 1900 | 0.46 | 451 | 173 |
| Mat2 | gravel | 900 | 450 | 0.33 | 2000 | 1.09 | 1068 | 410 |
| Mat3 | conglomerate | 2200 | 1200 | 0.28 | 2200 | 8.32 | 6303 | 3250 |
| Mat4 | sandstone | 2800 | 1500 | 0.3 | 2400 | 13.9 | 11583 | 5346 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hakimov, F.; Havenith, H.-B.; Ischuk, A.; Reicherter, K. Assessment of Site Effects and Numerical Modeling of Seismic Ground Motion to Support Seismic Microzonation of Dushanbe City, Tajikistan. Geosciences 2024, 14, 117. https://doi.org/10.3390/geosciences14050117
Hakimov F, Havenith H-B, Ischuk A, Reicherter K. Assessment of Site Effects and Numerical Modeling of Seismic Ground Motion to Support Seismic Microzonation of Dushanbe City, Tajikistan. Geosciences. 2024; 14(5):117. https://doi.org/10.3390/geosciences14050117
Chicago/Turabian StyleHakimov, Farkhod, Hans-Balder Havenith, Anatoly Ischuk, and Klaus Reicherter. 2024. "Assessment of Site Effects and Numerical Modeling of Seismic Ground Motion to Support Seismic Microzonation of Dushanbe City, Tajikistan" Geosciences 14, no. 5: 117. https://doi.org/10.3390/geosciences14050117
APA StyleHakimov, F., Havenith, H.-B., Ischuk, A., & Reicherter, K. (2024). Assessment of Site Effects and Numerical Modeling of Seismic Ground Motion to Support Seismic Microzonation of Dushanbe City, Tajikistan. Geosciences, 14(5), 117. https://doi.org/10.3390/geosciences14050117












