Laboratory Hydraulic Tensile Strength Correlation with Strength-Based Brittleness Indices for Carbonate Reservoirs
Abstract
1. Introduction
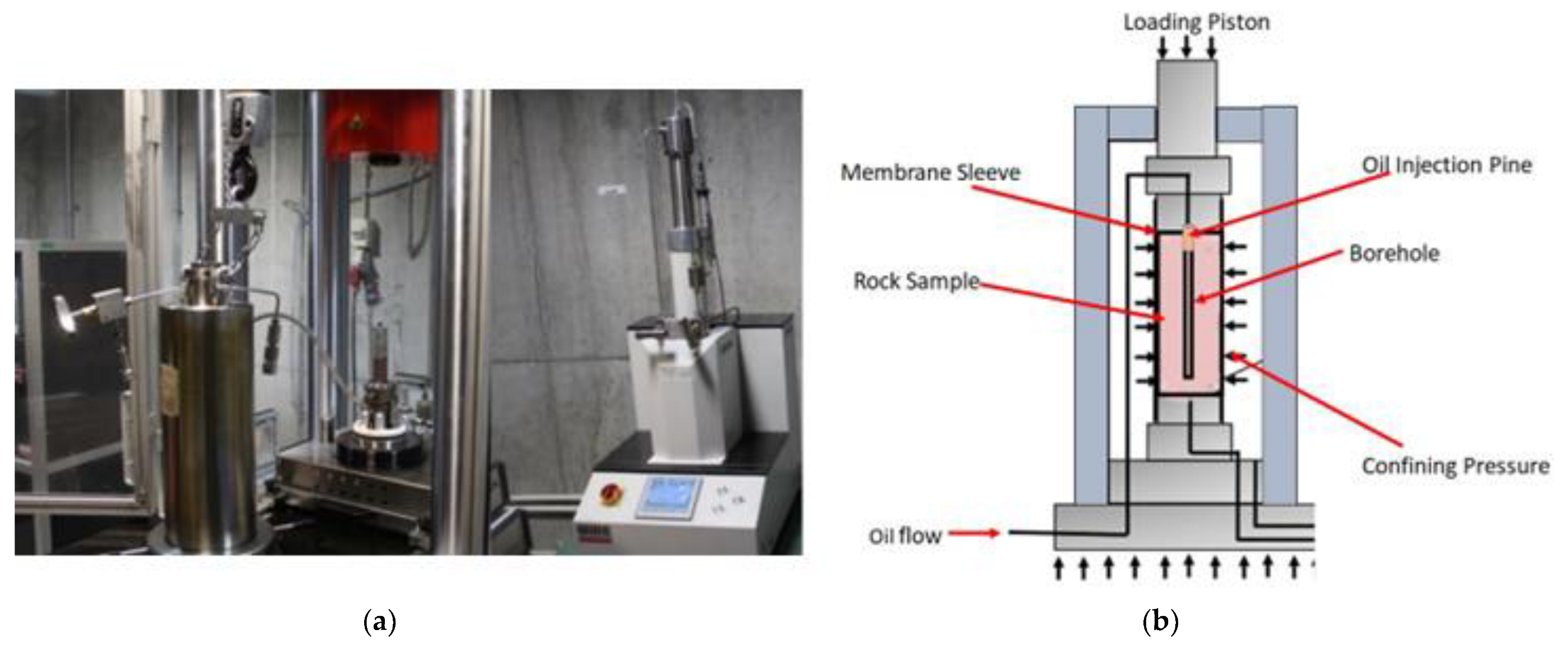
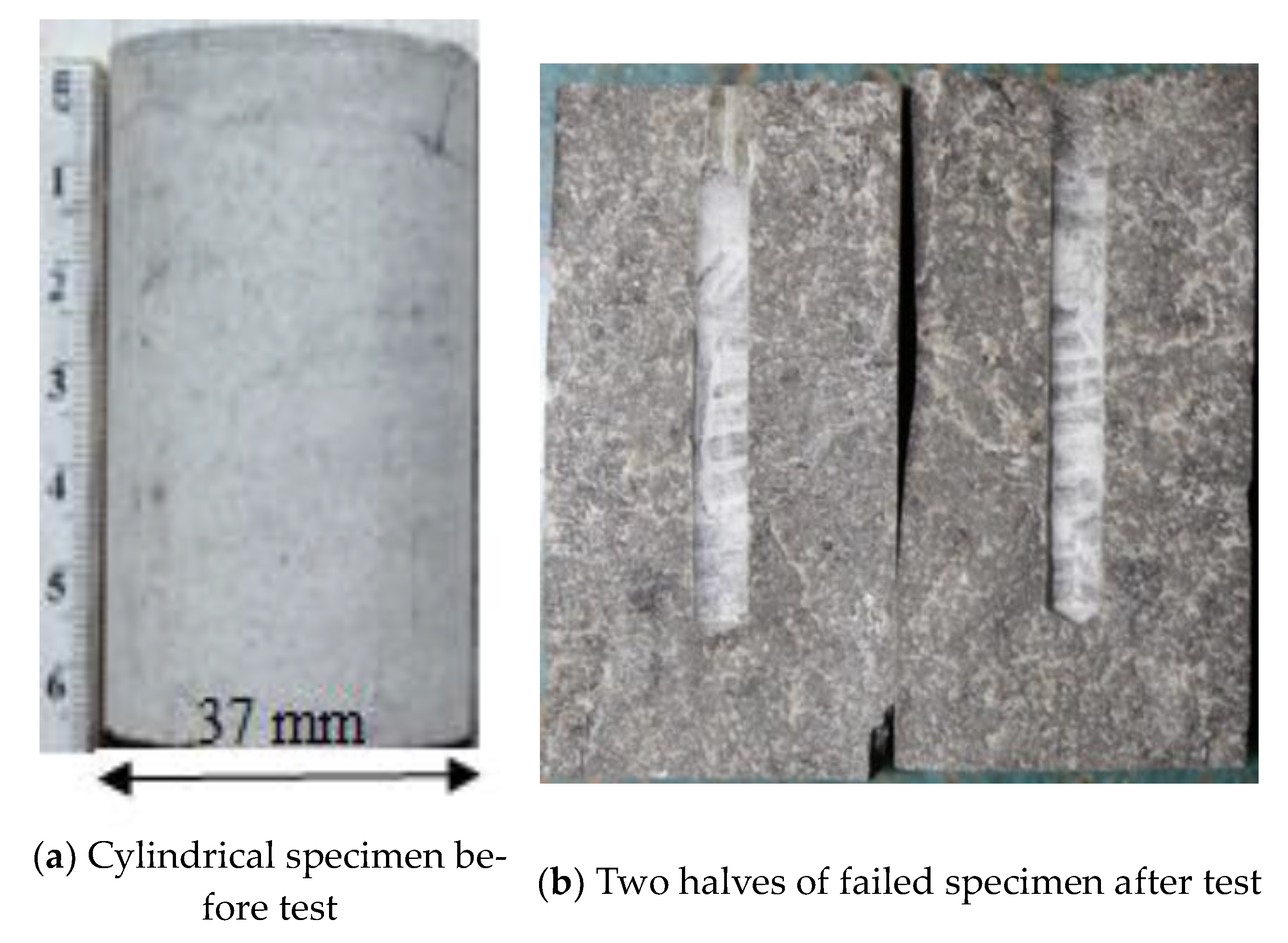
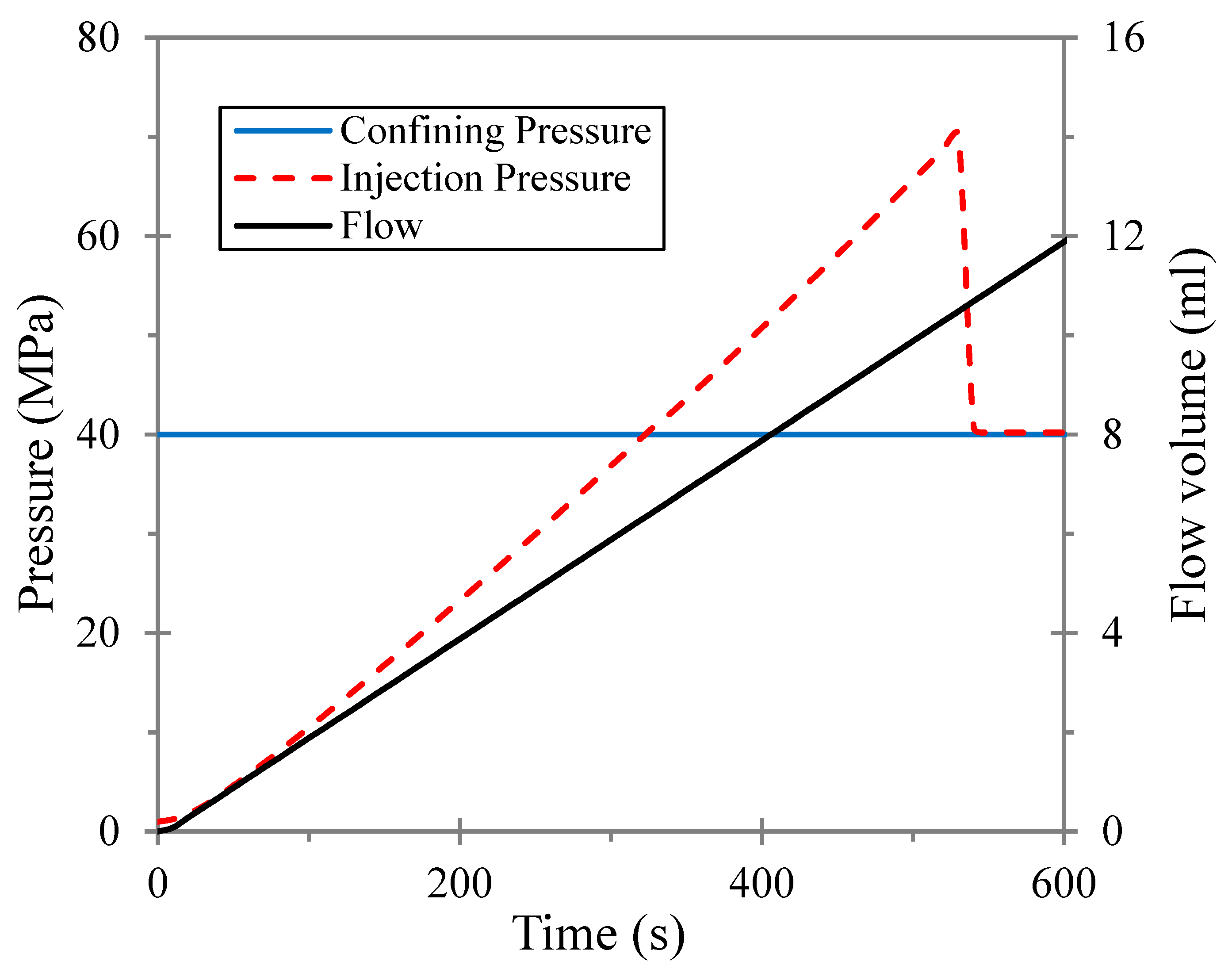
2. Materials and Methods
3. Experimental Results
4. Analysis
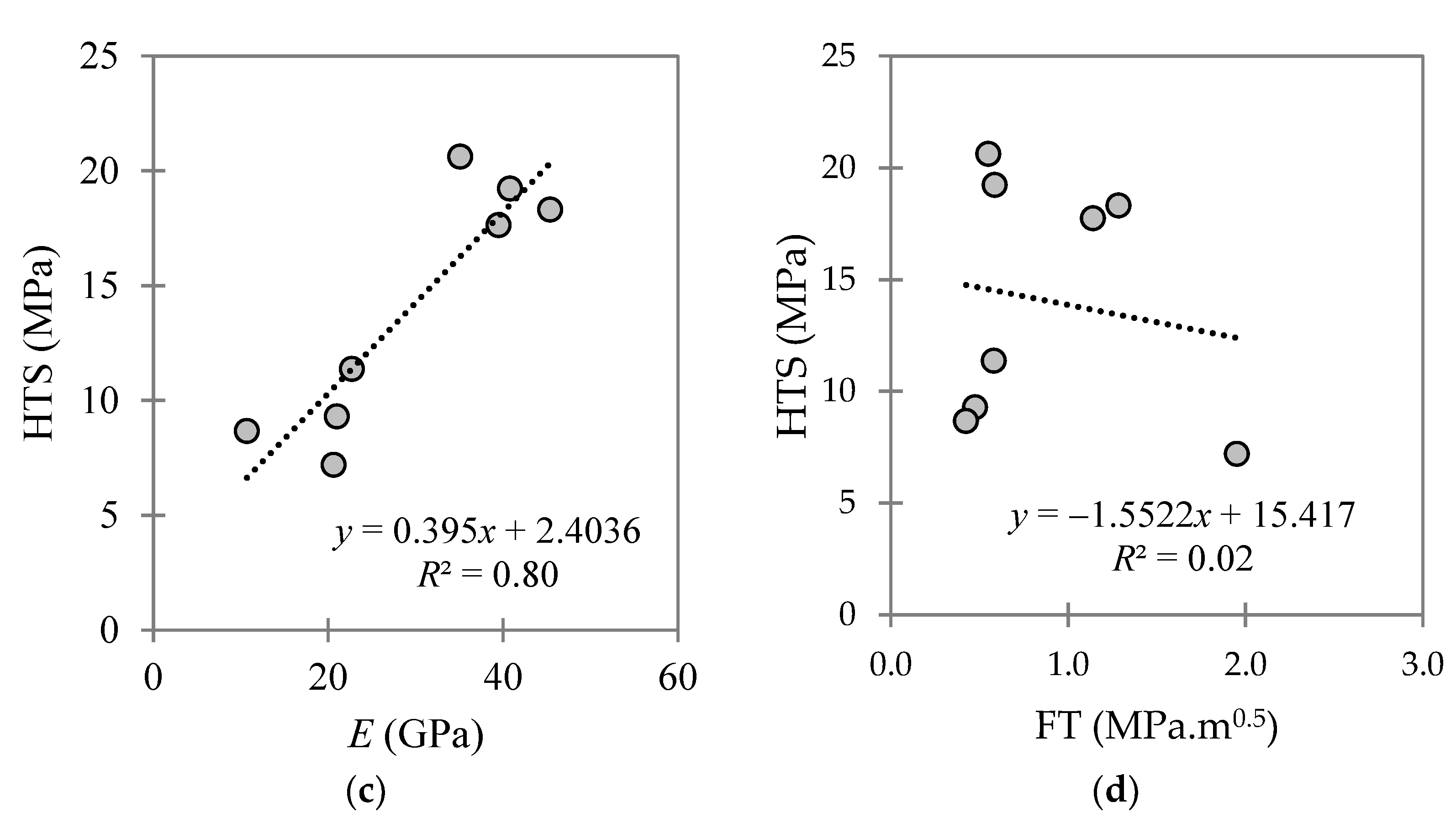
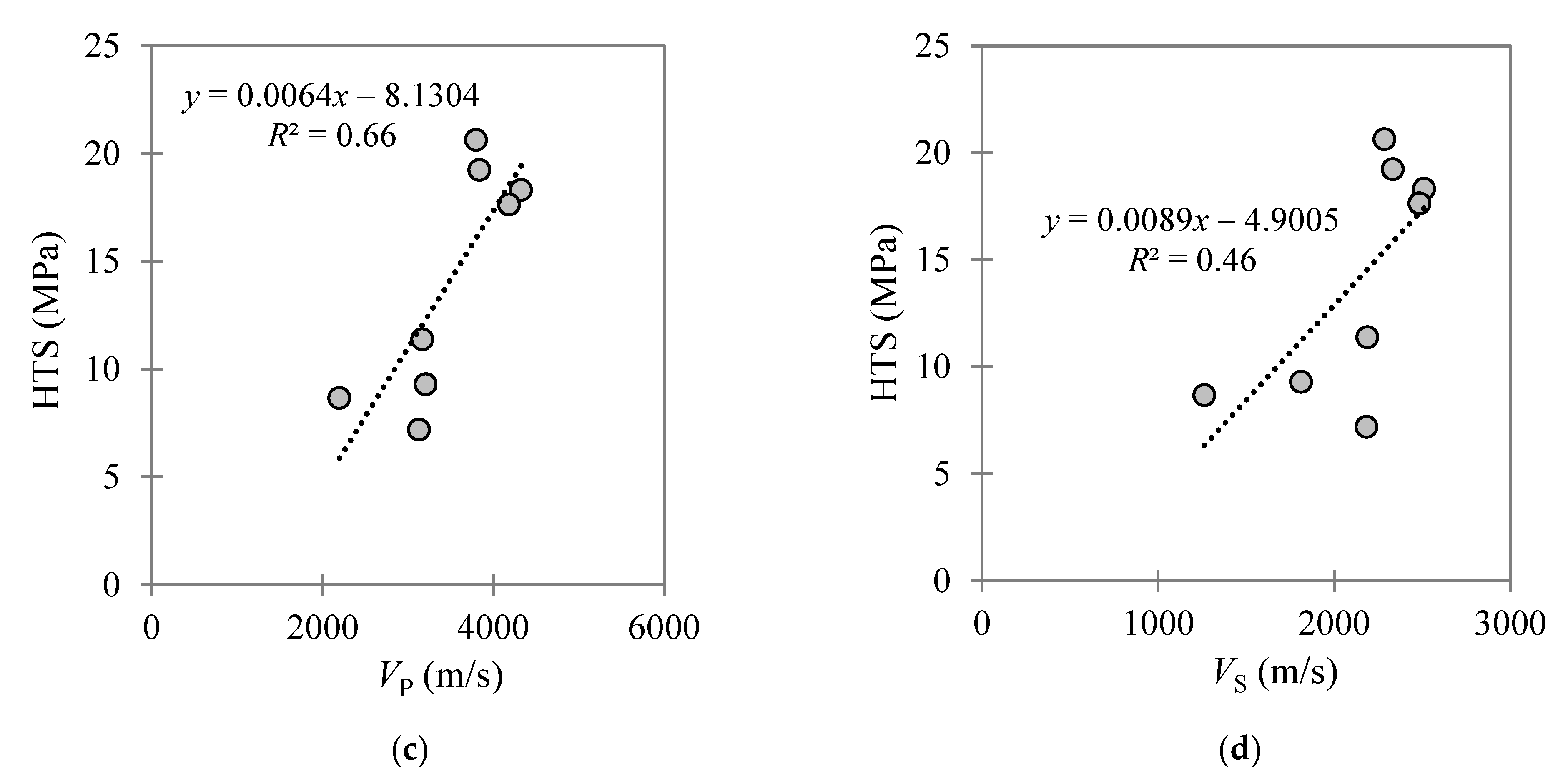
4.1. HTS Correlation with Other Rock Parameters
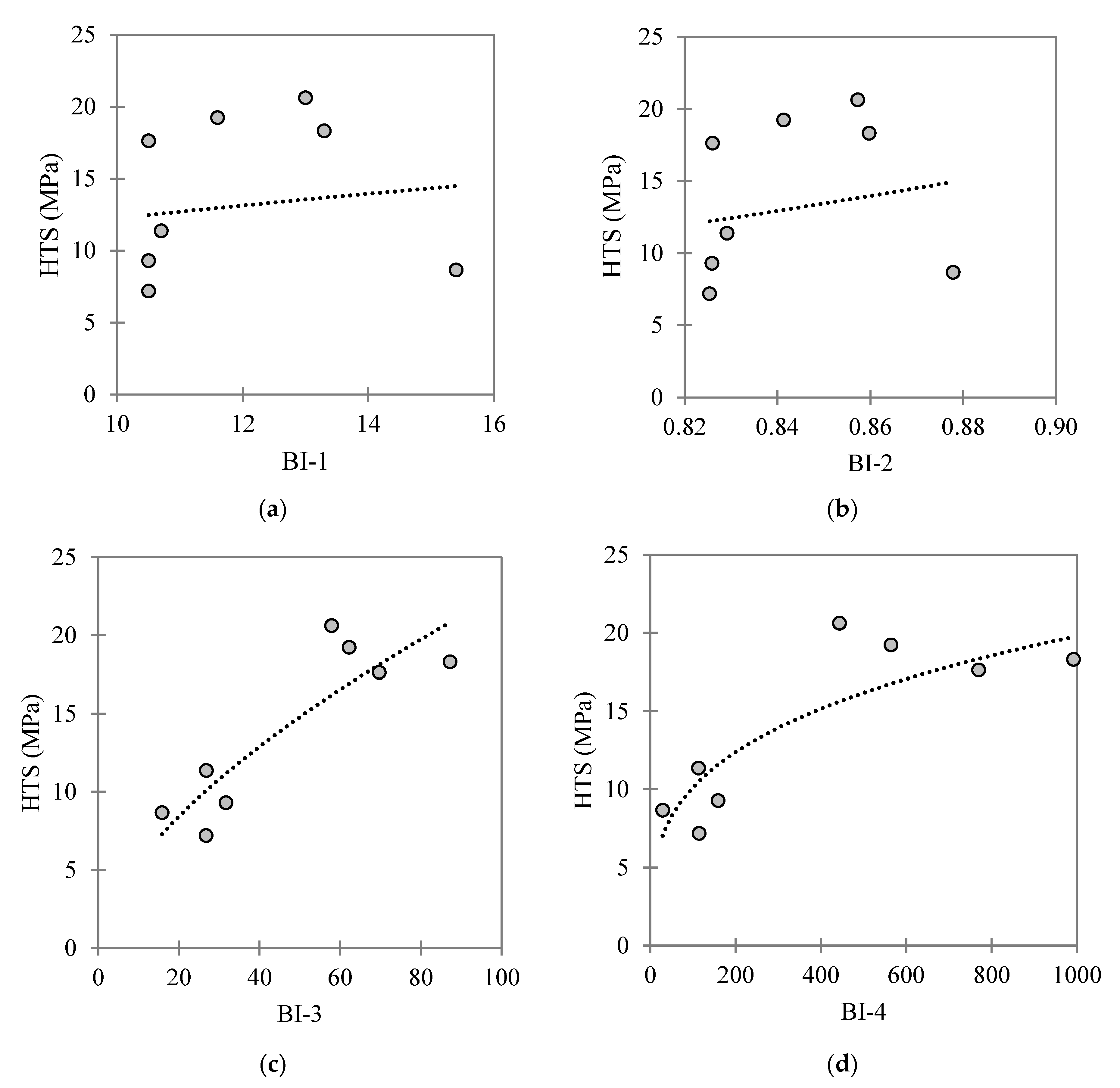
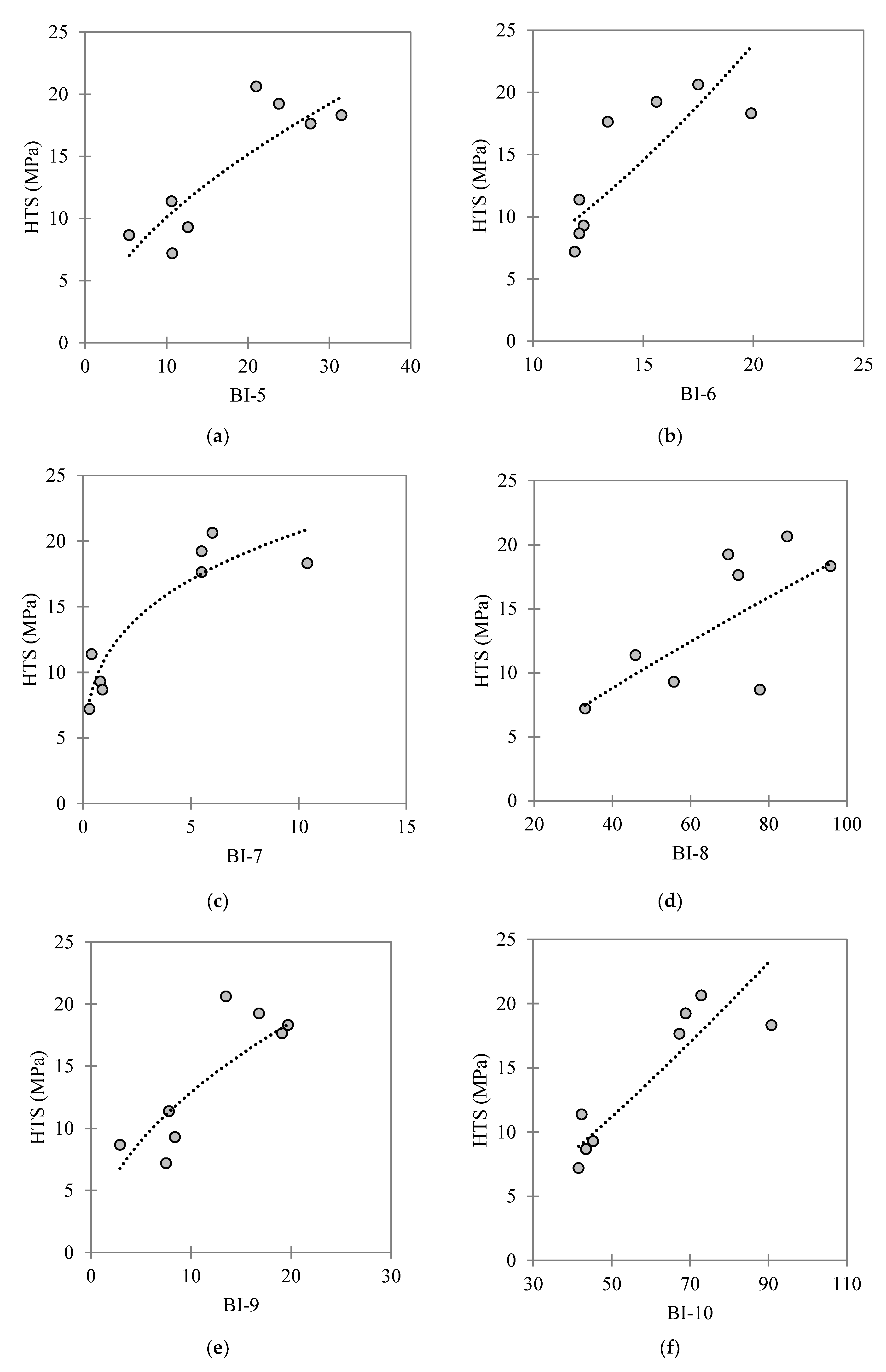
4.2. HTS Correlation with BIs
5. Discussion
6. Summary and Conclusions
- UCS, BTS, E, ρ, VP, and VS directly correlated with the HTS, whereas the rock porosity was found to inversely correlate with the HTS. The correlations between the HTS and the UCS, BTS, and E produced the best agreements.
- Seven of the ten investigated BIs correlated with the HTS over 69% (R2 ≥ 0.69). Whereas the indices BI-1 and BI-2 were found to produce weak or no correlations with the HTS, since they are considered as not satisfactory indicators of rock brittleness.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| BI | brittleness index |
| BI-1 to BI-10 | brittleness index correlations (numbered 1 to 10) |
| BTS | Brazilian tensile strength |
| HF | hydraulic fracturing |
| HTS | hydraulic tensile strength |
| FT | fracture toughness |
| GU | geomechanical unit |
| MAPE | mean absolute percentage error |
| RMSE | root-mean-square error |
| UCS | uniaxial compressive strength |
Notations
| a, b, and c | coefficients of power equation |
| C | apparent cohesion (cohesion intercept) |
| E | Young’s modulus |
| R2 | coefficient of determination |
| VP | compressional wave velocity |
| VS | shear wave velocity |
| γ | bulk unit weight |
| ν | Poisson’s ratio |
| φ | internal friction angle |
| ρ | bulk density |
| σc | uniaxial compressive strength |
| σt | Brazilian tensile strength |
References
- Economides, M.J.; Nolte, K.G. (Eds.) Reservoir Stimulation; Wiley: Chichester, UK, 2000. [Google Scholar]
- Lu, Y.; Zeng, L.; Xie, Q.; Jin, Y.; Hossain, M.M.; Saeedi, A. Analytical modelling of wettability alteration-induced micro-fractures during hydraulic fracturing in tight oil reservoirs. Fuel 2019, 249, 434–440. [Google Scholar] [CrossRef]
- Lebedev, M.; Zhang, Y.; Sarmadivaleh, M.; Barifcani, A.; Al-Khdheeawi, E.; Iglauer, S. Carbon geosequestration in limestone: Pore-scale dissolution and geomechanical weakening. Int. J. Greenh. Gas Control 2017, 66, 106–119. [Google Scholar] [CrossRef]
- Odling, N.W.A.; Elphick, S.C.; Meredith, P.; Main, I.; Ngwenya, B.T. Laboratory measurement of hydrodynamic saline dispersion within a micro-fracture network induced in granite. Earth Planet. Sci. Lett. 2007, 260, 407–418. [Google Scholar] [CrossRef]
- Zhuang, L.; Kim, K.Y.; Jung, S.G.; Diaz, M.; Min, K.B. Effect of water infiltration, injection rate and anisotropy on hydraulic fracturing behavior of granite. Rock Mech. Rock Eng. 2019, 52, 575–589. [Google Scholar] [CrossRef]
- Rickman, R.; Mullen, M.; Petre, E.; Grieser, B.; Kundert, D. A practical use of shale petrophysics for stimulation design optimization: All shale plays are not clones of the Barnett shale. In Proceedings of the 2008 Society of Petroleum Engineers (SPE) Annual Technical Conference and Exhibition, Denver, CO, USA, 21–24 September 2008; SPE: Richardson, TX, USA, 2008. [Google Scholar] [CrossRef]
- Jin, Z.H.; Johnson, S.E. Effects of elastic anisotropy on primary petroleum migration through buoyancy-driven crack propagation. Geomech Geophys. Geo-Energy Geo-Resour. 2017, 3, 365–378. [Google Scholar] [CrossRef]
- Geng, Z.; Chen, M.; Jin, Y.; Yang, S.; Yi, Z.; Fang, X.; Du, X. Experimental study of brittleness anisotropy of shale in triaxial compression. J. Nat. Gas Sci. Eng. 2016, 36 Pt A, 510–518. [Google Scholar] [CrossRef]
- Gholami, R.; Rasouli, V.; Sarmadivaleh, M.; Minaeian, V.; Fakhari, N. Brittleness of gas shale reservoirs: A case study from the north Perth basin, Australia. J. Nat. Gas Sci. Eng. 2016, 33, 1244–1259. [Google Scholar] [CrossRef]
- Rastegarnia, A.; Sharifi Teshnizi, E.; Hosseini, S.; Shamsi, H.; Etemadifar, M. Estimation of punch strength index and static properties of sedimentary rocks using neural networks in south west of Iran. Measurement 2018, 128, 464–478. [Google Scholar] [CrossRef]
- Jin, X.; Shah, S.N.; Roegiers, J.C.; Zhang, B. An integrated petrophysics and geomechanics approach for fracability evaluation in shale reservoirs. SPE J. 2015, 20, 518–526. [Google Scholar] [CrossRef]
- Guo, J.; Feng, G.R.; Qi, T.Y.; Wang, P.; Yang, J.; Li, Z.; Bai, J.; Du, X.; Wang, Z. Dynamic mechanical behavior of dry and water saturated igneous rock with acoustic emission monitoring. Shock. Vib. Deep. Min. Sci. 2018, 281, 2348394. [Google Scholar] [CrossRef]
- Rastegarnia, A.; Lashkaripour, G.R.; Sharifi Teshnizi, E.; Ghafoori, M. Evaluation of engineering characteristics and estimation of static properties of clay-bearing rocks. Environ. Earth Sci. 2021, 80, 621. [Google Scholar] [CrossRef]
- Noori, M.; Khanlari, G.; Rafiei, B.; Sarfarazi, V.; Zaheri, M. Correction to: Estimation of brittleness indexes from petrographic characteristics of different sandstone types (Cenozoic and Mesozoic Sandstones), Markazi Province, Iran. Rock Mech. Rock Eng. 2022, 55, 6519. [Google Scholar] [CrossRef]
- Chong, Z.; Yao, Q.; Li, X.; Zhu, L.; Tang, C. Effect of rock brittleness on propagation of hydraulic fractures in shale reservoirs with bedding-planes. Energy Sci. Eng. 2020, 8, 2352–2370. [Google Scholar] [CrossRef]
- Oyarhossein, M.; Dusseault, M.B. Sensitivity analysis of factors affecting fracture height and aperture. Upstream Oil Gas Technol. 2022, 9, 100079. [Google Scholar] [CrossRef]
- Perez Altamar, R.; Marfurt, K.J. Identification of brittle/ductile areas in unconventional reservoirs using seismic and microseismic data: Application to the Barnett Shale. Interpretation 2015, 3, T233–T243. [Google Scholar] [CrossRef]
- Zhou, C.; Sun, Z.; Xiao, Y.; Huang, G.; Kuang, D.; Li, M. Study on brittleness characteristics and fracturing crack propaga-tion law of deep thin-layer tight sandstone in Longdong, Changqing. Processes 2023, 11, 2636. [Google Scholar] [CrossRef]
- Zhang, B.; Ji, B.; Liu, W. The study on mechanics of hydraulic fracture propagation direction in shale and numerical simulation. Geomech Geophys. Geo-Energy Geo-Resour. 2018, 4, 119–127. [Google Scholar] [CrossRef]
- Karrari, S.S.; Heidari, M.; Hamidi, J.K.; Tashnizi, E.S. Evaluation brittleness indices of rocks, implication for estimating their toughness modulus. Iran. J. Eng. Geol. 2020, 13, 125–129. [Google Scholar]
- Karrari, S.S.; Haidari, M.; Hamidi, J.K.; Tashnizi, E.S. The assessment of rock brittleness effect on drillability. Adv. Appl. Geol. 2022, 11, 671–689. [Google Scholar] [CrossRef]
- de Pater, C.J.; Cleary, M.P.; Quinn, T.S.; Barr, D.T.; Johnson, D.E.; Weijers, L. Experimental verification of dimensional analysis for hydraulic fracturing. SPE Prod. Facil. 1994, 9, 230–238. [Google Scholar] [CrossRef]
- Kaka, S.I.; Reyes-Montes, J.M.; Al-Shuhail, A.; Al-Shuhail, A.A.; Jervis, M. Analysis of microseismic events during a multistage hydraulic stimulation experiment at a shale gas reservoir. Pet. Geosci. 2017, 23, 386–394. [Google Scholar] [CrossRef]
- Zhao, H.; Wang, X.; Liu, Z. Experimental investigation of hydraulic sand fracturing on fracture propagation under the influence of coal macrolithotypes in Hancheng block, China. J. Pet. Sci. Eng. 2019, 175, 60–71. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, Y.; Wang, W.; Wan, W.; Tang, J. Testing study on crack propagation due to rheological fracture in quasi-brittle material under compression-shear or double torsional loading. Geotech. Geol. Eng. 2016, 34, 1655–1667. [Google Scholar] [CrossRef]
- Lin, H.; Yang, H.; Wang, Y.; Zhao, Y.; Cao, R. Determination of the stress field and crack initiation angle of an open flaw tip under uniaxial compression. Theor. Appl. Fract. Mech. 2019, 104, 102358. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, H.; Lin, H.; Zhao, Y.; Liu, Y. Fracture behaviour of central-flawed rock plate under uniaxial compression. Theor. Appl. Fract. Mech. 2020, 106, 102503. [Google Scholar] [CrossRef]
- Song, I.; Suh, M.; Won, K.S.; Haimson, B. A laboratory study of hydraulic fracturing breakdown pressure in tablerock sandstone. Geosci. J. 2001, 5, 263–271. [Google Scholar] [CrossRef]
- Zoback, M.D.; Rummel, F.; Jung, R.; Raleigh, C.B. Laboratory hydraulic fracturing experiments in intact and pre-fractured rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1977, 14, 49–58. [Google Scholar] [CrossRef]
- Haimson, B.C.; Zhao, Z. Effect of borehole size and pressurization rate on hydraulic fracturing breakdown pressure. In Proceedings of the 32nd U.S. Symp. Rock Mechanics (USRMS), Norman, OK, USA, 10–12 July 1991. [Google Scholar]
- Molenda, M.; Stöckert, F.; Brenne, S.; Alber, M. Comparison of hydraulic and conventional tensile strength tests. In Proceedings of the Int. Conf. for Effective and Sustainable Hydraulic Fracturing, Brisbane, Australia, 20–22 May 2013; pp. 981–992. [Google Scholar] [CrossRef][Green Version]
- Zhou, J.; Jin, Y.; Chen, M. Experimental investigation of hydraulic fracturing in random naturally fractured blocks. Int. J. Rock Mech. Min. Sci. 2010, 47, 1193–1199. [Google Scholar] [CrossRef]
- Athavale, A.S.; Miskimins, J.L. Laboratory hydraulic fracturing tests on small homogeneous and laminated blocks. In Proceedings of the 42nd U.S. Rock Mechanics Symposium (USRMS), San Francisco, CA, USA, 29 June–2 July 2008. [Google Scholar]
- Ispas, I.; Eve, R.A.; Hickman, R.J.; Keck, R.G.; Willson, S.M.; Olson, K.E. Laboratory testing and numerical modelling of fracture propagation from deviated wells in poorly consolidated formations. In Proceedings of the 2012 Society of Petroleum Engineers (SPE) Annual Technical Conference and Exhibition, San Antonio, TX, USA, 8–10 October 2012. [Google Scholar] [CrossRef]
- Schmitt, D.R.; Zoback, M.D. Infiltration effects in the tensile rupture of thin walled cylinders of glass and granite: Implications for the hydraulic fracturing breakdown equation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 289–303. [Google Scholar] [CrossRef]
- Bieniawski, Z.T.; Hawkes, I. Suggested methods for determining tensile strength of rock materials. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1978, 15, 99–103. [Google Scholar] [CrossRef]
- Plinninger, R.J.; Thomée, B.; Wolski, K. The modified tension test (MTT)—Evaluation and testing experiences with a new and simple direct tension test. In Proceedings of the EUROCK 2004 & 53rd Geomechanics Colloquium, Salzburg, Austria, 7–9 October 2004; pp. 545–548. [Google Scholar]
- Erarslan, N.; Williams, D.J. Experimental, numerical and analytical studies on tensile strength of rocks. Int. J. Rock Mech. Min. Sci. 2012, 49, 21–30. [Google Scholar] [CrossRef]
- Aliabadian, Z.; Zhao, G.F.; Russell, A.R. Failure, crack initiation and the tensile strength of transversely isotropic rock using the Brazilian test. Int. J. Rock Mech. Min. Sci. 2019, 122, 104073. [Google Scholar] [CrossRef]
- Meng, F.; Wong, L.N.Y.; Zhou, H. Rock brittleness indices and their applications to different fields of rock engineering: A review. J. Rock Mech. Geotech. Eng. 2021, 13, 221–247. [Google Scholar] [CrossRef]
- Hucka, V.; Das, B. Brittleness determination of rocks by different methods. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1974, 11, 389–392. [Google Scholar] [CrossRef]
- Özfırat, M.K.; Yenice, H.; Şimşir, F.; Yaralı, O. A new approach to rock brittleness and its usability at prediction of drillability. J. Afr. Earth Sci. 2016, 119, 94–101. [Google Scholar] [CrossRef]
- Altindag, R. The evaluation of rock brittleness concept on rotary blast hole drills. J. South. Afr. Inst. Min. Metall. 2002, 102, 61–66. [Google Scholar]
- Altindag, R. Assessment of some brittleness indexes in rock-drilling efficiency. Rock Mech. Rock Eng. 2010, 43, 361–370. [Google Scholar] [CrossRef]
- Yagiz, S.; Gokceoglu, C. Application of fuzzy inference system and nonlinear regression models for predicting rock brittleness. Expert Syst. Appl. 2010, 37, 2265–2272. [Google Scholar] [CrossRef]
- Khandelwal, M.; Shirani Faradonbeh, R.; Monjezi, M.; Armaghani, D.J.; Majid, M.Z.B.A.; Yagiz, S. Function development for appraising brittleness of intact rocks using genetic programming and non-linear multiple regression models. Eng. Comput. 2017, 33, 13–21. [Google Scholar] [CrossRef]
- Nejati, H.R.; Moosavi, S.A. A new brittleness index for estimation of rock fracture toughness. J. Min. Environ. 2017, 8, 83–91. [Google Scholar] [CrossRef]
- Ghadernejad, S.; Nejati, H.R.; Yagiz, S. A new rock brittleness index on the basis of punch penetration test data. Geomech. Eng. 2020, 21, 391–399. [Google Scholar] [CrossRef]
- Kashfi, M.S. Geology of the Permian “Super-Giant” gas reservoirs in the Greater Persian Gulf area. J. Pet. Geol. 1992, 15, 465–480. [Google Scholar] [CrossRef]
- Aali, J.; Rahimpour-Bonab, H.; Kamali, M.R. Geochemistry and origin of natural gas in the world’s largest gas field from Persian Gulf, Iran. J. Pet. Sci. Eng. 2006, 50, 163–175. [Google Scholar] [CrossRef]
- ASTM D5550; Standard Test Method for Specific Gravity of Soil Solids by Gas Pycnometer. ASTM International: West Conshohocken, PA, USA, 2014.
- ASTM D4404; Standard Test Method for Determination of Pore Volume and Pore Volume Distribution of Soil and Rock by Mercury Intrusion Porosimetry. ASTM International: West Conshohocken, PA, USA, 2018.
- ASTM D1188; Standard Test Method for Bulk Specific Gravity and Density of Compacted Asphalt Mixtures Using Coated Samples. ASTM International: West Conshohocken, PA, USA, 2022.
- Kahraman, S.; Altindag, R. A brittleness index to estimate fracture toughness. Int. J. Rock Mech. Min. Sci. 2004, 41, 343–348. [Google Scholar] [CrossRef]



| Equation | Strength-Based BI Correlation | Reference | Application |
|---|---|---|---|
| Equation (1) | [41] | Assess rock fragmentation efficiency. Predict possibility of rock burst. | |
| Equation (2) | |||
| Equation (3) | [42] | Investigate relationship between rock brittleness and drillability, cutability, drilling rate, etc. Equation (4) is used to predict FT. | |
| Equation (4) | [43] | ||
| Equation (5) | [44] | ||
| Equation (6) | [45] | Developed by linear fitting or regression analysis. Applications for these BIs were not reported. | |
| Equation (7) | [46] | ||
| Equation (8) | |||
| Equation (9) | [47] | Estimating FT modes I and II. Finding the positive linear interconnection of BI-9 and modes I and II. | |
| Equation (10) | [48] | Used when punch penetration test tools are not available. |
| GU | Lithology | Specific Gravity (g/cm3) | Porosity, n (%) | Bulk Density, ρ (g/cm3) | VP (m/s) | VS (m/s) |
|---|---|---|---|---|---|---|
| 1 | Dolomite and anhydritic dolomite | 2.87 | 5.25 | 2.80 | 3835 | 2332 |
| 2 | Anhydritic dolomite | 2.73 | 16.61 | 2.28 | 3207 | 1811 |
| 3 | Limestone | 2.71 | 1.57 | 2.50 | 3797 | 2284 |
| 4 | Anhydritic dolomite | 2.86 | 1.89 | 2.75 | 4325 | 2510 |
| 5 | Anhydritic dolomite | 2.87 | 0.46 | 2.54 | 4182 | 2483 |
| 6 | Limestone and dolomite | 2.71 | 19.29 | 2.50 | 2199 | 1262 |
| 7 | Limestone | 2.88 | 12.81 | 2.52 | 3129 | 2183 |
| 8 | Limestone | 2.77 | 24.41 | 2.09 | 3169 | 2189 |
| GU | UCS (MPa) | E (GPa) | ν | C (MPa) | φ (Degree) | BTS (MPa) | FT (MPa.m0.5) |
|---|---|---|---|---|---|---|---|
| 1 | 114.4 ± 0.5 | 40.8 | 0.25 | 30.6 | 43.1 | 9.86 ± 0.5 | 0.585 |
| 2 | 57.7 ± 0.5 | 21.0 | 0.20 | 15. 7 | 32.3 | 5.50 ± 0.5 | 0.474 |
| 3 | 107.4 ± 0.5 | 35.1 | 0.25 | 27.9 | 39.6 | 8.25 ± 0.5 | 0.551 |
| 4 | 162.2 ± 0.5 | 45.4 | 0.20 | 34.5 | 46.7 | 12.23 ± 0.5 | 1.285 |
| 5 | 127.1 ± 0.5 | 39.5 | 0.21 | 25.4 | 49.0 | 12.11 ± 0.5 | 1.140 |
| 6 | 29.7 ± 0.5 | 10.7 | 0.29 | 12.5 | 26.9 | 1.93 ± 0.5 | 0.425 |
| 7 | 48.8 ± 0.5 | 20.6 | 0.30 | 9.9 | 26.3 | 4.67 ± 0.5 | 1.950 |
| 8 | 49.0 ± 0.5 | 22.7 | 0.27 | 14.8 | 21.2 | 4.58 ± 0.5 | 0.580 |
| GU | Test Specimen | Confinement Pressure (MPa) | Breakdown Pressure (MPa) | HTS (MPa) |
|---|---|---|---|---|
| 1 | A | 20.0 ± 0.5 | 45.2 ± 0.5 | 19.24 |
| B | 30.0 ± 0.5 | 63.7 ± 0.5 | ||
| C | 40.0 ± 0.5 | 72.8 ± 0.5 | ||
| 2 | A | 20.0 ± 0.5 | 33.0 ± 0.5 | 9.30 |
| B | 30.0 ± 0.5 | 48.5 ± 0.5 | ||
| C | 40.0 ± 0.5 | 57.7 ± 0.5 | ||
| 3 | A | 20.0 ± 0.5 | 36.0 ± 0.5 | 20.63 |
| B | 30.0 ± 0.5 | 49.6 ± 0.5 | ||
| C | 40.0 ± 0.5 | 53.1 ± 0.5 | ||
| 4 | A | 20.0 ± 0.5 | 42.0 ± 0.5 | 18.32 |
| B | 30.0 ± 0.5 | 59.0 ± 0.5 | ||
| C | 40.0 ± 0.5 | 67.2 ± 0.5 | ||
| 5 | A | 20.0 ± 0.5 | 42.2 ± 0.5 | 17.75 |
| B | 30.0 ± 0.5 | 56.0 ± 0.5 | ||
| C | 40.0 ± 0.5 | 67.1 ± 0.5 | ||
| 6 | A | 20.0 ± 0.5 | 32.5 ± 0.5 | 8.67 |
| B | 30.0 ± 0.5 | 39.0 ± 0.5 | ||
| C | 40.0 ± 0.5 | 54.8 ± 0.5 | ||
| 7 | A | 5.0 ± 0.5 | 11.5 ± 0.5 | 7.20 |
| B | 10.0 ± 0.5 | 15.0 ± 0.5 | ||
| C | 15.0 ± 0.5 | 19.7 ± 0.5 | ||
| 8 | A | 10.0 ± 0.5 | 20.1 ± 0.5 | 11.38 |
| B | 20.0 ± 0.5 | 30.6 ± 0.5 | ||
| C | 30.0 ± 0.5 | 38.4 ± 0.5 |
| Correlation | R2 | RMSE | MAPE |
|---|---|---|---|
| HTS = 0.1003 × UCS + 5.318 | 0.75 | 2.53 | 16.33 |
| HTS = 1.1912 × BTS + 5.243 | 0.69 | 2.81 | 19.27 |
| HTS = 0.395 × E + 2.4036 | 0.80 | 2.26 | 15.38 |
| HTS = −0.484 × n + 19.026 | 0.67 | 2.90 | 22.27 |
| HTS = 12.861 × ρ − 18.073 | 0.30 | 4.27 | 33.31 |
| HTS = 0.0064 × VP − 8.1304 | 0.66 | 2.96 | 23.18 |
| HTS = 0.0089 × VS − 4.9005 | 0.46 | 3.75 | 28.45 |
| GU | Equation (1) | Equation (2) | Equation (3) | Equation (4) | Equation (5) | Equation (6) | Equation (7) | Equation (8) | Equation (9) | Equation (10) |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 11.6 | 0.84 | 62.2 | 564.2 | 23.8 | 15.6 | 5.5 | 69.6 | 16.8 | 68.9 |
| 2 | 10.5 | 0.83 | 31.6 | 158.6 | 12.6 | 12.3 | 0.8 | 55.7 | 8.4 | 45.3 |
| 3 | 13.0 | 0.86 | 57.8 | 443.0 | 21.0 | 17.5 | 6.0 | 84.7 | 13.5 | 72.9 |
| 4 | 13.3 | 0.86 | 87.2 | 991.9 | 31.5 | 19.9 | 10.4 | 95.8 | 19.7 | 90.8 |
| 5 | 10.5 | 0.83 | 69.6 | 769.7 | 27.7 | 13.4 | 5.5 | 72.2 | 19.1 | 67.3 |
| 6 | 15.4 | 0.88 | 15.8 | 28.6 | 5.4 | 12.1 | 0.9 | 77.7 | 2.9 | 43.5 |
| 7 | 10.5 | 0.83 | 26.7 | 114.0 | 10.7 | 11.9 | 0.3 | 33.0 | 7.5 | 41.6 |
| 8 | 10.7 | 0.83 | 26.8 | 112.3 | 10.6 | 12.1 | 0.4 | 45.9 | 7.8 | 42.4 |
| Correlation | Correlation Equation | R2 | RMSE | MAPE |
|---|---|---|---|---|
| HTS–BI-1 | 0.21 | 5.69 | 49.96 | |
| HTS–BI-2 | 0.07 | 4.89 | 39.00 | |
| HTS–BI-3 | 0.69 | 2.95 | 21.08 | |
| HTS–BI-4 | 0.74 | 2.56 | 18.89 | |
| HTS–BI-5 | 0.74 | 3.65 | 20.37 | |
| HTS–BI-6 | 0.94 | 1.55 | 3.45 | |
| HTS–BI-7 | 0.86 | 1.95 | 13.90 | |
| HTS–BI-8 | 0.50 | 3.73 | 24.37 | |
| HTS–BI-9 | 0.72 | 2.66 | 17.92 | |
| HTS–BI-10 | 0.87 | 0.88 | 4.31 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ezazi, M.; Ghorbani, E.; Shafiei, A.; Sharifi Teshnizi, E.; O’Kelly, B.C. Laboratory Hydraulic Tensile Strength Correlation with Strength-Based Brittleness Indices for Carbonate Reservoirs. Geosciences 2024, 14, 52. https://doi.org/10.3390/geosciences14020052
Ezazi M, Ghorbani E, Shafiei A, Sharifi Teshnizi E, O’Kelly BC. Laboratory Hydraulic Tensile Strength Correlation with Strength-Based Brittleness Indices for Carbonate Reservoirs. Geosciences. 2024; 14(2):52. https://doi.org/10.3390/geosciences14020052
Chicago/Turabian StyleEzazi, Mohammad, Ebrahim Ghorbani, Ali Shafiei, Ebrahim Sharifi Teshnizi, and Brendan C. O’Kelly. 2024. "Laboratory Hydraulic Tensile Strength Correlation with Strength-Based Brittleness Indices for Carbonate Reservoirs" Geosciences 14, no. 2: 52. https://doi.org/10.3390/geosciences14020052
APA StyleEzazi, M., Ghorbani, E., Shafiei, A., Sharifi Teshnizi, E., & O’Kelly, B. C. (2024). Laboratory Hydraulic Tensile Strength Correlation with Strength-Based Brittleness Indices for Carbonate Reservoirs. Geosciences, 14(2), 52. https://doi.org/10.3390/geosciences14020052









