Exploring the Deuterium Excess of Cretaceous Arctic Paleoprecipitation Using Stable Isotope Composition of Clay Minerals from the Prince Creek Formation (Maastrichtian) in Northern Alaska
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Clay Mineralogy and Chemical Composition
3.2. δ18O and δD of Meteoric Water from Pedogenic Phyllosilicates
| Sample | Formation | δ18Omix V-SMOW (±0.3%) | δDmix V-SMOW (±4%) | Wt. % Kaolinite (±5%) | a 103ln18α2:1-water | b 103lnDα2:1-water = −2.2*106*T−2+ | c 103ln18αmix-water | d 103lnDαmix-water= −2.2*106*T−2+ | e δDmeteoric water V-SMOW | f δ18Ometeoric water V-SMOW |
|---|---|---|---|---|---|---|---|---|---|---|
| Paleosol | ||||||||||
| 06 SH 9.2 | Prince Creek | 11.8 | −3 | 23 | 2.84 × 106 × T−2–7.03 | −18.51 | 2.82 × 106 × T−2–6.96 | −13.40 | −106 | −17.2 |
| 06 SH 14.4 | Prince Creek | 10.3 | −83 | 15 | 2.83 × 106 × T−2–6.90 | −19.43 | 2.82 × 106 × T−2–6.87 | −15.48 | −95 | −18.8 |
| 06 SH 15.1 | Prince Creek | 11.5 | −72 | 25 | 2.81 × 106 × T−2–7.00 | −18.28 | 2.80 × 106 × T−2–6.93 | −12.97 | −86 | −17.3 |
| Bentonite | ||||||||||
| PFDV 17 | Prince Creek | 5.03 | −136 | 0 | 2.55 × 106 × T−2–4.05 | −19.38 | 2.55 × 106 × T−2–4.05 | −19.38 | −147 | −23.6 |
| PFDV 17 duplicate | Prince Creek | 5.03 | −132 | 0 | 2.55 × 106 × T−2–4.05 | −19.38 | 2.55 × 106 × T−2–4.05 | −19.38 | −142 | −23.6 |
| 06KKT-20.5 | Prince Creek | 4.96 | −169 | 0 | 2.55 × 106 × T−2–4.05 | −15.44 | 2.55 × 106 × T−2–4.05 | −15.44 | −177 | −24.6 |
| 06KKT-20.5 duplicate | Prince Creek | 4.96 | −172 | 0 | 2.55 × 106 × T−2–4.05 | −15.44 | 2.55 × 106 × T−2–4.05 | −15.44 | −179 | −24.6 |
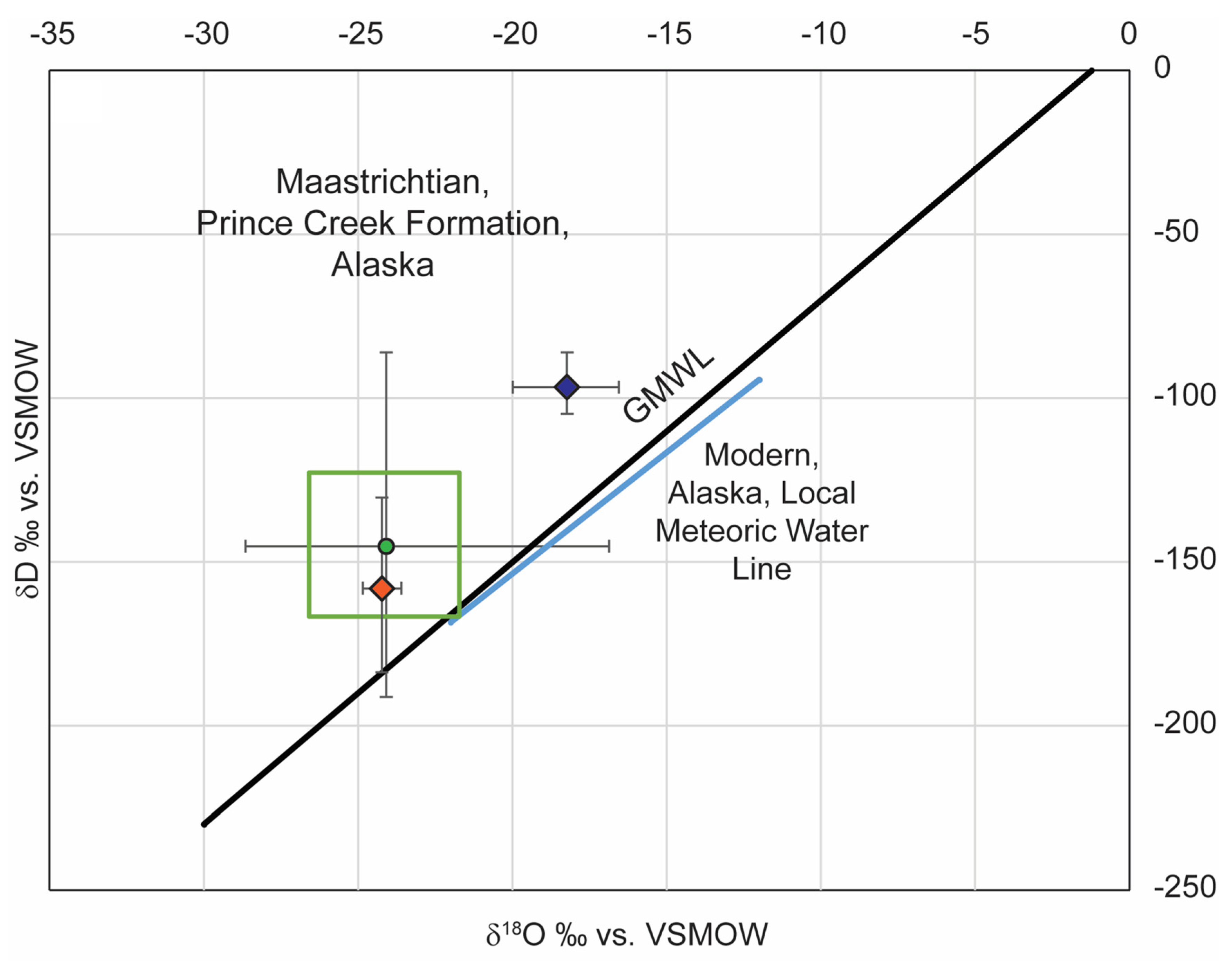
4. Discussion
4.1. Stable Isotope Composition of Phyllosilicates and Use as Paleoclimate Proxies
- Phyllosilicates analyzed from a paleosol are authigenic.
- Phyllosilicates have not been diagenetically altered since the time of formation.
- The relationship between the oxygen and hydrogen isotope compositions of soil water is known.
4.2. Implications of δ18O and δD of Meteoric Water from Pedogenic Phyllosilicates
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barron, E.J. Warm, equable Cretaceous: The nature of the problem. Earth Sci. Rev. 1983, 19, 305–338. [Google Scholar] [CrossRef]
- Ruddiman, W.F. Earth’s Climate: Past and Future, 2nd ed.; W. H. Freeman and Company: New York, NY, USA, 2008. [Google Scholar]
- Poulsen, C.J. Palaeoclimate: A balmy Arctic. Nature 2004, 432, 814. [Google Scholar] [CrossRef] [PubMed]
- Suarez, C.A.; Ludvigson, G.A.; Gonzalez, L.; Fiorillo, A.; Flaig, P.; McCarthy, P.J. Use of Multiple Oxygen Isotope Proxies for Elucidating Arctic Cretaceous Palaeo-Hydrology. In Isotopic Studies in Cretaceous Research; Bojar, A.-V., Melinte-Dobrinescu, M.C., Smit, J., Eds.; Geological Society London, Special Publications: London, UK, 2013; Volume 382, pp. 185–202. [Google Scholar]
- Ludvigson, G.A.; Diefendorf, A.F.; Suarez, M.B.; González, L.A.; Corcoran, M.C.; Schlanser, K.; Flaig, P.P.; McCarthy, P.J.; van der Kolk, D.; Houseknecht, D.; et al. Stable Isotope Tracers of Cretaceous Arctic Paleoprecipitation. Geosciences 2022, 12, 143. [Google Scholar] [CrossRef]
- Moore, T.E.; Wallace, W.K.; Bird, K.J.; Karl, S.M.; Mull, C.G.; Dillon, J.T. Geology of Northern Alaska. In The Geology of Alaska: The Geology of North America; Plafker, G., Berg, H.C., Eds.; v. G-1; The Geological Society of America: Boulder, CO, USA, 1994; pp. 49–140. [Google Scholar]
- Cole, F.; Bird, K.J.; Toro, J.; Roure, F.; O’Sullivan, P.B.; Pawlewicz, M.; Howell, D.G. An integrated model for the tectonic development of the frontal Brooks Range and Colville Basin 250 km west of the Trans-Alaska Crustal Transect. J. Geophys. Res. 1997, 102, 20685–20708. [Google Scholar] [CrossRef]
- Mull, C.G.; Houseknecht, D.W.; Bird, K.J. Revised Cretaceous and Tertiary Stratigraphic Nomenclature in the Colville Basin, Northern Alaska; Professional Paper; United States Geological Survey: Reston, VA, USA, 2003; Volume 1673, pp. 1–51.
- Flaig, P.P.; McCarthy, P.J.; Fiorillo, A.R. A tidally influenced, high-latitude coastal plain: The Upper Cretaceous (Maastrichtian) Prince Creek Formation, North Slope, Alaska. In From River to Rock Record: The Preservation of Fluvial Sediments and Their Subsequent Interpretation; Davidson, S., Lelu, S., North, C., Eds.; SEPM Special Publication: Tulsa, Oklahoma, 2011; Volume 97, pp. 233–264. [Google Scholar]
- Flaig, P.P.; McCarthy, P.J.; Fiorillo, A.R. Anatomy, evolution and paleoenvironmental interpretation of an ancient Arctic coastal plain: Integrated paleopedology and palynology from the Upper Cretaceous (Maastrichtian) Prince Creek Formation, North Slope, Alaska. In New Frontiers in Paleopedology and Terrestrial Paleoclimatology; Driese, S.G., Nordt, L.D., Eds.; SEPM Special Publication: Tulsa, Oklahoma, 2013; Volume 104, pp. 179–230. [Google Scholar]
- Fiorillo, A.R.; McCarthy, P.J.; Flaig, P.P.; Brandlen, E.; Norton, D.; Jacobs, L.; Zippi, P.; Gangloff, R.A. Paleontology and Paleoenvironmental Interpretation of the Kikak-Tegoseak Dinosaur Quarry (Prince Creek Formation: Late Cretaceous), Northern Alaska: A Multi-Disciplinary Study of an Ancient, High-Latitude Ceratopsian Dinosaur Bonebed, In New Perspectives on Horned Dinosaurs. The Royal Tyrell Museum Ceraptopsian Symposium; Ryan, M.J., Chinner-Algeier, B.J., Eberth, D.A., Eds.; University Press: Bloomington, IN, USA, 2010; pp. 456–477. [Google Scholar]
- Brouwers, E.M.; Clemens, W.A.; Spicer, R.A.; Ager, T.A.; Carter, L.D.; Sliter, W.V. Dinosaurs on the North Slope, Alaska: High latitude latest Cretaceous environments. Science 1987, 25, 1608–1610. [Google Scholar] [CrossRef] [PubMed]
- Witte, W.K.; Stone, D.B.; Mull, C.G. Paleomagnetism, paleobotany, and paleogeography of the Cretaceous North Slope, Alaska. In Alaska North Slope Geology; Tailleur, I., Weimer, P., Eds.; The Pacific Section: Alameda, CA, USA; SEPM: Tulsa, Oklahoma; Alaska Geological Society: Anchorage, AK, USA, 1987; Volume 1, pp. 571–579. [Google Scholar]
- Salazar Jaramillo, S.; McCarthy, P.J.; Trainor, T.; Fowell, S.J.; Fiorillo, A.R. Origin of clay minerals in alluvial paleosols, Prince Creek Formation, North Slope, Alaska: Influence of volcanic ash on pedogenesis in the Late Cretaceous Arctic. J. Sediment. Res. 2015, 85, 192–208. [Google Scholar] [CrossRef]
- Rich, T.H.; Gangloff, R.A.; Hammer, W.H. Polar Dinosaurs. Science 2002, 295, 979–980. [Google Scholar] [CrossRef]
- Fiorillo, A.R.; McCarthy, P.J.; Kobayashi, Y.; Suarez, M.A. Cretaceous dinosaurs across Alaska show the role paleoclimate played in structuring large ancient herbivore populations. Geosciences 2022, 12, 161. [Google Scholar] [CrossRef]
- Stern, L.A.; Chamberlain, C.P.; Reynolds, R.C.; Johnson, G.D. Oxygen isotope evidence of climate change from pedogenic clay minerals in the Himalayan molasses. Geochim. Cosmochim. Acta 1997, 61, 731–744. [Google Scholar] [CrossRef]
- Tabor, N.J.; Montañez, I.P.; Southard, R.J. Paleoenvironmental reconstruction from chemical and isotopic compositions of Permo-Pennsylvanian pedogenic minerals. Geochim. Cosmochim. Acta 2002, 66, 3093–3107. [Google Scholar] [CrossRef]
- Vitali, F.; Longstaffe, F.J.; McCarthy Plint, P.J.; Guy, A.; Caldwell, W.; Glen, E. Stable isotopic investigation of clay minerals and pedogenesis in an interfluve paleosol from the Cenomanian Dunvegan Formation, N.E. British Columbia, Canada. Chem. Geol. 2002, 192, 269–287. [Google Scholar] [CrossRef]
- Tabor, N.J.; Montañez, I.P. Oxygen and hydrogen isotope compositions of pedo-genic phyllosilicates: Development of modern surface domain arrays and implications for paleotemperature reconstructions. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2005, 223, 127–146. [Google Scholar] [CrossRef]
- Myers, T.S.; Tabor, N.J.; Jacobs, L.L.; Mateus, O. Palaeoclimate of the Late Jurassic of Portugal: Comparison with the western United States. Sedimentology 2012, 59, 1695–1717. [Google Scholar] [CrossRef]
- Rosenau, N.A.; Tabor, N.J. Oxygen and hydrogen isotope compositions of paleosol phyllosilicates: Differential burial histories and determination of Middle-Late Pennsylvanian low-latitude terrestrial paleotemperatures. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2013, 392, 383–397. [Google Scholar] [CrossRef]
- Andrzejewski, K.A.; Tabor, N.J. Paleoenvironmental and paleoclimatic reconstruction of Cretaceous (Aptian-Cenomanian) terrestrial formations of Texas and Oklahoma using phyllosilicates. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2020, 543, 109491. [Google Scholar] [CrossRef]
- Savin, S.M.; Epstein, S. The oxygen and hydrogen isotope geochemistry of clay minerals. Goechim. Cosmochim. Acta 1970, 34, 25–42. [Google Scholar] [CrossRef]
- Lawrence, J.R.; Taylor, H.P. Deuterium and 18O correlation: Clay minerals and hydroxides in Quaternary soils compared to meteoric waters. Geochim. Cosmochim. Acta 1971, 35, 993–1003. [Google Scholar] [CrossRef]
- Moore, D.M.; Reynolds, R.C. X-ray Diffraction and the Identification and Analysis of Clay Minerals; Oxford University Press: New York, NY, USA, 1997; p. 378. [Google Scholar]
- Clayton, R.N.; Maydeda, T.K. The use of bromine pentafluoride in the extraction of oxygen from oxides and silicates for isotopic analysis. Geochim. Cosmochim. Acta 1963, 27, 43–52. [Google Scholar] [CrossRef]
- Gonfiantini, R. Advisory Group Meeting on Stable Isotope Reference Samples for Geochemical and Hydrological Investigations; Report International Atomic Energy Agency: Vienna, Austria, 1984. [Google Scholar]
- Salazar-Jaramillo, S.; McCarthy, P.J.; Ochoa, A.; Fowell, S.J.; Longstaffe, F.J. Data supporting Maastrichtian paleoclimate variables applying a multi proxy approach to a paleosol profile, Arctic Alaska. Data Brief 2020, 29, 105191. [Google Scholar] [CrossRef] [PubMed]
- Sharp, Z.D.; Atudorei, V.; Durakiewicz, T. A rapid method for determination of hydrogen and oxygen isotope ratios from water and hydrous minerals. Chem. Geol. 2001, 178, 197–210. [Google Scholar] [CrossRef]
- VanDeVelde, J.H.; Bowen, G.J. Effects of chemical pretreatments on the hydrogen isotope composition of 2:1 clay minerals. Rapid Commun. Mass Spectrom. 2013, 27, 1143–1148. [Google Scholar] [CrossRef] [PubMed]
- Bauer, K.K.; Vennemann, T.W. Analytical methods for the measurement of hydrogen isotope composition and water content in clay minerals by TC/EA. Chem. Geol. 2014, 363, 229–240. [Google Scholar] [CrossRef]
- Qi, H.; Coplen, T.B.; Gehre, M.; Vennemann, T.W.; Brand, W.A.; Geilmann, H.; Olack, G.; Bindeman, I.N.; Palandri, J.; Huang, L.; et al. New biotite and muscovite isotopic reference materials, USGS57 and USGS58, for δ2H measurements—A replacement for NBS 30. Chem. Geol. 2017, 467, 89–99. [Google Scholar] [CrossRef]
- Kanik, N.J.; Longstaffe, F.J.; Kuligiewicz, A.; Derkowski, A. Systematics of smectite hydrogen-isotope composition: Structural hydrogen versus absorbed water. Appl. Clay Sci. 2022, 216, 106338. [Google Scholar] [CrossRef]
- Coplen, T.B. New guidelines for reporting stable hydrogen, carbon, and oxygen isotope-ratio data. Geochim. Cosmochim. Acta 1996, 60, 3359–3360. [Google Scholar] [CrossRef]
- Savin, S.M.; Lee, M. Isotopic Studies of Phyllosilicates. Rev. Mineral. Geochem. 1988, 19, 189–223. [Google Scholar]
- Sheppard, S.M.F.; Gilg, H.A. Stable isotope geochemistry of clay minerals. Clay Miner. 1996, 31, 1–24. [Google Scholar] [CrossRef]
- Spicer, R.A.; Herman, A.B. The Late Cretaceous environment of the Arctic: A quantitative reassessment based on plant fossils. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2010, 295, 423–442. [Google Scholar] [CrossRef]
- Ludvigson, G.A.; González, L.A.; Fowle, D.A.; Roberts, J.A.; Driese, S.G.; Villarreal, M.A.; Smith, J.J.; Suarez, M.B. Paleoclimatic applications and modern process studies of pedogenic siderite. In New Frontiers in Paleopedology and Terrestrial Paleoclimatology; Driese, S.G., Nordt, L.C., McCarthy, P.J., Eds.; SEPM Special Publication: Broken Arrow, OK, USA, 2013; Volume 104, pp. 79–87. [Google Scholar]
- Gilg, H.A.; Sheppard, S.M.F. Hydrogen isotope fractionation between smectites and water. Terra Nova 1995, 7, 329. [Google Scholar]
- Rozanski, K.; Araguás-Araguás, L.; Gonfiantini, R. Isotopic patterns in modern global precipitation. Climate Change in Continental Isotopic Records. Am. Geophys. Monogr. 1993, 78, 1–36. [Google Scholar]
- Burns, W.M.; Hayba, D.O.; Rowan, E.L.; Houseknecht, D.W. Estimating the amount of eroded section in a partially exhumed basin from geophysical well logs: An example from the North Slope. In Studies by the U.S. Geological Survey in Alaska; Special Paper; Haeussler, P.J., Galloway, J.P., Eds.; U.S. Geological Survey: Reston, VA, USA, 2005; Volume 1732, pp. 1–18. [Google Scholar]
- Robinson, M.S. Kerogen Microscopy of Coal and Shales from the North Slope of Alaska; Public Data File; Alaska Division of Geological and Geophyscial Surveys: Fairbanks, AK, USA, 1989; Volume 89. [Google Scholar]
- Johnson, M.J.; Howell, D.G. Thermal Maturity of Sedimentary Basins in Alaska: An Overview; U.S. Geological Survey: Reston, VA, USA, 1996; Volume 2142, pp. 1–131.
- Barker, C.E.; Pawlewicz, M.J. The correlation of vitrinite reflectance with maximum paleotemperature in humic organic matter. In Paleogeothermics; Springer: New York, NY, USA, 1986; pp. 79–93. [Google Scholar]
- Meunier, A. Clays; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–472. [Google Scholar]
- Craig, H. Isotopic variations in meteoric waters. Science 1961, 133, 1702–1708. [Google Scholar] [CrossRef] [PubMed]
- Yapp, C.J. Climatic implications of surface domains in arrays of δD and δ18O from hydroxyl minerals: Goethite as an example. Geochim. Cosmichim. Acta 2000, 64, 2009–2025. [Google Scholar] [CrossRef]
- Dansgaard, W. Stable isotopes in precipitation. Tellus 1964, 16, 436–468. [Google Scholar] [CrossRef]
- Bowen, G.J.; Cai, Z.; Fiorella, R.P.; Putman, A.L. Isotopes in the Water Cycle: Regional- to Global-Scale Patterns and Applications. Annu. Rev. Earth Planet. Sci. 2019, 47, 453–479. [Google Scholar] [CrossRef]
- Craig, H.; Gordon, L.I. Deuterium and Oxygen-18 Variations in the Ocean and the Marine Atmosphere. In Proceedings of the Conference on Stable Isotopes in Oceanographic Studies and Paleotemperatures, Pisa, Italy, 26–30 July 1965; Tongiorgi, E., Ed.; pp. 9–130. [Google Scholar]
- Gat, J.R.; Bowser, C.J.; Kendall, C. The contribution of evaporation from the Great Lakes to the continental atmosphere: Estimate based on stable isotope data. Geophys. Res. Lett. 1994, 21, 557–560. [Google Scholar] [CrossRef]
- Good, S.P.; Mallia, D.V.; Lin, J.C.; Bowen, G.J. Stable isotope analysis of precipitation samples obtained via crowdsourcing reveals the spatiotemporal evolution of Superstorm Sandy. PLoS ONE 2014, 9, e91117. [Google Scholar] [CrossRef]
- Cropper, S.; Solander, K.; Newman, B.D.; Tuinenburg, O.A.; Staal, A.; Theeuwen, J.J.E.; Xu, C. Comparing deuterium excess to large-scale precipitation recycling models in the tropics npj. Clim. Atmos. Sci. 2021, 4, 60. [Google Scholar] [CrossRef]
- Talbot, M.R.; Kelts, K. Primary and diagenetic carbonates in the anoxic sediments of Lake Bosumtwi, Ghana. Geology 1986, 14, 912–916. [Google Scholar] [CrossRef]
- Mozley, P.S.; Wersin, P. Isotopic composition of siderite as an indicator of depositional environment. Geology 1992, 20, 817–820. [Google Scholar] [CrossRef]
- North, J.C.; Frew, R.D.; Van Hale, R. Can stable isotopes be used to monitor landfill leachate impact on surface waters? J. Geophys. Explor. 2006, 88, 49–53. [Google Scholar] [CrossRef]
- Andrei, F.; Barbieri, M.; Sappa, G. Application of 2H and 18O isotopes for tracing municipal solid waste landfill contamination of groundwater: Two Italian case histories. Water 2021, 13, 1065. [Google Scholar] [CrossRef]
- Castañeda, S.; Sucgang, R.; Almoneda, R.; Mendoza, N.; David, C. Environmental isotopes and major ions for tracing leachate contamination from a municipal landfill in Metro Manila, Philippines. J. Environ. Radioact. 2012, 110, 20–37. [Google Scholar] [CrossRef] [PubMed]


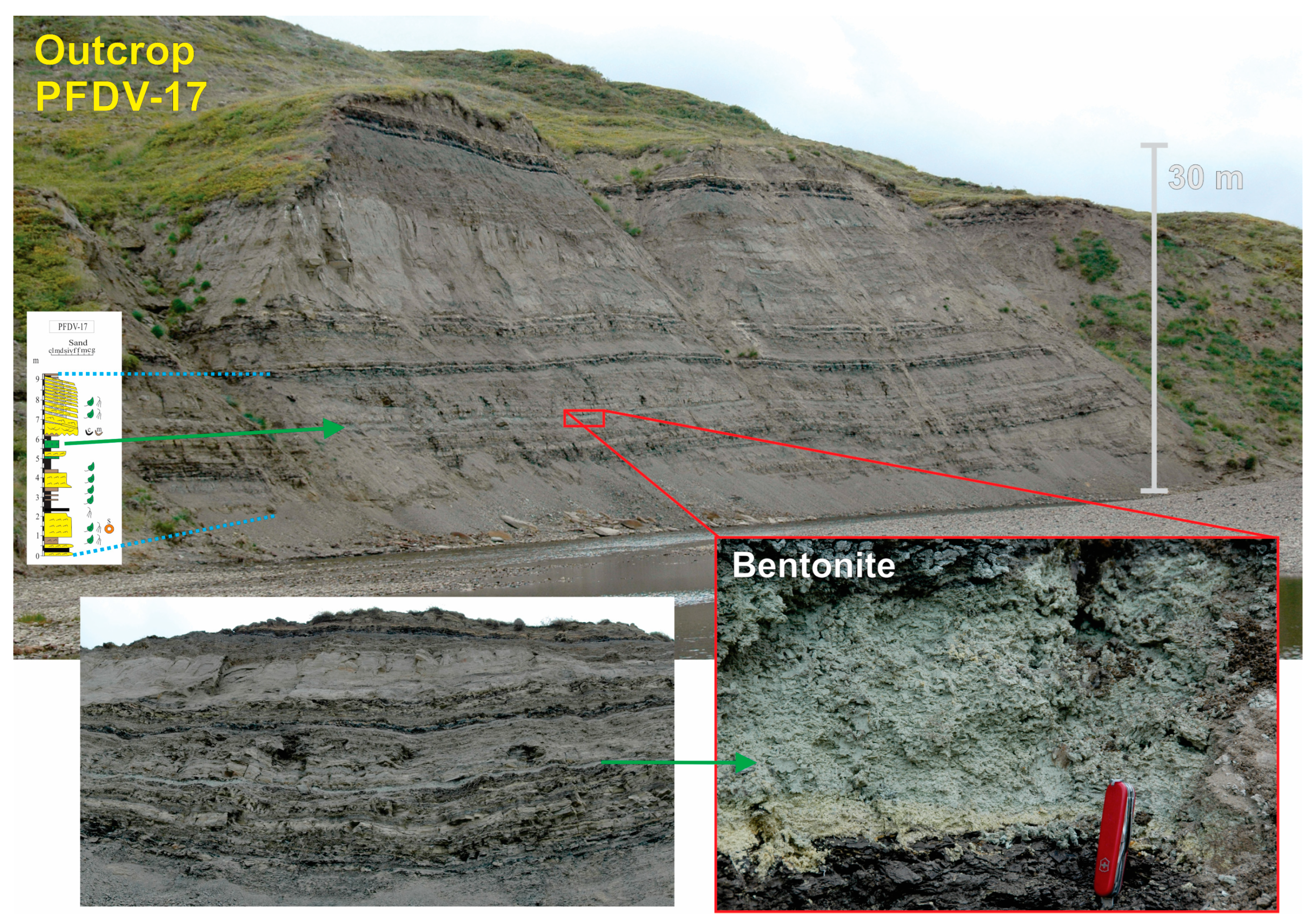

| Sample | Formation | Na2O | MgO | MnO | Al2O3 | SiO2 | K2O | CaO | Fe2O3 | TiO2 | Total | 2:1 Phyllosilicate (wt.%) | Kaolinite (wt.%) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Paleosol | |||||||||||||
| 06 SH 9.2 | Prince Creek | 2.51 | 1.68 | 0.01 | 24.57 | 56.99 | 2.56 | 0.03 | 5.56 | 1.04 | 94.95 | 77 | 23 |
| 06 SH 14.4 | Prince Creek | 2.28 | 1.67 | 0.02 | 23.40 | 57.39 | 2.50 | 0.03 | 6.51 | 1.01 | 94.81 | 85 | 15 |
| 06 SH 15.1 | Prince Creek | 2.01 | 2.18 | 0.04 | 24.29 | 57.23 | 3.21 | 0.11 | 5.25 | 1.09 | 95.41 | 75 | 25 |
| Bentonite | |||||||||||||
| * PFDV 17 | Prince Creek | 2.24 | 1.82 | 0.02 | 19.31 | 66.96 | 0.49 | 1.37 | 4.71 | 0.19 | 97.11 | 100 | 0 |
| ** 06 KKT-20.5 | Prince Creek | 0.19 | 1.84 | 0.01 | 20.67 | 60.78 | 0.07 | 1.91 | 6.04 | 0.21 | 91.72 | 100 | 0 |
| Sample | Formation | Chemical Formulae |
|---|---|---|
| Paleosol | ||
| 06 SH 9.2 | Prince Creek | (K0.26Na0.39)(Al1.37Fe0.33Mg0.20Ti0.06)(Si3.61Al0.39)O10(OH)2 |
| 06 SH 14.4 | Prince Creek | (K0.23Na0.32)(Al1.37Fe0.36Mg0.18Ti0.06)(Si3.67Al0.33)O10(OH)2 |
| 06 SH 15.1 | Prince Creek | (K0.33Na0.31Ca0.01)(Al1.33Fe0.32Mg0.26Ti0.07)(Si3.61Al0.39)O10(OH)2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andrzejewski, K.; Ludvigson, G.; Suarez, M.; McCarthy, P.; Flaig, P. Exploring the Deuterium Excess of Cretaceous Arctic Paleoprecipitation Using Stable Isotope Composition of Clay Minerals from the Prince Creek Formation (Maastrichtian) in Northern Alaska. Geosciences 2023, 13, 273. https://doi.org/10.3390/geosciences13090273
Andrzejewski K, Ludvigson G, Suarez M, McCarthy P, Flaig P. Exploring the Deuterium Excess of Cretaceous Arctic Paleoprecipitation Using Stable Isotope Composition of Clay Minerals from the Prince Creek Formation (Maastrichtian) in Northern Alaska. Geosciences. 2023; 13(9):273. https://doi.org/10.3390/geosciences13090273
Chicago/Turabian StyleAndrzejewski, Kate, Greg Ludvigson, Marina Suarez, Paul McCarthy, and Peter Flaig. 2023. "Exploring the Deuterium Excess of Cretaceous Arctic Paleoprecipitation Using Stable Isotope Composition of Clay Minerals from the Prince Creek Formation (Maastrichtian) in Northern Alaska" Geosciences 13, no. 9: 273. https://doi.org/10.3390/geosciences13090273
APA StyleAndrzejewski, K., Ludvigson, G., Suarez, M., McCarthy, P., & Flaig, P. (2023). Exploring the Deuterium Excess of Cretaceous Arctic Paleoprecipitation Using Stable Isotope Composition of Clay Minerals from the Prince Creek Formation (Maastrichtian) in Northern Alaska. Geosciences, 13(9), 273. https://doi.org/10.3390/geosciences13090273










