Cyclic Steps Created by Flowing Water on Ice Surface
Abstract
1. Introduction
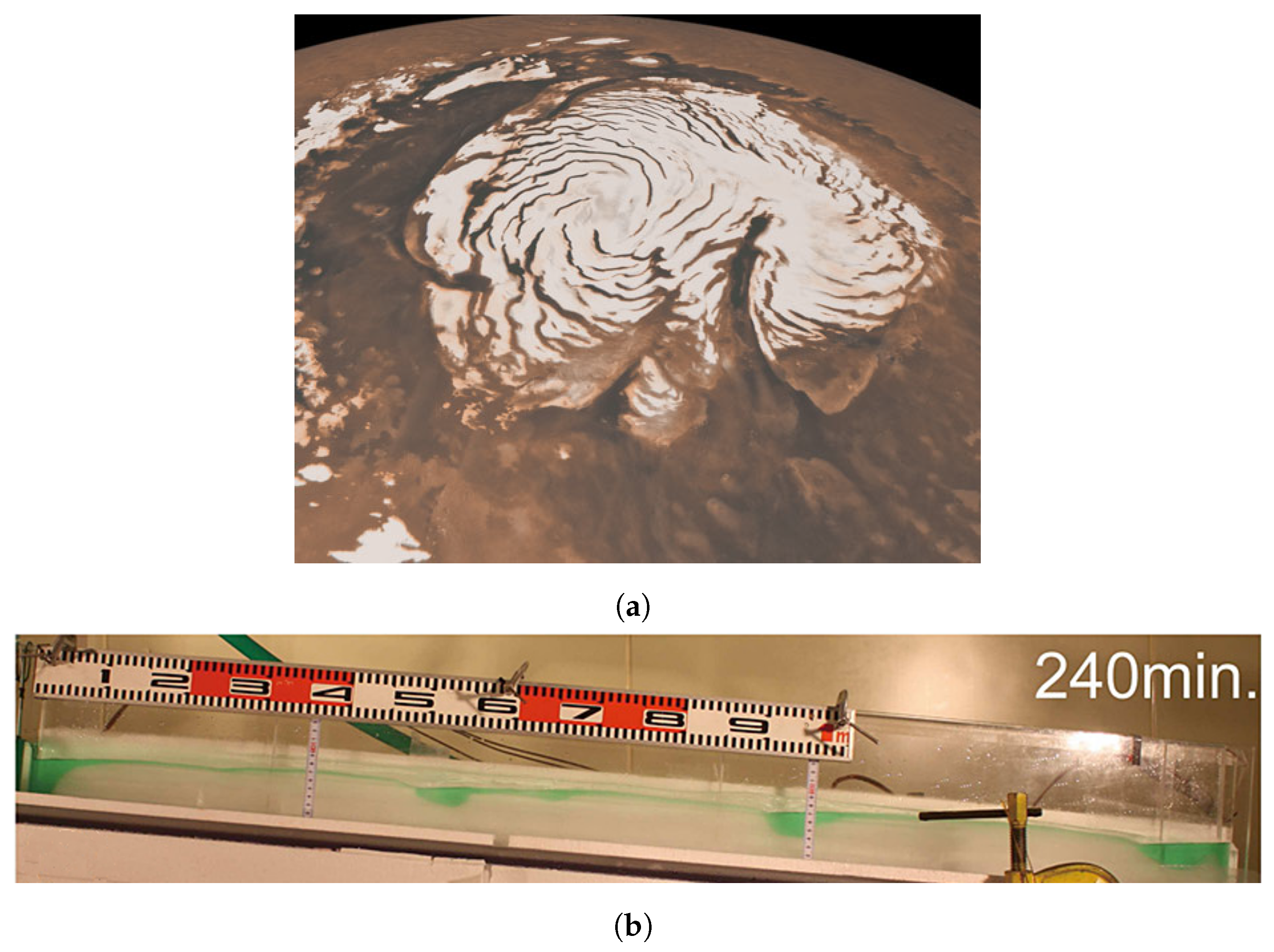
2. Methods
2.1. Formulation
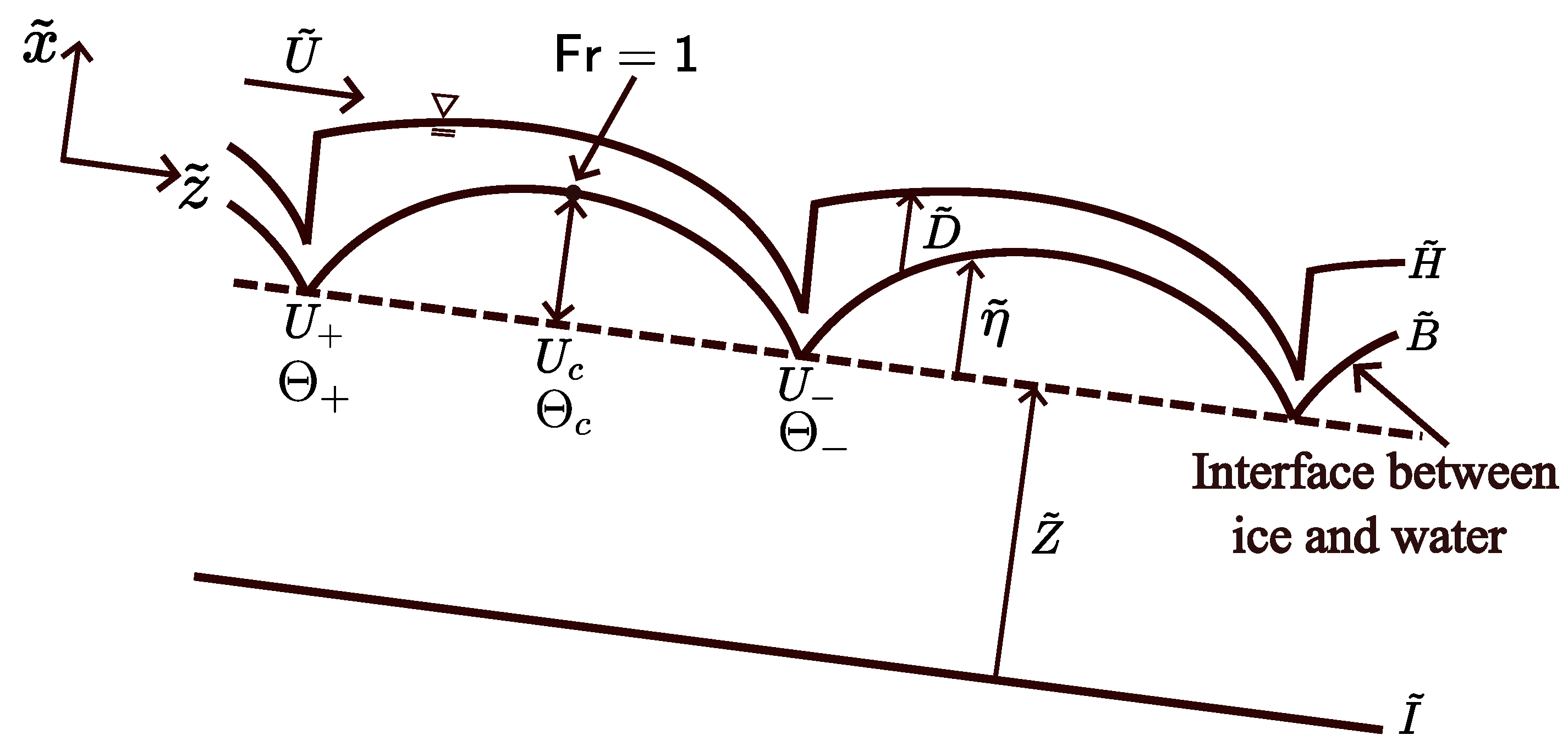
2.1.1. Governing Equations
2.1.2. Normalization
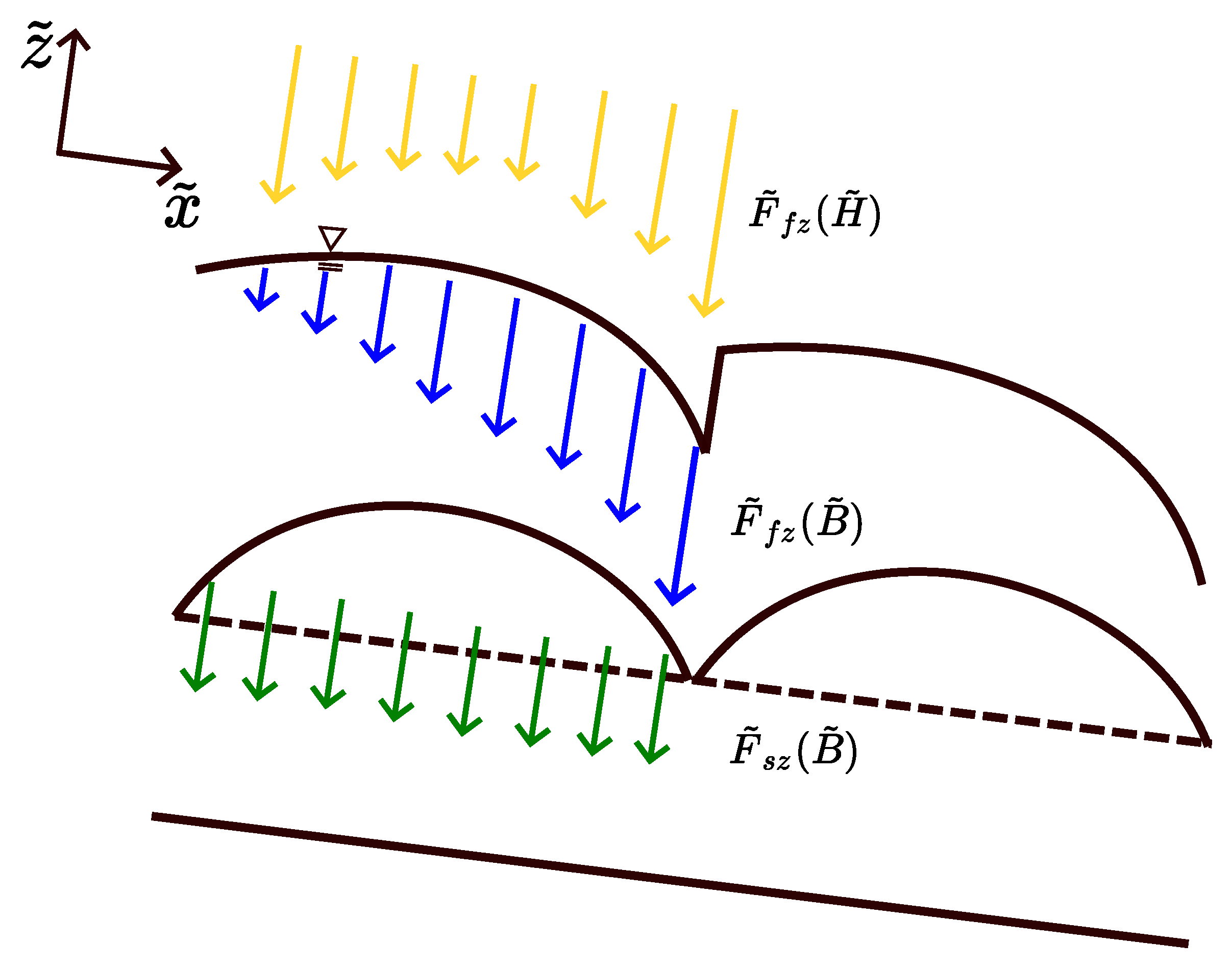
2.1.3. Boundary Conditions
2.2. Solution Method
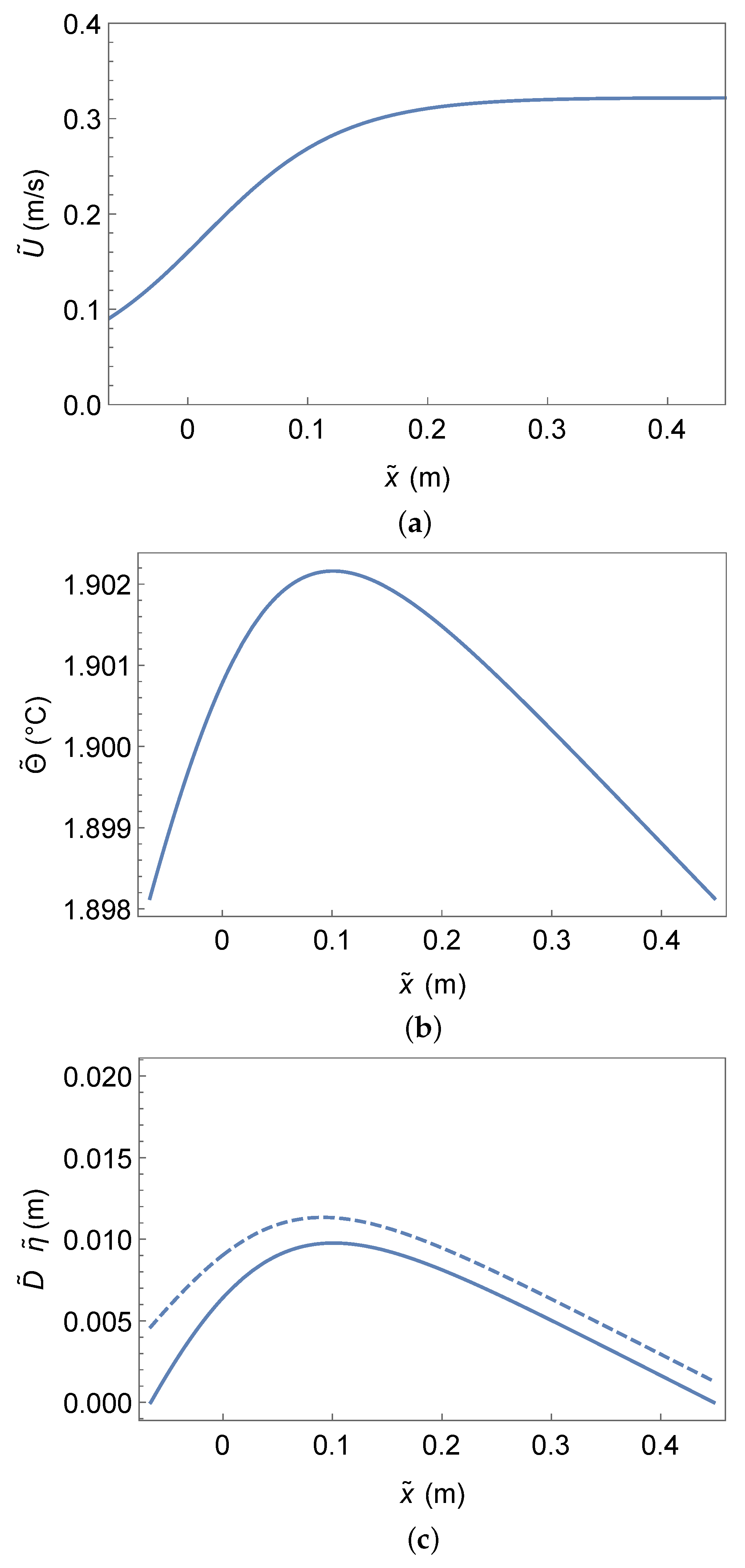
3. Results
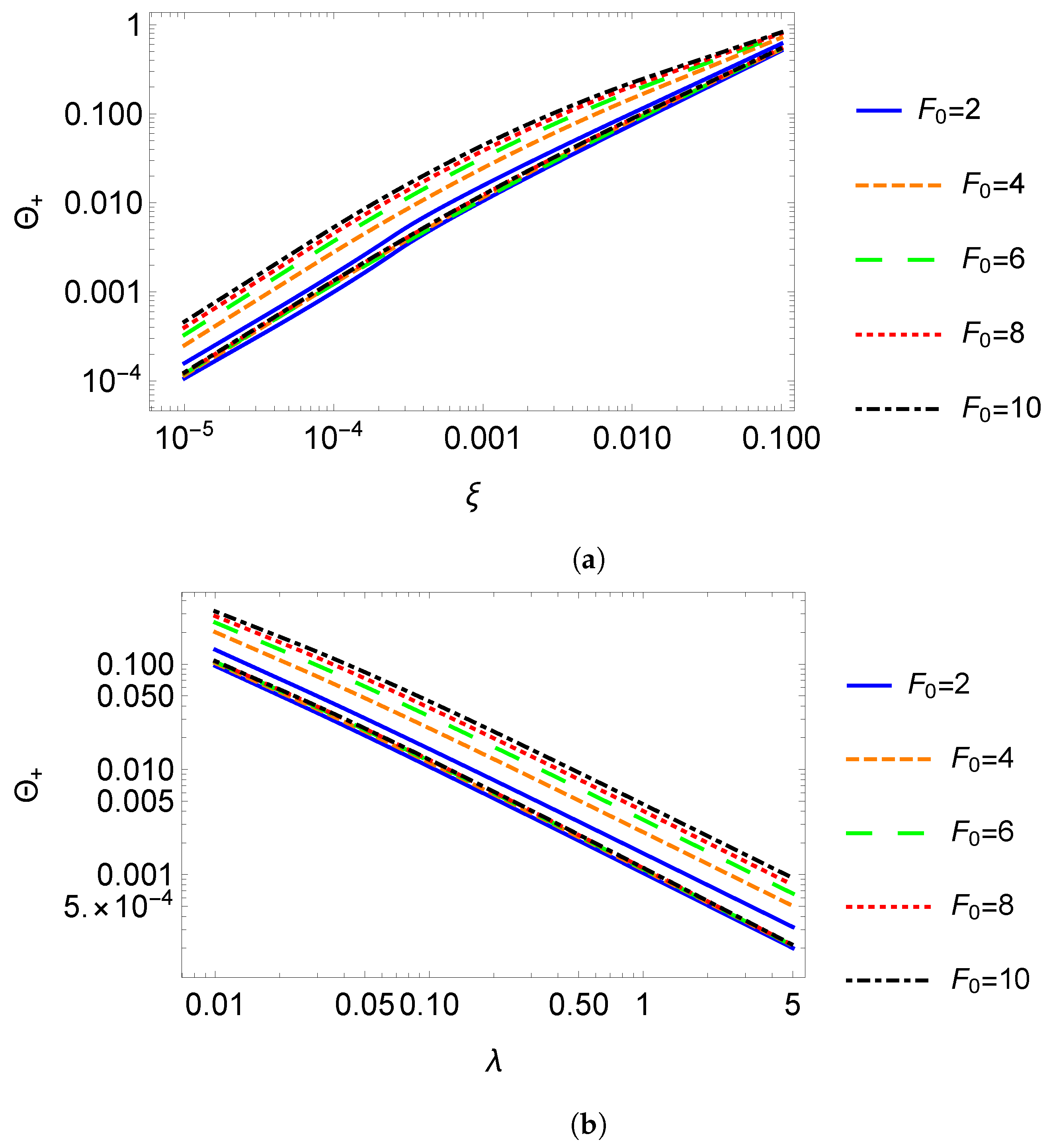
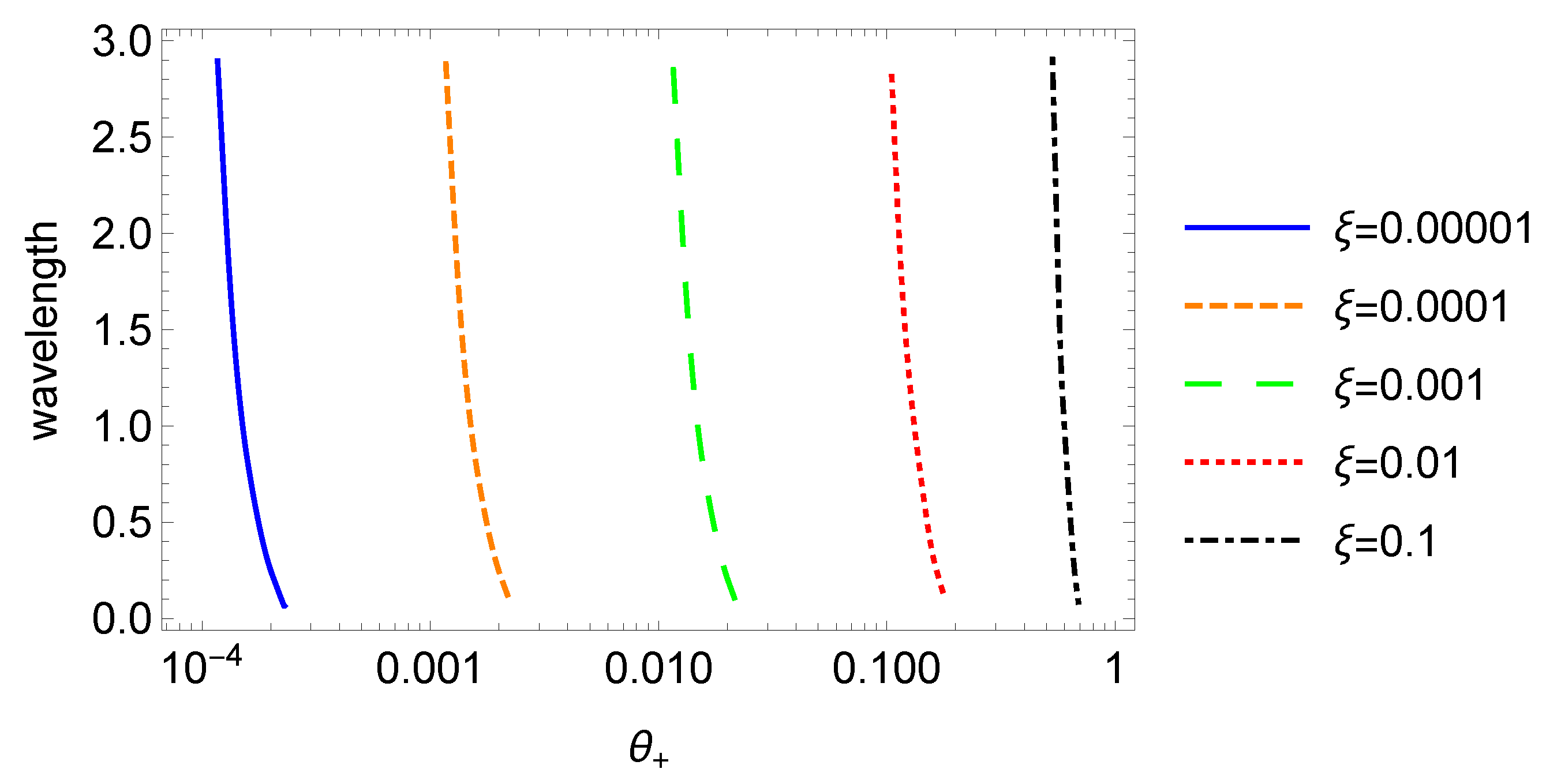
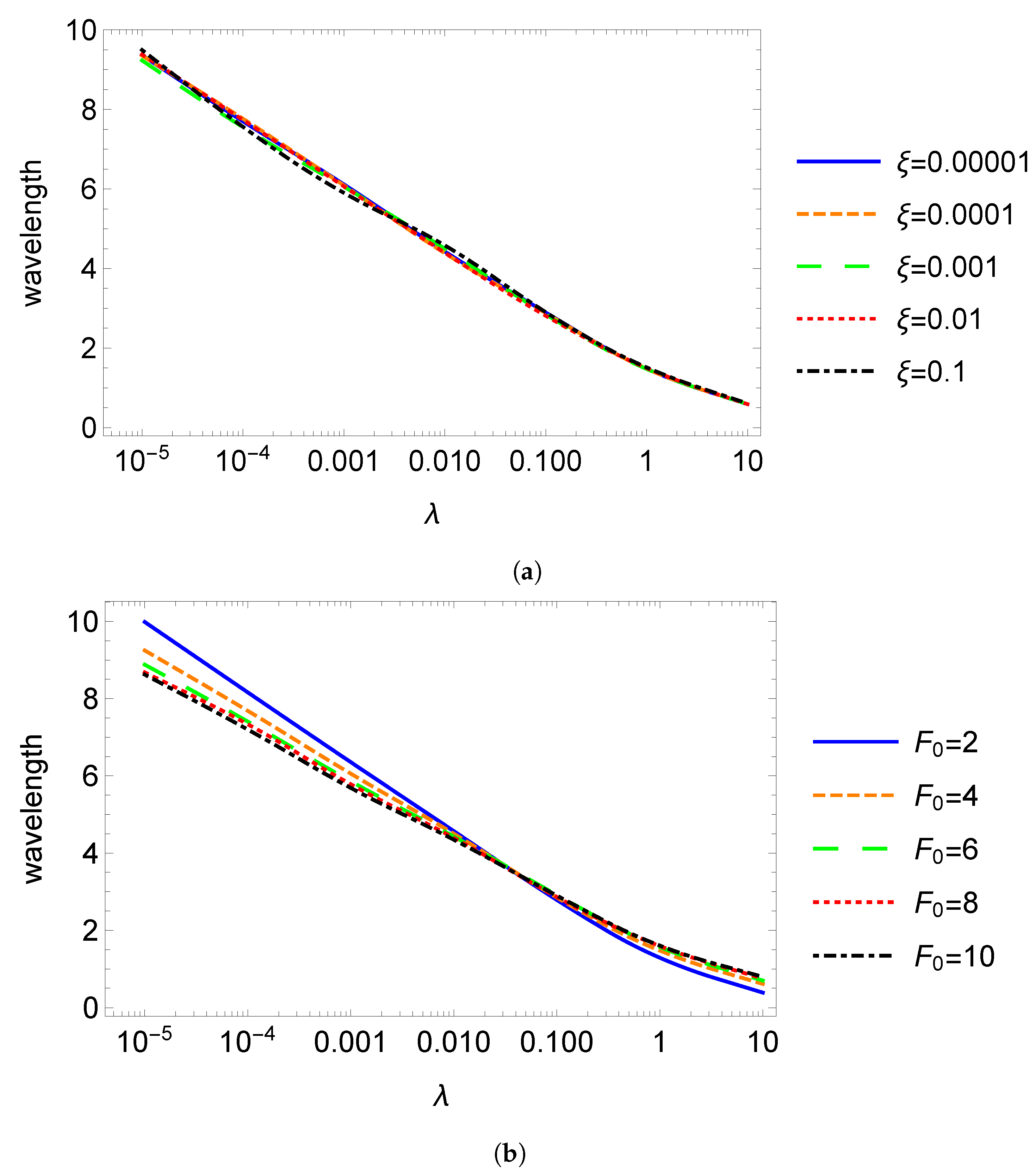
3.1. Calculation for Wavelength
3.2. The Relationships among Parameters
4. Discussion
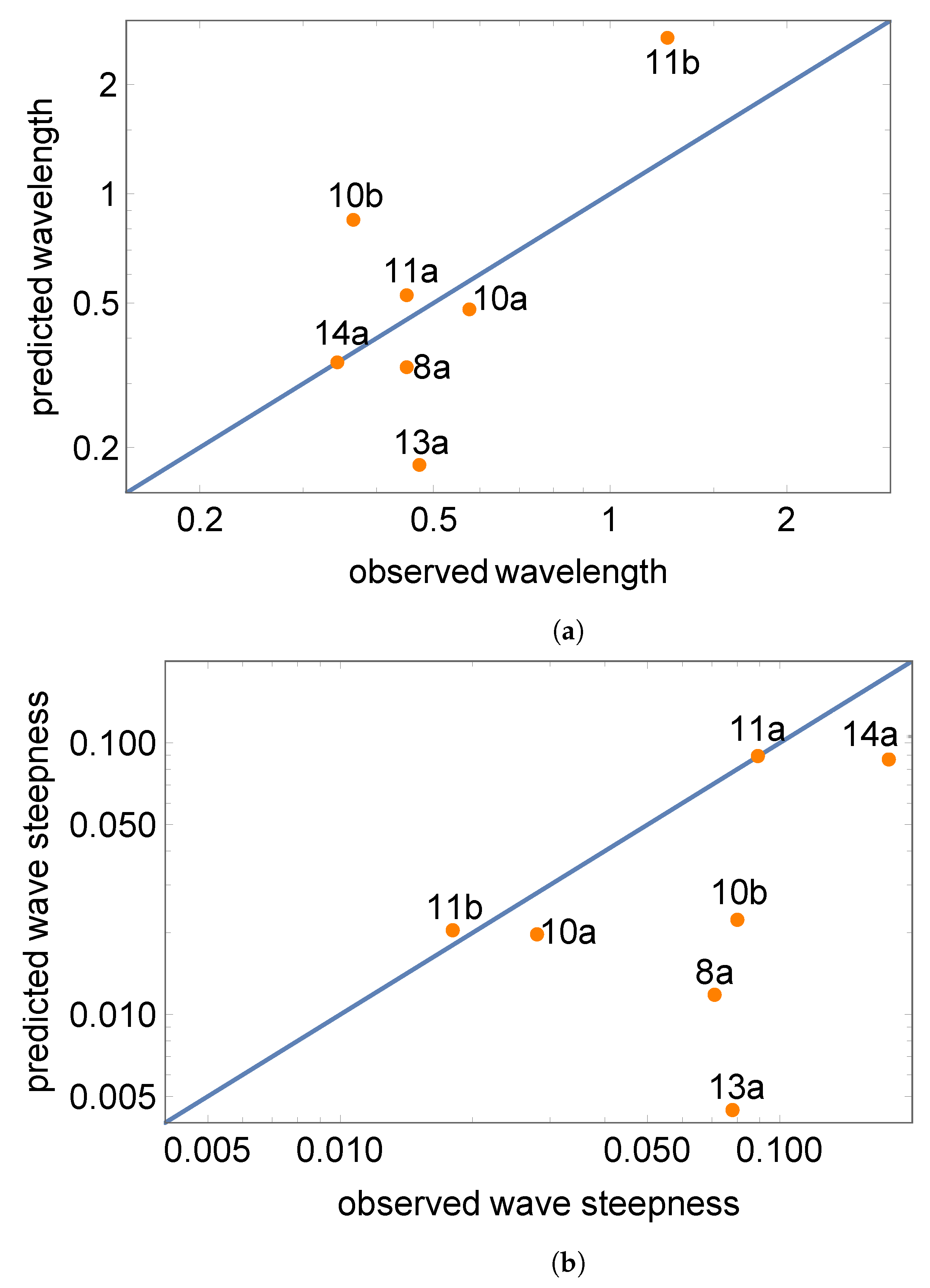
4.1. Comparison with Observed and Predicted Results
4.2. The Formation of Cyclic Steps
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notations
Appendix A. Derivations of Equation and Formula
Appendix A.1. Depth-Integrated Heat Transfer Equations of Flowing Water
Appendix A.2. The Calculation of the Drag Coefficient
References
- Carey, K.L.; Wis, M. Observed configuration and computed roughness of the underside of river ice, St. Croix River, Wisconsin. Geol. Surv. Res. 1966, 2, B192–B198. [Google Scholar]
- Gilpin, R.R.; Hirata, T.; Cheng, K.C. Wave formation and heat transfer at an ice-water interface in the presence of a turbulent flow. Fluid Mech. 1980, 99, 619–640. [Google Scholar] [CrossRef]
- Camporeale, C.; Ridolfi, L. Ice ripple formation at large Reynolds numbers. J. Fluid Mech. 2012, 694, 225–251. [Google Scholar] [CrossRef]
- Parker, G.; Izumi, N. Purely erosional cyclic and solitary steps created by flow over a cohesive bed. J. Fluid Mech. 2000, 419, 203–238. [Google Scholar] [CrossRef]
- Lang, J.; Winsemann, J. Lateral and vertical facies relationships of bedforms deposited by aggrading supercritical flows: From cyclic steps to humpback dunes. Sediment. Geol. 2013, 296, 36–54. [Google Scholar] [CrossRef]
- Fildani, A.; Normark, W.R.; Kostic, S.; Parker, G. Channel formation by flow stripping: Large-scale scour features along the Monterey East Channel and their relation to sediment waves. Sedimentology 2006, 53, 1265–1287. [Google Scholar] [CrossRef]
- Zhong, G.; Cartigny, M.J.; Kuang, Z.; Wang, L. Cyclic steps along the south Taiwan shoal and west Penghu submarine canyons on the northeastern continental slope of the South China Sea. GSA Bull. 2015, 127, 804–824. [Google Scholar] [CrossRef]
- Paull, C.K.; Talling, P.J.; Maier, K.L.; Parsons, D.; Xu, J.; Caress, D.W.; Gwiazda, R.; Lundsten, E.M.; Anderson, K.; Barry, J.P.; et al. Powerful turbidity currents driven by dense basal layers. Nat. Commun. 2018, 9, 4114. [Google Scholar] [CrossRef]
- Ventra, D.; Cartigny, M.J.; Bijkerk, J.F.; Acikalin, S. Supercritical-flow structures on a Late Carboniferous delta front: Sedimentologic and paleoclimatic significance. Geology 2015, 43, 731–734. [Google Scholar] [CrossRef]
- Fricke, A.T.; Sheets, B.A.; Nittrouer, C.A.; Allison, M.A.; Ogston, A.S. An examination of Froude-supercritical flows and cyclic steps on a subaqueous lacustrine delta, Lake Chelan, Washington, USA. J. Sediment. Res. 2015, 85, 754–767. [Google Scholar] [CrossRef]
- Casalbore, D.; Romagnoli, C.; Bosman, A.; Chiocci, F.L. Large-scale seafloor waveforms on the flanks of insular volcanoes (Aeolian Archipelago, Italy), with inferences about their origin. Mar. Geol. 2014, 355, 318–329. [Google Scholar] [CrossRef]
- Lowe, D.G.; Arnott, R. Composition and architecture of braided and sheetflood-dominated ephemeral fluvial strata in the Cambrian–Ordovician Potsdam Group: A case example of the morphodynamics of early Phanerozoic fluvial systems and climate change. J. Sediment. Res. 2016, 86, 587–612. [Google Scholar] [CrossRef]
- Wang, J.; Plink-Bjorklund, P. Stratigraphic complexity in fluvial fans: Lower Eocene Green River Formation, Uinta Basin, USA. Basin Res. 2019, 831, 892–919. [Google Scholar] [CrossRef]
- Karlstrom, L.; Zok, A.; Manga, M. Near-surface permeability in a supraglacial drainage basin on the Llewellyn Glacier, Juneau Icefield, British Columbia. Cryosphere 2014, 8.2, 537–546. [Google Scholar] [CrossRef]
- Spiga, A.; Smith, I. Katabatic jumps in the Martian northern polar regions. Icarus 2017, 308, 197–208. [Google Scholar] [CrossRef]
- Smith, I.B.; Holt, J.W.; Spiga, A.; Howard, A.D.; Parker, G. The title of the cited article. The spiral troughs of Mars as cyclic steps. J. Geophys. Res. Planets 2013, 118, 1835–1857. [Google Scholar] [CrossRef]
- Fahnestock, M.A.; Scambos, T.A.; Shuman, C.A.; Arthern, R.J.; Winebrenner, D.P.; Kwok, R. Snow megadune fields on the East Antarctic Plateau: Extreme atmosphere-ice interaction. Geophys. Res. Lett. 2000, 27, 3719–3722. [Google Scholar] [CrossRef]
- Frezzotti, M.; Gandolfi, S.; Urbini, S. Snow megadunes in Antarctica: Sedimentary structure and genesis. J. Geophys. Res. Atmos. 2002, 107, ACL-1. [Google Scholar] [CrossRef]
- Taki, K.; Parker, G. Transportational cyclic steps created by flow over an erodible bed. part 1. experiments. J. Hydraul. Res. 2005, 143, 488–501. [Google Scholar] [CrossRef]
- Sun, T.; Parker, G. Transportationalcyclicstepscreatedbyflowoveran erodible bed. part 2. theory and numerical simulation. J. Hydraul. Res. 2005, 43, 502–514. [Google Scholar] [CrossRef]
- Wu, Z.; Izumi, N. Transportational Cyclic Steps Created by Submarine Long-Runout Turbidity Currents. Geosciences 2022, 12, 263. [Google Scholar] [CrossRef]
- Yokokawa, M.; Izumi, N.; Naito, K.; Parker, G.; Yamada, T.; Greve, R. Cyclic steps on ice. J. Geophys. Res. Earth Surf. 2016, 121, 1023–1048. [Google Scholar] [CrossRef]
- Naito, K.; Izumi, N.; Yokokawa, M.; Yamada, T. Downstream migrating steps on ice. J. Jpn. Soc. Civ. Eng. Ser. B1 (Hydraul. Eng.) 2013, 69, I1123–I1128. [Google Scholar]
- Sumida, T.; Izumi, N.; Yokokawa, M.; Yamada, T. Boundary waves formed on the ice floor due to katabatic wind. J. Jpn. Soc. Civ. Eng. Ser. B1 (Hydraul. Eng.) 2016, 72, I739–I744. [Google Scholar]
- Komori, S.; Kurose, R.; Takagaki, N.; Ohtsubo, S.; Iwano, K.; Handa, K.; Shimada, S. Sensible and latent heat transfer across the air–water interface wind-driven turbulence. In Gas Transfer at Water Surfaces; Kyoto University: Kyoto, Japan, 2010; pp. 78–89. [Google Scholar]
- Shirasawa, K.; Ingram, R.G. Currents and turbulent fluxes under the first-year sea ice in Resolute Passage, Northwest Territories, Canada. J. Mar. Syst. 1997, 11.1–11.2, 21–32. [Google Scholar] [CrossRef]
- Hamblin, P.F.; Carmack, E.C. On the rate of heat transfer between a lake and an ice sheet. Cold Reg. Sci. Technol. 1990, 18, 173–182. [Google Scholar] [CrossRef]
- Li, N.; Tuo, Y.; Deng, Y.; Li, J.; Liang, R.; An, R. Heat transfer at ice-water interface under conditions of low flow velocities. J. Hydrodyn. 2016, 28, 603–609. [Google Scholar] [CrossRef]
| Run Number | s | (m/s) | ( m) | () | () | () | |||
|---|---|---|---|---|---|---|---|---|---|
| CSIM120908A | 0.0875 | 5.5 | 0.7 | 1.52 | 0.22 | 2.05 | 2.26 | 1.58 | 3.79 |
| CSIM120910A | 0.0875 | 9.3 | 1.9 | 2.42 | 0.29 | 1.45 | 9.54 | 4.02 | 1.49 |
| CSIM120910B | 0.0875 | 5.7 | 0.7 | 3.17 | 0.40 | 1.65 | 8.59 | 6.89 | 0.87 |
| CSIM120911A | 0.3640 | 5.5 | 0.5 | 4.31 | 0.62 | 2.12 | 2.69 | 3.06 | 1.96 |
| CSIM120911B | 0.0875 | 5.2 | 0.2 | 2.89 | 0.68 | 5.70 | 2.10 | 5.73 | 1.05 |
| CSIM120913A | 0.0875 | 5.3 | 2.2 | 1.11 | 0.17 | 2.29 | 5.82 | 0.84 | 7.10 |
| CSIM120914A | 0.3640 | 5.4 | 0.5 | 4.37 | 0.48 | 1.25 | 2.85 | 3.15 | 1.91 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Z.; Izumi, N. Cyclic Steps Created by Flowing Water on Ice Surface. Geosciences 2023, 13, 128. https://doi.org/10.3390/geosciences13050128
Wu Z, Izumi N. Cyclic Steps Created by Flowing Water on Ice Surface. Geosciences. 2023; 13(5):128. https://doi.org/10.3390/geosciences13050128
Chicago/Turabian StyleWu, Zhuyuan, and Norihiro Izumi. 2023. "Cyclic Steps Created by Flowing Water on Ice Surface" Geosciences 13, no. 5: 128. https://doi.org/10.3390/geosciences13050128
APA StyleWu, Z., & Izumi, N. (2023). Cyclic Steps Created by Flowing Water on Ice Surface. Geosciences, 13(5), 128. https://doi.org/10.3390/geosciences13050128








