Application of an Integrated 3D–2D Modeling Approach for Pillar Support Design in a Western US Underground Coal Mine
Abstract
1. Introduction
2. Site Description, Monitoring, and Panel-Scale FLAC3D Model Setup
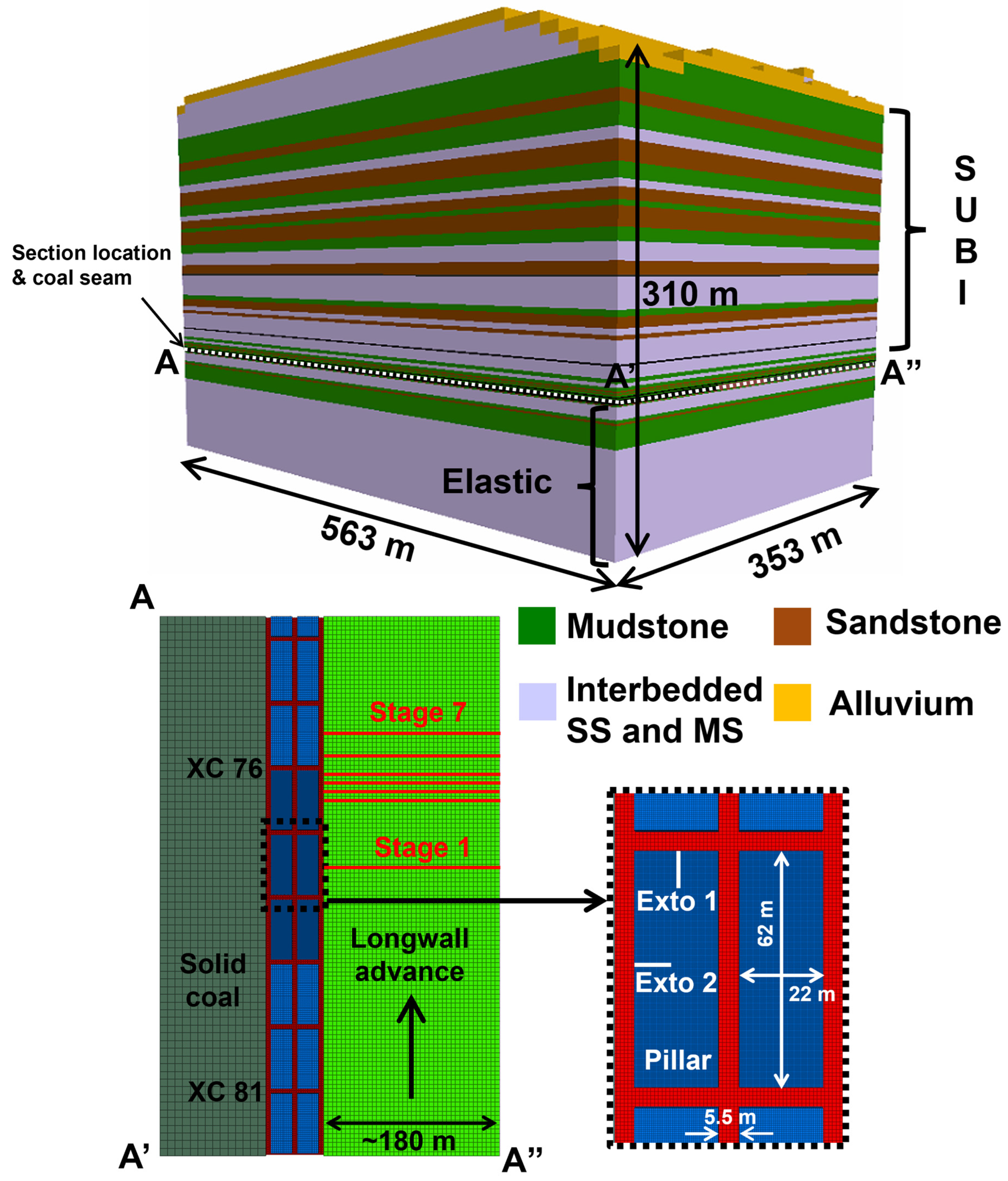
2.1. Description of the Site and Instrumentation
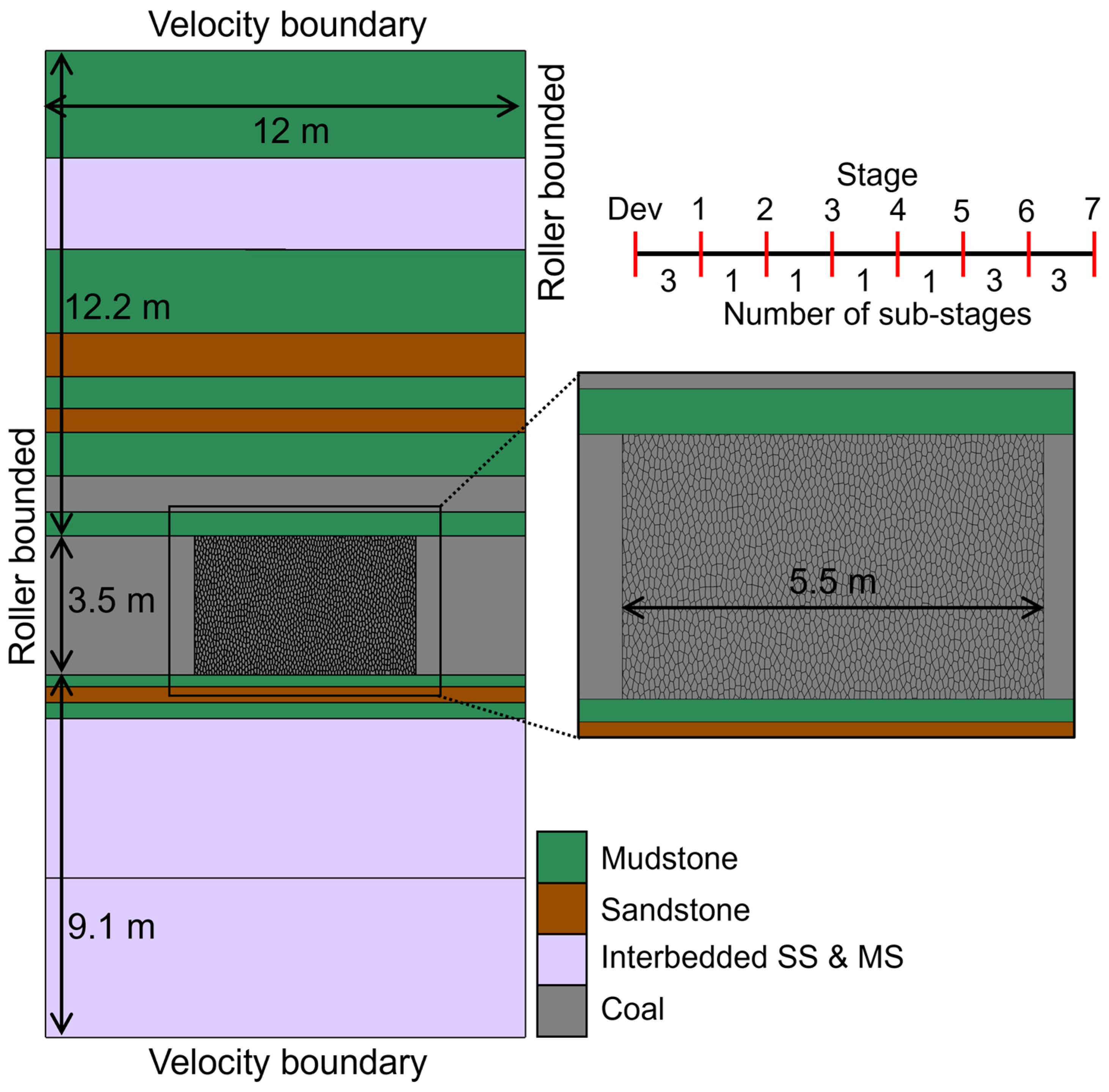
2.2. FLAC3D Model Setup
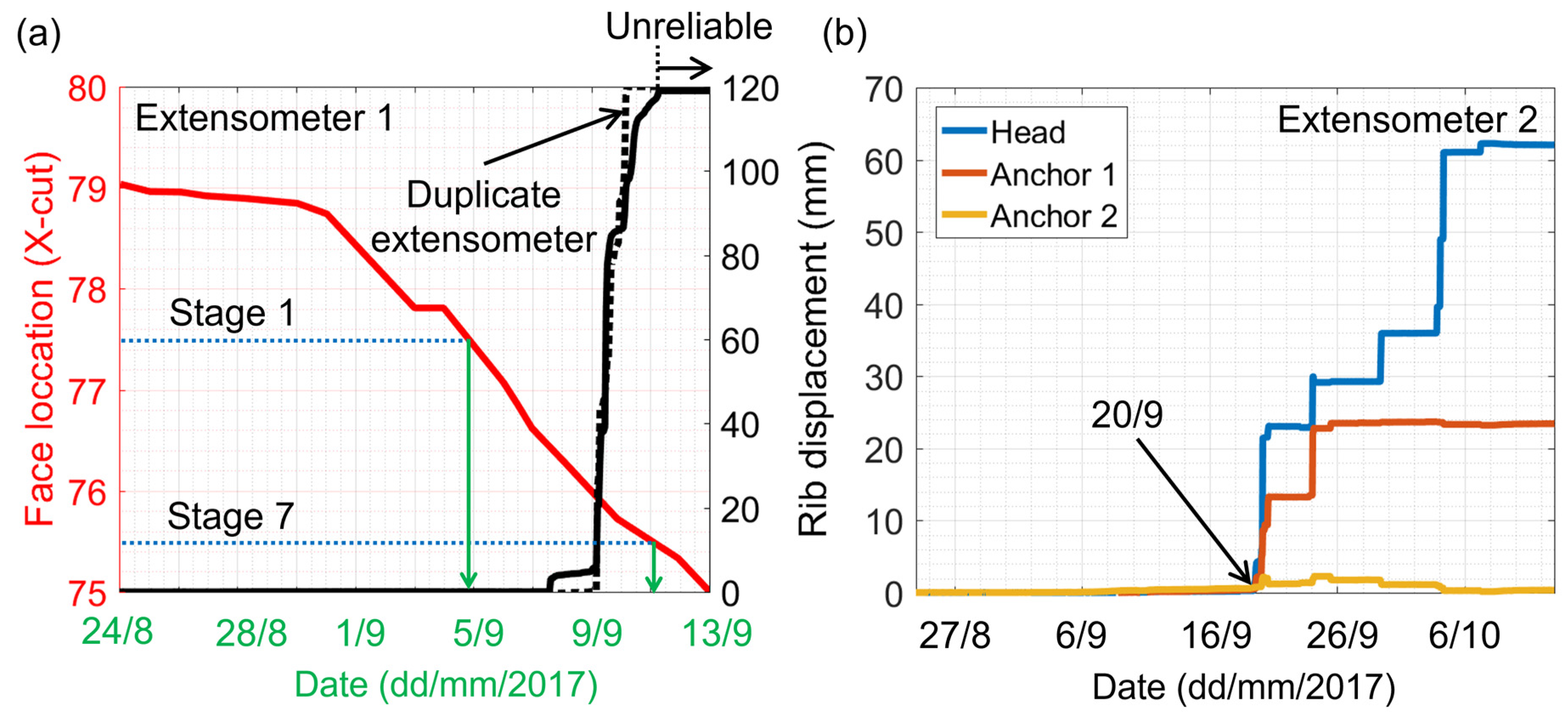
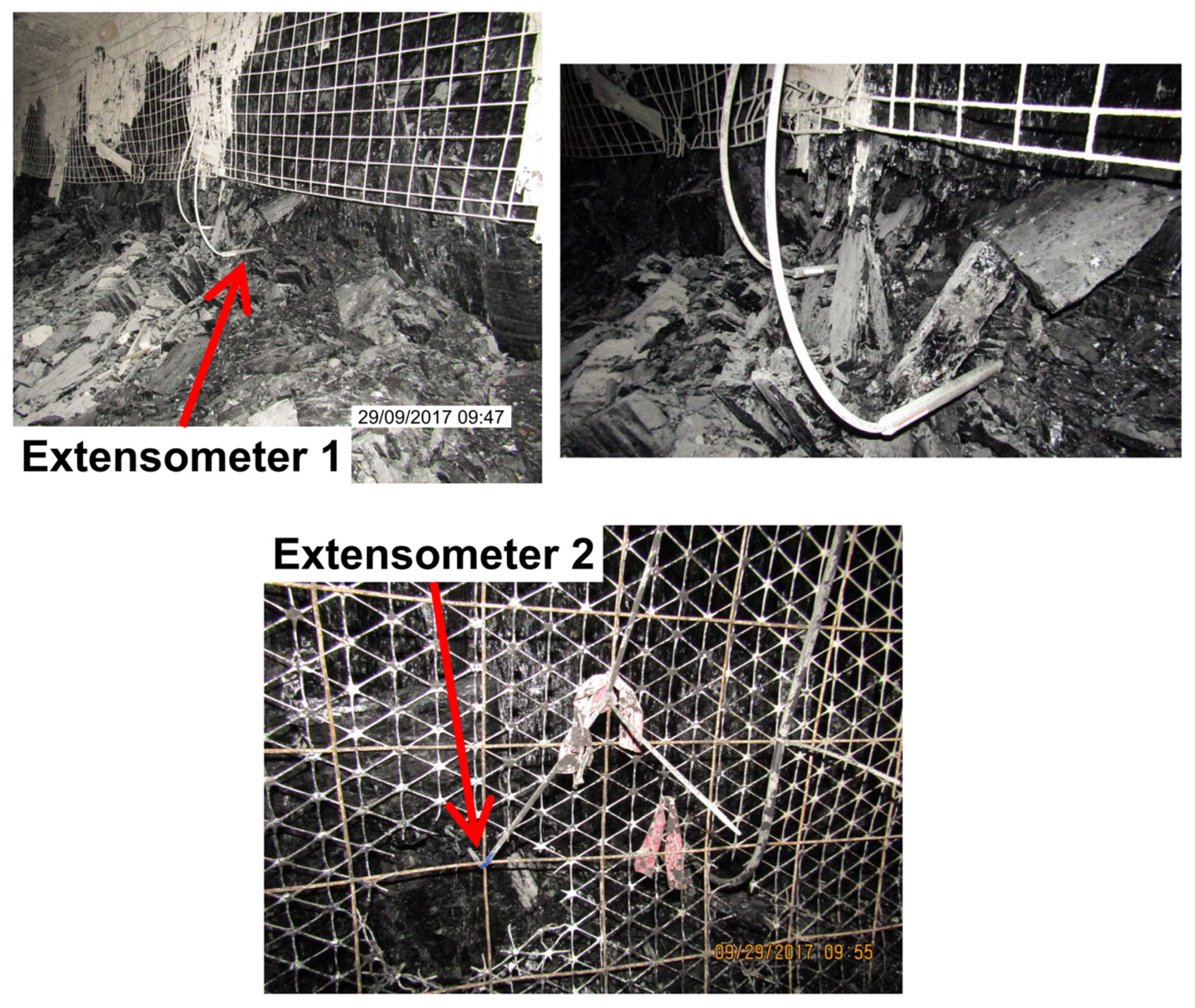
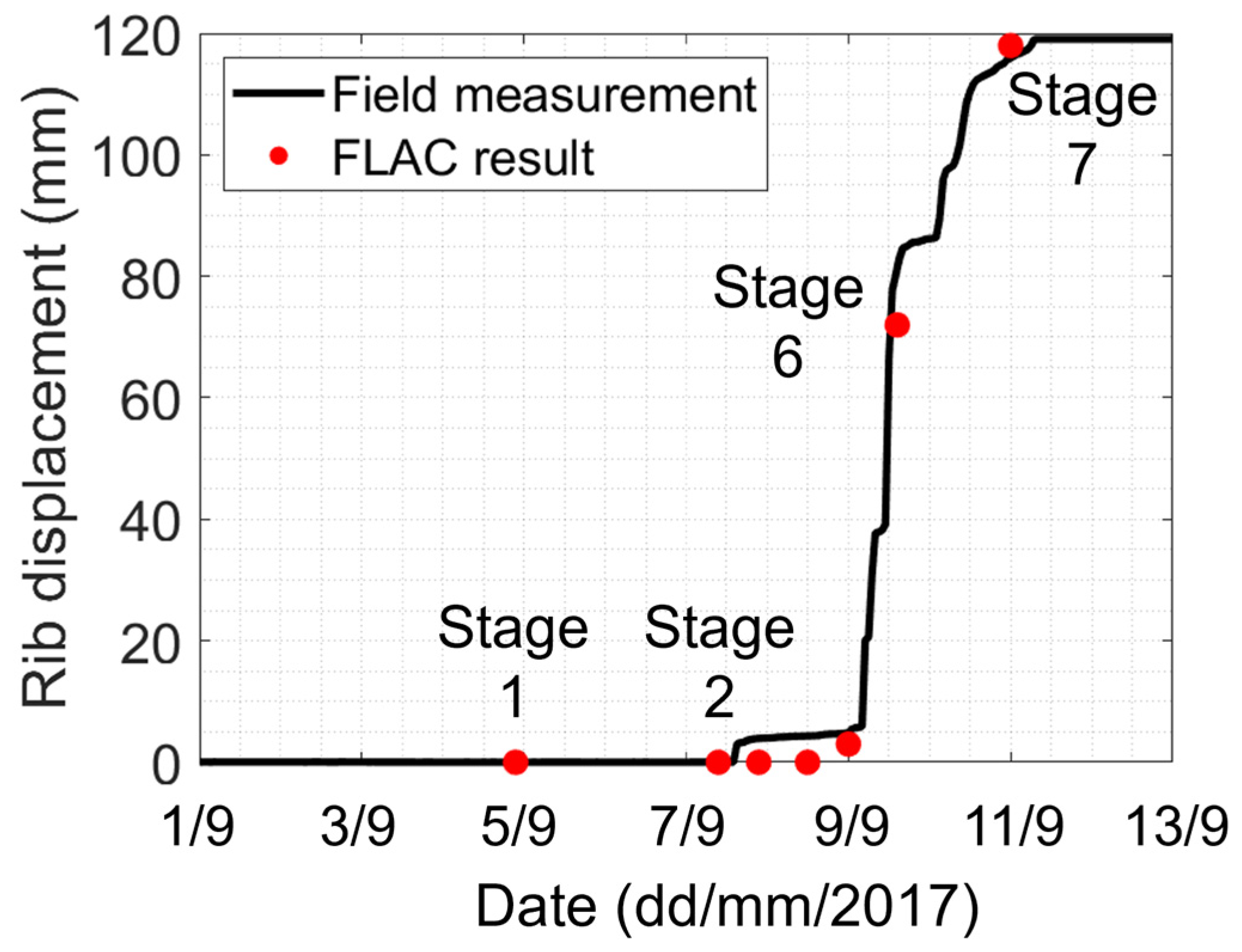
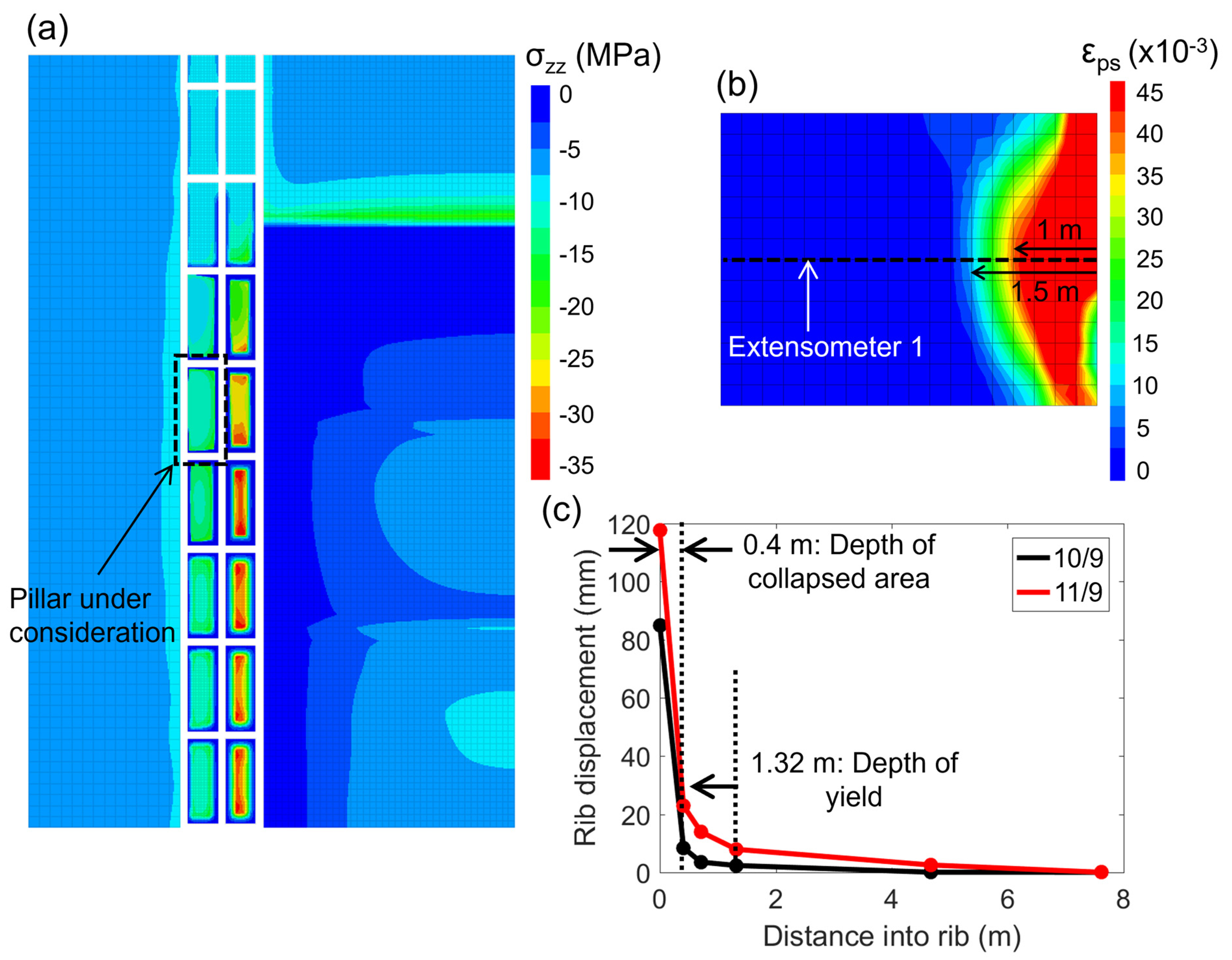
2.3. Extensometer Data and Model Considerations
3. FLAC3D Model Setup, Calibration, and Results
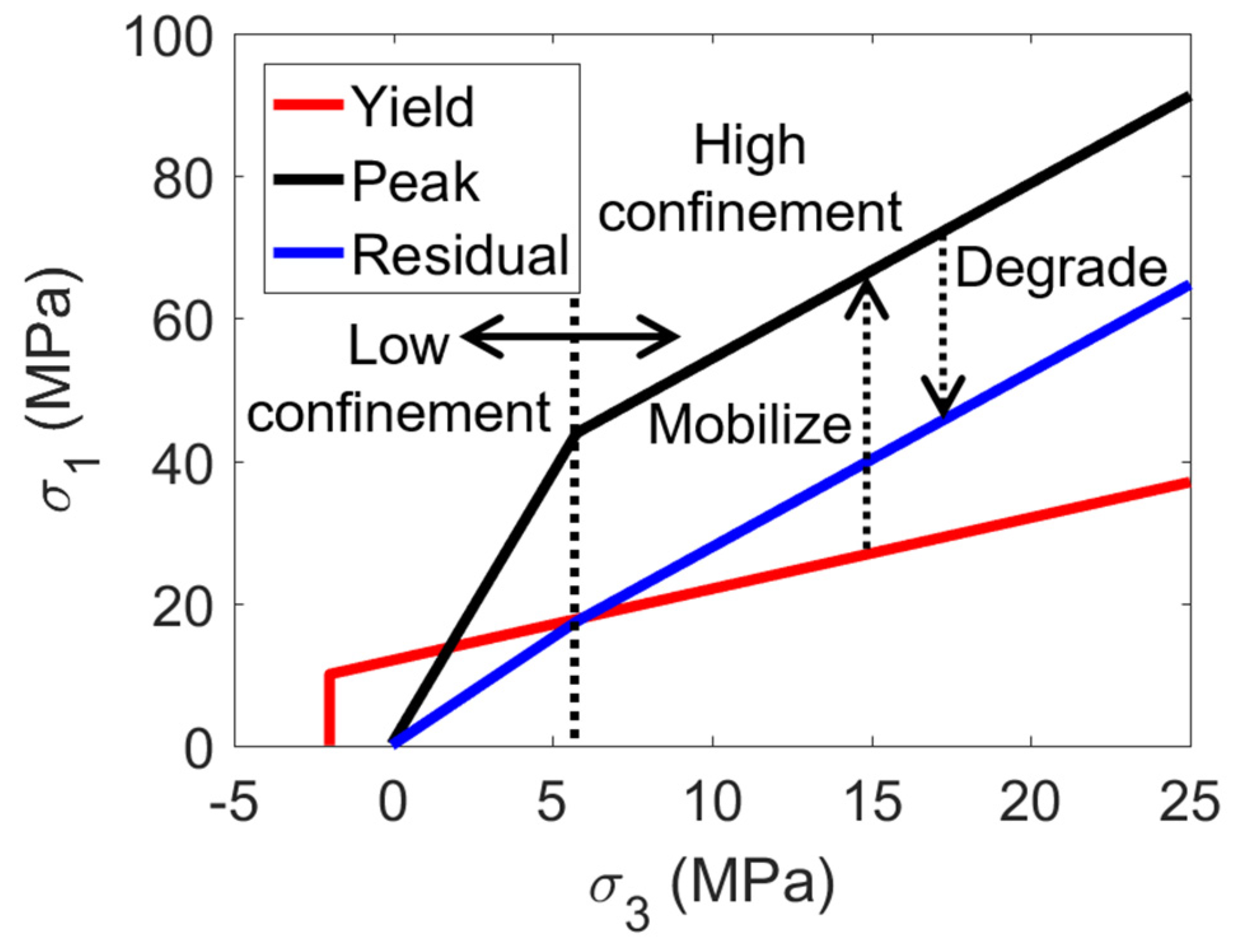
3.1. Progressive S-Shaped Criterion and Preliminary Coal Pillar Parameters
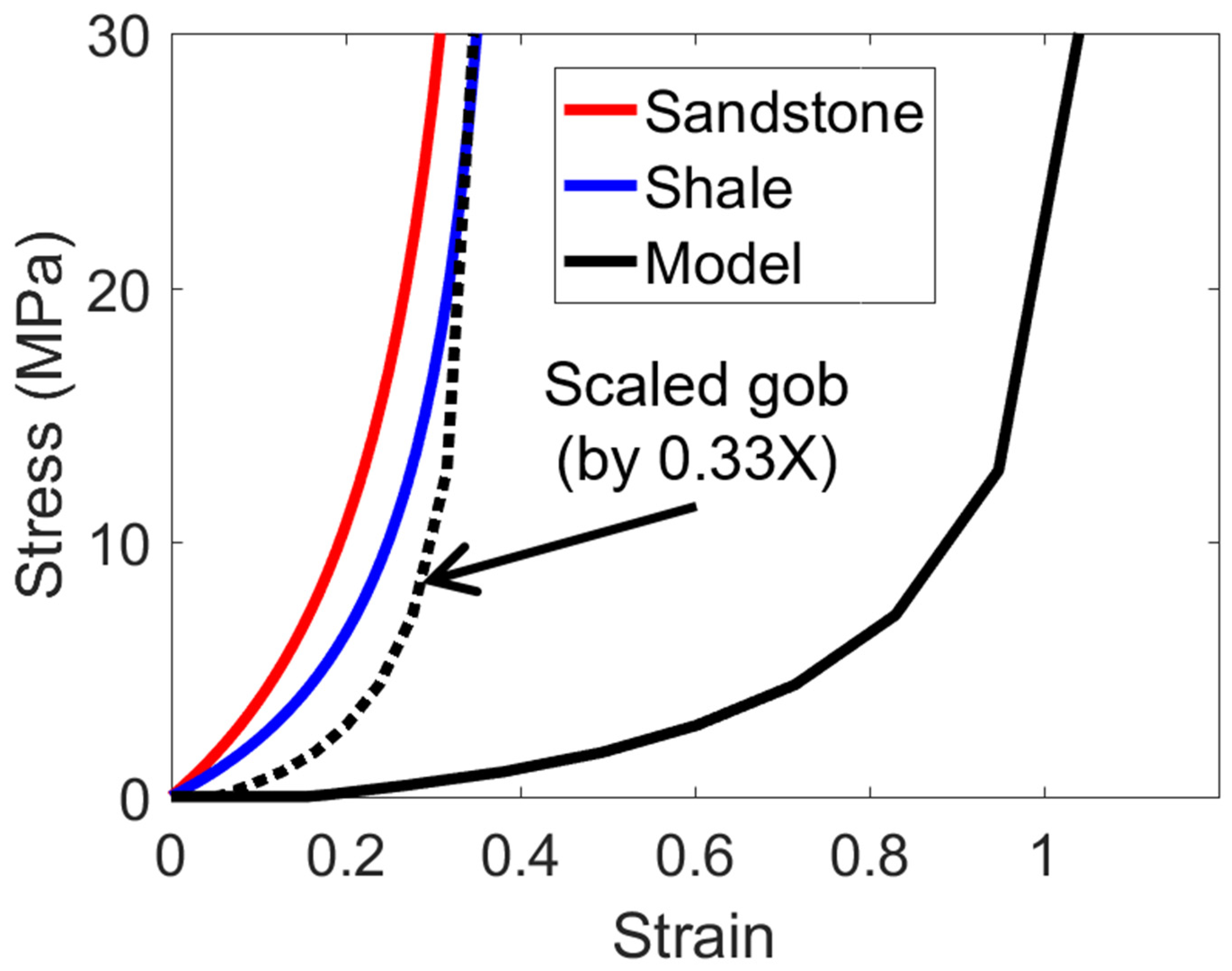
3.2. Gob and Overburden Parameters
3.3. Calibration of the Coal Parameters and Associated Results
4. The Integrated Modeling Approach—Setup and Results
4.1. Bonded Block Model (BBM) Setup for Mine A
- In situ stresses corresponding to those in the FLAC3D model at the instrumented location were applied to the entire BBM, and the model was brought to mechanical equilibrium. Next, the entry was excavated, and the unbalanced forces were relaxed in 10 stages using UDEC’s built-in ZONK function. This gradual relaxation is necessary in order to avoid unrealistic yielding/fracturing along the entry due to a sudden increase in the unbalanced forces in the model.
- In order to apply the same load path to the BBM as was experienced by the calibrated FLAC3D pillar section, a strain-controlled loading approach was adopted. In particular, the vertical displacements at gridpoints corresponding to the top and bottom boundaries of the BBM were recorded for each of the seven stages, segmented into multiple sub-stages, and then applied to the BBM via a velocity boundary condition. The segmentation was performed to avoid applying a large displacement to the BBM in one step, which could result in unrealistic fracturing. The number of sub-stages was based on the displacement difference between two consecutive stages (when the difference was large, a larger number of sub-stages was used). To segment a stage, the difference in displacement from the previous stage to the stage of interest was split equally into the required number of sub-stages. Ultimately, 13 total sub-stages were considered in the final BBM such that the average vertical displacement increase along the loading boundaries did not exceed 1 mm between any two sub-stages. A roller boundary was assigned to the vertical edges of the model in order to simplify the loading procedure.
- Instead of applying the displacement differences as a velocity in the BBM, it was scaled up by 10 times so that the model would need to be stepped for only 1/10th of a second of model time (~30,000 solution steps) instead of 1 s to apply the appropriate displacements. Once the model was stepped by 0.1 s, the boundaries were fixed, and then stepping continued until mechanical equilibrium was attained. This loading mechanism was repeated for all seven stages (including the 13 sub-stages).
4.2. Model Calibration and Results of Unsupported BBM
4.3. Effect of Rockbolts on Rib Behavior
4.3.1. Effect of Support Density
4.3.2. Effect of Rockbolt Position
5. Conclusions
- The FLAC3D model could reproduce the rib deformations very well but failed to match the depth of fracturing.
- The BBM, in contrast, exhibited a close correspondence with both the deformation and the depth of fracturing. This suggests that a perfect replication of the observed depth of yield is not necessary to obtain a reasonable approximation of the boundary conditions to be applied to the BBM.
- The inclusion of one rockbolt at the rib mid-height reduced the fracturing and bulking significantly, while the use of two rockbolts stabilized the rib to an extent where only distributed fracturing occurred in the central portion of the rib. The behaviors of the rockbolts were generally interpreted to be realistic based on a comparison against the literature.
- Some other interesting behaviors were noted during the analysis of the rockbolt position, and it was concluded that the specific rockbolt location can affect the extent of damage (making the damage profile more anisotropic across the rib height) and magnitude of bulking along the rib.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Horino, F.G.; Duvall, W.I.; Brady, B.T. The Use of Rock Bolts or Wire Rope to Increase the Strength of Fractured Model Pillars; U.S. Bureau of Mines Report of Investigation, RI-7568; US Department of the Interior, Bureau of Mines: Washington, DC, USA, 1971; pp. 1–24.
- Guana, M. Angle bolts control rib side at No. 4 Mine, Brockwood, Alabama. In Proceedings of the SME-AIME Fall Meeting and Exhibit, Salt Lake City, UT, USA, 19–21 October 1983. Preprint number 83–310. [Google Scholar]
- Dolinar, D.R.; Tadolini, S.C. Entry Stabilization Using Rib Bolting Procedures; U.S. Bureau of Mines Report of Investigation, RI-9366; US Department of the Interior, Bureau of Mines: Washington, DC, USA, 1991; pp. 1–13.
- Smith, W.C. Rib Stability: Practical Considerations to Optimize Rib Design; US Department of the Interior, Bureau of Mines: Washington, DC, USA, 1992. [Google Scholar]
- Colwell, M.; Mark, C. Analysis and design of rib support (ADRS)—A rib support design methodology for Australian collieries. In Proceedings of the 24th International Conference on Ground Control in Mining, Morgantown, WV, USA, 2–4 August 2005; Volume 24, pp. 12–22. [Google Scholar]
- Colwell, M.G. A Study of the Mechanics of Coal Mine Rib Deformation and Rib Support as a Basis for Engineering Design. Ph.D. Thesis, University of Queensland, Gatton, Australia, 2006. [Google Scholar]
- Guana, M.; Mark, C. Protecting underground coal miners from rib falls. In Proceedings of the 30th International Conference on Ground Control in Mining, Morgantown, WV, USA, 26–28 July 2011; Volume 30, pp. 126–134. [Google Scholar]
- Mohamed, K.M.; Tulu, I.B.; Klemetti, T. Numerical simulation of deformation and failure process of coal-mass. In Proceedings of the 49th US Rock Mechanics/Geomechanics Symposium, American Rock Mechanics Association, San Francisco, CA, USA, 29 June–1 July 2015. Paper No. 363. [Google Scholar]
- Mohamed, K.M.; Murphy, M.M.; Lawson, H.E.; Klemetti, T. Analysis of the current rib support practices and techniques in U.S. coal mines. Int. J. Min. Sci. Technol. 2016, 26, 77–87. [Google Scholar] [CrossRef]
- Zhang, P.; Mohamed, K.M.; Trackemas, J. Coal rib failure and support in longwall gate entries. In Proceedings of the 51th US Rock Mechanics/Geomechanics Symposium, San Francisco, CA, USA, 25–28 June 2017. Paper No. 886. [Google Scholar]
- Sears, M.M.; Rusnak, J.; Van Dyke, M.; Rashed, G.; Mohamed, K.M.; Sloan, M. Coal rib response during bench mining: A case study. Int. J. Min. Sci. Technol. 2018, 28, 107–113. [Google Scholar] [CrossRef]
- Slaker, B.; Gangrade, V.; Murphy, M.M.; Winfield, J. Seismic data interpretation of a development pillar at a steeply dipping underground limestone mine. In Proceedings of the 52nd US Rock Mechanics/Geomechanics Symposium, Seattle, WA, USA, 17–20 June 2018. Paper No. 194. [Google Scholar]
- Slaker, B.; Mohamed, K.M. A practical application of photogrammetry to performing rib characterization measurements in an underground coal mine using a DSLR camera. Int. J. Min. Sci. Technol. 2017, 27, 83–90. [Google Scholar] [CrossRef]
- MSHA. Mining Industry Accident, Injuries, Employment and Production Statistics and Reports. 1 March 2019. Available online: https://arlweb.msha.gov/ACCINJ/accinj.htm (accessed on 15 May 2019).
- Gao, Y.; Liu, D.; Zhang, X.; He, M. Analysis and optimization of entry stability in underground longwall mining. Sustainability 2017, 9, 2079. [Google Scholar] [CrossRef]
- Shabanimashcool, M.; Li, C.C. A numerical study of stress changes in barrier pillars and a border area in a longwall coal mine. Int. J. Coal Geol. 2013, 106, 39–47. [Google Scholar] [CrossRef]
- Shabanimashcool, M.; Li, C.C. Numerical modelling of longwall mining and stability analysis of the gates in a coal mine. Int. J. Rock Mech. Min. Sci. 2012, 51, 24–34. [Google Scholar] [CrossRef]
- Zhang, G.; Liang, S.; Tan, Y.; Xie, F.; Chen, S.; Jia, H. Numerical modeling for longwall pillar design: A case study from a typical longwall panel in China. J. Geophys. Eng. 2015, 15, 121–134. [Google Scholar] [CrossRef]
- Basarir, H.; Oge, I.F.; Aydin, O. Prediction of the stresses around main and tail gates during top coal caving by 3D numerical analysis. Int. J. Rock Mech. Min. Sci. 2015, 76, 88–97. [Google Scholar] [CrossRef]
- Esterhuizen, E.; Mark, C.; Murphy, M.M. Numerical model calibration for simulation coal pillars, gob and overburden response. In Proceedings of the 29th International Conference on Ground Control in Mining, Morgantown, WV, USA, 27–29 July 2010; pp. 46–57. [Google Scholar]
- Sinha, S.; Walton, G. A progressive S-shaped yield criterion and its application to the study of rock pillar behavior. Int. J. Rock Mech. Min. Sci. 2018, 105, 98–109. [Google Scholar] [CrossRef]
- Sinha, S.; Walton, G. Modeling behaviors of a coal pillar rib using the progressive S-shaped yield criterion. J. Rock Mech. Geotech. Eng. 2020, 12, 484–492. [Google Scholar] [CrossRef]
- Lorig, L.J.; Varona, P. Guidelines for numerical modelling of rock support for mines. In Proceedings of the 7th International Symposium on Ground Support in Mining and Underground Construction, Australian Centre for Geomechanics, Perth, Australia, 13–15 May 2013; pp. 81–105. [Google Scholar]
- Bahrani, N.; Hadjigeorgiou, J. Influence of stope excavation of drift convergence and support behavior: Insights from 3D continuum and discontinuum models. Rock Mech. Rock Eng. 2018, 51, 2395–2413. [Google Scholar] [CrossRef]
- Renani, H.R.; Martin, C.D.; Hudson, R. Back analysis of rock mass displacements around a deep shaft using two- and three- dimensional continuum modeling. Rock Mech. Rock Eng. 2016, 49, 1313–1327. [Google Scholar] [CrossRef]
- Sinha, S.; Walton, G. Understanding continuum and discontinuum models of rock-support interaction for excavations undergoing stress-induced spalling. Int. J. Rock Mech. Min. Sci. 2019, 123, 104089. [Google Scholar] [CrossRef]
- Sinha, S.; Walton, G. Modeling the behavior of a coal pillar rib using bonded block models with emphasis on ground-support interaction. Int. J. Rock Mech. Min. Sci. 2021, 148, 104965. [Google Scholar] [CrossRef]
- Sunkpal, M.; Sherizadeh, T. Exploring the Deformation Mechanics of Coal Ribs Using the Distinct Element Modeling Approach. Rock Mech. Rock Eng. 2022, 55, 2879–2898. [Google Scholar] [CrossRef]
- Sunkpal, M.; Sherizadeh, T.; Guner, D. Evaluating the Effect of Overburden Depth, Mining Height, and Support Density on Coal Rib Damage Using DEM Modeling. Geosciences 2023, 13, 77. [Google Scholar] [CrossRef]
- Sunkpal, M.; Sherizadeh, T.; Guner, D. Quantifying coal rib stability and support requirements using bonded block modeling and reliability analysis based on the random set theory. Int. J. Rock Mech. Min. Sci. 2023, 163, 105332. [Google Scholar] [CrossRef]
- Bai, Q.; Tu, S.; Zhang, C.; Zhu, D. Discrete element modeling of progressive failure of wide coal roadway from water-rich roofs. Int. J. Coal Geol. 2016, 167, 215–229. [Google Scholar] [CrossRef]
- Farahmand, K.; Vazaios, I.; Diederichs, M.S.; Vlachopoulos, N. Investigating the scale-dependency of the geometrical and mechanical properties of a moderately jointed rock using a synthetic rock mass (SRM) approach. Comput. Geotech. 2018, 95, 162–179. [Google Scholar] [CrossRef]
- Yang, X.; Wang, E.; Wang, Y.; Gao, Y.; Wang, P. A study of the large deformation mechanism and control techniques for deep soft rock roadways. Sustainability 2018, 10, 1100. [Google Scholar] [CrossRef]
- Garza-Cruz, T.; Bouzeran, L.; Pierce, M.; Jalbout, A.; Ruest, M. Evaluation of ground support design at Eleonore Mine via Bonded Block Modelling. In Proceedings of the 9th International Symposium on Ground Support in Mining and Underground Construction, Perth, Australia, 23–25 October 2019; pp. 41–356. [Google Scholar]
- Bai, Q.; Tu, S. Numerical observations of the failure of a laminated and jointed roof and the effective of different support schemes: A case study. Environ. Earth Sci. 2020, 79, 1–8. [Google Scholar] [CrossRef]
- Garza-Cruz, T.V.; Pierce, M.; Board, M. Effect of shear stresses on pillar stability—A back-analysis of the Troy Mine experience to forward predict pillar performance at Montanore. In Proceedings of the 52nd US Rock Mechanics/Geomechanics Symposium, Seattle, WA, USA, 17–20 June 2018. Paper No. 1158. [Google Scholar]
- Sinha, S.; Walton, G. Integration of three-dimensional continuum model and two-dimensional bonded block model for studying the damage process in a granite pillar at the Creighton Mine, Sudbury, Canada. J. Rock Mech. Geotech. Eng. 2021, 13, 275–288. [Google Scholar] [CrossRef]
- Potyondy, D.O.; Cundall, P.A. A bonded-particle model for rock. Int. J. Rock Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Cai, M.; Kaiser, P.K.; Morioka, H.; Minami, M.; Maejima, T.; Tasaka, Y.; Kurose, H. FLAC/PFC coupled numerical simulation of AE in large-scale underground excavations. Int. J. Rock Mech. Min. Sci. 2007, 44, 550–564. [Google Scholar] [CrossRef]
- Saiang, D. Stability analysis of the blast-induced damage zone by continuum and coupled continuum–discontinuum methods. Eng. Geol. 2010, 116, 1–11. [Google Scholar] [CrossRef]
- Zhang, F.; Dontsov, E.; Mack, M. Fully coupled simulation of a hydraulic fracture interacting with natural fractures with a hybrid discrete-continuum method. Int. J. Numer. Anal. Methods Geomech. 2017, 41, 1430–1452. [Google Scholar] [CrossRef]
- Babcock, C.O. Equations for the analysis of Borehole Pressure Cell data. In Proceedings of the 27th U.S. Symposium on Rock Mechanics (USRMS), Tuscaloosa, Alabama, 23–25 June 1986. [Google Scholar]
- Westman, E.; Luxbacher, K.; Schafrik, S. Passive seismic tomography for three-dimensional time-lapse imaging of mining-induced rock mass changes. Lead. Edge 2012, 31, 338–345. [Google Scholar] [CrossRef]
- Ezersky, M.; Eppelbaum, L. Geophysical monitoring of underground constructions and its theoretical basis. Int. J. Georesources Environ. 2017, 3, 56–72. [Google Scholar] [CrossRef][Green Version]
- Barczak, T.M. Longwall tailgates: The technology for roof support has improved but optimization is still not there. In Proceedings of the Longwall USA, International Exhibition and Conference, Pittsburgh, PA, USA, 3–5 June 2003; pp. 105–130. [Google Scholar]
- Peng, S.S.; Chiang, H.S. Longwall Mining; Wiley: New York, NY, USA, 1984. [Google Scholar]
- Itasca. FLAC3D Version 5.0: Theory and Background; Itasca Consulting Group: Minneapolis, MN, USA, 2016. [Google Scholar]
- Tulu, I.B.; Esterhuizen, G.S.; Mohamed, K.M.; Klemetti, T.M. Verification of a calibrated longwall model with field measurements. In Proceedings of the 51st US Rock Mechanics/Geomechanics Symposium, San Francisco, CA, USA, 25–28 June 2017. Paper No. 238. [Google Scholar]
- Li, W.; Bai, J.; Peng, S.; Wang, X.; Xu, Y. Numerical modeling for yield pillar design: A case study. Rock Mech. Rock Eng. 2015, 48, 305–318. [Google Scholar] [CrossRef]
- Heidbach, O.; Rajabi, M.; Reiter, K.; Ziegler, M. World Stress Map 2016. GFZ Data Service. 2016. Available online: https://dataservices.gfz-potsdam.de/wsm/showshort.php?id=escidoc:1680899 (accessed on 1 August 2023).
- Mark, C. Horizontal stress and its effects on longwall ground control. Min. Eng. 1991, 43, 1356–1360. [Google Scholar]
- Diederichs, M.S. The 2003 Canadian Geotechnical Colloquium: Mechanistic interpretation and practical application of damage and spalling prediction criteria for deep tunnelling. Can. Geotech. J. 2007, 44, 1082–1116. [Google Scholar] [CrossRef]
- Sinha, S.; Walton, G.; Kim, B.H. Difficulties in determining the Crack Initiation (CI) thresholds for three different rock types. In Proceedings of the 54th US Rock Mechanics/Geomechanics Symposium, Golden, CO, USA, 28 June–1 July 2020. Paper No. 1074. [Google Scholar]
- Mark, C.; Iannacchione, A.T. Coal pillar mechanics: Theoretical models and field measurements compared. In Proceedings of the Workshop on Coal Pillar Mechanics and Design, Pittsburgh, PA, USA, 7 June 1992. [Google Scholar]
- Das, M.N. Influence of width/height ratio on post-failure behaviour of coal. Int. J. Min. Geol. Eng. 1986, 4, 79–87. [Google Scholar] [CrossRef]
- Dolinar, D.R.; Esterhuizen, G.S. Evaluation of the effect of length on the strength of slender pillars in limestone mines using numerical modeling. In Proceedings of the 26th International Conference on Ground Control in Mining, Morgantown, WV, USA, 31 July–2 August 2007; pp. 304–313. [Google Scholar]
- Sinha, S.; Walton, G. Numerical analyses of pillar behaviors with variation in yield criterion, dilatancy, rock heterogeneity and length to width ratio. J. Rock Mech. Geotech. Eng. 2019, 11, 46–60. [Google Scholar] [CrossRef]
- Pappas, D.M.; Mark, C. Behavior of Simulated Gob Material; U.S. Bureau of Mines: Pittsburgh, PA, USA, 1993; RI 9458. [Google Scholar]
- Abbasi, B.; Chugh, Y.P.; Gurley, H. An analysis of the possible fault displacements associated with a retreating longwall face in Illinois. In Proceedings of the 48th U.S. Rock Mechanics/Geomechanics Symposium, Minneapolis, MN, USA, 1–4 June 2014. Paper No. 7632. [Google Scholar]
- Peng, S.S. Longwall Mining; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Su, D.W.H. Finite Element Modeling of Subsidence induced by underground coal mining: The influence of material nonlinearity and shearing along existing planes of weakness. In Proceedings of the 10th International Conference on Ground Control in Mining, Morgantown, WV, USA, 10–12 June 1991; pp. 287–300. [Google Scholar]
- Vermeer, P.A.; De Borst, R. Non-associated plasticity for soils, concrete and rock. Heron 1984, 29. [Google Scholar] [CrossRef]
- Zhao, X.G.; Cai, M.; Cai, M. Considerations of rock dilation on modeling failure and deformation of hard rocks—A case study of the mine-by test tunnel in Canada. J. Rock Mech. Geotech. Eng. 2010, 2, 338–349. [Google Scholar]
- Corkum, A.G.; Lorig, L.J.; DeGagne, D.O. Continuum representation of brittle rock failure bulking-induced displacements around tunnels. In Proceedings of the 46th US Rock Mechanics/ Geomechanics Symposium, Chicago, IL, USA, 24–27 June 2012. Paper No. 176. [Google Scholar]
- Klemetti, T.M.; Van Dyke, M.A.; Compton, C.S.; Tulu, I.B.; Tuncay, D.; Wickline, J. Longwall gateroad yield pillar response and model verification–A case study. In Proceedings of the 53rd US Rock Mechanics/Geomechanics Symposium, New York, NY, USA, 23–26 June 2019. Paper No. 1553. [Google Scholar]
- Feng, G.; Wang, P.; Chugh, Y.P. Stability of gate roads next to an irregular yield pillar: A case study. Rock Mech. Rock Eng. 2019, 52, 2741–2760. [Google Scholar] [CrossRef]
- Basarir, H.; Sun, Y.; Li, G. Gateway stability analysis by global-local modeling approach. Int. J. Rock Mech. Min. Sci. 2019, 113, 31–40. [Google Scholar] [CrossRef]
- Klemetti, T.M.; Van Dyke, M.A.; Tulu, I.B.; Tuncay, D. A case study of the stability of a non-typical bleeder entry system at a US longwall mine. Int. J. Min. Sci. Technol. 2020, 30, 25–31. [Google Scholar] [CrossRef]
- Kim, B. (National Institute for Occupational Safety and Health, Spokane, Washington, United States). Personal Communication, 2020.
- Bouzeran, L.; Furtney, J.; Pierce, M.; Hazzard, J.; Lemos, J.V. Simulation of ground support performance in highly fractured and bulked rock masses with advanced 3DEC bolt model. In Proceedings of the Eighth International Conference on Deep and High Stress Mining, Perth, Australia, 28–30 March 2017; pp. 667–680. [Google Scholar]
- Sinha, S. Advancing Continuum and Discontinuum Models of Brittle Rock Damage and Rock-Support Interaction. Ph.D. Thesis, Colorado School of Mines, Golden, CO, USA, 2020. [Google Scholar]
- Galvin, J.M. Ground Engineering-Principles and Practices for Underground Coal Mining; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Gao, F.; Stead, D.; Kang, H. Simulation of roof shear failure in coal mine roadways using an innovative UDEC Trigon approach. Comput. Geotech. 2014, 61, 33–41. [Google Scholar] [CrossRef]
- Gao, F.; Stead, D.; Kang, H. Numerical simulation of squeezing failure in a coal mine roadway due to mining-induced stresses. Rock Mech. Rock Eng. 2015, 48, 1635–1645. [Google Scholar] [CrossRef]
- Kang, H.P.; Lin, J.; Fan, M.J. Investigation on support pattern of a coal roadway within soft rocks—A case study. Int. J. Coal Geol. 2015, 140, 31–40. [Google Scholar] [CrossRef]
- Wu, W.D.; Bai, J.B.; Wang, X.Y.; Yan, S.; Wu, S.X. Numerical study of failure mechanisms and control techniques for a gob-side yield pillar in the Sijiazhuang coal mine, China. Rock Mech. Rock Eng. 2019, 52, 1231–1245. [Google Scholar] [CrossRef]
- Mohamed, K.; Van Dyke, M.; Rashed, G.; Sears, M.M.; Kimutis, R. Preliminary rib support requirements for solid coal ribs using a coal pillar rib rating (CPRR). Int. J. Min. Sci. Technol. 2021, 31, 15–22. [Google Scholar] [CrossRef]





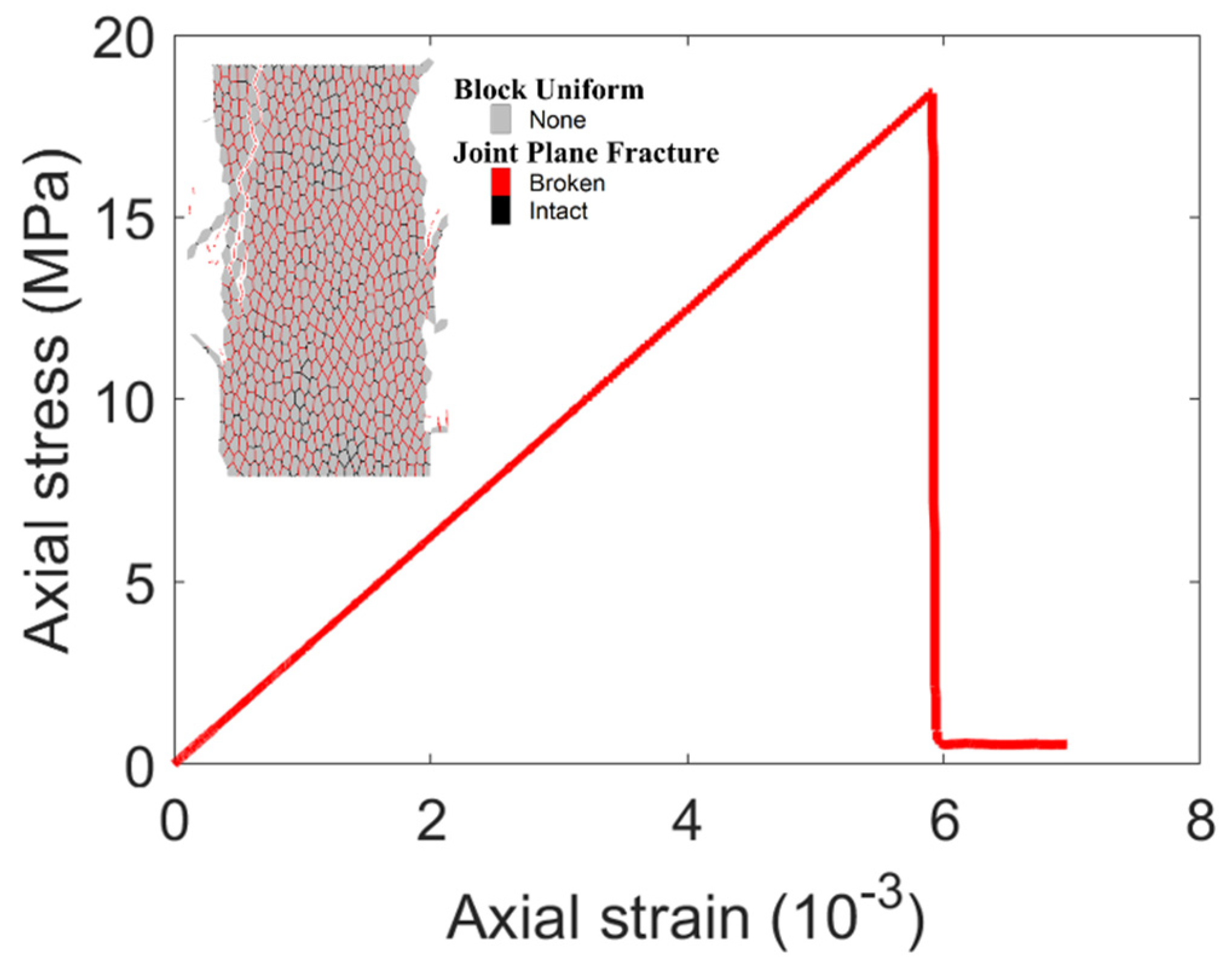
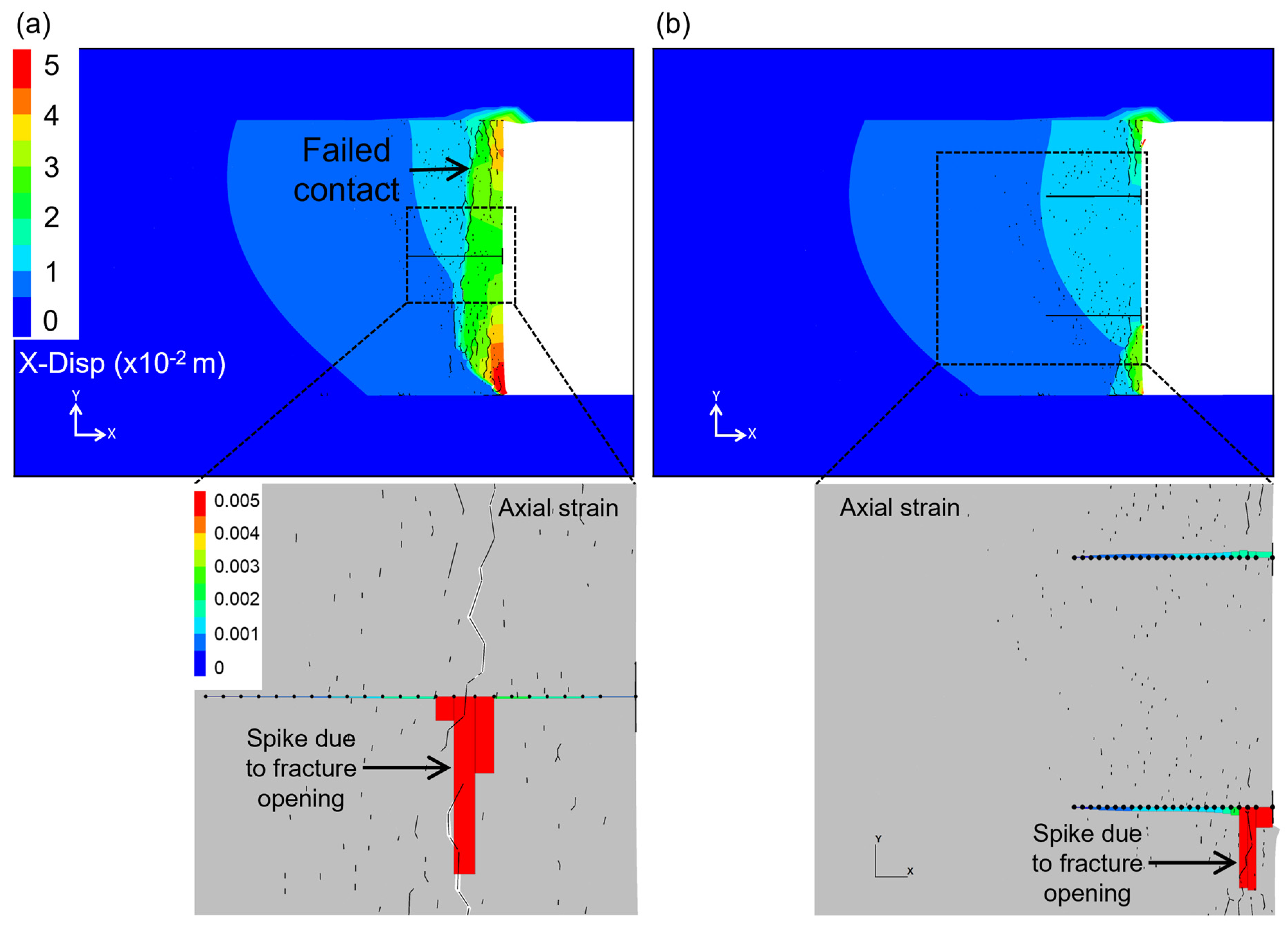
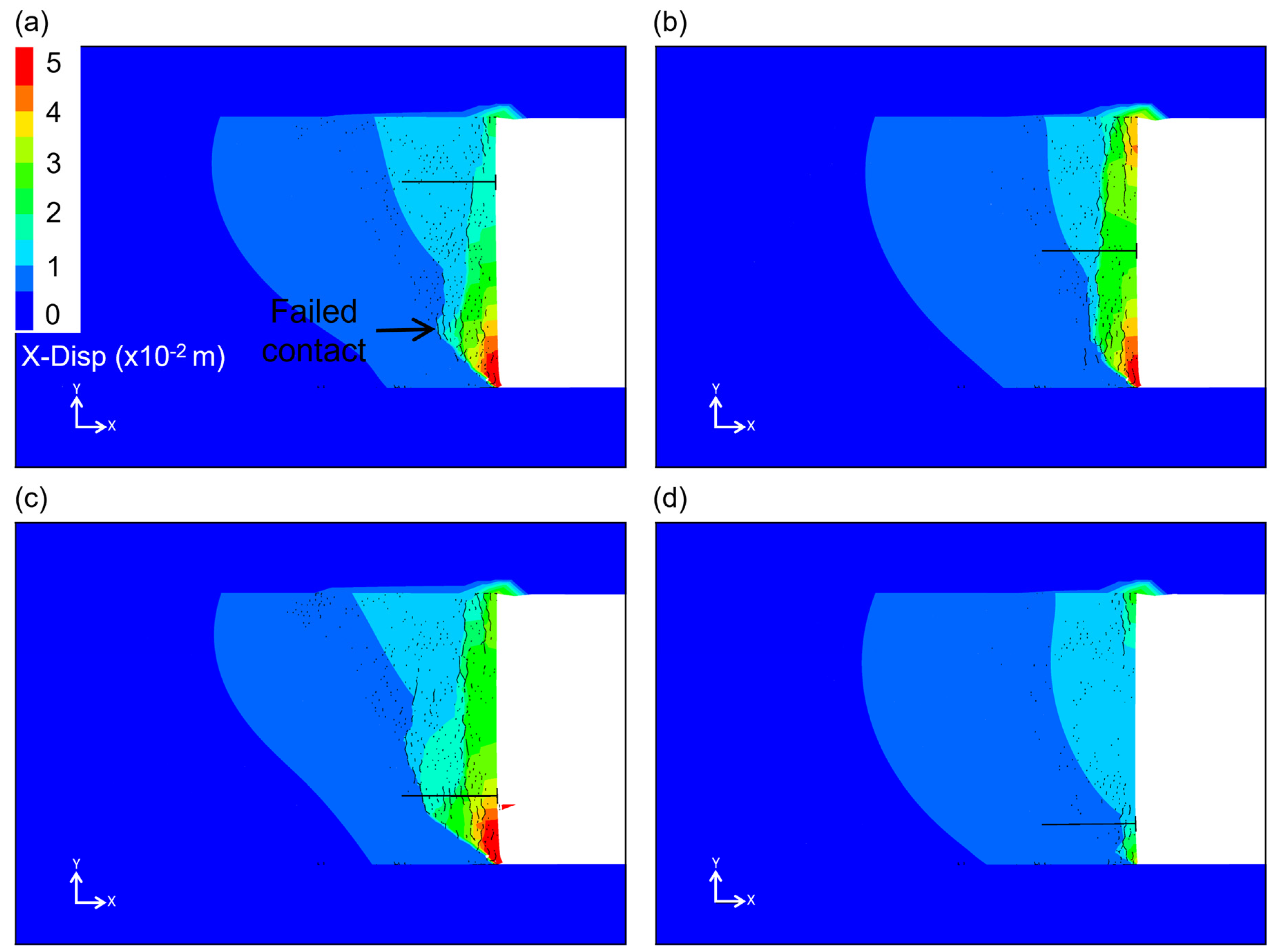
| Parameters | Values |
|---|---|
| Yield threshold (cohesion, MPa) | 6.0 |
| Yield threshold (friction angle, degrees) | 0 |
| Peak threshold; left side (cohesion, MPa) | 0.1 |
| Peak threshold; left side (friction angle, degrees) | 50 |
| Peak threshold; right side (cohesion, MPa) | 9.5 |
| Peak threshold; right side (friction angle, degrees) | 25 |
| Residual threshold; left side (cohesion, MPa) | 0.05 |
| Residual threshold; left side (friction angle, degrees) | 22 |
| from yield to peak (millistrain) | 9 |
| from yield to residual (millistrain) | 45 |
| Dilation angle (°) | 15 |
| Layer | E (GPa), v | Matrix | Ubiquitous Joint (Parallel to Bedding) 2 | ||||
|---|---|---|---|---|---|---|---|
| Cohesion (MPa) 1 | Friction Angle (°) 2 | Tensile Strength (MPa) 1 | Cohesion (MPa) | Friction Angle (°) | Tensile Strength (MPa) | ||
| SS | 10.5, 0.25 | 5.22 | 28 | 1.74 | 3 | 18 | 0.21 |
| MS | 7.59, 0.25 | 2.03 | 20 | 0.58 | 0.2 | 5.0 | 0.06 |
| Interbedded SS and MS | 8.31, 0.25 | 3.05 | 20 | 0.87 | 0.25 | 5 | 0.09 |
| Alluvium 3 | 2, 0.28 | 0.12 | 10 | 0.035 | - | - | - |
| 0 | 0.09 | 0.10 | 0.15 | 0.22 | 0.28 | 0.35 | 0.41 | 0.48 | 0.54 | 0.58 | |
| Cohesion (MPa) | 0.001 | 0.001 | 0.04 | 0.22 | 0.49 | 0.87 | 1.4 | 2.2 | 3.57 | 6.41 | 15 |
| Parameters | Values |
|---|---|
| Yield threshold (cohesion, MPa) | 4.3 |
| Yield threshold (friction angle, degrees) | 20 |
| Peak threshold; left side (cohesion, MPa) | 0.1 |
| Peak threshold; left side (friction angle, degrees) | 60.0 |
| Residual threshold; left side (cohesion, MPa) | 0.05 |
| Residual threshold; left side (friction angle, degrees) | 30 |
| from yield to peak (millistrain) | 9 |
| from yield to residual (millistrain) | 45 |
| Dilation angle (°) | 37 |
| Zones—Strain Softening | Contacts | ||
|---|---|---|---|
| E (GPa) | 3.0 | cpeak (MPa) | 7.8 |
| Peak cohesion (MPa) | 7.0 | cres (MPa) | 0 |
| Residual cohesion (MPa) | 0.5 | φpeak (o) | 40 |
| Peak friction angle (o) | 20 | φres (o) | 14.8 |
| Residual friction angle (o) | 20 | σt (MPa) | 3 |
| Tensile strength (MPa) | 3 | Normal stiffness (GPa/m/m) | 500 |
| Critical plastic shear strain from peak to residual | 0.0025 | Shear stiffness (GPa/m/m) | 250 |
| Parameters | Values |
|---|---|
| Grout cohesive capacity (MN/m) | 0.126 |
| Grout friction angle (o) | 60 |
| Stiffness of grout (MN/m/m) | 1800 |
| Modulus (GPa) | 210 |
| Tensile strength (kN) | 105 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sinha, S.; Walton, G. Application of an Integrated 3D–2D Modeling Approach for Pillar Support Design in a Western US Underground Coal Mine. Geosciences 2023, 13, 333. https://doi.org/10.3390/geosciences13110333
Sinha S, Walton G. Application of an Integrated 3D–2D Modeling Approach for Pillar Support Design in a Western US Underground Coal Mine. Geosciences. 2023; 13(11):333. https://doi.org/10.3390/geosciences13110333
Chicago/Turabian StyleSinha, Sankhaneel, and Gabriel Walton. 2023. "Application of an Integrated 3D–2D Modeling Approach for Pillar Support Design in a Western US Underground Coal Mine" Geosciences 13, no. 11: 333. https://doi.org/10.3390/geosciences13110333
APA StyleSinha, S., & Walton, G. (2023). Application of an Integrated 3D–2D Modeling Approach for Pillar Support Design in a Western US Underground Coal Mine. Geosciences, 13(11), 333. https://doi.org/10.3390/geosciences13110333






