Fracture Transmissivity in Prospective Host Rocks for Enhanced Geothermal Systems (EGS)
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Material
2.2. Methods
3. Results
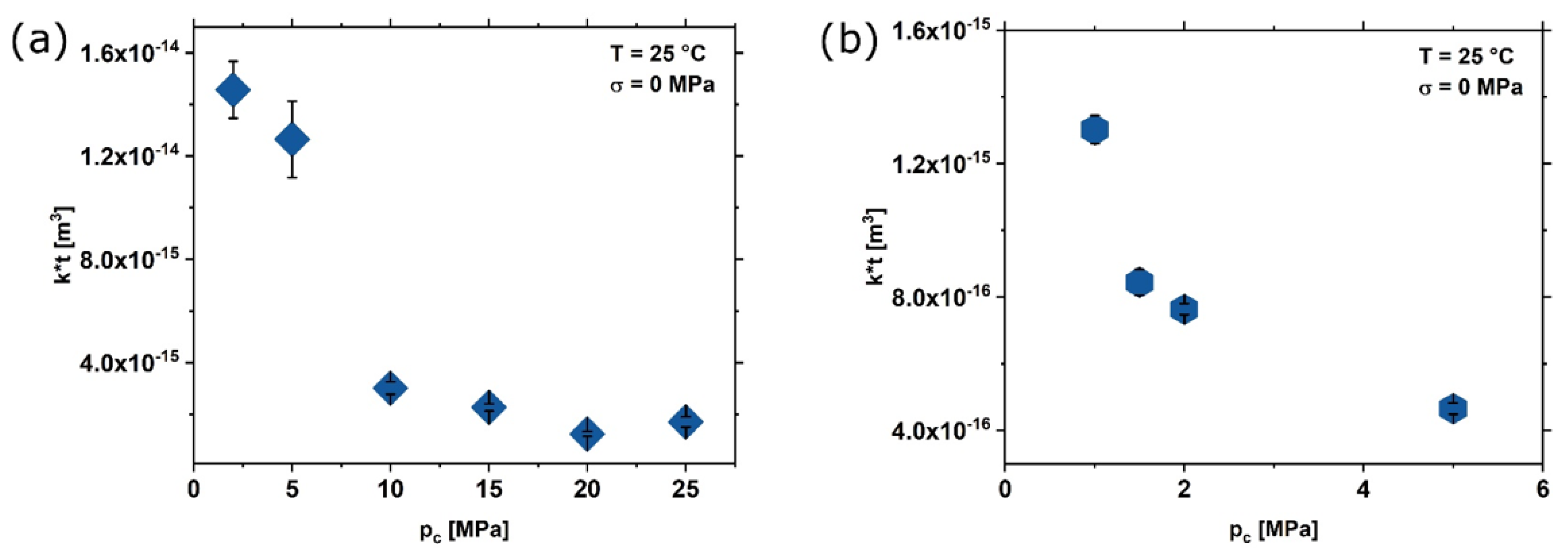
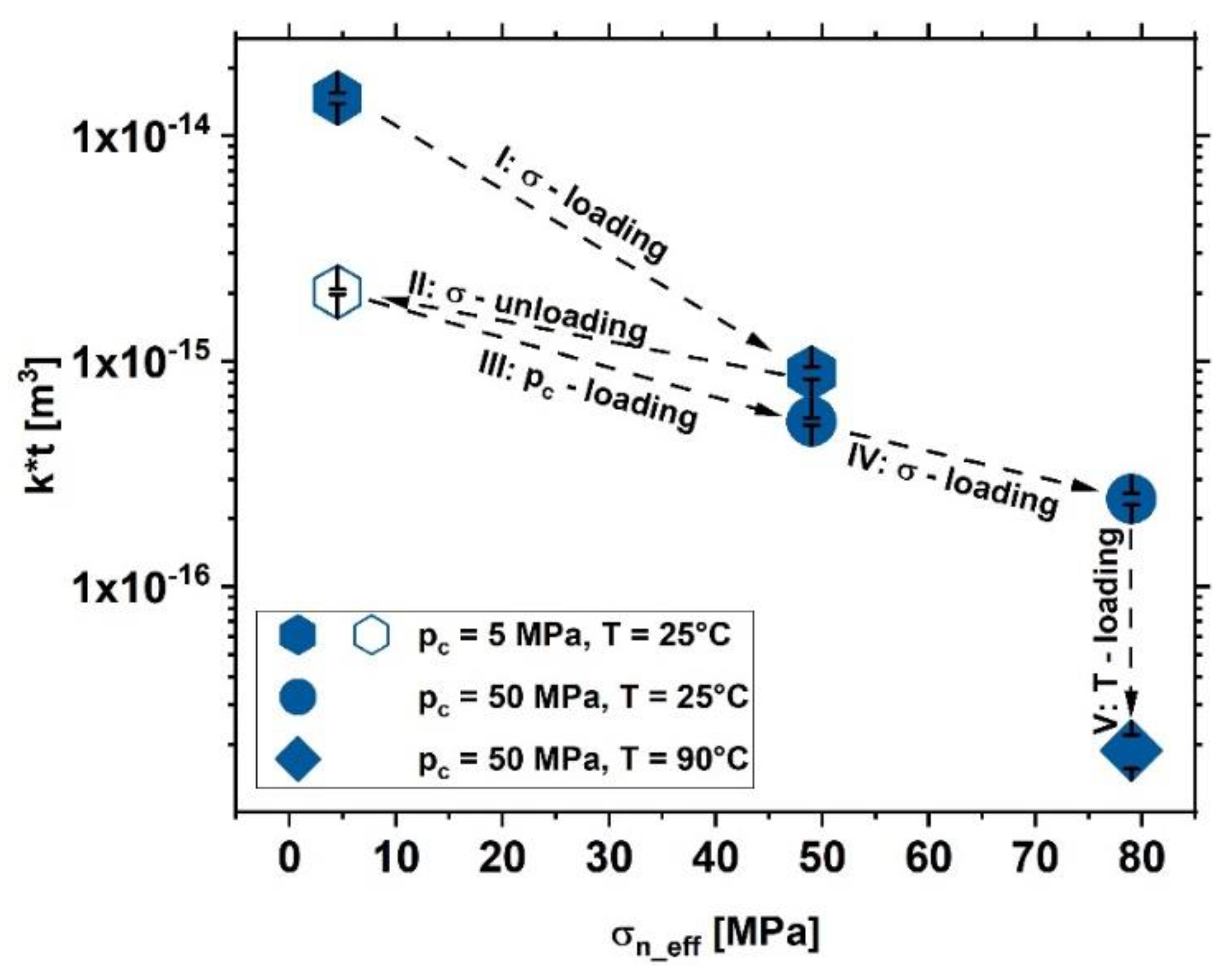
3.1. Effect of Thermodynamic Boundary Conditions
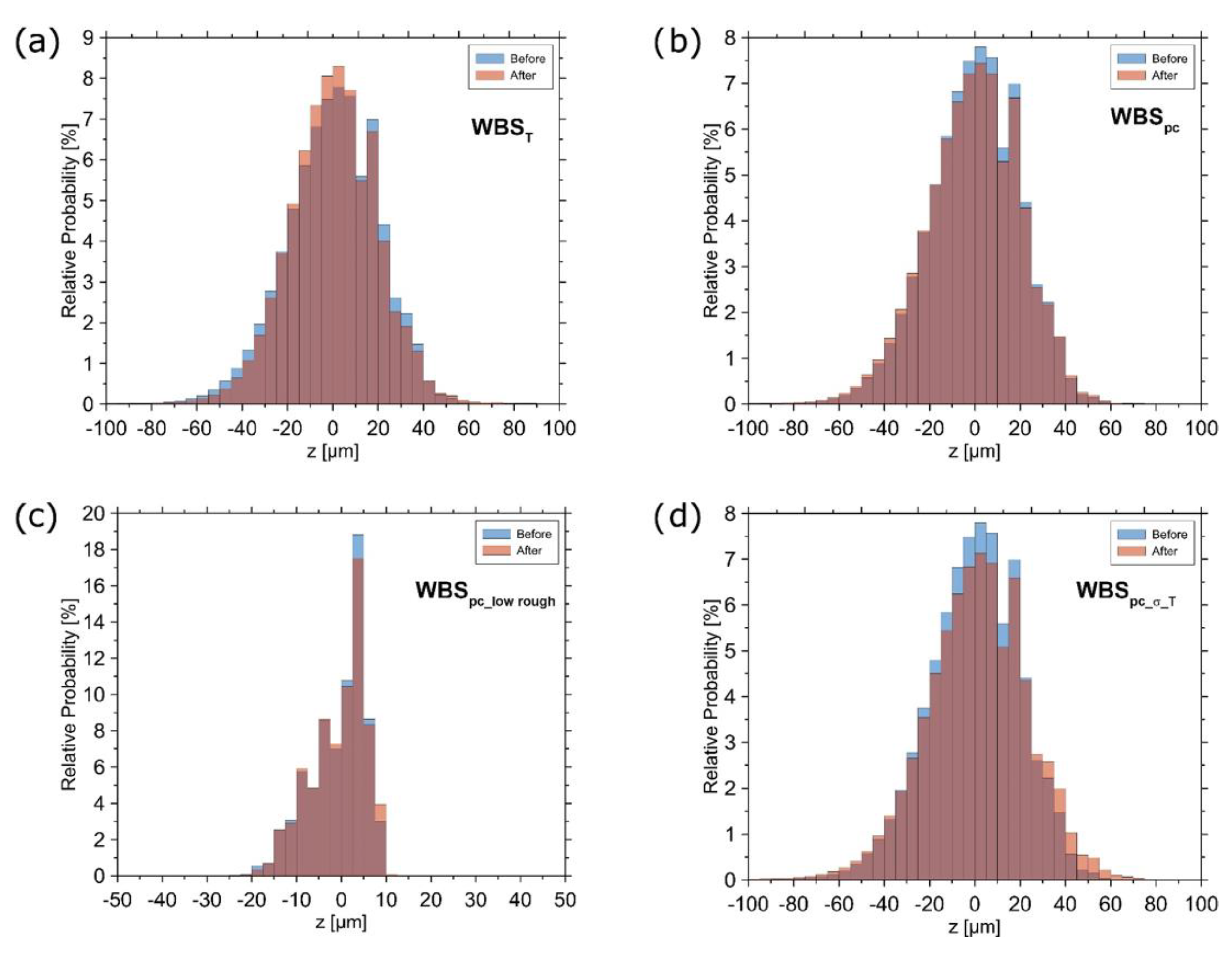
3.2. Effect of Fracture Surface Roughness
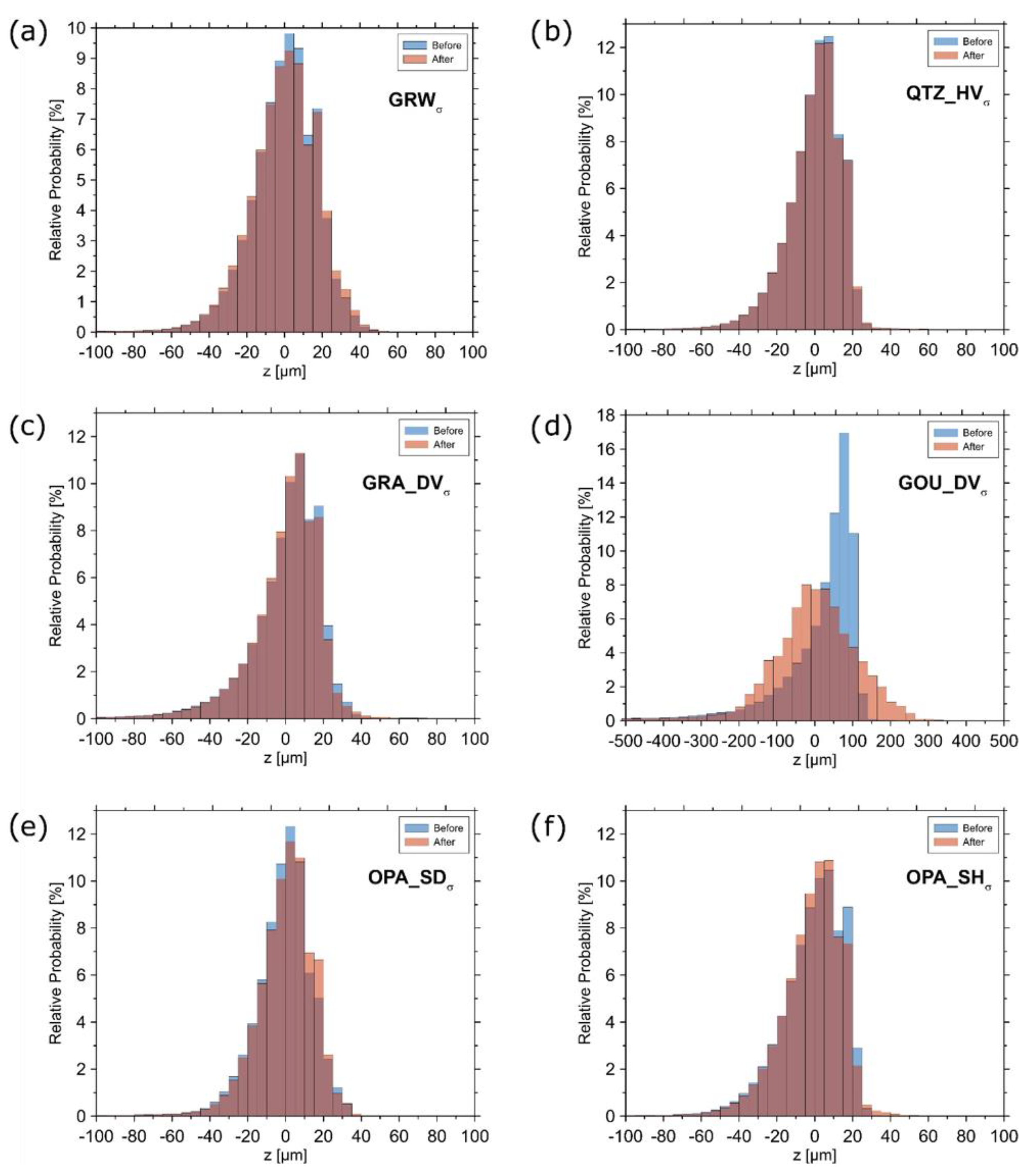
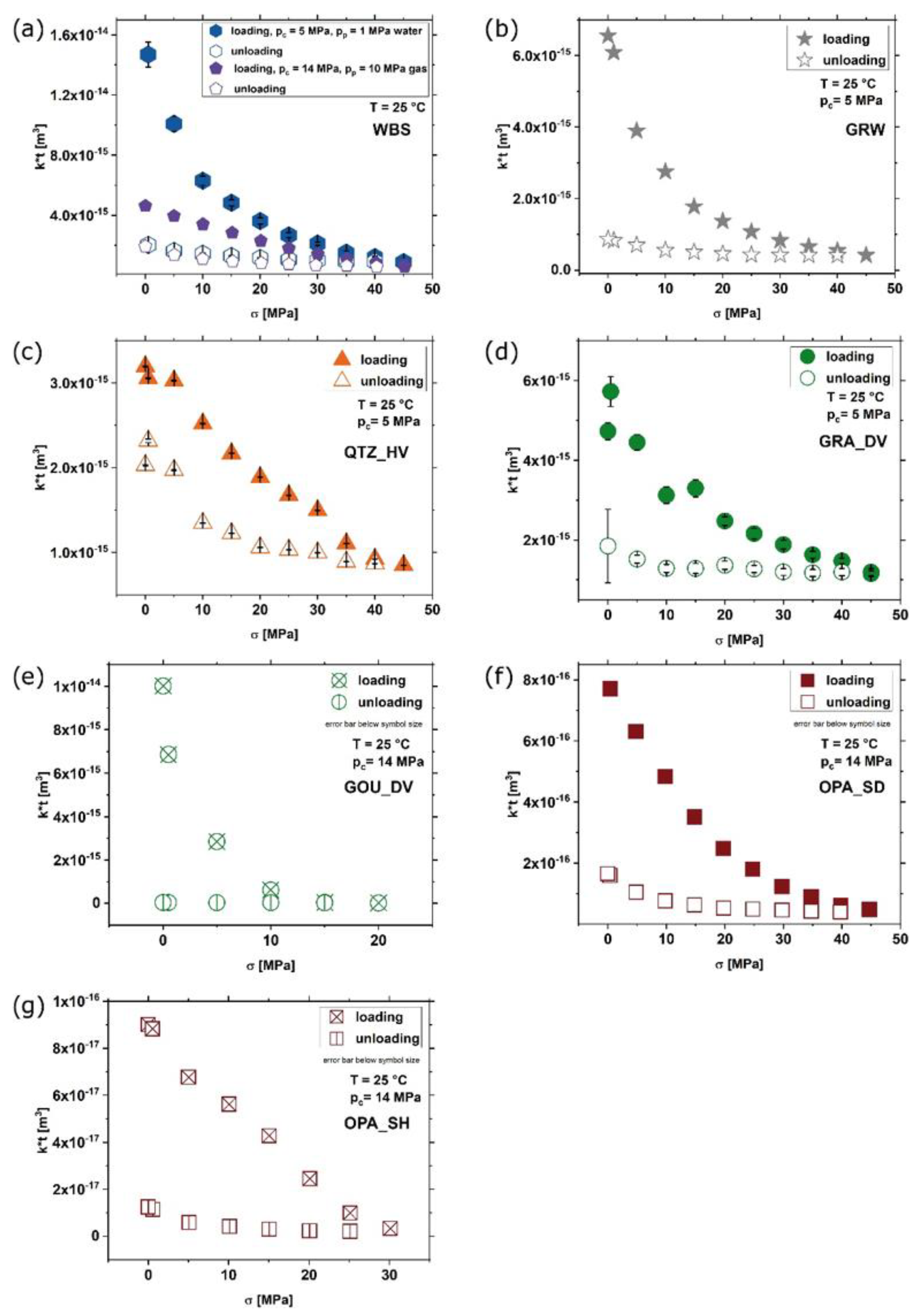
3.3. Effect of Sample Composition
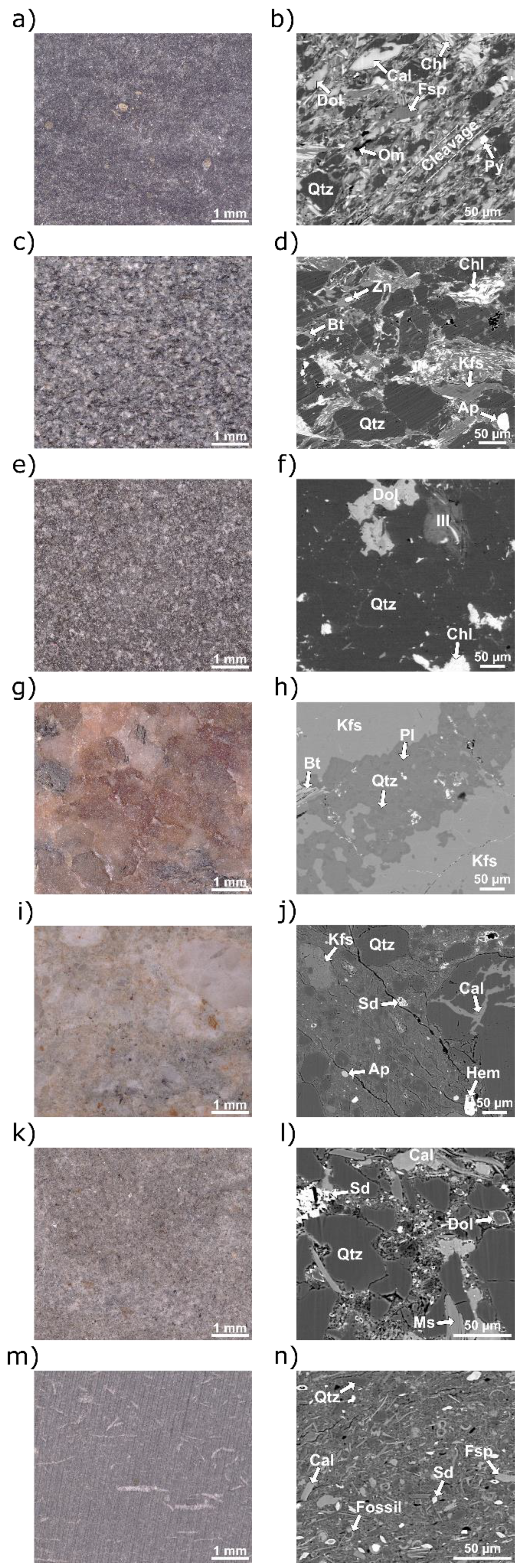
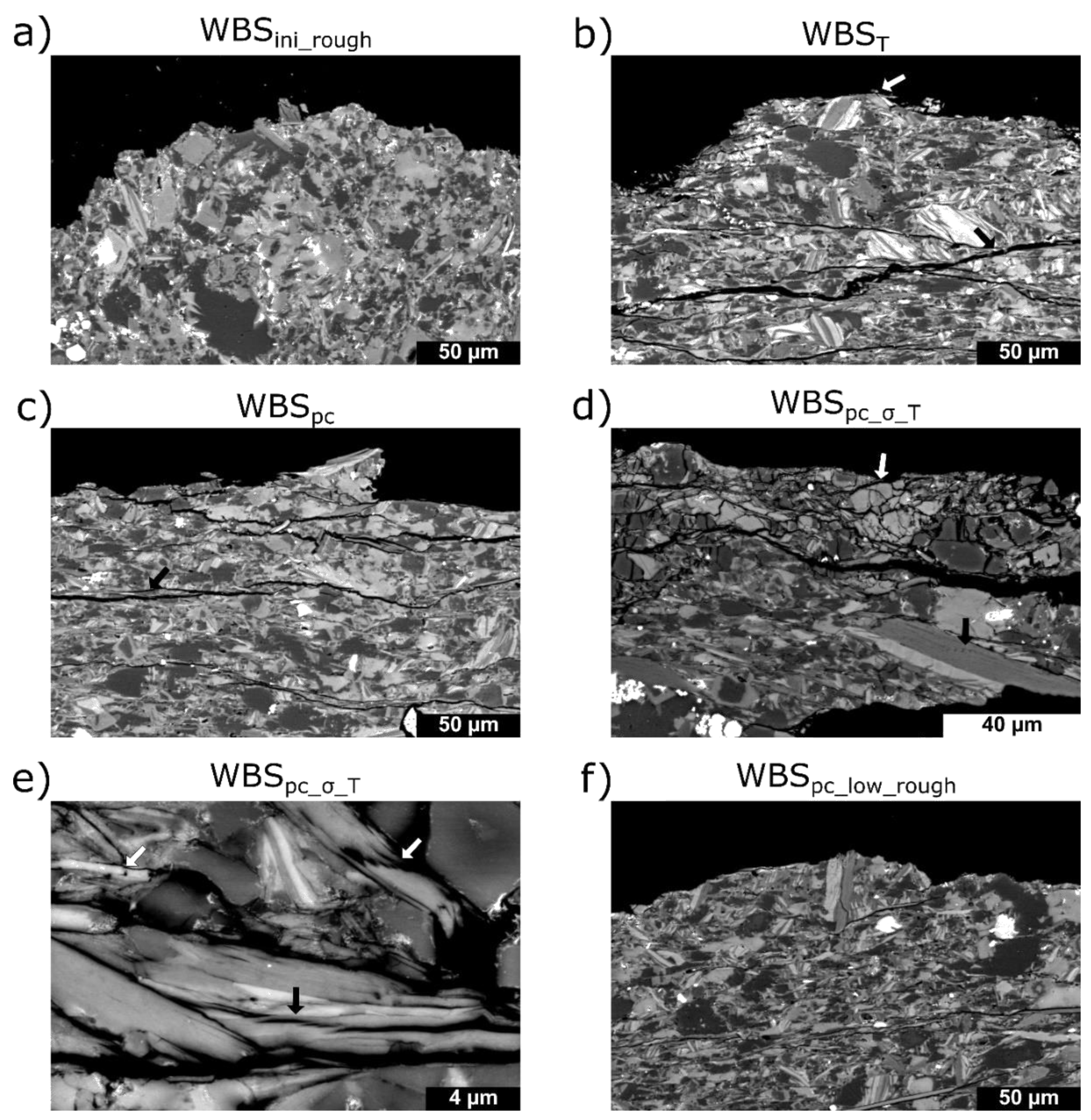
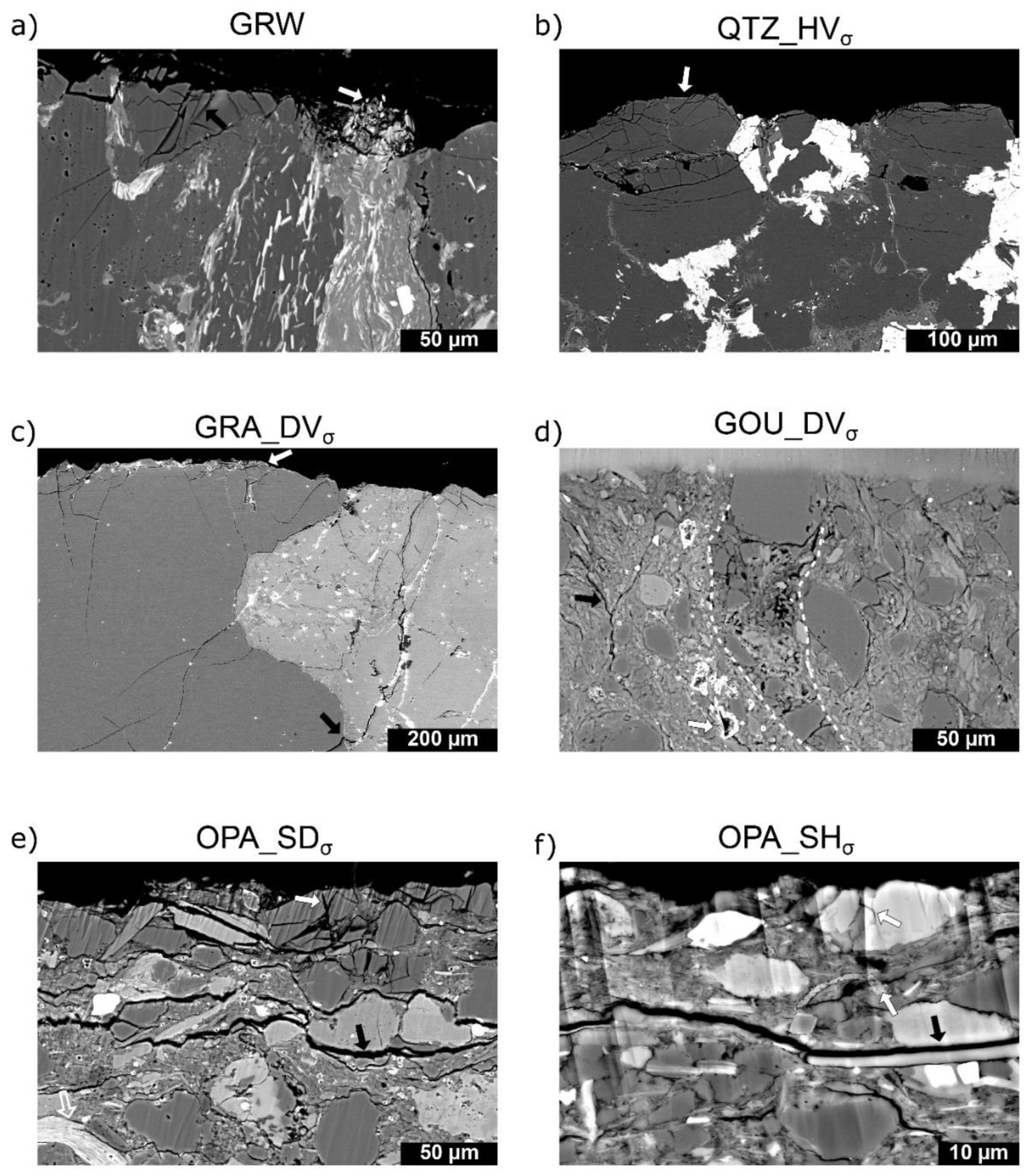
3.4. Microstructures
4. Discussion
4.1. Influence of Thermodynamic Boundary Conditions (T, pc, σ) on Fracture Transmissivity of Wissenbach Slate
4.2. Sample Composition and Mechanical Properties
4.3. Implications for EGS in Different Host Rocks
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

| Symbol | Description | Unit |
|---|---|---|
| ρ | Bulk density | g/cm3 |
| ρg | Grain density | g/cm3 |
| ϕHe | Sample Porosity (using Helium pycnometry) | vol% |
| kmatrix | Matrix permeability | m2 |
| σUCS | Uniaxial compressive strength | MPa |
| σTCS | Triaxial compressive strength | MPa |
| E | Static Young’s modulus | GPa |
| pc | Confining pressure | MPa |
| pp | Fluid pressure | Pa |
| T | Temperature | °C |
| σ | Axial deviatoric stress | MPa |
| Sq | Fracture surface roughness (root mean square) | mm |
| k | Fracture permeability | m2 |
| t | Fracture thickness/hydraulic aperture | mm |
| Q | Volumetric flow rate | ml/min |
| η | Dynamic viscosity | Pa∗s |
| 2a | Distance between up- and downstream borehole | mm |
| r0 | Radius of up- and downstream borehole | mm |
| ∆pp | Differential pore pressure within the fracture | Pa |
| ∆x | Distance within the fracture over which is ∆pp measured | mm |
| B | Geometry factor | / |
| Bcompo | Brittleness based on composition | / |
| wxx | Mineral weighting factor | / |
| fxx | Mineral fraction | wt% |
References
- Vogler, D.; Amann, F.; Bayer, P.; Elsworth, D. Permeability evolution in natural fractures subject to cyclic loading and gouge formation. Rock Mech. Rock Eng. 2016, 49, 3463–3479. [Google Scholar] [CrossRef]
- Lu, S.M. A global review of enhanced geothermal system (EGS). Renew. Sustain. Energy Rev. 2018, 81, 2902–2921. [Google Scholar] [CrossRef]
- Favier, A.; Lardeaux, J.M.; Corsini, M.; Verati, C.; Navelot, V.; Géraud, Y.; Diraison, M.; Ventalon, S.; Voitus, E. Characterization of an exhumed high-temperature hydrothermal system and its application for deep geothermal exploration: An example from Terre-de-Haut Island (Guadeloupe archipelago, Lesser Antilles volcanic arc). J. Volcanol. Geotherm. Res. 2021, 418, 107256. [Google Scholar] [CrossRef]
- Roche, V.; Bouchot, V.; Beccaletto, L.; Jolivet, L.; Guillou-Frottier, L.; Tuduri, J.; Bozkurt, E.; Oguz, K.; Tokay, B. Structural, lithological, and geodynamic controls on geothermal activity in the Menderes geothermal Province (Western Anatolia, Turkey). Int. J. Earth Sci. 2019, 108, 301–328. [Google Scholar] [CrossRef] [Green Version]
- Beauchamps, G.; Ledésert, B.; Hébert, R.; Navelot, V.; Favier, A. The characterisation of an exhumed high-temperature paleo-geothermal system on Terre-de-Haut Island (the Les Saintes archipelago, Guadeloupe) in terms of clay minerals and petrophysics. Geotherm. Energy 2019, 7, 6. [Google Scholar] [CrossRef]
- Gringarten, A.C.; Witherspoon, P.A.; Ohnishi, Y. Theory of heat extraction from fractured hot dry rock. J. Geophys. Res. 1975, 80, 1120–1124. [Google Scholar] [CrossRef]
- Liotta, D.; Brogi, A.; Ruggieri, G.; Zucchi, M. Fossil vs. active geothermal systems: A field and laboratory method to disclose the relationships between geothermal fluid flow and geological structures at depth. Energies 2021, 14, 993. [Google Scholar] [CrossRef]
- Brogi, A.; Alçiçek, M.C.; Liotta, D.; Capezzuoli, E.; Zucchi, M.; Matera, P.F. Step-over fault zones controlling geothermal fluid-flow and travertine formation (Denizli Basin, Turkey). Geothermics 2021, 89, 101941. [Google Scholar] [CrossRef]
- Li, Q.; Xing, H.; Liu, J.; Liu, X. A review on hydraulic fracturing of unconventional reservoir. Petroleum 2015, 1, 8–15. [Google Scholar] [CrossRef] [Green Version]
- Milsch, H.H.; Spangenberg, E.; Kulenkampff, J.; Meyhöfer, S. A new apparatus for long-term petrophysical investigations on geothermal reservoir rocks at simulated in-situ conditions. Transp. Porous Media 2008, 74, 73–85. [Google Scholar] [CrossRef] [Green Version]
- Voltolini, M.; Ajo-Franklin, J. Evolution of propped fractures in shales: The microscale controlling factors as revealed by in situ X-Ray microtomography. J. Pet. Sci. Eng. 2020, 188, 106861. [Google Scholar] [CrossRef]
- Crandall, D.; Moore, J.; Gill, M.; Stadelman, M. CT scanning and flow measurements of shale fractures after multiple shearing events. Int. J. Rock Mech. Min. Sci. 2017, 100, 177–187. [Google Scholar] [CrossRef]
- Carey, J.W.; Lei, Z.; Rougier, E.; Mori, H.; Viswanathan, H. Fracture-permeability behavior of shale. J. Unconv. Oil Gas Resour. 2015, 11, 27–43. [Google Scholar] [CrossRef]
- Leung, C.T.O.; Zimmerman, R.W. Estimating the Hydraulic Conductivity of Two-Dimensional Fracture Networks Using Network Geometric Properties. Transp. Porous Media 2012, 93, 777–797. [Google Scholar] [CrossRef]
- Stober, I.; Bucher, K. Hydraulic conductivity of fractured upper crust: Insights from hydraulic tests in boreholes and fluid-rock interaction in crystalline basement rocks. Geofluids 2015, 15, 161–178. [Google Scholar] [CrossRef]
- Cho, Y.; Apaydin, O.G.; Ozkan, E. Pressure-dependent natural-fracture permeability in shale and its effect on shale-gas well production. SPE Reserv. Eval. Eng. 2013, 16, 216–228. [Google Scholar] [CrossRef]
- Gutierrez, M.; Øino, L.E.; Nygård, R. Stress-dependent permeability of a de-mineralised fracture in shale. Mar. Pet. Geol. 2000, 17, 895–907. [Google Scholar] [CrossRef]
- Liu, E. Effects of fracture aperture and roughness on hydraulic and mechanical properties of rocks: Implication of seismic characterization of fractured reservoirs. J. Geophys. Eng. 2005, 2, 38–47. [Google Scholar] [CrossRef]
- Rutter, E.H.; Mecklenburgh, J. Influence of normal and shear stress on the hydraulic transmissivity of thin cracks in a tight quartz sandstone, a granite, and a shale. J. Geophys. Res. Solid Earth 2018, 123, 1262–1285. [Google Scholar] [CrossRef] [Green Version]
- Blöcher, G.; Kluge, C.; Milsch, H.; Cacace, M.; Jacquey, A.B.; Schmittbuhl, J. Permeability of matrix-fracture systems under mechanical loading—Constraints from laboratory experiments and 3-D numerical modelling. Adv. Geosci. 2019, 49, 95–104. [Google Scholar] [CrossRef] [Green Version]
- Cheng, C.; Milsch, H. Permeability variations in illite-bearing sandstone: Effects of temperature and NaCl fluid salinity. J. Geophys. Res. Solid Earth 2020, 125, 9. [Google Scholar] [CrossRef]
- Cheng, C.; Herrmann, J.; Wagner, B.; Leiss, B.; Stammeier, J.A.; Rybacki, E.; Milsch, H. Long-Term Evolution of Fracture Permeability in Slate: An Experimental Study with Implications for Enhanced Geothermal Systems (EGS). Geosciences 2021, 11, 443. [Google Scholar] [CrossRef]
- Walsh, S.D.C.; Smith, M.; Carroll, S.A.; Crandall, D. Non-invasive measurement of proppant pack deformation. Int. J. Rock Mech. Min. Sci. 2016, 87, 39–47. [Google Scholar] [CrossRef]
- Zhang, J.; Ouyang, L.; Zhu, D.; Hill, A.D. Experimental and numerical studies of reduced fracture conductivity due to proppant embedment in the shale reservoir. J. Pet. Sci. Eng. 2015, 130, 37–45. [Google Scholar] [CrossRef] [Green Version]
- Watanabe, N.; Ishibashi, T.; Ohsaki, Y.; Tsuchiya, Y.; Tamagawa, T.; Hirano, N.; Okabe, H.; Tsuchiya, N. X-ray CT based numerical analysis of fracture flow for core samples under various confining pressures. Eng. Geol. 2011, 123, 338–346. [Google Scholar] [CrossRef]
- Schill, E.; Genter, A.; Cuenot, N.; Kohl, T. Hydraulic performance history at the Soultz EGS reservoirs from stimulation and long-term circulation tests. Geothermics 2017, 70, 110–124. [Google Scholar] [CrossRef]
- Vidal, J.; Genter, A. Overview of naturally permeable fractured reservoirs in the central and southern Upper Rhine Graben: Insights from geothermal wells. Geothermics 2018, 74, 57–73. [Google Scholar] [CrossRef]
- Vanbrabant, Y.; Stenmans, V.; Burlet, C.; Petitclerc, E.; Meyvis, B.; Stasi, G.; Abarim, R.; Bar, K.; Goovaerts, T. Havelange deep borehole (Belgium): A study case for the evaluation of metasedimentary formations as potential geothermal reservoir—H2020 MEET project. In Proceedings of the EGU General Assembly Conference 2020, Online, 4–8 May 2020; p. 10943. [Google Scholar]
- Leiss, B.; Tanner, D.; Vollbrecht, A.; Wemmer, K. Neue Untersuchungen zur Geologie der Leinetalgrabenstruktur; Universitätsverlag Göttingen: Göttingen, Germany, 2011. [Google Scholar]
- Leiss, B.; Tanner, D.; Vollbrecht, A.; Wemmer, K. Tiefengeothermisches Potential in der Region Göttingen—Geologische Rahmenbedingungen. In Neue Untersuchungen zur Geologie der Leinetalgrabenstruktur; Leiss, B., Tanner, D., Vollbrecht, A., Arp, G., Eds.; Universitätsverlag Göttingen: Göttingen, Germany, 2011; pp. 163–170. [Google Scholar] [CrossRef]
- Leiss, B.; Wagner, B.; Heinrichs, T.; Romanov, D.; Tanner, D.C.; Vollbrecht, A.; Wemmer, K. Integrating deep, medium and shallow geothermal energy into district heating and cooling system as an energy transition approach for the Göttingen University Campus. In Proceedings of the World Geothermal Congress 2021, Reykjavik, Iceland, 24–27 October 2021; pp. 1–9. [Google Scholar]
- Brinckmann, J.; Brüning, U. (Eds.) Das Bundesbohrprogramm im West-Harz, Paläogeographische Ergebnisse (The Federal Drilling Program in the Western Harz Mountains: Paleogeographic Results and Five Additional Contributions to the Geology of the Western Harz Mountains); Geologisches Jahrbuch Reihe D: Hanover, Germany, 1986; Band D. [Google Scholar]
- Gerling, P.; Kockel, F.; Krull, P. The HC Potential of Pre-Westphalian Sediments in the North German Basin—A Synthesis; DGMK-Research Report 43; 1999. Available online: https://www.osti.gov/etdeweb/biblio/695510 (accessed on 7 April 2022).
- Littke, R.; Krooss, B.; Uffmann, A.K.; Schulz, H.M.; Horsfield, B. Unconventional gas resources in the Paleozoic of Central Europe. Oil Gas Sci. Technol. 2011, 66, 953–977. [Google Scholar] [CrossRef] [Green Version]
- Schubert, M. Die Dysaerobe Biofazies der Wissenbach Schiefer (Rheinisches Schiefergebirge, Harz, Devon); Institute der Georg-August-Universit: Göttingen, Germany, 1996. [Google Scholar]
- Sáez, R.; Moreno, C.; González, F.; Almodóvar, G.R. Black shales and massive sulfide deposits: Causal or casual relationships? Insights from Rammelsberg, Tharsis, and Draa Sfar. Miner. Depos. 2011, 46, 585–614. [Google Scholar] [CrossRef]
- Klee, J.; Potel, S.; Ledésert, B.; Hébert, R.; Chabani, A.; Barrier, P.; Trullenque, G. Fluid-rock interactions in a Paleo-geothermal reservoir (Noble Hills Granite, California, USA). Part 1: Granite pervasive alteration processes away from fracture zones. Geosciences 2021, 11, 325. [Google Scholar] [CrossRef]
- Klee, J.; Chabani, A.; Ledésert, B.A.; Potel, S.; Hébert, R.L.; Trullenque, G. Fluid-rock interactions in a paleo-geothermal reservoir (Noble hills granite, california, usa). part 2: The influence of fracturing on granite alteration processes and fluid circulation at low to moderate regional strain. Geosciences 2021, 11, 433. [Google Scholar] [CrossRef]
- Amann, F.; Wild, K.M.; Loew, S.; Yong, S.; Thoeny, R.; Frank, E. Geomechanical behaviour of Opalinus Clay at multiple scales: Results from Mont Terri rock laboratory (Switzerland). Swiss J. Geosci. 2017, 110, 151–171. [Google Scholar] [CrossRef]
- Bossart, P.; Bernier, F.; Birkholzer, J.; Bruggeman, C.; Connolly, P.; Dewonck, S.; Fukaya, M.; Hexfort, M.; Jensen, M.; Matray, J.-M.; et al. Mont Terri rock laboratory, 20 years of research: Introduction, site characteristics and overview of experiments. Swiss J. Geosci. 2017, 110, 3–22. [Google Scholar] [CrossRef] [Green Version]
- Schuster, V.; Rybacki, E.; Bonnelye, A.; Herrmann, J.; Schleicher, A.; Dresen, G. Experimental deformation of Opalinus Clay at elevated temperature and pressure conditions: Mechanical properties and the influence of rock fabric. Rock Mech. Rock Eng. 2021, 54, 4009–4039. [Google Scholar] [CrossRef]
- Nussbaum, C.; Kloppenburg, A.; Caër, T.; Bossart, P. Tectonic evolution around the Mont Terri rock laboratory, northwestern Swiss Jura: Constraints from kinematic forward modelling. Swiss J. Geosci. 2017, 110, 39–66. [Google Scholar] [CrossRef] [Green Version]
- Yu, C.; Matray, J.M.; Gonçalvès, J.; Jaeggi, D.; Gräsle, W.; Wieczorek, K.; Vogt, T.; Skyes, E. Comparative study of methods to estimate hydraulic parameters in the hydraulically undisturbed Opalinus Clay (Switzerland). Swiss J. Geosci. 2017, 110, 85–104. [Google Scholar] [CrossRef] [Green Version]
- McKernan, R.; Mecklenburgh, J.; Rutter, E.; Taylor, K. Microstructural controls on the pressure-dependent permeability of Whitby mudstone. Geol. Soc. 2017, 454, 39–66. [Google Scholar] [CrossRef] [Green Version]
- Ji, Y.; Hofmann, H.; Rutter, E.H.; Xiao, F.; Yang, L. Revisiting the Evaluation of Hydraulic Transmissivity of Elliptical Rock Fractures in Triaxial Shear-Flow Experiments. Rock Mech. Rock Eng. 2022, 1–9. [Google Scholar] [CrossRef]
- Bieniawski, Z.T.; Bernede, M.J. Suggested methods for determining the uniaxial compressive strength and deformability of rock materials: Part 1. Suggested method for determining deformability of rock materials in uniaxial compression. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1979, 16, 138–140. [Google Scholar] [CrossRef]
- Paterson, M.S. A high-pressure, high-temperature apparatus for rock deformation. Int. J. Rock Mech. Min. Sci. 1970, 7, 517–526. [Google Scholar] [CrossRef]
- Rybacki, E.; Reinicke, A.; Meier, T.; Makasi, M.; Dresen, G. What controls the mechanical properties of shale rocks?—Part I: Strength and Young’s modulus. J. Pet. Sci. Eng. 2015, 135, 702–722. [Google Scholar] [CrossRef]
- von Terzaghi, K. Die Berechnung der Durchas- sigkeitsziffer des Tones aus dem Veriauf der hydrodynamischen Spannungserscheinungen, Sitzungsber. Akad. Wiss. Wien Math Naturwiss. 1923, 132, 105. [Google Scholar]
- Cheng, C.; Milsch, H. Evolution of fracture aperture in quartz sandstone under hydrothermal conditions: Mechanical and chemical effects. Minerals 2020, 10, 657. [Google Scholar] [CrossRef]
- Li, F.B.; Sheng, J.C.; Zhan, M.L.; Xu, L.M.; Wu, Q.; Jia, C.L. Evolution of limestone fracture permeability under coupled thermal, hydrological, mechanical, and chemical conditions. J. Hydrodyn. 2014, 26, 234–241. [Google Scholar] [CrossRef]
- Lima, M.G.; Vogler, D.; Querci, L.; Madonna, C.; Hattendorf, B.; Saar, M.O.; Kong, X.Z. Thermally driven fracture aperture variation in naturally fractured granites. Geotherm. Energy 2019, 7, 23. [Google Scholar] [CrossRef]
- Yasuhara, H.; Kinoshita, N.; Ohfuji, H.; Lee, D.S.; Nakashima, S.; Kishida, K. Temporal alteration of fracture permeability in granite under hydrothermal conditions and its interpretation by coupled chemo-mechanical model. Appl. Geochem. 2011, 26, 2074–2088. [Google Scholar] [CrossRef]
- Kamali-Asl, A.; Ghazanfari, E.; Perdrial, N.; Bredice, N. Experimental study of fracture response in granite specimens subjected to hydrothermal conditions relevant for enhanced geothermal systems. Geothermics 2018, 72, 205–224. [Google Scholar] [CrossRef]
- Yasuhara, H.; Elsworth, D. Compaction of a rock fracture moderated by competing roles of stress corrosion and pressure solution. Pure Appl. Geophys. 2008, 165, 1289–1306. [Google Scholar] [CrossRef] [Green Version]
- Rutqvist, J. Fractured rock stress-permeability relationships from in situ data and effects of temperature and chemical-mechanical couplings. Geofluids 2015, 15, 48–66. [Google Scholar] [CrossRef] [Green Version]
- Cao, N.; Lei, G.; Dong, P.; Li, H.; Wu, Z.; Li, Y. Stress-dependent permeability of fractures in tight reservoirs. Energies 2019, 12, 117. [Google Scholar] [CrossRef] [Green Version]
- Rutter, E.H.; Mecklenburgh, J. Hydraulic conductivity of bedding-parallel cracks in shale as a function of shear and normal stress. Geol. Soc. 2017, 454, 67. [Google Scholar] [CrossRef]
- Yasuhara, H.; Elsworth, D. Evolution of permeability in a natural fracture: Significant role of pressure solution. J. Geophys. Res. 2004, 109, 03204. [Google Scholar] [CrossRef]
- Bowden, F.P.; Tabor, D. The Friction and Lubrication of Solids; Clarendon Press: Oxford, UK, 1964; Volume 2, No. 2. [Google Scholar]
- Huo, D.; Benson, S.M. An Experimental Investigation of Stress-Dependent Permeability and Permeability Hysteresis Behavior in Rock Fractures. Dynamics of Fluids and Transport in Complex Fractured-Porous Systems. Geophys. Monogr. 2015, 210, 99–114. [Google Scholar]
- Gangi, A.F. Variation of whole and fractured porous rock permeability with confining pressure. Int. J. Rock Mech. Min. Sci. 1978, 15, 249–257. [Google Scholar] [CrossRef]
- Tsang, Y.W.; Witherspoon, P.A. The dependence of fracture mechanical and fluid flow properties on fracture roughness and sample size. J. Geophys. Res. 1983, 88, 2359–2366. [Google Scholar] [CrossRef] [Green Version]
- Walsh, J.B. Effect of pore pressure and confining pressure on fracture permeability. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 429–435. [Google Scholar] [CrossRef]
- Witherspoon, P.A.; Wang, J.S.Y.; Iwai, K.; Gale, J.E. Validity of cubic law for fluid-flow in a deformable rock fracture. Water Resour. Res. 1980, 16, 1016–1024. [Google Scholar] [CrossRef] [Green Version]
- Zimmerman, R.W.; Chen, D.W.; Cook, N.G.W. The effect of contact area on the permeability of fractures. J. Hydrol. 1992, 139, 79–96. [Google Scholar] [CrossRef] [Green Version]
- Barton, N.; Bandis, S.; Bakhtar, K. Strength, Deformation and Conductivity Coupling of Rock Joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 121–140. [Google Scholar] [CrossRef]
- Gale, J.E. The effects of fracture type induced versus natural on the stress-fracture closure-fracture permeability relationships. In Proceedings of the 23rd U.S. Symposium on Rock Mechanics, University of California, Berkeley, CA, USA, 25–27 August 1982; pp. 290–298. [Google Scholar]
- Rybacki, E.; Meier, T.; Dresen, G. What controls the mechanical properties of shale rocks?—Part II: Brittleness. J. Pet. Sci. Eng. 2016, 144, 39–58. [Google Scholar] [CrossRef] [Green Version]
- Herrmann, J.; Rybacki, E.; Sone, H.; Dresen, G. Deformation experiments on Bowland and Posidonia shale—Part I: Strength and Young’s modulus at ambient and in situ pc-T conditions. Rock Mech. Rock Eng. 2018, 51, 3645–3666. [Google Scholar] [CrossRef] [Green Version]
- Ulusay, R. The ISRM Suggested Methods for Rock Characterization, Testing and Monitoring 2007–2014; Springer: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Durham, W.B.; Bonner, B.P. Self-propping and fluid flow in slightly offset joints at high effective pressures. J. Geophys. Res. 1994, 99, 9391–9399. [Google Scholar] [CrossRef]
- Reinicke, A.; Rybacki, E.; Stanchits, S.; Huenges, E.; Dresen, G. Hydraulic fracturing stimulation techniques and formation damage mechanisms—Implications from laboratory testing of tight sandstone–proppant systems. Geochemistry 2010, 70 (Suppl. S3), 107–117. [Google Scholar] [CrossRef]






| Formation | Sample ID | Depth [m] | ρ [g/cm3] | ρg [g/cm3] | ϕHe [%] | Phyl [wt%] | Cb [wt%] | QFSO [wt%] | Sq_ini [mm] | Sq_def [mm] | Mechanical Properties | Experimental Conditions | Fluid Medium |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| WBS | 1134–1210 | 2.76 | 2.82 | 2.0 | 46 | 26 | 28 | 0.006 (low) | Perpendicular: σUCS = 219 ± 2 MPa E = 27.4 ± 0.3 GPa | UCS | - | ||
| 0.019 (high) | Parallel: σUCS = 124 ± 1 MPa E = 31.4 ± 0.3 GPa | UCS | - | ||||||||||
| Perpendicular: σTCS = 498 ± 20 MPa E = 69 ± 14 GPa | Triaxial: pc = 50 MPa T = 100 °C = 5 × 10−4 s−1 | - | |||||||||||
| WBST | 0.019 | Hydrostatic: pc = 5 MPa pp = 1 MPa, T = 25–100 °C | H2O | ||||||||||
| WBSpc | 0.020 | Hydrostatic: pc = 2–25 MPa pp = 1 MPa, T = 25 °C | H2O | ||||||||||
| WBSpc_σ_T | 0.021 | Triaxial: pc = 5–50 MPa pp = 1 MPa, σ = 0–45 MPa T = 25–90 °C | H2O | ||||||||||
| WBSσAR | 0.020 | Triaxial: pc = 14 MPa pp = 10 MPa, σ = 0–45 MPa T = 25 °C | Ar | ||||||||||
| WBSpc_lowrough | 0.006 | Triaxial: pc = 1–5 MPa pp = 0.5–1 MPa, T = 25 °C | H2O | ||||||||||
| GRW | 843 | 2.67 | 2.69 | 0.7 | 57 | 2 | 41 | 0.016 | σUCS = 185 ± 2 MPa E = 35.2 ± 0.3 GPa | UCS | - | ||
| GRWσ | 0.016 | Triaxial: pc = 5 MPa, pp = 1 MPa, σ = 0–45 MPa T = 25 °C | H2O | ||||||||||
| QTZ_HV | 4732 | 2.69 | 2.7 | 0.2 | 20 | 3 | 77 | 0.014 | σUCS = 175 ± 2 MPa E = 35.6 ± 0.4 GPa | UCS | - | ||
| QTZ_HVσ | 0.014 | Triaxial: pc = 5 MPa pp = 1 MPa, σ = 0–45 MPa T = 25 °C | H2O | ||||||||||
| GRA_DV | OC | 2.63 | 2.66 | 1.3 | 9 | 1 | 90 | 0.019 | σUCS = 160 ± 2 MPa E = 31.7 ± 0.3 GPa | UCS | - | ||
| GRA_DVσ | 0.019 | Triaxial: pc = 5 MPa pp = 1 MPa, σ = 0–45 MPa T = 25 °C | H2O | ||||||||||
| GOU_DV | OC | 2.24 | 2.56 | 12.6 | 34 | 7 | 59 | 0.247 | σUCS = 4.5 ± 0.7 MPa E = 0.7 ± 0.3 GPa | UCS | - | ||
| GOU_DVσ | 0.175 | Triaxial: pc = 14 MPa pp = 10 MPa, σ = 0–20 MPa T = 25 °C | Ar | ||||||||||
| OPA_SD | MT_URL | 2.38 | 2.7 | 11.8 | 30 | 13 | 57 | 0.012 | σUCS = 49.6 ± 0.5 MPa E = 5.3 ± 0.1 GPa | UCS | - | ||
| OPA_SDσ | 0.012 | Triaxial: pc = 14 MPa pp = 10 MPa, σ = 0–45 MPa T = 25 °C | Ar | ||||||||||
| OPA_SH | MT_URL | 2.39 | 2.77 | 13.8 | 74 | 11 | 15 | 0.016 | σUCS = 34.9 ± 0.3 MPa E = 1.8 ± 0.1 GPa | UCS | - | ||
| OPA_SHσ | 0.015 | Triaxial: pc = 14 MPa pp = 10 MPa, σ = 0–30 MPa T = 25 °C | Ar |
| T [°C] | pp [MPa] | k∗t [m3] |
|---|---|---|
| 25 | 1 | 1.33 × 10−14 |
| 40 | 1 | 1.02 × 10−14 |
| 60 | 1 | 1.02 × 10−14 |
| 80 | 1 | 1.07 × 10−14 |
| 100 | 1 | 9.40 × 10−15 |
| pc [MPa] | pp [MPa] | k∗t [m3] |
|---|---|---|
| 2 | 1 | 1.46 × 10−14 |
| 5 | 1 | 1.26 × 10−14 |
| 10 | 1 | 3.01 × 10−15 |
| 15 | 1 | 2.27 × 10−15 |
| 20 | 1 | 1.24 × 10−15 |
| 25 | 1 | 1.71 × 10−15 |
| σ [MPa] | pp [MPa] | k∗t [m3] |
|---|---|---|
| 0.5 | 1 | 1.47 × 10−14 |
| 5 | 1 | 1.01 × 10−14 |
| 10 | 1 | 6.30 × 10−15 |
| 15 | 1 | 4.83 × 10−15 |
| 20 | 1 | 3.62 × 10−15 |
| 25 | 1 | 2.67 × 10−15 |
| 30 | 1 | 2.12 × 10−15 |
| 35 | 1 | 1.51 × 10−15 |
| 40 | 1 | 1.22 × 10−15 |
| 45 | 1 | 8.87 × 10−16 |
| 40 | 1 | 9.11 × 10−16 |
| 35 | 1 | 9.22 × 10−16 |
| 30 | 1 | 1.01 × 10−15 |
| 25 | 1 | 1.04 × 10−15 |
| 20 | 1 | 1.16 × 10−15 |
| 15 | 1 | 1.29 × 10−15 |
| 10 | 1 | 1.44 × 10−15 |
| 5 | 1 | 1.63 × 10−15 |
| 0.5 | 1 | 2.03 × 10−15 |
| σ [MPa] | pp [MPa] | k∗t [m3] |
|---|---|---|
| 0 | 10 | 4.62 × 10−15 |
| 5 | 10 | 3.95 × 10−15 |
| 10 | 10 | 3.4 × 10−15 |
| 15 | 10 | 2.83 × 10−15 |
| 20 | 10 | 2.3 × 10−15 |
| 25 | 10 | 1.8 × 10−15 |
| 30 | 10 | 1.41 × 10−15 |
| 35 | 10 | 1.08 × 10−15 |
| 40 | 10 | 8.4 × 10−16 |
| 45 | 10 | 6.58 × 10−16 |
| 40 | 10 | 5.82 × 10−16 |
| 35 | 10 | 6.41 × 10−16 |
| 30 | 10 | 6.84 × 10−16 |
| 25 | 10 | 7.44 × 10−16 |
| 20 | 10 | 8.25 × 10−16 |
| 15 | 10 | 9.3 × 10−16 |
| 10 | 10 | 1.1 × 10−15 |
| 5 | 10 | 1.37 × 10−15 |
| 0 | 10 | 1.93 × 10−15 |
| Step | σ [MPa] | pc [MPa] | T [°C] | pp [MPa] | k∗t [m3] |
|---|---|---|---|---|---|
| I | 0.5 | 5 | 25 | 1 | 1.47 × 10−14 |
| 45 | 5 | 25 | 1 | 8.87 × 10−16 | |
| II | 0.5 | 5 | 25 | 1 | 2.03 × 10−15 |
| III | 0 | 50 | 25 | 1 | 5.39 × 10−16 |
| IV | 30 | 50 | 25 | 1 | 2.44 × 10−16 |
| V | 30 | 50 | 90 | 1 | 1.88 × 10−17 |
| pc [MPa] | pp [MPa] | k∗t [m3] |
|---|---|---|
| 1 | 0.5 | 1.30 × 10−15 |
| 1.5 | 0.5 | 8.44 × 10−16 |
| 2 | 0.5 | 7.63 × 10−16 |
| 5 | 1 | 4.65 × 10−16 |
| Formation | Fluid | σ [MPa] | pc [MPa] | pp [MPa] | k∗t [m3] |
|---|---|---|---|---|---|
| GRW | H2O | 0 | 5 | 1 | 6.55 × 10−15 |
| 1 | 6.08 × 10−15 | ||||
| 5 | 3.89 × 10−15 | ||||
| 10 | 2.75 × 10−15 | ||||
| 15 | 1.77 × 10−15 | ||||
| 20 | 1.37 × 10−15 | ||||
| 25 | 1.07 × 10−16 | ||||
| 30 | 8.26 × 10−16 | ||||
| 35 | 6.55 × 10−16 | ||||
| 40 | 5.44 × 10−16 | ||||
| 45 | 4.12 × 10−16 | ||||
| 40 | 4.14 × 10−16 | ||||
| 35 | 4.19 × 10−16 | ||||
| 30 | 4.20 × 10−16 | ||||
| 25 | 4.26 × 10−16 | ||||
| 20 | 4.56 × 10−16 | ||||
| 15 | 5.02 × 10−16 | ||||
| 10 | 5.53 × 10−16 | ||||
| 5 | 7.00 × 10−16 | ||||
| 1 | 8.26 × 10−16 | ||||
| 0 | 8.68 × 10−16 | ||||
| QTZ_HV | H2O | 0 | 5 | 1 | 3.20 × 10−15 |
| 0.5 | 3.06 × 10−15 | ||||
| 5 | 3.03 × 10−15 | ||||
| 10 | 2.52 × 10−15 | ||||
| 15 | 2.17 × 10−15 | ||||
| 20 | 1.89 × 10−15 | ||||
| 25 | 1.67 × 10−15 | ||||
| 30 | 1.50 × 10−15 | ||||
| 35 | 1.11 × 10−15 | ||||
| 40 | 9.22 × 10−16 | ||||
| 45 | 8.50 × 10−16 | ||||
| 40 | 8.68 × 10−16 | ||||
| 35 | 8.90 × 10−16 | ||||
| 30 | 9.98 × 10−16 | ||||
| 25 | 1.03 × 10−15 | ||||
| 20 | 1.06 × 10−15 | ||||
| 15 | 1.23 × 10−15 | ||||
| 10 | 1.35 × 10−15 | ||||
| 5 | 1.97 × 10−15 | ||||
| 0.5 | 2.32 × 10−15 | ||||
| 0 | 2.03 × 10−15 | ||||
| GRA_DV | H2O | 0 | 5 | 1 | 4.73 × 10−15 |
| 0.5 | 5.72 × 10−15 | ||||
| 5 | 4.44 × 10−15 | ||||
| 10 | 3.13 × 10−15 | ||||
| 15 | 3.30 × 10−15 | ||||
| 20 | 2.48 × 10−15 | ||||
| 25 | 2.16 × 10−15 | ||||
| 30 | 1.88 × 10−15 | ||||
| 35 | 1.63 × 10−15 | ||||
| 40 | 1.47 × 10−15 | ||||
| 45 | 1.16 × 10−15 | ||||
| 40 | 1.20 × 10−15 | ||||
| 35 | 1.17 × 10−15 | ||||
| 30 | 1.20 × 10−15 | ||||
| 25 | 1.27 × 10−15 | ||||
| 20 | 1.37 × 10−15 | ||||
| 15 | 1.28 × 10−15 | ||||
| 10 | 1.29 × 10−15 | ||||
| 5 | 1.52 × 10−15 | ||||
| 0 | 1.84 × 10−15 | ||||
| GOU_DV | Argon | 0 | 14 | 10 | 1.00 × 10−14 |
| 0.5 | 6.86 × 10−15 | ||||
| 5 | 2.85 × 10−15 | ||||
| 10 | 6.17 × 10−16 | ||||
| 15 | 4.69 × 10−17 | ||||
| 20 | 2.14 × 10−17 | ||||
| 15 | 2.84 × 10−17 | ||||
| 10 | 3.02 × 10−17 | ||||
| 5 | 3.22 × 10−17 | ||||
| 0.5 | 3.81 × 10−17 | ||||
| 0 | 3.86 × 10−17 | ||||
| OPA_SD | Argon | 0.5 | 14 | 10 | 7.71 × 10−16 |
| 5 | 6.30 × 10−16 | ||||
| 10 | 4.83 × 10−16 | ||||
| 15 | 3.51 × 10−16 | ||||
| 20 | 2.48 × 10−16 | ||||
| 25 | 1.79 × 10−16 | ||||
| 30 | 1.23 × 10−16 | ||||
| 35 | 8.95 × 10−17 | ||||
| 40 | 6.05 × 10−17 | ||||
| 45 | 4.78 × 10−17 | ||||
| 40 | 3.91 × 10−17 | ||||
| 35 | 4.22 × 10−17 | ||||
| 30 | 4.52 × 10−17 | ||||
| 25 | 4.88 × 10−17 | ||||
| 20 | 5.20 × 10−17 | ||||
| 15 | 6.23 × 10−17 | ||||
| 10 | 7.58 × 10−17 | ||||
| 5 | 1.04 × 10−16 | ||||
| 0.5 | 1.59 × 10−16 | ||||
| 0 | 1.65 × 10−16 | ||||
| OPA_SH | Argon | 0 | 14 | 10 | 8.99 × 10−17 |
| 0.5 | 8.82 × 10−17 | ||||
| 5 | 6.76 × 10−17 | ||||
| 10 | 5.61 × 10−17 | ||||
| 15 | 4.27 × 10−17 | ||||
| 20 | 2.44 × 10−17 | ||||
| 25 | 9.86 × 10−18 | ||||
| 30 | 1.85 × 10−18 | ||||
| 25 | 2.06 × 10−18 | ||||
| 20 | 2.30 × 10−18 | ||||
| 15 | 2.98 × 10−18 | ||||
| 10 | 4.20 × 10−18 | ||||
| 5 | 5.81 × 10−18 | ||||
| 0.5 | 1.13 × 10−17 | ||||
| 0 | 1.24 × 10−17 |
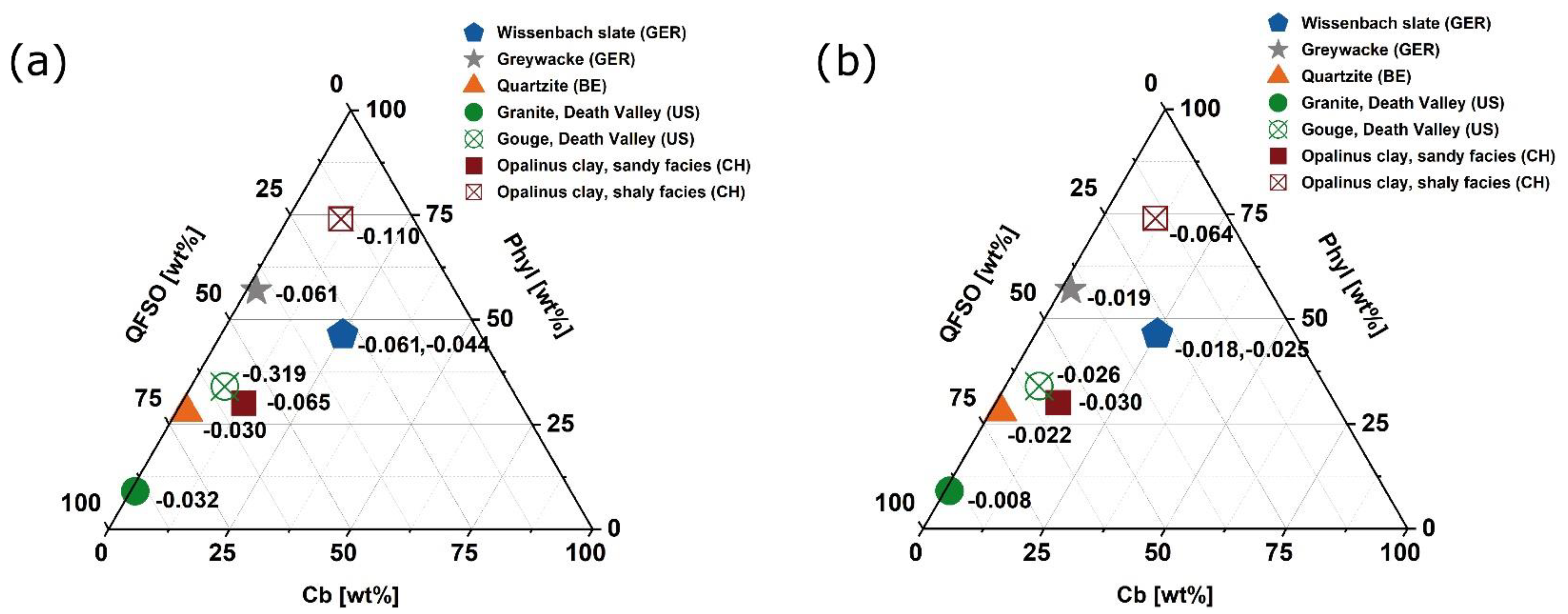
| Sample | α | β | Bcompo |
|---|---|---|---|
| WBS | −0.061 ± 0.002 | −0.018 ± 0.002 | 0.32 |
| WBSAr | −0.044 ± 0.001 | −0.025 ± 0.002 | 0.32 |
| GRW | −0.061 ± 0.003 | −0.019 ± 0.003 | 0.41 |
| QTZ_HV | −0.030 ± 0.001 | −0.022 ± 0.003 | 0.71 |
| GRA_DV | −0.032 ± 0.001 | −0.008 ± 0.002 | 0.90 |
| GOU_DV | −0.319 ± 0.023 | −0.026 ± 0.003 | 0.61 |
| OPA_SD | −0.065 ± 0.001 | −0.030 ± 0.005 | 0.61 |
| OPA_SH | −0.110 ± 0.014 | −0.064 ± 0.008 | 0.16 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Herrmann, J.; Schuster, V.; Cheng, C.; Milsch, H.; Rybacki, E. Fracture Transmissivity in Prospective Host Rocks for Enhanced Geothermal Systems (EGS). Geosciences 2022, 12, 195. https://doi.org/10.3390/geosciences12050195
Herrmann J, Schuster V, Cheng C, Milsch H, Rybacki E. Fracture Transmissivity in Prospective Host Rocks for Enhanced Geothermal Systems (EGS). Geosciences. 2022; 12(5):195. https://doi.org/10.3390/geosciences12050195
Chicago/Turabian StyleHerrmann, Johannes, Valerian Schuster, Chaojie Cheng, Harald Milsch, and Erik Rybacki. 2022. "Fracture Transmissivity in Prospective Host Rocks for Enhanced Geothermal Systems (EGS)" Geosciences 12, no. 5: 195. https://doi.org/10.3390/geosciences12050195
APA StyleHerrmann, J., Schuster, V., Cheng, C., Milsch, H., & Rybacki, E. (2022). Fracture Transmissivity in Prospective Host Rocks for Enhanced Geothermal Systems (EGS). Geosciences, 12(5), 195. https://doi.org/10.3390/geosciences12050195






