Viewpoint on the Integration of Geochemical Processes into Tracer Transport Models for the Marine Environment
Abstract
1. Introduction
2. Materials and Methods
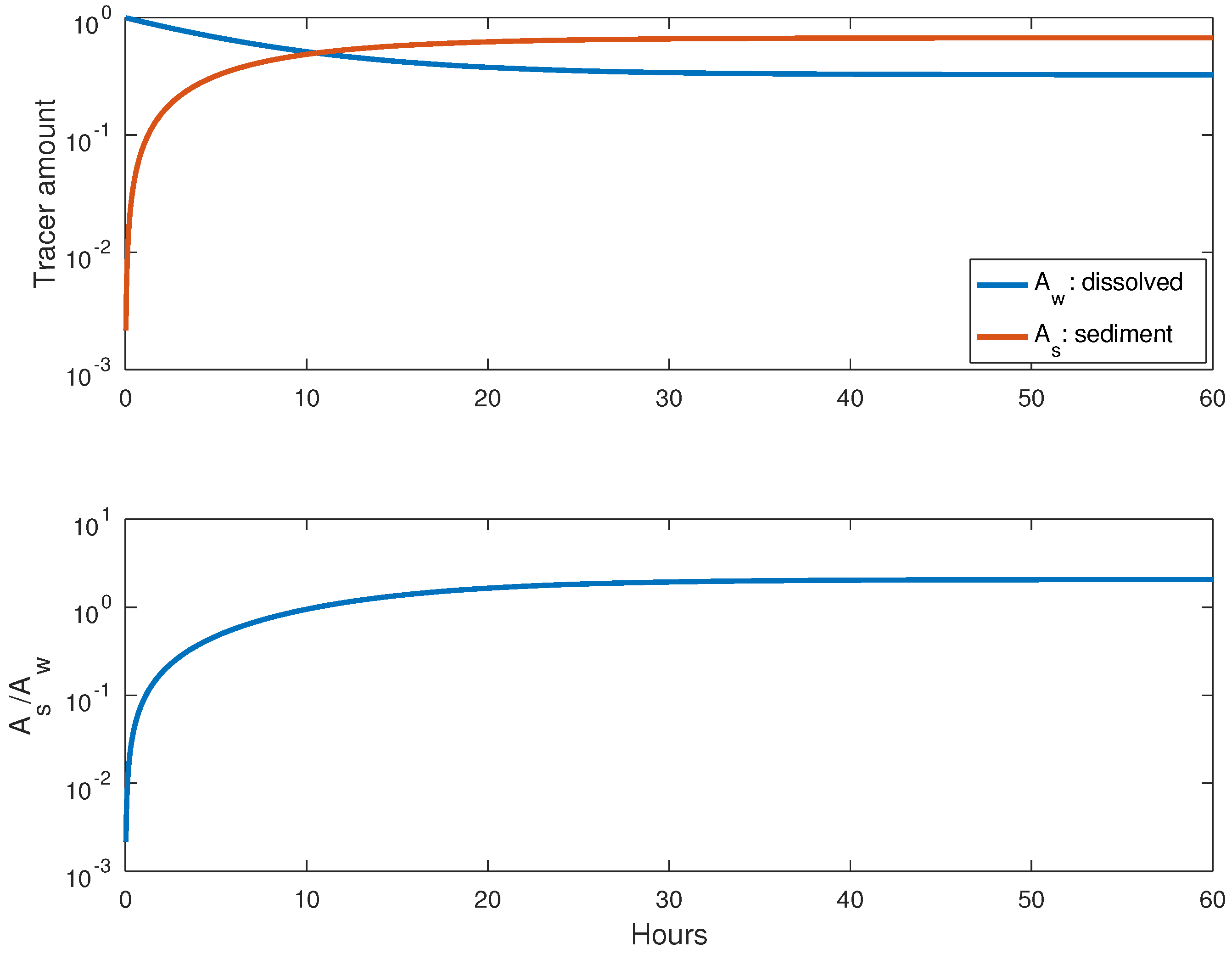
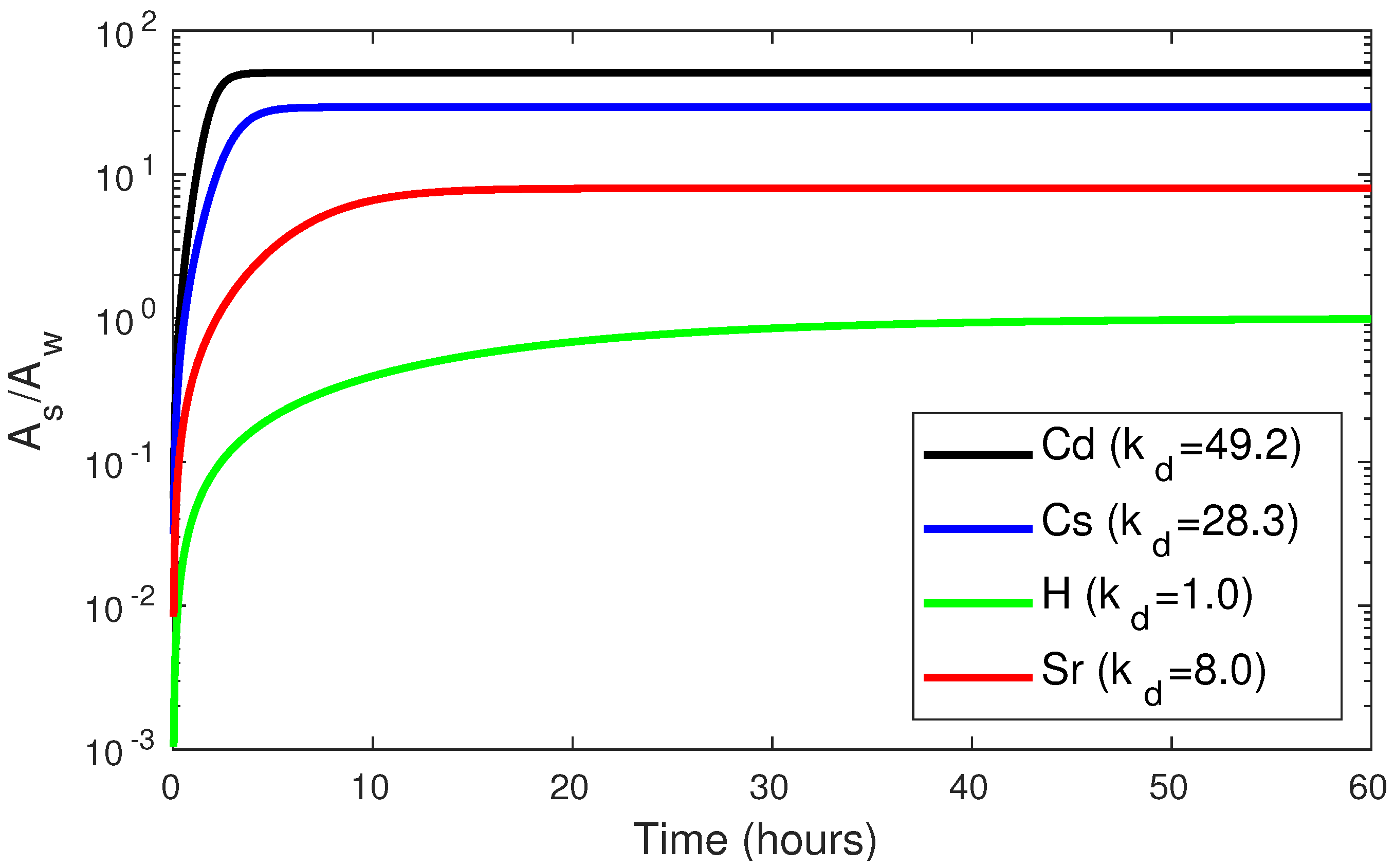
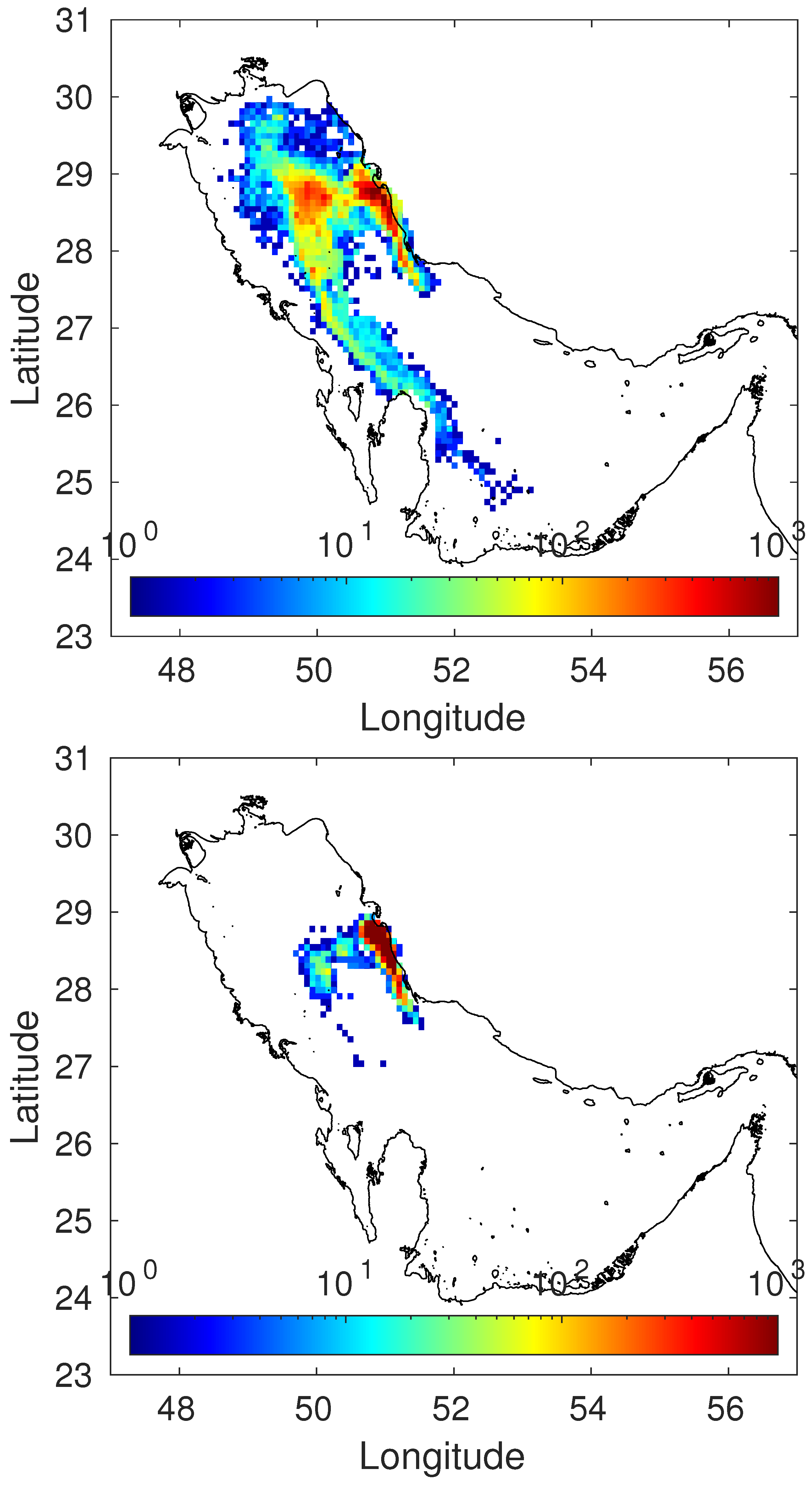
3. Results
4. Conclusions
- Concentrations in the sediments of the release area are overestimated in the short term and underestimated in the long term.
- The mobility of tracers released to the marine environment is overestimated.
- Point 2 implies that concentrations in the sediments at long distances from the release area are also overestimated in the short term.
- The well-known behavior of sediments as a long-term, delayed source of pollutants in the water column cannot be described.
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Periáñez, R. Environmental modelling in the Gulf of Cadiz: Heavy metal distributions in water and sediments. Sci. Total Environ. 2009, 407, 3392–3406. [Google Scholar] [PubMed]
- Periáñez, R. APERTRACK: A particle-tracking model to simulate radionuclide transport in the Arabian/Persian Gulf. Prog. Nucl. Energy 2021, 142, 103998. [Google Scholar] [CrossRef]
- Zhao, C.; Wang, G.; Zhang, M.; Wang, G.; de With, G.; Bezhenar, R.; Maderich, V.; Xia, C.; Zhao, B.; Jung, K.T.; et al. Transport and dispersion scenarios of tritium from the radioactive water of the Fukushima Dai-ichi nuclear plant. Mar. Pollut. Bull. 2021, 169, 112515. [Google Scholar] [CrossRef] [PubMed]
- Periáñez, R.; Qiao, F.; Zhao, C.; de With, G.; Jung, K.; Sangmanee, C.; Wang, G.; Xia, C.; Zhang, M. Opening Fukushima floodgates: Modelling 137Cs impact in marine biota. Mar. Pollut. Bull. 2021, 170, 112645. [Google Scholar] [CrossRef] [PubMed]
- Bezhenar, R.; Takata, H.; de With, G.; Maderich, V. Planned release of contaminated water from the Fukushima storage tanks into the ocean: Simulation scenarios of radiological impact for aquatic biota and human from seafood consumption. Mar. Pollut. Bull. 2021, 173, 112969. [Google Scholar] [CrossRef] [PubMed]
- Hunt, J.; Leonard, K.; Hughes, L. Artificial radionuclides in the Irish Sea from Sellafield: Remobilisation revisited. J. Radiol. Prot. 2013, 33, 261–279. [Google Scholar] [CrossRef] [PubMed]
- Lepicard, S.; Heling, R.; Maderich, V. POSEIDON/RODOS model for radiological assessment of marine environment after accidental releases: Application to coastal areas of the Baltic, Black and North seas. J. Environ. Radioact. 2004, 72, 153–161. [Google Scholar] [CrossRef]
- Iosjpe, M.; Karcher, M.; Gwynn, J.; Harms, I.; Gerdes, R.; Kauker, F. Improvement of the dose assessment tools on the basis of dispersion of the 99Tc in the Nordic Seas and the Arctic Ocean. Radioprotection 2009, 44, 531–536. [Google Scholar] [CrossRef][Green Version]
- Maderich, V.; Bezhenar, R.; Heling, R.; de With, G.; Jung, K.T.; Myoung, J.G.; Cho, Y.K.; Qiao, F.; Robertson, L. Regional long-term model of radioactivity dispersion and fate in the northwestern Pacific and adjacent seas: Application to the Fukushima Dai-ichi accident. J. Environ. Radioact. 2014, 131, 4–18. [Google Scholar] [CrossRef] [PubMed]
- Periáñez, R.; Bezhenar, R.; Brovchenko, I.; Duffa, C.; Iosjpe, M.; Jung, K.T.; Kim, K.O.; Kobayashi, T.; Liptak, L.; Maderich, V.; et al. Marine radionuclide dispersion modelling: Recent developments, problems and challenges. Environ. Model. Softw. 2019, 122, 104523. [Google Scholar] [CrossRef]
- IAEA. Sediment Distribution Coefficients and Concentration Factors for Biota in the Marine Environment; Technical Reports Series; IAEA: Vienna, Austria, 2004; Volume 422. [Google Scholar]
- Periáñez, R. Redissolution and long-term transport of radionuclides released from a contaminated sediment: A numerical modelling study. Estuar. Coast. Shelf Sci. 2003, 56, 5–14. [Google Scholar] [CrossRef]
- Periáñez, R.; Brovchenko, I.; Jung, K.T.; Kim, K.O.; Maderich, V. The marine kd and water/sediment interaction problem. J. Environ. Radioact. 2018, 192, 635–647. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, K.; Tagami, K.; Uchida, S.; Ishii, N. Model estimation of 137-Cs concentration change with time in seawater and sediment around the Fukushima Daiichi Nuclear Power Plant site considering fast and slow reactions in the seawater-sediment systems. J. Radioanal. Nucl. Chem. 2015, 304, 867–881. [Google Scholar] [CrossRef]
- Kusakabe, M.; Takata, H. Temporal trends of 137-Cs concentration in seawaters and bottom sediments in coastal waters around Japan: Implications for the Kd concept in the dynamic marine environment. J. Radioanal. Nucl. Chem. 2020, 323, 567–580. [Google Scholar] [CrossRef]
- Maderich, V.; Jung, K.T.; Brovchenko, I.; Kim, K.O. Migration of radioactivity in multi-fraction sediments. Environ. Fluid Mech. 2017, 17, 1207–1231. [Google Scholar] [CrossRef]
- Otosaka, S.; Kambayashi, S.; Fukuda, M.; Tsuruta, T.; Misonou, T.; Suzuki, T.; Aono, T. Behavior of radiocesium in sediments in Fukushima coastal waters: Verification of desorption potential through pore water. Environ. Sci. Technol. 2020, 54, 13778–13785. [Google Scholar] [CrossRef]
- Vives i Batlle, J.; Beresford, N.; Beaugelin-Seiller, K.; Bezhenar, R.; Brown, J.; Cheng, J.J.; Cujic, M.; Dragovic, S.S.; Duffa, C.; Fievet, B.; et al. Inter-comparison of dynamic models for radionuclide transfer to marine biota in a Fukushima accident scenario. J. Environ. Radioact. 2016, 153, 31–50. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Periáñez, R. Viewpoint on the Integration of Geochemical Processes into Tracer Transport Models for the Marine Environment. Geosciences 2022, 12, 152. https://doi.org/10.3390/geosciences12040152
Periáñez R. Viewpoint on the Integration of Geochemical Processes into Tracer Transport Models for the Marine Environment. Geosciences. 2022; 12(4):152. https://doi.org/10.3390/geosciences12040152
Chicago/Turabian StylePeriáñez, Raúl. 2022. "Viewpoint on the Integration of Geochemical Processes into Tracer Transport Models for the Marine Environment" Geosciences 12, no. 4: 152. https://doi.org/10.3390/geosciences12040152
APA StylePeriáñez, R. (2022). Viewpoint on the Integration of Geochemical Processes into Tracer Transport Models for the Marine Environment. Geosciences, 12(4), 152. https://doi.org/10.3390/geosciences12040152





