Successive Modes of Carbonate Precipitation in Microbialites along the Hydrothermal Spring of La Salsa in Laguna Pastos Grandes (Bolivian Altiplano)
Abstract
:1. Introduction
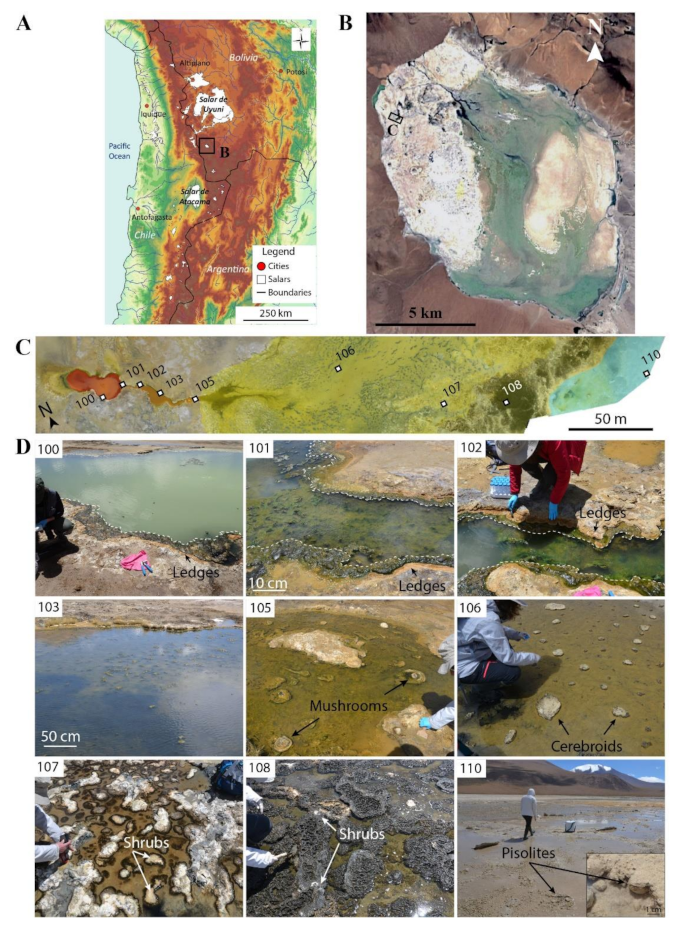
2. Geological Setting
3. Previous Results on La Salsa Spring
4. Materials and Methods
4.1. Sampling and Analytical Methods
4.2. PHREEQC Thermodynamic Modeling
4.3. Workflow of DIC Isotopic Mass Balance Calculations
4.4. Microbial Mat Fixation and Microscopy Methods
5. Results
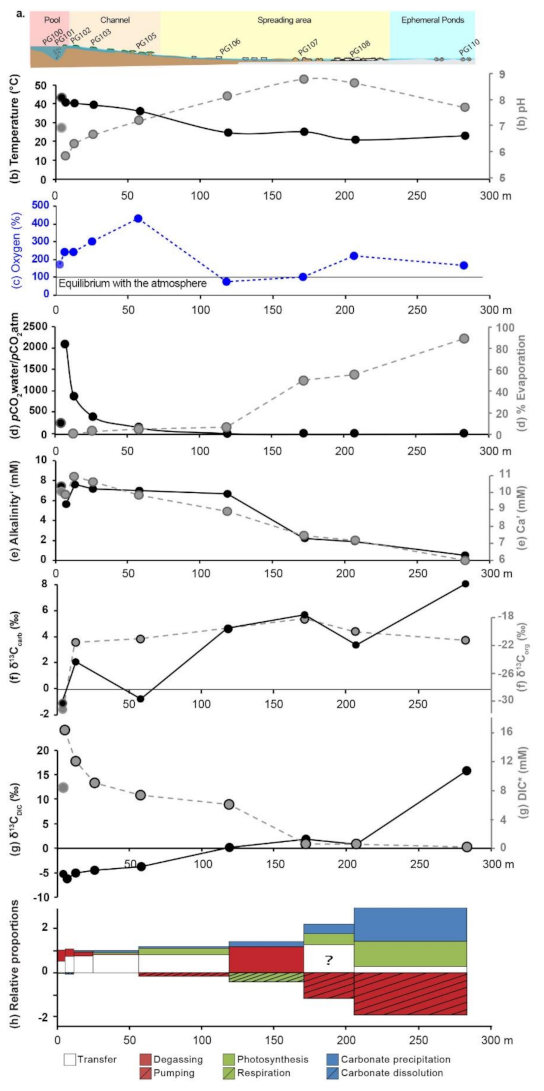
5.1. Water Chemistry along La Salsa Spring
5.2. DIC Isotope Mass Balance
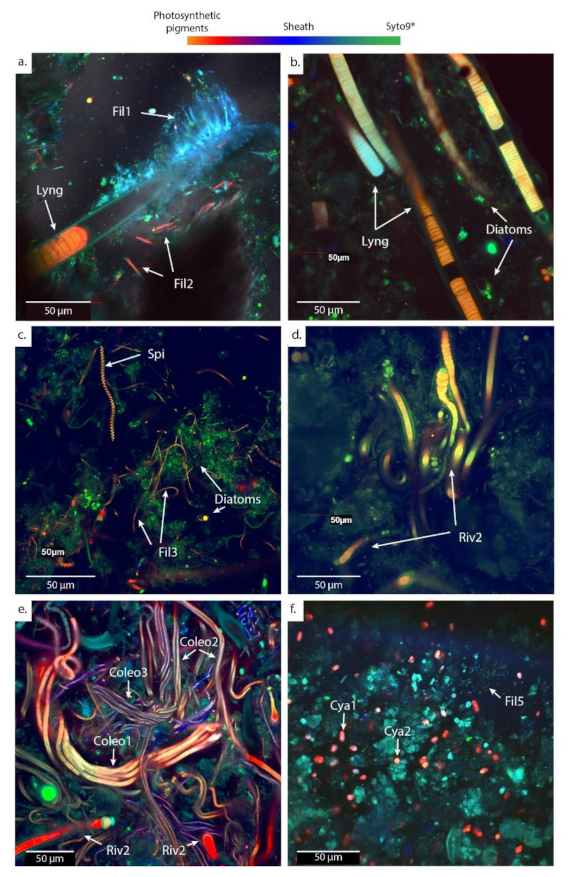
5.3. Morphology-Based Assessment of Dominant Cyanobacteria
5.4. Mineral–Microorganism Interactions
6. Discussion
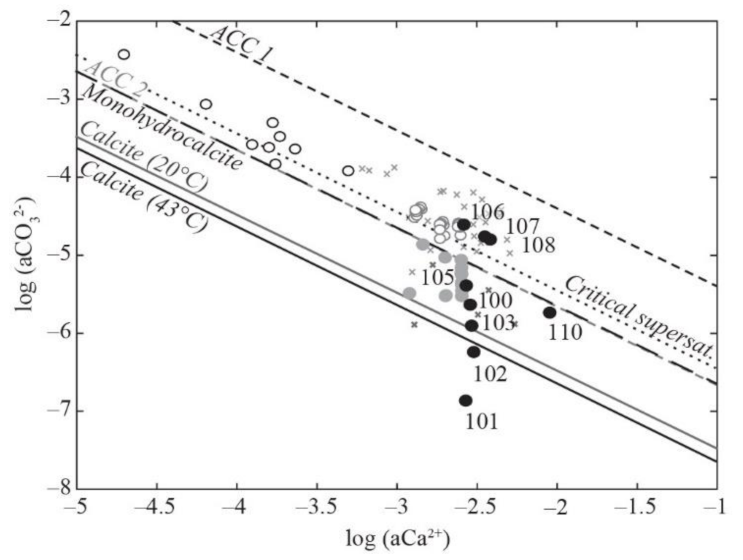
6.1. Calcification of Microbial Mats in Solutions Undersaturated with Calcite
6.2. Pervasively Calcified Microbial Mats in Calcite Supersaturated Waters
6.3. Calcite Precipitation in Hypersaline Saturated Waters
7. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Burne, R.V.; Moore, L.S. Microbialites; organosedimentary deposits of benthic microbial communities. Palaios 1987, 2, 241–254. [Google Scholar] [CrossRef]
- Grotzinger, J.P.; Knoll, A.H. Stromatolites in Precambrian carbonates: Evolutionary mileposts or environmental dipsticks? Annu. Rev. Earth Planet. Sci. 1999, 27, 313–358. [Google Scholar] [CrossRef] [PubMed]
- Fouke, B.W.; Farmer, J.D.; Des Marais, D.J.; Pratt, L.; Sturchio, N.C.; Burns, P.C.; Discipulo, M.K. Depositional facies and aqueous-solid geochemistry of travertine-depositing hot springs (Angel Terrace, Mammoth Hot Springs, Yellowstone National Park, USA). J. Sed. Res. 2000, 70, 565–585. [Google Scholar] [CrossRef] [PubMed]
- Shiraishi, F.; Hanzawa, Y.; Nakamura, Y.; Eno, Y.; Morikawa, A.; de Mattos, R.F.; Asada, J.; Cury, L.F.; Bahniuk, A.M. Abiotic and biotic processes controlling travertine deposition: Insights from eight hot springs in Japan. Sedimentology 2021, 69, 592–623. [Google Scholar] [CrossRef]
- Della Porta, G.; Hoppert, M.; Hallmann, C.; Schneider, D.; Reitner, J. The influence of microbial mats on travertine precipitation in active hydrothermal systems (Central Italy). Depos. Rec. 2021, 1–45. [Google Scholar] [CrossRef]
- Laval, B.; Cady, S.L.; Pollack, J.C.; McKay, C.P.; Bird, J.S.; Grotzinger, J.P.; Ford, D.C.; Bohm, H.R. Modern freshwater microbialite analogues for ancient dendritic reef structures. Nature 2000, 407, 626–629. [Google Scholar] [CrossRef] [PubMed]
- Arp, G.; Wedemeyer, N.; Reitner, J. Fluvival tufa formation in a hard-water creek (Deinschwanger Bach, Franconian Alb, Germany). Facies 2001, 44, 1–22. [Google Scholar] [CrossRef]
- Roche, A.; Vennin, E.; Bundeleva, I.; Bouton, A.; Payandi-Rolland, D.; Amiotte-Suchet, P.; Gaucher, E.C.; Courvoisier, H.; Visscher, P.T. The role of the substrate on the mineralization potential of microbial mats in a modern freshwater River (Paris Basin, France). Minerals 2019, 9, 359. [Google Scholar] [CrossRef] [Green Version]
- Arp, G.; Helms, G.; Karlinska, K.; Schumann, G.; Reimer, A.; Reitner, J.; Trichet, J. Photosynthesis versus exopolymer degradation in the formation of microbialites on the atoll of Kiritimati, Republic of Kiribati, Central Pacific. Geomicrobiol. J. 2012, 29, 29–65. [Google Scholar] [CrossRef]
- Pace, A.; Bourillot, R.; Bouton, A.; Vennin, E.; Galaup, S.; Bundeleva, I.; Patrier, P.; Dupraz, C.; Thomazo, C.; Sansjofre, P.; et al. Microbial and diagenetic steps leading to the mineralisation of Great Salt Lake microbialites. Sci. Rep. 2016, 6, 31495. [Google Scholar] [CrossRef] [Green Version]
- Zeyen, N.; Benzerara, K.; Beyssac, O.; Daval, D.; Muller, E.; Thomazo, C.; Tavera, R.; López-García, P.; Moreira, D.; Duprat, E. Integrative analysis of the mineralogical and chemical composition of modern microbialites from ten Mexican lakes: What do we learn about their formation? GCA 2021, 305, 148–184. [Google Scholar] [CrossRef]
- Harris, P.M.M.; Ellis, J.; Purkis, S.J. Assessing the extent of carbonate deposition in early rift settings Carbonate Deposition in Early Rift Settings. AAPG Bull. 2013, 97, 27–60. [Google Scholar] [CrossRef]
- Chagas, A.A.P.; Webb, G.E.; Burne, R.V.; Southam, G. Modern lacustrine microbialites: Towards a synthesis of aqueous and carbonate geochemistry and mineralogy. Earth-Sci. Rev. 2016, 162, 338–363. [Google Scholar] [CrossRef]
- Falkowski, P.G.; Fenchel, T.; Delong, E.F. The microbial engines that drive Earth’s biogeochemical cycles. Science 2008, 320, 1034–1039. [Google Scholar] [CrossRef] [Green Version]
- Dupraz, C.; Visscher, P.T. Microbial lithification in marine stromatolites and hypersaline mats. Trends Microbiol. 2005, 13, 429–438. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gérard, E.; Ménez, B.; Couradeau, E.; Moreira, D.; Benzerara, K.; Tavera, R.; López-García, P. Specific carbonate–microbe interactions in the modern microbialites of Lake Alchichica (Mexico). ISME J. 2013, 7, 1997. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gomez, F.J.; Mlewski, C.; Boidi, F.J.; Farías, M.E.; Gérard, E. Calcium carbonate precipitation in diatom-rich microbial mats: The Laguna Negra hypersaline lake, Catamarca, Argentina. J. Sediment. Res. 2018, 88, 727–742. [Google Scholar] [CrossRef]
- Shiraishi, F.; Bissett, A.; de Beer, D.; Reimer, A.; Arp, G. Photosynthesis, respiration and exopolymer calcium-binding in biofilm calcification (Westerhöfer and Deinschwanger Creek, Germany). Geomicrobiol. J. 2008, 25, 83–94. [Google Scholar] [CrossRef]
- Shiraishi, F.; Reimer, A.; Bissett, A.; de Beer, D.; Arp, G. Microbial effects on biofilm calcification, ambient water chemistry and stable isotope records in a highly supersaturated setting (Westerhöfer Bach, Germany). Palaeogeogr. Palaeoclimatol. Palaeoecol. 2008, 262, 91–106. [Google Scholar] [CrossRef]
- Bissett, A.; Reimer, A.; de Beer, D.; Shiraishi, F.; Arp, G. Metabolic microenvironmental control by photosynthetic biofilms under changing macroenvironmental temperature and pH conditions. Appl. Environ. Microbiol. 2008, 74, 6306–6312. [Google Scholar] [CrossRef] [Green Version]
- Dupraz, C.; Reid, R.P.; Braissant, O.; Decho, A.W.; Norman, R.S.; Visscher, P.T. Processes of carbonate precipitation in modern microbial mats. Earth-Sci. Rev. 2009, 96, 141–162. [Google Scholar] [CrossRef]
- Couradeau, E.; Benzerara, K.; Gérard, E.; Moreira, D.; Bernard, S.; Brown, G.E.; López-García, P. An early-branching microbialite cyanobacterium forms intracellular carbonates. Science 2012, 336, 459–462. [Google Scholar] [CrossRef] [Green Version]
- Gérard, E.; De Goeyse, S.; Hugoni, M.; Agogué, H.; Richard, L.; Milesi, V.; Guyot, F.; Lecourt, L.; Borensztajn, S.; Joseph, M.-B.; et al. Key role of alphaproteobacteria and cyanobacteria in the formation of stromatolites of Lake Dziani Dzaha (Mayotte, Western Indian Ocean). Front. Microbiol. 2018, 9, 796. [Google Scholar] [CrossRef] [Green Version]
- Decho, A.W. Microbial exopolymer secretions in ocean environments: Their role(s) in food webs and marine processes. Oceanogr. Mar. Biol. Annu. Rev. 1990, 28, 73–153. [Google Scholar]
- Benzerara, K.; Menguy, N.; López-García, P.; Yoon, T.H.; Kazmierczak, J.; Tyliszczak, T.; Guyot, F.; Brown, G.E., Jr. Nanoscale detection of organic signatures in carbonate microbialites. Proc. Natl. Acad. Sci. USA 2006, 103, 9440–9445. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Braissant, O.; Decho, A.W.; Przekop, K.M.; Gallagher, K.L.; Glunk, C.; Dupraz, C.; Visscher, P.T. Characteristics and turnover of exopolymeric substances in a hypersaline microbial mat. FEMS Microbiol. Ecol. 2009, 67, 293–307. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bougeault, C.; Vennin, E.; Durlet, C.; Muller, E.; Mercuzot, M.; Chavez, M.; Gérard, E.; Ader, M.; Virgone, A.; Gaucher, E.C. Biotic–Abiotic Influences on Modern Ca–Si-Rich Hydrothermal Spring Mounds of the Pastos Grandes Volcanic Caldera (Bolivia). Minerals 2019, 9, 380. [Google Scholar] [CrossRef] [Green Version]
- Bougeault, C.; Durlet, C.; Vennin, E.; Muller, E.; Ader, M.; Ghaleb, B.; Gérard, E.; Virgone, A.; Gaucher, E.C. Variability of Carbonate Isotope Signatures in a Hydrothermally Influenced System: Insights from the Pastos Grandes Caldera (Bolivia). Minerals 2020, 10, 989. [Google Scholar] [CrossRef]
- Risacher, F.; Eugster, H.P. Holocene pisoliths and encrustations associated with spring-fed surface pools, Pastos Grandes, Bolivia. Sedimentology 1979, 26, 253–270. [Google Scholar] [CrossRef]
- Salisbury, M.J.; Jicha, B.R.; de Silva, S.L.; Singer, B.S.; Jiménez, N.C.; Ort, M.H. 40Ar/39Ar chronostratigraphy of Altiplano-Puna volcanic complex ignimbrites reveals the development of a major magmatic province. Bulletin 2011, 123, 821–840. [Google Scholar] [CrossRef]
- De Silva, S.L.; Francis, P.W. Volcanoes of the Central Andes; Springer: Berlin, Germany, 1991. [Google Scholar]
- Peel, M.C.; Finlayson, B.L.; McMahon, T.A. Updated world map of the Köppen-Geiger climate classification. Hydrol. Earth Syst. Sci. 2007, 4, 439–473. [Google Scholar] [CrossRef] [Green Version]
- Risacher, F.; Fritz, B. Geochemistry of Bolivian salars, Lipez, southern Altiplano: Origin of solutes and brine evolution. Geochim. Cosmochim. Acta 1991, 55, 687–705. [Google Scholar] [CrossRef]
- Jones, B.; Renaut, R.W. Crystal fabrics and microbiota in large pisoliths from Laguna Pastos Grandes, Bolivia. Sedimentology 1994, 41, 1171–1202. [Google Scholar] [CrossRef]
- Kasemann, S.A.; Meixner, A.; Erzinger, J.; Viramonte, J.G.; Alonso, R.N.; Franz, G. Boron isotope composition of geothermal fluids and borate minerals from salar deposits (central Andes/NW Argentina). J. S. Am. Earth Sci. 2004, 16, 685–697. [Google Scholar] [CrossRef]
- Ahlfeld, F. Sodaseen in Lipez (Bolivien). Neues Jb. Miner. Mh. 1956, 6, 128–136. [Google Scholar]
- Ballivian, O.; Risacher, F. Los Salares del Altiplano Boliviano: Métodos de Estudio y Estimación Económica; ORSTOM: Paris, France, 1981; 246p. [Google Scholar]
- Muller, E.; Gaucher, E.C.; Durlet, C.; Moquet, J.; Moreira, M.; Rouchon, V.; Louvat, P.; Bardoux, G.; Noirez, S.; Bougeault, C.; et al. The Origin of Continental Carbonates in Andean Salars: A Multi-Tracer Geochemical Approach in Laguna Pastos Grandes (Bolivia). Geochim. Cosmochim. Acta 2020, 279, 220–237. [Google Scholar] [CrossRef] [Green Version]
- Assayag, N.; Jézéquel, D.; Ader, M.; Viollier, E.; Michard, G.; Prévot, F.; Agrinier, P. Hydrological budget, carbon sources and biogeochemical processes in Lac Pavin (France): Constraints from δ18O of water and δ13C of dissolved inorganic carbon. Appl. Geochem. 2008, 23, 2800–2816. [Google Scholar] [CrossRef]
- Assayag, N.; Rivé, K.; Ader, M.; Jézéquel, D.; Agrinier, P. Improved method for isotopic and quantitative analysis of dissolved inorganic carbon in natural water samples. Rapid Commun. Mass Spectrom. 2006, 20, 2243–2251. [Google Scholar] [CrossRef]
- Lebeau, O.; Busigny, V.; Chaduteau, C.; Ader, M. Organic matter removal for the analysis of carbon and oxygen isotope compositions of siderite. Chem. Geol. 2014, 372, 54–61. [Google Scholar] [CrossRef]
- Parkhurst, D.L.; Appelo, C.A.J. Description of input and examples for PHREEQC version 3–a computer program for speciation, batch-reaction, one-dimensional transport, and inverse geochemical calculations. US Geol. Surv. Tech. Methods 2013, 6, 497. [Google Scholar]
- Blanc, P.; Lassin, A.; Piantone, P.; Azaroual, M.; Jacquemet, N.; Fabbri, A.; Gaucher, E.C. Thermoddem: A geochemical database focused on low temperature water/rock interactions and waste materials. Appl. Geochem. 2012, 27, 2107–2116. [Google Scholar] [CrossRef]
- Blair, N.E.A.L.; Leu, A.; Olsen, J.; Kwong, E.; Des Marais, D. Carbon isotopic fractionation in heterotrophic microbial metabolism. Appl. Environ. Microbiol. 1985, 50, 996–1001. [Google Scholar] [CrossRef] [Green Version]
- Mook, W.G. 13C in atmospheric CO2. Neth. J. Sea Res. 1986, 20, 211–223. [Google Scholar] [CrossRef]
- Zeebe, R.E.; Wolf-Gladrow, D. CO2 in Seawater: Equilibrium, Kinetics, Isotopes; Halpern, D., Ed.; Elsevier Oceanography Series No. 65; Elsevier: Amsterdam, The Netherlands, 2001. [Google Scholar]
- Anderson, G.M. Error propagation by the Monte Carlo method in geochemical calculations. Geochim. Cosmochim. Acta 1976, 40, 1533–1538. [Google Scholar] [CrossRef]
- Beeler, S.R.; Gomez, F.J.; Bradley, A.S. Controls of extreme isotopic enrichment in modern microbialites and associated abiogenic carbonates. Geochim. Cosmochim. Acta 2020, 269, 136–149. [Google Scholar] [CrossRef]
- Brecevic, L.; Nielsen, A.E. Solubility of amorphous calcium carbonate. J. Cryst. Growth 1989, 98, 504–510. [Google Scholar] [CrossRef]
- Kellermeier, M.; Picker, A.; Kempter, A.; Cölfen, H.; Gebauer, D. A straightforward treatment of activity in aqueous CaCO3 solutions and the consequences for nucleation theory. J. Adv. Mater. 2014, 26, 752–757. [Google Scholar] [CrossRef] [PubMed]
- Fukushi, K.; Matsumiya, H. Control of Water Chemistry in Alkaline Lakes: Solubility of Monohydrocalcite and Amorphous Magnesium Carbonate in CaCl2–MgCl2–Na2CO3 Solutions. ACS Earth Space Chem. 2018, 2, 735–744. [Google Scholar] [CrossRef]
- Plummer, L.N.; Busenberg, E. The solubilities of calcite, aragonite and vaterite in CO2-H2O solutions between 0 and 90 °C, and an evaluation of the aqueous model for the system CaCO3-CO2-H2O. Geochim. Cosmochim. Acta 1982, 46, 1011–1040. [Google Scholar] [CrossRef]
- Arp, G.; Reimer, A.; Reitner, J. Photosynthesis-induced biofilm calcification and calcium concentrations in Phanerozoic oceans. Science 2001, 292, 1701–1704. [Google Scholar] [CrossRef] [Green Version]
- Castenholz, R.W.; Wilmotte, A.; Herdman, M.; Rippka, R.; Waterbury, J.B.; Iteman, I.; Hoffmann, L. Phylum BX. cyanobacteria. In Bergey’s Manual® of Systematic Bacteriology; Springer: New York, NY, USA, 2001; pp. 473–599. [Google Scholar]
- Siegesmund, M.A.; Johansen, J.R.; Karsten, U.; Friedl, T. Coleofasciculus gen. nov. (Cyanobacteria): Morphological and Molecular Criteria for Revision of the Genus Microcoleus Gomont1. J. Phycol. 2008, 44, 1572–1585. [Google Scholar] [CrossRef] [PubMed]
- Giuffre, A.J.; Hamm, L.M.; Han, N.; De Yoreo, J.J.; Dove, P.M. Polysaccharide chemistry regulates kinetics of calcite nucleation through competition of interfacial energies. Proc. Natl. Acad. Sci. USA 2013, 110, 9261–9266. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schneider, J.; Le Campion-Alsumard, T. Construction and destruction of carbonates by marine and freshwater cyanobacteria. Eur. J. Phycol. 1999, 34, 417–426. [Google Scholar] [CrossRef]
- Golubić, S. The relationship between blue-green algae and carbonate deposits. In The Biology of Blue-Green Algae; Carr, N.G., Whitton, B.A., Eds.; University of California Press: Oakland, CA, USA, 1973; Volume 9, pp. 434–472. [Google Scholar]
- Fouke, B.W. Hot-spring Systems Geobiology: Abiotic and biotic influences on travertine formation at Mammoth Hot Springs, Yellowstone National Park, USA. Sedimentology 2011, 58, 170–219. [Google Scholar] [CrossRef]
- Arp, G.; Bissett, A.; Brinkmann, N.; Cousin, S.; De Beer, D.; Friedl, T.; Mohr, K.I.; Neu, T.R.; Reimer, A.; Shiraishi, F.; et al. Tufa-forming biofilms of German karstwater streams: Microorganisms, exopolymers, hydrochemistry and calcification. Geol. Soc. London Spec. Publ. 2010, 336, 83–118. [Google Scholar] [CrossRef]
- Arp, G.; Hofmann, J.; Reitner, J. Microbial fabric formation in spring mounds (“microbialites”) of alkaline salt lakes in the Badain Jaran sand sea, PR China. Palaios 1998, 13, 581–592. [Google Scholar] [CrossRef] [Green Version]
- Obst, M.; Dynes, J.J.; Lawrence, J.R.; Swerhone, G.D.W.; Benzerara, K.; Karunakaran, C.; Kaznatcheeva, K.; Tyliszczak, T.; Hitchcock, A.P. Precipitation of amorphous CaCO3 (aragonite-like) by cyanobacteria: A STXM study of the influence of EPS on the nucleation process. Geochim. Cosmochim. Acta 2009, 73, 4180–4198. [Google Scholar] [CrossRef]
- Messabeb, H.; Contamine, F.; Cézac, P.; Serin, J.P.; Pouget, C.; Gaucher, E.C. Experimental Measurement of CO2 Solubility in Aqueous CaCl2 Solution at Temperature from 323.15 to 423.15 K and Pressure up to 20 MPa Using the Conductometric Titration. J. Chem. Eng. Data 2017, 62, 4228–4234. [Google Scholar] [CrossRef]
- Gomez, F.J.; Kah, L.C.; Bartley, J.K.; Astini, R.A. Microbialites in a high-altitude Andean lake: Multiple controls on carbonate precipitation and lamina accretion. Palaios 2014, 29, 233–249. [Google Scholar] [CrossRef]
- Mlewski, E.C.; Pisapia, C.; Gomez, F.; Lecourt, L.; Soto Rueda, E.; Benzerara, K.; Menez, B.; Borensztajn, S.; Jamme, F.; Réfrégiers, M.; et al. Characterization of pustular mats and related rivularia-rich laminations in oncoids from the Laguna Negra lake (Argentina). Front. Microbiol. 2018, 9, 996. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez-Blanco, J.D.; Shaw, S.; Bots, P.; Roncal-Herrero, T.; Benning, L.G. The role of Mg in the crystallization of monohydrocalcite. Geochim. Cosmochim. Acta 2014, 127, 204–220. [Google Scholar] [CrossRef]
- Blue, C.R.; Giuffre, A.; Mergelsberg, S.; Han, N.; De Yoreo, J.J.; Dove, P.M. Chemical and physical controls on the transformation of amorphous calcium carbonate into crystalline CaCO3 polymorphs. Geochim. Cosmochim. Acta 2017, 196, 179–196. [Google Scholar] [CrossRef] [Green Version]
- Mergelsberg, S.T.; De Yoreo, J.J.; Miller, Q.R.S.; Marc Michel, F.; Ulrich, R.N.; Dove, P.M. Metastable solubility and local structure of amorphous calcium carbonate (ACC). Geochim. Cosmochim. Acta 2020, 289, 196–206. [Google Scholar] [CrossRef]
- Mercedes-Martín, R.; Rogerson, M.; Prior, T.J.; Brasier, A.T.; Reijmer, J.J.; Billing, I.; Matthews, A.; Love, T.; Lepley, S.; Pedley, M. Towards a morphology diagram for terrestrial carbonates: Evaluating the impact of carbonate supersaturation and alginic acid in calcite precipitate morphology. Geochim. Cosmochim. Acta 2021, 306, 340–361. [Google Scholar] [CrossRef]
- Purgstaller, B.; Mavromatis, V.; Immenhauser, A.; Dietzel, M. Transformation of Mg-bearing amorphous calcium carbonate to Mg-calcite–In situ monitoring. Geochim. Cosmochim. Acta 2016, 174, 180–195. [Google Scholar] [CrossRef]
- Buongiorno, J.; Gomez, F.J.; Fike, D.A.; Kah, L.C. Mineralized microbialites as archives of environmental evolution, Laguna Negra, Catamarca Province, Argentina. Geobiology 2019, 17, 199–222. [Google Scholar] [CrossRef] [PubMed]
- Valero-Garcés, B.L.; Delgado-Huertas, A.; Ratto, N.; Navas, A. Large 13C enrichment in primary carbonates from Andean Altiplano lakes, northwest Argentina. Earth Planet. Sci. Lett. 1999, 171, 253–266. [Google Scholar] [CrossRef] [Green Version]
- Pentecost, A.; Spiro, B. Stable carbon and oxygen isotope composition of calcites associated with modern freshwater cyanobacteria and algae. Geomicrobiol. J. 1990, 8, 17–26. [Google Scholar] [CrossRef]
- Chaffaut, I.; Coudrain-Ribstein, A.; Michelot, J.L.; Pouyaud, B. Précipitations d’altitude du Nord-Chili, origine des sources de vapeur et données isotopiques. Bull. Inst. Fr. Etudes Andin. 1998, 27, 367–384. (In French) [Google Scholar]


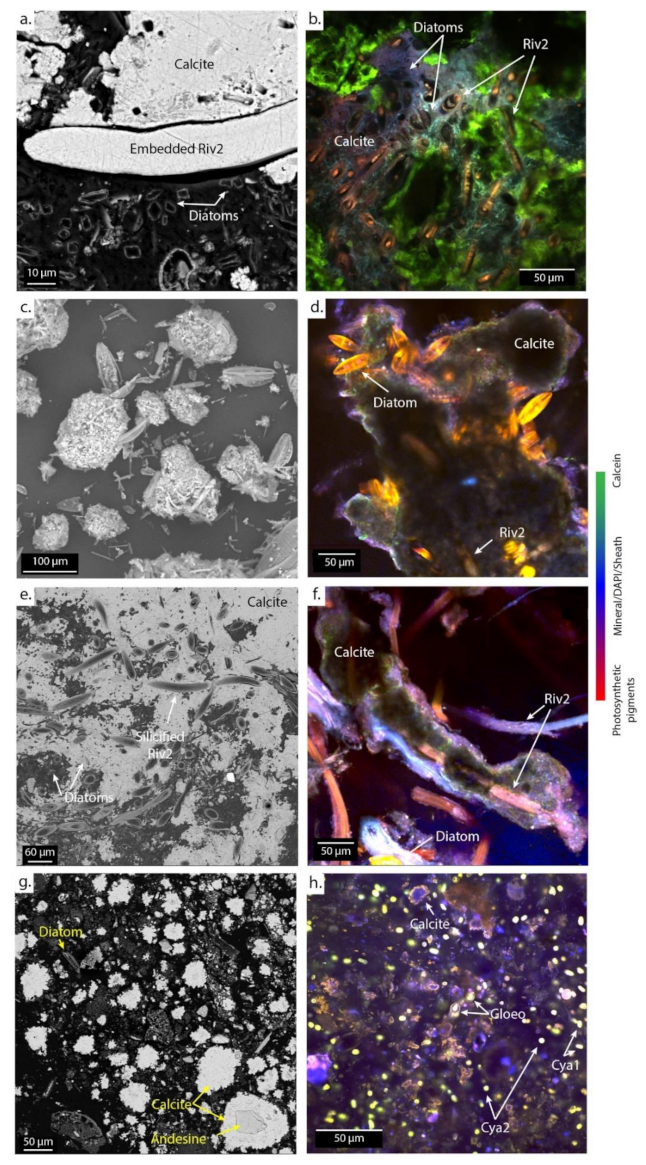
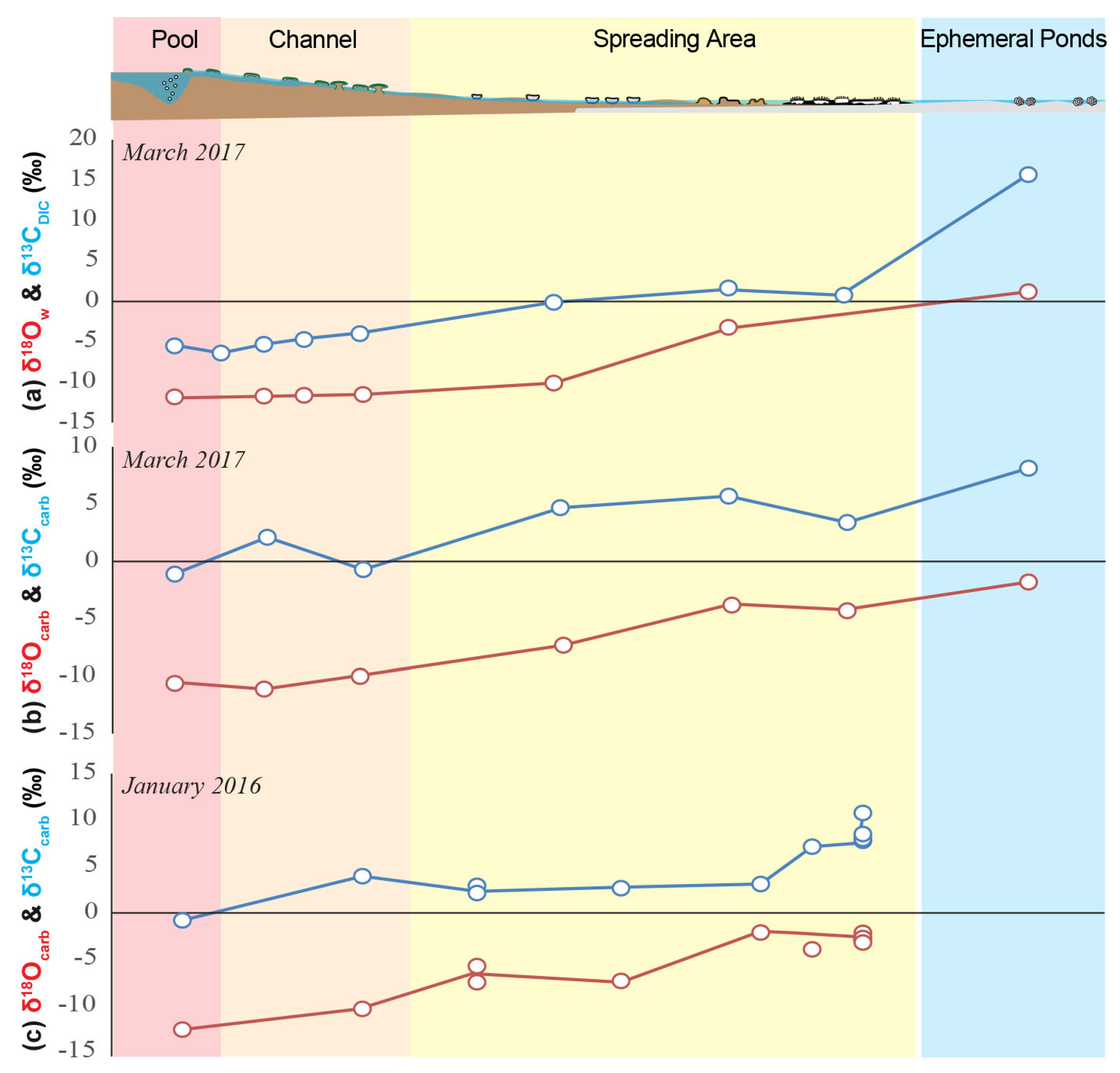
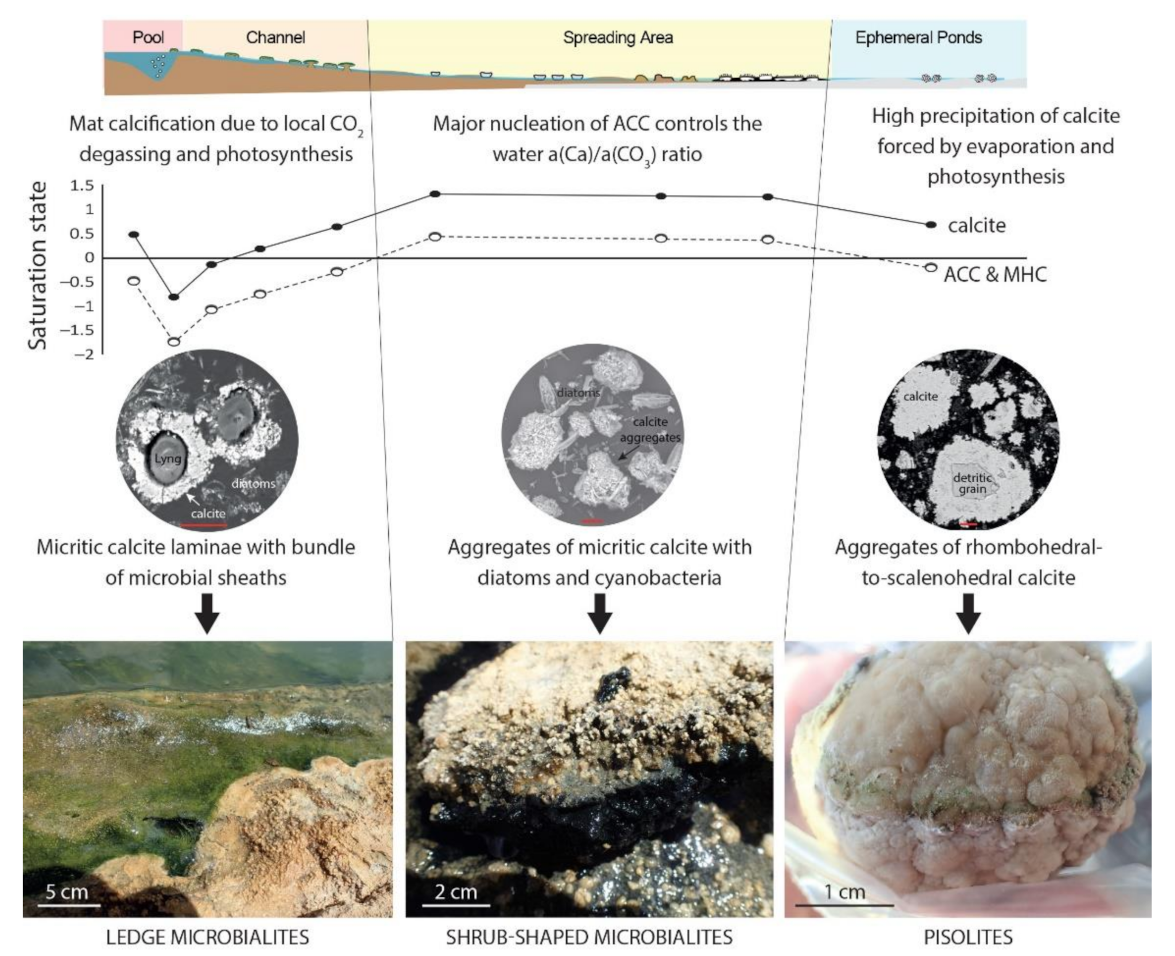
| Point | PG100 | PG101 | PG102 | PG103 | PG105 | PG106 | PG107 | PG108 | PG110 |
|---|---|---|---|---|---|---|---|---|---|
| Latitude | 21.61934 | 21.61934 | 21.619367 | 21.619475 | 21.619594 | 21.619651 | 21.620015 | 21.620123 | 21.620351 |
| Longitude | 67.84846 | 67.848348 | 67.848299 | 67.84822 | 67.847936 | 67.84732 | 67.846932 | 67.846605 | 67.84591 |
| Distance (m) | 4 | 7 | 13 | 26 | 58 | 119 | 172 | 207 | 283 |
| pH (±0.1) | 6.9 | 5.8 | 6.3 | 6.6 | 7.2 | 8.1 | 8.8 | 8.6 | 7.7 |
| Oxygen (±0.1%) | 166 | 238 | 235 | 297 | 426 | 69 | 94 | 215 | 160 |
| Temperature (±0.3 °C) | 43.4 | 40.9 | 40.3 | 39.4 | 36.1 | 24.6 | 25.0 | 20.7 | 22.9 |
| Cond. (mS/cm ± 0.5%) | 26.4 | 26.7 | 26.3 | 26.9 | 27.2 | 27.0 | 31.9 | 48.0 | 156.3 |
| Na+ (mM ± 5%) | 173 | 208 | 205 | 207 | 213 | 223 | 386 | 412 | 1760 |
| K+ | 12.5 | 12.3 | 12.2 | 12.2 | 12.7 | 13.3 | 23.0 | 24.3 | 117 |
| Ca2+ | 10.1 | 9.9 | 11.0 | 10.7 | 9.9 | 9.4 | 14.7 | 16.3 | 56.1 |
| Mg2+ | 5.2 | 5.2 | 5.4 | 5.3 | 5.4 | 5.6 | 9.7 | 10.6 | 48.0 |
| SO42− | 2.3 | 3.0 | 2.7 | 2.5 | 3.4 | 3.1 | 4.9 | 5.7 | 20.4 |
| Cl− | 197 | 255 | 226 | 236 | 239 | 248 | 460 | 534 | 2188 |
| Li+ | 9.9 | 9.9 | 9.9 | 9.9 | 10.4 | 10.8 | 18.9 | 20.2 | 93.9 |
| Sr2+ | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.2 | 0.2 | 1.0 |
| Fe | <dl | <dl | <dl | 0.02 | 0.01 | <dl | <dl | <dl | <dl |
| B | 3.3 | 3.1 | 3.3 | 3.3 | 3.3 | 3.6 | 6.3 | 6.9 | 26.8 |
| Br- | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.4 |
| NICB (%) | 4.2 | −1.2 | 4.3 | 2.7 | 2.5 | 2.8 | 0.2 | −3.7 | −1.2 |
| SiO2 (mg/L ± 5%) | 116 | 116 | 114 | 116 | 112 | 96.6 | 112 | 116 | 110 |
| Salinity (g/L ± 5%) | 11.5 | 14.9 | 13.2 | 13.8 | 14.0 | 14.5 | 26.9 | 31.2 | 128 |
| δDwater (±0.8‰) | −93.9 | −94.2 | −93.5 | −92.9 | −87.2 | −52.6 | −25.5 | ||
| δ18Owater (±0.1‰) | −11.8 | −11.7 | −11.6 | −11.5 | −10.1 | −3.2 | 1.2 | ||
| [DIC]mes (mM ± 10%) | 9.7 | 10.5 | 9.6 | 8.8 | 8.4 | 6.5 | 1.2 | 1.4 | 2.0 |
| Alkalinity (mM ± 1%) | 7.38 | 5.66 | 7.57 | 7.48 | 7.34 | 7.34 | 3.03 * | 4.43 | 4.56 |
| δ13CDIC (±0.2‰) | −5.5 | −6.4 | −5.3 | −4.7 | −4.0 | −0.1 | 1.7 | 0.8 | 15.7 |
| δ13Corg (±0.1‰) | −31.3 | −21.6 | −21.0 | −19.8 | −18.2 | −19.9 | −21.2 | ||
| δ13Ccarb (±0.1‰) | −1.1 | 2.1 | −0.7 | 4.7 | 5.7 | 3.4 | 8.1 | ||
| δ18Ocarb (‰ ±0.3‰) | −10.6 | −11.1 | −10.0 | −7.3 | −3.8 | −4.3 | −1.7 |
| Point | PG100 | PG101 | PG102 | PG103 | PG105 | PG106 | PG107 | PG108 | PG110 |
|---|---|---|---|---|---|---|---|---|---|
| [DIC] (mM) | 8.4 | 16.3 | 12.1 | 9.4 | 7.8 | 6.6 | 1.2 * | 1.8 | 2.0 |
| [CO2] (mM) | 1.10 | 10.65 | 4.51 | 1.99 | 0.55 | 0.06 | 0.002 | 0.004 | 0.02 |
| [CO32−] (mM) | 0.009 | 0.0006 | 0.002 | 0.005 | 0.02 | 0.10 | 0.31 | 0.08 | 0.01 |
| [HCO3−] (mM) | 6.49 | 5.04 | 6.69 | 6.59 | 6.35 | 5.57 | 0.88 | 1.24 | 1.16 |
| pCO2(water)/*pCO2(atm) | 227 | 2100 | 877 | 380 | 98 | 9 | 0 | 1 | 5 |
| Degassed CO2 (mbar) | 432 | - | 282 | 115 | 65 | 21 | 2 | 0 | −1 |
| Evaporation extent (%) | n.d. | n.d. | 0 | 4.0 | 5.4 | 8.8 | 50.8 | 57.5 | 89.6 |
| SI Calcite | 0.46 | −0.83 | −0.16 | 0.16 | 0.61 | 1.29 | 1.21 | 1.24 | 0.69 |
| SI Monohydrocalcite | −0.49 | −1.76 | −1.09 | −0.77 | −0.31 | 0.40 | 0.33 | 0.36 | −0.22 |
| PG100 | PG101 | PG102 | PG103 | PG105 | PG106 | PG107 | PG108 | PG110 | |
|---|---|---|---|---|---|---|---|---|---|
| [DIC]carb–diss (mM) | −0.20 | −1.10 | 0.80 | 0.90 | 0.70 | 1.40 | 0.27 | 1.10 | |
| Fractionation factors and δ13C of major DIC components (‰) | |||||||||
| εCO2aq-HCO3 | −7.05 | −7.30 | −7.36 | −7.45 | −7.78 | −9.02 | −8.97 | −9.45 | −9.21 |
| εCO3-HCO3 | −0.22 | −0.24 | −0.25 | −0.25 | −0.28 | −0.39 | −0.39 | −0.43 | −0.41 |
| εCO2aq-CO2g | −0.99 | −1.00 | −1.00 | −1.00 | −1.02 | −1.06 | −1.06 | −1.08 | −1.07 |
| δ13CHCO3(aq) | −5.03 | −1.70 | −2.71 | −3.40 | −3.82 | −0.01 | 1.78 | 1.08 | 26.91 |
| δ13CCO2(aq) | −12.04 | −8.98 | −10.04 | −10.82 | −11.57 | −9.02 | −7.20 | −8.39 | 17.45 |
| Isotopic compositions of the end-members (‰) | |||||||||
| δ13Cdeg | −11.05 | −7.98 | −9.04 | −9.81 | −10.55 | −7.96 | −6.14 | −7.31 | 18.52 |
| δ13Corg | −31.26 | −31.26 | −21.59 | −21.29 | −21.00 | −19.75 | −18.22 | −19.94 | −21.24 |
| δ13Ccarb | −1.11 | −1.11 | 2.10 | 2.10 | −0.71 | 4.69 | 5.67 | 3.35 | 8.11 |
| Participation of the end-members to the DIC balance (%) | |||||||||
| Ptransf | 52% | 74% | 75% | 81% | 82% | 1% | 125% | 28% | |
| Pdeg–pump | 51% | 33% | 17% | 3% | −20% | 116% | −119% (−144%) | −192% (−476%) | |
| Pphoto–resp | −2% | 0% | 2% | 7% | 28% | −41% | 50% (73%) | 114% (158%) | |
| Pcarb–diss | −1% | −7% | 6% | 10% | 10% | 23% | 44% | 150% | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Muller, E.; Ader, M.; Aloisi, G.; Bougeault, C.; Durlet, C.; Vennin, E.; Benzerara, K.; Gaucher, E.C.; Virgone, A.; Chavez, M.; et al. Successive Modes of Carbonate Precipitation in Microbialites along the Hydrothermal Spring of La Salsa in Laguna Pastos Grandes (Bolivian Altiplano). Geosciences 2022, 12, 88. https://doi.org/10.3390/geosciences12020088
Muller E, Ader M, Aloisi G, Bougeault C, Durlet C, Vennin E, Benzerara K, Gaucher EC, Virgone A, Chavez M, et al. Successive Modes of Carbonate Precipitation in Microbialites along the Hydrothermal Spring of La Salsa in Laguna Pastos Grandes (Bolivian Altiplano). Geosciences. 2022; 12(2):88. https://doi.org/10.3390/geosciences12020088
Chicago/Turabian StyleMuller, Elodie, Magali Ader, Giovanni Aloisi, Cédric Bougeault, Christophe Durlet, Emmanuelle Vennin, Karim Benzerara, Eric C. Gaucher, Aurélien Virgone, Marco Chavez, and et al. 2022. "Successive Modes of Carbonate Precipitation in Microbialites along the Hydrothermal Spring of La Salsa in Laguna Pastos Grandes (Bolivian Altiplano)" Geosciences 12, no. 2: 88. https://doi.org/10.3390/geosciences12020088
APA StyleMuller, E., Ader, M., Aloisi, G., Bougeault, C., Durlet, C., Vennin, E., Benzerara, K., Gaucher, E. C., Virgone, A., Chavez, M., Souquet, P., & Gérard, E. (2022). Successive Modes of Carbonate Precipitation in Microbialites along the Hydrothermal Spring of La Salsa in Laguna Pastos Grandes (Bolivian Altiplano). Geosciences, 12(2), 88. https://doi.org/10.3390/geosciences12020088








