An Approach for the Automatic Characterization of Underwater Dunes in Fluviomarine Context
Abstract
:1. Introduction
2. Underwater Dunes
2.1. Formation of the Underwater Dunes
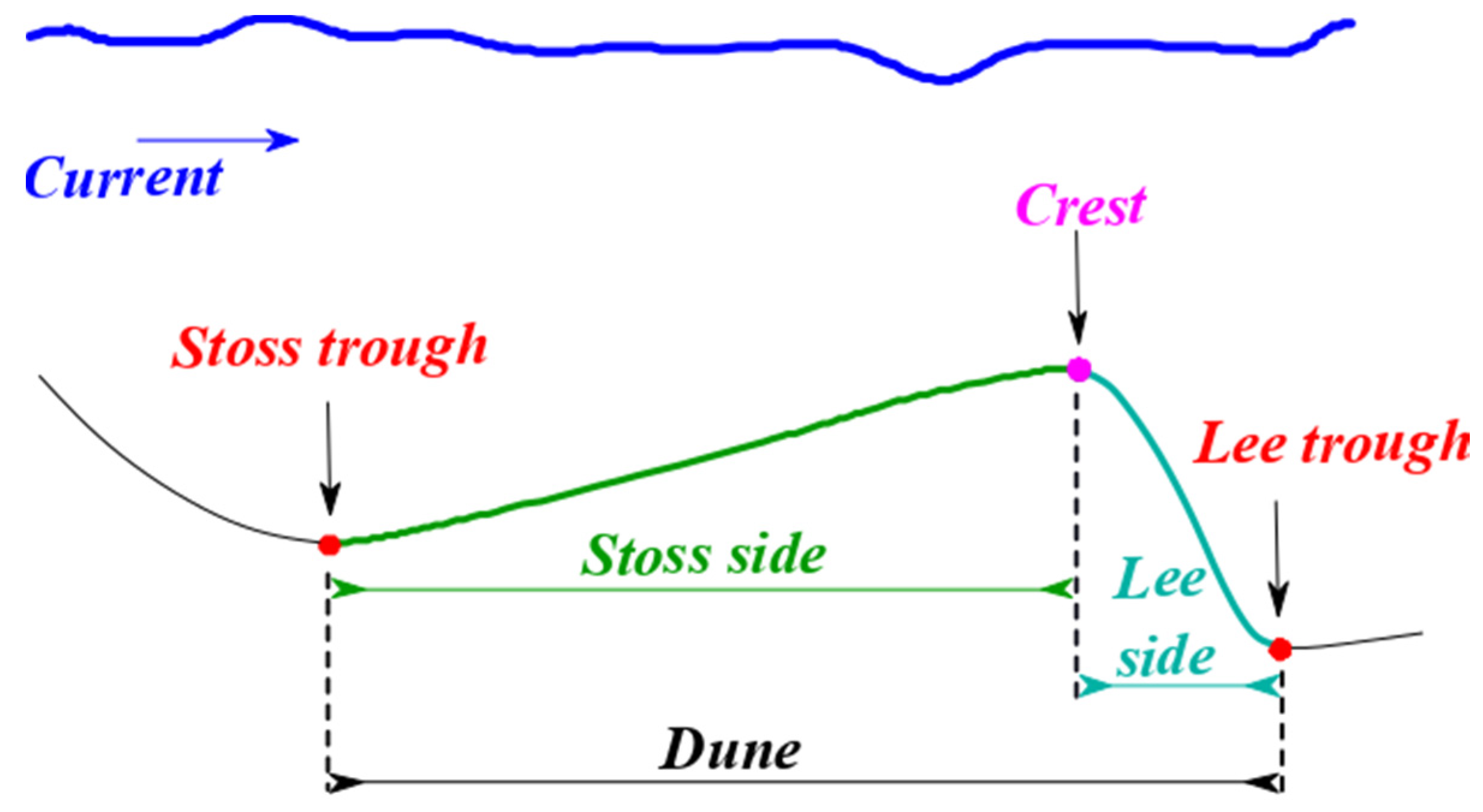
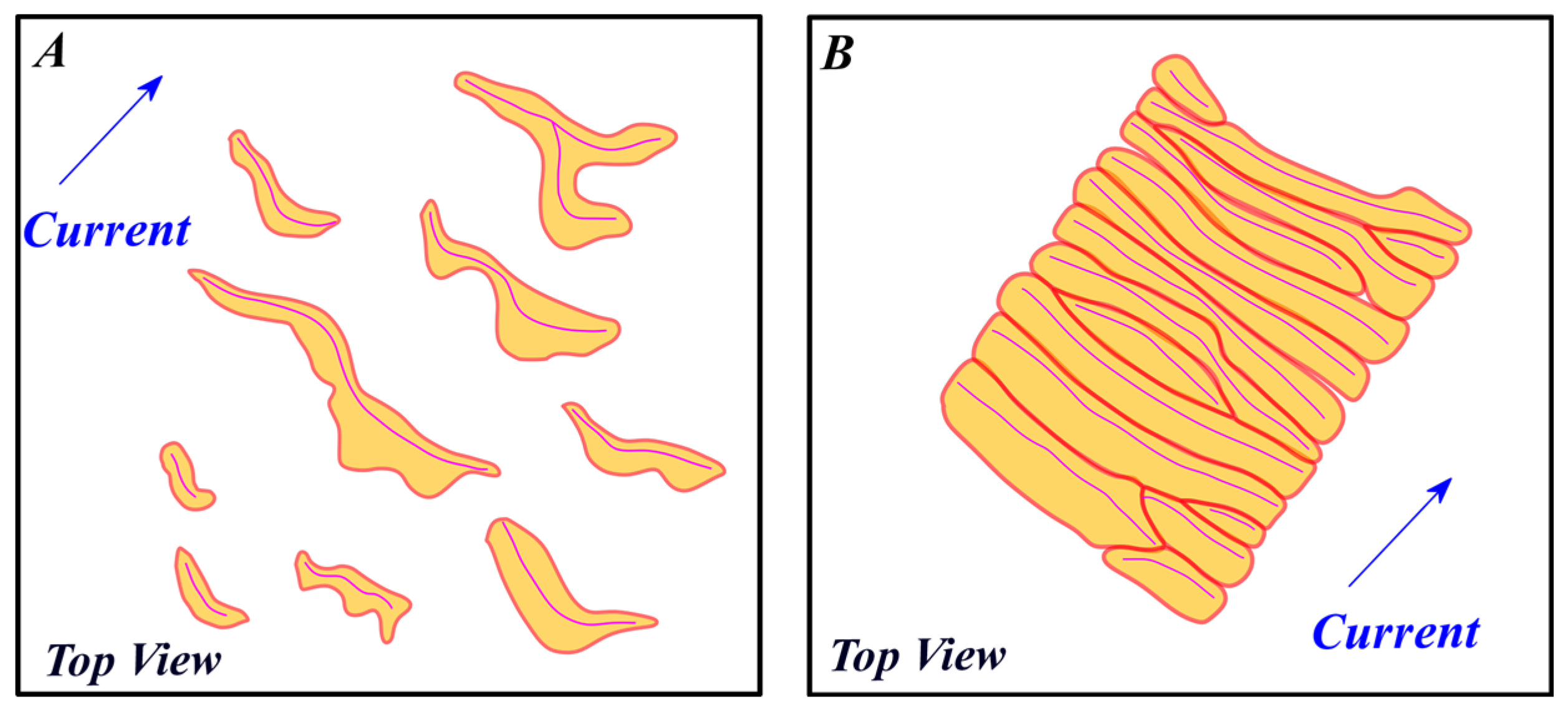
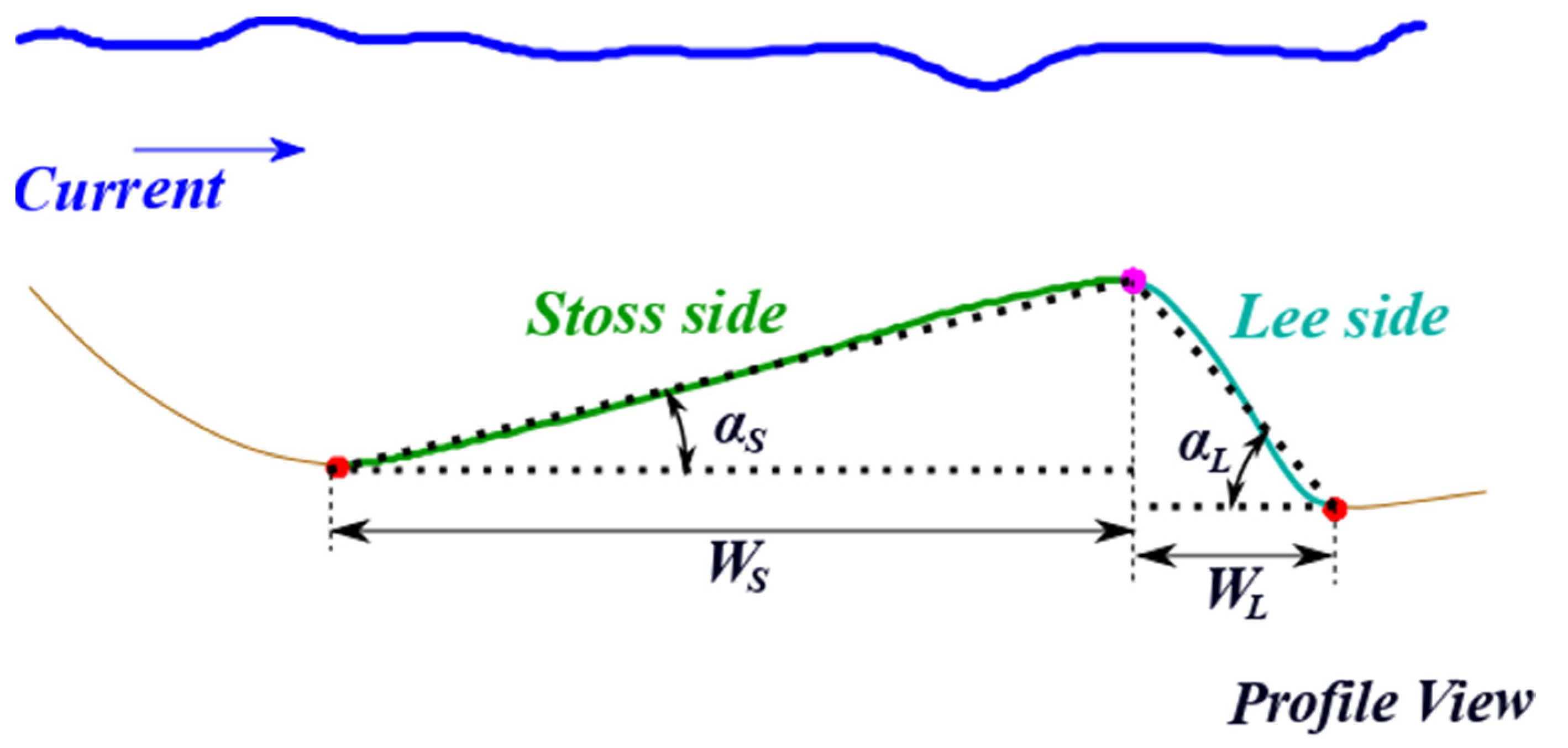
2.2. Morphological Descriptors of the Dunes
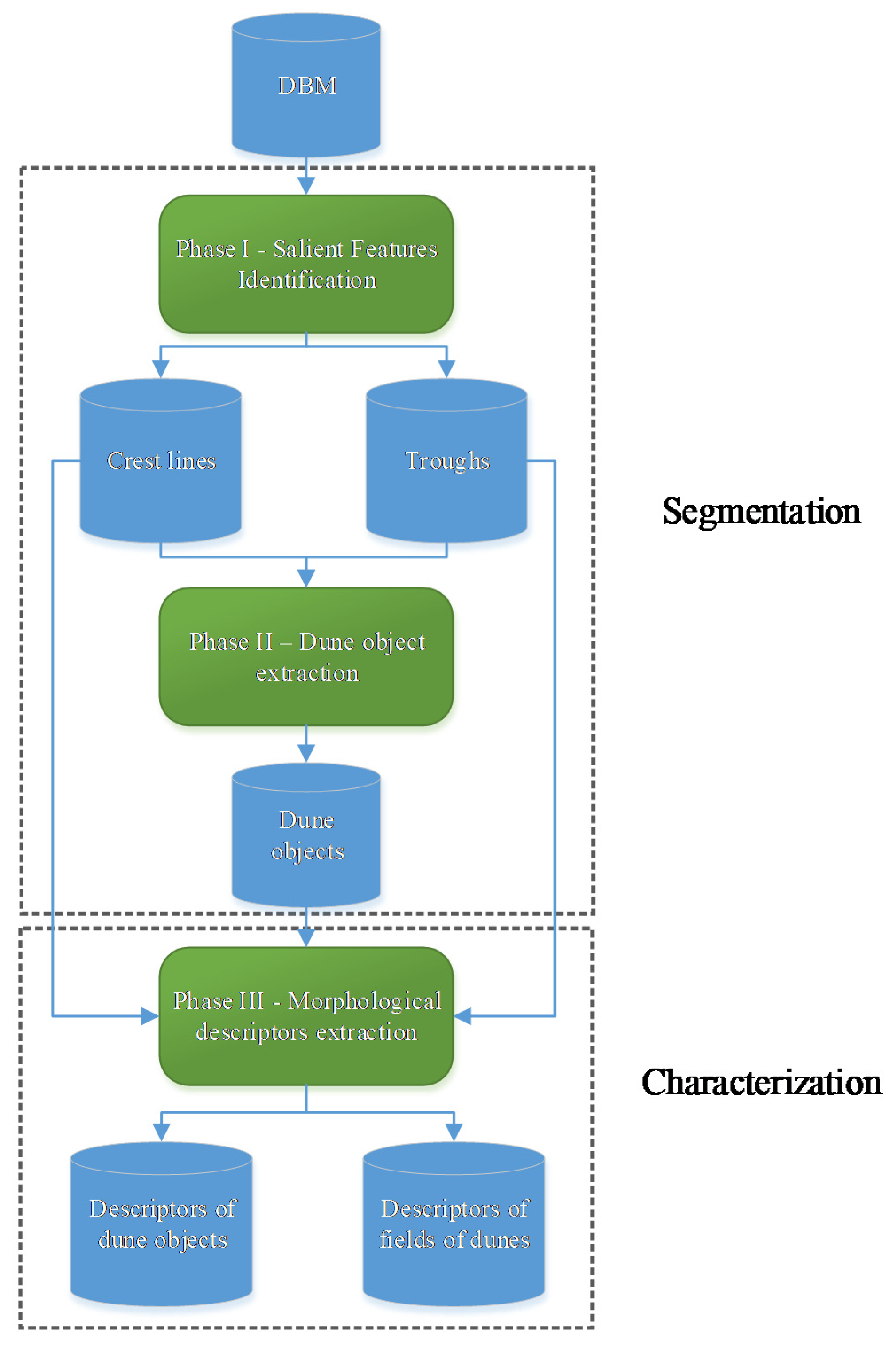
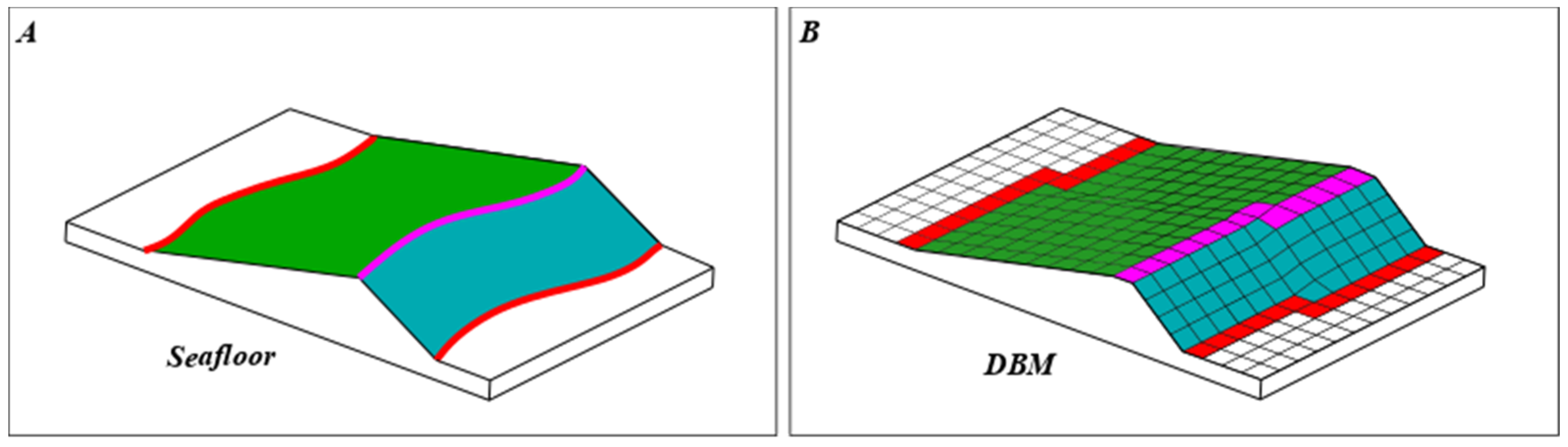
3. Underwater Dunes Characterization from a DBM
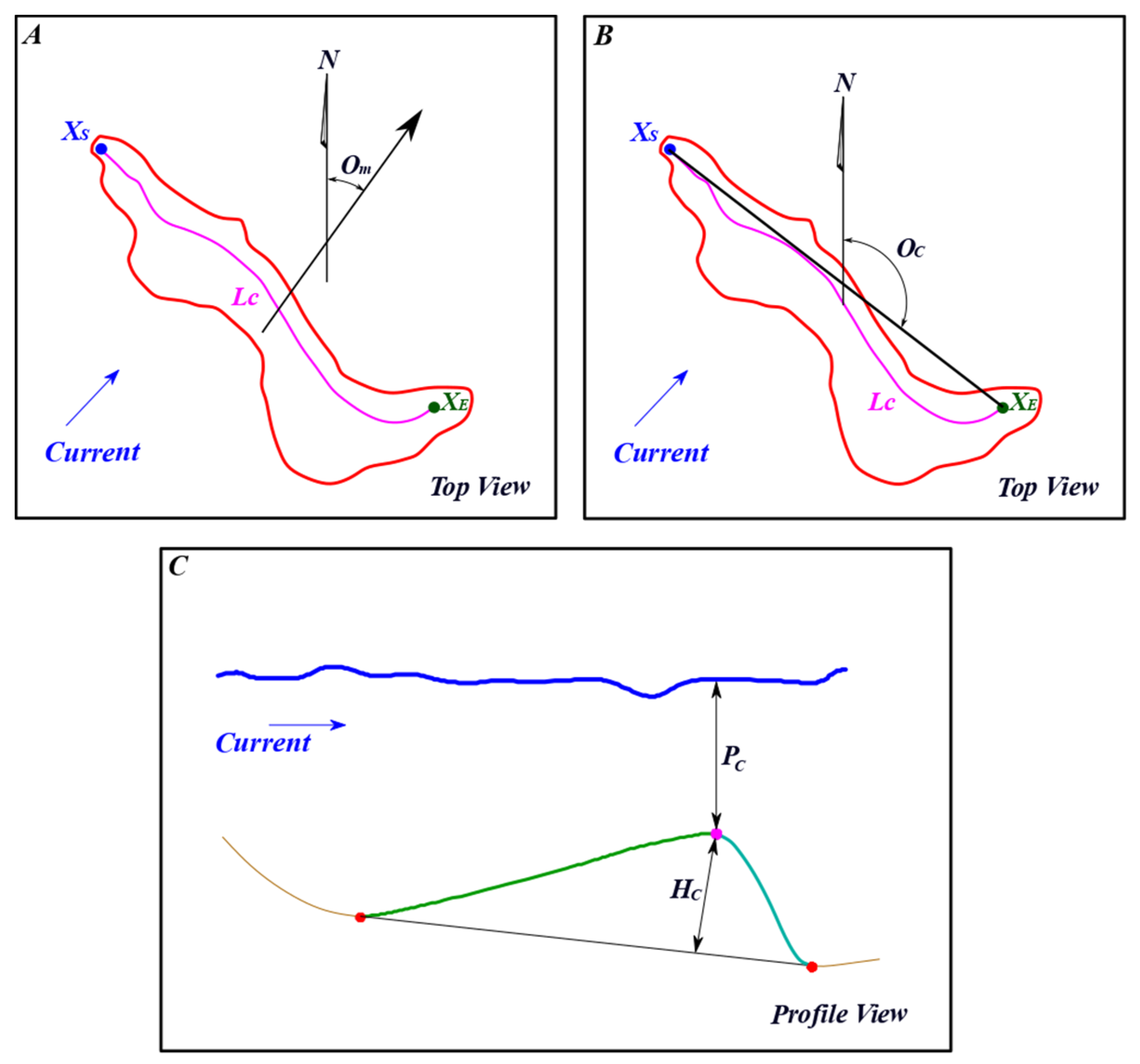
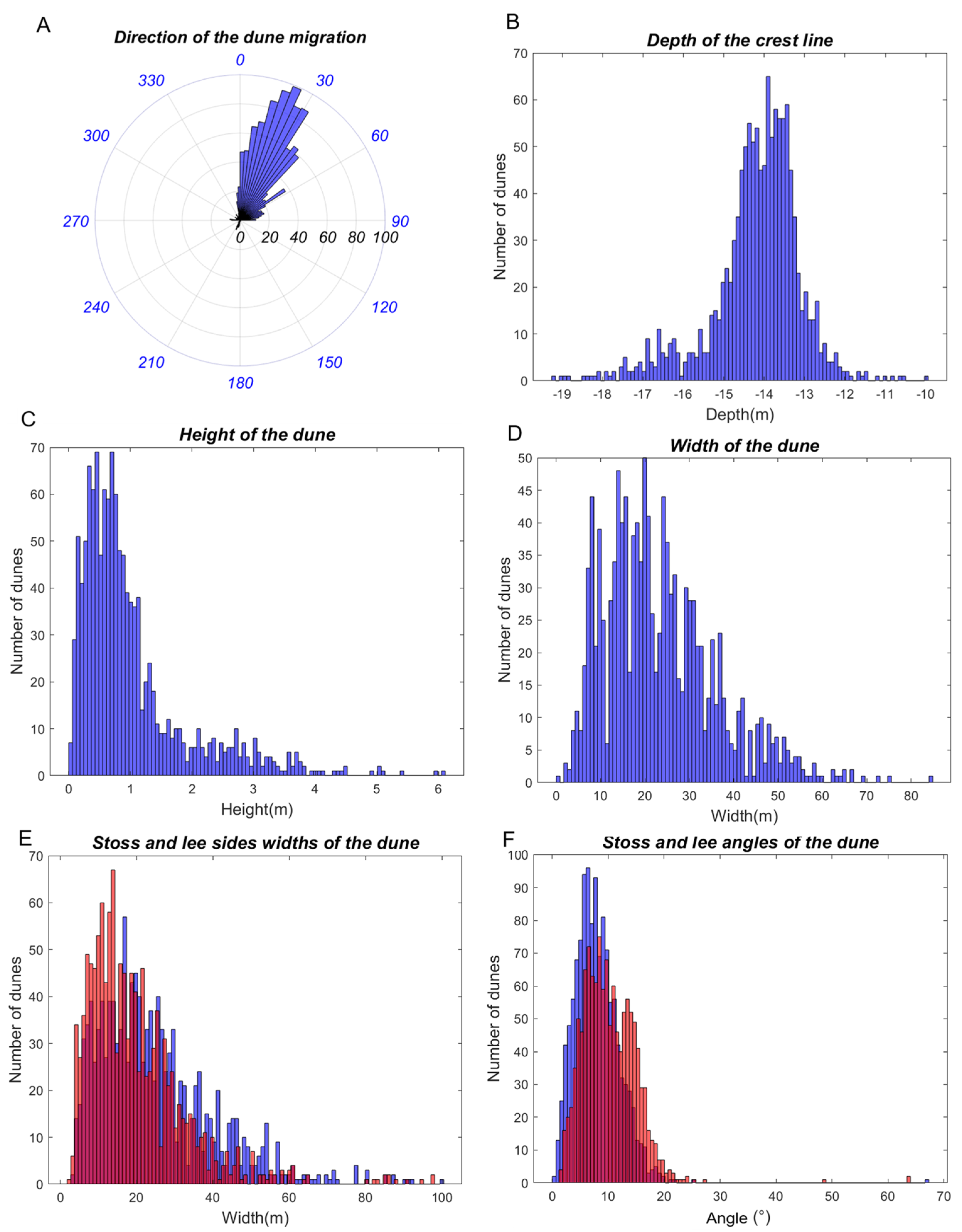
- The dune orientation is computed using the segment joining the starting and ending pixel of the crest line. We considered the direction facing the lee side of the dune. The segment orientation angle is measured from the north (Om).
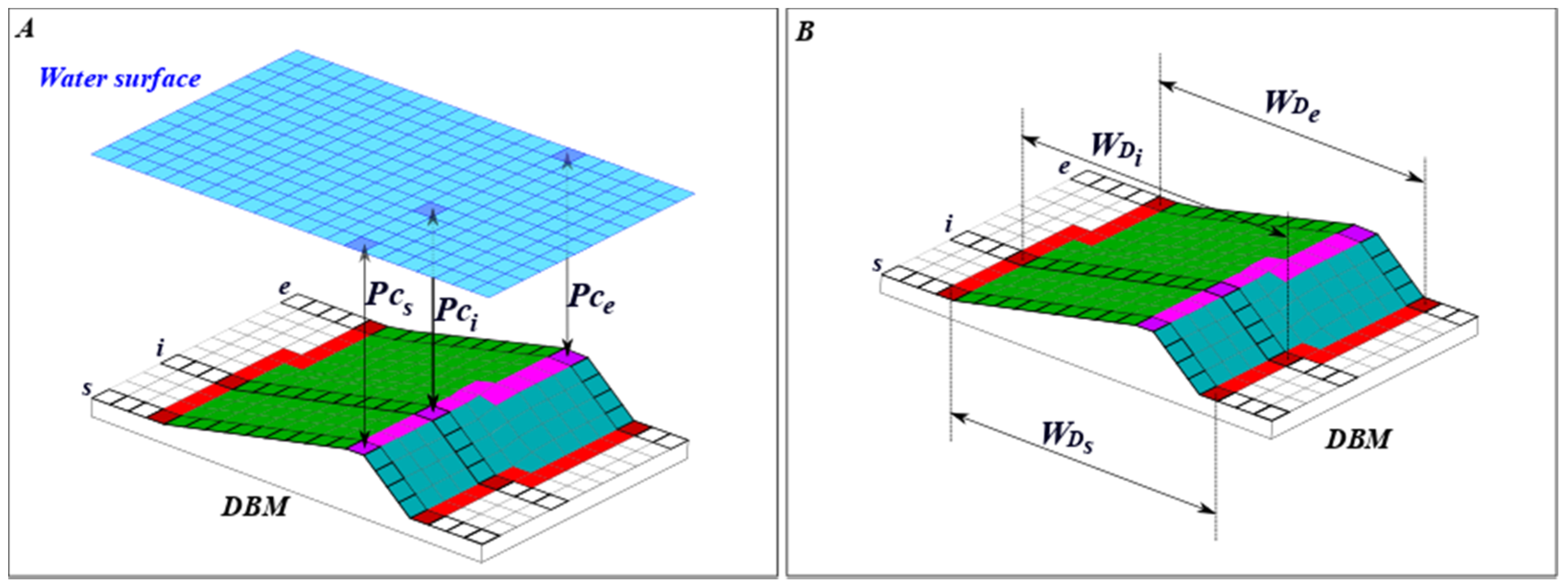
- The depth (PC) of a dune is computed using the depth of each pixel of the crest line, as illustrated in Figure 11A. The minimum value among these pixels (i.e., closest to the water surface) is considered as the dune depth, since this information is valuable to detect dunes representing a risk for safe navigation.
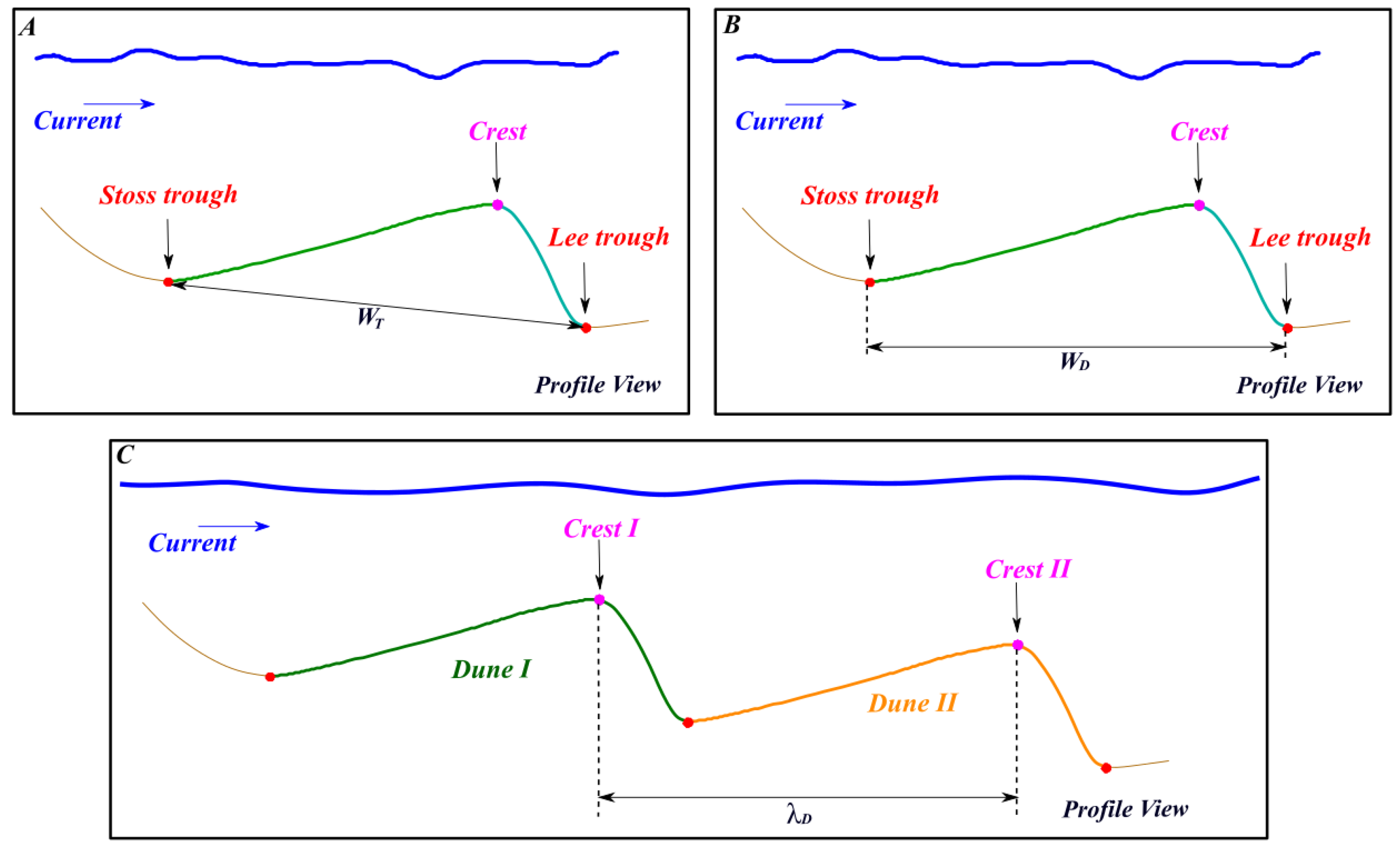
- The width (WD) is defined as the horizontal distance between the dune lee and stoss troughs. To compute this measure, we considered the stoss and lee trough pixels matched with each crest line pixel. Therefore, a width value is computed for each pixel composing the crest line, as illustrated in Figure 11B. The median value among all crest line pixels is considered as the dune width.
- The height of a dune (HC) also considers the matching between the pixels of the crest line and the troughs. A height value is computed for each crest line pixel as the distance between the crest line pixel and the line joining the stoss and lee troughs (see Figure 4C). The median value among all the crest line pixels is considered as the height of the dune.
- The stoss and lee angles (αS and αL), as well as stoss and lee widths (WS and WL), are computed in a similar fashion as the measures related to the crest line. The median values computed are considered as the stoss and lee angles, as well as stoss and lee widths of the dune.
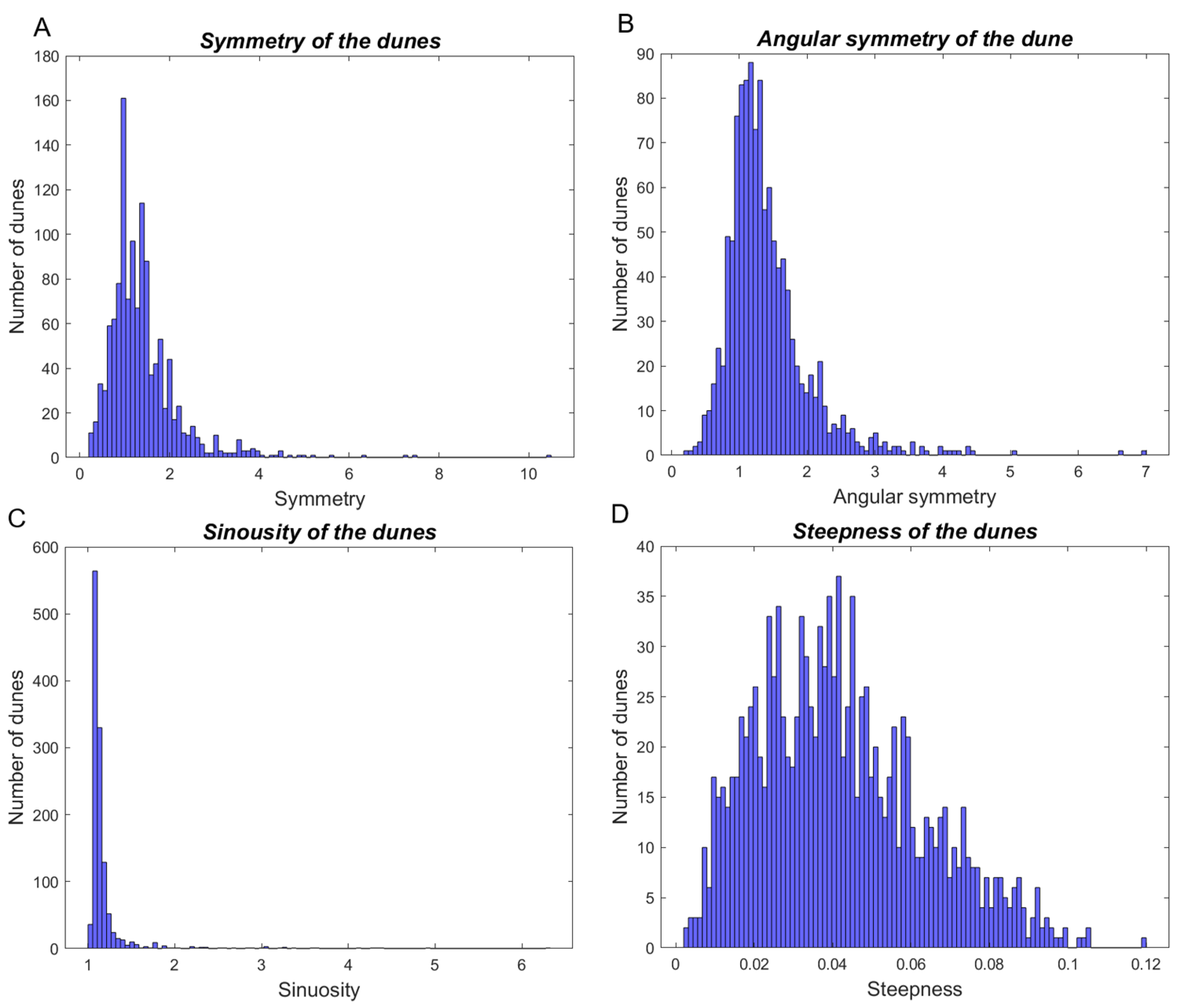
- The sinuosity index () is computed considering (3). The geodetic distance () is calculated as illustrated in Figure 7. Please note that the length of the crest line () is not computed as the number of pixels composing the crest line. Instead, the geometric distance between the centers of the pixels is used to increase accuracy given the variability of the crest line sinuosity.
- The steepness and symmetry indexes are computed for each dune object considering the values previously estimated for the width (WD, WS, and WL) and height (HC), as defined in (1) and (2).
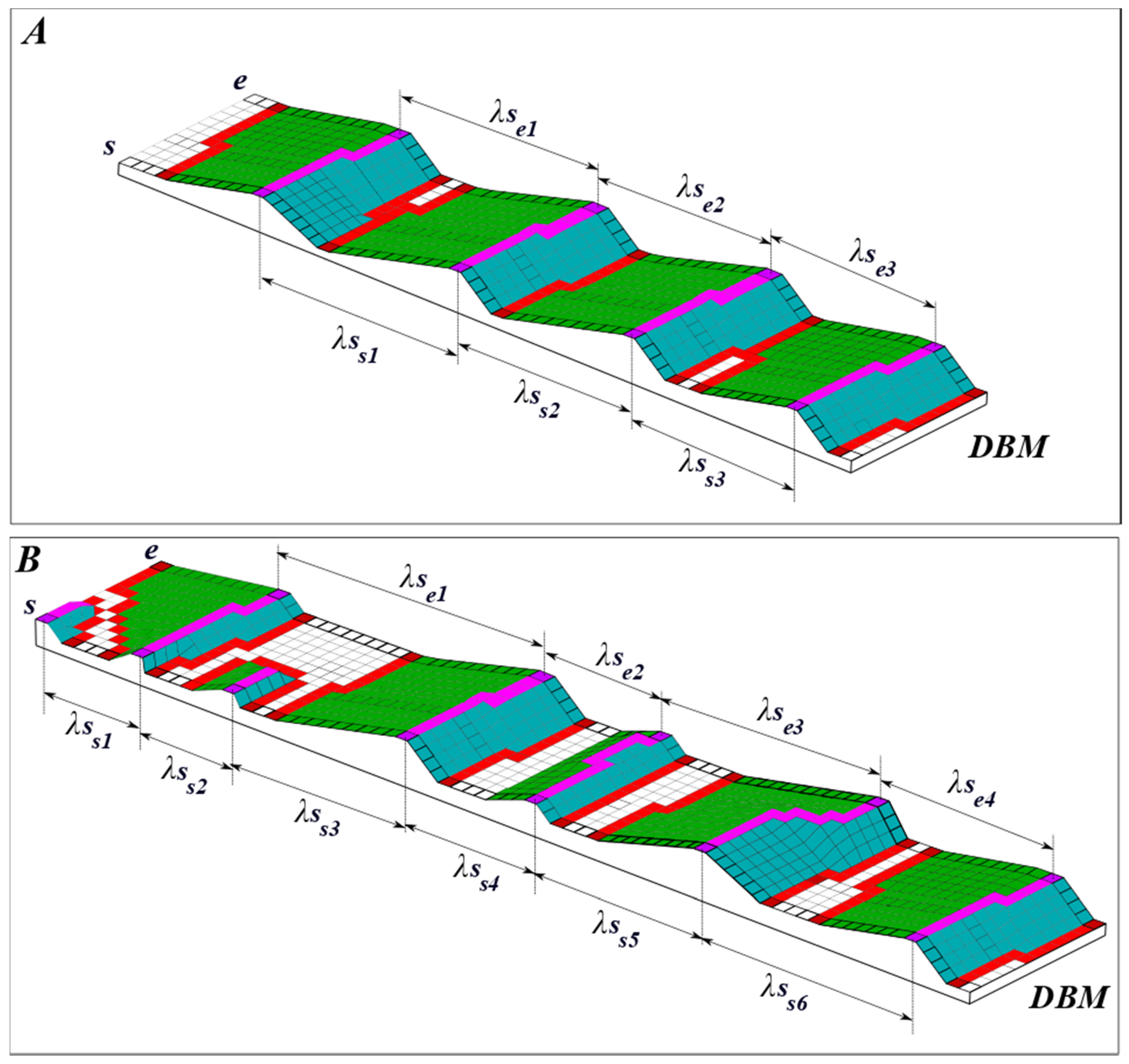
- The spacing between two consecutive dunes in a field is computed considering the distances between the crest lines of each dune. The computation of the spacing requires a direction. Therefore, the median value of the dune orientation of the objects located on the field is considered here. The spacing is computed for each pixel of the crest lines, as illustrated in Figure 12. Then, the mean value is considered as the spacing (λS) of the dunes of a field.
- The standard deviation of the spacing () is computed considering all the spacing values of the dunes on a field. This descriptor is useful to characterize the dunes dispersion on the seafloor surface.
- The dunes density () is computed using the ratio between the surface of the field covered by dunes () and the total surface of the field (), as described in (4). is a third-order descriptor mentioned by the authors of [24], therein also called fullbeddedness or fraction of the seafloor covered by dunes.
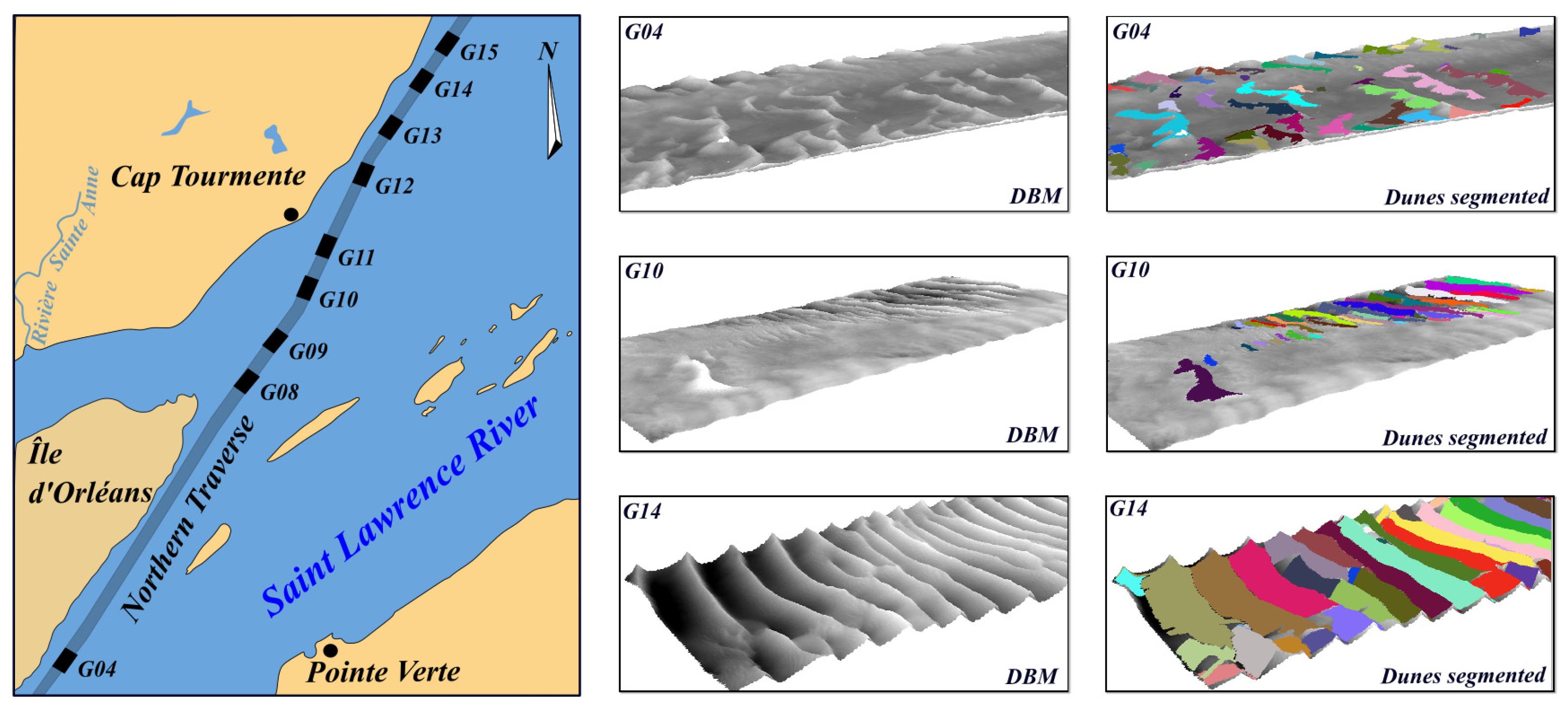
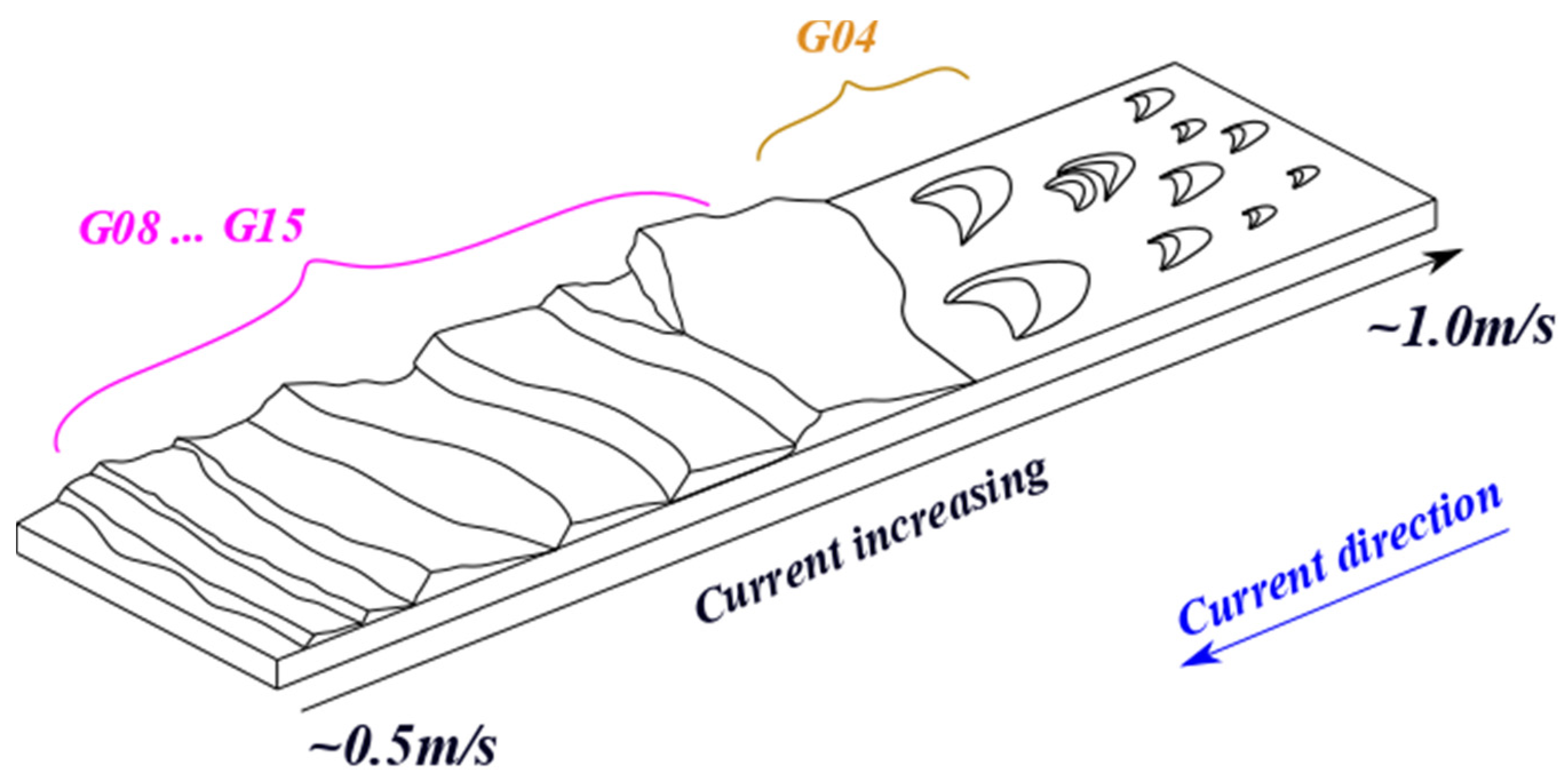
4. Characterization of the Dunes of the Northern Traverse of the Saint Lawrence River
4.1. The Northern Traverse of the Saint Lawrence River
4.2. Morphological Descriptors of the Dunes of the Northern Traverse
5. Analysis and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Le Bot, S. Morphodynamique de Dunes Sous-Marines Sous Influence des Marées et des Tempêtes. Processus Hydro-Sédimentaires et Enregistrement. Exemple du Pas-de-Calais. Ph.D. Thesis, Université de Lille I, Villeneuve-d’Ascq, France, 2001. [Google Scholar]
- Cheng, H.Q.; Kostaschuk, R.; Shi, Z. Tidal currents, bed sediments, and bedforms at the South Branch and the South Channel of the Changjiang (Yangtze) Estuary, China: Implications for the ripple-dune transition. Estuaries 2004, 27, 861–866. [Google Scholar] [CrossRef]
- Boggs, S. Sedimentary structures. In Principles of Sedimentology and Stratigraphy, 4th ed.; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2006; Chapter 4; pp. 74–116. ISBN 0-13-154728-3. [Google Scholar]
- Nichols, G. Processes of transport and sedimentary structures. In Sedimentology and Stratigraphy, 2nd ed.; Wiley-Blackwell, A., Ed.; John Wiley & Sons Publication: West Sussex, UK, 2009; Chapter 4; pp. 44–68. [Google Scholar]
- Best, J.L. The fluid dynamics of river dunes: A review and some future research directions. J. Geophys. Res. 2005, 110, F04S02. [Google Scholar] [CrossRef]
- Andreotti, B.; Claudin, P.; Devauchelle, O.; Duràn, O.; Fourrière, A. Bedforms in a turbulent stream: Ripples, chevrons and antidunes. J. Fluid Mech. 2012, 690, 94–128. [Google Scholar] [CrossRef] [Green Version]
- Lefebvre, A.; Paarlberg, A.J.; Winter, C. Characterising natural bedform morphology and its influence on flow. Geo-Mar. Lett. 2016, 36, 379–393. [Google Scholar] [CrossRef]
- Debese, N.; Jacq, J.J.; Garlan, T. Extraction of sandy bedforms features through geodesic morphometry. Geomorphology 2016, 267, 82–97. [Google Scholar] [CrossRef] [Green Version]
- Lecours, V.; Dolan, M.F.J.; Micallef, A.; Lucieer, V.L. A review of marine geomorphometry, the quantitative study of the seafloor. Hydrol. Earth Syst. Sci. 2016, 20, 3207–3244. [Google Scholar] [CrossRef] [Green Version]
- Debese, N.; Jacq, J.-J.; Degrendele, K.; Roche, M. Toward Reliable Volumetric Monitoring of Sandbanks. In Geomorphometry; Elsevier Science: Boulder, CO, USA, 2018. [Google Scholar]
- Yan, G.; Cheng, H.Q.; Jiang, Z.Y.; Tengm, L.Z.; Tang, M.; Shi, T.; Jiang, Y.H.; Yang, G.Q.; Zhou, Q.P. Recognition of fluvial bank erosion along the main stream of the Yangtze River. Engineering 2021, in press. [CrossRef]
- Cheng, H.Q.; Chen, W.; Li, J.F.; Jiang, Y.H.; Hu, X.; Zhang, X.L.; Zhou, F.N.; Hu, F.X.; Stive, M.J.F. Morphodynamic changes of the Yangtze Estuary under the impact of the Three Gorge Dam, of estuarine engineering interventions and of climate-induced sea level rise. Earth Planet. Sci. Lett. 2022, 580, 117385. [Google Scholar] [CrossRef]
- van Dijk, T.A.G.P.; van Dalfsen, J.A.; Van Lancker, V.; van Overmeeren, R.A.; van Heteren, S.; Doornenbal, P.J. 13—Benthic Habitat Variations over Tidal Ridges, North Sea, the Netherlands. In Seafloor Geomorphology as Benthic Habitat; Elsevier: Amsterdam, The Netherlands, 2012; pp. 241–249. ISBN 9780123851406. [Google Scholar] [CrossRef]
- Parsons, D.R.; Best, J.L.; Orfeo, O.; Hardy, R.J.; Kostaschuk, R.; Lane, S.N. Morphology and flow fields of three-dimensional dunes, Rio Paranà, Argentina: Results from simultaneous multibeam echo sounding and acoustic Doppler current profiling. J. Geophys. Res. 2005, 110, F04S03. [Google Scholar] [CrossRef] [Green Version]
- Di Stefano, M.; Mayer, L.A. An automatic procedure for the quantitative characterization of submarine bedforms. Geosciences 2018, 8, 28. [Google Scholar] [CrossRef] [Green Version]
- Jasiewicz, J.; Stepinski, T.F. Geomorphons—A pattern reconigtion approach to classification and mapping of landforms. Geomorphology 2012, 182, 147–156. [Google Scholar] [CrossRef]
- Cassol, W.N.; Daniel, S.; Guilbert, É. A Segmentation Approach to Identify Underwater Dunes from Digital Bathymetric Models. Geoscience 2021, 11, 361. [Google Scholar] [CrossRef]
- Lebrec, U.; Riera, R.; Paumard, V.; O’Leary, M.J.; Lang, S.C. Automatic Mapping and Characterisation of Linear Depositional Bedforms: Theory and Application Using Bathymetry from the North West Shelf of Australia. Remote Sens. 2022, 14, 280. [Google Scholar] [CrossRef]
- Hains, D.; Bergmann, M.; Cawthra, H.C.; Cove, K.; Echeverry, P.; Maschke, J.; Mihailov, M.E.; Obura, V.; Oei, P.; Pang, P.Y.; et al. Hydrospatial…A global movement. Inter. Hydrogr. Rev. 2021, 26, 147–153. [Google Scholar]
- Amos, C.L.; King, E.L. Bedforms of the Canadian eastern seaboard: A comparison with global occurrences. Mar. Geol. 1984, 57, 167–208. [Google Scholar] [CrossRef]
- Bartholdy, J.; Flemming, B.W.; Bartholomä, A.; Ernstsen, V.B. Flow and grain size control of depth-independent simple subaqueous dunes. J. Geophys. Res. 2005, 110, F04S16. [Google Scholar] [CrossRef]
- Landeghem, K.J.J.; Wheeler, A.J.; Mitchell, N.C.; Sutton, G. Variations in sediment wave dimensions across the tidally dominated Irish Sea, NW Europe. Mar. Geol. 2009, 263, 108–119. [Google Scholar] [CrossRef]
- Thibaud, R.; Del Mondo, G.; Garlan, T.; Mascret, A.; Carpentier, C. A Spatio-Temporal Graph Model for Marine Dune Dynamics Analysis and Representation. Trans. GIS 2013, 17, 742–762. [Google Scholar] [CrossRef]
- Ashley, G.M. Classification of large-scale subaqueous bedforms: A new look to an old problem. J. Sediment. Petrol. 1990, 60, 160–172. [Google Scholar]
- Gutierrez, R.R.; Abad, J.D.; Parsons, D.R.; Best, J.L. Discrimination of bed form scales using robust spline filters and wavelet transforms: Methods and application to synthetic signals and bed forms of the Rio Paraná, Argentina. J. Geophys. Res. Earth Surf. 2013, 118, 1400–1419. [Google Scholar] [CrossRef]
- Ferret, Y. Morphodynamique de Dunes Sous-Marines en Contexte de Plate-Forme Mégatidale (Manche Orientale). Approche Multi-Échelles Spatio-Temporelles. Interfaces Continentales, Environnement; Université de Rouen: Rouen, France, 2011. [Google Scholar]
- van der Mark, C.F.; Blom, A. A New & Widely Applicable Bedform Tracking Tool; University of Twente, Faculty of Engineering Technology, Department of Water Engineering and Management: Enschede, The Netherlands, 2007; 44p. [Google Scholar]
- Knaapen, M.A.F. Sandwave migration predictor based on shape information. J. Geophys. Res. 2005, 110, F04S11. [Google Scholar] [CrossRef] [Green Version]
- Ogor, J. Design of Algorithms for the Automatic Characterization of Marine Dune Morphology and Dynamics. Ocean, Atmosphere. Ph.D. Thesis, ENSTA-Bretagne, Brest, France, 2018. [Google Scholar]
- Garlan, T. Study on Marine Sandwave Dynamics. Int. Hydrogr. Rev. 2007, 8, 26–37. [Google Scholar]
- Garlan, T. GIS and Mapping of Moving Marine Sand Dunes. In Proceedings of the 24th International Cartography Conference, Santiago, Chili, 15–21 November 2009. [Google Scholar]
- Berné, S. Architecture et Dynamique des Dunes Tidales. Exemples de la Marge Atlantique Française. Ph.D. Thesis, Université des Sciences et Techniques de Lille Flandres-Artois, Westhoek, France, 1991. [Google Scholar]
- Drapeau, G. Dynamique sédimentaire des littoraux de l’estuaire du Saint Laurent. Géographie Phys. Quat. 1992, 46, 233–242. [Google Scholar] [CrossRef] [Green Version]
- Comité de concertation navigation de Saint Laurent Vision 2000. Stratégie de Navigation Durable Pour le Saint-Laurent; Ministère des Transports du Québec et Pêches et Océans: Québec, QC, Canada, 2004; 114p. [Google Scholar]
- Kenyon, N.H. Sand Ribbons of European tidal Seas. Mar. Geol. 1970, 9, 25–39. [Google Scholar] [CrossRef]
- Masetti, G.; Mayer, L.A.; Ward, L.G. A Bathymetry- and Reflectivity-Based Approach for Seafloor Segmentation. Geosciences 2018, 8, 14. [Google Scholar] [CrossRef] [Green Version]



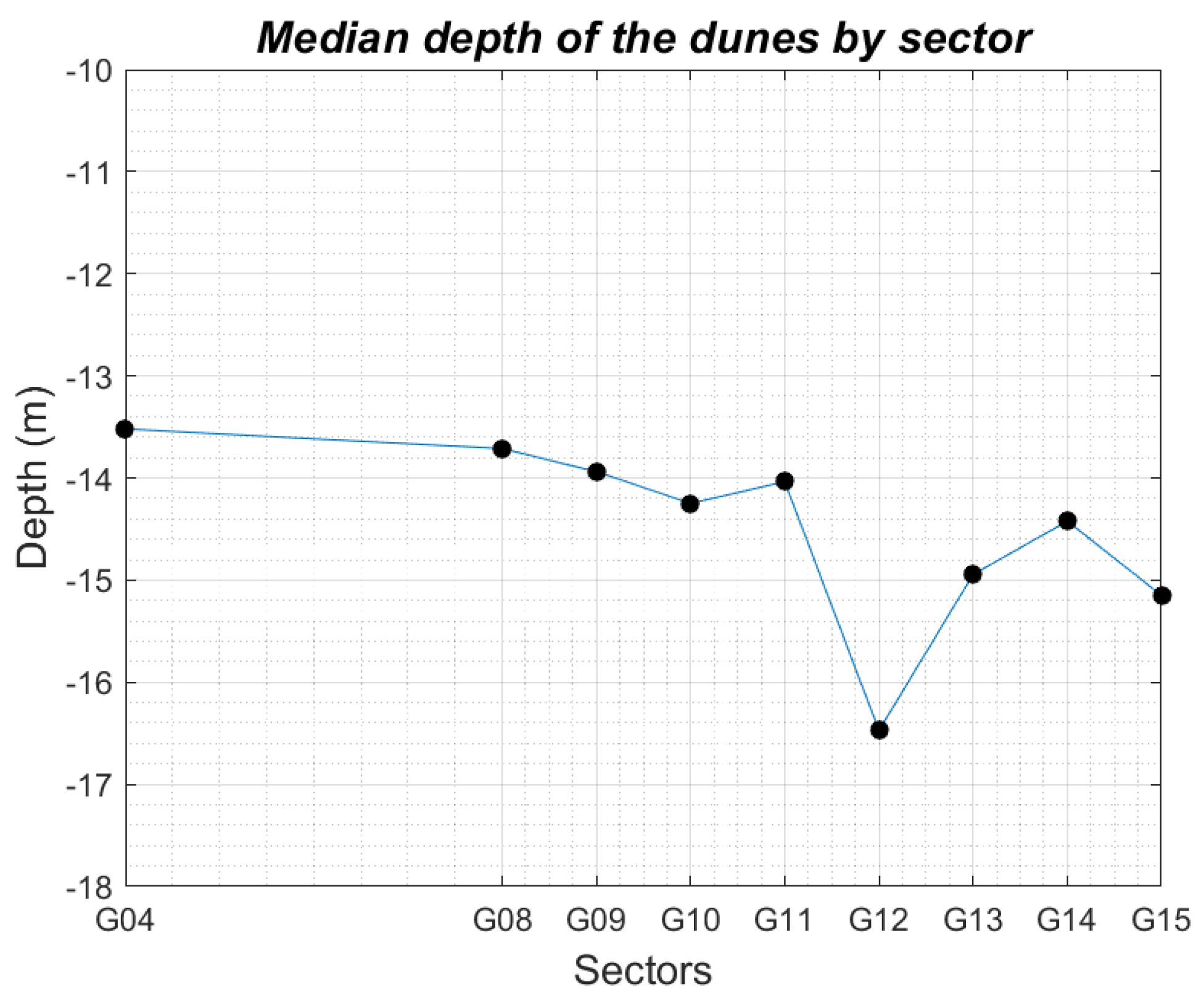

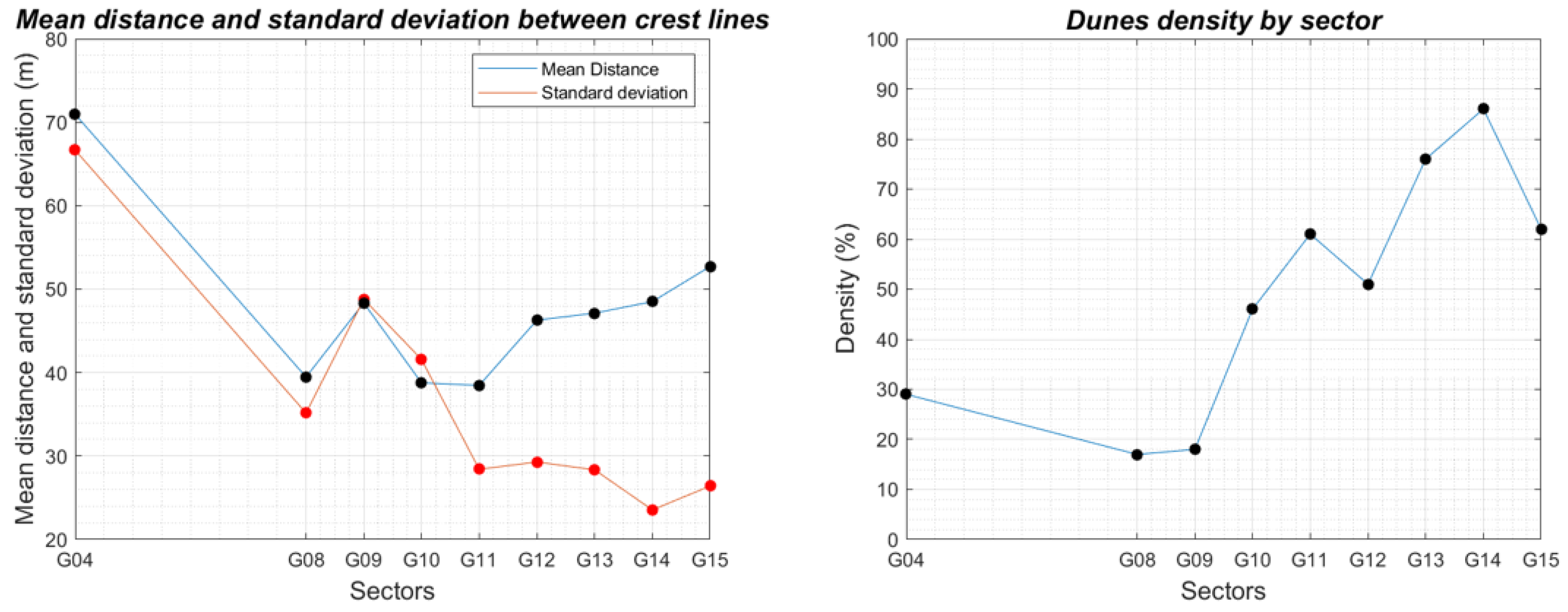
| Sector | Om (°) | PC (m) | HC (m) | WD (m) | ||
|---|---|---|---|---|---|---|
| G04 | 57.18 | 13.52 | 0.60 | 16.97 | 1.16 | 1.49 |
| G08 | 33.69 | 13.71 | 0.43 | 20.81 | 1.11 | 1.43 |
| G09 | 21.92 | 13.94 | 0.54 | 8.49 | 1.10 | 1.34 |
| G10 | 20.22 | 14.25 | 0.48 | 12.00 | 1.10 | 1.10 |
| G11 | 21.80 | 14.03 | 0.78 | 23.42 | 1.10 | 1.16 |
| G12 | 23.33 | 16.47 | 0.84 | 21.00 | 1.11 | 1.52 |
| G13 | 27.21 | 14.94 | 2.45 | 34.00 | 1.09 | 1.25 |
| G14 | 36.19 | 14.42 | 2.64 | 36.00 | 1.09 | 1.24 |
| G15 | 201.48 | 15.15 | 1.66 | 28.00 | 1.10 | 1.54 |
| Sector | λS (m) | ||
|---|---|---|---|
| G04 | 70.95 | 66.66 | 29 |
| G08 | 39.47 | 35.16 | 17 |
| G09 | 48.35 | 48.80 | 18 |
| G10 | 38.78 | 41.58 | 46 |
| G11 | 38.47 | 28.42 | 61 |
| G12 | 46.29 | 29.29 | 51 |
| G13 | 47.13 | 28.35 | 76 |
| G14 | 48.51 | 23.55 | 86 |
| G15 | 52.72 | 26.41 | 62 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cassol, W.N.; Daniel, S.; Guilbert, É. An Approach for the Automatic Characterization of Underwater Dunes in Fluviomarine Context. Geosciences 2022, 12, 89. https://doi.org/10.3390/geosciences12020089
Cassol WN, Daniel S, Guilbert É. An Approach for the Automatic Characterization of Underwater Dunes in Fluviomarine Context. Geosciences. 2022; 12(2):89. https://doi.org/10.3390/geosciences12020089
Chicago/Turabian StyleCassol, Willian Ney, Sylvie Daniel, and Éric Guilbert. 2022. "An Approach for the Automatic Characterization of Underwater Dunes in Fluviomarine Context" Geosciences 12, no. 2: 89. https://doi.org/10.3390/geosciences12020089
APA StyleCassol, W. N., Daniel, S., & Guilbert, É. (2022). An Approach for the Automatic Characterization of Underwater Dunes in Fluviomarine Context. Geosciences, 12(2), 89. https://doi.org/10.3390/geosciences12020089







