Exploiting Ground-Penetrating Radar Signal Enhancements by Water-Saturated Bulb Surrounding Defective Waterpipes for Leak Detection
Abstract
1. Introduction
2. Laboratory Experiment
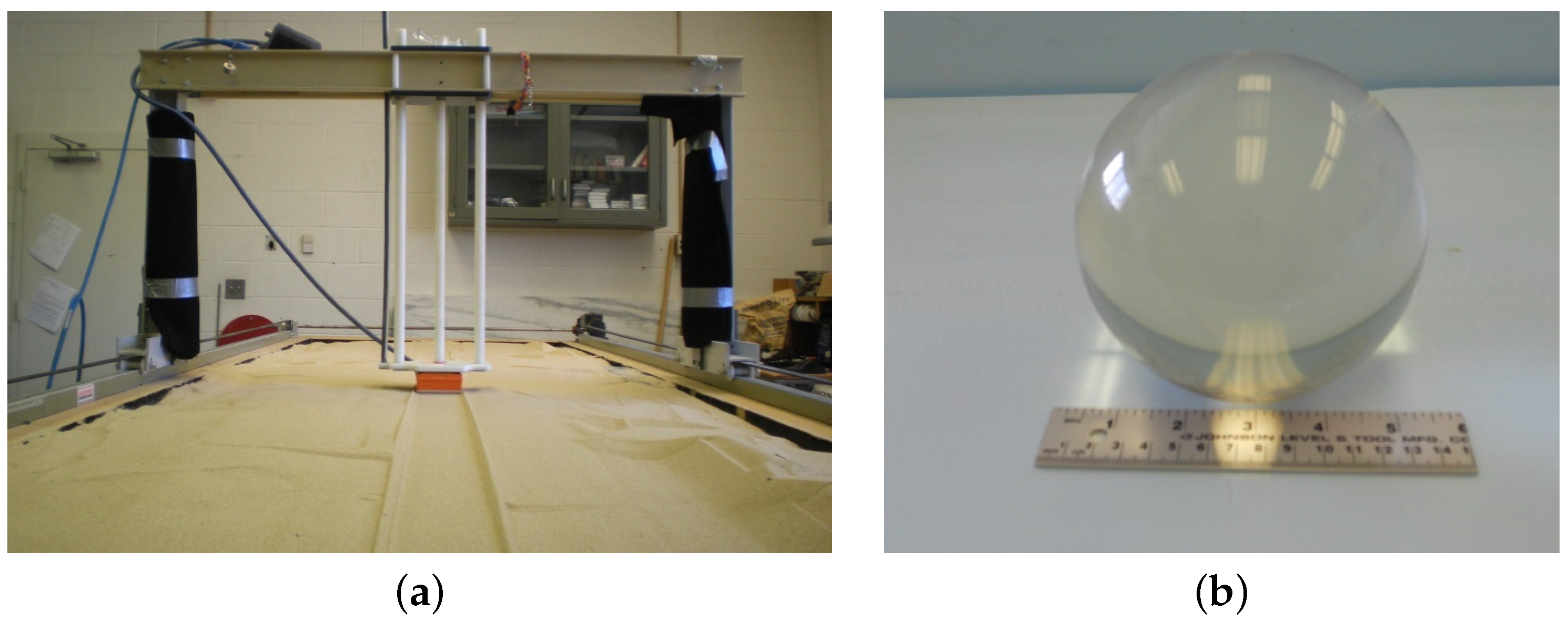
2.1. Laboratory Setup
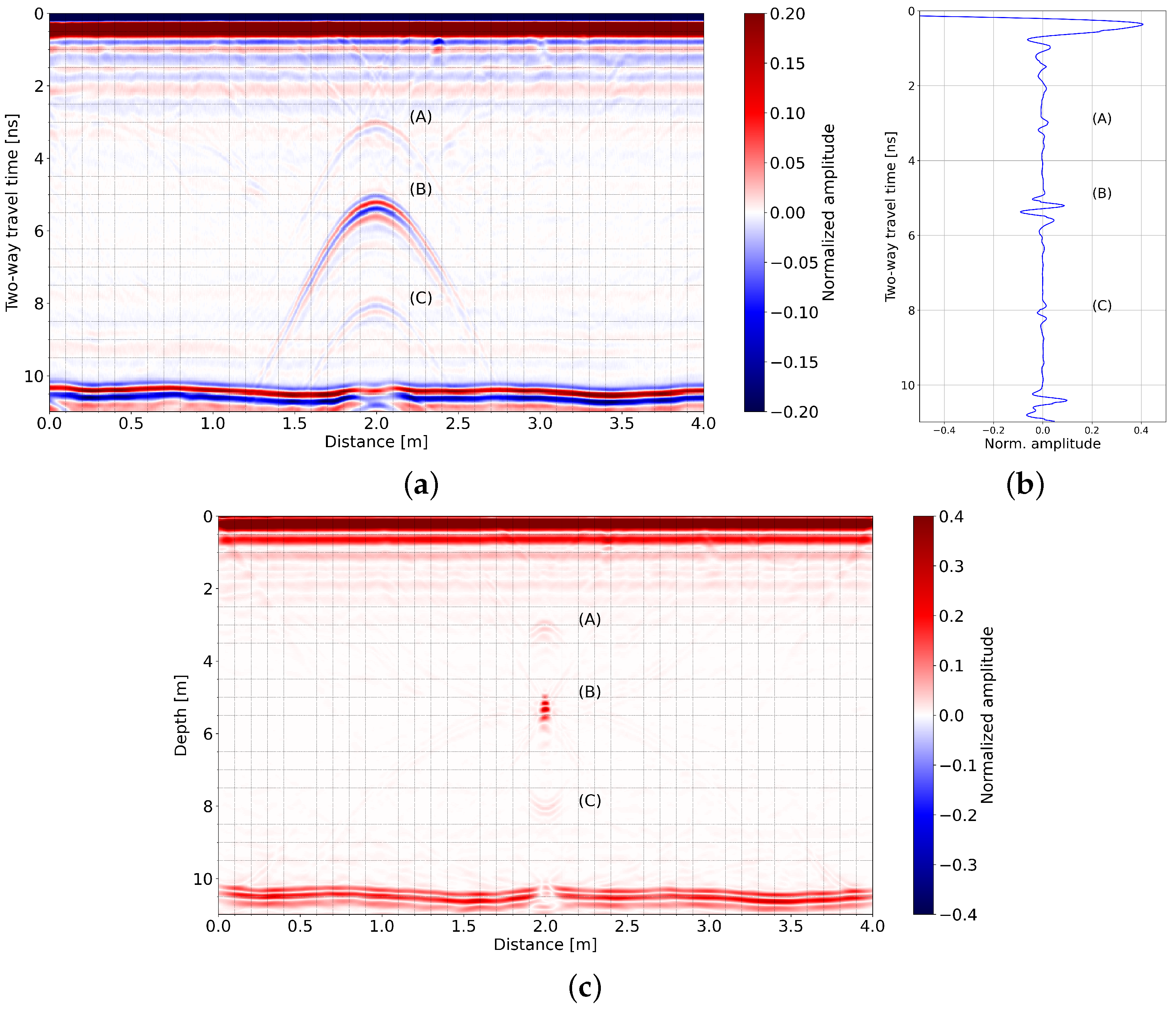
2.2. Results
3. Numerical Experiments
3.1. Cylinder in Dry Sand Conditions
3.2. Cylinder versus Sphere Simulation
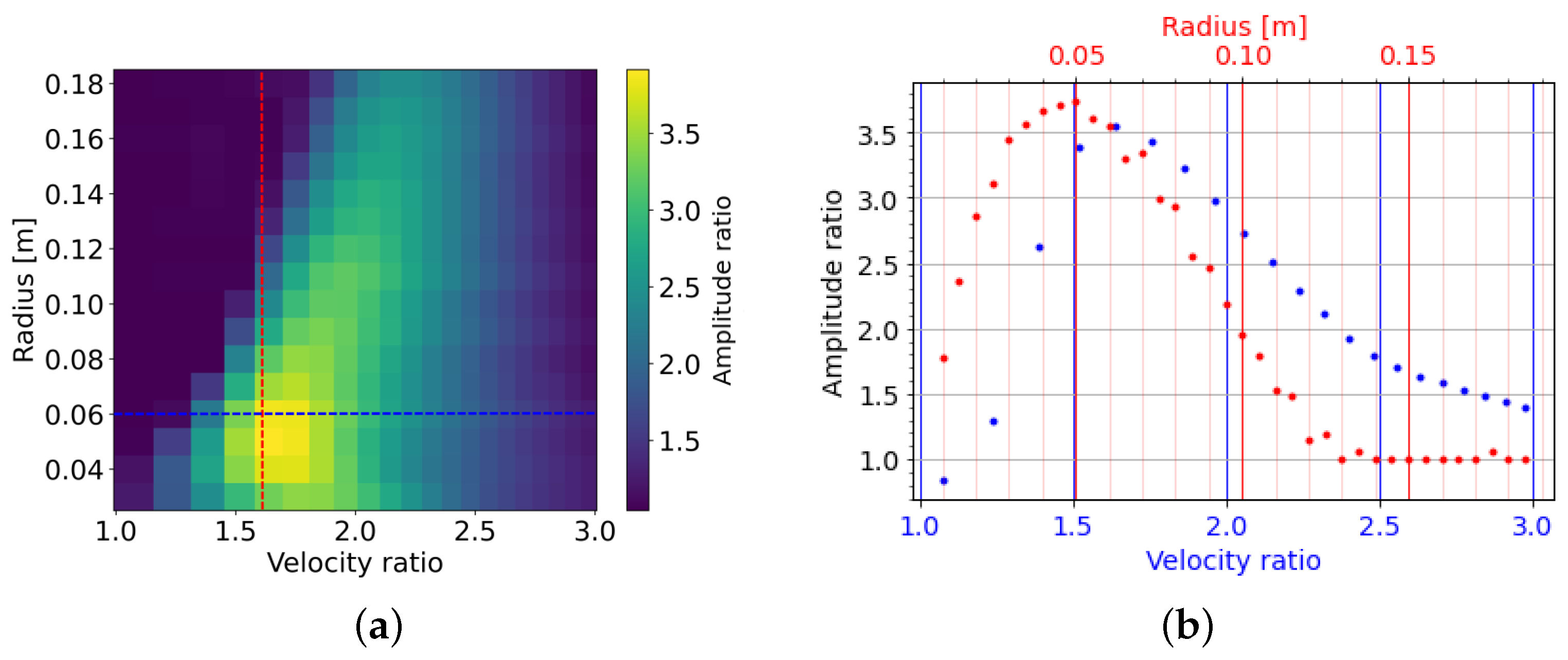
3.3. Amplification Sensitivity to the Anomaly Size and Velocity
3.4. Leaking Pipe Model
4. Field Test-Case Experiment
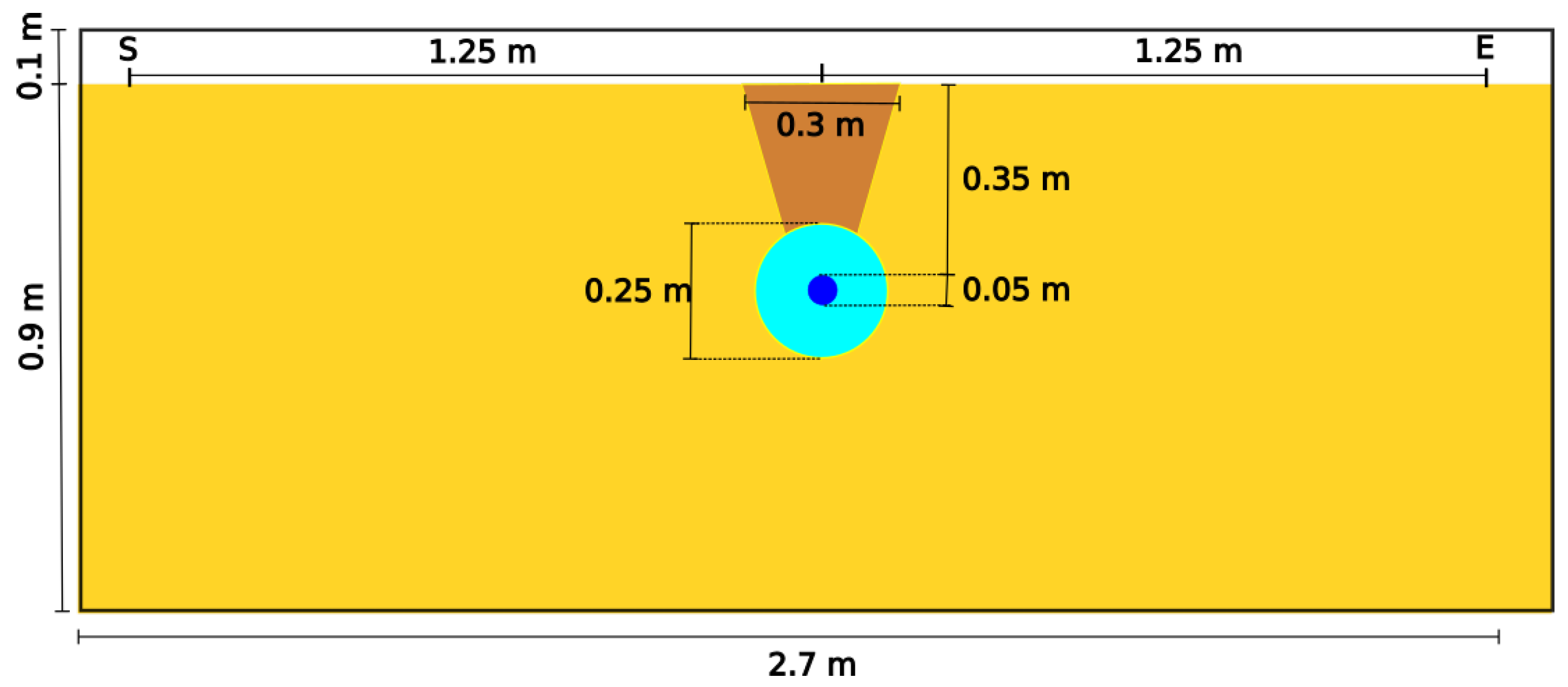
4.1. Setup
4.2. Results
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pachauri, R.K.; Allen, M.R.; Barros, V.R.; Broome, J.; Cramer, W.; Christ, R.; Church, J.A.; Clarke, L.; Dahe, Q.; Dasgupta, P.; et al. Climate Change 2014: Synthesis Report. Contribution of Working Groups I, II and III to the fifth Assessment Report of the Intergovernmental Panel on Climate Change; IPCC: Geneva, Switzerland, 2014. [Google Scholar]
- Li, W.; Ling, W.; Liu, S.; Zhao, J.; Liu, R.; Chen, Q.; Qiang, Z.; Qu, J. Development of systems for detection, early warning, and control of pipeline leakage in drinking water distribution: A case study. J. Environ. Sci. 2011, 23, 1816–1822. [Google Scholar] [CrossRef]
- Robertshaw, J.; Brown, P.D. Geophysical methods of exploration and their application to civil engineering problems. Proc. Inst. Civ. Eng. 1955, 4, 644–676. [Google Scholar] [CrossRef]
- Shön, J. Physical Properties of Rocks: Fundamentals and Principles of Petrophysics; Elsevier: Amsterdam, The Netherlands, 2015; Volume 65. [Google Scholar]
- Bièvre, G.; Lacroix, P.; Oxarango, L.; Goutaland, D.; Monnot, G.; Fargier, Y. Integration of geotechnical and geophysical techniques for the characterization of a small earth-filled canal dyke and the localization of water leakage. J. Appl. Geophys. 2017, 139, 1–15. [Google Scholar] [CrossRef]
- Cataldo, A.; Persico, R.; Leucci, G.; De Benedetto, E.; Cannazza, G.; Matera, L.; De Giorgi, L. Time domain reflectometry, ground penetrating radar and electrical resistivity tomography: A comparative analysis of alternative approaches for leak detection in underground pipes. NDT E Int. 2014, 62, 14–28. [Google Scholar] [CrossRef]
- Olhoeft, G.R. Maximizing the information return from ground penetrating radar. J. Appl. Geophys. 2000, 43, 175–187. [Google Scholar] [CrossRef]
- Ristic, A.V.; Petrovacki, D.; Govedarica, M. A new method to simultaneously estimate the radius of a cylindrical object and the wave propagation velocity from GPR data. Comput. Geosci. 2009, 35, 1620–1630. [Google Scholar] [CrossRef]
- Leparoux, D.; Gibert, D.; Cote, P. Adaptation of prestack migration to multi-offset Ground Penetrating Radar (GPR) data. Geophys. Prospect. 2001, 49, 374–386. [Google Scholar] [CrossRef]
- Sagnard, F.; Norgeot, C.; Derobert, X.; Baltazart, V.; Merliot, E.; Derkx, F.; Lebental, B. Utility detection and positioning on the urban site Sense-City using Ground-Penetrating Radar systems. Measurement 2016, 88, 318–330. [Google Scholar] [CrossRef]
- Klotzsche, A.; van der Kruk, J.; Linde, N.; Doetsch, J.; Vereecken, H. 3-D characterization of high-permeability zones in a gravel aquifer using 2-D crosshole GPR full-waveform inversion and waveguide detection. Geophys. J. Int. 2013, 195, 932–944. [Google Scholar] [CrossRef]
- Jazayeri, S.; Klotzsche, A.; Kruse, S. Improving estimates of buried pipe diameter and infilling material from ground-penetrating radar profiles with full-waveform inversion. Geophysics 2018, 83, H27–H41. [Google Scholar] [CrossRef]
- Nakhkash, M.; Mahmood-Zadeh, M.R. Water leak detection using ground penetrating radar. In Proceedings of the Tenth International Conference on Grounds Penetrating Radar, GPR 2004, Delft, The Netherlands, 21–24 June 2004; pp. 525–528. [Google Scholar]
- Crocco, L.; Prisco, G.; Soldovieri, F.; Cassidy, N. Early-stage leaking pipes GPR monitoring via microwave tomographic inversion. J. Appl. Geophys. 2009, 67, 270–277. [Google Scholar] [CrossRef]
- Demirci, S.; Yigit, E.; Eskidemir, I.H.; Ozdemir, C. Ground penetrating radar imaging of water leaks from buried pipes based on back-projection method. Ndt E Int. 2012, 47, 35–42. [Google Scholar] [CrossRef]
- Lai, W.W.; Chang, R.K.; Sham, J.F.; Pang, K. Perturbation mapping of water leak in buried water pipes via laboratory validation experiments with high-frequency ground penetrating radar (GPR). Tunn. Undergr. Space Technol. 2016, 52, 157–167. [Google Scholar] [CrossRef]
- Adler, C.L.; Lock, J.A.; Stone, B.R.; Garcia, C.J. High-order interior caustics produced in scattering of a diagonally incident plane wave by a circular cylinder. J. Opt. Soc. Am. A 1997, 14, 1305–1315. [Google Scholar] [CrossRef]
- Lock, J.A.; Adler, C.L.; Hovenac, E.A. Exterior caustics produced in scattering of a diagonally incident plane wave by a circular cylinder: Semiclassical scattering theory analysis. J. Opt. Soc. Am. A 2000, 17, 1846–1856. [Google Scholar] [CrossRef]
- Chen, Z.; Taflove, A.; Backman, V. Photonic nanojet enhancement of backscattering of light by nanoparticles: A potential novel visible-light ultramicroscopy technique. Opt. Express 2004, 12, 1214–1220. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Lecler, S. Light Scattering by Sub-Micrometric Particles. Ph.D. Thesis, Université Louis Pasteur, Strasbourg, France, 2005. [Google Scholar]
- Van de Hulst, H.C. Light Scattering by Small Particles John; Wiley & Sons Inc.: New York, NY, USA, 1957; Volume 470. [Google Scholar]
- Rheinstein, J. Backscatter from spheres: A short pulse view. IEEE Trans. Antennas Propag. 1968, 16, 89–97. [Google Scholar] [CrossRef]
- Saintenoy, A.; Schneider, S.; Tucholka, P. Evaluating Ground Penetrating Radar use for water infiltration monitoring. Vadose Zone J. 2008, 7, 208–214. [Google Scholar] [CrossRef]
- Léger, E.; Saintenoy, A.; Tucholka, P.; Coquet, Y. Hydrodynamic parameters of a sandy soil determined by ground-penetrating radar monitoring of Porchet infiltrations. IEEE J. Sel. Top. Appl. Earth Obs. Remote. Sens. 2015, 9, 188–200. [Google Scholar] [CrossRef]
- Arcone, S.A. The subbottom stratigraphy of Mirror Lake, Woodstock, NH: GPR profiles of Gyttja, glacial deposits and till. In Proceedings of the 15th International Conference on GPR, Brussels, Belgium, 30 June–4 July 2014. [Google Scholar]
- Arcone, S.A. Sedimentary architecture beneath lakes subjected to storms: Control by turbidity current bypass and turbidite armouring, interpreted from ground-penetrating radar images. Sedimentology 2018, 65, 1413–1446. [Google Scholar] [CrossRef]
- Arcone, S.A.; Yankielun, N.E. 1.4 GHz radar penetration and evidence of drainage structures in temperate ice: Black Rapids Glacier, Alaska, USA. J. Glaciol. 2000, 46, 477–490. [Google Scholar] [CrossRef]
- Rignot, E.J.; Ostro, S.J.; Van Zyl, J.J.; Jezek, K.C. Unusual radar echoes from the Greenland ice sheet. Science 1993, 261, 1710–1713. [Google Scholar] [CrossRef] [PubMed]
- Hagfors, T.; Gold, T.; Ierkic, H.M. Refraction scattering as origin of the anomalous radar returns of Jupiter’s satellites. Nature 1985, 315, 637–640. [Google Scholar] [CrossRef]
- Hagfors, T.; Dahlstrøm, I.; Gold, T.; Hamran, S.E.; Hansen, R. Refraction scattering in the anomalous reflections from icy surfaces. Icarus 1997, 130, 313–322. [Google Scholar] [CrossRef]
- Le Gall, A.; Janssen, M.; Paillou, P.; Lorenz, R.; Wall, S.; The Cassini Radar Team. Radar-bright channels on Titan. Icarus 2010, 207, 948–958. [Google Scholar] [CrossRef]
- Fenske, K.; Misra, D. Dielectric materials at microwave frequencies. Appl. Microw. Wirel. 2000, 12, 92–100. [Google Scholar]
- Stolt, R. Migration by Fourier transform. Geophysics 1978, 43, 23–48. [Google Scholar] [CrossRef]
- Warren, C.; Giannopoulos, A.; Giannakis, I. GprMax: Open source software to simulate electromagnetic wave propagation for Ground Penetrating Radar. Comput. Phys. Commun. 2016, 209, 163–170. [Google Scholar] [CrossRef]
- Yee, K. Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar]
- Saintenoy, A.; Léger, E.; Grenier, C.; Thiéry, N. Perspectives in Ground-Penetrating Radar at High Latitudes: From Occasional Imaging to Automated Continuous Monitoring. Eur. Assoc. Geosci. Eng. 2021, 2021, 1–5. [Google Scholar] [CrossRef]




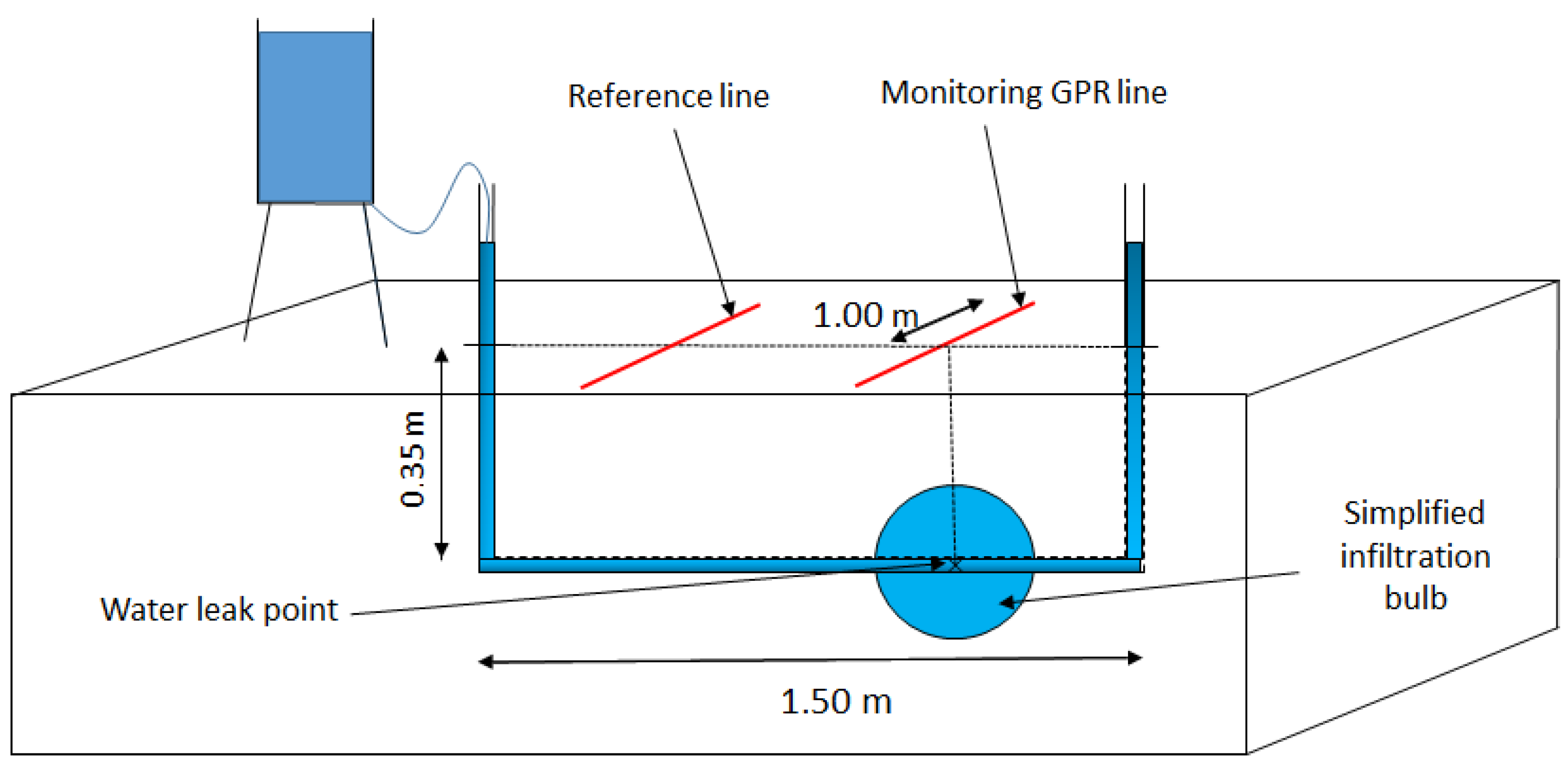
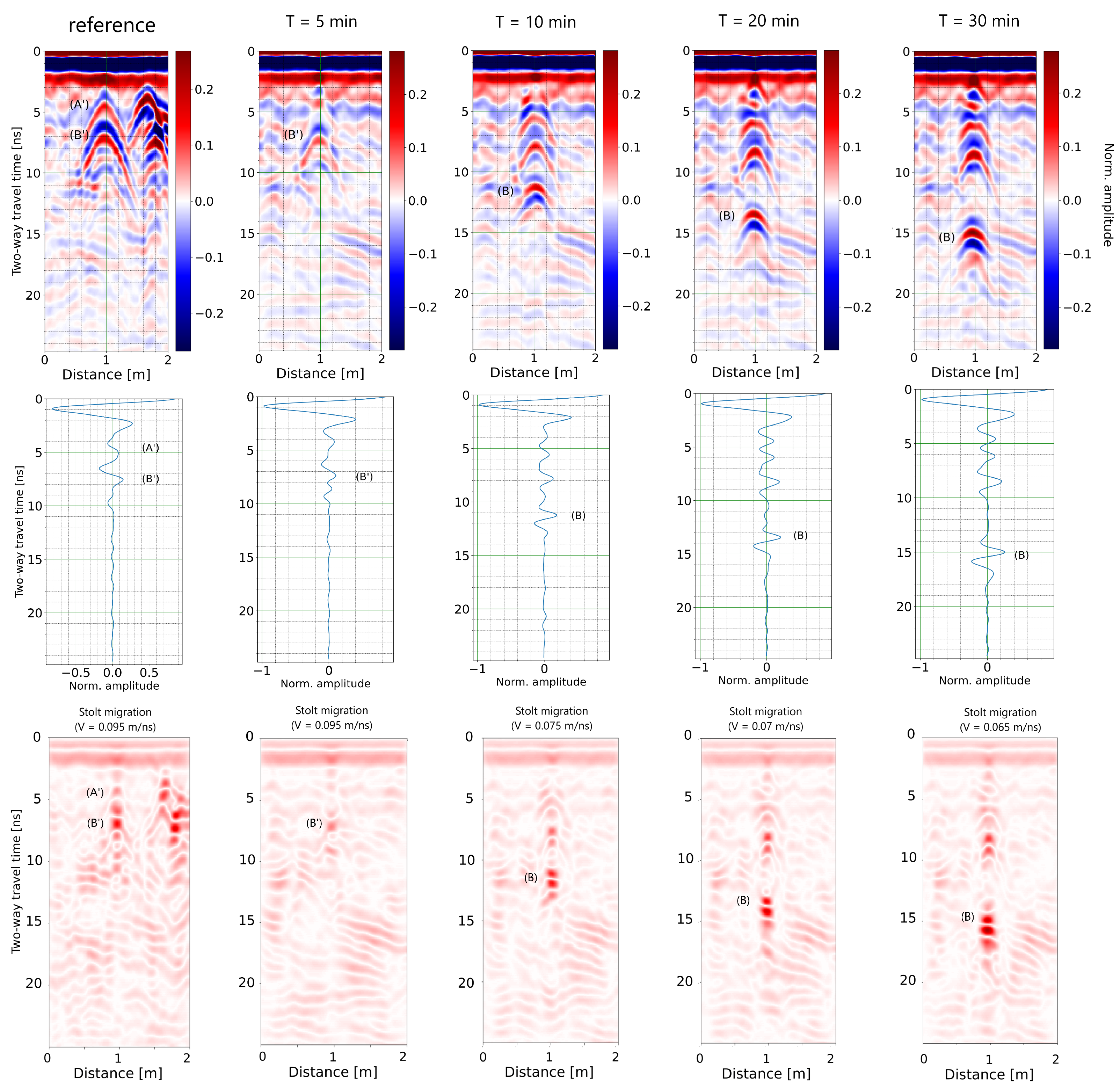
| Radargram | TWT (ns) | Velocity (m/ns) | Depth (m) | Amplitude () |
|---|---|---|---|---|
| Reference | 7.5 | 0.095 ± 0.005 | 0.35 ± 0.02 | 0.4 |
| 5 min | 7.5 | 0.095 ± 0.005 | 0.35 ± 0.02 | 0.3 |
| 10 min | 11.3 | 0.075 ± 0.005 | 0.43 ± 0.03 | 0.55 |
| 20 min | 13.5 | 0.07 ± 0.005 | 0.47 ± 0.035 | 0.6 |
| 30 min | 15 | 0.065 ± 0.005 | 0.49 ± 0.038 | 0.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carrive, P.; Saintenoy, A.; Léger, E.; Arcone, S.A.; Sailhac, P. Exploiting Ground-Penetrating Radar Signal Enhancements by Water-Saturated Bulb Surrounding Defective Waterpipes for Leak Detection. Geosciences 2022, 12, 368. https://doi.org/10.3390/geosciences12100368
Carrive P, Saintenoy A, Léger E, Arcone SA, Sailhac P. Exploiting Ground-Penetrating Radar Signal Enhancements by Water-Saturated Bulb Surrounding Defective Waterpipes for Leak Detection. Geosciences. 2022; 12(10):368. https://doi.org/10.3390/geosciences12100368
Chicago/Turabian StyleCarrive, Pierre, Albane Saintenoy, Emmanuel Léger, Steven A. Arcone, and Pascal Sailhac. 2022. "Exploiting Ground-Penetrating Radar Signal Enhancements by Water-Saturated Bulb Surrounding Defective Waterpipes for Leak Detection" Geosciences 12, no. 10: 368. https://doi.org/10.3390/geosciences12100368
APA StyleCarrive, P., Saintenoy, A., Léger, E., Arcone, S. A., & Sailhac, P. (2022). Exploiting Ground-Penetrating Radar Signal Enhancements by Water-Saturated Bulb Surrounding Defective Waterpipes for Leak Detection. Geosciences, 12(10), 368. https://doi.org/10.3390/geosciences12100368









