Physics and Modeling of Various Hazardous Landslides
Abstract
1. Introduction
2. Landslides, Types and Classification
3. Physical Laws of Landslide Motion
3.1. Slope Stability, Rupture Lines and Zones
3.2. The Physical Laws of Landslide Motion and Associated Equations
3.2.1. Types (i) and (ii)
3.2.2. Type (iii)
4. Subaqueous Slides and Tsunamis
5. Constructions of Landslide Models
5.1. Integral Models
5.1.1. Type (i)
5.1.2. Type (ii)
5.2. Pseudostationary Flow and Slope Stability
5.3. Translatory Wave Theory Used on Landslide Flow
6. Case Studies of Hazards, Probability and Mitigation
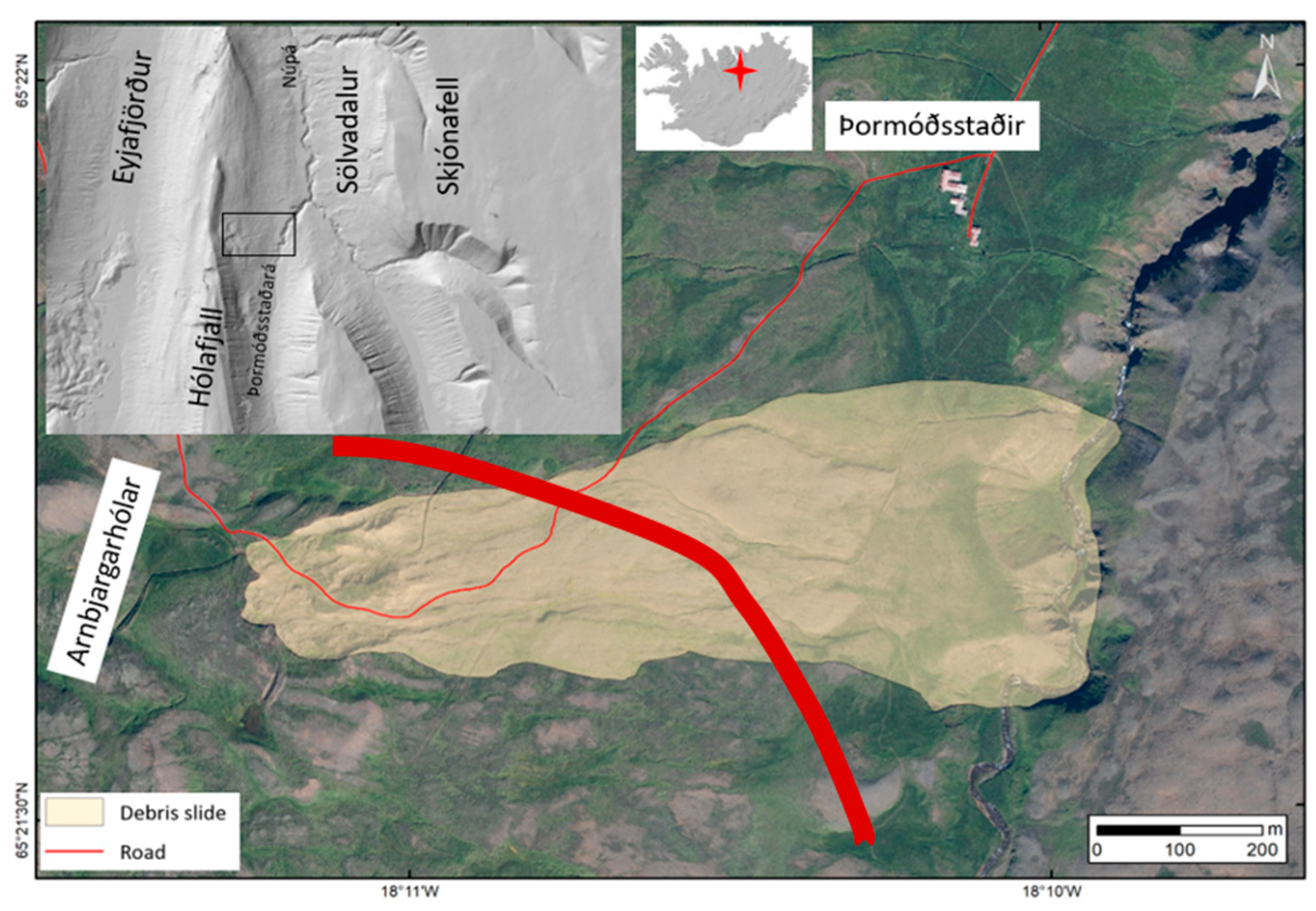
6.1. Case Study A. Landslide in the Sölvadalur Valley, North Iceland
6.1.1. The Event
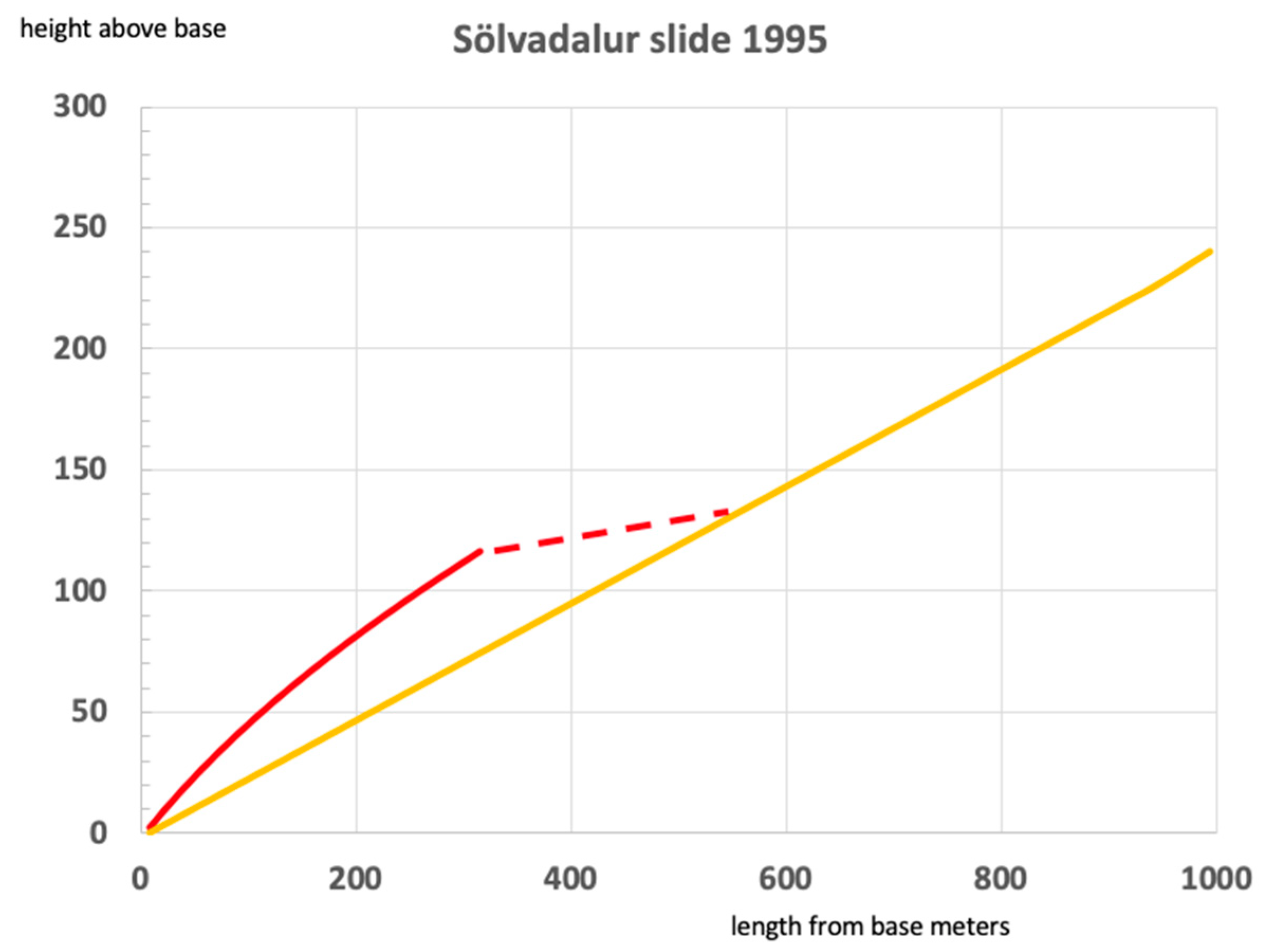
6.1.2. The Slide
6.1.3. Evaluation
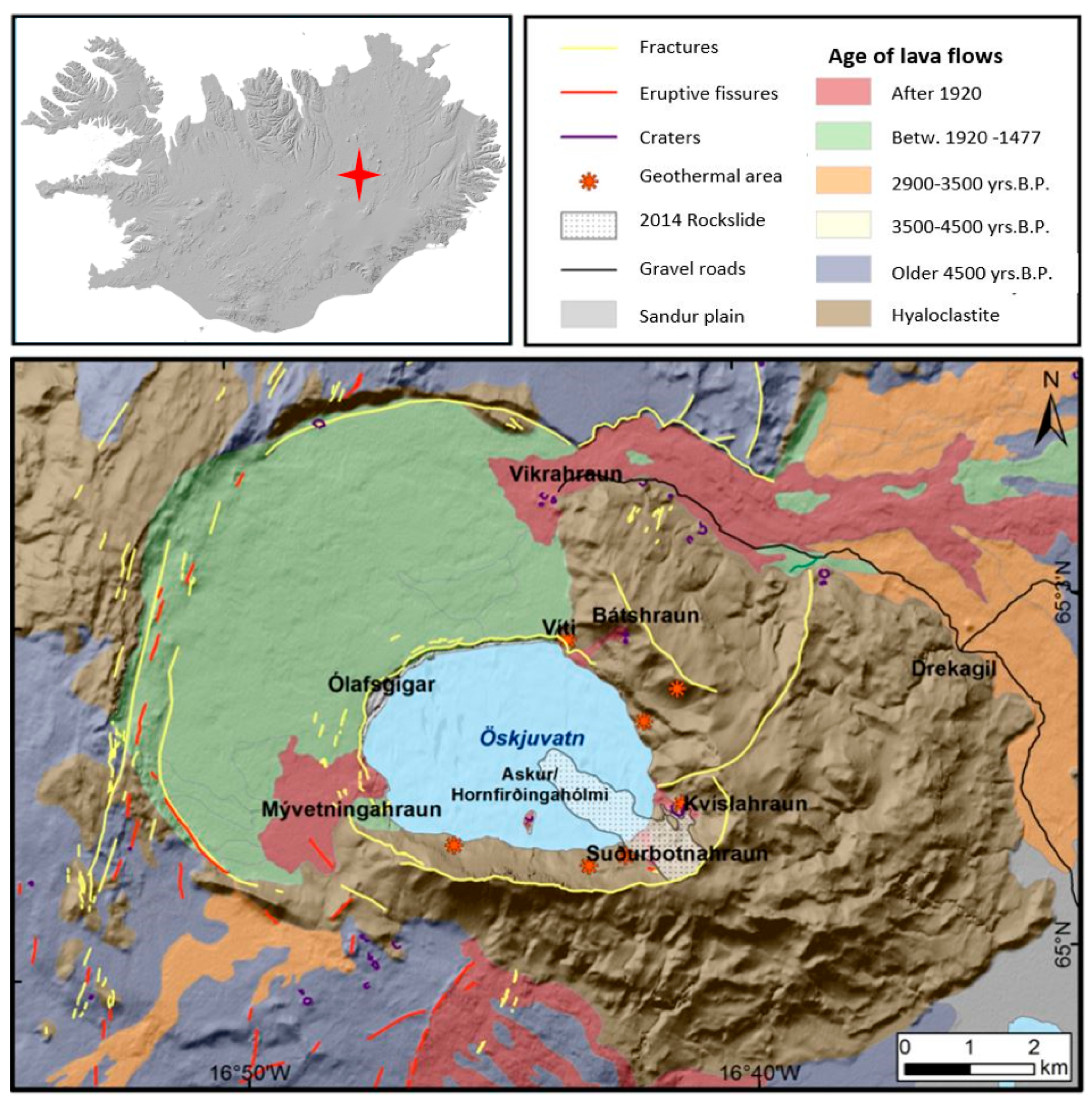
6.2. Case Study B: The Rockslide in the Askja Caldera in East Iceland
6.2.1. The Event
6.2.2. The Slide
6.2.3. Evaluation
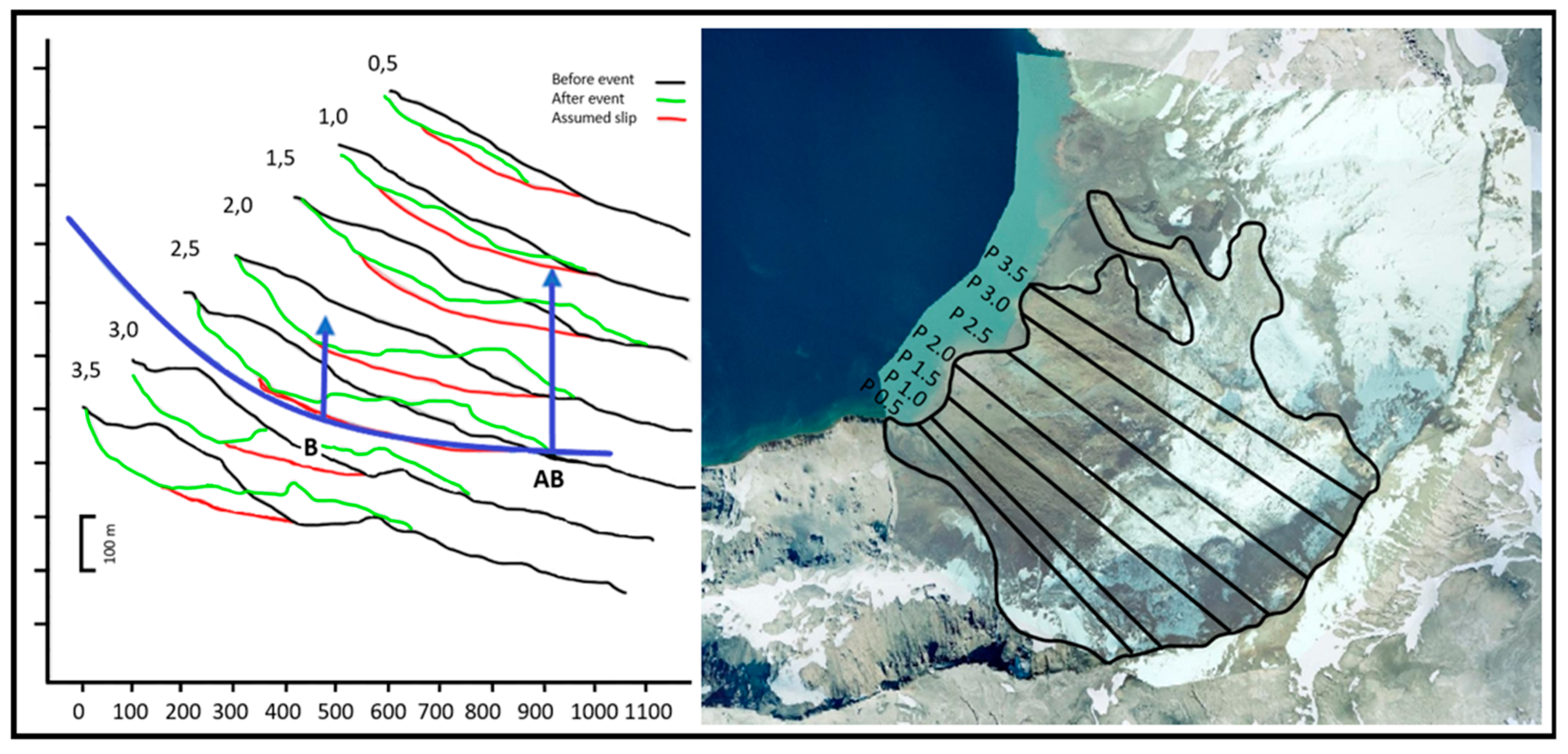
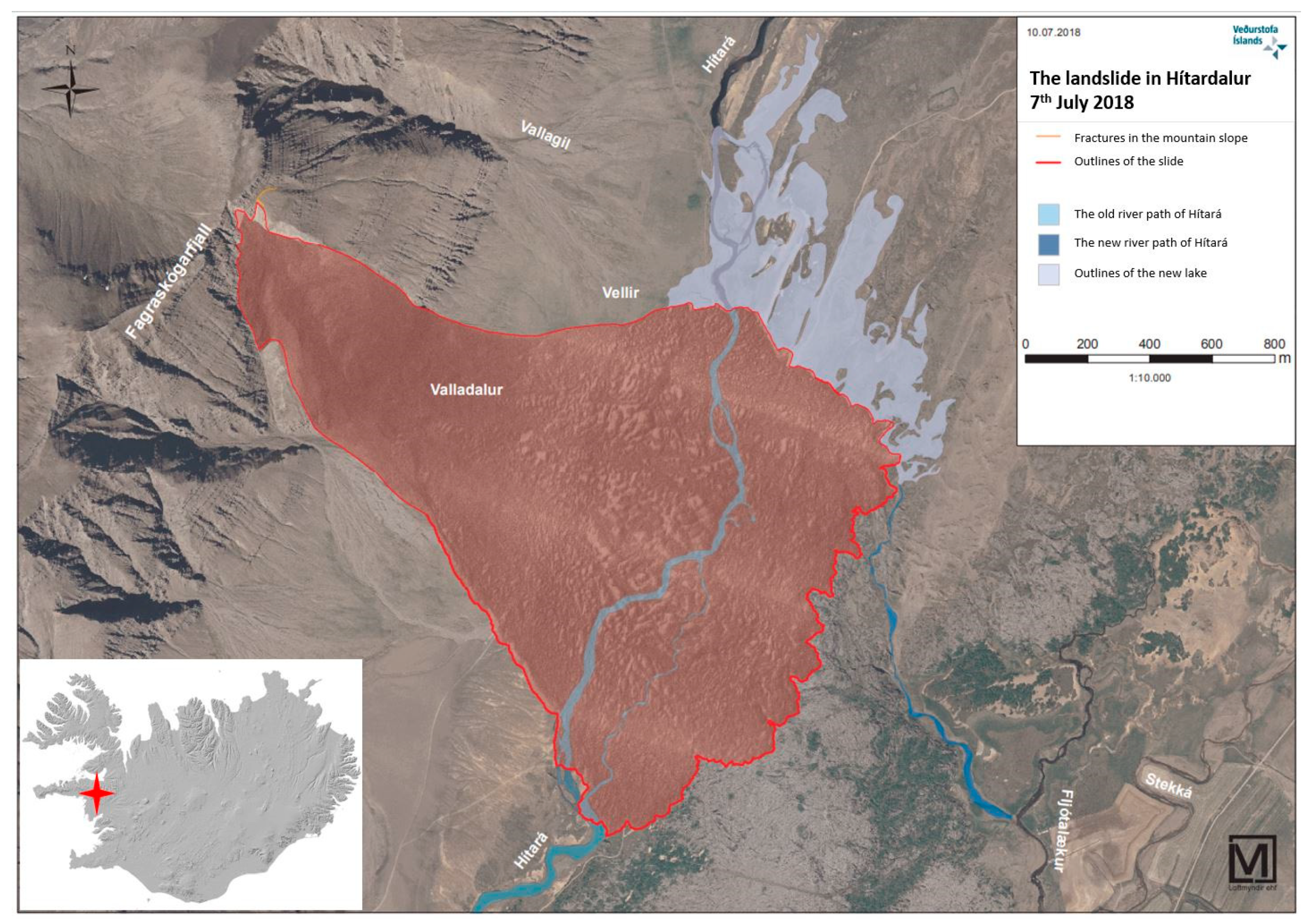
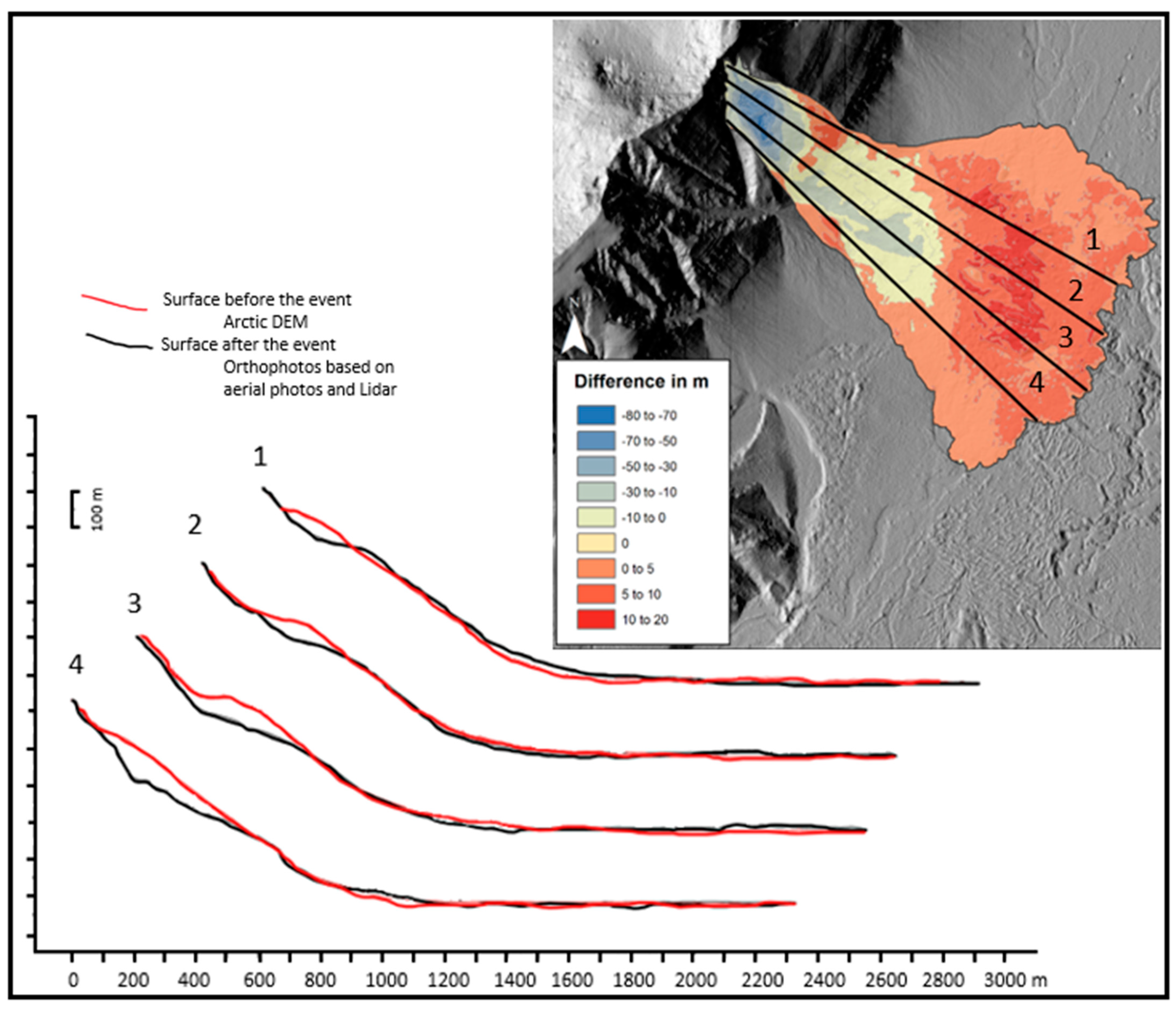
6.3. The Landslide in Mt. Fagraskógarfjall in the Hítardalur Valley, West Iceland
6.3.1. The Event
6.3.2. The Slide
7. Discussion
8. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Casagli, N.; Frodella, W.; Morelli, S.; Tofani, V.; Ciampalini, A.; Intrieri, E.; Lu, P. Spaceborne, UAV and ground-based remote sensing techniques for landslide mapping, monitoring and early warning. Geoenviron. Disasters 2017, 4, 9. [Google Scholar]
- Arabameri, A.; Karimi-Sangchini, E.; Pal, S.C.; Saha, A.; Chowdhuri, I.; Lee, S.; Bui, D.T. Novel Credal Decision Tree-Based Ensemble Approaches for Predicting the Landslide Susceptibility. Remote. Sens. 2020, 12, 3389. [Google Scholar] [CrossRef]
- Chowdhuri, I.; Pal, S.C.; Arabameri, A.; Ngo, P.T.T.; Chakrabortty, R.; Malik, S.; Das, B.; Roy, P. Ensemble approach to develop landslide susceptibility map in landslide dominated Sikkim Himalayan region, India. Environ. Earth Sci. 2020, 79, 1–28. [Google Scholar] [CrossRef]
- Pal, S.C.; Das, B.; Malik, S. Potential Landslide Vulnerability Zonation Using Integrated Analytic Hierarchy Process and GIS Technique of Upper Rangit Catchment Area, West Sikkim, India. J. Indian Soc. Remote. Sens. 2019, 47, 1643–1655. [Google Scholar] [CrossRef]
- Pal, S.C.; Chowdhuri, I. GIS-based spatial prediction of landslide susceptibility using frequency ratio model of Lachung River basin, North Sikkim, India. SN Appl. Sci. 2019, 1, 416. [Google Scholar] [CrossRef]
- Pham, B.T.; Prakash, I.; Bui, D.T. Spatial prediction of landslides using a hybrid machine learning approach based on Random Subspace and Classification and Regression Trees. Geomorphol. 2018, 303, 256–270. [Google Scholar] [CrossRef]
- Highland, L.M.; Bobrowsky, P. The Landslide Handbook—A Guide to Understanding Landslides, United States Geological Survey, Landslide Program and National Landslide Information Center, Mail Stop 966, Box 25046; Denver Fed-eral Center: Denver, CO, USA, 2008. Available online: https://pubs.usgs.gov/circ/1325/ (accessed on 23 February 2021).
- Komac, M. A landslide susceptibility model using the Analytical Hierarchy Process method and multivariate statistics in perialpine Slovenia. Geomorphology 2006, 74, 17–28. [Google Scholar] [CrossRef]
- Guzzetti, F.; Malamud, B.D.; Turcotte, D.L.; Reichenbach, P. Power-law correlations of landslide areas in central Italy. Earth Planet. Sci. Lett. 2002, 195, 169–183. [Google Scholar] [CrossRef]
- Masson, D.G.; Harbitz, C.B.; Wynn, R.B.; Pedersen, G.; Løvholt, F. Subaqueous landslides: Processes, triggers and hazard prediction. Philosophical Transactions of the Royal Society A: Mathematical. Phys. Eng. Sci. 2006, 364, 2009–2039. [Google Scholar]
- Sæmundsson, Þ.; Morino, C.; Helgason, J.K.; Conway, S.J.; Pétursson, H.G. The triggering factors of the Móafellshyrna debris slide in northern Iceland: Intense precipitation, earthquake activity and thawing of mountain permafrost. Sci. Total. Environ. 2018, 621, 1163–1175. [Google Scholar] [CrossRef]
- Elíasson, J. Initial Wave Height and Total Energy of Landslide-Generated Tsunamis from Translatory Wave Theory. In Proceedings of the Geotechnics and Earthquake Geotechnics towards Global Sustainability; Springer International Publishing: Berlin/Heidelberg, Germany, 2018; pp. 159–174. [Google Scholar]
- Eliasson, J. Earthquake-Generated Landslides and Tsunamis. In Earthquakes—Impact, Community Vulnerability and Resilience; IntechOpen: London, UK, 2019. [Google Scholar]
- Cruden, D.M. A simple definition of a landslide. Bull. Int. Assoc. Eng. Geol. 1991, 43, 27–29. [Google Scholar] [CrossRef]
- Varnes, D.J. Slope Movement Types and Processes. Transportation Research Board Special Report: 11–33. In Special Report 176: Landslides: Analysis and Control; Transportation Research Board: Washington, DC, USA, 1978. [Google Scholar]
- Cruden, D.M.; Varnes, D. Landslide types and processes. In Landslides Investigation and Mitigation; Transportation Research Board: Washington, DC, USA, 1996; pp. 36–75. [Google Scholar]
- Hungr, O.; Evans, S.G.; Bovis, M.J.; Hutchinson, J.N. A review of the classification of landslides of the flow type. Environ. Eng. Geosci. 2001, 7, 221–238. [Google Scholar] [CrossRef]
- Hutchinson, J.H. Morphological and Geotechnical Parameters of Landslides in Relation to Geology and Hydrogeology. In Proceedings of the 4th International Symposium on Landslides; Balkema: Rotterdam, The Netherlands, 1988; pp. 3–35. [Google Scholar]
- Julian, M.; Anthony, E.J. Landslides and climatic variables with specific reference to the Maritime Alps of southeast-ern France. In Temporal Occurrence and Forecasting of Landslides in the European Community, Programme EPOCH; Casale, R., Fantechi, R., Flageolle, J.C., Eds.; European Commission: Brussels, Belgium, 1994; pp. 697–721. [Google Scholar]
- Hungr, O.; Leroueil, S.; Picarelli, L. The Varnes classification of landslide types, an update. Landslides 2014, 11, 167–194. [Google Scholar] [CrossRef]
- Casadei, M.; Dietrich, W.E.; Miller, N.L. Testing a model for predicting the timing and location of shallow land-slide initiation in soil-mantled landscapes. Earth Surface Process. Landf. 2003, 28, 925–950. [Google Scholar] [CrossRef]
- Crozier, M.J. Prediction of rainfall-triggered landslides: A test of the antecedent water status model. Earth Surface Process. Landf. 1999, 24, 825–833. [Google Scholar] [CrossRef]
- Tsai, T.-L.; Yang, J.-C. Modeling of rainfall-triggered shallow landslide. Environ. Earth Sci. 2006, 50, 525–534. [Google Scholar] [CrossRef]
- Hergarten, S.; Neugebauer, H.J. Self-organized criticality in a landslide model. Geophys. Res. Lett. 1998, 25, 801–804. [Google Scholar] [CrossRef]
- Hussin, H.Y.; Luna, B.Q.; Van Westen, C.J.; Christen, M.; Malet, J.P.; Van Asch, T.W. Parameterization of a nu-merical 2-D debris flow model with entrainment: A case study of the Faucon catchment, Southern French Alps. Nat. Hazards Earth Syst. Sci. 2012, 12, 3075–3090. [Google Scholar] [CrossRef]
- Farahmand, A.M.; Aghakouchak, A.A. A satellite-based global landslide model. Nat. Hazards Earth Syst. Sci. 2013, 13, 1259–1267. [Google Scholar] [CrossRef]
- Terzaghi, K. Theoretical Soil Mechanics; Wiley: New York, NY, USA, 1943. [Google Scholar]
- Salvatici, T.; Di Roberto, A.; Di Traglia, F.; Bisson, M.; Morelli, S.; Fidolini, F.; Bertagnini, A.; Pompilio, M.; Hungr, O.; Casagli, N. From hot rocks to glowing avalanches: Numerical modelling of gravity-induced pyroclastic density currents and hazard maps at the Stromboli volcano (Italy). Geomorphology 2016, 273, 93–106. [Google Scholar] [CrossRef]
- Sottili, G.; Martino, S.; Palladino, D.M.; Paciello, A.; Bozzano, F. Effects of tidal stresses on volcanic activity at Mount Etna, Italy. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Elíasson, J.; Sæmundsson, Þ. The Translatory Wave Model for Landslides. In Landslides—Investigation and Monitoring; IntechOpen: London, UK, 2020. [Google Scholar]
- Eliasson, J.; Kjaran, S.P.; Holm, S.L.; Gudmundsson, M.T.; Larsen, G. Large hazardous floods as translatory waves. Environ. Model. Softw. 2007, 22, 1392–1399. [Google Scholar] [CrossRef]
- Pedersen, F.B. Environmental Hydraulics: Stratified Flows: Stratified Flows; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Bryant, E. Tsunami: The Underrated Hazard, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Lovholt, F.; Harbitz, C.B.; Haugen, K.B. A parametric study of tsunamis generated by subaqueous slides in the Ormen Lange/Storegga area off western Norway. Mar. Pet. Geol. 2005, 22, 219–231. [Google Scholar] [CrossRef]
- Bondevik, S.; Mangerud, J.; Dawson, S.; Dawson, A.; Lohne, Ø. Record-breaking height for 8000-year-old tsunami in the North Atlantic. Eos 2003, 84, 289–293. [Google Scholar] [CrossRef]
- Grauert, M.; Bjorck, S.; Bondevik, S. Storegga tsunami deposits in a coastal lake on Suduroy, the Faroe Islands. Boreas 2001, 30, 263–271. [Google Scholar] [CrossRef]
- Yeh, H.; Chadha, R.K.; Francis, M.; Katada, T.; Latha, G.; Peterson, C.; Raghuraman, G.; Singh, J.P. Tsunami Runup Survey along the Southeast Indian Coast. Earthq. Spectra 2006, 22, 173–186. [Google Scholar] [CrossRef]
- Mori, N.; Takahashi, T.; Yasuda, T.; Yanagisawa, H. Survey of 2011 Tohoku earthquake tsunami inundation and run-up. Geophys. Res. Lett. 2011, 38. [Google Scholar] [CrossRef]
- Grilli, S.T.; Taylor, O.D.S.; Baxter, C.D.P.; Maretzki, S. A probabilistic approach for determining subaqueous land-slide tsunami hazard along the upper east coast of the United States. Mar. Geol. 2009, 264, 74–97. [Google Scholar] [CrossRef]
- Gylfadóttir, S.S.; Kim, J.; Helgason, J.K.; Brynjólfsson, S.; Höskuldsson, Á.; Jóhannesson, T.; Harbitz, C.B.; Løvholt, F. The 2014 Lake Askja rockslide-induced tsunami: Optimization of numerical tsunami model using observed data. J. Geophys. Res. Oceans 2017, 122, 4110–4122. [Google Scholar] [CrossRef]
- L’Heureux, J.-S.; Locat, A.; Leroueil, S.; Demers, D.; Locat, J. (Eds.) Landslides in Sensitive Clays—From Geosciences to Risk Management. In Advances in Natural and Technological Hazards Research; Springer: Berlin/Heidelberg, Germany, 2014; Volume 36. [Google Scholar]
- McDougall, S.; Hungr, O. A model for the analysis of rapid landslide motion across three-dimensional terrain. Can. Geotech. J. 2004, 41, 1084–1097. [Google Scholar] [CrossRef]
- Quinn, P.; Diederichs, M.; Rowe, R.; Hutchinson, D. A new model for large landslides in sensitive clay using a fracture mechanics approach. Can. Geotech. J. 2011, 48, 1151–1162. [Google Scholar] [CrossRef]
- Locat, A.; Locat, P.; Demers, D.; Leroueil, S.; Robitaille, D.; Lefebvre, G. The Saint-Jude landslide of 10 May 2010, Quebec, Canada: Investigation and characterization of the landslide and its failure mechanism. Can. Geotech. J. 2017, 54, 1357–1374. [Google Scholar] [CrossRef]
- Amirahmadi, A.; Pourhashemi, S.; Karami, M.; Akbari, E. Modeling of landslide volume estimation. Open Geosci. 2016, 8, 360–370. [Google Scholar] [CrossRef][Green Version]
- Pétursson, H.G. Potential danger of landslides in Sölvadalur, Report to the Icelandic Civil Defense Authorities; Icelandic Government: Akureyri, Iceland, 1997. (In Icelandic) [Google Scholar]
- Sæmundsson, Þ.; Pétursson, H.G. The Sölvadalur debris-slide. In Proceedings of the Nordic Geological Winter Meeting, Aarhus, Denmark, 13–16 January 1998. [Google Scholar]
- Pétursson, H.G.; Sæmundsson, Þ. The 1995 Sölvadalur debris slide in Central North Iceland. In Proceedings of the First Science Meeting of the European Science Foundation ESF—Network SEDIFLUX, Sauðárkrókur, Iceland, 18–21 June 2004; Beylich, A.A., Sæmundsson, Þ., Decaulne, A., Sandberg, O., Eds.; European Science Foundation (ESF): Strasbourg, France, 2004. [Google Scholar]
- Moorbath, S.; Sigurdsson, H.; Goodwin, R. K-Ar ages of oldest exposed rocks in Iceland. Earth Planet. Sci. Lett. 1968, 26, 197–205. [Google Scholar] [CrossRef]
- McDougall, I.; Kristjansson, L.; Sæmundsson, K. Magnetostratigraphy and geochronology of NW-Iceland. J. Geophys. Res. 1984, 89, 7029–7060. [Google Scholar] [CrossRef]
- Watkins, N.D.; Walker, G.P.L. Magnetostratigraphy of eastern Iceland. Am. J. Sci. 1977, 277, 513–584. [Google Scholar] [CrossRef]
- Sæmundsson, K.; Kristjánsson, L.; McDougal, I.; Warkins, N.D. K-Ar dating, geological and paleomagnetic study of a 5-km lava succession in Northern Iceland. J. Geophys. Res. 1980, 85, 3628–3646. [Google Scholar] [CrossRef]
- Sæmundsson, K. Outline of the geology of Iceland. Jökull 1979, 29, 7–28. [Google Scholar]
- Jóhannesson, H. Yfirlit um jarðfræði Tröllaskaga (Miðskaga); Overview of the Geology of Tröllaskagi; Árbók Ferðafélags Íslands: Reykjavík, Iceland, 1991; pp. 39–56. (In Icelandic) [Google Scholar]
- Pétursson, H.G.; Larsen, G. An early Holocene basaltic tephra bed in North Iceland, a possible equivalent to the Saksunar-vatn Ash Bed. In Proceedings of the 20th Nordic Geological Winter Meeting, Reykjavík, Iceland, 7–10 January 1992; p. 133. [Google Scholar]
- Sæmundsson, Þ.; Helgason, J.K.; Brynjólfsson, S.; Höskuldsson, Á.; Hjartardóttir, Á.R.; Sigmundsson, F. The rockslide in the Askja caldera on 21 July 2014. In Proceedings of the European Geosciences Union, General Assembly 2015, Vienna, Austria, 12–17 April 2015. [Google Scholar]
- Sæmundsson, Þ.; Helgason, J.K.; Brynjólfsson, S.; Höskuldsson, Á.; Hjartardóttir, Á.R.; Sigmundsson, F. What did trigger the rockslide in the Askja caldera on 21 July 2014. In Proceedings of the 32nd Nordic Geological Winter Meeting 2016, Helsinki, Finland, 13–15 January 2015. [Google Scholar]
- Helgason, J.K.; Gylfadóttir, S.S.; Brynjólfsson, S.; Grímsdóttir, H.; Höskuldsson, Á.; Sæmundsson, Þ.; Hjartardóttir, Á.R.; Sigmundsson, F.; Jóhannesson, T. Berghlaupið í Öskju 21. júlí 2014. Náttúrufræðingurinn 2019, 89, 5–21, (The rockslide in Askja, in Icelandic). [Google Scholar]
- Helgason, J.K.; Sæmundsson, Þ.; Drouin, V.; Jóhannesson, T.; Grímsdóttir, H.; Jónsson, M.H.; Gylfadóttir, S.S. The Hítardalur landslide in West Iceland in July 2018. In Proceedings of the 21st EGU General Assembly, Vienna, Austria, 7–12 April 2019. [Google Scholar]
- Ragnarsdóttir, K.V. Jarðlagaskipan Fagraskógarfjalls og Vatnshlíðar í Hítardal (e. Geology of Mt. Fagraskógarfjall and Mt. Vatnshlíðarfjall in Hítardalur Valley, in Icelandic). Bachelor Dissertation, University of Iceland, Reykjavík, Iceland, 1979. [Google Scholar]
- Mulder, T.; Alexander, J. The physical character of subaqueous sedimentary density flows and their deposits. Sedimentology 2001, 48, 269–299. [Google Scholar] [CrossRef]
- Moscardelli, L.; Wood, L. Morphometry of mass-transport deposits as a predictive tool. GSA Bull. 2015, 128, B31221. [Google Scholar] [CrossRef]
- Lee, H.J. Undersea landslides: Extent and significance in the Pacific Ocean, an update. Nat. Hazards Earth Syst. Sci. 2005, 5, 877–892. [Google Scholar] [CrossRef]



| Hillslope | Data | 0.28 | |
|---|---|---|---|
| Length | Data | 1000 | m |
| LVS | Data | 350 | m |
| B | Data | 400 | m |
| VS | Data | 1750 | m |
| c | Data | 12.9 | m/s |
| y0 m | VS/LVS | 5 | m |
| Sf | sin(atan(0,3)) | 0.29 | |
| C | c/√(g y0 Sf) | 3.4 | |
| t | L/c | 13 | s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elíasson, J.; Sæmundsson, Þ. Physics and Modeling of Various Hazardous Landslides. Geosciences 2021, 11, 108. https://doi.org/10.3390/geosciences11030108
Elíasson J, Sæmundsson Þ. Physics and Modeling of Various Hazardous Landslides. Geosciences. 2021; 11(3):108. https://doi.org/10.3390/geosciences11030108
Chicago/Turabian StyleElíasson, Jόnas, and Þorsteinn Sæmundsson. 2021. "Physics and Modeling of Various Hazardous Landslides" Geosciences 11, no. 3: 108. https://doi.org/10.3390/geosciences11030108
APA StyleElíasson, J., & Sæmundsson, Þ. (2021). Physics and Modeling of Various Hazardous Landslides. Geosciences, 11(3), 108. https://doi.org/10.3390/geosciences11030108






