The Equilibrium Concept, or…(Mis)concept in Beaches

Abstract

Beaches, as deposits of unconsolidated material at the land/water interface, are open systems where input and output items constitute the sediment budget. Beach evolution depends on the difference between the input/output to the system; if positive the beach advances, if negative the beach retreats. Is it possible that this difference is zero and the beach is stable? The various processes responsible for sediment input and output in any beach system are here considered by taking examples from the literature. Results show that this can involve movement of a volume of sediments ranging from few, to over a million cubic meters per year, with figures continuously changing so that the statistical possibility for the budget being equal can be considered zero. This can be attributed to the fact that very few processes are feedback-regulated, which is the only possibility for a natural system to be in equilibrium. Usage of the term “beach equilibrium” must be reconsidered and used with great caution.

1. Introduction

Equilibrium is a term having many different meanings. In physics, it describes the average condition of a system, as measured through one of its elements or attributes over a specific period of time. However, the geomorphological concept of equilibrium has many confusing meanings [1], e.g., see below plus, quasi-equilibrium, time independence, and its semantics have been seemingly lost in a vast array of papers, e.g., the authors of [2,3,4,5] showed that catastrophe theory even suggests that any system might have numerous equilibrium states.

Equilibrium theory arises from Newton’s laws of motion (F = MLT⁻²) and refers to where an object’s velocity is constant (no acceleration), or if an object is stationary (at rest) and any force acting on it has its vector sum as zero, i.e., force and reaction are balanced and the system’s properties are unchanged over time. This is static equilibrium. Dean ([6], p.399), referring to beach dynamic equilibrium, defined it as “the tendency for beach geometry to fluctuate about an equilibrium, which also changed through time, but much more slowly.” He added that our ability to predict quantitatively these changes is likely to remain poor for the coming decades.

A system can also exhibit other states:

• Steady: where the average system’s condition trajectory is unchanged through time.
• Stable: where a system has a tendency to return to the same equilibrium state once it experiences disturbance.
• Unstable: where a system returns to a new equilibrium, post any disturbance.
• Static: where reactive forces and moments must balance the externally applied forces and moments.
• Metastable: where additional energy must be introduced before an object can reach true stability.

Further readings about these states may be found in [7,8].

Additionally, Renwick [9] has argued that if one has equilibrium (absence of a discernible trend over the period under observation), then its inverse is a disequilibrium state, i.e., a landform that tends towards equilibrium, but has not had sufficient time to reach it, i.e., a decaying state. The end result is confusion. It is not only with respect to beaches that the term is frequently used; care should be taken in usage of the term, as it has been strewn around the literature in many guises, e.g., equilibrium shoreline evolution models [10]; equilibrium types in planforms of bay beaches [11]. The latter’s findings ranged from “dynamic” planforms where there is a constant sediment throughput to maintain beach stability, to a “static” position, as a result of a reduced/ceasing of an updrift sediment supply. Jackson and Cooper ([11], p.112), further commented that “Static equilibrium models represented a convenient yardstick with which to ascertain a particular shoreline’s current stability status.” However, they urged caution to the approach in identifying equilibrium and non-equilibrium shorelines, mentioning reliance on contemporary beach morphometrics as an input, and omission of other dynamic variables (secondary wave motions, tidal, and river currents).

Its geomorphological origin can be traced to Gilbert’s (1877) classic work on sediment flux at the drainage basin scale in the Henry Mountains, USA [12], later quantified by Ahnert [13], relating to rock erosion and resistance, the concept of a systems approach in geomorphology and negative feedback. It is a process-based approach, and most discussion of the term has been in reference to hydrological processes and concepts. Gilbert [12] argued that all streams worked towards a graded condition (dynamic equilibrium) where the net effect of the stream is neither erosion nor deposition. Willgoose et al. [14] expanded this arguing that within river catchments episodic fluctuations can occur, but over time in dynamic equilibrium, a balance exists between uplift and erosion.

Equilibrium in any system has to be time dependent but the particular attributes must be defined over a particular period, but how is the scale defined? A classic paper on the fundamental importance of scale was that of Schumm and Lichty [15]. They showed that input/output relationships could change within any timespan, leading to the concept of fast and slow variables. Beattie and Oppenheim [16], dealing with thermodynamics, argued that processes can produce different equilibrium states that take place so slowly that no discernible displacement in the presumed equilibrium state can detected. Is this true in geomorphology and particularly in a beach environment? Usage of the equilibrium term as being scale-dependent is perhaps questionable, as it depends on the observer’s view of the term. Howard ([17], p.71) was of the opinion that, “Equilibrium systems are generally not applicable to physical systems that exhibit oscillatory, threshold, hysteretic, multivalued or strongly unpredictable responses to constant or slowly changing inputs.” He further stated that a change of time/scale could make a system giving complex responses be predictable or in equilibrium. Bracken and Wainwright [18] even posed the question whether equilibrium was a myth or a metaphor, arguing for the latter? Is the concept testable? Richards [19] even suggested that it was impossible to measure equilibrium.

For beaches, which are the concern of this paper, equilibrium infers a lack of change in a system (a component set gathered into a whole), as inputs and outputs remain in balance. If changes do occur moving it to a new position (dynamic equilibrium), then feedbacks (an output causing system changes to the inputs) will allow for correction (Figure 1). Feedbacks can be positive, i.e., exacerbating the size of the systems normal elements through time, or negative that can dampen or reverse the size of the systems elements/attributes. It is assumed that self-regulation occurs. Any beach can be thought of as an open system, i.e., energy/matter relating to the sediment budget can arrive and depart and if these are in balance then equilibrium is assumed to exist [20], e.g., beach sediment arrival equating to sediment removal. When this is broken, e.g., not only by human intervention (damming rivers, coastal structures, etc.), but also by the natural system itself, as occurred before the appearance of man on Earth, it is argued that the system will change in order to bring it back to equilibrium.

If one analyses the above closely, three key points emerge:

• The number of items involved in the sediment budget is so high that is statistically impossible for the balance to close at zero.
• Input and output volumes for each item change continuously and even if equilibrium is reached, it lasts one moment.
• Almost all the processes are not feedback regulated, which is the only chance for natural systems to be stable.

Does this infer that beaches can never be stable?

Among the forms that make up the Earth’s surface, beaches are certainly those subject to the most rapid variations. A storm, even a modest one, is enough for the beach to assume a different morphology. The shape and position of the shoreline varies, as does the slope of the swash zone, the seabed, the position, size, and number of submerged bars. Moreover, from a granulometric viewpoint, the changes are significant and frequent, e.g., a small hole dug on the shoreline allows one to see more or less coarse sand levels that have been deposited in the previous days in different marine weather conditions.

Taking into consideration the whole beach (emerged and submerged part), these variations, both morphological and granulometric, are mainly due to movement of sand to and from the shore, and the overall volume of sediments that make up the beach does not vary as consistently as the changes in its morphology might suggest. For a short time scale, the beach is subject to feedback processes that make it assume the form that best dissipates wave energy, and as this changes moment by moment, even the beach adapts and changes. However, in these short periods some sediment can enter/exit, but does this infer that a beach is stable? Ahnert’s ([21], p.322) comment that, “the huge concentration of energy in littoral processes means that all artificial disturbances of the natural dynamic equilibrium (sic) on the seacoast have very rapid and often unforeseen effects,” is particularly pertinent here.

Beach stability is a concept frequently present in research papers, official documents and the press. Generally, claiming that beaches are not stable relates to concern for coastal sectors experiencing erosion, as accretion, in most cases, is not a problem. For the press and general public out of the three possible conditions for a beach, i.e., accretion, erosion, and stability, the latter is frequently considered as the natural one and the two-remaining are attributed to disturbances governed by anthropogenic actions, although beaches appeared, disappeared, grew and reduced before the appearance of Homo sapiens.

Hourly, daily, and seasonally changes in dry beach width are recognized in every coast, due to tide, air pressure, and waves, but these are considered as “normal” oscillations around longer-term “stable” conditions. Their magnitude order can range from few centimeters in micro-tidal sheltered coasts, e.g., many Mediterranean beaches, to hundreds of meters in macro-tidal exposed environments, e.g., the Glamorgan Heritage Coast, Wales, UK. Many papers/books refer to the sweep zone or envelope: a large sweep zone delineating an unstable beach, a very narrow one a stable (equilibrium) beach.

Bogaert et al. [22] distinguished between behavior and evolution. However, it is not the shoreline position which determinates beach condition but the beach sediment budget, calculated for a selected coastal segment and extending from the first dune (or anthropogenic structure) to the depth of closure [23]. The problem of high-frequency beach morphological changes around a hypothetical equilibrium has frequently been resolved by referring to the concept of dynamic equilibrium [6], but this status cannot be attributed to a beach as it refers to a continuous process which does not change the shape, temperature, or chemical constitution of an object. In chemistry, dynamic equilibrium is when, e.g., substances transition between the reactants and products at equal rates, meaning there is no net change, and can only occur in reversible reactions, i.e., when the rate of the forward reaction is equal to the rate of the reverse reaction.

The equilibrium concept could be applied to pocket beach rotation [24], where grains eroded on one side are deposited on the other one, but also in this case the balance between input and output is questionable. What is under discussion here is the existence of that hypothetical equilibrium around which the beach should fluctuate. The approach to this problem in this paper starts with processes that form the beach sediment budget looks at how they can modify sediment input and output from the beach, considers whether related processes are feedback regulated or not, and, finally, to try to find a functional definition of beach stability (if any).

A stable beach is one that oscillates “slightly” around a mean, as against a high oscillatory component that can spill into an erosion/deposition mode, i.e., an unstable beach has the potential to enter this phase. The time factor must be long enough to dampen out any irregularities due to transient storm activity. Many studies rely on the estimation done via interviews by Bird [25] who found that over 70% of shorelines are retreating because of climate change related processes, and this trend is increasing [26]. If these shorelines are in an eroding mode, then they are by definition unstable [26]. It should be noted that the figure quoted should refer to beaches that have been studied, as all beaches in the world were not investigated. Alternatively, Luijendijk et al. [27], through machine learning and image processing techniques on 1.9 million historical Landsat images, carried out global scale studies concluding that 24% of the beaches are eroding at a rate >0.5 m/year. Applying the same method, 48% of world beaches resulted as stable, but within a range of ±0.5 m/year.

2. Beach Sediment Budget

Each stretch of beach, even if delimited by coastal structures, such as harbors, jetties, groins, and detached breakwaters, is not a closed system where the amount of initial free energy is less easily available as the system moves towards a state with maximum entropy [20], as it might appear from previous descriptions, but it is crossed by a significant flow of entering and exiting material, some entering and some exiting, all contributing to 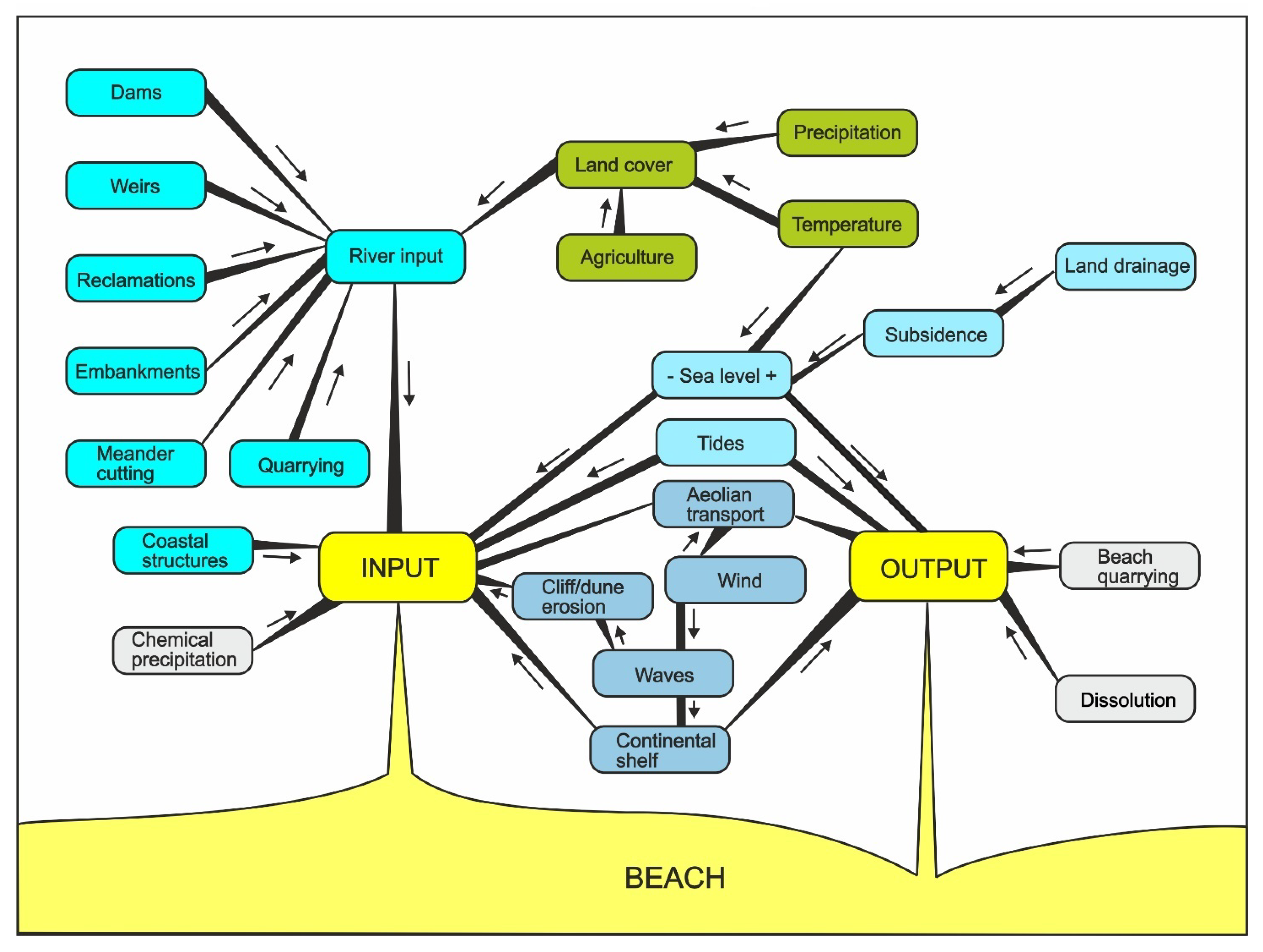a beach sediment budget [28]. It comprises multiple input and output items, each of them directly influenced by several factors, which, in their turn, respond to other forces, and none of these is stable over the time. In any beach the volume of input and output items can be many orders of magnitude different, and continuously changing, to make it statistically impossible for the statement to be equal.

2.1. Riverine Input

Most world beaches owe their origin and survival to the sedimentary contribution from rivers (more than 90% according to Pethick [30] and 75% according to Best and Griggs [31] for the Santa Monica littoral cell). It is precisely due to the variations in river input that many of the shoreline oscillations that occurred in historical times are still in progress today. There are numerous natural and anthropogenic factors which, more or less directly, influence river input; some are easily identifiable, and quantification of their effects is rather simple, others are difficult to study and of uncertain effect.

2.1.1. Changes in Rainfall

Certainly, rainfall regime variations directly influence soil erosion rates [32] and modify transport capacities of rivers, so much so that an increase in rainfall should result in an increase in sedimentary input and a progradation of beaches. This, in general, can be considered true, but there are certainly cases in which the increase in rainfall determines an increase in vegetation cover which, in turn, reduces erodibility of the soils and surface runoff, so much so as to lead to a reduction of river input. A similar effect is most likely in semi-arid areas [33], where soil erosion rates are high despite low rainfall [34].

2.1.2. Variations in Vegetation Cover

Vegetation cover is also influenced by temperature (Figure 1); an increase in this, particularly in Mediterranean areas, can cause a reduction in vegetation with a consequent increase in erodibility. However, this temperature variation should lead to an upward shift of the tree vegetation limit in mountains [35] with a consequent reduction in erosion rates in the upper parts of catchment areas where, due to the greater relief, the production of sediments is greater [36].

Variations of this type certainly occurred in the Holocene and may have modified, even significantly, the sedimentary balance of some coasts. Unfortunately, studies of this nature are extremely scarce due to the objective difficulties of appreciating a phenomenon that is largely overshadowed by a much more relevant one that in turn is linked to variations of the vegetation cover induced by anthropic intervention. Forests cutting to convert land to pasture and agriculture have been recognized to be the main cause of coastal progradation in historical time [37,38,39].

Conversely, agricultural abandonment can lead to forest growth with a reduction of soil erosion [40,41,42], but where this favored collapse of terrace stone walls, as in many parts of the Mediterranean countries, this process increased [43].

2.1.3. Hydraulic Works

Among interventions carried out on rivers, it is not only deforestation that increases fluvial input; modifications of water courses and embankments construction have a similar effect. Cutting meanders, carried out to prevent alluvial plain flooding, imposes a shorter path, and therefore a greater slope, favoring sediment transport to the sea. From 1338 to 1771, the River Arno course from Pisa to the sea was shortened by approximately 5 km (from 12 to 7 km) by cutting three meanders, which almost halved its slope. On the same river, upstream and downstream from the town, embankments were built by the Romans together with reclamation works, in order to transform the marshy area into an agricultural area, as centurion footprints still show [44]. Together with the watershed deforestation this helped the river to build its delta and extend its course for 7.5 km (which reduces the slope). However, most anthropogenic interventions carried out on river channels has led to a reduction in sedimentary input: the construction of “diversions” to introduce the “slurries” into reclamation basins [41] and that of the bridles along river channels [45] have often caused a drastic reduction in the amount of sediment transported to the sea.

After reclamation, rivers can be redirected to the sea, enriching the sediment input, but the same can be done by diverting river course previously emptying into a lagoon in a natural way. This has been carried out with rivers on the western Veneto coast to prevent Venice Lagoon siltation and expanding beaches near the new river mouth [46]. Additionally, weirs can be removed, releasing sediments they trapped [47], but more important, and well studied, is the effect of dams on riverine input to the coast [48].

From large dams, e.g., the Nile River at Aswan [49] and that of the Seven Gorges on the Yangtze River [50], to smaller ones, this impact has been well documented, e.g., rivers emptying into the Catania Gulf, Italy [51]. More than 100 × 10⁹ tons of sediment has been sequestered in reservoirs constructed largely within the past 50 years [52]. Against this, several dam removals have been performed during the last few decades and channel evolution documented [53,54], but none had the tremendous impact on the coast as that of the Elwha River [55]. Here, a two years post-removal monitoring showed that 2.2 million m³ of sand and gravel were deposited on the seafloor offshore at the river mouth [56]. However, each intervention does not have an instantaneous impact on the coast and its effect increases and reduces over time, never maintaining the same effect.

2.1.4. River Bed Quarrying

One of the most effective causes of reduction in sediment river input to the coast is riverbed extraction of aggregates [57]. This activity was conducted on an occasional basis and with modest means up to the last century and has become a real “industry” in recent decades, driven by the demand for construction materials for building and communication routes [58]. From river beds, each year materials have been removed with volumes far greater than the bed load of the rivers themselves, so much so that their level has been lowered by several meters, which has triggered serious problems of instability in banks and bridges. For example, ten times for the Po River [59], Italy; forty times according to for the Vembanad Lake catchments, India [60]. This operation was also favored by the fight against floods, as widening and deepening of river beds leads to an increase in the hydraulic variables. The fallout area of these interventions, the coastal strip, is too far, physically and politically, from populations affected by the floods and the fight against extractions has not yet been won everywhere. Damage caused by this activity is such that, even where mining has been banned for more than twenty years (e.g., Cecina River, Tuscany), the morphology of the rivers has not yet assumed the natural configuration and deep holes are still evident in their longitudinal and transverse profiles; actually, approximately 4 × 10⁶ m³ of aggregates was extracted on the 40 km terminal river course from the 1960s to early 1970s [61].

2.2. Coastal Processes

2.2.1. Beach and Dune Mining

Aggregates from beaches and dunes were traditionally used for construction in all coastal settlements, until its negative effect on coastal evolution was perceived; nevertheless, this activity is still, legally or illegally, carried out in many countries. Portobello beach, the 19th century fashionable watering place near Edinburgh, UK, had been the quartz rich sand quarry for glassware manufacturing from 1834 to mid-1930s, until all the white sand was lost, the promenade collapsed, and tourists disappeared [62]. At Poetto, the urban beach of Cagliari, Italy, approximately 2 million cubic meters of sediments were quarried on the beach, mostly for reconstruction of the town after WWII bombing, and this had been the main cause of the beach erosion [63]. In China, during the 1980s, 4000 million tons of beach sands was removed annually [57]. Dune mining along the 18 km of Monterey Bay from 1940 to 1984 was 128,000 m³/year [64], i.e., approximately 7 m³/m/year; when mines were closed the erosion rate decreased, but not significantly.

2.2.2. Relative Sea Level Change

Coastal plains have always been subject to subsidence, as sediments recently deposited are subject to compaction. This process is faster on river deltas, where deposition rates are higher, but can reach massive values where oil, gas, and water extraction is carried out. The high coastal erosion rate found at Ravenna, Italy, is mostly induced by oil and gas extraction both inland and offshore that produced a 40 mm/year subsidence in 1970–1977, the period of most intensive activity [65]. Satellite interferometry measured a value of 30 mm/year at the Gudao Oilfield, on the Yellow River Delta [66], and in the Piombino alluvial plain, natural subsidence rate shifted from 1 mm/year in the Holocene to the present 10 mm/year, as a consequence of water pumping for agriculture and a steel mill [67].

Sea level rise, as a cause of sediment loss, has its rationale in the Bruun Rule [68] which was firstly enthusiastically accepted, and later strongly rejected; it passed through several modifications, see, e.g., in [69,70,71,72], and is related to those sediments deposited near the depth of closure that subsequently exit the active beach following the “apparent deepening” caused by the rise in sea level. Although suggestions have been made that it is time to abandon the Bruun Rule [73], no operative alternatives have been proposed, but it is evident that if water depth increases, sediments moved to the limit of the active beach enter the “inactive” part of the profile. However, sea level rise can also induce sediment shifting to the coast, as occurred during the last 18,000 years, when barrier islands emerged and moved ashore [74,75], somehow contradicting the Bruun Rule. This input had been significant during the Holocene Sea Level Rise, but there have been no studies to investigate if it is still operating.

2.2.3. Aeolian Transport

Wind, with its direct effect on sediment erosion/transport/deposition, influences the “fine sediment” budget although wind drifted gravel has been documented by Zenkovich [76]. Aeolian processes are responsible both for input and output in the beach budget, although the former only has been generally considered, except for alongshore transport [77]. Offshore transport is evident from satellite images, but almost exclusively concerns silt-sized sediments coming from inland areas and not the beach itself. Inland transport has been documented in Neolithic times (burial of Skara Brea, Orkney [78]); historical times, e.g., abandonment of the Roman Via Julia, Wales after the year 1344 [79] and burial of the church at Skagen [80]; and in the present time, examples can be found in Spain [81], South Africa [82], and Australia [83].

2.2.4. Wave Energy

Waves influence the beach sediment budget and are the predominant process: waves and associated storm surges, move sediment longshore, offshore and onshore; the latter direction seldom produces sediment deficit unless sand is deposited in urbanized areas and not returned to the beach, e.g., if polluted by traffic [84]. Cross-shore [85] and long-shore [86] variability in sediment transport rate is well-known and documented. Net sediment long-shore transport ranges from few to millions cubic meters per year among the different coastal sectors. But the same magnitude order differences can be found locally at different times. Episodicity in long-shore sediment transport was studied by, e.g., Seymour et al. [87] who found that in one day sediment transport can be more than 600 times the mean daily net transport.

Shi-Leng and LiuTeh-Fu [88], studying a sector of the Mauritania coast, found that long-term variation in sediment transport follow a Gumbel distribution, but it is not necessary to wait for extreme events to have huge volumes of sediments moved along the coast: the net long-shore transport near Oregon Inlet (Outer Banks, NC, USA) is between one-half million and one million m³/year [89]. Along the Mediterranean coast, a “protected sea environment” according to the Davies classification [90], a net sediment transport of more than 400,000 m³ was calculated for a point near the harbor of Ashkelon, Israel [91].

With respect to long-shore transport, Raynor [92] introduced the term “cascade of uncertainties” involving the triple interaction of air, sea, and sediment into geomorphological literature. This is very pertinent when echoed in the concept of beach equilibrium. Pilkey and Cooper ([93], p.579) were of the opinion that “Sand volumes perhaps should be expressed as broad categories such as small, intermediate or large in recognition of the fact that meaningful determination of net annual transport of sand is probably impossible in this complex, dynamic and changing natural system.” A case study for the Northwest coast of Portugal [94], showed that the annual littoral long-shore transport values exhibit a large variability with a maximum of 2.24 million m³ year⁻¹, which exceeds the long-term mean magnitude by 105%, and a minimum, 108,000 m³ year⁻¹, 10 times less than the mean value. Taborda et al. ([94], p.466) pointed out that, “The long-shore transport estimates reported in the literature for this coastal stretch were made through different techniques from cartographic comparison with mathematical modeling, whose results led to a wide range of values from 200,000 (Abecasis 1955) to 3.5 million m³ year⁻¹ (Teixeira 1994), although most values converge toward a mean value of around 1 million m³ year⁻¹.”

Storms are known to have strongly modified the coast in specific periods of the Middle ages, e.g., the 1634 storm surge divided the German island of Strand in two parts, now known as Pellworm and Nordstrand islands [95]. In South Wales, UK, extreme storm events have been recorded in monastic records since the 3rd century culminating in the 14th and 15th centuries that saw much coastal erosion along with washovers. The storm events peaked in the 16th century, when they became especially ferocious causing much flooding and massive washover sand amounts formed the coastal dune fringe of South Wales [96,97]. Extreme events have been studied over a 6000-year-long period in Australia [98] surveying and dating beach ridges, showing the importance of the association of wave height and storm surge to build up those morphologies.

Climate change occurring during the last decades has modified storm frequency and intensity, e.g., along the Atlantic coastlines of Europe [99] and future scenarios forecast a further increase in storminess. This is an additional variable to the future beach sediment budget. Changes in wave directional distribution will modify long-shore transport direction and possibly invert its resultant direction, shifting convergence points, or completely modifying coastal cell geometry.

2.2.5. Biogenic Production and Chemical Precipitation, e.g., Inside Posidonia Prairies, Mangroves

Although most coastal sediments come from inland erosion, in some areas the beach comprises a consistent percentage of shell fragment and skeletons of marine animals. In Western Australia, these components are in the majority [100], but the same can be found in mid Latitude coasts, such as, Sardinia, where on the northern granite coast, the beach of Pelosa has more carbonate sand than quartz [101], or even in Scotland, where Coral Beach, Hebrides, has an abundance of calcareous seaweed [102]. Their production is influenced by water temperature, nutrients richness, and turbidity, all of which are extremely variable. Since historical times, this material was quarried to amend acid soils, e.g., in Scotland [103].

2.2.6. Hard Rock Coasts

Contrary to what one is led to believe, erosion of high coasts generally provides a very low sedimentary input (less than 5% according to Inman [104]), and those models of coastal evolution, which see the retreat of promontories and the filling in of the gulfs to the complete rectification of the coast, appear to be linked to a Davisian concept of coastal geomorphology by now largely outdated. The Cycle of Normal Erosion by Davies held sway for many years and hindered acceptance of the Gilbertian viewpoint that came to the fore again in the evolution of quantitative geography in the 1950s.

Nevertheless, rock coast erosion can be locally significant and constitute the main sediment source not only for pocket beaches, but also for significant segments of open coasts. Erosion is very dependent upon lithological setting and can be very high in favorable conditions, e.g., soft rocks strata. On some sectors of the Algarve coast, a 10 to 50 m high soft rock cliff is retreating for 1–2 m/year [105], but the highest cliff retreat rate was measured on pyroclastic deposits on Nishinoshima Island (Japan), with 80 m/year [106]. In East Anglia, UK, at Dunwich, coastal retreat has been 2 km since the Roman time, with 13 parishes disappeared after XII century, the last one in 1919 [107]; this sediment input feeds the coast down to Orford Ness, more than 30 km south. Obviously, sediment input from a rocky coast is extremely discontinuous [108] and beaches taking advantage of this follow an uneven evolution [109]. As protection of Fairlight Cove, Sussex, UK was being undertaken, French [103,110] noted that “cliff stabilization represents a net loss of sediments to the coastal budget”, as an input of 9750 m³/year was interrupted.

2.2.7. Coastal Structures

Sediment offshore dispersion is favored by coastal structures, both in harbor and with shore protection projects. These, not only modify long-shore sediment distribution, favoring or penalizing different sectors, but through wave reflection, especially with shore parallel structures: [111,112] can induce topographically controlled rip currents through the structures’ gaps [113]. Structures orthogonal or oblique to the coast [114,115] favor the shift of sediments to deeper areas, where they can be lost or from where they hardly return to the near-shore further downdrift [116]. This is well known and considered by researchers, but here we want to highlight the variability of their effect.

In front of shore parallel structures, beach profiles gradually lower [117,118], so wave energy dissipation reduces, and the structure becomes hit with increasing intensity, under a positive feedback-regulated process. However, when depth is so large that no shoaling processes are present and waves are fully reflected, no impact on sediment transport is to be expected; but this terminal condition is hard to be achieved. If this happens, a contributing cause is a regional negative sediment budget, e.g., at Marina di Pisa, Italy, where breakwaters located at an initial depth of 2.5 m, now have a depth of up to 7.0 m at the seaward side [112].

With respect to groins, trapping capacity reduces with time, until sediments bypass the obstacle; at this point, offshore dispersion is increased, although some material can overpass the structure (if it does not extend over the depth of closure). Therefore, updrift accumulation, offshore dispersion and input to the downdrift sectors change over time [119,120].

3. Conclusions

Most of the above examples dealt with in this paper are not linked by feedback processes, e.g., an eroding beach cannot send a message upstream to ask the river for more sediment. Without feedback any equilibrium is impossible not only in nature, but also in, for example, economics or human sciences. The beach is subject to feedback-regulated processes [121], that act within the beach and do not operate linking it with the external environment (atmosphere, catchment, etc.). Beach slope changes under the attack of different waves (to better dissipate wave energy), bars formed at the breaker line induce more waves to break there (a positive feedback) but waves can demolish the bar if it is too elevated (negative feedback) giving it a well-defined height.

Even without considering variations in each of these input and output items, each of which involves from few to millions of cubic meters of sediments a year, it is statistically impossible that their algebraic sum is zero, or even not far from zero. When a beach is classified to be in equilibrium, it almost certainly means that data could not reveal changes that were of the same order of magnitude; and one may question the data accuracy.

For the Tuscany coast a stable classification refers to sectors where the mean shoreline displacement of two surveyed shorelines was between +/−5 m. As far as the real sedimentary budget is considered, i.e., the sand volume change, the surveys accuracy is generally unsuitable to monitor actual values. Echo sounding accuracy is between 5 and 10 cm, and in the worst conditions depth changes of +/−20 cm are not significant [122]. If the depth of closure is 1 km offshore, 200 m³ per meter of coast is the expected error, which equals a medium artificial nourishment project.

Assessing the possibility for a beach to be in equilibrium is contentious and socially dangerous. To give stakeholders this idea makes them ask for this stability and turns away the possibility of allowing the beach to achieve its due resilience. In many papers, “stability” has been used, but clearly exhibiting such a beach evolution trend refers to cases where observed changes are within any measurement error.

Many coastal geomorphic features are relatively stable and form changes take place slowly; others are less stable and have comparatively rapid change in response to dynamic environmental factors [123]. Quickly changing trends are a major problem for the present, and for the temporal dimension, which we usually use to build the knowledge, explanations, and predictions [44,124].

Unconsolidated beach materials respond rapidly to changes in the dynamic environment. Comments made in many books and documents of the last century, were to the effect that if a beach for an adequate period has been subject to environmental forces, then the profile will respond to both long-term and short-term changes, which tends to restore an equilibrium profile. The view was that equilibrium—the amount of sediment deposited by waves and currents—will be balanced by the amount removed, with many researchers even producing equations for the slope of natural profiles [125]. Cooper and Pilkey ([126], p. 605) concluded an overview of long-shore transport modeling, assessing, “that our present understanding permits only a qualitative estimate of direction of long-shore drift and identification of some of the controls”; but are the controls reliable?

So what is beach stability and how is it (if at all) entwined with dynamic equilibrium, or has the term dynamic been corrupted and used as a useful blanket adjective? As has been shown, it is virtually impossible for subsequent beach profiling to cover the exact same profile, so the stability (equilibrium) concept must be questioned. A beach is certainly a dynamic entity as it constantly changes shape as sediment is moved around. Is dynamic equilibrium simply an idea for a beach striving to assume an ideal shape? Is stability neither erosion nor deposition? Is an unstable beach one undergoing strong erosion only—if so what about strong deposition? Subjectivity of input selection, survey accuracy and also the potential for geological, dynamic, and sedimentological constraints reflect the uncertainty in the quest for the elusive conundrum of equilibrium. Is it akin to Lewis Carroll’s [127] snark, is it a Boojum (a particularly nasty type of snark, which if glimpsed by a person, he/she disappears, so perhaps the term might disappear from the literature), or an allegory of an attempt to discover an absolute measure that is doomed to failure? The concept is so theoretical as to be functionally irrelevant.

To end, we believe that the word “stability” should not be used for beaches, unless it is accompanied by a value of the accuracy with which this condition is assessed and the time period to which it refers.