Sinkhole Stability in Elliptical Cavity under Collapse and Blowout Conditions
Abstract
:1. Introduction
2. Problem Definition and FELA Model
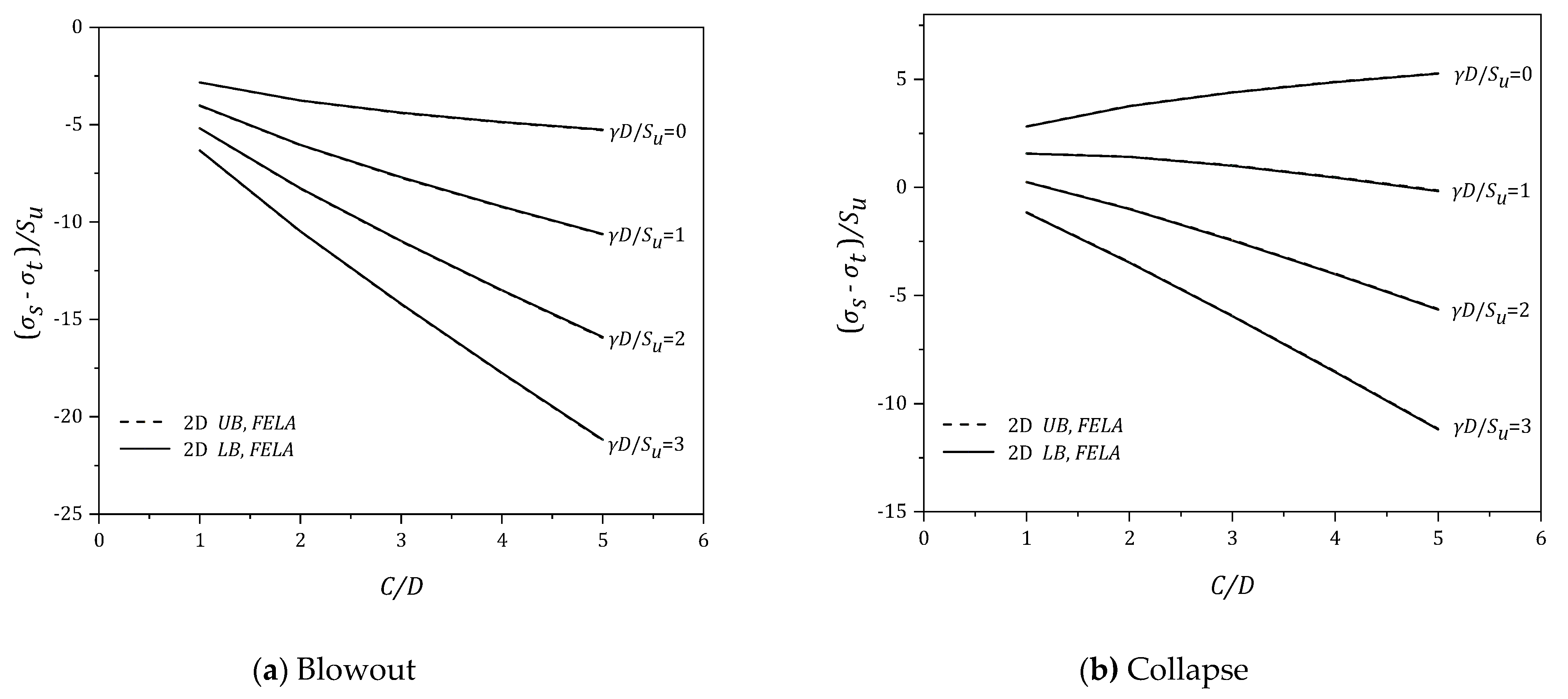
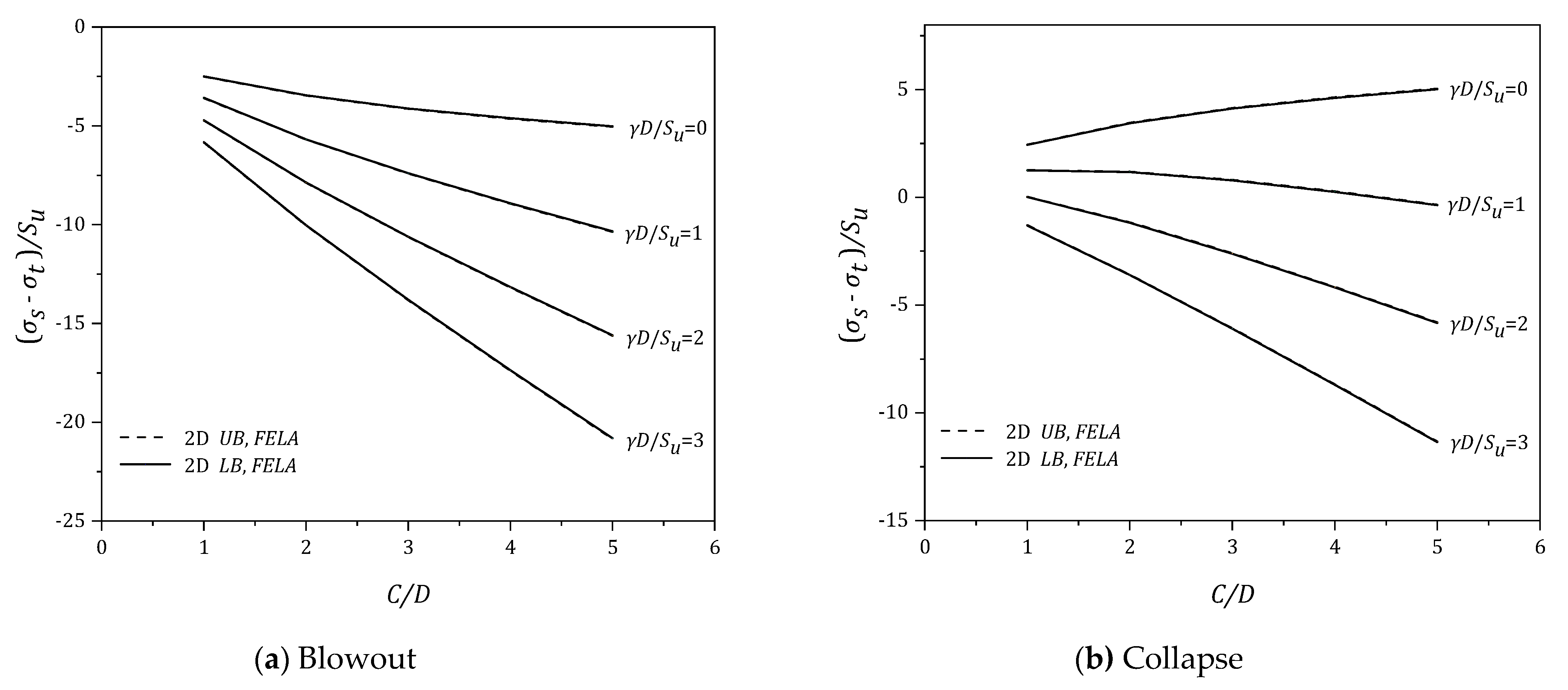
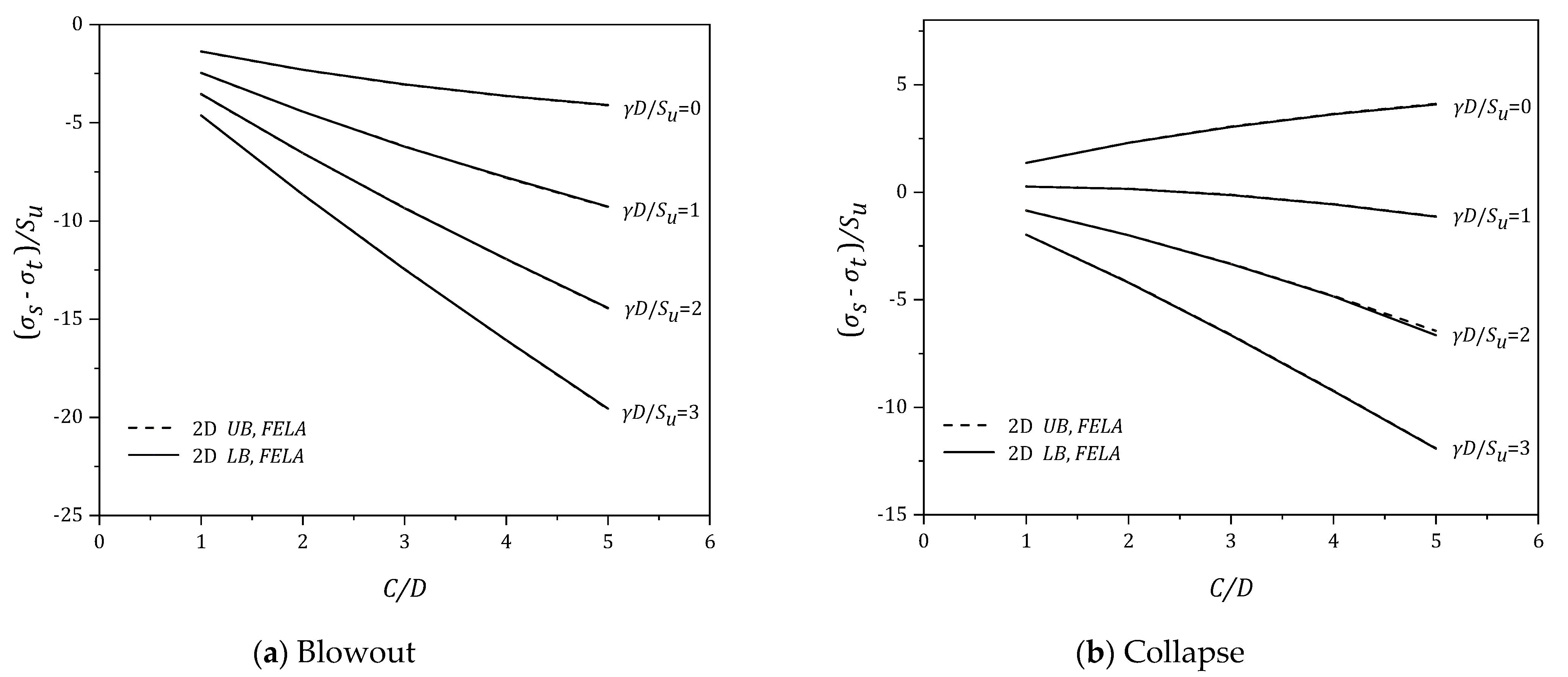
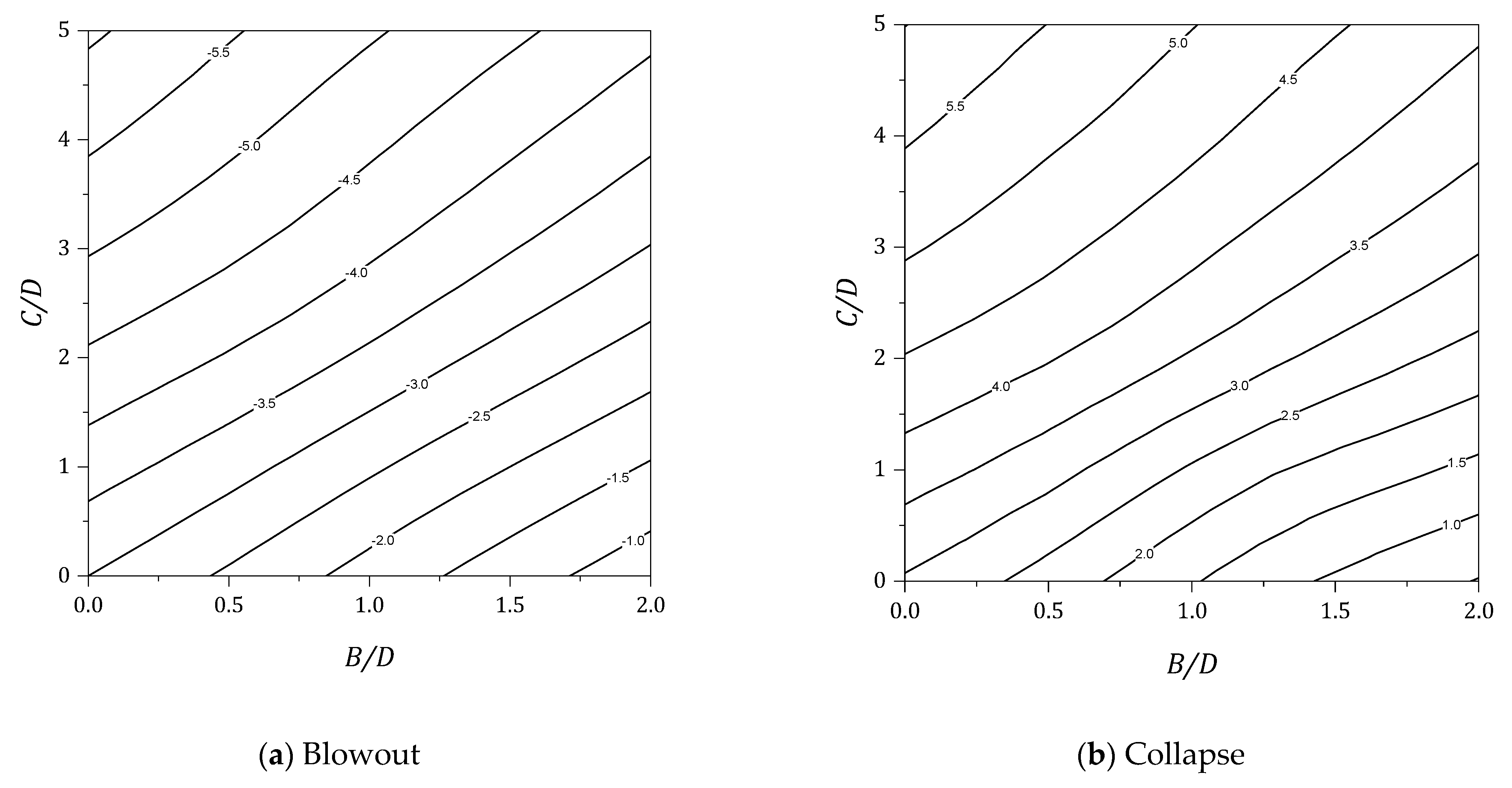
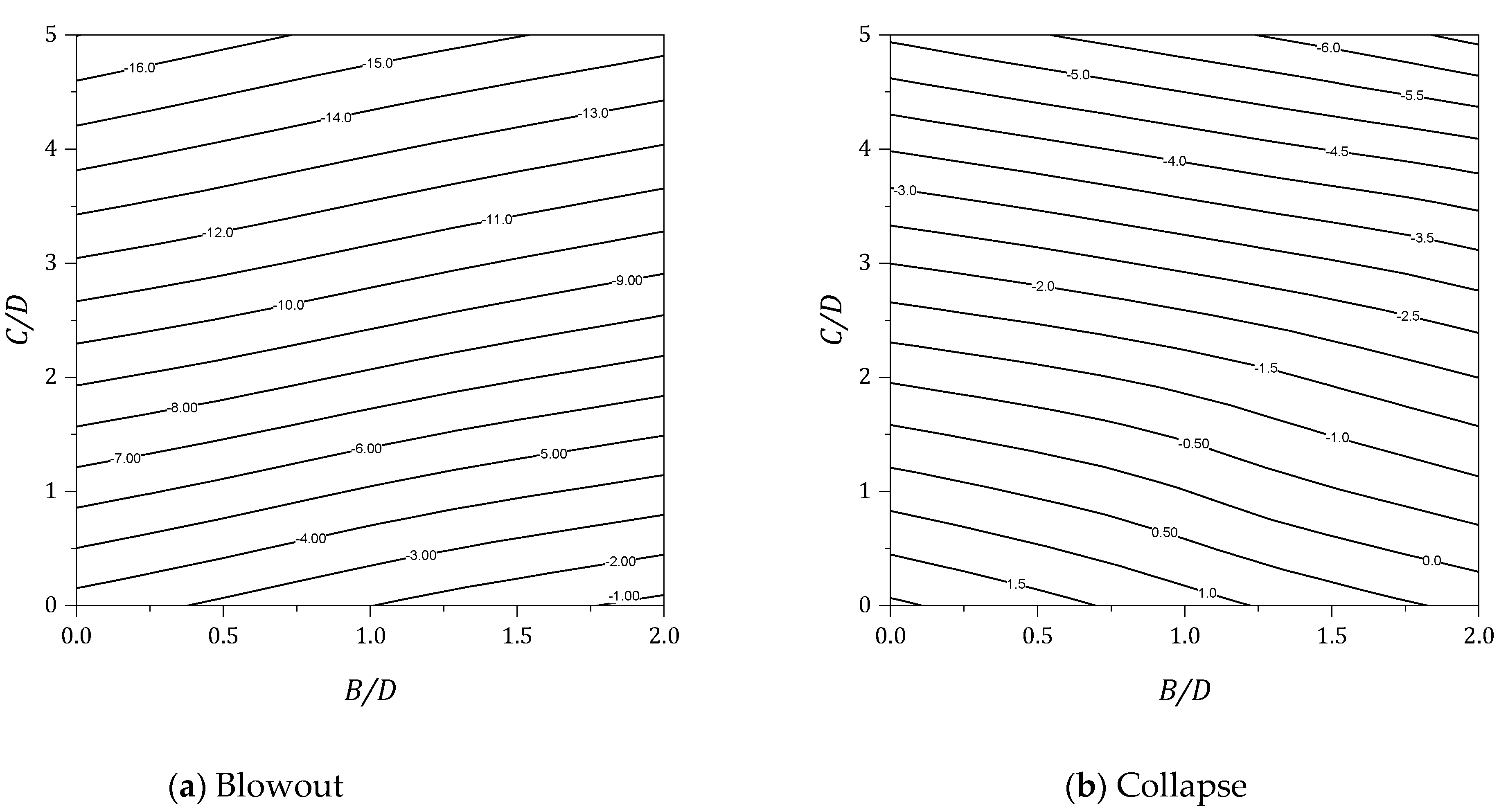
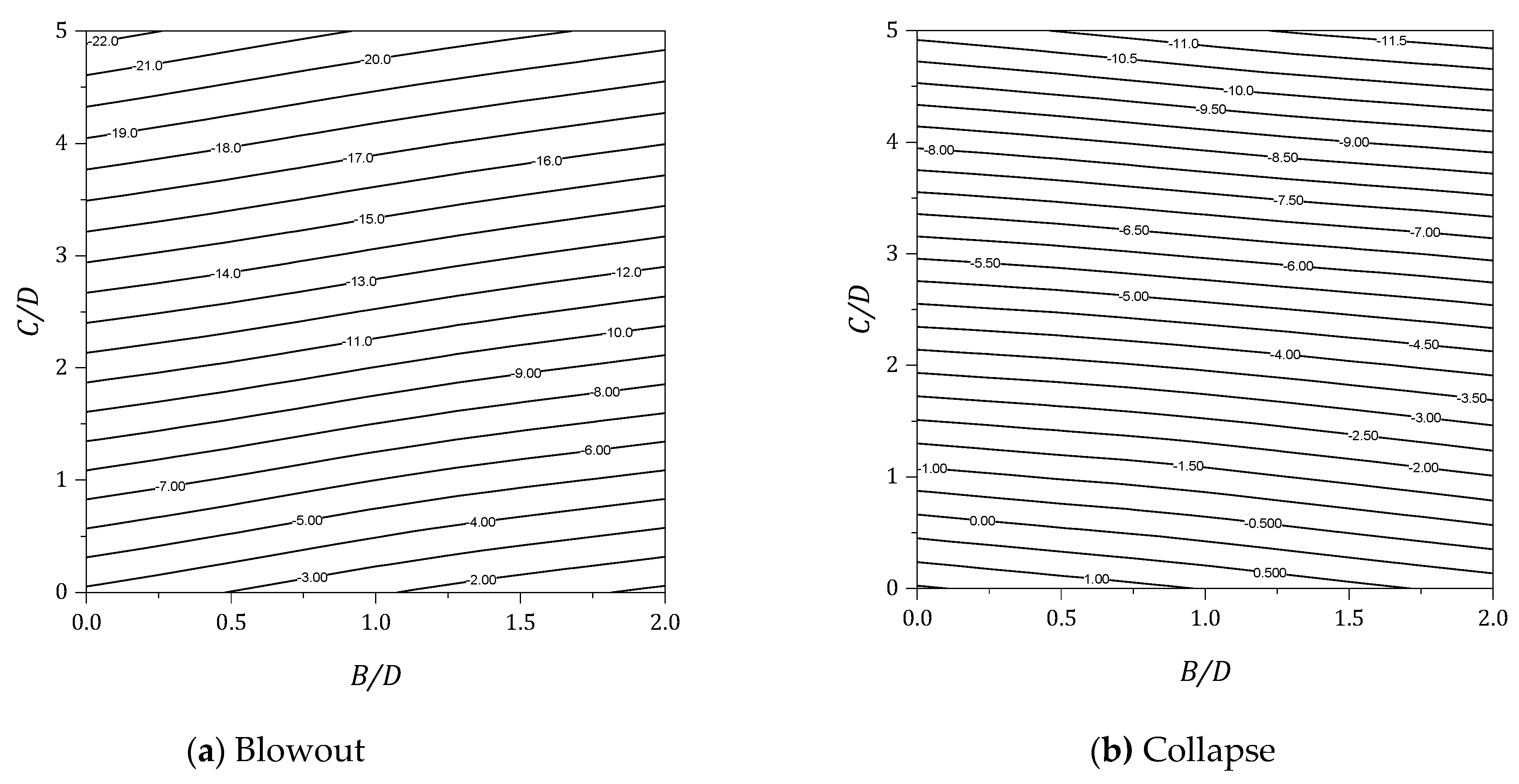
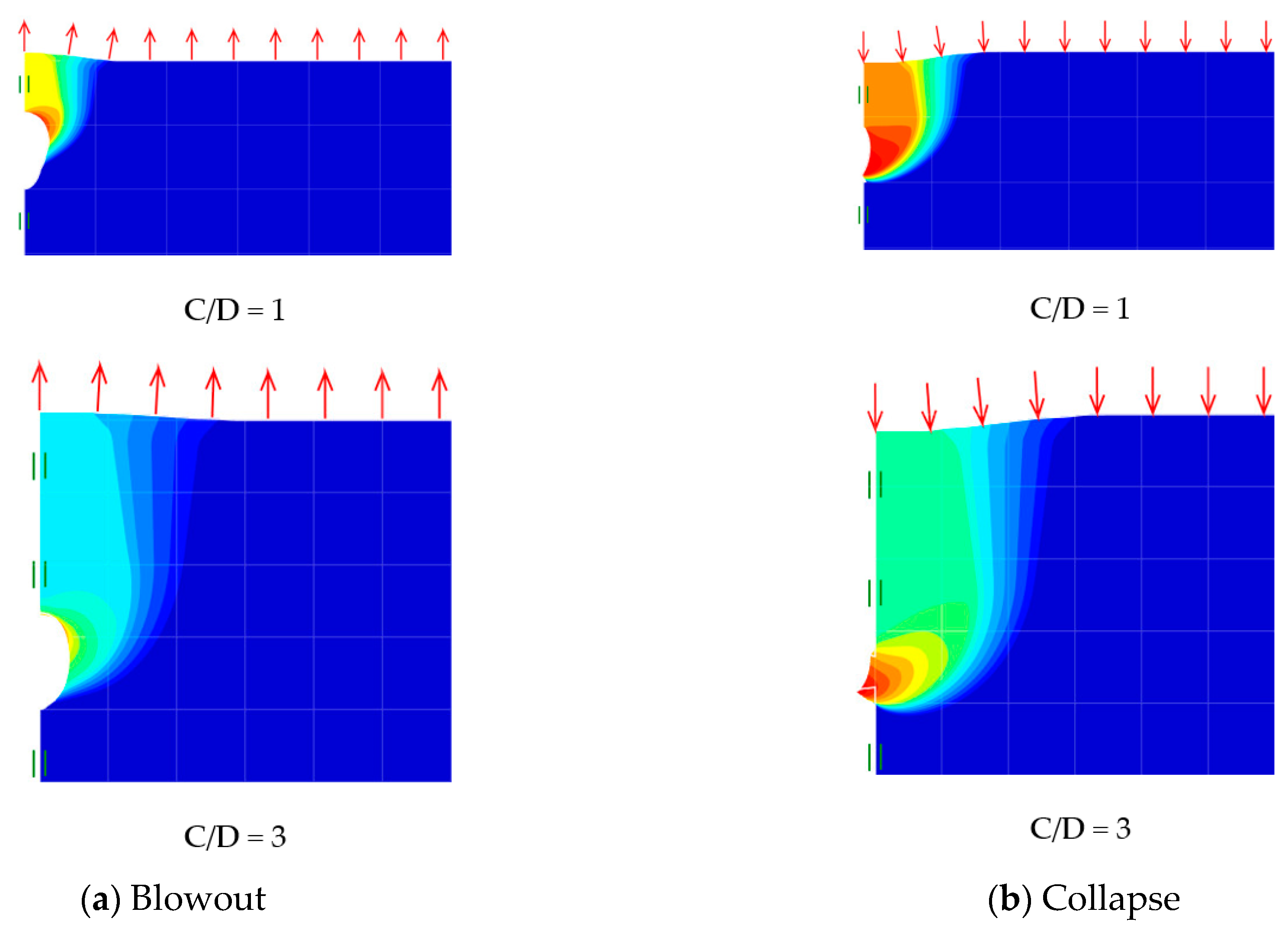
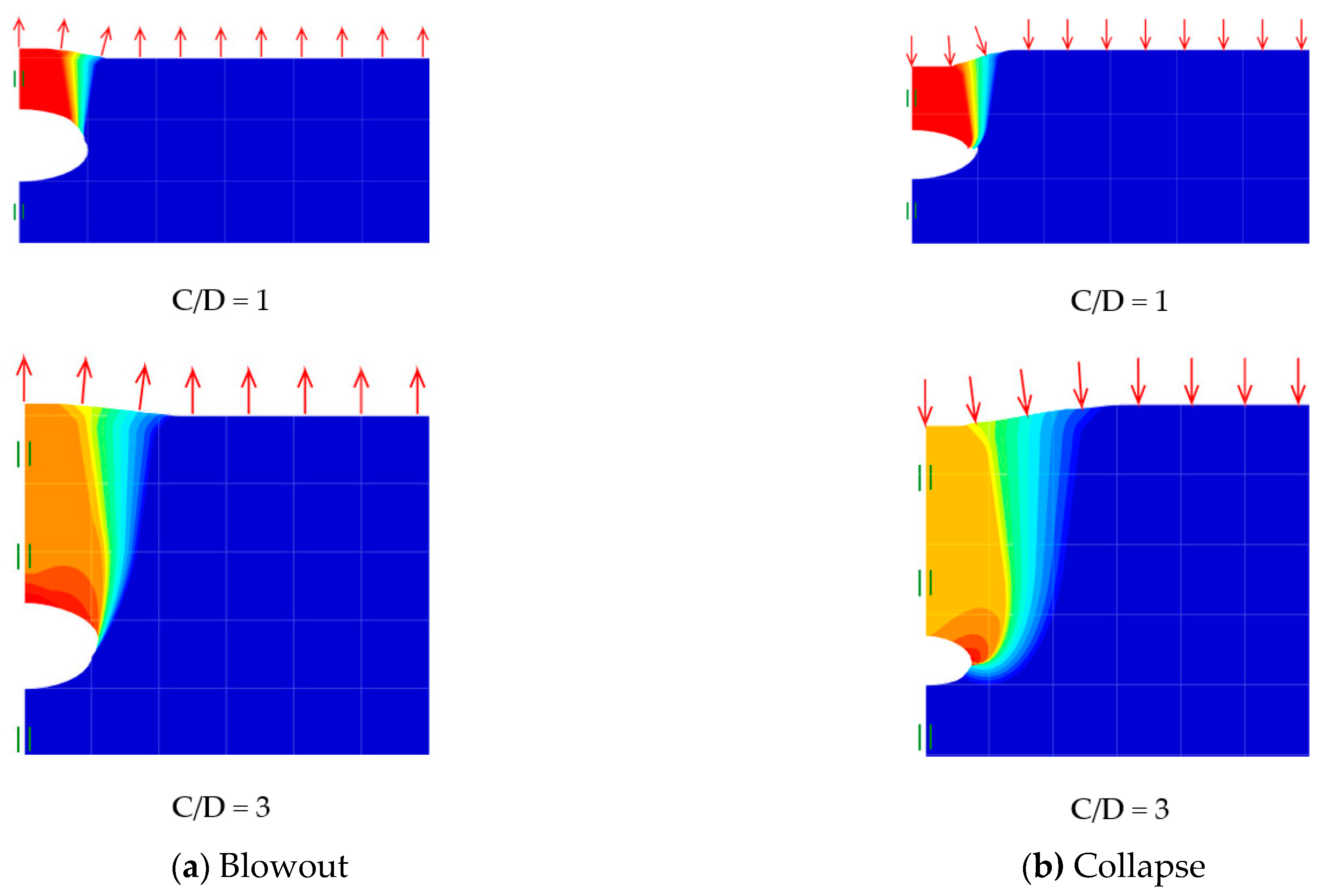
3. Results and Discussion
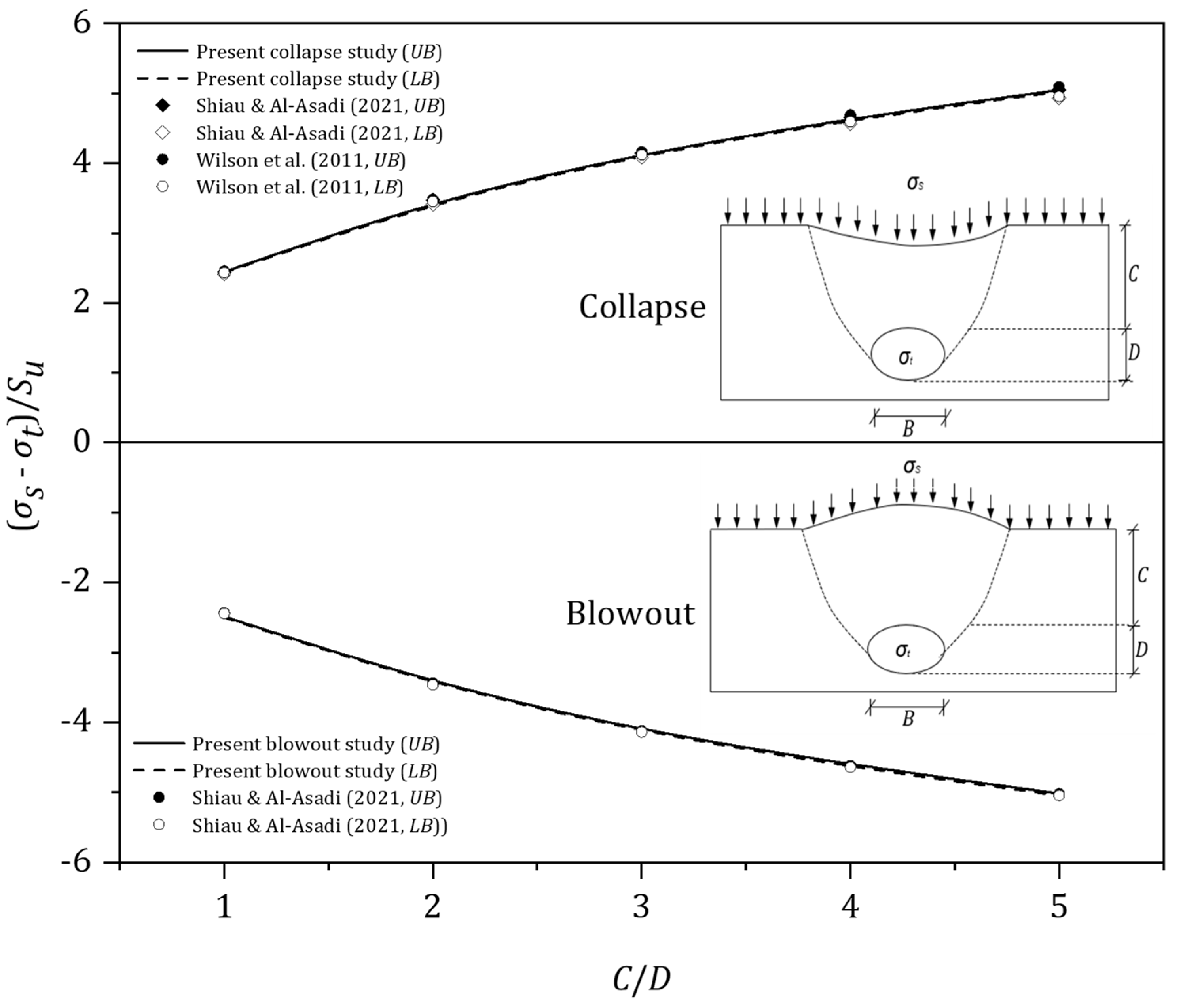
4. Comparison and Examples
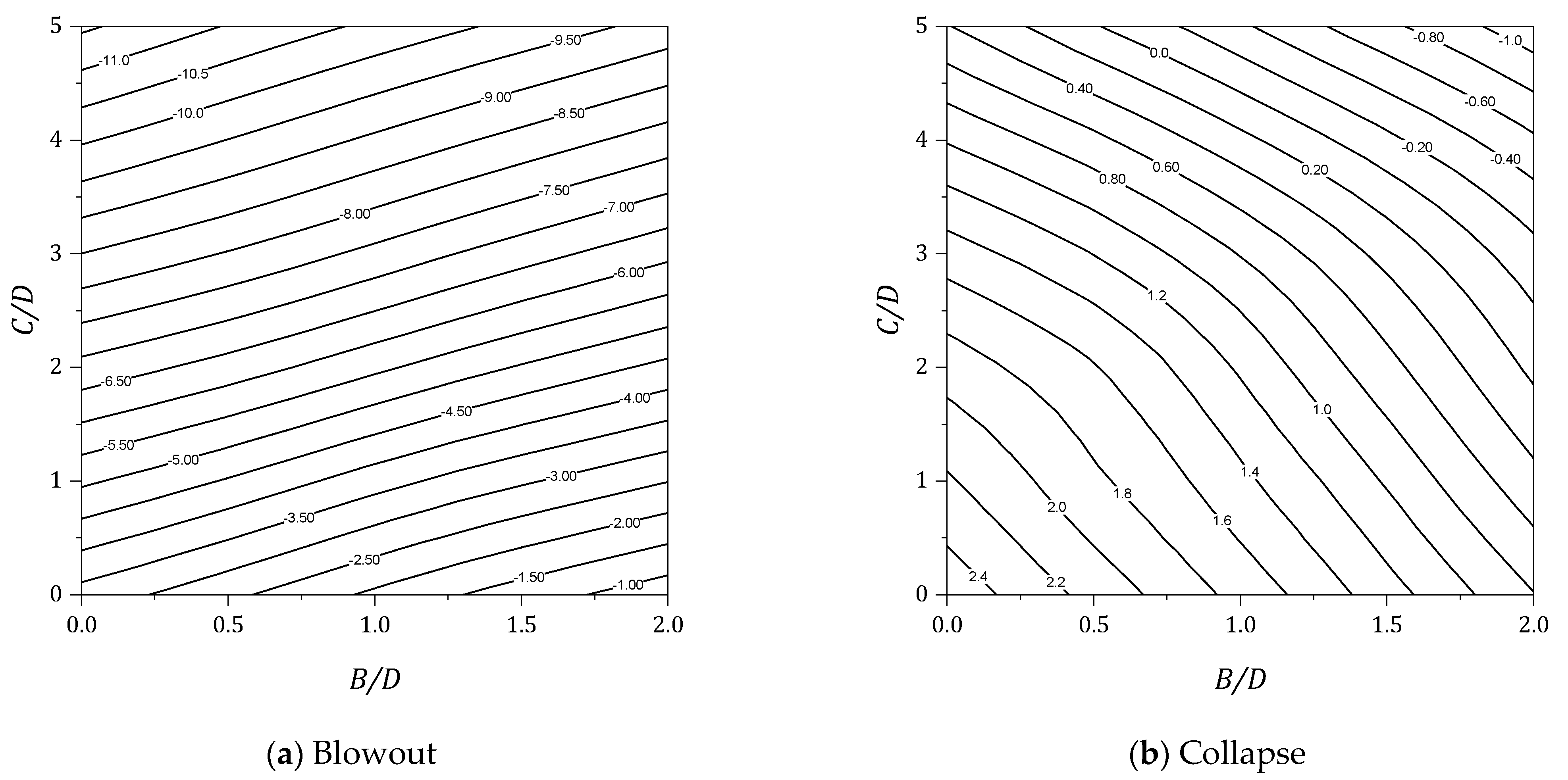
- Both the cover depth ratio and the width depth ratio are C/D = 3 and B/D =0.5, respectively.
- The strength ratio: SR = γD/Su = (20 × 2/40) = 1.
- Using Figure 14a, for C/D = 3 and B/D = 0.5, the critical pressure ratio is calculated as PR = (σs – σt)/Su = −7.9.
- Since σs = 100 and Su = 40 kPa, σt is calculated as 416 kPa. Theoretically, the support pressure should not be greater than 416 kPa, or a ground blow out failure occurs.
- Both the cover depth ratio and the width depth ratio are C/D = 3 and B/D = 0.5, respectively.
- The strength ratio: SR = γD/Su = (20 × 2/40) = 1.
- Using Figure 14b, for C/D = 3 and B/D = 0.5, the critical pressure ratio is calculated as PR = (σs – σt)/Su = 1.2.
- Since σs = 100 and Su = 40 kPa, σt is calculated as 52 kPa. Theoretically, the cavity requires a support pressure of 52 kPa, or a ground collapse failure occurs.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ali, H.; Choi, J. A review of underground pipeline leakage and sinkhole monitoring methods based on wireless sensor networking. Sustainability 2019, 11, 4007. [Google Scholar] [CrossRef] [Green Version]
- Waltham, T.; Bell, F.; Culshaw, M. Sinkholes and Subsidence: Karst and Cavernous Rocks in Engineering and Construction; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Gutierrez, F.; Parise, M.; De Waele, J.; Jourde, H. A review on natural and human-induced geohazards and impacts in karst. Earth Sci. Rev. 2014, 138, 61–88. [Google Scholar] [CrossRef]
- Parise, M. Sinkholes. In Encyclopedia of Caves, 3rd ed.; White, W.B., Culver, D.C., Pipan, T., Eds.; Academic Press: Cambridge, MA, USA; Elsevier: Amsterdam, The Netherlands, 2019; pp. 934–942. [Google Scholar]
- Service Restored after Water Main Breaks in Front of Perry Hall Home-Opera News. 2021. Available online: https://www.dailyadvent.com/gb/news/4721acb8259e28c6cdd1ef23b70e1f6c-Service-Restored-After-Water-Main-Breaks-In-Front-Of-Perry-Hall-Home (accessed on 1 July 2021).
- News Break. Storm Sewer Collapse Leads to Sinkhole in Maryville. 2021. Available online: https://www.newsbreak.com/news/2198607773329/storm-sewer-collapse-leads-to-sinkhole-in-maryville (accessed on 1 July 2021).
- NewsComAu. Pipe Bursts in Sydney Street before Sinkhole Opens Up. 2021. Available online: https://www.news.com.au/national/nsw-act/news/sinkhole-opens-up-on-newport-nsw-road-after-water-pipe-bursts/news-story/1068cc414cd5da051d5453a88ecf4ef5 (accessed on 1 May 2021).
- WionNews (WION). Italy: Giant Sinkhole in Rome Swallows Two Parked Cars. 2021. Available online: https://www.wionews.com/world/italy-giant-sinkhole-in-rome-swallows-two-parked-cars-387462 (accessed on 2 July 2021).
- Indiketiya, S.; Jegatheesan, P.; Rajeev, P.; Kuwano, R. The influence of pipe embedment material on sinkhole formation due to erosion around defective sewers. Transp. Geotech. 2019, 19, 110–125. [Google Scholar] [CrossRef]
- Sloan, S.W.; Assadi, A.; Purushothaman, N. Undrained stability of a trapdoor. Géotechnique 1990, 40, 45–62. [Google Scholar] [CrossRef]
- Martin, C.M. Undrained collapse of a shallow plane-strain trapdoor. Géotechnique 2009, 59, 855–863. [Google Scholar] [CrossRef]
- Shiau, J.; Lamb, B.; Sams, M. The use of sinkhole models in advanced geotechnical engineering teaching. Int. J. GEOMATE 2016, 10, 1718–1724. [Google Scholar] [CrossRef]
- Wang, L.; Leshchinsky, B.; Evans, T.M.; Xie, Y. Active and passive arching stress in C’-Φ’ soils: A sensitivity study using computational limit analysis. Comput. Geotech. 2017, 84, 47–55. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Undrained stability of an active planar trapdoor in non-homogeneous clays with a linear increase of strength with depth. Comput. Geotech. 2017, 81, 284–293. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Undrained stability of plane strain active trapdoors in anisotropic and non-homogeneous clays. Tunn. Undergr. Space Technol. 2021, 107, 103628. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Likitlersuang, S. Undrained stability of active trapdoors in two-layered clays. Undergr. Space 2021, 6, 446–454. [Google Scholar] [CrossRef]
- Shiau, J.; Al-Asadi, F. Three-Dimensional Analysis of Circular Tunnel Headings Using Broms and Bennermark’s Original Stability Number. Int. J. Geomech. 2020, 20, 06020015. [Google Scholar] [CrossRef]
- Shiau, J.; Hassan, M.M. Undrained stability of active and passive trapdoors. Geotech. Res. 2020, 7, 40–48. [Google Scholar] [CrossRef] [Green Version]
- Shiau, J.; Chudal, B.; Mahalingasivam, K.; Keawsawasvong, S. Pipeline burst-related ground stability in blowout condition. Transp. Geotech. 2021, 29, 100587. [Google Scholar] [CrossRef]
- Fazio, N.L.; Perrotti, M.; Lollino, P.; Parise, M.; Vattano, M.; Madonia, G.; Di Maggio, C. A three-dimensional back analysis of the collapse of an underground cavity in soft rocks. Eng. Geol. 2017, 238, 301–311. [Google Scholar] [CrossRef]
- Fiore, A.; Fazio, N.L.; Lollino, P.; Luisi, M.; Miccoli, N.M.; Pagliarulo, R.; Perrotti, M.; Pisano, L.; Spalluto, L.; Vennari, C.; et al. Evaluating the susceptibility to anthropogenic sinkholes in Apulian calcarenites, southern Italy. In Advances in Karst Research: Theory, Fieldwork and Applications; Parise, M., Gabrovsek, F., Kaufmann, G., Ravbar, N., Eds.; Special Publications; Geological Society: London, UK, 2018; Volume 466, pp. 381–396. [Google Scholar]
- Perrotti, M.; Lollino, P.; Fazio, N.L.; Pisano, L.; Vessia, G.; Parise, M.; Fiore, A.; Luisi, M. Finite Element–based stability charts for underground cavities in soft calcarenites. Int. J. Geomech. 2018, 18, 04018071. [Google Scholar] [CrossRef]
- Lollino, P.; Perrotti, M.; Fazio, N.L.; Parise, M. Sinkhole Susceptibility Assessment of Underground Caves in Soft Rocks by Means of FEM-Based Charts; American Rock Mechanics Association Symposium: New York, NY, USA, 2019; 19-A-2026-ARMA. [Google Scholar]
- Dutta, P.; Bhattacharya, P. Determination of internal pressure for the stability of dual elliptical tunnels in soft clay. Geomech. Geoengin. 2021, 16, 67–79. [Google Scholar] [CrossRef]
- Yang, F.; Sun, X.; Zou, J.; Zheng, X. Analysis of an elliptical tunnel affected by surcharge loading. Proc. Inst. Civ. Eng.-Geotech. Eng. 2019, 172, 312–319. [Google Scholar] [CrossRef]
- Zhang, J.; Yang, J.; Yang, F.; Zhang, X.; Zheng, X. Upper-bound solution for stability number of an elliptical tunnel in cohesionless soils. Int. J. Geomech. 2017, 17, 06016011. [Google Scholar] [CrossRef]
- Yang, F.; Zhang, J.; Yang, J.; Zhao, L.; Zheng, X. Stability analysis of unlined elliptical tunnel using finite element upper-bound method with rigid translatory moving elements. Tunn. Undergr. Space Technol. 2015, 50, 13–22. [Google Scholar] [CrossRef]
- Yang, F.; Sun, X.; Zheng, X.; Yang, J. Stability analysis of a deep buried elliptical tunnel in cohesive–frictional (c–ϕ) soils with a nonassociated flow rule. Can. Geotech. J. 2017, 54, 736–741. [Google Scholar] [CrossRef]
- Yang, M.Z.; Drumm, E.C. Stability evaluation for the siting of municipal landfills in karst. Eng. Geol. 2002, 65, 185–195. [Google Scholar] [CrossRef]
- Drumm, E.C.; Aktürk, Ö.; Akgün, H.; Tutluoğlu, L. Stability charts for the collapse of residual soil in karst. J. Geotech. Geoenvironmental Eng. 2009, 135, 925–931. [Google Scholar] [CrossRef]
- Ukritchon, B.; Yoang, S.; Keawsawasvong, S. Three-dimensional stability analysis of the collapse pressure on flexible pavements over rectangular trapdoors. Transp. Geotech. 2019, 21, 100277. [Google Scholar] [CrossRef]
- OptumCE, OptumG2. Copenhagen, Denmark: Optum Computational Engineering. 2021. Available online: https://optumce.com/ (accessed on 25 June 2021).
- Broms, B.B.; Bennermark, H. Stability of clay at vertical openings. J. Soil Mech. Found. Div. 1967, 93, 71–94. [Google Scholar] [CrossRef]
- Davis, E.; Gunn, M.; Mair, R.; Seneviratine, H. ‘The stability of shallow tunnels and underground openings in cohesive material. Geotechnique 1980, 30, 397–416. [Google Scholar] [CrossRef]
- Shiau, J.; Al-Asadi, F. Revisiting circular tunnel stability using Broms and Bennermarks’ Original Stability Number. Int. J. Geomech. 2021, 21, 06021009. [Google Scholar] [CrossRef]
- Wilson, D.W.; Abbo, A.J.; Sloan, S.W.; Lyamin, A.V. Undrained stability of a circular tunnel where the shear strength increases linearly with depth. Can. Geotech. J. 2011, 48, 1328–1342. [Google Scholar] [CrossRef]
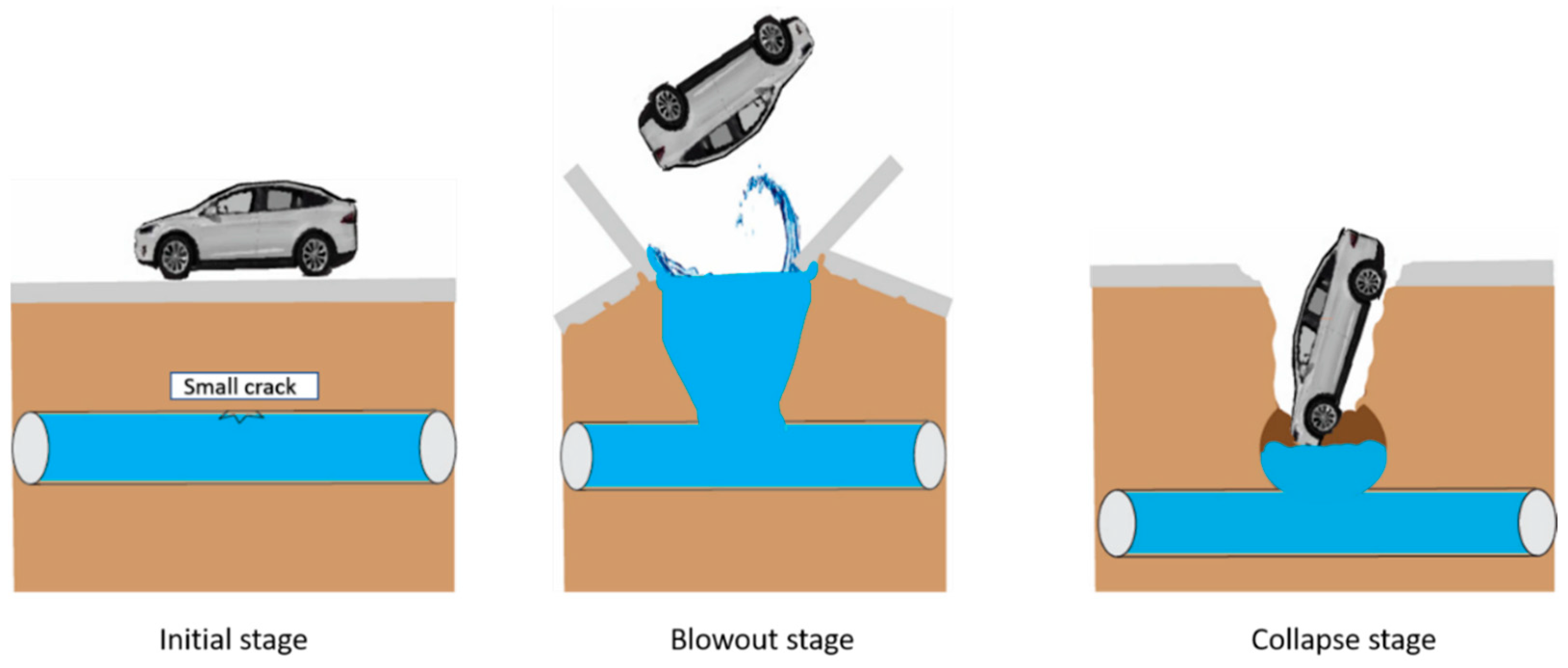



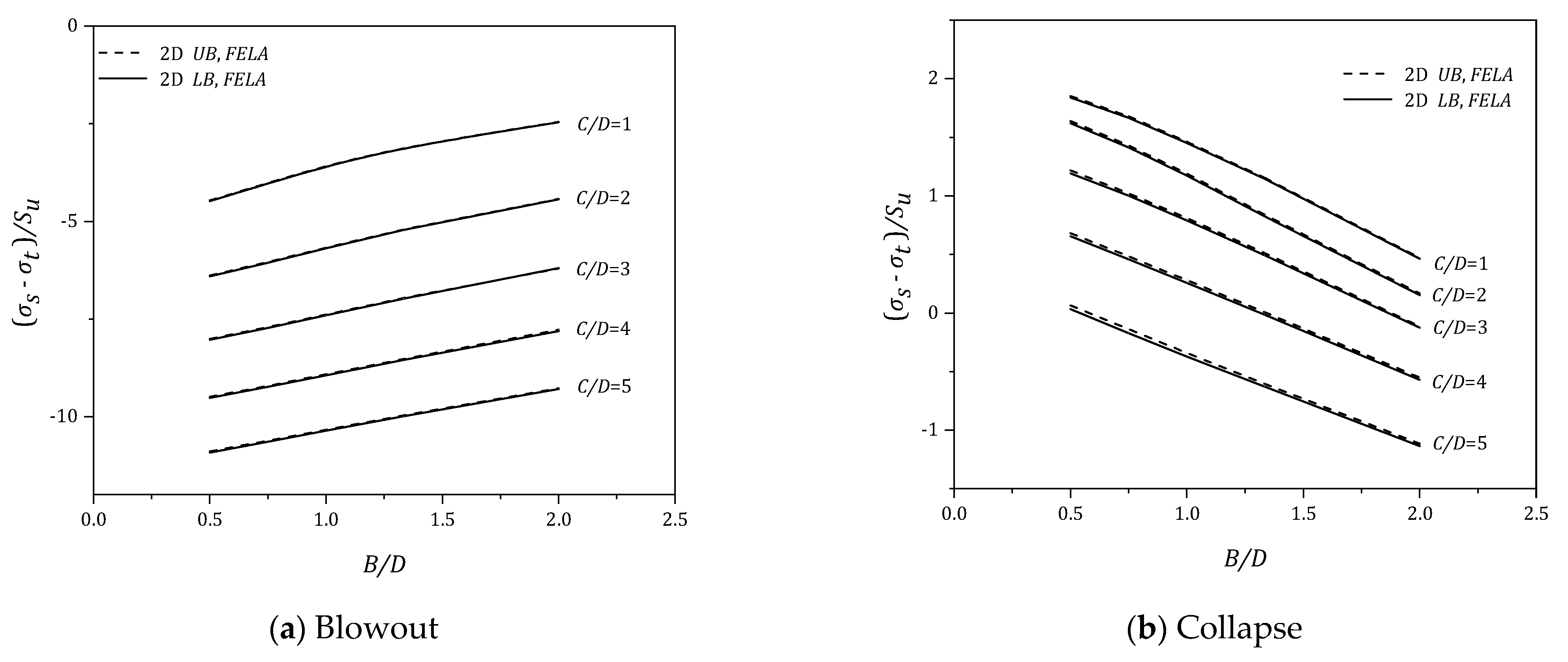





| Date | Location | Cause of Catastrophe | Effects | Reference |
|---|---|---|---|---|
| April 2021 | Maryland, U.S | water main break | damaged the home yard, and water spewing about 30 feet in the air. | (Opera News, [5]) |
| Jan 2021 | Sydney, Australia | water main burst | the spurt of water several meters up in the sky | (NewsComAu, [6]) |
| April 2021 | Tennessee, US | storm sewer pipe collapsed | road damaged | (News Break, [7]) |
| May 2021 | Roma, Italy | pipeline leakage | swallowed two parked cars | (WinNews, [8]) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shiau, J.; Keawsawasvong, S.; Chudal, B.; Mahalingasivam, K.; Seehavong, S. Sinkhole Stability in Elliptical Cavity under Collapse and Blowout Conditions. Geosciences 2021, 11, 421. https://doi.org/10.3390/geosciences11100421
Shiau J, Keawsawasvong S, Chudal B, Mahalingasivam K, Seehavong S. Sinkhole Stability in Elliptical Cavity under Collapse and Blowout Conditions. Geosciences. 2021; 11(10):421. https://doi.org/10.3390/geosciences11100421
Chicago/Turabian StyleShiau, Jim, Suraparb Keawsawasvong, Bishal Chudal, Kiritharan Mahalingasivam, and Sorawit Seehavong. 2021. "Sinkhole Stability in Elliptical Cavity under Collapse and Blowout Conditions" Geosciences 11, no. 10: 421. https://doi.org/10.3390/geosciences11100421
APA StyleShiau, J., Keawsawasvong, S., Chudal, B., Mahalingasivam, K., & Seehavong, S. (2021). Sinkhole Stability in Elliptical Cavity under Collapse and Blowout Conditions. Geosciences, 11(10), 421. https://doi.org/10.3390/geosciences11100421







