Insights into Mechanical Properties of the 1980 Irpinia Fault System from the Analysis of a Seismic Sequence
Abstract
1. Introduction
2. Seismotectonic Setting
2.1. Structural Setting of Southern Apennines
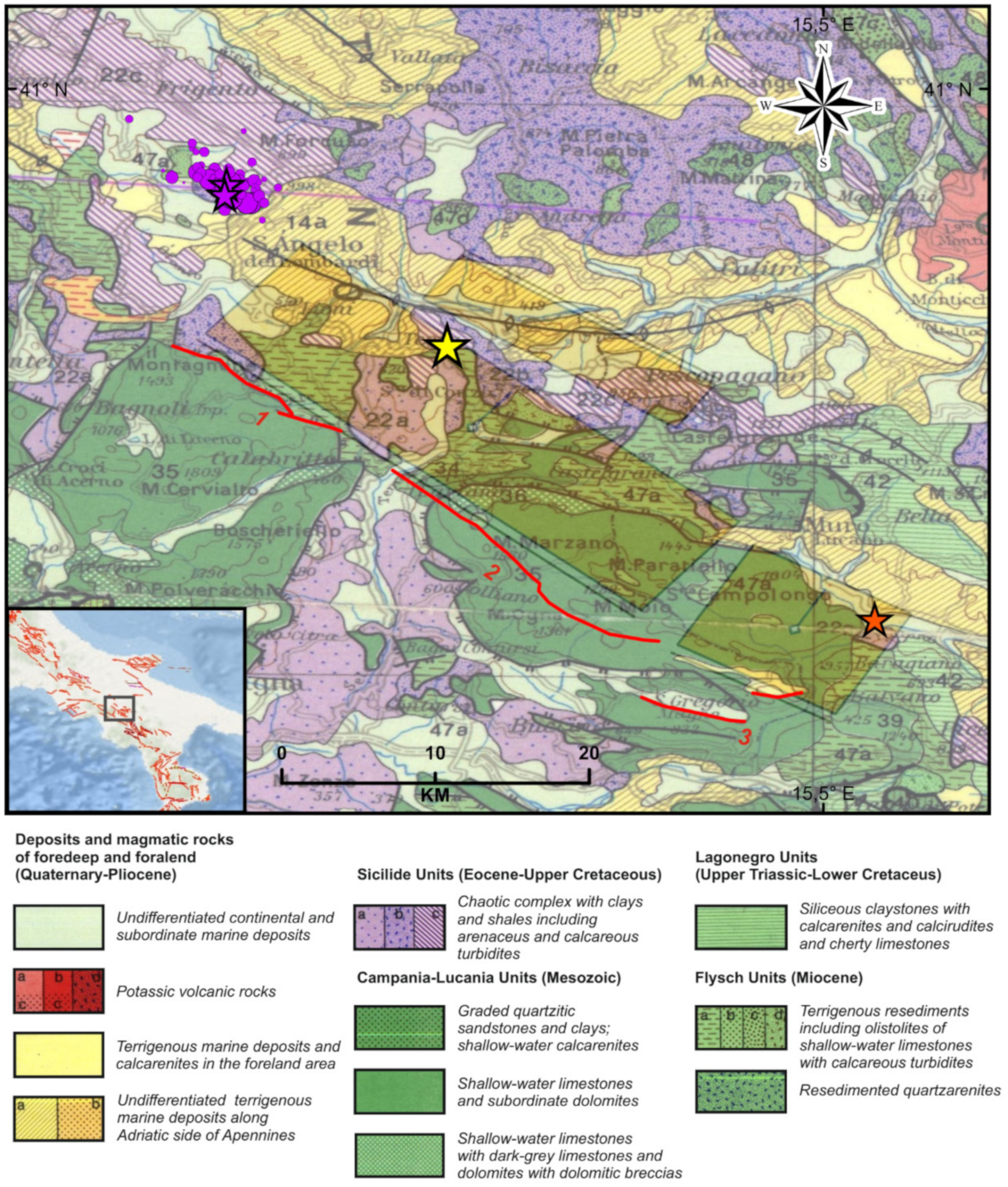
2.2. Seismogenic Normal Faults
2.3. 1980 Irpinia Earthquake
2.4. Recent Seismicity of the Irpinia Region
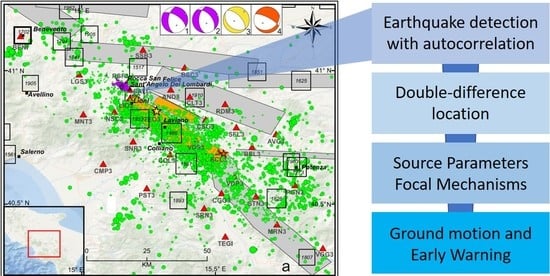
3. Data
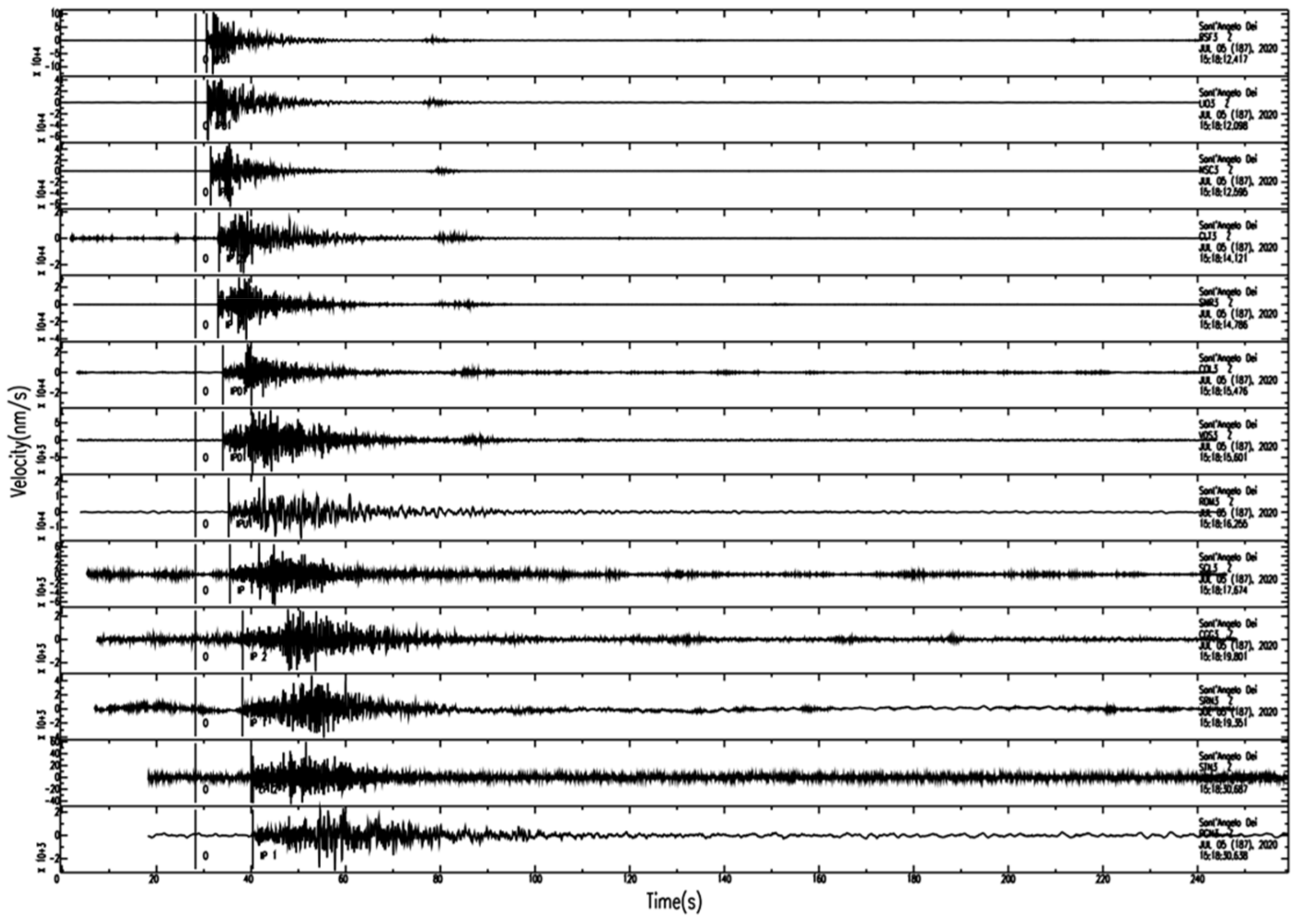
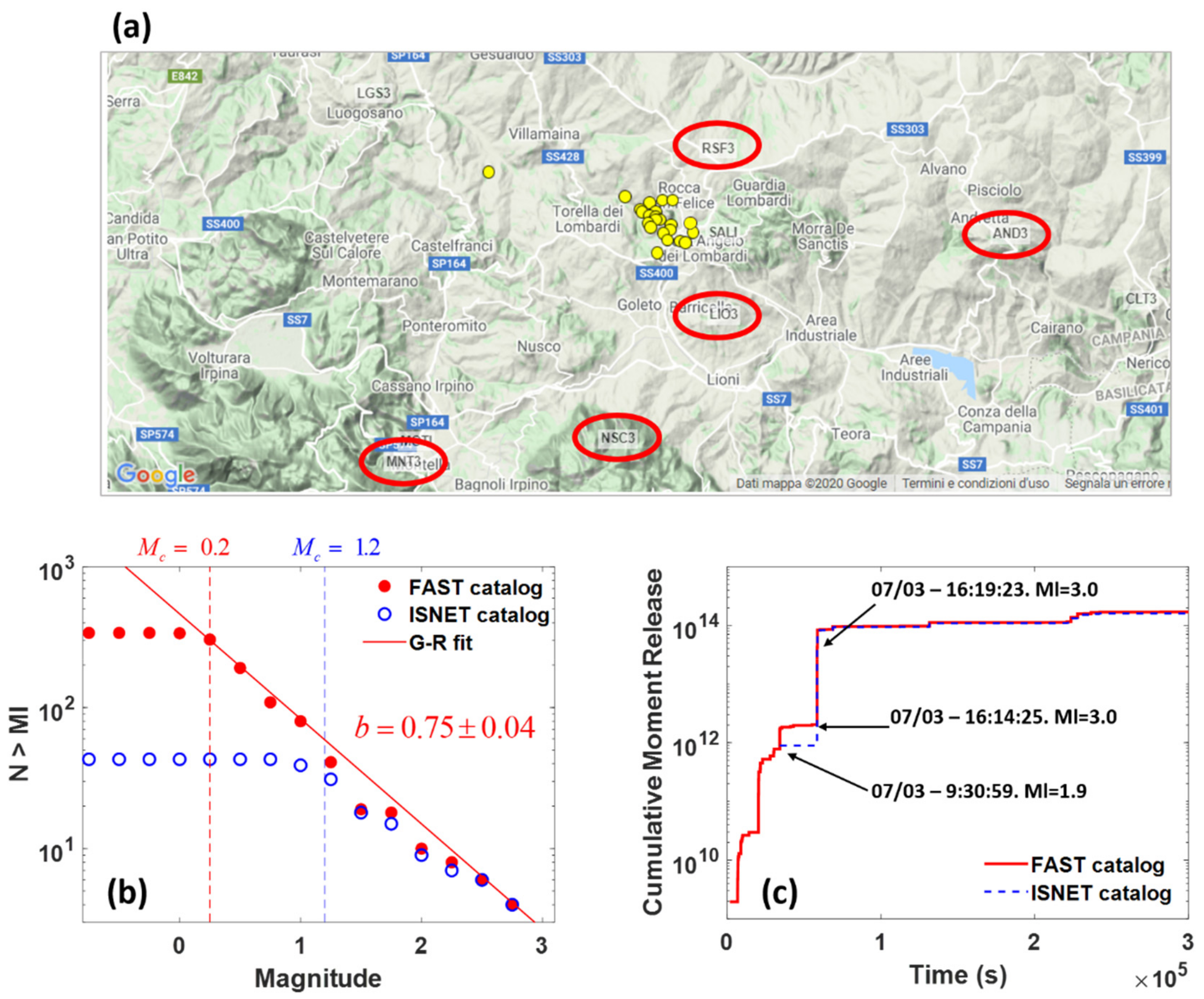
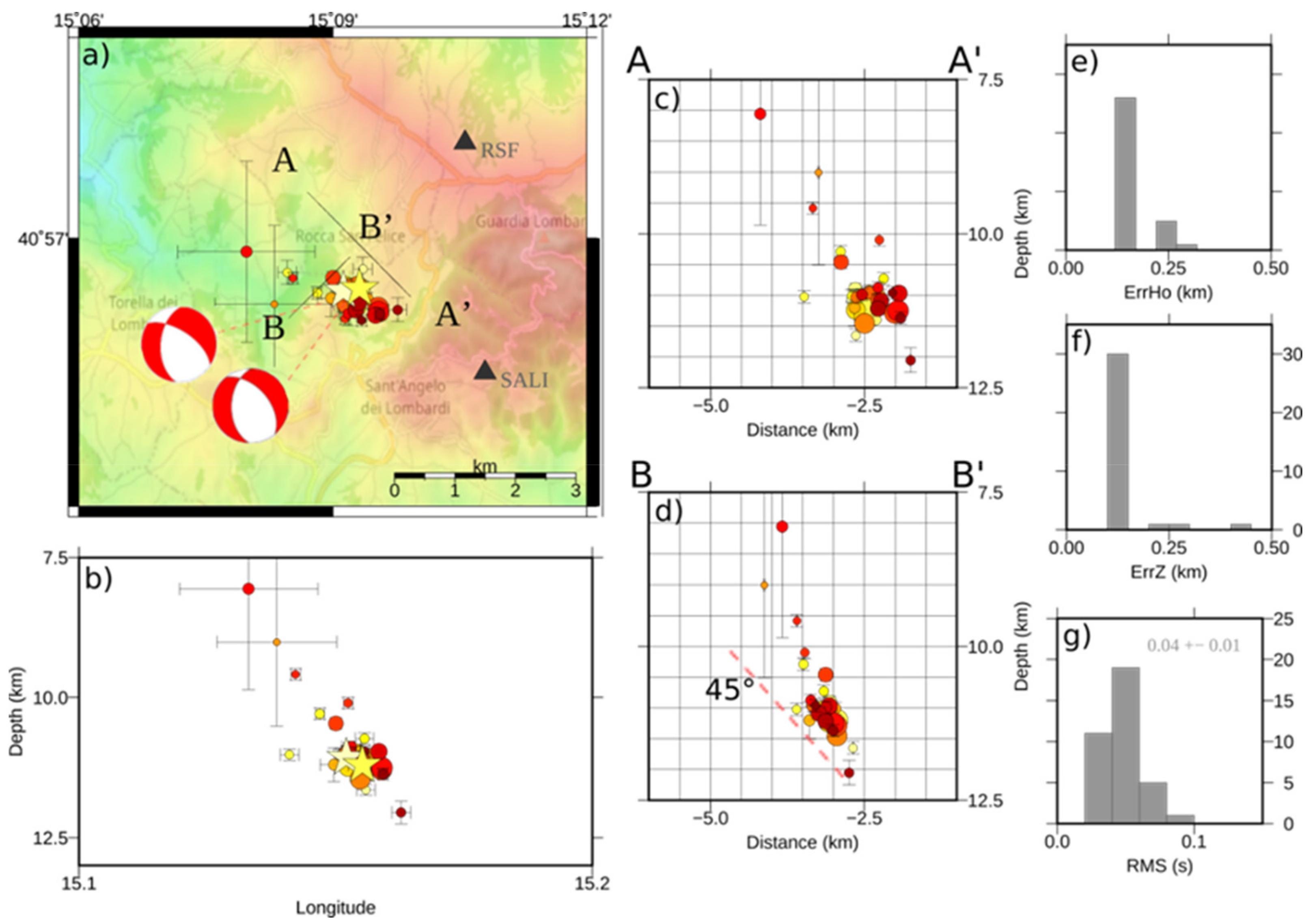
4. Refined Seismic Catalog
5. Accurate Earthquake Location
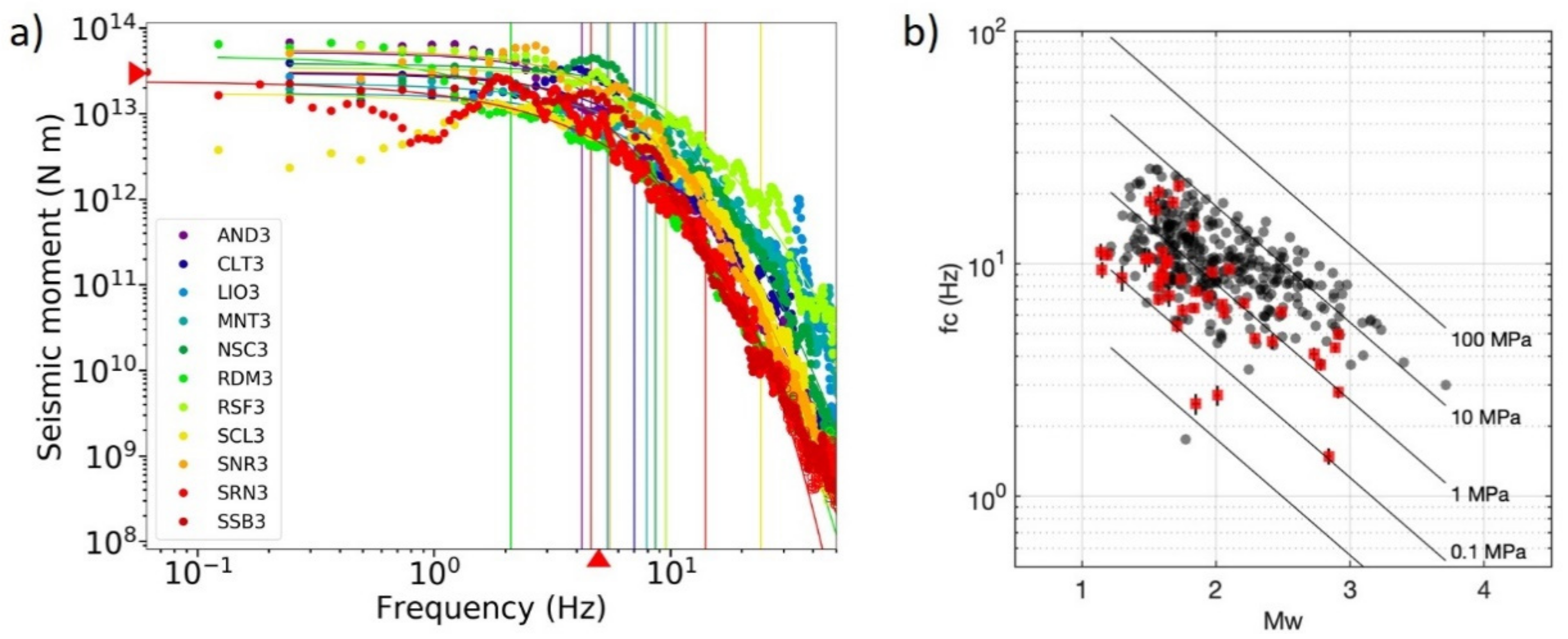
6. Source Parameters
7. Focal Mechanism Solutions
8. Ground Motion
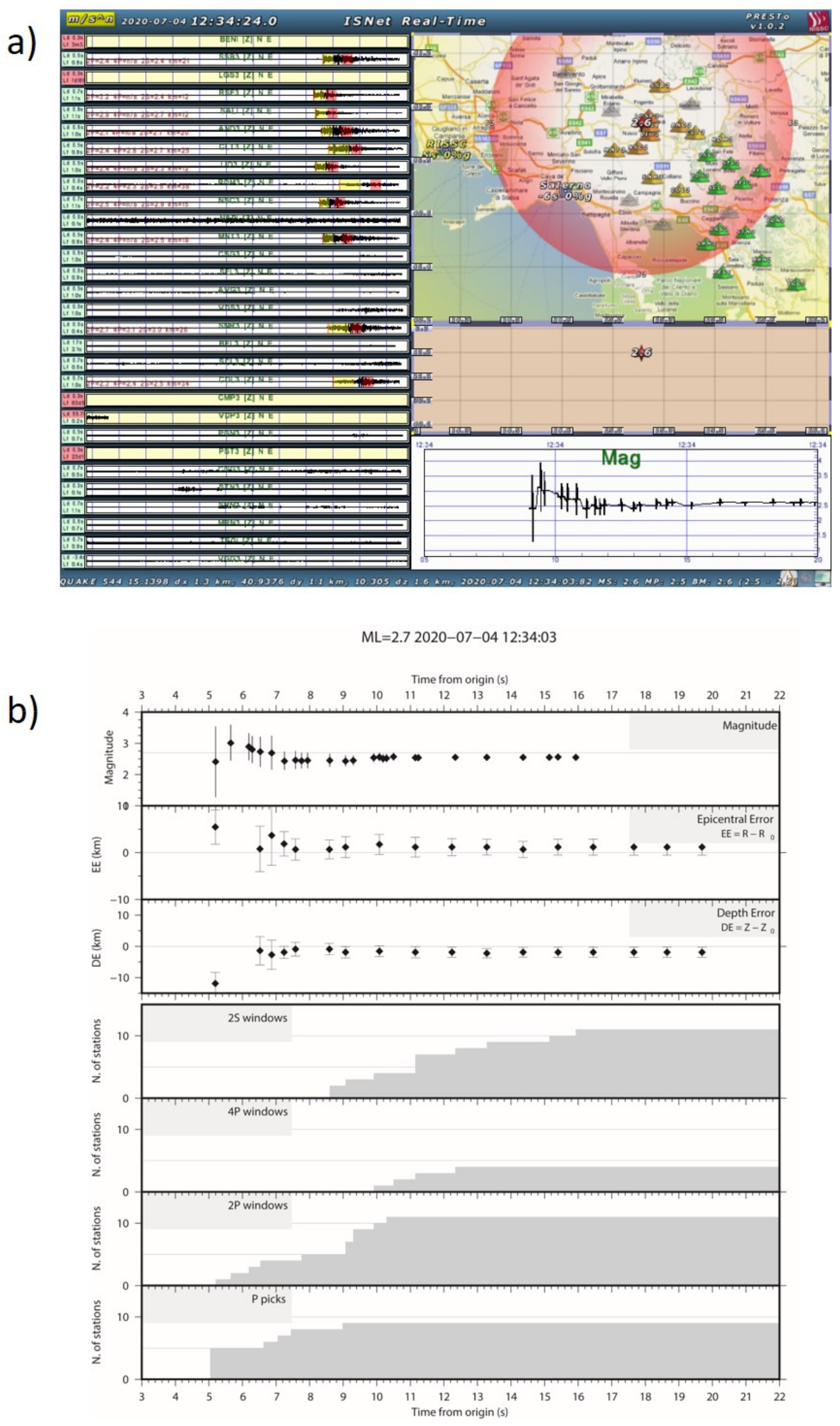
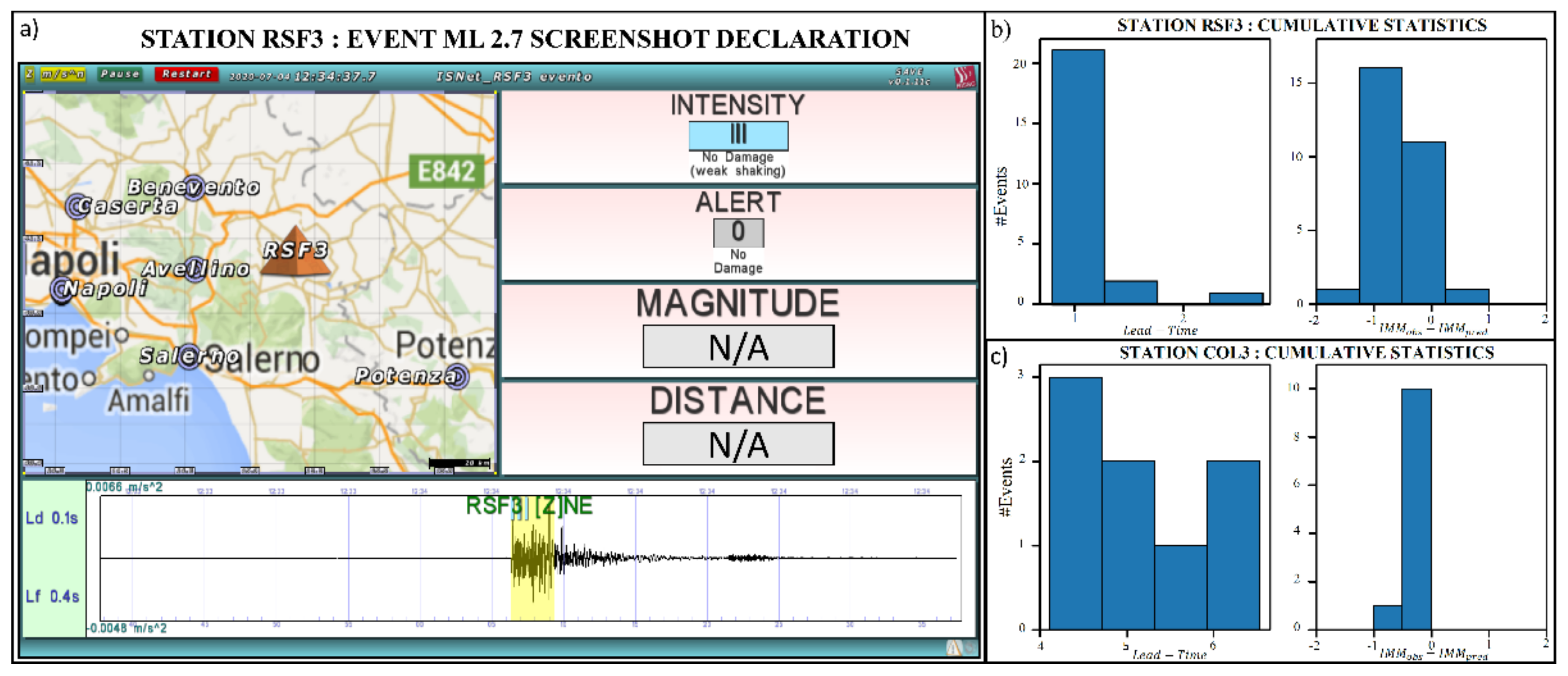
9. Early Warning Analysis
10. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lomax, A.; Virieux, J.; Volant, P.; Berge-Thierry, C. Probabilistic Earthquake Location in 3D and Layered Models. In Advances in Seismic Event Location; Modern Approaches in Geophysics, Volume 18; Thurber, C.H., Rabinowitz, N., Eds.; Springer: Dordrecht, The Netherlands, 2000. [Google Scholar] [CrossRef]
- Satriano, C.; Lomax, A.; Zollo, A. Real-Time Evolutionary Earthquake Location for Seismic Early Warning. Bull. Seism. Soc. Am. 2008, 98, 1482–1494. [Google Scholar] [CrossRef]
- Waldhauser, F.; Ellsworth, W.L. A double-difference earthquake location algorithm: Method and application to the northern Hayward Fault, California. Bull. Seismol. Soc. Am. 2000, 90, 1353–1368. [Google Scholar] [CrossRef]
- Stabile, T.A.; Satriano, C.; Orefice, A.; Festa, G.; Zollo, A. Anatomy of a microearthquake sequence on an active normal fault. Sci. Rep. 2012, 2, 410. [Google Scholar] [CrossRef] [PubMed]
- Rowe, C.A.; Thurber, C.H.; White, R.A. Dome growth behavior at Soufriere Hills Volcano, Montserrat, revealed by relocation of volcanic event swarms, 1995–1996. J. Volcanol. Geotherm. Res. 2004, 134, 199–221. [Google Scholar] [CrossRef]
- Valoroso, L.; Chiaraluce, L.; Piccinini, D.; Di Stefano, R.; Schaff, D.; Waldhauser, F. Radiography of a normal fault system by 64,000 high-precision earthquake locations: The 2009 L’Aquila (central Italy) case study. J. Geophys. Res. Solid Earth 2013, 118, 1156–1176. [Google Scholar] [CrossRef]
- Chen, X.; Shearer, P.M.; Abercrombie, R.E. Spatial migration of earthquakes within seismic clusters in Southern California: Evidence for fluid diffusion. J. Geophys. Res. Space Phys. 2012, 117. [Google Scholar] [CrossRef]
- Miller, S.A. Aftershocks are fluid-driven and decay rates controlled by permeability dynamics. Nat. Commun. 2020, 11, 5787. [Google Scholar] [CrossRef]
- Abercrombie, R.E. Earthquake source scaling relationships from− 1 to 5 ML using seismograms recorded at 2.5-km depth. J. Geophys. Res. 1995, 100, 24015–24036. [Google Scholar] [CrossRef]
- Prieto, G.A.; Shearer, P.M.; Vernon, F.L.; Kilb, D. Earthquake source scaling and self-similarity estimation from stacking P and S spectra. J. Geophys. Res. 2004, 109. [Google Scholar] [CrossRef]
- Abercrombie, R.E.; Rice, J.R. Can observations of earthquake scaling constrain slip weakening? Geophys. J. Int. 2005, 162, 406–424. [Google Scholar] [CrossRef]
- Oth, A.; Parolai, S.; Bindi, D.; Wenzel, F. Source Spectra and Site Response from S Waves of Intermediate-Depth Vrancea, Romania, Earthquakes. Bull. Seism. Soc. Am. 2009, 99, 235–254. [Google Scholar] [CrossRef]
- Miller, S.A. Properties of large ruptures and the dynamical influence of fluids on earthquakes and faulting. J. Geophys. Res. Solid Earth 2002, 107, ESE-3. [Google Scholar] [CrossRef]
- Dublanchet, P.; Godano, M.; Bernard, P. Inferring fault mechanical conditions from the source parameters of a complex microseismic multiplet in the Corinth rift, Greece. J. Geophys. Res. Solid Earth 2015, 120, 7655–7682. [Google Scholar] [CrossRef]
- Amoroso, O.; Russo, G.; De Landro, G.; Zollo, A.; Garambois, S.; Mazzoli, S.; Parente, M.; Virieux, J. From velocity and attenuation tomography to rock physical modeling: Inferences on fluid-driven earthquake processes at the Irpinia fault system in southern Italy. Geophys. Res. Lett. 2017, 44, 6752–6760. [Google Scholar] [CrossRef]
- Bouchon, M.; Durand, V.; Marsan, D.; Karabulut, H.; Schmittbuhl, J. The long precursory phase of most large interplate earthquakes. Nat. Geosci. 2013, 6, 299–302. [Google Scholar] [CrossRef]
- Trugman, D.T.; Ross, Z.E. Pervasive Foreshock Activity Across Southern California. Geophys. Res. Lett. 2019, 46, 8772–8781. [Google Scholar] [CrossRef]
- Ende, M.P.A.V.D.; Ampuero, J.-P. On the Statistical Significance of Foreshock Sequences in Southern California. Geophys. Res. Lett. 2020, 47. [Google Scholar] [CrossRef]
- Gibbons, S.J.; Ringdal, F. The detection of low magnitude seismic events using array-based waveform correlation. Geophys. J. Int. 2006, 165, 149–166. [Google Scholar] [CrossRef]
- Yoon, C.E.; O’Reilly, O.; Bergen, K.J.; Beroza, G.C. Earthquake detection through computationally efficient similarity search. Sci. Adv. 2015, 1, e1501057. [Google Scholar] [CrossRef]
- Picozzi, M.; Bindi, D.; Zollo, A.; Festa, G.; Spallarossa, D. Detecting long-lasting transients of earthquake activity on a fault system by monitoring apparent stress, ground motion and clustering. Sci. Rep. 2019, 9, 16268. [Google Scholar] [CrossRef]
- Iannaccone, G.; Zollo, A.; Elia, L.; Convertito, V.; Satriano, C.; Martino, C.; Festa, G.; Lancieri, M.; Bobbio, A.; Stabile, T.A.; et al. A prototype system for earthquake early-warning and alert management in southern Italy. Bull. Earthq. Eng. 2010, 8, 1105–1129. [Google Scholar] [CrossRef]
- Satriano, C.; Elia, L.; Martino, C.; Lancieri, M.; Zollo, A.; Iannaccone, G. PRESTo, the earthquake early warning system for Southern Italy: Concepts, capabilities and future perspectives. Soil Dyn. Earthq. Eng. 2011, 31, 137–153. [Google Scholar] [CrossRef]
- Doglioni, C. Geological remarks on the relationships between extension and convergent geodynamic settings. Tectonophysics 1995, 252, 253–267. [Google Scholar] [CrossRef]
- Patacca, E.; Scandone, P. Geology of the southern Apennines. Boll. Soc. Geol. It. 2007, 7, 75–119. [Google Scholar]
- Cavazza, W.; Roure, F.M.; Spakman, W.; Stampfli, G.M.; Ziegler, P.A. The TRANSMED Atlas. The Mediterranean Region from Crust to Mantle: The Mediterranean Region from Crust to Mantle: Geological and Geophysical Framework of the Mediterranean and the Surrounding Areas; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Casero, P.; Roure, F.; Vially, R. Tectonic framework and petroleum potential of the southern Apennines. Gener. Accumul. Prod. Eur. Hydrocarb. 1991, 1, 381–387. [Google Scholar]
- Bally, A.; Burbi, L.; Cooper, C.; Ghelardoni, R. Balanced cross sections and seismic reflection profiles across the central Apennines. Memorie della Societa Geologica Italiana 1986, 35, 275–310. [Google Scholar]
- Patacca, E.; Sartori, R.; Scandone, P. Tyrrhenian basin and Apenninic arcs: Kinematic relations since late Tortonian times. Memorie della Societa Geologica Italiana 1990, 45, 425–451. [Google Scholar]
- Frepoli, A.; Amato, A. Contemporaneous extension and compression in the Northern Apennines from earthquake fault-plane solutions. Geophys. J. Int. 1997, 129, 368–388. [Google Scholar] [CrossRef]
- D’Agostino, N.; Avallone, A.; Cheloni, D.; D’Anastasio, E.; Mantenuto, S.; Selvaggi, G. Active tectonics of the Adriatic region from GPS and earthquake slip vectors. J. Geophys. Res. Solid Earth 2008, 113. [Google Scholar] [CrossRef]
- Ascione, A.; Mazzoli, S.; Petrosino, P.; Valente, E. A decoupled kinematic model for active normal faults: Insights from the 1980, MS = 6.9 Irpinia earthquake, southern Italy. GSA Bull. 2013, 125, 1239–1259. [Google Scholar] [CrossRef]
- Chiarabba, C.; Jovane, L.; Distefano, R. A new view of Italian seismicity using 20 years of instrumental recordings. Tectonophysics 2005, 395, 251–268. [Google Scholar] [CrossRef]
- Improta, L.; De Gori, P.; Chiarabba, C. New insights into crustal structure, Cenozoic magmatism, CO2degassing, and seismogenesis in the southern Apennines and Irpinia region from local earthquake tomography. J. Geophys. Res. Solid Earth 2014, 119, 8283–8311. [Google Scholar] [CrossRef]
- Pantosti, D.; Schwartz, D.P.; Valensise, G. Paleoseismology along the 1980 surface rupture of the Irpinia Fault: Implications for earthquake recurrence in the southern Apennines, Italy. J. Geophys. Res. Solid Earth 1993, 98, 6561–6577. [Google Scholar] [CrossRef]
- Buck, W.R. flexural rotation of normal faults. Tectonics 1988, 7, 959–973. [Google Scholar] [CrossRef]
- King, G.; Ellis, M. The origin of large local uplift in extensional regions. Nature 1990, 348, 689–693. [Google Scholar] [CrossRef]
- Ghisetti, F.; Vezzani, L. Normal faulting, transcrustal permeability and seismogenesis in the Apennines (Italy). Tectonophysics 2002, 348, 155–168. [Google Scholar] [CrossRef]
- Westaway, R.; Jackson, J. Surface faulting in the southern Italian Campania-Basilicata earthquake of 23 November 1980. Nat. Cell Biol. 1984, 312, 436–438. [Google Scholar] [CrossRef]
- Westaway, R.; Jackson, J. The earthquake of 1980 November 23 in Campania--Basilicata (southern Italy). Geophys. J. Int. 1987, 90, 375–443. [Google Scholar] [CrossRef]
- Bernard, P.; Zollo, A. The Irpinia (Italy) 1980 earthquake: Detailed analysis of a complex normal faulting. J. Geophys. Res. Solid Earth 1989, 94, 1631–1647. [Google Scholar] [CrossRef]
- Pantosti, D.; Valensise, G. Faulting mechanism and complexity of the November 23, 1980, Campania-Lucania Earthquake, inferred from surface observations. J. Geophys. Res. Solid Earth 1990, 95, 15319–15341. [Google Scholar] [CrossRef]
- Amoruso, A.; Crescentini, L.; Scarpa, R. Faulting geometry for the complex 1980 Campania-Lucania earthquake from levelling data. Geophys. J. Int. 2005, 162, 156–168. [Google Scholar] [CrossRef]
- Amato, A.; Chiarabba, C.; Malagnini, L.; Selvaggi, G. Three-dimensional P-velocity structure in the region of the MS = 6.9 Irpinia, Italy, normal faulting earthquake. Phys. Earth Planet. Inter. 1992, 75, 111–119. [Google Scholar] [CrossRef]
- Amato, A.; Selvaggi, G. Aftershock location and P-velocity structure in the epicentral region, of the 1980 Irpinia earthquake. Annali di Geophysica 1993, 36, 237–243. [Google Scholar]
- Patella, D.; Petrillo, Z.; Siniscalchi, A.; Improta, L.; Di Fiore, B. Magnetotelluric profiling along the CROP-04 section in the Southern Apennines. In CROP PROJECT: Deep Seismic Exploration of the CENTRAL Mediterranean and Italy; Finetti, I.R., Ed.; Elsevier: Amsterdam, The Netherlands, 2005; pp. 263–280. [Google Scholar]
- De Matteis, R.; Matrullo, E.; Rivera, L.; Stabile, T.A.; Pasquale, G.; Zollo, A. Fault Delineation and Regional Stress Direction from the Analysis of Background Microseismicity in the southern Apennines, Italy. Bull. Seism. Soc. Am. 2012, 102, 1899–1907. [Google Scholar] [CrossRef]
- De Landro, G.; Amoroso, O.; Stabile, T.A.; Matrullo, E.; Lomax, A.; Zollo, A. High-precision differential earthquake location in 3-D models: Evidence for a rheological barrier controlling the microseismicity at the Irpinia fault zone in southern Apennines. Geophys. J. Int. 2015, 203, 1821–1831. [Google Scholar] [CrossRef]
- Adinolfi, G.M.; Cesca, S.; Picozzi, M.; Heimann, S.; Zollo, A. Detection of weak seismic sequences based on arrival time coherence and empiric network detectability: An application at a near fault observatory. Geophys. J. Int. 2019, 218, 2054–2065. [Google Scholar] [CrossRef]
- Vassallo, M.; Festa, G.; Bobbio, A.; Serra, M. Low shear velocity in a normal fault system imaged by ambient noise cross correlation: The case of the Irpinia fault zone, Southern Italy. J. Geophys. Res. Solid Earth 2016, 121, 4290–4305. [Google Scholar] [CrossRef]
- Chiarabba, C.; Chiodini, G. Continental delamination and mantle dynamics drive topography, extension and fluid discharge in the Apennines. Geology 2013, 41, 715–718. [Google Scholar] [CrossRef]
- Italiano, F.; Martelli, M.; Martinelli, G.; Nuccio, P.M. Geochemical evidence of melt intrusions along lithospheric faults of the Southern Apennines, Italy: Geodynamic and seismogenic implications. J. Geophys. Res. Solid Earth 2000, 105, 13569–13578. [Google Scholar] [CrossRef]
- Chiodini, G.; Granieri, D.; Avino, R.; Caliro, S.; Costa, A.; Minopoli, C.; Vilardo, G. Non-volcanic CO2Earth degassing: Case of Mefite d’Ansanto (southern Apennines), Italy. Geophys. Res. Lett. 2010, 37. [Google Scholar] [CrossRef]
- Miller, S.A.; Collettini, C.; Chiaraluce, L.; Cocco, M.; Barchi, M.; Kaus, B.J.P. Aftershocks driven by a high-pressure CO2 source at depth. Nature 2004, 427, 724–727. [Google Scholar] [CrossRef] [PubMed]
- Lucente, F.P.; De Gori, P.; Margheriti, L.; Piccinini, D.; Di Bona, M.; Chiarabba, C.; Agostinetti, N.P. Temporal variation of seismic velocity and anisotropy before the 2009 MW6.3 L’Aquila earthquake, Italy. Geology 2010, 38, 1015–1018. [Google Scholar] [CrossRef]
- Johnson, C.E.; Bittenbinder, A.; Bogaert, B.; Dietz, L.; Kohler, W. Earthworm: A flexible approach to seismic network processing. Iris Newsl. 1995, 14, 1–4. [Google Scholar]
- Cocco, M.; Chiarabba, C.; Di Bona, M.; Selvaggi, G.; Margheriti, L.; Frepoli, A.; Lucente, F.P.; Basili, A.; Jongmans, D.; Campillo, M. The April 1996 Irpinia seismic sequence: Evidence for fault interaction. J. Seism. 1999, 3, 105–117. [Google Scholar] [CrossRef]
- Bergen, K.J.; Beroza, G.C. Detecting earthquakes over a seismic network using single-station similarity measures. Geophys. J. Int. 2018, 213, 1984–1998. [Google Scholar] [CrossRef]
- Andoni, A.; Indyk, P. Near-Optimal Hashing Algorithms for Approximate Nearest Neighbor in High Dimensions. In Proceedings of the 47th Annual IEEE Symposium on Foundations of Computer Science (FOCS’06), Berkeley, CA, USA, 21–24 October 2006; pp. 459–468. [Google Scholar]
- Vassallo, M.; Festa, G.; Bobbio, A. Seismic Ambient Noise Analysis in Southern Italy. Bull. Seism. Soc. Am. 2012, 102, 574–586. [Google Scholar] [CrossRef]
- Michelini, A.; Lomax, A. The effect of velocity structure errors on double-difference earthquake location. Geophys. Res. Lett. 2004, 31. [Google Scholar] [CrossRef]
- Amoroso, O.; Ascione, A.; Mazzoli, S.; Virieux, J.; Zollo, A. Seismic imaging of a fluid storage in the actively extending Apennine mountain belt, southern Italy. Geophys. Res. Lett. 2014, 41, 3802–3809. [Google Scholar] [CrossRef]
- Brune, J.N. Tectonic stress and the spectra of seismic shear waves from earthquakes. J. Geophys. Res. 1970, 75, 4997–5009. [Google Scholar] [CrossRef]
- Supino, M.; Festa, G.; Zollo, A. A probabilistic method for the estimation of earthquake source parameters from spectral inversion: Application to the 2016–2017 Central Italy seismic sequence. Geophys. J. Int. 2019, 218, 988–1007. [Google Scholar] [CrossRef]
- Zollo, A.; Orefice, A.; Convertito, V. Source parameter scaling and radiation efficiency of microearthquakes along the Irpinia fault zone in southern Apennines, Italy. J. Geophys. Res. Solid Earth 2014, 119, 3256–3275. [Google Scholar] [CrossRef]
- Festa, G.; Zollo, A.; Lancieri, M. Earthquake magnitude estimation from early radiated energy. Geophys. Res. Lett. 2008, 35. [Google Scholar] [CrossRef]
- Reasenberg, P.; Oppenheimer, D. FPFIT, FPPLOT and FPPAGE: Fortran Computer Programs for Calculating and Displaying Earthquake Fault-Plane Solutions; Open File Report 85–739; US Geological Survey: Menlo Park, CA, USA, 1985; pp. 85–739.
- Zhu, T.J.; Heidebrecht, A.C.; Tso, W.K. Effect of peak ground acceleration to velocity ratio on ductility demand of inelastic systems. Earthq. Eng. Struct. Dyn. 1988, 16, 63–79. [Google Scholar] [CrossRef]
- Ameri, G.; Emolo, A.; Pacor, F.; Gallovič, F. Ground-motion simulations for the 1980 M 6.9 Irpinia earthquake (Southern Italy) and scenario events. Bull. Seismol. Soc. Am. 2011, 101, 1136–1151. [Google Scholar] [CrossRef]
- Kwon, O.S.; Elnashai, A. The effect of material and ground motion uncertainty on the seismic vulnerability curves of RC structure. Eng. Struct. 2006, 28, 289–303. [Google Scholar] [CrossRef]
- Emolo, A.; Convertito, V.; Cantore, L. Ground-motion predictive equations for low-magnitude earthquakes in the Campania–Lucania area, Southern Italy. J. Geophys. Eng. 2011, 8, 46–60. [Google Scholar] [CrossRef]
- Wald, D.J.; Quitoriano, V.; Heaton, T.H.; Kanamori, H.; Scrivner, C.W.; Worden, C.B. TriNet “ShakeMaps”: Rapid Generation of Peak Ground Motion and Intensity Maps for Earthquakes in Southern California. Earthq. Spectra 1999, 15, 537–555. [Google Scholar] [CrossRef]
- Caruso, A.; Colombelli, S.; Elia, L.; Picozzi, M.; Zollo, A. An on-site alert level early warning system for Italy. J. Geophys. Res. Solid Earth 2017, 122, 2106–2118. [Google Scholar] [CrossRef]
- Colombelli, S.; Carotenuto, F.; Elia, L.; Zollo, A. Design and implementation of a mobile device app for network-based earthquake early warning systems (EEWSs): Application to the PRESTo EEWS in southern Italy. Nat. Hazards Earth Syst. Sci. 2020, 20. [Google Scholar] [CrossRef]
- Deschamps, A.; King, G.C.P. The Campania-Lucania (southern Italy) earthquake of 23 November 1980. Earth Planet. Sci. Lett. 1983, 62, 296–304. [Google Scholar] [CrossRef]
- Scholz, C.H. The frequency-magnitude relation of microfracturing in rock and its relation to earthquakes. Bull. Seismol. Soc. Am. 1968, 58, 399–415. [Google Scholar]
- Festa, G.; Picozzi, M.; Caruso, A.; Colombelli, S.; Cattaneo, M.; Chiaraluce, L.; Elia, L.; Martino, C.; Marzorati, S.; Supino, M.; et al. Performance of Earthquake Early Warning Systems during the 2016–2017 Mw 5–6.5 Central Italy Sequence. Seism. Res. Lett. 2018, 89, 1–12. [Google Scholar] [CrossRef]
- Nazeri, S.; Colombelli, S.; Zollo, A. Fast and accurate determination of earthquake moment, rupture length and stress release for the 2016–2017 Central Italy seismic sequence. Geophys. J. Int. 2019, 217, 1425–1432. [Google Scholar] [CrossRef]
- Bigi, G.; Coli, M.; Cosentino, D.; Parotto, M.; Praturlon, A.; Sartori, R.; Scandone, P.; Turco, E. Structural Model of Italy scale 1:500.000, sheet 4. In C.N.R., Progetto Finalizzato Geodinamica; SELCA: Firenze, Italy, 1992. [Google Scholar]


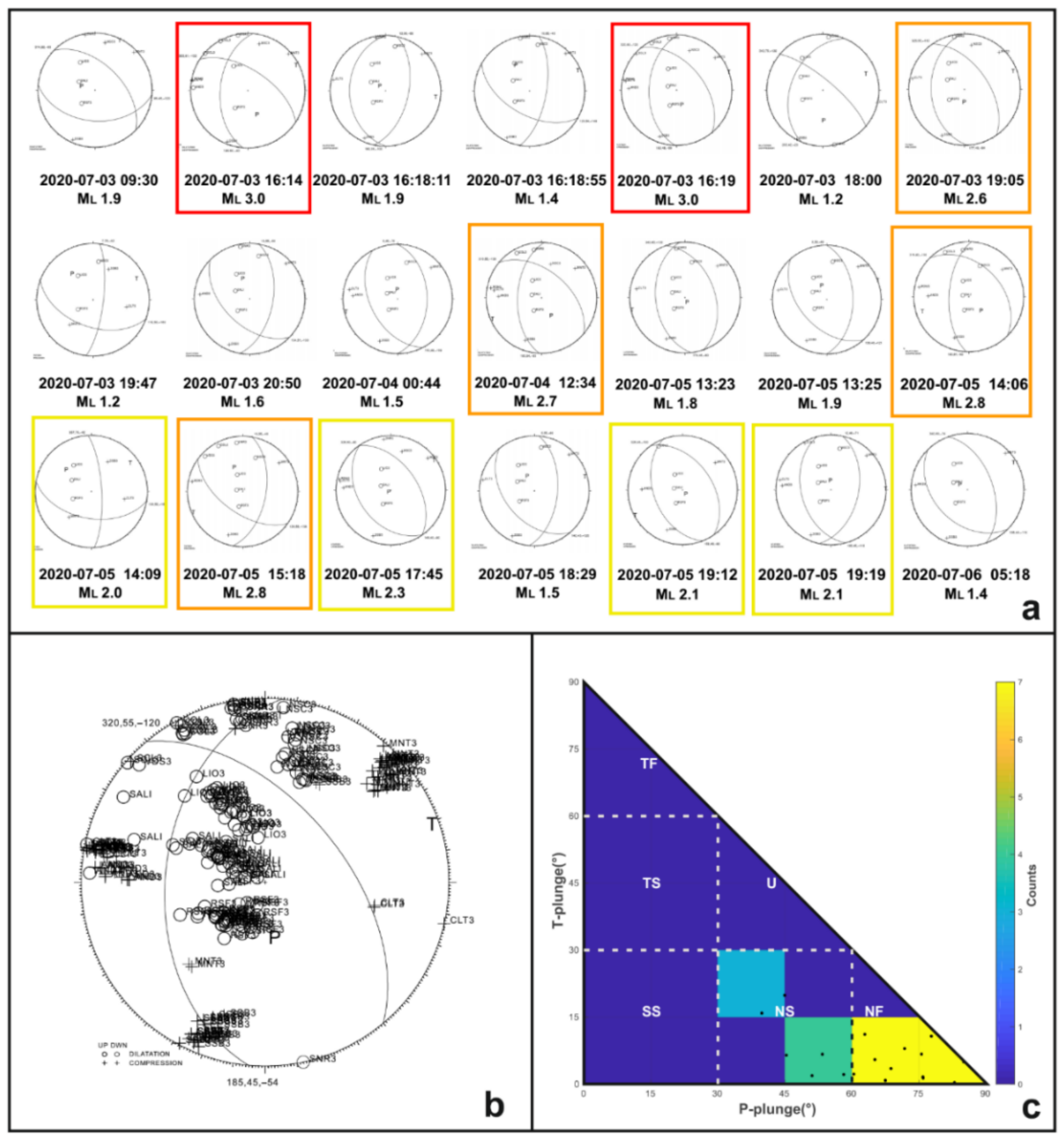

| Station | Polar. | No. | Polar. | No. |
|---|---|---|---|---|
| AND3 | UP | 9 | DOWN | 1 |
| CLT3 | UP | 16 | DOWN | 1 |
| COL3 | UP | 1 | DOWN | 6 |
| LIO3 | UP | 0 | DOWN | 30 |
| MNT3 | UP | 27 | DOWN | 0 |
| NSC3 | UP | 8 | DOWN | 18 |
| RDM3 | UP | 6 | DOWN | 0 |
| RSF3 | UP | 1 | DOWN | 33 |
| SALI | UP | 0 | DOWN | 38 |
| SCL3 | UP | 1 | DOWN | 1 |
| SNR3 | UP | 4 | DOWN | 11 |
| SSB3 | UP | 22 | DOWN | 0 |
| VDS3 | UP | 0 | DOWN | 1 |
| Event ID | Ml | M_PRESTo | ΔM |
|---|---|---|---|
| 16975 | 1.4 | 1.5 | 0.1 |
| 16973 | 2.1 | 2.1 | 0 |
| 16972 | 2.1 | 2.5 | 0.4 |
| 16967 | 2.8 | 3.4 | 0.6 |
| 16964 | 2 | 2 | 0 |
| 16963 | 2.8 | 3 | 0.2 |
| 16962 | 1.9 | 1.9 | 0 |
| 16961 | 1.8 | 1.2 | −0.6 |
| 16958 | 1.3 | 1.2 | −0.1 |
| 16956 | 2.7 | 3.1 | 0.4 |
| City | Epicentral Distance (km) | Lead-Time (s) |
|---|---|---|
| Avellino | 25.4 | 5.8 |
| Benevento | 33.4 | 7.7 |
| Caserta | 65.4 | 16.6 |
| Napoli | 69.1 | 17.5 |
| Salerno | 39.6 | 9.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Festa, G.; Adinolfi, G.M.; Caruso, A.; Colombelli, S.; De Landro, G.; Elia, L.; Emolo, A.; Picozzi, M.; Scala, A.; Carotenuto, F.; et al. Insights into Mechanical Properties of the 1980 Irpinia Fault System from the Analysis of a Seismic Sequence. Geosciences 2021, 11, 28. https://doi.org/10.3390/geosciences11010028
Festa G, Adinolfi GM, Caruso A, Colombelli S, De Landro G, Elia L, Emolo A, Picozzi M, Scala A, Carotenuto F, et al. Insights into Mechanical Properties of the 1980 Irpinia Fault System from the Analysis of a Seismic Sequence. Geosciences. 2021; 11(1):28. https://doi.org/10.3390/geosciences11010028
Chicago/Turabian StyleFesta, Gaetano, Guido Maria Adinolfi, Alessandro Caruso, Simona Colombelli, Grazia De Landro, Luca Elia, Antonio Emolo, Matteo Picozzi, Antonio Scala, Francesco Carotenuto, and et al. 2021. "Insights into Mechanical Properties of the 1980 Irpinia Fault System from the Analysis of a Seismic Sequence" Geosciences 11, no. 1: 28. https://doi.org/10.3390/geosciences11010028
APA StyleFesta, G., Adinolfi, G. M., Caruso, A., Colombelli, S., De Landro, G., Elia, L., Emolo, A., Picozzi, M., Scala, A., Carotenuto, F., Gammaldi, S., Iaccarino, A. G., Nazeri, S., Riccio, R., Russo, G., Tarantino, S., & Zollo, A. (2021). Insights into Mechanical Properties of the 1980 Irpinia Fault System from the Analysis of a Seismic Sequence. Geosciences, 11(1), 28. https://doi.org/10.3390/geosciences11010028