Non-Associated Flow Rule-Based Elasto-Viscoplastic Model for Clay
Abstract
1. Introduction
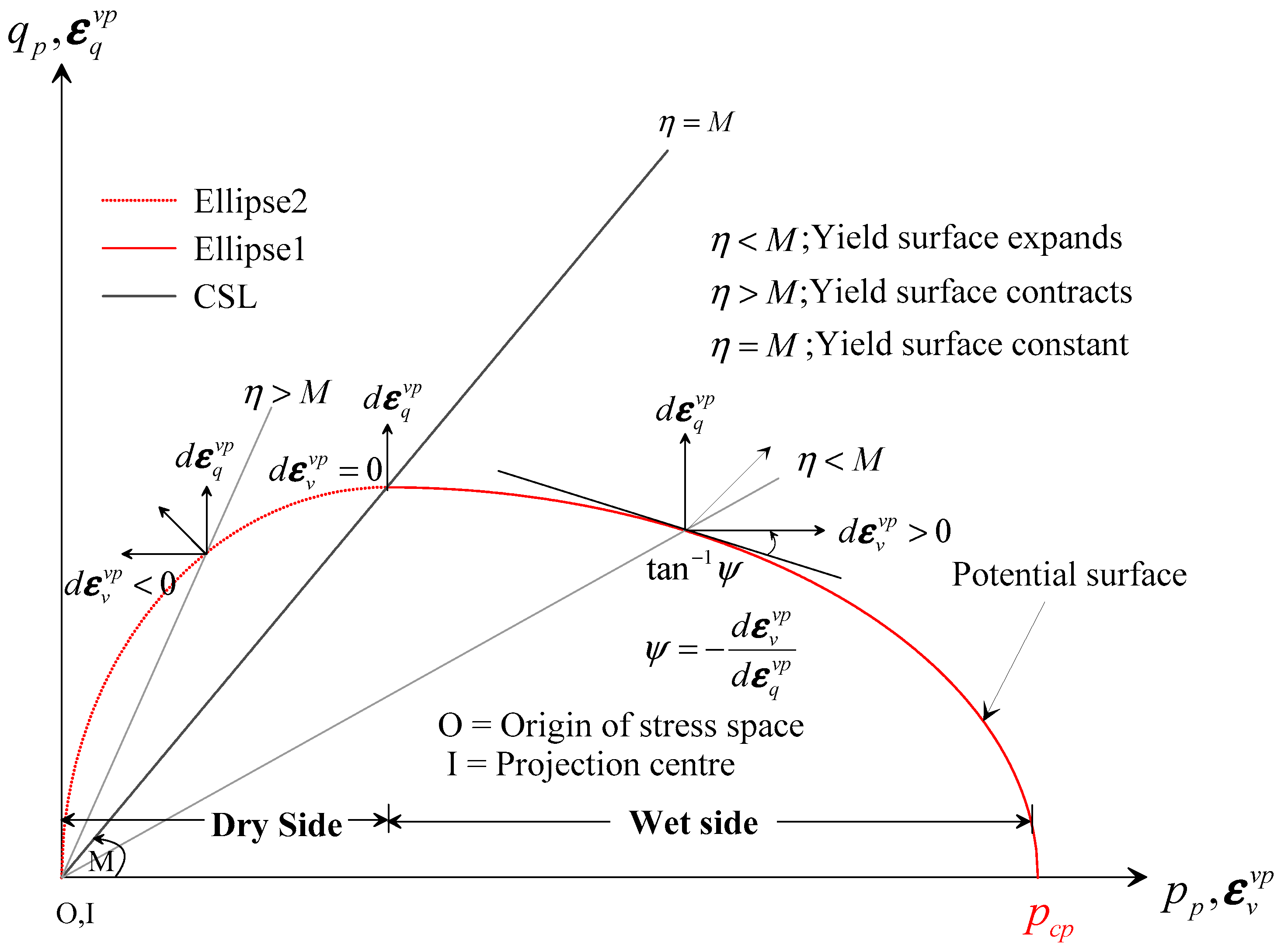
2. Importance of the Non-Associated Flow Rule
3. Numerical Modeling
3.1. Governing Equations
3.2. Constitutive Assumptions
3.2.1. Strain Rate Tensor of the EVP Model
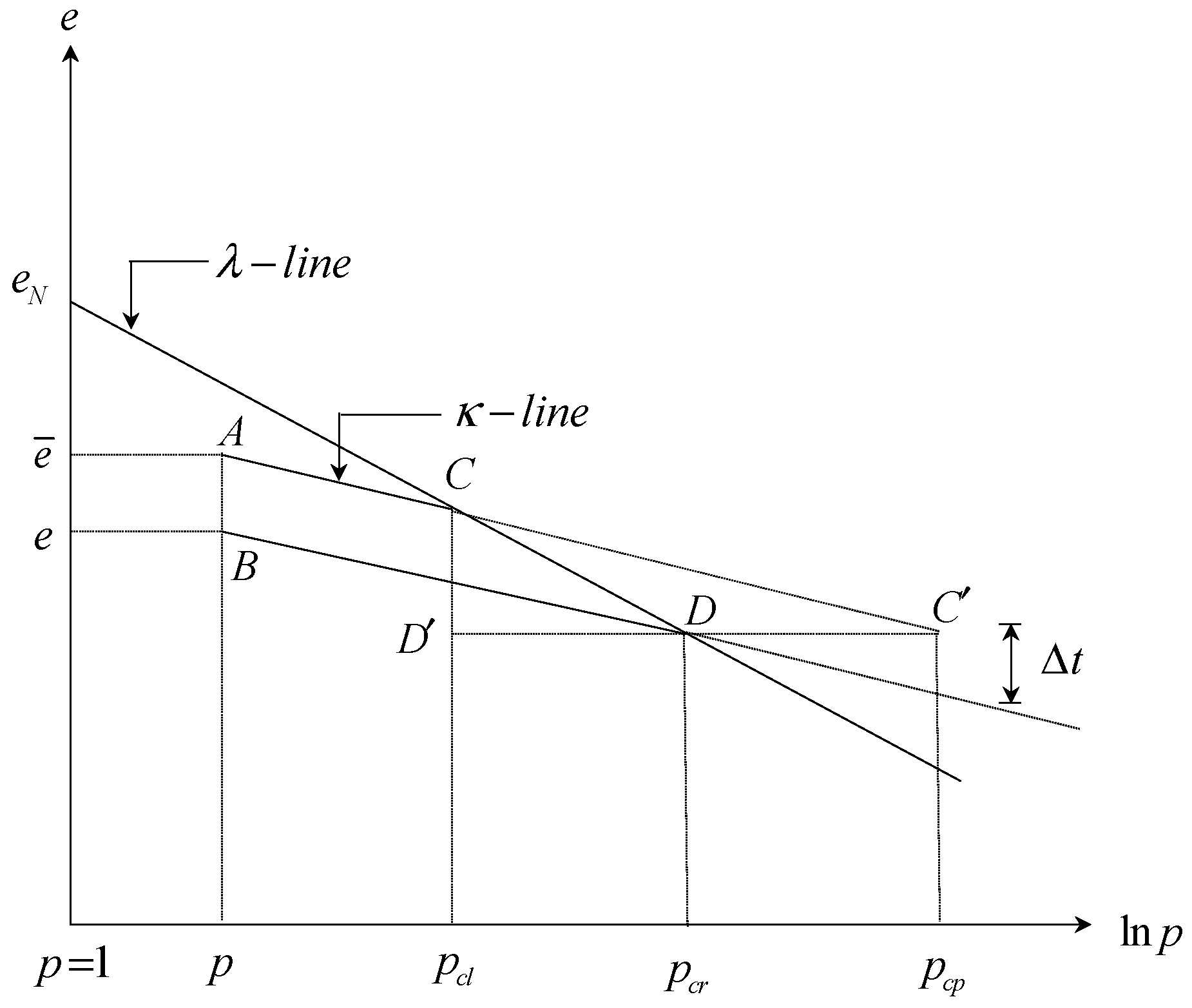
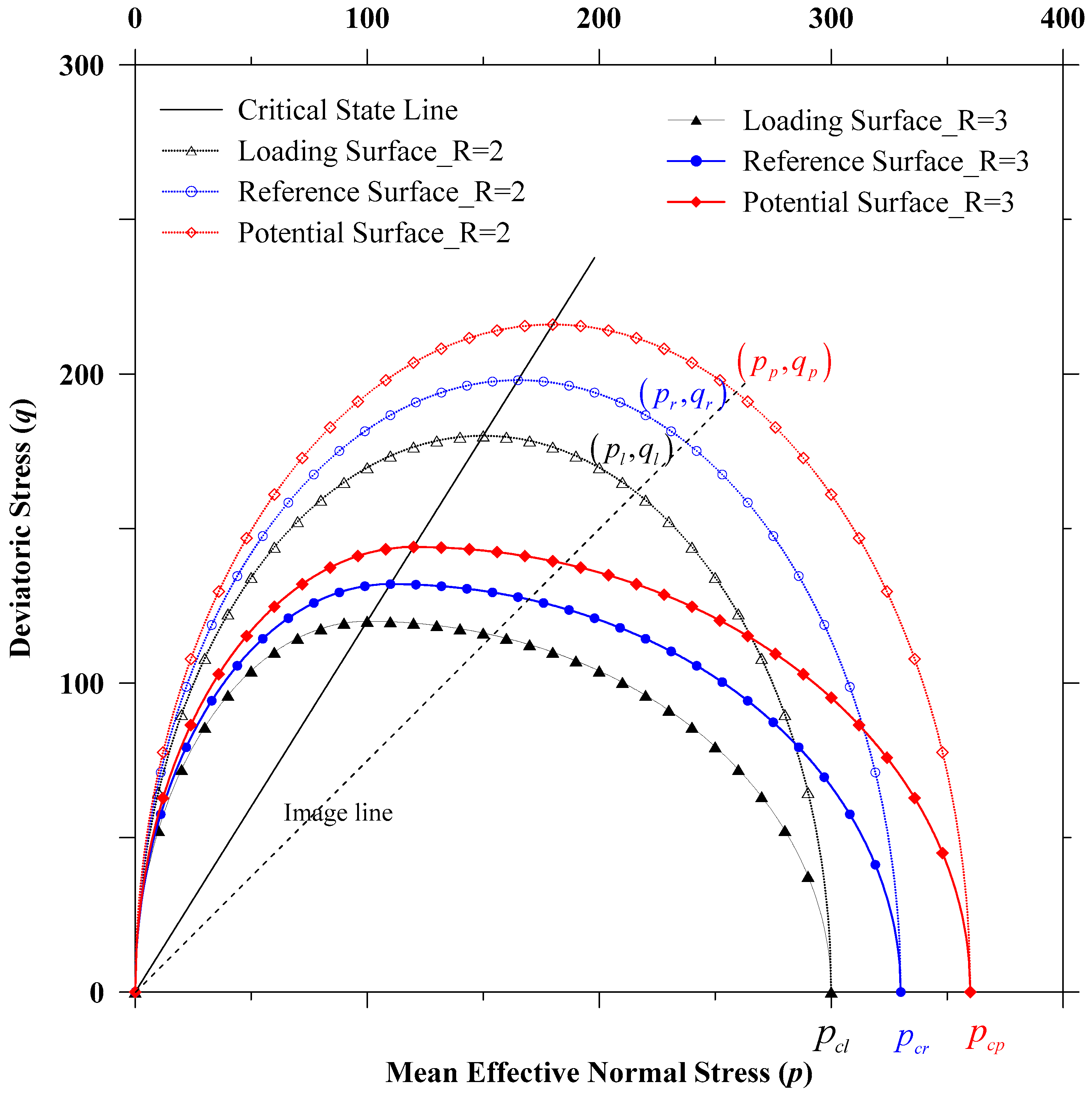
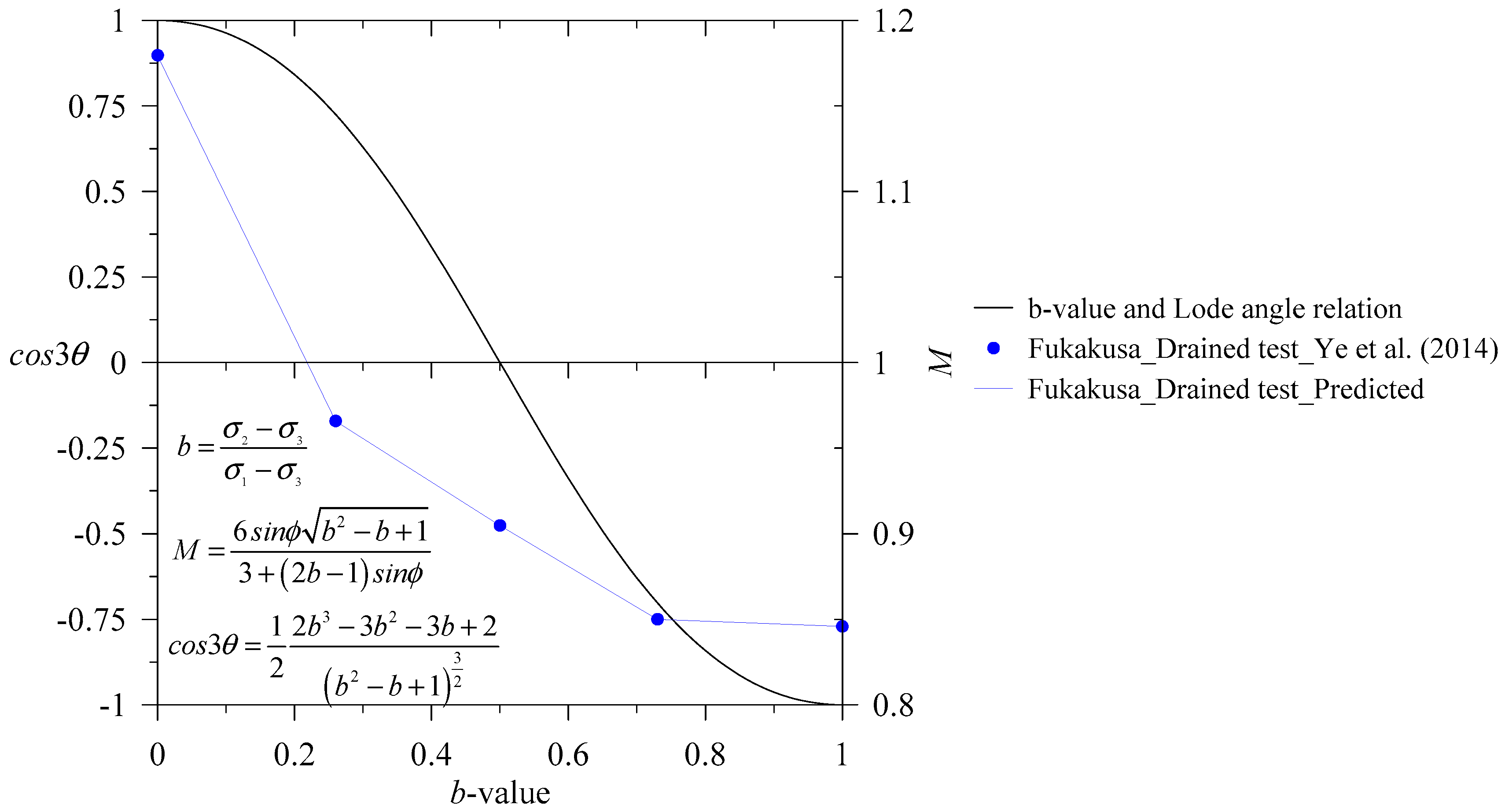
3.2.2. Bounding Surfaces of the EVP Model
3.2.3. Image Parameters of the EVP Model
3.3. Finite Element Implementation
3.3.1. Couple Finite Element Formulation
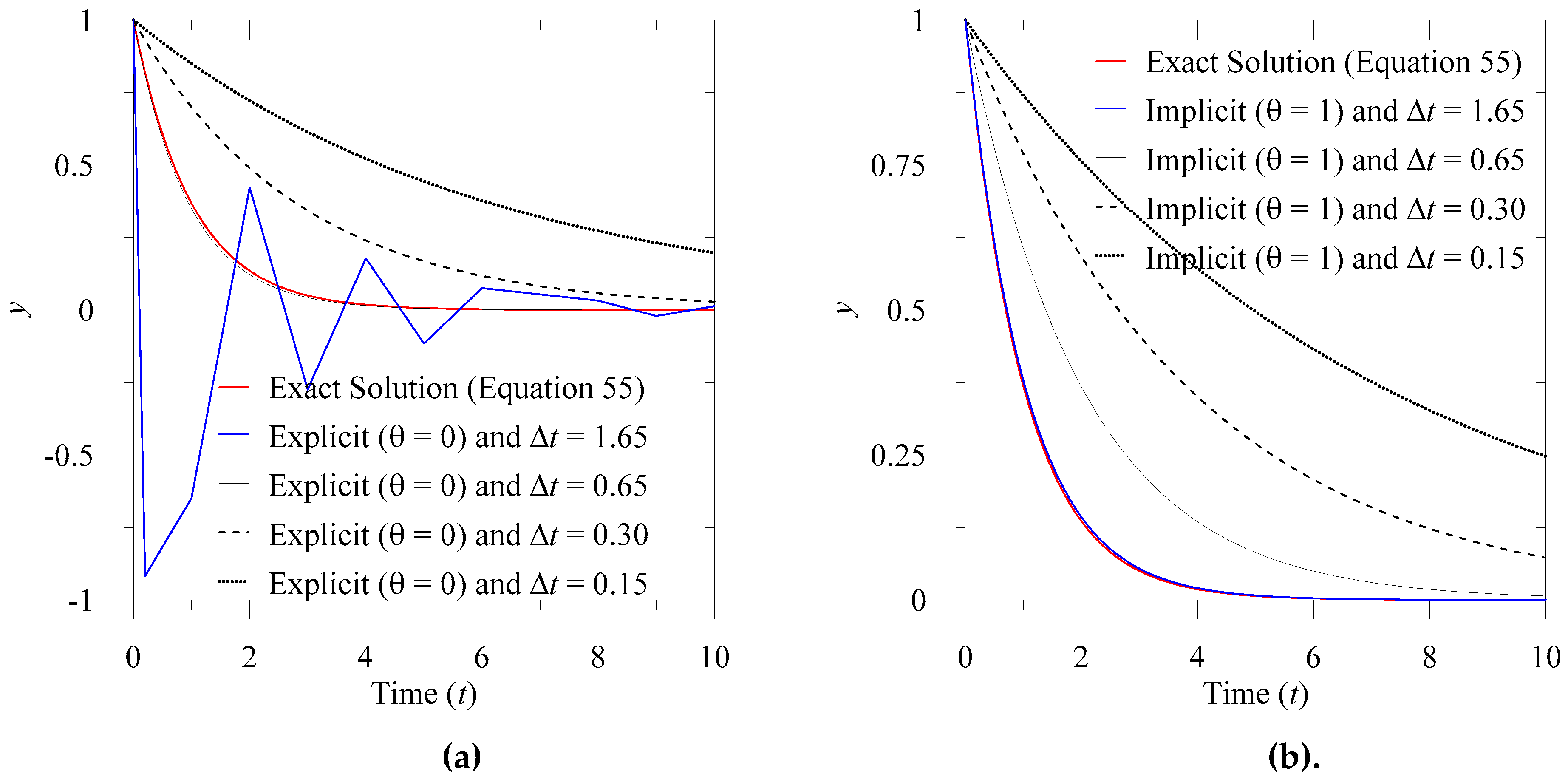
3.3.2. Time Integration
3.3.3. Incremental Stress and Strain
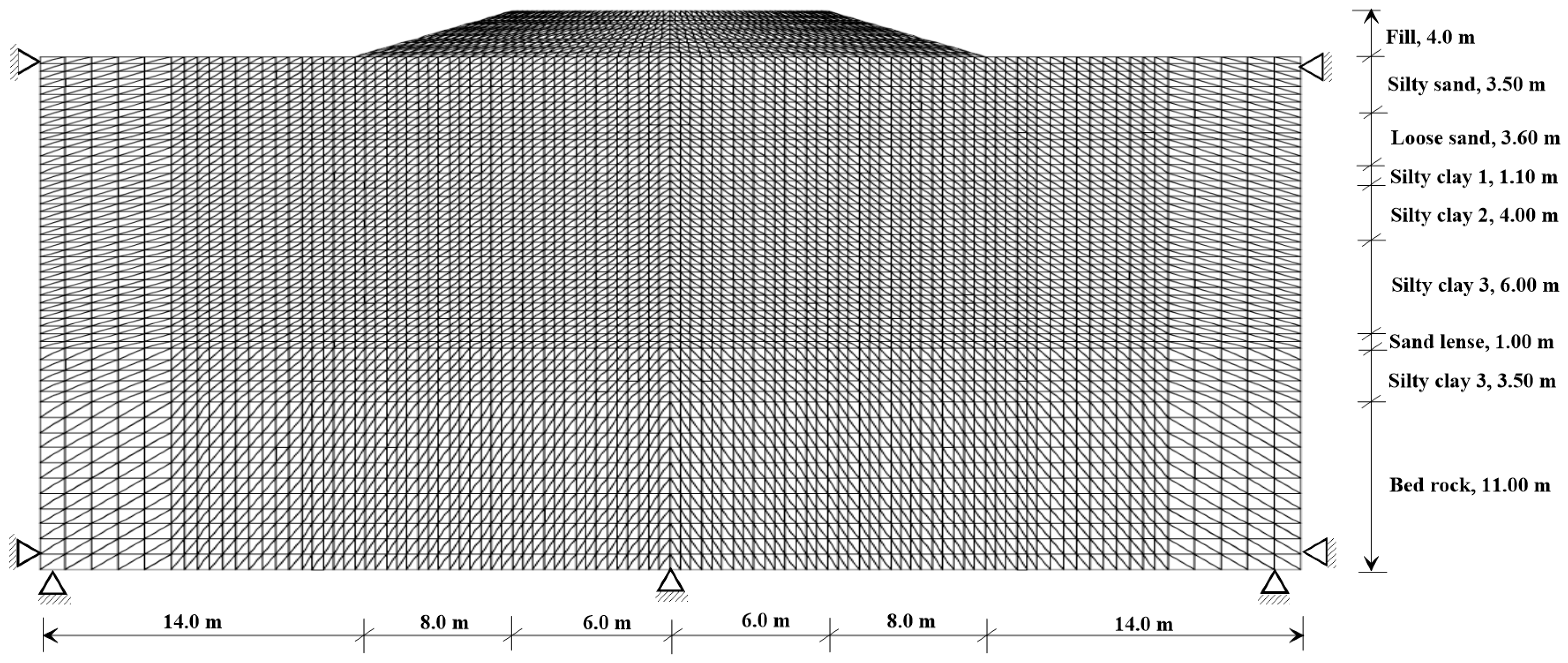
3.4. Initial and Boundary Conditions
3.5. Model Parameters
4. Results and Discussion
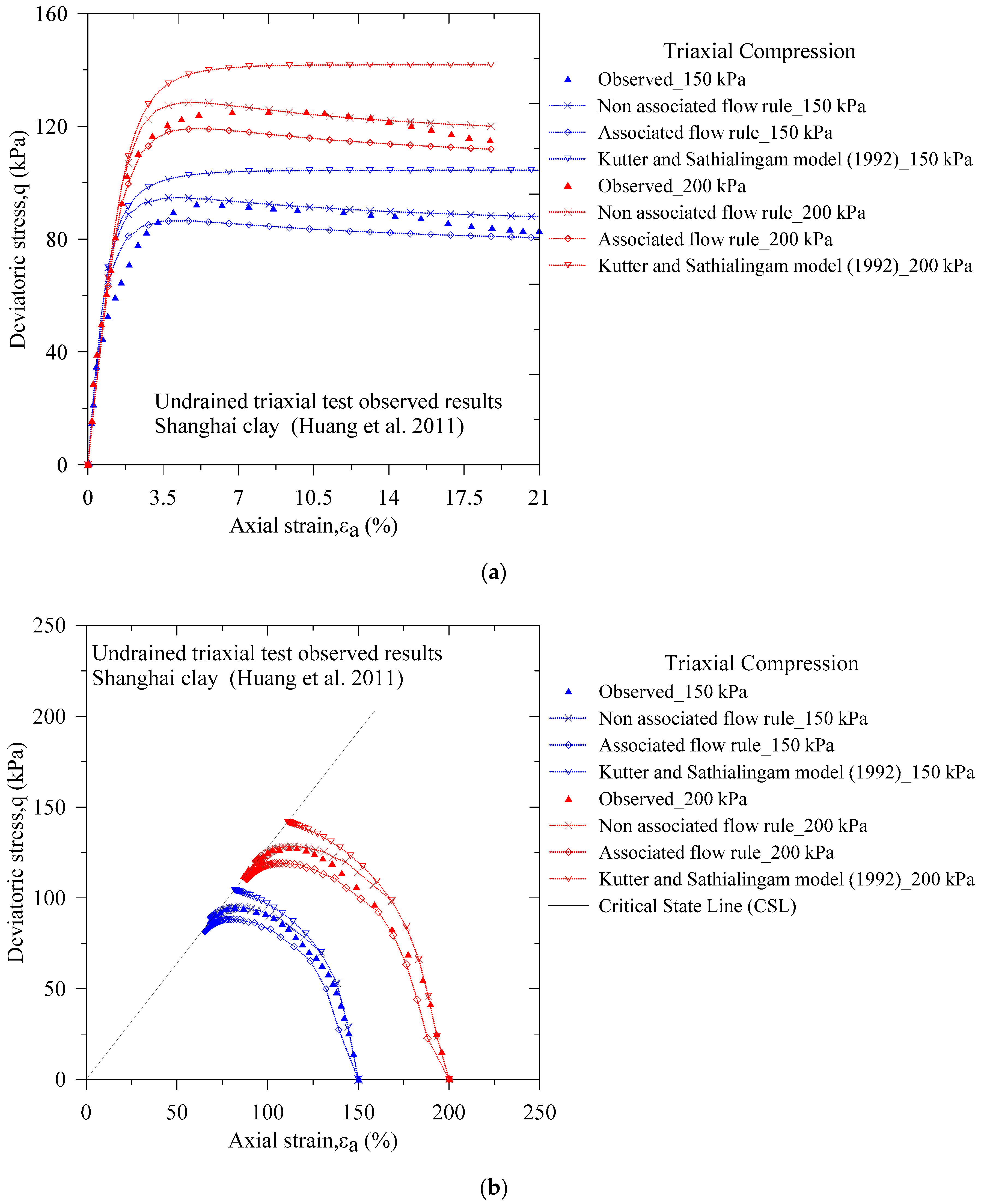
4.1. Shanghai Clay
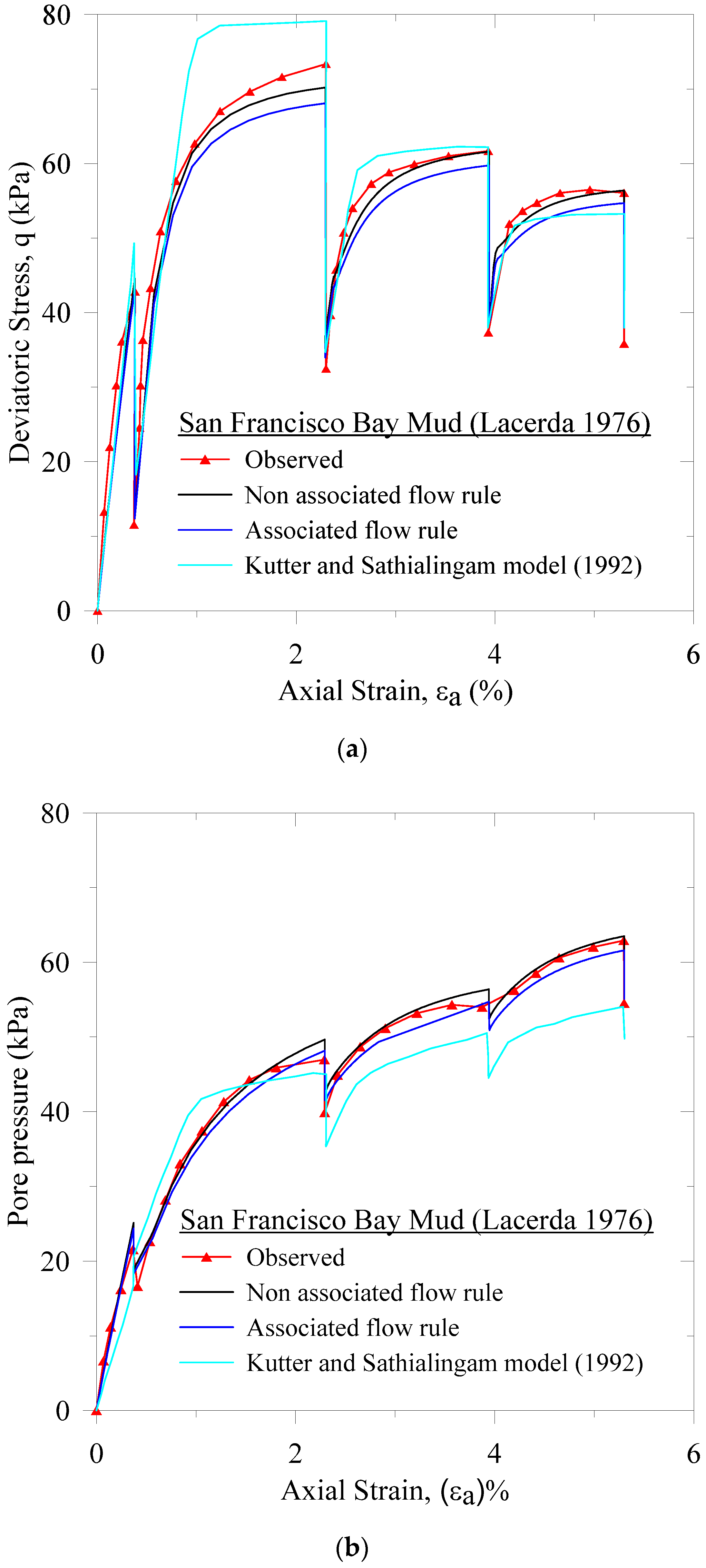
4.2. San Francisco Bay Mud Clay
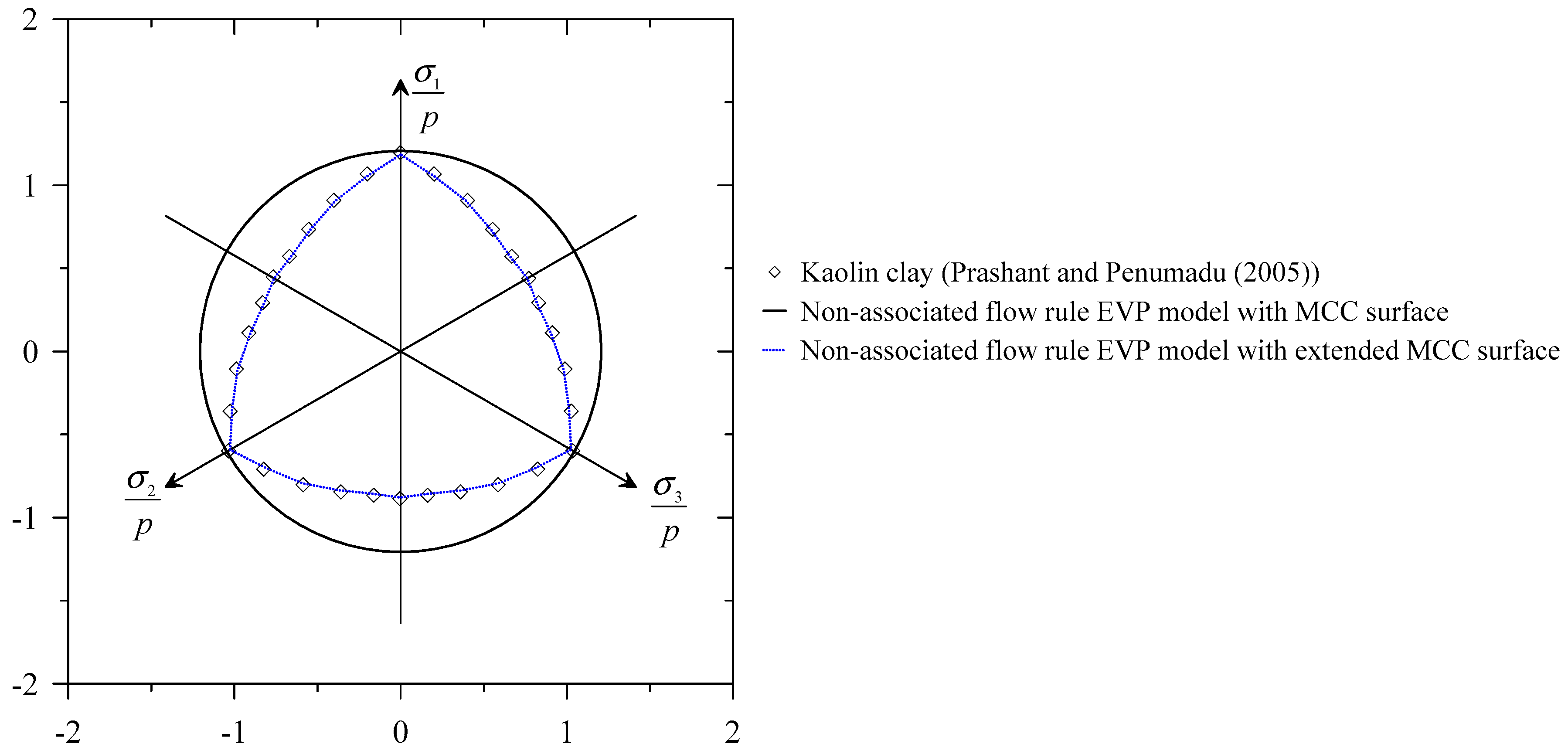
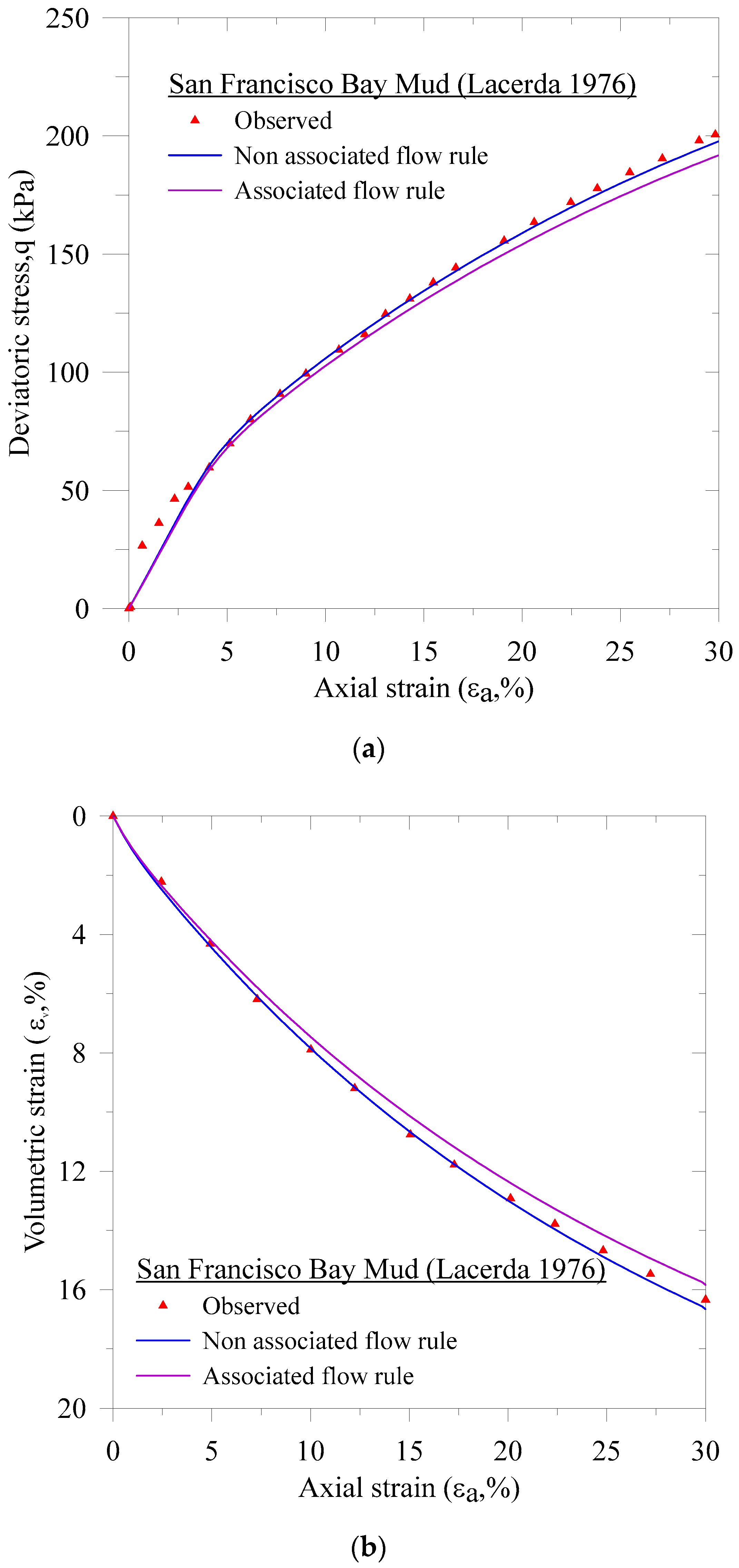
4.3. Kaolin Clay
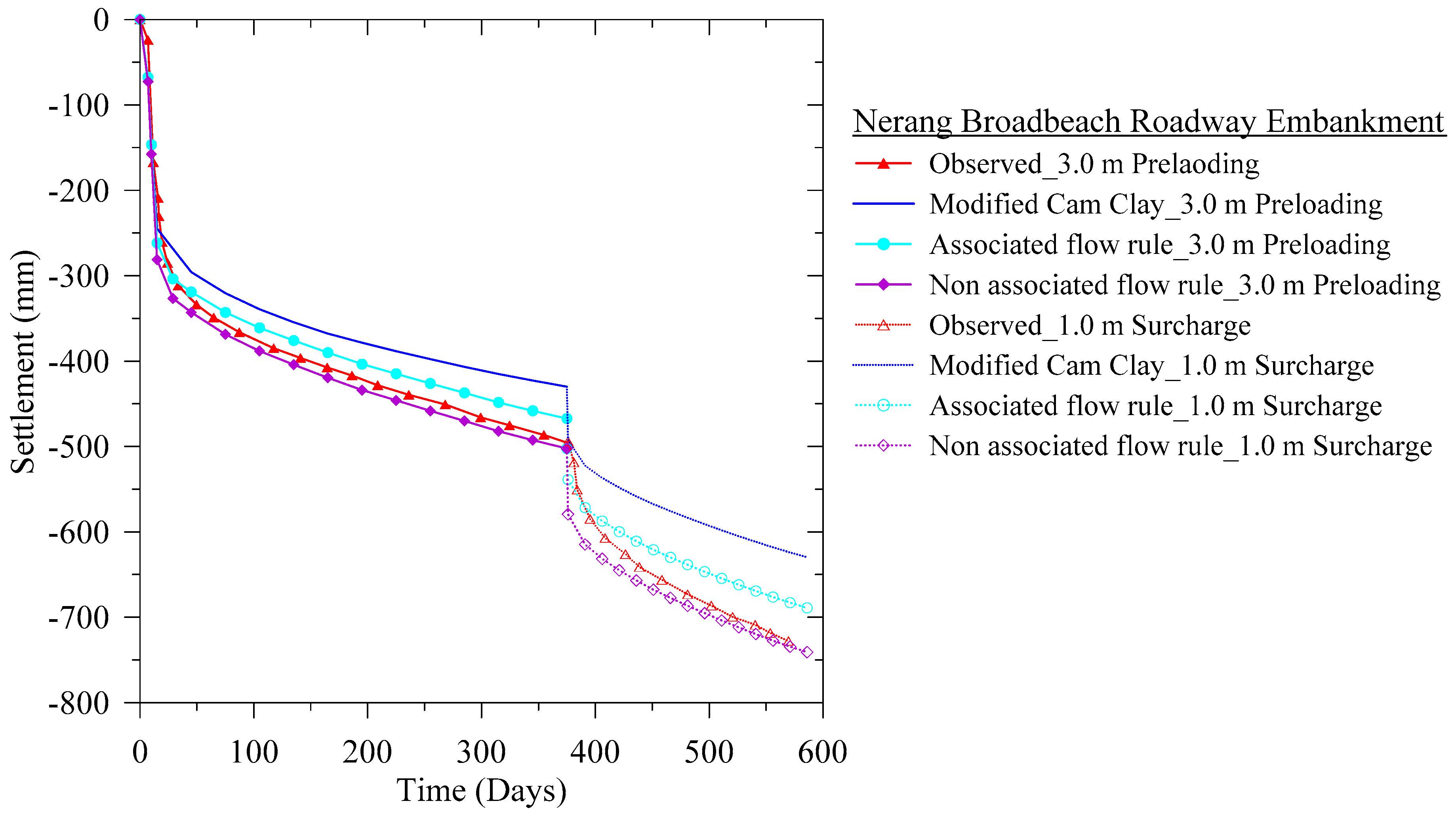
5. Application of the EVP Models
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Derivation of Φ
Appendix B. Derivation of
References
- Terzaghi, K. Theoretical Soil Mechanics; Wiley: New York, NY, USA, 1943. [Google Scholar]
- Brand, E.W.; Brenner, R.P. Soft Clay Engineering; Libra Press: Hong Kong, China, 1981. [Google Scholar]
- Wang, L.; Zhou, F. Analysis of elastic-viscoplastic creep model based on variable-order differential operator. Appl. Math. Model. 2020, 81, 37–49. [Google Scholar] [CrossRef]
- Burland, J.B.; Jamiolkowski, M.; Squeglia, N.; Viggiani, C. The leaning tower of pisa. In Geotechnics and Heritage; Bilotta, E., Elora, A., Lirer, S., Viggiani, C., Eds.; Taylor and Francis: London, UK, 2013; pp. 207–227. [Google Scholar]
- Thyagaraj, T. Ground Improvement Techniques and Geosynthetics; Springer: Berlin, Germany, 2019. [Google Scholar]
- Islam, M.N.; Bunger, A.P.; Huerta, N.; Dilmore, R. Bentonite extrusion into near-borehole fracture. Geosciences 2019, 9, 495. [Google Scholar] [CrossRef]
- Stephansson, O.; Hudson, J.A.; Jing, L. Coupled Thermo-Hydro-Mechanical-Chemical Processes in Geo-Systems: Fundamental Modelling, Experiments and Applications; Elsevier: New York, NY, USA, 2004. [Google Scholar]
- Oka, F.; Kimoto, S. Computational Modeling of Multiphase Geomaterials; Kimoto, S., Oka, F., Eds.; Limited Liability Company: New York, NY, USA, 2013. [Google Scholar]
- Adachi, T.; Okano, M. A constitutive equation for normally consolidated clay. Soils Found. 1974, 14, 55–73. [Google Scholar] [CrossRef]
- Roscoe, K.H.; Burland, J.B. On the generalized stress-strain behavior of wet clay. In Engineering Plasticity; Heyman, J., Leckie, F.A., Eds.; Cambridge University Press: Cambridge, UK, 1968; pp. 535–609. [Google Scholar]
- Liingaard, M.; Augustesen, A.; Lade, P. Characterization of Models for Time-Dependent Behavior of Soils. Int. J. Geomech. 2004, 4, 157–177. [Google Scholar] [CrossRef]
- Chaboche, J.L. A review of some plasticity and viscoplasticity constitutive theories. Int. J. Plast. 2008, 24, 1642–1693. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and non-associated visco-plasticity and plasticity in soil mechanics. Geotechnique 1975, 25, 671–689. [Google Scholar] [CrossRef]
- Islam, M.N.; Gnanendran, C.T.; Massoudi, M. Finite Element Simulations of an Elasto-Viscoplastic Model for Clay. Geosciences 2019, 9, 145. [Google Scholar] [CrossRef]
- Yu, H.S. Plasticity and Geotechnics; Springer: New York, NY, USA, 2006. [Google Scholar]
- Perzyna, P. Fundamental problems in viscoplasticity. Adv. Appli. Mech. 1966, 9, 244–377. [Google Scholar]
- Borja, R.I.; Kavazanjian, J.E. A constitutive model for the stress-strain & time behaviour of ‘wet’ clays. Geotechnique 1985, 35, 283–298. [Google Scholar]
- Hashiguchi, K. Elastoplasticity Theory, 2nd ed.; Springer: New York, NY, USA, 2014. [Google Scholar]
- Carter, J.P.; Balaam, N.P. AFENA User’s Manual, Version 5.0; Center for Geotechnical Research, University of Sydney: Sydney, Australia, 1995.
- Islam, M.N.; Gnanendran, C.T.; Sivakumar, S.T. Prediction of embankments time-dependent behavior on soft soils: Effects of preloading, surcharging, and choice of lab versus field test data for soft soil parameters. In Ground Improvement Case Histories; Indraratna, B., Chu, J., Cholachat, R., Eds.; Butterworth-Heinemann: Oxford, UK, 2015; pp. 359–379. [Google Scholar]
- Schofield, A.N.; Wroth, P. Critical State Soil Mechanics; McGrawHill: London, UK, 1968. [Google Scholar]
- McDowell, G.R.; Hau, K.W. A Simple Non-associated three surface kinematic hardening model. Geotechnique 2003, 53, 433–437. [Google Scholar] [CrossRef]
- Islam, M.N.; Gnanendran, C.T. Elastic-viscoplastic model for clays: Development, validation, and application. J. Eng. Mech. 2017, 143. [Google Scholar] [CrossRef]
- Bear, J.; Bachmat, Y. Introduction to Modeling of Transport Phenomena in Porous Media; Kluwer Academic Publishers: London, UK, 1990; Volume 4. [Google Scholar]
- Lubliner, J. Plasticity Theory; Dover Publications: New York, NY, USA, 1990. [Google Scholar]
- Kaliakin, V.N.; Dafalias, Y.F. Verification of the elastoplastic-viscopalstic bounding surface model for cohesive soils. Soils Found. 1990, 30, 25–36. [Google Scholar] [CrossRef]
- Kutter, B.L.; Sathialingam, N. Elastic-viscoplastic modelling of the rate-dependent behaviour of clays. Géotechnique 1992, 42, 427–441. [Google Scholar] [CrossRef]
- Prashant, A.; Penumadu, D. A laboratory study of normally consolidated kaolin clay. Can. Geotech. J. 2005, 42, 27–37. [Google Scholar] [CrossRef]
- Lade, P.V. Triaxial Testing of Soils; John Wiley & Sons: Hoboken, NJ, USA, 2016; p. 88. [Google Scholar]
- Ye, G.L.; Ye, B.; Zhang, F. Strength and dilatancy of overconsolidated clays in drained true triaxial tests. J. Geotech. Geoenviron. Eng. 2014, 140, 06013006. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.L.; Zhu, J.Z. The Finite Element Method: Its Basis and Fundamentals, 6th ed.; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Owen, D.R.J.; Hinton, E. Finite Elements in Plasticity: Theory and Practice; Pineridge Press Limited: Swansea, UK, 1980. [Google Scholar]
- Segerlind, L.J. Applied Finite Element Analysis, 2nd ed.; John Wiley and Sons: Hoboken, NJ, USA, 1984. [Google Scholar]
- Potts, D.M.; Zdravkovic, L. Finite Element Analysis in Geotechnical Engineering: Theory; Thomas Telford Publishing: London, UK, 1999. [Google Scholar]
- Mesri, G.; Castro, A. Cα/Cc Concept and K0 during secondary compression. J. Geo. Eng. 1987, 113, 230–247. [Google Scholar] [CrossRef]
- Tonni, L.; Simonini, P. Evaluation of secondary compression of sands and silts from CPTU. Geomech. Geoeng. 2012, 8, 141–154. [Google Scholar] [CrossRef]
- Dafalias, Y.F.; Herrmann, L.R. Bounding surface plasticity. II: Application to isotropic cohesive Soils. J. Eng. Mech. 1986, 112, 1263–1291. [Google Scholar] [CrossRef]
- Huang, M.; Liu, Y.; Sheng, D. Simulation of yielding and stress-stain behavior of Shanghai soft clay. Comput. Geotech. 2011, 38, 341–353. [Google Scholar] [CrossRef]
- Lacerda, W.A. Stress-Relaxation and Creep Effects on Soil Deformation. Ph.D. Thesis, University of California, Berkeley, CA, USA, December 1976. [Google Scholar]
- Herrmann, L.R.; Shen, C.K.; Jafroudi, S.; DeNatale, J.S.; Dafalias, Y.F. A Verification Study for the Bounding Surface Plasticity Model for Cohesive Soils; Final report to the Civil Engineering Laboratory; Naval Construction Battalion Center: Port Hueneme, CA, USA, March 1982. [Google Scholar]
- Whittle, A.; Kavvadas, M. Formulation of MIT-E3 Constitutive Model for Overconsolidated Clays. J. Geotech. Eng. 1994, 120, 173–198. [Google Scholar] [CrossRef]
- Liu, M.D.; Carter, J.P. A structured Cam Clay model. Can. Geotech. J. 2002, 39, 1313–1332. [Google Scholar] [CrossRef]

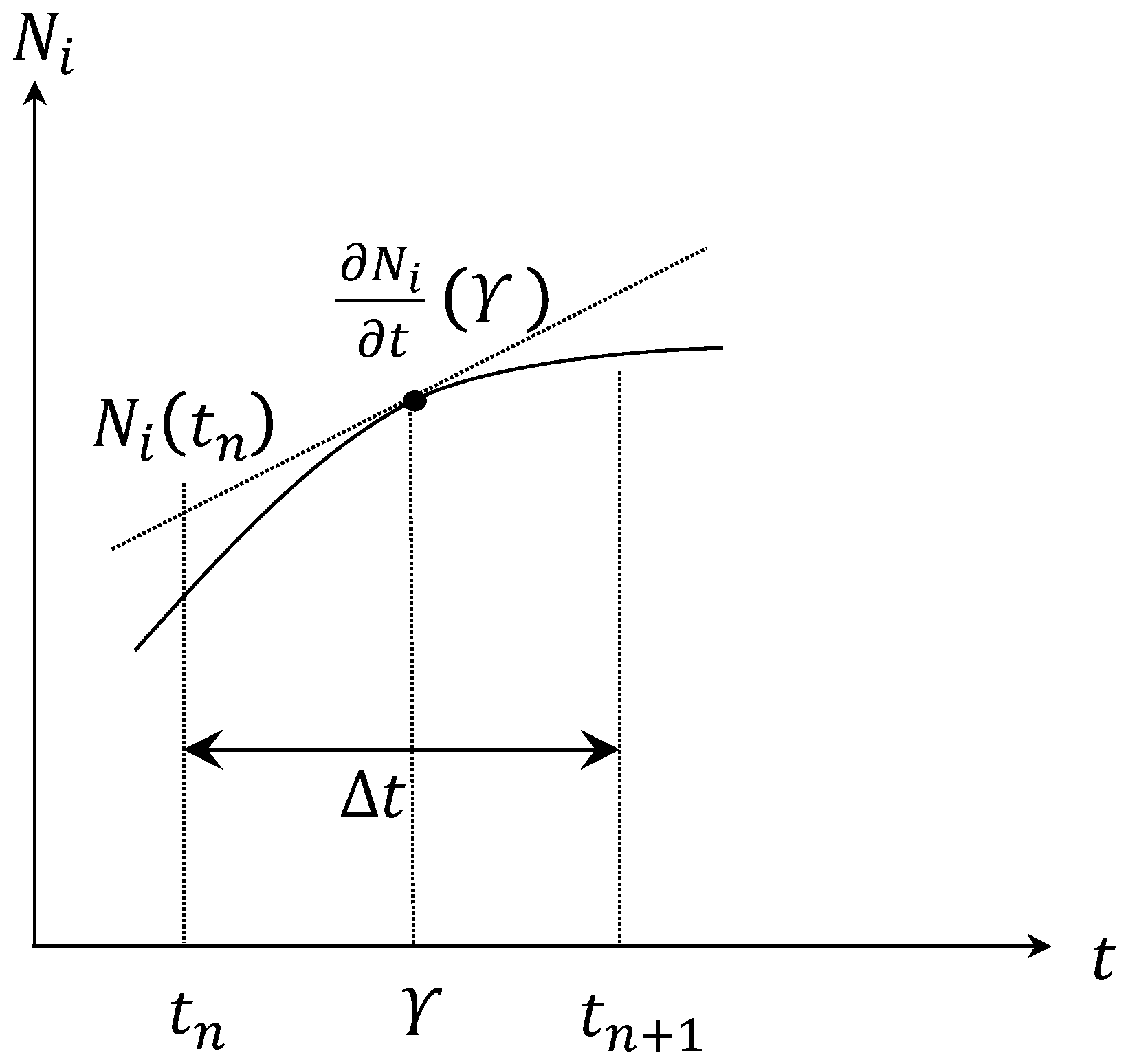



| Parameters | Meaning | Method of Determination |
|---|---|---|
| Slope of NCL | Triaxial or oedometer test | |
| Slope of SL | Triaxial or oedometer test | |
| Slope of CSL | Triaxial test | |
| Poisson’s ratio | Assumed | |
| Void ratio at p = 1 with NCL at | Triaxial or oedometer test | |
| Creep parameter | Triaxial or oedometer test | |
| Shape parameter | Undrained triaxial test |
| Clay | EVP Model Parameters in This Paper | |||||||
|---|---|---|---|---|---|---|---|---|
| Shanghai clay [38] | 0.22 | 0.046 | 1.28 | --- | 0.30 | 2.23 | 0.016 | 2.00 |
| SFBM clay [39] | 0.37 | 0.054 | 1.40 | --- | G | 3.17 | 0.053 | 2.10 |
| Kaolin clay [40] | 0.15 | 0.018 | 1.25 | 0.95 | 0.30 | 1.51 | 0.014 | 2.50 |
| Phase | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| Test | Shear | *Relax. | Shear | *Relax. | Shear | *Relax. | Shear | *Relax. |
| 1.5 | 0 | 1.5 | 0 | 0.0162 | 0 | 0.00081 | 0 | |
| 0–0.38 | 0.38 | 0.38–2.3 | 2.3 | 2.3–3.94 | 3.94 | 3.94–5.3 | 5.3 | |
| Time | 0.25 | 3070 | 1.28 | 1320 | 101.24 | 2700 | 1679 | 8370 |
| Soil Layer | |||||||
|---|---|---|---|---|---|---|---|
| Fill | 3000 kPa, 300,5.0 kPa | --- | |||||
| Silty sand | 5000 kPa, 350,2.5 kPa | --- | |||||
| Loose sand | 7000 kPa, 330,1.5 kPa | --- | |||||
| Silty clay 1 | 1.28 | 0.36 | 0.060 | 2.10 | 159.52 | 0.029 | 2.10 |
| Silty clay 2 | 1.25 | 0.42 | 0.043 | 3.73 | 105.36 | 0.033 | 2.10 |
| Silty clay 3 | 1.20 | 0.29 | 0.030 | 2.61 | 132.20 | 0.023 | 2.10 |
| Sand lense | 3000 kPa, 350,5.0 kPa | --- | |||||
| Silty clay 3 | 1.20 | 0.29 | 0.030 | 2.61 | 287.18 | 0.023 | 2.10 |
| Bedrock | ,000 kPa, 360,50.0 kPa | --- | |||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, M.; Gnanendran, C. Non-Associated Flow Rule-Based Elasto-Viscoplastic Model for Clay. Geosciences 2020, 10, 227. https://doi.org/10.3390/geosciences10060227
Islam M, Gnanendran C. Non-Associated Flow Rule-Based Elasto-Viscoplastic Model for Clay. Geosciences. 2020; 10(6):227. https://doi.org/10.3390/geosciences10060227
Chicago/Turabian StyleIslam, Mohammad, and Carthigesu Gnanendran. 2020. "Non-Associated Flow Rule-Based Elasto-Viscoplastic Model for Clay" Geosciences 10, no. 6: 227. https://doi.org/10.3390/geosciences10060227
APA StyleIslam, M., & Gnanendran, C. (2020). Non-Associated Flow Rule-Based Elasto-Viscoplastic Model for Clay. Geosciences, 10(6), 227. https://doi.org/10.3390/geosciences10060227





