Some Remarks on the Use of Deterministic and Probabilistic Approaches in the Evaluation of Rock Slope Stability
Abstract
1. Introduction
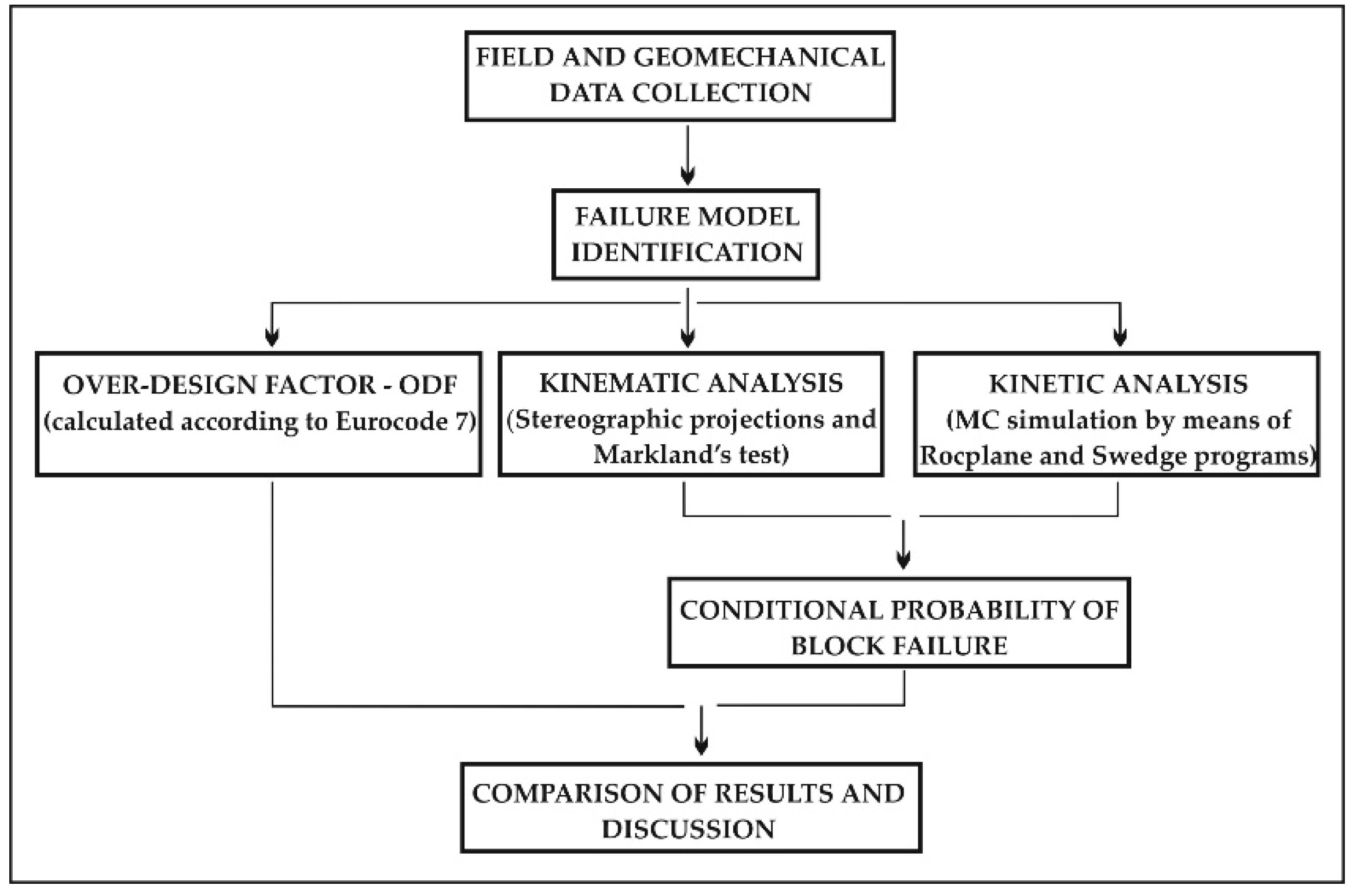
2. Adopted Methods
2.1. Over-Design Factor (ODF) Calculation
2.2. Kinematic Analysis
2.3. Kinetic Analysis by Means of Monte Carlo Simulation
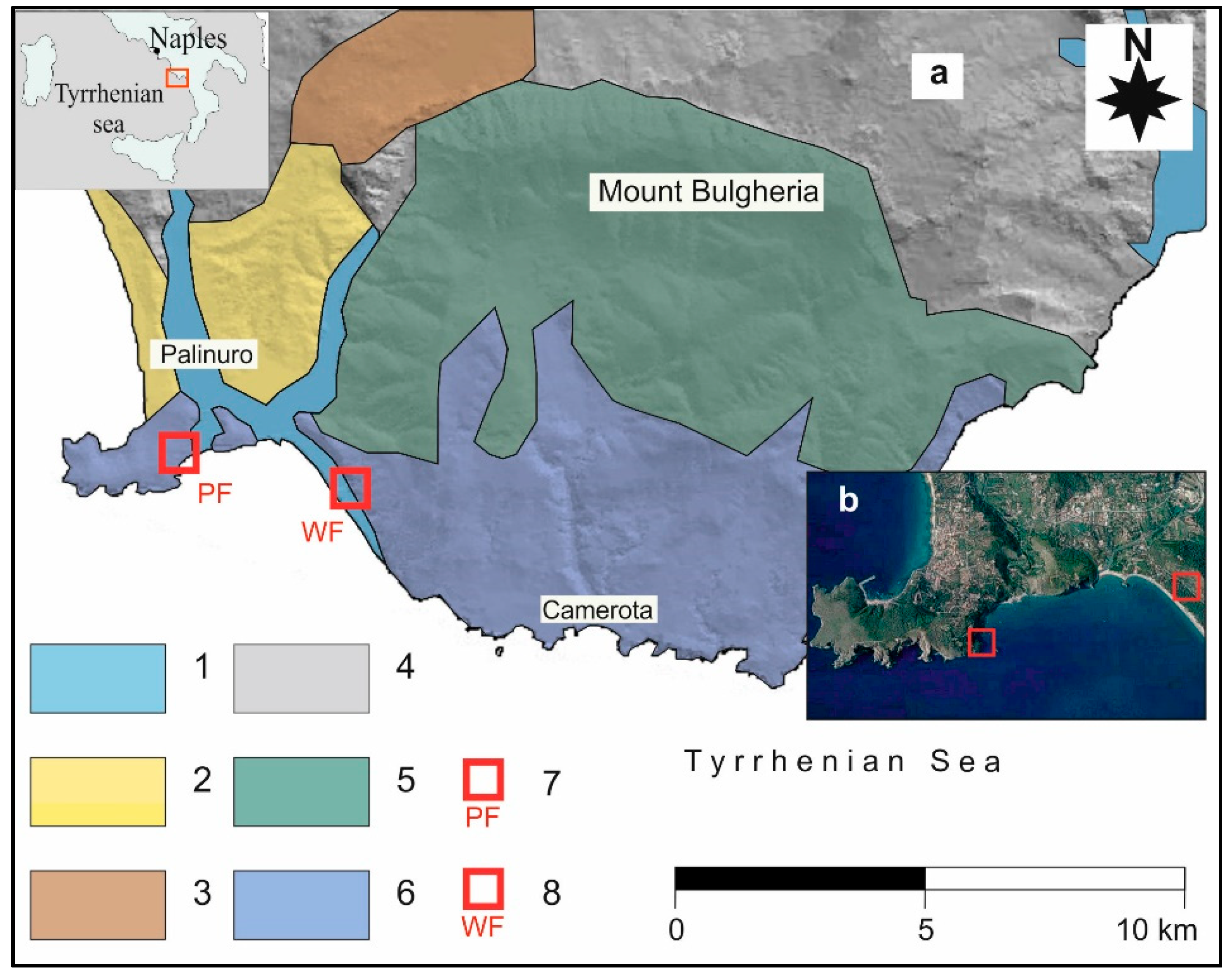
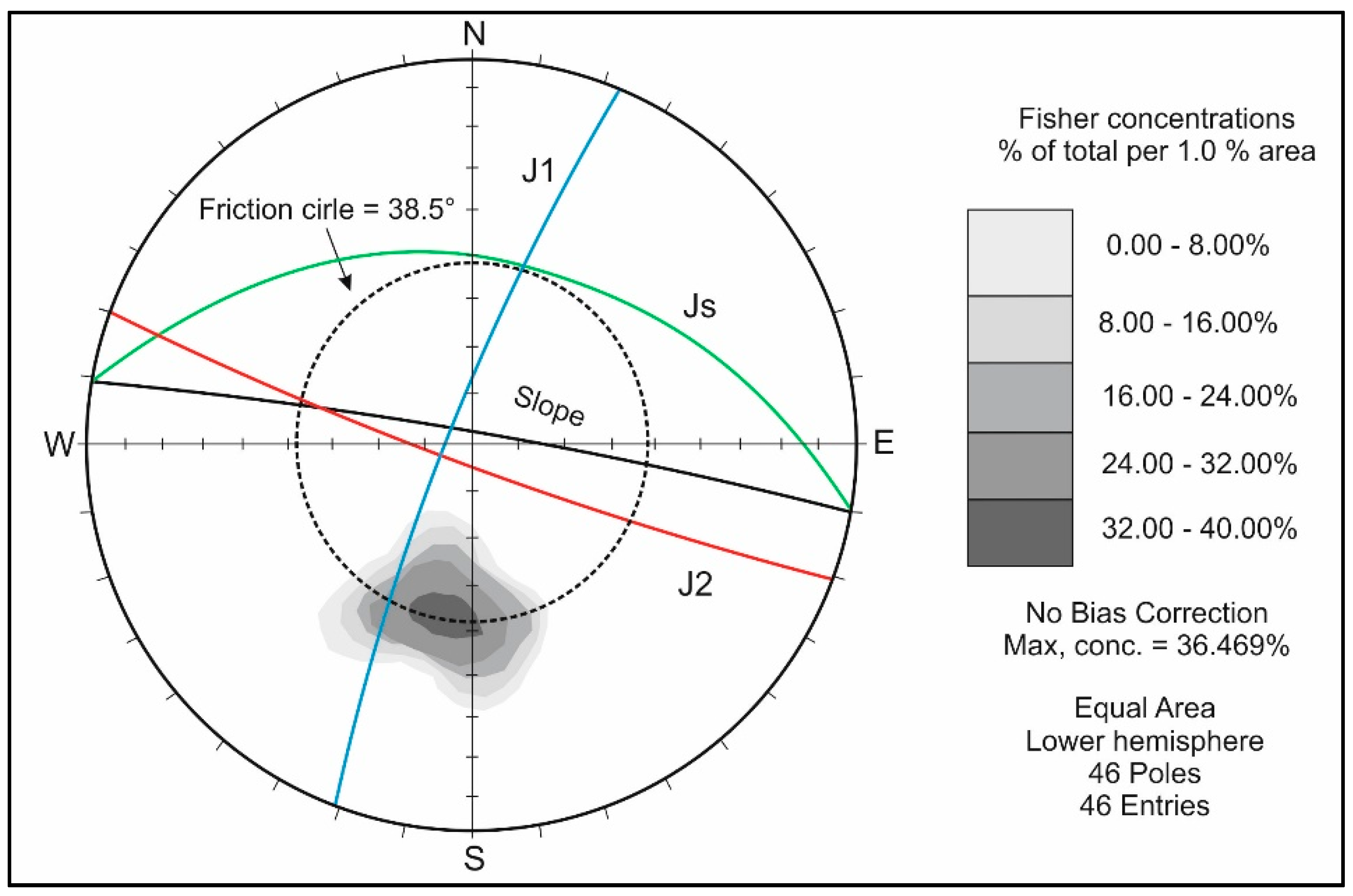
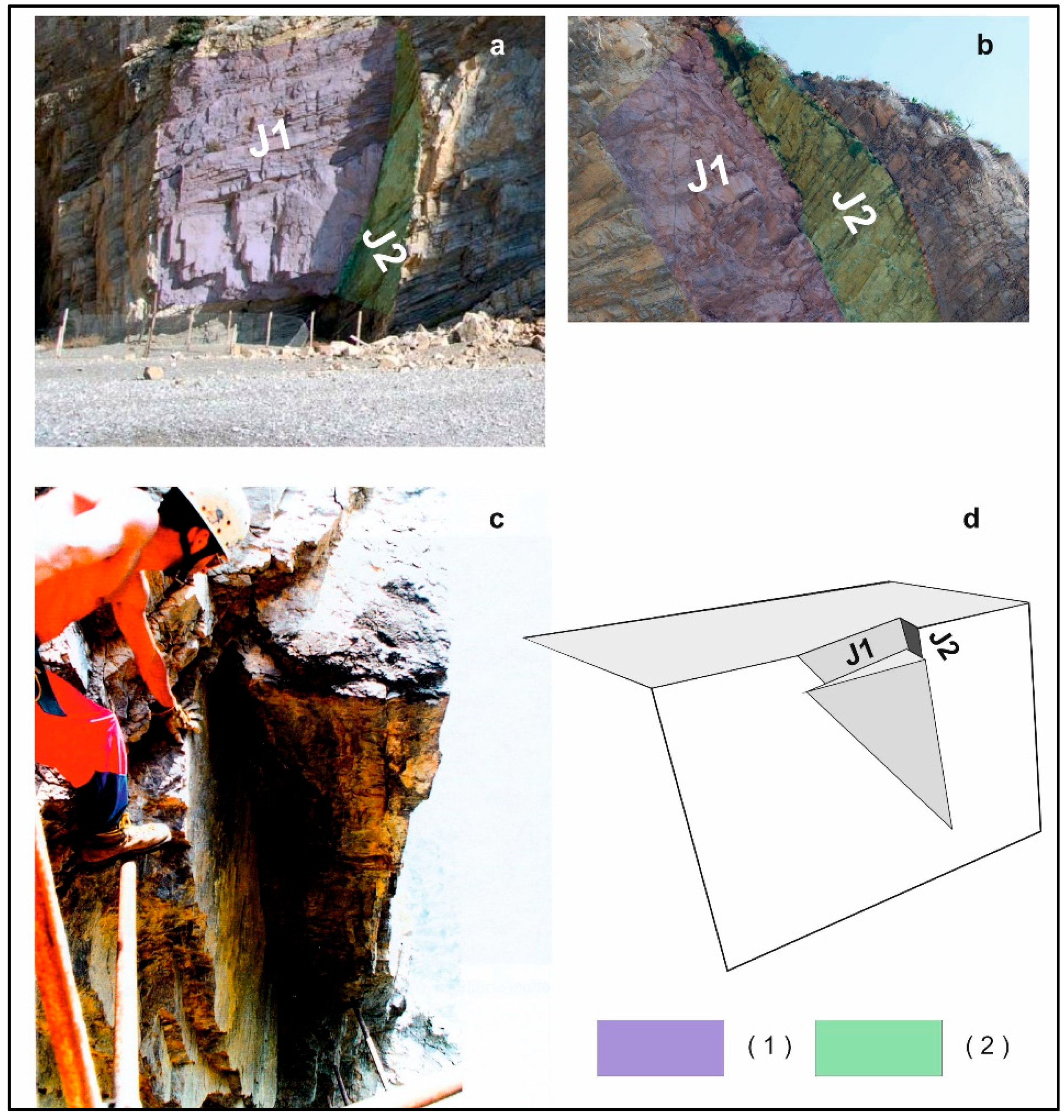
3. Geological Setting
4. Results
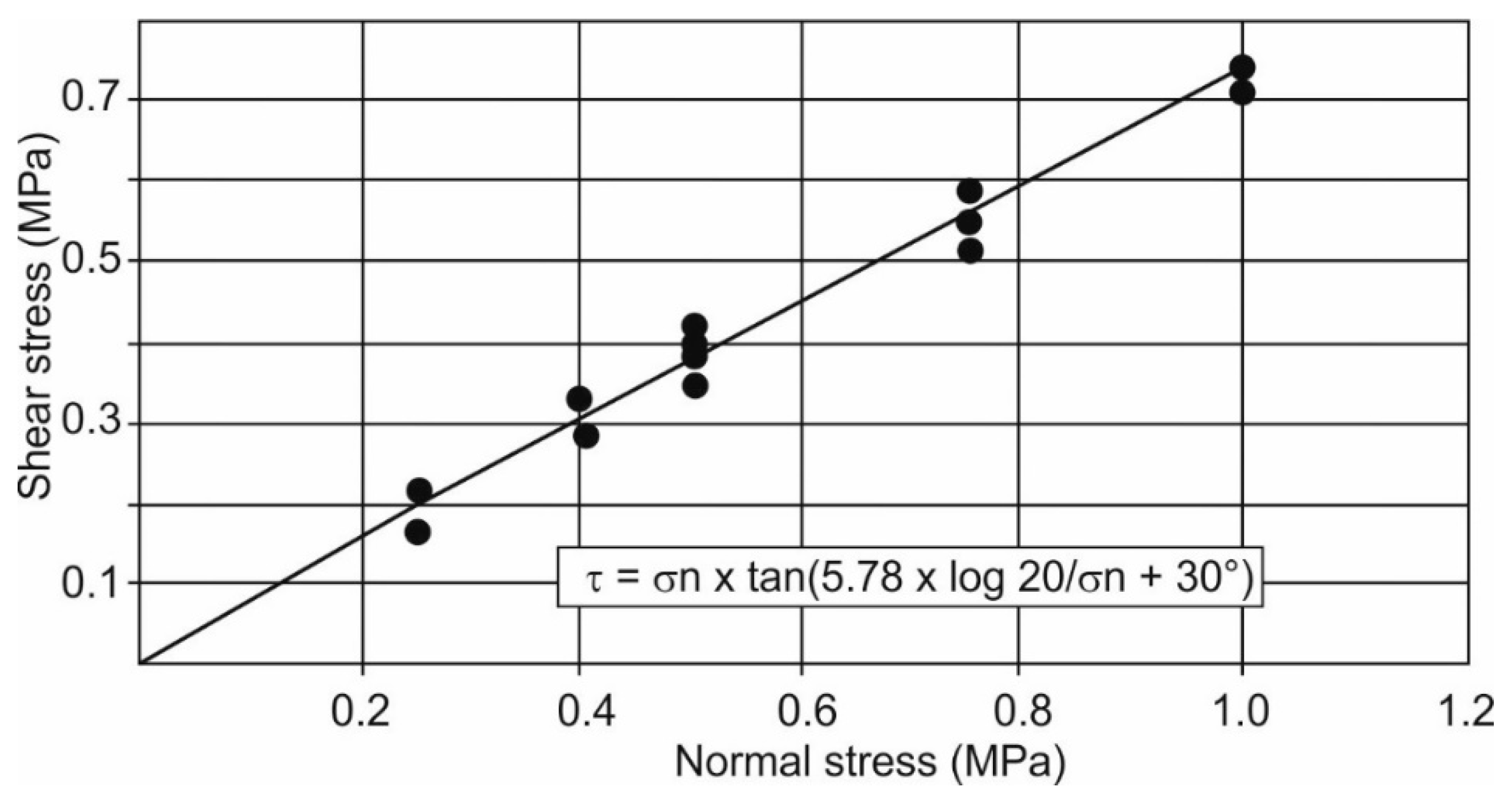
4.1. Geo-mechanical Characterization
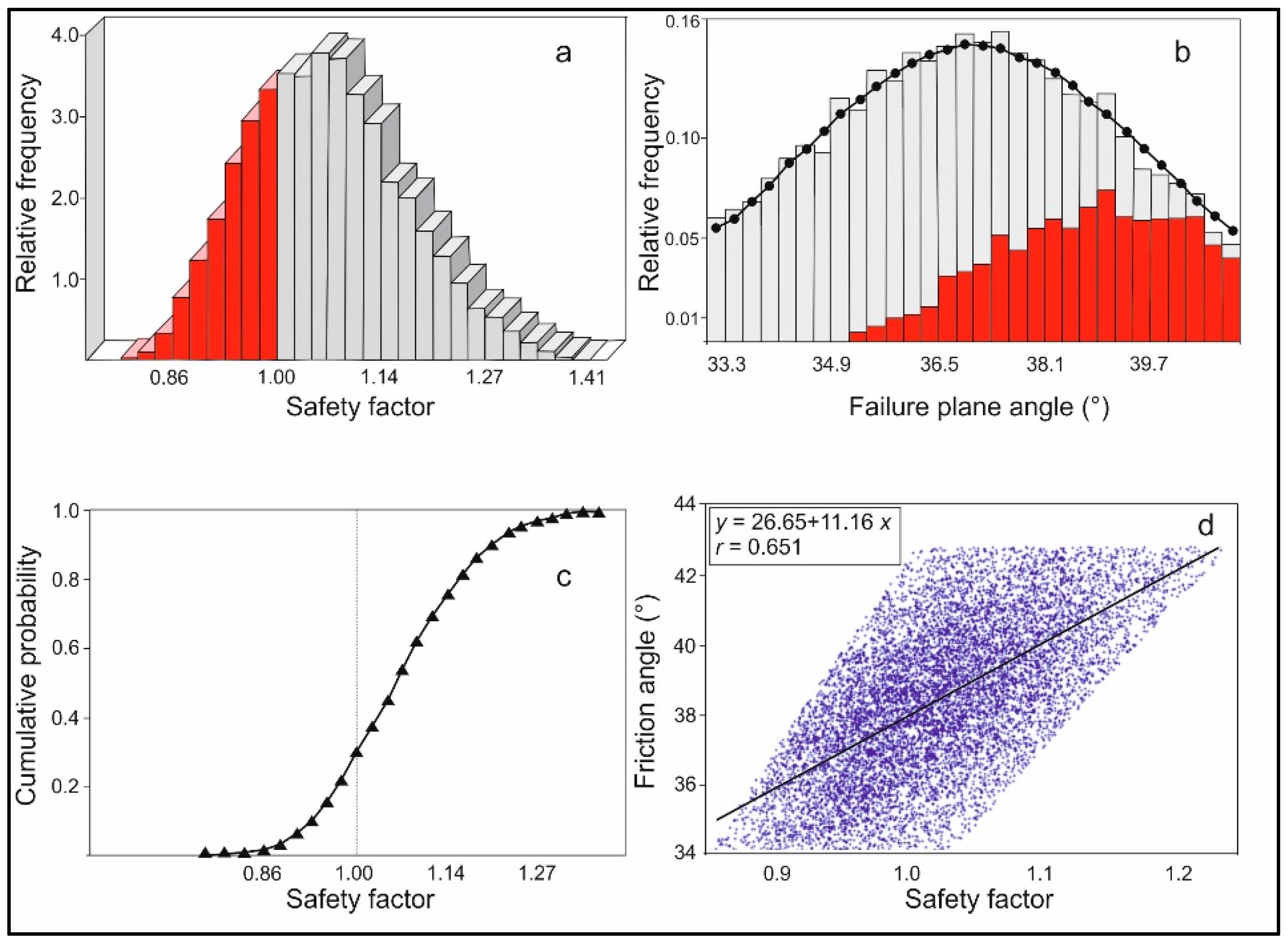
4.2. Plane Failure
- –
- Joint apertures range from “partly open” to “moderately wide” (0.25–6 mm), but also, apertures up to 10 mm are present. Moreover, some joints show hard calcite veins infilling;
- –
- The roughness, measured by means of the Barton comb [28], results “smooth undulating” for bedding planes (Joint Roughness Coefficient (JRC) values 5–10), whereas it is “smooth nearly planar” (JRC values 6–8) for joints belonging to the other sets;
- –
- The main joint terminations halt against other discontinuities in the exposure (“J/J type” terminations);
- –
- The joint surfaces are “moderately” weathered, with some rock bridges.
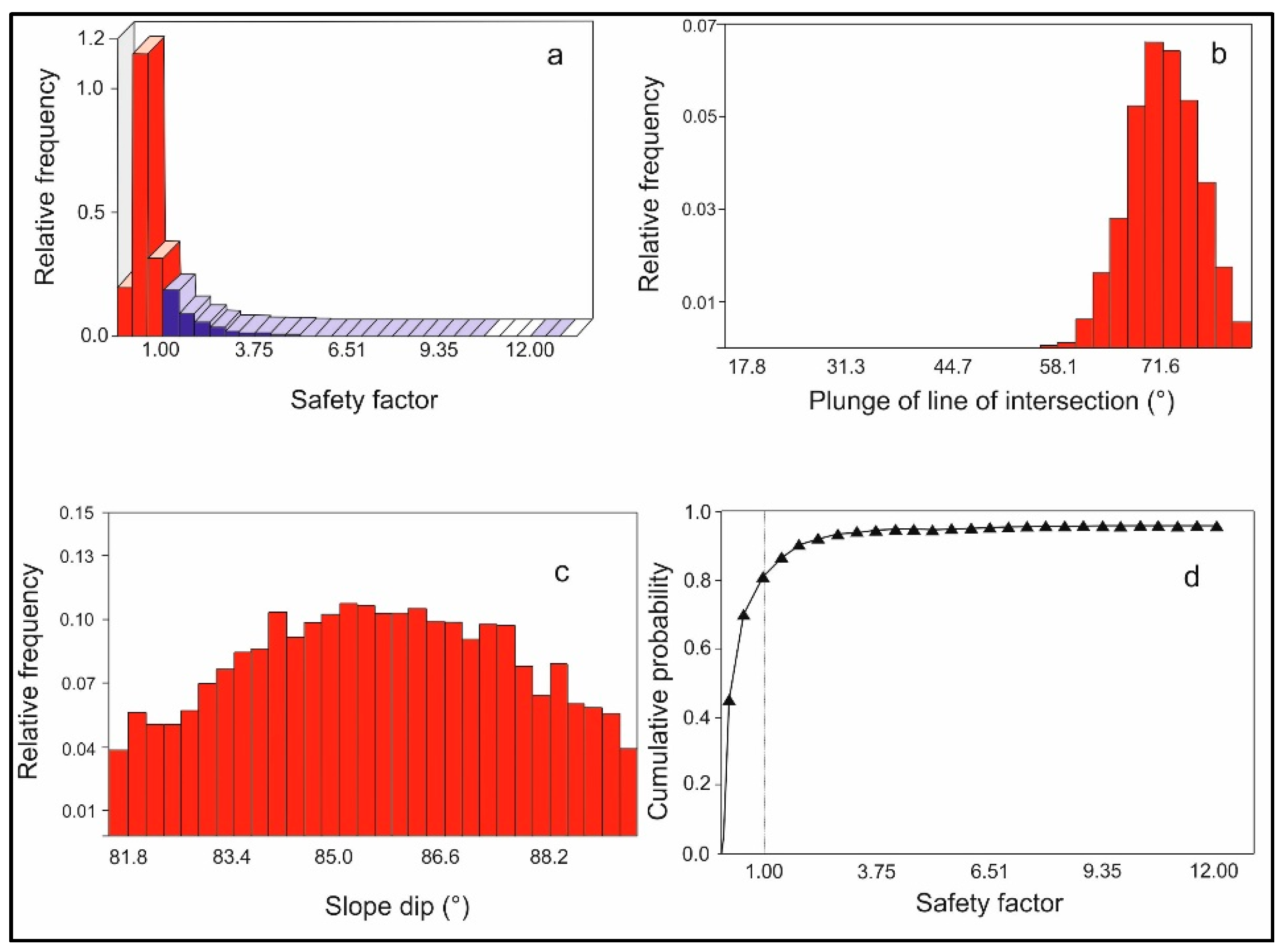
4.3. Wedge Failure
5. Discussion
6. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- International Society for Rock Mechanics and Rock Engineering. The Blue Book—The Complete ISRM Suggested Methods for Rock Characterisation, Testing and Monitoring: 1974–2006; Ulusay, R., Hudson, J.A., Eds.; ISRM & Turkish National Group of ISRM: Ankara, Turkey, 2007. [Google Scholar]
- Gibson, W. Probabilistic methods for slope analysis and design. Aust. Geomech. 2011, 46, 1–12. [Google Scholar]
- Chen, S.L.; Cheng, C.P. Influence of failure probability due to parameter and anchor variance of a freeway dip slope slide—A case study in Taiwan. Entropy 2017, 19, 431. [Google Scholar] [CrossRef]
- El-Ramly, H.H.; Morgenstern, N.R.; Cruden, D.M. Probabilistic slope stability analysis for practice. Can. Geotech. J. 2002, 39, 665–683. [Google Scholar] [CrossRef]
- Park, H.; West, T.R. Development of a probabilistic approach for rock wedge failure. Eng. Geol. 2001, 59, 233–251. [Google Scholar] [CrossRef]
- Markland, J.T. A Useful Technique for Estimating the Stability of Rock Slopes When the Rigid Wedge Sliding Type of Failure Is Expected; Imperial College of Science & Technology: London, UK, 1972; p. 20. [Google Scholar]
- Goodman, R.E. Introduction to Rock Mechanics; Wiley: New York, NY, USA, 1989; p. 562. [Google Scholar]
- Hocking, G. A method for distinguishing between single and double plane sliding of tetrahedral wedges. Int. J. Rock Mech. Min. Sci. 1976, 13, 225–226. [Google Scholar] [CrossRef]
- Park, H.; West, T.R.; Woo, I. Probabilistic analysis of rock slope stability and random properties of discontinuity parameters, interstate highway 40, Western North Carolina, USA. Eng. Geol. 2005, 79, 230–250. [Google Scholar] [CrossRef]
- Einstein, H.H. Risk and risk analysis in rock engineering. Tunn. Undergr. Space Technol. 1996, 11, 141–155. [Google Scholar] [CrossRef]
- Obregon, C.; Mitri, H. Probabilistic approach for open pit bench slope stability analysis—A mine case study. Int. J. Min. Sci. Technol. 2019, 29, 629–640. [Google Scholar] [CrossRef]
- Mat Radhi, M.S.; Mohd Pauzi, N.I.; Omar, H. Probabilistic Approach of Rock Slope Stability Analysis Using Monte Carlo Simulation. In Proceedings of the International Conference on Construction and Building Technology, Kuala Lumpur, Malaysia, 16–20 June 2008; pp. 449–469. [Google Scholar]
- Budetta, P.; de Luca, C. Wedge failure hazard assessment by means of a probabilistic approach for an unstable sea-cliff. Nat. Hazards 2015, 76, 1219–1239. [Google Scholar] [CrossRef]
- Zhao, H.; Li, Z.; Kong, C.S. Probabilistic method to determine the overall rock block failure based on failure mode. Eng. Trans. 2016, 64, 105–113. [Google Scholar]
- Mostyn, G.R.; Li, K.S. Probabilistic slope analysis-state of play. In Proceedings of the Conference on Probabilistic Methods in Geotechnical Engineering, Canberra, Australia, 10–12 February 1993; pp. 89–109. [Google Scholar]
- Read, J.; Stacey, P. Guidelines for Open Pit Slope Design; CSIRO Publishing: Collingwood, Australia, 2009; p. 487. [Google Scholar] [CrossRef]
- European Standard EN 1997-1. Eurocode 7: Geotechnical Design—Part. 1: General Rules; European Committee for Standardisation (CEN): Brussels, Belgium, 2004; p. 168. Available online: http://eurocodes.jrc.ec.europa.eu/ (accessed on 3 December 2019).
- Hoek, E.; Bray, J. Rock Slope Engineering, 3rd ed.; Taylor & Francis: London, UK, 1981; p. 357. [Google Scholar]
- Nilsen, B. Rock slope stability analysis according to Eurocode 7, discussion of some dilemmas with particular focus on limit equilibrium analysis. Bull. Eng. Geol. Environ. 2017, 76, 1229–1236. [Google Scholar] [CrossRef]
- Cherubini, C.; Vessia, G. Reliability analyses of rock slope stability. In Proceedings of the 2nd Int Symp on Geothecnical Safety and Risk, Gifu, Japan, 11–12 June 2009; pp. 83–88. [Google Scholar]
- Rocscience Inc. DIPS An Interactive Analysis of Orientation Based Geological Data Software Program (Version 7); Toronto, ON, Canada, 2016. Available online: http://www.rocscience.com (accessed on 12 December 2019).
- Wyllie, D.C.; Mah, C.W. Rock Slope Engineering Civil and Mining, 4th ed.; Taylor & Francis e-Library: London, UK, 2005; p. 431. Available online: https://civilenglineering.files.wordpress.com/2014/10/rock_slope_engineering_civil_and_mining.pdf (accessed on 3 December 2019).
- Rocscience Inc. ROCPLANE (Version 4.001) and SWEDGE (Version 7.001) Software Programs. Toronto, ON, Canada. 2019. Available online: http://www.rocscience.com (accessed on 16 December 2019).
- Grenon, M.; Hadjigeorgiou, J. A design methodology for rock slopes susceptible to wedge failure using fracture system modelling. Eng. Geol. 2008, 96, 78–93. [Google Scholar] [CrossRef]
- Bonardi, G.; Ciarcia, S.; di Nocera, S.; Matano, F.; Sgrosso, I.; Torre, M. Carta delle principali unità cinematiche dell’Appennino meridionale-Nota illustrative. Italian J. Geosci. 2009, 128, 47–60. (In Italian) [Google Scholar]
- Scrocca, D. Southern Apennines: Structural Setting and Tectonic Evolution. J. Virtual Explor. 2010. Available online: http://virtualexplorer.com.au/article/2009/225/southern-apennines-evolution (accessed on 9 January 2020). [CrossRef]
- International Society Rock Mechanics (ISRM). Suggested Methods for Determining Water Content, Porosity, Density, Absorption and Related Properties and Swelling and Slake-Durability Index Properties; Pergamon Press Ltd.: Oxford, UK, 1977; pp. 143–151. [Google Scholar]
- Barton, N.R.; Choubey, V. The shear strength of rock joints in theory and practice. Rock Mech. 1977, 10, 1–54. [Google Scholar] [CrossRef]
- Barton, N.R. The shear strength of rock and rock joints. Int. J. Mech. Min. Sci. Geomech. Abstr. 1976, 13, 1–24. [Google Scholar] [CrossRef]
- Seidel, J.P.; Haberfield, C.M. The application of energy principles to the determination of the sliding resistance of rock joints. Rock Mech. Rock Eng. 1995, 28, 211–226. [Google Scholar] [CrossRef]
- Ladanyi, B.; Archambault, G. Simulation of shear behaviour of a jointed rock mass. In Proceedings of the 11th U.S. Symposium on Rock Mechanics, Berkeley, CA, USA, 16–19 June 1969; pp. 105–125. [Google Scholar]
- Basahel, H.; Mitri, H. Probabilistic assessment of rock slopes stability using the response surface approach-A case study. Int. J. Min. Sci. Technol. 2019, 29, 357–370. [Google Scholar] [CrossRef]
- Aladejare, A.E.; Akeju, V.O. Design and sensitivity analysis of rock slope using Monte Carlo simulation. Geotech. Geol. Eng. 2020, 38, 573–585. [Google Scholar] [CrossRef]


| Sample (N.) | Calcite (%) | Dolomite (%) | γs (kN/m3) | γ (kN/m3) | γd (kN/m3) | n (%) | w (%) |
|---|---|---|---|---|---|---|---|
| 1 2 | 60.23 60.88 | 25.11 25.31 | 28.1 27.8 | 26.0 25.8 | 25.4 25.0 | 9.58 10.11 | 2.35 3.25 |
| 3 4 5 6 7 μ σ | 61.75 61.97 60.45 61.84 61.50 61.23 0.71 | 26.31 26.31 26.40 25.79 24.97 25.74 0.61 | 28.7 28.1 27.6 27.9 28.3 28.1 0.36 | 28.3 26.8 26.4 26.0 26.5 26.5 0.85 | 27.8 25.7 27.3 27.1 25.5 26.3 1.11 | 2.48 8.39 9.34 10.10 9.37 8.48 2.71 | 1.12 4.11 4.35 5.60 2.36 3.31 1.50 |
| Parameter | μ | σ | Min | Max | Distribution |
|---|---|---|---|---|---|
| Upper face | 37° | 2.73° | 32° | 41° | Normal |
| Failure plane | 37° | 2.73° | 33° | 41° | Normal |
| Tension crack | 85° | 2.73° | 81° | 89° | Normal |
| Dist. from crest | 4.25 m | 2.73 m | 0.25 m | 8.25 m | Normal |
| Friction angle | 38.5° | 2.44° | 35° | 42° | Normal |
| Parameter | μ | σ | Min | Max | Distribution |
|---|---|---|---|---|---|
| Dip of the slope | 85.5° | 3° | 81° | 90° | Normal |
| Dip dir. of the slope | 293.5° | 2.87° | 289° | 298° | Normal |
| Dip of J1 | 73° | 6° | 63° | 83° | Normal |
| Dip dir. of J1 | 285° | 9° | 270° | 300° | Normal |
| Dip of J2 Dip dir. of J2 Dip of the upper face Dip dir. of the upper face Friction angle on J1 and J2 | 78° 250° 5° 293° 38.5° | 6° 9° 3° 3° 2.44° | 68° 235° 0° 288° 35° | 88° 265° 10° 298° 42° | Normal Normal Normal Normal Normal |
| Failure Model | ODF | Probability of Failure | ||
|---|---|---|---|---|
| Kinematic | Kinetic | Final | ||
| Plane | 0.84 | 0.369 | 0.293 | 0.108 |
| Wedge | 0.48 | 0.231 | 0.802 | 0.185 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Budetta, P. Some Remarks on the Use of Deterministic and Probabilistic Approaches in the Evaluation of Rock Slope Stability. Geosciences 2020, 10, 163. https://doi.org/10.3390/geosciences10050163
Budetta P. Some Remarks on the Use of Deterministic and Probabilistic Approaches in the Evaluation of Rock Slope Stability. Geosciences. 2020; 10(5):163. https://doi.org/10.3390/geosciences10050163
Chicago/Turabian StyleBudetta, Paolo. 2020. "Some Remarks on the Use of Deterministic and Probabilistic Approaches in the Evaluation of Rock Slope Stability" Geosciences 10, no. 5: 163. https://doi.org/10.3390/geosciences10050163
APA StyleBudetta, P. (2020). Some Remarks on the Use of Deterministic and Probabilistic Approaches in the Evaluation of Rock Slope Stability. Geosciences, 10(5), 163. https://doi.org/10.3390/geosciences10050163





