A Dumbbell Shaped Piezoelectric Motor Driven by the First-Order Torsional and the First-Order Flexural Vibrations
Abstract
1. Introduction
2. Configuration and Working Principle
2.1. Design Procedure
- (1)
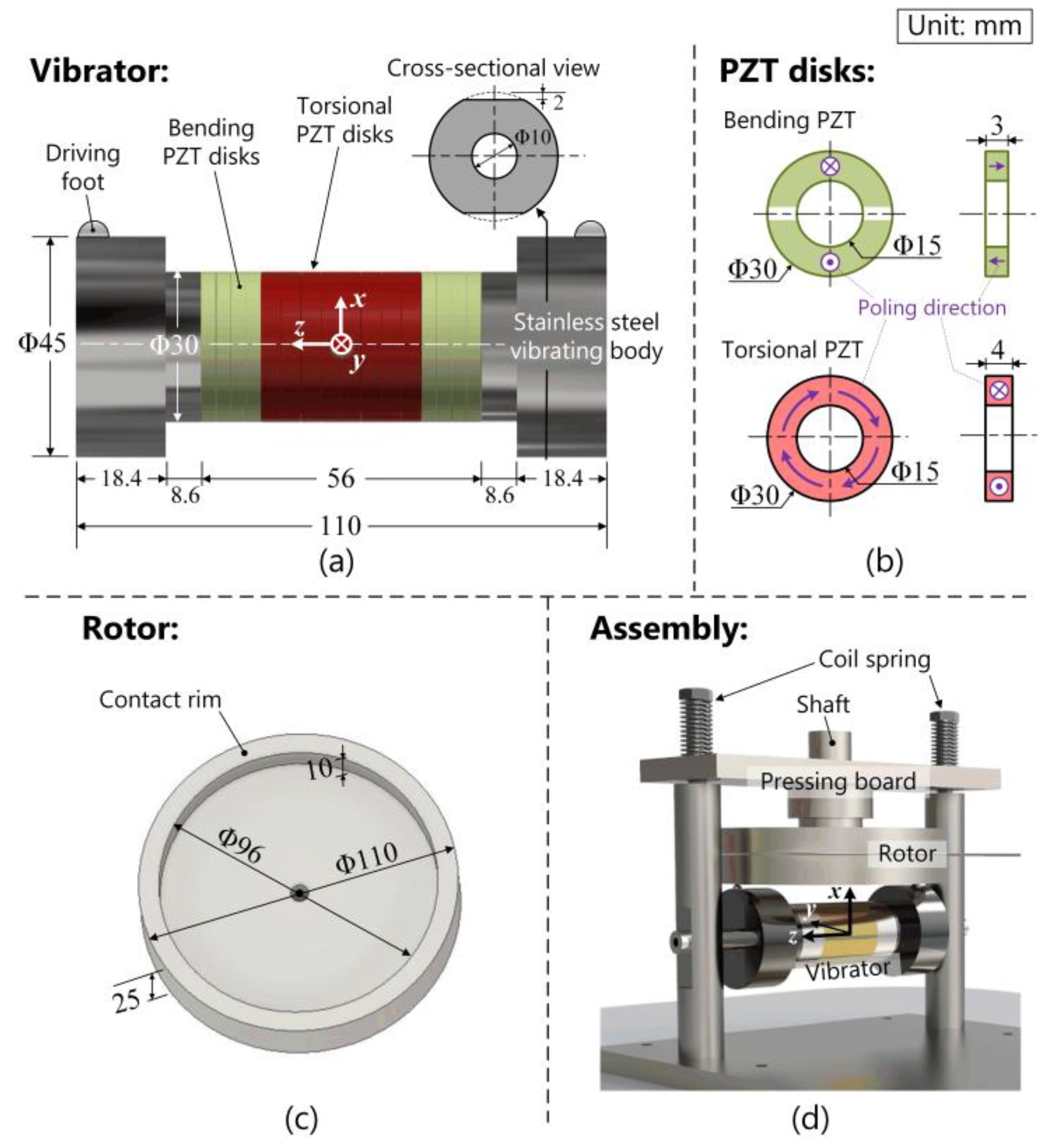
- Determine the material of each part. Here, stainless steel (elastic modulus: 197 GPa, density: 7800 kg/m3, and Poisson’s ratio: 0.29) was chosen for the vibrating bodies. The density of the PZT ceramics, provided with our agent, is 7700 kg/m3 and the elastic, piezoelectric, and dielectric matric are as follows:andFigure 1b shows the dimensions and the poling directions of PZT disks. The flexural PZT disks (30 mm in outer diameter, 15 mm in inner diameter, and 3 mm in thickness) were divided into two parts with opposite poling directions, while the torsional ones (30 mm in outer diameter, 15 mm in inner diameter, and 4 mm in thickness) were poled in the circumferential direction. The PZT disks were clamped between the vibrating bodies with a stainless-steel screw.
- (2)
- Arrange the PZT disks. Since the motor works in the 1st-order torsional and the 1st-order flexural modes, the torsional PZT disks were set in the middle part of the vibrator. Additionally, the central position of the flexural PZT disks located at the node of the flexural mode. By solving the Equation [21]:it can be found that the distance between the flexural mode’s node and the vibrator’s middle surface (Δl) should be equal to 0.275 times of the vibrator’s entire length (l). Then, the number of PZT disks were determined according to the distance between each node and the PZT disks’ thicknesses.
- (3)
- Decide the dominant diameters. Here, the diameters of the vibrating bodies adjacent to the PZT disks were set to 30 mm, as torsional PZT in this diameter is easy to produce.
- (4)
- Conduct modal degeneration. Through the finite element method (FEM; software ANSYS 14.0, ANSYS Incorporated, Canonsburg, PA, USA.), the lengths of the vibrating bodies’ bilateral outer parts were adjusted to gather the resonance frequencies of the flexural and torsional vibrations. Here, the FEM model of the vibrator was meshed with hexahedron elements, whose types were SOLID227 and SOLID5 for, respectively, the vibrating bodies and the PZT disks. The electrodes were neglected as they are too thin for meshing. Figure 1a also gives the final values of each dimension. The vibrating bodies include two steps: the outer step with a circumferential surface are 45 mm in diameter and 18.4 mm in length, while the inner step 30 mm in diameter and 8.6 mm in length contacts the PZT disks.
- (5)
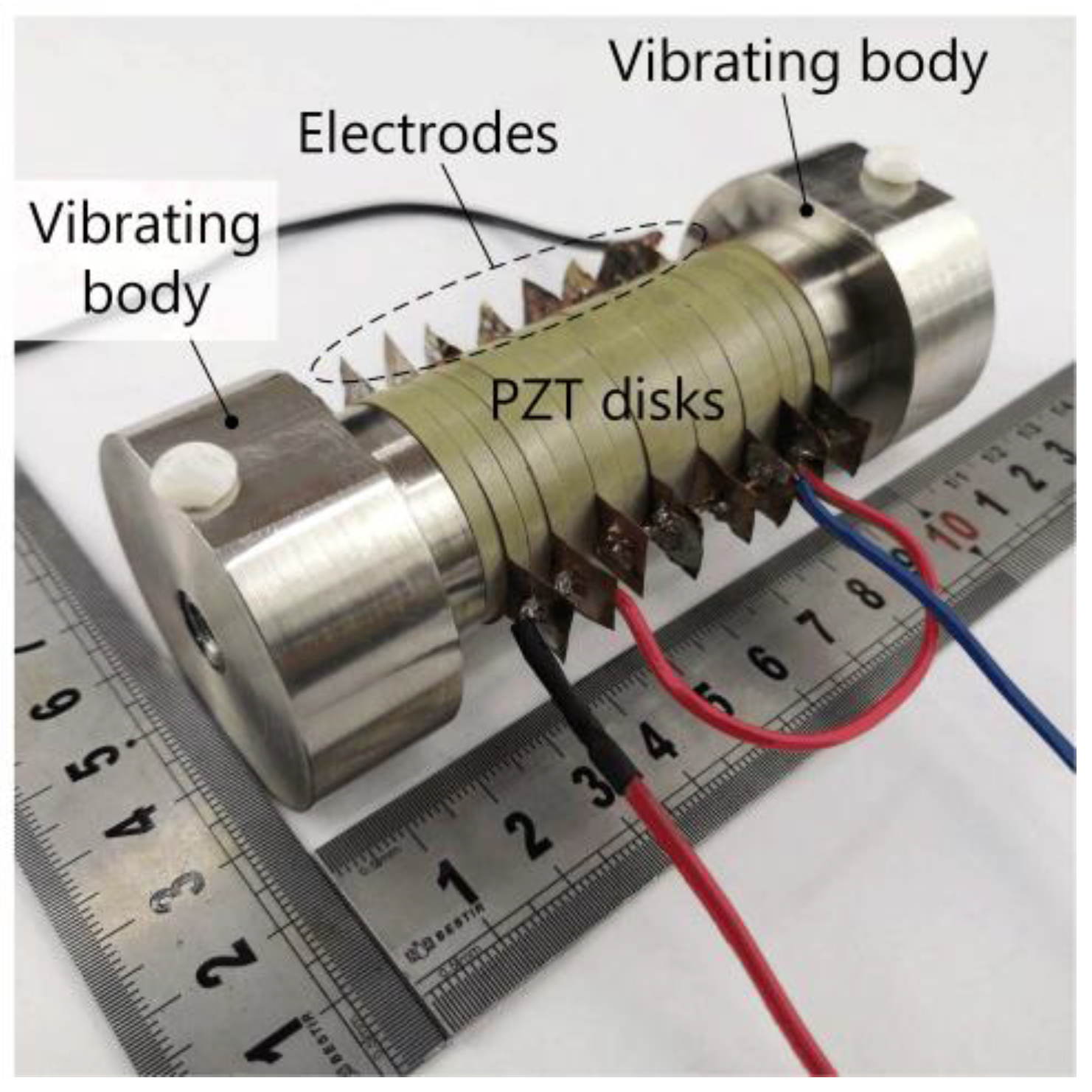
- Design the accessory components. First, two polymetric hemispheres were fixed near the vibrator’s edges as the driving feet. Second, Figure 1c illustrates the configuration of the rotor, whose contact rim had the width and height of, respectively, 12 and 10 mm. Third, Figure 1d shows the assembly of the T/F motor. Observably, the rotor was pressed orthogonally to the vibrator by tightening two coil springs. Since the driving-feet distance was approximately equal to the rotor’s diameter, the elliptical motions existing on two driving feet frictionally drove the rotor.
2.2. Working Principle
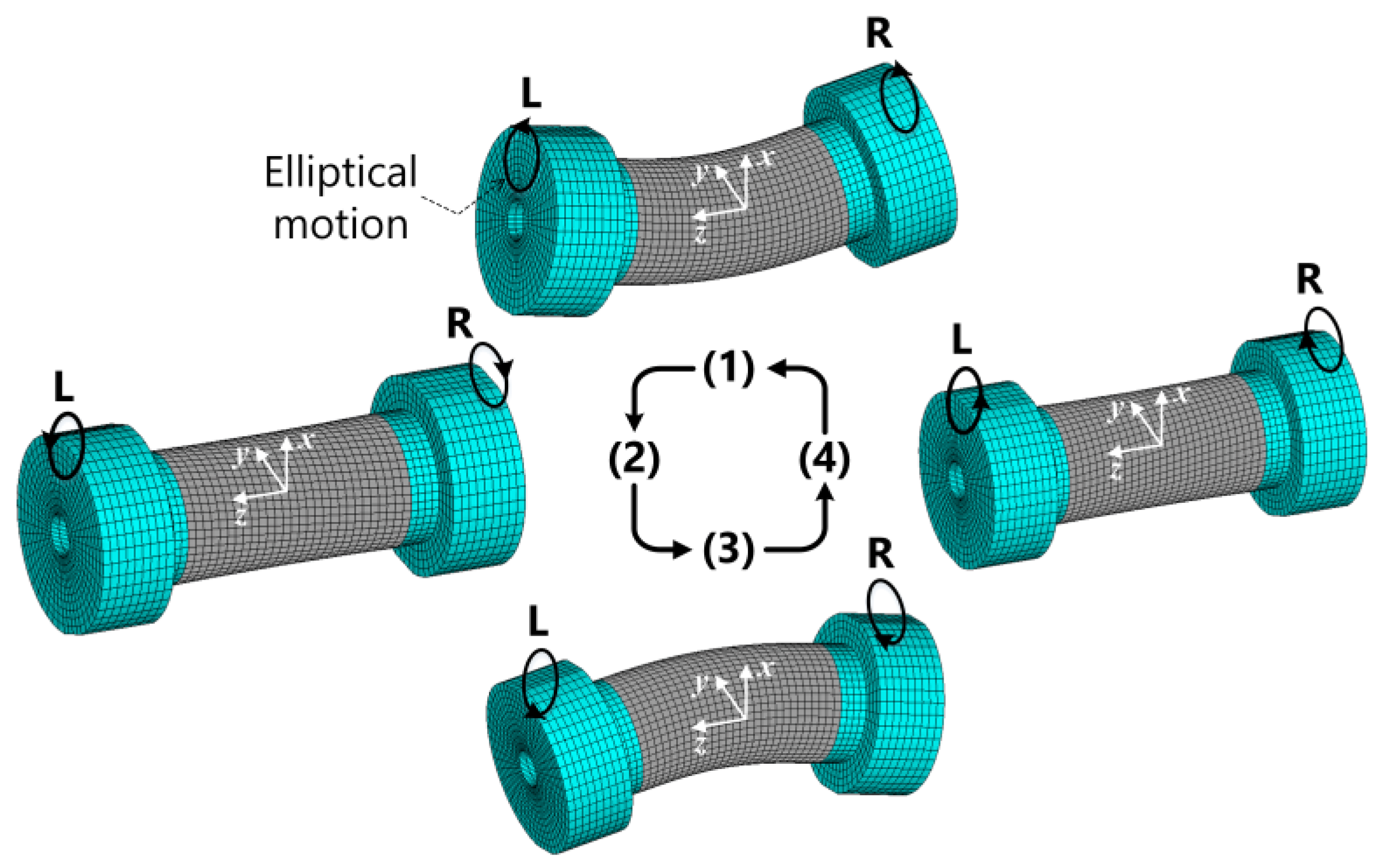
- (1)
- At ωt = 0, the torsional vibration velocity reaches the peak value. Meanwhile, the flexural vibration displacement is maximal in the +x axis. Since the vibrator operates in the 1st torsional mode, the torsional vibration velocities at left (L) and right (R) points are in inverse directions.
- (2)
- At ωt = 90°, the torsional vibration velocity and the flexural vibration displacement are zero.
- (3)
- At ωt = 180°, the torsional vibration velocity is maximal but the direction is inverse to those in step (1). On the other hand, the flexural vibration displacement is maximal in the-x axis.
- (4)
- At ωt = 270°, the torsional vibration velocity and the flexural vibration displacement are zero.
3. Vibration Properties
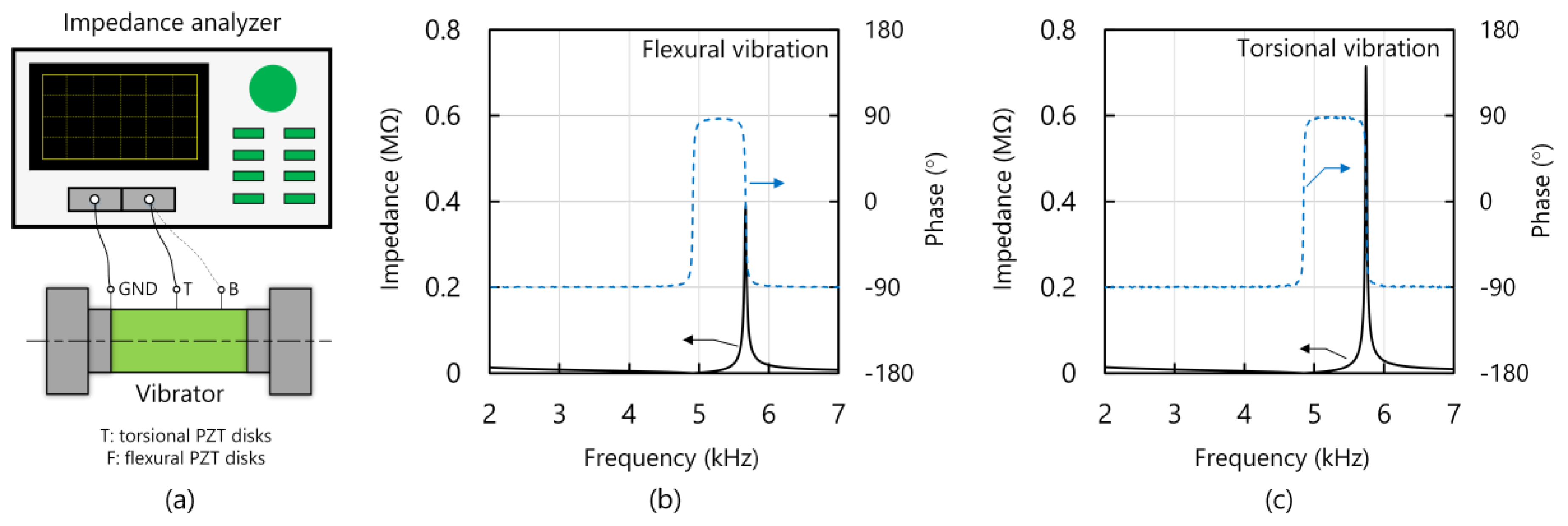
3.1. Impedance Characteristics
3.2. Vibration Velocity Distribution
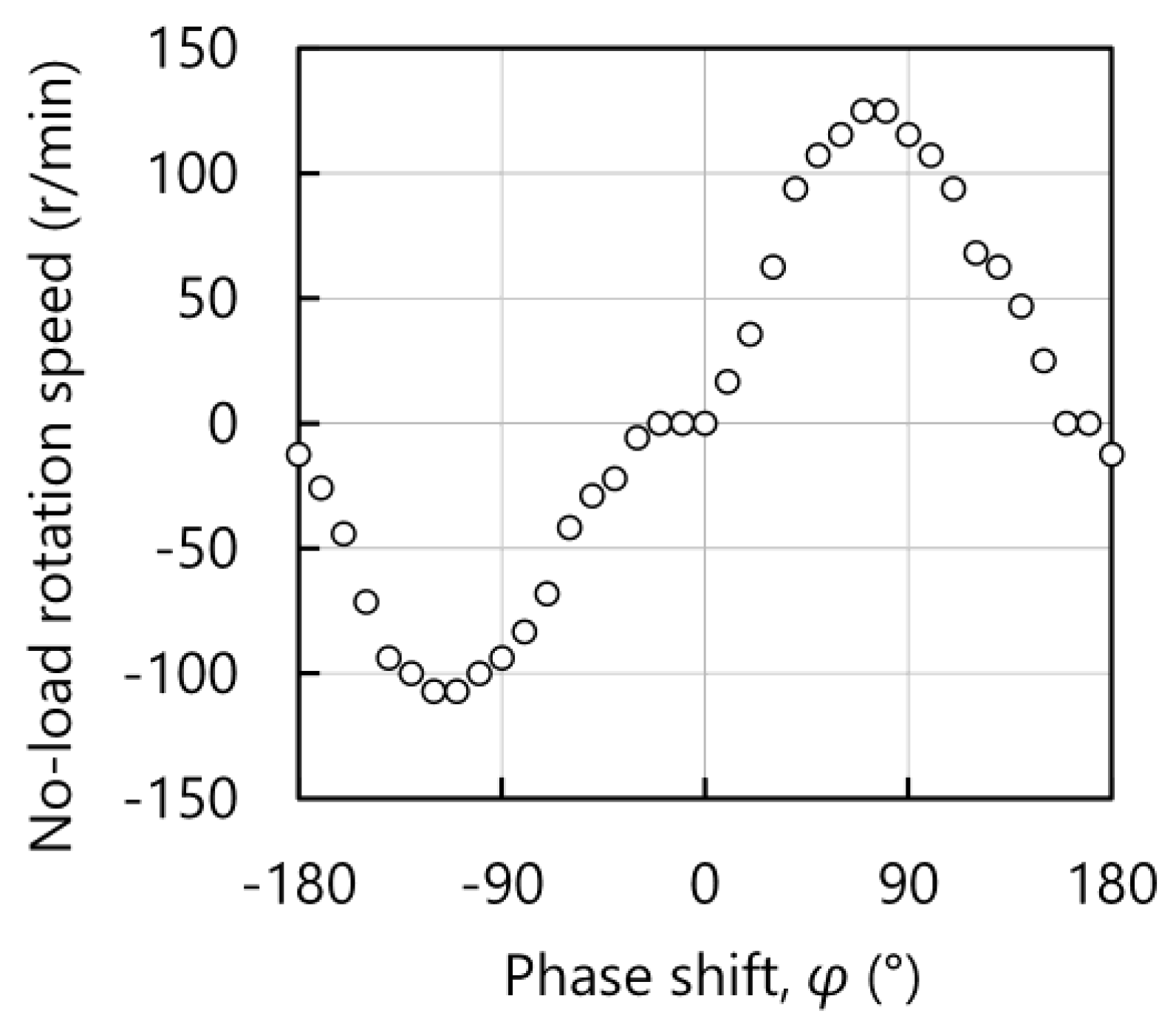
4. Load Characteristics
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ueha, S.; Tomikawa, Y.; Kurosawa, M.; Nakamura, K. Ultrasonic Motors—Theory and Applications; Oxford University Press: New York, NY, USA, 1993; pp. 4–13. [Google Scholar]
- Yan, L.; Liu, D.; Lan, H.; Jiao, Z. Compact traveling wave micromotor based on shear electromechanical coupling. IEEE/ASME Trans. Mechatron. 2016, 21, 1572–1580. [Google Scholar] [CrossRef]
- Dong, S.; Zhang, J.; Kim, H.W.; Strauss, M.T.; Uchino, K.; Viehland, D. Flexural traveling wave excitation based on shear-shear mode. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2004, 51, 1240–1246. [Google Scholar] [CrossRef]
- Gao, X.; Xin, X.; Wu, J.; Chu, Z.; Dong, S. A multilayered-cylindrical piezoelectric shear actuator operating in shear (d15) mode. Appl. Phys. Lett. 2018, 112, 152902. [Google Scholar] [CrossRef]
- Satonobu, J.; Torii, N.; Nakamura, K.; Ueha, S. Construction of mega-torque hybrid transducer type ultrasonic motor. Jpn. J. Appl. Phys. 1997, 35, 5038–5042. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, W.; Liu, J.; Yang, X. A high-power linear ultrasonic motor using bending vibration transducer. IEEE Trans. Ind. Electron. 2012, 60, 5160–5166. [Google Scholar] [CrossRef]
- Wang, L.; Hofmann, V.; Bai, F.; Jin, J.; Twiefel, J. Modeling of coupled longitudinal and bending vibrations in a sandwich type piezoelectric transducer utilizing the transfer matrix method. Mech. Syst. Signal Process. 2018, 108, 216–237. [Google Scholar] [CrossRef]
- Nakamura, K.; Kurosawa, M.; Ueha, S. Design of a hybrid transducer type ultrasonic motor. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1993, 40, 395–3401. [Google Scholar] [CrossRef]
- Nakamura, K.; Ueha, S. Potential ability of ultrasonic motors: A discussion focused on the friction control mechanism. Electron. Commun. Jpn. Part II Electron. 1998, 81, 57–68. [Google Scholar] [CrossRef]
- Cao, T.; Li, X.; Wang, B.; Mi, Y.; Zhao, G.; Twiefel, J.; Wu, D. Viscoelastic analytical model and design of polymer-based bimodal piezoelectric motor. Mech. Syst. Signal Process. 2020, 145, 106960. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, W.; Liu, Y.; Liu, J.; Jiang, Q. A frog-shaped linear piezoelectric actuator using first-order longitudinal vibration mode. IEEE Trans. Ind. Electron. 2016, 64, 2188–2195. [Google Scholar] [CrossRef]
- Xu, D.; Liu, Y.; Shi, S.; Chen, W.; Wang, L. Development of a non-resonant piezoelectric motor with nanometer resolution driving ability. IEEE/ASME Trans. Mechatron. 2018, 23, 444–451. [Google Scholar] [CrossRef]
- Deng, J.; Liu, Y.; Chen, W.; Yu, H. A XY transporting and nano-positioning piezoelectric robot operated by leg rowing mechanism. IEEE/ASME Trans. Mechatron. 2019, 24, 207–217. [Google Scholar] [CrossRef]
- Wu, J.; Mizuno, Y.; Nakamura, K. Polymer-based ultrasonic motors utilizing high-order vibration modes. IEEE/ASME Trans. Mechatron. 2018, 23, 788–799. [Google Scholar] [CrossRef]
- Nakamura, K.; Isago, R.; Koyama, D. Endoscopic optical coherence elastography using acoustic radiation force and a vibrating fiber. AIP Conf. Proc. 2012, 1474, 247–250. [Google Scholar]
- Liu, Y.; Yan, J.; Wang, L.; Chen, W. A Two-dof ultrasonic motor using a longitudinal-bending hybrid sandwich transducer. IEEE Trans. Ind. Electron. 2019, 66, 3041–3050. [Google Scholar] [CrossRef]
- Pan, S.; Wang, D.; Huang, W. A novel small motor measurement system based on ultrasonic bearings. Measurement 2021, 168, 108307. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, W.; Yang, X.; Liu, J. A rotary piezoelectric actuator using the third and fourth bending vibration modes. IEEE Trans. Ind. Electron. 2014, 61, 4366–4373. [Google Scholar] [CrossRef]
- Wu, J.; Mizuno, Y.; Tabaru, M.; Mizuno, Y. Ultrasonic motors with polymer-based vibrators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 2169–2178. [Google Scholar] [CrossRef]
- Huang, Z.; Shi, S.; Chen, W.; Wang, L.; Wu, L.; Liu, Y. Development of a novel spherical stator multi-DOF ultrasonic motor using in-plane non-axisymmetric mode. Mech. Syst. Signal Process. 2020, 140, 106658. [Google Scholar] [CrossRef]
- Graff, K.F. Wave Motions in Elastic Solids; Dover: New York, NY, USA, 1991; pp. 169–180. [Google Scholar]
- Wu, J.; Mizuno, Y.; Nakamura, K. Piezoelectric motor utilizing an alumina/PZT transducer. IEEE Trans. Ind. Electron. 2020, 67, 6762–6772. [Google Scholar] [CrossRef]
- Nakamura, K. Ultrasonic Transducers: Materials and Design for Sensors, Actuators, and Medical Applications; Woodhead Publishing Limited: Cambridge, UK, 2012; pp. 677–704. [Google Scholar]
- Morita, T. Piezoelectric Phenomena; Morikita Publication: Tokyo, Japan, 2017; pp. 86–94. (In Japanese) [Google Scholar]
- Deng, J.; Chen, W.; Wang, Y.; Zhang, S.; Liu, Y. Modeling and experimental evaluations of a four-legged stepper rotary precision piezoelectric stage. Mech. Syst. Signal Process. 2019, 132, 153–167. [Google Scholar] [CrossRef]
- Wang, L.; Guan, Y.; Liu, Y.; Deng, J.; Liu, J. A compact cantilever-type ultrasonic motor with nanometer resolution: Design and performance evaluation. IEEE Trans. Ind. Electron. 2021, 68, 734–743. [Google Scholar] [CrossRef]
- Nakamura, K. Evaluation methods for materials for power ultrasonic applications. Jpn. J. Appl. Phys. 2020, 59, SK0801. [Google Scholar] [CrossRef]
- Izuhara, S.; Mashimo, T. Linear piezoelectric motor using a hollow rectangular stator. Sens. Actuators A Phys. 2020, 309, 112002. [Google Scholar] [CrossRef]
- Wang, L.; Xue, C.; Hofmann, V.; Bai, F.; Jin, J.; Twiefel, J. Semi-analytical modeling and optimization of a traveling wave sandwich piezoelectric transducer with a beam-ring combined structure. Mech. Syst. Signal Process. 2019, 122, 171–191. [Google Scholar] [CrossRef]
- Zhang, S.; Liu, Y.; Deng, J.; Tian, X.; Gao, X. Development of a two-DOF inertial rotary motor using a piezoelectric actuator constructed on four bimorphs. Mech. Syst. Signal Process. 2021, 149, 107213. [Google Scholar] [CrossRef]
- Dong, H.; Yu, Z.; Grattan, K.T.V.; Sun, T.; Li, T. Acoustic standing wave field measurement using a laser doppler vibrometer based on the Hankel Fourier algorithm. IEEE Access 2019, 7, 139013–139020. [Google Scholar] [CrossRef]
- Slabki, M.; Wu, J.; Weber, M.; Breckner, P.; Isaia, D.; Nakamura, K.; Koruza, J. Anisotropy of the high-power piezoelectric properties of Pb(Zr, Li)O3. J. Am. Ceram. Soc. 2019, 102, 6008–6017. [Google Scholar] [CrossRef]
- Wu, J.; Mizuno, Y.; Nakamura, K. Structural parameter study on polymer-based ultrasonic motor. Smart Mater. Struct. 2017, 26, 115022. [Google Scholar] [CrossRef]
- Xu, Z.; Pan, S.; Chen, L.; Di, S.; Huang, W. A continuously variable beam expander driven by ultrasonic motors. Rev. Sci. Instrum. 2019, 90, 096107. [Google Scholar] [CrossRef]
- Li, X.; Yao, Z.; Li, R.; Wu, D. Dynamics modeling and control of a V-shaped ultrasonic motor with two Langevin-type transducers. Smart Mater. Struct. 2020, 29, 025018. [Google Scholar] [CrossRef]
- An, D.; Yang, M.; Zhuang, X.; Yang, T.; Meng, F.; Dong, Z. Dual traveling wave rotary ultrasonic motor with single active vibrator. Appl. Phys. Lett. 2017, 110, 143507. [Google Scholar] [CrossRef]
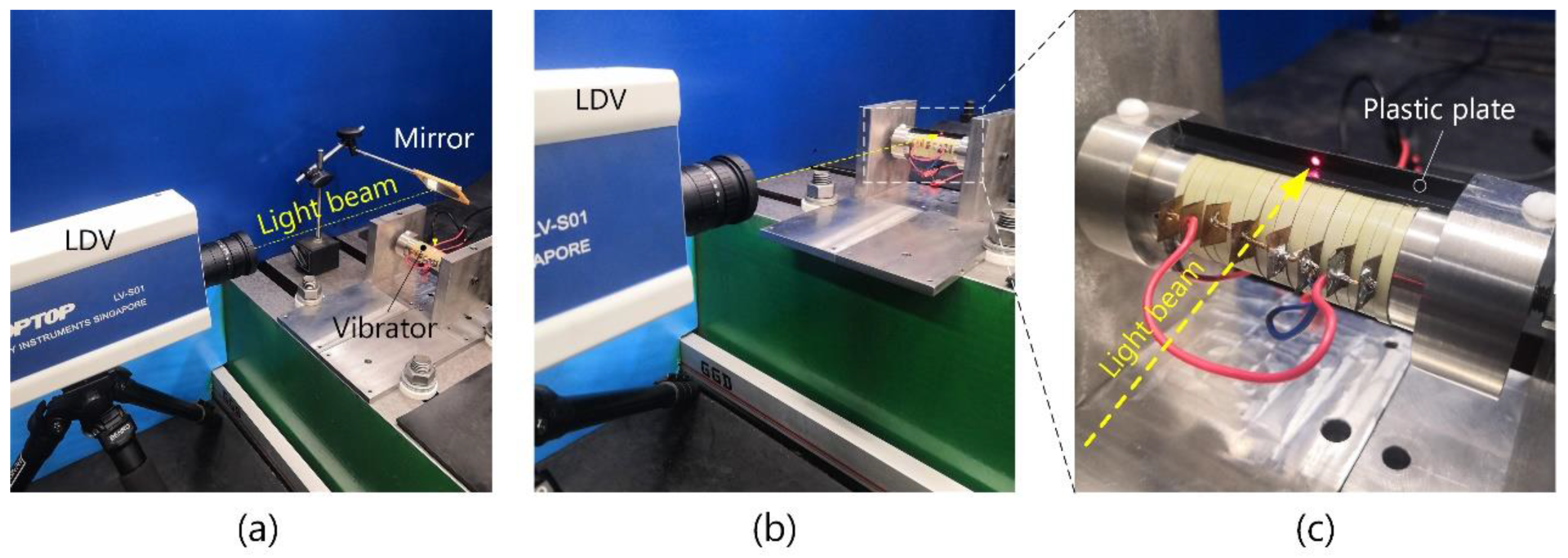

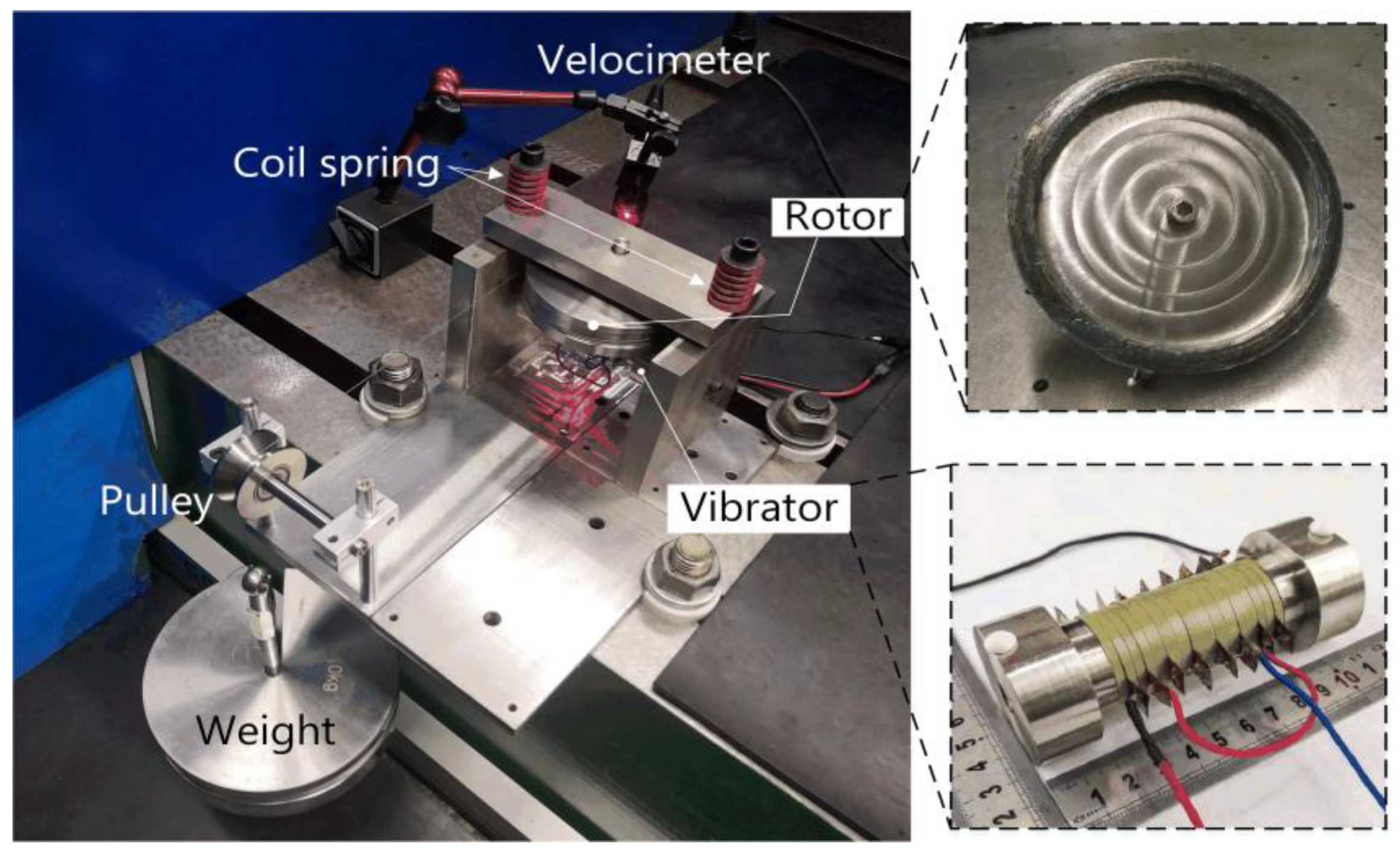

| Time | Point | Vibration Velocity | Vibration Displacement | ||
|---|---|---|---|---|---|
| Flexural | Torsional | Flexural | Torsional | ||
| ωt = 0 + 360° n | L | 0 | ωAt | Af | 0 |
| R | 0 | −ωAt | Af | 0 | |
| ωt = 90° + 360° n | L | ωAf | 0 | 0 | At |
| R | ωAf | 0 | 0 | −At | |
| ωt = 180° + 360° n | L | 0 | −ωAt | −Af | 0 |
| R | 0 | ωAt | −Af | 0 | |
| ωt = 270° + 360° n | L | −ωAf | 0 | 0 | −At |
| R | −ωAf | 0 | 0 | At | |
| Flexural Vibration | Torsional Vibration | |
|---|---|---|
| Resonance frequency, fr (kHz) | 4.911 | 4.838 |
| Anti-resonance frequency, fa (kHz) | 5.070 | 5.058 |
| Electromechanical coupling factor, k | 24.8% | 29.2% |
| Mechanical quality factor, Qm | 215 | 302 |
| Motional admittance, Ym0 (mS) | 9.3 | 14.1 |
| Motional resistance, Rm (Ω) | 107.7 | 70.9 |
| Motional inductance, Lm (H) | 0.750 | 0.705 |
| Motional capacitance, Cm (nF) | 1.40 | 1.53 |
| This Motor | [2] | [5] | |
|---|---|---|---|
| Vibration modes | T/F | T/T | T/L |
| Structure | Cylindrical | Square bar | Cylindrical |
| Dimension (mm) | Φ45 × 110 | 1.55 × 0.75 × 0.75 | Φ60 × 72 |
| Weight (kg) | 0.89 | 1.9 × 10−5 | 1.1 |
| Maximal torque (Nm) | 4.3 | 2.5 × 10−6 | 8 |
| No-load rotation speed (r/min) | 125 | 700 | – |
| Maximal output power (W) | 16.9 | 8.3 × 10−5 | 3 |
| Maximal efficiency | 10.5% | – | <15% |
| Torque density (Nm/kg) | 4.8 | 0.1 | 7.3 |
| Power density (W/kg) | 19.0 | 4.3 | 2.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niu, J.; Wu, J.; Liu, Q.; Chen, L.; Guo, S. A Dumbbell Shaped Piezoelectric Motor Driven by the First-Order Torsional and the First-Order Flexural Vibrations. Actuators 2020, 9, 124. https://doi.org/10.3390/act9040124
Niu J, Wu J, Liu Q, Chen L, Guo S. A Dumbbell Shaped Piezoelectric Motor Driven by the First-Order Torsional and the First-Order Flexural Vibrations. Actuators. 2020; 9(4):124. https://doi.org/10.3390/act9040124
Chicago/Turabian StyleNiu, Jianye, Jiang Wu, Qiming Liu, Li Chen, and Shijie Guo. 2020. "A Dumbbell Shaped Piezoelectric Motor Driven by the First-Order Torsional and the First-Order Flexural Vibrations" Actuators 9, no. 4: 124. https://doi.org/10.3390/act9040124
APA StyleNiu, J., Wu, J., Liu, Q., Chen, L., & Guo, S. (2020). A Dumbbell Shaped Piezoelectric Motor Driven by the First-Order Torsional and the First-Order Flexural Vibrations. Actuators, 9(4), 124. https://doi.org/10.3390/act9040124




