Numerical Modeling and Structure Optimization for Magnetic Levitation Planar Machine Using PCB Coils
Abstract
1. Introduction
2. Numerical Model Design and Computation
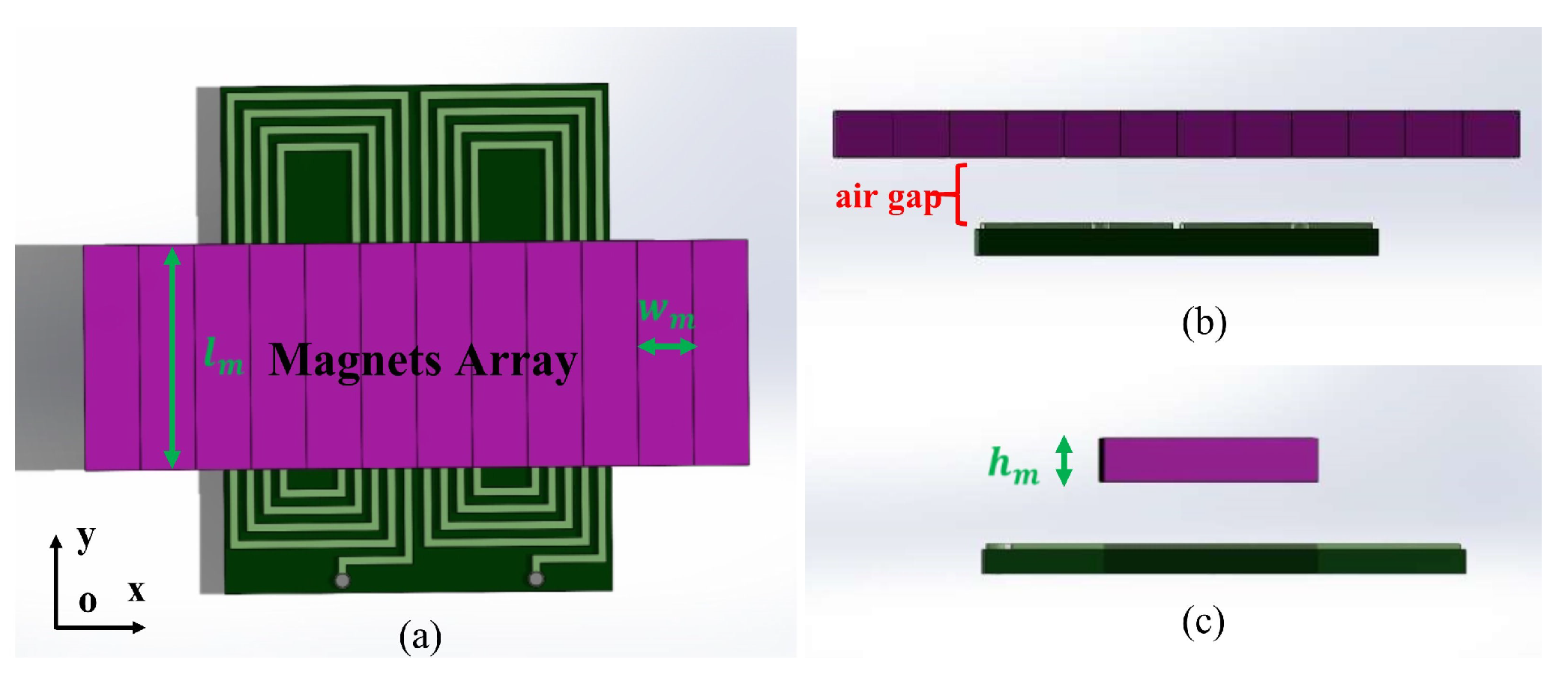
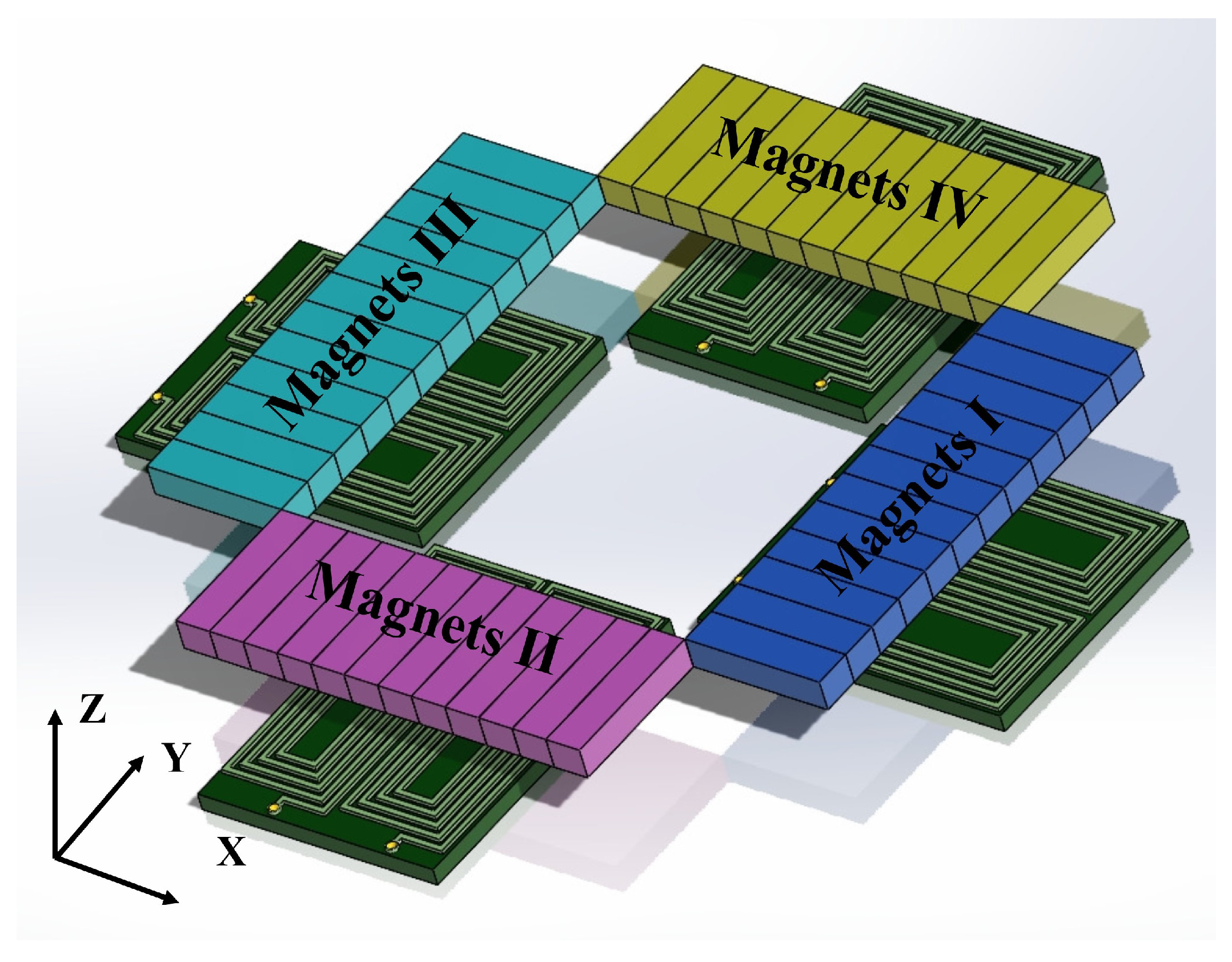
2.1. Working Principle of ML System
2.2. Construction of Numerical Model
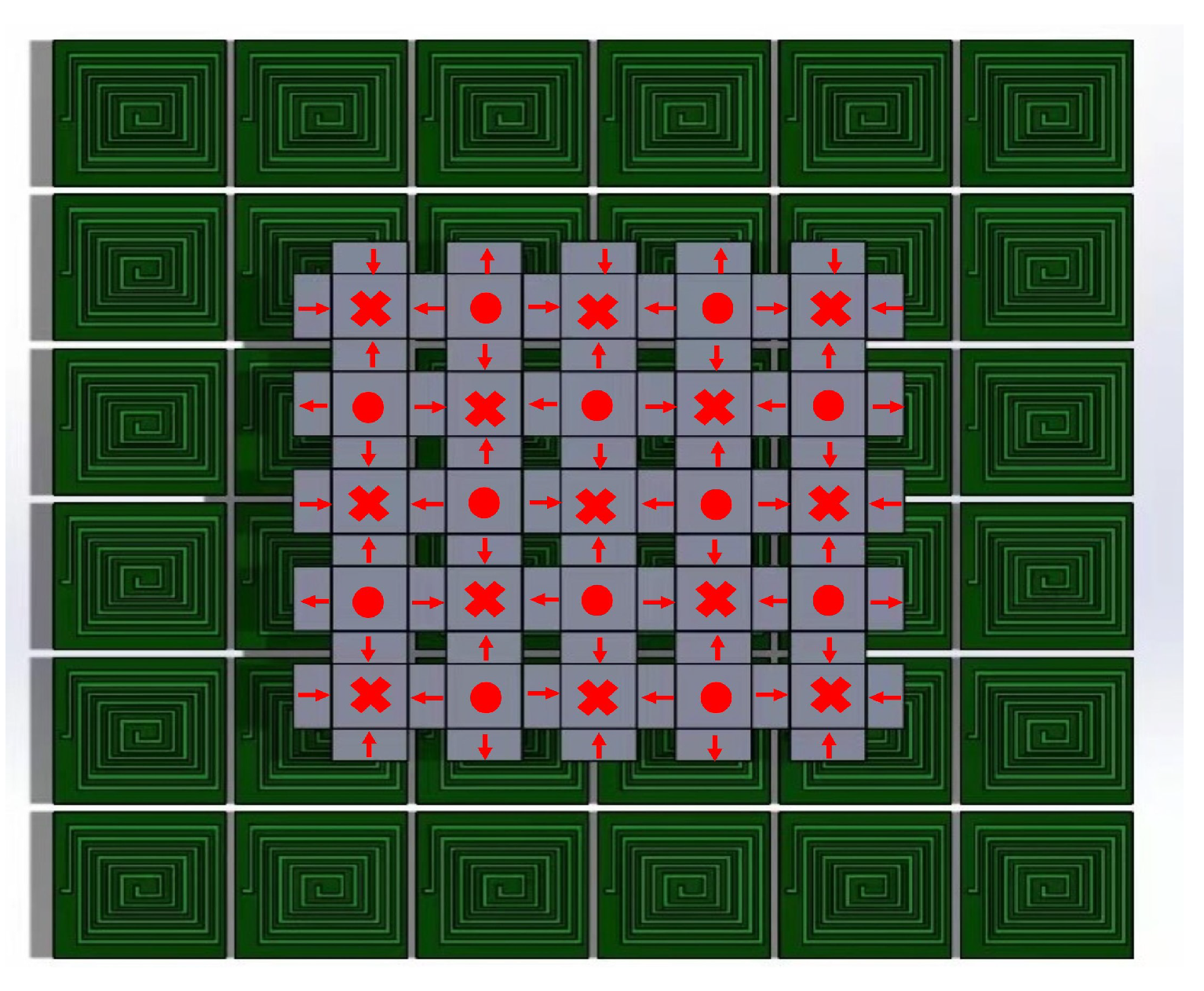
2.2.1. Magnetic Nodes
2.2.2. Coordinate Transformation
2.2.3. Computation of Magnetic Force
2.2.4. Gaussian Quadrature
2.3. Heat Transfer Equation for PCB
2.4. Power Loss Caused by Resistance and Voltage Drop
3. Optimal Design for the ML System
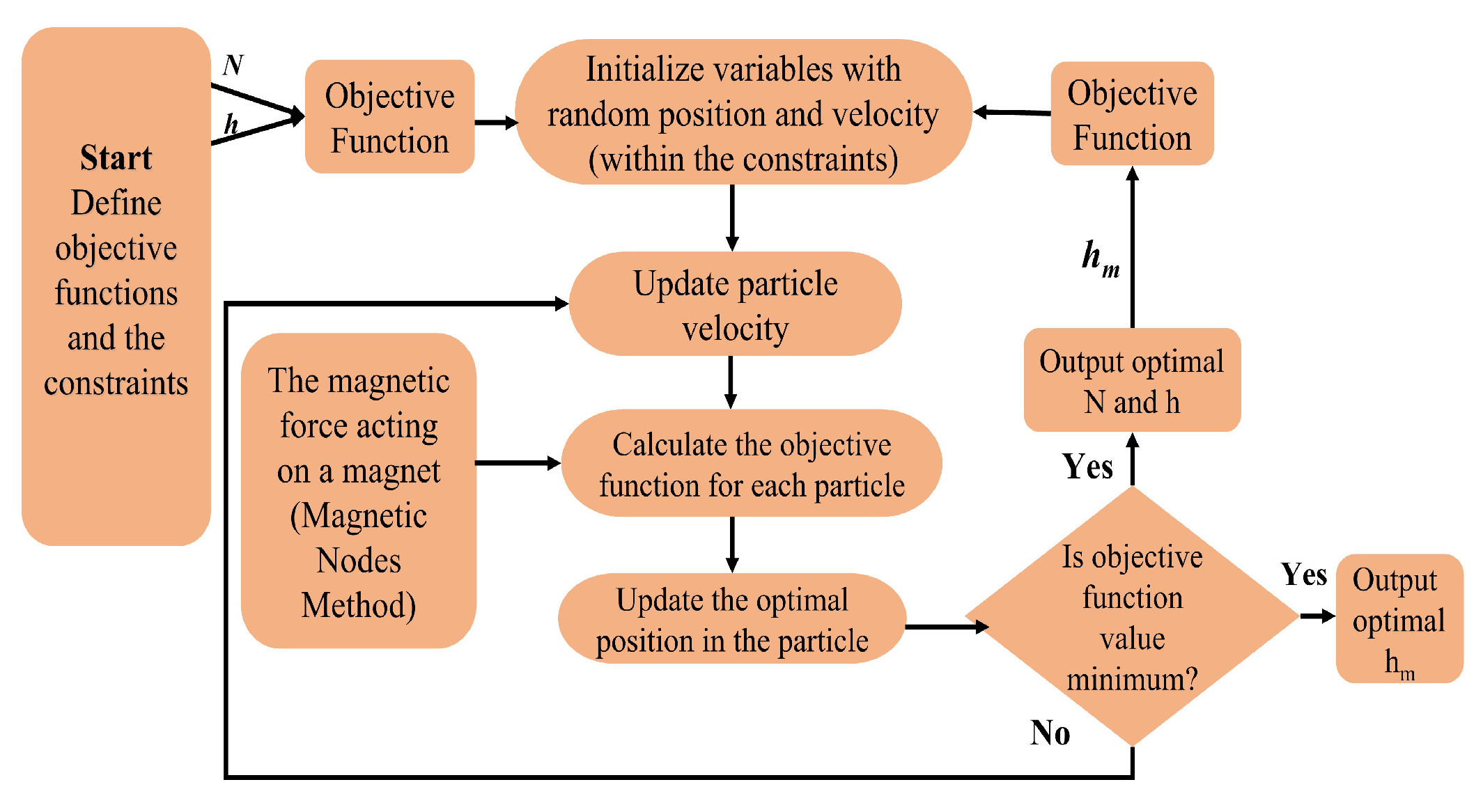
3.1. Optimization of Variables and Objective Functions
3.2. Optimization Algorithms and Constraints
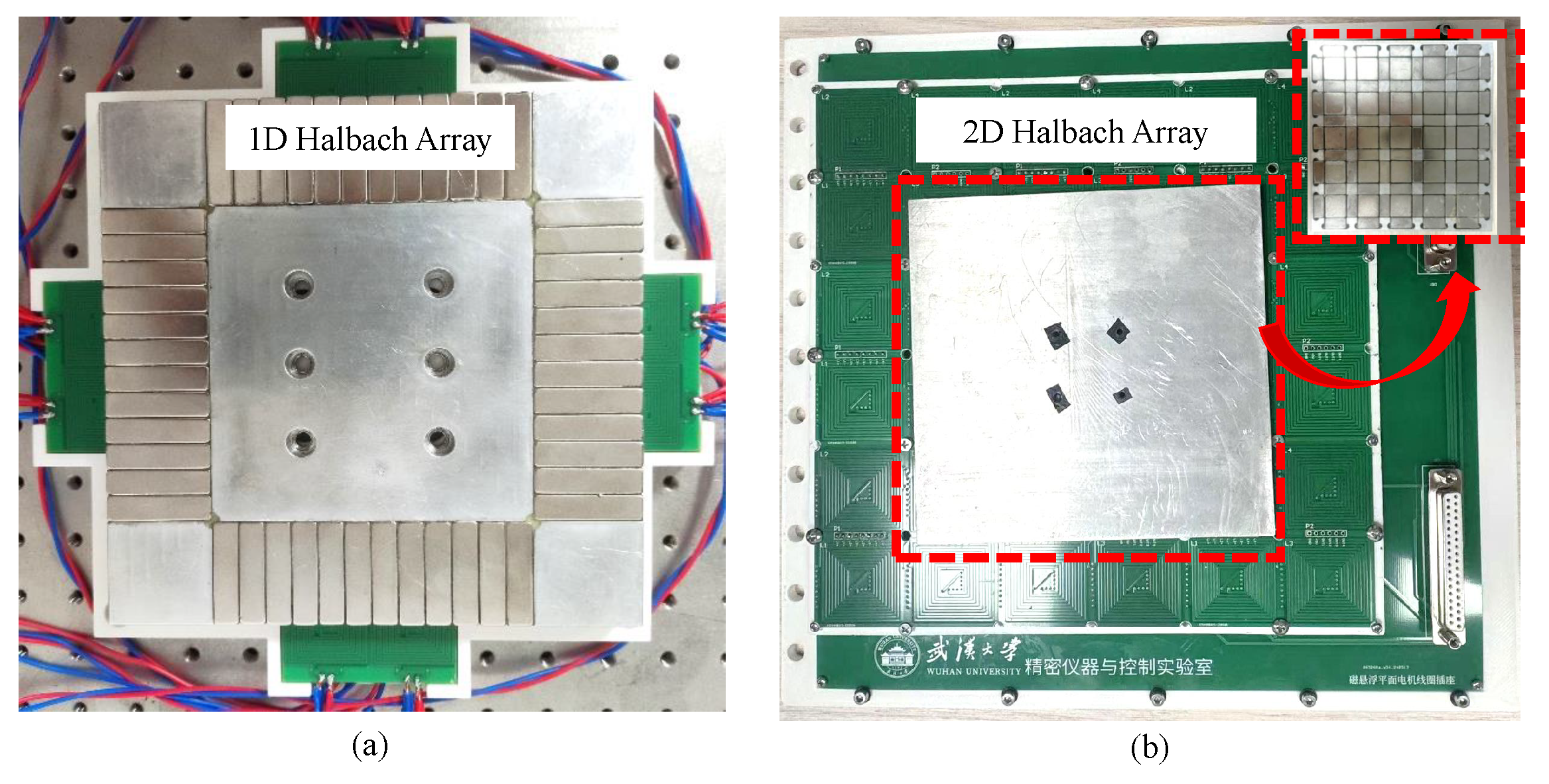
4. Validation and Verification of Magnetic Model and Optimization Process
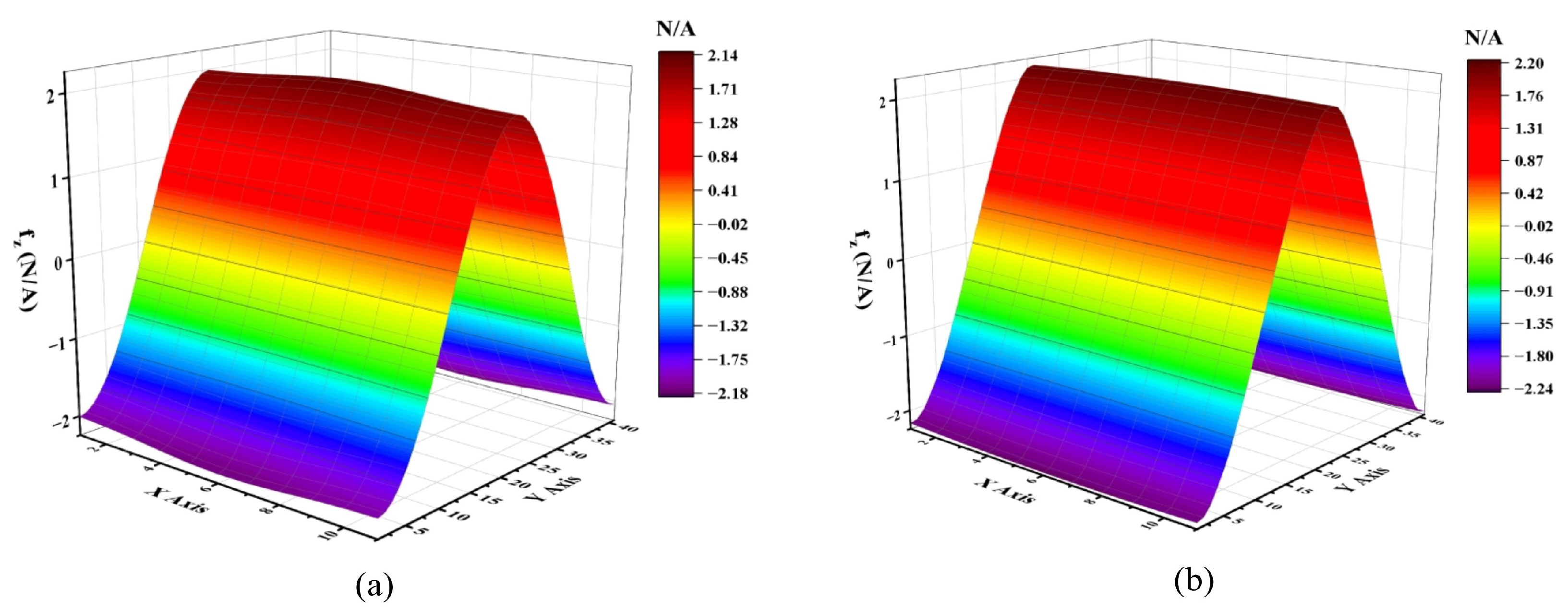
4.1. Feasibility of the Modified Magnetic Node Model
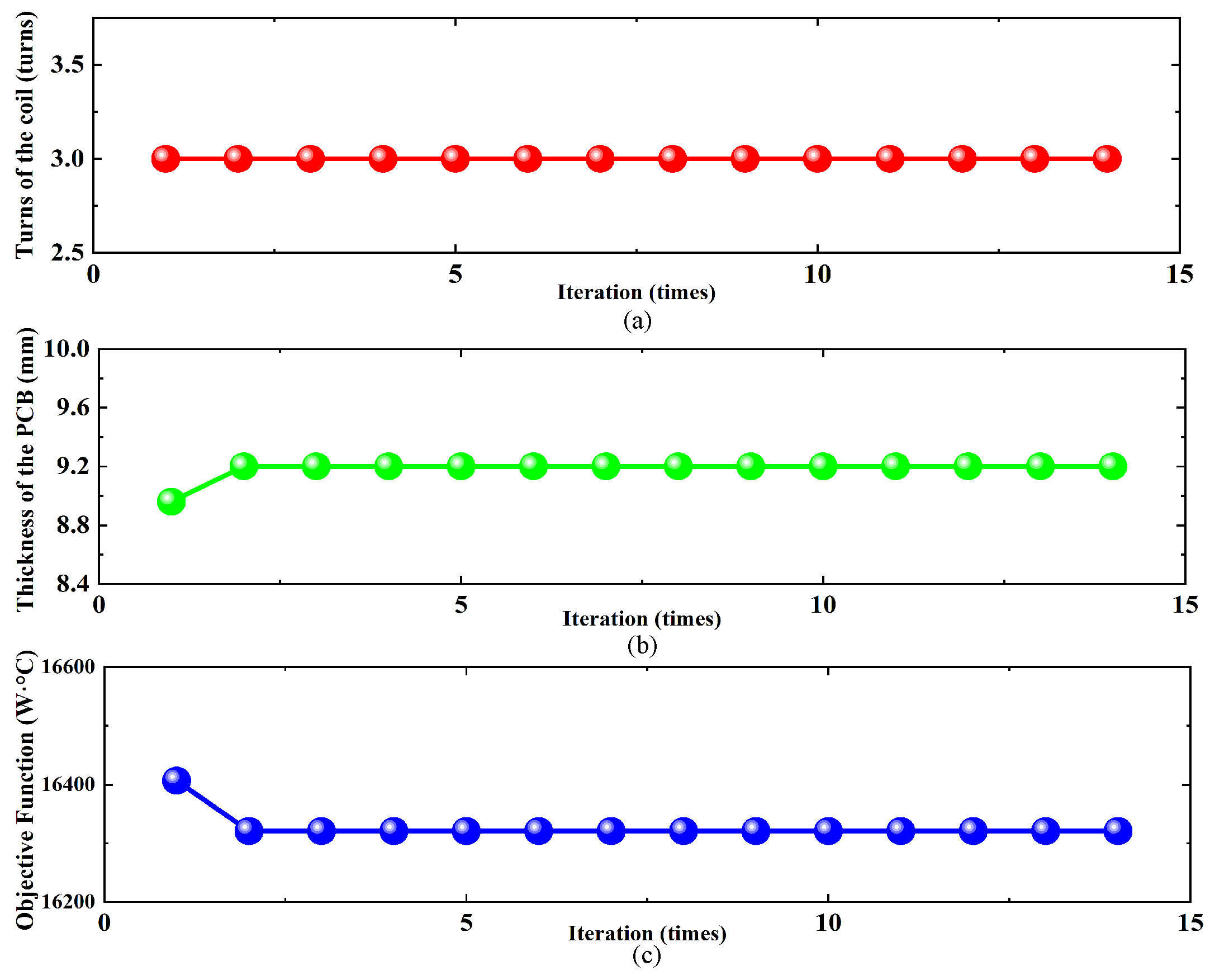
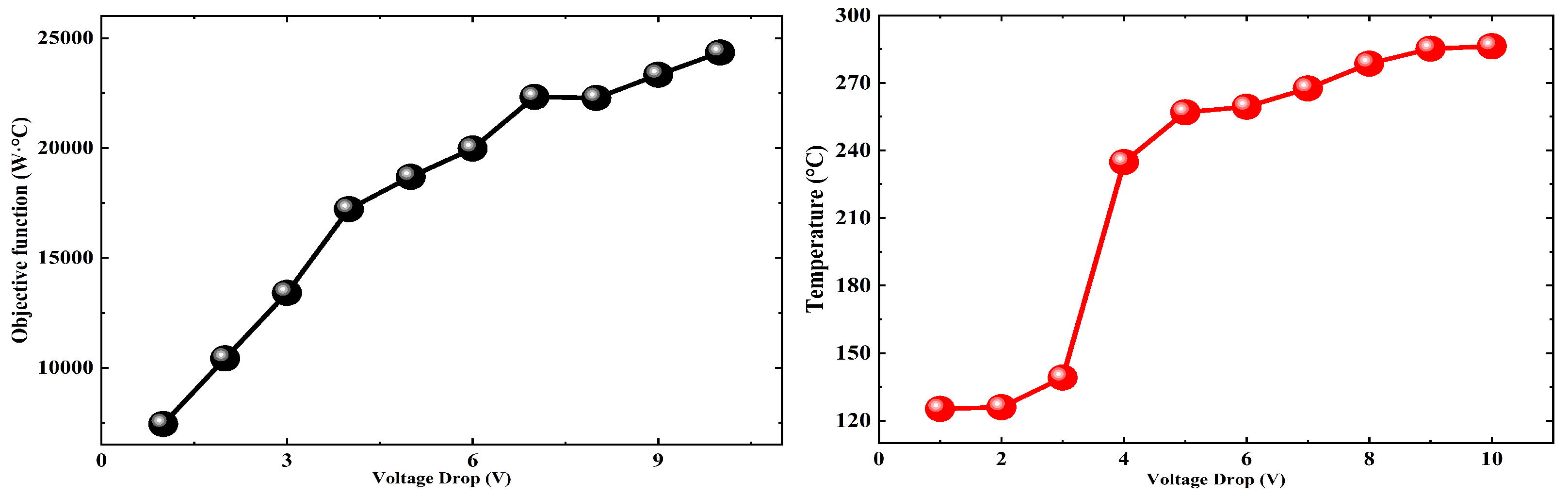
4.2. Optimization Process and Results
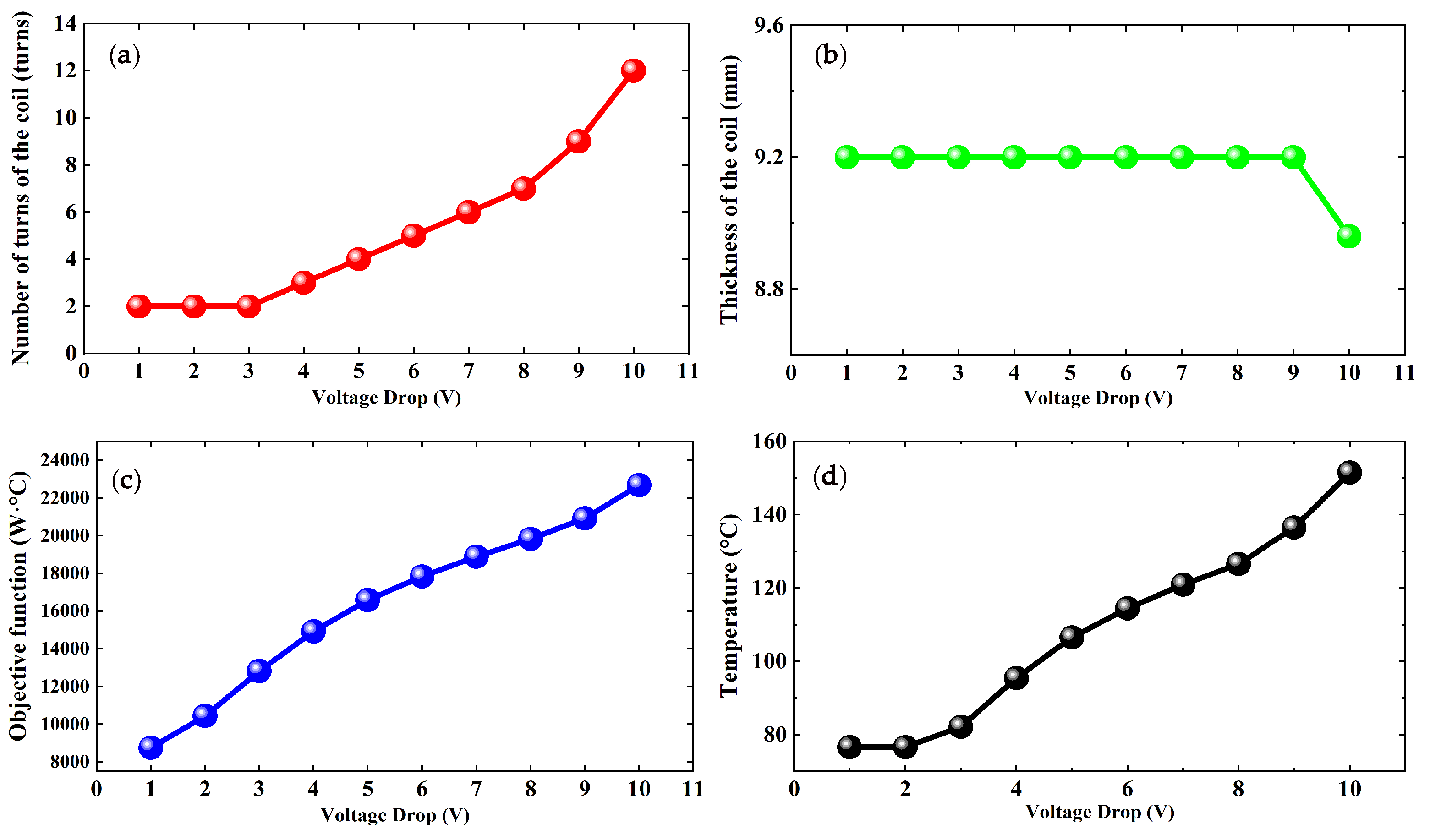
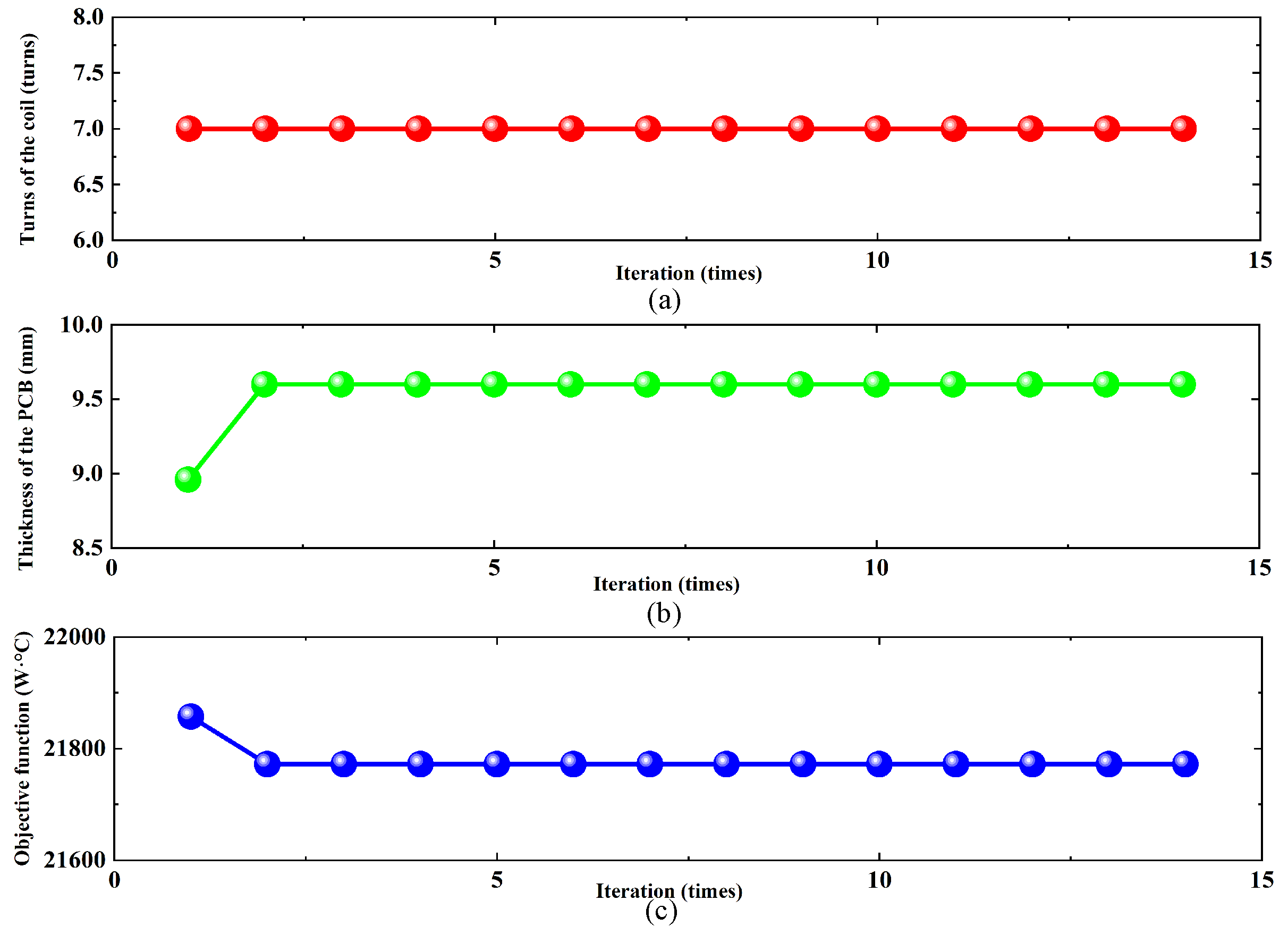
4.3. Effectiveness and Versatility of the Proposed Optimization
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pieters, R.; Tung, H.; Charreyron, S.; Sargent, D.F.; Nelson, B.J. RodBot: A rolling microrobot for micromanipulation. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation, Seattle, WA, USA, 26–30 May 2015; pp. 4042–4047. [Google Scholar]
- Jeong, J.; Ryu, J.; Gweon, D. Feedforward reference compensation using bilinear interpolation for long range motion of six degrees-of-freedom magnetic levitation planar motor. In Proceedings of the 2017 8th International Conference on Mechanical and Aerospace Engineering, Prague, Czech Republic, 22–25 July 2017; pp. 75–78. [Google Scholar]
- Almobaied, M.; Al-Nahhal, H.S.; Arrieta, O.; Vilanova, R. Design a robust Proportional-Derivative Gain-Scheduling control for a magnetic levitation system. Mathematics 2023, 11, 4040. [Google Scholar] [CrossRef]
- Week, E.M.; Wahner, U. Linear magnetic bearing and levitation system for machine tools. CIRP Ann. 1998, 47, 311–314. [Google Scholar] [CrossRef]
- He, Z.; Wen, T.; Liu, X.; Suo, Y. Loss Estimation and Thermal Analysis of a Magnetic Levitation Reaction Flywheel with PMB and AMB for Satellite Application. Energies 2020, 15, 1584. [Google Scholar] [CrossRef]
- Xu, F.; Lu, X.; Zheng, T.; Xu, X. Motion Control of a Magnetic Levitation Actuator Based on a Wrench Model Considering Yaw Angle. IEEE Trans. Ind. Electron. 2020, 67, 8545–8554. [Google Scholar] [CrossRef]
- Zhang, K.; Xu, F.; Xu, X. Observer-based fast nonlinear MPC for multi-DOF maglev positioning system: Theory and experiment. Control Eng. Pract. 2021, 114, 104860. [Google Scholar] [CrossRef]
- Peng, R.; Zheng, T.; Lu, X.; Xu, X.; Xu, F. Simulation of a synchronous Planar magnetically levitated motion system based on a Real-Time Analytical Force model. Energies 2020, 13, 6367. [Google Scholar] [CrossRef]
- Jansen, J.; Lierop, C.; Lomonova, E.; Vandenput, A. Modeling of Magnetically Levitated Planar Actuators with Moving Magnets. IEEE Trans. Magn. 2007, 43, 15–25. [Google Scholar] [CrossRef]
- Schaeffel, C.; Katzschmann, M.; Mohr, H.; Gloess, R.; Rudolf, C.; Mock, C.; Walenda, C. 6D planar magnetic levitation system—PIMag 6D. J. Mech. Eng. 2016, 3, 15-00111. [Google Scholar] [CrossRef][Green Version]
- Kurita, N.; Inomata, R.; Gruber, W.; Okayasu, T. Examination of flex PCB configuration for a winding of a magnetic levitation motor. In Proceedings of the 2020 23rd International Conference on Electrical Machines and Systems (ICEMS), Hamamatsu, Japan, 24–27 November 2020; pp. 1655–1658. [Google Scholar]
- Lu, X.; Usman, I. 6D direct-drive technology for planar motion stages. CIRP Ann. 2012, 61, 359–362. [Google Scholar] [CrossRef]
- Hsu, A.; Pelrine, R.; De Gouvea Pinto, R.; Howe, A.S.; Schaler, E.W. Design of a Novel Lunar Transportation System (FLOAT) consisting of Diamagnetically-Levitated Robots on a Flexible Film Track. In Proceedings of the 2023 IEEE Aerospace Conference, Big Sky, MT, USA, 4–11 March 2023; pp. 1–20. [Google Scholar]
- Zou, M.; Song, M.; Zhou, S.; Xu, X.; Xu, F. Force and Torque Model of Magnetically Levitated System with 2D Halbach Array and Printed Circuit Board Coils. Sensors 2023, 23, 8735. [Google Scholar] [CrossRef]
- Lee, H.; Lee, S.; Won, S.; Lee, J. Optimal design of high-precision maglev system using simulation-based DOE and FEM. IEE Proc.-Electr. Power Appl. 2006, 153, 773–779. [Google Scholar] [CrossRef]
- Saaideh, M.A.; Alatawneh, N.; Janaideh, M.A. Multi-objective optimization of a reluctance actuator for precision motion applications. J. Magn. Magn. Mater. 2022, 546, 168652. [Google Scholar] [CrossRef]
- Li, Z.; Wu, Q.; Liu, B.; Gong, Z. Optimal Design of Magneto-Force-Thermal Parameters for Electromagnetic Actuators with Halbach Array. Actuators 2021, 10, 231. [Google Scholar] [CrossRef]
- Guo, L.; Zhang, H.; Galea, M.; Li, J.; Gerada, C. Multiobjective Optimization of a Magnetically Levitated Planar Motor with Multilayer Windings. IEEE Trans. Ind. Electron. 2016, 63, 3522–3532. [Google Scholar] [CrossRef]
- Lohrasbi, S.; Hammer, R.; Essl, W.; Reiss, G.; Defregger, S.; Sanz, W. A Comprehensive Review on the Core Thermal Management Improvement Concepts in Power Electronics. IEEE Access 2020, 8, 166880–166906. [Google Scholar] [CrossRef]
- Moore, L.; Shi, L. Emerging challenges and materials for thermal management of electronics. Mater. Today 2014, 17, 163–174. [Google Scholar] [CrossRef]
- Zaman, M.S.; Michalak, A.; Nasr, M.; Da Silva, C.; Mills, J.K.; Amon, C.H.; Trescases, O. Multiphysics Optimization of Thermal Management Designs for Power Electronics Employing Impingement Cooling and Stereolithographic Printing. IET Power Electron. 2021, 36, 12769–12780. [Google Scholar] [CrossRef]
- De Bock, H.P.; Huitink, D.; Shamberger, P.; Lundh, J.S.; Choi, S.; Niedbalski, N.; Boteler, L. A system to package perspective on transient thermal management of electronics. J. Electron. Packag. 2020, 142, 041111. [Google Scholar] [CrossRef]
- Mendizábal, J.K.; Montazeri, M.; Huitink, D.; Miljkovic, N. Direct cooling of a planar magnetic converter using dielectric liquid forced convection enabled by additive manufacturing. Int. J. Heat Mass Transf. 2022, 191, 122809. [Google Scholar] [CrossRef]
- Bancel, F. Magnetic nodes. J. Phys. D Appl. Phys. 1999, 32, 2155–2161. [Google Scholar] [CrossRef]
- Kahaner, D.; Moler, C.; Nash, S. Numerical Methods in Software and Analysis; Prentice-Hall: Englewood Cliffs, NJ, USA, 1989. [Google Scholar]
- Elleaume, P.; Chubar, O.; Chavanne, J. Computing 3D Magnetic Field from Insertion Devices. In Proceedings of the 1997 Particle Accelerator Conference, Vancouver, BC, Canada, 16 May 1997; pp. 3509–3511. [Google Scholar]
- Lu, X.; Zheng, T.; Xu, F.; Xu, X. Semi-Analytical Solution of Magnetic Force and Torque for a Novel Magnetically Levitated Actuator in Rotary Table. IEEE Trans. Magn. 2019, 55, 1–8. [Google Scholar] [CrossRef]
- Zhu, H.; Pang, K.; Teo, J. Analysis and control of a 6 DOF maglev positioning system with characteristics of end-effects and eddy current damping. Mechatronics 2017, 47, 183–194. [Google Scholar] [CrossRef]
- Golub, G.; Welsch, H. Calculation of Gauss quadrature rules. Math. Comput. 1969, 23, 221–230. [Google Scholar] [CrossRef]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; Dewitt, D.P. Fundamentals of Heat and Mass Transfer, 8th ed.; Wiley: New York, NY, USA, 2017. [Google Scholar]
- Akbari, M.; Rezaei-Zare, A. Thermal Analysis of Power Transformers Under Geomagnetically Induced Current. IEEE T Power Deliver. 2023, 38, 4114–4121. [Google Scholar] [CrossRef]
- Ordonez, L.C.; Exposito, A.D.; Cervera, P.A.; Bakic, M.; Wijekoon, T. Fast and Accurate Analytical Thermal Modeling for Planar PCB Magnetic Components. IEEE Trans. Power Electron. 2023, 38, 7480–7491. [Google Scholar] [CrossRef]
- Cengel, Y. Heat and Mass Transfer: A Practical Approach, 3rd ed.; McGraw-Hill: New York, NY, USA, 2007. [Google Scholar]
- Zhang, Y. Improved Numerical-Analytical Thermal Modeling Method of the PCB with Considering Radiation Heat Transfer and Calculation of Components Temperature. IEEE Access 2021, 9, 92925–92940. [Google Scholar] [CrossRef]
- Zhang, Y. Modeling Methodology for Reliability-Concerned Current Density Analysis of PCB Tracks and Thermal Analysis of PCB Structure. Ph.D. Dissertation, Department of Information Engineering, University of Pisa, Pisa, Italy, 2013. [Google Scholar]
- Yang, C.; Xia, Y. A multi-objective optimization strategy of load-dependent sensor number determination and placement for on orbit modal identification. Measurement 2022, 200, 111682. [Google Scholar] [CrossRef]
- Nourmohammadi, A.; Asteraki, M.H.; Feiz, S.; Habibi, M. A generalized study of coil-core-aspect ratio optimization for noise reduction and snr enhancement in search coil magnetometers at low frequencies. IEEE Sens. J. 2015, 15, 6454–6459. [Google Scholar] [CrossRef]
- Shiri, A.; Allahyari, M. Sensitivity analysis and optimization of railguns using circuit model. IEEE Trans. Plasma Sci. 2020, 48, C2. [Google Scholar] [CrossRef]
- Wang, D.; Tan, D.; Liu, L. Particle swarm optimization algorithm: An overview. Soft Comput. 2017, 22, 387–408. [Google Scholar] [CrossRef]
- Goldbarg, E.; Souza, G.; Goldbarg, M. Particle swarm optimization for traveling salesman problem. In Proceedings of the EvoCOP2006, Budapest, Hungary, 10–12 April 2006; pp. 99–110. [Google Scholar]
- Parvin, M.; Yousefi, H.; Noorollahi, Y. Techno-economic optimization of a renewable micro grid using multi-objective particle swarm optimization algorithm. Energy Convers. Manag. 2023, 277, 116639. [Google Scholar] [CrossRef]
- Baktash, A.; Vahedi, A. Design of a wound core pulse transformer using multiobjective optimization method. IEEE Trans. Plasma Sci. 2015, 43, 857–863. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Heat transfer coefficient () | 15 W/m2k |
| Inner length of the coil (L) | 60 mm |
| Inner width of the coil (W) | 10 mm |
| Distance from the inner to the outer coil (D) | 10 mm |
| Thickness of copper (h) | 0.2 mm |
| Distance between each wire (d) | 0.1 mm |
| Resistivity () | mm |
| Air gap (g) | 1.5 mm |
| Length of the magnet () | 40 mm |
| Width of the magnet () | 10 mm |
| Remanence () | 1.2 T |
| The relative permeability of magnet () | 1.1 |
| Density of the magnet () | |
| Height of the magnets () | 8 mm |
| Constant weight of the stator () | 20 N |
| Room temperature | 25 °C |
| Type | Size | Results | ||
|---|---|---|---|---|
| Turns (turns) | Thickness (mm) | in MATLAB (N/A) | in Radia (N/A) | |
| Figure 4 | 3 | 3.84 | 0.599 | 0.605 |
| 3 | 4.48 | 0.666 | 0.675 | |
| 3 | 5.76 | 0.782 | 0.796 | |
| 3 | 7.68 | 0.917 | 0.936 | |
| 7 | 8.32 | 1.905 | 1.950 | |
| 10 | 8.32 | 2.858 | 2.737 | |
| 13 | 8.32 | 3.810 | 3.647 | |
| Basic Parameter | Values for 1D ML System | Values for 2D ML System |
|---|---|---|
| Length of the magnets () | 40 mm | 16 mm |
| Width of the magnets () | 10 mm | 8 mm |
| Height of the magnets () | 12 mm | 11 mm |
| Constant weight of the stator () | 23 N | 10 N |
| Thickness of the PCB coil () | 9.2 mm | 8.32 mm |
| Total width of one PCB coil () | 30 mm | 48 mm |
| Total length of one PCB coil () | 80 mm | 48 mm |
| Total turns of one PCB coil () | 180 turns | 171 turns |
| Number of layer of PCB | 20 layers | 19 layers |
| Thickness of the copper (h) | 0.2 mm | 0.2 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; He, J.; Xu, X.; Wang, R.; Xu, M.; Xu, F. Numerical Modeling and Structure Optimization for Magnetic Levitation Planar Machine Using PCB Coils. Actuators 2025, 14, 33. https://doi.org/10.3390/act14010033
Zhang H, He J, Xu X, Wang R, Xu M, Xu F. Numerical Modeling and Structure Optimization for Magnetic Levitation Planar Machine Using PCB Coils. Actuators. 2025; 14(1):33. https://doi.org/10.3390/act14010033
Chicago/Turabian StyleZhang, Han, Jiawen He, Xianze Xu, Rui Wang, Manman Xu, and Fengqiu Xu. 2025. "Numerical Modeling and Structure Optimization for Magnetic Levitation Planar Machine Using PCB Coils" Actuators 14, no. 1: 33. https://doi.org/10.3390/act14010033
APA StyleZhang, H., He, J., Xu, X., Wang, R., Xu, M., & Xu, F. (2025). Numerical Modeling and Structure Optimization for Magnetic Levitation Planar Machine Using PCB Coils. Actuators, 14(1), 33. https://doi.org/10.3390/act14010033







