Comprehensive Analysis and Development of Electric-Drive-Wheel with Idler Gear
Abstract
1. Introduction
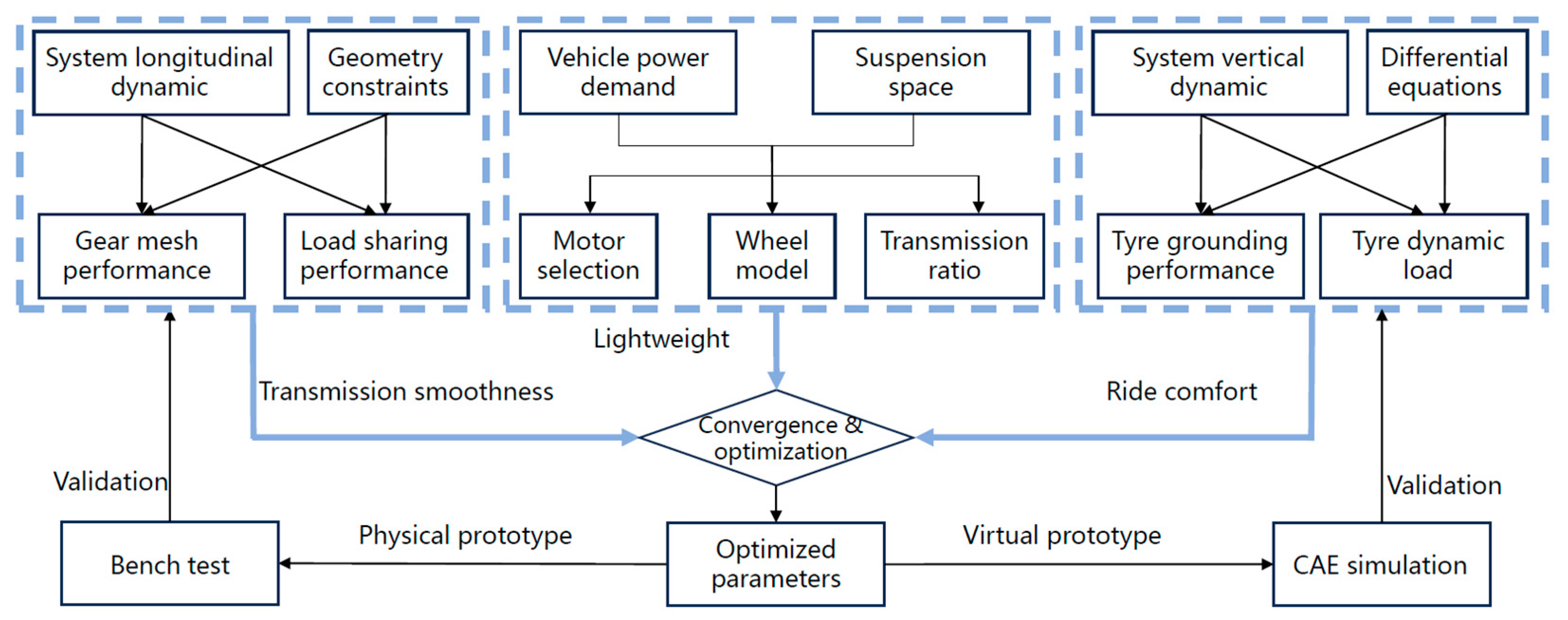
2. Research Objective
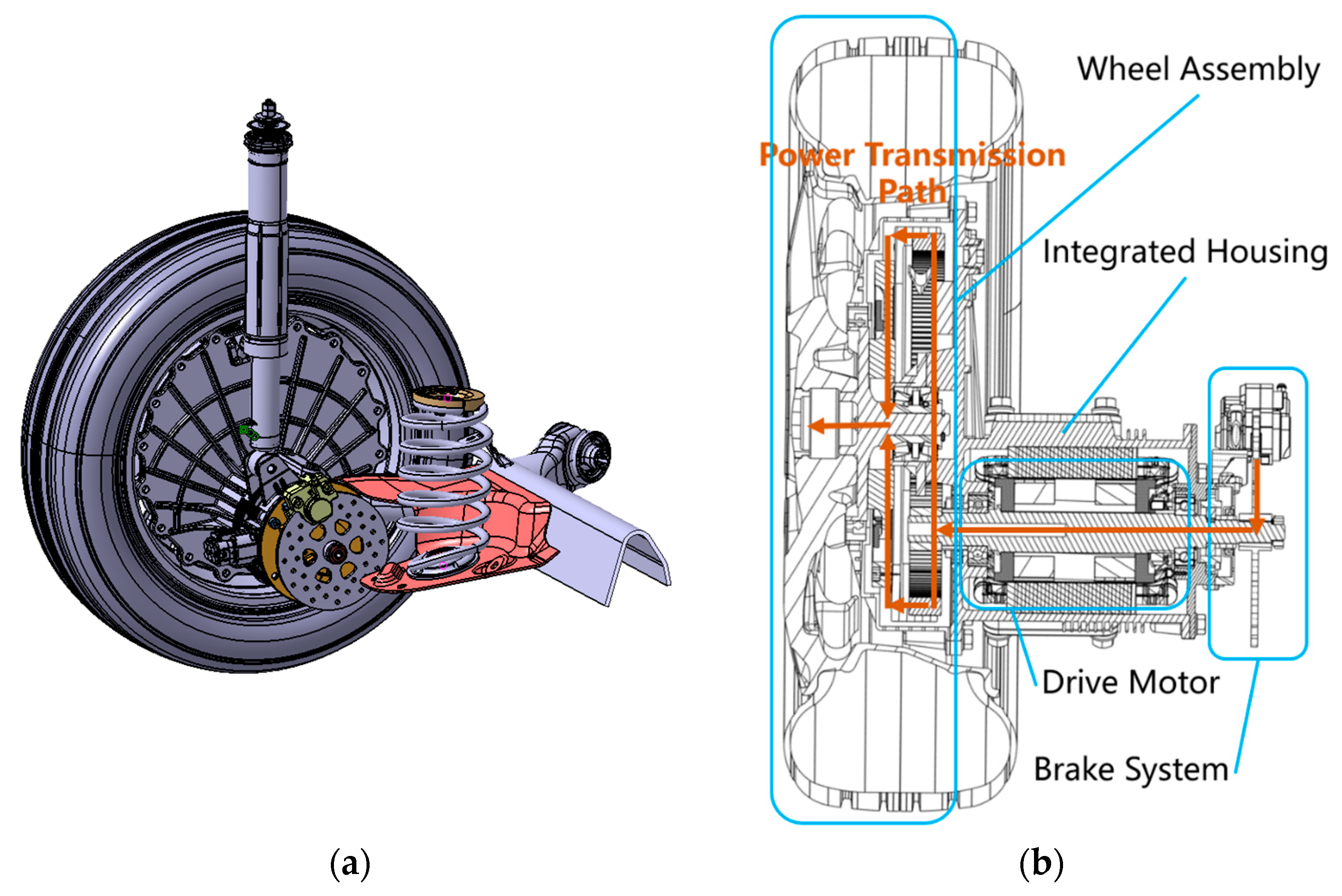
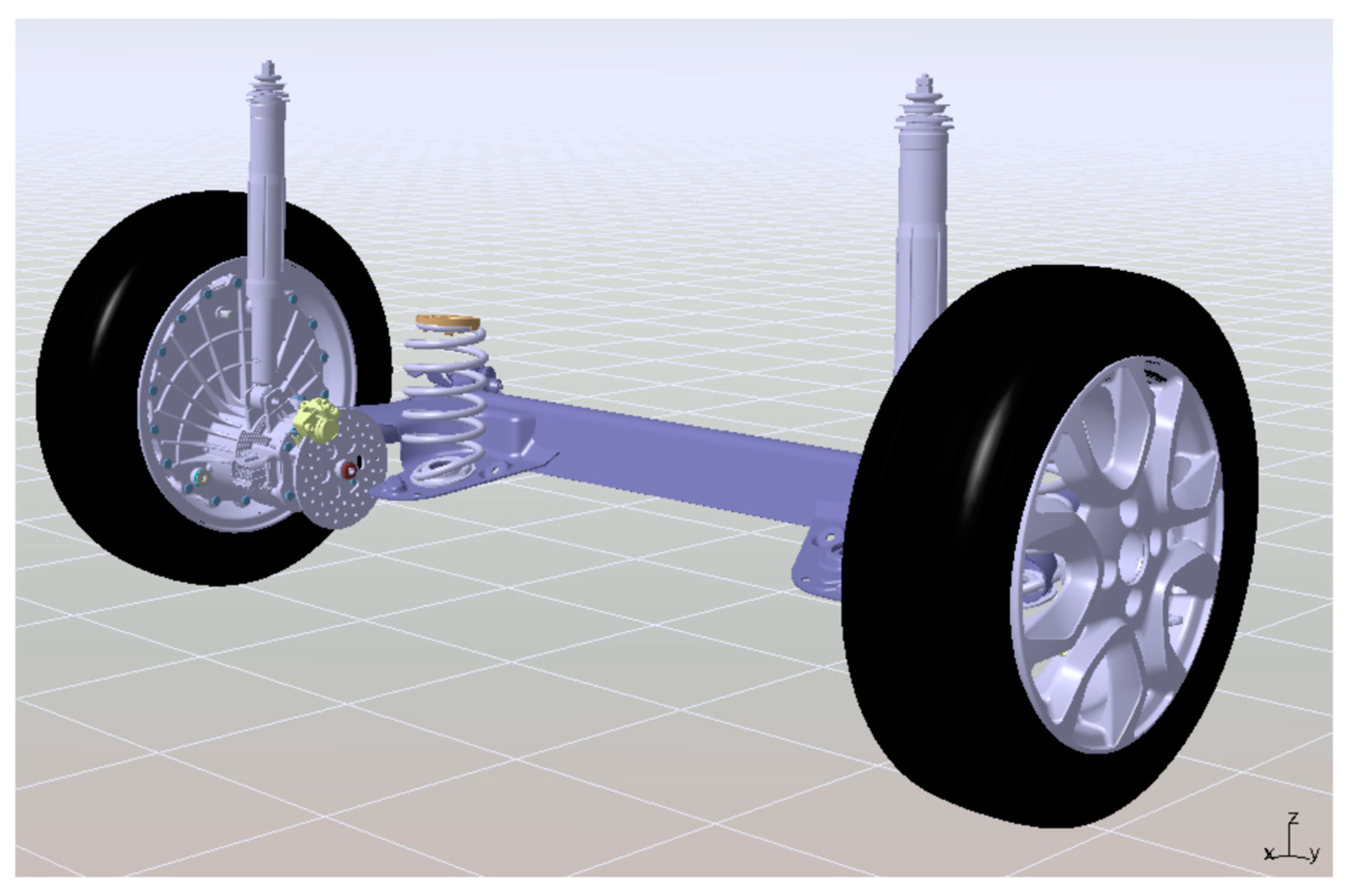
2.1. Basic Structure
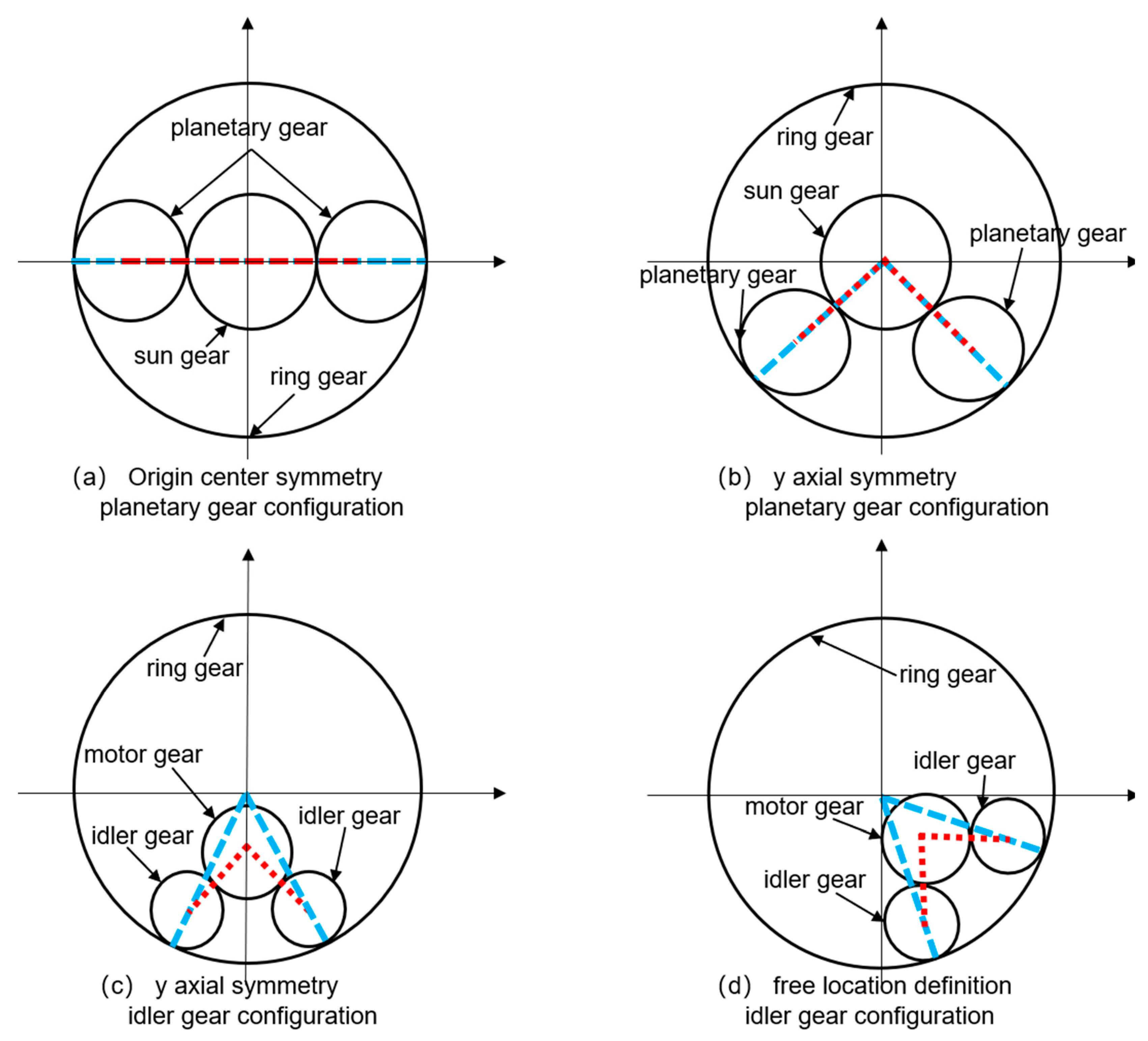
2.2. Configuration Evolution
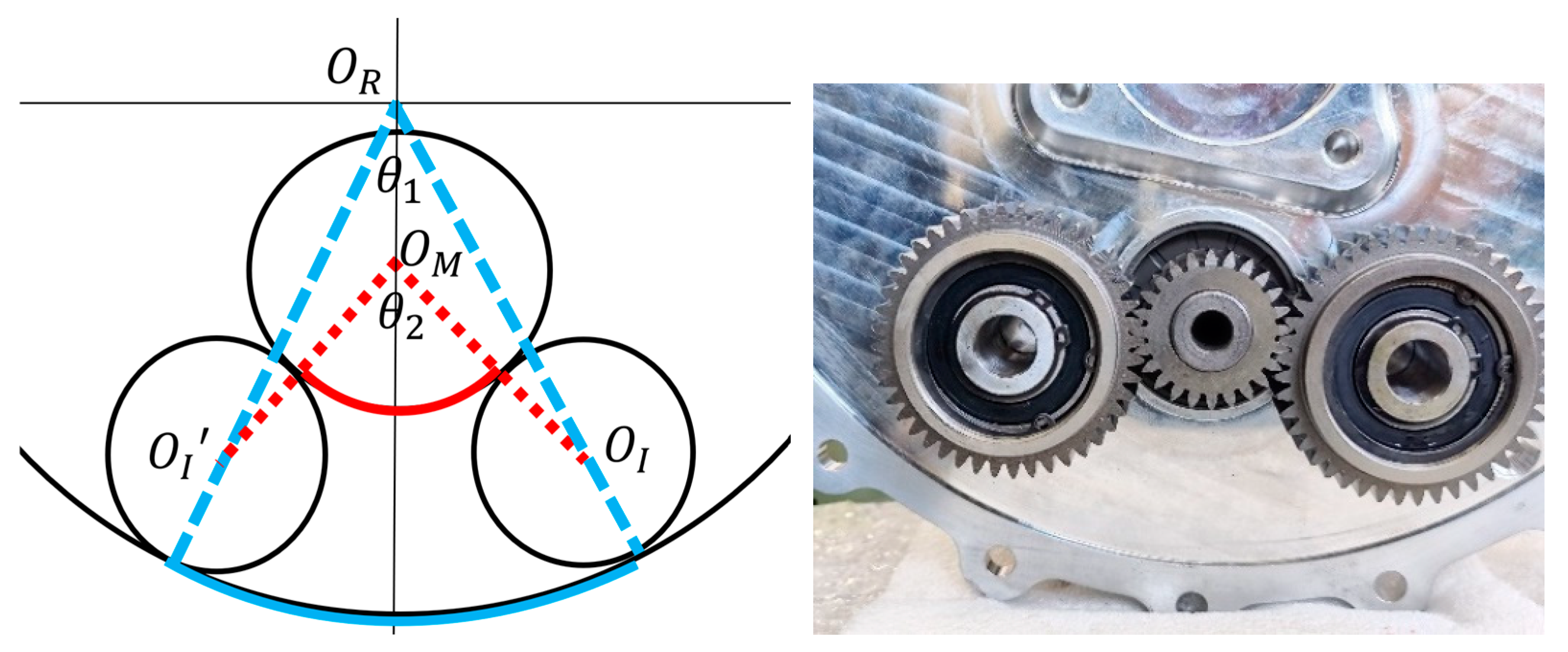
2.3. Constraint Conditions
3. Longitudinal Characteristic Analysis
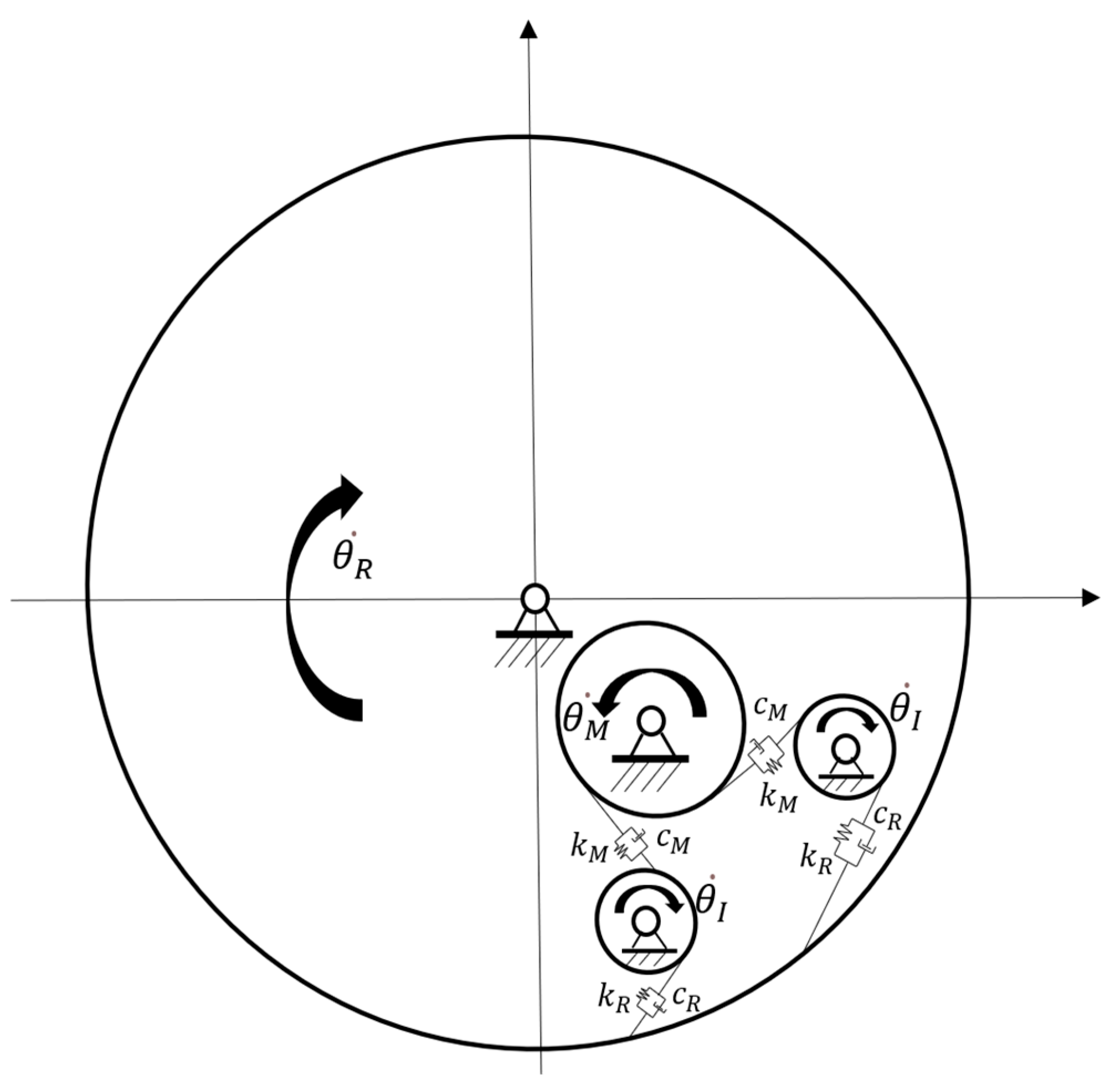
3.1. Power System Model
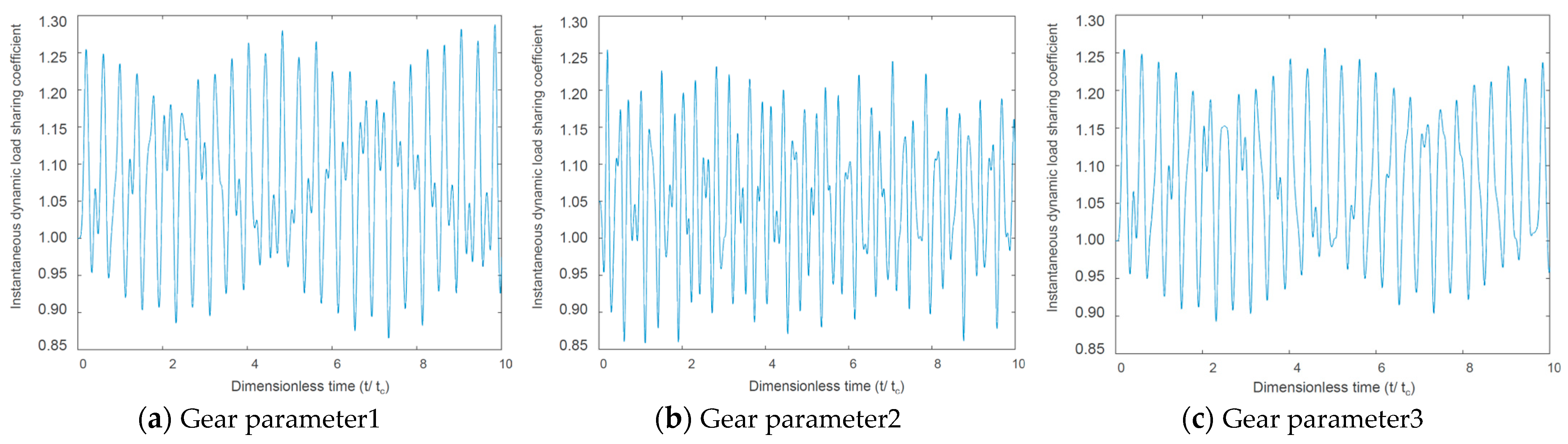
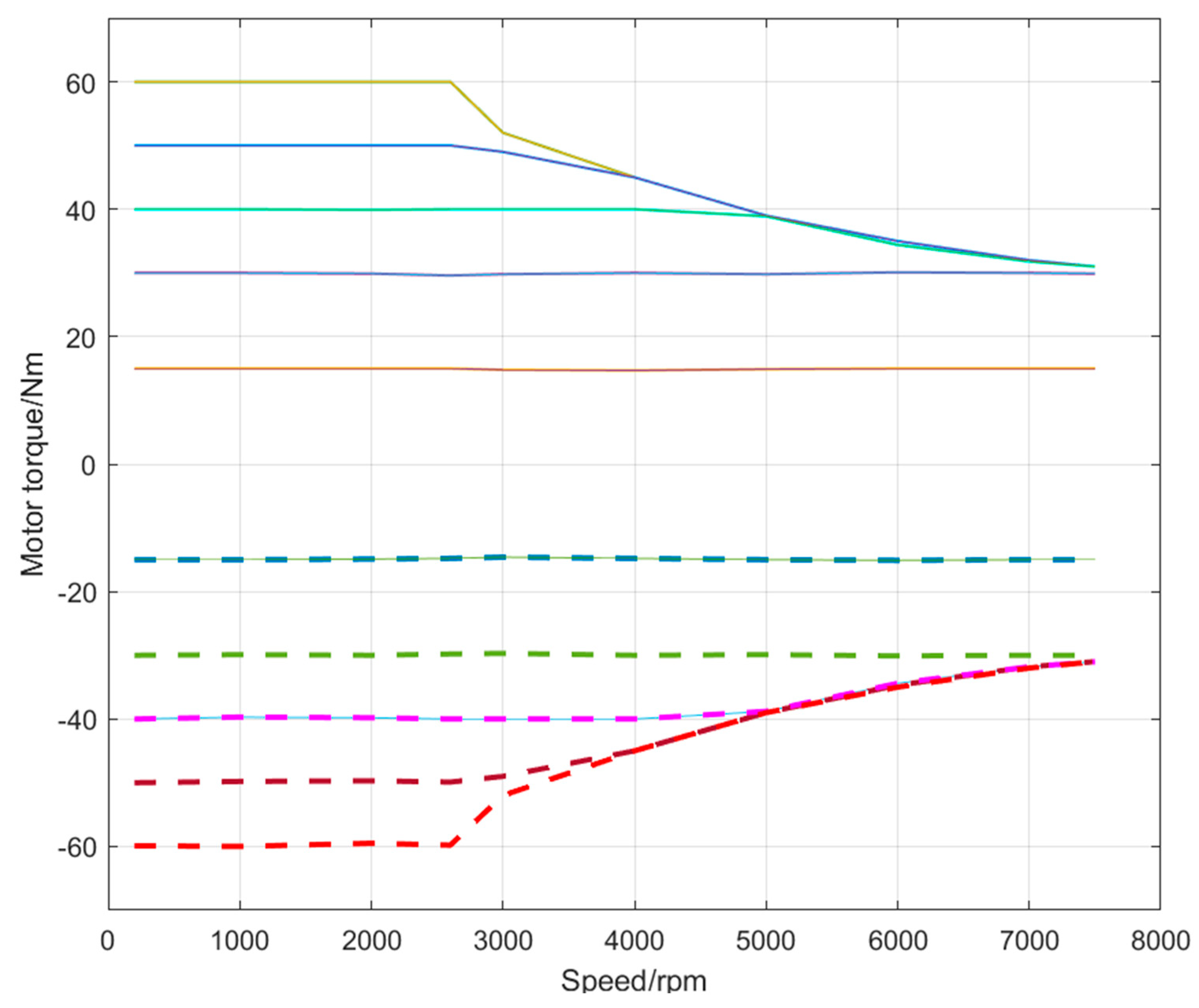
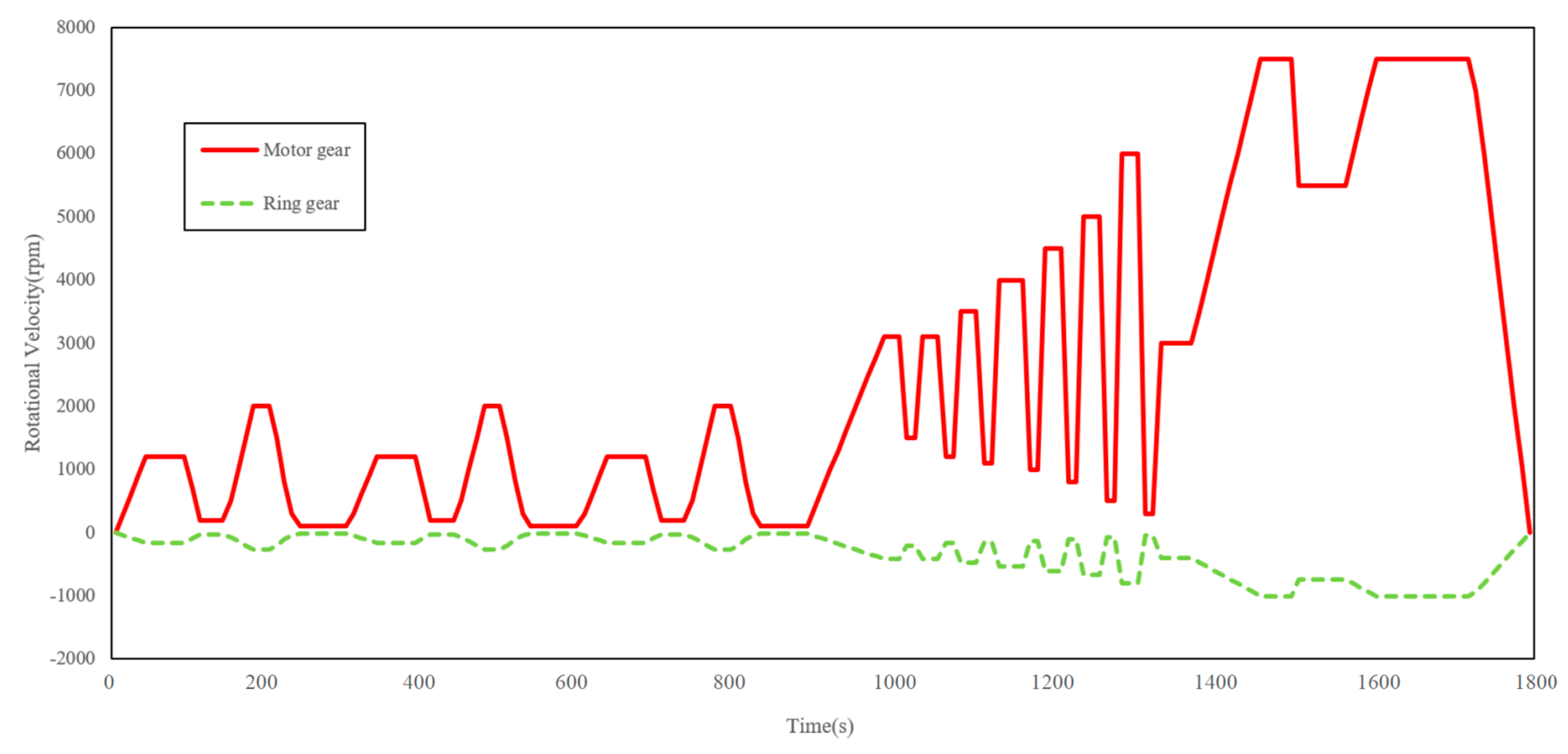
3.2. Load-Sharing Performance
4. Vertical Characteristic Analysis
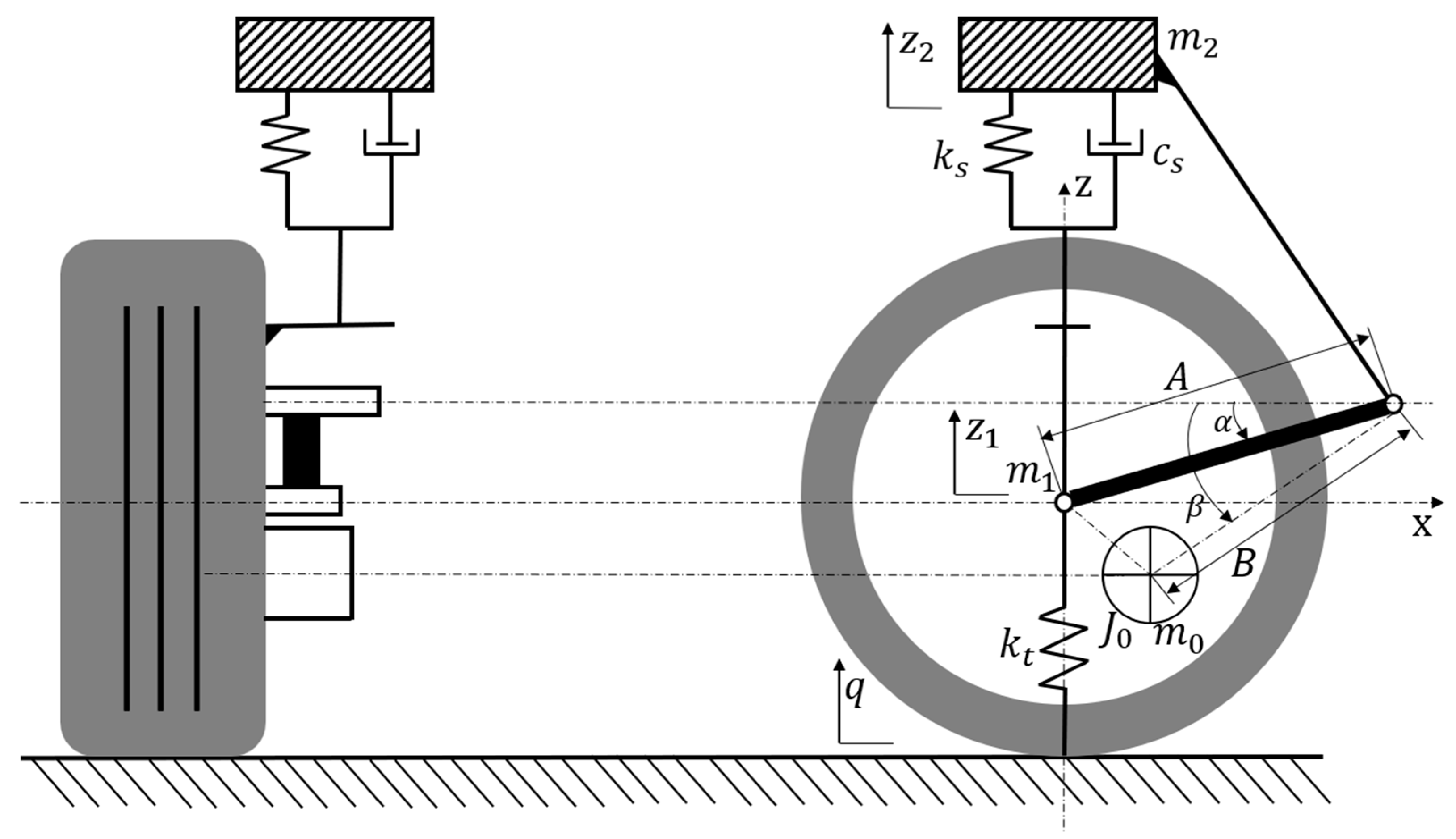
4.1. Vertical Vibration Model
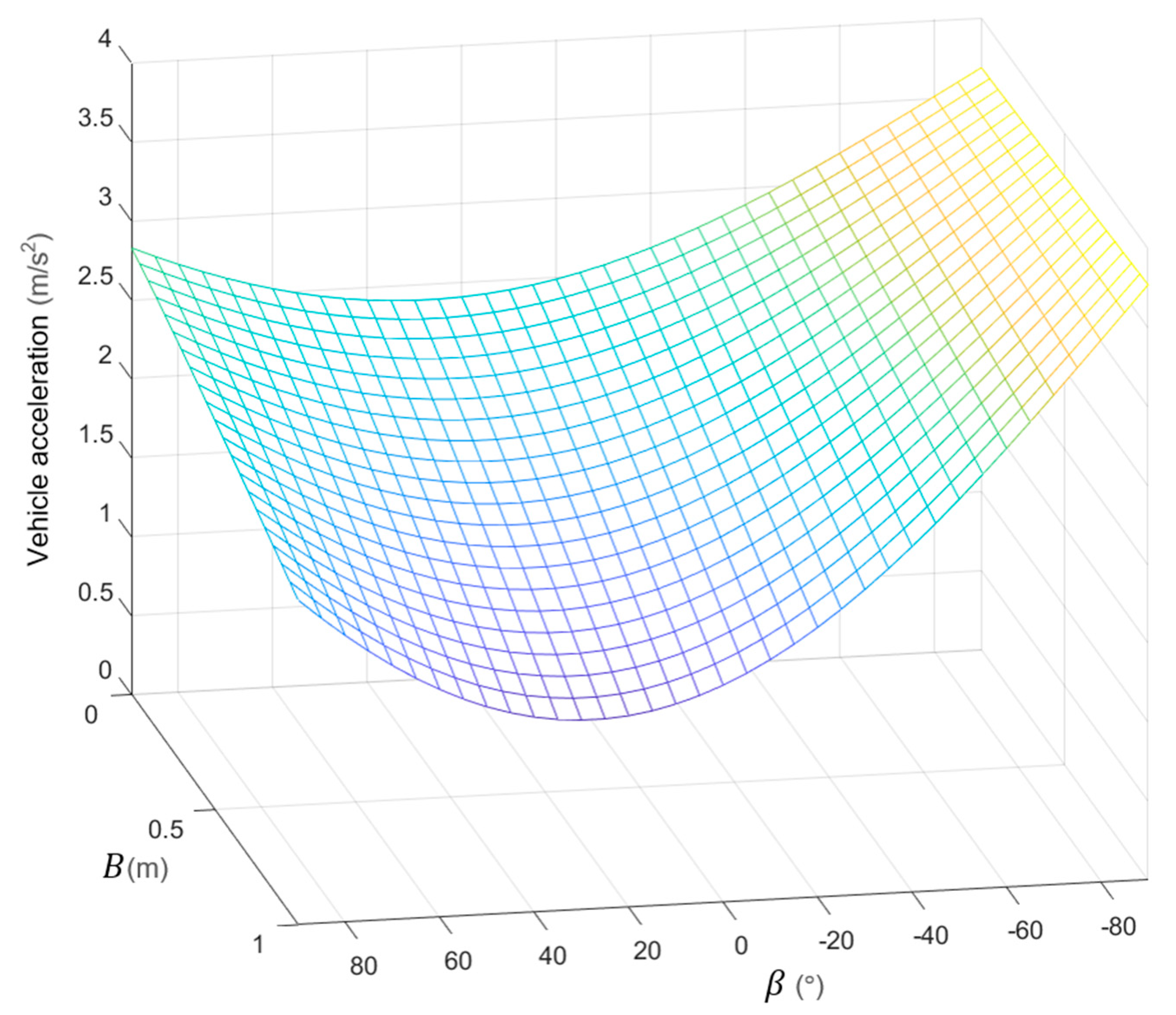
4.2. Dynamic Analysis
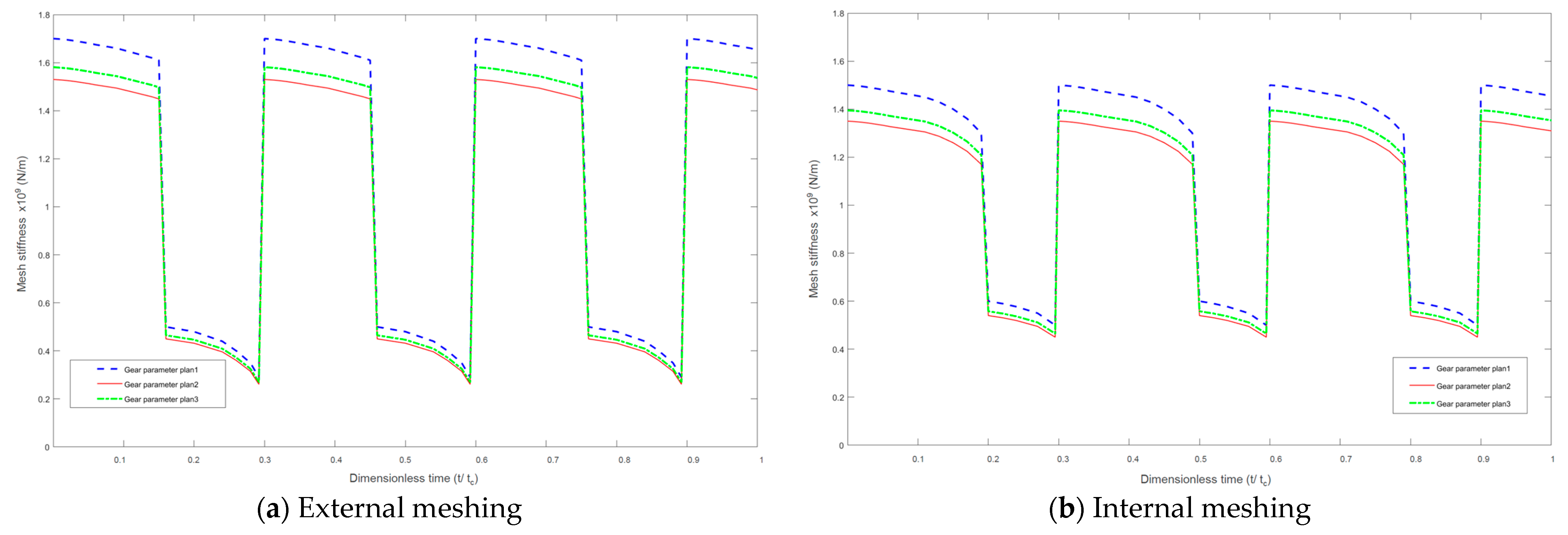
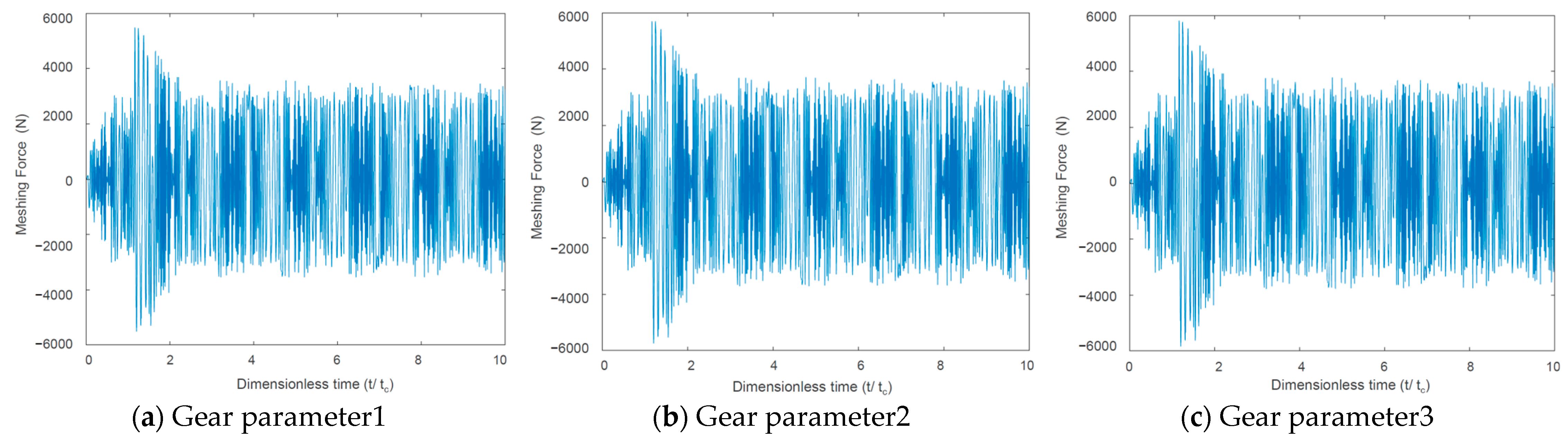
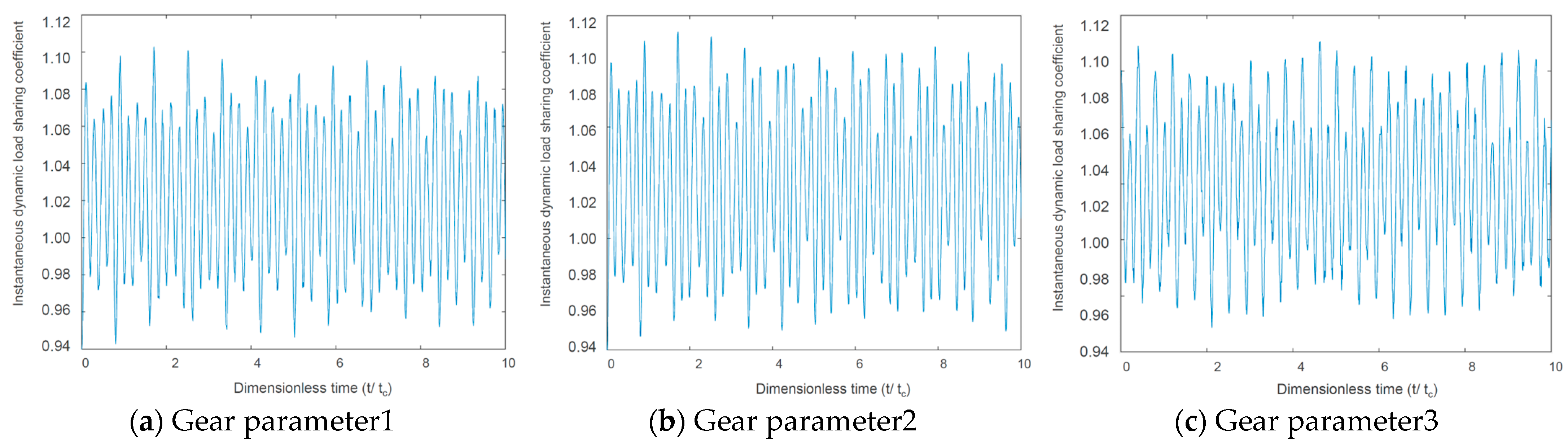
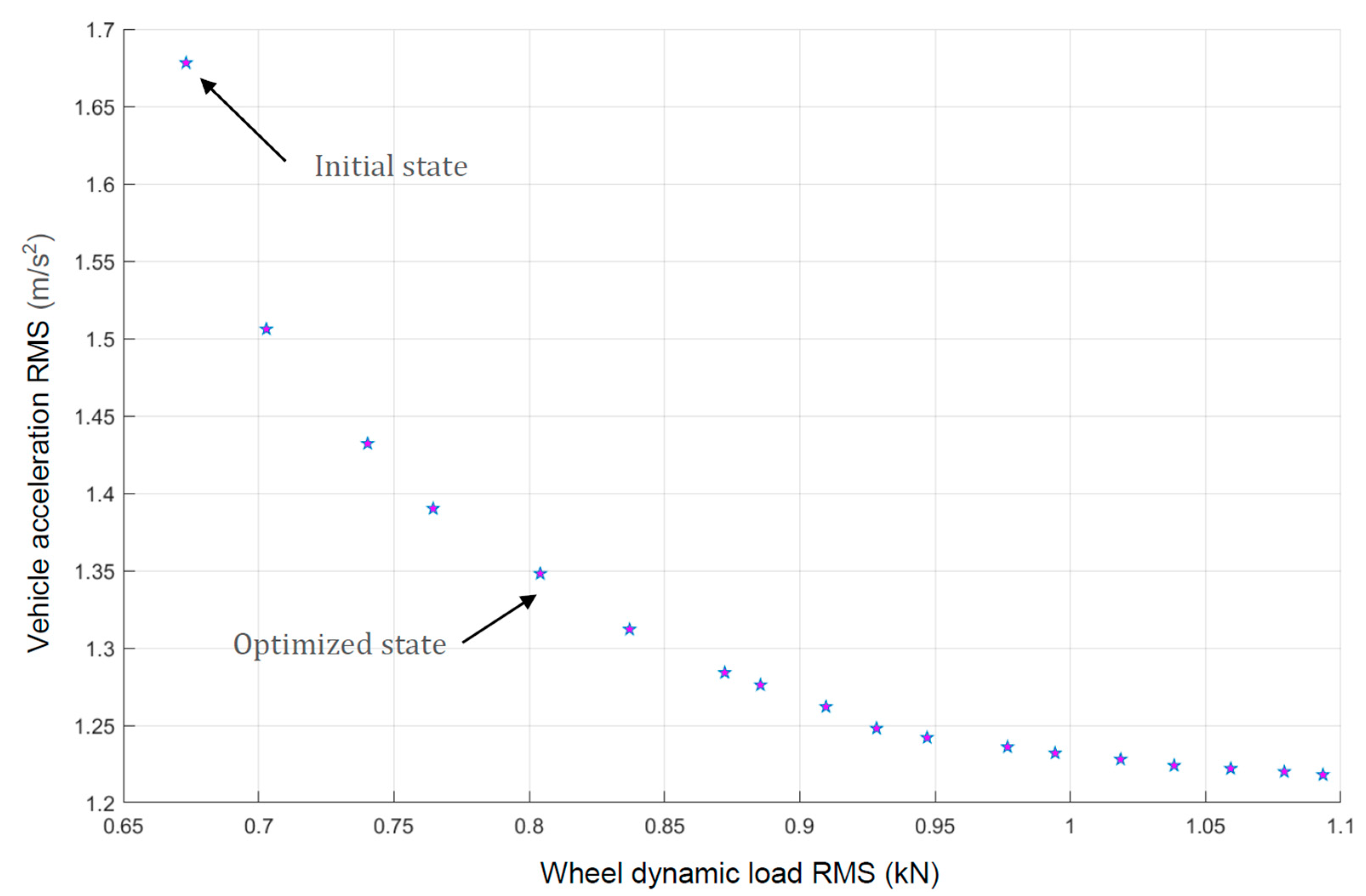
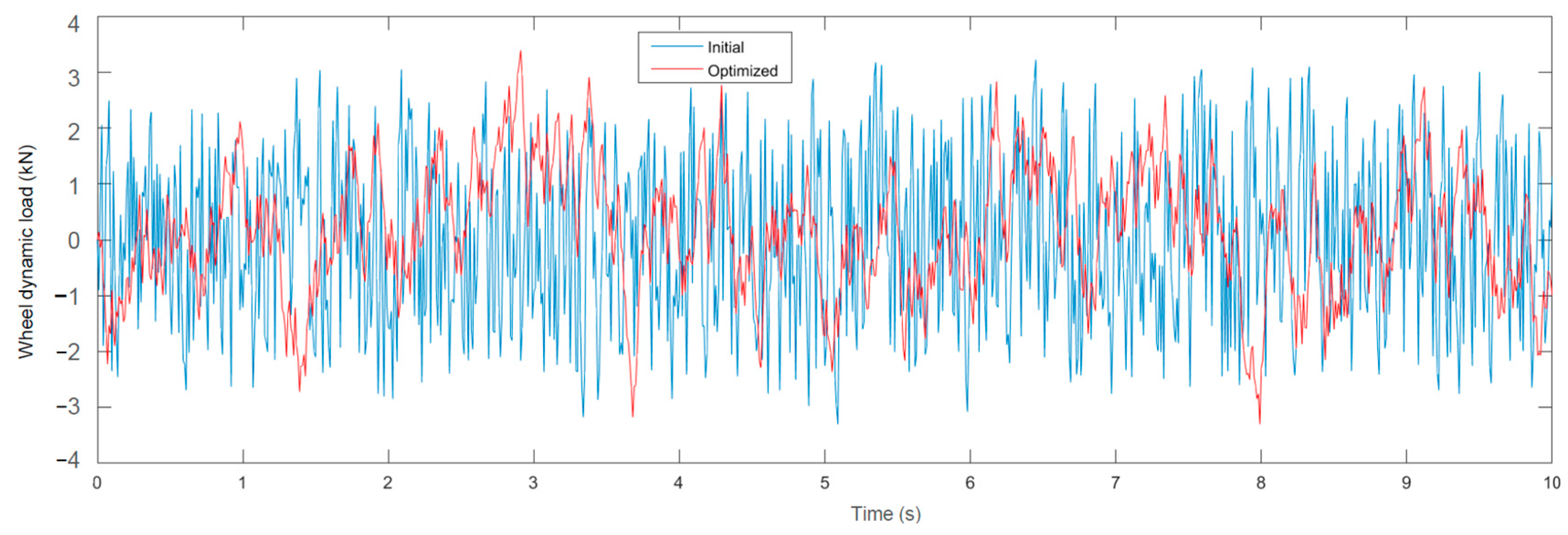
4.3. Parameter Optimization
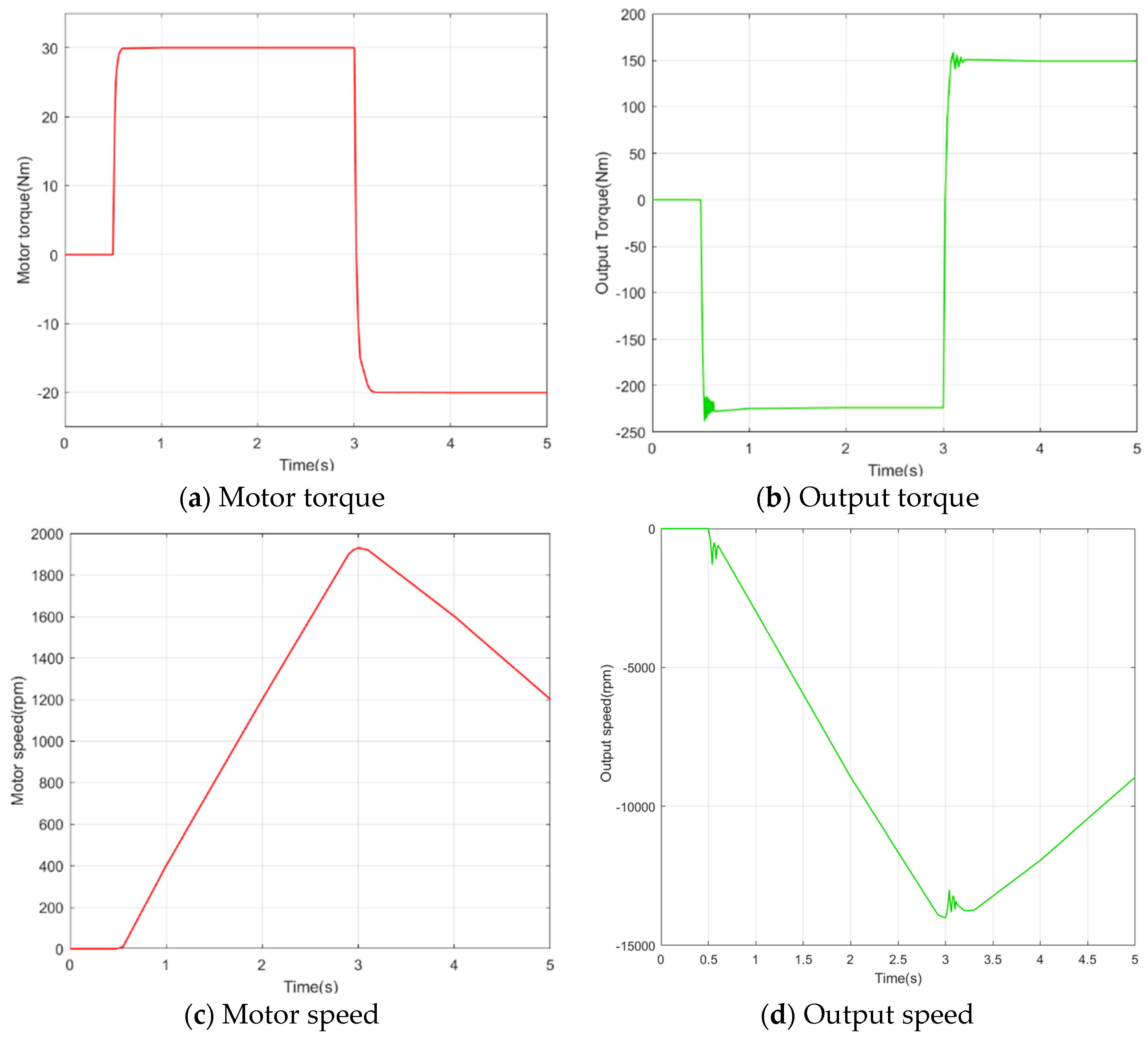
4.4. Simulation Analysis
5. Prototype and Test
6. Conclusions
7. Patents
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Agamloh, E.; Jouanne, A.V.; Yokochi, A. An overview of electric machine trends in modern electric vehicles. Machines 2020, 8, 20. [Google Scholar] [CrossRef]
- Boldea, I.; Tutelea, L.N.; Parsa, L. Automotive electric propulsion systems with reduced or no permanent magnets: An overview. IEEE Trans. Ind. Electron. 2014, 61, 5696–5711. [Google Scholar] [CrossRef]
- Hilton, C. Wheel Torque and Speed in a Vehicle with In-Wheel Motors; Protean Electric Ltd.: Farnham, UK, 2024; p. 8. [Google Scholar]
- Biek, M.; Connes, R.; Omerovi, S. The bearing stiffness effect on In-Wheel Motors. Sustainability 2020, 12, 4070. [Google Scholar] [CrossRef]
- De Santis, M.; Agnelli, S.; Patanè, F.; Giannini, O.; Bella, G. Experimental study for the assessment of the measurement uncertainty associated with electric powertrain efficiency using the back-to-back direct method. Energies 2018, 11, 3536. [Google Scholar] [CrossRef]
- Pedro, J.; Weinholtz, J.; Sousa, L.; Reis, L. A New Concept for a wheel-embedded assembly for electric vehicles. J. Mech. Des. 2013, 135, 124502. [Google Scholar] [CrossRef]
- Drexler, D.; Hou, Z. Simulation analysis on vertical vehicle dynamics of three in-wheel motor drive configurations. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2023, 09544070231163925. [Google Scholar] [CrossRef]
- Ciobotaru, T.; Grigore, L.S.; Barothi, L. Kinematic and dynamic analysis of a planetary gear box in steady-state working regime. IOP Conf. Ser. Mater. Sci. Eng. 2024, 1303, 012015. [Google Scholar] [CrossRef]
- Jian, L.; Chau, K.T. Design and analysis of an integrated Halbach-magnetic-geared permanent-magnet motor for electric vehicles. J. Asian Electr. Veh. 2009, 7, 1213–1219. [Google Scholar] [CrossRef]
- Shi, Y.; Wei, J.; Deng, Z.; Jian, L. A novel electric vehicle powertrain system supporting multi-path power flows: Its architecture, parameter determination and system simulation. Energies 2017, 10, 216. [Google Scholar] [CrossRef]
- Shi, Y.; Jian, L. A novel Dual-Permanent-Magnet-Excited Machine with Flux Strengthening Effect for low-speed large-torque applications. Energies 2018, 11, 153. [Google Scholar] [CrossRef]
- Jian, L.; Deng, Z.; Shi, Y.; Wei, J.; Chan, C.C. The mechanism how coaxial magnetic gear transmits magnetic torques between its two rotors: Detailed analysis of torque distribution on modulating ring. IEEE/ASME Trans. Mechatron. 2019, 24, 763–773. [Google Scholar] [CrossRef]
- Perovic, D.K. Making the impossible, possible–overcoming the design challenges of In-Wheel Motors. World Electr. Veh. J. 2012, 5, 514–519. [Google Scholar] [CrossRef]
- Theling, J.; Brimmers, J.; Brecher, C. Reducing mass while improving the operational behavior form optimization of planetary gearbox housings. Forsch. Im Ingenieurwesen 2021, 85, 543–551. [Google Scholar] [CrossRef]
- Kahraman, A. Load sharing characteristics of planetary transmissions. Mech. Mach. Theory 1994, 29, 1151–1165. [Google Scholar] [CrossRef]
- Howard, S.I. Calculation of the combined torsional mesh stiffness of spur gears with two-and three-dimensional parametrical FE Models. J. Mech. Eng. 2011, 57, 810–818. [Google Scholar]
- Xue, S.; Howard, I. Ring-planet mesh stiffness study with different boundary conditions and crack locations. In Proceedings of the ASME International Design Engineering Technical Conferences & Computers & Information in Engineering Conference 2015, Boston, MA, USA, 2–5 August 2015. [Google Scholar] [CrossRef]
- Chen, X.; Yin, J.; Wang, W.; Wu, L.; Tang, F. Approaches to diminish large unsprung mass negative effects of wheel side drive electric vehicles. J. Adv. Mech. Des. Syst. Manuf. 2016, 10, JAMDSM0064. [Google Scholar] [CrossRef]
- Wang, W.; Chen, X. Design methodology for wheel corner module topology based on position and orientation characteristics. Mech. Mach. Theory 2019, 136, 122–140. [Google Scholar] [CrossRef]
- Qin, Y.; Wang, Z.; Yuan, K. Comprehensive analysis and optimization of dynamic vibration-absorbing structures for electric vehicles driven by In-Wheel Motors. Automot. Innov. 2020, 3, 192. [Google Scholar] [CrossRef]
- Abe, W.A. Development of an in-wheel drive with advanced dynamic-damper mechanism. JSAE Rev. 2003, 24, 477–481. [Google Scholar] [CrossRef]
- Frajnkovic, M.; Omerovic, S.; Rozic, U. Structural integrity of In-Wheel Motors. In Proceedings of the International Powertrains, Fuels & Lubricants Meeting, Heidelberg, Germany, 17–20 October 2018; Volume 1, p. 11. [Google Scholar]
- Ding, X.Y.; Kong, A.; Chen, X. An Electric Drive Wheel That Can Sense the Wheel Load in Real Time with the Sensing System. Chinese Patent CN117774559A, 29 March 2024. [Google Scholar]
- Hu, Y.; Talbot, D.; Kahraman, A. A gear load distribution model for a planetary gear set with a flexible ring gear having external splines. J. Mech. Des. 2018, 141, 053301. [Google Scholar] [CrossRef]
- Zhao, N.; Wang, L.I. A general mathematical design method of the torque-split gear transmission with idler pinion. J. Adv. Mech. Des. Syst. Manuf. 2018, 12, JAMDSM0127. [Google Scholar] [CrossRef]
- Cardon, A.; Tordion, G.V. Numerical implementation of complex potentials for gear tooth stress analysis. ASME J. Mech. Des. 1981, 103, 460–465. [Google Scholar]
- Comell, R.W. Compliance and stress sensitivity of spur gear teeth. ASME J. Mech Des. 1981, 103, 447–459. [Google Scholar]
- Liu, H.; Zhong, Z.; Jing, H. Simulation on the dynamic characteristics of In-Wheel-Motor powertrain in distributed drive electric vehicle. Automot. Eng. 2014, 36, 597–602. [Google Scholar]
- Kahraman, A.; Ligata, H.; Singh, A. Influence of ring gear rim thickness on planetary gear set behavior. J. Mech. Des. 2010, 132, 021002. [Google Scholar] [CrossRef]
- Hedlund, J.; Lehtovaara, A. A parameterized numerical model for the evaluation of gear mesh stiffness variation of a helical gear pair. Drive Syst. Tech. 2008, 222, 1321–1327. [Google Scholar] [CrossRef]
- Sánchez, M.B.; Pleguezuelos, M.; Pedrero, J.I. Approximate equations for the meshing stiffness and the load sharing ratio of spur gears including hertzian effects. Mech. Mach. Theory 2017, 109, 231–249. [Google Scholar] [CrossRef]
- Kahraman, M.I. A theoretical and experimental investigation of modulation sidebands of planetary gear sets. J. Sound Vib. 2009, 323, 677–696. [Google Scholar] [CrossRef]
- Kim, W.; Lee, J.Y.; Chung, J. Dynamic analysis for a planetary gear with time-varying pressure angles and contact ratios. J. Sound Vib. 2012, 331, 883–901. [Google Scholar] [CrossRef]
- Kahraman, A. Static load sharing characteristics of transmission planetary gear sets: Model and experiment. SAE Trans. 1999, 108, 1954–1963. [Google Scholar] [CrossRef]
- Chen, X.; Wang, W.; Wang, X. Research on vertical dynamics of electric drive systems with inhibition of negative effects of unsprung mass. J. Auto. Eng. 2014, 3, 430–437. [Google Scholar]
- Ning, G.; Wan, G. The present research situation of the influences on vehicle vertical performances induced by direct wheel drives system. Auto. Technol. 2007, 3, 21–25. [Google Scholar]
- Andrés, E.; Rojas, R.; Niederkofler, H.; Willberger, J. Comfort and Safety Enhancement of PassengerVehicles with In-Wheel Motors; SAE Technical Papers; SAE International: Warrendale, PA, USA, 2010. [Google Scholar] [CrossRef]






| Parameters (Unit) | Value |
|---|---|
| the vehicle weight (kg) | 1400 |
| Front/rear load distribution | 50%/50% |
| Tire model | 175 60/R15 |
| the rolling radius of the wheel (mm) | 295.5 |
| Motor type | PMSM |
| Motor peak power (kW) | 15 |
| Motor peak torque (Nm) | 60 |
| Motor rated speed (rpm) | 2600 |
| Motor peak speed (rpm) | 7500 |
| Parameter (Unit) | Motor Gear | Idler Gear | Ring Gear |
|---|---|---|---|
| Gear Number | 1 | 2 | 1 |
| Gear Teeth Number | Plan1: 18 | Plan1: 33 | Plan1: 133 |
| Plan2: 24 | Plan2: 44 | Plan2: 179 | |
| Plan3: 36 | Plan3: 65 | Plan3: 269 | |
| Module (mm) | Plan1: 1 | Plan1: 1 | Plan1: 1 |
| Plan2: 1.5 | Plan2: 1.5 | Plan2: 1.5 | |
| Plan3: 2 | Plan3: 2 | Plan3: 2 | |
| Pressure Angle (°) | 20 | 20 | 20 |
| Teeth Width (mm) | 24.5 | 24.5 | 24.5 |
| Addendum coefficient | 1.25 | 1.25 | 1.25 |
| Elastic modulus (GPa) | 206 | 206 | 206 |
| Mass (kg) | Plan1: 0.13 | Plan1: 0.34 | Plan1: 1.92 |
| Plan2: 0.13 | Plan2: 0.35 | Plan2: 1.99 | |
| Plan3: 0.14 | Plan3: 0.38 | Plan3: 2.11 | |
| Moment of inertia (kgm2) | Plan1: 2.07 × 10−5 | Plan1: 1.47 × 10−4 | Plan1: 0.021 |
| Plan2: 2.07 × 10−5 | Plan2: 1.48 × 10−4 | Plan2: 0.021 | |
| Plan3: 2.07 × 10−5 | Plan3: 1.49 × 10−4 | Plan3: 0.022 |
| Parameters (Unit) | Value |
|---|---|
| Wheel mass (kg) | 20 |
| Motor mass (kg) | 30 |
| (kg) | 350 |
| Leverage length of suspension arm A (m) | 0.4 |
| Leverage length of electric motor B (m) | 0~1 |
| Initial balance suspension angle (°) | 20 |
| Initial balance motor angle (°) | −90~90 |
| Motor moment of inertia J0 (kg·m2) | 0.2 |
| Vertical stiffness of tire (N/m) | 225,000 |
| Suspension stiffness (N/m) | 25,000 |
| Suspension damping (N·s/m) | 1600 |
| Parameter (Unit) | Initial | Optimized | Change Rate |
|---|---|---|---|
| Suspension stiffness | 25,000 | 21,042 | −15.83% |
| Suspension damping | 2000 | 1598 | −20.10% |
| Vehicle body acceleration RMS (m/s2) | 1.683 | 1.343 | −20.21% |
| Wheel dynamic load RMS (kN) | 0.679 | 0.802 | +18.11% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, X.; Kong, A.; Zhang, J.; Chen, X. Comprehensive Analysis and Development of Electric-Drive-Wheel with Idler Gear. Actuators 2024, 13, 336. https://doi.org/10.3390/act13090336
Ding X, Kong A, Zhang J, Chen X. Comprehensive Analysis and Development of Electric-Drive-Wheel with Idler Gear. Actuators. 2024; 13(9):336. https://doi.org/10.3390/act13090336
Chicago/Turabian StyleDing, Xiaoyu, Aijing Kong, Jiantao Zhang, and Xinbo Chen. 2024. "Comprehensive Analysis and Development of Electric-Drive-Wheel with Idler Gear" Actuators 13, no. 9: 336. https://doi.org/10.3390/act13090336
APA StyleDing, X., Kong, A., Zhang, J., & Chen, X. (2024). Comprehensive Analysis and Development of Electric-Drive-Wheel with Idler Gear. Actuators, 13(9), 336. https://doi.org/10.3390/act13090336





