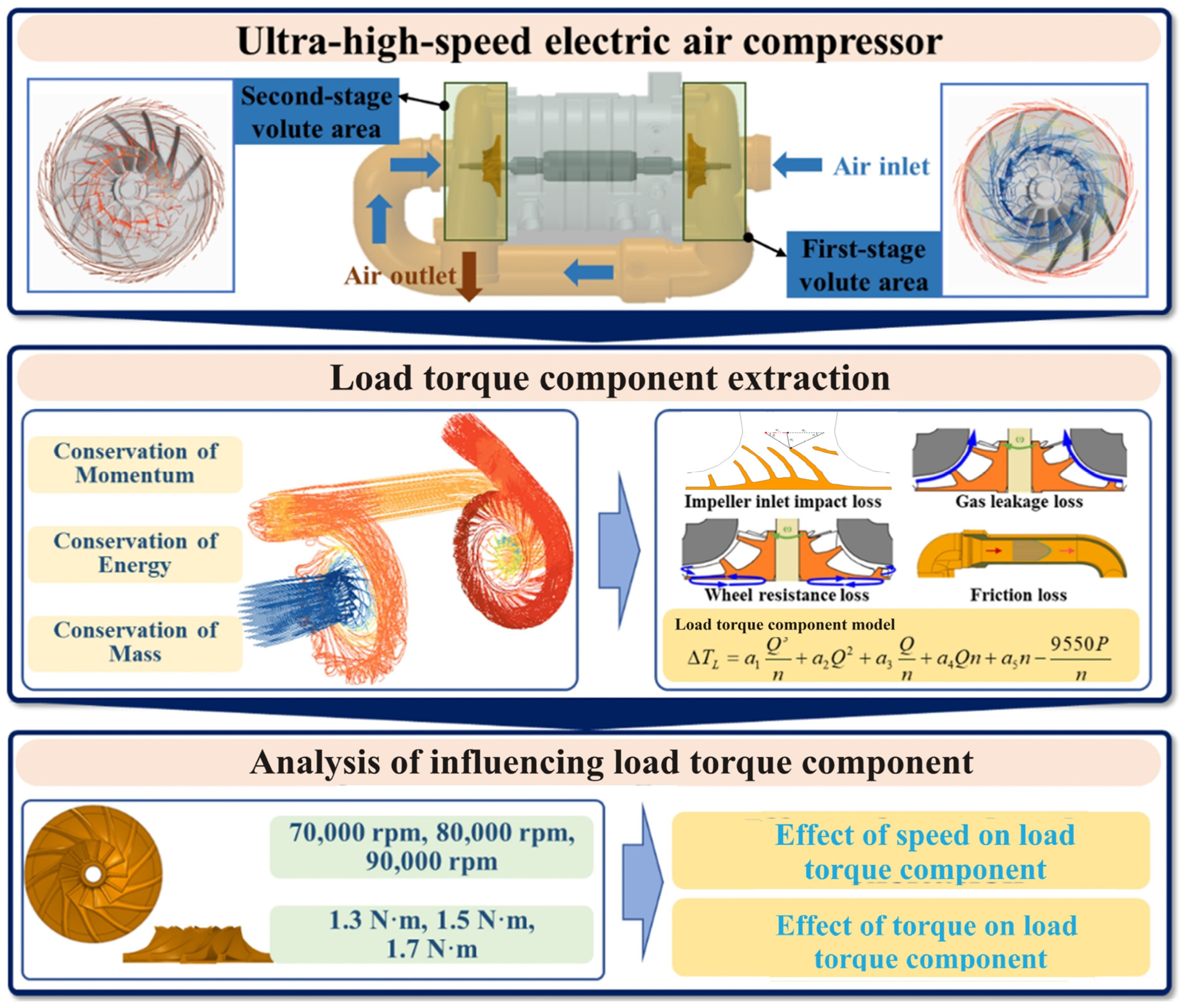
Load Torque Component Extraction and Analysis of Ultra-High-Speed Electric Air Compressors for Fuel Cell Vehicles
Abstract
1. Introduction
- (1)
- Considering the uneven distribution of gas density in the UHSEAC, the fluid model of the UHSEAC is established, the energy variation of the UHSEAC is analyzed, and the load torque component is extracted.
- (2)
- By selecting the working speed and torque of the UHSEAC, the control single variable method is used to analyze the influence of speed and torque on the UHSEAC, and the variation law of the load torque component under UHSOC is revealed.
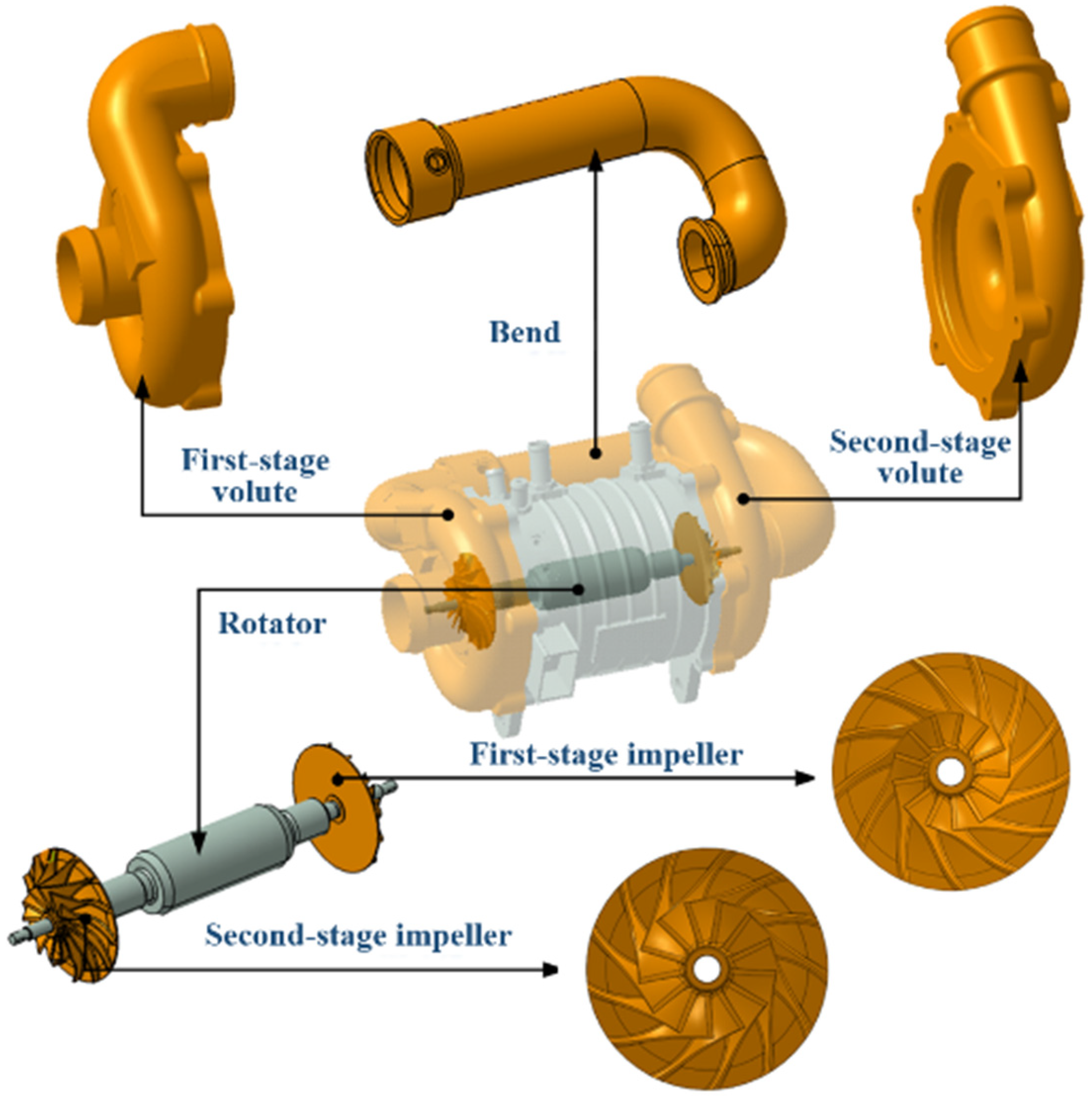
2. Modeling and Verification
2.1. Fluid Model
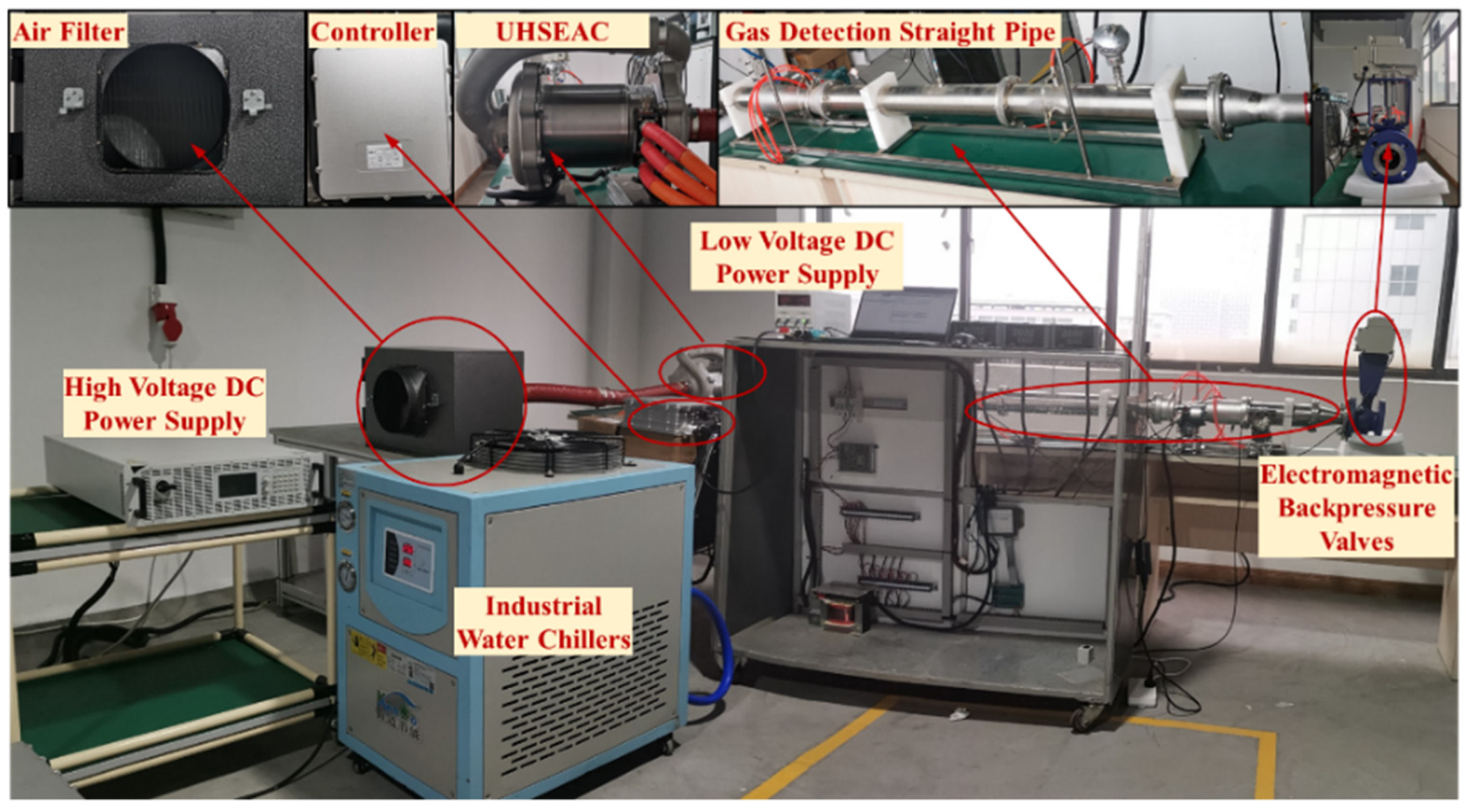
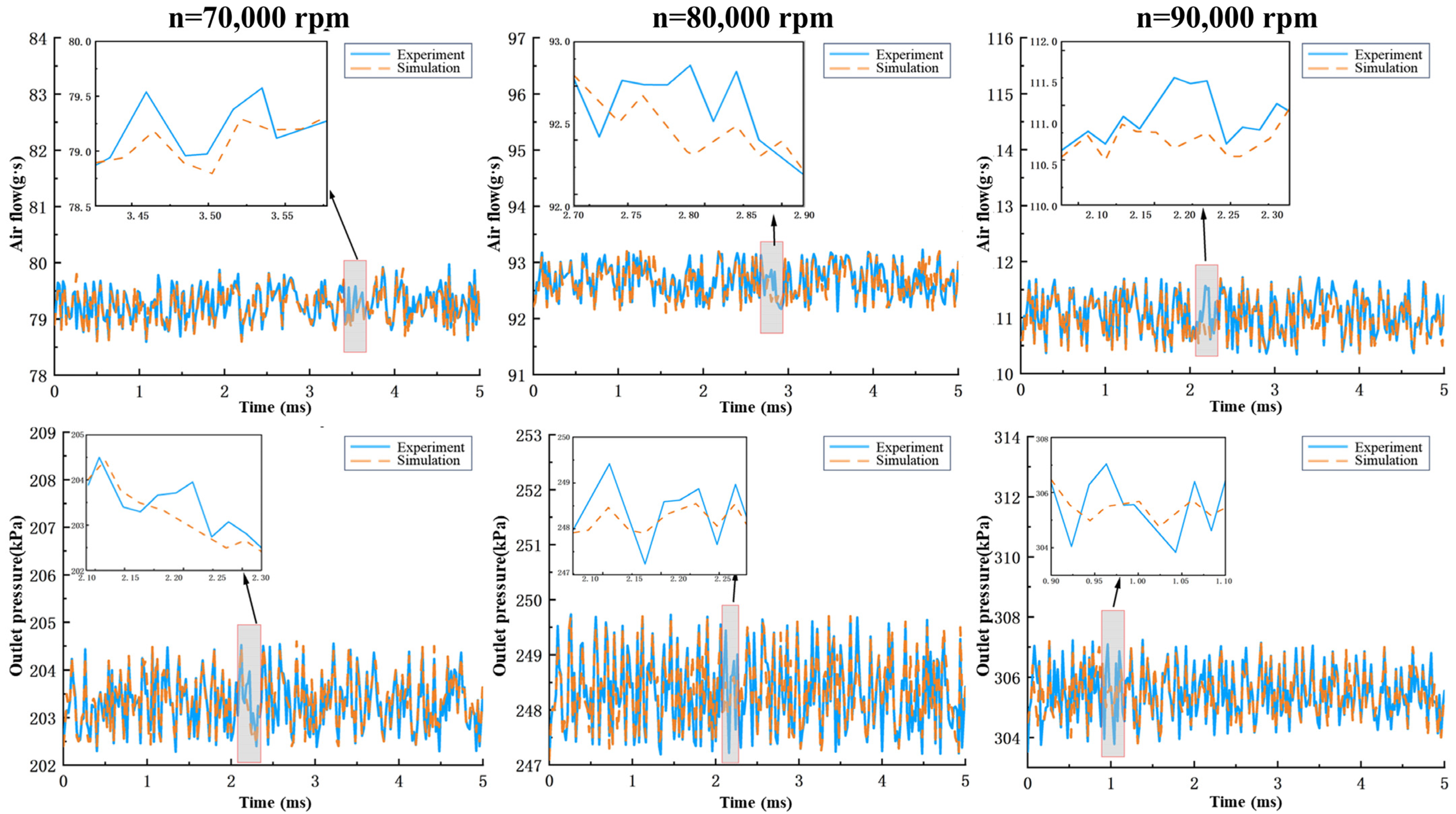
2.2. Experimental Verification
- (1)
- Adjust the opening of the back pressure valve to 36% (known to be greater than the surge opening of the UHSEAC), open the data sending window of the upper computer software, and send the target speed;
- (2)
- Set the sensor acquisition period to 20 ms in the upper computer software, and start data reading after the speed of the air compressor is stable;
- (3)
- The inlet and outlet pressures and flow rates collected during the experiment are saved in the computer;
- (4)
- Continue to set the next target speed and repeat the above steps.
3. Load Torque Component Extraction
4. Load Torque Component Analysis
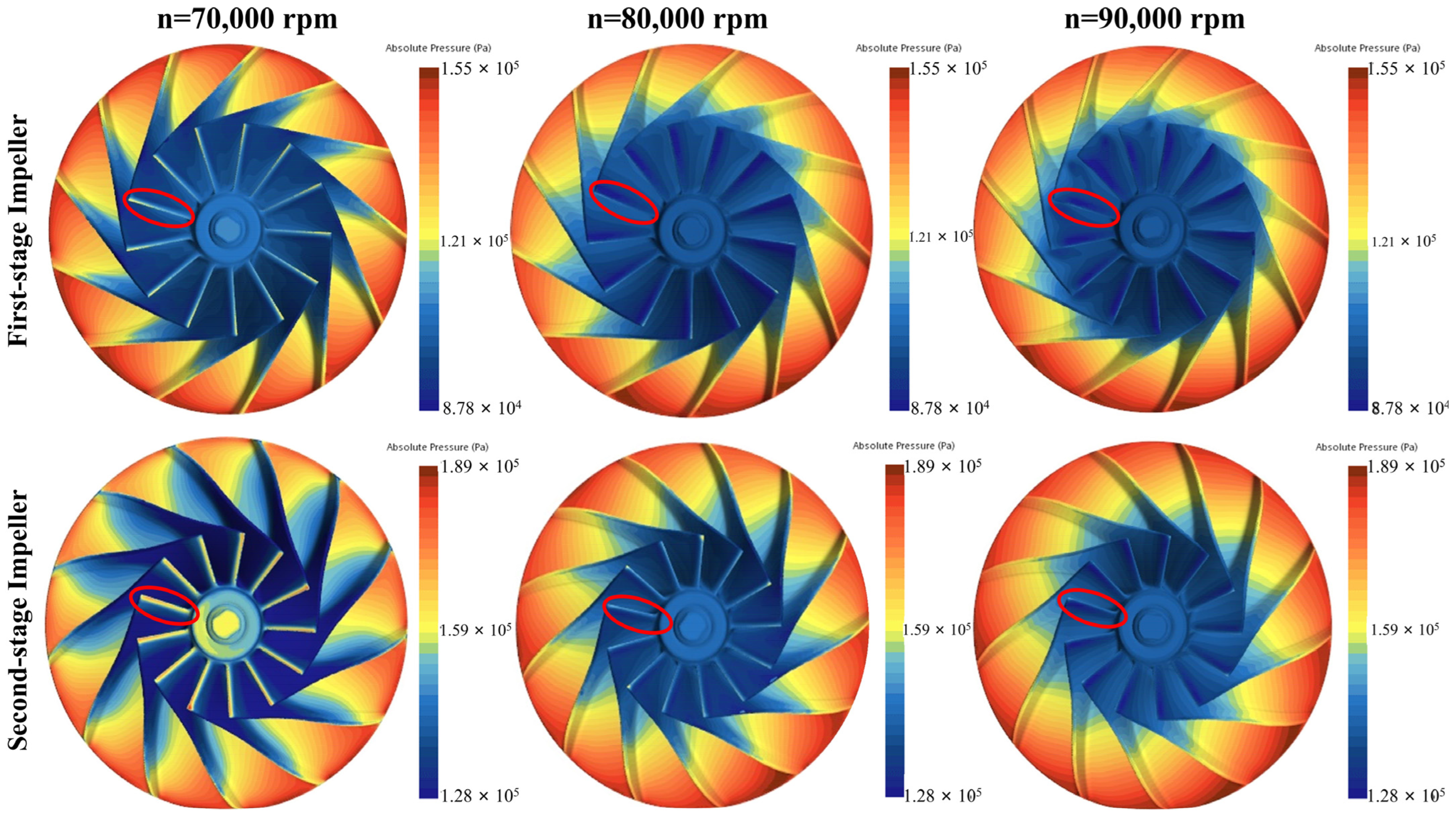
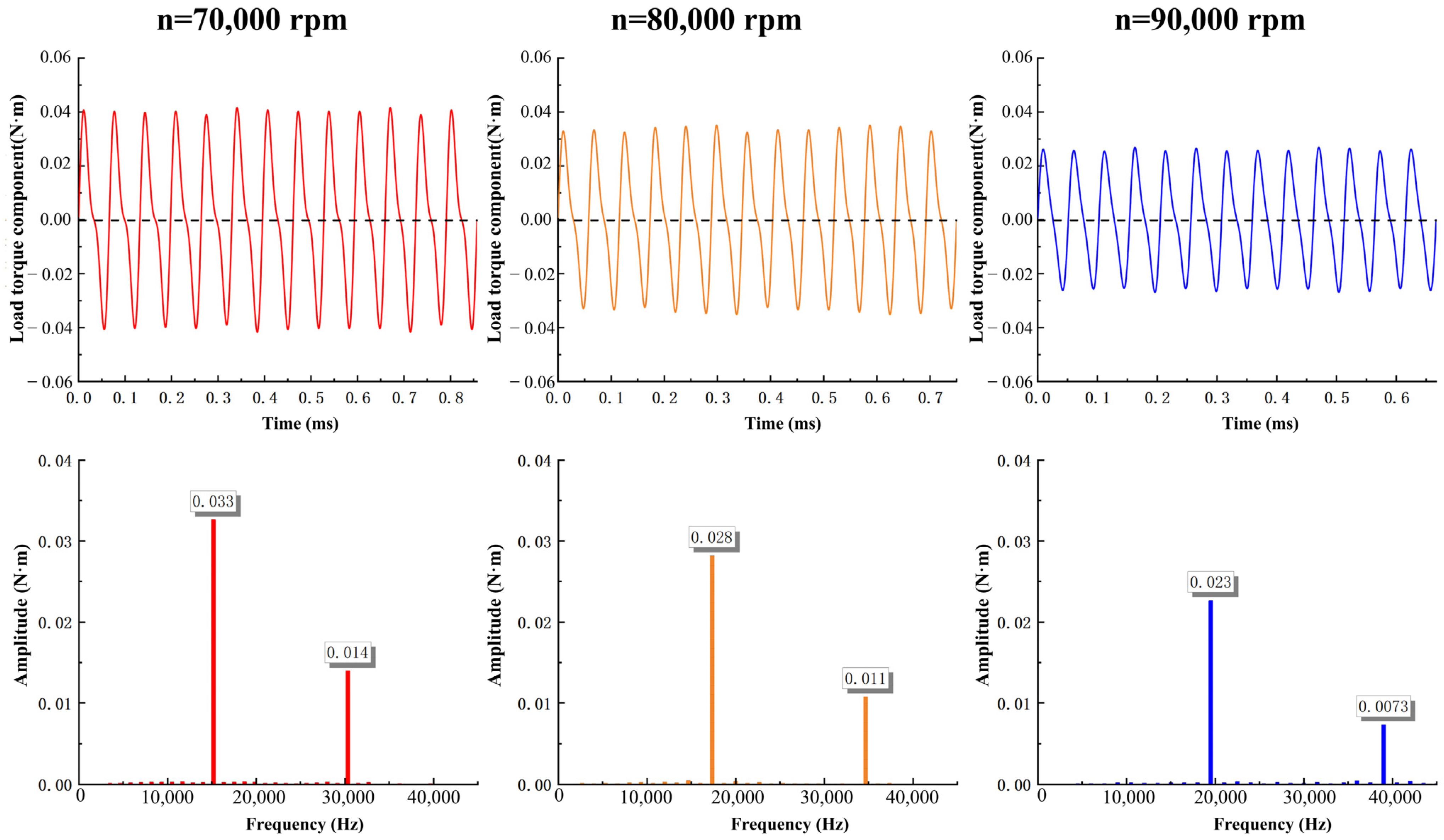
4.1. Speed Characteristic
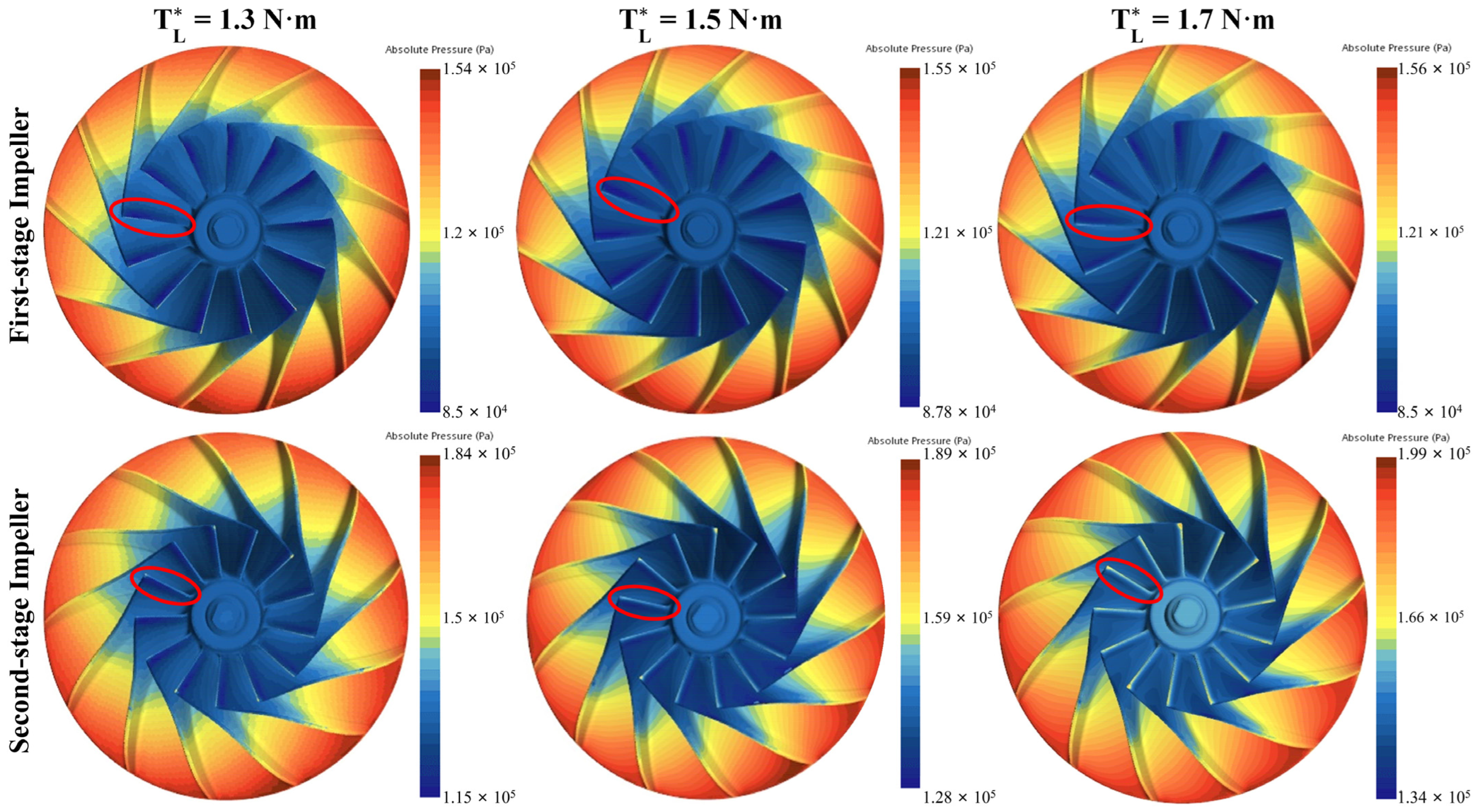
4.2. Torque Characteristic
5. Conclusions
- (1)
- Under constant torque, as the speed of the UHSEAC increases, the inlet mass flow rate decreases. This weakens the airflow impact on the impeller’s leading edge, reducing the pressure difference and consequently decreasing the amplitude of the load torque component. At 70,000 rpm, the inlet mass flow is the highest, with a load torque component amplitude of 0.033 N·m and a frequency of 15,166 Hz. At 90,000 rpm, the inlet mass flow is the lowest, resulting in a load torque component amplitude of 0.023 N·m and a frequency of 17,333 Hz. This shows that with increasing speed under UHSOC, the amplitude of load torque component decreases while the frequency increases.
- (2)
- Under constant speed, as the torque of the UHSEAC increases, the inlet mass flow rate increases. This intensifies the airflow impact on the impeller’s leading edge, creating a larger pressure difference and consequently increasing the amplitude of the load torque component. When the torque is 1.3 N·m, the inlet mass flow rate is the smallest, resulting in a load torque component amplitude of 0.023 N·m and a frequency of 17,333 Hz. When the torque is 1.7 N·m, the inlet mass flow rate is the largest, resulting in a load torque component amplitude of 0.039 N·m and the same frequency of 17,333 Hz. This shows that with increasing torque under UHSOC, the amplitude of load torque component increases while the frequency remains unchanged.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, D.; Hou, W.; Xiang, C.; Lu, D.; Yang, Q.; Li, J.; Wang, J. Waste heat utilization performance verification of Heat Exchanger Only Thermal Management System for fuel cell vehicle. J. Clean. Prod. 2023, 428, 139479. [Google Scholar] [CrossRef]
- Li, Y.; Pei, P.; Ma, Z.; Ren, P.; Huang, H. Analysis of air compression, progress of compressor and control for optimal energy efficiency in proton exchange membrane fuel cell. Renew. Sustain. Energy Rev. 2020, 133, 110304. [Google Scholar] [CrossRef]
- Jia, C.; He, H.; Zhou, J.; Li, K.; Li, J.; Wei, Z. A performance degradation prediction model for PEMFC based on bi-directional long short-term memory and multi-head self-attention mechanism. Int. J. Hydrogen Energy 2024, 60, 133–146. [Google Scholar] [CrossRef]
- Jiao, K.; Sun, H.; Li, X.; Wu, H.; Krivitzky, E.; Schram, T.; Larosiliere, L.M. Numerical simulation of air flow through turbocharger compressors with dual volute design. Appl. Energy 2009, 86, 2494–2506. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, S.; Wan, Y. Performance improvement of centrifugal compressors for fuel cell vehicles using the aerodynamic optimization and data mining methods. Int. J. Hydrogen Energy 2020, 45, 11276–11286. [Google Scholar] [CrossRef]
- Ahsan, N.; Al Rashid, A.; Zaidi, A.A.; Imran, R.; Qadir, S.A. Performance analysis of hydrogen fuel cell with two-stage turbo compressor for automotive applications. Energy Rep. 2021, 7, 2635–2646. [Google Scholar] [CrossRef]
- Hou, J.; Yang, M.; Ke, C.; Zhang, J. Control logics and strategies for air supply in PEM fuel cell engines. Appl. Energy 2020, 269, 115059. [Google Scholar] [CrossRef]
- Simons, S.; Hinchliff, M.; White, B.; Talabisco, G.C.; Kurz, R.; Ji, M. Compressor System Design and Analysis. In Compression Machinery for Oil and Gas; Gulf Professional Publishing: Houston, TX, USA, 2019; pp. 427–447. [Google Scholar]
- Fu, J.; Wang, H.; Sun, X.; Bao, H.; Wang, X.; Liu, J. Multi-objective optimization for impeller structure parameters of fuel cell air compressor using linear-based boosting model and reference vector guided evolutionary algorithm. Appl. Energy 2024, 363, 123057. [Google Scholar] [CrossRef]
- Handschuh, P.; Dobrica, M.; Çokşen, A.; Wiedmann, G. Turbo Compressors for the Cathode and Anode Supply of Fuel Cells. MTZ Worldw. 2022, 83, 66–71. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, Y.; Liu, G.; Yang, Q.; Li, L.; Gao, Z. Air and hydrogen supply systems and equipment for PEM fuel cells: A review. Int. J. Green Energy 2022, 19, 331–348. [Google Scholar] [CrossRef]
- Schröter, J.; Graf, T.; Frank, D.; Bauer, C.; Kallo, J.; Willich, C. Influence of pressure losses on compressor performance in a pressurized fuel cell air supply system for airplane applications. Int. J. Hydrogen Energy 2021, 46, 21151–21159. [Google Scholar] [CrossRef]
- Deng, Z.; Chen, Q.; Zhang, L.; Fu, Z. Data driven NARMAX modeling for PEMFC air compressor. Int. J. Hydrogen Energy 2020, 45, 20321–20328. [Google Scholar] [CrossRef]
- Lei, L.; Li, F.; Xu, B.; Egusquiza, M.; Luo, X.; Zhang, J.; Egusquiza, E.; Chen, D.; Jiang, W.; Patelli, E. Time-frequency domain characteristics analysis of a hydro-turbine governor system considering vortex rope excitation. Renew. Energy 2022, 183, 172–187. [Google Scholar] [CrossRef]
- Sheng, L.; Li, W.; Jiang, S.; Chen, J.; Liu, A. Nonlinear torsional vibration analysis of motor rotor system in shearer semi-direct drive cutting unit under electromagnetic and load torque component. Nonlinear Dyn. 2019, 96, 1677–1691. [Google Scholar] [CrossRef]
- Burella, G.; Moro, L.; Oldford, D. Analysis and validation of a procedure for a lumped model of Polar Class ship shafting systems for transient torsional vibrations. J. Mar. Sci. Technol. 2018, 23, 633–646. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, J.; Egusquiza, M.; Chen, D.; Li, F.; Behrens, P.; Egusquiza, E. A review of dynamic models and stability analysis for a hydro-turbine governing system. Renew. Sustain. Energy Rev. 2021, 144, 110880. [Google Scholar] [CrossRef]
- Zhao, L.; Zhen, T.; Liu, X.; Bo, X. Simulation analysis of load characteristic of thin seam shearer drum. J. Syst. Simul. 2020, 27, 3102–3108. [Google Scholar]
- Tanaka, S.; Nagumo, K.; Yamamoto, M.; Chiba, H.; Yoshida, K.; Okano, R. Fuel cell system for Honda CLARITY fuel cell. ETransportation 2020, 3, 100046. [Google Scholar] [CrossRef]
- Shu, M.; Yang, B.; Yang, M.; Martinez-Botas, R.F.; Deng, K. Investigation on the unsteadiness of centrifugal compressor exposed to pulsating backpressure. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2021, 235, 2212–2225. [Google Scholar] [CrossRef]
- Tao, R.; Wang, Z. Comparative modeling and analysis of the flow asymmetricity in a centrifugal pump impeller at partial load. Proc. Inst. Mech. Eng. Part A J. Power Energy 2020, 234, 237–247. [Google Scholar] [CrossRef]
- Fu, J.; Wang, H.; Bao, H.; Sun, X.; Liu, J. Multi-parameter optimization for the performance of the fuel cell air compressor based on computational fluid dynamics analysis at part load. Therm. Sci. Eng. Prog. 2023, 44, 102057. [Google Scholar] [CrossRef]
- Wang, H.; Yang, D.; Zhu, Z.; Zhang, H.; Zhang, Q. Effect of Interstage Pipeline on the Performance of Two-Stage Centrifugal Compressors for Automotive Hydrogen Fuel Cells. Appl. Sci. 2022, 13, 503. [Google Scholar] [CrossRef]
- Li, W.; Feng, G. Design and experimental study of centrifugal compressor in fuel cell vehicle. Mechanics 2021, 27, 52–61. [Google Scholar] [CrossRef]
- Hu, D.; Wang, Y.; Li, J.; Wang, J.; Yang, Q. Energy saving control of waste heat utilization subsystem for fuel cell vehicle. IEEE Trans. Transp. Electrif. 2023, 10, 1. [Google Scholar] [CrossRef]
- Zhou, S.; Jin, J.; Wei, Y. Research on online diagnosis method of fuel cell centrifugal air compressor surge fault. Energies 2021, 14, 3071. [Google Scholar] [CrossRef]
- Chen, H.; Liu, Z.; Ye, X.; Yi, L.; Xu, S.; Zhang, T. Air flow and pressure optimization for air supply in proton exchange membrane fuel cell system. Energy 2022, 238, 121949. [Google Scholar] [CrossRef]
- Alizadeh, H.; Jahangir, M.H.; Ghasempour, R. CFD-based improvement of Savonius type hydrokinetic turbine using optimized barrier at the low-speed flows. Ocean Eng. 2020, 202, 107178. [Google Scholar] [CrossRef]
- Liu, Y.; Zhao, Y.; Yang, Q.; Liu, G.; Li, L.; Gao, Z. Performance study of centrifugal air compressor for proton exchange membrane fuel cell systems. Energy Sci. Eng. 2022, 10, 208–218. [Google Scholar] [CrossRef]
- Shi, T.; Huang, H.; Peng, X.; Feng, J.; Guo, Y. Experimental study on the static and dynamic performances of gas foil bearings for the centrifugal air compressor used in fuel cell vehicles. Int. J. Energy Res. 2022, 46, 4417–4433. [Google Scholar] [CrossRef]
- Tempiam, A.; Kachapongkun, P.; Rattanadecho, P.; Prommas, R. Experimental investigation of vortex tube for reduction air inlet of a reciprocating air compressor. Case Stud. Therm. Eng. 2020, 19, 100617. [Google Scholar] [CrossRef]
- Zou, Z.; Xu, P.; Fu, C. An evaluation model for wall heat transfer effects on micro centrifugal compressors and its application in aerodynamic design. Appl. Therm. Eng. 2024, 236, 121554. [Google Scholar] [CrossRef]
- Liu, Z.; Li, Z.; Xie, D.; Wu, H. Unsteady characteristic and flow mechanism of a scroll compressor in small-scale compressed air energy storage system. J. Energy Storage 2022, 51, 104368. [Google Scholar] [CrossRef]
- Gouda, E.M.; Benaouicha, M.; Neu, T.; Fan, Y.; Luo, L. Flow and heat transfer characteristics of air compression in a liquid piston for compressed air energy storage. Energy 2022, 254, 124305. [Google Scholar] [CrossRef]
- Khaljani, M.; Vennard, A.; Harrison, J.; Surplus, D.; Murphy, A.; Mahmoudi, Y. Experimental and modelling analysis of efficiency enhancement in a liquid piston gas compressor using metal plate inserts for compressed air energy storage application. J. Energy Storage 2021, 43, 103240. [Google Scholar] [CrossRef]
- Kan, K.; Xu, Z.; Chen, H.; Xu, H.; Zheng, Y.; Zhou, D.; Muhirwa, A.; Maxime, B. Energy loss mechanisms of transition from pump mode to turbine mode of an axial-flow pump under bidirectional conditions. Energy 2022, 257, 124630. [Google Scholar] [CrossRef]
- Zheng, S.; Wei, M.; Song, P.; Hu, C.; Tian, R. Thermodynamics and flow unsteadiness analysis of trans-critical CO2 in a scroll compressor for mobile heat pump air-conditioning system. Appl. Therm. Eng. 2020, 175, 115368. [Google Scholar] [CrossRef]
- Bacak, A.; Pınarbaşı, A.; Dalkılıç, A.S. An experimental study on the determination of a hermetic reciprocating compressor’s discharge and suction losses. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2024, 238, 1330–1344. [Google Scholar] [CrossRef]
- Kan, K.; Zhang, Q.; Xu, Z.; Zheng, Y.; Gao, Q.; Shen, L. Energy loss mechanism due to tip leakage flow of axial flow pump as turbine under various operating conditions. Energy 2022, 255, 124532. [Google Scholar] [CrossRef]

| Content | Model |
|---|---|
| State equation | Ideal gas |
| Energy | Separation energy |
| Time | Unsteady |
| Rapids | K-omega turbulence |
| Parameter | Value | Unit |
|---|---|---|
| Rated power | 22 | kW |
| Rated voltage | 380 | V |
| Rated speed | 80,000 | rpm |
| Pole pairs | 1 | |
| Number of slots | 24 | |
| Stator inner diameter | 36.85 | mm |
| Stator outer diameter | 82 | mm |
| Stator length | 70 | mm |
| Stacking Coefficient | 0.85 | |
| Rotor inner diameter | 5.85 | mm |
| Rotor outer diameter | 35.85 | mm |
| Rotor shaft length | 70 | mm |
| Magnet thickness | 6 | mm |
| Magnet span | 60 |
| Condition | Parameter | Speed (rpm) | Value | Frequency | Maximum Amplitude |
|---|---|---|---|---|---|
| fixed outlet pressure | air flow rate | 70,000 | 79.241 g/s | 15,166 Hz | 0.362 g/s |
| 80,000 | 92.690 g/s | 17,333 Hz | 0.460 g/s | ||
| 90,000 | 111.071 g/s | 19,500 Hz | 0.612 g/s | ||
| fixed air flow rate | outlet pressure | 70,000 | 203.488 kPa | 15,166 Hz | 0.920 kPa |
| 80,000 | 248.402 kPa | 17,333 Hz | 1.240 kPa | ||
| 90,000 | 305.534 kPa | 19,500 Hz | 1.681 kPa |
| Parameter | Value | Unit |
|---|---|---|
| Cross-sectional areas of entrance, S1 | 2.132 × 10−3 | m2 |
| Cross-sectional areas of exit, S2 | 1.479 × 10−3 | m2 |
| Heights of gas inlet, Z1 | 0 | m |
| Heights of gas outlet, Z2 | 0.101 | m |
| Installation angle of the FSI, β1 | 40 | deg |
| Installation angle of the SSI, β2 | 54 | deg |
| Nominal diameter of the FSI, D1 | 7.598 × 10−2 | m2 |
| Nominal diameter of the SSI, D2 | 7.250 × 10−2 | m2 |
| Loss coefficient, λc | 1.500 × 10−2 | |
| Wheel resistance loss coefficient, βdf | 2.000 × 10−2 | |
| Friction resistance coefficient, λ | 1.900 × 10−2 | |
| Average equivalent diameter of the volute flow passage, dh | 4.531 × 10−2 | m |
| Length of process, l | 0.384 | m |
| Number of impeller blades, b | 13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, J.; Shu, X.; Zhang, J.; Yi, F.; Hu, D.; Zhang, C.; Li, Y. Load Torque Component Extraction and Analysis of Ultra-High-Speed Electric Air Compressors for Fuel Cell Vehicles. Actuators 2024, 13, 320. https://doi.org/10.3390/act13080320
Zhou J, Shu X, Zhang J, Yi F, Hu D, Zhang C, Li Y. Load Torque Component Extraction and Analysis of Ultra-High-Speed Electric Air Compressors for Fuel Cell Vehicles. Actuators. 2024; 13(8):320. https://doi.org/10.3390/act13080320
Chicago/Turabian StyleZhou, Jiaming, Xing Shu, Jinming Zhang, Fengyan Yi, Donghai Hu, Caizhi Zhang, and Yanzhao Li. 2024. "Load Torque Component Extraction and Analysis of Ultra-High-Speed Electric Air Compressors for Fuel Cell Vehicles" Actuators 13, no. 8: 320. https://doi.org/10.3390/act13080320
APA StyleZhou, J., Shu, X., Zhang, J., Yi, F., Hu, D., Zhang, C., & Li, Y. (2024). Load Torque Component Extraction and Analysis of Ultra-High-Speed Electric Air Compressors for Fuel Cell Vehicles. Actuators, 13(8), 320. https://doi.org/10.3390/act13080320









