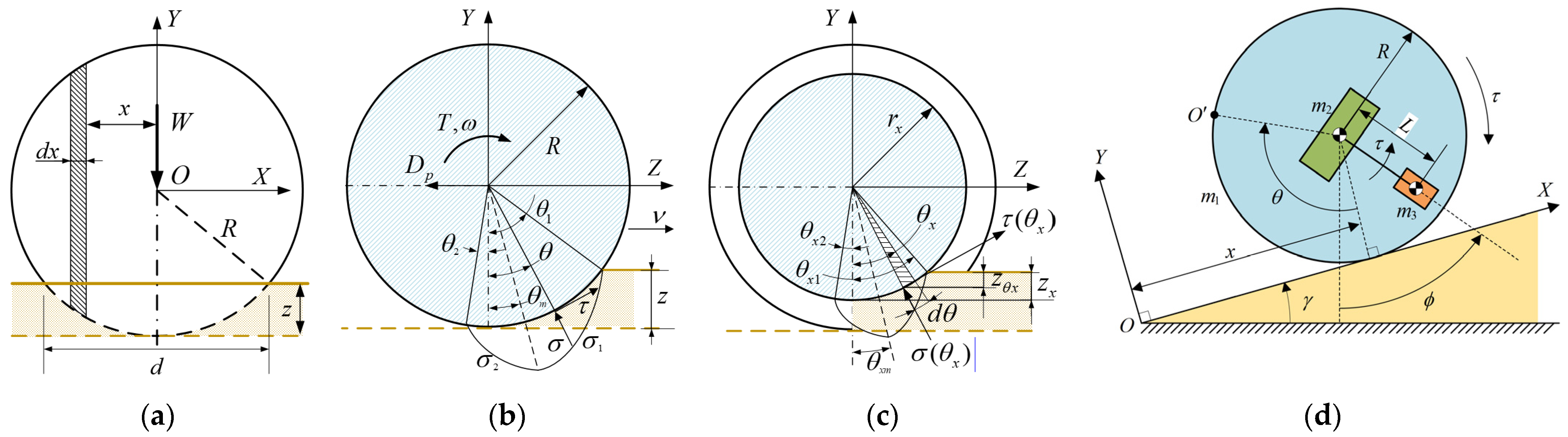
We design two main experiments: the experiment of factors affecting the performance of the spherical robot which is carried out by the orthogonal test method; and the experiment of the forced sliding of the spherical robot on sandy terrain which is carried out by the forced sliding principle. The following is a detailed introduction to the two main experiments.
3.1. Spherical Robot Longitudinal Motion Performance Influencing Factor Experiment
The longitudinal motion performance of spherical robots on sandy terrain is influenced by the robot’s load, joint angular acceleration, maximum joint angular velocity, and maximum driving torque. The maximum driving torque of the robot is related to the rated power of the motor, the load, and the pendulum length. Under conditions of constant rated power and pendulum length, the maximum driving torque of the spherical robot is determined by the load. The prototype spherical robot used in the experiment [
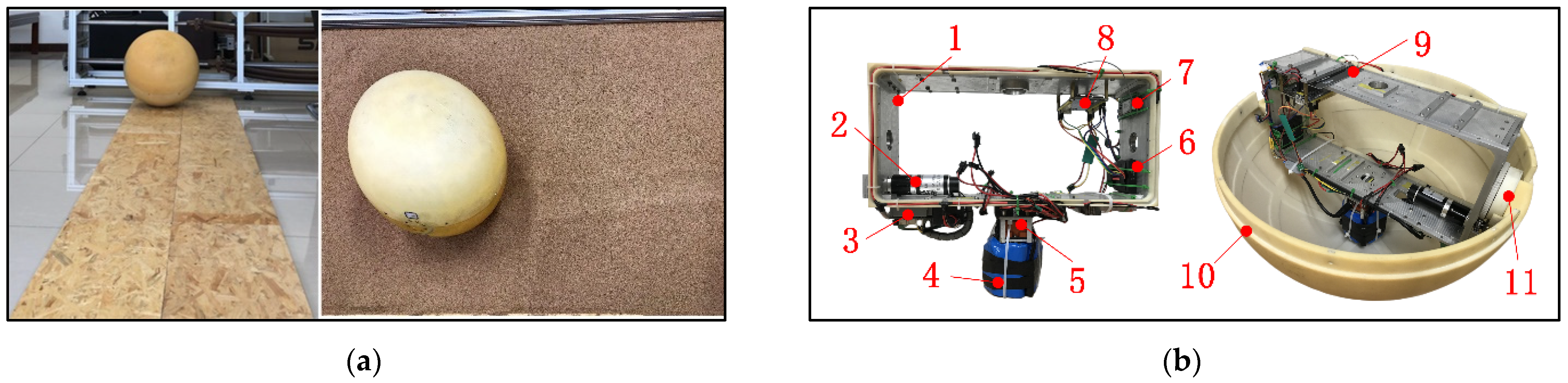
4] had an initial total mass of 7.7 kg. This study adjusted the robot’s load by changing the pendulum mass in increments of 300 g. The spherical shell material is glass-fiber-reinforced polymer (GFRP). The experiment was conducted on surfaces of European oak boards and dry fine sand, with uniformly laid hardwood floorboards and a sand tank, as shown in
Figure 2a. The sand tank had a depth of 15 mm; the dimensions of the tank are
. The dimensions of the hardwood floorboards are
. Rolling experiments were conducted on both terrains, and the motor current of the robot was recorded in real-time and converted into output torque.
The experimental platform, as shown in
Figure 3a, includes the following instruments, as shown in
Figure 2b: a brushless DC servo motor with a Hall effect encoder (ECG2845, Beijing Patses Technology Co., Ltd., Beijing, China), a servo motor driver (WHI-A20/100, Elmo Motion Control Ltd., Tehran, Israel), an inertial measurement unit (IMU) system (Xsens MTi-300, MicoBo Technology (Shanghai) Co., Ltd., Beijing, China), and a wireless module (Microhard HP900, Microhard Systems Inc., Ottawa, Canada). The rolling process of the robot was recorded using a stereo depth camera (Intel RealSense D435, Intel Corporation, San Francisco, CA, USA), and the rolling speed and distance of the spherical robot in the videos were measured using Tracker software (Version 6.1.3), as shown in
Figure 3b.
The motion of spherical robots on sandy terrain can be divided into four stages: the hardwood board acceleration stage, the sand tank penetration stage, the stable motion stage, and the deceleration stop stage. Starting from the hardwood board with a given joint angular acceleration and maximum angular velocity, the spherical robot accelerates. As the robot enters the sand tank from the hardwood board, gravity and inertia cause compression, penetration, and shearing of the sand particles in the tank, leading to subsidence and uplift of the sand. Upon contact between the sphere and the sand, a significant horizontal impact occurs, resulting in initial traction and substantial subsidence. Subsequently, under the resistance of the sand and the action of internal driving mechanisms, the robot gradually enters the stable motion stage. In this stage, the subsidence of the sand tank is relatively uniform. When the robot lacks power or enters the deceleration stop stage, the pendulum swings downward, reducing the sphere’s speed. The entire weight of the robot and the inertia during the pendulum swing process act completely on the contact surface between the sand tank and the sphere, causing increased subsidence of the sand tank, increased height of uplift, and increased earth-moving resistance, until the robot stops rolling.
This study varies robot mass, joint angular acceleration, and maximum joint angular velocity as experimental factors, with robot rolling distance as the experimental criterion, setting up an experimental factor level table. When the prototype is unloaded, the total mass of the machine is 7.7 kg, and the maximum load is 600 g. Therefore, the mass factor levels are taken as 7.7 kg, 8.0 kg, and 8.3 kg. Secondly, based on the performance of the driving motor, the levels of joint angular acceleration factors are 0.3 rot/s
2, 0.4 rot/s
2, and 0.5 rot/s
2, and the levels of maximum joint angular velocity are 1 rot/s, 1.125 rot/s, and 1.25 rot/s. Based on this, a three-factor, three-level orthogonal experiment was conducted using an L
9(3
4) orthogonal table, as shown in
Table 2, with nine experimental runs (N = 9).
In
Table 2,
K1 represents the sum of the values of the test indicators corresponding to the “1” level;
K2 represents the sum of the values of the test indicators corresponding to the “2” level;
K3 represents the sum of the values of the test indicators corresponding to the “3” level;
R is the range, and the larger
R is, the more significant the impact will be. According to the results in
Table 2, the primary order of the three factors that affect the longitudinal movement distance of the spherical robot on dry sandy soil is as follows: load, joint angular acceleration, and joint angular velocity. The optimal combination is as follows: a mass of 7.7 kg, a joint angular acceleration of 0.5 rad/s
2, a joint angular velocity of 1.25 rad/s, and the passing distance is 2.387 m.
In
Table 3, we used SPSS for analysis of variance and selected the S-N-K model for analysis; the table lists the sum of square, standard deviation, and other information for each group. Based on the comparison results, the differences between each group can be determined. According to the table of S-N-K method, if the
p-value between two groups is less than 0.05, it indicates a significant difference; if the
p-value is greater than 0.05, it indicates that there is no significant difference. We found that the load had the most significant effect, followed by joint angular acceleration. The results of the variance analysis were consistent with the range analysis, indicating that the results were generally reliable.
When the robot’s driving torque is limited, the robot’s load has the most significant impact on its longitudinal motion performance, followed by joint angular acceleration. Therefore, under rated power conditions for spherical robots, with the same levels of angular acceleration and maximum angular velocity, optimizing the robot’s load significantly improves its performance in traversing soft terrain.
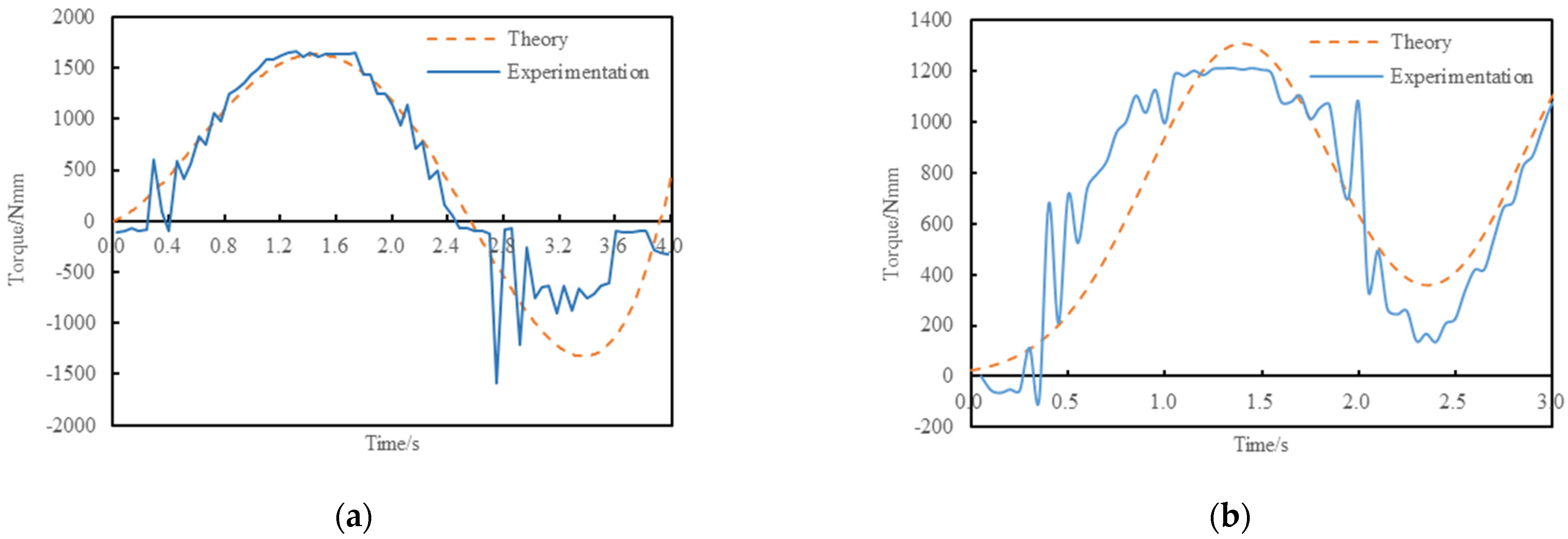
We take the results of the ninth test in
Table 2 as an example, comparing the numerical calculations and physical experimental results of the driving torque–time variation for spherical robots rolling in a straight line on hardwood floorboards and loose dry sand, as shown in
Figure 4. It is observed that within the same timeframe, the trends of variation in the numerical calculations and the physical experimental results are generally consistent.
Under constant joint angular velocity, the variation of joint torque over time forms a sinusoidal curve. In
Figure 4a, the curve is symmetric about y = 0, while in
Figure 4b, the curve is symmetric about y = 700. This indicates a significant increase in the driving torque required for spherical robots on loose dry sand, highlighting the non-negligible frictional resistance between the sphere and the terrain.
Although there are still differences between the numerical calculations and the physical measurements in some time intervals, the introduction of the coefficient of determination (R2) to characterize the fit between the numerical calculation data and the experimental data shows that the correlation coefficients are both around 0.9, indicating a good agreement between the numerical calculations and the experimental measurements.
3.2. Experiment on Forced Sliding of Spherical Robot on Sandy Terrain
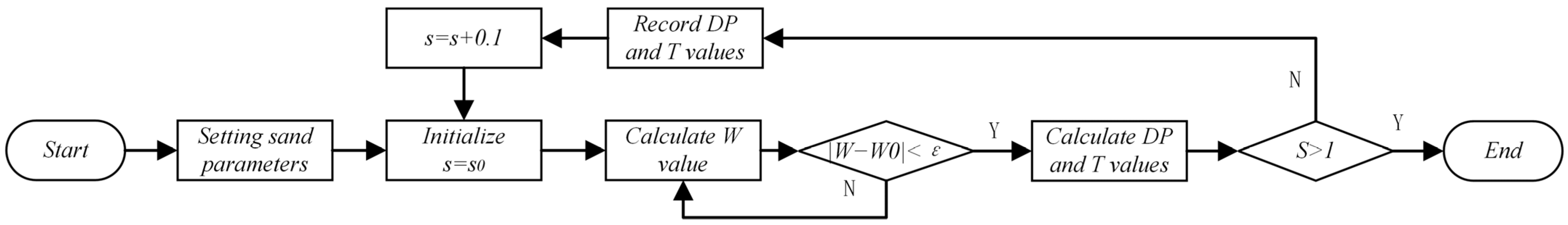
The above experiments were conducted under the assumption of zero slip ratio for the spherical robot. In order to quantitatively analyze the relationship between different slip ratios and the driving torque, as well as the traction force, we performed numerical calculations on the performance of the sphere’s motion with slip under two different loads (300 N and 150 N) and two different shell radii (0.3 m and 0.15 m) based on Equations (4)–(10). The calculation process is shown in
Figure 5.
Firstly, we defined the parameters of the sand, initialized the slip ratio to 0, and then calculated the theoretical load W of the sphere based on Equation (4). If the absolute deviation between the theoretical load W and the actual load W0 is within a certain range , then according to Equations (5) and (6), we calculated the traction force DP and the driving torque T. If the slip ratio is less than 1, the slip ratio is increased by 0.1, and the calculation process is repeated.
From the results in
Figure 6, the following conclusions can be drawn: Under conditions where the shell radius is 0.3 m, increasing the load leads to increases in traction force, driving torque, and subsidence, but these increases are not linear. The traction force and driving torque stabilize after the slip ratio reaches 0.5. Under a load of 300 N, increasing the shell radius results in increased traction force and driving torque, but decreased subsidence. Therefore, increasing the shell radius increases the contact area, resulting in less subsidence under the same load conditions, and the traction force and driving torque also stabilize after the slip ratio reaches 0.5.
To validate the accuracy of our theoretical modeling in Equations (4)~(6) and (9)~(10), we constructed a forced slip test platform for spherical robots. The principle of forced slip involves rotating the sphere at a certain angular velocity, generating a theoretical translational speed. Simultaneously, the sphere is forced to translate at a certain linear velocity, resulting in an actual translational speed. When the theoretical translational speed exceeds the actual translational speed, the sphere exhibits slipping movement.
First, we calibrated the parameters of the experimental sand material: the angle of repose and the density of the sand were determined using the funnel method and the graduated cylinder method, respectively. The friction parameters between the sand and the sphere shell were calibrated using the slope method. For the interaction parameters between sand particles in contact, empirical values from the literature [
24] were selected based on the sand density. The dry sand used in the experiment had very minimal moisture content and an uneven particle size distribution, but particles in the range of 0.1 to 0.5 mm diameter constituted over 90% by weight, dominating the overall particle medium analysis.
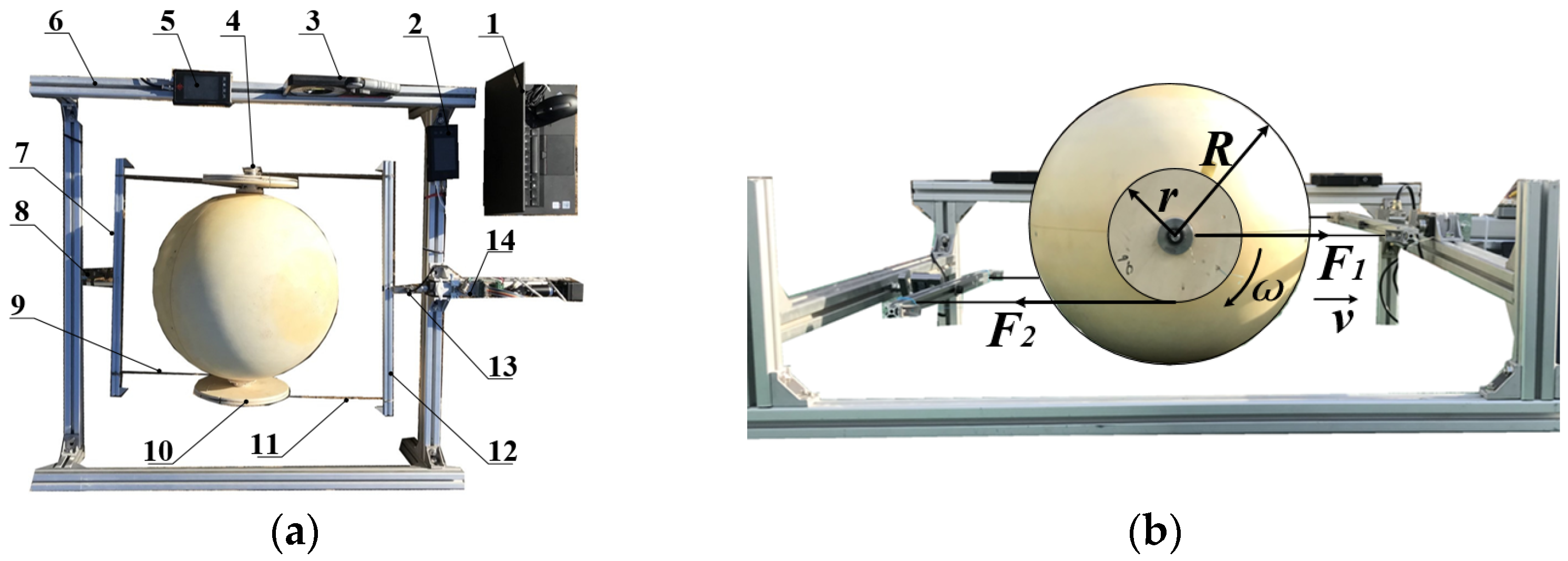
The structure of the forced slip test platform is shown in
Figure 7a. A slip disc is securely attached to each side of the sphere shell. Depending on the required number of rotations for the sphere shell in the experiment, a driving rope is wound around the slip discs. The direction of winding ensures that the driving rope can unwind from the underside of the slip disc and is tied to a fixed rod at the end of the driving rope. The fixed rod of the driving rope is connected to a force sensor, which is in turn fixed to a stationary frame. A pulley is mounted at the center of the slip disc, allowing relative rotation with respect to the sphere shell. One end of a traction rope is tied to the pulley, and the other end is tied to a fixed rod for the traction rope, ensuring the traction rope is 100 mm longer than the radius of the sphere shell to prevent collision with the fixed rod. The fixed rod of the traction rope is connected to one end of a traction force sensor, with the other end of the sensor fixed to the end of an electric cylinder push rod.
The electric cylinder push rod drives the fixed rod of the traction rope to pull the sphere shell to the right at a constant speed. During the movement of the sphere shell, the driving rope continuously unwinds. Because the driving rope is not on the same horizontal line as the sphere center, it generates a torque on the sphere shell, causing it to rotate at a certain angular velocity. Due to the smaller radius of the slip discs compared to the sphere shell, the actual movement speed of the sphere shell is less than the theoretical speed, thereby achieving forced slip. Data from the two sensors are collected by a data recorder and subsequently stored on a computer. To ensure the horizontal alignment of the fixed bracket and all connecting rods, a gradienter is used for calibration before the experiment begins. The driving rope and traction rope used in this study are made of 0.5 mm diameter PE braided line. Compared to steel wire rope, PE braided line is softer, non-rebounding, easy to tie and wind, and has low stretchability, high strength, and abrasion resistance. It can withstand a tensile force of up to 40 kg, meeting the experimental requirements.
The side view of the forced slip test platform is shown in
Figure 7b. Let
r denote the radius of the slip discs, and let
R denote the radius of the sphere shell. The magnitude of the traction force measured by the traction force sensor is
, and the magnitude of the pulling force measured by the driving force sensor is
. When the sphere shell rotates uniformly for one revolution, the length of the driving rope unwound is
, while the distance moved by the sphere shell is
. Due to the unequal lengths, the sphere shell undergoes slip, and the slip ratio of the sphere shell can be expressed as follows:
The traction force of the spherical shell can be expressed as follows:
The driving torque of the spherical shell can be expressed as follows:
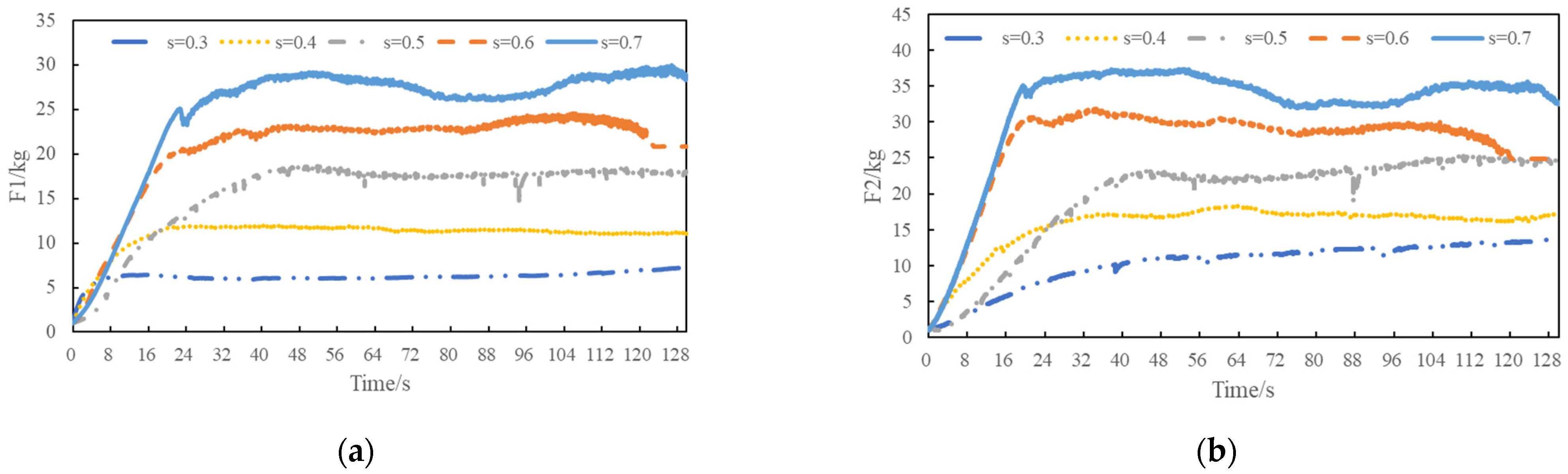
In numerical calculations, we found that the traction force and driving torque tend to stabilize around a slip ratio of 0.5. Therefore, we selected slip ratios of 0.3, 0.4, 0.5, 0.6, and 0.7 for the physical experiments. The specific steps of the forced slip experiment are as follows: Firstly, the sand is leveled and compacted, and the fixed bracket is adjusted to ensure the bracket is horizontal. Next, the slip discs and pulleys are mounted on both sides of the sphere shell, ensuring that the fixing rods at both ends are horizontal and that the sphere shell is centered. The height and width of the traction rope are adjusted to ensure it remains straight in both the horizontal and vertical directions. The sensor values are zeroed out, the electric cylinder speed is set to 28 mm/min, the distance is set to 150 mm, and the data are collected and recorded from the two tension sensors. The slip discs are replaced with different diameters and the above steps are repeated to obtain tension data at different slip ratios. For each set of slip ratio settings, experiments are repeated multiple times; a stable dataset is selected as the measurement result. The data are processed accordingly, as shown in
Figure 8. The values of F1 and F2 when they reach stability were substituted into Equations (13) and (14) to obtain the corresponding values for DP and T, as shown in
Table 4.
The experimental sphere shell radius is 0.22 m with a load of 150 N. The traction force–slip ratio curves and the driving torque–slip ratio curves from the numerical calculations and the physical experiments are shown in
Figure 9a,b. In the figure, Theory 1 represents the numerical calculation results without considering pushing resistance or additional resistance, while Theory 2 represents the numerical calculation results considering these resistances. In
Figure 9a, the average percentage error between theoretical value 1 and the experimental value is 9.6%, and the average percentage error between theoretical value 2 and the experimental value is 0.5%. In
Figure 9b, the average percentage error between theoretical value 1 and the experimental value is 15.7%, and the average percentage error between theoretical value 2 and the experimental value is 3.3%.
From the result of
Figure 9, it can be observed that without considering resistance, the traction force and driving torque calculated numerically at the same slip ratio are higher than the experimental results. When considering both pushing resistance and additional resistance, the numerical calculation results align well with the results from physical experiments. The physical experiment results indicate that the actual peak hook traction force and driving torque both occur around s = 0.5, and beyond a slip ratio of 0.5, both traction force and driving torque decrease. This suggests that spherical robots rolling on sandy terrain should avoid excessively high slip ratios. If a spherical robot cannot traverse the sandy terrain even when the slip ratio reaches 0.5, alternative strategies for extrication should be considered.
It is not difficult to observe that the force conditions of spherical robots on sandy terrain, as calculated numerically, correspond well with the results of the physical experiments. The gradual trends in the various data also align with physical principles, which to some extent validates the effectiveness of the model. Given the various parameter combinations yielding similar numerical results, and recognizing that we cannot measure physical experiment values with 100% accuracy, we select parameter combinations that are closest to reality within the permissible range of engineering. Due to factors such as simplification in theoretical models, inaccuracies in the calibration of parameters in physical experiments, and data measurement, achieving a high consistency between theoretical and physical experiment results is challenging. Nevertheless, the effectiveness of this modeling approach cannot be denied.