A High Torque Density Dual-Stator Flux-Reversal-Machine with Multiple Poles Halbach Excitation on Outer Stator
Abstract
1. Introduction
2. Evolution Process of Topology and Operating Principle
2.1. Machine Configuration
2.2. PM Poles Selection
2.3. Armature Winding Configuration
2.4. Evolution Process
2.5. Operating Principle
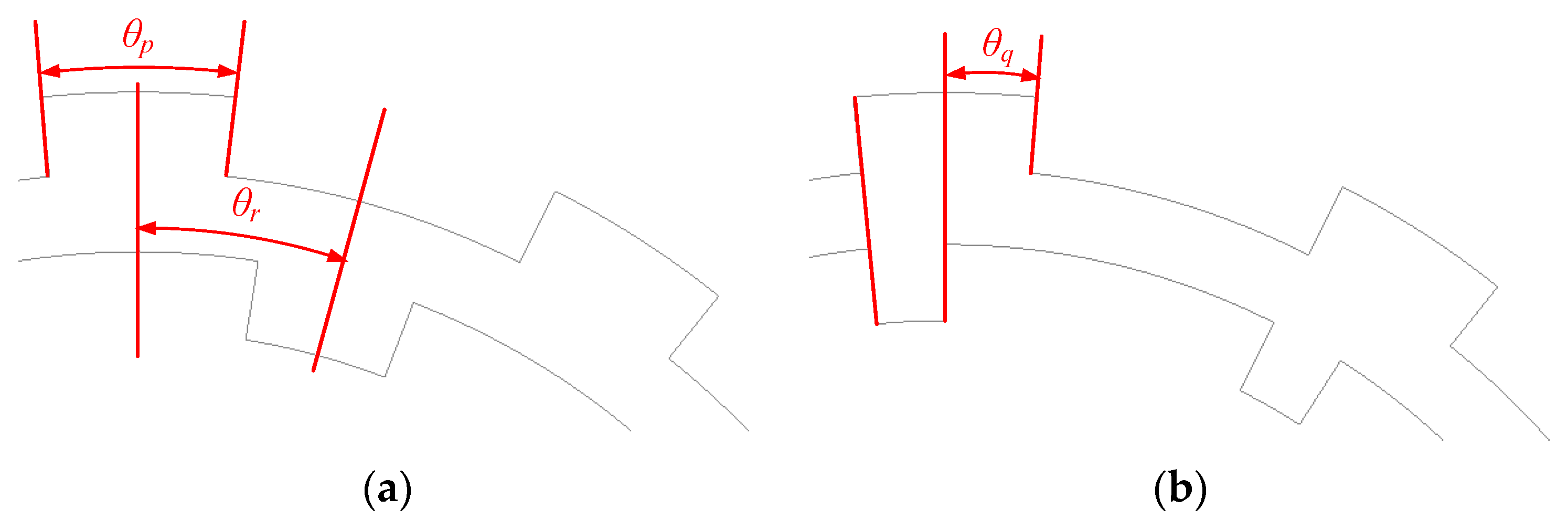
3. Key Parameters Design of the Proposed MPHE-DSFRM
3.1. Position Relationship of Two Stators
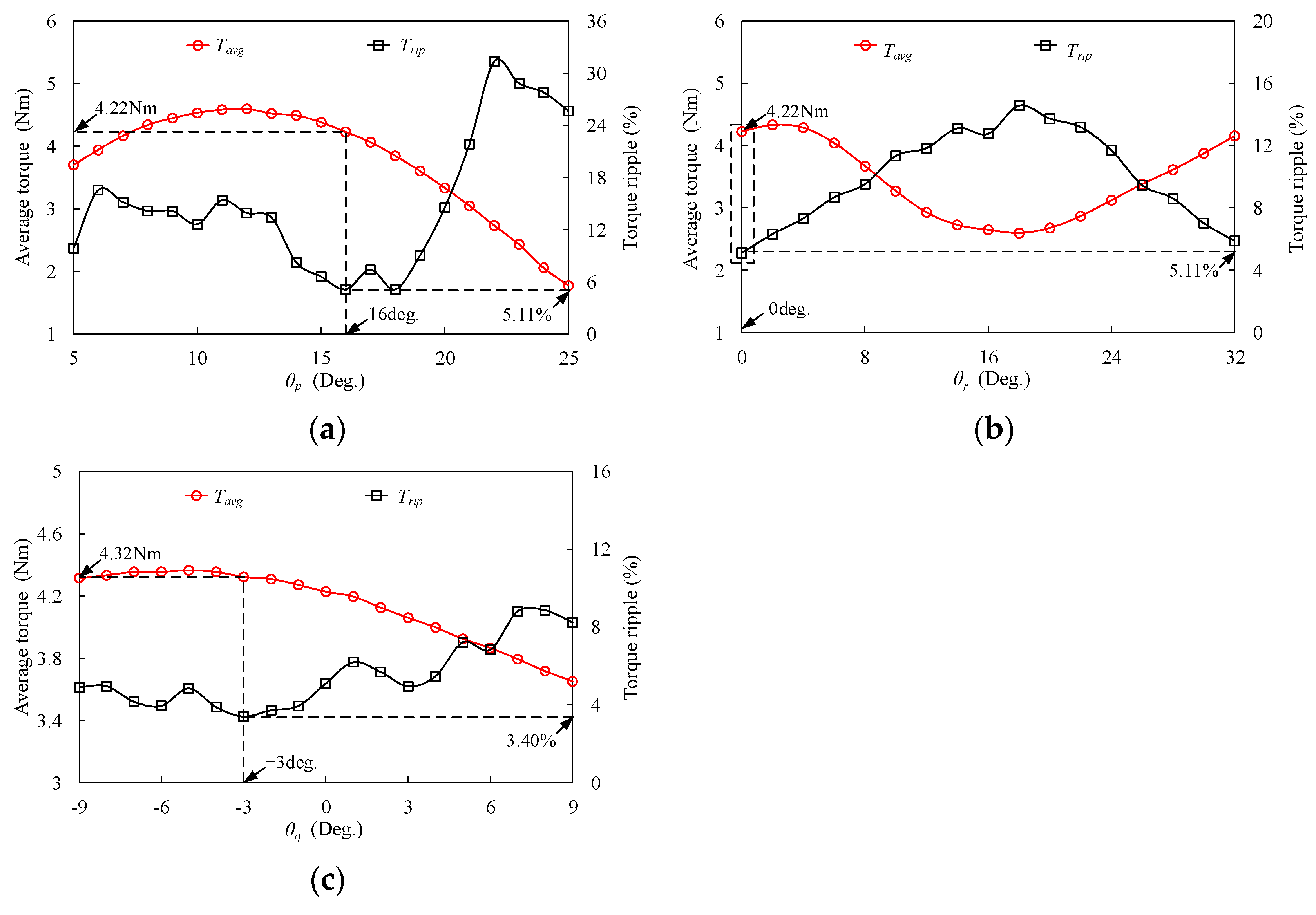
3.2. Width of Stator PM
3.3. Design Parameters for Rotor
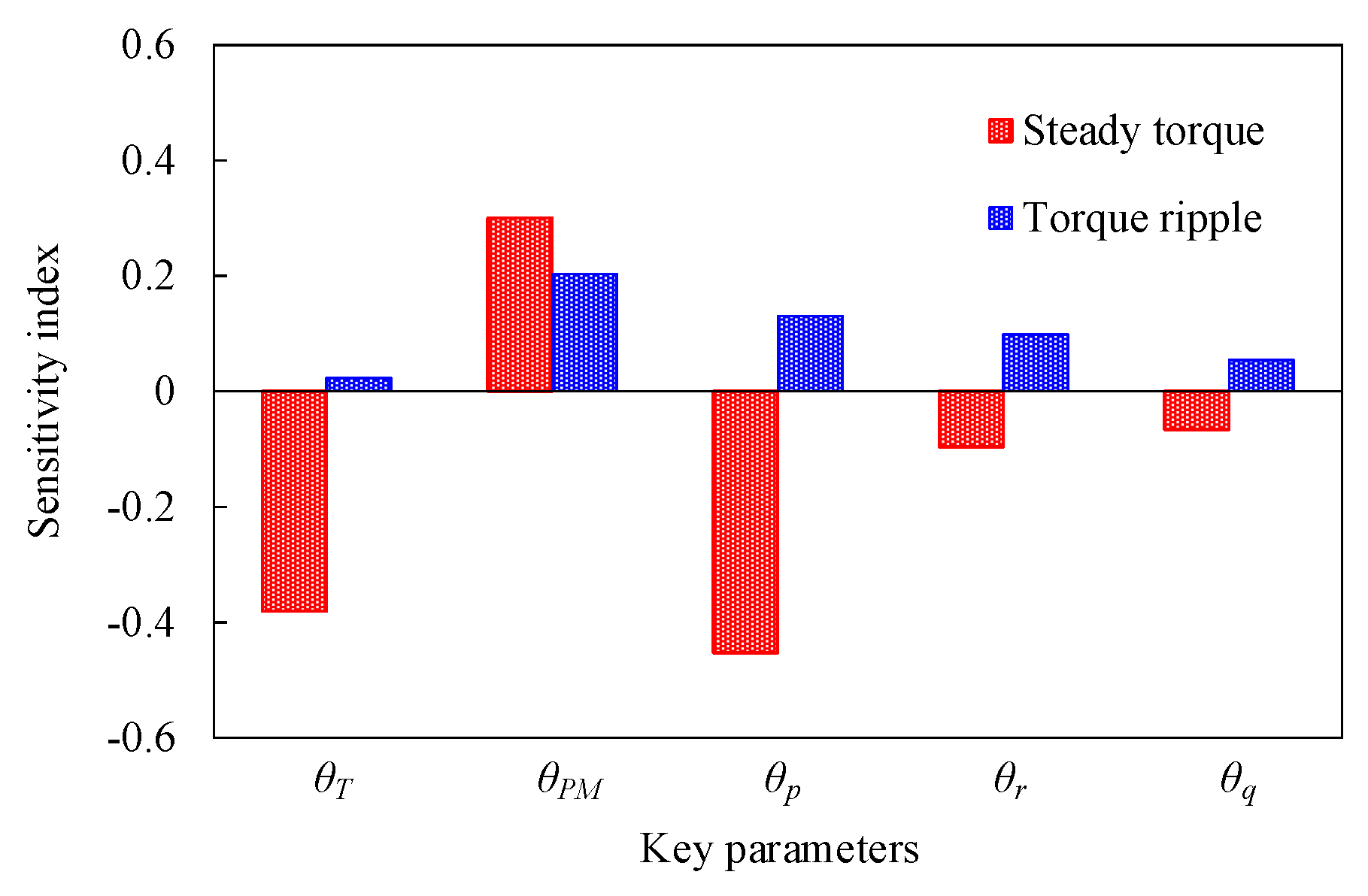
3.4. Sensitivity Analysis of Key Parameters
4. Electromagnetic Performance Analysis
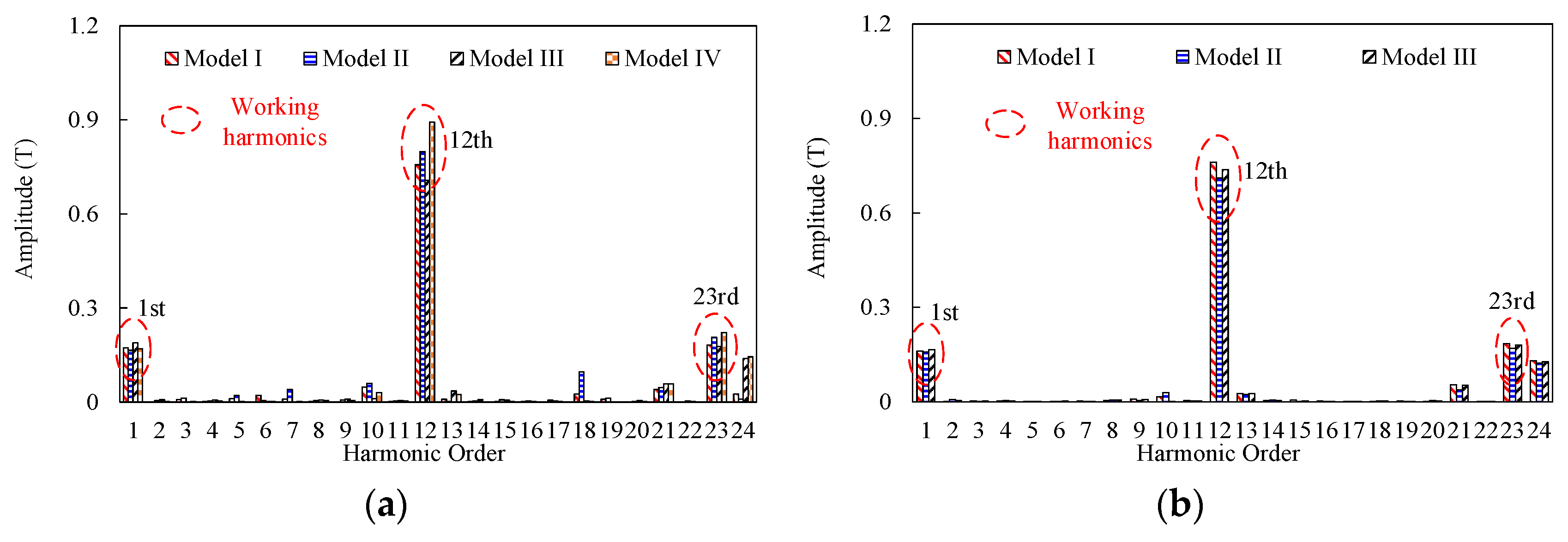
4.1. No-Load Airgap Flux Density
4.2. No-Load Magnetic Field Distribution
4.3. No-Load Back-EMF
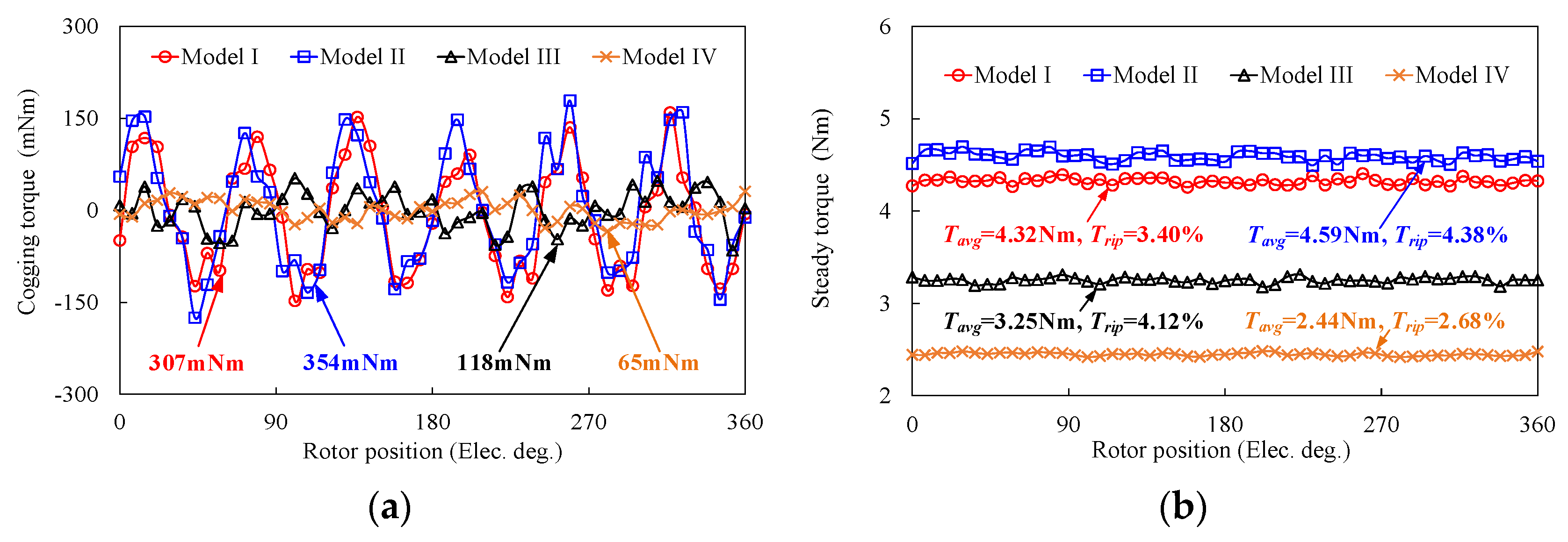
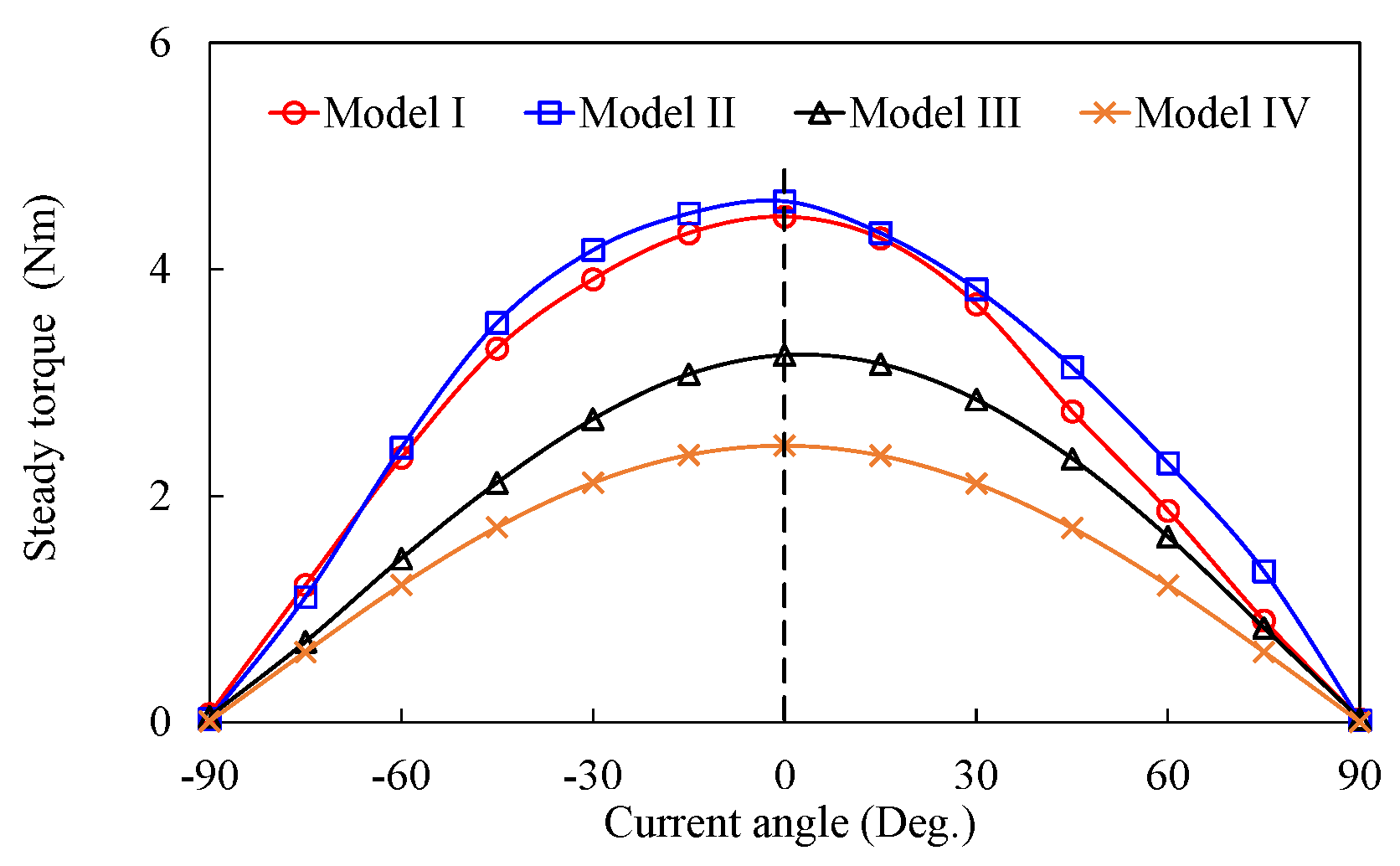
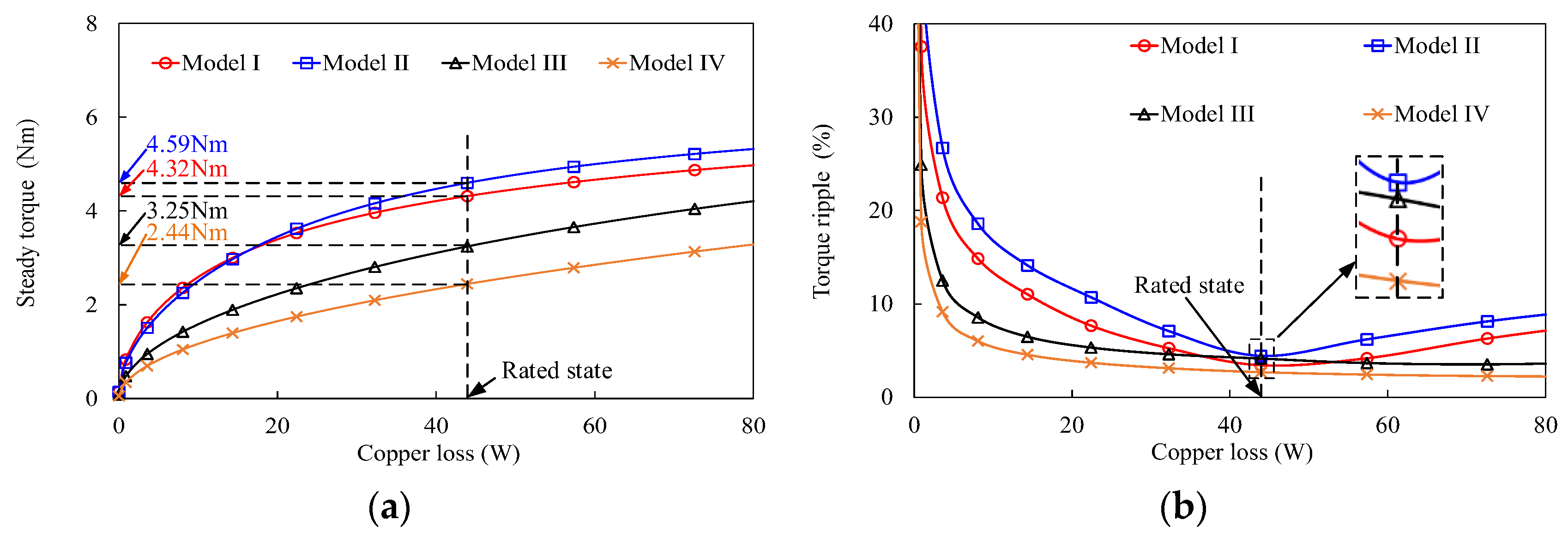
4.4. Torque Characteristics
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, D.; Peng, C.; Wang, B.; Feng, Z.; Li, J. Permanent magnet synchronous machines with nonuniformly distributed teeth. IEEE Trans. Ind. Electron. 2022, 69, 8705–8715. [Google Scholar] [CrossRef]
- Qiao, G.; Liu, Y.; Wang, M.; Liu, F.; Zheng, P. Study of a high-efficiency series–parallel-connected hybrid-PM variable-flux permanent magnet synchronous machine. IEEE Trans. Magn. 2022, 58, 8201207. [Google Scholar] [CrossRef]
- Zhao, H.; Liu, C.; Song, Z. Design of an effective double-rotor machine with robust mechanical structure. IEEE Trans. Magn. 2020, 56, 7503407. [Google Scholar] [CrossRef]
- Xu, X.; Chen, J.; Lin, Z.; Qiao, Y.; Chen, X.; Zhang, Y.; Xu, Y.; Li, Y. Optimization design for the planetary gear train of an electric vehicle under uncertainties. Actuators 2022, 11, 49. [Google Scholar] [CrossRef]
- Zhao, W.; Hu, Q.; Ji, J.; Ling, Z.; Li, Z. Torque generation mechanism of dual-permanent-magnet-excited vernier machine by air-gap field modulation theory. IEEE Trans. Ind. Electron. 2023, 70, 9799–9810. [Google Scholar] [CrossRef]
- Zhu, X.; Lee, C.H.T.; Chan, C.C.; Xu, L.; Zhao, W. Overview of flux-modulation machines based on flux-modulation principle: Topology, theory, and development prospects. IEEE Trans. Transp. Electrif. 2020, 6, 612–624. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, Q.; Ding, S.; Li, W.; Hang, J. Investigation of air-gap field modulation effect in spoke-type PM machines. IEEE Trans. Transp. Electrif. 2023, 9, 845–855. [Google Scholar] [CrossRef]
- Wang, H.; He, C.; Xu, W.; Zhu, H.; Yang, J. Design and analysis of a new separated type permanent magnet machine based on bidirectional field modulation effect. IEEE Trans. Energy Convers. 2023, 38, 2202–2210. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Yu, J.; Wang, Y. A novel partitioned-stator flux reversal machine with two sets of permanent magnet. IEEE Trans. Magn. 2023, 59, 8102506. [Google Scholar] [CrossRef]
- Gao, Y.; Kosaka, T.; Liu, Y.; Doppelbauer, M.; Qu, R. Comparative analysis of double flux modulation permanent magnet machines with different stator PM arrangements. IEEE Trans. Ind. Appl. 2022, 58, 1941–1951. [Google Scholar] [CrossRef]
- Gao, Y.; Doppelbauer, M.; Qu, R.; Li, D.; Ding, H. Synthesis of a flux modulation machine with permanent magnets on both stator and rotor. IEEE Trans. Ind. Appl. 2021, 57, 294–305. [Google Scholar] [CrossRef]
- Zhao, H.; Liu, C.; Song, Z.; Wang, W.; Lubin, T. A dual-modulator magnetic-geared machine for tidal-power generation. IEEE Trans. Magn. 2020, 56, 6703607. [Google Scholar] [CrossRef]
- Xu, G.; Jian, L.; Gong, W.; Zhao, W. Quantitative comparison of flux-modulated interior permanent magnet machines with distributed windings and concentrated windings. Prog. Electromagn. Res. 2012, 129, 109–123. [Google Scholar] [CrossRef]
- Jian, L.; Shi, Y.; Liu, C.; Xu, G.; Gong, Y.; Chan, C.C. A novel dual-permanent-magnet-excited machine for low-speed large-torque applications. IEEE Trans. Magn. 2013, 49, 2381–2384. [Google Scholar] [CrossRef]
- Su, P.; Wang, Y.; Li, Y.; Hua, W.; Shen, Y. Design and analysis of axial-modular flux-switching permanent magnet machine. IEEE Trans. Transp. Electrif. 2023, 9, 3649–3661. [Google Scholar] [CrossRef]
- Meng, Y.; Fang, S.; Pan, Z.; Liu, W.; Qin, L. Design and analysis of a new partitioned stator hybrid-excited flux reversal machine with dual-PM. IEEE Trans. Magn. 2022, 58, 8100806. [Google Scholar] [CrossRef]
- Yang, H.; Zhu, Z.Q.; Lin, H.; Li, H.; Lyu, S. Analysis of consequent-pole flux reversal permanent magnet machine with biased flux modulation theory. IEEE Trans. Ind. Electron. 2020, 67, 2107–2121. [Google Scholar] [CrossRef]
- Zahid, A.; Khan, F.; Ahmad, N.; Sami, I.; Ullah, W.; Ullah, N.; Ullah, N.; Alkhammash, H.I. Design and analysis of dual mover multi-tooth permanent magnet flux switching machine for ropeless elevator applications. Actuators 2021, 10, 81. [Google Scholar] [CrossRef]
- Meng, Y.; Fang, S.; Li, Y.; Zhong, Y.; Qin, L. Design and analysis of new dual-stator flux modulated machines with dual-PM excitation. IEEE Trans. Ind. Appl. 2023, 59, 1383–1393. [Google Scholar] [CrossRef]
- Yu, J.; Liu, C.; Liu, S.; Zhao, H. Comparative study of double-stator interior-PM vernier machines based on electromagnetic-structural coupling analysis. IEEE Trans. Ind. Electron. 2021, 68, 10510–10520. [Google Scholar] [CrossRef]
- Zhang, X.; Yang, H.; Li, Y.; Niu, S. Comparative study of novel dual-stator machines having different biased PM configurations. IEEE Trans. Magn. 2022, 58, 8103706. [Google Scholar] [CrossRef]
- Yang, H.; Lyu, S.; Zhu, Z.Q.; Lin, H.; Wang, S.; Fang, S.; Huang, Y. Novel Dual-Stator Machines With Biased Permanent Magnet Excitation. IEEE Trans. Energy Convers. 2018, 33, 2070–2080. [Google Scholar] [CrossRef]
- Baloch, N.; Kwon, B.-I.; Gao, Y. Low-Cost High-torque-density dual-stator consequent-pole permanent magnet vernier machine. IEEE Trans. Magn. 2018, 54, 8206105. [Google Scholar] [CrossRef]
- Liu, X.; Zhao, Y.; Zhu, J.; Chen, Z.; Huang, S. Multi-objective robust optimization of a dual-flux-modulator magnetic geared machine with hybrid uncertainties. IEEE Trans. Energy Convers. 2020, 35, 2106–2115. [Google Scholar] [CrossRef]
- Kim, D.; Hwang, H.; Bae, S.; Lee, C. Analysis and design of a double-stator flux-switching permanent magnet machine using ferrite magnet in hybrid electric vehicles. IEEE Trans. Magn. 2016, 52, 8106604. [Google Scholar] [CrossRef]
- Ho, S.L.; Niu, S.; Fu, W.N. A new dual-stator bidirectional-modulated pm machine and its optimization. IEEE Trans. Magn. 2014, 50, 8103404. [Google Scholar] [CrossRef]
- Ding, Z.; He, C.; Feng, C.; Yang, J. A new dual stator permanent magnet machine based on field modulation theory. Sustainability 2023, 15, 281. [Google Scholar] [CrossRef]
- Meng, Y.; Fang, S.; Zhu, Y.; Yu, Y.; Qin, L. Investigation of new dual-stator consequent-pole flux reversal permanent magnet arc machines. IEEE Trans. Appl. Superconduct. 2024, 1–5. [Google Scholar] [CrossRef]
- Wang, H.; Fang, S.; Lu, X.; Ni, H.; Yang, H.; Lin, H. Analysis of a new dual-stator vernier machine with hybrid magnet flux-reversal arrangement. IEEE Trans. Appl. Superconduct. 2019, 29, 0600805. [Google Scholar] [CrossRef]
- Pan, Z.; Fang, S.; Lin, H.; Yang, H.; Xue, S. A new double-sided flux reversal arc permanent magnet machine with enhanced torque density capability. IEEE Trans. Magn. 2019, 55, 8102506. [Google Scholar] [CrossRef]
- Meng, Y.; Fang, S.; Wang, H.; Pan, Z.; Qin, L. Design and analysis of a new dual-stator consequent-pole flux reversal machine with triple-PM excitation. IEEE Trans. Magn. 2021, 57, 8105904. [Google Scholar] [CrossRef]
- Kwon, J.-W.; Kwon, B.-I. High-efficiency dual output stator-PM machine for the two-mode operation of washing machines. IEEE Trans. Energy Convers. 2018, 33, 2050–2059. [Google Scholar] [CrossRef]
- Yu, J.; Liu, C.; Zhao, H. Design and optimization procedure of a mechanical-offset complementary-stator flux-reversal permanent-magnet machine. IEEE Trans. Magn. 2019, 55, 8204007. [Google Scholar] [CrossRef]
- Wei, L.; Nakamura, T. A novel dual-stator hybrid excited permanent magnet vernier machine with Halbach-array PMs. IEEE Trans. Magn. 2021, 57, 8101705. [Google Scholar] [CrossRef]
- Shi, Y.; Jian, L. A novel dual-permanent-magnet-excited machine with flux strengthening effect for low-speed large-torque applications. Energies 2018, 11, 153. [Google Scholar] [CrossRef]
- Cao, L.; Zhou, Y.; Yang, G.; He, Y.; Xie, S.; Lee, C.H.T. Decoupling analysis of brushless dual-mechanical-port dual- electrical-port machines. IEEE Trans. Ind. Electron. 2024, 71, 4361–4374. [Google Scholar] [CrossRef]
- Wang, H.; Xu, W.; Xu, Y.; He, C. Design and analysis of permanent magnet machines with multiple arc pole ratios in stator teeth. IEEE Trans. Energy Convers. 2024, 39, 1278–1287. [Google Scholar] [CrossRef]
- Jian, L.; Deng, Z.; Shi, Y.; Wei, J.; Chan, C.C. The mechanism how coaxial magnetic gear transmits magnetic torques between its two rotors: Detailed analysis of torque distribution on modulating ring. IEEE/ASME Trans. Mechatron. 2019, 24, 763–773. [Google Scholar] [CrossRef]
- Ma, C.; Qu, L. Multiobjective optimization of switched reluctance motors based on design of experiments and particle swarm optimization. IEEE Trans. Energy Convers. 2015, 30, 1144–1153. [Google Scholar] [CrossRef]



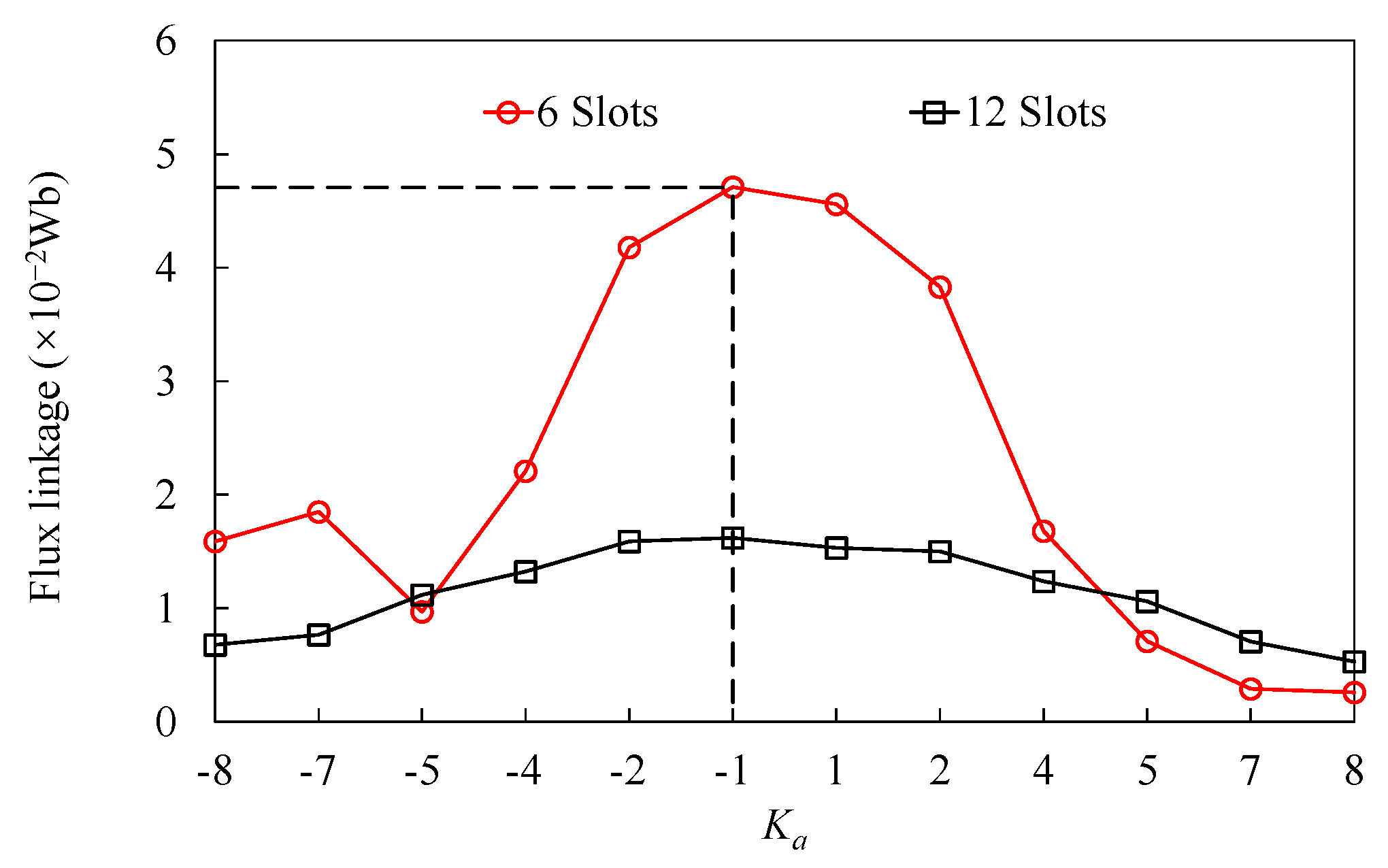
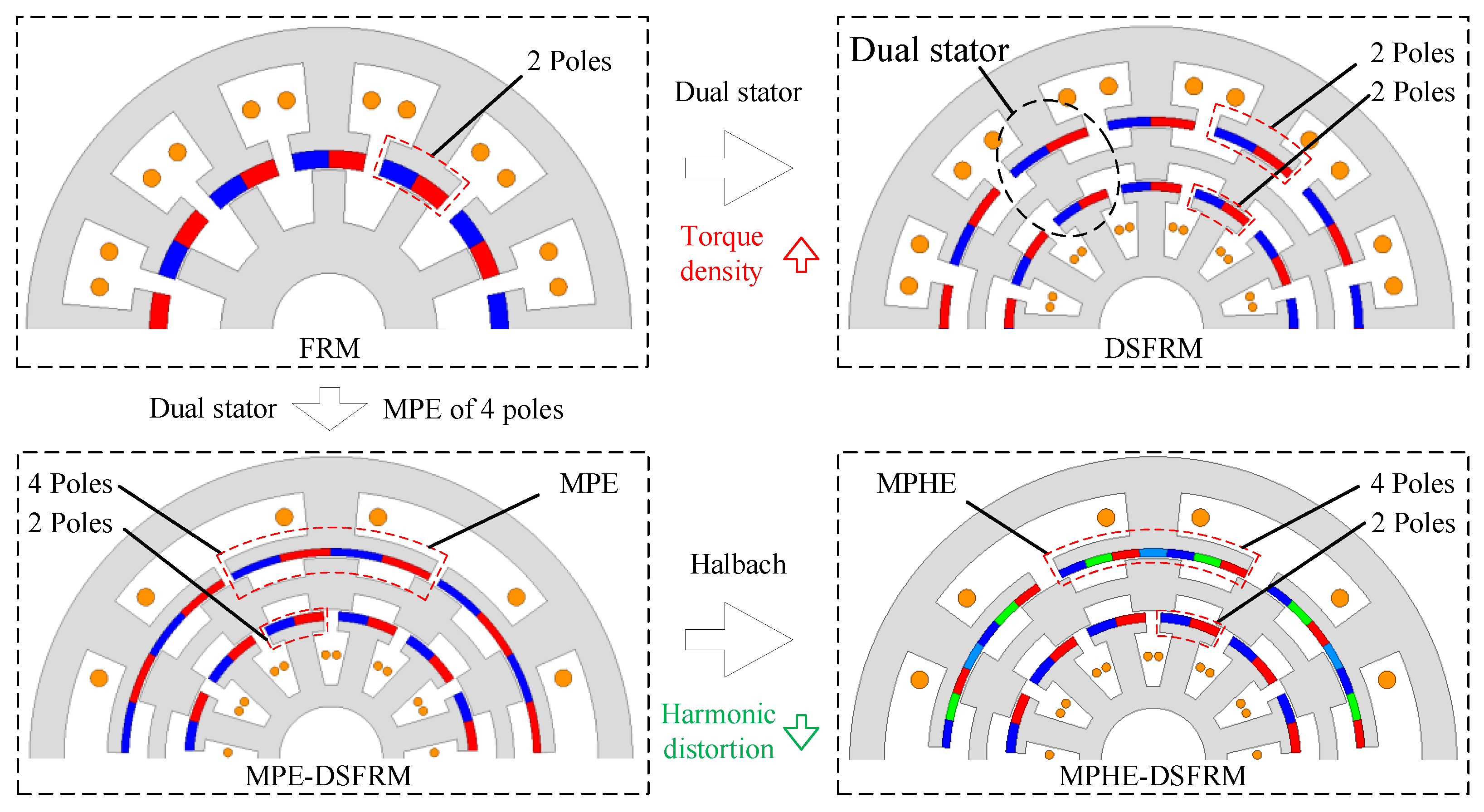
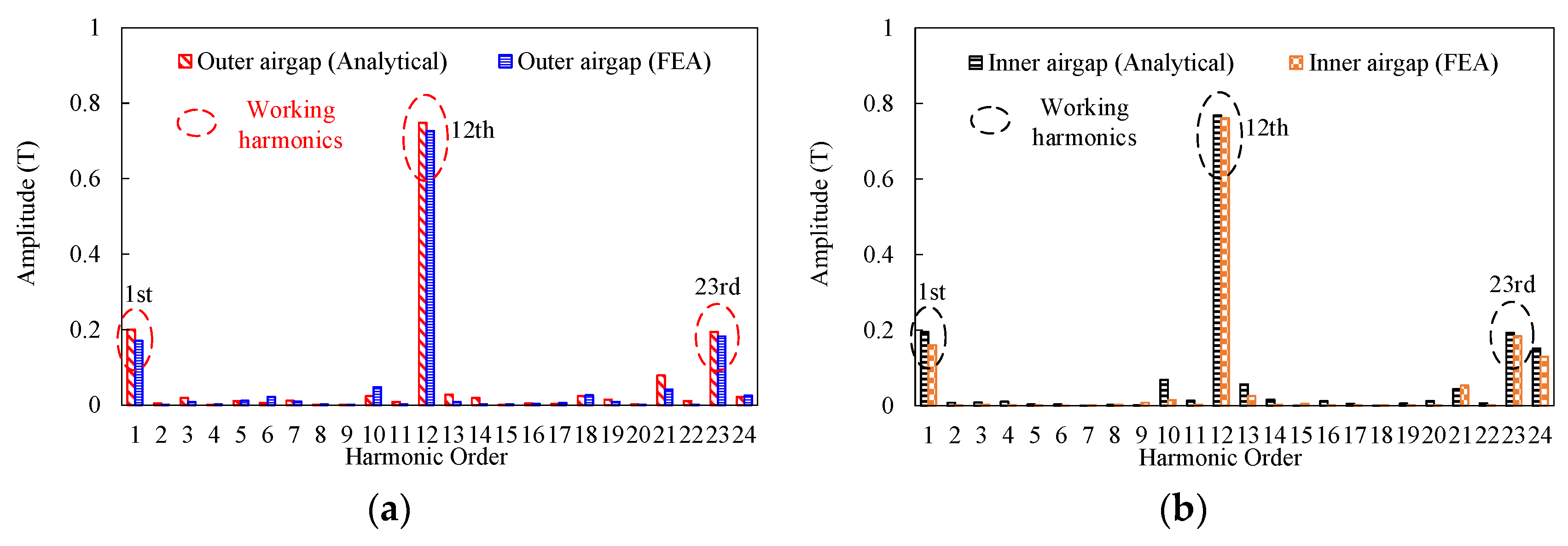
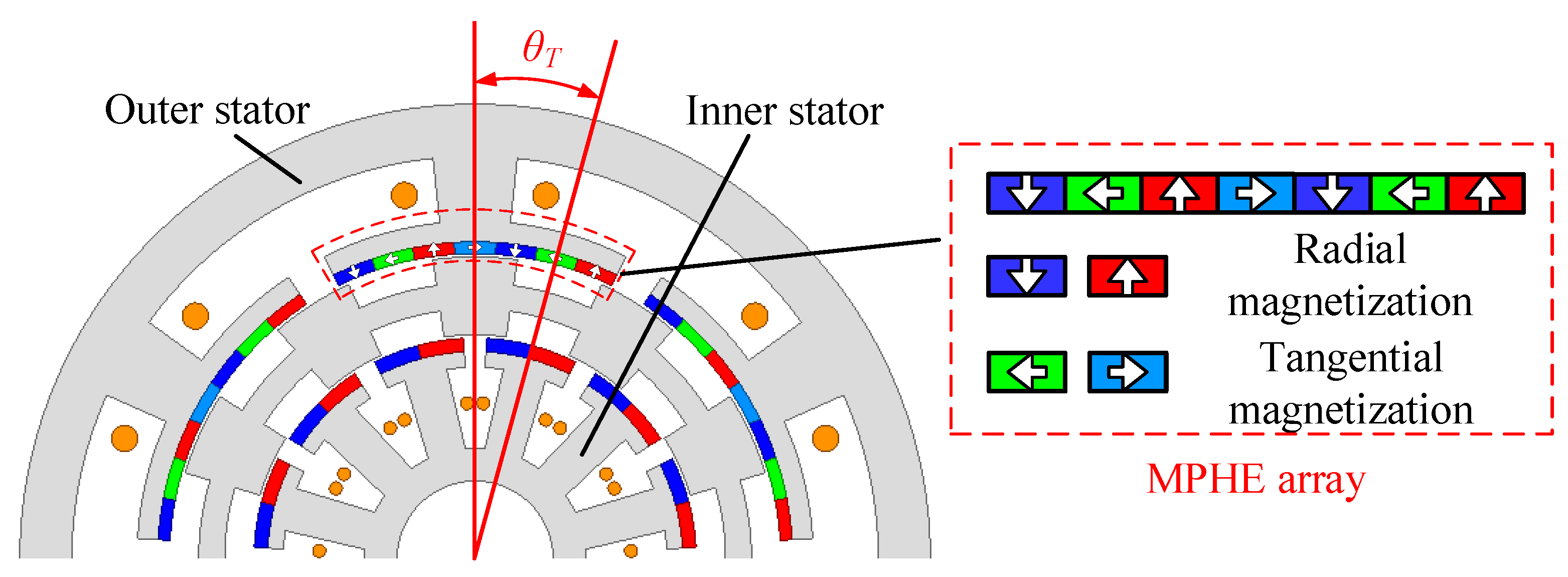






| Ns | Nr | PPN | kd | kp | kw | Ub (V) | ψf (10−2 Wb) | Ns | Nr | PPN | kd | kp | kw | Ub (V) | ψf (10−2 Wb) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 6 | 4 | 8 | 1 | 0.866 | 0.866 | 5.27 | 1.59 | 12 | 16 | 8 | 1 | 0.866 | 0.866 | 1.55 | 0.68 |
| 5 | 7 | 1 | 0.5 | 0.5 | 10.51 | 1.85 | 17 | 7 | 1 | 0.933 | 0.933 | 2.34 | 0.77 | ||
| 7 | 5 | 1 | 0.5 | 0.5 | 1.52 | 0.97 | 19 | 5 | 1 | 0.933 | 0.933 | 3.51 | 1.12 | ||
| 8 | 4 | 1 | 0.866 | 0.866 | 7.03 | 2.21 | 20 | 4 | 1 | 0.866 | 0.866 | 4.13 | 1.33 | ||
| 10 | 2 | 1 | 0.866 | 0.866 | 6.68 | 4.18 | 22 | 2 | 1 | 0.5 | 0.5 | 4.95 | 1.59 | ||
| 11 | 1 | 1 | 0.5 | 0.5 | 14.79 | 4.71 | 23 | 1 | 1 | 0.5 | 0.5 | 5.07 | 1.62 | ||
| 13 | 1 | 1 | 0.5 | 0.5 | 14.20 | 4.56 | 25 | 1 | 1 | 0.5 | 0.5 | 4.77 | 1.53 | ||
| 14 | 2 | 1 | 0.866 | 0.866 | 6.12 | 3.83 | 26 | 2 | 1 | 0.5 | 0.5 | 4.71 | 1.50 | ||
| 16 | 4 | 1 | 0.866 | 0.866 | 4.98 | 1.68 | 28 | 4 | 1 | 0.866 | 0.866 | 3.89 | 1.24 | ||
| 17 | 5 | 1 | 0.5 | 0.5 | 1.20 | 0.71 | 29 | 5 | 1 | 0.933 | 0.933 | 3.28 | 1.06 | ||
| 19 | 7 | 1 | 0.5 | 0.5 | 0.94 | 0.29 | 31 | 7 | 1 | 0.933 | 0.933 | 2.17 | 0.71 | ||
| 20 | 8 | 1 | 0.866 | 0.866 | 0.91 | 0.26 | 32 | 8 | 1 | 0.866 | 0.866 | 1.67 | 0.53 |
| Items | S(yi) | C(yi) | Sensitivity | |
|---|---|---|---|---|
| Savg | Srip | |||
| θT | −0.38 | 0.02 | 0.24 | √ |
| θPM | 0.30 | 0.21 | 0.26 | √ |
| θp | −0.45 | 0.13 | 0.32 | √ |
| θr | −0.09 | 0.10 | 0.09 | × |
| θq | −0.07 | 0.05 | 0.06 | × |
| Items | Model I | Model II | Model III | Model IV |
|---|---|---|---|---|
| Outer/inner pole number of PM | 4/2 | 4/2 | 2/2 | 2/- |
| Outer/inner stator slot number | 6/12 | 6/12 | 12/12 | 12/- |
| Outer/inner diameter of outer stator (mm) | 140/97.62 | 140/97.37 | 140/98 | 140/83 |
| Outer/inner diameter of inner stator (mm) | 64/24 | - | ||
| Outer/inner diameter of rotor (mm) | 93/69 | 73.8/26 | ||
| Active length of outer/inner airgap (mm) | 0.5/0.5 | 0.5/- | ||
| PPN of rotor | 11 | |||
| Active stack length (mm) | 20 | |||
| PPN of the outer/inner PM | 12/12 | 12/- | ||
| PPN of the outer/inner armature reaction | 1/1 | 1/- | ||
| PM volume (cm3) | 16.26 | |||
| PM grade | N38SH | |||
| Rated speed (rpm) | 273 | |||
| RMS value of rated current (Arms) | 2.97 | |||
| Current density at rated state (A/mm2) | 5.35 | |||
| θT (deg.) | 15 | 15 | 0 | - |
| Outer/inner θPM (deg.) | 7.57/12 | 14.25/12 | 12/12 | 12/- |
| θr (deg.) | 16 | 16 | 12 | 12 |
| θp (deg.) | 0 | 0 | 0 | - |
| θq (deg.) | −3 | −6 | 0 | - |
| Items | Model I | Model II | Model III | Model IV |
|---|---|---|---|---|
| Amplitude of back-EMF (V) | 26.51 | 24.93 | 14.87 | 11.06 |
| THD of back-EMF (%) | 9.68 | 6.29 | 1.06 | 2.02 |
| Peak-to-peak cogging torque (mNm) | 307 | 354 | 118 | 65 |
| Steady torque at rated state (Nm) | 4.32 | 4.59 | 3.25 | 2.44 |
| Torque ripple (%) | 3.40 | 4.38 | 4.12 | 2.68 |
| Torque quality, TQ | 1.27 | 1.05 | 0.79 | 0.91 |
| Machine volume (cm3) | 307.87 | |||
| Machine weight (kg) | 2.42 | |||
| Torque density (×10−2 Nm/cm3) | 1.40 | 1.49 | 1.05 | 0.79 |
| Specific torque (Nm/kg) | 1.78 | 1.89 | 1.34 | 1.01 |
| Torque per PM volume (Nm/cm3) | 0.265 | 0.282 | 0.199 | 0.150 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, S.; Xu, Y.; He, C.; Yang, J. A High Torque Density Dual-Stator Flux-Reversal-Machine with Multiple Poles Halbach Excitation on Outer Stator. Actuators 2024, 13, 275. https://doi.org/10.3390/act13080275
Tang S, Xu Y, He C, Yang J. A High Torque Density Dual-Stator Flux-Reversal-Machine with Multiple Poles Halbach Excitation on Outer Stator. Actuators. 2024; 13(8):275. https://doi.org/10.3390/act13080275
Chicago/Turabian StyleTang, Siwei, Yuanying Xu, Chao He, and Jiquan Yang. 2024. "A High Torque Density Dual-Stator Flux-Reversal-Machine with Multiple Poles Halbach Excitation on Outer Stator" Actuators 13, no. 8: 275. https://doi.org/10.3390/act13080275
APA StyleTang, S., Xu, Y., He, C., & Yang, J. (2024). A High Torque Density Dual-Stator Flux-Reversal-Machine with Multiple Poles Halbach Excitation on Outer Stator. Actuators, 13(8), 275. https://doi.org/10.3390/act13080275






