Cooperative Integrated Guidance and Control for Active Target Protection in Three-Player Conflict
Abstract
1. Introduction
- Advanced IGC Modeling for Active Protection: An advanced modeling approach for active protection is introduced that uniquely combines linearization of both translational and rotational dynamics around the center of mass. Although the linearization technique is a well-established practice in aerial vehicle design, our contribution lies in applying this approach to the IGC design in a three-player conflict scenario. This modeling framework allows for a more comprehensive and accurate representation of the characteristics of aerial vehicles compared to previous studies.
- Analytical Derivation and Solution of Riccati Equation: Through the application of differential game theory and the sweep method, this study derives and solves the Riccati differential equation, providing an analytical expression for the optimal control strategy and in turn presenting possibilities for real-time onboard calculation.
- Theoretical Rigor in Solution Analysis: The theoretical rigor of the proposed approach is provided through a proof of optimality sufficiency. Additionally, we examine factors that influence the computational accuracy of the Riccati equation solution using singular values of the control matrix and condition numbers of the solution matrix.
2. Problem Formulation
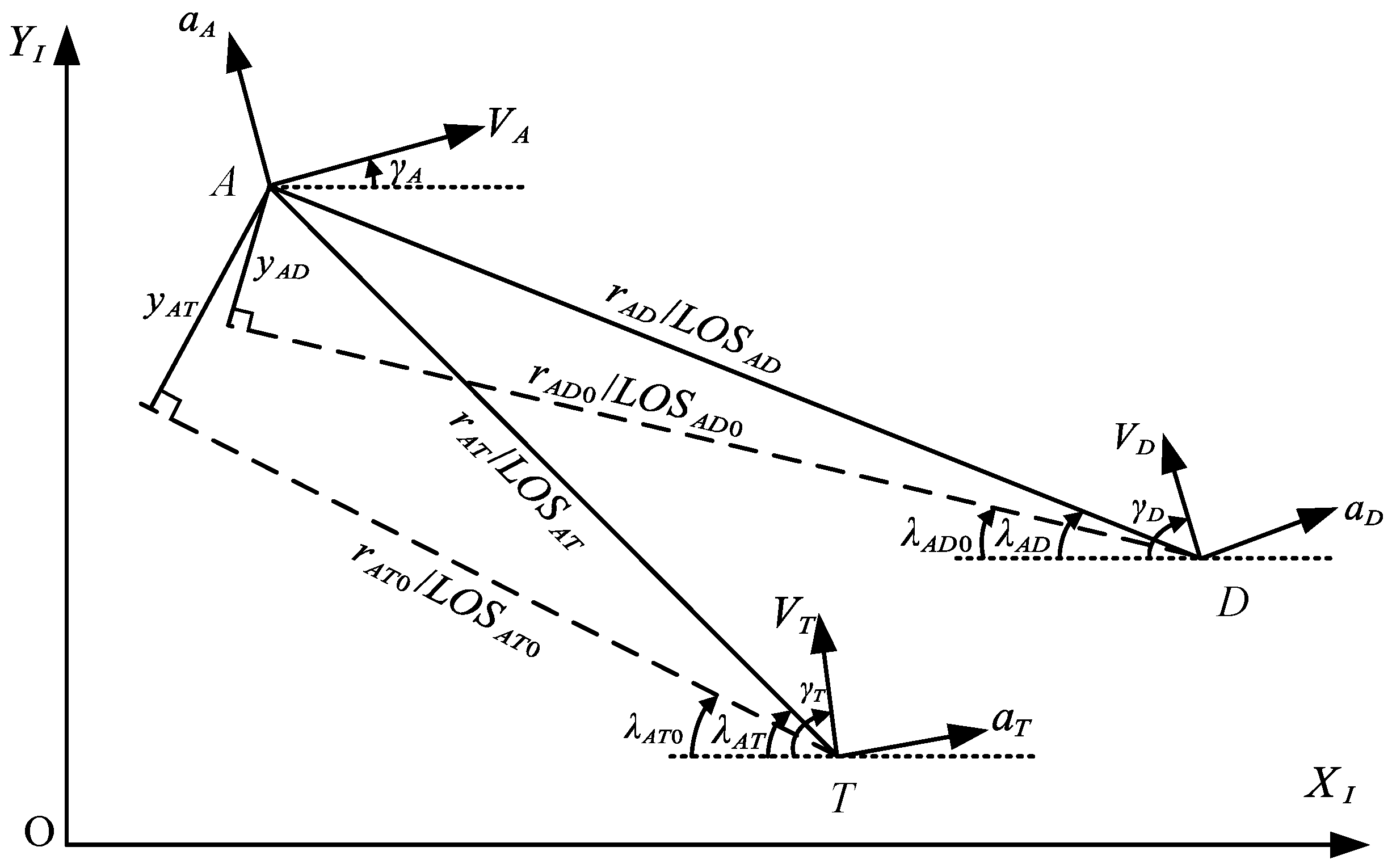
2.1. Nonlinear Engagement Model
2.2. Linearization and Order Reduction
- Constant Velocity: The velocities of the entities are assumed to be constant.
- Co-planar Movement: The three entities are considered to move within the same plane; the defender is launched by the target, meaning that and coincide.
- Linear Plant Models: The dynamics of each entity are approximated as linear systems.
- Linearization of Collision Triangles: It is assumed that the two collision triangles can be linearized along their respective initial lines of sight (LOS).
- Linearization of the airframe: Linearization of the airframe dynamics is typically performed around a trim condition, which represents a steady-state flight condition; in our case, this corresponds to flight at a constant velocity and a small angle of attack. This approach is widely accepted in aerospace control systems design, especially for short-period dynamics, as deviations from this trim condition during maneuvers are usually small enough to maintain the validity of the linear model.
- Linearization of engagement geometry: For the engagement geometry, we employ linearization around a nominal collision course. This approach is commonly used and justified in terminal guidance problems, particularly in the endgame phase, where the relative geometry changes are relatively small and can be approximated linearly.
3. Derivation of IGC Active Protection Strategy
4. Theoretical Analysis of IGC Active Protection Strategy
4.1. Saddle Point Sufficiency Proof
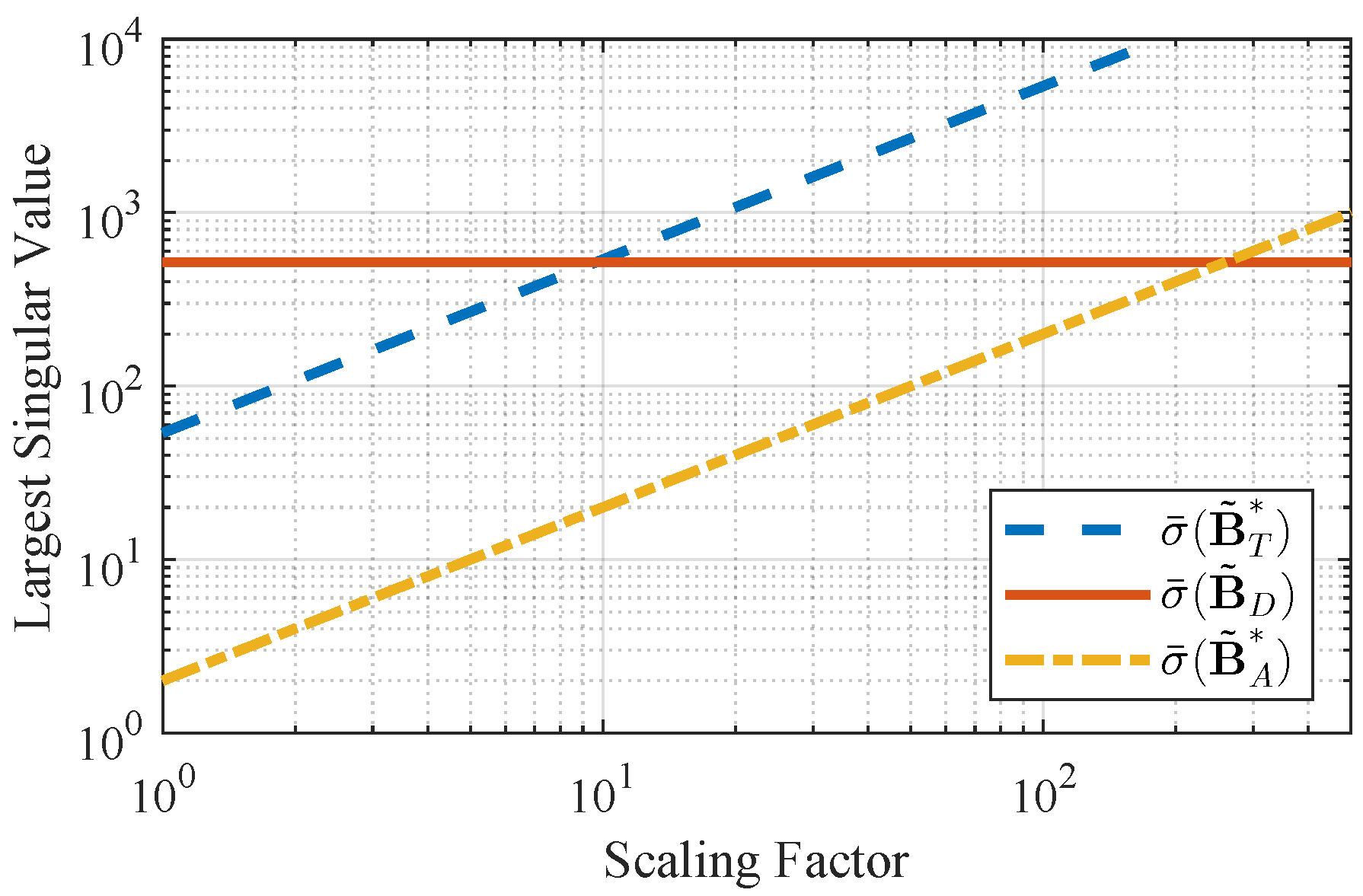
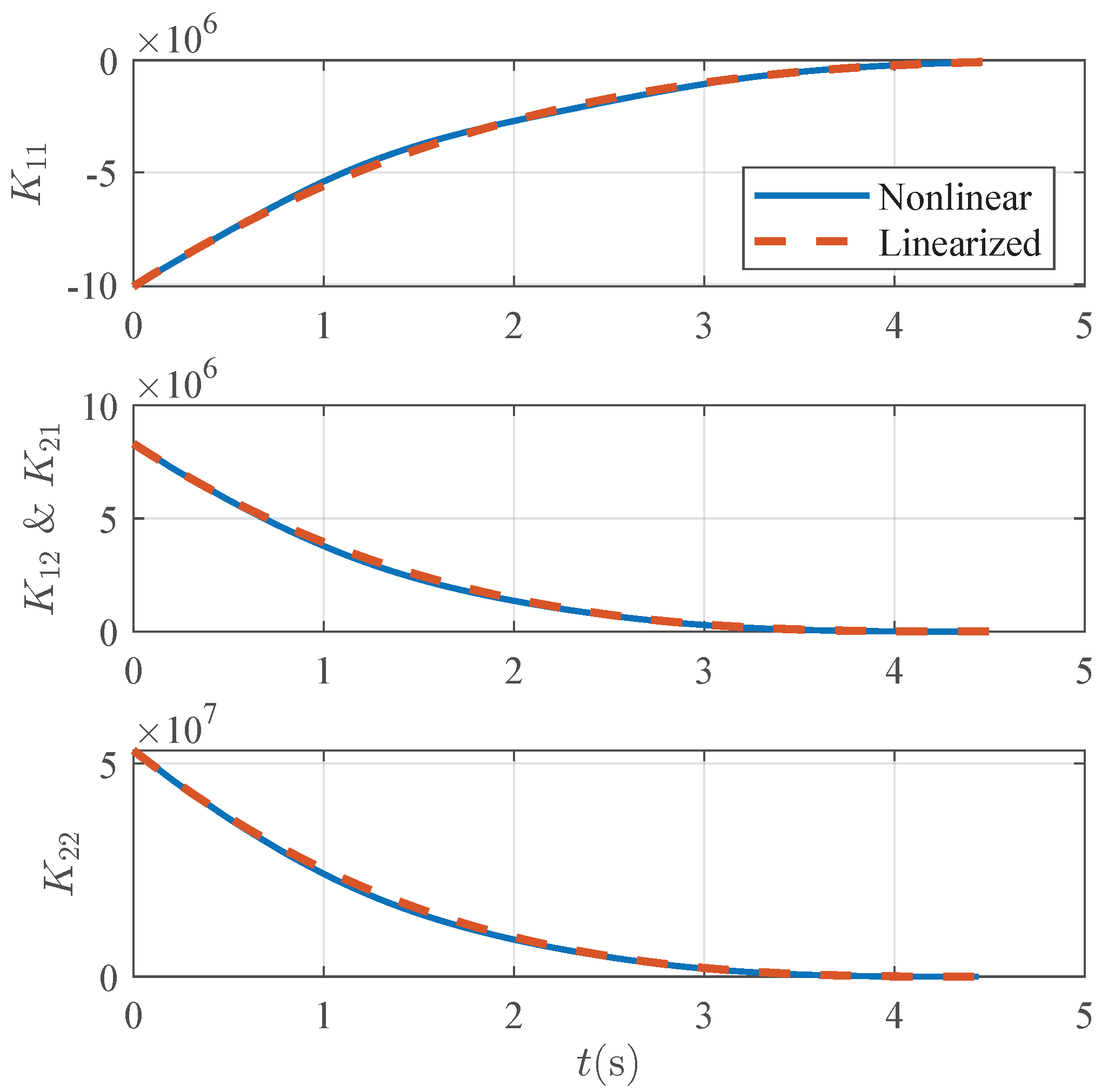
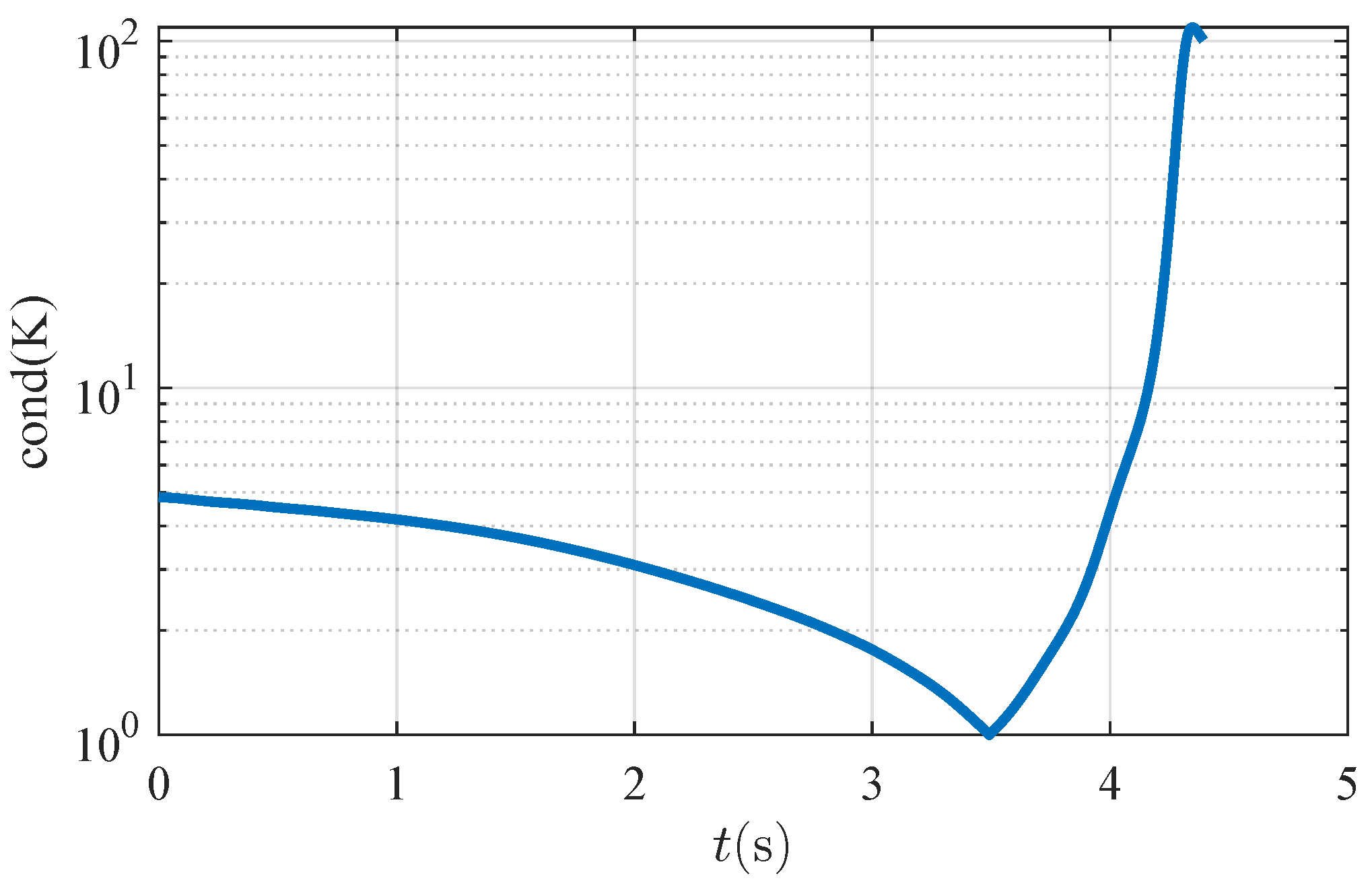
4.2. Analysis of the Invertibility of Matrix
4.3. Simplified IGC Active Protection Strategy
5. Simulation Results and Analysis
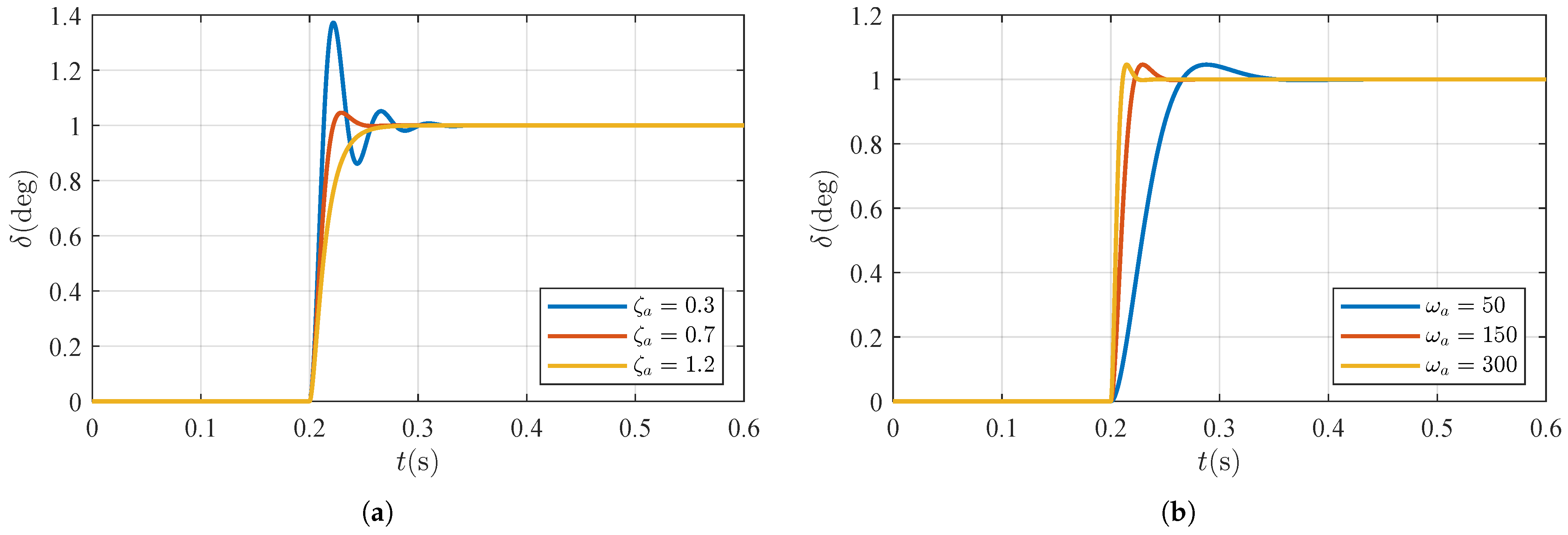
5.1. Simulation Setup
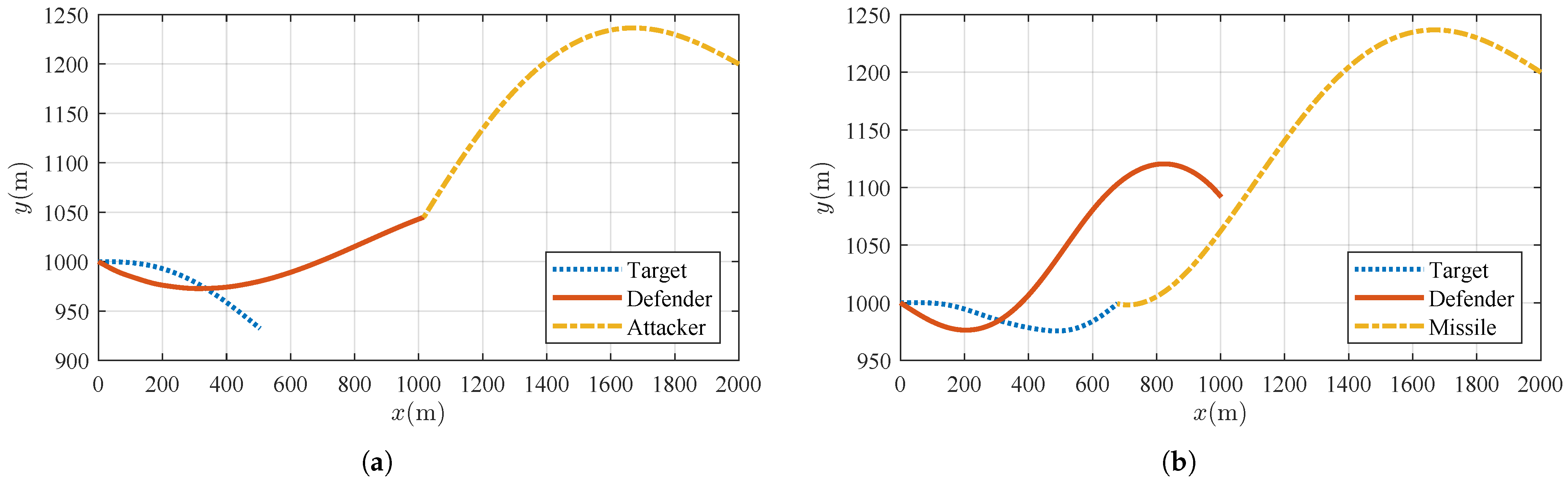
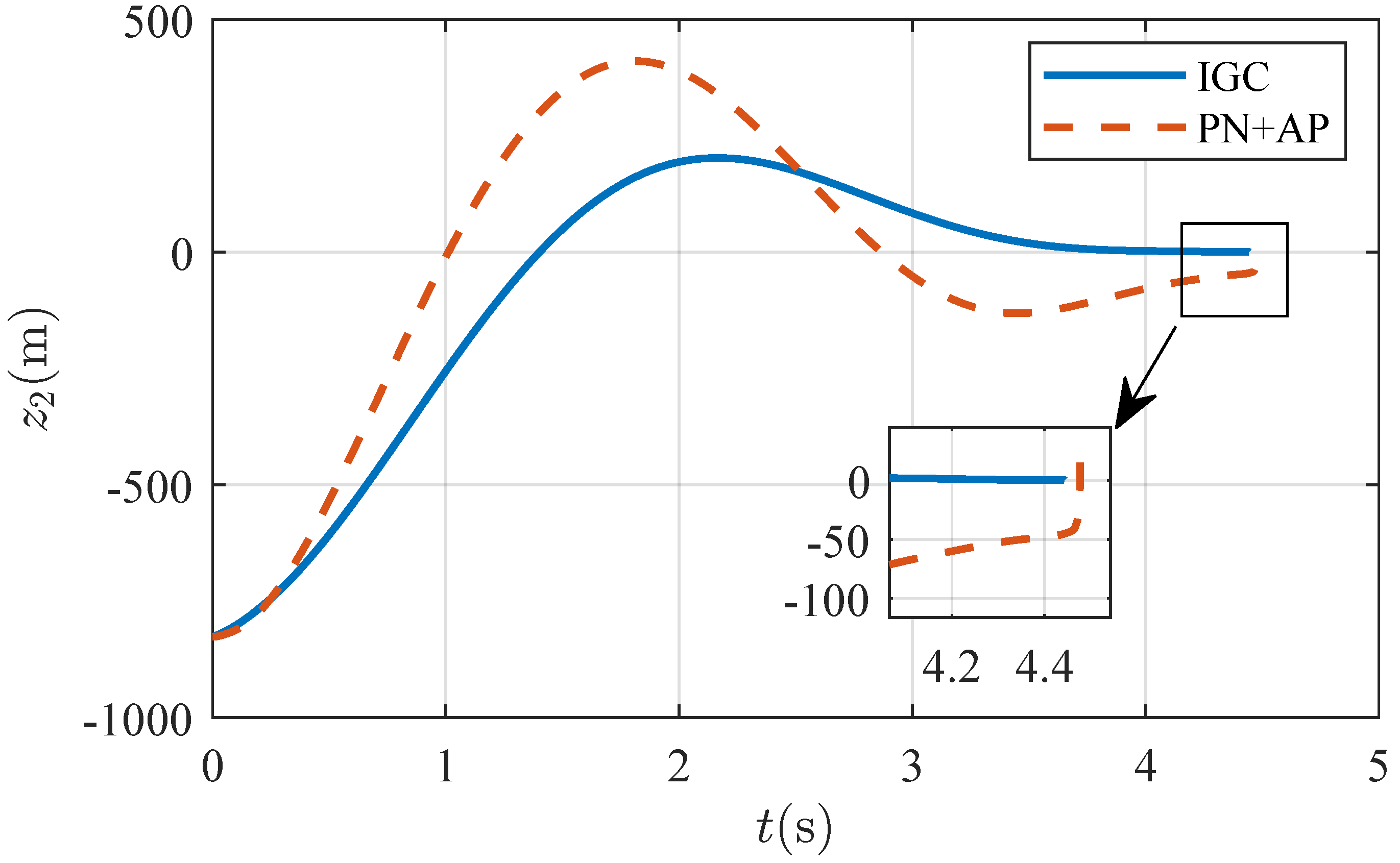
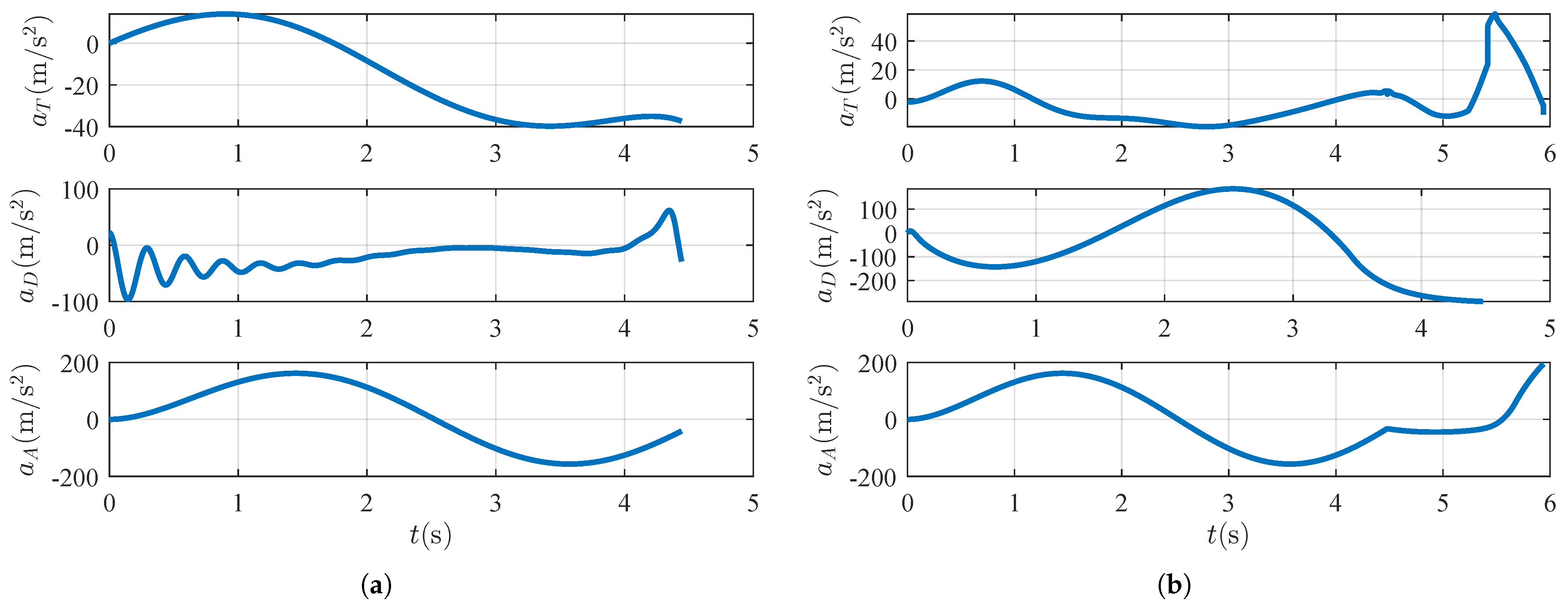
5.2. Simulation Scenario I
5.3. Simulation Scenario II
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Isaacs, R. Differential Games: A Mathematical Theory with Applications to Warfare and Pursuit, Control and Optimization; Dover Publications: Mineola, NY, USA, 1999. [Google Scholar]
- Wei, X.; Yang, J. Optimal Strategies for Multiple Unmanned Aerial Vehicles in a Pursuit/Evasion Differential Game. J. Guid. Control Dyn. 2018, 41, 1799–1806. [Google Scholar] [CrossRef]
- Hayoun, S.Y.; Shima, T. On guaranteeing point capture in linear n -on-1 endgame interception engagements with bounded controls. Automatica 2017, 85, 122–128. [Google Scholar] [CrossRef]
- Buzikov, M.; Galyaev, A. The Game of Two Identical Cars: An Analytical Description of the Barrier. J. Optim. Theory Appl. 2023, 198, 988–1018. [Google Scholar] [CrossRef]
- Singh, S.K.; Reddy, P.V. Dynamic Network Analysis of a Target Defense Differential Game with Limited Observations. IEEE Trans. Control Netw. Syst. 2023, 10, 308–320. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, S.; Yao, Y.; Sun, Q.; Wang, Y. A target defence–intrusion game with considering the obstructive effect of target. IET Control Theory Appl. 2024. [Google Scholar] [CrossRef]
- Liang, L.; Deng, F.; Wang, J.; Lu, M.; Chen, J. A Reconnaissance Penetration Game With Territorial-Constrained Defender. IEEE Trans. Autom. Control 2022, 67, 6295–6302. [Google Scholar] [CrossRef]
- Weintraub, I.E.; Pachter, M.; Garcia, E. An Introduction to Pursuit-evasion Differential Games. In Proceedings of the 2020 American Control Conference (ACC), Denver, CO, USA, 1–3 July 2020; pp. 1049–1066. [Google Scholar] [CrossRef]
- Garcia, E.; Casbeer, D.W.; Pachter, M. Pursuit in the Presence of a Defender. Dyn. Games Appl. 2019, 9, 652–670. [Google Scholar] [CrossRef]
- Garcia, E.; Casbeer, D.W.; Pachter, M. The Complete Differential Game of Active Target Defense. J. Optim. Theory Appl. 2021, 191, 675–699. [Google Scholar] [CrossRef]
- Liang, L.; Deng, F.; Peng, Z.; Li, X.; Zha, W. A differential game for cooperative target defense. Automatica 2019, 102, 58–71. [Google Scholar] [CrossRef]
- Liang, L.; Deng, F.; Lu, M.; Chen, J. Analysis of Role Switch for Cooperative Target Defense Differential Game. IEEE Trans. Autom. Control 2021, 66, 902–909. [Google Scholar] [CrossRef]
- Nayak, S.P.; Rajawat, A.P.; Kothari, M. Inverse Geometric Guidance Strategy for a Three-Body Differential Game. In Proceedings of the AIAA Scitech 2021 Forum, Reston, VA, USA, 11–15 & 19–21 January 2021; AIAA SciTech Forum. pp. 1–17. [Google Scholar] [CrossRef]
- Garcia, E.; Casbeer, D.W.; Pachter, M. Cooperative Strategies for Optimal Aircraft Defense from an Attacking Missile. J. Guid. Control Dyn. 2015, 38, 1510–1520. [Google Scholar] [CrossRef]
- Harini Venkatesan, R.; Sinha, N.K. A New Guidance Law for the Defense Missile of Nonmaneuverable Aircraft. IEEE Trans. Control Syst. Technol. 2015, 23, 2424–2431. [Google Scholar] [CrossRef]
- Garcia, E.; Casbeer, D.W.; Fuchs, Z.E.; Pachter, M. Cooperative Missile Guidance for Active Defense of Air Vehicles. IEEE Trans. Aerosp. Electron. Syst. 2018, 54, 706–721. [Google Scholar] [CrossRef]
- Zarchan, P. Tactical and Strategic Missile Guidance, 6th ed.; Progress in Astronautics and Aeronautics; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012; Volume 239. [Google Scholar] [CrossRef]
- Yanushevsky, R. Modern Missile Guidance, 2nd ed.; Taylor et Francis/CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2019. [Google Scholar]
- Shaferman, V.; Oshman, Y. Stochastic Cooperative Interception Using Information Sharing Based on Engagement Staggering. J. Guid. Control Dyn. 2016, 39, 2127–2141. [Google Scholar] [CrossRef]
- Prokopov, O.; Shima, T. Linear Quadratic Optimal Cooperative Strategies for Active Aircraft Protection. J. Guid. Control Dyn. 2013, 36, 753–764. [Google Scholar] [CrossRef]
- Shima, T. Optimal Cooperative Pursuit and Evasion Strategies Against a Homing Missile. J. Guid. Control Dyn. 2011, 34, 414–425. [Google Scholar] [CrossRef]
- Perelman, A.; Shima, T.; Rusnak, I. Cooperative Differential Games Strategies for Active Aircraft Protection from a Homing Missile. J. Guid. Control Dyn. 2011, 34, 761–773. [Google Scholar] [CrossRef]
- Alkaher, D.; Moshaiov, A. Game-Based Safe Aircraft Navigation in the Presence of Energy-Bleeding Coasting Missile. J. Guid. Control Dyn. 2016, 39, 1539–1550. [Google Scholar] [CrossRef]
- Liu, F.; Dong, X.; Li, Q.; Ren, Z. Cooperative differential games guidance laws for multiple attackers against an active defense target. Chin. J. Aeronaut. 2022, 35, 374–389. [Google Scholar] [CrossRef]
- Rubinsky, S.; Gutman, S. Three-Player Pursuit and Evasion Conflict. J. Guid. Control Dyn. 2014, 37, 98–110. [Google Scholar] [CrossRef]
- Rubinsky, S.; Gutman, S. Vector Guidance Approach to Three-Player Conflict in Exoatmospheric Interception. J. Guid. Control Dyn. 2015, 38, 2270–2286. [Google Scholar] [CrossRef]
- Qi, N.; Sun, Q.; Zhao, J. Evasion and pursuit guidance law against defended target. Chin. J. Aeronaut. 2017, 30, 1958–1973. [Google Scholar] [CrossRef]
- Shaferman, V.; Shima, T. Cooperative Multiple-Model Adaptive Guidance for an Aircraft Defending Missile. J. Guid. Control Dyn. 2010, 33, 1801–1813. [Google Scholar] [CrossRef]
- Shaferman, V.; Shima, T. Cooperative Differential Games Guidance Laws for Imposing a Relative Intercept Angle. J. Guid. Control Dyn. 2017, 40, 2465–2480. [Google Scholar] [CrossRef]
- Saurav, A.; Kumar, S.R.; Maity, A. Cooperative Guidance Strategies for Aircraft Defense with Impact Angle Constraints. In Proceedings of the AIAA Scitech 2019 Forum, Reston, VA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]
- Liang, H.; Wang, J.; Liu, J.; Liu, P. Guidance strategies for interceptor against active defense spacecraft in two-on-two engagement. Aerosp. Sci. Technol. 2020, 96, 105529. [Google Scholar] [CrossRef]
- Shalumov, V.; Shima, T. Weapon–Target-Allocation Strategies in Multiagent Target–Missile–Defender Engagement. J. Guid. Control Dyn. 2017, 40, 2452–2464. [Google Scholar] [CrossRef]
- Chen, Z.; Chen, W.; Liu, X.; Cheng, J. Three-dimensional fixed-time robust cooperative guidance law for simultaneous attack with impact angle constraint. Aerosp. Sci. Technol. 2021, 110, 106523. [Google Scholar] [CrossRef]
- Li, G.; Liu, L.; Liu, J.; Wu, Y.; Zhao, J. Three-dimensional low-order fixed-time integrated guidance and control for STT missile with strap-down seeker. J. Frankl. Inst. 2023, 360, 9788–9811. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, X.; Zhang, H.; Zhang, F. Three-dimensional approximate cooperative integrated guidance and control with fixed-impact time and azimuth constraints. Aerosp. Sci. Technol. 2023, 142, 108617. [Google Scholar] [CrossRef]
- Williams, D.; Richman, J.; Friedland, B. Design of an integrated strapdown guidance and control system for a tactical missile. In Proceedings of the Guidance and Control Conference, Reston, VA, USA, 15–August 1983. [Google Scholar] [CrossRef]
- Santoso, F.; Garratt, M.A.; Anavatti, S.G. State-of-the-Art Integrated Guidance and Control Systems in Unmanned Vehicles: A Review. IEEE Syst. J. 2021, 15, 3312–3323. [Google Scholar] [CrossRef]
- Yao, C.; Liu, Z.; Zhou, H.; Gao, C.; Li, J.; Zhang, Z. Integrated guidance and control for underactuated fixed-trim moving mass flight vehicles. Aerosp. Sci. Technol. 2023, 142, 108680. [Google Scholar] [CrossRef]
- Shima, T.; Idan, M.; Golan, O.M. Sliding-Mode Control for Integrated Missile Autopilot Guidance. J. Guid. Control Dyn. 2006, 29, 250–260. [Google Scholar] [CrossRef]
- Xingling, S.; Honglun, W. Back-stepping active disturbance rejection control design for integrated missile guidance and control system via reduced-order ESO. ISA Trans. 2015, 57, 10–22. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Jiang, B.; Zhang, K. Adaptive Fault-Tolerant H-Infinity Output Feedback Control for Lead-Wing Close Formation Flight. IEEE Trans. Syst. Man, Cybern. Syst. 2019, 50, 2804–2814. [Google Scholar] [CrossRef]
- Yan, H.; Hou, M. A Small-Gain Approach for Three-Dimensional Integrated Guidance and Control in Pursuit-Evasion Games. In Proceedings of the 2020 Chinese Control And Decision Conference (CCDC), Hefei, China, 22–24 August 2020; pp. 4069–4076. [Google Scholar] [CrossRef]
- Stevens, B.L.; Lewis, F.L.; Johnson, E.N. Aircraft Control and Simulation: Dynamics, Controls Design, and Autonomous Systems, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Green, M.; Limebeer, D.J.N. Linear Robust Control, dover ed.; Dover Publications Inc.: Mineola, NY, USA, 2012. [Google Scholar]
- Yechout, T.R.; Morris, S.L.; Bossert, D.E.; Hallgren, W.F.; Hall, J.K. Introduction to Aircraft Flight Mechanics: Performance, Static Stability, Dynamic Stability, Classical Feedback Control, and State-Space Foundations, 2nd ed.; AIAA Education Series; American Institute of Aeronautics and Astronautics Inc.: Reston, VA, USA, 2014. [Google Scholar]




| Parameter | Value |
|---|---|
| (0, 1000) m | |
| (0, 1000) m | |
| (2000, 1200) m | |
| 0.5 s | |
| 40 g |
| Noise and Deviation | 0 | 10% | 15% | 25% |
| Success Rate | 100% | 100% | 100% | 100% |
| Initial Parameters | Value |
|---|---|
| (0, 1000) m | |
| (0, 1000) m | |
| (3500, 800) m | |
| 0.5 s | |
| 20 g |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, X.; Chen, W.; Chen, Z. Cooperative Integrated Guidance and Control for Active Target Protection in Three-Player Conflict. Actuators 2024, 13, 245. https://doi.org/10.3390/act13070245
Gong X, Chen W, Chen Z. Cooperative Integrated Guidance and Control for Active Target Protection in Three-Player Conflict. Actuators. 2024; 13(7):245. https://doi.org/10.3390/act13070245
Chicago/Turabian StyleGong, Xiaopeng, Wanchun Chen, and Zhongyuan Chen. 2024. "Cooperative Integrated Guidance and Control for Active Target Protection in Three-Player Conflict" Actuators 13, no. 7: 245. https://doi.org/10.3390/act13070245
APA StyleGong, X., Chen, W., & Chen, Z. (2024). Cooperative Integrated Guidance and Control for Active Target Protection in Three-Player Conflict. Actuators, 13(7), 245. https://doi.org/10.3390/act13070245







