Stability Analysis and Delay Compensation for Space Instable Target Simulator
Abstract
1. Introduction
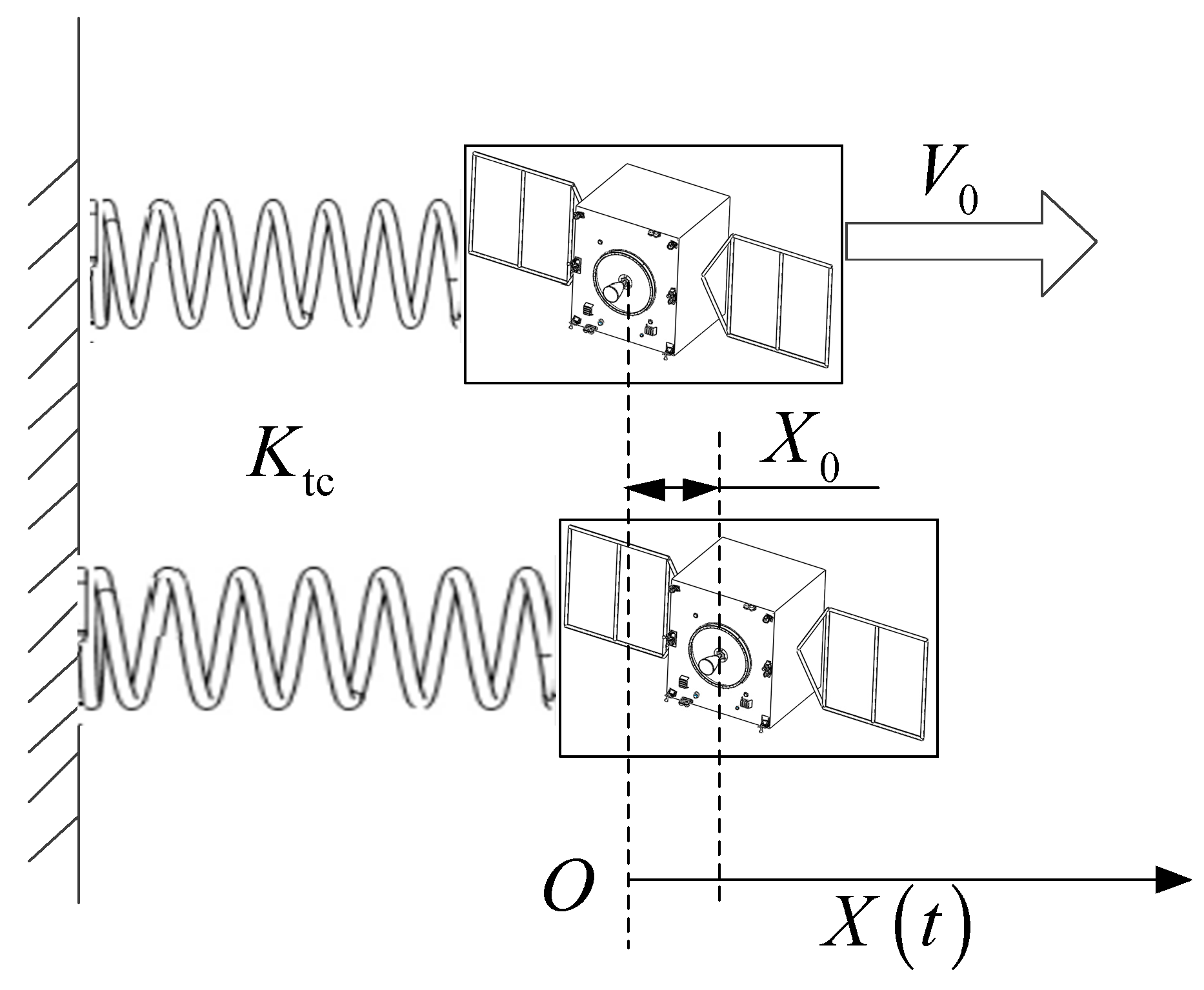
2. Effect of Different Delays on SITS Stability
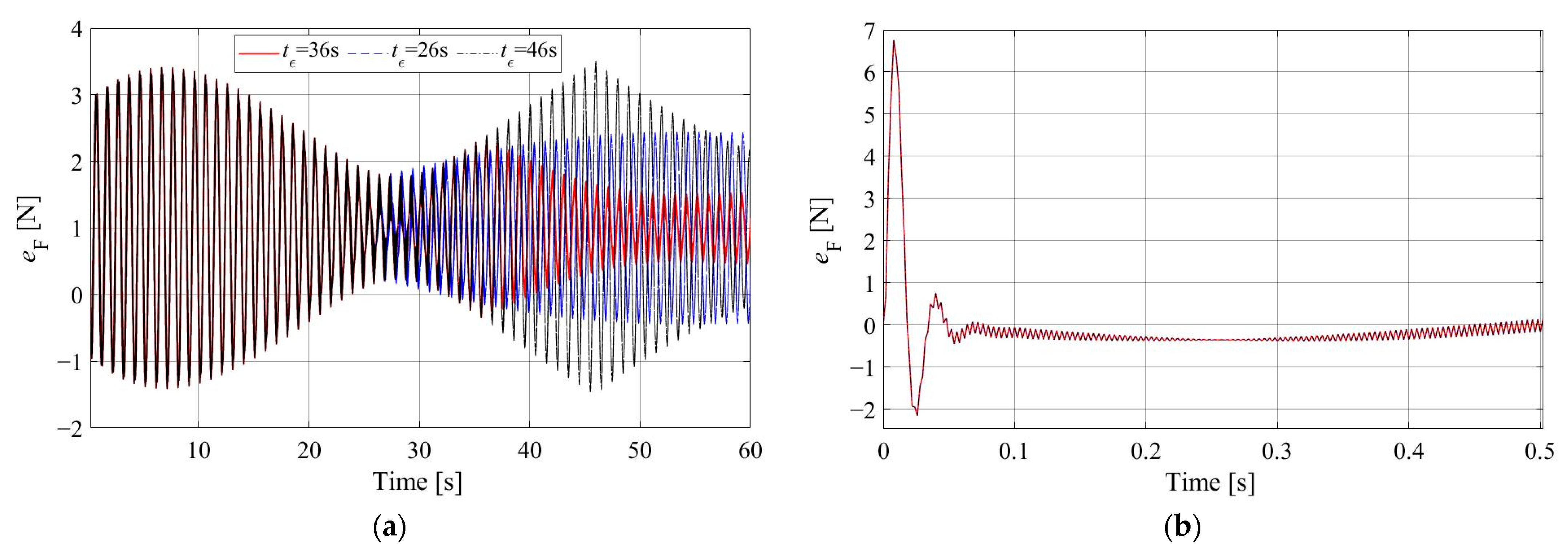
2.1. Effect of Force Measurement Delay on System Stability
2.2. Effect of Control Cycle on System Stability
2.3. Effect of Simulator Response Delay (SRD) on System Stability
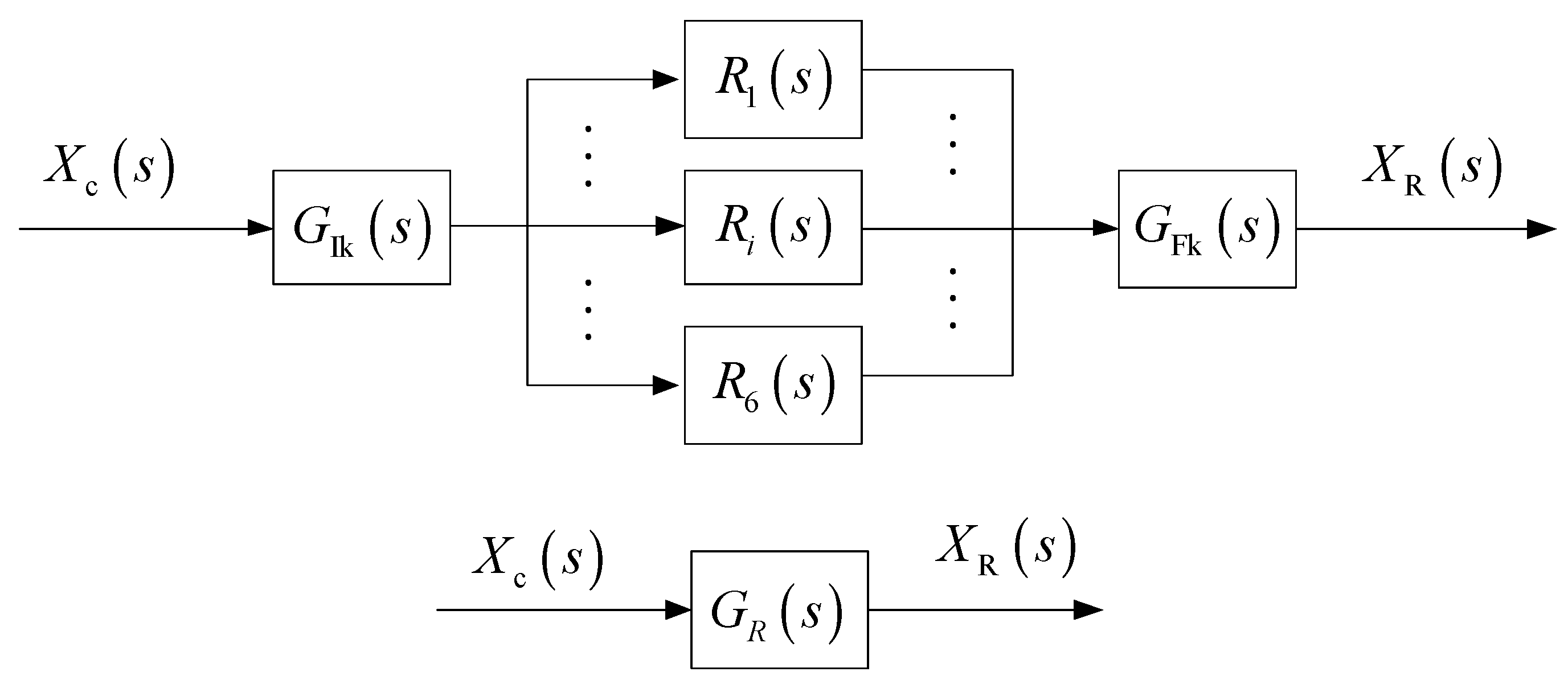
3. Mathematical Modeling and Stability Analysis of Hybrid Simulator
3.1. Mathematical Modeling of Hybrid Simulator
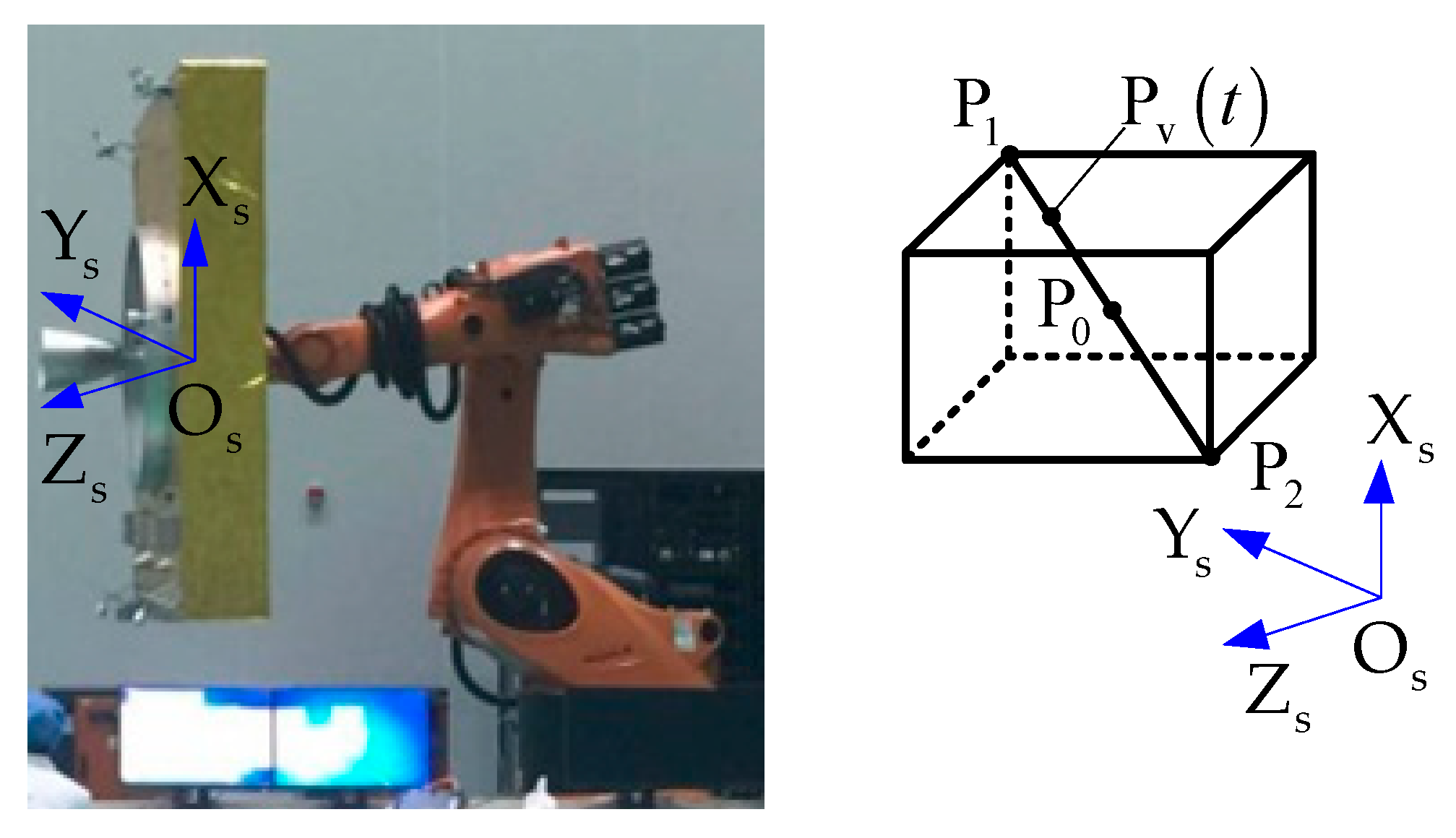
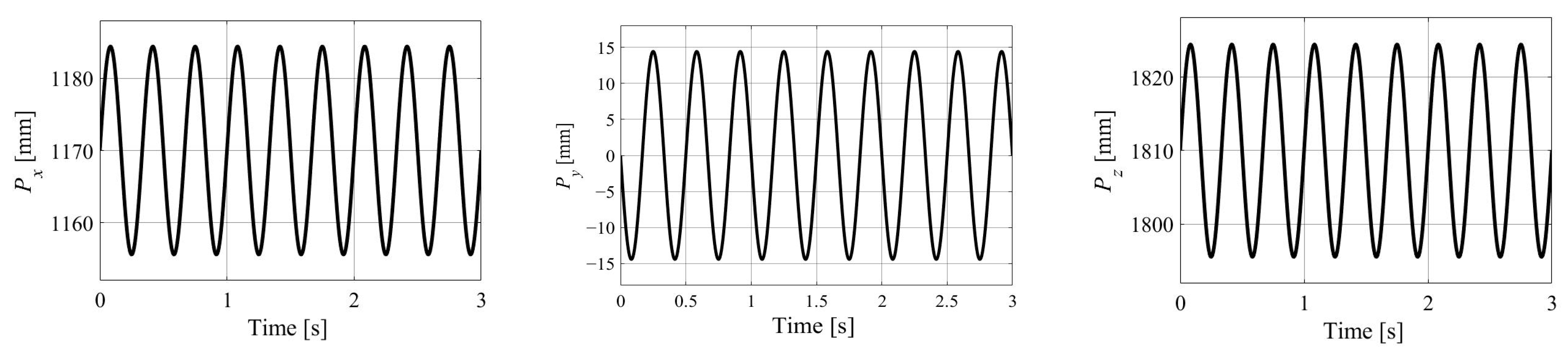
3.2. Model Identification of SITS
3.3. Stability and Steady-State Error Analysis
4. Delay Compensation Method of Hybrid Simulator
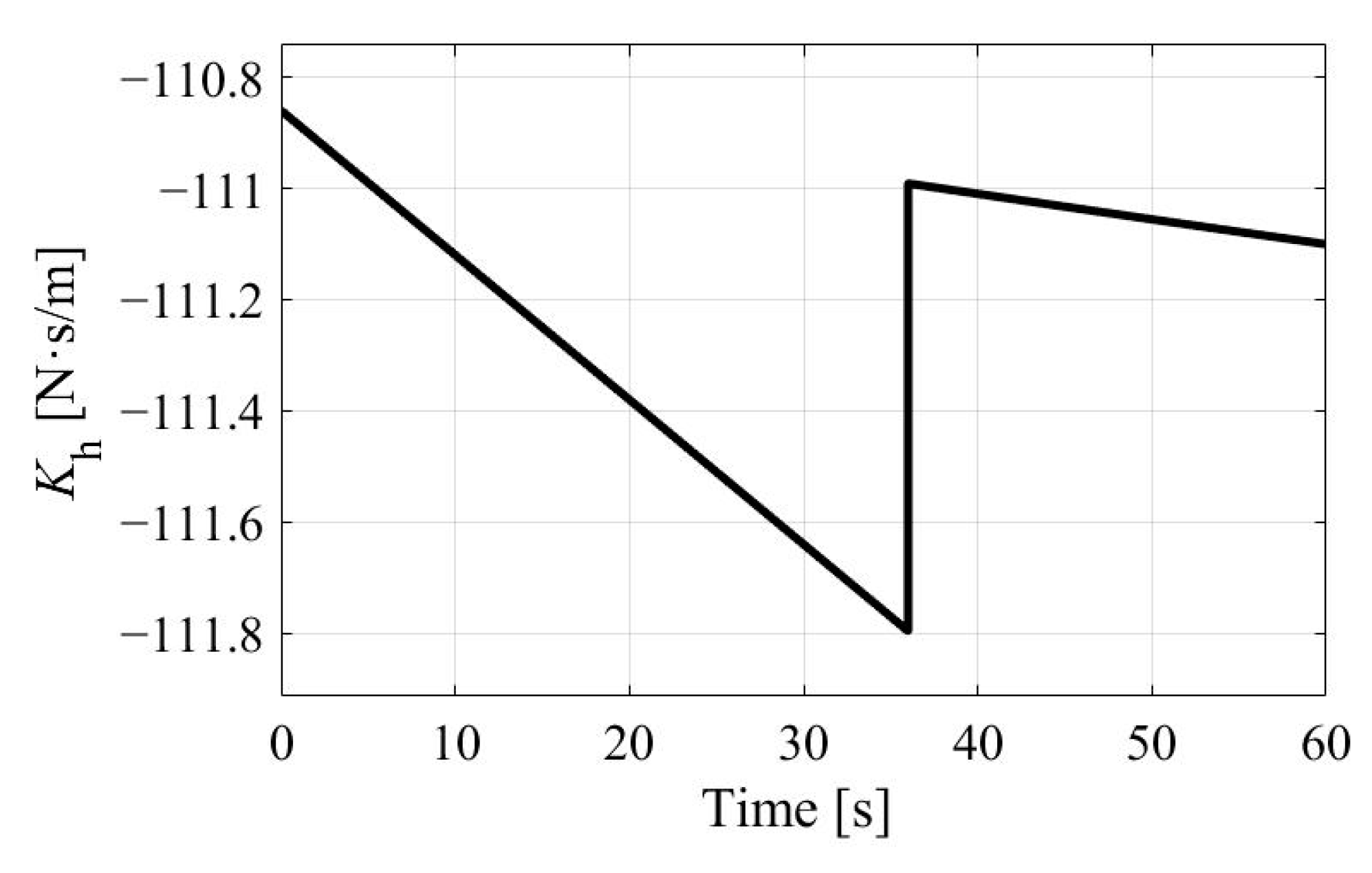
4.1. Modeling of Switching Compensator with Variable Gain
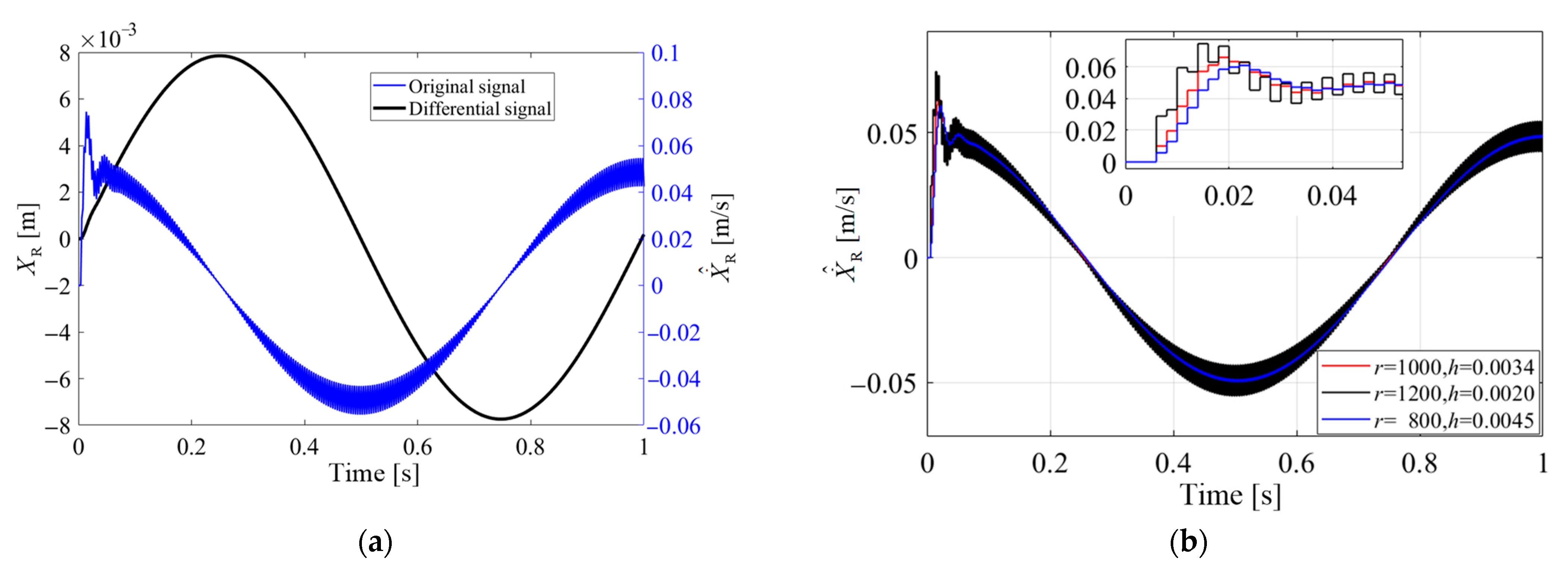
4.2. Velocity Estimation Based on Tracking Differentiator
5. Simulation Study
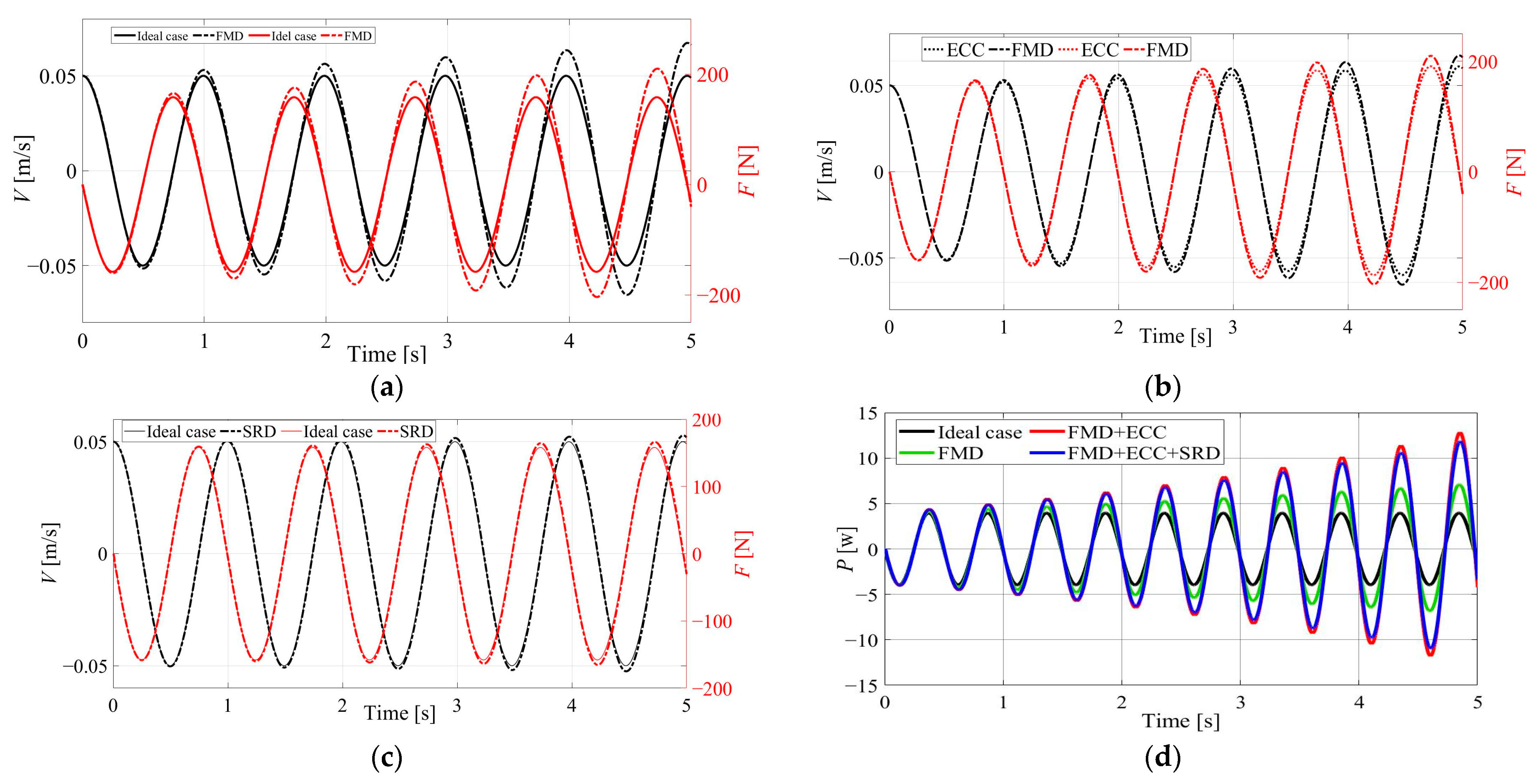
5.1. Simulation Verification of Delay Effect on SITS Stability of 1D Undamped Vibration
5.2. Identification Simulation of SITS
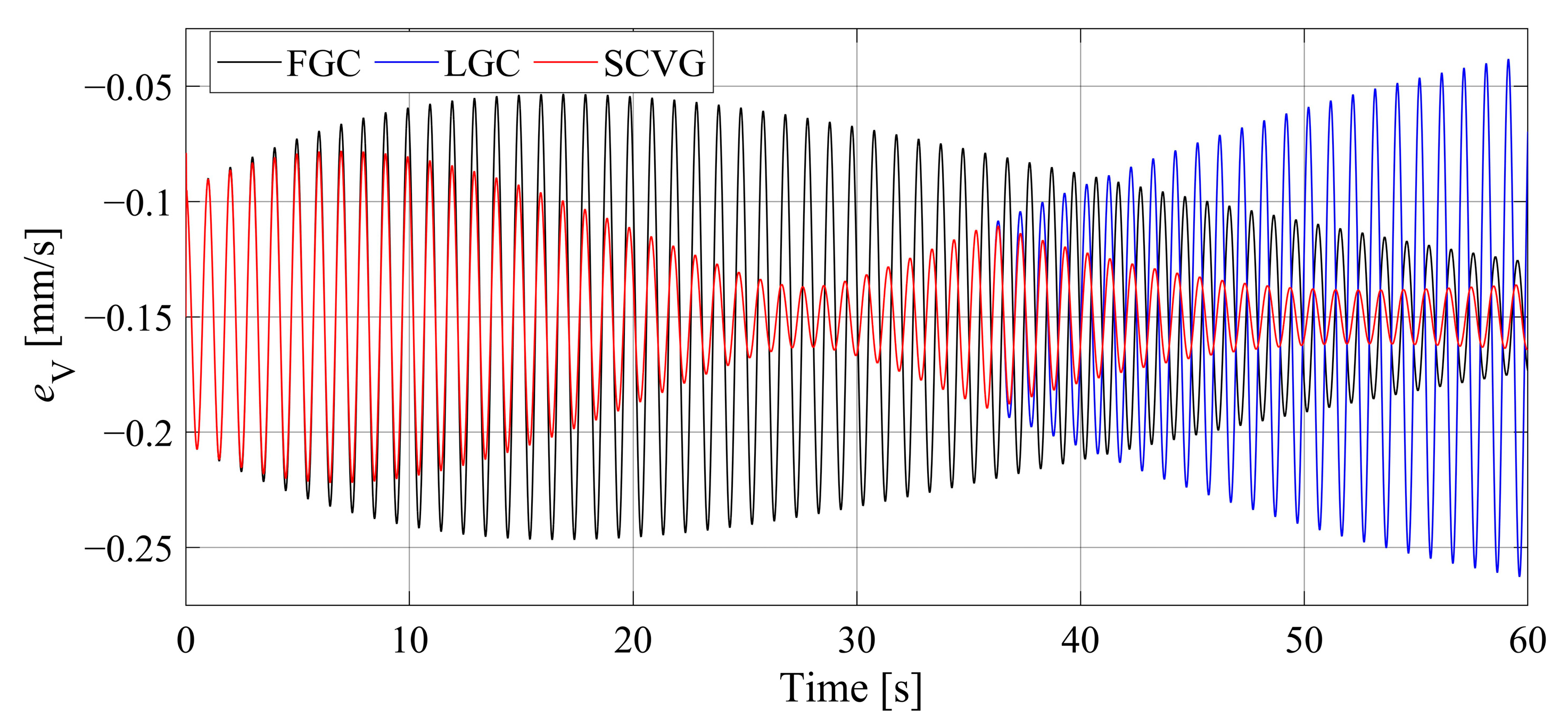
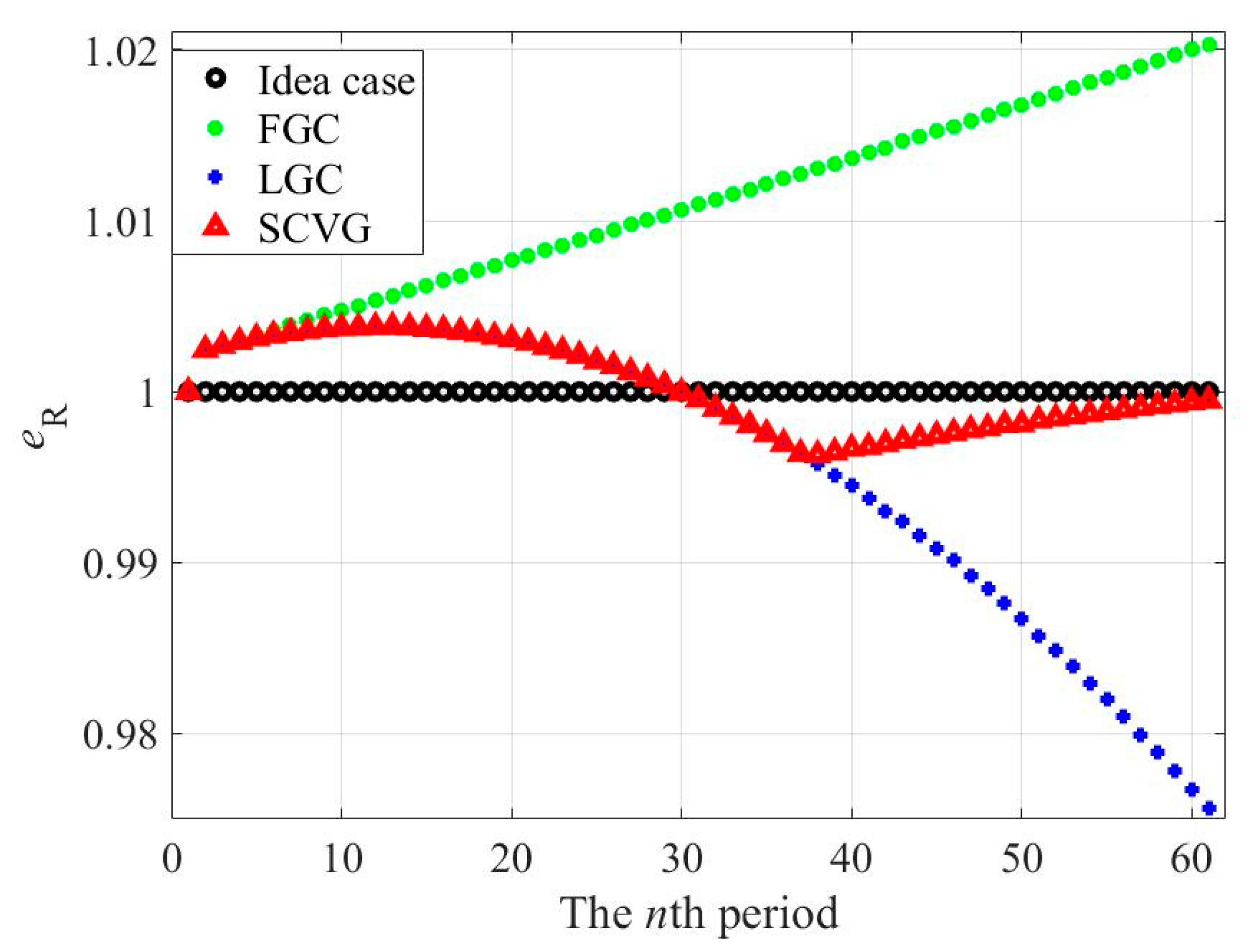
5.3. Simulation Verification of the Effect for SCVG
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Keles, O.F. Telecommunications and Space Debris: Adaptive Regulation Beyond Earth. Telecommun. Policy 2023, 47, 102517. [Google Scholar] [CrossRef]
- Takeichi, N.; Tachibana, N. A tethered plate satellite as a sweeper of small space debris. Acta Astronaut. 2021, 189, 429–436. [Google Scholar] [CrossRef]
- Witze, A. The quest to conquer earth’s space junk problem. Nature 2018, 561, 24–26. [Google Scholar] [CrossRef] [PubMed]
- Nanjangud, A.; Blacker, P.C.; Bandyopadhyay, S.; Gao, Y. Robotics and AI-Enabled On-Orbit Operations with Future Generation of Small Satellites. Proc. IEEE 2018, 106, 429–439. [Google Scholar] [CrossRef]
- Inaba, N.; Oda, M.; Asano, M. Rescuing a Stranded Satellite in Space-Experimental Robotic Capture of Non-Cooperative Satellites. Trans. Jpn. Soc. Aeronaut. Space Sci. 2006, 48, 213–220. [Google Scholar] [CrossRef]
- Papadopoulos, E.; Aghili, F.; Ma, O.; Lampariello, R. Robotic Manipulation and Capture in Space: A Survey. Front. Robot. AI 2021, 8, 686723. [Google Scholar] [CrossRef] [PubMed]
- Rybus, T. Obstacle avoidance in space robotics: Review of major challenges and proposed solutions. Prog. Aerosp. Sci. 2018, 101, 31–48. [Google Scholar] [CrossRef]
- Hatch, H.G.; Pennington, J.E.; Cobb, J.B. Dynamic Simulation of Lunar Module Docking with Apollo Command Module in Lunar Orbit; NASA Tech. Note TN D-3972; National Aeronautics and Space Administration: Washington, DC, USA, 1967; pp. 1–26. [Google Scholar]
- Uyama, N.; Fujii, Y.; Nagaoka, K.; Yoshida, K. Experimental evaluation of contact-impact dynamics between a space robot with a compliant wrist and a free-flying object. In Proceedings of the International Symposium on Artificial Intelligence, Robotics and Automation in Space, Turin, Italy, 4–6 September 2012. [Google Scholar]
- Wei, Y.; Yang, X.; Xu, Z.; Bai, X. Novel ground microgravity experiment system for a spacecraft-manipulator system based on suspension and air-bearing. Aerosp. Sci. Technol. 2023, 141, 108587. [Google Scholar] [CrossRef]
- Ma, O.; Wang, J. Model order reduction for impact-contact dynamics simulations of flexible manipulators. Robotica 2007, 25, 397–407. [Google Scholar] [CrossRef]
- Flores-Abad, A.; Ma, O.; Pham, K.; Ulrich, S. A review of space robotics technologies for on-orbit servicing. Prog. Aerosp. Sci. 2014, 68, 1–26. [Google Scholar] [CrossRef]
- Yaskevich, A. Real time math simulation of contact interaction during spacecraft docking and berthing. J. Mech. Eng. Autom. 2014, 4, 1–15. [Google Scholar]
- Roe, F.D.; Howard, R.T.; Murphy, L. Automated rendezvous and capture system development and simulation for NASA. Modeling, Simulation, and Calibration of Space-based Systems. In Proceedings of the Defense and Security, Orlando, FL, USA, 12–16 April 2004; SPIE: Bellingham, WA, USA, 2004; Volume 5420, pp. 118–125. [Google Scholar]
- Mitchell, J.D.; Cryan, S.P.; Baker, K.; Martin, T.; Goode, R.; Key, K.W.; Chien, C.H. Integrated docking simulation and testing with the Johnson space center six-degree-of-freedom dynamic test system. In Proceedings of the Space Technology and Applications International Forum, Albuquerque, NM, USA, 10–14 February 2008; pp. 709–716. [Google Scholar]
- Boge, T.; Ma, O. Using advanced industrial robotics for spacecraft Rendezvous and Docking simulation. In Proceedings of the 2011 IEEE International Conference on Robotics and Automation, Shanghai, China, 9–13 May 2011; pp. 1–4. [Google Scholar]
- Ma, O.; Flores-Abad, A.; Boge, T. Use of industrial robots for hardware-in-the-loop simulation of satellite rendezvous and docking. Acta Astronaut. 2012, 81, 335–347. [Google Scholar] [CrossRef]
- Wei, Y.Q.; Yang, X.; Bai, X.L.; Xu, Z.G. Hardware-in-the-loop based ground test system for space berthing and docking mechanism of small spacecraft. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2023, 237, 3486–3495. [Google Scholar] [CrossRef]
- Zebenay, M.; Lampariello, R.; Boge, T.; Choukroun, D. A new contact dynamics model tool for hardware-in-the-loop docking simulation. In Proceedings of the International Symposium on Artificial Intelligence, Robotics and Automation in Space, Turin, Italy, 4–6 September 2012. [Google Scholar]
- Zebenay, M.; Boge, T.; Krenn, R.; Choukroun, D. Analytical and experimental stability investigation of a hardware-in-the-loop satellite docking simulator. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2015, 229, 666–681. [Google Scholar] [CrossRef]
- Qi, C.; Ren, A.; Gao, F.; Zhao, X.; Wang, Q.; Sun, Q. Compensation of velocity divergence caused by dynamic response for hardware-in-the-loop docking simulator. IEEE/ASME Trans. Mechatron. 2016, 22, 422–432. [Google Scholar] [CrossRef]
- Stefano, M.D.; Balachandran, R.; Secchi, C. A Passivity-Based Approach for Simulating Satellite Dynamics with Robots: Discrete-Time Integration and Time-Delay Compensation. IEEE Trans. Robot. 2020, 36, 189–203. [Google Scholar] [CrossRef]
- Rao, L.S.; Rao, P.S.; Chhabra, I.M.; Kumar, L.S. Modeling and Compensation of Time-Delay Effects in HILS of Aerospace Systems. IETE Tech. Rev. 2022, 39, 375–388. [Google Scholar] [CrossRef]
- Osaki, K.; Konno, A.; Uchiyama, M. Delay time compensation for a hybrid simulator. Adv. Robot. 2010, 24, 1081–1098. [Google Scholar] [CrossRef]
- Abiko, S.; Satake, Y.; Jiang, X.; Tsujita, T.; Uchiyama, M. Delay time compensation based on coefficient of restitution for collision hybrid motion simulator. Adv. Robot. 2014, 28, 1177–1188. [Google Scholar] [CrossRef]
- Ma, O.; Wang, J.; Misra, S.; Liu, M. On the validation of SPDM task verification facility. J. Robot. Syst. 2004, 21, 219–235. [Google Scholar] [CrossRef]
- Hayati, S.; Song, W. Design and performance evaluation of an optimal discrete-time feedforward controller for servo-hydraulic compensation. J. Eng. Mech. 2018, 144, 04017163. [Google Scholar] [CrossRef]
- Sahu, G.N.; Vashisht, S.; Wahi, P.; Law, M. Validation of a hardware-in-the-loop simulator for investigating and actively damping regenerative chatter in orthogonal cutting. CIRP J. Manuf. Sci. Technol. 2020, 29, 115–129. [Google Scholar] [CrossRef]
- Xu, W.; Guo, T.; Chen, C.; Chen, M.; Chen, K. Experimental verification of a frequency domain evaluation index-based compensation for real-time hybrid simulation. Struct. Control. Health Monit. 2020, 27, e2641. [Google Scholar] [CrossRef]
- Qi, C.; Li, D.; Ma, W.; Wei, Q.; Zhang, W.; Wang, W.; Hu, H.; Gao, F. Distributed delay compensation for a hybrid simulation system of space manipulator capture. IEEE/ASME Trans. Mechatron. 2021, 27, 2367–2378. [Google Scholar] [CrossRef]
- He, J.; Shen, M. Hybrid Force/Velocity Control for Simulating Contact Dynamics of Satellite Robots on a Hardware-in-the-Loop Simulator. IEEE Access 2022, 10, 59277–59289. [Google Scholar] [CrossRef]
- Tsokanas, N.; Pastorino, R.; Stojadinovic, B. Adaptive model predictive control for actuation dynamics compensation in real-time hybrid simulation. Mech. Mach. Theory 2022, 172, 104817. [Google Scholar] [CrossRef]
- Khusainov, D.Y.; Diblík, J.; Růžičková, M.; Lukáčová, J. Representation of a solution of the Cauchy problem for an oscillating system with pure delay. Nonlinear Oscil. 2008, 11, 276–285. [Google Scholar] [CrossRef]
- Ricardo, L.G.G.; Lagomasino, G.L. Strong asymptotics of multi-level Hermite-Padé polynomials. J. Math. Anal. Appl. 2024, 531, 127801. [Google Scholar] [CrossRef]
- Đukić, D.; Mutavdžić Đukić, R.; Reichel, L.; Spalević, M. Optimal averaged Padé-type approximants. Electron. Trans. Numer. Anal. 2023, 59, 145–156. [Google Scholar] [CrossRef]
- Motoyama, Y.; Yoshimi, K.; Otsuki, J. Robust analytic continuation combining the advantages of the sparse modeling approach and the Padé approximation. Phys. Rev. B 2022, 105, 035139. [Google Scholar] [CrossRef]
- Mehmood, K.; Chaudhary, N.I.; Cheema, K.M.; Khan, Z.A.; Raja, M.A.Z.; Milyani, A.H.; Alsulami, A. Design of nonlinear marine predator heuristics for hammerstein autoregressive exogenous system identification with key-term separation. Mathematics 2023, 11, 2512. [Google Scholar] [CrossRef]
- Beltran-Perez, C.; Serrano, A.A.; Solís-Rosas, G.; Martínez-Jiménez, A.; Orozco-Cruz, R.; Espinoza-Vázquez, A.; Miralrio, A. A general use QSAR-ARX model to predict the corrosion inhibition efficiency of drugs in terms of quantum mechanical descriptors and experimental comparison for lidocaine. Int. J. Mol. Sci. 2022, 23, 5086. [Google Scholar] [CrossRef] [PubMed]
- Mehmood, K.; Chaudhary, N.I.; Khan, Z.A.; Cheema, K.M.; Raja, M.A.Z.; Shu, C.M. Novel knacks of chaotic maps with Archimedes optimization paradigm for nonlinear ARX model identification with key term separation. Chaos Soliton Fract. 2023, 175, 114028. [Google Scholar] [CrossRef]
- Wen, Q.; Wang, M.; Li, X.; Chang, Y. Learning-based design optimization of second-order tracking differentiator with application to missile guidance law. Aerosp. Sci. Technol. 2023, 137, 108302. [Google Scholar] [CrossRef]
- Park, J.H.; Park, T.S.; Kim, S.H. Asymptotically convergent higher-order switching differentiator. Mathematics 2020, 8, 185. [Google Scholar] [CrossRef]
- Feng, H.; Qian, Y. A linear differentiator based on the extended dynamics approach. IEEE Trans. Autom. Control 2022, 67, 6962–6967. [Google Scholar] [CrossRef]
- Oliveira, T.R.; Estrada, A.; Fridman, L.M. Global and exact HOSM differentiator with dynamic gains for output-feedback sliding mode control. Automatica 2017, 81, 156–163. [Google Scholar] [CrossRef]







| Parameter | Value | Unit |
|---|---|---|
| 0.05 | m/s | |
| 500 | Kg | |
| 20,000 | N/m | |
| 0.002 | s | |
| 0.004 | s | |
| 50 | N·s/m | |
| 0.025 | m | |
| 6π | rad/s | |
| 0.002 | s | |
| 1501 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, X.; Li, X.; Zhao, Z.; Xu, Z.; Lu, H.; Liu, M. Stability Analysis and Delay Compensation for Space Instable Target Simulator. Actuators 2024, 13, 123. https://doi.org/10.3390/act13040123
Bai X, Li X, Zhao Z, Xu Z, Lu H, Liu M. Stability Analysis and Delay Compensation for Space Instable Target Simulator. Actuators. 2024; 13(4):123. https://doi.org/10.3390/act13040123
Chicago/Turabian StyleBai, Xinlin, Xiwen Li, Zhen Zhao, Zhigang Xu, Han Lu, and Mingyang Liu. 2024. "Stability Analysis and Delay Compensation for Space Instable Target Simulator" Actuators 13, no. 4: 123. https://doi.org/10.3390/act13040123
APA StyleBai, X., Li, X., Zhao, Z., Xu, Z., Lu, H., & Liu, M. (2024). Stability Analysis and Delay Compensation for Space Instable Target Simulator. Actuators, 13(4), 123. https://doi.org/10.3390/act13040123






